Configuration of the Power Reflux Hydro-Mechanical Transmission System
Abstract
1. Introduction
2. Configuration Principle of the PHTS
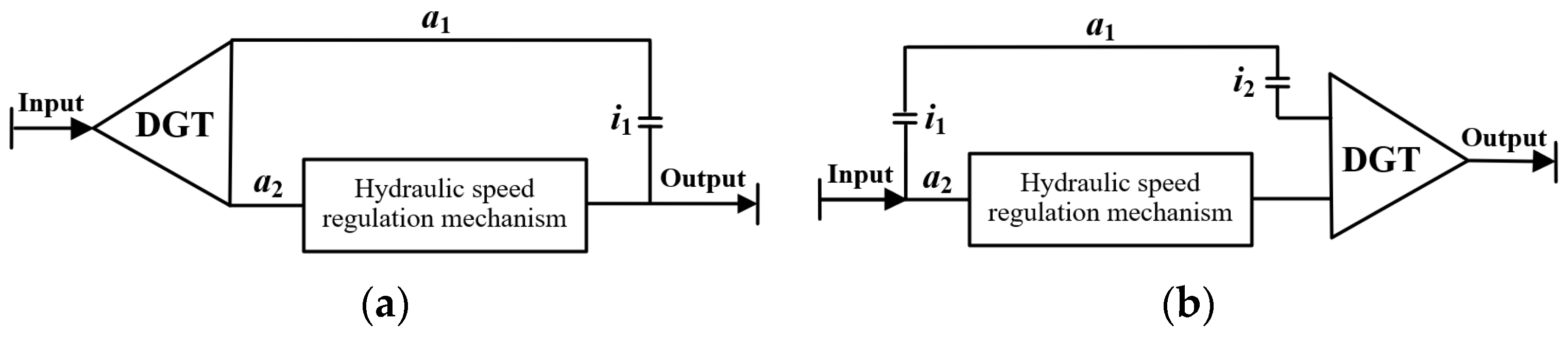
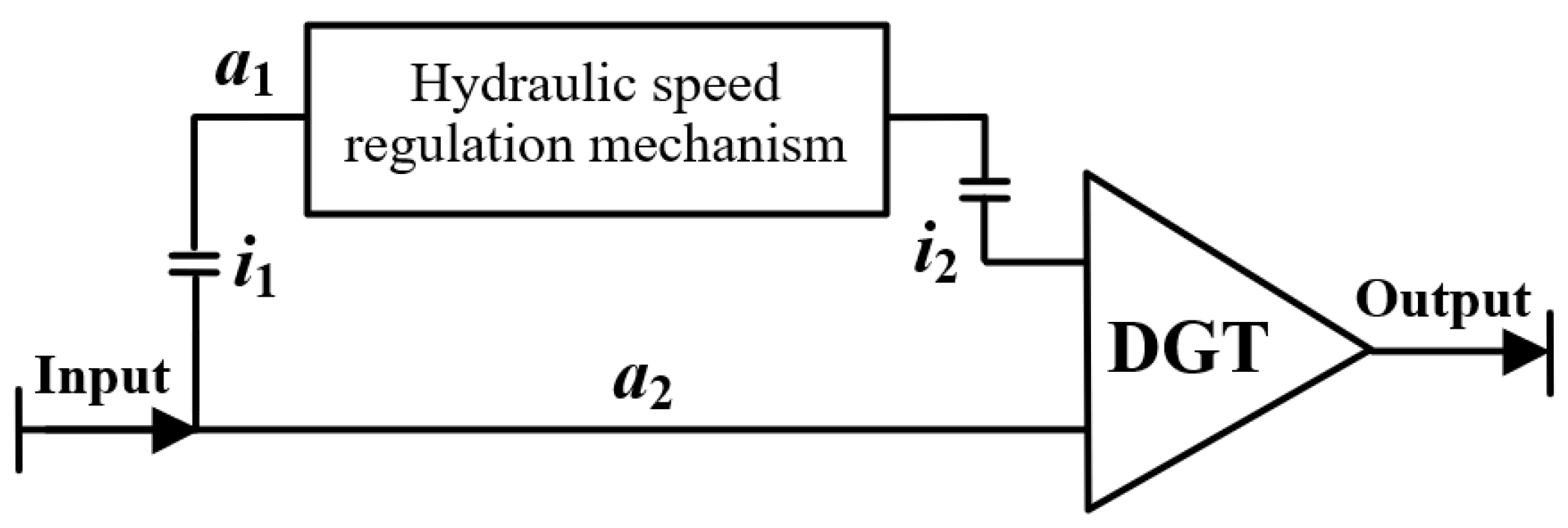
2.1. Basic Configuration of the PHTS
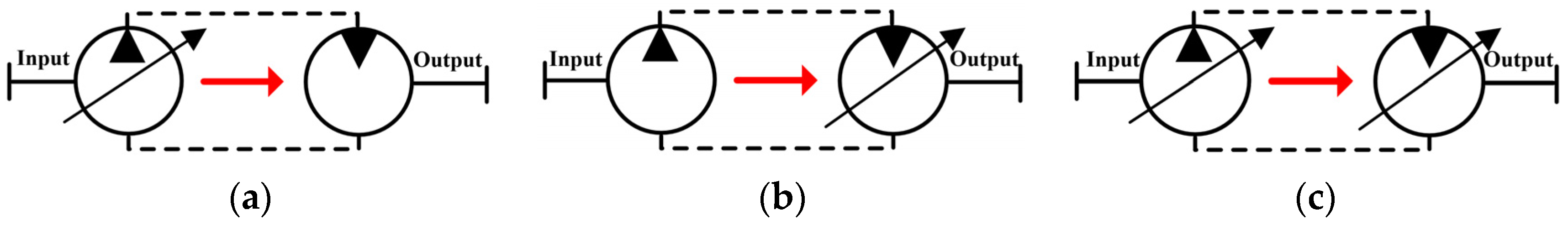
2.2. Structural Form of Hydraulic Speed Regulation Mechanism
3. Power-Flow Transmission Characteristics of PHTS
4. Transmission Characterization of PHTS
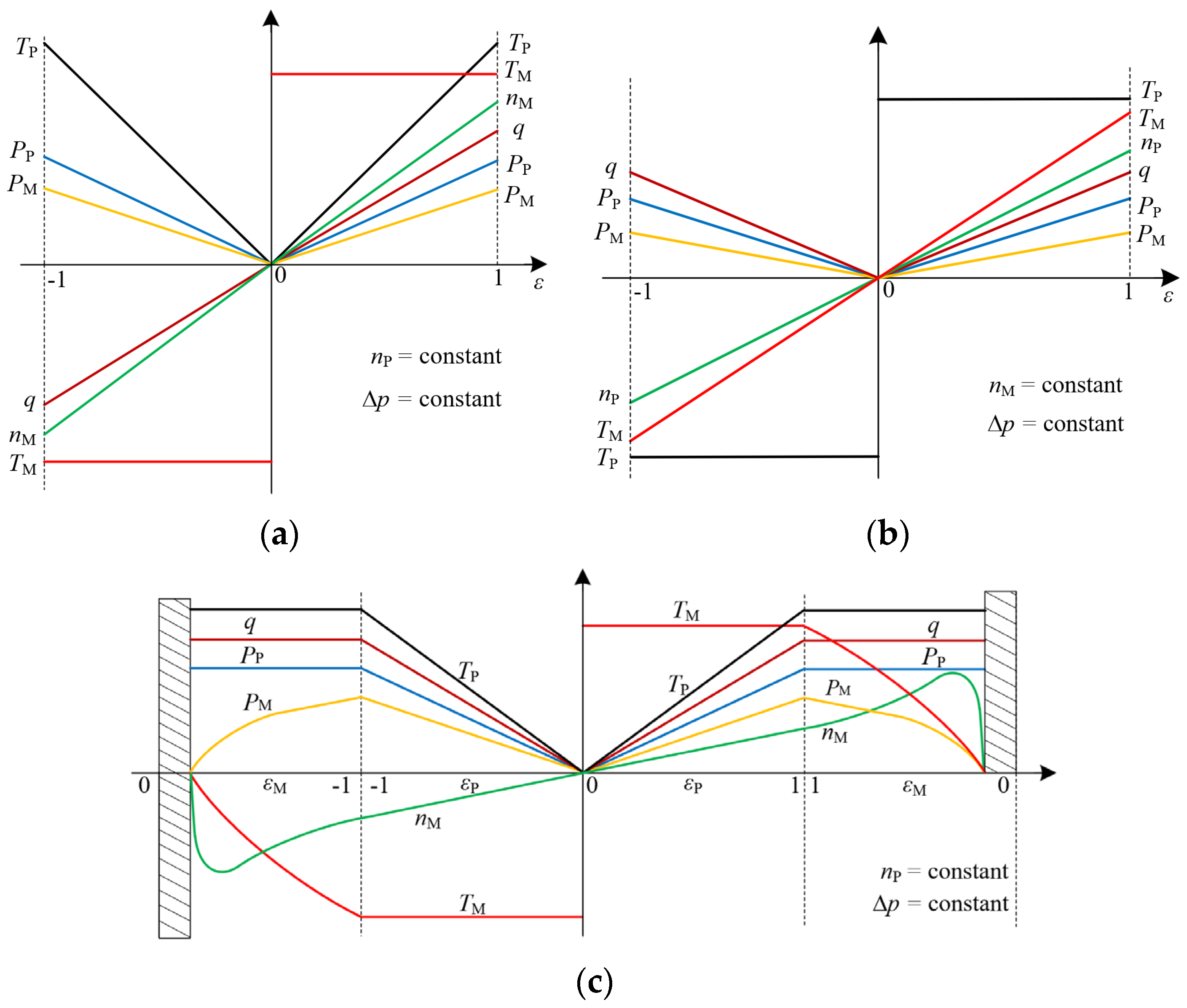
4.1. Speed Regulation Characteristics of the PHTS
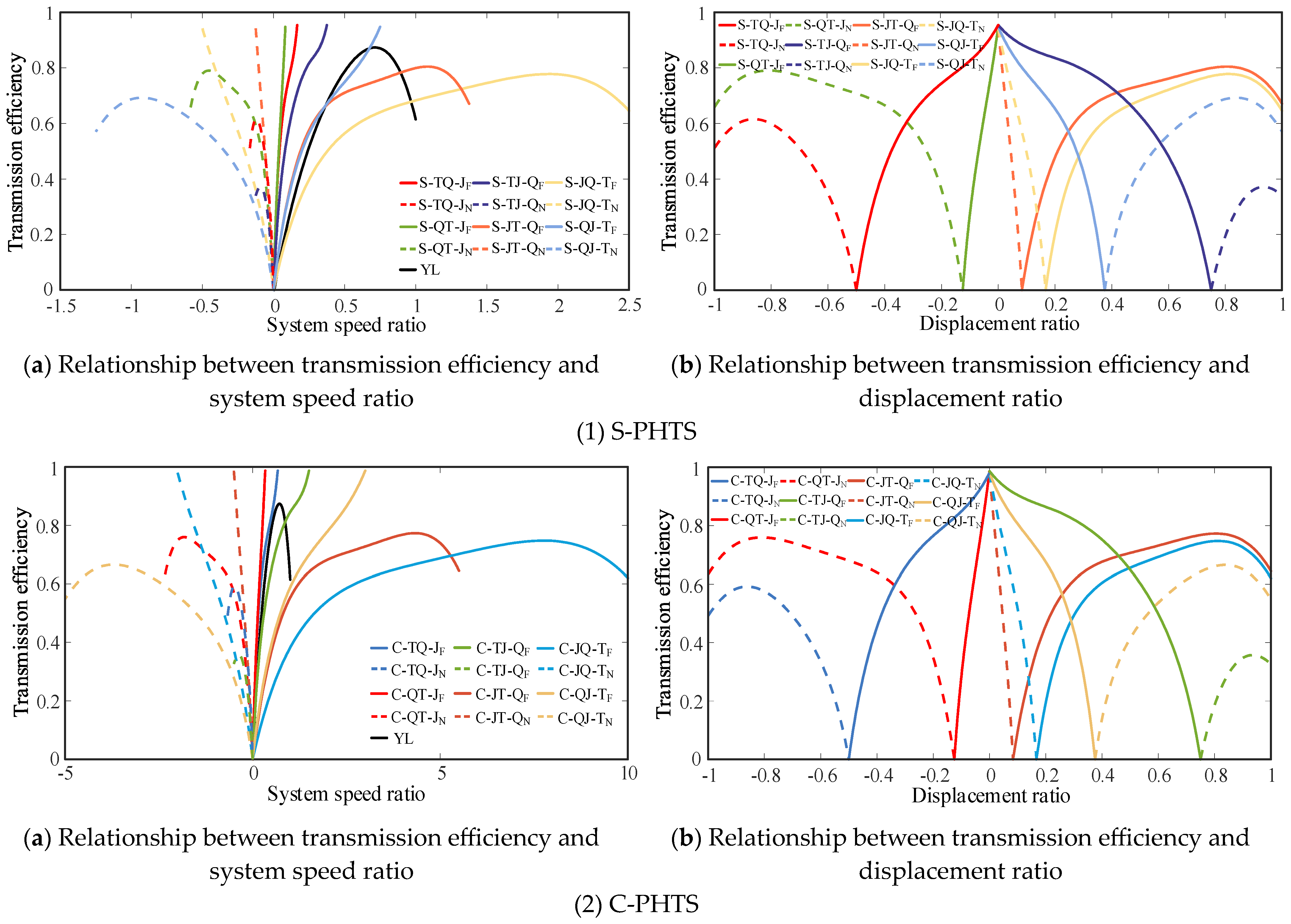
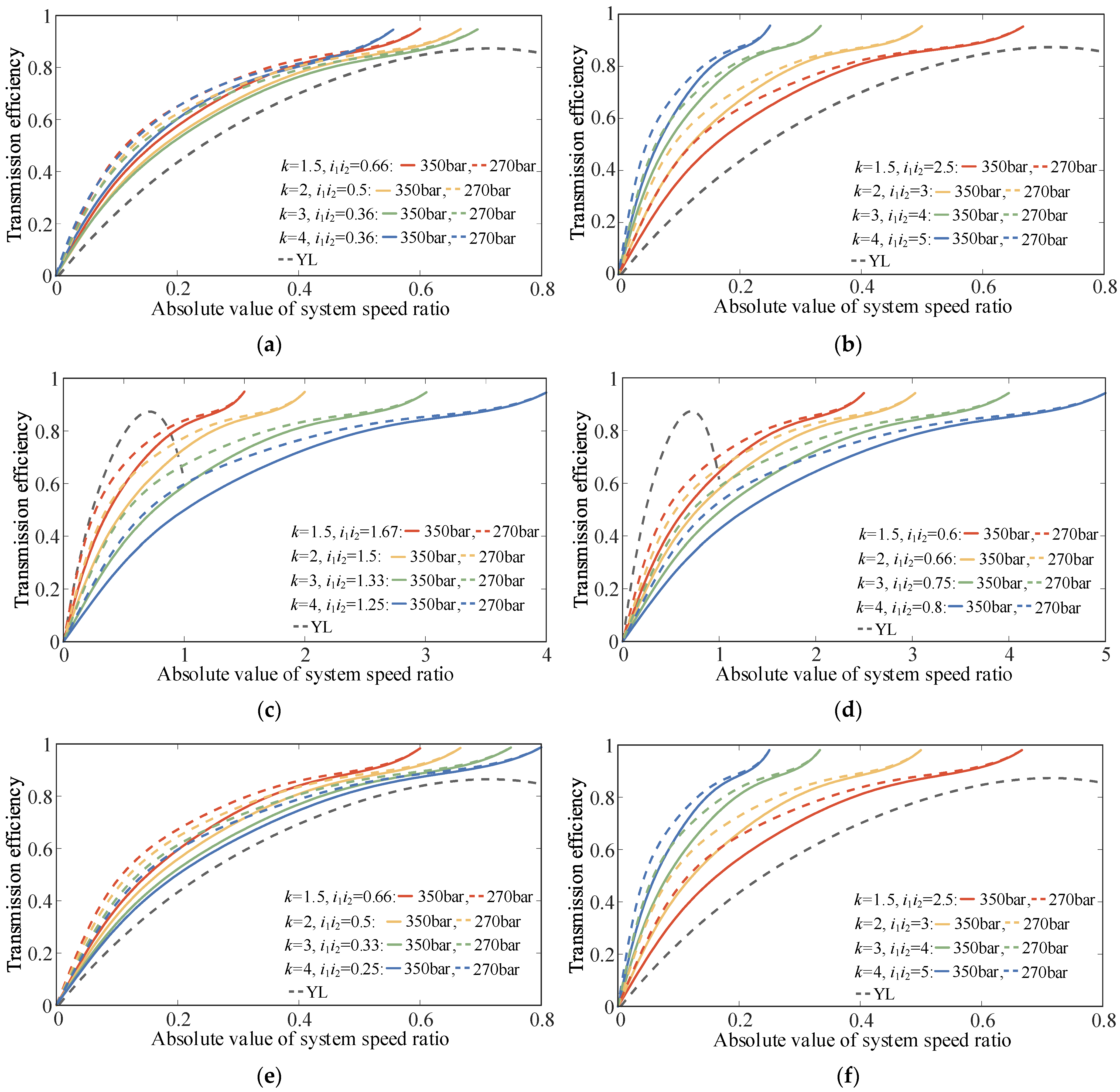
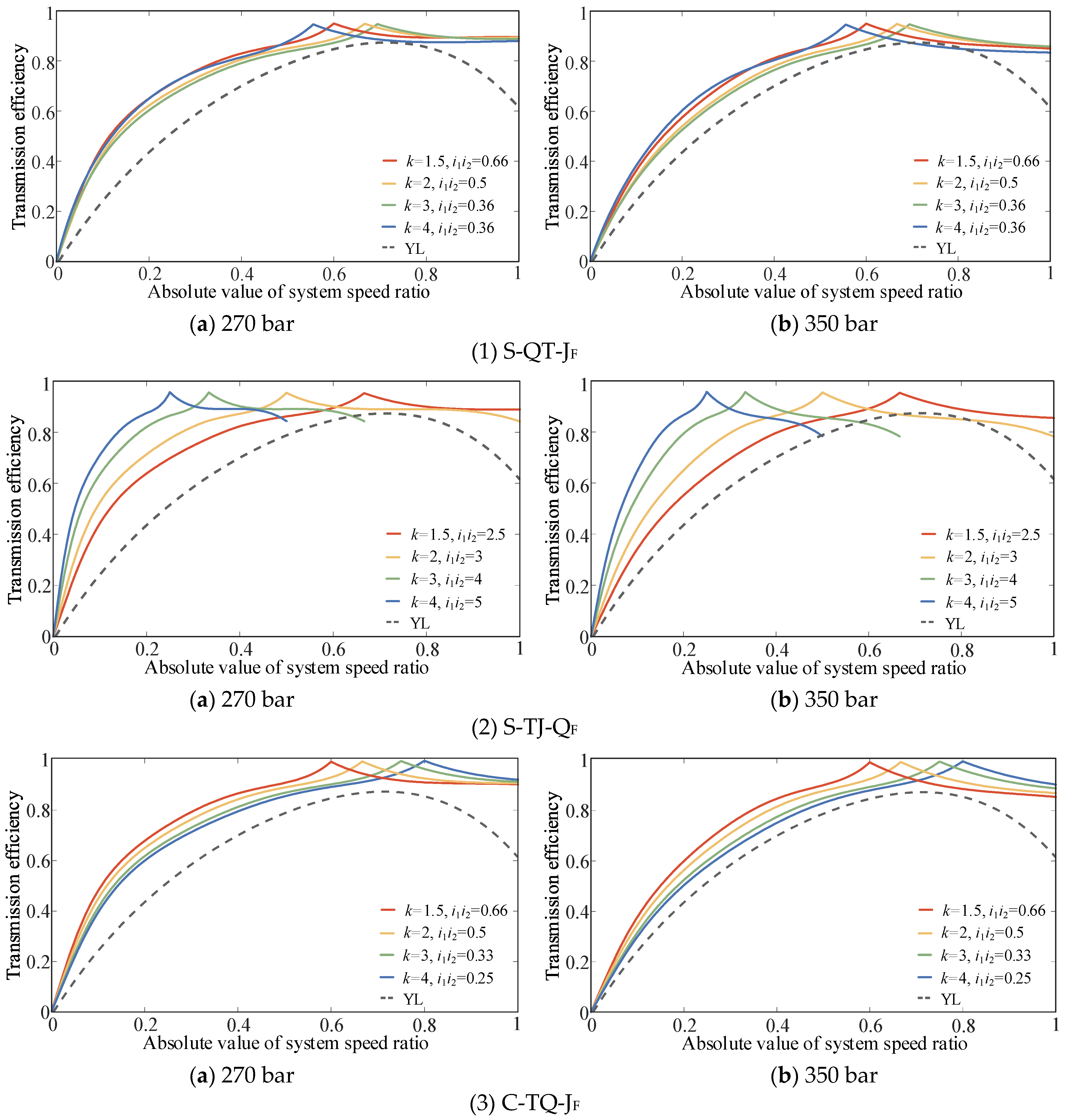
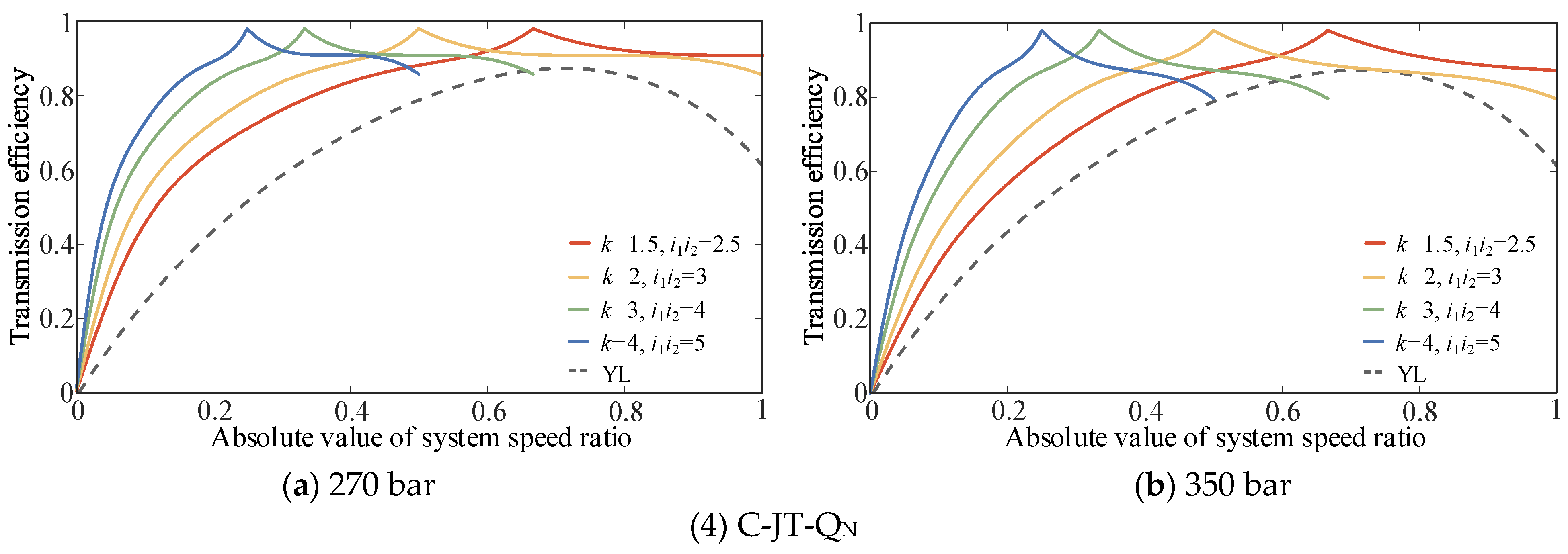
4.2. Transmission Efficiency Characteristics of the PHTS
4.2.1. Calculation of the Transmission Efficiency
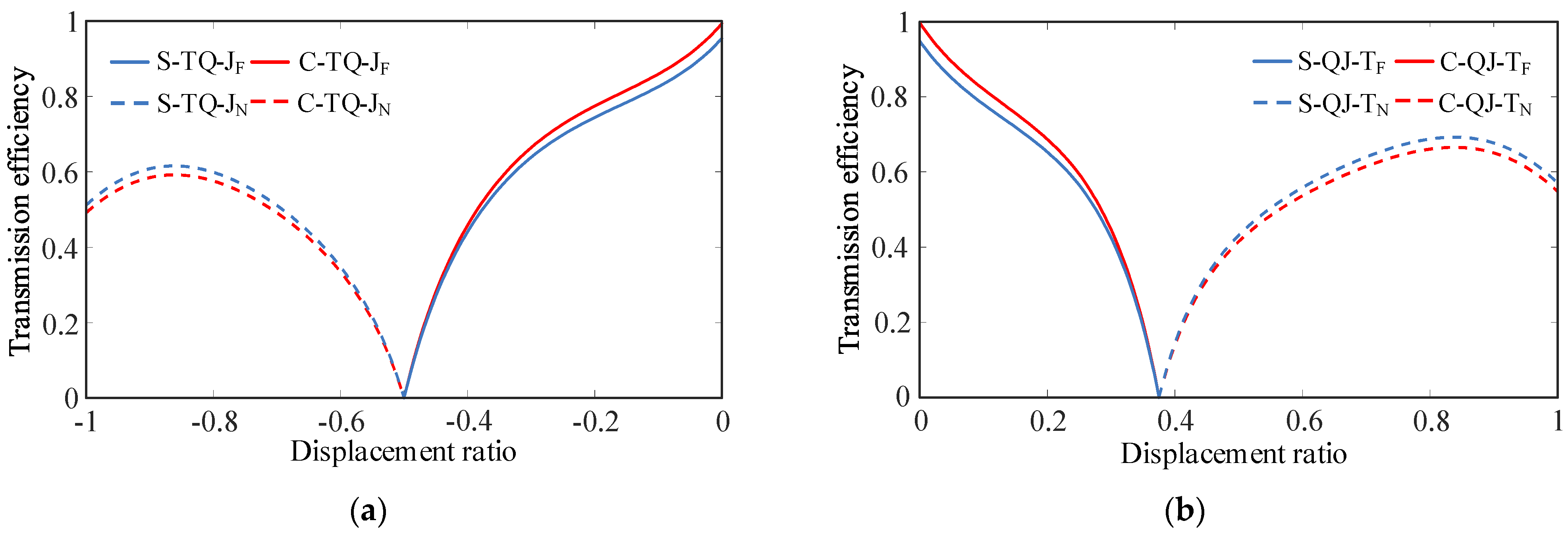
4.2.2. Efficiency Comparison between C-PHTS and S-PHTS
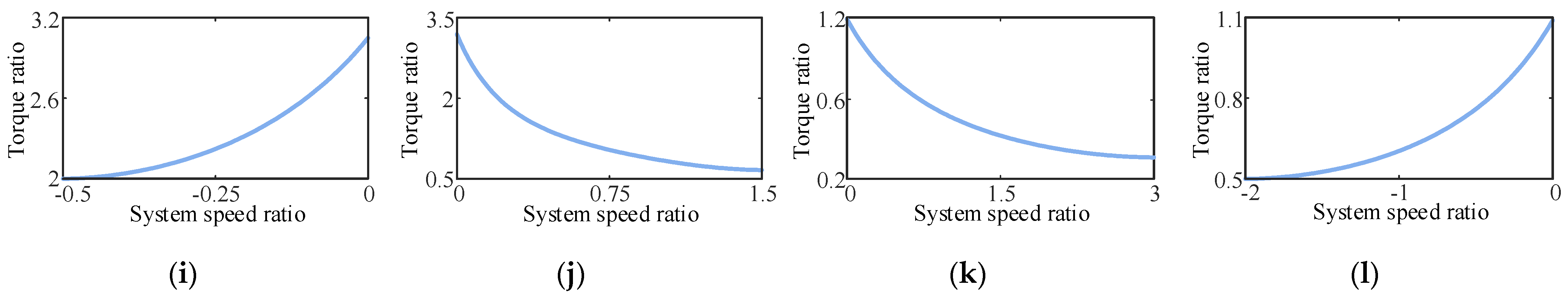
4.3. Low-Speed Torque Increase Characteristics of the PHTS
5. Feasibility Analysis of the PHTS
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| HMTS | Hydro-mechanical transmission system |
| PHTS | Power reflux hydro-mechanical transmission system |
| S-PHTS | Split-shaft power reflux hydro-mechanical transmission system |
| C-PHTS | Combined-shaft power reflux hydro-mechanical transmission system |
| CLHS | Closed-loop hydraulic system |
| DGT | Differential gear train |
| PSTM | Power split transmission mode |
| PRTM | Power reflux transmission mode |
| ε | Displacement ratio |
| nV | Actual rotation speed of the variable-displacement hydraulic components, rpm |
| nF | Actual rotation speed of the fixed-displacement hydraulic components, rpm |
| VP-FM | Variable displacement pump-fixed displacement motor closed-loop system |
| FP-VM | Fixed displacement pump-variable displacement motor closed-loop system |
| ne | Rotation speed of the power source, rpm |
| ns | Rotation speed of the sun wheel, rpm |
| nr | Rotation speed of the gear ring, rpm |
| np | Rotation speed of the planetary carrier, rpm |
| i1,i2 | Transmission ratios of fixed-axis gears |
| k | Characteristic parameter of the planetary gear |
| ib | System speed ratio of the PHTS |
| iab | Ratio of the rotation speed of elements a and b in the PHTS |
| Ratio of the rotation speed of elements b and c relative to element a in the PHTS | |
| ηP-M | Transmission efficiency of the CLHS |
| Δp | System differential pressure of the CLHS |
| φX | Power loss coefficient of the DGT when the planetary carrier is fixed |
| ηg | Total transmission efficiency of fixed-axis gears i1 and i2 |
| Pls1 | Power loss in the S-PHTS in the mechanical–hydraulic reflux transmission form, kW |
| Plc1 | Power loss in the C-PHTS in the mechanical–hydraulic reflux transmission form, kW |
| Ptm | Total power input to the mechanical transmission path in the mechanical–hydraulic reflux transmission form, kW |
| Pls2 | Power loss in the S-PHTS in the hydraulic–mechanical reflux transmission form, kW |
| Plc2 | Power loss in the C-PHTS in the hydraulic–mechanical reflux transmission form, kW |
| Pth | Total power input to the hydraulic transmission path in the hydraulic–mechanical reflux transmission form, kW |
| b | Power reflux ratio, defined as the ratio of the reflux power to the total power input to the DGT |
| Pd1 | Difference in power loss between the S-PHTS and C-PHTS in the mechanical–hydraulic reflux transmission form, kW |
| Pd2 | Difference in power loss between the S-PHTS and C-PHTS in the hydraulic–mechanical reflux transmission form, kW |
| Pr | Power transmitted by the gear ring, kW |
| Pe | Input power of the power source, kW |
| Ps | Power transmitted by the sun wheel, kW |
| Ts | Torque of the sun wheel, Nm |
| Tr | Torque of the gear ring, Nm |
| Tp | Torque of the planetary carrier, Nm |
| K | Torque ratio, expressed as the ratio of the output torque to the input torque of the system |
| ηpm | Transmission efficiency of the CLHS when the displacement ratio of the CLHS is maximum or minimum (|ε| = |ε|max) |
| ηpmmax | Maximum transmission efficiency of the CLHS |
References
- Li, C.; Meng, F.; Xi, J.; Zhai, Y. Starting shift control of heavy-duty automatic transmissions based on the optimal trajectory of turbine speed. Mech. Syst. Signal Process. 2019, 126, 490–504. [Google Scholar] [CrossRef]
- Karaoglan, M.U.; Vatansever, Y.; Kuralay, N.S. Simulation of 8 × 8 heavy duty truck for evaluating effects of torque converter characteristics on vehicle performance. Mechanika 2018, 24, 232–239. [Google Scholar] [CrossRef]
- Xiong, P.; Chen, X.; Sun, H.; Zhong, J.; Wu, L.; Gao, H. Effect of the blade shaped by Joukowsky airfoil transformation on the characteristics of the torque converter. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2021, 235, 3314–3321. [Google Scholar]
- Kim, B.S.; Ha, S.B.; Lim, W.S.; Cha, S.W. Performance estimation model of a torque converter part I: Correlation between the internal flow field and energy loss coefficient. Int. J. Automot. Technol. 2008, 9, 141–148. [Google Scholar] [CrossRef]
- Do, H.T.; Park, H.G.; Ahn, K.K. Application of an adaptive fuzzy sliding mode controller in velocity control of a secondary controlled hydrostatic transmission system. Mechatronics 2014, 24, 1157–1165. [Google Scholar] [CrossRef]
- Guo, X.; Vacca, A. Advanced design and optimal sizing of hydrostatic transmission systems. Actuators 2021, 10, 243. [Google Scholar] [CrossRef]
- Comellas, M.; Pijuan, J.; Nogues, M.; Roca, J. Efficiency analysis of a multiple axle vehicle with hydrostatic transmission overcoming obstacles. Veh. Syst. Dyn. 2018, 56, 55–77. [Google Scholar] [CrossRef]
- Yu, J.; Dong, X.; Song, Y.; Zhang, Y.; Zhang, H.; Yang, X.; Xu, Z.; Liu, Y. Energy efficiency optimization of a compound coupled hydro-mechanical transmission for heavy-duty vehicles. Energy 2022, 252, 123937. [Google Scholar] [CrossRef]
- Savaresi, S.M.; Taroni, F.L.; Previdi, F.; Bittanti, S. Control system design on a power-split CVT for high-power agricultural tractors. IEEE/ASME Trans. Mechatron. 2004, 9, 569–579. [Google Scholar] [CrossRef]
- Cao, F.Y.; Zhou, Z.L.; Zhao, H.J. Design of steering wheel control system of tracked vehicle of hydro-mechanical differential turning. Adv. Mater. Res. 2012, 472–475, 753–756. [Google Scholar]
- Liu, X. Vehicle Transmission System Analysis; National Defense Industry Press: Beijing, China, 1998. [Google Scholar]
- Rossetti, A.; Macor, A. Multi-objective optimization of hydro-mechanical power split transmissions. Mech. Mach. Theory 2013, 62, 112–128. [Google Scholar] [CrossRef]
- Pettersson, K.; Heybroek, K.; Mattsson, P.; Krus, P. A novel hydromechanical hybrid motion system for construction machines. Int. J. Fluid Power 2017, 18, 17–28. [Google Scholar] [CrossRef]
- Liu, F.; Wu, W.; Hu, J.; Yuan, S. Design of multi-range hydro-mechanical transmission using modular method. Mech. Syst. Signal Process. 2019, 126, 1–20. [Google Scholar] [CrossRef]
- Wu, W.; Luo, J.; Wei, C.; Liu, H.; Yuan, S. Design and control of a hydro-mechanical transmission for all-terrain vehicle. Mech. Mach. Theory 2020, 154, 104052. [Google Scholar] [CrossRef]
- Troin, P.E.; Gostomski, V.G. Application Considerations with the Cummins Sundstrand DMT-25 Hydromechanical Transmission; SAE Technical Paper 750732; SAE International: Warrendale, PA, USA, 1975. [Google Scholar]
- Brenninger, M.M. Fendt Vario CVT in Agricultural Tractors; SAE Technical Paper 4205; SAE International: Warrendale, PA, USA, 2007. [Google Scholar]
- Wu, Q. 800 Vario Series Continuously Variable New Tractors from FENDT Company. Tract. Farm Transp. 2016, 43, 6–11. [Google Scholar]
- Pohlenz, J.; Gruhle, W.D. The ECCOM continuously variable hydrostatic-variable power-split transmission for use in tractors. VDI Berichte 2001, 1592, 451–468. [Google Scholar]
- Messenger, B. Hydrostatic transmission designed for compact mobile applications. Diesel Prog. Int. Ed. 2003, 22, 28. [Google Scholar]
- Nagao, T.; Ichiryu, K.; Masuzawa, K. Development of SATV [Super All Terrain Vehicle] and HMT [Hydro Mechanical Transmission] System; SAE Technical Paper 021448; SAE International: Warrendale, PA, USA, 2002. [Google Scholar]
- Liu, X.; Sun, D. An improved design of power-cycling hydrodynamic mechanical transmission. J. Mech. Sci. Technol. 2020, 34, 3165–3179. [Google Scholar] [CrossRef]
- Cheng, Z.; Lu, Z.; Qian, J. A new non-geometric transmission parameter optimization design method for HMCVT based on improved GA and maximum transmission efficiency. Comput. Electron. Agric. 2019, 167, 105034. [Google Scholar] [CrossRef]
- Wang, H.; Sun, D. Theory and application on power-cycling variable transmission system. J. Mech. Design 2017, 139, 24501. [Google Scholar] [CrossRef]
- You, Y.; Sun, D.; Qin, D.; Wu, B.; Feng, J. A new continuously variable transmission system parameters matching and optimization based on wheel loader. Mech. Mach. Theory 2020, 150, 103876. [Google Scholar] [CrossRef]
- Mucino, V.H.; Lu, Z.; Smith, J.E.; Kimcikiewicz, M.; Cowan, B. Design of continuously variable power split transmission systems for automotive applications. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2001, 215, 469–478. [Google Scholar] [CrossRef]
- Suo, X.; Jiang, Y.; Wang, W. Hydraulic axial plunger pump: Gaseous and vaporous cavitation characteristics and optimization method. Eng. Appl. Comput. Fluid Mech. 2021, 15, 712–726. [Google Scholar] [CrossRef]
- Xiao, M.; Zhao, J.; Wang, Y.; Zhang, H.; Lu, Z.; Wei, W. Fuel economy of multiple conditions self-adaptive tractors with hydro-mechanical CVT. Int. J. Agric. Biol. Eng. 2018, 11, 102–109. [Google Scholar] [CrossRef]
- Lee, W.; Park, J.; Kim, N. Analysis of transmission efficiency of a plug-in hybrid vehicle based on operating modes. Int. J. Precis. Eng. Manuf.-Green Technol. 2021, 8, 165–175. [Google Scholar] [CrossRef]
- Cheng, Z.; Lu, Z.; Dai, F. Research on HMCVT efficiency model based on the improved SA algorithm. Math. Probl. Eng. 2019, 2019, 2856908. [Google Scholar] [CrossRef]
- Kauranne, H. Effect of operating parameters on efficiency of swash-plate type axial piston pump. Energies 2022, 15, 4030. [Google Scholar] [CrossRef]
- Zhao, Z. Automotive Design; China Machine Press: Beijing, China, 2019. [Google Scholar]
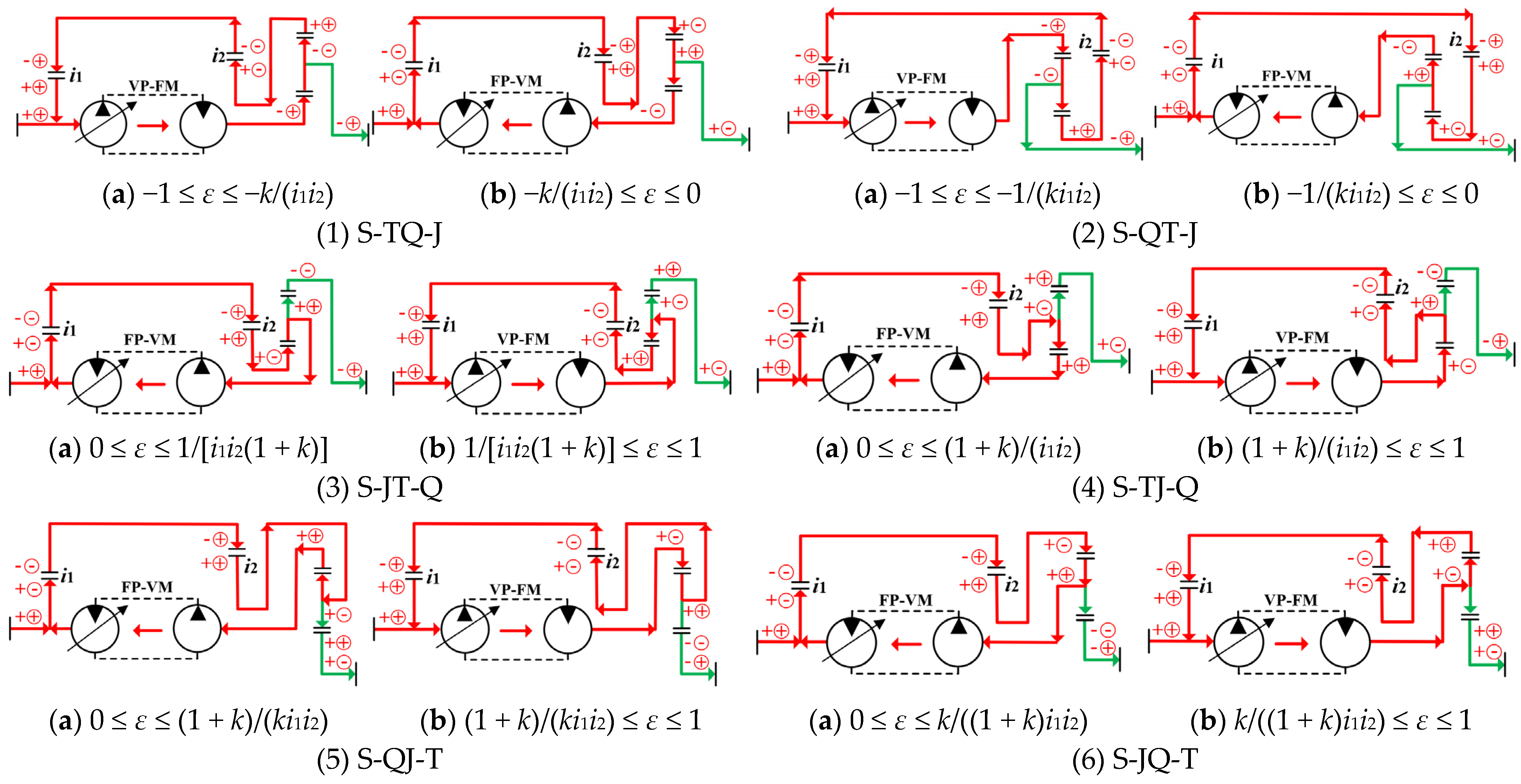
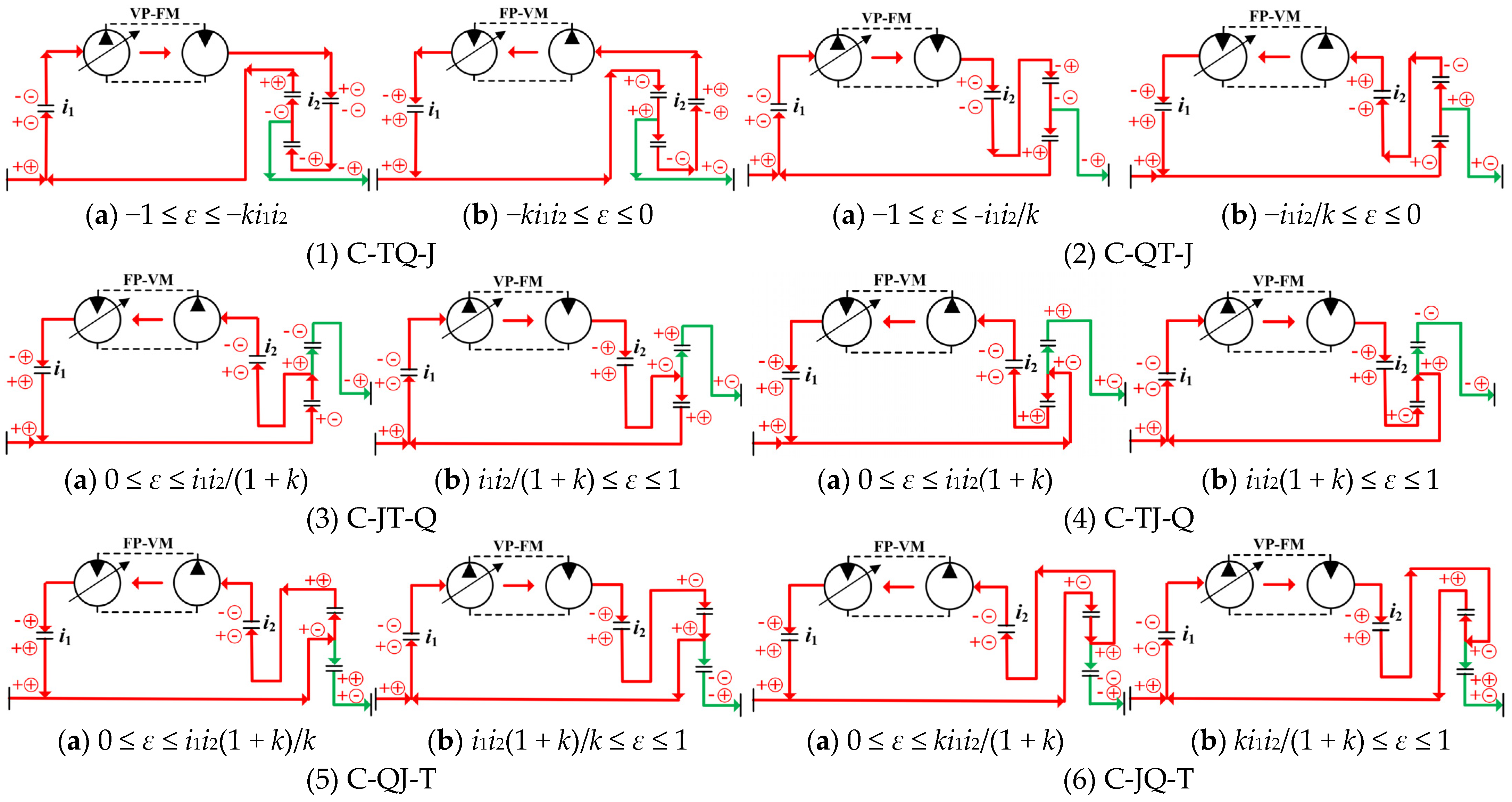
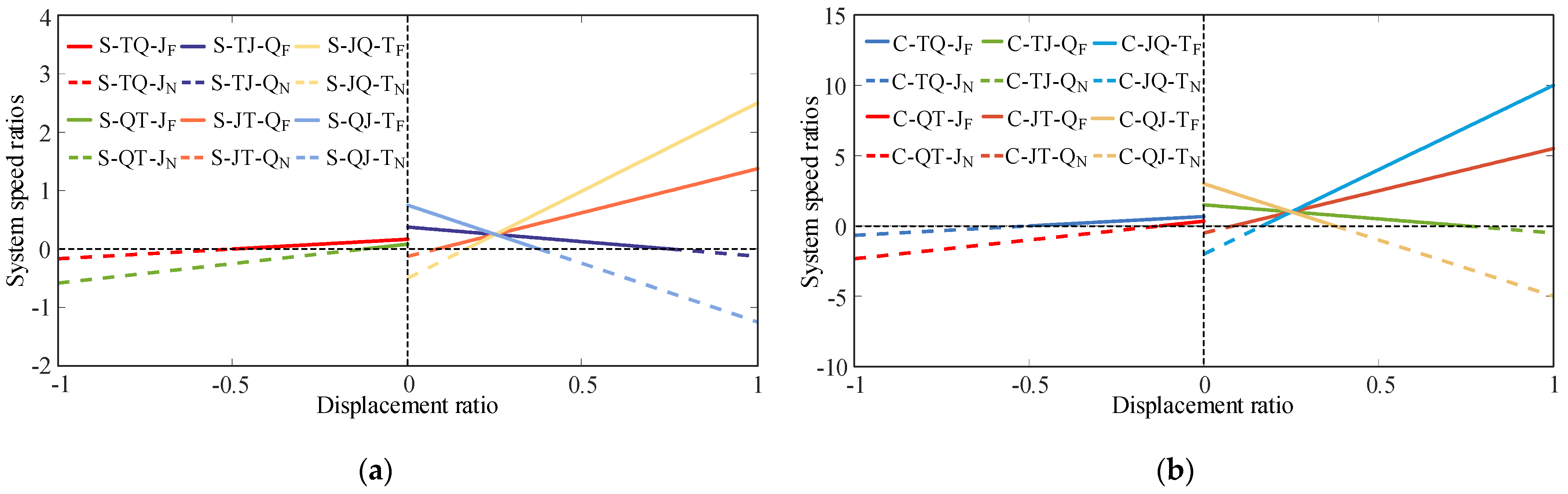
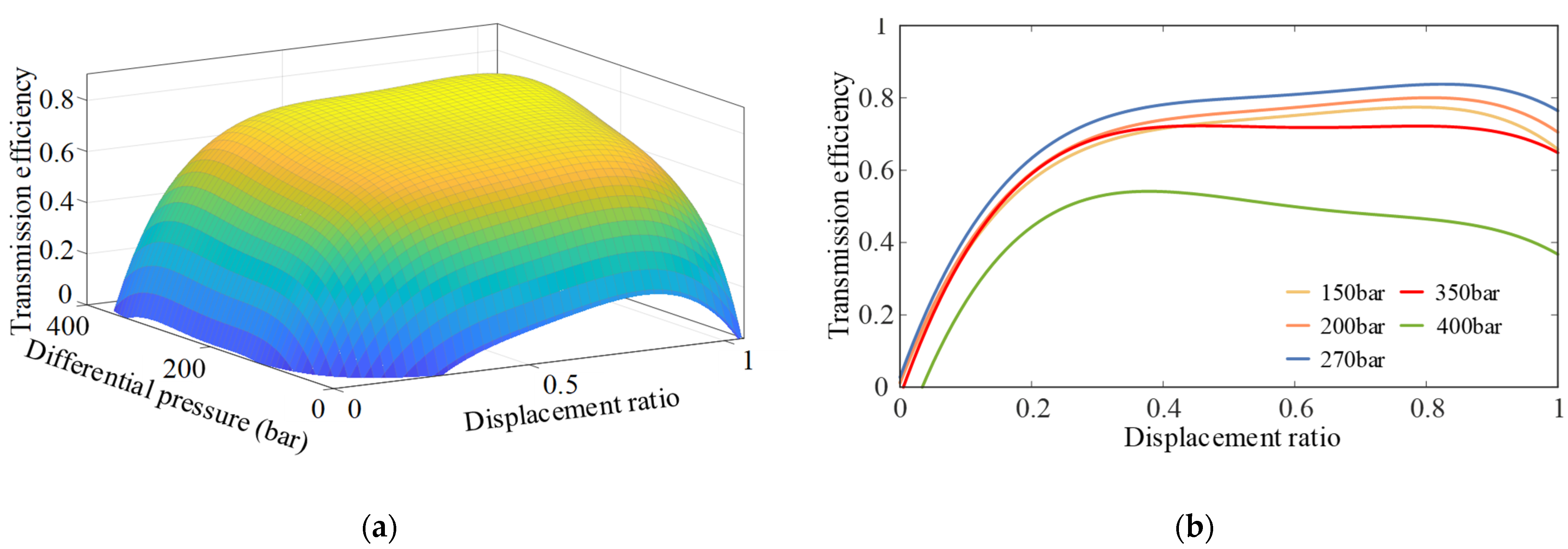


| Configuration | System Speed Ratio of the S-PHTS | Configuration | System Speed Ratio of the D-PHTS |
|---|---|---|---|
| S-TQ-J | C-TQ-J | ||
| S-QT-J | C-QT-J | ||
| S-JT-Q | C-JT-Q | ||
| S-TJ-Q | C-TJ-Q | ||
| S-QJ-T | C-QJ-T | ||
| S-JQ-T | C-JQ-T |
| Configuration | Transmission Efficiency of the S-PHTS | Configuration | Transmission Efficiency of the C-PHTS |
|---|---|---|---|
| S-TQ-JN | C-TQ-JN | ||
| S-TQ-JF | C-TQ-JF | ||
| S-QT-JN | C-QT-JN | ||
| S-QT-JF | C-QT-JF | ||
| S-JT-QN | C-JT-QN | ||
| S-JT-QF | C-JT-QF | ||
| S-TJ-QF | C-TJ-QF | ||
| S-TJ-QN | C-TJ-QN | ||
| S-QJ-TF | C-QJ-TF | ||
| S-QJ-TN | C-QJ-TN | ||
| S-JQ-TN | C-JQ-TN | ||
| S-JQ-TF | C-JQ-TF |
| Configuration | Torque Ratio of the S-PHTS | Configuration | Torque Ratio of the C-PHTS |
|---|---|---|---|
| S-TQ-JN | C-TQ-JN | ||
| S-TQ-JF | C-TQ-JF | ||
| S-QT-JN | C-QT-JN | ||
| S-QT-JF | C-QT-JF | ||
| S-JT-QN | C-JT-QN | ||
| S-JT-QF | C-JT-QF | ||
| S-TJ-QF | C-TJ-QF | ||
| S-TJ-QN | C-TJ-QN | ||
| S-QJ-TF | C-QJ-TF | ||
| S-QJ-TN | C-QJ-TN | ||
| S-JQ-TN | C-JQ-TN | ||
| S-JQ-TF | C-JQ-TF |
| Configuration | Maximum Torque Ratio | Configuration | Maximum Torque Ratio | ||||||
|---|---|---|---|---|---|---|---|---|---|
| k = 1.5 | k = 2 | k = 3 | k = 4 | k = 1.5 | k = 2 | k = 3 | k = 4 | ||
| S-TQ-JF | 29.94 | 32.72 | 36.10 | 37.11 | C-TQ-JF | 8.50 | 7.65 | 6.80 | 6.38 |
| S-QT-JF | 28.92 | 31.40 | 37.80 | 44.87 | C-QT-JF | 12.75 | 15.29 | 20.39 | 25.49 |
| S-JT-QN | 14.77 | 18.90 | 26.92 | 34.79 | C-JT-QN | 7.65 | 10.20 | 15.29 | 20.39 |
| S-TJ-QF | 14.96 | 18.05 | 22.26 | 26.17 | C-TJ-QF | 3.06 | 3.40 | 3.82 | 4.08 |
| S-QJ-TF | 7.72 | 5.99 | 4.15 | 3.19 | C-QJ-TF | 2.04 | 1.70 | 1.27 | 1.02 |
| S-JQ-TN | 7.41 | 5.78 | 4.05 | 3.13 | C-JQ-TN | 3.40 | 2.55 | 1.70 | 1.27 |
| Configuration | Maximum Speed Ratio Upper Limit | Configuration | Maximum Speed Ratio Upper Limit | ||||||
|---|---|---|---|---|---|---|---|---|---|
| k = 1.5 | k = 2 | k = 3 | k = 4 | k = 1.5 | k = 2 | k = 3 | k = 4 | ||
| S-TQ-JF | 0.40 | 0.33 | 0.25 | 0.20 | S-JQ-TN | −2.50 | −3.03 | −4 | −5 |
| S-QT-JF | 0.61 | 0.66 | 0.69 | 0.55 | C-TQ-JF | 0.6 | 0.66 | 0.75 | 0.8 |
| S-JT-QN | −1.67 | −1.38 | −0.93 | −0.69 | C-QT-JF | 0.40 | 0.33 | 0.25 | 0.20 |
| S-TJ-QF | 0.66 | 0.50 | 0.33 | 0.25 | C-JT-QN | −0.67 | −0.50 | −0.33 | −0.25 |
| S-QJ-TF | 1.50 | 2 | 3 | 4 | C-TJ-QF | 1.67 | 1.50 | 1.33 | 1.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Sun, D.; Wang, J.; Liao, G. Configuration of the Power Reflux Hydro-Mechanical Transmission System. Actuators 2024, 13, 120. https://doi.org/10.3390/act13040120
Wang J, Sun D, Wang J, Liao G. Configuration of the Power Reflux Hydro-Mechanical Transmission System. Actuators. 2024; 13(4):120. https://doi.org/10.3390/act13040120
Chicago/Turabian StyleWang, Jiezhong, Dongye Sun, Jianhua Wang, and Guangliang Liao. 2024. "Configuration of the Power Reflux Hydro-Mechanical Transmission System" Actuators 13, no. 4: 120. https://doi.org/10.3390/act13040120
APA StyleWang, J., Sun, D., Wang, J., & Liao, G. (2024). Configuration of the Power Reflux Hydro-Mechanical Transmission System. Actuators, 13(4), 120. https://doi.org/10.3390/act13040120





