Development of a Self-Regulating Solar Shading Actuator Based on the Thermal Shape Memory Effect
Abstract
1. Introduction
2. Concept of the Self-Regulating Solar Shading Actuator
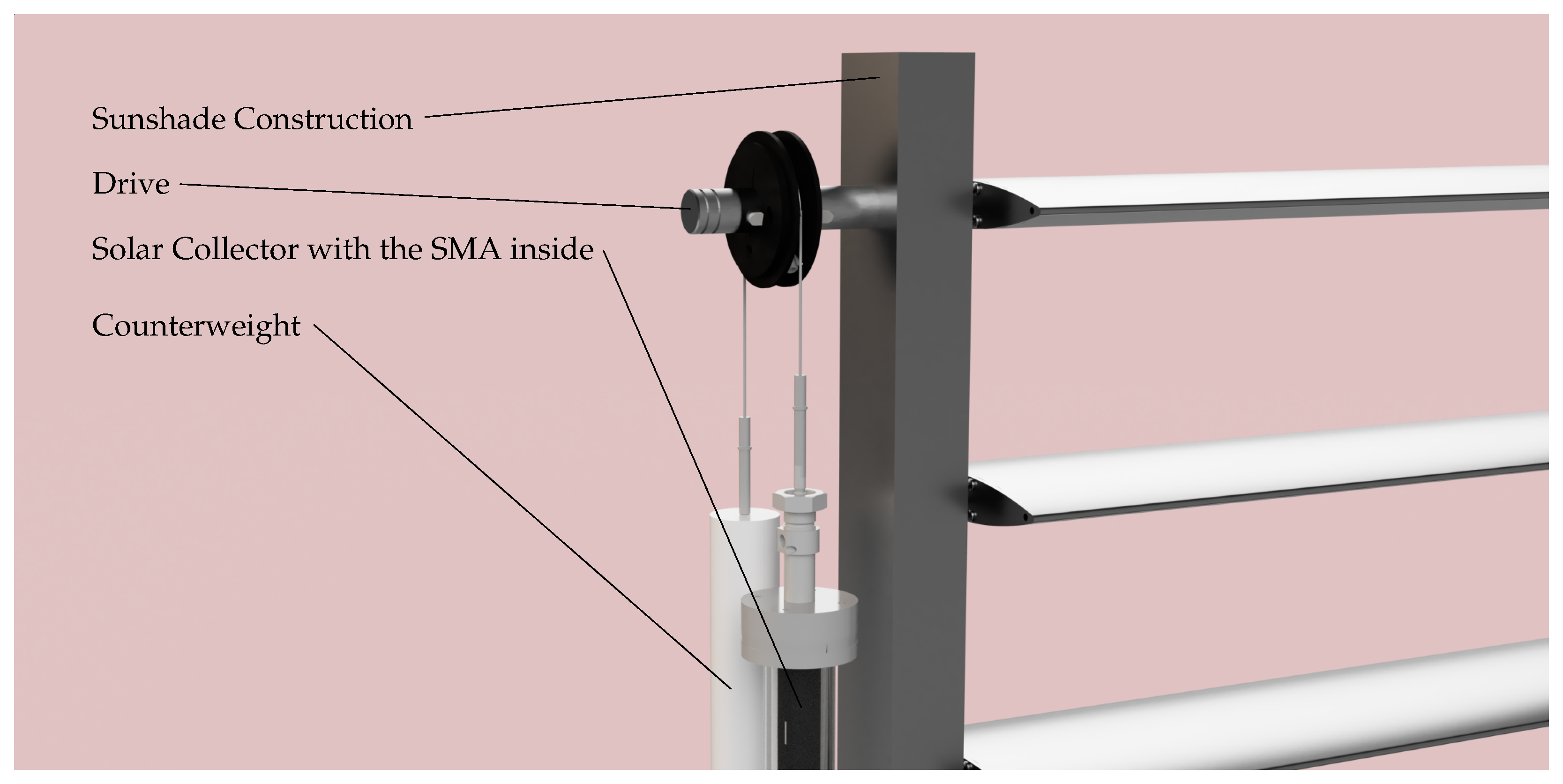
2.1. Sunshade Construction
2.2. Shape Memory Effect
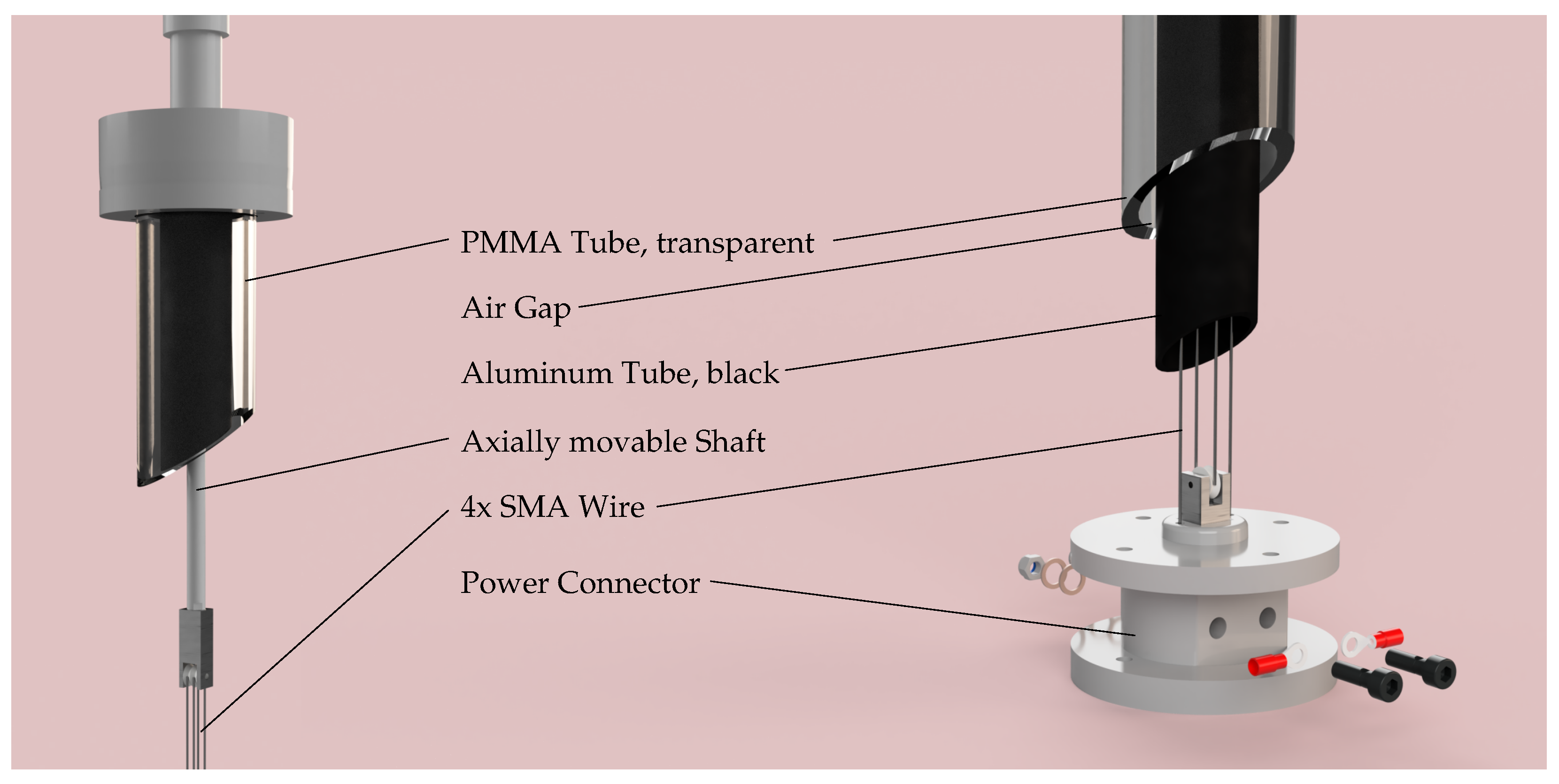
2.3. Actuator Concept
3. Actuator Component Design
3.1. Actuator Parameters
3.2. Definition of the Shading Requirement
3.3. Determination of the Design Temperatures for the SMA Transformation
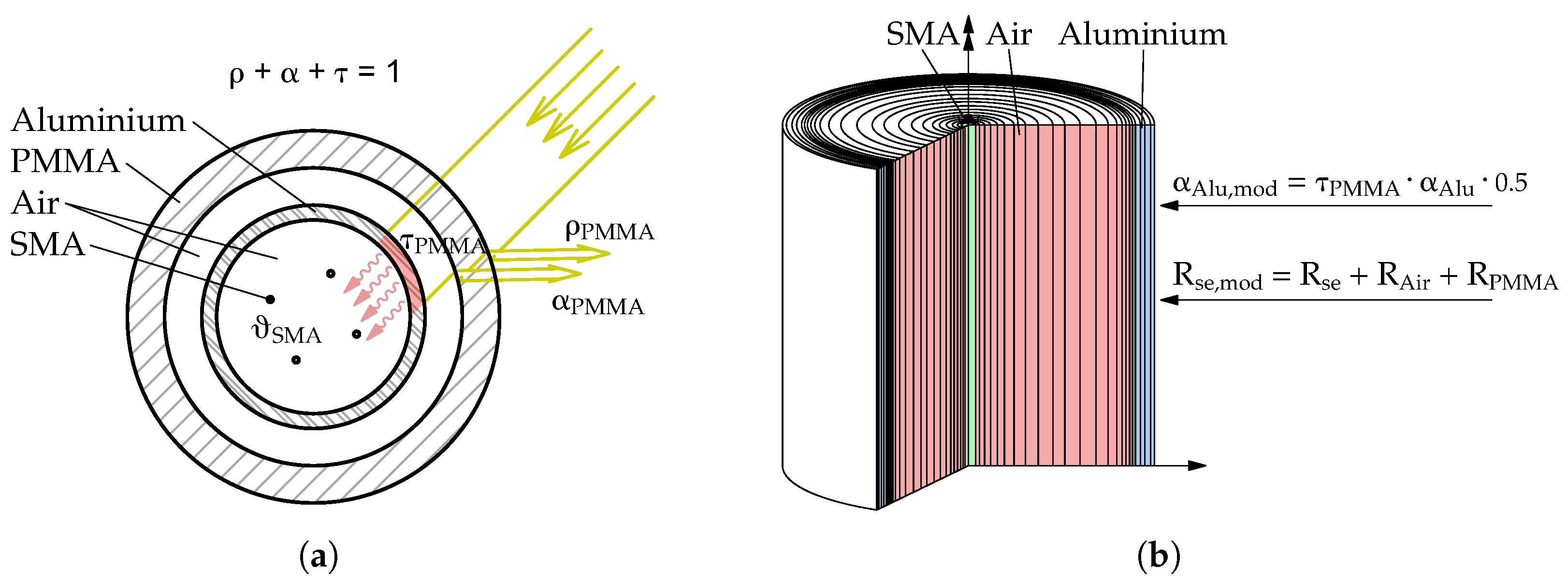
3.3.1. Model Approach for the Thermal Description of the SMA-Wire
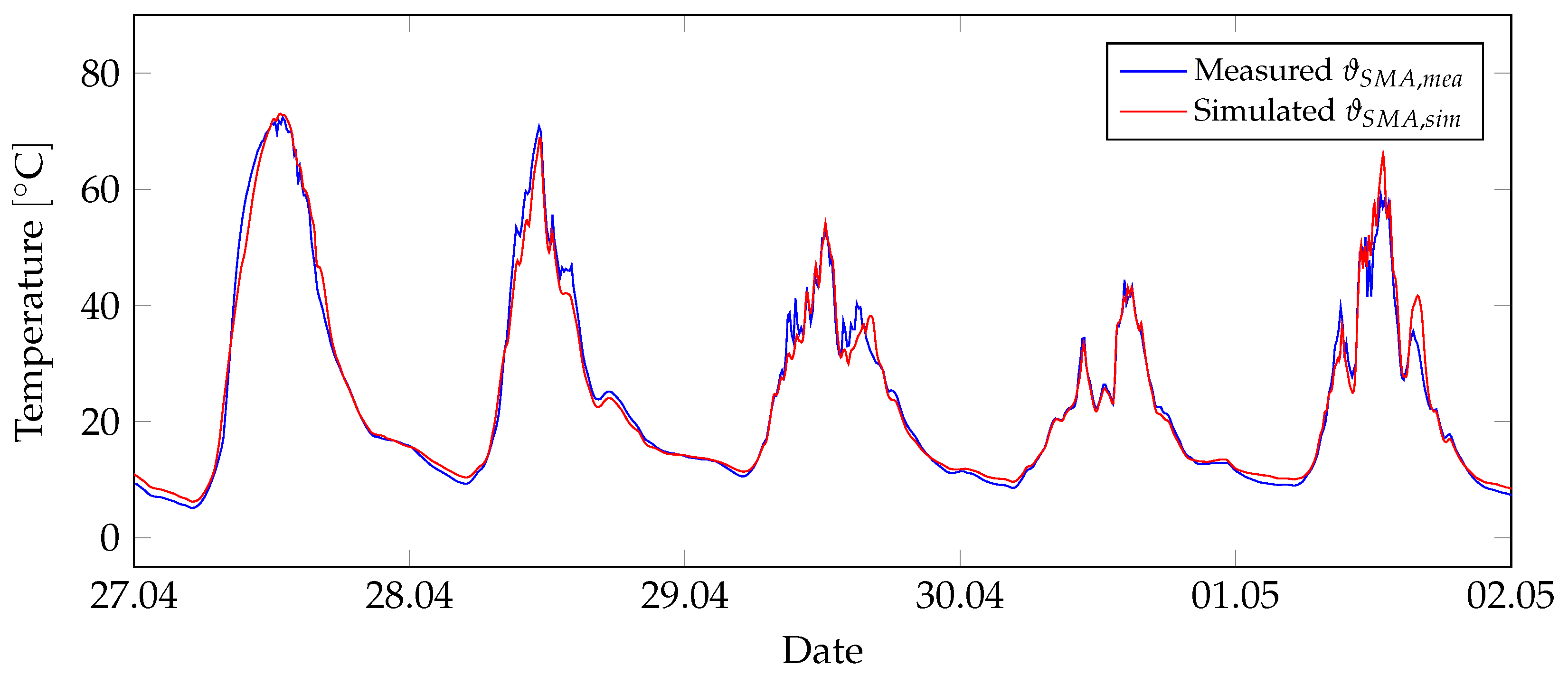
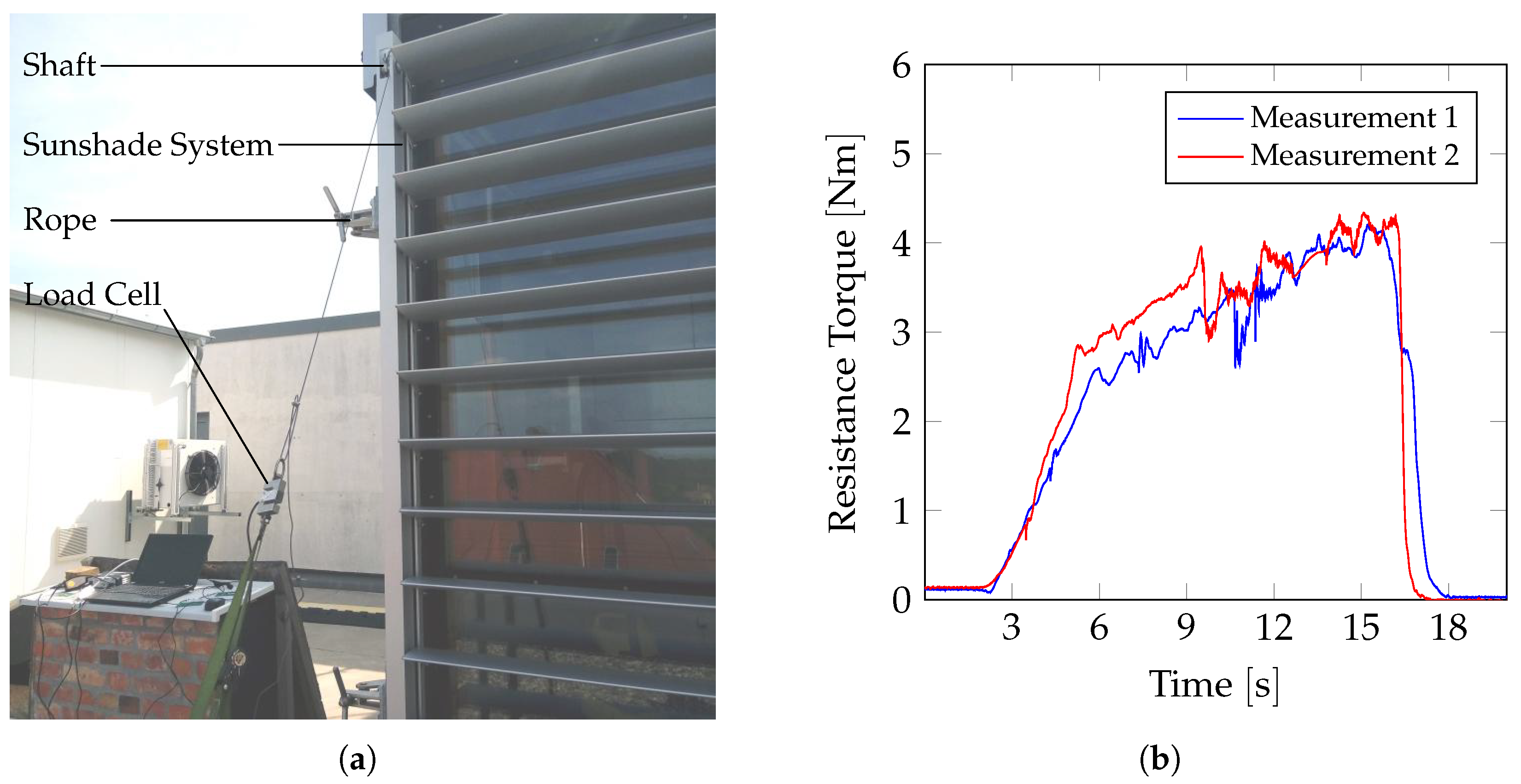
3.3.2. Experimental Validation of the Model
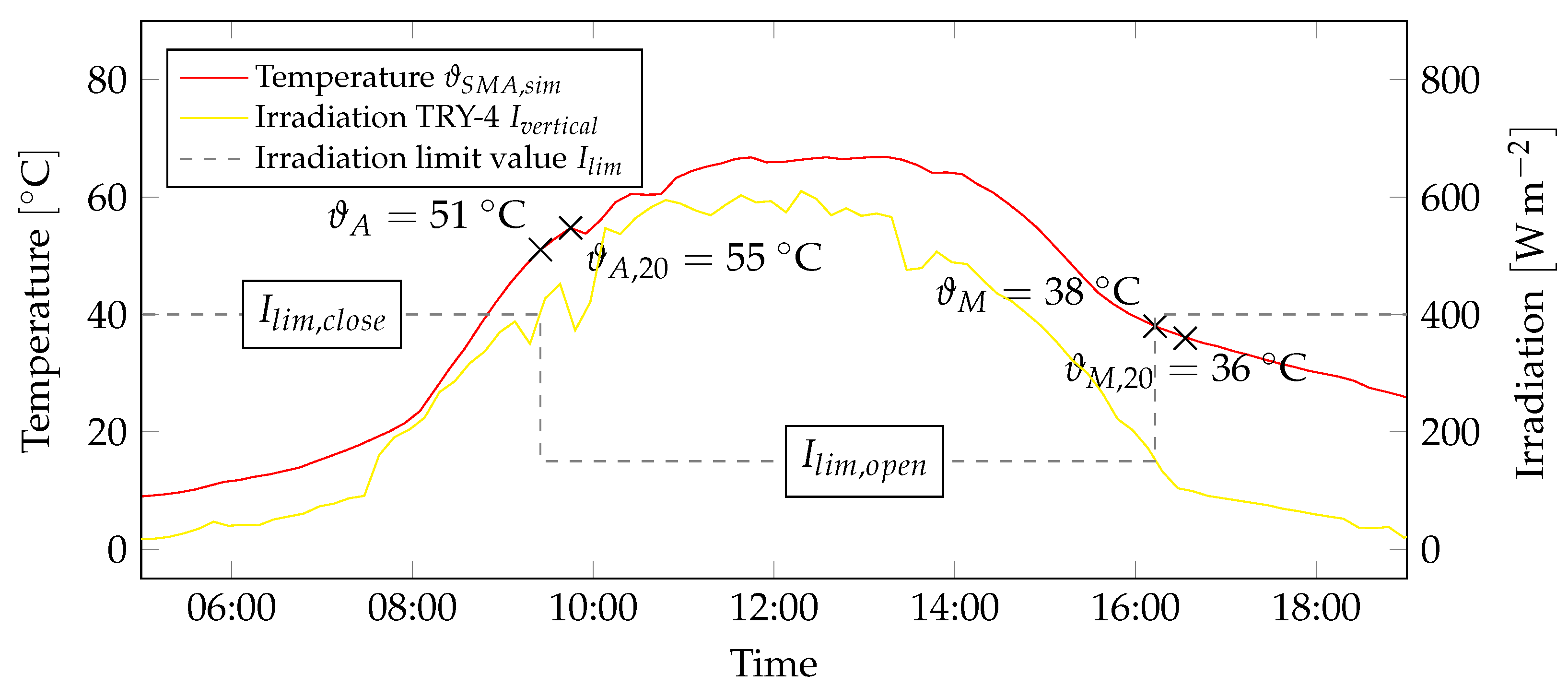
3.3.3. Defining SMA Setpoint Switching Temperatures
3.4. Determination of the System Resistance
3.5. Selection of the Shape Memory Alloy
4. Validation of the Full-Scale Demonstrator
4.1. The Demonstrator Setup
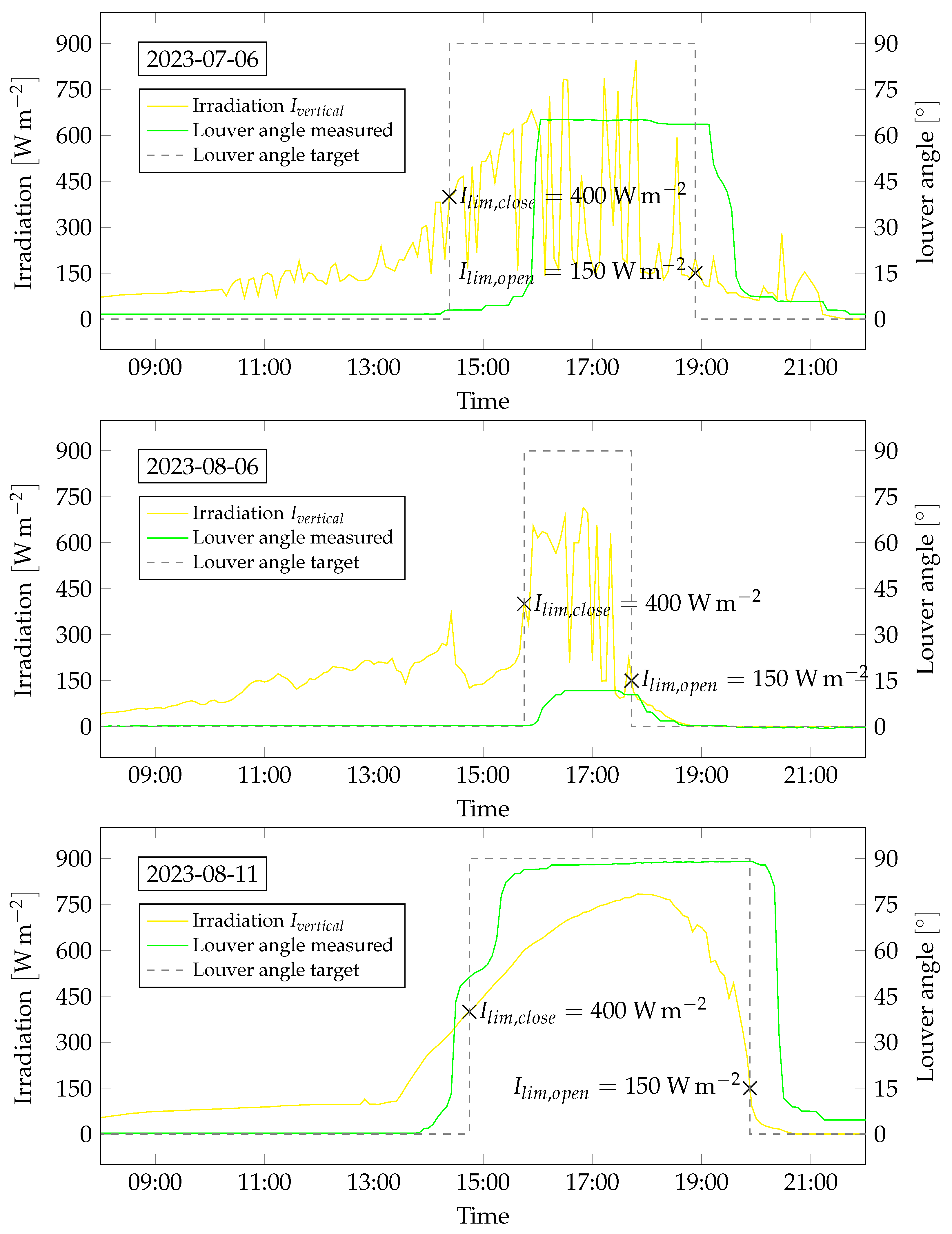
4.2. Sunshade Function
4.3. Solar Collector
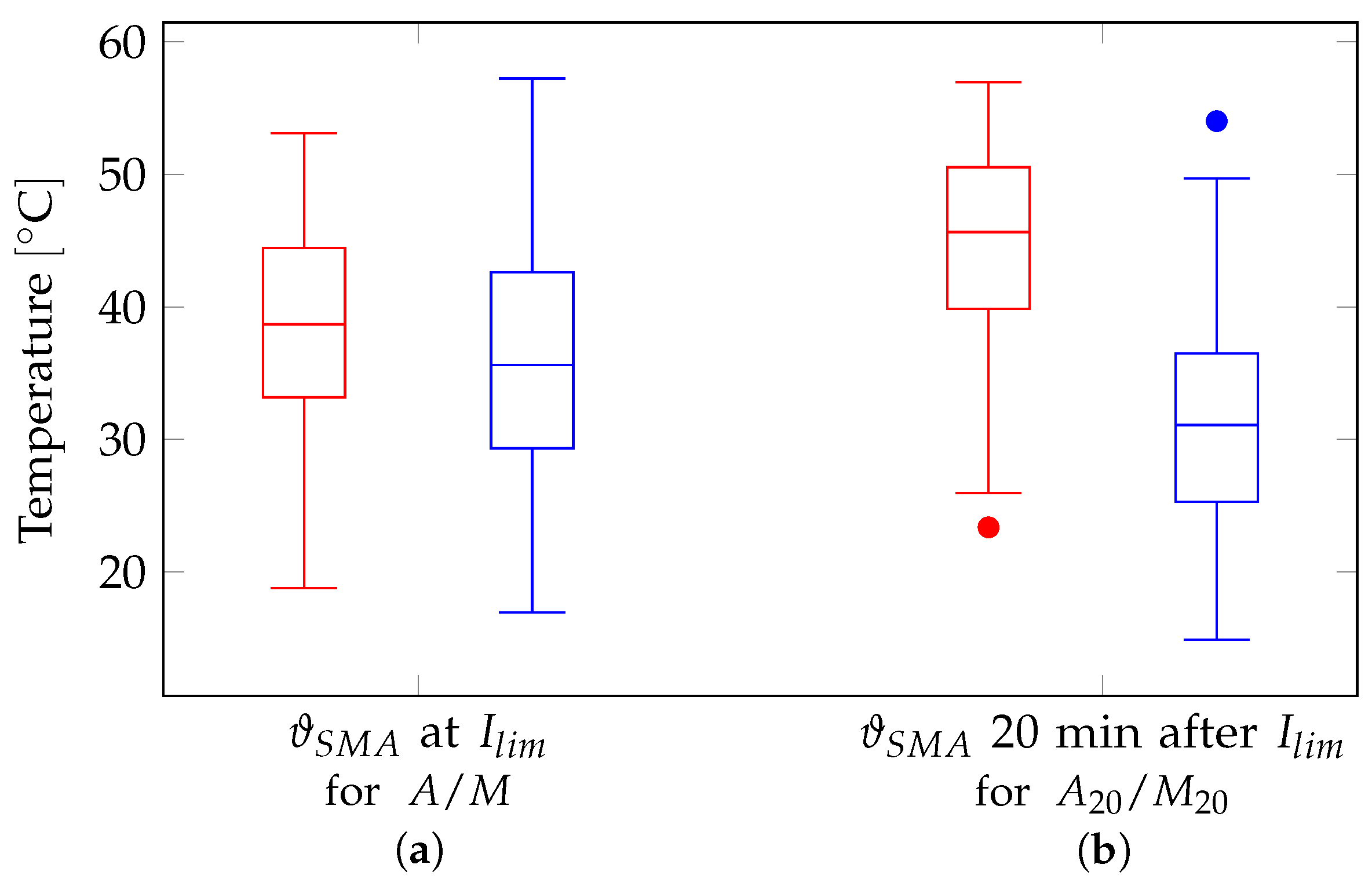
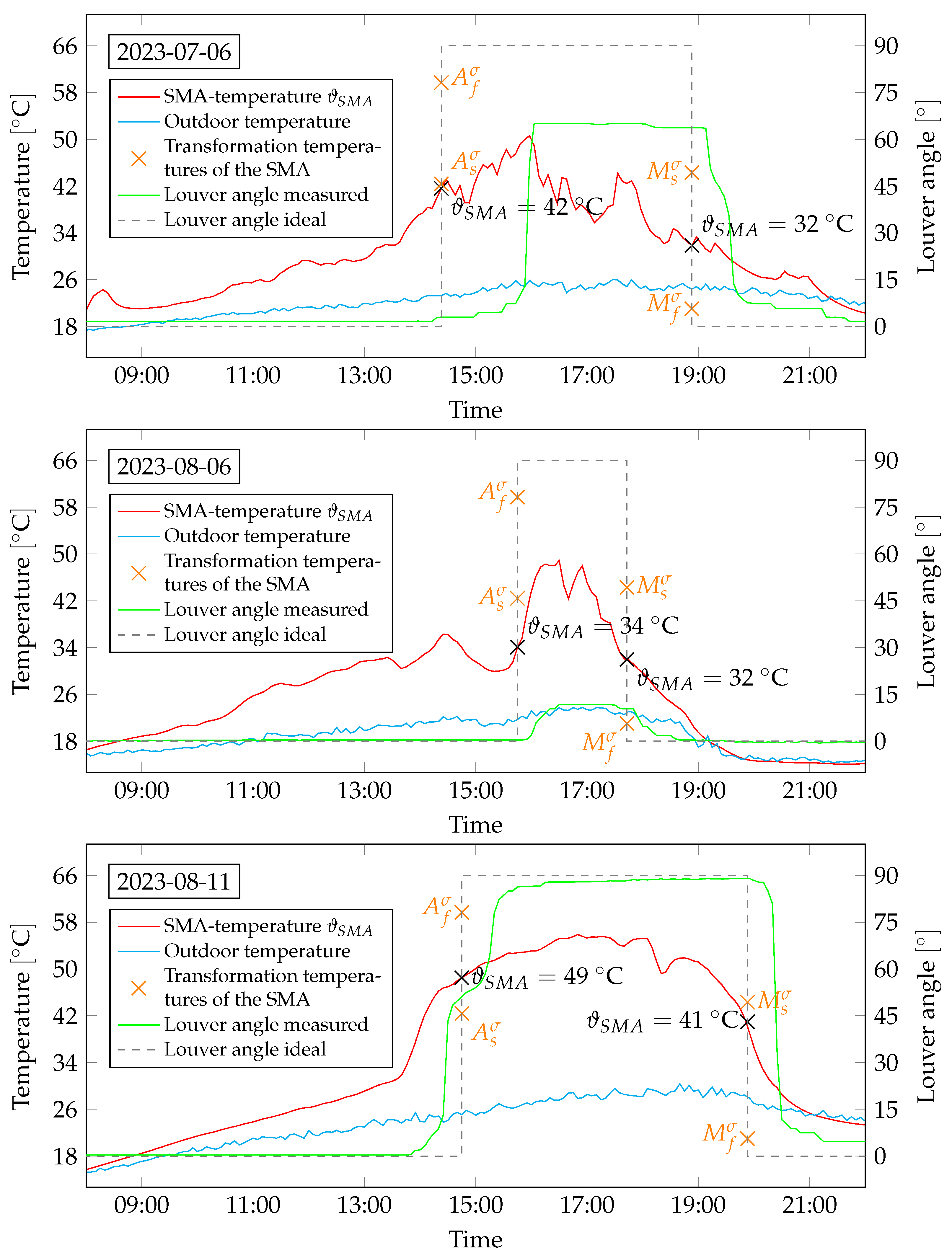
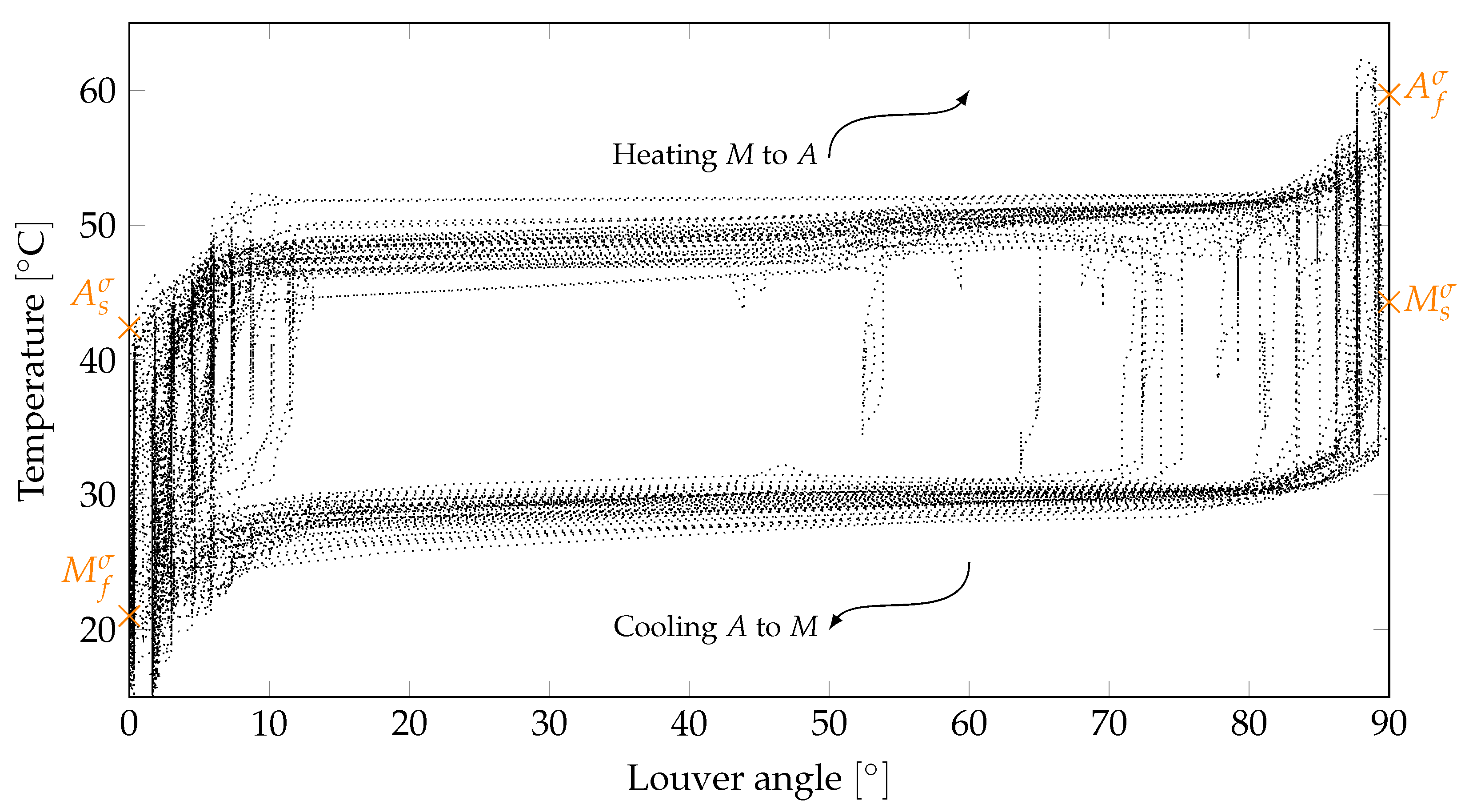
4.4. Analysis of SMA Characteristics
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Stelzmann, M.; Schreier, M.; Navarro de Sosa, I.; Schurig, M.; Syga, K.; Nemati, A. Schlussbericht Smartskinreal: Zwanzig20—Smart3—Verbundvorhaben—SmertSkinReal—Entwicklung und Monitoring eines Verschattungssystems mit FGL-Steuerung; TP1: Ermittlung von Einflüssen auf Schalttemperaturen; Report; Tibleibniz Information Centre for Science and Technology University Library: Hanover, Germany, 2023. [Google Scholar]
- Navarro de Sosa, I. Smartskin—Entwicklung von Selbstregulierenden Sonnenschutzkomponenten für die Gebäudehülle auf Basis des Thermischen Formgedächtniseffektes: Teilprojekt: FGL-Aktorsystem: Akronym: S3-Smartskin-VI: Schlussbericht; Report; Tibleibniz Information Centre for Science and Technology University Library: Hanover, Germany, 2017. [Google Scholar]
- Lagoudas, D.C. Shape Memory Alloys. Modeling and Engineering Applications; Springer: New York, NY, USA, 2008; pp. 5–15. [Google Scholar]
- Navarro de Sosa, I.; Ruff, M.; Kirmse, S.; Kahnt, A.; Drossel, W.-G. Self-regulating Solar Shading System for the Building Envelope Based on Thermal Shape Memory Effect. In Proceedings of the ACTUATOR 2018: 16th International Conference on New Actuators, Bremen, Germany, 25–27 June 2018; pp. 522–526. [Google Scholar]
- Großlamellen aus Aluminim für den Sonnenschutz Colt Solarfin—Colt. (n.d.). Available online: https://www.colt-info.de/grosslamellensystem-solarfin.html (accessed on 27 June 2023).
- EN 13659; Shutters and External Venetian Blinds—Performance Requirements Including Safety. European Committee for Standardization: Bruxelles, Belgium, 2015.
- Duerig, T.W.; Melton, K.N.; Stöckel, D.; Wayman, C.M. Engineering Aspects of Shape Memory Alloys; Butterworth-Heinemann: Oxford, UK, 1990; pp. 115–170. [Google Scholar]
- Liu, Y.; Humbeeck, J.V.; Stalmans, R.; Delaey, L. Some aspects of the properties of NiTi shape memory alloy. J. Alloys Compd. 1997, 1–2, 115–121. [Google Scholar] [CrossRef]
- Arghavani, J.; Auricchio, F.; Naghdabadi, R.; Sohrabpour, S. A 3-D phenomenological constitutive model for shape memory alloys under multiaxial loadings. Int. J. Plast. 2010, 26, 976–991. [Google Scholar] [CrossRef]
- Goel, V.; Hans, V.S.; Singh, S.; Kumar, R.; Pathak, S.K.; Singla, M.; Saini, R.P. A comprehensive study on the progressive development and applications of solar air heaters. Sol. Energy 2021, 229, 112–147. [Google Scholar] [CrossRef]
- Lopes Leal, M., Jr.; Pino, L.; Barati, M.; Saint-Sulpice, L.; Daniel, L.; Chirani, S.A. Modeling of functional fatigue of SMA-based actuators under thermomechanical loading and Joule heating. Int. J. Fatigue 2024, 179, 108055. [Google Scholar] [CrossRef]
- Yang, S.; Kang, S.; Lim, Y.-M.; Lee, Y.; Kim, J.; Nam, T. Emperature profiles in a Ti–45Ni–5Cu (at%) shape memory alloy developed by the Joule heating. J. Alloys Compd. 2010, 490, L28–L32. [Google Scholar] [CrossRef]
- Song, H.; Kubica, E.; Gorbet, R. Resistance modelling of SMA wire actuators. In Proceedings of the International Workshop Smart Materials Structures and NDT in Aerospace, Montreal, QC, Canada, 2–4 November 2011. [Google Scholar]
- IEC 61140; Protection against Electric Shock—Common Aspects for Installation and Equipment. IEC International: Geneva, Switzerland, 2016.
- Carl, M.; Zhang, B.; Young, M.L. Texture and Strain Measurements from Bending of NiTi Shape Memory Alloy Wires. Shape Mem. Superelast. 2016, 2, 254–263. [Google Scholar] [CrossRef]
- Ho, H.V.; Choi, E.; Park, S.J. Investigating stress distribution of crimped SMA fibers during pullout behavior using experimental testing and a finite element model. Compos. Struct. 2021, 272, 114254. [Google Scholar] [CrossRef]
- Mirzaeifar, R.; DesRoches, R.; Yavari, A.; Gall, K. On superelastic bending of shape memory alloy beams. Int. J. Solids Struct. 2013, 50, 1664–1680. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, X. Transformation Temperature Predictions Through Computational Intelligence for NiTi-Based Shape Memory Alloys. Shap. Mem. Superelast. 2020, 6, 374–386. [Google Scholar] [CrossRef]
- Melton, K.; Mercier, O. The mechanical properties of NiTi-based shape memory alloys. Acta Metall. 1981, 2, 393–398. [Google Scholar] [CrossRef]
- Bouaissi, A.; Radhi, N.S.; Morad, K.F.; Hafiz, K.F.; Atiyah, A.A. Optimization of nickel content on some properties of (niti) shape memory alloy. Knowl.-Based Eng. Sci. 2020, 1, 40–47. [Google Scholar] [CrossRef]
- DIN 4108-2; Thermal Protection and Energy Economy in Buildings—Part 2: Minimum Requirements to Thermal Insulation. Beuth: Berlin, Germany, 2013.
- Da Silva, P.C.; Leal, V.; Andersen, M. Influence of shading control patterns on the energy assessment of office spaces. Energy Build. 2012, 50, 35–48. [Google Scholar] [CrossRef]
- Karlsen, L.; Heiselberg, P.; Bryn, I.; Johra, H. Solar shading control strategy for office buildings in cold climate. Energy Build. 2016, 118, 316–328. [Google Scholar] [CrossRef]
- Yun, G.; Park, D.Y.; Kim, K.S. Appropriate activation threshold of the external blind for visual comfort and lighting energy saving in different climate conditions. Build. Environ. 2017, 113, 247–266. [Google Scholar] [CrossRef]
- Tabadkani, A.; Roetzel, A.; Li, H.X.; Tsangrassoulis, A.; Attia, S. Analysis of the impact of automatic shading control scenarios on occupant’s comfort and energy load. Appl. Energy 2021, 294, 116904. [Google Scholar] [CrossRef]
- Tabadkani, A.; Roetzel, A.; Li, H.X.; Tsangrassoulis, A. A review of automatic control strategies based on simulations for adaptive facades. Build. Environ. 2020, 175, 106801. [Google Scholar] [CrossRef]
- Goswami, D.Y. Solar Thermal Collectors. In Principles of Solar Engineering; CRC Press: Boca Raton, FL, USA, 2022; pp. 119–204. [Google Scholar]
- Nicolai, A.; Scheffler, G.A.; Grunewald, J.; Plagge, R. An Efficient Numerical Solution Method and lmplementation for Coupled Heat, Moisture, and Salt Transport: The DELPHIN Simulation Program. In Simulation of Time Dependent Degradation of Porous Materials, Final Report on Priority Program 1122, Funded by the German Research Foundation DFG; Franke, L., Deckelmann, G., Espinosa-Marzal, R., Eds.; Cuvillier: Göttingen, Germany, 2009; pp. 85–100. [Google Scholar]
- Spekat, A. Aktualisierte und Erweiterte Testreferenzjahre von Deutschland für Mittlere, Extreme und Zukünftige Witterungsverhältnisse; Bundesamt für Bauwesen und Raumordnung (BBR) Self-Published: Offenbach, Germany, 2011; pp. 16–18. [Google Scholar]
- Karakoc, O.; Yegin, Y.; Ozdogan, M.; Salman, M.; Nagabandi, N.; Yegin, C.; Yurukcu, M.; Murat Sari, M. Smart and state-of-the-art materials in oil and gas industry. Sustain. Mater. Transit. Altern. Energy 2021, 2, 1–51. [Google Scholar]
- Nam, T.H.; Saburi, T.; Shimizu, K. Cu-Content Dependence of Shape Memory Characteristics in Ti–Ni–Cu Alloys. Mater. Trans. JIM 1990, 31, 959–967. [Google Scholar] [CrossRef]
- Frotscher, M.; Burow, J.; Schön, P.; Neuking, K.; Böckmann, R.; Eggeler, G. Characterization of the mechanical properties of ultra-fine grained NiTiCr-wires. Mater. Werkst. 2009, 40, 17–22. [Google Scholar] [CrossRef]
- Isalgue, A.; Torra, V.; Yawny, A.; Lovey, F.C. Metastable effects on martensitic transformation in SMA. J. Therm. Anal. Calorim. 2008, 91, 991–998. [Google Scholar] [CrossRef]




| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Solar irradiation during the time when the sunshade should close | 400 | W/m2 | |
| Solar irradiation during the time when the sunshade should open | 150 | W/m2 |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Sunshade during the closing process | 45.7 | °C | |
| Sunshade during the opening process | 31.1 | °C | |
| Sunshade opening process is complete | >20.0 | °C |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Length of the SMA-wire | 1.92 | m | |
| Actuator radius | 0.0343 | m | |
| SMA cross-sectional | 1.81 | mm2 | |
| Weight force | 147.0 | N | |
| Resistance torque | 4.5 | Nm | |
| Resistance force | 131.0 | N | |
| SMA tension | 71.4 | N mm−2 | |
| SMA strain | 2.9 | % | |
| SMA stress influence coefficient | C | 4.425 | MPa K−1 |
| Martensite start temperature | 28.2 | °C | |
| Martensite finish temperature | 4.9 | °C | |
| Austenite start temperature | 26.3 | °C | |
| Austenite finish temperature | 43.6 | °C | |
| Martensite start temperature under tensile stress | 44.3 | °C | |
| Martensite finish temperature under tensile stress | 21.0 | °C | |
| Austenite start temperature under tensile stress | 42.4 | °C | |
| Austenite finish temperature under tensile stress | 59.7 | °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stelzmann, M.; Zakner, F.; Navarro de Sosa, I.; Nemati, A.; Kahnt, A.; Maaß, B.; Drossel, W.-G. Development of a Self-Regulating Solar Shading Actuator Based on the Thermal Shape Memory Effect. Actuators 2024, 13, 85. https://doi.org/10.3390/act13030085
Stelzmann M, Zakner F, Navarro de Sosa I, Nemati A, Kahnt A, Maaß B, Drossel W-G. Development of a Self-Regulating Solar Shading Actuator Based on the Thermal Shape Memory Effect. Actuators. 2024; 13(3):85. https://doi.org/10.3390/act13030085
Chicago/Turabian StyleStelzmann, Mario, Felix Zakner, Iñaki Navarro de Sosa, Amir Nemati, Alexander Kahnt, Burkhard Maaß, and Welf-Guntram Drossel. 2024. "Development of a Self-Regulating Solar Shading Actuator Based on the Thermal Shape Memory Effect" Actuators 13, no. 3: 85. https://doi.org/10.3390/act13030085
APA StyleStelzmann, M., Zakner, F., Navarro de Sosa, I., Nemati, A., Kahnt, A., Maaß, B., & Drossel, W.-G. (2024). Development of a Self-Regulating Solar Shading Actuator Based on the Thermal Shape Memory Effect. Actuators, 13(3), 85. https://doi.org/10.3390/act13030085






