Reduced-Order Observer-Based Position Control of a Magnetic-Geared Servo Drive
Abstract
:1. Introduction
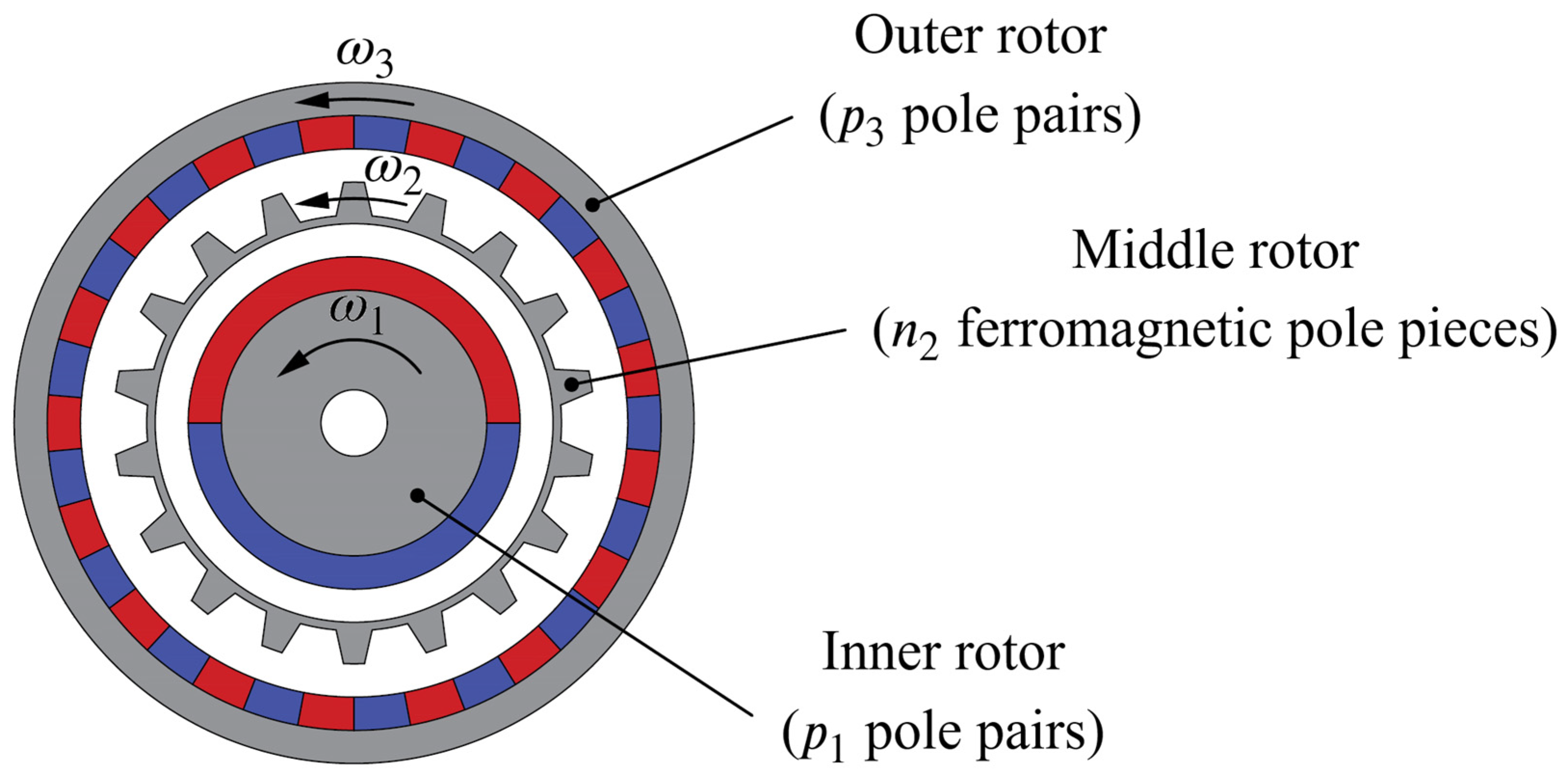
1.1. Essentials of Coaxial MGs
1.2. Control of Magnetic-Geared Drives: State-of-the-Art
1.3. Contribution and Overview of the Paper
- Most of the research in this field has been focusing on speed rather than position control. The latter is, however, often used, especially in servo systems such as industrial robotic manipulators and computer numerically controlled (CNC) machine tools for which high-precision positioning is of great importance.
- Magnetic-geared drives are weakly damped systems in which fast torque actuation can easily excite torsional oscillations on both rotors. Therefore, control systems for magnetic-geared drives should be carefully designed in order to suppress those oscillations and ensure their high-performance dynamic behaviour.
2. Mathematical Model of a Magnetic-Geared PMSM
2.1. Mathematical Model of a PMSM
2.2. Mathematical Model of an MG
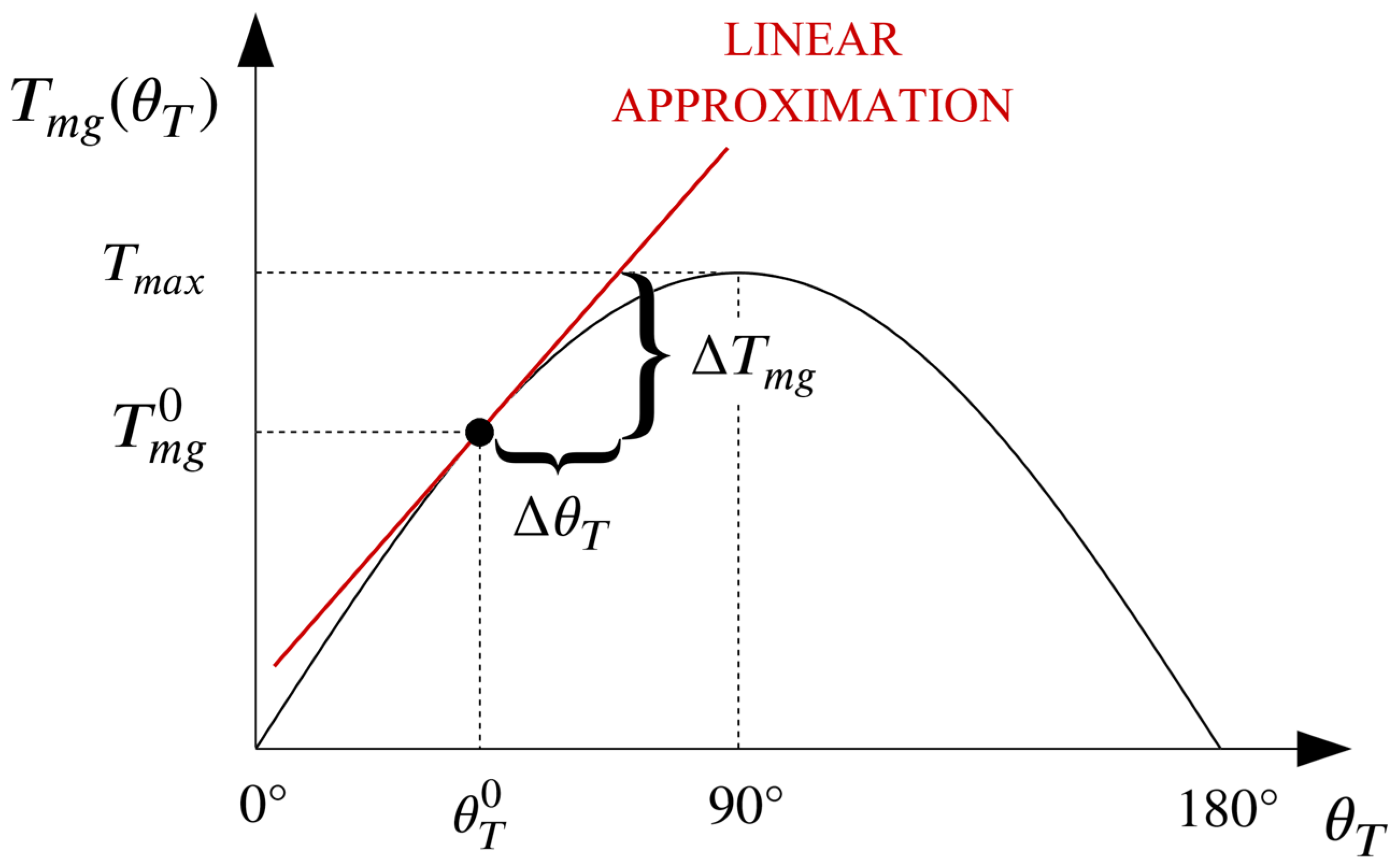
2.2.1. Nonlinear Model of an MG
2.2.2. Linearized Model of an MG
3. Observer-Based Position Control System
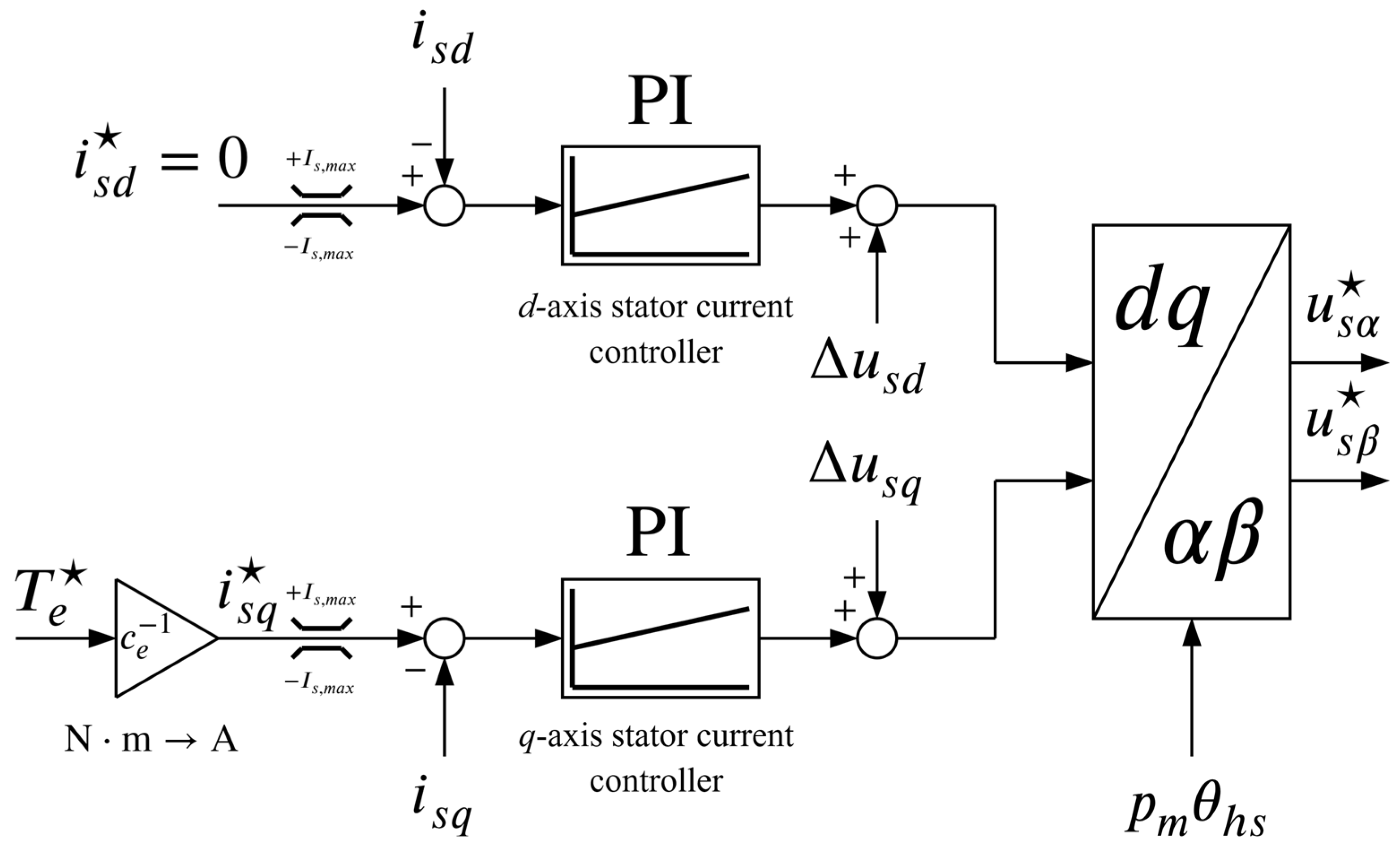
3.1. Design of the State Feedback Position Controller
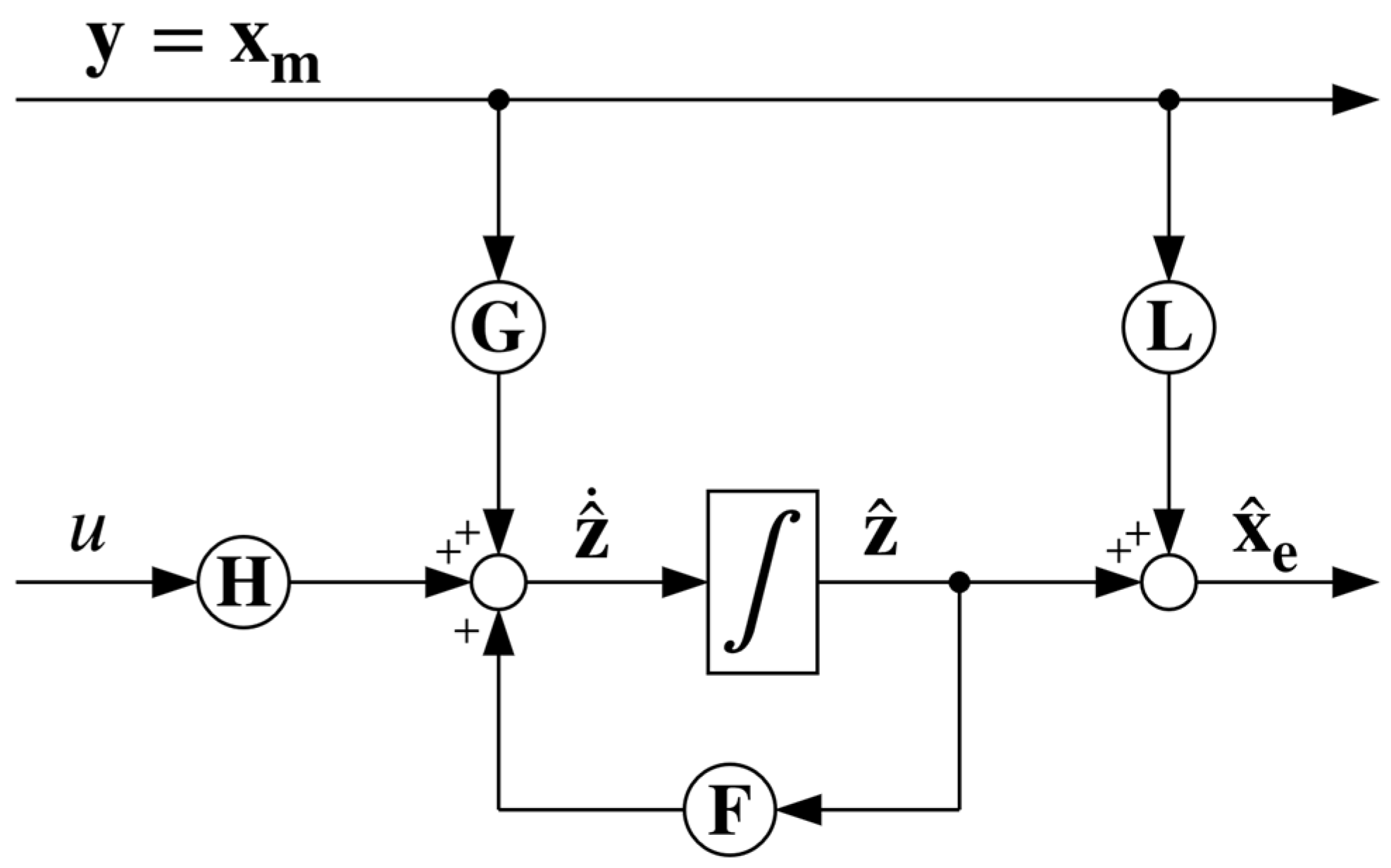
3.2. Design of the Reduced-Order ESO
3.3. Compensation of the Steady-State Estimation Error
4. Real-Time Implementation and Experimental Evaluation
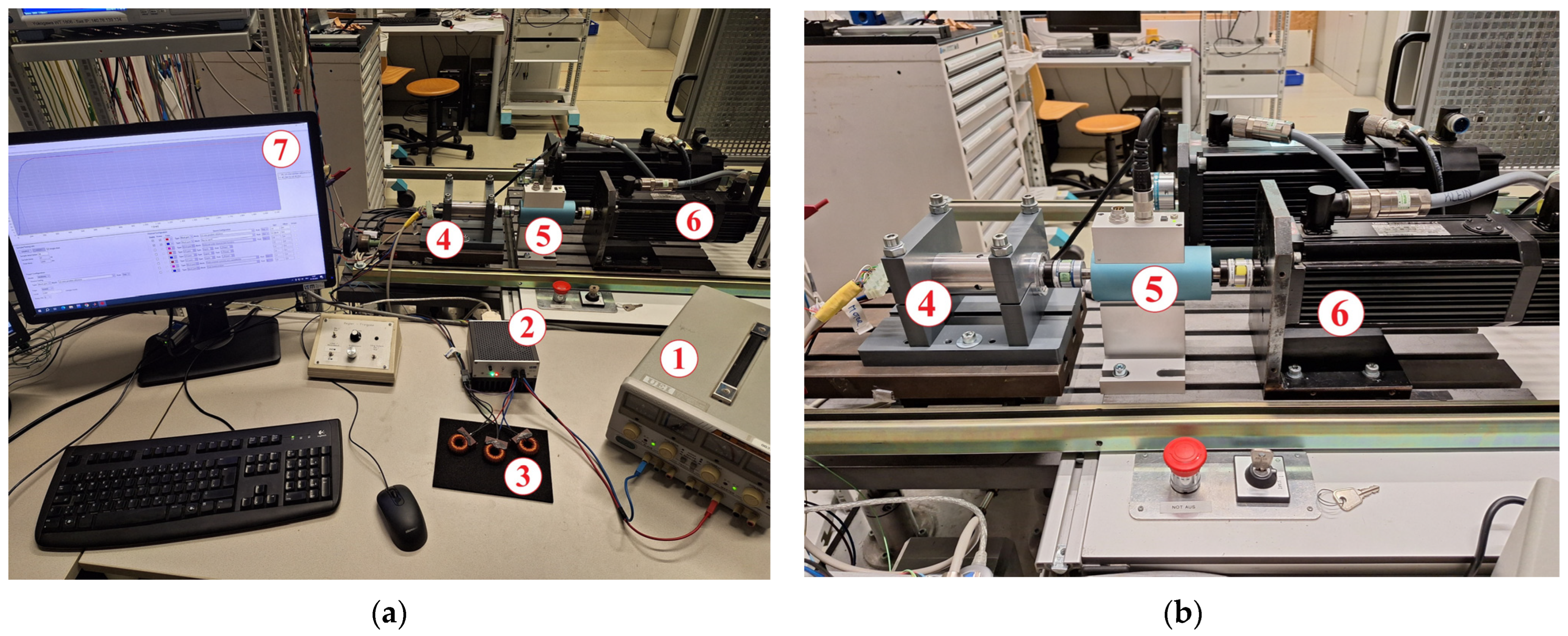
4.1. Experimental Setup
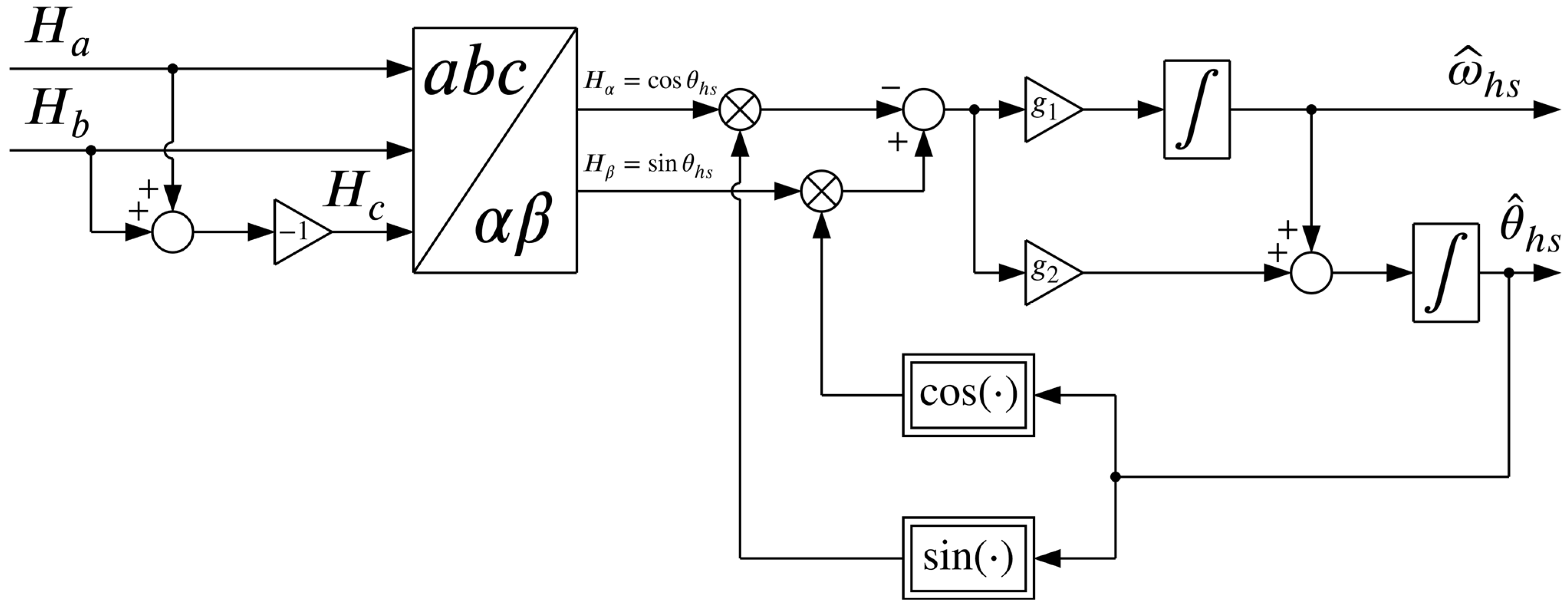
4.2. Measurement Processing
4.3. Static Measurements
4.4. Experimental Evaluation of the Presented Position Control System
4.4.1. Experimental Results: Current Control
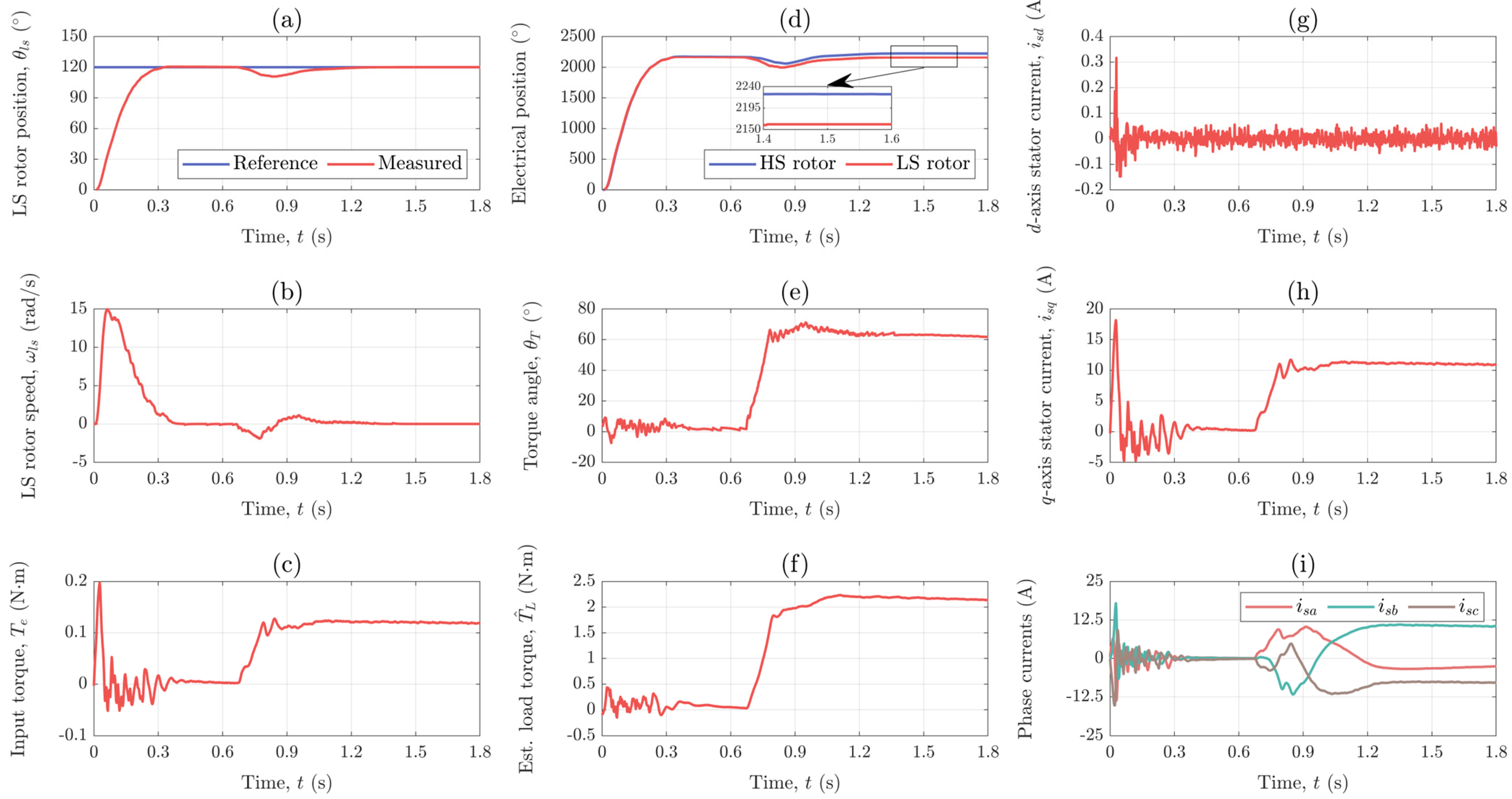
4.4.2. Experimental Results: Position Control (Scenario I)
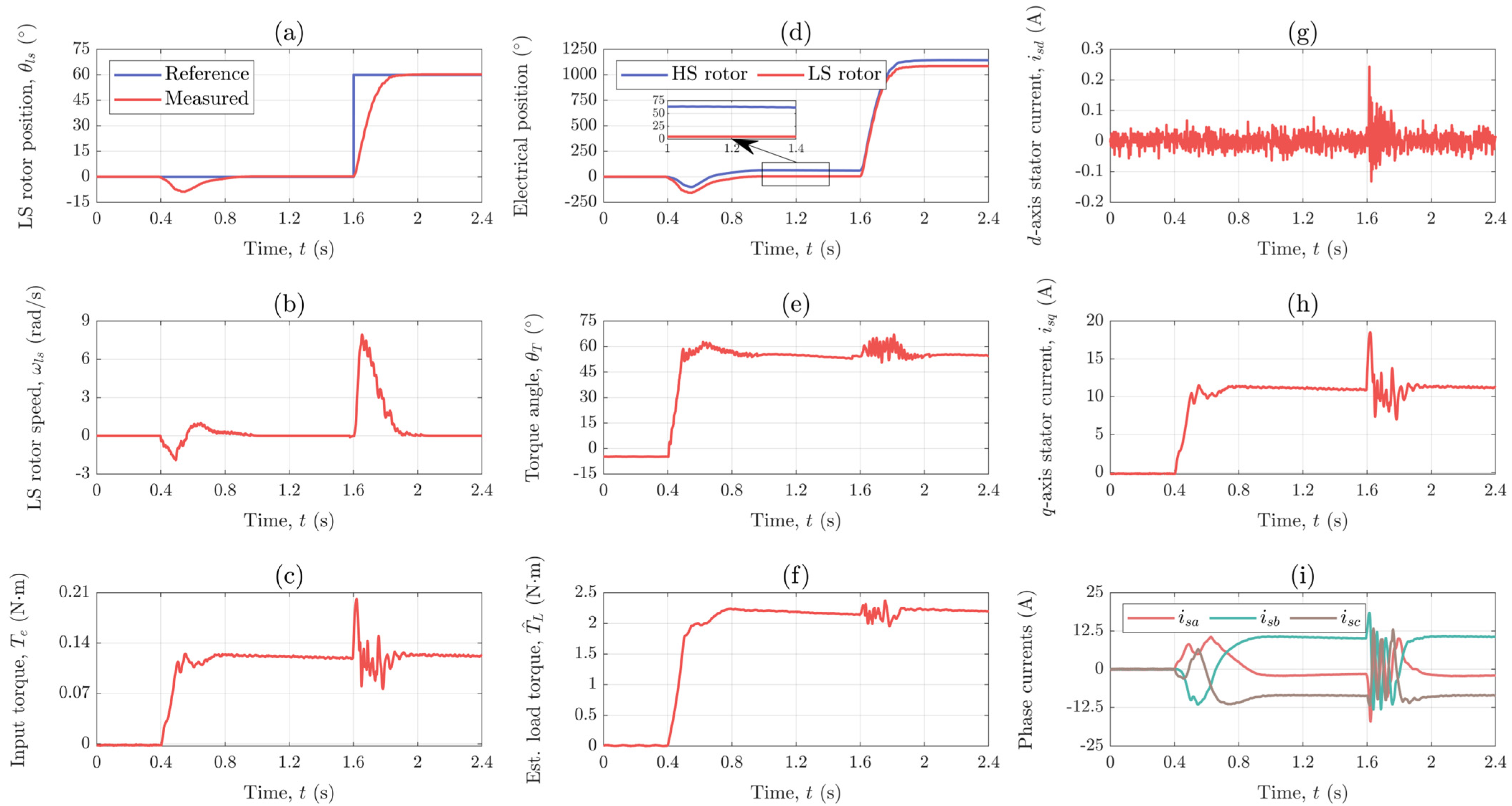
4.4.3. Experimental Results: Position Control (Scenario II)
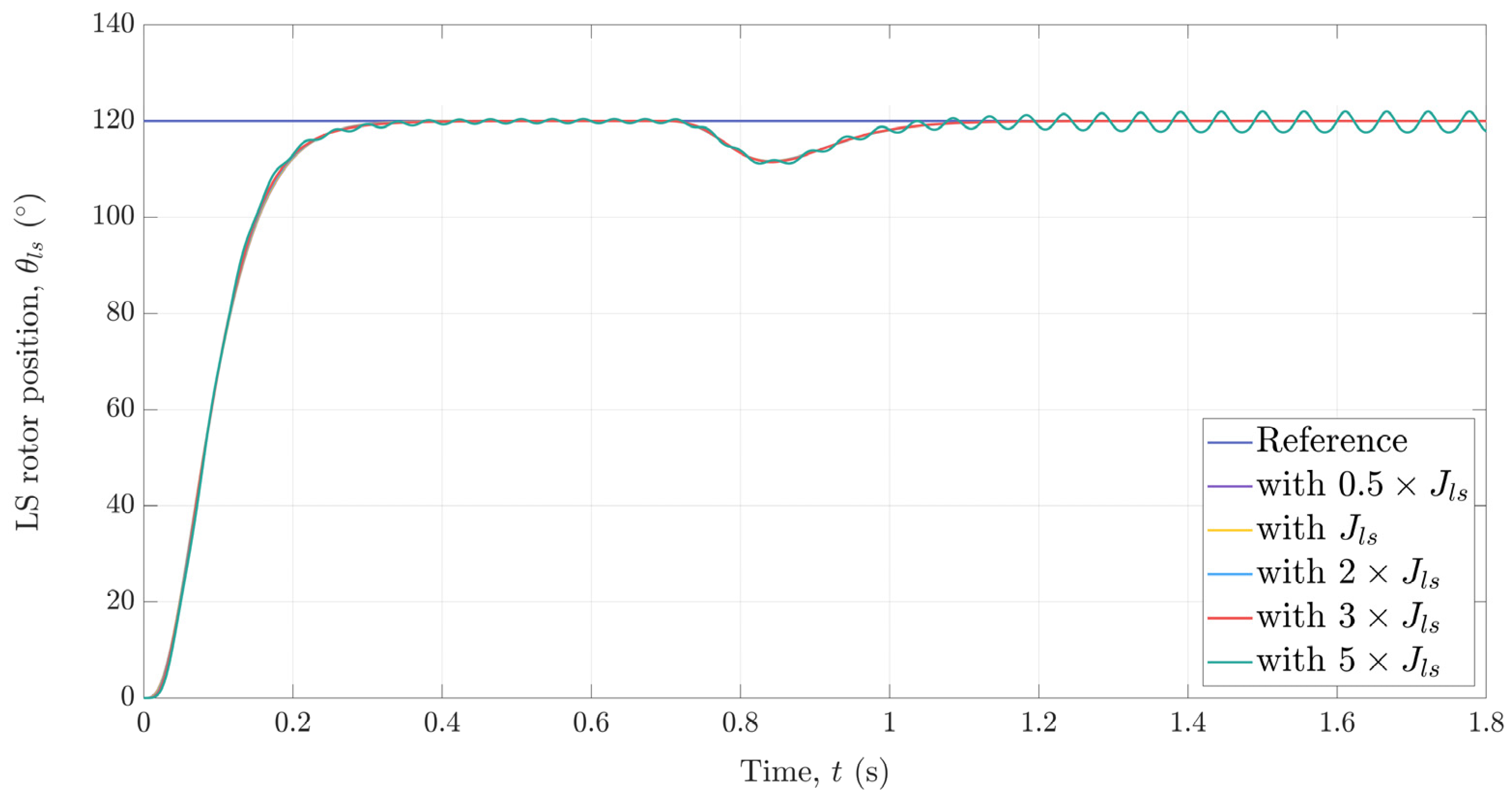
5. Simulation-Based Robustness Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
| Physical Quantity | Value | Unit |
|---|---|---|
| 13.45 | V | |
| 9.30 | A | |
| 22,644 | rpm |
| Physical Quantity | Value | Unit |
|---|---|---|
| 0.0650 | Ω | |
| d- | 2.56 × 10−5 | H |
| q- | 2.94 × 10−5 | H |
| 0.0073 | V·s | |
| 1 | - |
| Physical Quantity | Value | Unit |
|---|---|---|
| 0.07 | Ω | |
| 29.3 × 10−5 | H |
| Physical Quantity | Value | Unit |
|---|---|---|
| 2.489 | N·m | |
| 1 | - | |
| 1.3186 × 10−5 | kg·m2 | |
| 3.2930 × 10−6 | N·m·s | |
| 18 | - | |
| 1.3437 × 10−5 | kg·m2 | |
| 2.7380 × 10−4 | kg·m2 | |
| 2.2797 × 10−4 | N·m·s |
Appendix C
| Torque Angle, (°) | 0 | 11.08 | 20.58 | 30.05 | 39.60 | 50.63 | 60.10 | 69.60 | 80.70 | 89.40 |
| Transmitted torque, (N·m) | 0.081 | 0.479 | 0.812 | 1.138 | 1.430 | 1.778 | 2.030 | 2.220 | 2.402 | 2.489 |
References
- Nielsen, S.S.; Holm, R.K.; Rasmussen, P.O. Conveyor System With a Highly Integrated Permanent Magnet Gear and Motor. IEEE Trans. Ind. Appl. 2020, 56, 2550–2559. [Google Scholar] [CrossRef]
- Frandsen, T.V.; Mathe, L.; Berg, N.I.; Holm, R.K.; Matzen, T.N.; Rasmussen, P.O.; Jensen, K.K. Motor Integrated Permanent Magnet Gear in a Battery Electrical Vehicle. IEEE Trans. Ind. Appl. 2015, 51, 1516–1525. [Google Scholar] [CrossRef]
- Jian, L.; Chau, K.T.; Jiang, J.Z. A Magnetic-Geared Outer-Rotor Permanent-Magnet Brushless Machine for Wind Power Generation. IEEE Trans. Ind. Appl. 2009, 45, 954–962. [Google Scholar] [CrossRef]
- Hazra, S.; Kamat, P.; Bhattacharya, S.; Ouyang, W.; Englebretson, S. Power Conversion With a Magnetically-Geared Permanent Magnet Generator for Low-Speed Wave Energy Conversion. IEEE Trans. Ind. Appl. 2020, 56, 5308–5318. [Google Scholar] [CrossRef]
- Martin, T.B. Magnetic Transmission. U.S. Patent 3378710A, 16 April 1968. [Google Scholar]
- Atallah, K.; Howe, D. A Novel High-Performance Magnetic Gear. IEEE Trans. Magn. 2001, 37, 2844–2846. [Google Scholar] [CrossRef]
- Atallah, K.; Calverley, S.D.; Howe, D. Design, analysis and realization of a high-performance magnetic gear. IEE Proc.-Electr. Power Appl. 2004, 151, 135–143. [Google Scholar] [CrossRef]
- Rasmussen, P.O.; Andersen, T.O.; Jørgensen, F.T.; Nielsen, O. Development of a High-Performance Magnetic Gear. IEEE Trans. Ind. Appl. 2005, 41, 764–770. [Google Scholar] [CrossRef]
- Jungmayr, G.; Loeffler, J.; Winter, B.; Jeske, F.; Amrhein, W. Magnetic Gear: Radial Force, Cogging Torque, Skewing, and Optimization. IEEE Trans. Ind. Appl. 2016, 52, 3822–3830. [Google Scholar] [CrossRef]
- Göbl, E.; Jungmayr, G.; Marth, E.; Amrhein, W. Optimization and Comparison of Coaxial Magnetic Gears With and Without Back Iron. IEEE Trans. Magn. 2018, 54, 1–4. [Google Scholar] [CrossRef]
- Wong, H.Y.; Baninajar, H.; Dechant, B.W.; Southwick, P.; Bird, J.Z. Experimentally Testing a Halbach Rotor Coaxial Magnetic Gear With 279 Nm/L Torque Density. IEEE Trans. Energy Convers. 2023, 38, 507–518. [Google Scholar] [CrossRef]
- Wang, Y.; Filippini, M.; Bianchi, N.; Alotto, P. A Review on Magnetic Gears: Topologies, Computational Models, and Design Aspects. IEEE Trans. Ind. Appl. 2019, 55, 4557–4566. [Google Scholar] [CrossRef]
- Chen, Y.; Fu, W.N.; Ho, S.L.; Liu, H. A Quantitative Comparison Analysis of Radial-Flux, Transverse-Flux, and Axial-Flux Magnetic Gears. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Gardner, M.C.; Johnson, M.; Toliyat, H. Comparison of Surface Mounted Permanent Magnet Axial and Radial Flux Coaxial Magnetic Gears. IEEE Trans. Energy Convers. 2018, 33, 2250–2259. [Google Scholar] [CrossRef]
- Jian, L.; Deng, Z.; Shi, Y.; Wei, J.; Chan, C.C. The Mechanism How Coaxial Magnetic Gear Transmits Magnetic Torques Between Its Two Rotors: Detailed Analysis of Torque Distribution on Modulating Ring. IEEE/ASME Trans. Mechatron. 2019, 24, 763–773. [Google Scholar] [CrossRef]
- Montague, R.; Bingham, C. Nonlinear Control of Magnetically-geared Drive-trains. Int. J. Autom. Comput. 2013, 10, 319–326. [Google Scholar] [CrossRef]
- Verbanac, N.; Bulić, N.; Jungmayr, G.; Marth, E. Nonlinear Position Control of a Magnetic-Geared Servo Drive With Unknown Load. In Proceedings of the 2022 International Conference on Smart Systems and Technologies (SST), Osijek, Croatia, 19–20 October 2022; pp. 263–268. [Google Scholar] [CrossRef]
- Montague, R.G.; Bingham, C.M.; Atallah, K. Magnetic gear overload detection and remedial strategies for servo-drive systems. In Proceedings of the 2010 International Symposium on Power Electronics, Electrical Drives, Automation and Motion (SPEEDAM), Pisa, Italy, 14–16 June 2010; pp. 523–528. [Google Scholar] [CrossRef]
- Montague, R.; Bingham, C.; Atallah, K. Servo Control of Magnetic Gears. IEEE/ASME Trans. Mechatron. 2012, 17, 269–278. [Google Scholar] [CrossRef]
- Liao, X.; Bingham, C.; Zolotas, A.; Zhang, Q.; Smith, T. Servo Control of Drive-Trains Incorporating Magnetic Couplings. IEEE Trans. Ind. Appl. 2022, 58, 3674–3684. [Google Scholar] [CrossRef]
- Liao, X.; Bingham, C.; Smith, T. Speed Control of Magnetic Drive-Trains with Pole-Slipping Amelioration. Energies 2022, 15, 8148. [Google Scholar] [CrossRef]
- Bouheraoua, M.; Wang, J.; Atallah, K. Design and implementation of an observer-based state feedback controller for a pseudo direct drive. IET Electr. Power Appl. 2013, 7, 643–653. [Google Scholar] [CrossRef]
- Bouheraoua, M.; Wang, J.; Atallah, K. Speed Control for a Pseudo Direct Drive Permanent-Magnet Machine With One Position Sensor on Low-Speed Rotor. IEEE Trans. Ind. Appl. 2014, 50, 3825–3833. [Google Scholar] [CrossRef]
- Bouheraoua, M.; Wang, J.; Atallah, K. Slip Recovery and Prevention in Pseudo Direct Drive Permanent-Magnet Machines. IEEE Trans. Ind. Appl. 2015, 51, 2291–2299. [Google Scholar] [CrossRef]
- Bouheraoua, M.; Wang, J.; Atallah, K. Rotor Position Estimation of a Pseudo Direct-Drive PM Machine Using Extended Kalman Filter. IEEE Trans. Ind. Appl. 2017, 53, 1088–1095. [Google Scholar] [CrossRef]
- Montague, R.G.; Bingham, C.; Atallah, K. Magnetic Gear Pole-Slip Prevention Using Explicit Model Predictive Control. IEEE/ASME Trans. Mechatron. 2012, 18, 1535–1543. [Google Scholar] [CrossRef]
- Montague, R.G.; Bingham, C.M.; Atallah, K. Dual-observer-based position-servo control of a magnetic gear. IET Electr. Power Appl. 2011, 5, 708–714. [Google Scholar] [CrossRef]
- Ohm, D.Y. Analysis of PID and PDF compensators for motion control systems. In Proceedings of the 1994 IEEE Industry Applications Society Annual Meeting, Denver, CO, USA, 2–6 October 1994; pp. 1923–1929. [Google Scholar] [CrossRef]
- O’Sullivan, T.M.; Bingham, C.M.; Schofield, N. High-Performance Control of Dual-Inertia Servo-Drive Systems Using Low-Cost Integrated SAW Torque Transducers. IEEE Trans. Ind. Electron. 2006, 53, 1226–1237. [Google Scholar] [CrossRef]
- Chiasson, J. Modeling and High-Performance Control of Electric Machines, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005. [Google Scholar]
- Bodson, M.; Chiasson, J.N.; Novotnak, R.T.; Rekowski, R.B. High-Performance Nonlinear Feedback Control of a Permanent Magnet Stepper Motor. IEEE Trans. Control. Syst. Technol. 1993, 1, 5–14. [Google Scholar] [CrossRef]
- Harnefors, L.; Nee, H.-P. Model-Based Current Control of AC Machines Using the Internal Model Control Method. IEEE Trans. Ind. Appl. 1998, 34, 133–141. [Google Scholar] [CrossRef]
- Yuan, M.; Manzie, C.; Good, M.; Shames, I.; Gan, L.; Keynejad, F.; Robinette, T. A review of industrial tracking control algorithms. Control Eng. Pract. 2020, 102, 104536. [Google Scholar] [CrossRef]
- Saarakkala, S.E.; Hinkkanen, M. State-Space Control of Two-Mass Mechanical Systems: Analytical Tuning and Experimental Evaluation. IEEE Trans. Ind. Appl. 2014, 50, 3428–3437. [Google Scholar] [CrossRef]
- Ji, J.K.; Sul, S.K. Kalman Filter and LQ Based Speed Controller for Torsional Vibration Suppression in a 2-Mass Motor Drive System. IEEE Trans. Ind. Electron. 1995, 42, 564–571. [Google Scholar] [CrossRef]
- Chiasson, J. An Introduction to System Modeling and Control, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2022. [Google Scholar]
- Luenberger, D.G. An Introduction to Observers. IEEE Trans. Autom. Control 1971, 16, 596–602. [Google Scholar] [CrossRef]
- Jungmayr, G.; Weidenholzer, G.; Marth, E. Electrical Machine with Electric Motor and Magnetic Gear. U.S. Patent 20210265905A1, 26 August 2021. [Google Scholar]
- Jungmayr, G.; Marth, E.; Segon, G. Magnetic-Geared Motor in Side-by-Side Arrangement—Concept and Design. In Proceedings of the 2019 IEEE International Electric Machines & Drives Conference (IEMDC), San Diego, CA, USA, 12–15 May 2019; pp. 847–853. [Google Scholar] [CrossRef]
- Marth, E.; Jungmayr, G.; Amrhein, W.; Jeske, F. Magnetic-Geared Motor in Side-by-Side Arrangement—Optimization. In Proceedings of the 2019 IEEE International Electric Machines & Drives Conference (IEMDC), San Diego, CA, USA, 12–15 May 2019; pp. 839–845. [Google Scholar] [CrossRef]
- Power Inverters—X2C—Linz Center of Mechatronics. Available online: https://x2c.lcm.at/power-inverters/ (accessed on 5 October 2023).
- TM4C123BE6PZ—Texas Instruments. Available online: https://www.ti.com/product/TM4C123BE6PZ (accessed on 5 October 2023).
- X2C. Available online: https://x2c.lcm.at/ (accessed on 5 October 2023).
- Pole Placement Design—MATLAB Place—MathWorks. Available online: https://de.mathworks.com/help/control/ref/place.html (accessed on 6 October 2023).
- Harnefors, L.; Nee, H.-P. A General Algorithm for Speed and Position Estimation of AC Motors. IEEE Trans. Ind. Electron. 2000, 47, 77–83. [Google Scholar] [CrossRef]





| Controller | ||
|---|---|---|
| d-axis current controller | 0.9559 | 405 |
| q-axis current controller | 0.9673 | 405 |
| Gain | |||||
|---|---|---|---|---|---|
| Value | 0.0049 | 0.0532 | −0.0662 | −0.3340 | 6.1471 |
| Gain | |||
|---|---|---|---|
| Value | 0.8656 | 0.0042 | −0.0974 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Verbanac, N.; Jungmayr, G.; Marth, E.; Bulić, N. Reduced-Order Observer-Based Position Control of a Magnetic-Geared Servo Drive. Actuators 2024, 13, 6. https://doi.org/10.3390/act13010006
Verbanac N, Jungmayr G, Marth E, Bulić N. Reduced-Order Observer-Based Position Control of a Magnetic-Geared Servo Drive. Actuators. 2024; 13(1):6. https://doi.org/10.3390/act13010006
Chicago/Turabian StyleVerbanac, Nardi, Gerald Jungmayr, Edmund Marth, and Neven Bulić. 2024. "Reduced-Order Observer-Based Position Control of a Magnetic-Geared Servo Drive" Actuators 13, no. 1: 6. https://doi.org/10.3390/act13010006
APA StyleVerbanac, N., Jungmayr, G., Marth, E., & Bulić, N. (2024). Reduced-Order Observer-Based Position Control of a Magnetic-Geared Servo Drive. Actuators, 13(1), 6. https://doi.org/10.3390/act13010006






