Minimal Bio-Inspired Crawling Robots with Motion Control Capabilities
Abstract
:1. Introduction
2. Methods
2.1. Robot Design
2.2. Robot Prototyping
2.3. Robot Control
3. Mathematical Modeling
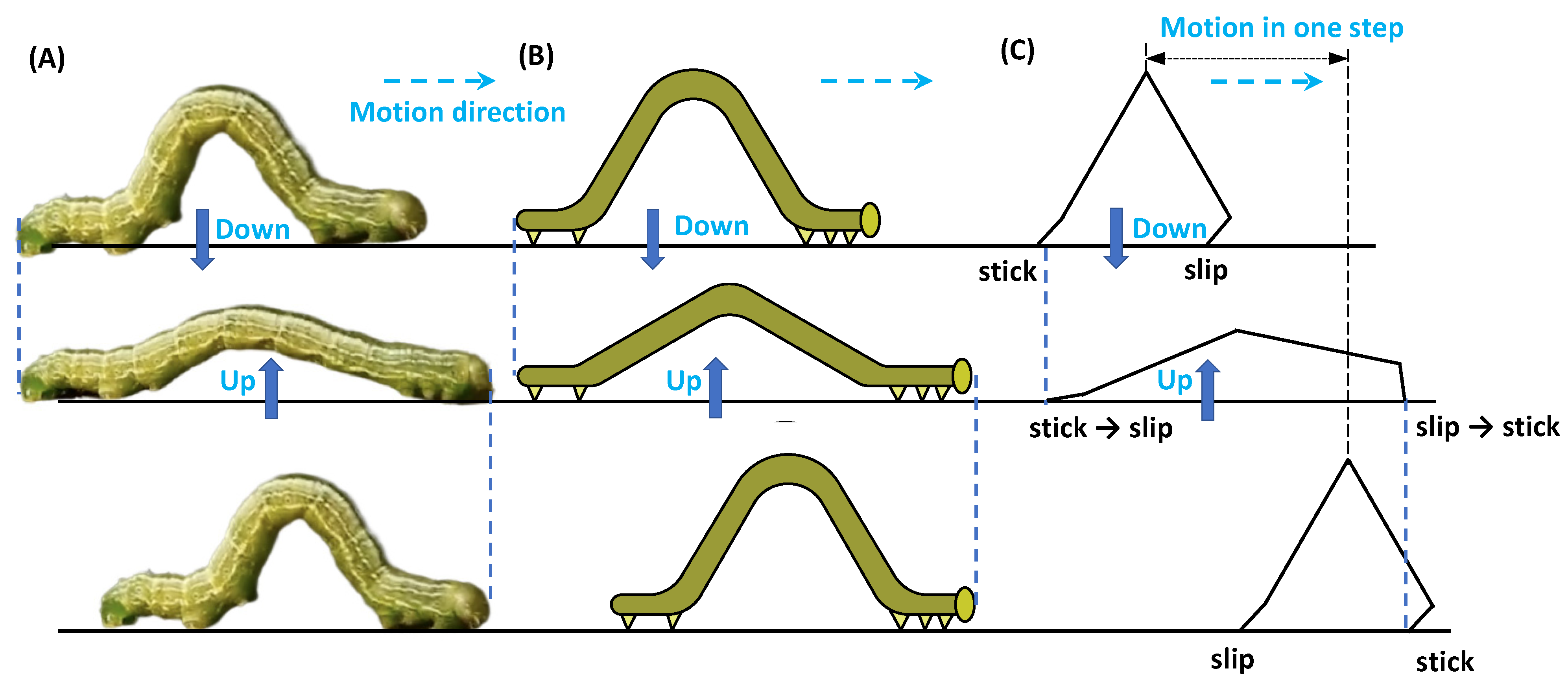
3.1. Modeling Straight Motion
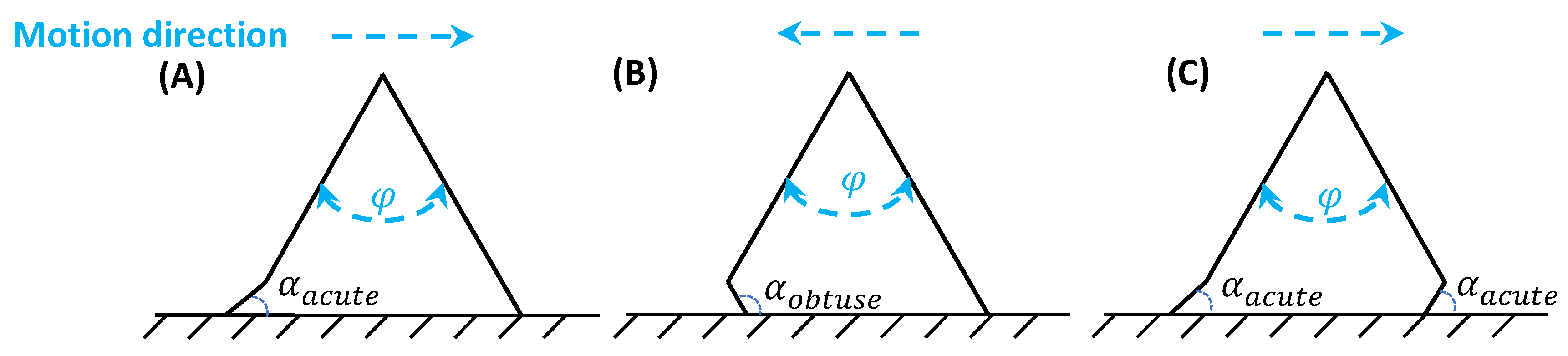
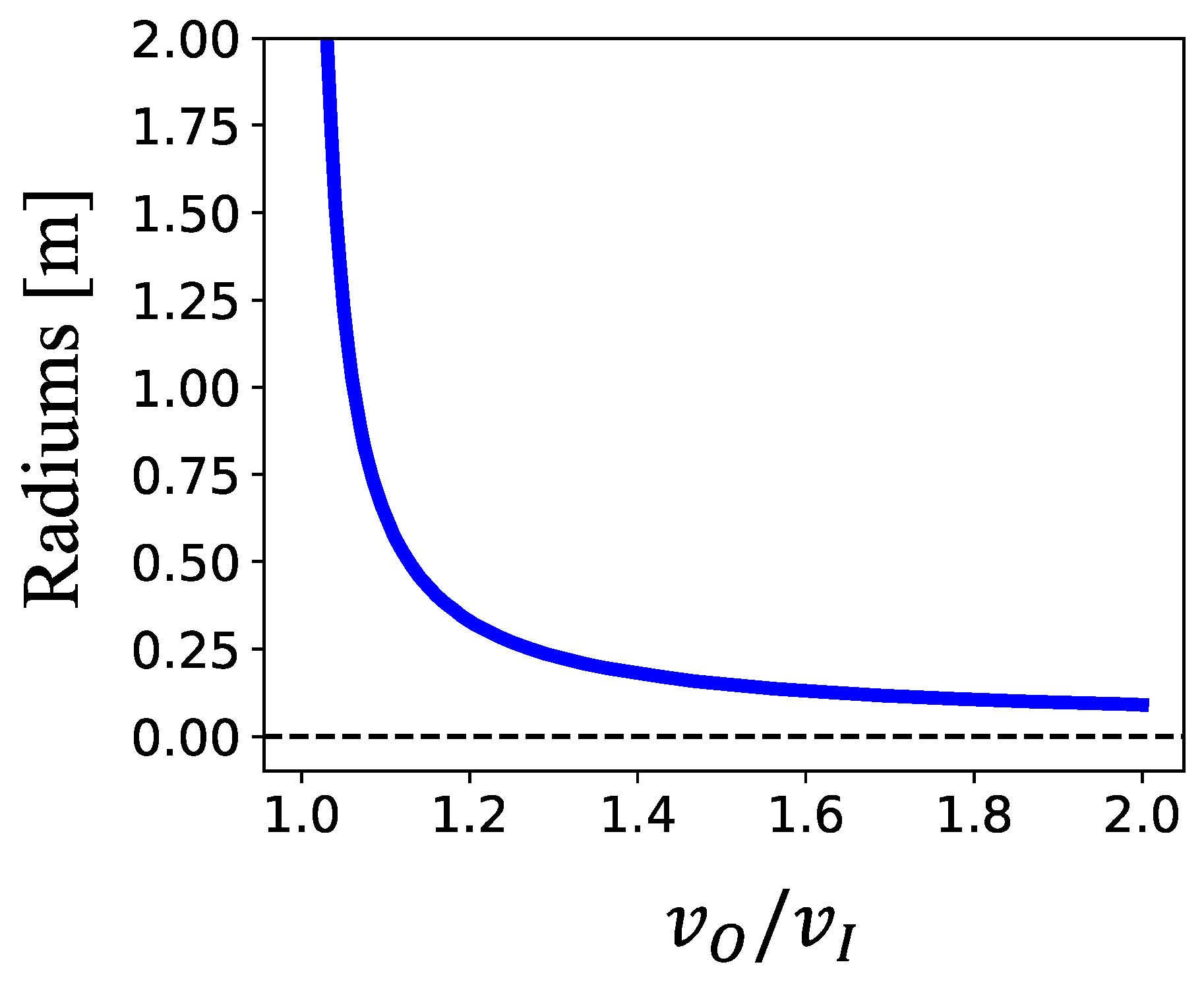
3.2. Modeling Curved Motion
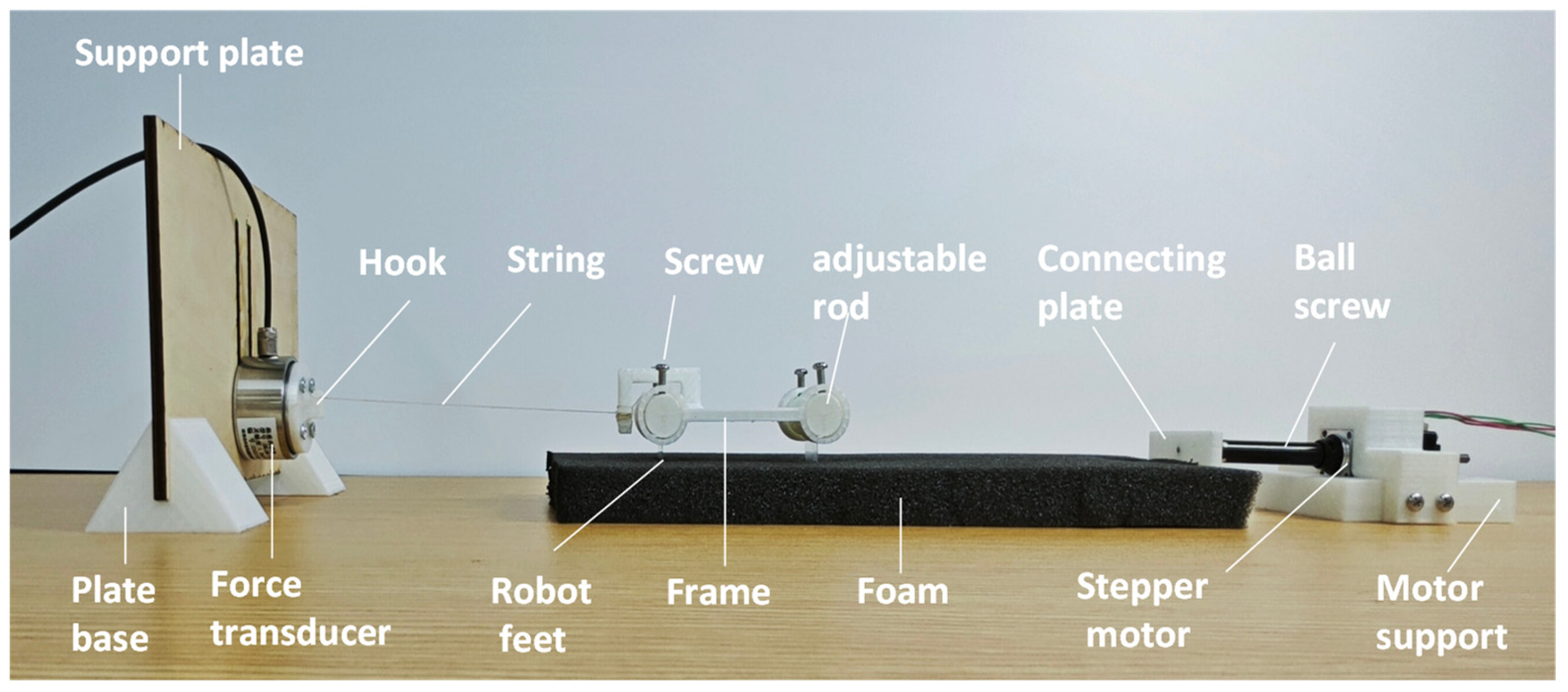
4. Experimental Verifications
4.1. Testing Straight Motion
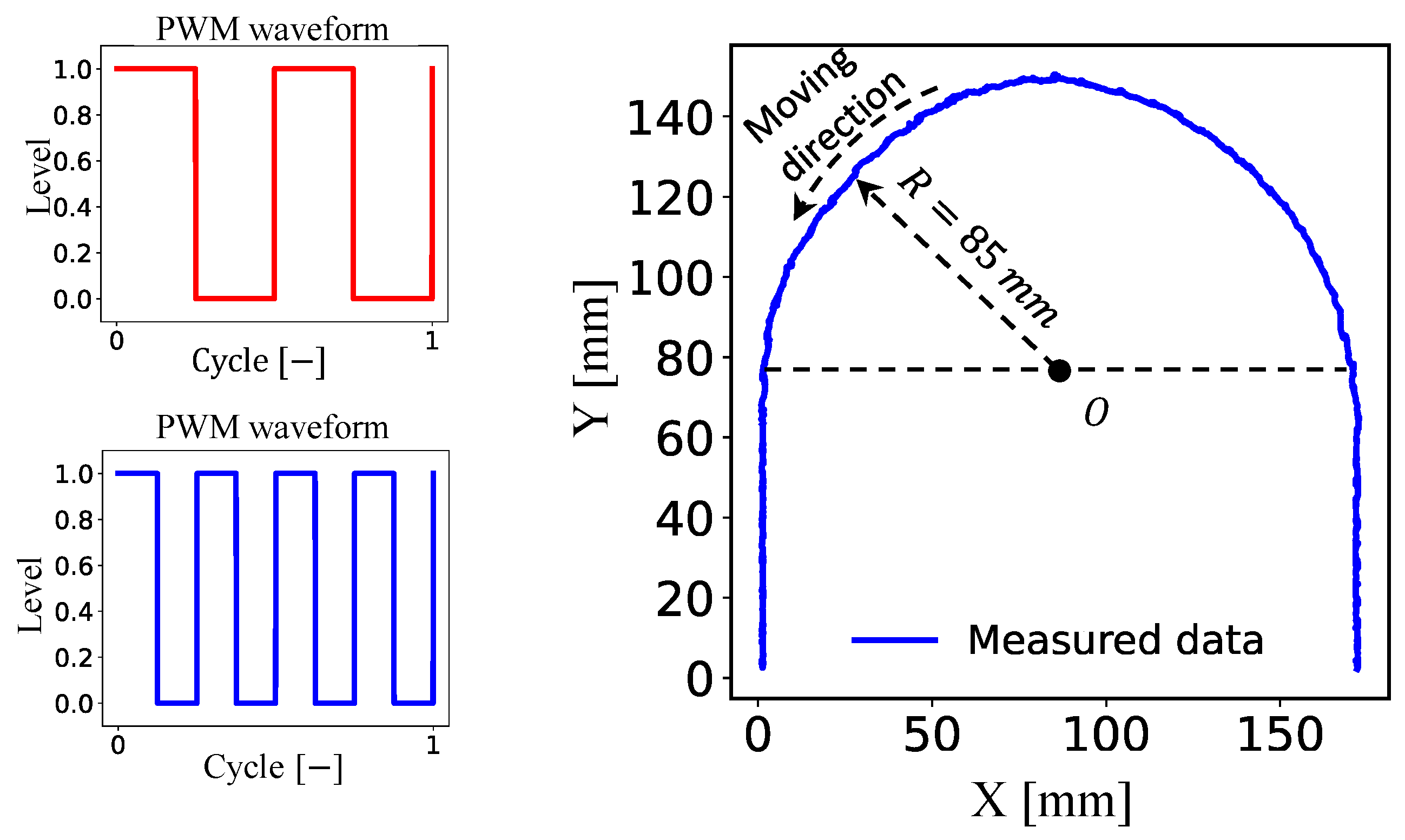
4.2. Testing Curved Motion
5. Conclusions
- (1)
- We have designed and prototyped two coin-sized robot versions with the simplest structures (cut-and-fold bodies) and actuators (fluid-driven soft actuators), namely single- and dual-actuator robots that perform straight motion alone or both straight and curved motion.
- (2)
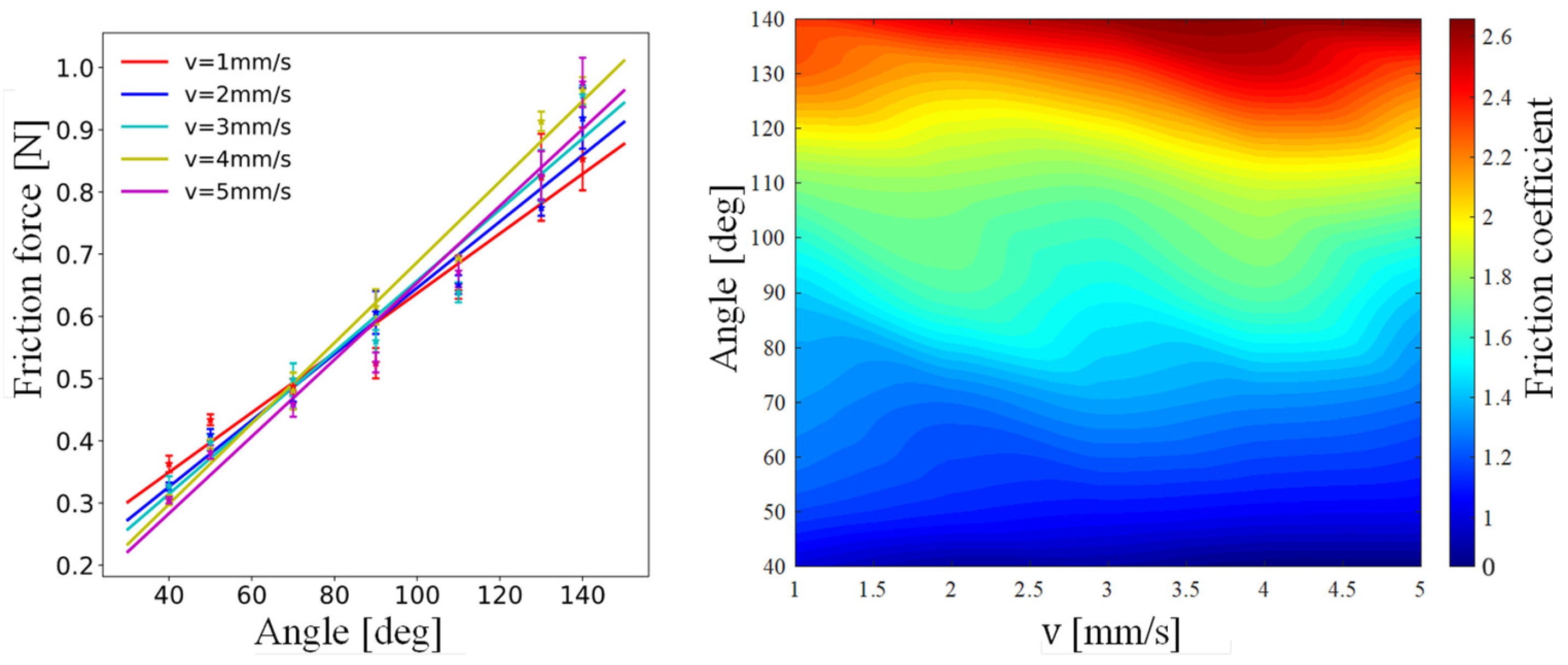
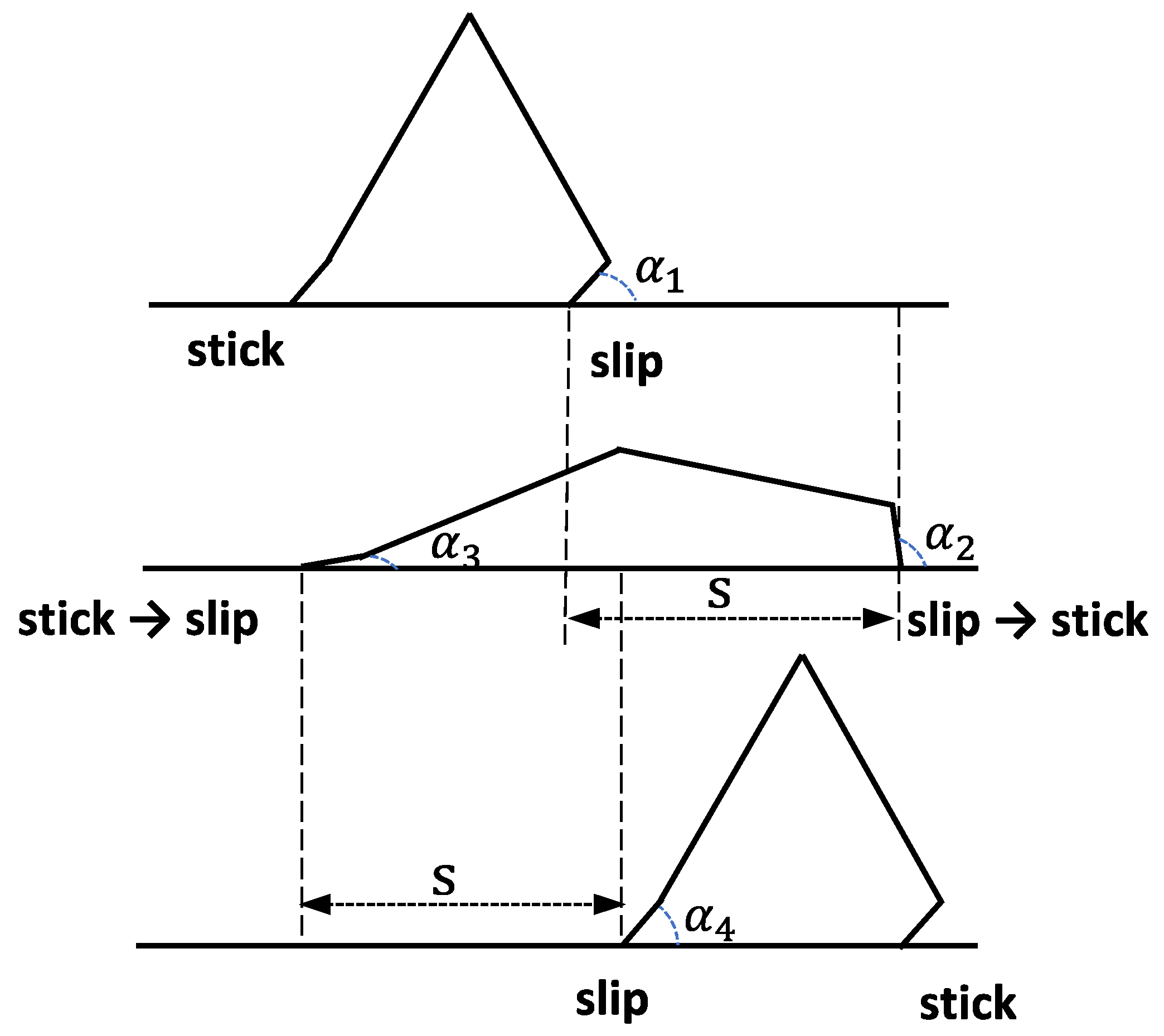
- We have studied the friction characteristics of the robot extremities in touch with the ground that deal with stick–slip contact conversions. We have understood how to design these contact points to achieve proper attack angles and anchoring capability that ensure crawling.
- (3)
- We have developed mathematical models for describing the robot motion in open-loop control. These straightforward techniques illustrate the crawling mechanism by giving predictions comparable to experiments.
- (4)
- We have conducted experiments showing that the robots can move up to 4.5 mm/s with straight motion (i.e., 0.1 body lengths per second) and perform cornering and U-turns while maintaining a constant curvature radius.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

References
- Ren, L.; Li, B.; Wei, G.; Wang, K.; Song, Z.; Wei, Y.; Ren, L.; Liu, Q. Biology and bioinspiration of soft robotics: Actuation, sensing, and system integration. Iscience 2021, 24, 103075. [Google Scholar] [CrossRef] [PubMed]
- Michael, T.T.; Shepherd, R.C.; Galloway, K.J.; Wood, R.; Whitesides, M.G. A resilient, untethered soft robot. Soft Robot. 2014, 1, 213–223. [Google Scholar]
- Duggan, T.; Horowitz, L.; Ulug, A.; Baker, E.; Petersen, K. Inchworm-inspired locomotion in untethered soft robots. In Proceedings of the 2019 2nd IEEE International Conference on Soft Robotics (RoboSoft), Seoul, Republic of Korea, 14–18 April 2019; pp. 200–205. [Google Scholar]
- Shepherd, R.F.; Ilievski, F.; Choi, W.; Morin, S.A.; Stokes, A.A.; Mazzeo, A.D.; Chen, X.; Wang, M.; Whitesides, G.M. Multigait soft robot. Proc. Natl. Acad. Sci. USA 2011, 108, 20400–20403. [Google Scholar] [CrossRef] [PubMed]
- Mao, S.; Dong, E.; Zhang, S.; Xu, M.; Yang, J. A new soft bionic starfish robot with multi-gaits. In Proceedings of the 2013 IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Wollongong, Australia, 9–12 July 2013; pp. 1312–1317. [Google Scholar]
- Yuk, H.; Lin, S.; Ma, C.; Takaffoli, M.; Fang, N.X.; Zhao, X. Hydraulic hydrogel actuators and robots optically and sonically camouflaged in water. Nat. Commun. 2017, 8, 14230. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Wang, T.; Wang, J.; Li, B.; Hong, J.; Zhang, J.X.; Wang, M.Y. Dynamic modeling and simulation of inchworm movement towards bio-inspired soft robot design. Bioinspiration Biomim. 2019, 14, 066012. [Google Scholar] [CrossRef]
- Mu, W.; Li, M.; Chen, E.; Yang, Y.; Yin, J.; Tao, X.; Liu, G.; Yin, R. Spiral-Shape Fast-Moving Soft Robots. Adv. Funct. Mater. 2023, 2023, 2300516. [Google Scholar] [CrossRef]
- Park, T.; Cha, Y. Soft mobile robot inspired by animal-like running motion. Sci. Rep. 2019, 9, 14700. [Google Scholar] [CrossRef]
- Niu, H.; Feng, R.; Xie, Y.; Jiang, B.; Sheng, Y.; Yu, Y.; Baoyin, H.; Zeng, X. Magworm: A biomimetic magnet embedded worm-like soft robot. Soft Robot. 2021, 8, 507–518. [Google Scholar] [CrossRef]
- Hu, W.; Lum, G.Z.; Mastrangeli, M.; Sitti, M. Small-scale soft-bodied robot with multimodal locomotion. Nature 2018, 554, 81–85. [Google Scholar] [CrossRef]
- Chung, H.J.; Parsons, A.M.; Zheng, L. Magnetically controlled soft robotics utilizing elastomers and gels in actuation: A review. Adv. Intell. Syst. 2021, 3, 2000186. [Google Scholar] [CrossRef]
- Bartlett, N.W.; Tolley, M.T.; Overvelde, J.T.; Weaver, J.C.; Mosadegh, B.; Bertoldi, K.; Whitesides, G.M.; Wood, R.J. A 3D-printed, functionally graded soft robot powered by combustion. Science 2015, 349, 161–165. [Google Scholar] [CrossRef] [PubMed]
- Wehner, M.; Truby, R.L.; Fitzgerald, D.J.; Mosadegh, B.; Whitesides, G.M.; Lewis, J.A.; Wood, R.J. An integrated design and fabrication strategy for entirely soft, autonomous robots. Nature 2016, 536, 451–455. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Li, K.; Li, X.; Cui, H.; Liu, G.; Xu, H.; Wu, X.; Yao, W.; Zhong, B.; Huang, X.; et al. Electro-thermally driven flexible robot arms based on stacking-controlled graphite nanocomposites. Carbon 2019, 152, 873–881. [Google Scholar] [CrossRef]
- Wu, S.; Baker, G.L.; Yin, J.; Zhu, Y. Fast thermal actuators for soft robotics. Soft Robot. 2022, 9, 1031–1039. [Google Scholar] [CrossRef] [PubMed]
- Calisti, M.; Picardi, G.; Laschi, C. Fundamentals of soft robot locomotion. J. R. Soc. Interface 2017, 14, 20170101. [Google Scholar] [CrossRef]
- Lvan Griethuijsen, I.; Trimmer, B.A. Caterpillar crawling over irregular terrain: Anticipation and local sensing. J. Comp. Physiol. A 2010, 196, 397–406. [Google Scholar] [CrossRef]
- Paoletti, P.; Mahadevan, L. A proprioceptive neuromechanical theory of crawling. Proc. R. Soc. B Biol. Sci. 2014, 281, 20141092. [Google Scholar] [CrossRef]
- Crooks, W.; Rozen-Levy, S.; Trimmer, B.; Rogers, C.; Messner, W. Passive gripper inspired by Manduca sexta and the Fin Ray® Effect. Int. J. Adv. Robot. Syst. 2017, 14, 1729881417721155. [Google Scholar] [CrossRef]
- Koh, J.-S.; Cho, K.-J. Omegabot: Biomimetic inchworm robot using SMA coil actuator and smart composite microstructures (SCM). In Proceedings of the 2009 IEEE International Conference on Robotics and Biomimetics (ROBIO), Guilin, China, 18–22 December 2009; pp. 1154–1159. [Google Scholar]
- Umedachi, T.; Vikas, V.; Trimmer, B.A. Highly deformable 3-D printed soft robot generating inching and crawling locomotions with variable friction legs. In Proceedings of the 2013 IEEE/RSJ International Conference on Intelligent Robots and Systems, Tokyo, Japan, 3–7 November 2013; pp. 4590–4595. [Google Scholar]
- Felton, S.M.; Tolley, M.T.; Onal, C.D.; Rus, D.; Wood, R.J. Robot self-assembly by folding: A printed inchworm robot. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; pp. 277–282. [Google Scholar]
- Noorani, M.R.S.; Ghanbari, A.; Aghli, S. Design and fabrication of a worm robot prototype. In Proceedings of the 2015 3rd RSI International Conference on Robotics and Mechatronics (ICROM), Tehran, Iran, 7–9 October 2015; pp. 073–078. [Google Scholar]
- Guo, H.; Zhang, J.; Wang, T.; Li, Y.; Hong, J.; Li, Y. Design and control of an inchworm-inspired soft robot with omega-arching locomotion. In Proceedings of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Singapore, 29 May–3 June 2017; pp. 4154–4159. [Google Scholar]
- Ning, J.; Ti, C.; Liu, Y. Inchworm inspired pneumatic soft robot based on friction hysteresis. J. Robot. Autom. 2017, 1, 54–63. [Google Scholar]
- Wang, J.; Min, J.; Fei, Y.; Pang, W. Study on nonlinear crawling locomotion of modular differential drive soft robot. Nonlinear Dyn. 2019, 97, 1107–1123. [Google Scholar] [CrossRef]
- Gamus, B.; Salem, L.; Gat, A.D.; Or, Y. Understanding inchworm crawling for soft-robotics. IEEE Robot. Autom. Lett. 2020, 5, 1397–1404. [Google Scholar] [CrossRef]
- Gamus, B.; Gat, A.D.; Or, Y. Dynamic inchworm crawling: Performance analysis and optimization of a three-link robot. IEEE Robot. Autom. Lett. 2020, 6, 111–118. [Google Scholar] [CrossRef]
- Wu, Q.; Jimenez, T.G.D.; Qu, J.; Zhao, C.; Liu, X. Regulating surface traction of a soft robot through electrostatic adhesion control. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; pp. 488–493. [Google Scholar]
- Vikas, V.; Cohen, E.; Grassi, R.; Sözer, C.; Trimmer, B. Design and locomotion control of a soft robot using friction manipulation and motor–tendon actuation. IEEE Trans. Robot. 2016, 32, 949–959. [Google Scholar] [CrossRef]
- Rus, D.; Sung, C. Spotlight on origami robots. Sci. Robot. 2018, 3, eaat0938. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, Q.; Zhou, J.; Song, A. Crab-inspired compliant leg design method for adaptive locomotion of a multi-legged robot. Bioinspiration Biomim. 2022, 17, 025001. [Google Scholar] [CrossRef]
- Du, L.; Ma, S.; Tokuda, K.; Tian, Y.; Li, L. Bidirectional locomotion of soft inchworm crawler using dynamic gaits. Front. Robot. AI 2022, 9, 899850. [Google Scholar] [CrossRef]
- Pedchenko, A.; Barth, E.J. Design and validation of a high energy density elastic accumulator using polyurethane. In Proceedings of the 2009 Dynamic Systems and Control Conference, Hollywood, CA, USA, 12–14 October 2009; Volume 48920, pp. 283–290. [Google Scholar]


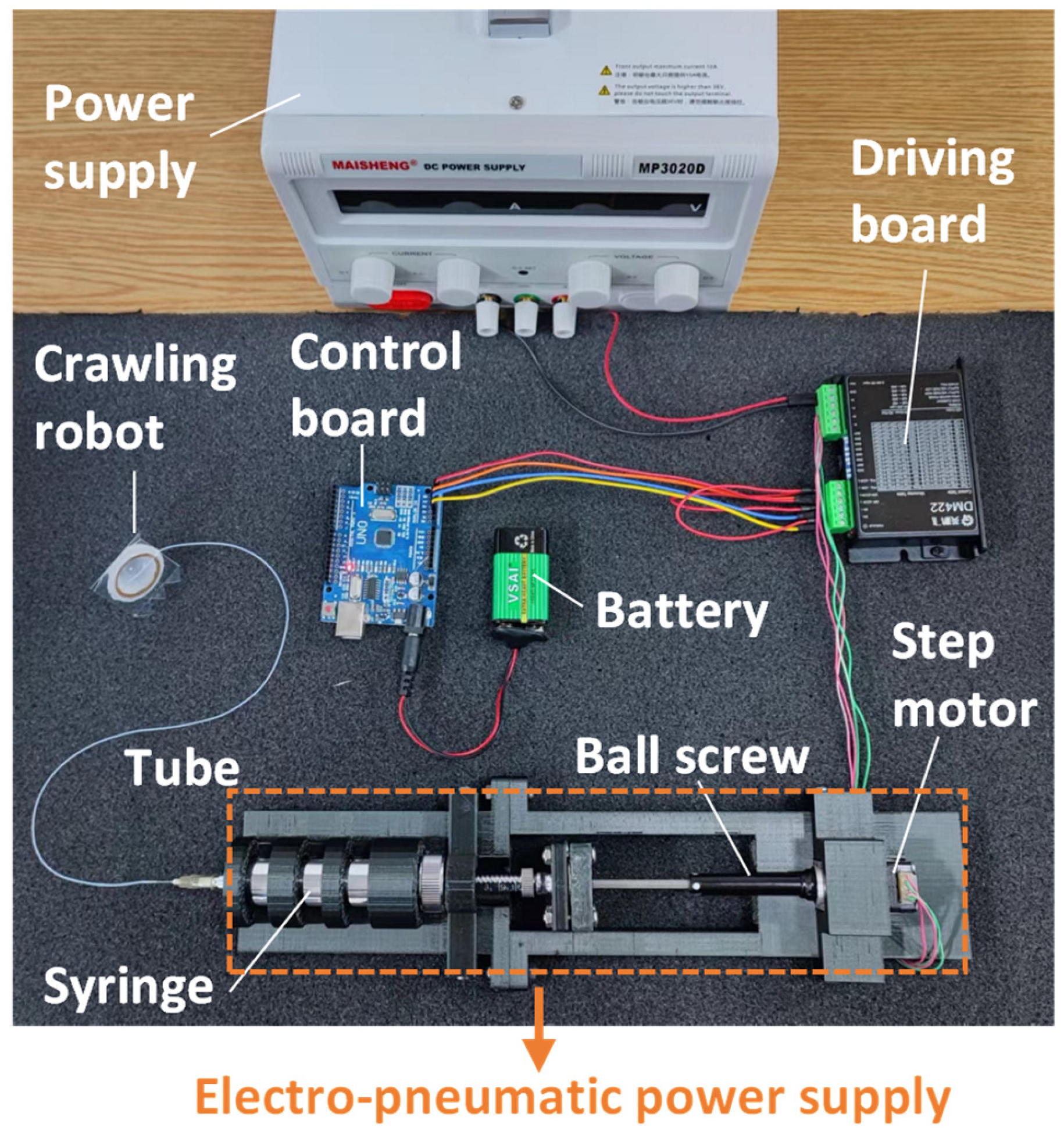
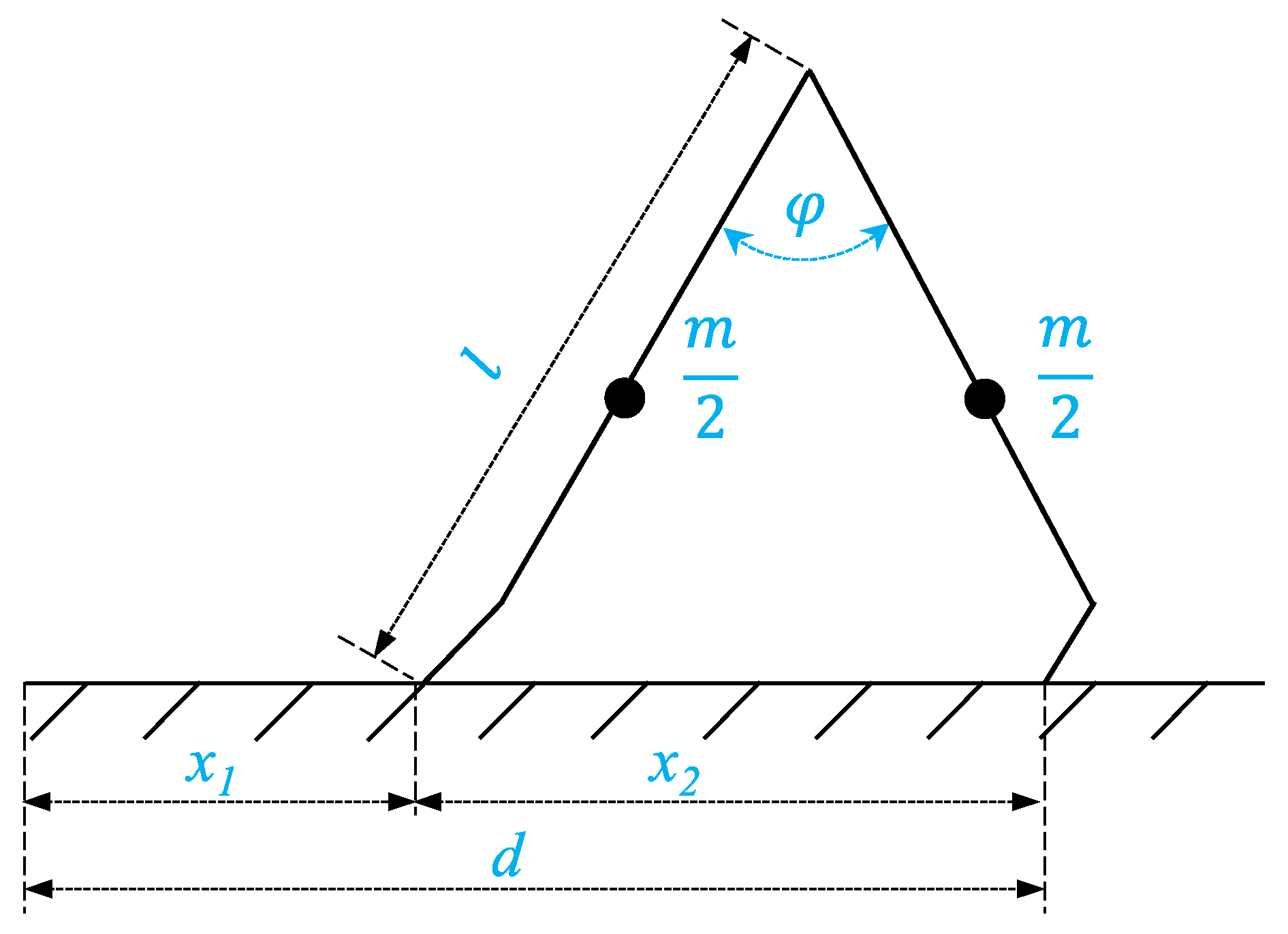

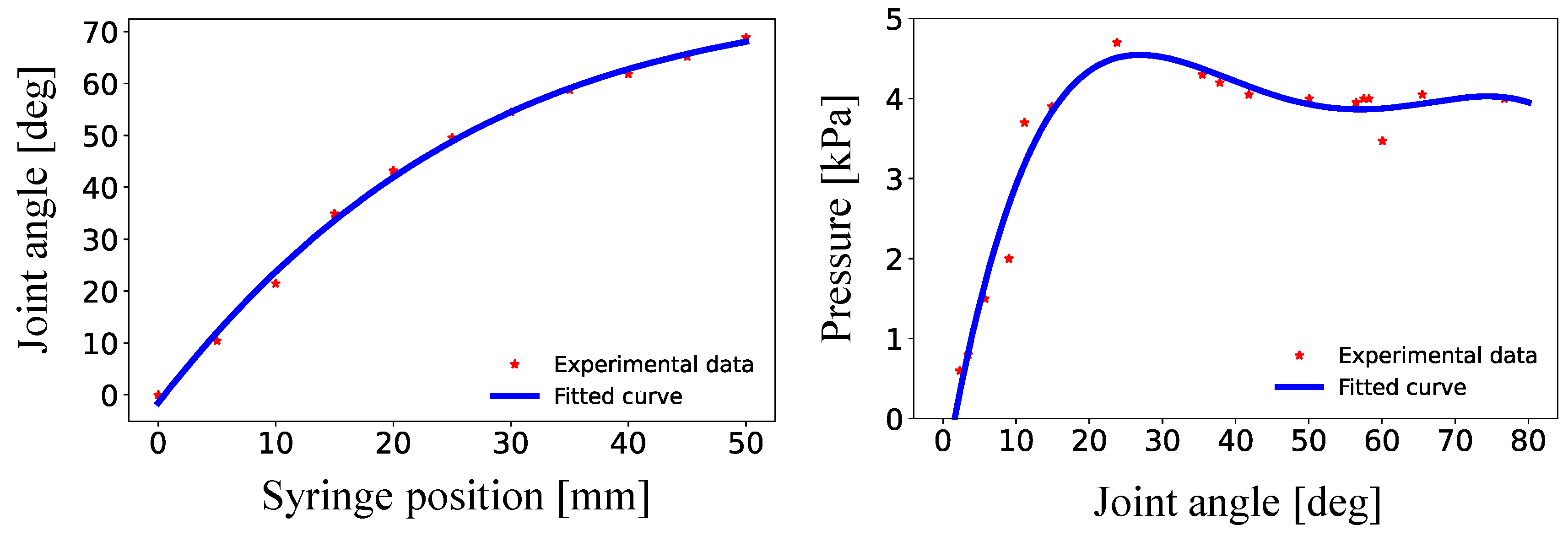
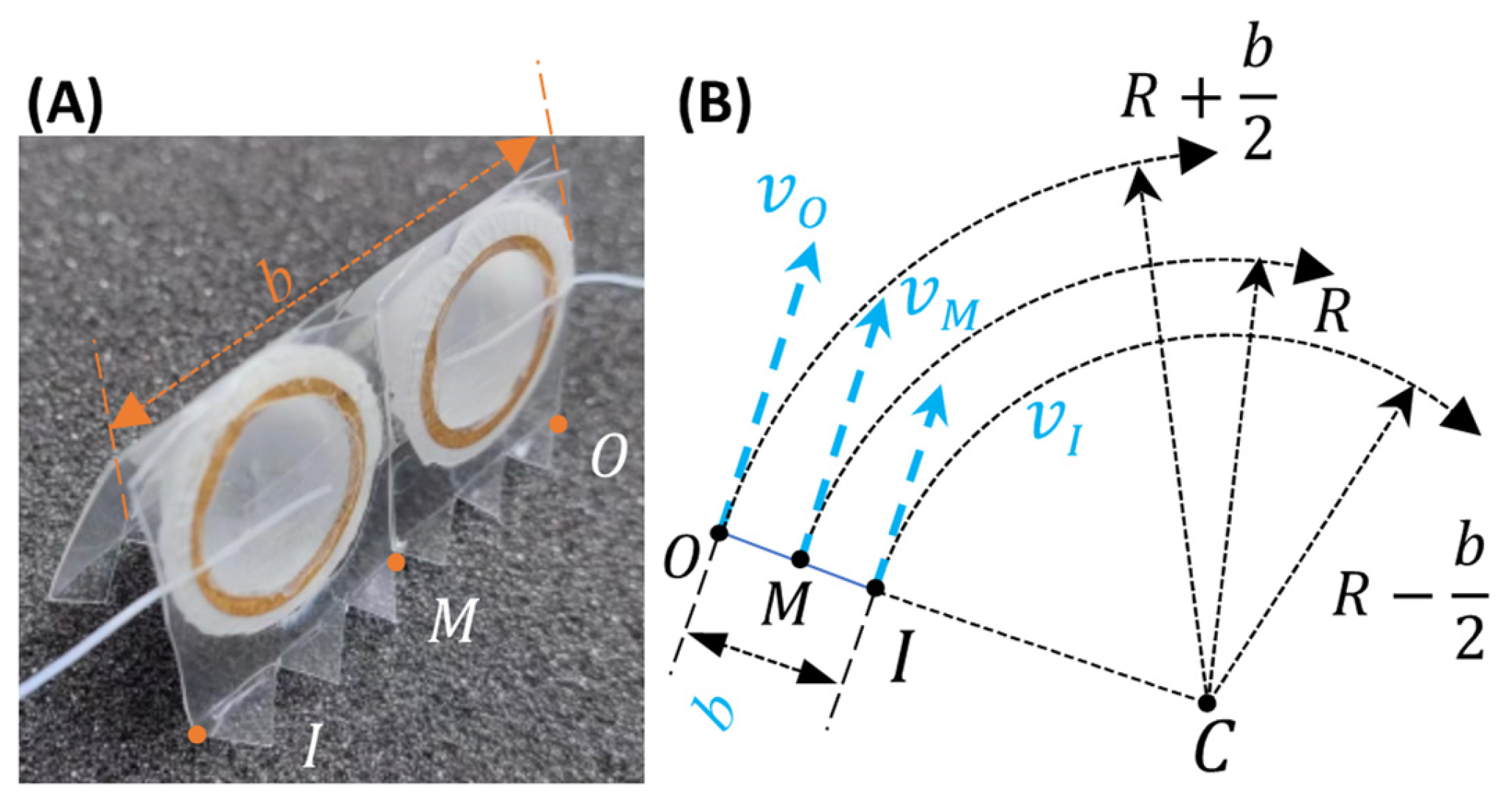

| Body | Actuator | Glue | Tube | Ring | |
|---|---|---|---|---|---|
| Material | PVC | Latex | A401 | Teflon l | Paper |
| Size | 16 cm2 | 16 cm2 | - | Ø 0.6 mm | Ø 24 mm |
| Mass | 0.8 g | 0.1 g | 0.2 g | 0.2 g | 0.016 g |
| Parameter | Notation | Value | Unit |
|---|---|---|---|
| Total mass | m | 0.002 | kg |
| Gravitational acceleration | g | 9.81 | m/s2 |
| COM height change | h | 0.0046 | m |
| Stride length | s | 0.020 | m |
| Friction force due to | 0.032 | N | |
| Friction force due to | 0.038 | N | |
| Friction force due to | 0.016 | N | |
| Friction force due to | 0.021 | N |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Liu, M.; Padovani, D. Minimal Bio-Inspired Crawling Robots with Motion Control Capabilities. Actuators 2024, 13, 4. https://doi.org/10.3390/act13010004
Wu J, Liu M, Padovani D. Minimal Bio-Inspired Crawling Robots with Motion Control Capabilities. Actuators. 2024; 13(1):4. https://doi.org/10.3390/act13010004
Chicago/Turabian StyleWu, Jintian, Mingyi Liu, and Damiano Padovani. 2024. "Minimal Bio-Inspired Crawling Robots with Motion Control Capabilities" Actuators 13, no. 1: 4. https://doi.org/10.3390/act13010004
APA StyleWu, J., Liu, M., & Padovani, D. (2024). Minimal Bio-Inspired Crawling Robots with Motion Control Capabilities. Actuators, 13(1), 4. https://doi.org/10.3390/act13010004








