A Review of Recent Developments in Permanent Magnet Eddy Current Couplers Technology
Abstract
1. Introduction
2. Working Principle, Structure, and Classification of PMECCs
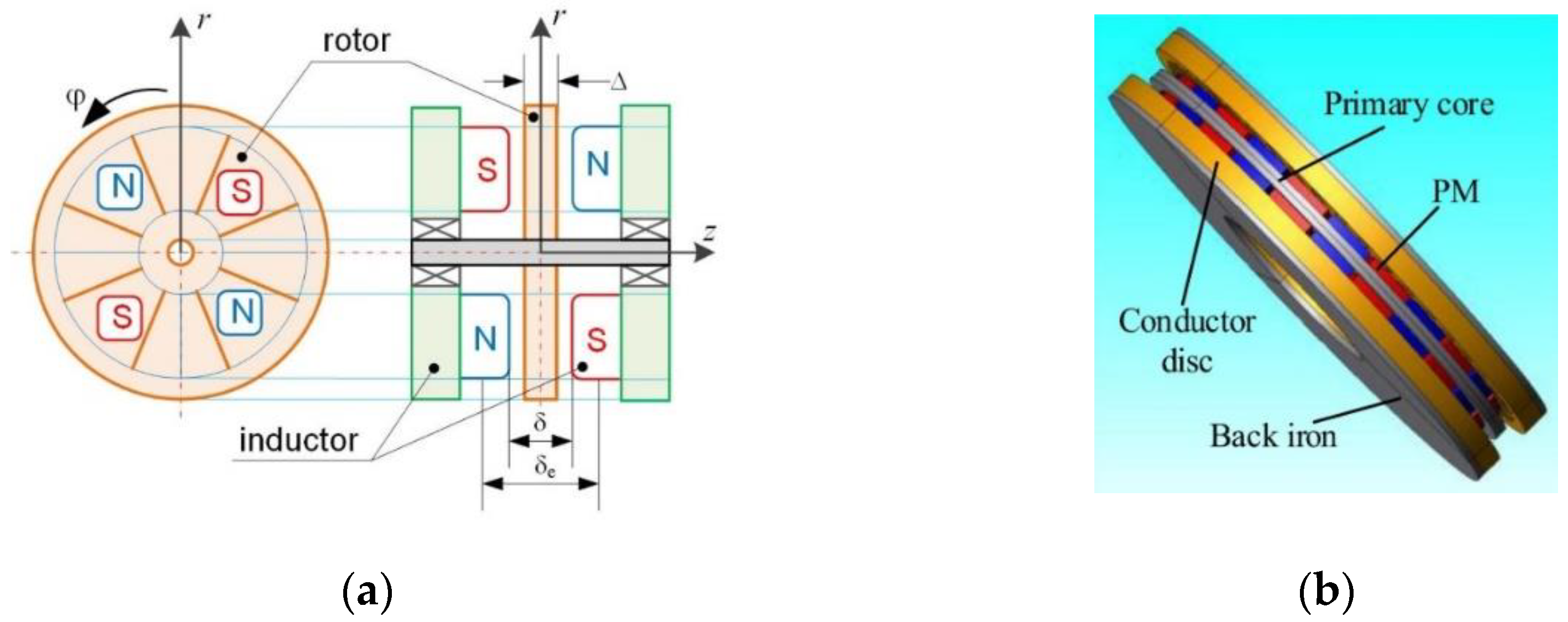
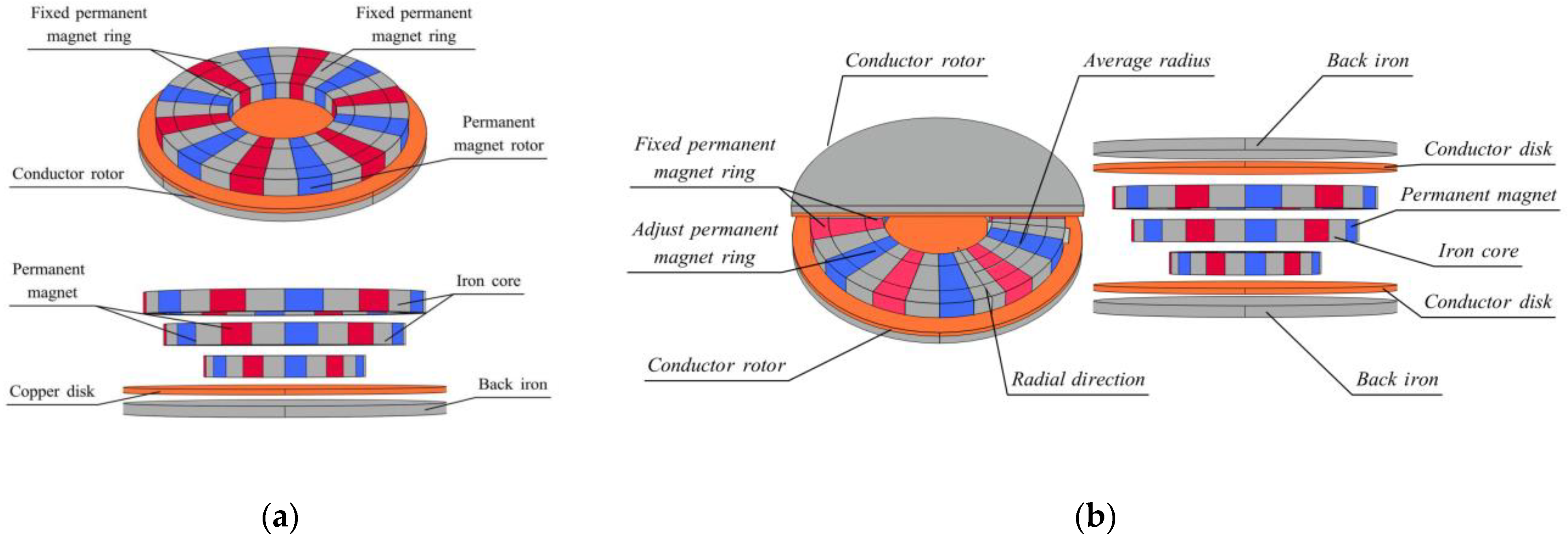
2.1. Axial-Flux PMECCs
2.1.1. Surface-Mounted
2.1.2. Surface-Inset
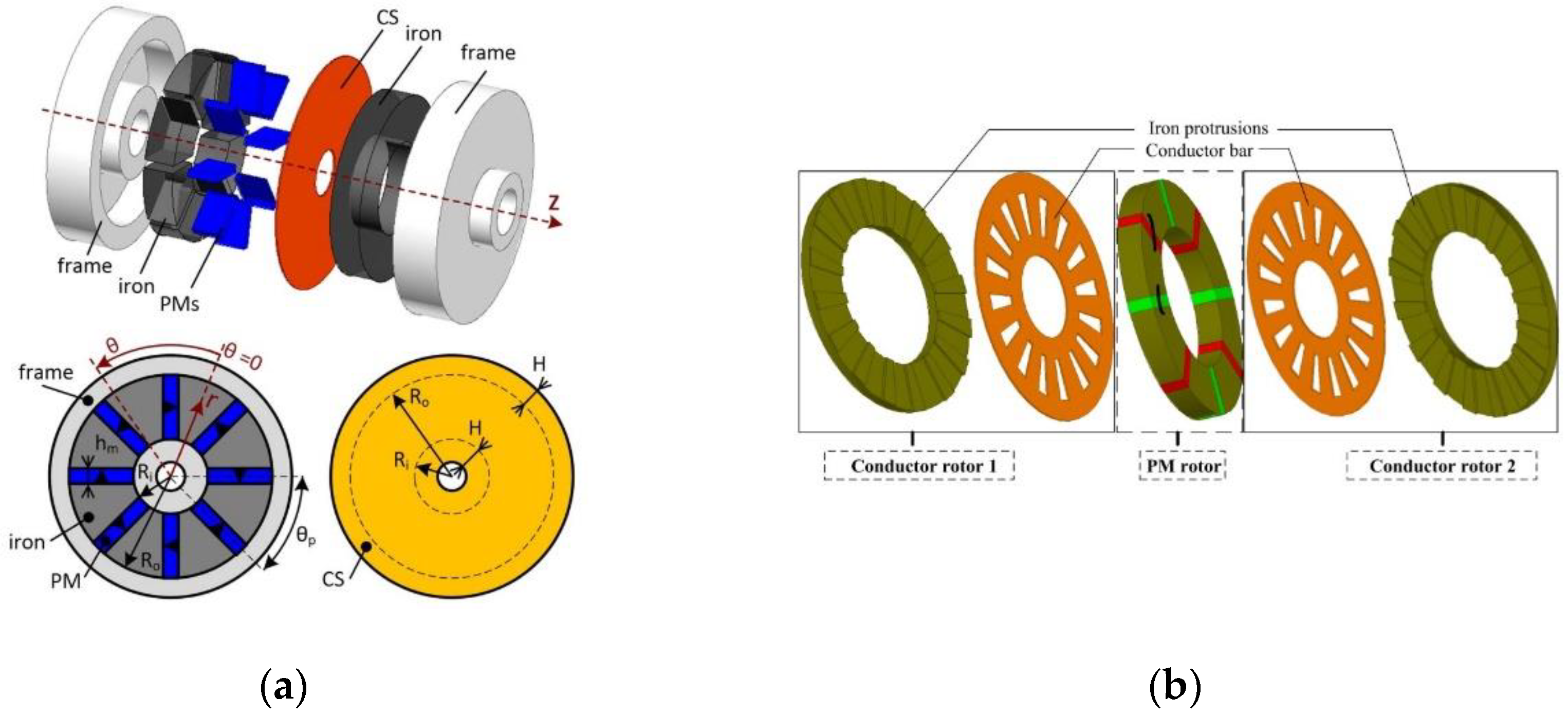
2.2. Radial-Flux PMECCs
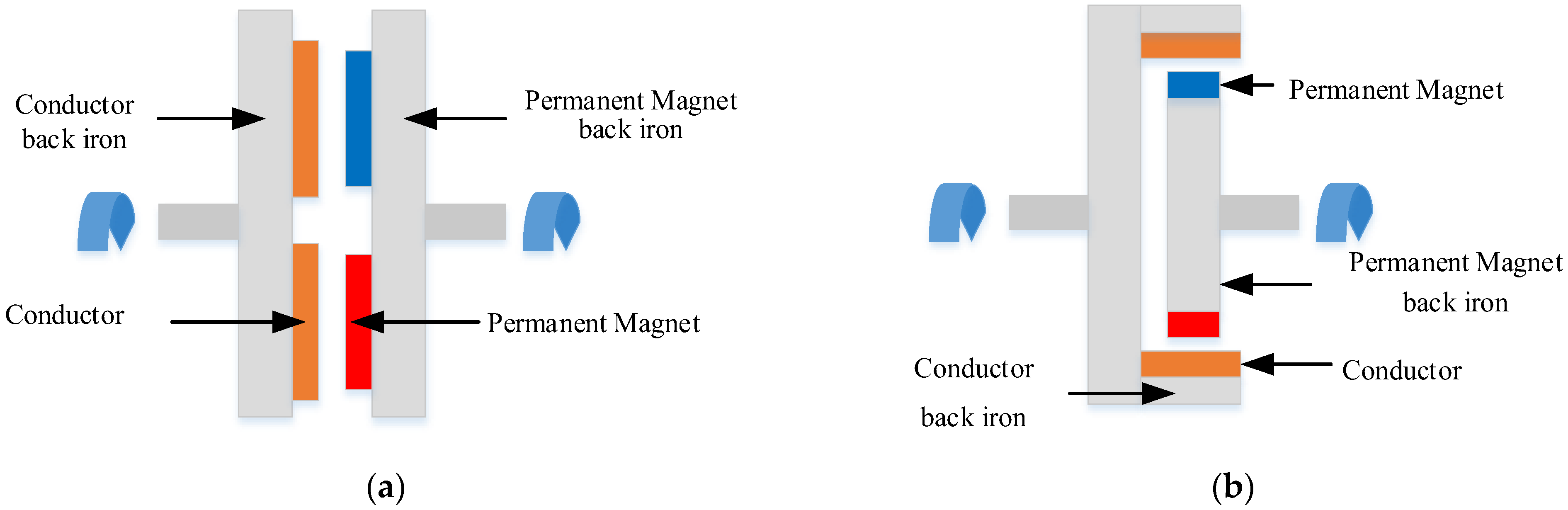
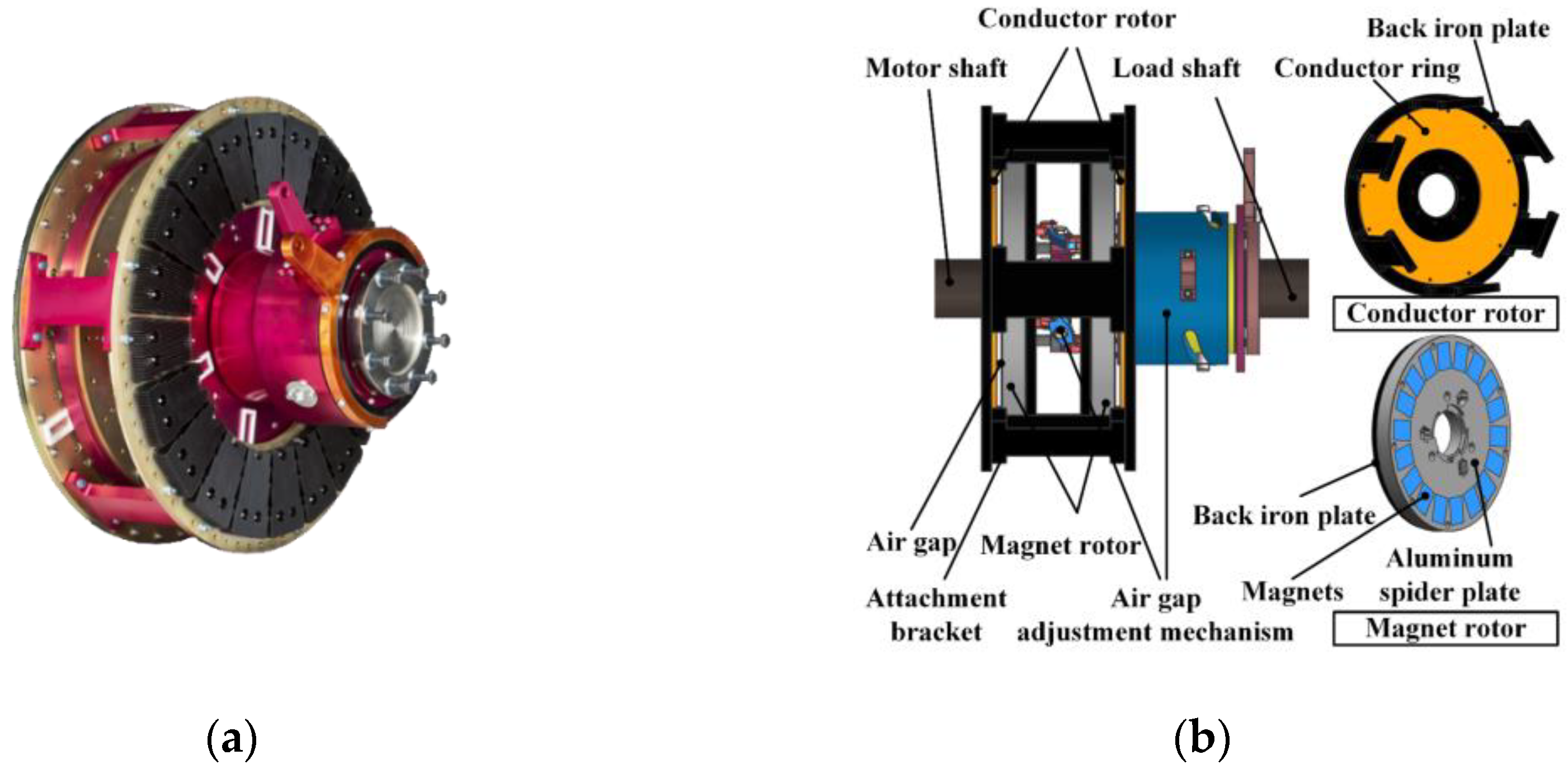
2.2.1. Surface-Mounted
2.2.2. Surface-Inset
2.3. Novel Design Topologies of PMECCs
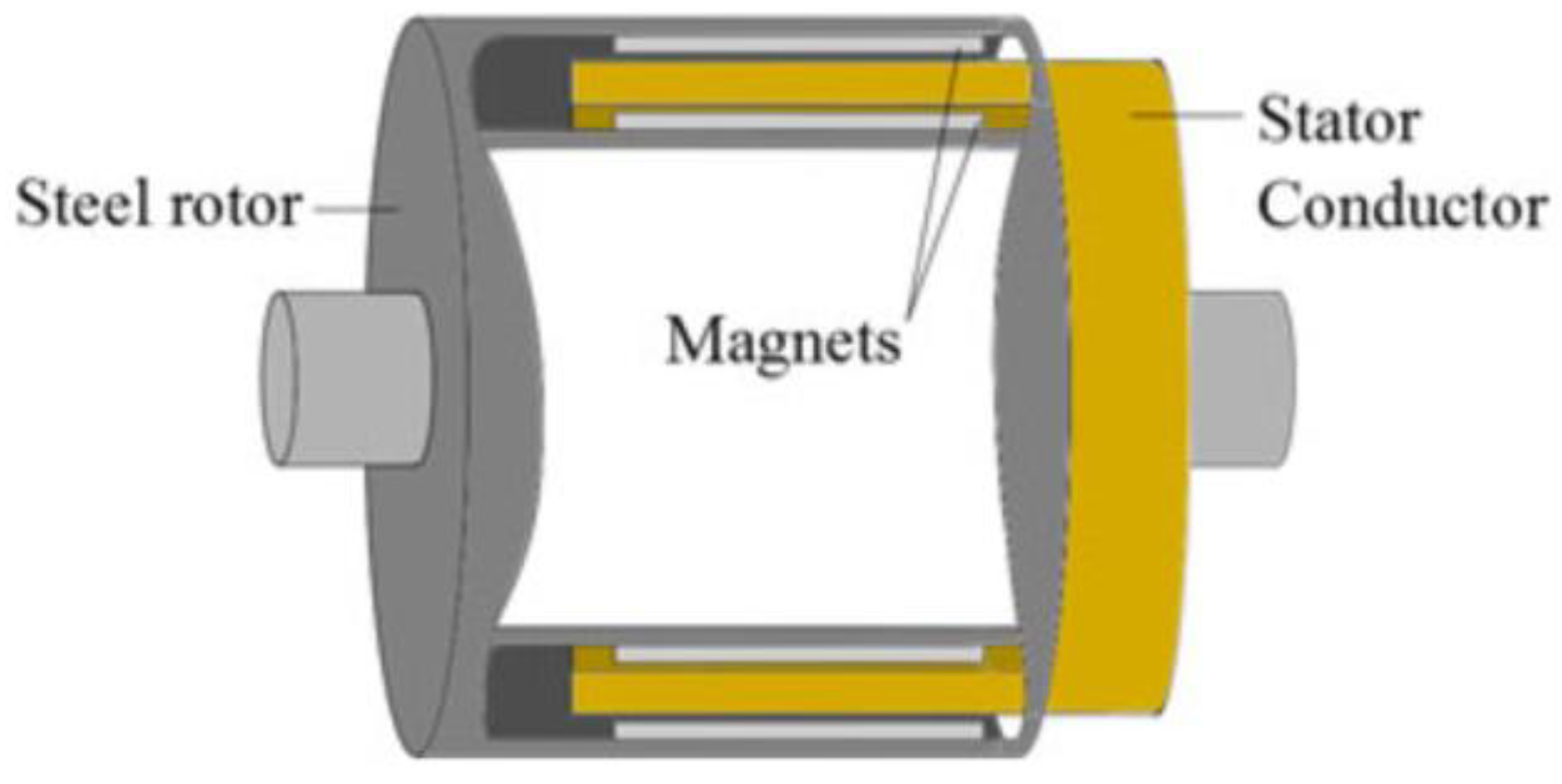
2.3.1. Axial–Radial Combined PMECCs
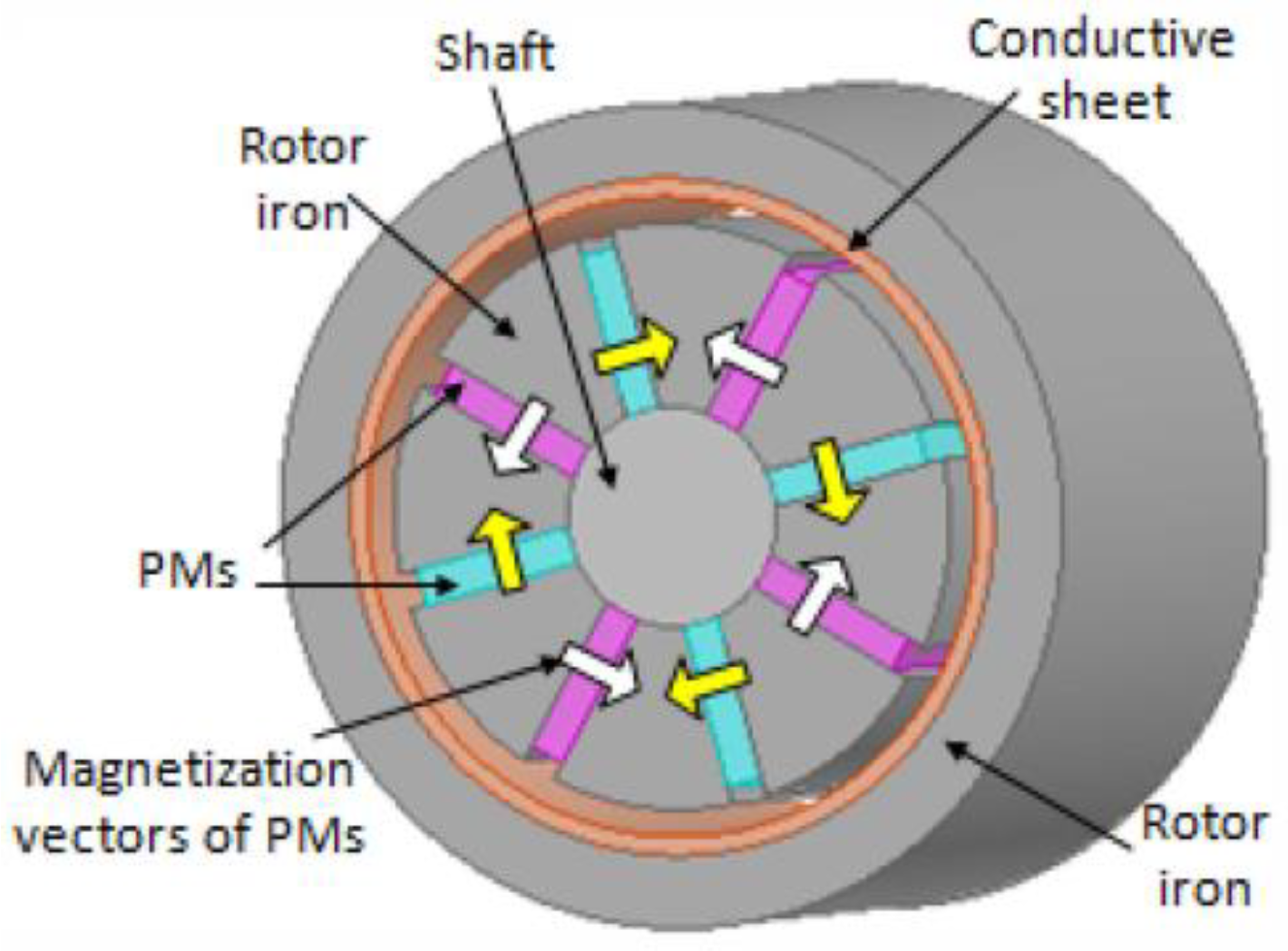
2.3.2. Novel Magnetization Method
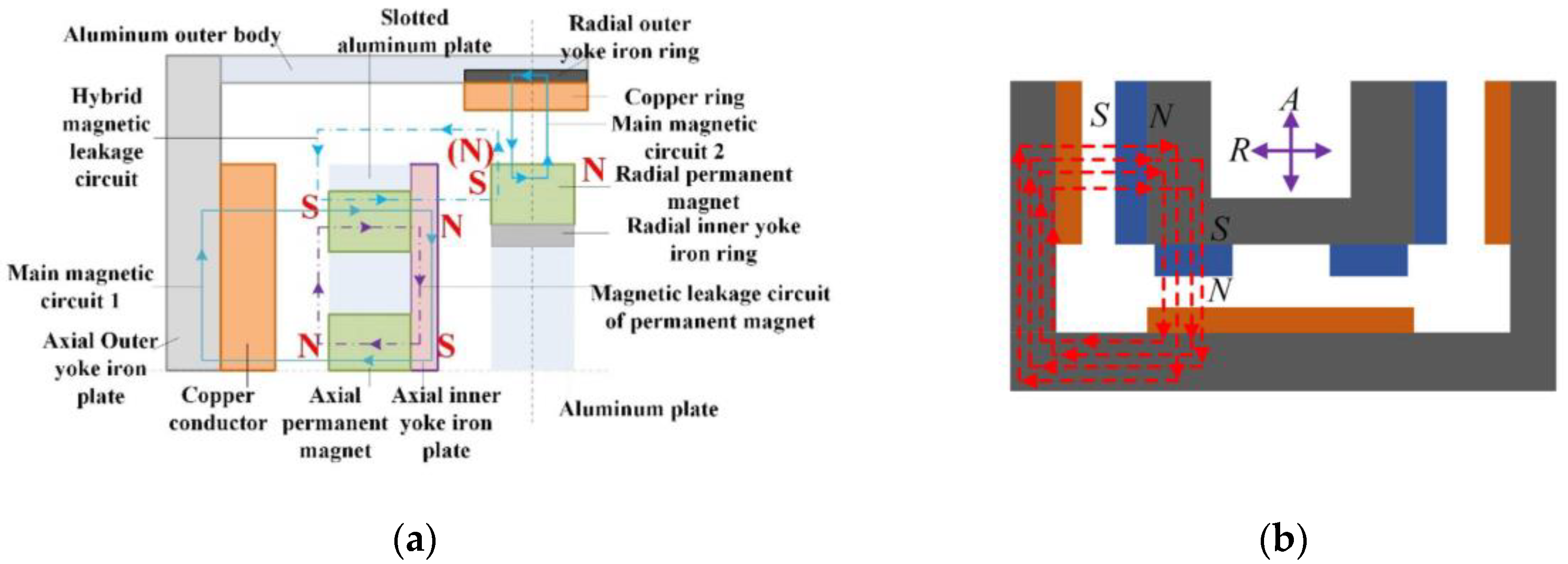
2.3.3. Slotted Conductor Rotor
2.3.4. Novel Flux Adjustable PMECCs
3. Modeling Methods for PMECC
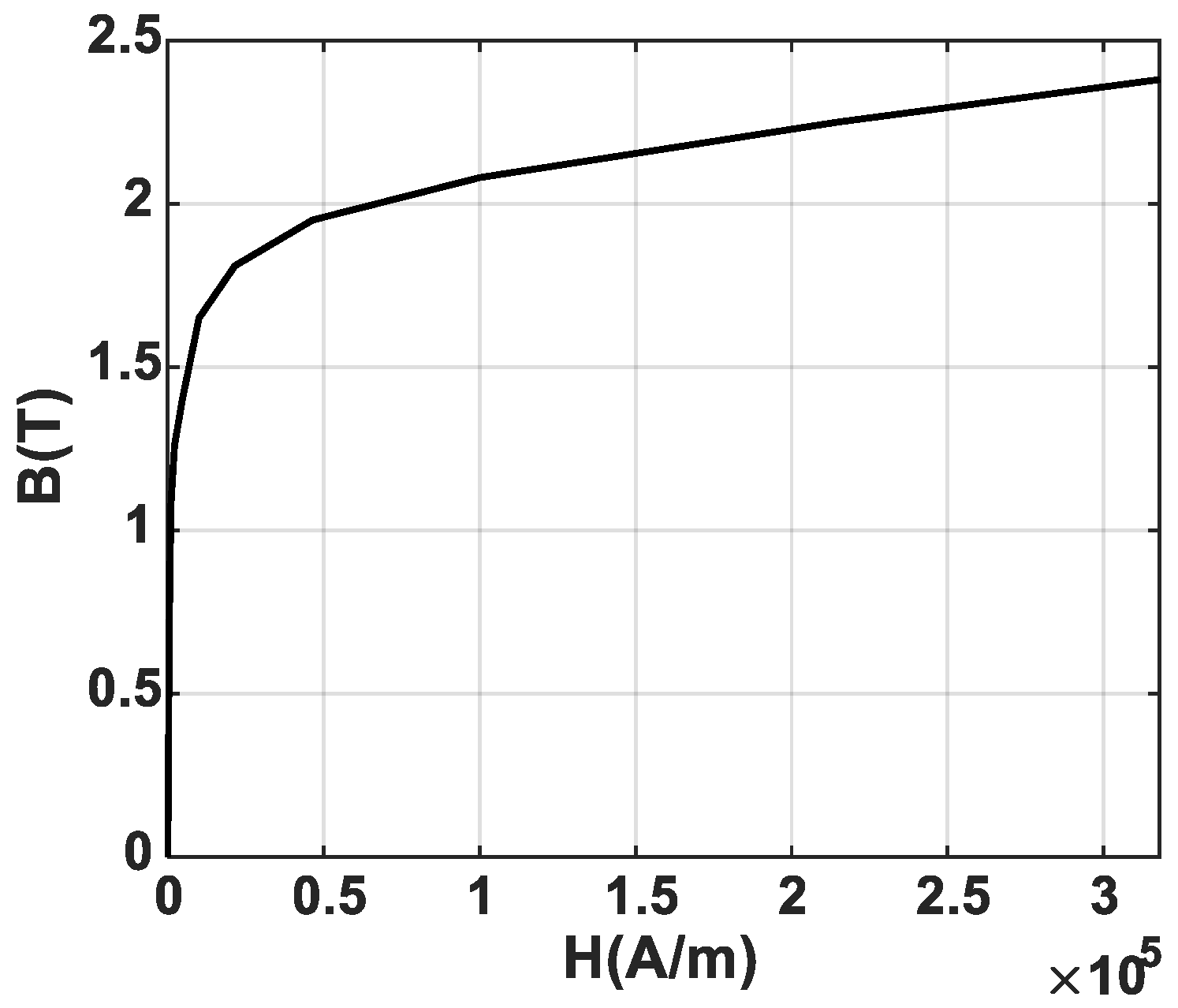
3.1. Numerical Methods
3.2. Analytical Methods
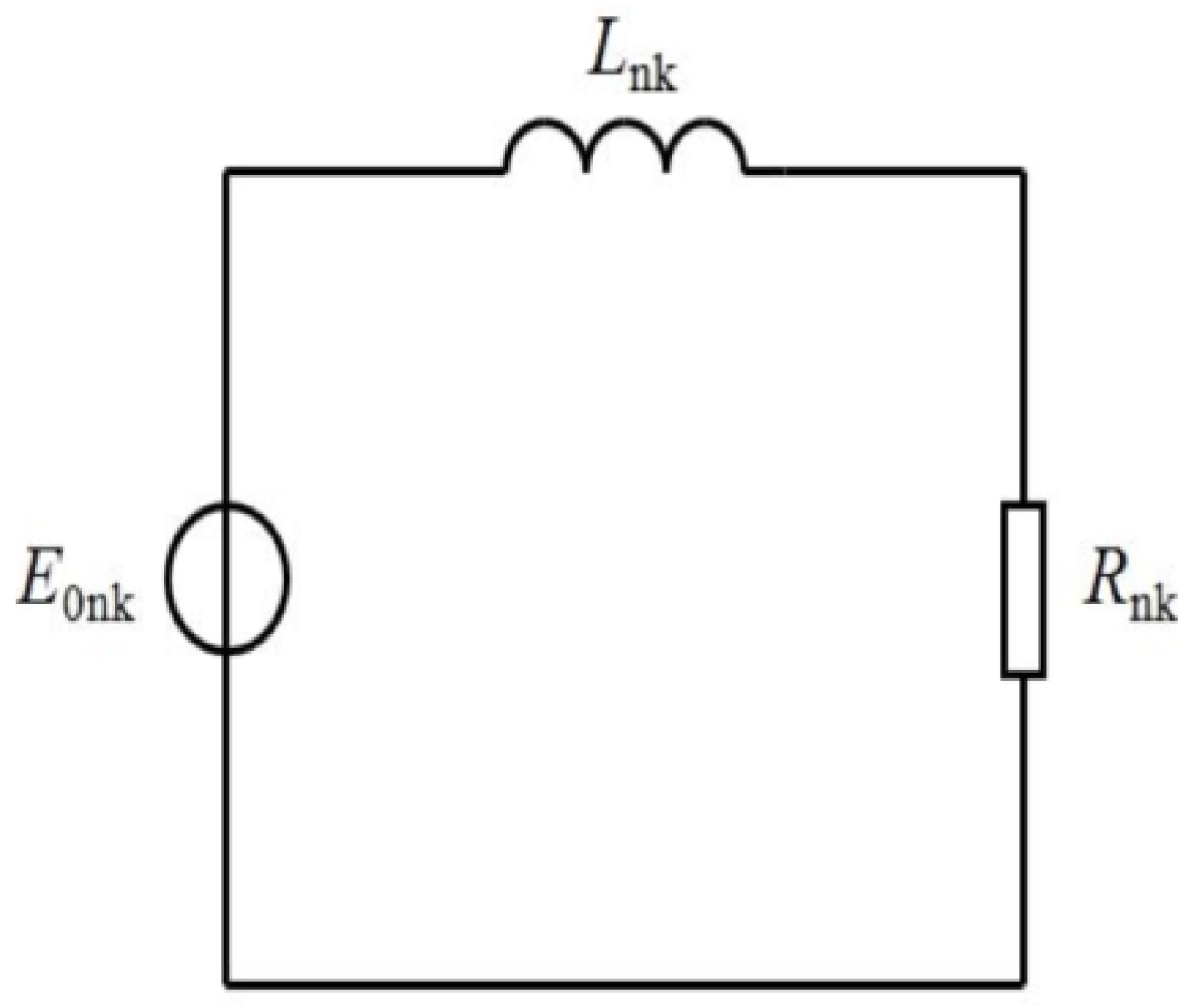
3.2.1. Magnetic Equivalent Circuit Method
3.2.2. Analytic Modeling Method Based on Maxwell’s Equations
3.3. Semi-Analytical Methods
3.4. Nonlinear Regression Modeling
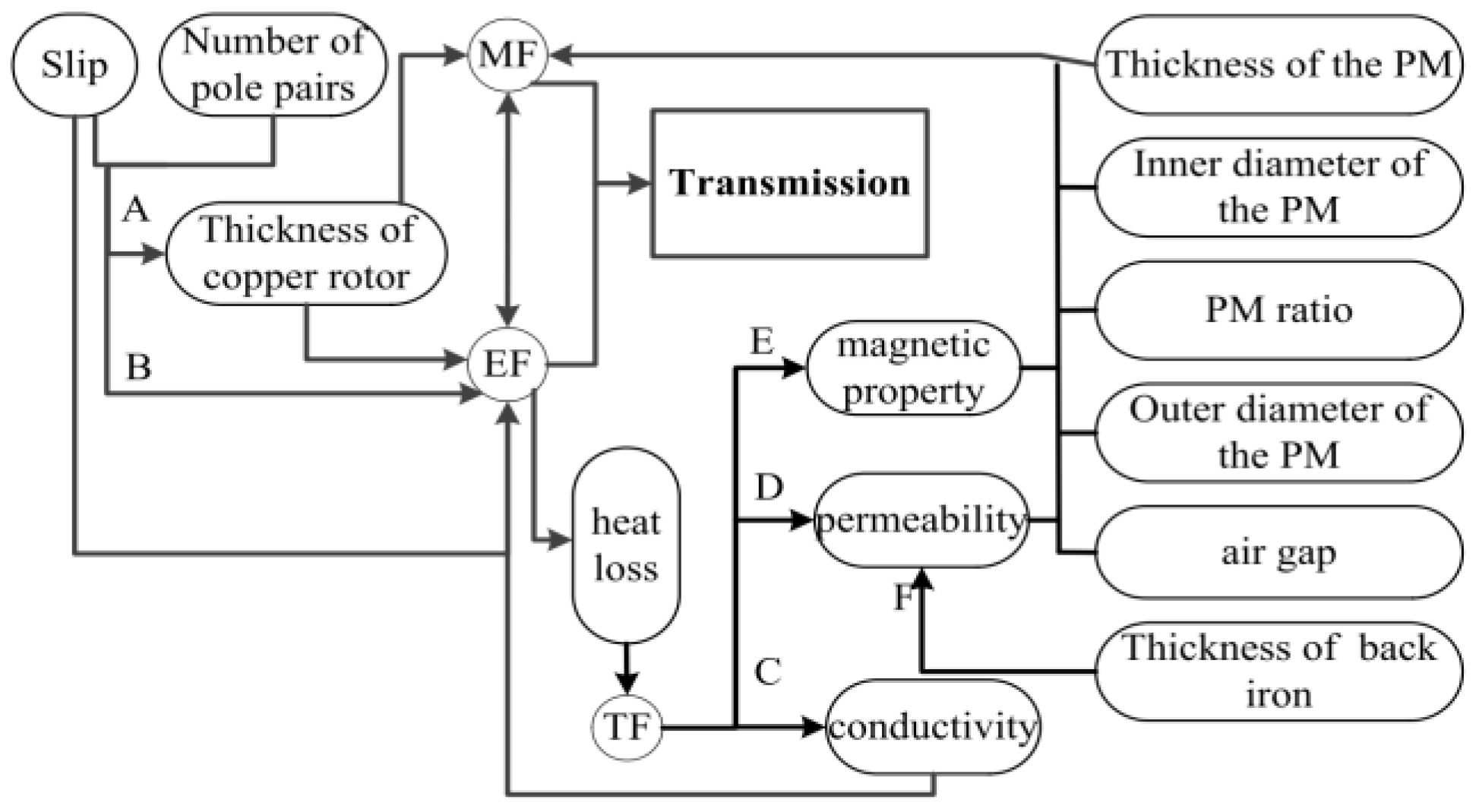
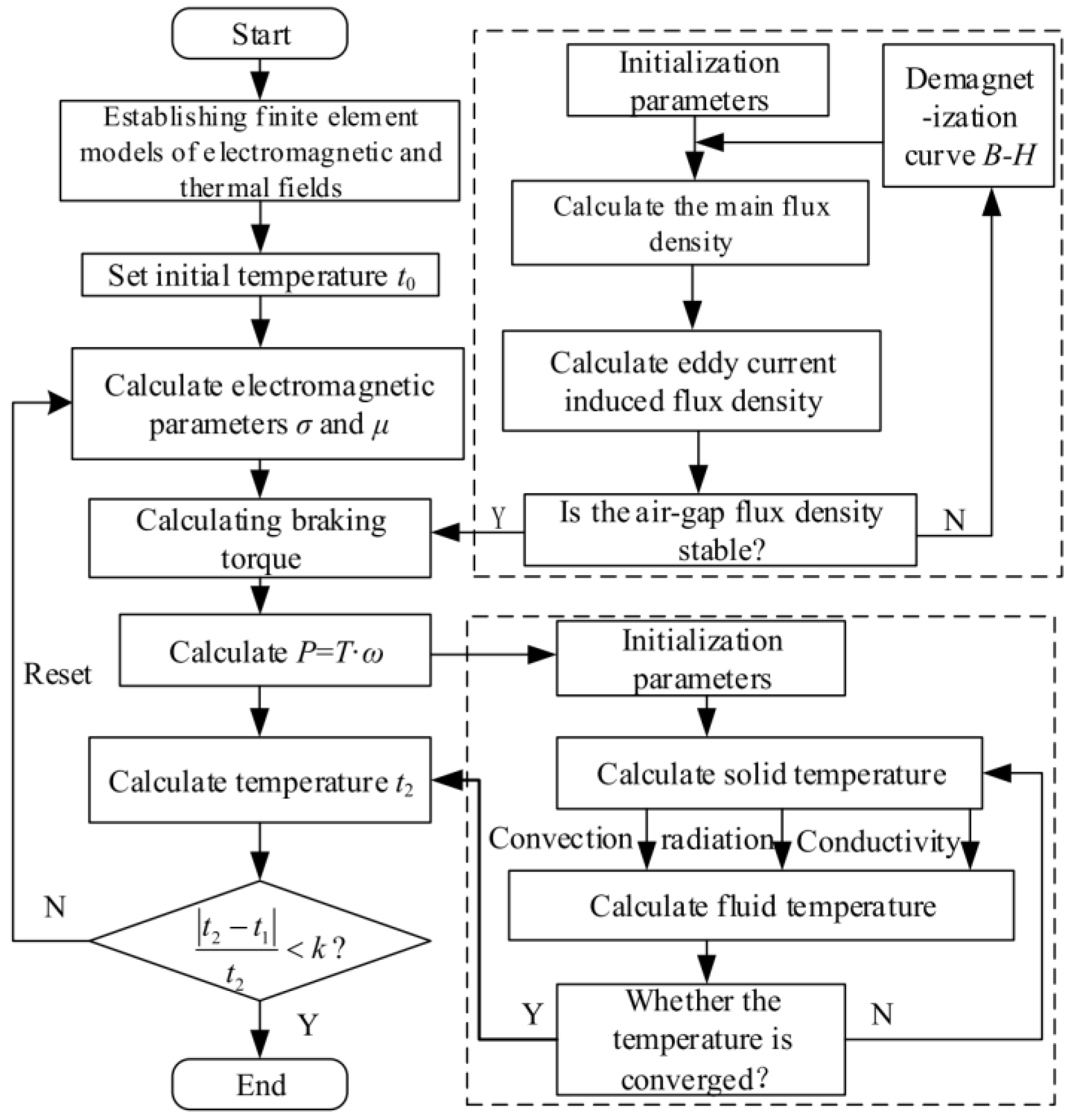
4. Loss Calculation and Thermal Analysis
4.1. Thermal Analysis Modeling Method
4.1.1. Analytical Thermal Model
4.1.2. Equivalent Thermal Network Model
4.1.3. Numerical Techniques
4.2. Multi-Field Coupling Analysis
5. Optimization Design
5.1. Optimization Classification
5.1.1. Single- and Multi-Objective Optimization
5.1.2. Component- and System-Level Optimization
5.2. Optimization Techniques
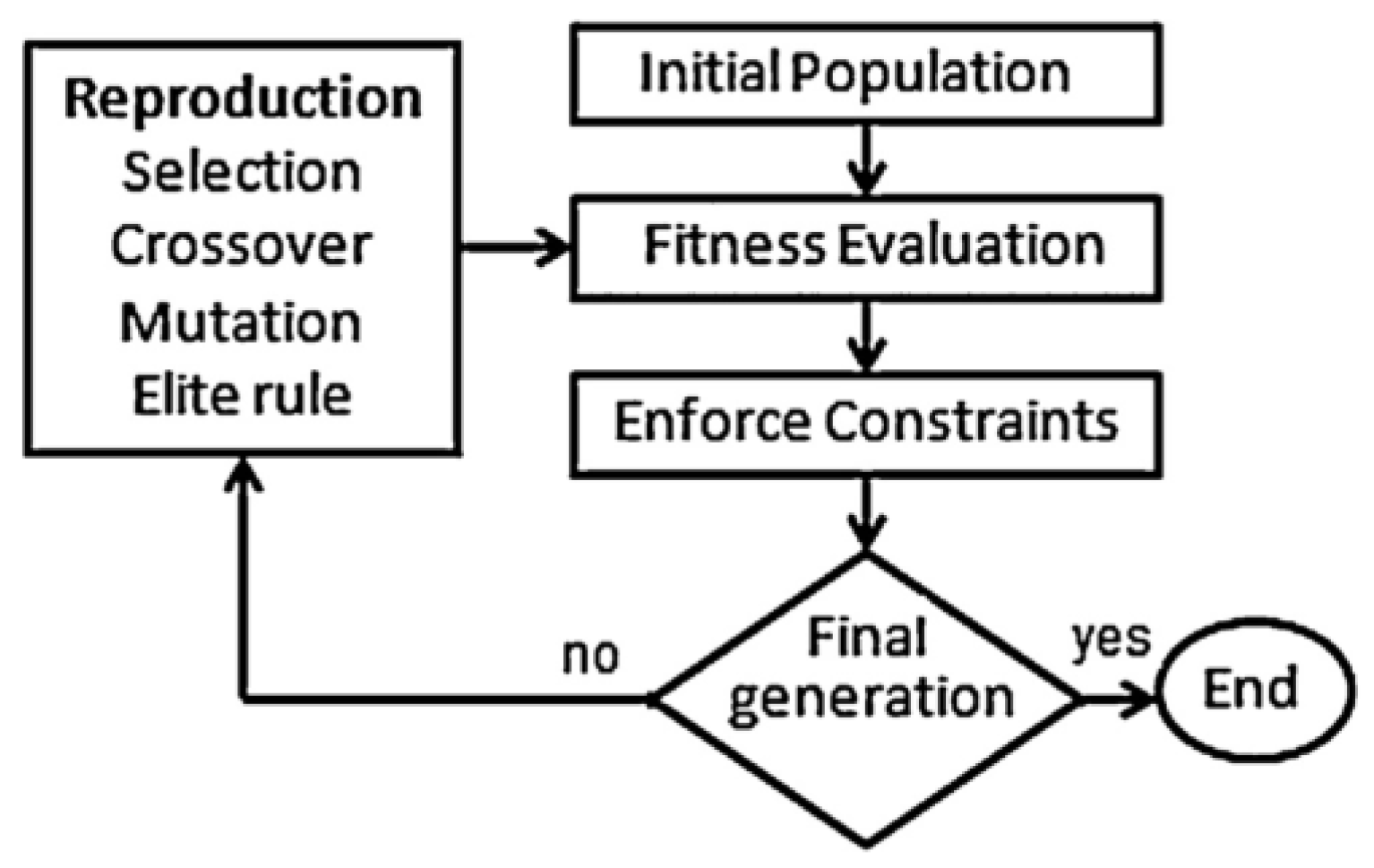
5.2.1. Genetic Algorithm (GA)
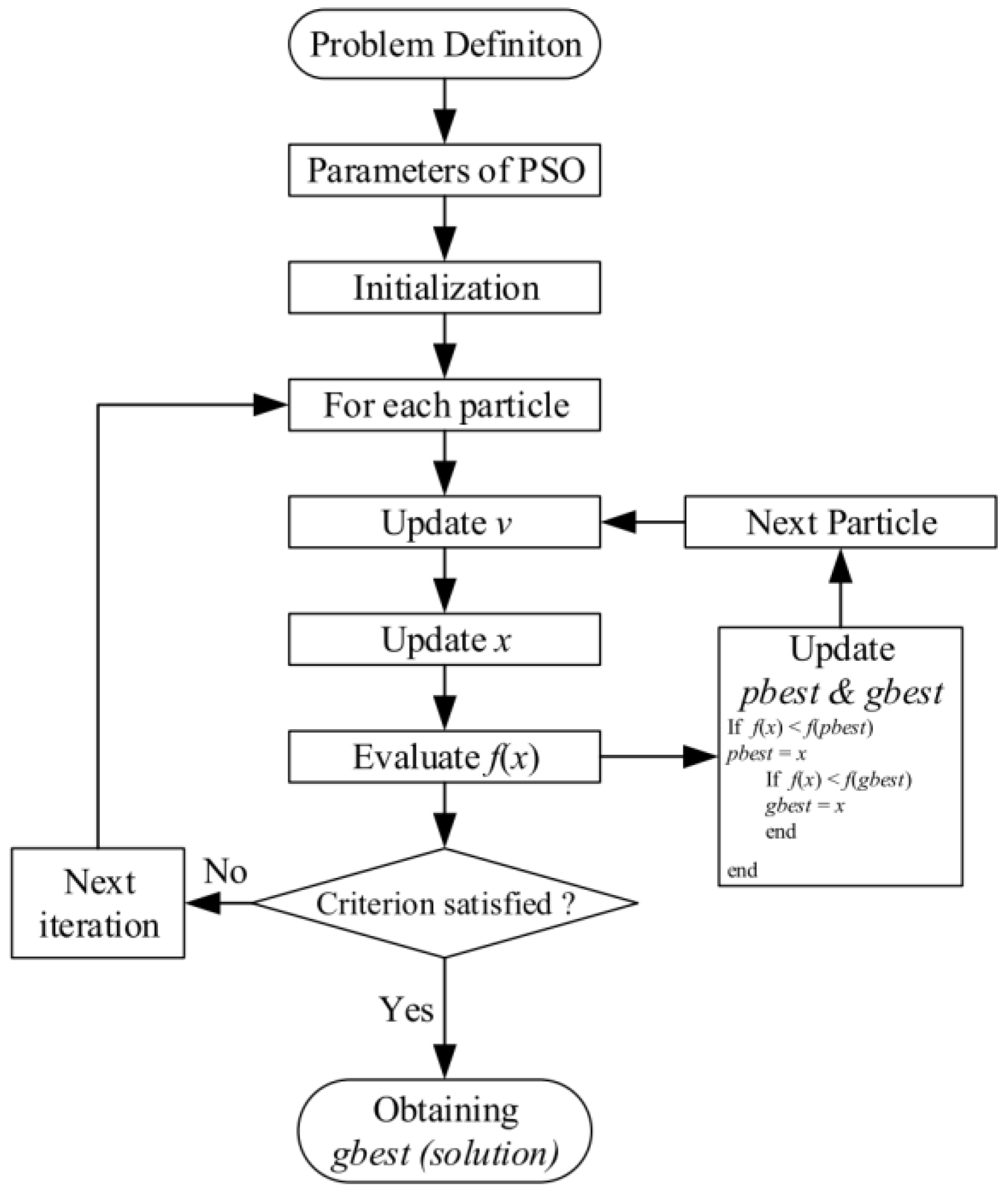
5.2.2. Particle Swarm Optimization (PSO)
5.2.3. Artificial Immune Systems (AIS)
6. Conclusions and Future Highlight
- From the perspective of the research problem, most of the current research is limited to the study of steady-state performance, and lacks in-depth analysis and the modeling of dynamic performance or the whole transmission system. For different transient conditions such as PMECCs start-up, sudden load addition and reduction, and input torque step response, a transient analysis model of PMECCs can be established to accurately reflect the dynamic characteristics of PMECCs.
- The current optimization methods are based on deterministic problems without considering uncertainty conditions, such as material variability, manufacturing tolerances, and device installation errors. Such problems need to be combined with actual production application scenarios; they need to introduce uncertainty data in the production and application processes, perform robust optimization, and make a comprehensive optimization of the PMECC in the manufacturing and product application processes.
- Many advanced methods and research tools currently applied to electromagnetic devices can be introduced for the study of PMECCs, such as physically constrained neural networks, dimensional analysis, fractal geometry theory, and multidisciplinary optimization design.
- The research on PMECCs should be developed in the direction of multidisciplinary integration, using advanced materials, processing processes, and control methods to expand the products, performance, and applications of PMECCs.
- In addition, research can be conducted from microscopic perspectives such as material composition and magnetization methods, and an in-depth analysis of the mechanism of eddy current generation, especially transient directional eddy current, is needed to explore the mechanism of eddy current heating and torque generation, to suppress eddy current loss, and to improve the transmission efficiency of PMECCs.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Alshammari, S.J.; Lazari, P.; Atallah, K. Comparison of Eddy Current Coupling Topologies for High Efficiency Mechanical Power Transmission. IEEE Trans. Energy Convers. 2022, 38, 982–992. [Google Scholar] [CrossRef]
- Waloyo, H.T.; Ubaidillah, U.; Tjahjana, D.D.D.P.; Nizam, M.; Koga, T. Mini Review on the Design of Axial Type Eddy Current Braking Technology. Int. J. Power Electron. Drive Syst. 2019, 10, 2198. [Google Scholar] [CrossRef]
- Putra, M.R.A.; Nizam, M.; Tjahjana, D.D.D.P.; Prabowo, A.R. Mini Review on Eddy Current Brakes Parameter. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1096, 012027. [Google Scholar] [CrossRef]
- Gulec, M.; Aydin, M.; Sergeant, P. Eddy Current Brakes: A Review on Working Principles and Technology Evolution. In Proceedings of the 2022 International Conference on Electrical Machines (ICEM), Valencia, Spain, 5–8 September 2022; IEEE: Piscataway, NJ, USA, 2022; pp. 441–447. [Google Scholar]
- Huang, Z.W.; Hua, X.G.; Chen, Z.Q.; Niu, H.W. Modeling, Testing, and Validation of an Eddy Current Damper for Structural Vibration Control. J. Aerosp. Eng. 2018, 31, 04018063. [Google Scholar] [CrossRef]
- Li, D.; Ikago, K.; Yin, A. Structural Dynamic Vibration Absorber Using a Tuned Inerter Eddy Current Damper. Mech. Syst. Signal Process. 2023, 186, 109915. [Google Scholar] [CrossRef]
- Li, S.; Li, Y.; Wang, J.; Chen, Z. Theoretical Investigations on the Linear and Nonlinear Damping Force for an Eddy Current Damper Combining with Rack and Gear. J. Vib. Control 2022, 28, 1035–1044. [Google Scholar] [CrossRef]
- Diez-Jimenez, E.; Rizzo, R.; Gómez-García, M.-J.; Corral-Abad, E. Review of Passive Electromagnetic Devices for Vibration Damping and Isolation. Shock. Vib. 2019, 2019, 1250707. [Google Scholar] [CrossRef]
- Zhang, H.Y.; Chen, Z.Q.; Hua, X.G.; Huang, Z.W.; Niu, H.W. Design and Dynamic Characterization of a Large-Scale Eddy Current Damper with Enhanced Performance for Vibration Control. Mech. Syst. Signal Process. 2020, 145, 106879. [Google Scholar] [CrossRef]
- Ockhuis, D.K.; Kamper, M.J. Static-FE Harmonic Analysis of PM Slip Couplers for Wind Turbine Applications. IEEE Trans. Ind. Appl. 2022, 59, 1446–1456. [Google Scholar] [CrossRef]
- Erasmus, A.S.; Kamper, M.J. Computationally Efficient Analysis of Double PM-Rotor Radial-Flux Eddy Current Couplers. IEEE Trans. Ind. Appl. 2017, 53, 3519–3527. [Google Scholar] [CrossRef]
- Erasmus, A.S.; Kamper, M.J. Analysis for Design Optimisation of Double PM-Rotor Radial Flux Eddy Current Couplers. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 6503–6510. [Google Scholar]
- Mouton, Z.; Kamper, M.J. Modeling and Optimal Design of an Eddy Current Coupling for Slip-Synchronous Permanent Magnet Wind Generators. IEEE Trans. Ind. Electron. 2014, 61, 3367–3376. [Google Scholar] [CrossRef]
- Potgieter, J.H.J.; Kamper, M.J. Optimum Design and Comparison of Slip Permanent-Magnet Couplings with Wind Energy as Case Study Application. IEEE Trans. Ind. Appl. 2014, 50, 3223–3234. [Google Scholar] [CrossRef]
- Mouton, Z.; Kamper, M.J. Design of an Eddy-Current Coupling for Slip-Synchronous Permanent Magnet Wind Generators. In Proceedings of the 2012 XXth International Conference on Electrical Machines, Marseille, France, 2–5 September 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 633–639. [Google Scholar]
- Bronzeri, R.B.; Chabu, I.E. Concept Validation of an Automotive Variable Flow Water Pump With an Eddy Current Magnetic Coupling. IEEE Trans. Transp. Electrif. 2021, 7, 2939–2950. [Google Scholar] [CrossRef]
- Tsai, M.-C.; Chiou, K.-Y.; Wang, S.-H.; Lin, C.-K. Characteristics Measurement of Electric Motors by Contactless Eddy-Current Magnetic Coupler. IEEE Trans. Magn. 2014, 50, 8203804. [Google Scholar] [CrossRef]
- Sinaga, N.; Syaiful; Yunianto, B.; Rifal, M. Experimental and Computational Study on Heat Transfer of a 150 KW Air-Cooled Eddy Current Dynamometer. J. Phys. Conf. Ser. 2019, 1373, 012020. [Google Scholar] [CrossRef]
- Atallah, K.; Wang, J. A Brushless Permanent Magnet Machine with Integrated Differential. IEEE Trans. Magn. 2011, 47, 4246–4249. [Google Scholar] [CrossRef]
- Bloxham, D.A.; Wright, M.T. Eddy-Current Coupling as an Industrial Variable-Speed Drive. Proc. Inst. Electr. Eng. UK 1972, 119, 1149. [Google Scholar] [CrossRef]
- Smythe, W.R. On Eddy Currents in a Rotating Disk. Electr. Eng. 1942, 61, 681–684. [Google Scholar] [CrossRef]
- Wang, J.; Lin, H.; Fang, S.; Huang, Y. A General Analytical Model of Permanent Magnet Eddy Current Couplings. IEEE Trans. Magn. 2014, 50, 8000109. [Google Scholar] [CrossRef]
- Wang, J.; Zhu, J. A Simple Method for Performance Prediction of Permanent Magnet Eddy Current Couplings Using a New Magnetic Equivalent Circuit Model. IEEE Trans. Ind. Electron. 2018, 65, 2487–2495. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Yang, H. A Novel Squirrel-Cage Rotor Permanent Magnet Adjustable Speed Drive With a Non-Rotary Mechanical Flux Adjuster. IEEE Trans. Energy Convers. 2021, 36, 1036–1044. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Huang, H.; Chen, C.; Yang, H. Analysis and Performance Evaluation of an Efficient Power-Fed Permanent Magnet Adjustable Speed Drive. IEEE Trans. Ind. Electron. 2019, 66, 784–794. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Huang, H.; Yang, H.; Tao, Q.; Fang, S. Analytical Analysis of a Novel Brushless Hybrid Excited Adjustable Speed Eddy Current Coupling. Energies 2019, 12, 308. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Yang, H.; Fang, S.; Wang, H. Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy-Current Coupling With a Movable Stator Ring. IEEE Trans. Magn. 2018, 54, 8000404. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Tao, Q.; Lu, X.; Yang, H.; Fang, S.; Wang, H. Analytical Analysis of an Adjustable-Speed Permanent Magnet Eddy-Current Coupling with a Non-Rotary Mechanical Flux Adjuster. IEEE Trans. Magn. 2019, 55, 8000805. [Google Scholar] [CrossRef]
- Tian, M.; Zhao, W.; Wang, X.; Wang, D.; Yang, Y.; Diao, J.; Ma, X. Analysis on a Novel Flux Adjustable Permanent Magnet Coupler with a Double-Layer Permanent Magnet Rotor. IEEE Trans. Magn. 2018, 54, 8109205. [Google Scholar] [CrossRef]
- Cheng, X.; Liu, W.; Luo, W.; Tan, Z.; Liu, S. Semi-Analytical Model for Temperature Estimation of Permanent Magnet Couplers. IEEE Trans. Energy Convers. 2021, 36, 2068–2078. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y.; Wang, L. An Improved Analytical Model of Permanent Magnet Eddy Current Magnetic Coupler Based on Electromagnetic-Thermal Coupling. IEEE Access 2020, 8, 95235–95250. [Google Scholar] [CrossRef]
- Fontchastagner, J.; Lubin, T.; Mezani, S.; Takorabet, N. Design Optimization of an Axial-Field Eddy-Current Magnetic Coupling Based on Magneto-Thermal Analytical Model. Open Phys. 2018, 16, 21–26. [Google Scholar] [CrossRef]
- Zheng, D.; Wang, D.; Li, S.; Shi, T.; Li, Z.; Yu, L. Eddy Current Loss Calculation and Thermal Analysis of Axial-Flux Permanent Magnet Couplers. AIP Adv. 2017, 7, 025117. [Google Scholar] [CrossRef]
- Wang, S.; Guo, Y.; Cheng, G.; Li, D. Performance Study of Hybrid Magnetic Coupler Based on Magneto Thermal Coupled Analysis. Energies 2017, 10, 1148. [Google Scholar] [CrossRef]
- Jin, Y.; Kou, B.; Li, L.; Pan, D. Fluid Flow and Thermal Analysis of an Axial Flux Permanent Magnet Eddy Current Brake. IEEE Trans. Veh. Technol. 2022, 71, 260–268. [Google Scholar] [CrossRef]
- Tian, J.; Li, D.; Ning, K.; Ye, L. A Timesaving Transient Magneto-Thermal Coupling Model for the Eddy Current Brake. IEEE Trans. Veh. Technol. 2020, 69, 10832–10841. [Google Scholar] [CrossRef]
- Monzón-Verona, J.M.; González-Domínguez, P.I.; García-Alonso, S.; Santana-Martín, F.J.; Cárdenes-Martín, J.F. Thermal Analysis of a Magnetic Brake Using Infrared Techniques and 3D Cell Method with a New Convective Constitutive Matrix. Sensors 2019, 19, 2028. [Google Scholar] [CrossRef] [PubMed]
- Gulec, M.; Lindh, P.; Aydin, M.; Pyrhonen, J. Cost Minimization of a Permanent Magnet Eddy Current Brake by Multiobjective Particle Swarm Optimization Based on Nonlinear Reluctance Network Modeling. IEEE Access 2021, 9, 157361–157370. [Google Scholar] [CrossRef]
- Niu, B.; Wang, D.; Pan, P. Multi-Objective Optimal Design of Permanent Magnet Eddy Current Retarder Based on NSGA-II Algorithm. Energy Rep. 2022, 9, 1448–1456. [Google Scholar] [CrossRef]
- Pan, P.; Wang, D.; Niu, B. Design Optimization of APMEC Using Chaos Multi-Objective Particle Swarm Optimization Algorithm. Energy Rep. 2021, 7, 531–537. [Google Scholar] [CrossRef]
- El-Wakeel, A.S. Design Optimization of PM Couplings Using Hybrid Particle Swarm Optimization-Simplex Method (PSO-SM) Algorithm. Electr. Power Syst. Res. 2014, 116, 29–35. [Google Scholar] [CrossRef]
- Tarvirdilu-Asl, R.; Yüksel, M.; Keysan, O. Multi-Objective Design Optimization of a Permanent Magnet Axial Flux Eddy Current Brake. Turk. J. Electr. Eng. Comput. Sci. 2019, 14, 998–1011. [Google Scholar] [CrossRef]
- Li, Z.; Li, Y.; Qu, B.; Yang, H.; Zhu, X.; Wang, D. Evaluation and Analysis of Flux-Regulated Permanent Magnet Linear Eddy Current Brakes. IEEE Trans. Ind. Appl. 2023, 59, 712–725. [Google Scholar] [CrossRef]
- Li, Z.; Yang, H.; Zhu, X. Performance Analysis of a Novel Double Sided Flux Adjustable Linear Permanent Magnet Eddy Current Brake. In Proceedings of the 2021 13th International Symposium on Linear Drives for Industry Applications (LDIA), Wuhan, China, 1 July 2021; IEEE: Piscataway, NJ, USA, 2021; pp. 1–4. [Google Scholar]
- Yang, P.; Zhou, G.; Zhu, Z.; Tang, C.; He, Z.; Wang, P. Linear Permanent Magnet Eddy Current Brake for Overwinding Protection. IEEE Access 2019, 7, 33922–33931. [Google Scholar] [CrossRef]
- Li, X.; Ye, L.; Li, M.; Lv, Q. Research on Temperature and Braking Performance of Water-Cooled Eddy Current Retarder. IEEE Access 2021, 9, 38991–38998. [Google Scholar] [CrossRef]
- Tian, J.; Li, D.; Ning, K.; Ye, L. Research on Heat Dissipation Optimization of a Novel Liquid-Cooling Eddy Current Brake. IEEE Trans. Energy Convers. 2021, 36, 131–138. [Google Scholar] [CrossRef]
- Colle, A.; Lubin, T.; Ayat, S.; Gosselin, O.; Leveque, J. Analytical Model for the Magnetic Field Distribution in a Flux Modulation Superconducting Machine. IEEE Trans. Magn. 2019, 55, 8107309. [Google Scholar] [CrossRef]
- Gulec, M.; Yolacan, E.; Aydin, M. Design, Analysis and Real Time Dynamic Torque Control of Single-rotor–Single-stator Axial Flux Eddy Current Brake. IET Electr. Power Appl. 2016, 10, 869–876. [Google Scholar] [CrossRef]
- Gulec, M.; Aydin, M.; Nerg, J.; Lindh, P.; Pyrhonen, J.J. Magneto-Thermal Analysis of an Axial-Flux Permanent-Magnet-Assisted Eddy-Current Brake at High-Temperature Working Conditions. IEEE Trans. Ind. Electron. 2021, 68, 5112–5121. [Google Scholar] [CrossRef]
- Dong, K.; Yu, H.; Hu, M.; Liu, J.; Huang, L.; Zhou, J. Study of Axial-Flux-Type Superconducting Eddy-Current Couplings. IEEE Trans. Appl. Supercond. 2017, 27, 0600905. [Google Scholar] [CrossRef]
- Dorget, R.; Lubin, T.; Ayat, S.; Leveque, J. 3-D Semi-Analytical Model of a Superconducting Axial Flux Modulation Machine. IEEE Trans. Magn. 2021, 57, 9000415. [Google Scholar] [CrossRef]
- Belguerras, L.; Mezani, S.; Lubin, T. Analytical Modeling of an Axial Field Magnetic Coupler with Cylindrical Magnets. IEEE Trans. Magn. 2021, 57, 8000305. [Google Scholar] [CrossRef]
- Belguerras, L.; Hadjout, L.; Mezani, S.; Lubin, T.; Rezzoug, A. Study of HTS Magnetic Coupler Using Analytical and Numerical Computations. IEEE Trans. Appl. Supercond. 2014, 24, 3600912. [Google Scholar] [CrossRef]
- Kenne Telezing, B.J.; Yang, C.; Ombolo, P.D.; Peng, Z.; Tai, J.; Zhu, L. Torque Characteristics Analysis of a Novel Hybrid Superconducting Magnetic Coupling with Axial-Flux Using a Magnetic Equivalent Circuit Model. IEEE Access 2022, 10, 45594–45604. [Google Scholar] [CrossRef]
- Dorget, R.; Lubin, T. Non-Linear 3-D Semi-Analytical Model for an Axial Flux Reluctance Magnetic Coupling. IEEE Trans. Energy Convers. 2022, 37, 2037–2047. [Google Scholar] [CrossRef]
- Lubin, T.; Vahaj, A.A.; Rahideh, A. Design Optimisation of an Axial-flux Reluctance Magnetic Coupling Based on a Two-dimensional Semi-analytical Model. IET Electr. Power Appl. 2020, 14, 901–910. [Google Scholar] [CrossRef]
- Huang, Y.; Ge, B.; Dong, J.; Lin, H.; Zhu, J.; Guo, Y. 3-D Analytical Modeling of No-Load Magnetic Field of Ironless Axial Flux Permanent Magnet Machine. IEEE Trans. Magn. 2012, 48, 2929–2932. [Google Scholar] [CrossRef]
- Lubin, T.; Fontchastagner, J.; Mezani, S.; Rezzoug, A. Comparison of Transient Performances for Synchronous and Eddy-Current Torque Couplers. In Proceedings of the 2016 XXII International Conference on Electrical Machines (ICEM), Lausanne, Switzerland, 4–7 September 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 695–701. [Google Scholar]
- Dolisy, B.; Mezani, S.; Lubin, T.; Leveque, J. A New Analytical Torque Formula for Axial Field Permanent Magnets Coupling. IEEE Trans. Energy Convers. 2015, 30, 892–899. [Google Scholar] [CrossRef]
- Park, J.; Paul, S.; Chang, J.; Hwang, T.; Yoon, J. Design and Comparative Survey of High Torque Coaxial Permanent Magnet Coupling for Tidal Current Generator. Int. J. Electr. Power Energy Syst. 2020, 120, 105966. [Google Scholar] [CrossRef]
- Zerioul, A.; Hadjout, L.; Ouazir, Y.; Mezani, S.; Messadi, M. 3D Fast Analytical Computation of a Permanent Magnets Axial Coupler. COMPEL 2022, 41, 1054–1071. [Google Scholar]
- Akcay, Y.; Giangrande, P.; Gerada, C.; Galea, M. Comparative Analysis Between Axial and Coaxial Magnetic Couplings. In Proceedings of the 10th International Conference on Power Electronics, Machines and Drives (PEMD 2020), Virtual, 15–17 December 2020; Institution of Engineering and Technology: Stevenage, UK, 2021; pp. 385–390. [Google Scholar]
- Akcay, Y.; Giangrande, P.; Tweedy, O.; Galea, M. Fast and Accurate 2D Analytical Subdomain Method for Coaxial Magnetic Coupling Analysis. Energies 2021, 14, 4656. [Google Scholar] [CrossRef]
- Lubin, T.; Mezani, S.; Rezzoug, A. Simple Analytical Expressions for the Force and Torque of Axial Magnetic Couplings. IEEE Trans. Energy Convers. 2012, 27, 536–546. [Google Scholar] [CrossRef]
- Jin, Y.; Kou, B.; Li, L. Improved Analytical Modeling of an Axial Flux Double-Sided Eddy-Current Brake with Slotted Conductor Disk. IEEE Trans. Ind. Electron. 2022, 69, 13277–13286. [Google Scholar] [CrossRef]
- Lubin, T.; Rezzoug, A. Steady-State and Transient Performance of Axial-Field Eddy-Current Coupling. IEEE Trans. Ind. Electron. 2015, 62, 2287–2296. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, D.; Wang, X.; Wang, X. Equivalent Circuit Model of Eddy Current Device. IEEE Trans. Magn. 2018, 54, 8001009. [Google Scholar] [CrossRef]
- Dai, X.; Cao, J.; Long, Y.; Liang, Q.; Mo, J.; Wang, S. Analytical Modeling of an Eddy-Current Adjustable-Speed Coupling System with a Three-Segment Halbach Magnet Array. Electr. Power Compon. Syst. 2015, 43, 1891–1901. [Google Scholar] [CrossRef]
- Chaojun, Y.; Yingzhi, W.; Kang, L.; Zhibao, L.; Weifeng, Z.; Ming, L. Analysis for Axial Force of Slotted-Type Axial-Flux Magnetic Coupler. In Proceedings of the 2017 5th International Conference on Mechanical, Automotive and Materials Engineering (CMAME), Guangzhou, China, 1–3 August 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 359–364. [Google Scholar]
- Mohammadi, S.; Mirsalim, M.; Vaez-Zadeh, S.; Talebi, H.A. Analytical Modeling and Analysis of Axial-Flux Interior Permanent-Magnet Couplers. IEEE Trans. Ind. Electron. 2014, 61, 5940–5947. [Google Scholar] [CrossRef]
- Li, Z.; Wang, D.; Zheng, D. Accurate Prediction and Analysis of Electromagnetic Fields and Forces in Flux-Focusing Eddy Current Coupling with Double Slotted Conductor Rotors. IEEE Access 2018, 6, 37685–37699. [Google Scholar] [CrossRef]
- Waloyo, H.T.; Nizam, M.; Tiahiana, D.; Ubaidillah. Parametric Design in Single Disk Axial Eddy Current Brake. In Proceedings of the 2018 5th International Conference on Electric Vehicular Technology (ICEVT), Surakarta, Indonesia, 30–31 October 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 132–135. [Google Scholar]
- Wang, J. A Generic 3-D Analytical Model of Permanent Magnet Eddy-Current Couplings Using a Magnetic Vector Potential Formulation. IEEE Trans. Ind. Electron. 2022, 69, 663–672. [Google Scholar] [CrossRef]
- Guo, B.; Li, D.; Shi, J.; Gao, Z. A Performance Prediction Model for Permanent Magnet Eddy-Current Couplings Based on the Air-Gap Magnetic Field Distribution. IEEE Trans. Magn. 2022, 58, 8000809. [Google Scholar] [CrossRef]
- Cheng, X.; Liu, W.; Luo, W.; Sun, M.; Zhang, Y. A Simple Modelling on Transmission Torque of Eddy-current Axial Magnetic Couplings Considering Thermal Effect. IET Electric. Power Appl. 2022, 16, 434–446. [Google Scholar] [CrossRef]
- Li, W.; Wang, D.; Kong, D.; Wang, S.; Hua, Z. A Novel Method for Modeling the Air Gap Flux Density of the Axial-Flux Permanent Magnet Eddy Current Coupler Considering the End-Effect. Energy Rep. 2021, 7, 508–514. [Google Scholar] [CrossRef]
- Mohammadi, S.; Kirtley, J.; Niaz Azari, M. Modelling of Axial-flux Eddy-current Couplers. IET Electr. Power Appl. 2020, 14, 1238–1246. [Google Scholar] [CrossRef]
- Li, W.; Wang, D.; Tong, T.; Kong, D.; Wang, S.; Hua, Z. A Simple 3-D Analytical Modeling Method and Sensitivity Analysis for the Axial-Flux Permanent Magnet Eddy Current Coupler. In Proceedings of the 2022 International Conference on Power Energy Systems and Applications (ICoPESA), Singapore, 25–27 February 2022; IEEE: Piscataway, NJ, USA, 2022; pp. 77–82. [Google Scholar]
- Lubin, T.; Rezzoug, A. 3-D Analytical Model for Axial-Flux Eddy-Current Couplings and Brakes Under Steady-State Conditions. IEEE Trans. Magn. 2015, 51, 8203712. [Google Scholar] [CrossRef]
- Mohammadi, S.; Mirsalim, M.; Vaez-Zadeh, S.; Lesani, H. Sensitivity Analysis and Prototyping of a Surface-Mounted Permanent-Magnet Axial-Flux Coupler. In Proceedings of the 5th Annual International Power Electronics, Drive Systems and Technologies Conference (PEDSTC 2014), Tehran, Iran, 5–6 February 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 568–573. [Google Scholar]
- Robert, R.S. 2D Model of Axial-Flux Eddy Current Brakes with Slotted Conductive Disk Rotor. In Proceedings of the 2017 International Siberian Conference on Control and Communications (SIBCON), Astana, Kazakhstan, 29–30 June 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 1–6. [Google Scholar]
- Canova, A.; Vusini, B. Design of Axial Eddy-Current Couplers. IEEE Trans. Ind. Appl. 2003, 39, 725–733. [Google Scholar] [CrossRef]
- Aberoomand, V.; Mirsalim, M.; Fesharakifard, R. Design Optimization of Double-Sided Permanent-Magnet Axial Eddy-Current Couplers for Use in Dynamic Applications. IEEE Trans. Energy Convers. 2019, 34, 909–920. [Google Scholar] [CrossRef]
- Meng, X.; Yang, G.; Song, L. Research on Torque Characteristics for a Novel Permanent Magnetic Coupler with Bilateral Structure. In Proceedings of the 2014 IEEE Conference and Expo Transportation Electrification Asia-Pacific (ITEC Asia-Pacific), Beijing, China, 31 August–3 September 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 1–5. [Google Scholar]
- Gulec, M.; Aydin, M.; Lindh, P.; Pyrhonen, J. Investigation of Braking Torque Characteristic for a Double-Stator Single-Rotor Axial-Flux Permanent-Magnet Eddy-Current Brake. In Proceedings of the 2018 XIII International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 793–797. [Google Scholar]
- Mohammadi, S.; Mirsalim, M.; Vaez-Zadeh, S.; Talebi, H.A. Design Analysis of a New Axial-Flux Interior Permanent-Magnet Coupler. In Proceedings of the 5th Annual International Power Electronics, Drive Systems and Technologies Conference (PEDSTC 2014), Tehran, Iran, 5–6 February 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 562–567. [Google Scholar]
- Li, Z.; Wang, D.; Zheng, D.; Yu, L. Analytical Modeling and Analysis of Magnetic Field and Torque for Novel Axial Flux Eddy Current Couplers with PM Excitation. AIP Adv. 2017, 7, 105303. [Google Scholar] [CrossRef]
- Jin, P.; Tian, Y.; Lu, Y.; Guo, Y.; Lei, G.; Zhu, J. 3-D Analytical Magnetic Field Analysis of the Eddy Current Coupling With Halbach Magnets. IEEE Trans. Magn. 2020, 56, 7501904. [Google Scholar] [CrossRef]
- Lu, X.; He, X.; Jin, P.; Huang, Q.; Yang, S.; Chen, M. General 3D Analytical Method for Eddy-Current Coupling with Halbach Magnet Arrays Based on Magnetic Scalar Potential and H-Functions. Energies 2021, 14, 8458. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y. An Improved Performance Prediction Model of Permanent Magnet Eddy Current Couplings Based on Eddy Current Inductance Characteristics. AIP Adv. 2019, 9, 035350. [Google Scholar] [CrossRef]
- Ye, L.; Li, D.; Ma, Y.; Jiao, B. Design and Performance of a Water-Cooled Permanent Magnet Retarder for Heavy Vehicles. IEEE Trans. Energy Convers. 2011, 26, 953–958. [Google Scholar] [CrossRef]
- Shin, K.-H.; Park, H.-I.; Cho, H.-W.; Choi, J.-Y. Semi-Three-Dimensional Analytical Torque Calculation and Experimental Testing of an Eddy Current Brake with Permanent Magnets. IEEE Trans. Appl. Supercond. 2018, 28, 5203205. [Google Scholar] [CrossRef]
- Guo, W.; Li, D.; Ye, L. A Model of Magnetic Field and Braking Torque in Liquid-Cooled Permanent-Magnet Retarder Accounting for the Skin Effect on Permeability. IEEE Trans. Veh. Technol. 2019, 68, 10618–10626. [Google Scholar] [CrossRef]
- Mohammadi, S.; Mirsalim, M.; Vaez-Zadeh, S. Nonlinear Modeling of Eddy-Current Couplers. IEEE Trans. Energy Convers. 2014, 29, 224–231. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, W.; Cheng, N. Magnetic Field and Eddy Current Analysis of Permanent Magnet Eddy Current Coupling. In Proceedings of the 2015 IEEE International Conference on Information and Automation, Lijiang, China, 8–10 August 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 2817–2822. [Google Scholar]
- Zhu, Y.; Wang, H.; Li, H.; Yang, C.; Gui, J. Transmission Performance of Halbach Array Cylindrical Permanent Magnet Governor. Electronics 2023, 12, 1161. [Google Scholar] [CrossRef]
- Dai, X.; Liang, Q.; Ren, C.; Cao, J.; Mo, J.; Wang, S. Analytical Solution of Magnetic Field in Permanent-Magnet Eddy-Current Couplings by Considering the Effects of Slots and Iron-Core Protrusions. J. Magn. 2015, 20, 273–283. [Google Scholar] [CrossRef]
- Liang, Q.; Mo, J.; Dai, X.; Long, Y.; Cao, J.; Wang, S. Modeling and Analysis of Field Modulated Permanent-Magnet Eddy-Current Couplings with a Slotted Conductor Rotor. Int. J. Appl. Electromagn. Mech. 2016, 52, 1295–1303. [Google Scholar] [CrossRef]
- Yang, J.; Wang, D.; Ge, Y. Experimental Research on Adjustable-Speed Characteristics of Squirrel Cage Asynchronous Magnetic Coupling. In Proceedings of the 2021 Photonics & Electromagnetics Research Symposium (PIERS), Hangzhou, China, 21–25 November 2021; IEEE: Piscataway, NJ, USA, 2021; pp. 220–229. [Google Scholar]
- Sun, K.; Shi, J.; Cui, W.; Meng, G. Theoretical Computational Model for Cylindrical Permanent Magnet Coupling. Electronics 2021, 10, 2026. [Google Scholar] [CrossRef]
- Mohammadi, S.; Mirsalim, M. Design Optimization of Double-Sided Permanent-Magnet Radial-Flux Eddy-Current Couplers. Electr. Power Syst. Res. 2014, 108, 282–292. [Google Scholar] [CrossRef]
- Mohammadi, S.; Mirsalim, M. Double-sided Permanent-magnet Radial-flux Eddy-current Couplers: Three-dimensional Analytical Modelling, Static and Transient Study, and Sensitivity Analysis. IET Electric. Power Appl. 2013, 7, 665–679. [Google Scholar] [CrossRef]
- Li, Z.; Yan, L.; Qu, B.; Fu, K. Analytical Prediction and Optimization of Torque Characteristic for Flux-Concentration Cage-Type Eddy-Current Couplings with Slotted Conductor Rotor Topology. Int. J. Appl. Electromagn. Mech. 2020, 62, 295–313. [Google Scholar] [CrossRef]
- Mohammadi, S.; Mirsalim, M.; Niazazari, M.; Talebi, H.A. A New Interior Permanent-Magnet Radial-Flux Eddy-Current Coupler. In Proceedings of the 5th Annual International Power Electronics, Drive Systems and Technologies Conference (PEDSTC 2014), Tehran, Iran, 5–6 February 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 500–505. [Google Scholar]
- Li, D.; Wang, S.; Guo, Y.; Ma, X. Modelling and Experimental Study of Halbach Array Magnetic Coupler. KSCE J. Civ. Eng. 2023, 27, 261–269. [Google Scholar] [CrossRef]
- Li, D.; Wang, S. Characteristics of New Permanent Magnetic Eddy Current Drive System of the Scraper Conveyor. J. Eng. 2021, 2021, 552–558. [Google Scholar] [CrossRef]
- Wang, S.; Guo, Y.; Li, D.; Su, C. Modelling and Experimental Research on the Equivalent Magnetic Circuit Network of Hybrid Magnetic Couplers Considering the Magnetic Leakage Effect. IET Electr. Power Appl. 2019, 13, 1413–1421. [Google Scholar] [CrossRef]
- Li, W.; Wang, D.; Gao, Q.; Kong, D.; Wang, S. Modeling and Performance Analysis of an Axial-Radial Combined Permanent Magnet Eddy Current Coupler. IEEE Access 2020, 8, 78367–78377. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Howe, D. Halbach Permanent Magnet Machines and Applications: A Review. IEE Proc. Electr. Power Appl. 2001, 148, 299. [Google Scholar] [CrossRef]
- Wang, J.; Lin, H.; Fang, S. Analytical Prediction of Torque Characteristics of Eddy Current Couplings Having a Quasi-Halbach Magnet Structure. IEEE Trans. Magn. 2016, 52, 8001209. [Google Scholar] [CrossRef]
- Tongyu, S.; Dazhi, W. 3D Analytical Model of Drag and Lift Force for a Conductive Plate Moving above a Halbach Magnet Array. Trans. Inst. Meas. Control. 2018, 40, 3515–3524. [Google Scholar] [CrossRef]
- Li, Z.; Wang, D.; Zheng, D. Analytical Predictions and Analysis of Electromagnetic Field and Torque for Eddy-Current Couplers with Quasi-Halbach Magnet Array. Int. J. Appl. Electromagn. Mech. 2018, 57, 347–366. [Google Scholar] [CrossRef]
- Park, M.-G.; Choi, J.-Y.; Shin, H.-J.; Jang, S.-M. Torque Analysis and Measurements of a Permanent Magnet Type Eddy Current Brake with a Halbach Magnet Array Based on Analytical Magnetic Field Calculations. J. Appl. Phys. 2014, 115, 17E707. [Google Scholar] [CrossRef]
- Zheng, D.; Guo, X. Analytical Prediction and Analysis of Electromagnetic-Thermal Fields in PM Eddy Current Couplings With Injected Harmonics Into Magnet Shape for Torque Improvement. IEEE Access 2020, 8, 60052–60061. [Google Scholar] [CrossRef]
- Razavi, H.K.; Lamperth, M.U. Eddy-Current Coupling with Slotted Conductor Disk. IEEE Trans. Magn. 2006, 42, 405–410. [Google Scholar] [CrossRef]
- Dai, X.; Liang, Q.; Cao, J.; Long, Y.; Mo, J.; Wang, S. Analytical Modeling of Axial-Flux Permanent Magnet Eddy Current Couplings with a Slotted Conductor Topology. IEEE Trans. Magn. 2016, 52, 8000315. [Google Scholar] [CrossRef]
- Shi, T.; Wang, D.; Zheng, D. Optimal Design and Analysis of a New Type of Eddy-Current Driver. In Proceedings of the 27th Chinese Control and Decision Conference (2015 CCDC), Qingdao, China, 23–25 May 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 6187–6191. [Google Scholar]
- You, S.-A.; Wang, S.-H.; Tsai, M.-C.; Mao, S.-H. Characteristic Analysis of Slotted-Type Axial-Flux Permanent Magnetic Couplers. In Proceedings of the 2012 15th International Conference on Electrical Machines and Systems, Sapporo, Japan, 21–24 October 2012; p. 5. [Google Scholar]
- Ubaidillah, U.; Suwolo, S.; Prayoga, A.R.; Nizam, M.; Waloyo, H.T. Magnetic Flux Distribution and Braking Torque of a Grooved Eddy Current Brake. In Proceedings of the 2020 2nd International Conference on Computer and Information Sciences (ICCIS), Sakaka, Saudi Arabia, 13–15 October 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 1–5. [Google Scholar]
- Prayoga, A.R.; Ubaidillah; Nizam, M.; Waloyo, H.T. The Influence of Aluminum Conductor Shape Modification on Eddy-Current Brake Using Finite Element Method. In Proceedings of the 2019 6th International Conference on Electric Vehicular Technology (ICEVT), Bali, Indonesia, 18–21 November 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 146–150. [Google Scholar]
- Deshan, K.; Dazhi, W.; Wenhui, L.; Sihan, W.; Zhong, H. Analysis of a Novel Flux Adjustable Axial Flux Permanent Magnet Eddy Current Coupler. IET Electric. Power Appl. 2023, 17, 181–194. [Google Scholar] [CrossRef]
- Kong, D.; Wang, D.; Ni, Y.; Song, K.; Qi, Y.; Li, Y. Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy Current Coupling with Double-Sided Conductor. Actuators 2023, 12, 105. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, L.; Qu, B.; Yang, H.; Wang, D. Evaluation and Analysis of Novel Flux-adjustable Permanent Magnet Eddy Current Couplings with Multiple Rotors. IET Electr. Power Appl. 2021, 15, 754–768. [Google Scholar] [CrossRef]
- Pyrhonen, J.; Jokinen, T.; Hrabovcová, V. Design of Rotating Electrical Machines, 2nd ed.; Wiley: Hoboken, NJ, USA, 2014; ISBN 978-1-118-58157-5. [Google Scholar]
- Gay, S.E.; Ehsani, M. Parametric Analysis of Eddy-Current Brake Performance by 3-D Finite-Element Analysis. IEEE Trans. Magn. 2006, 42, 319–328. [Google Scholar] [CrossRef]
- Chen, J.; Wang, S.; Shang, H.; Hu, H.; Peng, T. Finite Element Analysis of Axial Flux Permanent Magnetic Hysteresis Dampers Based on Vector Jiles-Atherton Model. IEEE Trans. Energy Convers. 2022, 37, 2472–2481. [Google Scholar] [CrossRef]
- Russell, R.L.; Norsworthy, K.H. Eddy Currents and Wall Losses in Screened-Rotor Induction Motors. Proc. IEE A Power Eng. UK 1958, 105, 163. [Google Scholar] [CrossRef]
- Chen, C.; Liu, Y.; Wang, Y. A Novel Model of Magnetic Eddy-Current Coupling Based on the Magnetic Equivalent Circuit Method. Dtmse 2017, 59, 1481–1491. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Wang, D.; Shi, T.; Bai, X. Performance Prediction and Analysis of Disk-Type Eddy-Current Drivers. Trans. Inst. Meas. Control 2018, 40, 1568–1578. [Google Scholar] [CrossRef]
- Li, Y.; Huang, J.; Gao, F.; Zhu, Z.; Han, Y.; Li, Y.; Sun, Q.; Zhang, D. The Analytical Comparative Analysis of Electromagnetic Characteristics of Four Typical Radial Flux Permanent Magnet Eddy Current Couplers. Energies 2021, 14, 8407. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y.; Wang, L. Nonlinear Modeling of Transmission Performance for Permanent Magnet Eddy Current Coupler. Math. Probl. Eng. 2019, 2019, 2098725. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, D.; Ning, Y.; Zheng, D. Performance Study of Axial-Flux Eddy Current Coupler Based on the Equivalent Circuit Model. IEEE Access 2018, 6, 65679–65689. [Google Scholar] [CrossRef]
- Yang, C.; Peng, Z.; Tai, J.; Zhu, L.; Telezing, B.J.K.; Ombolo, P.D. Torque Characteristics Analysis of Slotted-Type Eddy-Current Couplings Using a New Magnetic Equivalent Circuit Model. IEEE Trans. Magn. 2020, 56, 8000613. [Google Scholar] [CrossRef]
- Yang, F.; Zhu, J.; Yang, C.; Ding, Y.; Hang, T. A Simple Method to Calculate the Torque of Magnet-Rotating-Type Axial Magnetic Coupler Using a New Magnetic Equivalent Circuit Model. IEEE Trans. Magn. 2022, 58, 8003212. [Google Scholar] [CrossRef]
- Xikang, C.; Wei, L.; Yang, Z.; Sitong, L.; Weiqi, L. A Concise Transmitted Torque Calculation Method for Pre-Design of Axial Permanent Magnetic Coupler. IEEE Trans. Energy Convers. 2020, 35, 938–947. [Google Scholar] [CrossRef]
- Lubin, T.; Rezzoug, A. Improved 3-D Analytical Model for Axial-Flux Eddy-Current Couplings with Curvature Effects. IEEE Trans. Magn. 2017, 53, 8002409. [Google Scholar] [CrossRef]
- Shin, H.-J.; Choi, J.-Y.; Cho, H.-W.; Jang, S.-M. Analytical Torque Calculations and Experimental Testing of Permanent Magnet Axial Eddy Current Brake. IEEE Trans. Magn. 2013, 49, 4152–4155. [Google Scholar] [CrossRef]
- Zheng, D.; Guo, X.; Li, Y.; Cong, W.; Jiang, S. Torque Analysis of Axial-Flux Permanent Magnet Couplers with Skewed Slots Using Electromagnetic-Thermal Semi-Analytical Model. In Proceedings of the 2020 Chinese Control and Decision Conference (CCDC), Hefei, China, 22–24 August 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 4077–4081. [Google Scholar]
- Wang, A.; Wang, J.; Shi, C.; Zhao, F. Study on Nonlinear Regression Modeling Methods of the Permanent Magnet Drive. In Proceedings of the 2011 International Conference on Electronics and Optoelectronics, Dalian, China, 29–31 July 2011; IEEE: Piscataway, NJ, USA, 2011; pp. V2–V87. [Google Scholar]
- Wang, D.; Zheng, D.; Li, S.; Li, Z.; Liang, B.; Ni, Y. Electromagnetic-Thermal Analysis of Axial-Flux Permanent Magnet Eddy Current Couplers with Sub-Loop Calculation Method. J. Magn. 2018, 23, 509–516. [Google Scholar] [CrossRef]
- Ye, L.; Cao, M.; Liu, Y.; Li, D. Multi-Field Coupling Analysis and Demagnetization Experiment of Permanent Magnet Retarder for Heavy Vehicles (MAY 2018). IEEE Access 2019, 7, 50734–50745. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, D. Design Optimization of Axial-Flux Eddy Current Coupler. In Proceedings of the 2018 IEEE 3rd Advanced Information Technology, Electronic and Automation Control Conference (IAEAC), Chongqing, China, 12–14 October 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 989–993. [Google Scholar]
- Zhang, B.; Wan, Y.; Li, Y.; Feng, G. Optimized Design Research on Adjustable-Speed Permanent Magnet Coupling. In Proceedings of the 2013 IEEE International Conference on Industrial Technology (ICIT), Cape Town, South Africa, 25–28 February 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 380–385. [Google Scholar]
- Wang, S.; Hu, K.; Li, D. Optimal Design Method for the Structural Parameters of Hybrid Magnetic Coupler. J. Mech. Sci. Technol. 2019, 33, 173–182. [Google Scholar] [CrossRef]
- Li, J.; Yang, G.; Fan, Y. Characteristic Analysis of a Permanent Magnet Eddy-Current Brake Under Strong Impact Load. In Proceedings of the 2020 IEEE 19th Biennial Conference on Electromagnetic Field Computation (CEFC), Pisa, Italy, 16–19 November 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 1–4. [Google Scholar]
- Canova, A.; Freschi, F.; Gruosso, G.; Vusini, B. Genetic Optimisation of Radial Eddy Current Couplings. COMPEL—Int. J. Comput. Math. Electr. Electron. Eng. 2005, 24, 767–783. [Google Scholar] [CrossRef]
- Erasmus, A.S.; Kamper, M.J. Multi-Objective Design Optimisation and Pareto Front Visualisation of Radial-Flux Eddy Current Coupling for a Wind Generator Drivetrain. In Proceedings of the 2016 IEEE Energy Conversion Congress and Exposition (ECCE), Milwaukee, WI, USA, 18–22 September 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 1–8. [Google Scholar]
- Kennedy, J.; Eberhart, R. Particle Swarm Optimization. In Proceedings of the ICNN’95—International Conference on Neural Networks, Perth, WA, Australia, 27 November 1995; IEEE: Piscataway, NJ, USA, 1995; Volume 4, pp. 1942–1948. [Google Scholar]
- Coello, C.A.C.; Pulido, G.T.; Lechuga, M.S. Handling Multiple Objectives with Particle Swarm Optimization. IEEE Trans. Evol. Comput. 2004, 8, 256–279. [Google Scholar] [CrossRef]
- El-Wakeel, A.S.; Smith, A.C. Hybrid Fuzzy-Particle Swarm Optimization-Simplex (F-PSO-S) Algorithm for Optimum Design of PM Drive Couplings. Electr. Power Compon. Syst. 2015, 43, 1560–1571. [Google Scholar] [CrossRef]
- Zhao, L.; Dazhi, W.; Zhen, L. Multi-Objective Optimization Design of Permanent Magnet Drive Based on Kriging Model. In Proceedings of the 27th Chinese Control and Decision Conference (2015 CCDC), Qingdao, China, 23–25 May 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 847–851. [Google Scholar]
- De Castro, L.N.; Von Zuben, F.J. Artificial Immune Systems: Part I–Basic Theory and Applications; Dezembro de, Technical Report; Universidade Estadual de Campinas: Campinas, Brazil, 1999; Volume 210. [Google Scholar]
- Jerne, N.K. Towards a Network Theory of the Immune System. Ann. Immunol. 1974, 125, 373–389. [Google Scholar]
- Canova, A.; Freschi, F. Multiobjective Design Optimization and Pareto Front Analysis of a Radial Eddy Current Coupler. Int. J. Appl. Electromagn. Mech. 2010, 32, 219–236. [Google Scholar] [CrossRef]


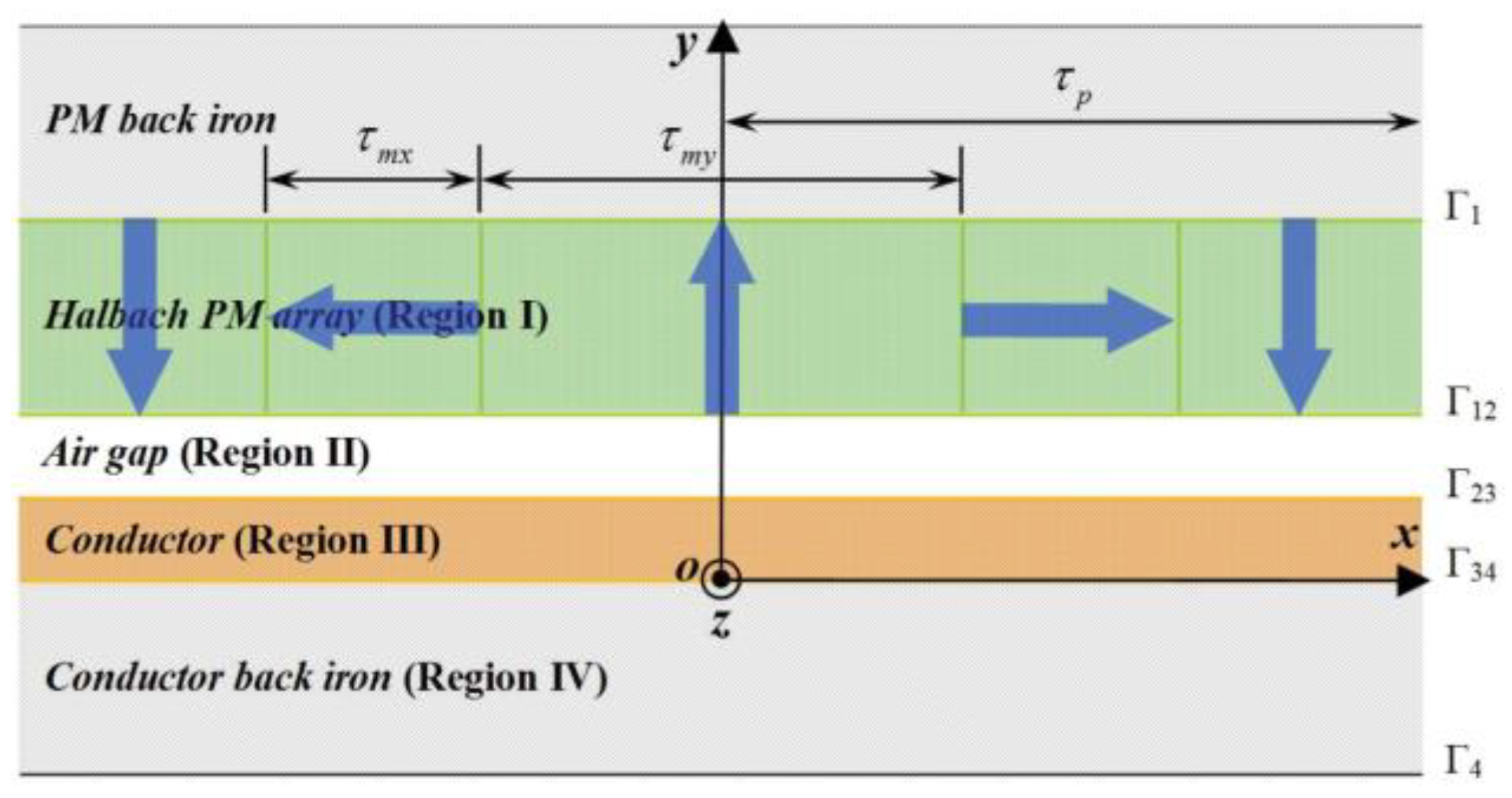





| Coordinate | Dimension | Reference System | Reference |
|---|---|---|---|
| Cartesian coordinate | 2-D | PM + Conductor Rotor | [77] |
| Cartesian coordinate | 2-D | PM Rotor | [67] |
| Cartesian coordinate | 2-D | Conductor Rotor | [72] |
| Cartesian coordinate | 2-D | Conductor Rotor | [117] |
| Cartesian coordinate | 2-D | Conductor Rotor | [104] |
| Cartesian coordinate | 3-D | PM Rotor | [79] |
| Cartesian coordinate | 3-D | Conductor Rotor | [58] |
| Cylindrical coordinate | 2-D | PM Rotor | [66] |
| Cylindrical coordinate | 2-D | Conductor Rotor | [72] |
| Cylindrical coordinate | 2-D | Conductor Rotor | [117] |
| Cylindrical coordinate | 2-D | Conductor Rotor | [104] |
| Cylindrical coordinate | 3-D | PM + Conductor Rotor | [79] |
| Cylindrical coordinate | 3-D | PM + Conductor Rotor | [58] |
| Cylindrical coordinate | 3-D | Conductor Rotor | [137] |
| Cylindrical coordinate | 3-D | Conductor Rotor | [138] |
| Cylindrical coordinate | 3-D | Conductor Rotor | [115] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Wang, D.; Wang, S.; Tong, T.; Sun, L.; Li, W.; Kong, D.; Hua, Z.; Sun, G. A Review of Recent Developments in Permanent Magnet Eddy Current Couplers Technology. Actuators 2023, 12, 277. https://doi.org/10.3390/act12070277
Wang J, Wang D, Wang S, Tong T, Sun L, Li W, Kong D, Hua Z, Sun G. A Review of Recent Developments in Permanent Magnet Eddy Current Couplers Technology. Actuators. 2023; 12(7):277. https://doi.org/10.3390/act12070277
Chicago/Turabian StyleWang, Jiaxing, Dazhi Wang, Sihan Wang, Tailai Tong, Lisong Sun, Wenhui Li, Deshan Kong, Zhong Hua, and Guofeng Sun. 2023. "A Review of Recent Developments in Permanent Magnet Eddy Current Couplers Technology" Actuators 12, no. 7: 277. https://doi.org/10.3390/act12070277
APA StyleWang, J., Wang, D., Wang, S., Tong, T., Sun, L., Li, W., Kong, D., Hua, Z., & Sun, G. (2023). A Review of Recent Developments in Permanent Magnet Eddy Current Couplers Technology. Actuators, 12(7), 277. https://doi.org/10.3390/act12070277






