Tracking-Differentiator-Based Position and Acceleration Feedback Control in Active Vibration Isolation with Electromagnetic Actuator

Abstract

In order to improve the performance of the active vibration isolation system (AVIS) with electromagnetic actuator, several problems of vibration control are studied. Position control is a critical component in suspension systems, and the position sensor noise can extremely affect the stability of the system, so a tracking differentiator (TD) is proposed to obtain effective differential signal from relative position sensor. In vibration control, the feedback of acceleration combined with PD position feedback is presented to suppress transmission of periodic vibrations. Then, taking the acceleration transmission as the evaluation index, the acceleration transmission under the presented control method is derived, and the influence of control parameters on vibration isolation performance is discussed in detail. The vibration isolation performance is improved from 24.47 dB to 2.4 dB at resonance frequency, and −34 dB attenuation is achieved at 100 Hz with respect to vibration isolation mount system tested on the ground. The experimental results demonstrate that the performance of active vibration isolation system are improved by the proposed acceleration feedback control.

1. Introduction

Mechanical vibration widely exists in the environment of the vehicle-mounted equipment. The good vibration isolation performance is the basic guarantee for the vehicle-mounted equipment, and it is of great significance for improving equipment performance. The vibration isolation system is mainly divided into passive vibration isolation systems and active vibration isolation systems (AVIS). Passive isolation is commonly achieved between the vibration source and the isolated object by introducing a material with known stiffness and damping characteristics [1,2]. Once the passive vibration isolation is designed, the vibration isolation bandwidth is predetermined, which is not easy to adjust, and the low-frequency vibration isolation performance is restricted.

AVIS has gained increasing visibility due to its ability to provide controllable and adjustable control forces, which enable it to achieve effective isolation even at low frequencies. AVIS with electromagnetic actuator has gained attention because of its advantages of large output force, wide adjustment range, and rapid response.

In recent years, numerous researchers have been focusing on the challenges associated with achieving vibration isolation through magnetic suspension. The nonlinear mass and damping effects of nonlinear electromagnetic shunt damping for vibration isolation systems is presented, and performance enhancement of a permanent magnet-based nonlinear vibration isolator is investigated [3]. To improve the performance of low-frequency vibration isolation compared with conventional variable stiffness elements, a novel compact and contactless multi-layer electromagnetic spring with tunable negative stiffness is proposed [4]. On the basis of electromagnetic technology and air spring technology, a magnet–air hybrid quasi-zero stiffness vibration isolation system is designed [5]. An active control system is developed for the proposed electromagnetic torsional active vibration absorber utilizing the filter-x least mean square (FxLMS) algorithm [6].

A new kind of vibration isolation platform with actuators based on the magnetic suspension techniques is presented [7]. The negative stiffness magnetic spring comprising a pair of coaxial ring permanent magnets is installed in parallel with the mechanical spring to counteract its positive stiffness [8]. A magnetic spring with negative stiffness has been proposed. This magnetic spring operates based on variable reluctance stress and consists of permanent magnets, a mover, and two stators. By utilizing these components, the system is able to create a negative stiffness effect in the axial direction of the spring [9]. A permanent and electromagnet composite vibration isolation system is designed based on negative stiffness theory, and the active control strategy based on the fuzzy PID algorithm is applied [10].

AVIS with magnetic actuator has been under development for decades, and various control approaches have been proposed. The control methods mainly include state feedback control, relative position feedback control, and force feedback control. The model of a single strut of the vibration isolation platform with the currents control are designed [11]. A multi loop feedback control scheme including position loop, velocity loop, acceleration loop and current loop is designed, and the feasibility of the control scheme through isolation experiment is verified [12]. The controller of the magnetic isolator with permanent magnets is designed with displacement feedback control [13]. The LMS acceleration feedback algorithm for the Stewart platform is investigated and validated through numerical simulations [14]. The robustness of magnetic spring to probable time delay, sensor measurement noise, and actuation error is simulated and verified, and LQR state feedback control is used to realize isolation [15]. A Stewart platform with piezoelectric actuators is presented. In the active control loop, the direct feedback of integrated forces is combined with the FxLMS-based adaptive feedback to dampen vibration of inherent modes and suppress transmission of periodic vibrations [16]. The cascade PID control with the force feedback is proposed for active vibration isolation [17]. A hybrid isolator consisting of maglev actuator and air spring is proposed and developed for application in active-passive vibration isolation system of ship machinery and the force/current gains of actuators are tested [18]. The force feedback and acceleration feedback implementation of the sky-hook damper is compared when it is used to isolate a flexible structure from a disturbance source [19]. The output force response of the fuzzy PID control semi-active vibration isolation system and passive system under the same excitation are simulated. The performance of the magnetic suspension vibration isolation system is tested with the proposed fuzzy control algorithm [20]. With respect to a fundamental limitation of using the PD type flotor acceleration controller, an integral and double integral type flotor acceleration controller is proposed [21]. A control law is proposed for the bistable nonlinear electromagnetic actuator with an elastic boundary system, which involves a polynomial function of the mover’s absolute velocity [22].

A new data-driven H∞ norm estimation algorithm for model-error modeling of multivariable AVIS is presented [23].

The performance of AVIS is often constrained by sensor noise. The sensor fusion technique has been used in order to increase the control bandwidth. Then, at low frequency, the low noise inertial instrument signal dominates the fusion to increase the control bandwidth, and other types of sensors (relative motion, smaller but noisier inertial, or force sensors) are used at higher frequencies to increase stability [24]. In the vibration isolation system, an active dynamic vibration absorber is used as an accelerometer in a low-frequency range, whereas it operates as a vibration control device in a high-frequency range [25]. The nano-g level optical accelerometer is suitable for low-frequency (less than 1 Hz) noise suppression, and it finds potential applications in seismic acceleration measurement for mineral exploration and active vibration isolation [26]. Sensor fusion is used to realize a low transmissibility of AVIS and to increase the damping ratio of internal vibration modes and to provide a high support stiffness [27].

The control methods of AVIS were discussed based on the current state of the art. The research related to magnetic suspension is mainly based on active and passive composite research or AVIS with voice coil actuator, but there are few studies on AVIS with electromagnetic actuator. This paper mainly studies the several problems in AVIS with electromagnetic actuator. The differential signal of the relative position sensor has a great influence on the stability of the system. The signal is generally filtered by the first-order inertial filter. In the vibration system, the vibration source is a broadband signal at 2–100 Hz. So, the obtained signal is required to have a small attenuation of amplitude and a small phase delay in the broadband frequency, but for the first-order inertial filter, there is a trade-off between filtering effect and phase delay. A tracking differentiator (TD) is proposed to obtain the differential signal with low noise and small phase delay. The contributions of this study mainly lie in the following three points:

• The tracking differentiator is introduced as lowpass filter to obtain the differential signal of the relative position sensor with less influence of sensor noise, and the system stability performance is enhanced.
• The acceleration feedback control is proposed to strengthen the connection with absolute space and to improve the performance of AVIS with electromagnetic actuator.
• The proposed method is applied to the vibration control problem of AVIS, where the noise of the relative position sensor affects the stability of system. Experimental tests under sinusoidal vibrating source show the effectiveness of the proposed controller.

The organization of this paper is as follows: In Section 2, the structure of AVIS with electromagnetic actuator is described. Then, the dynamic model of the AVIS is given. The stability of the plant model is analyzed. In Section 3, the controller design of the AVIS is proposed, and the parameters of position feedback control and acceleration feedback control is analyzed. The experimental results are presented, and the feasibility of the control scheme is verified by experiments in Section 4. Finally, conclusions are given in Section 5.

2. AVIS Modeling

The AVIS with a single degree of freedom in the vertical direction is taken as the research object, and the characteristics of the AVIS with electromagnetic actuator are analyzed after modeling.

2.1. AVIS Structure

As shown in Figure 1, the AVIS with electromagnetic actuator is a system that can perform single-degree-of-freedom movement in the vertical direction. The control force of the vibration isolation system is output by the upper and lower E-type electromagnets. The AVIS is achieved by controlling the current input to the two electromagnets and adjusting the output control force of the two electromagnets.

The vibration isolation system mainly includes the isolated object, vibration table, and the base. On the base, there are upper and lower E-type electromagnets which attract each other with the armature on the isolated object. The isolated object can move in the vertical direction under the electromagnetic force provided by the upper and lower electromagnets, and its stroke is 16 mm. The vibration table serves as the excitation source and is capable of generating different types of vibration signals. In this study, a sinusoidal vibration signal is produced using the vibration table, and the vibration isolation performance of the isolator is analyzed under the influence of this sinusoidal vibration. The base is fixedly connected with the vibration table, and the base vibrates together with the vibration table. The upper and lower electromagnets provide the active control force, which is regulated by the control differential. Therefore, the controller design is the key part of the vibration isolation system, and the performance of the controller directly affects the vibration isolation performance of the system.

This AVIS is equipped with two types of sensors, namely position sensor and accelerometer. Accelerometer #1 and accelerometer #2 measure the acceleration of the isolated object and the base, respectively. The position sensor measures the air gap. The controller design for achieving stable suspension and vibration isolation performance is based on the feedback signals from both types of sensors.

2.2. Dynamic Modeling

Before beginning the analysis of the electromagnetic suspension system, the electromagnetic force formula is based on the following assumptions: (a) the magnetic field distribution is uniform, (b) the armature is not saturated, (c) there is no magnetic leakage and hysteresis, (d) the frictional resistance of the isolated object during motion is neglected. In the designed structure of AVIS, the differential control is carried out to control two E-type electromagnets. The force of the isolated object is shown in Figure 2, and z is the absolute position of the isolated object, r is the absolute position of electromagnet, m is the equivalent mass of the isolated object, $F_{e1}$ and $F_{e2}$ are the electromagnetic forces of the lower and upper electromagnets, $i_1$ and $i_2$ are the total current input by the lower and upper electromagnetic coils, respectively, $h_1$ and $h_2$ are the gaps between the controlled object and the lower and upper electromagnets, respectively, $u_1$ and $u_2$ are the total voltage of the lower and upper electromagnet, respectively, and $R_1$ and $R_2$ are equivalent resistances of lower and upper electromagnet coils, respectively.

It is specified that the downward movement of the isolated object is the positive direction of the system, so the dynamic equation of the isolated object can be written as

$$\begin{array}{c}\hfill m\ddot{z}=F_{e1}-F_{e2}+mg,\end{array}$$

(1)

In Equation (1), the electromagnetic force of the upper and lower electromagnets can be expressed as

$$\begin{array}{c}\hfill F_{e1}=K{\left(i_1/h_1\right)}^2,\end{array}$$

(2)

$$\begin{array}{c}\hfill F_{e2}=K{\left(i_2/h_2\right)}^2.\end{array}$$

(3)

where $K={\mu }_{0}A{N}^2/4$, ${\mu }_0$ is vacuum permeability, A is effective magnetic circuit area of electromagnet, N is the number of turns of electromagnet coil. The currents of electromagnets can be expressed as

$$\left\{\begin{array}{c}\hfill i_1=i_{10}+\Delta i\hfill \\ \hfill i_2=i_{20}-\Delta i\hfill \end{array}\right.,$$

(4)

where $\Delta i$ is control current, $i_{10}$ and $i_{20}$ are both constant and denote the initial currents input by the lower and upper electromagnetic coils, respectively.

Then, the derivative of Equation (4) can be obtained:

$$\left\{\begin{array}{c}{i_1}^{\prime }=\Delta i^{\prime }\hfill \\ {i_2}^{\prime }=-\Delta i^{\prime }\hfill \end{array}\right..$$

(5)

The displacement of electromagnet in the system deviates from the center position. It is described by

$$\begin{array}{c}\hfill \Delta h=h_0-h_1=h_2-h_0=z-r,\end{array}$$

(6)

where $h_0$ is the stable suspension gap, and there is

$$\left\{\begin{array}{c}\hfill h_1=h_0-\Delta h\hfill \\ \hfill h_2=h_0+\Delta h\hfill \end{array}\right.,$$

(7)

The derivative of Equation (7) can be obtained:

$$\left\{\begin{array}{c}{h_1}^{\prime }=-\Delta h^{\prime }\hfill \\ {h_2}^{\prime }=\Delta h^{\prime }\hfill \end{array}\right..$$

(8)

After linearization of Equation (2) at the equilibrium point $(h_{10},i_0)$, we can obtain

$$\begin{array}{c}\hfill F_{e1}=F_1\left(i_{10},h_0\right)+K_{i1}\Delta i+K_{h1}\left(z-r\right),\end{array}$$

(9)

where $K_{i1}=\frac{2K{i}_{10}}{h_0^2}$, $K_{h1}=\frac{2K{i}_{10}^2}{h_0^3}$, $F_1\left(i_{10},h_0\right)=K{\left(i_{10}/h_0\right)}^2$.

After linearization of Equation (3) at the equilibrium point $(h_{20},i_0)$, we can obtain

$$\begin{array}{c}\hfill F_{e2}=F_2\left(i_{20},h_0\right)-K_{i2}\Delta i-K_{h2}\left(z-r\right),\end{array}$$

(10)

where $K_{i2}=\frac{2K{i}_{20}}{h_0^2}$, $K_{h2}=\frac{2K{i}_{20}^2}{h_0^3}$, $F_2\left(i_{20},h_0\right)=K{\left(i_{20}/h_0\right)}^2$.

When the system is in steady-state balance, the gap is $h_0$. At this time, the gaps satisfy $h_0=h_1=h_2$, and the forces satisfy

$$\begin{array}{c}\hfill F_1\left(i_{10},h_0\right)-F_2\left(i_{20},h_0\right)+mg=0,\end{array}$$

(11)

The manufacturing parameters of the upper and lower electromagnets, such as coil turns, resistance, inductance and structure size, are the same. Only the bias currents are different during the linearization process. Since the upper electromagnet bears the gravity of the system,

$$\left\{\begin{array}{c}{K}_{i1}+K_{i2}=K_i\hfill \\ K_{h1}+K_{h2}=K_h\hfill \end{array}\right.,$$

(12)

Combining Equations (9)–(12), then we can obtain

$$\begin{array}{c}\hfill F_{e1}-F_{e2}=-mg+K_i\Delta i+K_h(z-r),\end{array}$$

(13)

Combining Equation (1) and Equation (13), the linearized dynamics equation can be represented as

$$\begin{array}{c}\hfill m\ddot{z}=K_i\Delta i+K_h\left(z-r\right),\end{array}$$

(14)

The transfer function of the system from the control current to the position output of the isolated object after Laplace transform can be expressed as

$$\begin{array}{c}\hfill Z\left(s\right)=\frac{K_i}{m{s}^2-K_h}I\left(s\right)-\frac{K_h}{m{s}^2-K_h}R\left(s\right).\end{array}$$

(15)

In the electromagnetic actuators, the actual voltage of the system can be represented as

$$\left\{\begin{array}{c}\hfill u_1=u_{10}+\Delta u\hfill \\ \hfill u_2=u_{20}-\Delta u\hfill \end{array}\right.,$$

(16)

where $u_{10}$ and $u_{20}$ are the bias voltage of the lower and upper electromagnet respectively, $\Delta u$ is the control voltage. The electrical equations can be represented as:

$$\left\{\begin{array}{c}\hfill u_1=R_1{i}_1+\frac{2K}{h_1}{i^{\prime }}_1-\frac{2K}{h_1^2}{h^{\prime }}_1\hfill \\ \hfill u_2=R_2{i}_2+\frac{2K}{h_2}{i^{\prime }}_2-\frac{2K}{h_2^2}{h^{\prime }}_2\hfill \end{array}\right.,$$

(17)

In practical system, there is a delay between $\Delta u$ and $\Delta i$, when the delay is too great and the system will be unstable. Therefore, current feedback is introduced to enable the current in the electromagnet to track the control voltage quickly.

After linearizing the electrical nonlinear equation, the linearized electrical equation can be rewritten as

$$\begin{array}{c}\hfill \Delta u=R\Delta i+\frac{2}{h_0}K\Delta i^{\prime }\end{array},$$

(18)

From Equation (18), it can be seen that this part is a first-order inertial element, which can be equivalently represented as a proportional element. When its proportional coefficient is set to 1, the transfer function of the current feedback can be obtained as

$$\begin{array}{c}\hfill G_i\left(s\right)=\frac{I\left(s\right)}{U\left(s\right)}=1\end{array},$$

(19)

From Equation (19), when the current feedback loop is configured as 1, the transfer function between the control input $\Delta u$ and the position z in the frequency domain can be expressed as:

$$\begin{array}{c}\hfill \frac{Z\left(s\right)}{U\left(s\right)}=\frac{Z\left(s\right)}{I\left(s\right)}\frac{I\left(s\right)}{U\left(s\right)}=\frac{K_i}{m{s}^2-K_h}\end{array}.$$

(20)

Equation (20) shows that the simplified system is an unstable second-order system because of the value of the $K_h$ is large and negative, and the system has a pole at the right plane. So, it is necessary to design a controller to achieve system stability.

3. Stability Control Design

The dynamic model has been analyzed before. After the system model is established, the vibration isolation controller is designed based on the establishment and analysis of the system model.

3.1. Control Objective

The maglev isolation system is a vertical single-degree-of-freedom vibration isolation system. The basic goal of vibration isolation is to control the output response of the system and reduce the vibration transmission of the excitation source in the system. The control objective is that the isolated object keeps the vibration from being amplified when the vibration table excitation is at a low frequency, and when the vibration table is excited to vibrate at high frequency, the system will isolate the excitation signal. At the same time, under the different frequency excitation source signals, the whole system avoids the resonance frequency of the system and improves the vibration isolation performance under the resonance frequency.

3.2. Position Signal Processing with TD

The differential signal of relative position signal is a necessary to achieve the stability of the electromagnetic suspension. The traditional differentiator is very sensitive to signal noise. The TD used here has a good filtering effect on signal noise and can also obtain the effective differential signal.

In the process of measuring position signal, noise is inevitably introduced. If this noisy signal is directly differentiated, the noise of differential signal will be amplified, so the signal needs to be filtered. The first-order inertial filter is usually used to eliminate noise. This filter can improve the bandwidth and the fast tracking characteristics of the system by reducing the time constant $\tau$. The first-order inertial filter can be found as:

$$\begin{array}{c}\hfill H_f\left(s\right)=\frac{1}{\tau s+1}\end{array}.$$

(21)

In the vibration system, the vibration source is a broadband signal with in 2–100 Hz. The position signal and its derivative are required to have a small attenuation of amplitude and a small phase delay in this broadband frequency. When the first-order inertial filter is used for filtering, the design cut-off frequency is 100 Hz, and then the signal amplitude attenuation is within the controllable range, but the phase delay is too large to be used for position stability.

TD has been presented as a filter in [28], and TD can acquire the derivative signal of the tracking signal with less phase delay [29]. So, the TD for relative position signal is proposed as a filter to eliminate noise. For the double-integral system, it is the result of discretization of time optimal control. The concept of the boundary layer is proposed in the TD. The thickness of the boundary layer is changed to regulate the characteristics of TD. When the TD is used as a filter, it can improve the tracking delay characteristics of the signal compared with first-order inertial filter, and at same time, it can output the effective derivative of signal. In order to have the better filtering characteristic, the TD with a filtering factor $c_0$ [30] which determines the effect of noise filtering can be found as

$$\begin{array}{c}\hfill \left\{\begin{array}{c}u=fhan\left(x_1,x_2,r_0,c_{0}h\right)\\ d=r_{0}h,d_0=h_{0}d\\ y=x_1+h{x}_2\\ a_0=\sqrt{d^2+8r\left|y\right|}\\ a=\left\{\begin{array}{c}{x}_2+\frac{\left(a_0-d\right)}{2}sign\left(y\right),\left|y\right|>h^{2}r\\ x_2+y/h,\left|y\right|\le h^2{r}_0\end{array}\right.\\ fhan=-\left\{\begin{array}{c}{r}_{0}sign\left(a\right),\left|a\right|>d\\ r_0\frac{a}{d},\left|a\right|\le d\end{array}\right.\end{array}\right.\end{array},$$

(22)

where h is the integral step size, $r_0$ is the speed factor, sign is the sign function. The $fhan$$(x_1,x_2,r_0,c_{0}h)$ is function expression of TD. When TD is applied as a filter and differentiator, it can be described as

$$\left\{\begin{array}{c}u\left(k\right)=fhan\left(x_1\left(k\right)-v\left(k\right),x_2\left(k\right),r,c_{0}h\right)\hfill \\ x_1(k+1)=x_1\left(k\right)+h{x}_2\left(k\right)\hfill \\ \hfill x_2(k+1)=x_2\left(k\right)+hu\left(k\right),\left|u\left(k\right)\right|\le r,k=0,1,2,\dots \hfill \end{array}\right..$$

(23)

where $v\left(k\right)$ is the input signal to be differentiated. The input signal here is position signal, and the output signal is filtered position signal and its derivative.

3.3. Acceleration Control

The open-loop control of AVIS is unstable, so a closed-loop feedback system must be used to make the system stable. In the electromagnetic suspension system, the gap between electromagnet and the isolated object is the major signal for the control system to maintain the stable suspension. The control algorithm based on this gap signal is known as relative position feedback control. Relative position control goal is to maintain a stable position between the electromagnet and the isolated object. This method has been used in the early electromagnetic suspension system control. For AVIS with electromagnetic suspension, the aim is for the system to track the low-frequency excitation source, but this relative position feedback control scheme is not suitable for high-frequency disturbance. Therefore, the acceleration sensor information of the isolated object is considered to be added to the feedback control system so that there is absolute position information in the system controller. The relative position feedback control enhances the connection between the isolated object and the base. Intuitively, the relative position between the isolated object and the electromagnet is maintained to ensure stable suspension and no collision with the upper and lower electromagnets. The acceleration feedback channel enhances the connection between the isolated object and the absolute space, and the isolation control is the connection with the absolute space. The entire control system can be designed as a block diagram, as shown in Figure 3.

In Figure 3, $A\left(s\right)$ is the acceleration feedback control algorithm, and $C\left(s\right)$ is the relative position feedback control algorithm. In this control system, the absence of $A\left(s\right)$ corresponds to a relative position feedback control, whereas its presence defines an acceleration feedback control (which also includes relative position feedback part).

So, the closed-loop transfer function based on relative position feedback control is in the form of

$$\begin{array}{c}\hfill H_p\left(s\right)=\frac{Z\left(s\right)}{R\left(s\right)}=\frac{K_{i}C\left(s\right)-K_h}{m{s}^2+K_{i}C\left(s\right)-K_h}\end{array},$$

(24)

Then, acceleration transmissibility based on relative position feedback control can be expressed as

$$\begin{array}{c}\hfill T_p\left(s\right)=\frac{\ddot{Z}\left(s\right)}{\ddot{R}\left(s\right)}=\frac{K_{i}C\left(s\right)-K_h}{m{s}^2+K_{i}C\left(s\right)-K_h}\end{array},$$

(25)

So, the closed-loop transfer function based on acceleration feedback control is in the form of

$$\begin{array}{c}\hfill H_a\left(s\right)=\frac{Z\left(s\right)}{R\left(s\right)}=\frac{K_{i}C\left(s\right)-K_h}{\left[m+K_{i}A\left(s\right)\right]s^2+K_{i}C\left(s\right)-K_h}\end{array},$$

(26)

Then, acceleration transmissibility based on acceleration feedback control can be expressed as

$$\begin{array}{c}\hfill T_a\left(s\right)=\frac{\ddot{Z}\left(s\right)}{\ddot{R}\left(s\right)}=\frac{K_{i}C\left(s\right)-K_h}{\left[m+K_{i}A\left(s\right)\right]s^2+K_{i}C\left(s\right)-K_h}\end{array},$$

(27)

Then, it can be seen that the acceleration transmissibility formula is the same as the position formula. In the previous acceleration algorithm design process, acceleration feedback is commonly implemented using a PID control scheme. The existence of differential terms in $A\left(s\right)$ results in a higher system order. Therefore, $A\left(s\right)$ using absolute acceleration, its integration, and its double integration are put forward here:

$$\begin{array}{c}\hfill A\left(s\right)=K_a+K_v\frac{1}{s}+K_s\frac{1}{s^2}\end{array},$$

(28)

where $K_a$ is the coefficient of the acceleration term, $K_v$ is the coefficient of the acceleration integral term, and $K_s$ is the coefficient of the acceleration double integral.

Relative position control algorithm $C\left(s\right)$ can be expressed as

$$\begin{array}{c}\hfill C\left(s\right)=K_{hp}+K_{hd}s+K_{hi}\frac{1}{s}\end{array},$$

(29)

where $K_{hp}$ represents the coefficient of the proportional term, $K_{hd}$ represents the coefficient of the derivative, and $K_{hi}$ represents the coefficient of the integral term.

$K_{hi}$ is the integral term of gap signal, mainly used to adjust the steady-state error of position. In vibration isolation control, steady-state error is not a critical parameter to consider. Therefore, this term will be ignored in the subsequent analysis. In order to focus on the issue, $K_{hi}=0$, is assumed in this section. Then, substituting $A\left(s\right)$ in Equation (28) and $C\left(s\right)$ Equation (29) into Equation (25) and Equation (27) yields

$$T_p\left(s\right)=\frac{K_i{K}_{hd}s+K_i{K}_{hp}-K_h}{m{s}^2+K_i{K}_{hd}s+\left(K_i{K}_{hp}-K_h\right)},$$

(30)

$$T_a\left(s\right)=\frac{K_i{K}_{hd}s+K_i{K}_{hp}-K_h}{\left(m+K_i{K}_a\right)s^2+\left(K_i{K}_v+K_i{K}_{hd}\right)s+\left(K_i{K}_s+K_i{K}_{hp}-K_h\right)},$$

(31)

The system parameters being studied are presented in

Table 1. Parameter of the experimental apparatus.

Symbol	Physical Meaning	Value	Unit
m	Mass of isolated object	16	kg
R	Equivalent resistance of electromagnet coil	0.62	$\mathrm{\Omega }$
N	Turns of electromagnet coil	378
$h_0$	Stable suspension gap	8	mm
$i_{10}$	Initial current of lower electromagnet	3	A
g	Gravity acceleration	9.8	N/kg
${\mu }_0$	Vacuum permeability	$4\pi \times {10}^{-7}$	H/m
A	Magnetic circuit area of electromagnet	$7.2\times {10}^{-3}$	m${}^2$

. Then, the transmissibility of acceleration can be carried out with these parameters. It can be observed from the acceleration transmissibility curve shown in Figure 4 that the relative position feedback control exhibits a large transmissibility of 19.52 dB at 11.69 Hz, but the acceleration feedback control does not exhibit any resonant behavior, and over the entire frequency range, the acceleration feedback control demonstrates better vibration suppression performance.

4. Experimental Analysis of Vibration Isolation Performance

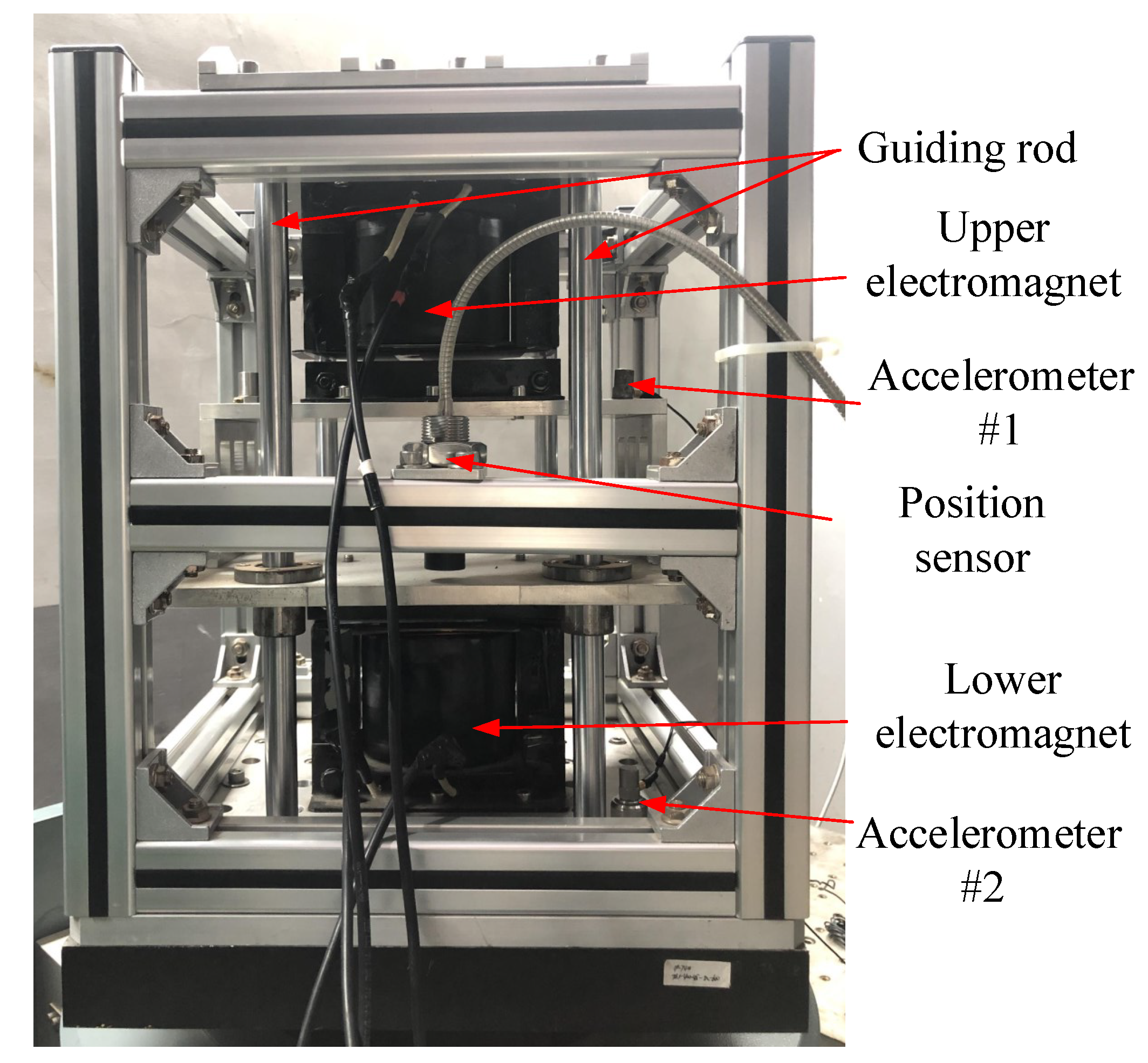
The electromagnetic suspension isolator is shown in Figure 5. The guiding rod is used to maintain the vertical motion of the isolated object and prevent any rotational movement. The accelerometer $\#1$ is mounted on the isolated object to measure its absolute acceleration, and an eddy-current positon sensor is used to measure the relative displacement $z-r$. The accelerometer $\#2$ is placed on the base to monitor its vibration but is not used in control.

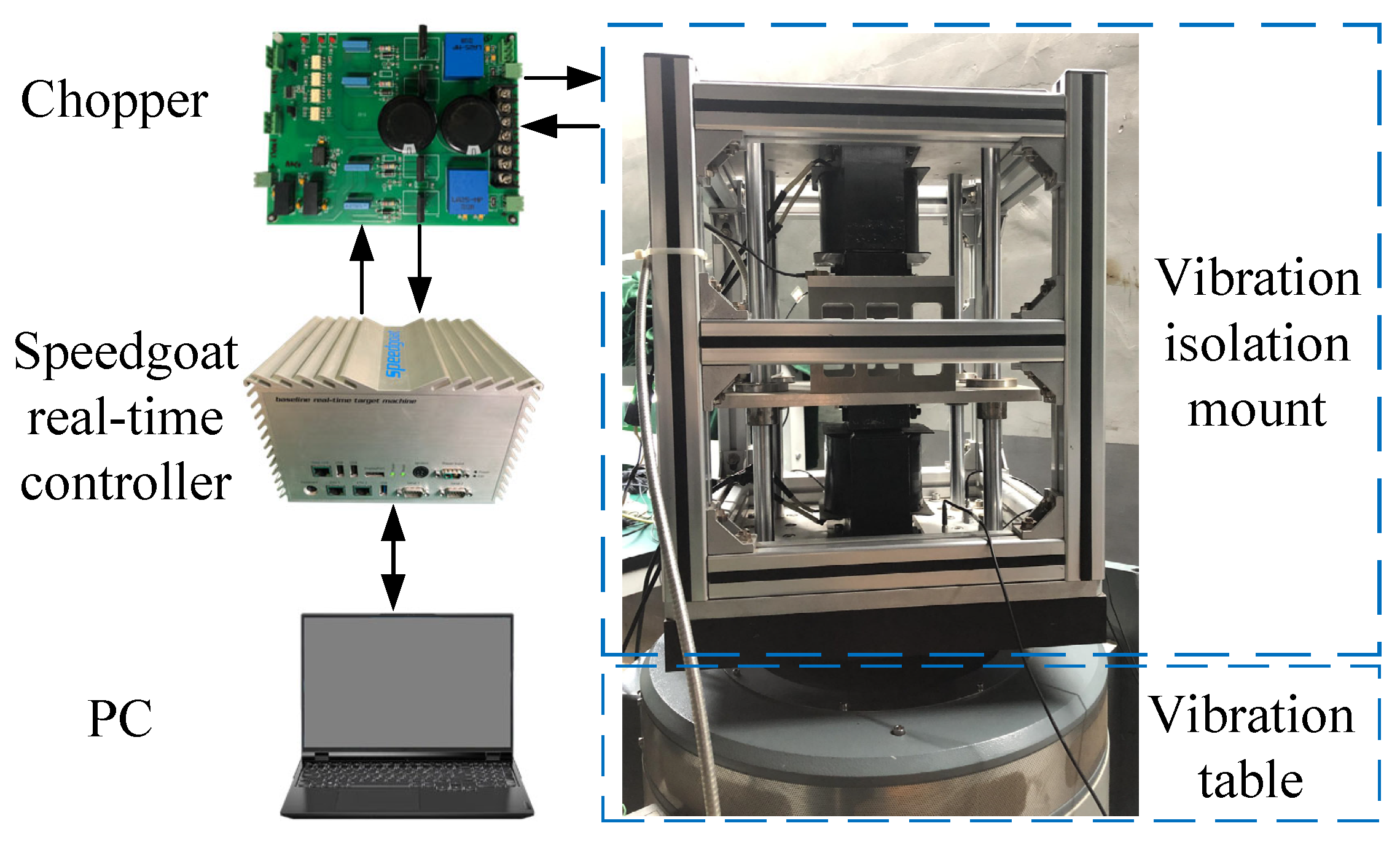
The schematic diagram of the AVIS with electromagnetic actuator test can be seen in Figure 6. The vibration table is used to apply a single-frequency sinusoidal vibration excitation in the frequency band of 2–100 Hz to the vibration isolation platform. The accelerometer has a measurement range of ±5 g. In the vibration isolation system experiment, the control forces are adjusted by changing the working current of the upper and lower electromagnets. The sensor signals are connected to 16 b analog-to-digital converters (ADCs). Low-pass filters (at 1 kHz) are used to reduce high-frequency noise and aliasing. The control is implemented using a speedgoat hosted by a PC. Relative position control and acceleration feedback control are applied to the vibration isolation system. Experimental parameters of AVIS are the same as the theoretical analysis parameters illustrated in Table 1. In order to verify the control strategy and to demonstrate the effectiveness of the proposed control methods, the experimental investigation is carried out with this electromagnetic suspension isolator.

4.1. Experiments of TD

The A/D module is set with a sampling frequency of $F_s$ = 5 kHz to collect the gap signal and acceleration signals. When the base is vibrating, the proposed TD is used to track the gap signal and produce the corresponding velocity signal, where the filtering factor is $c_0=8$ and the quickness factor is $r=1000$. The differentiation with first order inertial filter and the differentiation from TD is compared in the test.

The first-order inertial filter is simplified by FOIF in the test. The comparison results are presented in Figure 7. After conducting FFT analysis on the signal during this time interval, the frequency of the signal is found to be 33 Hz.

Figure 7 shows that the direct derivative of relative position signal has significant noise, which makes it unsuitable for use in control. The derivative signals obtained through the FOIF and TD methods exhibit superior filtering properties and smoother characteristics compared to directly acquired signals, and the TD method provides derivative signals with less phase delay compared to the FOIF method. With the proposed TD, the gap signals can be efficiently tracked with little error, and the derivative signals can be efficiently produced.

4.2. Experiments of Vibration Isolation Effect

The experimental parameters align with the theoretical analysis. The parameters of relative position feedback control are set as $K_p=1900$, $K_d=5$, and the parameters of acceleration feedback control are set as $K_a=0.01$, $K_v=200$, $K_s=1000$. The main objective of the isolation system is to attenuate the acceleration amplitude for the isolated object before and after isolation. Therefore, this paper is only focused on the attenuation of acceleration amplitude. In the experiment, the acceleration values of the base represent the vibration input, which can be considered as the vibration acceleration before isolation. The acceleration values of the isolated object represent the vibration output, which can be considered as the vibration acceleration after isolation. Comparing the acceleration before and after the isolation system is equivalent to comparing the acceleration values of the base and the isolated object. This comparison effectively reflects the isolation effectiveness of the system. Therefore, in this paper, the isolation effectiveness is evaluated by comparing the acceleration values between the base and the isolated object.

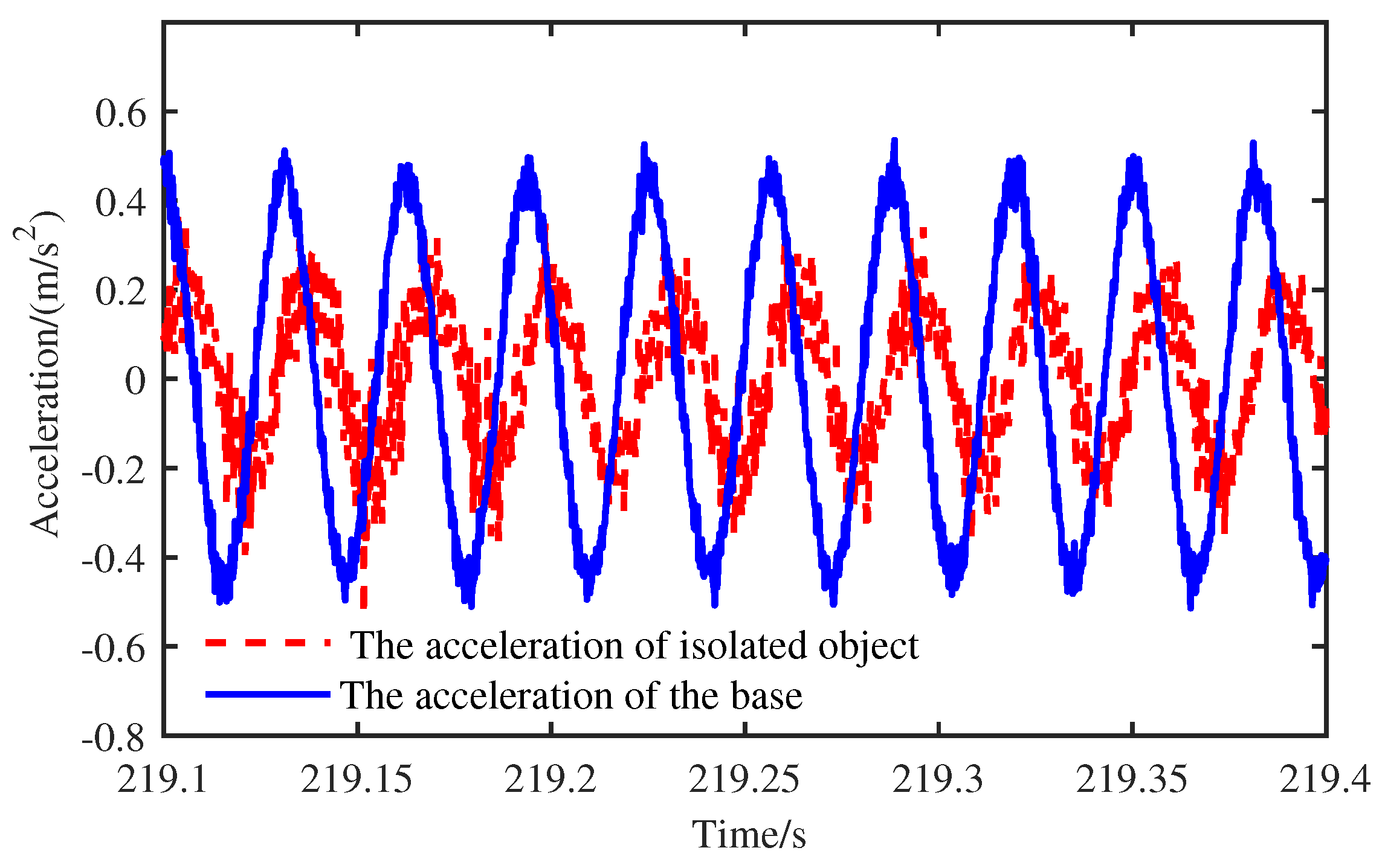
To facilitate a better comparison of the vibration isolation effectiveness between the two control algorithms, this study selects a set of representative signals from the experimental data. Under the condition of the same base vibration frequency, the acceleration amplitudes before and after isolation are compared to analyze the relative performance of the two algorithms in terms of vibration isolation. Sweeping frequency experiments ranging from 2 Hz to 100 Hz are conducted using the two control algorithms. By performing FFT transformation on a segment of the base vibration acceleration data, the frequency of the base vibration is obtained. 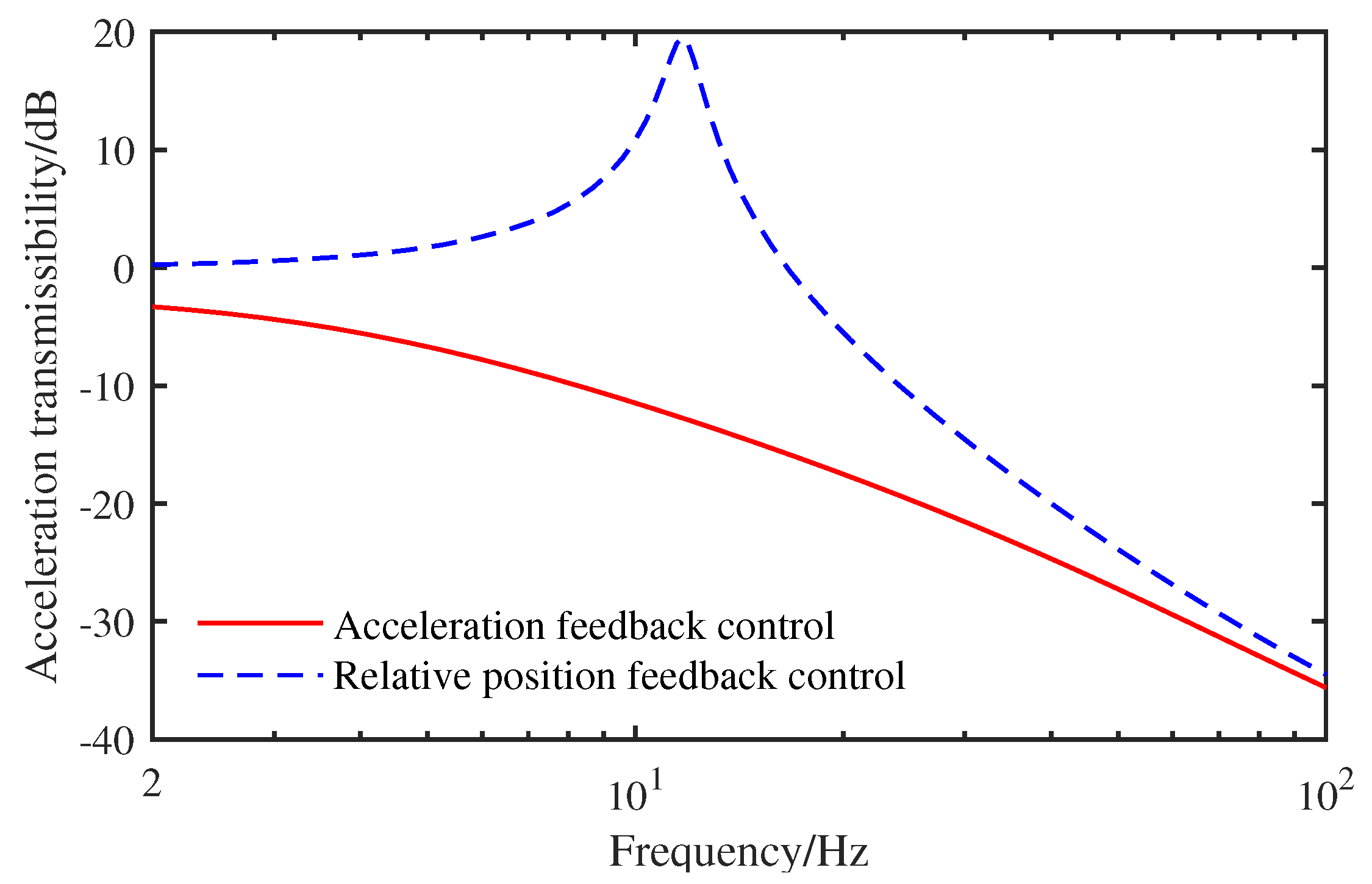In Figure 8 and Figure 9, the acceleration values of the isolated object and the base are shown for two control algorithms, with the base vibration signal frequency at 33.33 Hz. Compared to position control, the attenuation of acceleration from the base to the isolated object is greater and acceleration feedback control demonstrates a lower acceleration transmissibility. It can be observed that acceleration feedback control has better vibration isolation performance than position control.

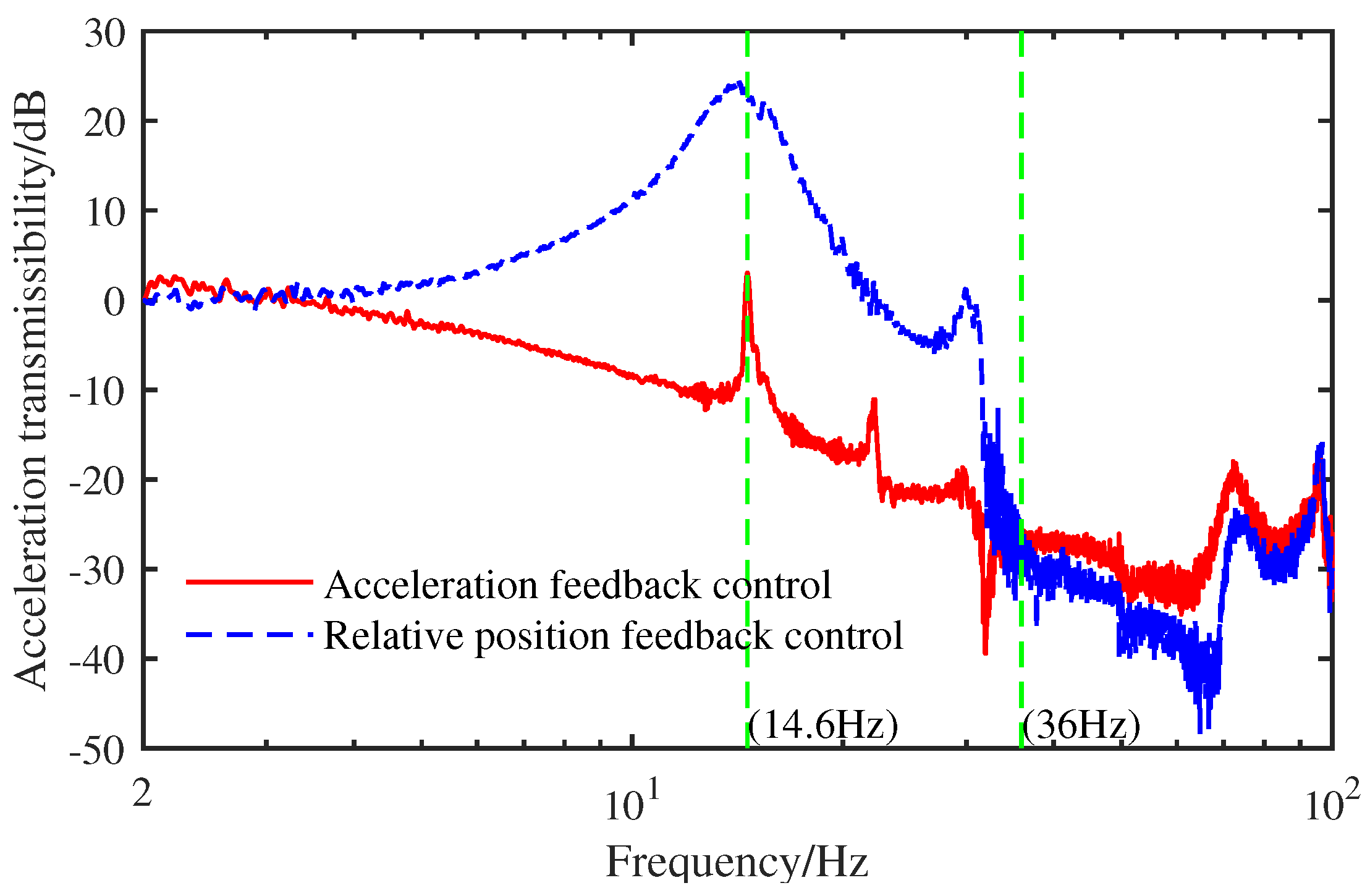
From Figure 10, an acceleration transmissibility comparison between the acceleration feedback control and the relative position feedback control is illustrated. The experimental data indicate that the vibration isolation performance in the low-frequency band is better for the acceleration feedback control compared to the relative position feedback control. At a frequency of 14.6 Hz, the relative position feedback control demonstrates a resonance peak, which corresponds to a transmissibility of 24.47 dB. Then, the acceleration feedback control system exhibits a resonance peak of 14.6 Hz, resulting in a transmissibility of 2.4 dB. The vibration isolation performance based on acceleration feedback is enhanced by 22.07 dB at the resonance frequency. Both curves exhibit additional peaks within the frequency range of 14.6 Hz to 36 Hz. In the high-frequency range of 36–100 Hz, the curves display similar trends, but the vibration isolation performance based on acceleration feedback is decreased. The experimental results confirm the effectiveness of the acceleration feedback method for vibration isolation in the low-frequency range. However, certain differences exist between the experimental and theoretical analysis performances as a result of limitations in model accuracy, system nonlinearity, and high sensor measurement noise.

5. Conclusions

In order to improve the vibration isolation performance of the AVIS with electromagnetic actuator, acceleration feedback control with TD is proposed. Theoretical analysis and experiment show that:

(i)

The proposed TD is aimed at improving the differential signal of the relative position sensor. A comparison between TD and a first-order inertial filter demonstrates that TD produces a superior differential signal;

(ii)

The results of the experiments indicate that the vibration isolation system with relative position feedback control experiences severe resonance in the low-frequency band. At the resonance frequency, the transmissibility can reach as high as 24.47 dB. However, the acceleration feedback control provides a better solution for vibration isolation in this frequency range. In fact, it improves the isolation performance by 22.07 dB near the resonance frequency compared to the position feedback control. Hence, the proposed acceleration feedback control approach is more effective in achieving superior vibration isolation performance.

The AVIS with electromagnetic actuator will be further studied by considering the changing frequency of vibrating source, designing the adaptive controller, and validating the theoretical results with a practical experimental platform.