Influence of Nonlinear Characteristics of Planetary Flywheel Inerter Actuator on Vehicle Active Suspension Performance
Abstract
1. Introduction
2. Structure of a Planetary Flywheel Inerter Actuator and Its Nonlinear Dynamic Model
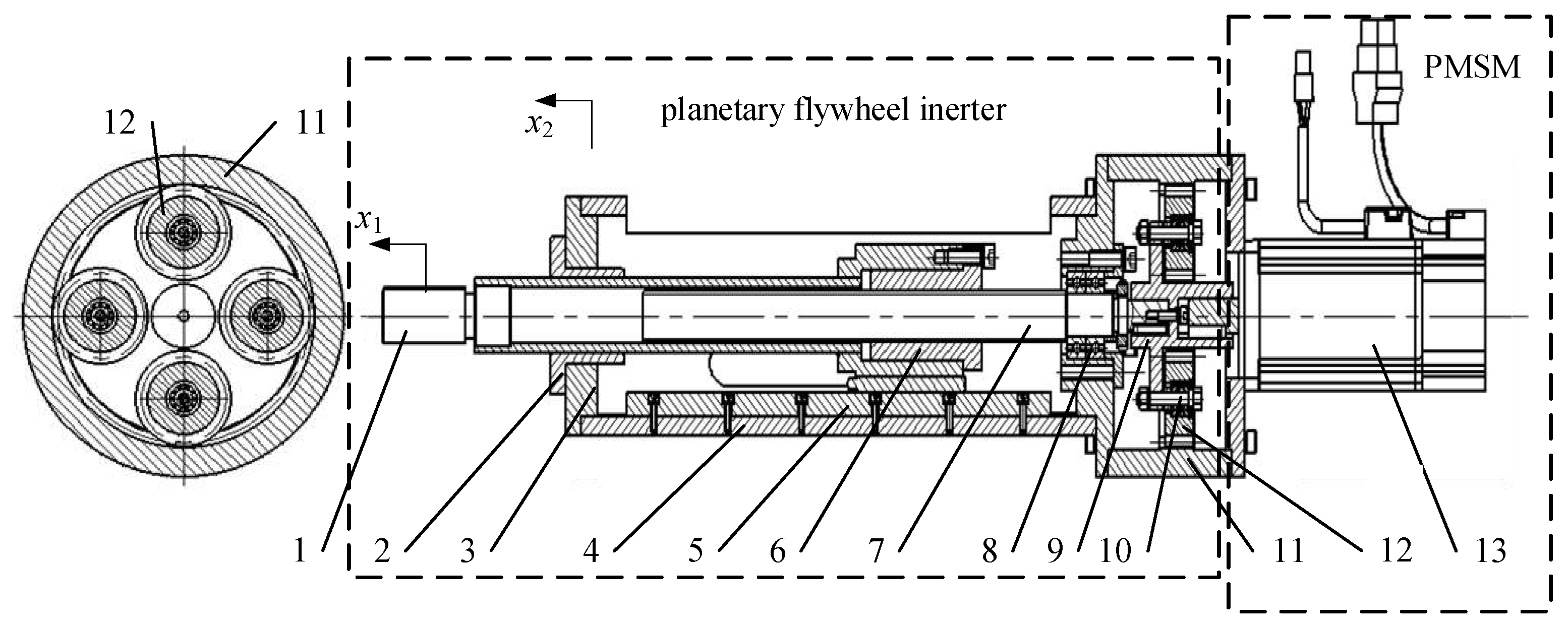
2.1. Structural Design and Fundamental Mechanical Model of Planetary Flywheel Inerter Actuator
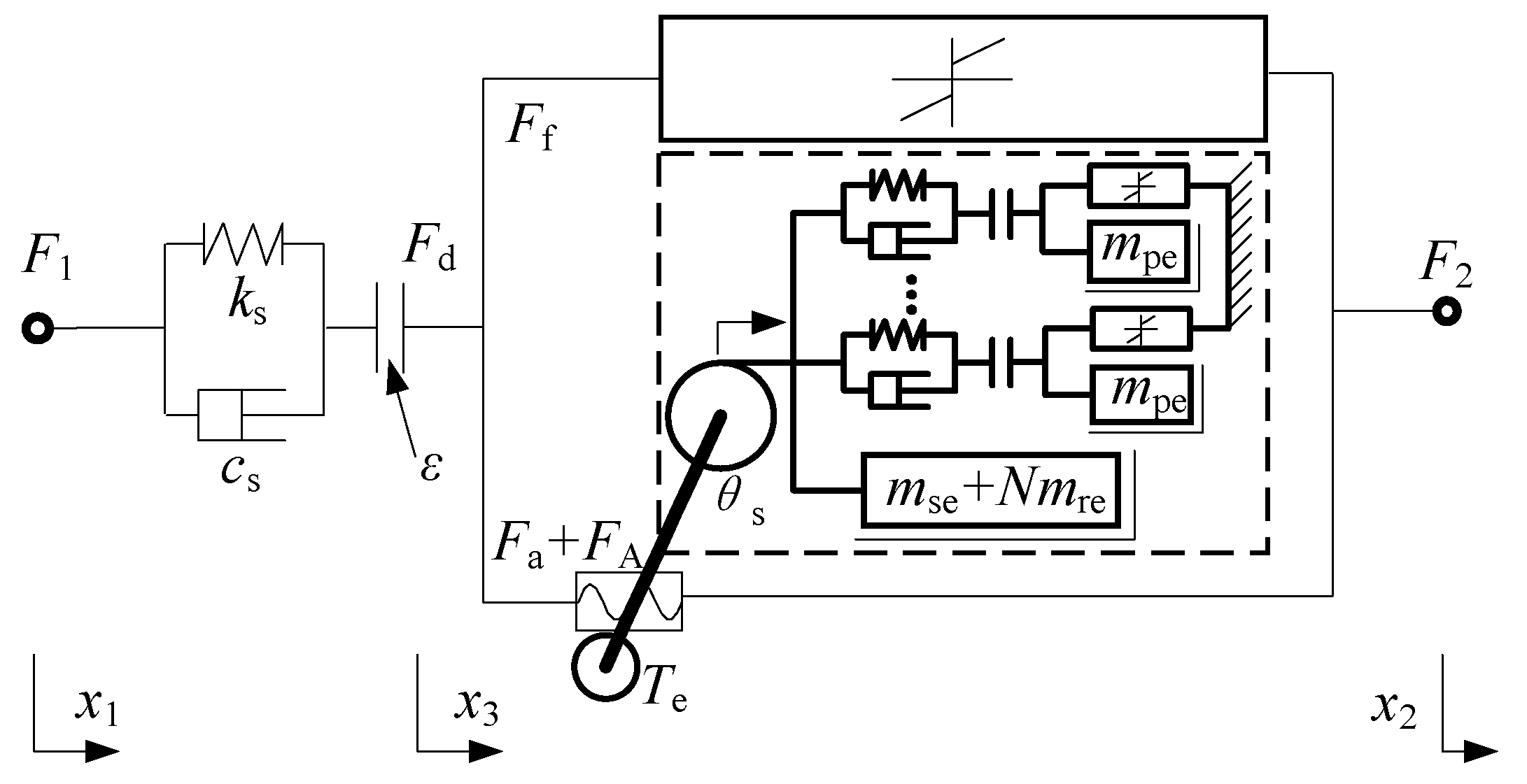
2.2. Mechanical Model of Passive Planetary Flywheel Inerter
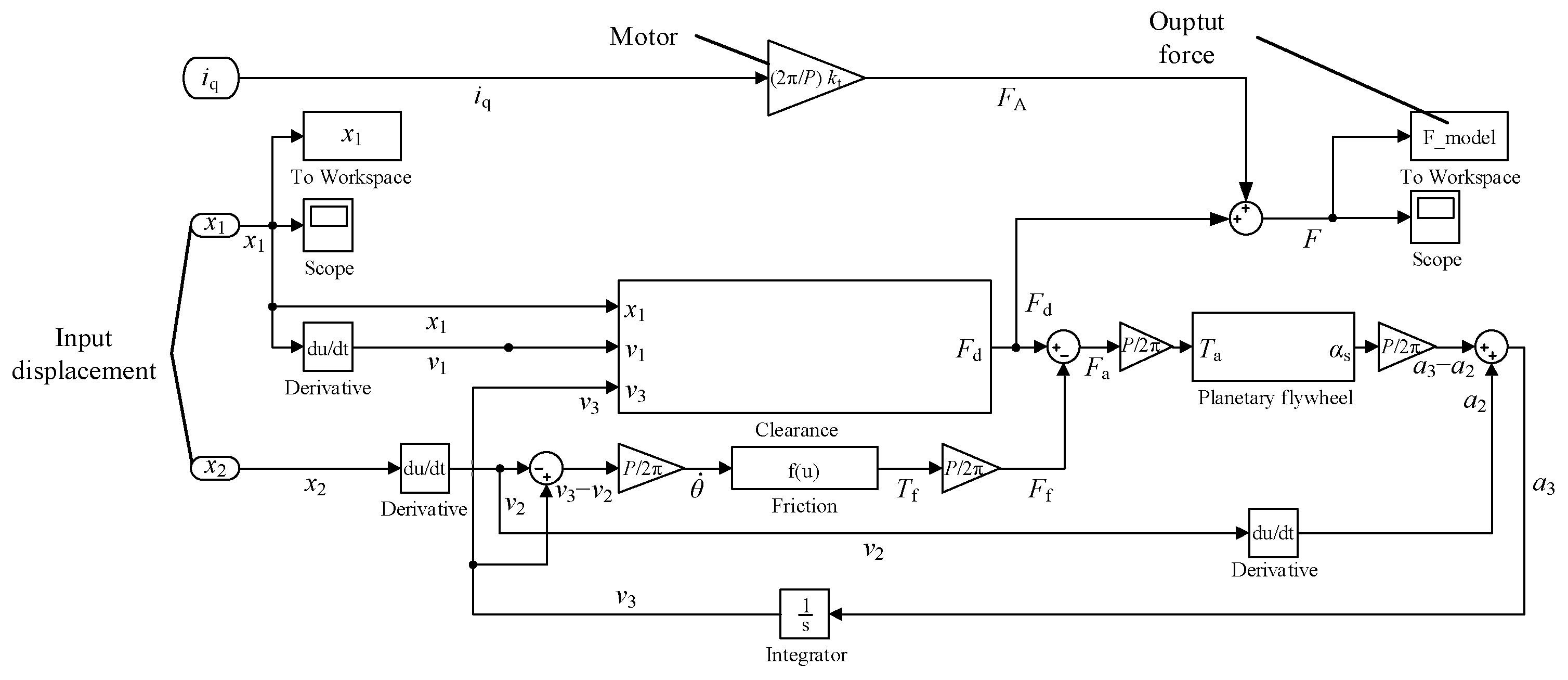
2.3. Dynamic Model of Planetary Flywheel Inerter Actuator
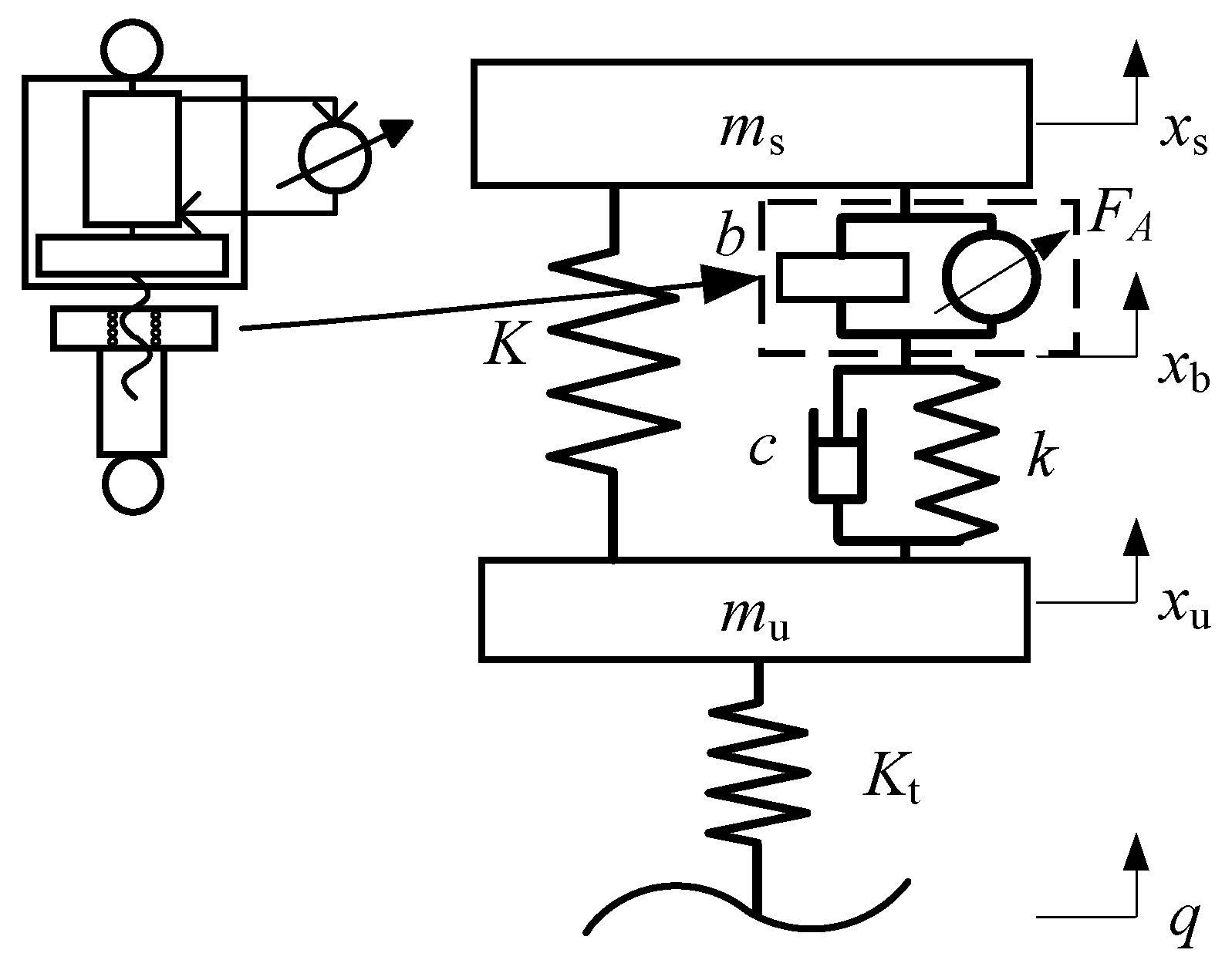
3. Structure and Performance Measure of Active Inerter Suspension
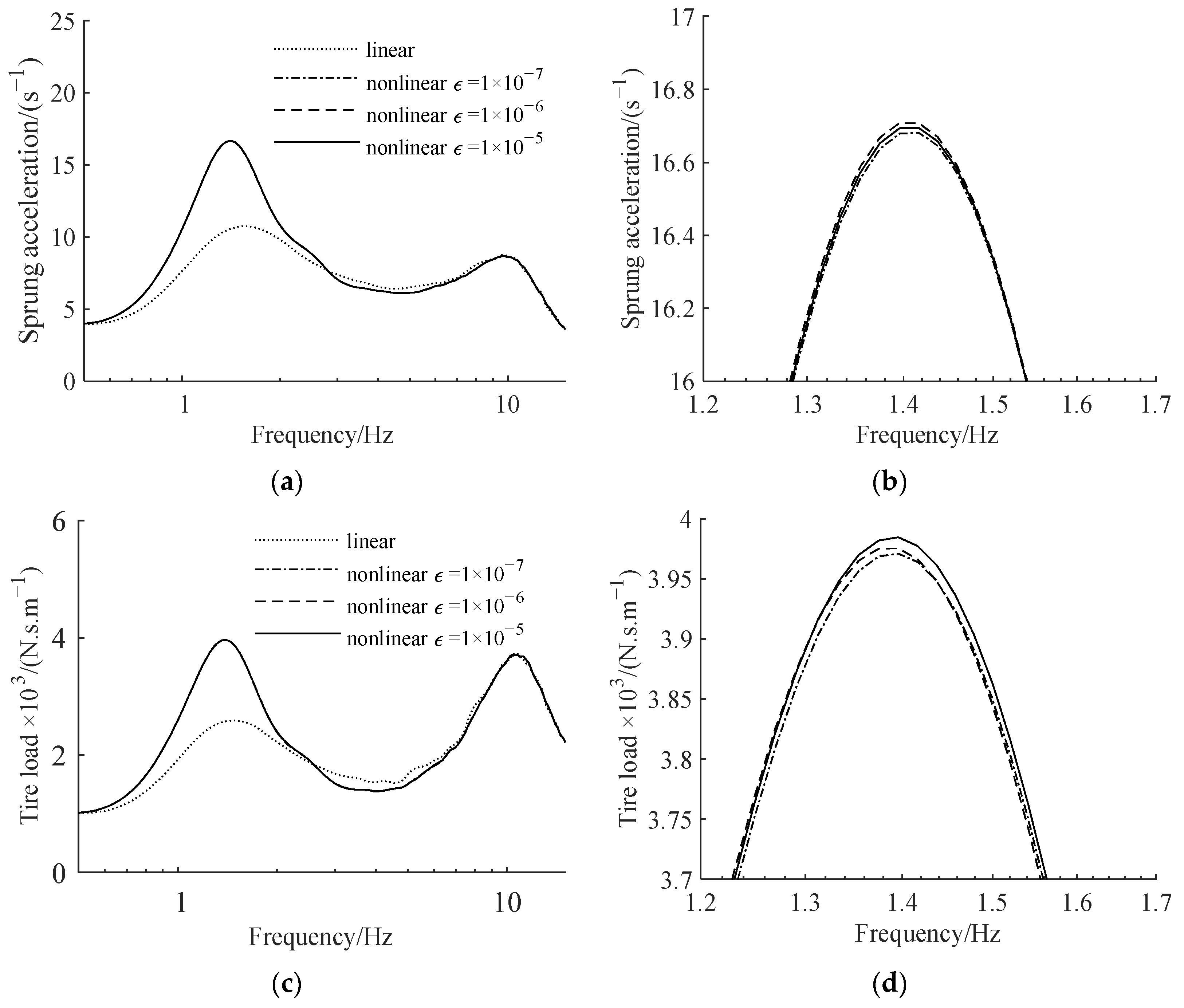
4. Comparison of Suspension Performance with Linear/Nonlinear Actuator Models
5. The Effect of Ball Screw Nonlinear Factors on the Performance of Active Inerter Suspension
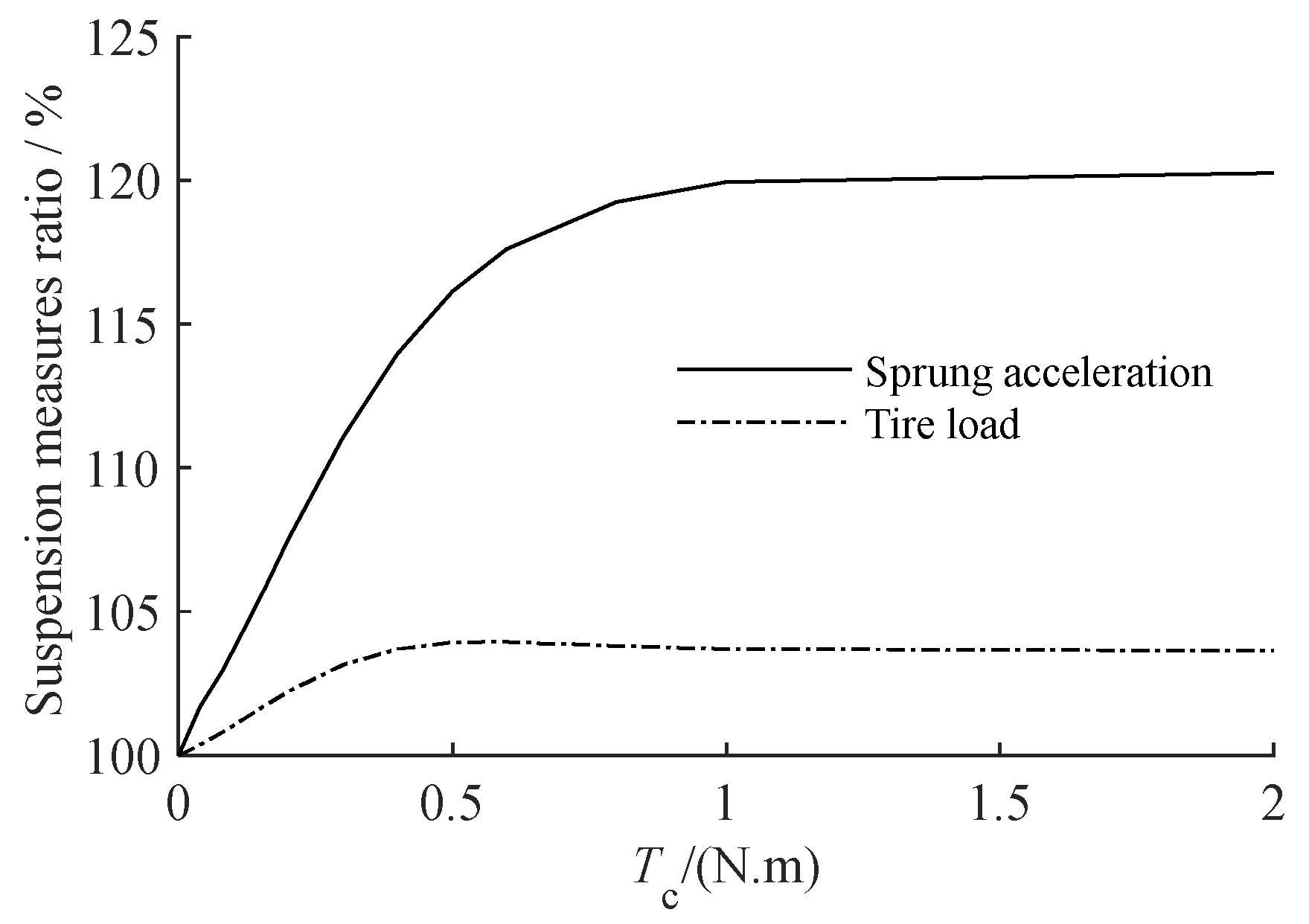
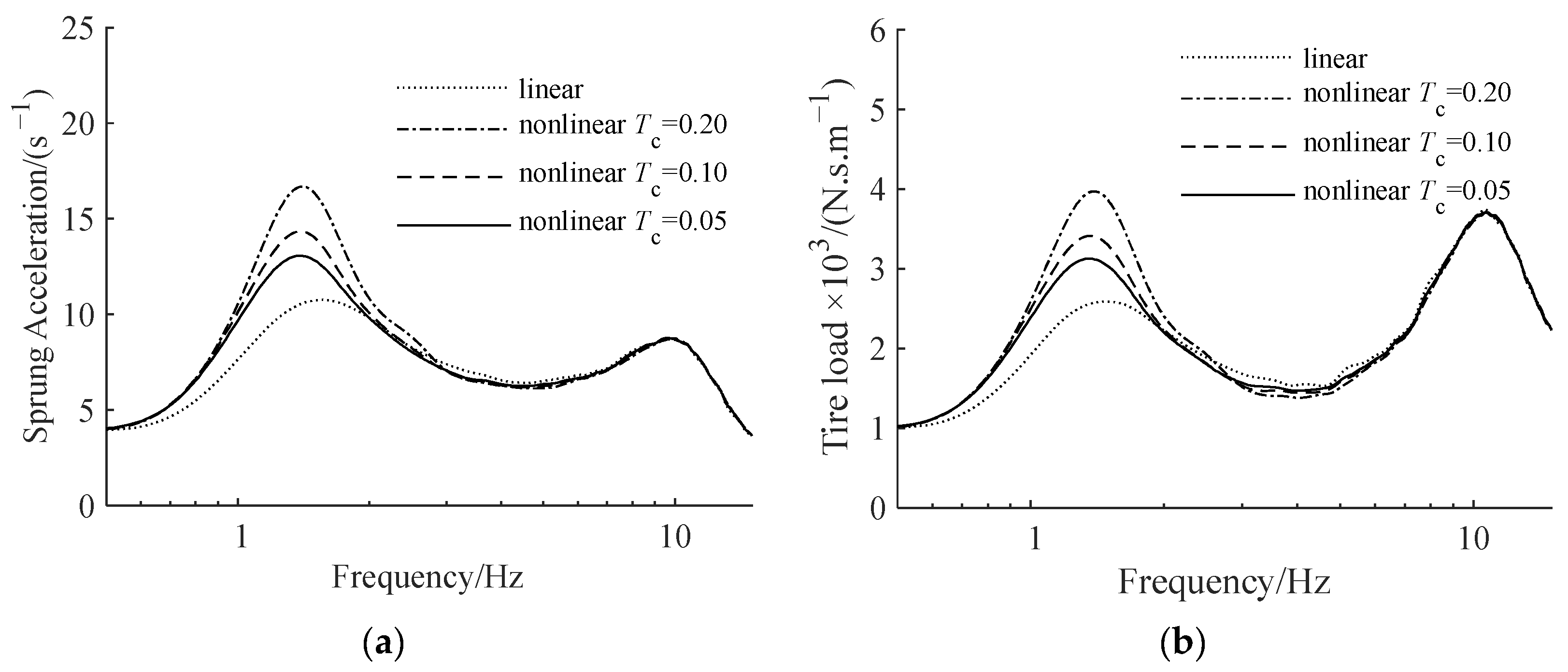
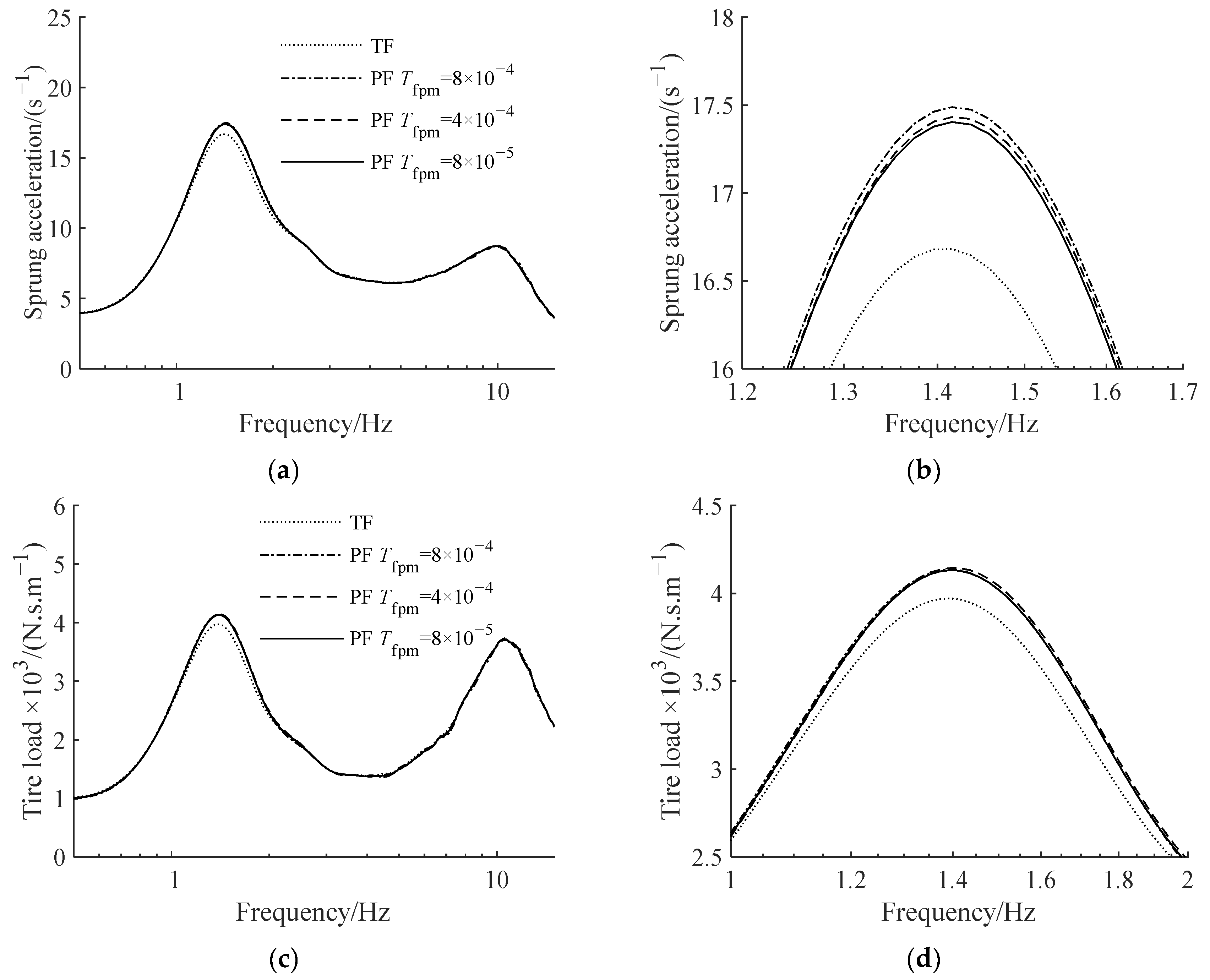
5.1. The Effect of Ball Screw Static Friction
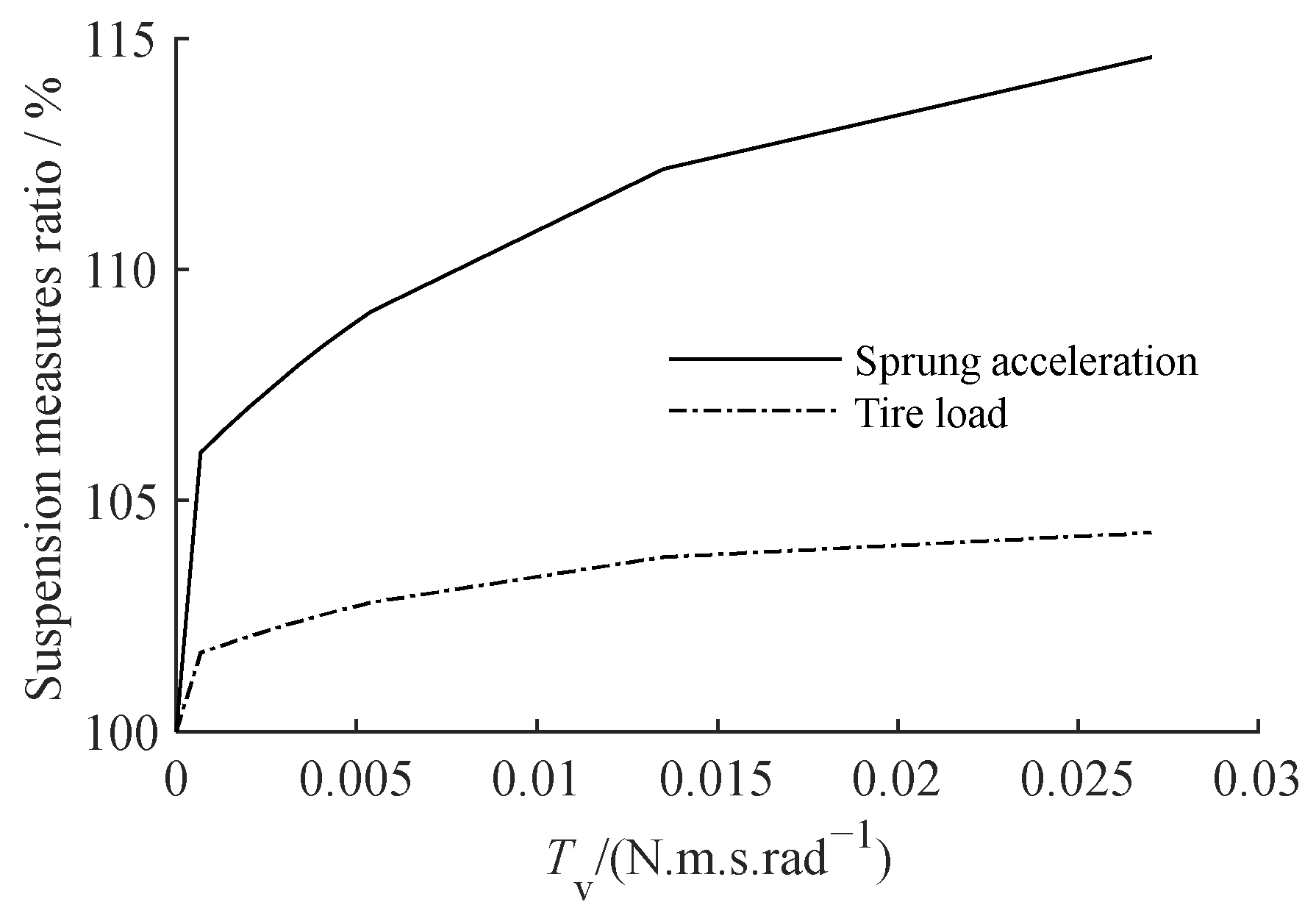
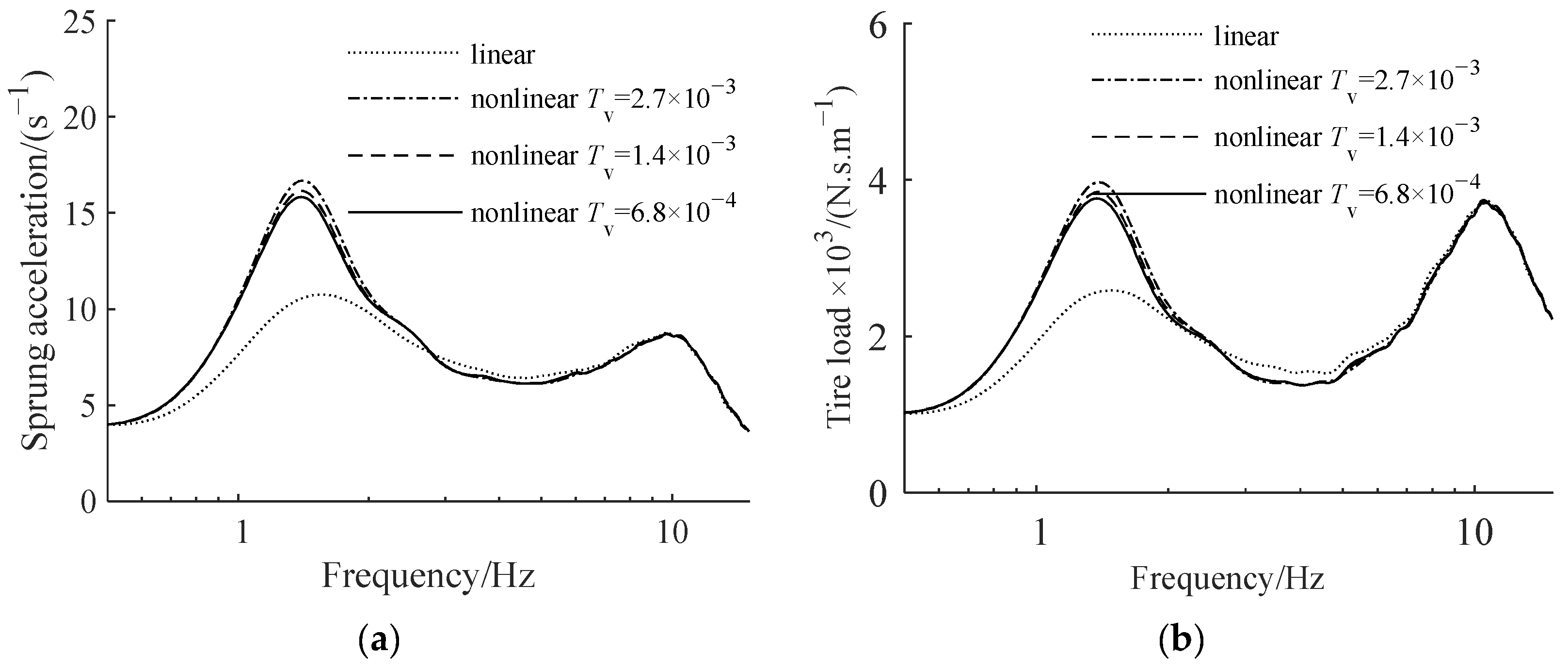
5.2. The Effect of Ball Screw Viscous Friction
5.3. The Effect of the Screw–Nut Pair Clearance
6. The Effect of Planetary Flywheel Nonlinear Factors on the Performance of Active Inerter Suspension
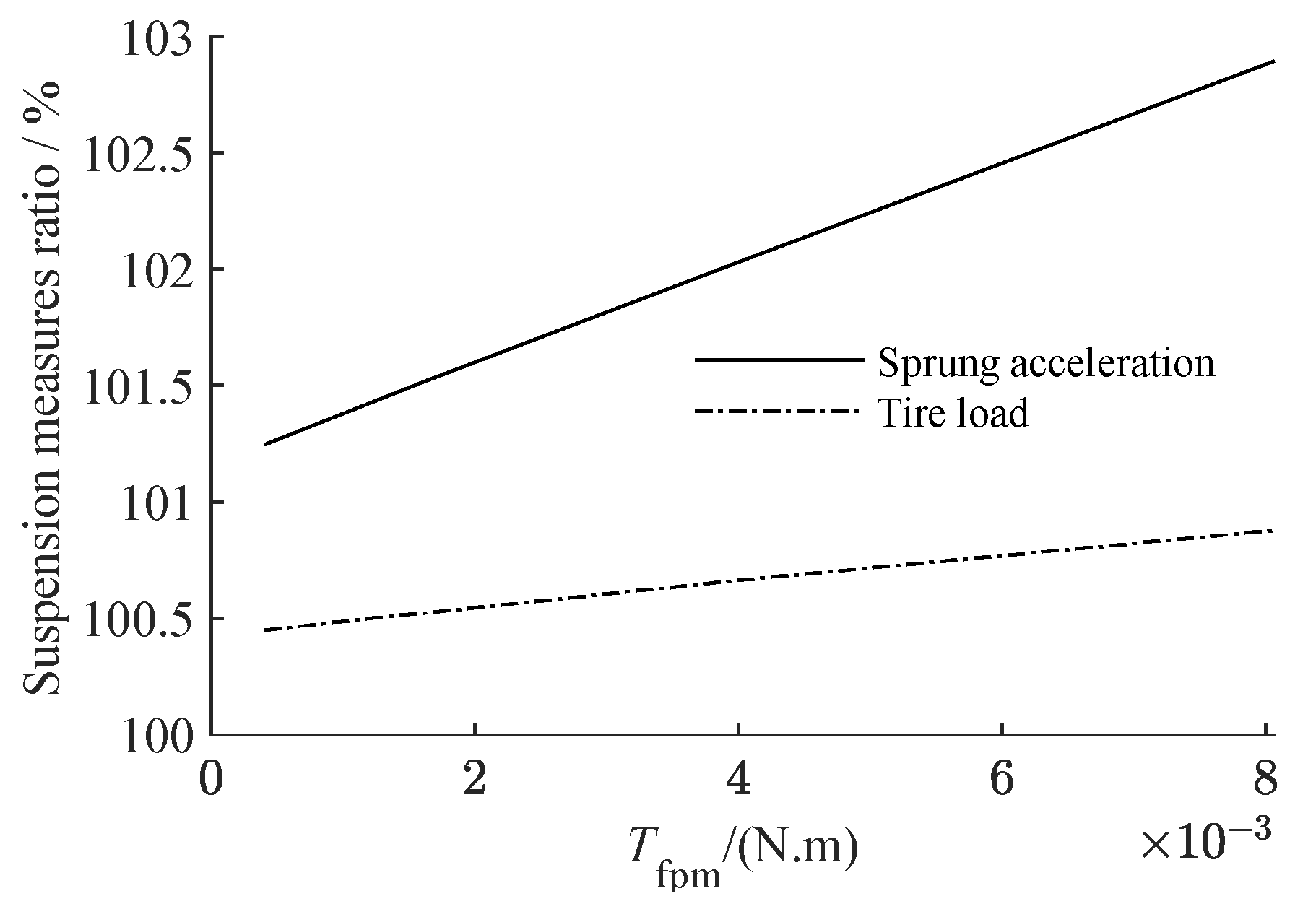
6.1. The Effect of Planetary Flywheel Static Friction
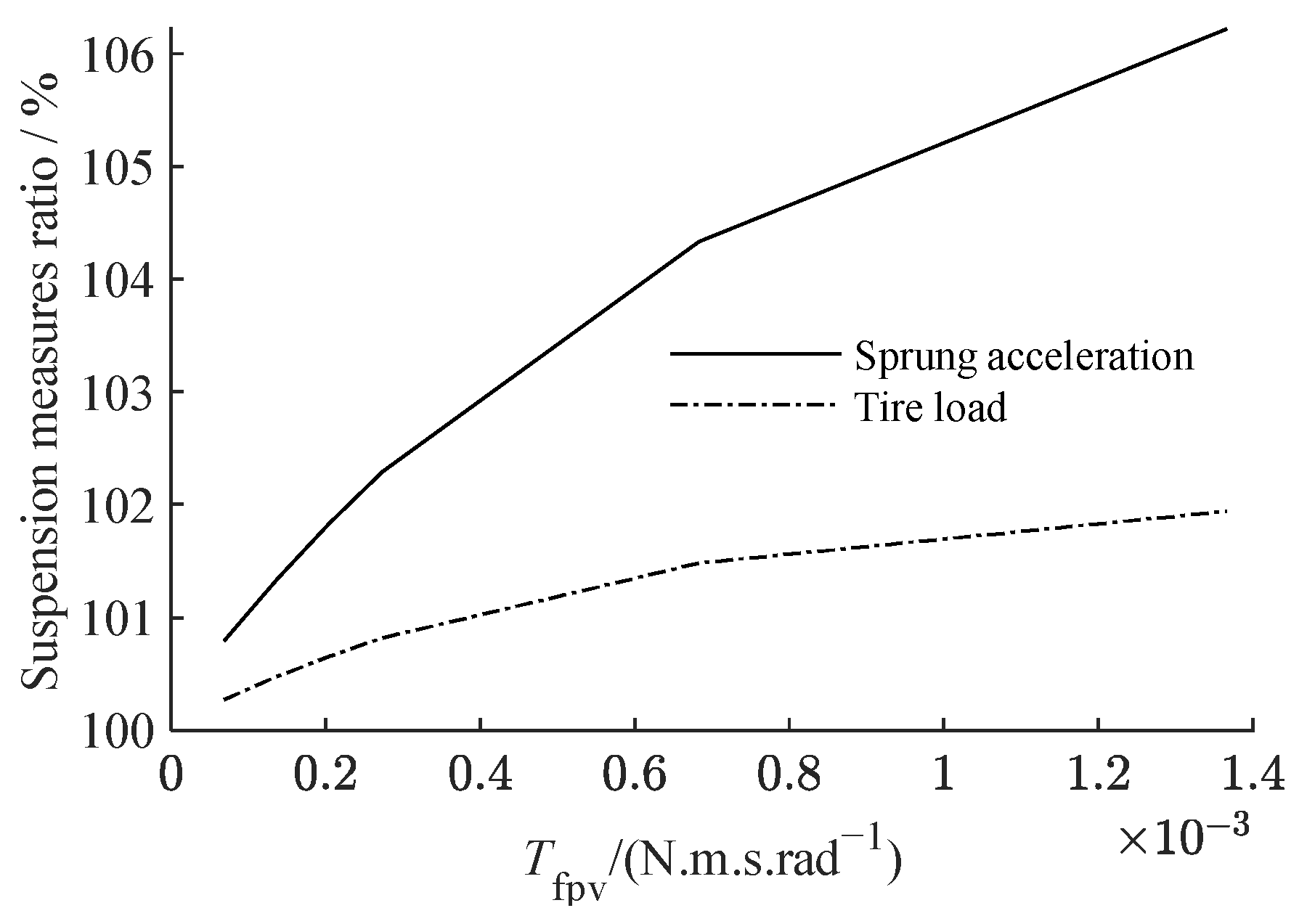
6.2. The Effect of Planetary Flywheel Viscous Friction
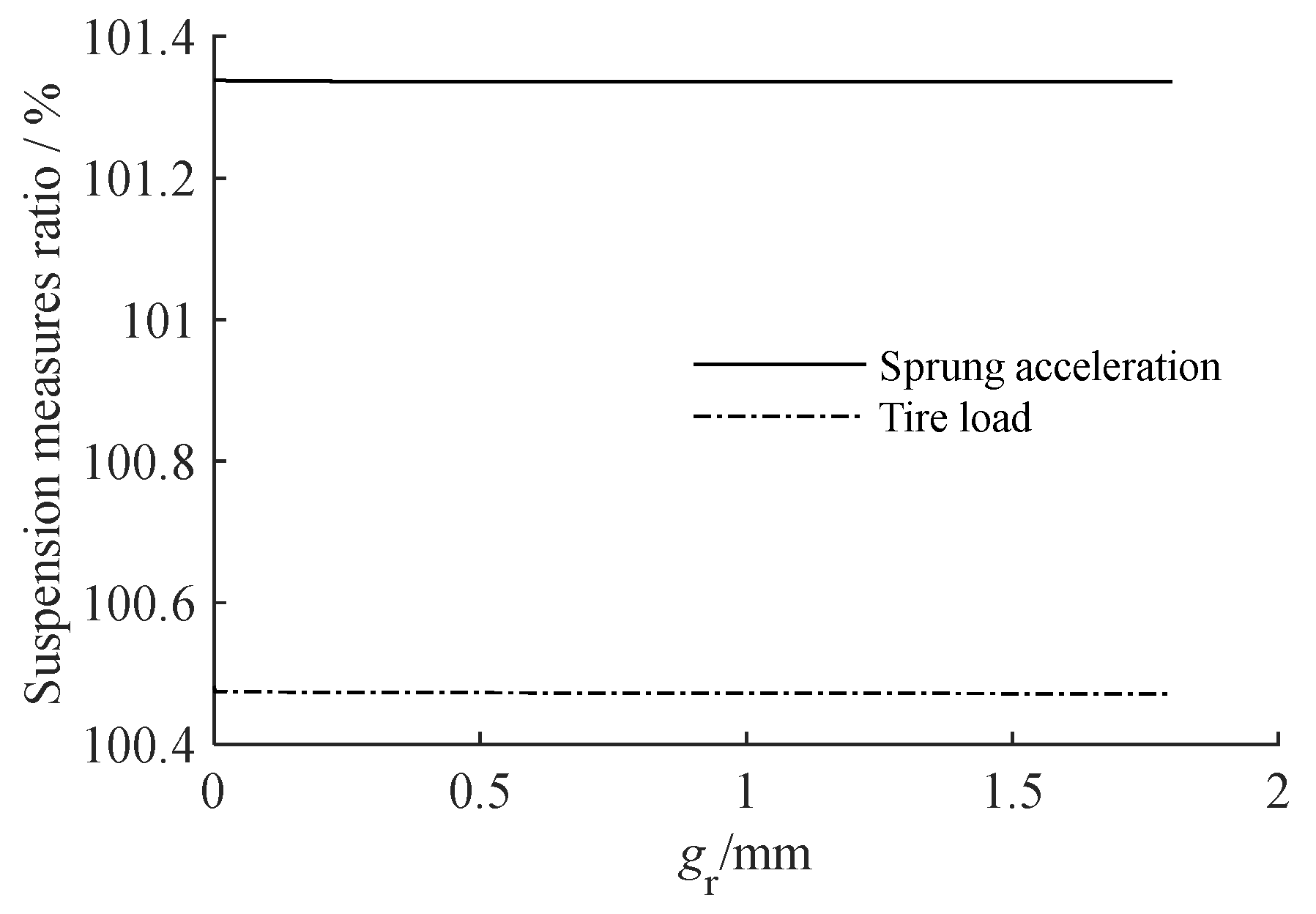
6.3. The Effect of Gear Backlash
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tseng, H.E.; Hrovat, D. State of the art survey: Active and semi-active suspension control. Veh. Syst. Dyn. 2015, 53, 1034–1062. [Google Scholar] [CrossRef]
- Smith, M.C. Synthesis of mechanical networks: The inerter. IEEE Trans. Automat. Contr. 2002, 47, 1648–1662. [Google Scholar] [CrossRef]
- Smith, M.C. The inerter: A retrospective. Annu. Rev. Control Rob. Auton. Syst. 2020, 3, 361–391. [Google Scholar] [CrossRef]
- Marian, L. The Tuned Mass Damper Inerter for Passive Vibration Control and Energy Harvesting in Dynamically Excited Structural Systems. Ph.D. Thesis, City University London, London, UK, 2015. [Google Scholar]
- Lazar, I.F.; Neild, S.A.; Wagg, D.J. Using an inerter-based device for structural vibration suppression. Earthq. Eng. Struct. Dyn. 2014, 43, 1129–1147. [Google Scholar] [CrossRef]
- Wang, H.; Shen, W.; Zhu, H.; Luo, H. Stochastic optimization of a nonlinear base isolation system with LRB and EIMD for building structures. Struct. Control Health Monit. 2023, 2023, 8392421. [Google Scholar] [CrossRef]
- Lazar, I.F.; Neild, S.A.; Wagg, D.J. Performance Analysis of Cables with Attached Tuned-Inerter-Dampers. In Proceedings of the 33rd IMAC, A Conference and Exposition on Structural Dynamics, Orlando, FL, USA, 2–5 February 2015. [Google Scholar]
- Luo, J.; Jiang, J.Z.; Macdonald, J.H.G. Cable vibration suppression with inerter-based absorbers. J. Eng. Mech. 2019, 145, 04018134. [Google Scholar] [CrossRef]
- Hanazawa, Y.; Suda, H.; Yamakita, M. Analysis and Experiment of Flat-Footed Passive Dynamic Walker with Ankle Inerter. In Proceedings of the 2011 IEEE International Conference on Robotics and Biomimetics, Karon Beach, Thailand, 7–11 December 2011. [Google Scholar]
- Xu, S.; He, B. A compliance modeling method of flexible rotary joint for collaborative robot using passive network synthesis theory. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2022, 236, 4038–4048. [Google Scholar] [CrossRef]
- Smith, M.C.; Wang, F.C. Performance benefits in passive vehicle suspensions employing inerters. Veh. Syst. Dyn. 2004, 42, 235–257. [Google Scholar] [CrossRef]
- Tran, T.T.; Hori, C.; Hasegawa, H. Integrated inerter design and application to optimal vehicle suspension system. Int. J. Comput.-Aided Technol. 2014, 1, 1–16. [Google Scholar]
- Hu, Y.; Chen, M.Z.Q.; Sun, Y. Comfort-oriented vehicle suspension design with skyhook inerter configuration. J. Sound Vib. 2017, 405, 34–47. [Google Scholar] [CrossRef]
- Shen, Y.; Hua, J.; Fan, W.; Liu, Y.; Yang, X.; Chen, L. Optimal design and dynamic performance analysis of a fractional-order electrical network-based vehicle mechatronic ISD suspension. Mech. Syst. Signal Process. 2023, 184, 109718. [Google Scholar] [CrossRef]
- Li, Y.; Jiang, J.Z.; Neild, S. Inerter-based configurations for main-landing-gear shimmy suppression. J. Aircr. 2017, 54, 684–693. [Google Scholar] [CrossRef]
- Stachiw, T.; Khouli, F.; Langlois, R.G.; Afagh, F.F. Landing gear mechanical network synthesis for improving comfort at landing considering aircraft flexibility. J. Aircr. 2021, 58, 1242–1253. [Google Scholar] [CrossRef]
- Chillemi, M.; Furtmüller, T.; Adam, C.; Pirrotta, A. Nonlinear mechanical model of a fluid inerter. Mech. Syst. Signal Process. 2023, 188, 109986. [Google Scholar] [CrossRef]
- Papageorgiou, C.; Smith, M.C. Laboratory Experimental Testing of Inerters. In Proceedings of the 44th IEEE Conference on Decision and Control, Seville, Spain, 15 December 2005. [Google Scholar]
- Papageorgiou, C.; Houghton, N.E.; Smith, M.C. Experimental testing and analysis of inerter devices. J. Dyn. Syst. Meas. Contr. 2009, 131, 011001. [Google Scholar] [CrossRef]
- Chen, P.C.; Chen, P.C.; Ting, G.C. Seismic response mitigation of buildings with an active inerter damper system. Struct. Control Health Monit. 2022, 29, e2975. [Google Scholar] [CrossRef]
- Madhamshetty, K.; Manimala, J.M. Low-rate characterization of a mechanical inerter. Machines 2018, 6, 32. [Google Scholar] [CrossRef]
- Wang, F.C.; Chan, H.A. Vehicle suspensions with a mechatronic network strut. Veh. Syst. Dyn. 2011, 49, 811–830. [Google Scholar] [CrossRef]
- Ge, Z.; Wang, W. Modeling, testing, and characteristic analysis of a planetary flywheel inerter. Shock Vib. 2018, 2018, 2631539. [Google Scholar] [CrossRef]
- Dai, J.; Xu, Z.; Gai, P. Tuned mass damper for self-excited vibration control: Optimization involving nonlinear aeroelastic effect. Eng. Struct. 2019, 199, 109585. [Google Scholar] [CrossRef]
- Bai, X.; Liang, Q.; Huo, L. Vibration control of beam-model using tuned inerter enhanced TMD. J. Sound Vib. 2021, 510, 116304. [Google Scholar] [CrossRef]
- Su, N.; Xia, Y.; Peng, S. Filter-based inerter location dependence analysis approach of Tuned mass damper inerter (TMDI) and optimal design. Eng. Struct. 2022, 250, 113459. [Google Scholar] [CrossRef]
- Dogan, H.; Sims, N.D.; Wagg, D.J. Design, testing and analysis of a pivoted-bar inerter device used as a vibration absorber. Mech. Syst. Signal Process. 2022, 171, 108893. [Google Scholar] [CrossRef]
- Zhang, M.; Xu, F. Tuned mass-damper-inerter control of wind-induced vibration of flexible structures based on inerter location. J. Wind. Eng. Ind. Aerod. 2022, 220, 104836. [Google Scholar] [CrossRef]
- Ge, Z.; Wang, W.; Li, G.; Rao, D. Design, parameter optimisation, and performance analysis of active tuned inerter damper (TID) suspension for vehicle. J. Sound Vib. 2022, 525, 116750. [Google Scholar] [CrossRef]
- Wagg, D.J. A review of the mechanical inerter: Historical context, physical realisations and nonlinear applications. Nonlinear Dyn. 2021, 104, 13–34. [Google Scholar] [CrossRef]
- Brzeski, P.; Perlikowski, P. Effects of play and inerter nonlinearities on the performance of tuned mass damper. Nonlinear Dyn. 2017, 88, 1027–1041. [Google Scholar] [CrossRef]
- Hu, Y.; Hua, T.; Chen, M.Z.Q.; Shi, S.; Sun, Y. Instability analysis for semi-active control systems with semi-active inerters. Nonlinear Dyn. 2021, 105, 99–112. [Google Scholar] [CrossRef]
- Soong, M.F.; Ramli, R.; Mahadi, W.N.L. Ride evaluation of vehicle suspension employing non-linear inerter. Appl. Mech. Mater. 2014, 471, 9–13. [Google Scholar] [CrossRef]
- Gonzalez-Buelga, A.; Lazar, I.F.; Jiang, J.Z.; Neild, S.A.; Inman, D.J. Assessing the effect of nonlinearities on the performance of a tuned inerter damper. Struct. Control Health Monit. 2017, 24, e1879. [Google Scholar] [CrossRef]
- Li, Z.; Xu, K.; Bi, K.; Han, Q.; Du, X. Inerter nonlinearity and its influence on control efficiency of TMDI for suppressing vortex-induced vibration of bridges. J. Bridge Eng. 2022, 27, 04022101. [Google Scholar] [CrossRef]
- Sun, X.Q.; Chen, L.; Wang, S.H.; Zhang, X.L.; Yang, X.F. Performance investigation of vehicle suspension system with nonlinear ball-screw inerter. Int. J. Automot. Technol. 2016, 17, 399–408. [Google Scholar] [CrossRef]
- Shen, Y.; Chen, L.; Liu, Y.; Zhang, X. Influence of fluid inerter nonlinearities on vehicle suspension performance. Adv. Mech. Eng. 2017, 9, 1–10. [Google Scholar] [CrossRef]
- Wang, F.C.; Su, W.J. Impact of inerter nonlinearities on vehicle suspension control. Veh. Syst. Dyn. 2008, 46, 575–595. [Google Scholar] [CrossRef]
- Liu, C.; Chen, L.; Zhang, X.; Yang, Y.; Nie, J. Design and tests of a controllable inerter with fluid-air mixture condition. IEEE Access 2020, 8, 125620–125629. [Google Scholar] [CrossRef]






| Parameter | Parameter Value |
|---|---|
| Sprung mass ms/kg | 229 |
| Unsprung mass mu/kg | 31 |
| Main spring stiffness K/(N‧m−1) | 20,213 |
| Tire stiffness Kt/(N‧m−1) | 127,976 |
| Tuned spring stiffness k/(N‧m−1) | 3304 |
| Tuned damping coefficient c/(N‧s‧m−1) | 747 |
| Inerter inertance b/kg | 385 |
| Compensation coefficient r/kg | 300 |
| Parameter | Parameter Value |
|---|---|
| Motor model | ECMA-C10604 |
| Rated power/W | 400 |
| Rated torque/(N‧m) | 1.27 |
| Rated speed/(r‧min−1) | 3000 |
| Rotor inertia/(kg‧m2) | 2.77 × 10−5 |
| Lead of screw P/mm | 10 |
| Rotation inertia of screw Js1/(kg‧m2) | 2.80 × 10−5 |
| Gear module | 2 |
| Gear pressure angle | 20° |
| Tooth number of external gear ring Z2 | 50 |
| Number of planetary gears N | 4 |
| Tooth number of planetary gear Z1 | 17 |
| Equivalent mass of planetary gear rotation mpe/kg | 7.52 × 10−3 |
| Equivalent mass of planetary gear revolution mre/kg | 7.22 × 10−2 |
| Equivalent mass of planetary flywheel carrier rotation mse/kg | 2.05 × 10−1 |
| Static friction torque coefficient of the screw–nut pair Tc/(N‧m) | 2.231 × 10−1 |
| Viscous friction torque coefficient of the screw–nut pair Tv/(N‧m‧s‧rad−1) | 2.703 × 10−3 |
| Total clearance between screw–nut pair and bearing ε/m | 1.006 × 10−7 |
| Gear backlash gr/m | 1.838 × 10−6 |
| Static friction torque coefficient of the gear Tfpm/(N‧m) | 8.067 × 10−4 |
| Viscous friction coefficient of the gear Tfpv/(N‧m‧s‧rad−1) | 1.366 × 10−4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ge, Z.; Li, G.; Chen, S.; Wang, W. Influence of Nonlinear Characteristics of Planetary Flywheel Inerter Actuator on Vehicle Active Suspension Performance. Actuators 2023, 12, 252. https://doi.org/10.3390/act12060252
Ge Z, Li G, Chen S, Wang W. Influence of Nonlinear Characteristics of Planetary Flywheel Inerter Actuator on Vehicle Active Suspension Performance. Actuators. 2023; 12(6):252. https://doi.org/10.3390/act12060252
Chicago/Turabian StyleGe, Zheng, Guangping Li, Shixiang Chen, and Weirui Wang. 2023. "Influence of Nonlinear Characteristics of Planetary Flywheel Inerter Actuator on Vehicle Active Suspension Performance" Actuators 12, no. 6: 252. https://doi.org/10.3390/act12060252
APA StyleGe, Z., Li, G., Chen, S., & Wang, W. (2023). Influence of Nonlinear Characteristics of Planetary Flywheel Inerter Actuator on Vehicle Active Suspension Performance. Actuators, 12(6), 252. https://doi.org/10.3390/act12060252






