Finite-Time Distributed Control of Non-Triangular Stochastic Nonlinear Multi-Agent Systems with Input Constraints
Abstract
1. Introduction
- (1)
- In comparison with command-filter-based finite-time distributed control algorithms for SNMASs [26,27], an improved finite-time distributed consensus control scheme is proposed for SNMASs with input constraints, which effectively attenuates the chattering phenomenon, and the consensus tracking errors converge to a sufficiently small neighborhood of the origin in a finite time.
- (2)
- Unlike the existing backstepping [16,17,18] and dynamic surface [19,20] distributed control strategies for non-triangular SNMASs, a finite-time command filter and fractional power error compensation mechanism are constructed to eliminate the problem of the “explosion of complexity” and remove the effect of filtered error in a finite time.
- (3)
2. Preliminaries and Problem Formulation
2.1. Graph Theory
2.2. Stochastic Theory
2.3. Problem Formulation
3. Main Results
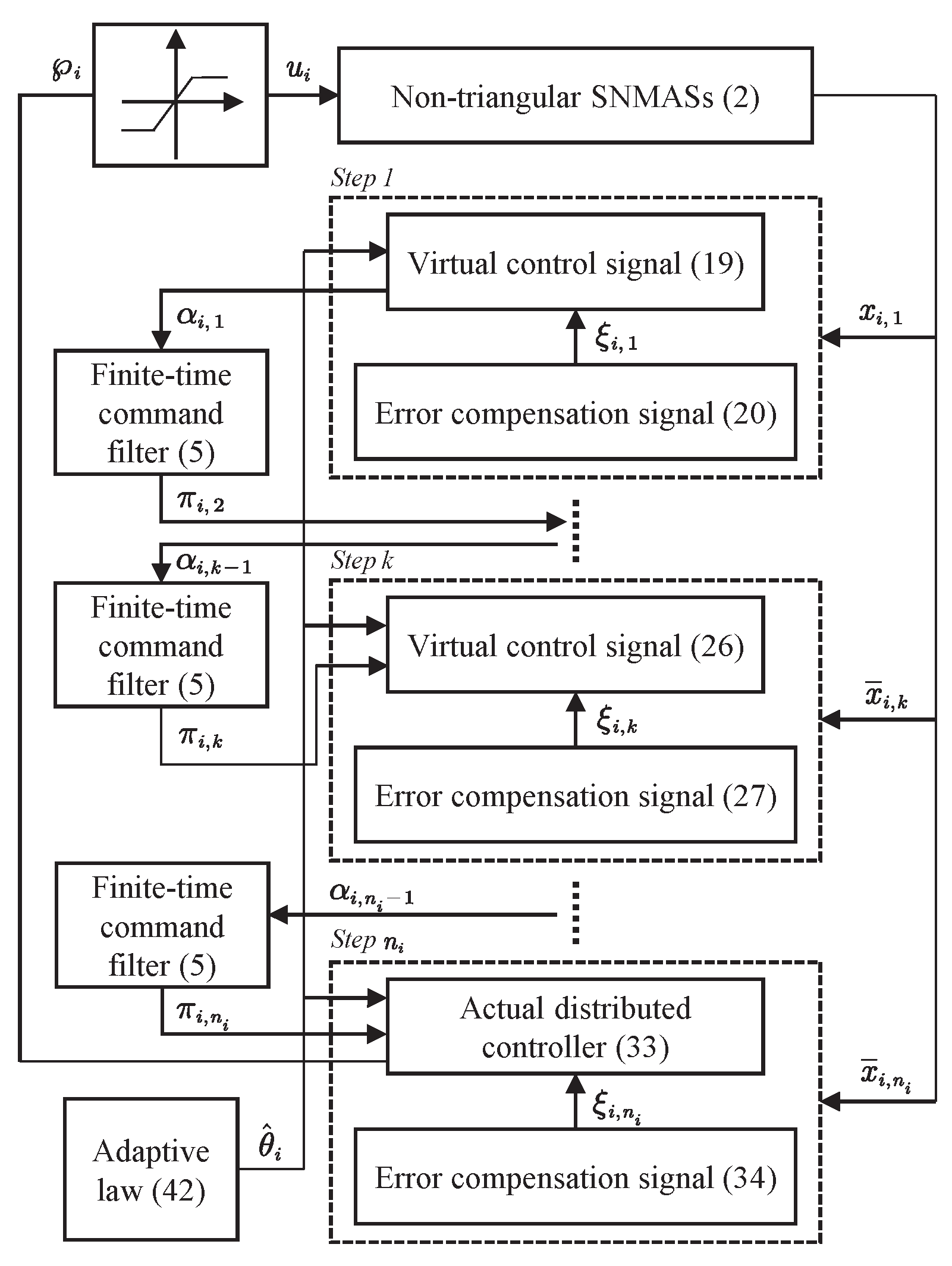
3.1. Finite-Time Distributed Controller Design
3.2. Stability Analysis
- All signals of the closed-loop system are semi-globally finite-time bounded in probability.
- The consensus tracking errors for the followers and the leader converge to a sufficiently small neighborhood of the origin in a finite time.
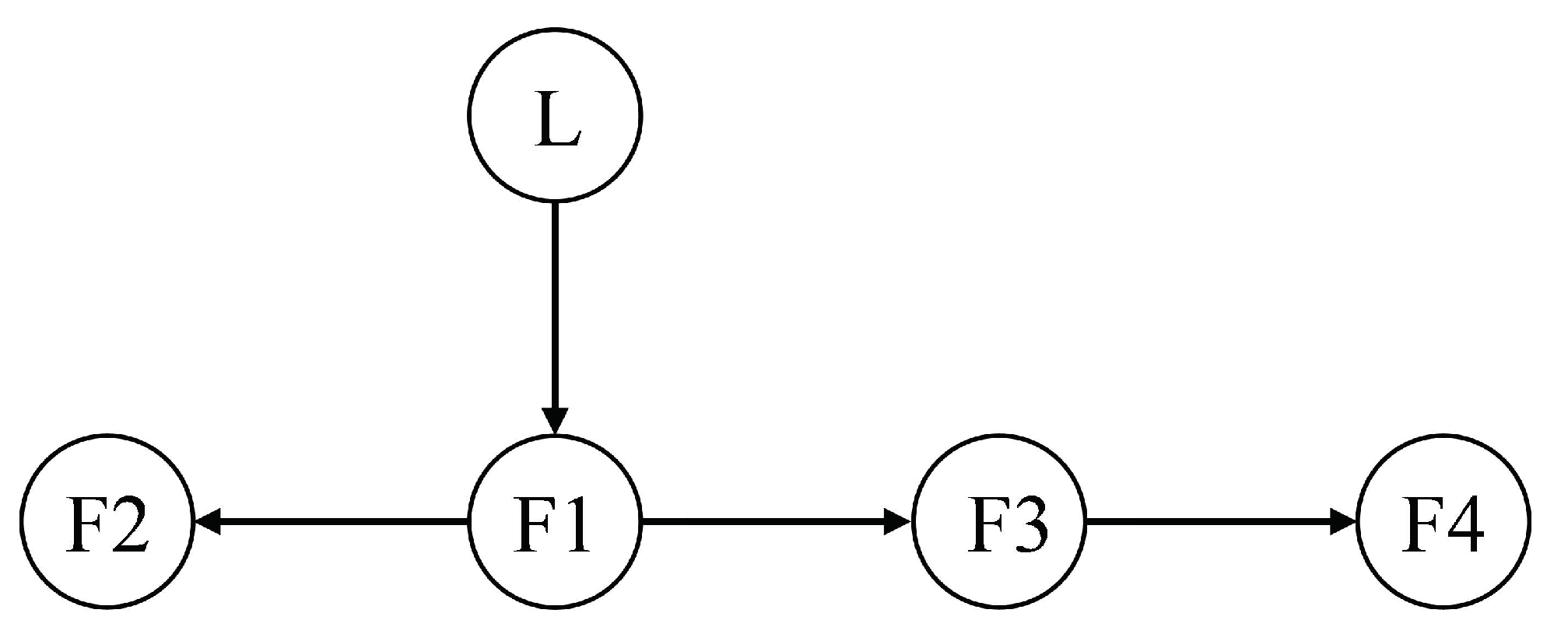
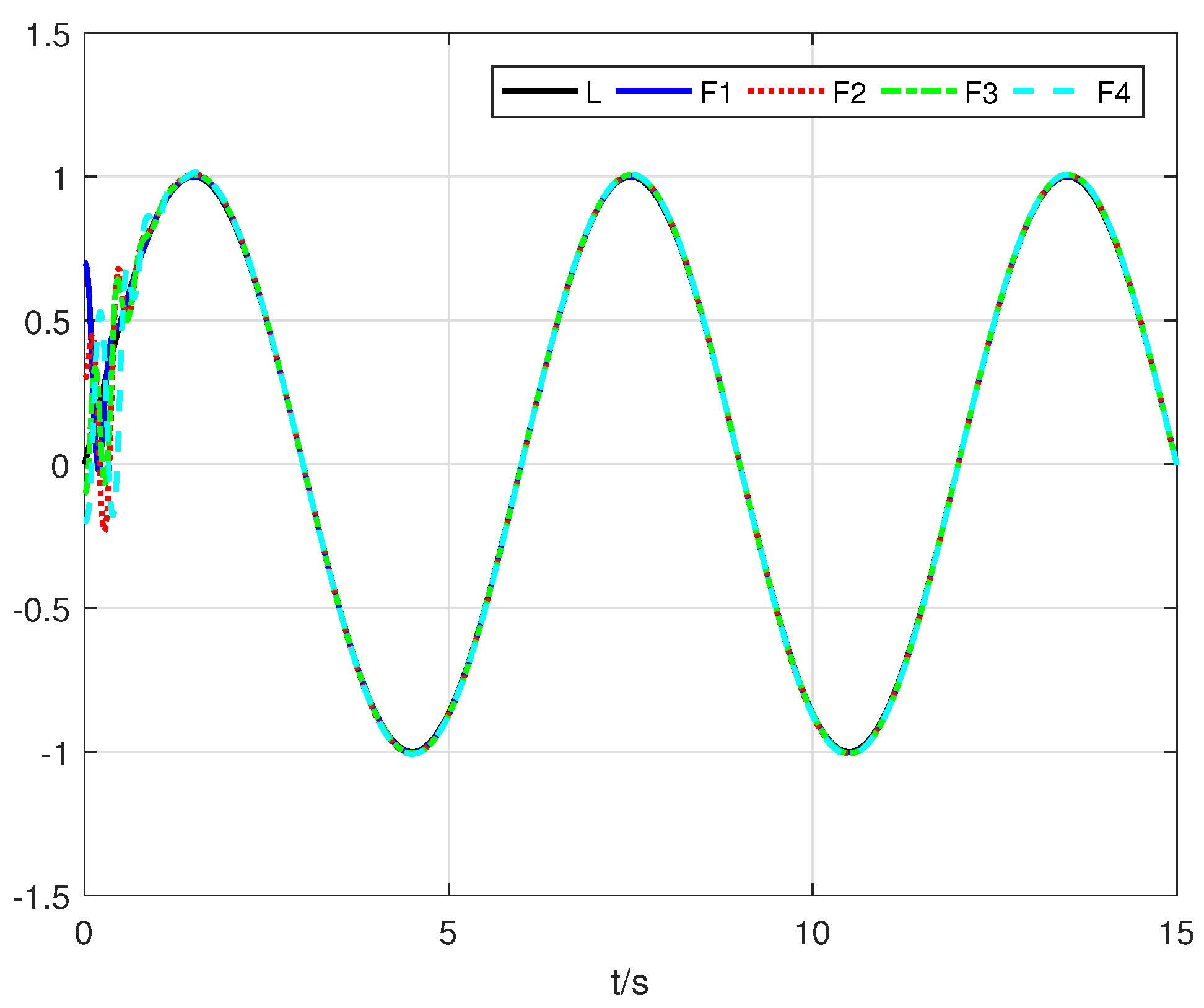
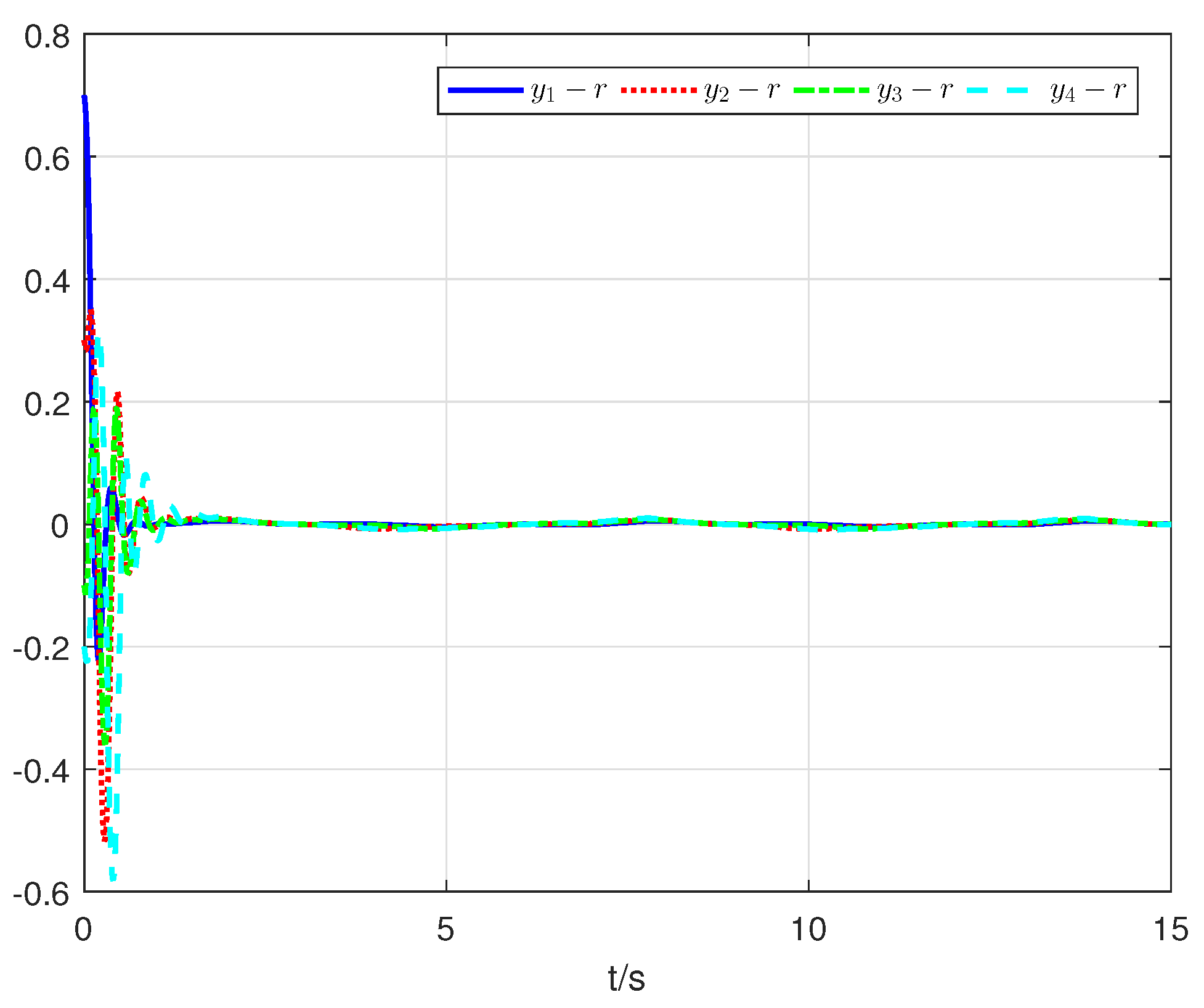
4. Simulation Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fax, J.A.; Murray, R.M. Information flow and cooperative control of vehicle formations. IEEE Trans. Autom. Control 2004, 49, 1465–1476. [Google Scholar] [CrossRef]
- Ren, W.; Beard, R.W. Distributed Consensus in Multi-Vehicle Cooperative Control; Springer: London, UK, 2008. [Google Scholar]
- Qin, J.; Ma, Q.; Shi, Y.; Wang, L. Recent advances in consensus of multi-agent systems: A brief survey. IEEE Trans. Ind. Electron. 2017, 64, 4972–4983. [Google Scholar] [CrossRef]
- Li, W.; Zhang, J. Distributed practical output tracking of high-order stochastic multi-agent systems with inherent nonlinear drift and diffusion terms. Automatica 2014, 50, 3231–3238. [Google Scholar] [CrossRef]
- Hua, C.; Li, Y.; Guan, X. Leader-following consensus for high-order nonlinear stochastic multiagent systems. IEEE Trans. Cybern. 2017, 47, 1882–1891. [Google Scholar] [CrossRef]
- You, X.; Hua, C.; Yu, H.; Guan, X. Leader-following consensus for high-order stochastic multi-agent systems via dynamic output feedback control. Automatica 2019, 107, 418–424. [Google Scholar] [CrossRef]
- Zou, W.; Ahn, C.K.; Xiang, Z. Event-triggered consensus tracking control of stochastic nonlinear multiagent systems. IEEE Syst. J. 2019, 13, 4051–4059. [Google Scholar] [CrossRef]
- Cui, G.; Yu, J.; Shi, P. Observer-based finite-time adaptive fuzzy control with prescribed performance for nonstrict-feedback nonlinear systems. IEEE Trans. Fuzzy Syst. 2022, 30, 767–778. [Google Scholar] [CrossRef]
- Cui, G.; Yang, W.; Yu, J. Neural network-based finite-time adaptive tracking control of nonstrict-feedback nonlinear systems with actuator failures. Inf. Sci. 2021, 545, 298–311. [Google Scholar] [CrossRef]
- Ren, C.E.; Chen, L.; Chen, C.P. Adaptive fuzzy leader-following consensus control for stochastic multiagent systems with heterogeneous nonlinear dynamics. IEEE Trans. Fuzzy Syst. 2017, 25, 181–190. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, J.; Tong, S. Fuzzy adaptive optimized leader-following formation control for second-order stochastic multi-agent systems. IEEE Trans. Ind. Informat. 2022, 18, 6026–6037. [Google Scholar] [CrossRef]
- Yang, Y.; Miao, S.; Yue, D.; Xu, C.; Ye, D. Adaptive neural containment seeking of stochastic nonlinear strict-feedback multi-agent systems. Neurocomputing 2020, 400, 393–400. [Google Scholar] [CrossRef]
- Guo, X.; Liang, H.; Pan, Y. Observer-based adaptive fuzzy tracking control for stochastic nonlinear multi-agent systems with dead-zone input. Appl. Math. Comput. 2020, 379, 125269. [Google Scholar] [CrossRef]
- Zhao, Y.; Yu, H.; Xia, X. Event-triggered adaptive consensus for stochastic multi-agent systems with saturated input and partial state constraints. Inf. Sci. 2022, 603, 16–41. [Google Scholar] [CrossRef]
- Li, K.; Li, Y. Fuzzy adaptive optimal consensus fault-tolerant control for stochastic nonlinear multi-agent systems. IEEE Trans. Fuzzy Syst. 2022, 30, 2870–2885. [Google Scholar] [CrossRef]
- Qin, Z.; He, X.; Li, G.; Wu, Y. Robust adaptive consensus of nonstrict-feedback multi-agent systems with quantized input and unmodeled dynamics. Inf. Sci. 2019, 498, 117–134. [Google Scholar] [CrossRef]
- Liang, H.; Liu, G.; Huang, T.; Lam, H.K.; Wang, B. Cooperative fault-tolerant control for networks of stochastic nonlinear systems with nondifferential saturation nonlinearity. IEEE Trans. Syst. Man Cybern. 2022, 52, 1362–1372. [Google Scholar] [CrossRef]
- Zhao, G.; Cao, L.; Li, X.; Zhou, Q. Observer-based dynamic event-triggered control for nonstrict-feedback stochastic nonlinear multiagent systems. Appl. Math. Comput. 2022, 430, 127289. [Google Scholar] [CrossRef]
- Wu, Y.; Pan, Y.; Liang, H. Observer-based distributed fault-tolerant containment control for stochastic nonlinear multi-agent systems with asymmetric hysteretic quantization. Int. J. Robust Nonlinear Control 2022, 32, 7408–7430. [Google Scholar] [CrossRef]
- Yan, B.; Niu, B.; Zhao, X.; Wang, H.; Chen, W.; Liu, X. Neural-network-based adaptive event-triggered asymptotically consensus tracking control for nonlinear nonstrict-feedback MASs: An improved dynamic surface approach. IEEE Trans. Neural Netw. Learn. Syst. 2022. [Google Scholar] [CrossRef]
- Farrell, J.A.; Polycarpou, M.; Sharma, M.; Dong, W. Command filtered backstepping. IEEE Trans. Autom. Control 2009, 54, 1391–1395. [Google Scholar] [CrossRef]
- Shi, Z.; Zhou, C.; Guo, J. Observer-based consensus tracking control for a class of nonstrict-feedback nonlinear multi-agent systems with prescribed performance and input quantization. Int. J. Robust Nonlinear Control 2022, 32, 10374–10395. [Google Scholar] [CrossRef]
- Shahvali, M.; Askari, J. Distributed containment output-feedback control for a general class of stochastic nonlinear multi-agent systems. Neurocomputing 2016, 179, 202–210. [Google Scholar] [CrossRef]
- Wu, Y.; Pan, Y.; Chen, M.; Li, H. Quantized adaptive finite-time bipartite NN tracking control for stochastic multiagent systems. IEEE Trans. Cybern. 2021, 51, 2870–2881. [Google Scholar] [CrossRef] [PubMed]
- Zhao, L.; Yu, J.; Lin, C.; Ma, Y. Adaptive neural consensus tracking for nonlinear multiagent systems using finite-time command filtered backstepping. IEEE Trans. Syst. Man Cybern. 2018, 48, 2003–2012. [Google Scholar] [CrossRef]
- Sedghi, F.; Arefi, M.M.; Abooee, A.; Yin, S. Distributed adaptive-neural finite-time consensus control for stochastic nonlinear multiagent systems subject to saturated inputs. IEEE Trans. Neural Netw. Learn. Syst. 2022. [Google Scholar] [CrossRef]
- Song, X.; Zhao, L. Adaptive fuzzy finite-time consensus tracking for high-order stochastic multi-agent systems with input saturation. Int. J. Fuzzy Syst. 2022, 24, 3781–3795. [Google Scholar] [CrossRef]
- Wen, C.; Zhou, J.; Liu, Z.; Su, H. Robust adaptive control of uncertain nonlinear systems in the presence of input saturation and external disturbance. IEEE Trans. Autom. Control 2011, 56, 1672–1678. [Google Scholar] [CrossRef]
- Hosseinzadeh, M.; Yazdanpanah, M.J. Robust adaptive passivity-based control of open-loop unstable affine nonlinear systems subject to actuator saturation. IET Control Theory Appl. 2017, 11, 2731–2742. [Google Scholar] [CrossRef]
- Wu, B.; Wu, J.; Zhang, J.; Tang, G.; Zhao, Z. Adaptive neural control of a 2DOF helicopter with input saturation and time-varying output constraint. Actuators 2022, 11, 336. [Google Scholar] [CrossRef]
- Cui, G.; Xu, S.; Lewis, F.L.; Zhang, B.; Ma, Q. Distributed consensus tracking for non-linear multi-agent systems with input saturation: A command filtered backstepping approach. IET Control Theory Appl. 2016, 10, 509–516. [Google Scholar] [CrossRef]
- Cao, L.; Li, H.; Dong, G.; Lu, R. Event-triggered control for multiagent systems with sensor faults and input saturation. IEEE Trans. Syst. Man Cybern. 2021, 51, 3855–3866. [Google Scholar] [CrossRef]
- Ji, H.; Xi, H. Adaptive output-feedback tracking of stochastic nonlinear systems. IEEE Trans. Autom. Control 2006, 51, 355–360. [Google Scholar] [CrossRef]
- Wang, L. Adaptive Fuzzy Systems and Control, Design and Stability Analysis; Prentice-Hall: Englewood Cliffs, NJ, USA, 1994. [Google Scholar]
- Qian, C.; Lin, W. A continuous feedback approach to global strong stabilization of nonlinear systems. IEEE Trans. Autom. Control 2001, 46, 1061–1079. [Google Scholar] [CrossRef]
- Hardy, G.H.; Littlewood, J.E.; Pólya, G. Inequalities; Cambridge University Press: Cambridge, UK, 1952. [Google Scholar]
- Zhang, H.; Liu, Y.; Dai, J.; Wang, Y. Command filter based adaptive fuzzy finite-time control for a class of uncertain nonlinear systems with hysteresis. IEEE Trans. Fuzzy Syst. 2021, 29, 2553–2564. [Google Scholar] [CrossRef]
- Cui, G.; Yu, J.; Wang, Q. Finite-time adaptive fuzzy control for MIMO nonlinear systems with input saturation via improved command-filtered backstepping. IEEE Trans. Syst. Man Cybern. 2022, 52, 980–989. [Google Scholar] [CrossRef]
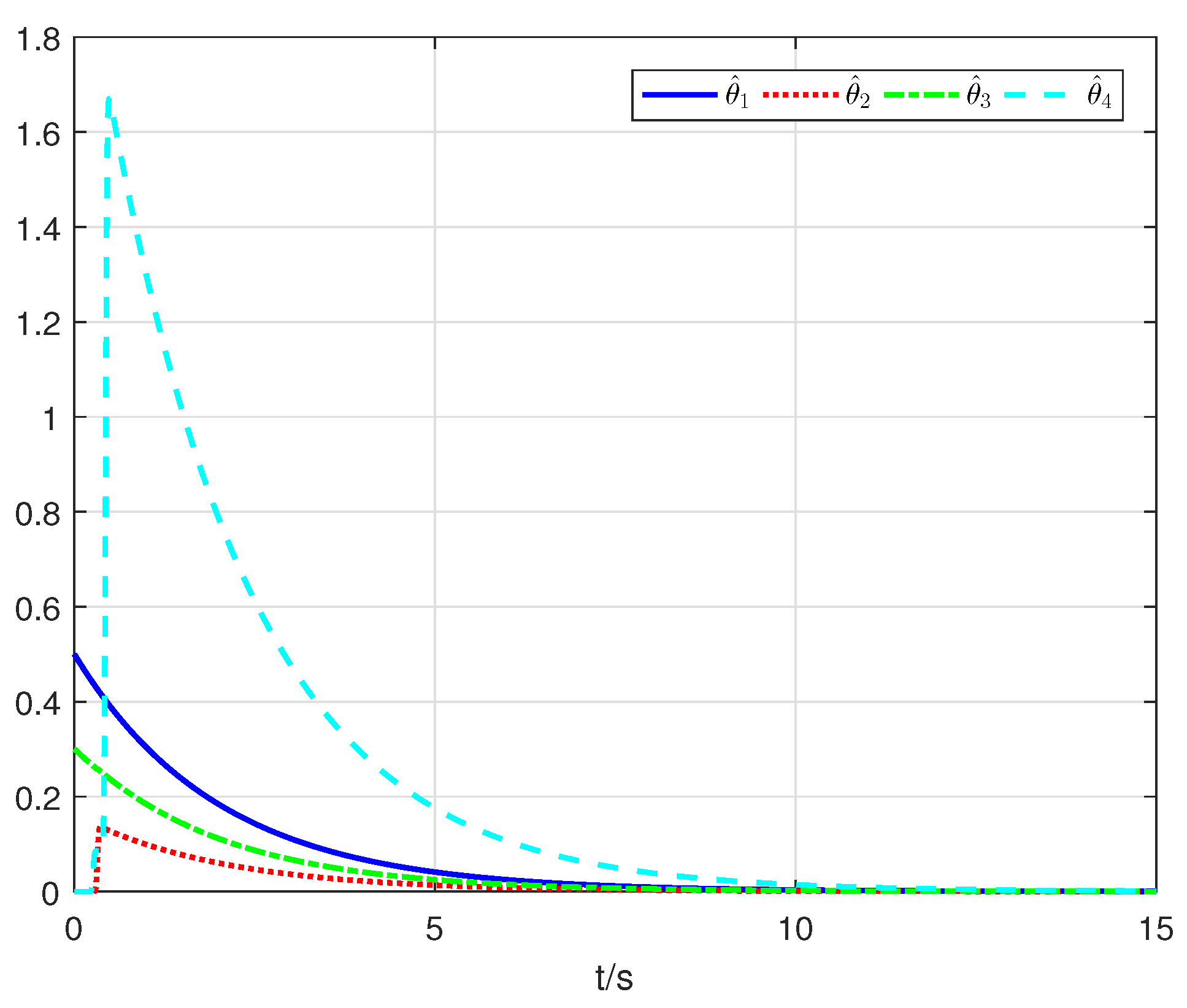
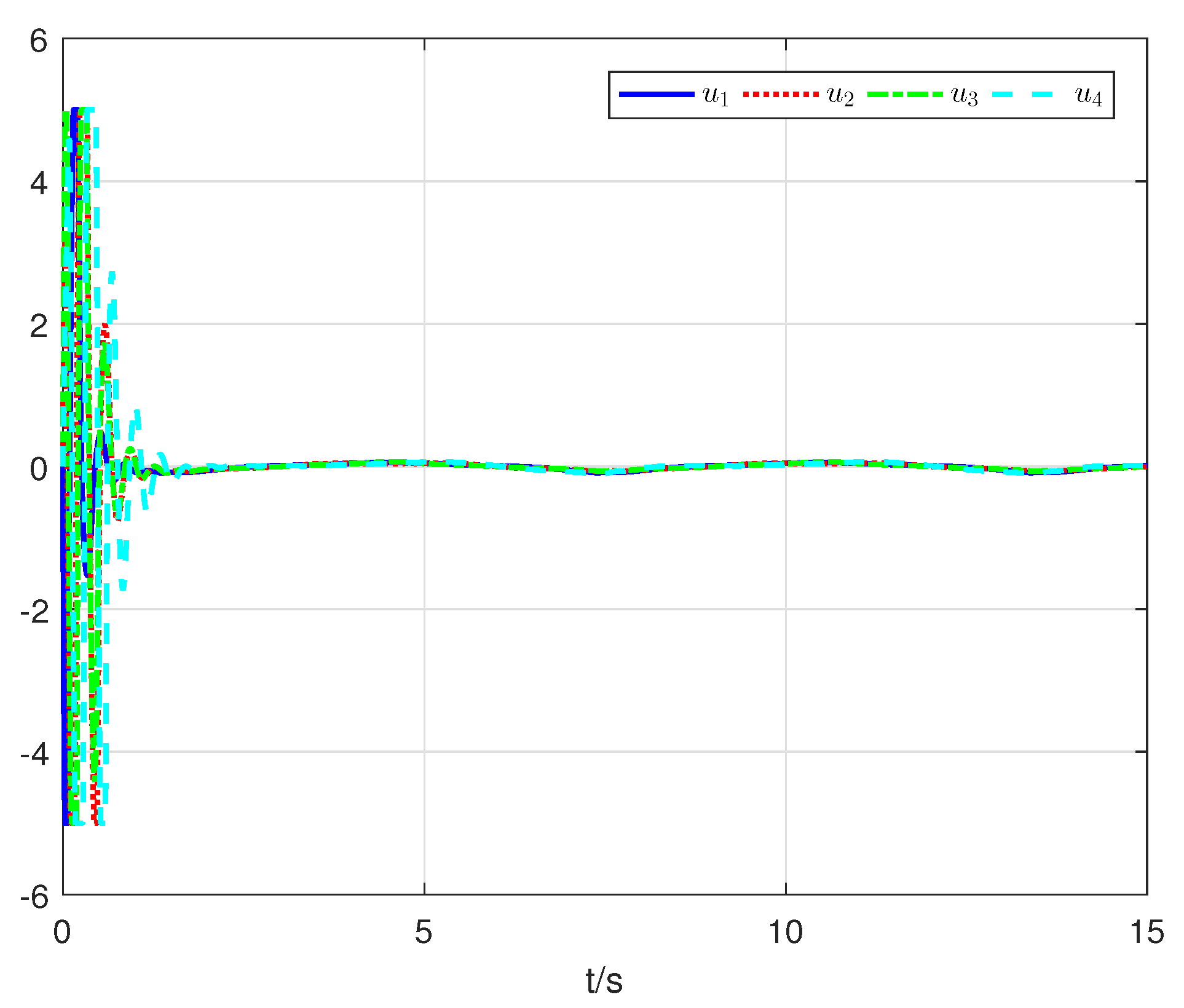
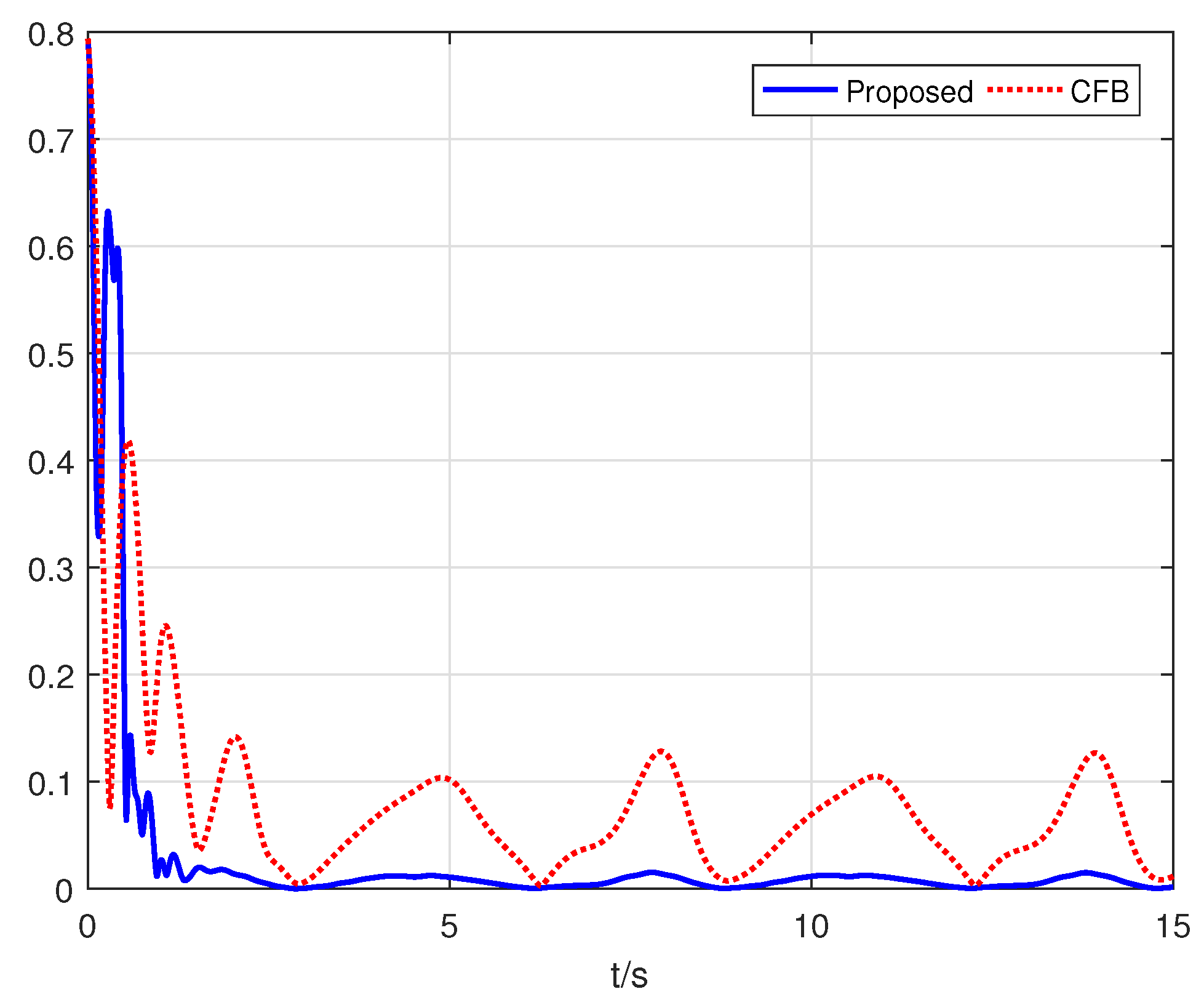
| Scheme | Settling Time (s) | RMSE |
|---|---|---|
| Proposed | 0.59 | 0.1032 |
| CFB in [23] | 1.31 | 0.1219 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Liu, Y.; Cui, G.; Li, Z.; Hao, W. Finite-Time Distributed Control of Non-Triangular Stochastic Nonlinear Multi-Agent Systems with Input Constraints. Actuators 2023, 12, 28. https://doi.org/10.3390/act12010028
Zhang Y, Liu Y, Cui G, Li Z, Hao W. Finite-Time Distributed Control of Non-Triangular Stochastic Nonlinear Multi-Agent Systems with Input Constraints. Actuators. 2023; 12(1):28. https://doi.org/10.3390/act12010028
Chicago/Turabian StyleZhang, Yuhang, Yifan Liu, Guozeng Cui, Ze Li, and Wanjun Hao. 2023. "Finite-Time Distributed Control of Non-Triangular Stochastic Nonlinear Multi-Agent Systems with Input Constraints" Actuators 12, no. 1: 28. https://doi.org/10.3390/act12010028
APA StyleZhang, Y., Liu, Y., Cui, G., Li, Z., & Hao, W. (2023). Finite-Time Distributed Control of Non-Triangular Stochastic Nonlinear Multi-Agent Systems with Input Constraints. Actuators, 12(1), 28. https://doi.org/10.3390/act12010028





