Numerical Study of the Fish-like Robot Swimming in Fluid with High Reynolds Number: Immersed Boundary Method
Abstract
:1. Introduction
2. Numerical Method
2.1. Governing Equation
2.2. Multidirect Forcing Method
2.3. Turbulence Model
- I.
- The Reynolds hypothesis is valid; namely, the parameter completely depends on k, ε, and grad ;
- II.
- The velocity fluctuations and the corresponding turbulence flow are isotropic;
- III.
- The convective transport of the fluctuating quantities corresponds to the convective transport of the diffusive transport;
- IV.
- is proportional to , and the proportionality factor is the turbulent eddy viscosity .
2.4. Solid Wall Boundary
3. Simulation of Fish-like Robot Swimming in Water
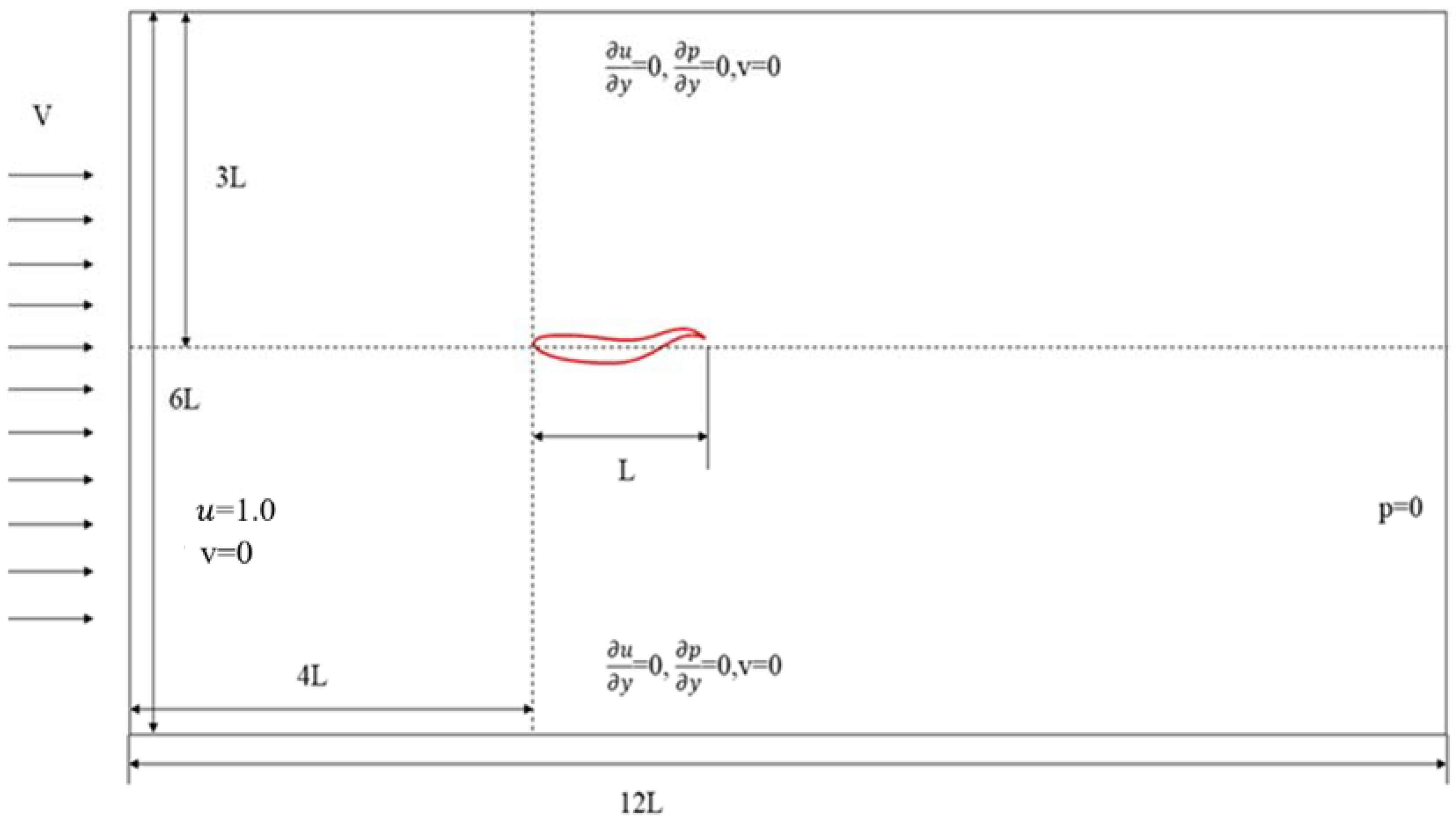
3.1. Model Verification through Flow over Square Object
3.2. Models of Fish Motion
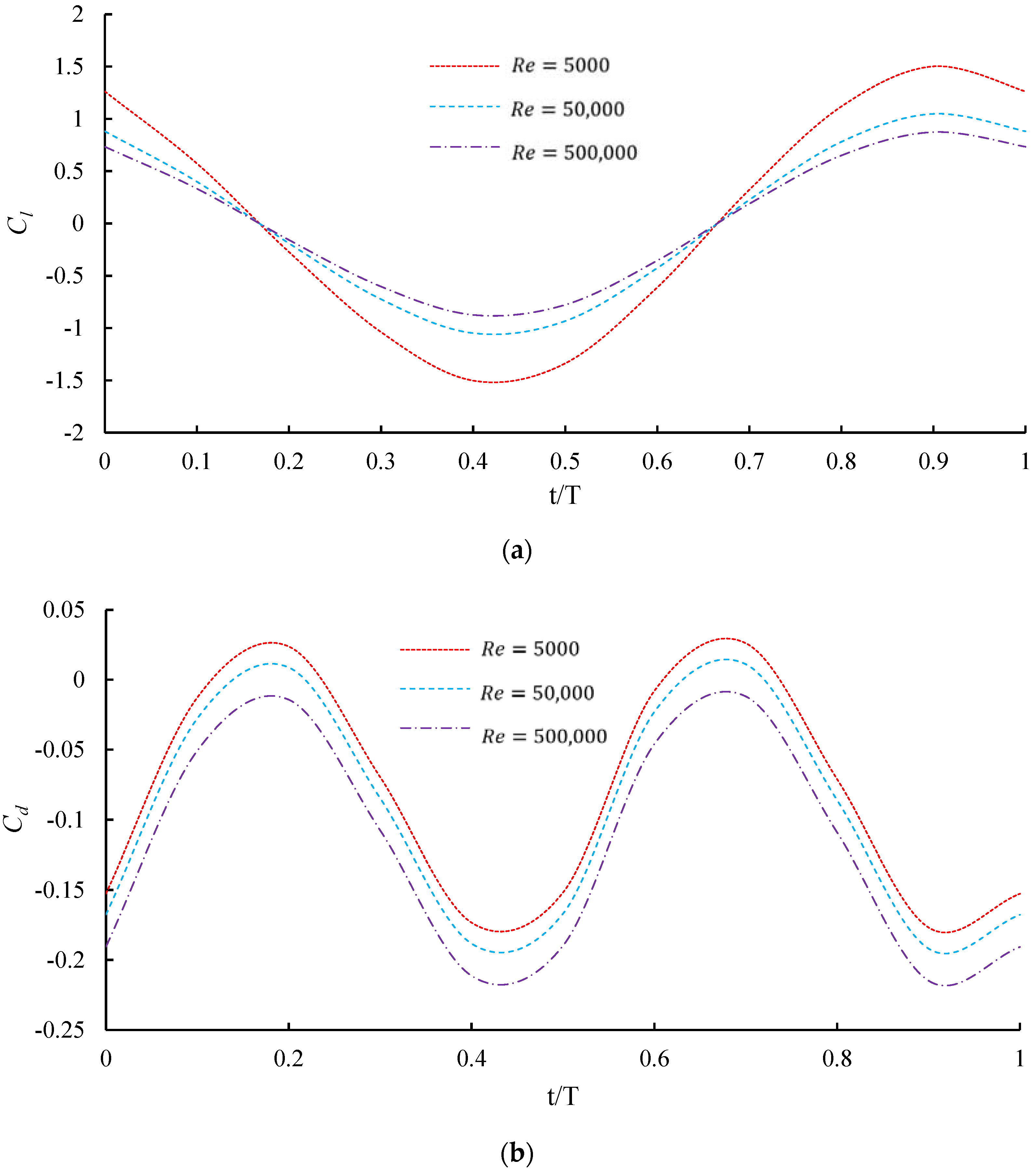
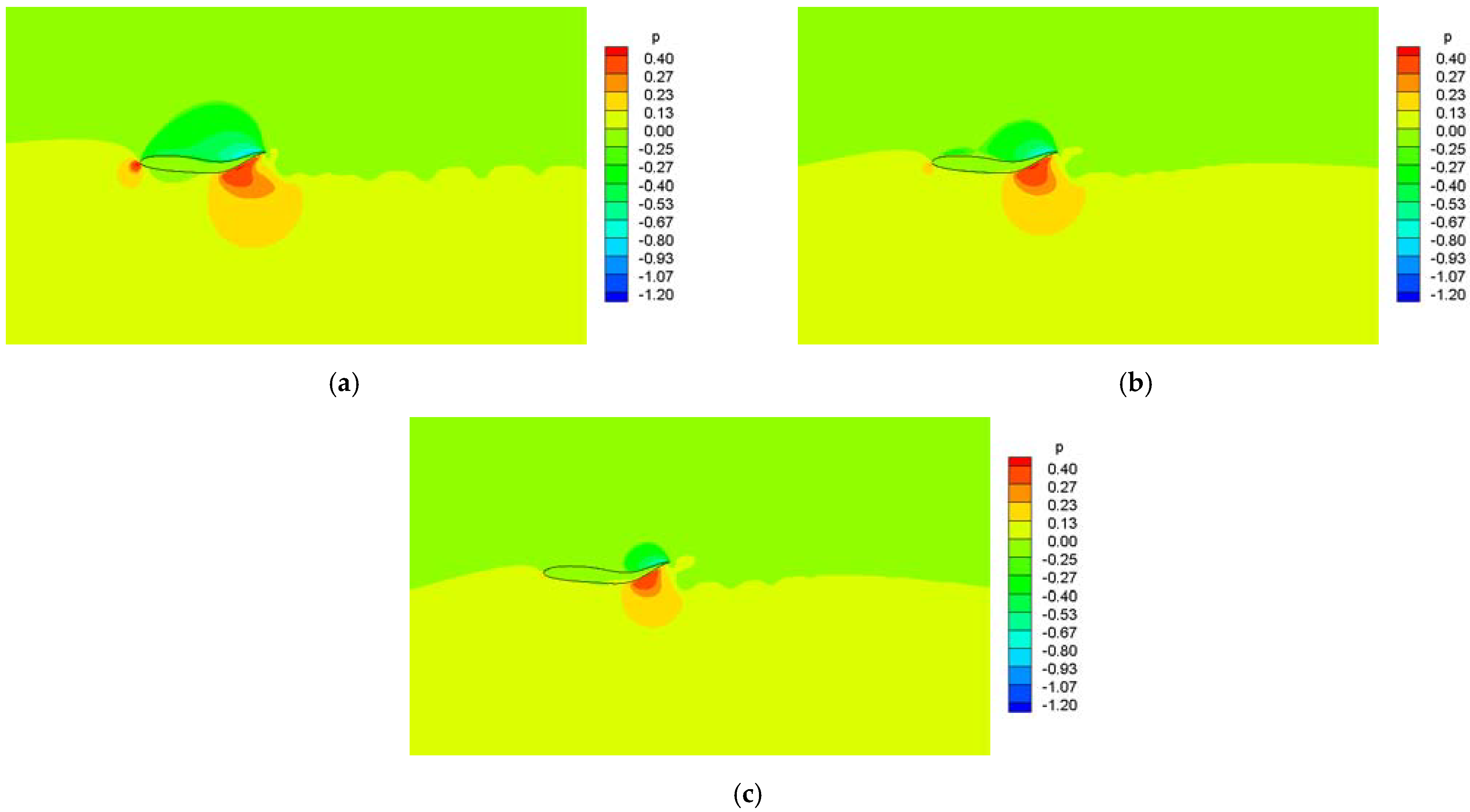
3.3. Influence of Different Reynolds Numbers
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aracri, S.; Giorgio-Serchi, F.; Suaria, G.; Sayed, M.E.; Stokes, A.A. Soft robots for ocean exploration and offshore operations: A perspective. Soft Robot. 2021, 8, 625–639. [Google Scholar] [CrossRef] [PubMed]
- Marcin, M.; Adam, S.; Jerzy, Z.; Marcin, M. Fish-like shaped robot for underwater surveillance and reconnaissance–Hull design and study of drag and noise. Ocean Eng. 2020, 217, 107889. [Google Scholar] [CrossRef]
- Glaze, J.; Salazar, R.; Vasconcellos, R.; Abdelkefi, A. Comparative design, hydrodynamic analysis, and physical performance of fish-like robots. Appl. Ocean Res. 2020, 106, 102443. [Google Scholar] [CrossRef]
- Chen, Y.; Qiao, J.; Liu, J.; Zhao, R.; An, D.; Wei, Y. Three-dimensional path following control system for net cage inspection using bionic robotic fish. Inf. Process. Agric 2021, 9, 2214–3173. [Google Scholar] [CrossRef]
- Utter, B.; Brown, A. Open-source five degree of freedom motion platform for investigating fish-robot interaction. HardwareX 2020, 7, e00107. [Google Scholar] [CrossRef] [PubMed]
- Shao, J.; Wang, L.; Yu, J. Development of an artificial fish-like robot and its application in cooperative transportation. Control Eng. Pract. 2008, 16, 569–584. [Google Scholar] [CrossRef]
- Giorgio-Serchi, F.; Weymouth, G.D. Drag cancellation by added-mass pumping. J. JFM 2016, 798. [Google Scholar] [CrossRef] [Green Version]
- Weymouth, G.; Giorgioserchi, F. Analytic Modeling of a Size-Changing Swimmer; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Armanini, C.; Farman, M.; Calisti, M.; Serchi, F.G.; Renda, F. Flagellate Underwater Robotics at Macroscale: Design, Modeling, and Characterization. IEEE Trans. Robot. 2021, 38, 731–747. [Google Scholar] [CrossRef]
- Liu, H.; Wassersug, R.J.; Kawachi, K.A. Computational fluid dynamics study of tadpole swimming. J. Exp. Biol. 1996, 199, 1245–1260. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Wassersug, R.J.; Kawachi, K.A. The three-dimensional hydrodynamics of tadpole locomotion. J. Exp. Biol 1997, 200, 2807–2819. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Ellington, C.P.; Kawachi, K.A.; Berg, C.V.D.; Willmott, A.P. A computational fluid dynamic study of hawkmoth hovering. J. Exp. Biol. 1998, 201, 461–477. [Google Scholar] [CrossRef] [PubMed]
- Carling, J.; Williams, T.; Bowtell, G. Self-propelled anguilliform swimming: Simultaneous solution of the two-dimensional Navier–Stokes equations and Newton’s laws of motion. J. Exp. Biol. 1998, 201, 3143–3166. [Google Scholar] [CrossRef] [PubMed]
- Togashi, F.; Ito, Y.; Murayama, M. Flow Simulation of flapping Wings of an Insect Using OVERSET unstructured Grid. In Proceedings of the Aiaa Computational Fluid Dynamics Conference, San Diego, CA, USA, 24–27 June 2013. [Google Scholar]
- Zhang, L.P.; Chang, X.H.; Duan, X.P.; Wang, Z.Y.; Zhang, H.X. A block LU-SGS implicit unsteady incompressible flow solver on hybrid dynamic grids for 2D external bio-fluid simulations. Comput. Fluids 2009, 38, 290–308. [Google Scholar] [CrossRef]
- Maertens, A.P.; Triantafyllou, M.S.; Yue, D. Efficiency of Fish Propulsion. Bioinspiration Biomim. 2015, 10, 4. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Peskin, C.S. Flow patterns around heart valves: A numerical method. J. Comput. Phys. 1972, 10, 252–271. [Google Scholar] [CrossRef]
- Weymouth, G.D.; Yue, K.P. Conservative Volume-of-Fluid method for free-surface simulations on Cartesian-grids. J. Comput. Phys. 2010, 229, 2853–2865. [Google Scholar] [CrossRef]
- Noor, D.Z.; Chern, M.J.; Horng, T.L. An immersed boundary method to solve fluid–solid interaction problems. Comput. Mechv. 2009, 44, 447–453. [Google Scholar] [CrossRef]
- Kress, R. Linear Integral Equations. Ann. Math. 2014, 82, 323–349. [Google Scholar]
- Pironneau, O. Analysis of the K-Epsilon Turbulence Model; Wiley: Hoboken, NJ, USA, 1993. [Google Scholar]
- Griebel, M.; Dornseifer, T.; Neunhoeffer, T. Numerical Simulation in Fluid Dynamics: A Practical Introduction; Society for Industrial and Applied Mathematics: Phidadelphia, PA, USA, 1998. [Google Scholar]
- Mohammadi, B.; Pironneau, O. Unsteady separated turbulent flows computation with wall-laws and k–ε model. Comput. Methods Appl. Mech. Eng. 1997, 148, 393–405. [Google Scholar] [CrossRef]
- Wardle, C.S.; Videler, J.J. Fish Swimming; Cambridge University Press: Britain, UK, 1980. [Google Scholar]

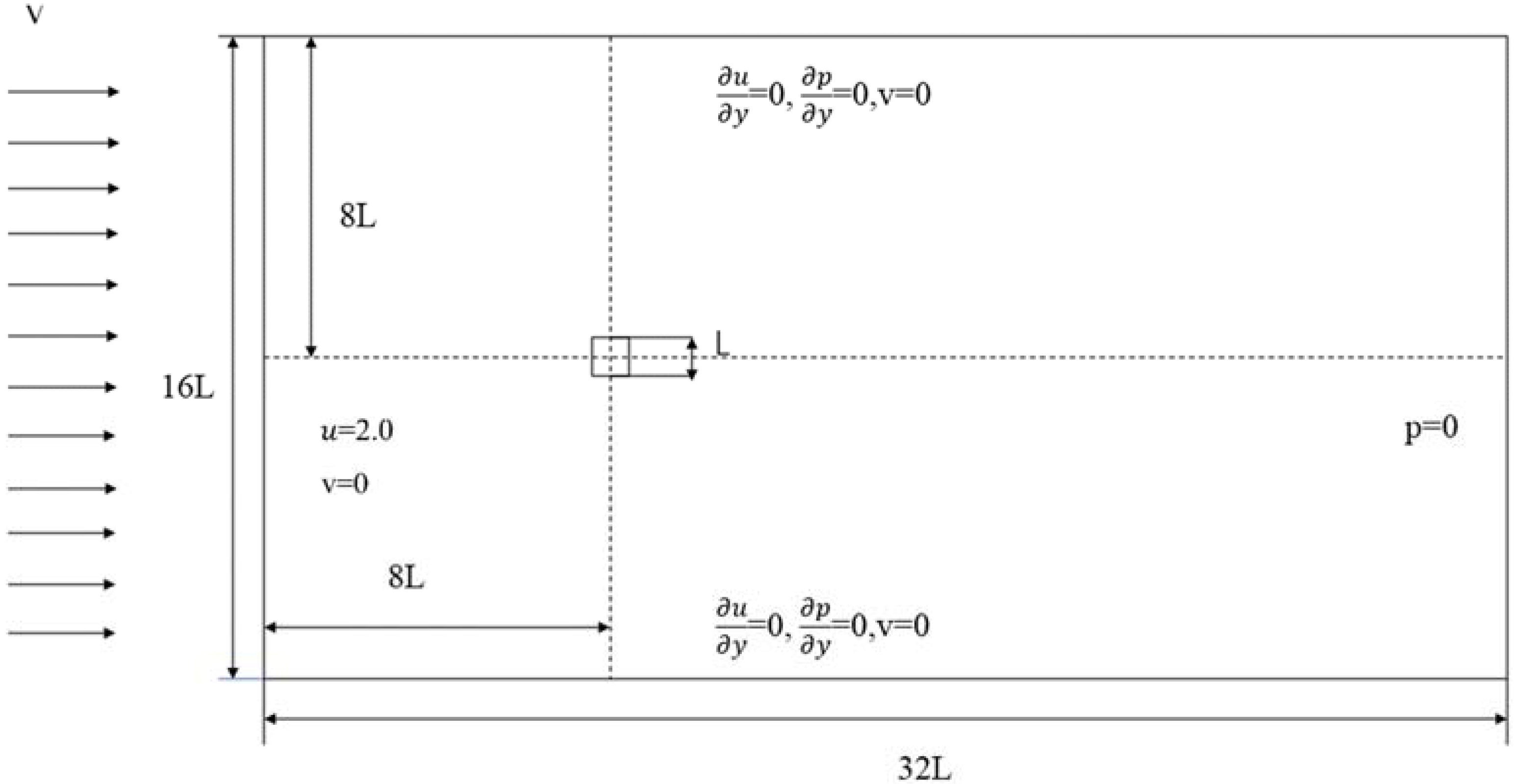
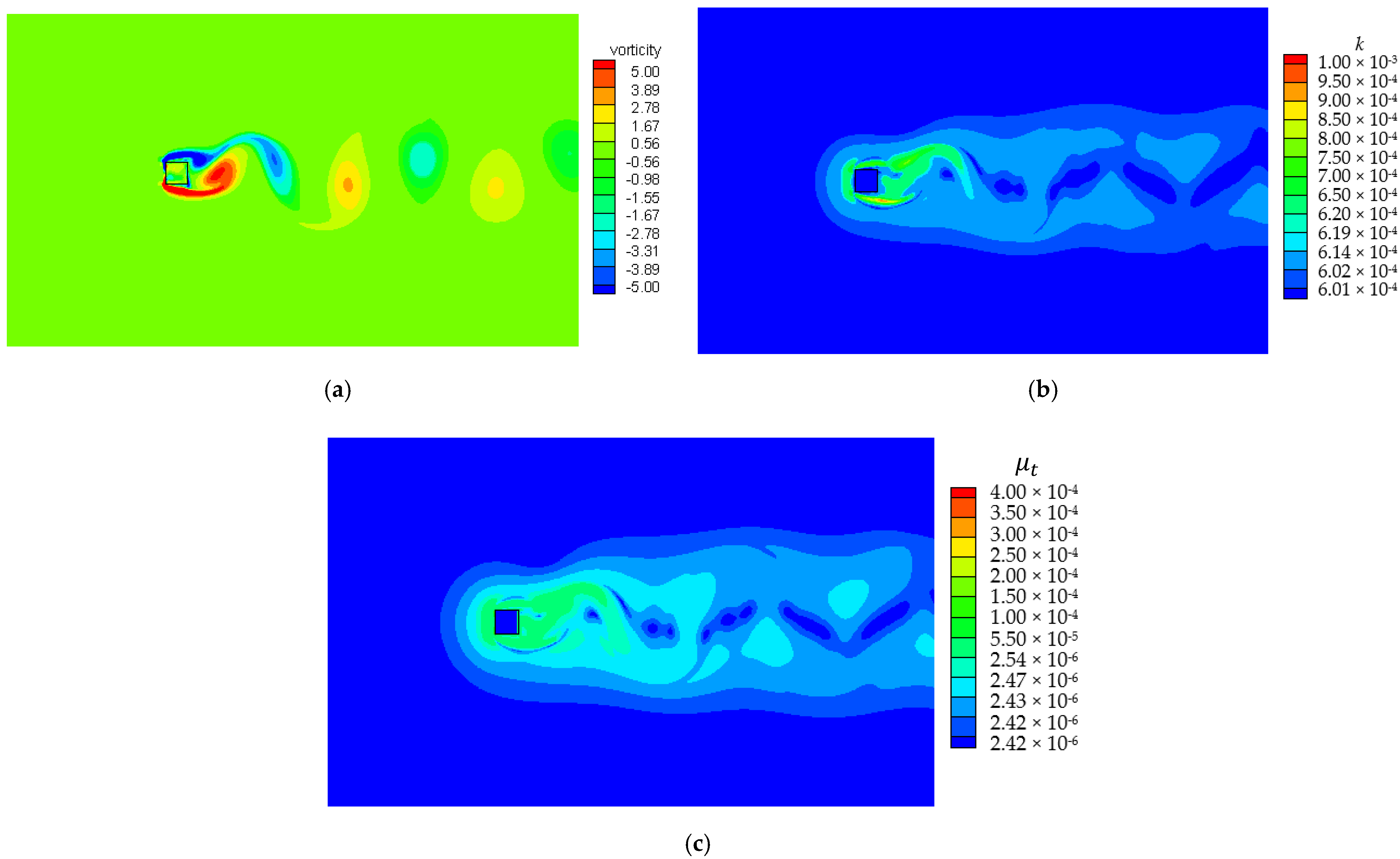
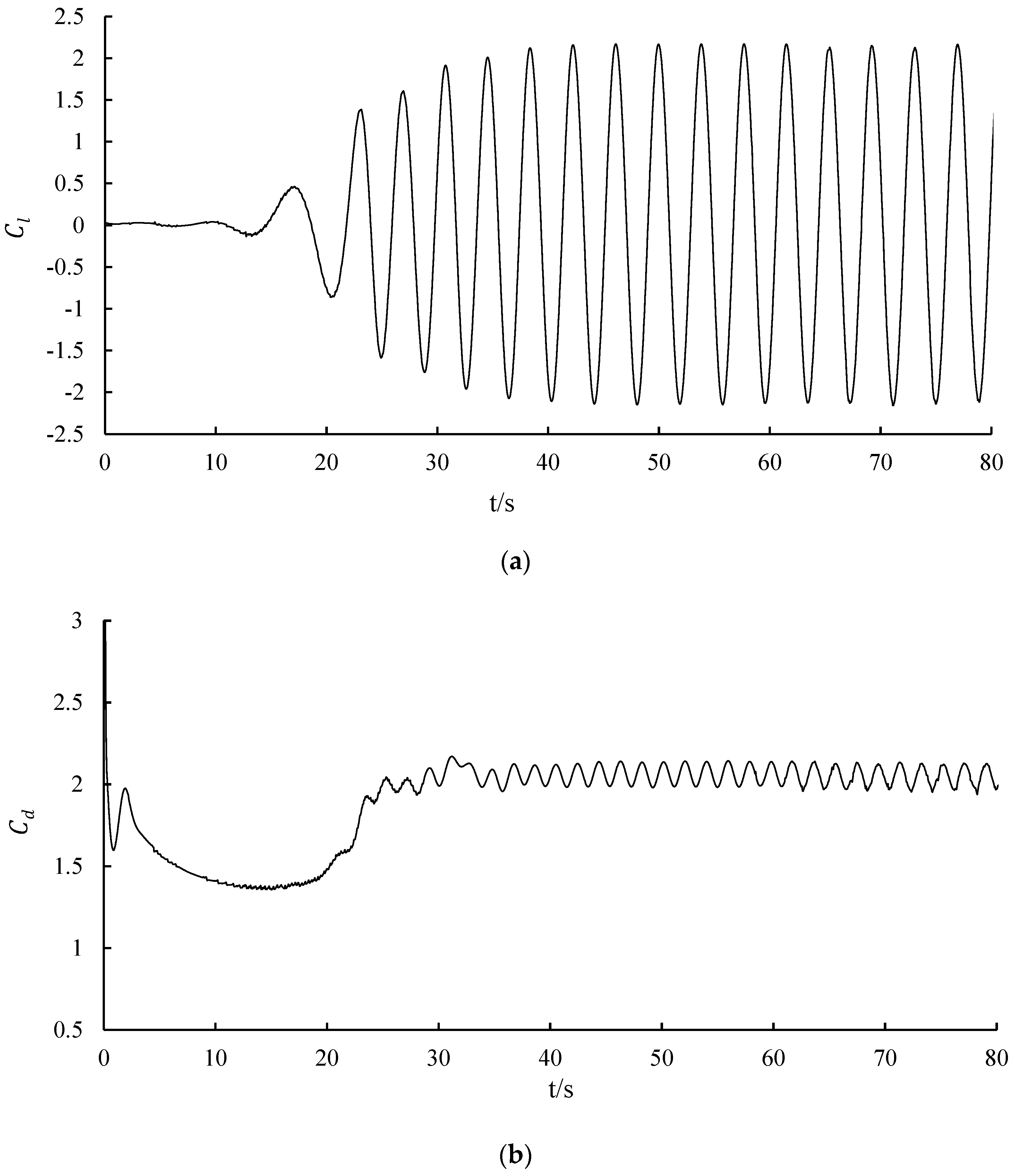



| / | St | ||
|---|---|---|---|
| This paper | 2.14 | 2.05 | 0.135 |
| Ref. [23] | 2.05 | 2.11 | 0.14 |
| Error | 4.3% | 2.8% | 3.6% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Lv, Z.; Hua, H.; Zhang, C.; Yu, H.; Jiao, Y. Numerical Study of the Fish-like Robot Swimming in Fluid with High Reynolds Number: Immersed Boundary Method. Actuators 2022, 11, 158. https://doi.org/10.3390/act11060158
Zhang J, Lv Z, Hua H, Zhang C, Yu H, Jiao Y. Numerical Study of the Fish-like Robot Swimming in Fluid with High Reynolds Number: Immersed Boundary Method. Actuators. 2022; 11(6):158. https://doi.org/10.3390/act11060158
Chicago/Turabian StyleZhang, Jun, Zhichao Lv, Haobo Hua, Chunming Zhang, Haiyang Yu, and Yanmei Jiao. 2022. "Numerical Study of the Fish-like Robot Swimming in Fluid with High Reynolds Number: Immersed Boundary Method" Actuators 11, no. 6: 158. https://doi.org/10.3390/act11060158
APA StyleZhang, J., Lv, Z., Hua, H., Zhang, C., Yu, H., & Jiao, Y. (2022). Numerical Study of the Fish-like Robot Swimming in Fluid with High Reynolds Number: Immersed Boundary Method. Actuators, 11(6), 158. https://doi.org/10.3390/act11060158







