Vibration Isolation Performance of an Adaptive Magnetorheological Elastomer-Based Dynamic Vibration Absorber
Abstract
:1. Introduction
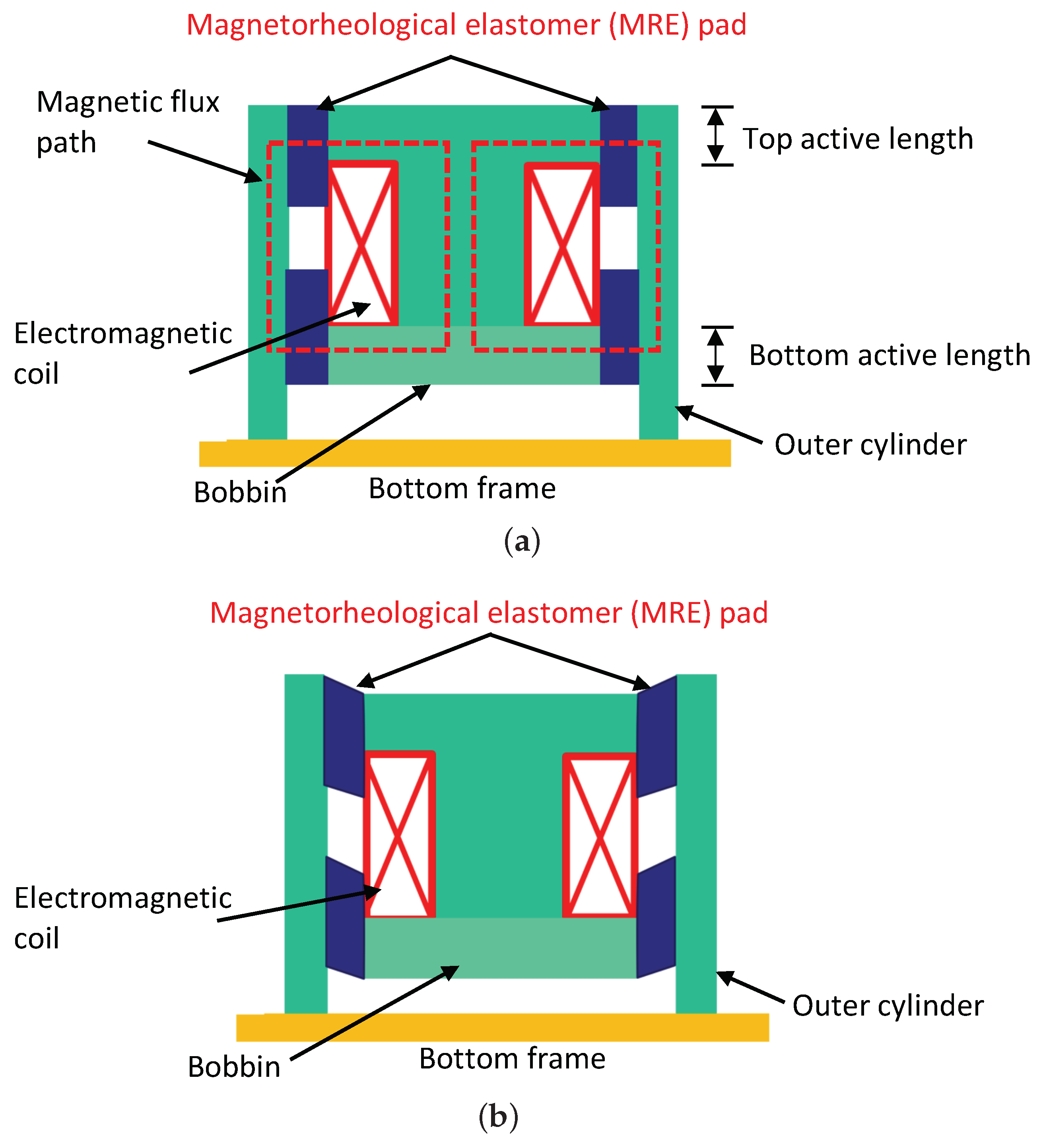
2. Magnetorheological Elastomer-Based Dynamic Vibration Absorber (MRE-DVA)
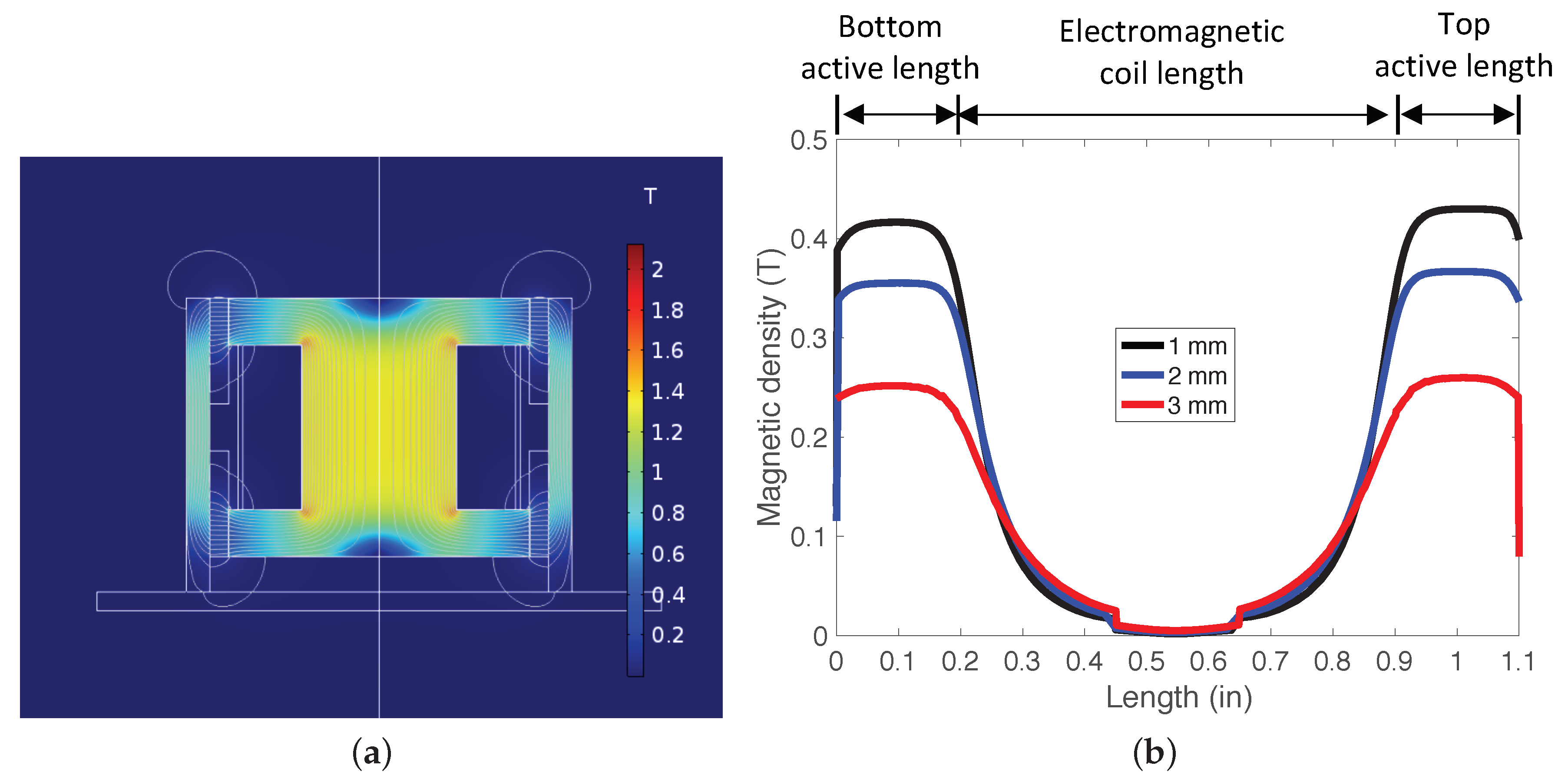
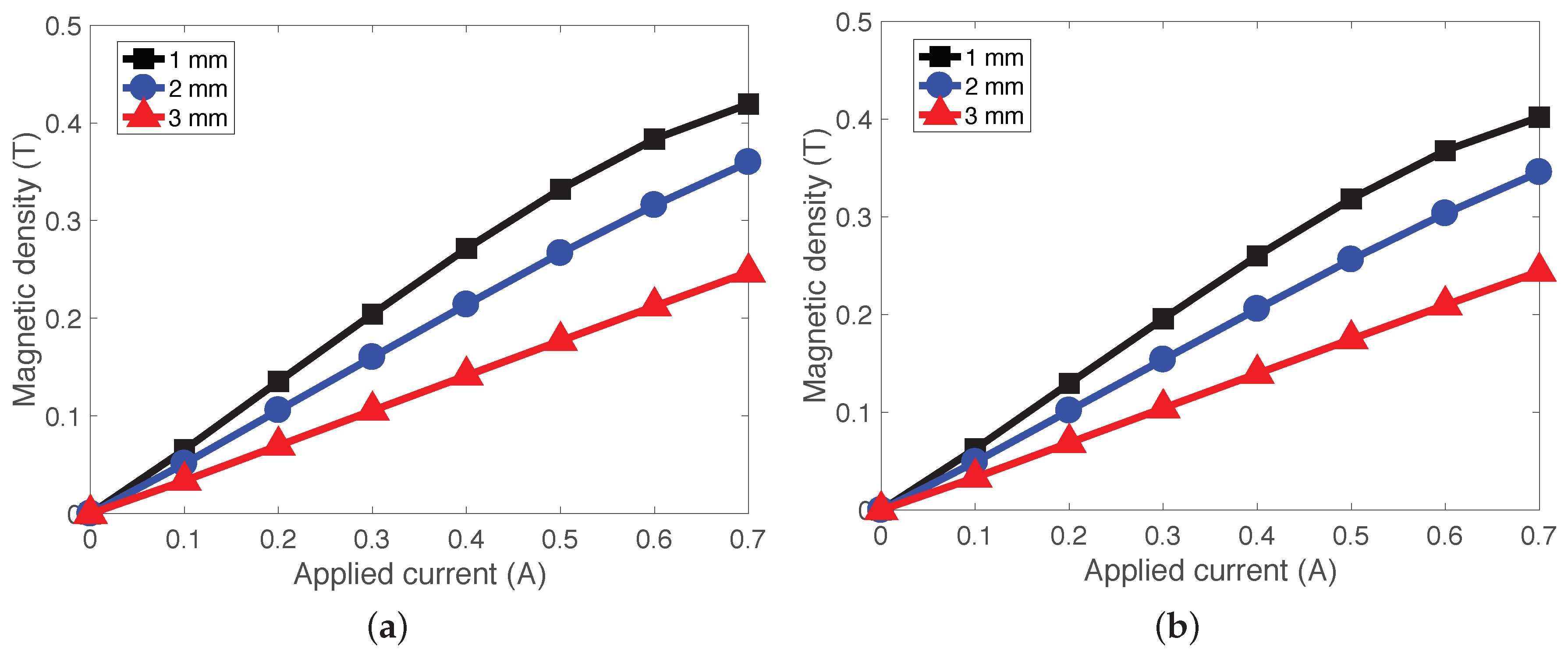
2.1. Electromagnetic Analysis
2.2. MRE Pad Material
2.3. Fabrication of the MRE-DVA
3. Damper Force Characteristics of the MRE-DVA
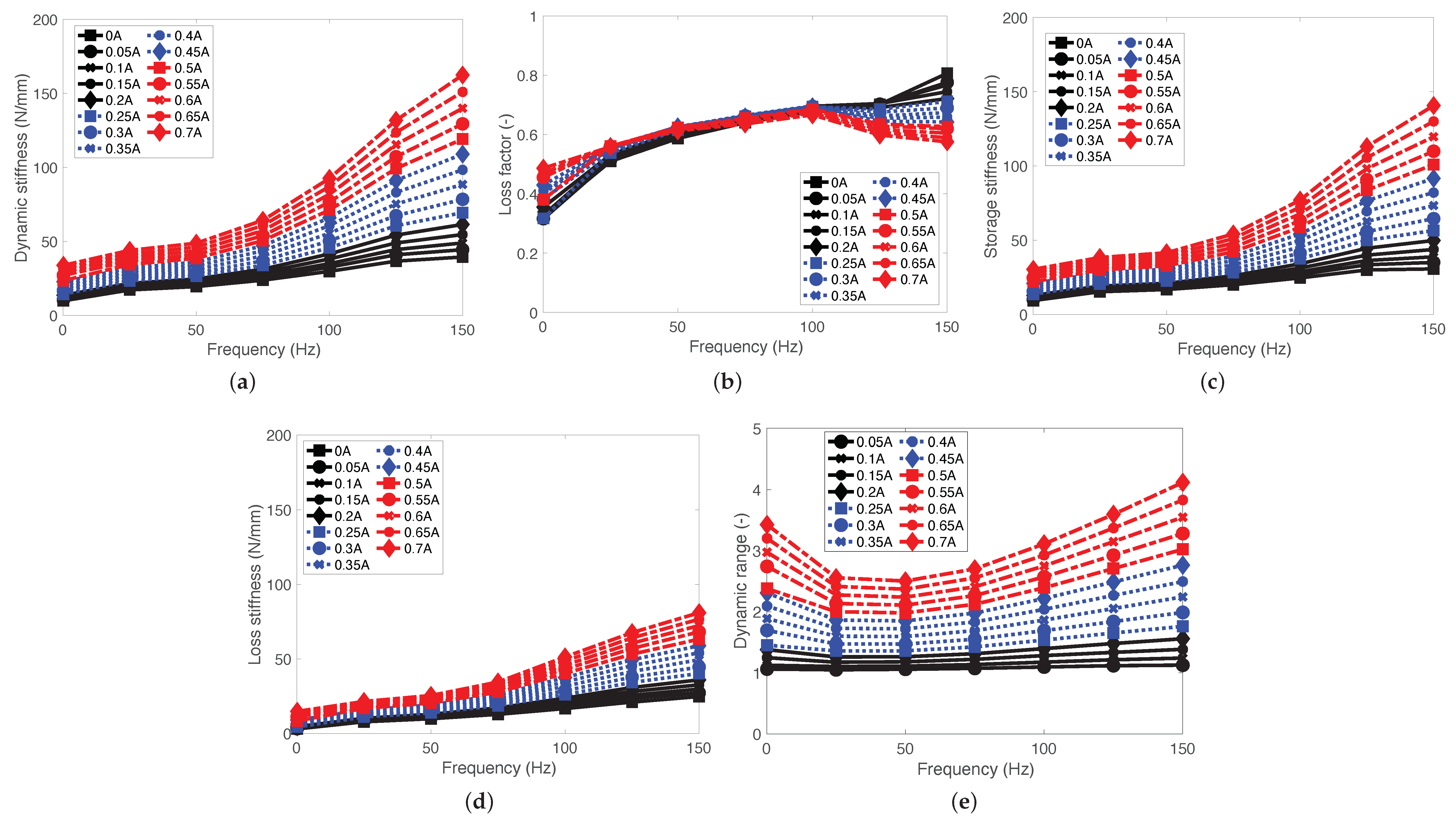
3.1. Complex Stiffness Analysis Method
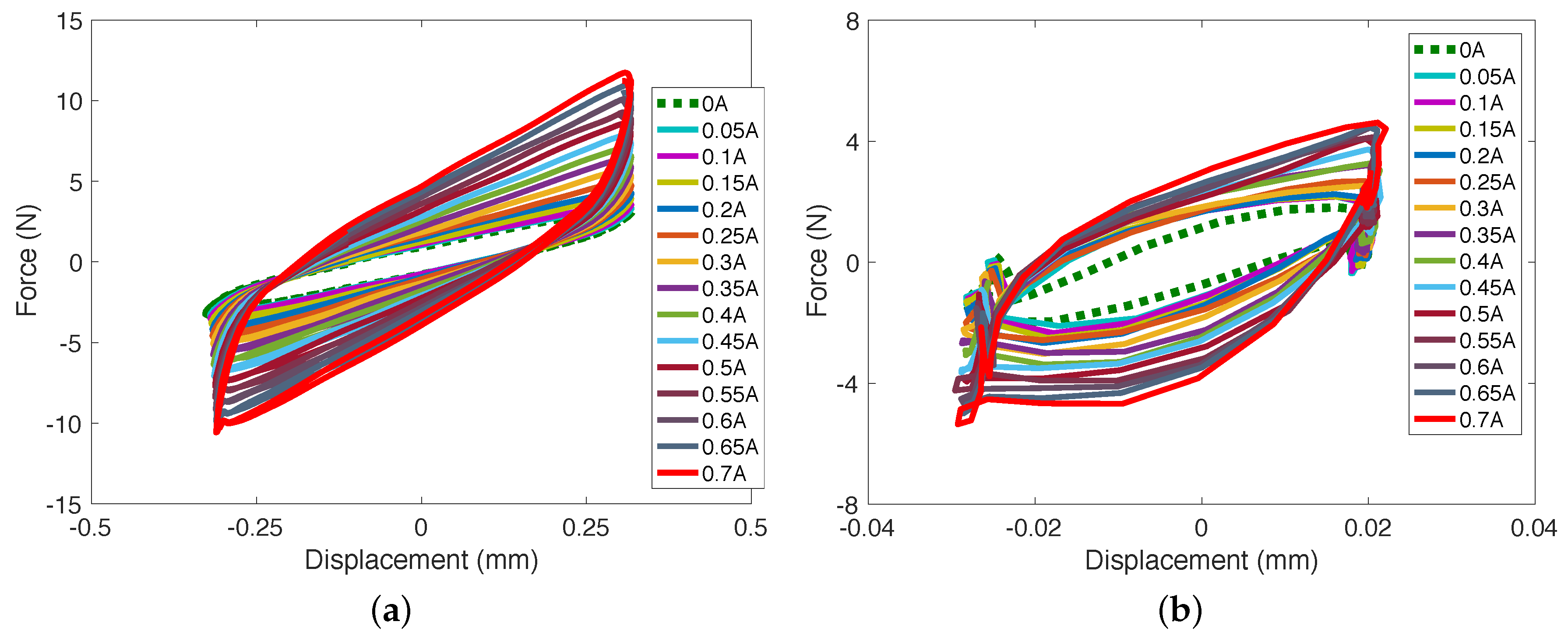
3.2. Experimental Results for the Damper Force Behavior
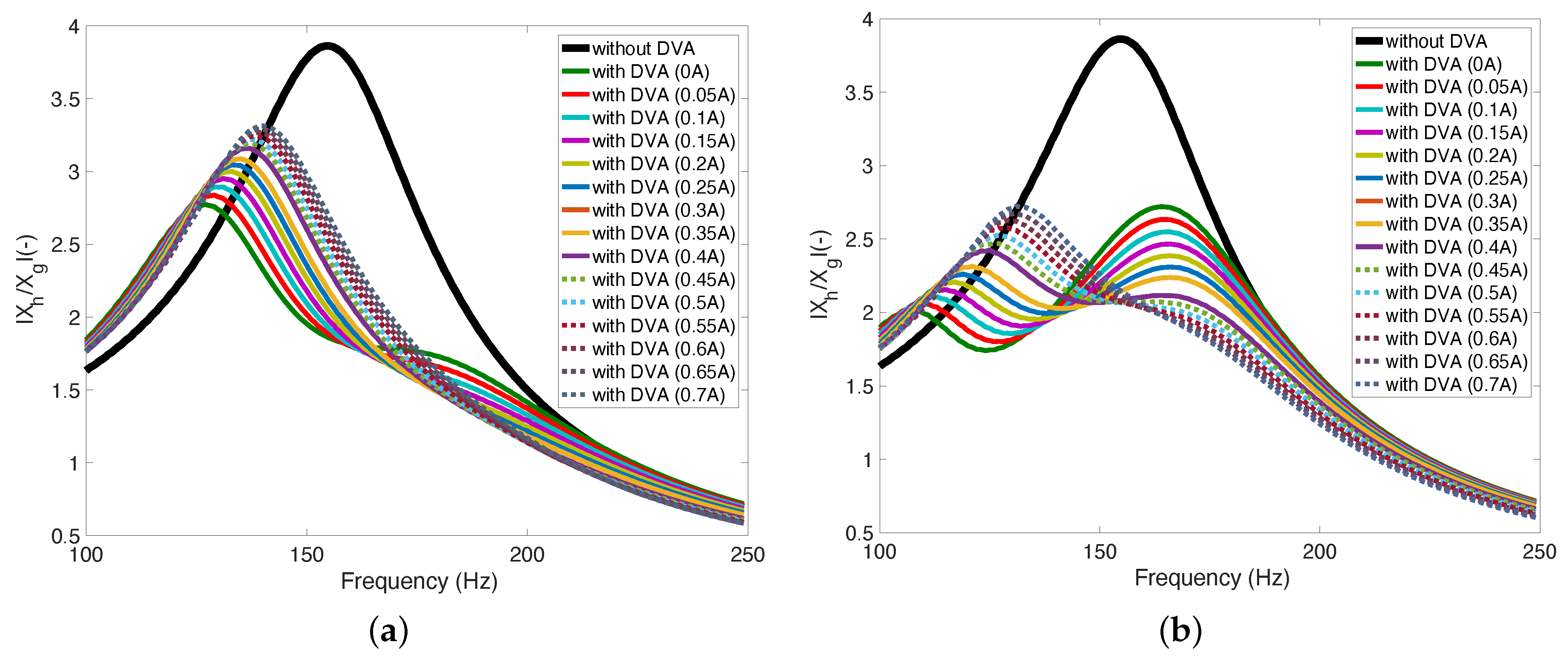
4. Vibration Isolation Performance of the MRE-DVA
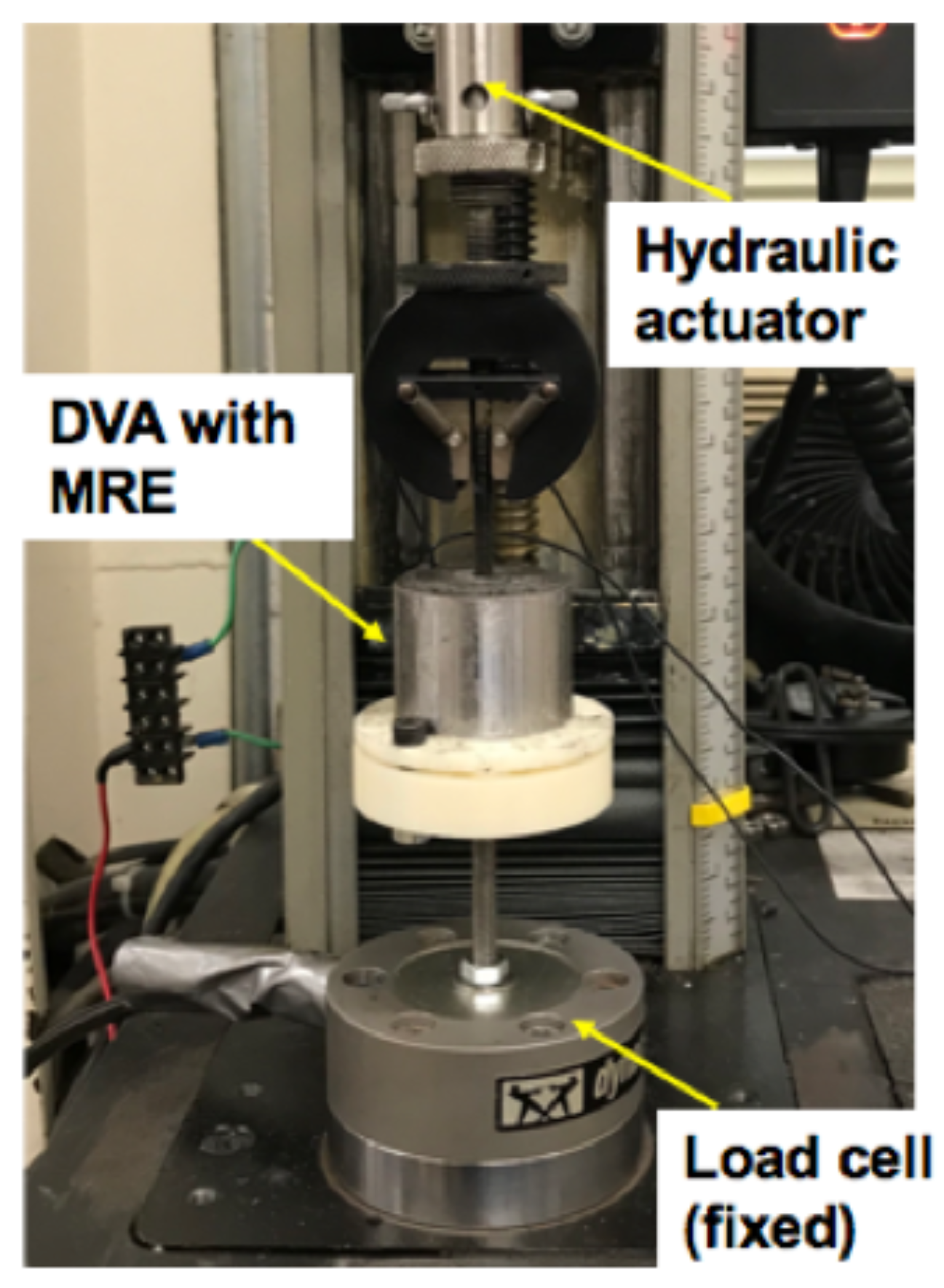
4.1. Experimental Setup and Results
4.2. Equivalent Mechanical Model of the MRE-DVA
4.3. Effect of the MRE Pad Thickness
5. Conclusions
- To soften the stiffness of the MRE pads, a silicone oil was added to the mixture of the liquid silicone rubber and carbonyl Fe particles before curing.
- From the complex stiffness analysis for the damper force cycle, it was confirmed that the dynamic range (DR) was at a relatively higher current input of above 0.5 A over the tested frequency range.
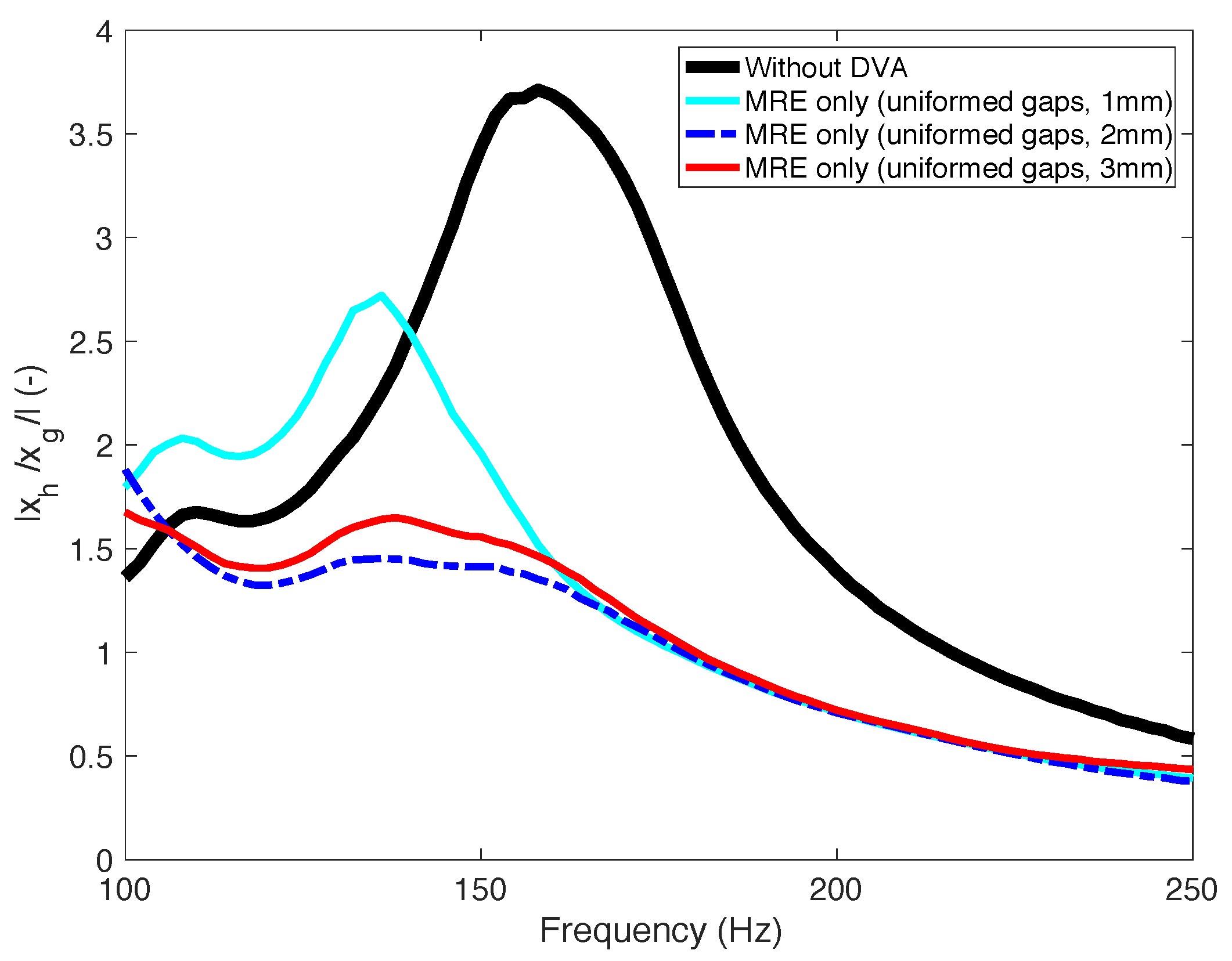
- From the vibration isolation testing, it was observed that the resonant frequencies of the MRE-DVAs could be substantially adjusted using an applied current input. For the 2 mm and 3 mm MRE pad thickness cases, the adjustable resonant frequency range by the current input was about 135–170 Hz. However, for the 1 mm MRE pad thickness case, the resonant frequency change was small because it was initially too stiff for no current input (0 A).
- From the minimum envelopes shown the in vibration frequency response plots for the tested frequency range from 100 Hz to 250 Hz, it was demonstrated that the system with the MRE-DVAs achieved much better vibration isolation performance than the baseline system with no DVA.
- It was confirmed that the design of the MRE-DVAs used in this study was simple but effective to reduce the vibration of the target system for the high frequency vibrations (i.e., 100–250 Hz).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Variables | Values |
|---|---|
| For the 1 mm MRE pad thickness case | |
| Stiffness of the MRE-DVA at no current input, | 107.8 kN/m |
| Viscous damping of the MRE-DVA at no current input, | 45.9 N · s/m |
| Coulomb friction damping force (i.e., yield force), | 0.51 N |
| of the MRE-DVA at a current input of 0.05 A | |
| For the 2 mm MRE pad thickness case | |
| Stiffness of the MRE-DVA at no current input, | 67.2 kN/m |
| Viscous damping of the MRE-DVA at no current input, | 27.2 N · s/m |
| Coulomb friction damping force (i.e., yield force), | 0.39 N |
| of the MRE-DVA at a current input of 0.05 A | |
| For the 3 mm MRE pad thickness case | |
| Stiffness of the MRE-DVA at no current input, | 60.9 kN/m |
| Viscous damping of the MRE-DVA at no current input, | 34.5 N · s/m |
| Coulomb friction damping force (i.e., yield force), | 0.26 N |
| of the MRE-DVA at a current input of 0.05 A |
References
- Hua, Y.; Wong, W.; Cheng, L. Optimal Design of a Beam-Based Dynamic Vibration Absorber Using Fixed-Points Theory. J. Sound Vib. 2018, 421, 111–131. [Google Scholar] [CrossRef]
- Phuc, V.; Tran, V.T. Optimization Design for Multiple Dynamic Vibration Absorbers on Damped Structures Using Equivalent Linearization Method. Proc. Inst. Mech. Eng. J. Multi-Body Dyn. 2021, 236, 41–50. [Google Scholar] [CrossRef]
- Ormondroyd, J.; Hartog, J.P.D. The Theory of Dynamic Vibration Absorber. ASME J. Appl. Mech. 1928, 50, 9–22. [Google Scholar]
- Yang, C.; Li, D.; Cheng, L. Dynamic Vibration Absorbers for Vibration Control within a Frequency Band. J. Sound Vib. 2011, 330, 1582–1598. [Google Scholar] [CrossRef]
- Brock, J.E. A Note on the Damped Vibration Absorber. ASME J. Appl. Mech. 1946, 13, A284. [Google Scholar] [CrossRef]
- Sun, J.Q.; Jolly, M.R.; Norris, M.A. Passive, Adaptive and Active Tuned Vibration Absorbers-A Survey. ASME J. Vib. Acoust. 1995, 117, 234–242. [Google Scholar] [CrossRef]
- Inman, D.J. Engineering Vibration; Prentice-Hall: Hoboken, NJ, USA, 2001. [Google Scholar]
- McDaid, A.J.; Mace, B.R. A Self-Tuning Electromagnetic Vibration Absorber with Adaptive Shunt Electronics. Smart Mater. Struct. 2013, 22, 105013. [Google Scholar] [CrossRef]
- Gonzalez-Buelga, A.; Clare, L.R.; Neild, S.A.; Burrow, S.G.; Inman, D.J. An Electromagnetic Vibration Absorber with Harvesting and Tuning Capabilities. Struct. Control Health Monit. 2015, 22, 1359–1372. [Google Scholar] [CrossRef]
- Herold, S.; Mayer, D. Adaptive Piezoelectric Absorber for Active Vibration Control. Actuator 2016, 5, 7. [Google Scholar] [CrossRef]
- Herold, S.; Mayer, D.; Roglin, T. Design and Testing of a Piezoelectric Inertial Mass Actuator for Active Vibration Control. Vib. Eng. Technol. Mach. 2014, 23, 587–597. [Google Scholar] [CrossRef]
- Mani, Y.; Senthikumar, M. Shape Memory Alloy-Based Adaptive-Passive Dynamic Vibration Absorber for Vibration Control in Piping Application. J. Vib. Control 2015, 21, 1838–1847. [Google Scholar] [CrossRef]
- Kumbhar, S.B.; Chavan, S.P.; Gawade, S.S. Adaptive Tuned Vibration Absorber Based on Magnetorheological Elastomer-Shape Memory Alloy Composite. Mech. Syst. Signal Process. 2018, 100, 208–223. [Google Scholar] [CrossRef]
- Liao, G.J.; Gong, X.L.; Kang, C.J.; Xuan, S.H. The Design of an Active-Adaptive Tuned Vibration Absorber Based on Magnetorheological Elastomer and its Vibration Attenuation Performance. Smart Mater. Struct. 2011, 20, 075015. [Google Scholar] [CrossRef]
- Li, Y.; Li, J.; Tian, T.; Li, W. A Highly Adjustable Magnetorheological Elastomer Based Isolator for Applications of Real-Time Adaptive Control. Smart Mater. Struct. 2013, 22, 095020. [Google Scholar] [CrossRef]
- Komatsuzaki, T.; Iwata, Y. Design of a Real-Time Adaptively Tuned Dynamic Vibration Absorber with a Variable Stiffness Property Using Magnetorheological Elastomer. Shock Vib. 2015, 2015, 676508. [Google Scholar] [CrossRef]
- Sun, S.; Deng, H.; Yang, J.; Li, W.; Du, H.; Alici, G.; Nakano, M. An Adaptive Tuned Vibration Absorber Based On Multilayered MR Elastomers. Smart Mater. Struct. 2015, 24, 045045. [Google Scholar] [CrossRef]
- Xin, F.L.; Bai, X.X.; Qian, L.L. Principle, Modeling, and Control of a Magnetorheological Elastomer Dynamic Vibration Absorber for Powertrain Mount Systems of Automobiles. J. Intell. Mater. Syst. Struct. 2017, 3, 2239–2254. [Google Scholar] [CrossRef]
- Bastola, A.K.; Li, L. A New Type of Vibration Isolator Based on Magnetorheological Elastomer. Mater. Des. 2018, 157, 431–436. [Google Scholar] [CrossRef]
- Bian, Y.; Liang, X.; Gao, Z. Vibration Reduction for a Flexible Arm Using Magnetorheological Elastomer Vibration Absorber. Shock. Vib. 2018, 2018, 9723538. [Google Scholar] [CrossRef]
- Yang, J.; Sun, S.S.; Ning, D.; Li, Z.; Deng, L.; Christie, M.; Du, H.; Zhang, S.W.; Li, W.H. Development and Evaluation of a Highly Adaptive MRF-Based Absorber with a Large Effective Frequency Range. Smart Mater. Struct. 2019, 28, 105003. [Google Scholar] [CrossRef]
- Komatsuzaki, T.; Inoue, T.; Iwata, Y. Experimental Investigation of an Adaptively Tuned Dynamic Absorber Incorporating Magnetorheological Elastomer with Self-Sensing Property. Exp. Mech. 2016, 56, 871–880. [Google Scholar] [CrossRef]
- Deng, H.X.; Gong, X.L. Application of Magnetorheological Elastomer to Vibration Absorber. Commun. Nonlinear Sci. Numer. Simul. 2008, 13, 1938–1947. [Google Scholar] [CrossRef]
- Gao, P.; Liu, H.; Xiang, C.; Yan, P.; Mahmoud, T. A New Magnetorheological Elastomer Torsional Vibration Absorber: Structural Design and Performance Test. Mech. Sci. 2021, 12, 321–332. [Google Scholar] [CrossRef]
- Sinko, R.; Karnes, M.; Koo, J.H.; Kim, Y.K.; Kim, K.S. Design and Test of an Adaptive Vibration Absorber Based on Magnetorheological Elastomers and a Hybrid Electromagnet. J. Intell. Mater. Syst. Struct. 2012, 24, 803–812. [Google Scholar] [CrossRef]
- Xu, Z.; Gong, X.; Liao, G.; Chen, X. An Active-Damping-Compensated Magnetorheological Elastomer Adaptive Tuned Vibration Absorber. J. Intell. Mater. Syst. Struct. 2010, 21, 1039–1047. [Google Scholar] [CrossRef]
- Liu, G.; Lu, K.; Zou, D.; Xie, Z.; Rao, Z.; Ta, N. Development of a Semi-Active Dynamic Vibration Absorber for Longitutdinal Vibration of Propulsion Shaft System Based on Magnetorheological Elastomer. Smart Mater. Struct. 2017, 26, 075009. [Google Scholar] [CrossRef]
- Deng, H.X.; Gong, X.L. Adaptive Tuned Vibration Absorber Based on Magnetorheological Elastomers. J. Intell. Mater. Syst. Struct. 2007, 18, 1205–1210. [Google Scholar] [CrossRef]
- Komatsuzaki, T.; Inoue, T.; Terashima, O. A Broadband Frequency-Tunable Dynamic Absorber for the Vibration Control of Structures. J. Phys. Conf. Ser. 2016, 744, 012167. [Google Scholar] [CrossRef]
- Komatsuzaki, T.; Inoue, T.; Terashima, O. Broadband Vibration Control of a Structure by Using a Magnetorheological Elastomer-Based Tuned Dynamic Absorber. Mechatronics 2016, 40, 128–136. [Google Scholar] [CrossRef]
- Sun, S.; Chen, Y.; Yang, Y.; Tian, T.; Deng, H. The Development of an Adaptive Tuned Magnetorheological Elastomer Absorber Working in Squeeze Mode. Smart Mater. Struct. 2014, 23, 075009. [Google Scholar] [CrossRef]
- Deng, H.X.; Gong, X.L.; Wang, L.H. Development of an Adaptive Tuned Vibration Absorber with Magnetorheological Elastomer. Smart Mater. Struct. 2006, 15, N111–N116. [Google Scholar] [CrossRef]
- Jeong, U.C. Application of Adaptive Tuned Magneto-Rheological Elastomer for Vibration Reduction of a Plate by a Variable-Unbalance Excitation. Appl. Sci. 2020, 10, 3934. [Google Scholar] [CrossRef]
- Samal, S.; Skodova, M.; Abate, L.; Blanco, I. Magneto-Rheological Elastomer Composite. A Review. Appl. Sci. 2020, 10, 4899. [Google Scholar] [CrossRef]
- Samal, S.; Vlach, J.; Kavan, P. Improved Mechanical Properties of Magneto Rheological Elastomeric Composite with Isotropic Iron Filler Distribution. Cienc. Tecnol. dos Mater. 2016, 28, 155–161. [Google Scholar] [CrossRef]
- Hartzell, C.M.; Choi, Y.T.; Wereley, N.M.; Leps, T.J. Performance of a Magnetorheological Fluid-Based Robotic End Effector. Smart Mater. Struct. 2019, 28, 035030. [Google Scholar] [CrossRef]
- Choi, Y.T.; Hartzell, C.M.; Leps, T.; Wereley, N.M. Gripping Characteristics of an Electromagnetically Activated Magnetorheological Fluid-Based Gripper. AIP Adv. 2018, 8, 056701. [Google Scholar] [CrossRef]
- Xie, L.; Choi, Y.T.; Liao, C.R.; Wereley, N.M. Long Term Stability of Magnetorheological Fluids Using High Viscosity Linear Polysiloxane Carrier Fluids. Smart Mater. Struct. 2016, 25, 075006. [Google Scholar] [CrossRef]
- Choi, Y.T.; Xie, L.; Wereley, N.M. Testing and Analysis of Magnetorheological Fluid Sedimentation in a Column Using a Vertical Axis Inductance Monitoring System. Smart Mater. Struct. 2016, 25, 04LT01. [Google Scholar] [CrossRef]
- Ahuré-Powell, L.A.; Choi, Y.T.; Hu, W.; Wereley, N.M. Nonlinear Modeling of Adaptive Magnetorheological Landing Gear Dampers under Impact Conditions. Smart Mater. Struct. 2016, 25, 115011. [Google Scholar] [CrossRef]
- Ladipo, I.; Fadly, J.D.; Faris, W.F. Characterization of Magnetorheological Elastomer (MRE) Engine Mounts. Mater. Today Proc. 2016, 3, 411–418. [Google Scholar] [CrossRef]
- Yarra, S.; Gordaninejad, F.; Behrooz, M. Performance of a Large-Scale Magnetorheological Elastomer Based Vibration Isolator for Highway Bridges. J. Intell. Mater. Syst. Struct. 2018, 29, 3890–3901. [Google Scholar] [CrossRef]
- Yu, Y.; Royel, S.; Li, J.; Li, Y.; Ha, Q. Magnetorheological Elastomer Based Isolator for Earthquake Response Mitigation on Building Structures: Modeling and Second-Order Sliding Mode Control. Earthq. Struct. 2016, 11, 943–966. [Google Scholar] [CrossRef]
- Li, Y.; Li, J.; Li, W.; Samali, B. Development and Characterization of a Magnetorheological Elastomer Based Adaptive Seismic Isolator. Smart Mater. Struct. 2013, 22, 035005. [Google Scholar] [CrossRef]
- Ismail, R.; Ibrahim, A.; Hamid, H.A. A Review of Magnetorheoogical Elastomers: Characterization Properties for Seismic Protection. In Proceedings of the International Civil and Infrastructure Engineering Conference 2013; Springer: Singapore, 2014; pp. 237–248. [Google Scholar] [CrossRef]
- Eem, S.H.; Jung, H.J.; Koo, J.H. Application of MR Elastomers for Improving Seismic Protection of Based-Isolated Structures. IEEE Trans. Magn. 2011, 47, 2901–2904. [Google Scholar] [CrossRef]
- Bastola, A.K.; Paudel, M.; Li, L. Magnetic Circuit Analysis to Obtain the Magnetic Permeability of Magnetorheological Elastomers. J. Intell. Mater. Syst. Struct. 2018, 29, 2946–2953. [Google Scholar] [CrossRef]
- Brigley, M.; Choi, Y.T.; Wereley, N.M. Experimental and Theoretical Development of Multiple Fluid Mode Magnetorheological Isolators. J. Guid. Control Dyn. 2008, 31, 449–459. [Google Scholar] [CrossRef]
- Boczkowska, A.; Awietjan, S. Microstructure and Properties of Magnetorheological Elastomers. In Advanced Elastomers; IntechOpen: London, UK, 2012; Chapter 6. [Google Scholar] [CrossRef]
- Abdul Aziz, S.A.; Mazlan, S.A.; Ismall, N.I.; Choi, S.-B.; Yunus, N.A.B. An Enhancement of Mechanical and Rheological Properties of Magnetorheological Elastomer with Multiwall Carbon Nanotubes. J. Intell. Mater. Syst. Struct. 2017, 28, 3127–3138. [Google Scholar] [CrossRef]
- Hapipi, N.; Mazlan, S.A.; Abdul Aziz, S.A.; Ubaidillah, U.; Mohamad, N.; Yazid, I.I.M.; Choi, S.-B. Effect of Curing Current on Stiffness and Damping Properties of Magnetorheological Elastomers. Int. J. Sustain. Transp. 2018, 1, 51–58. [Google Scholar] [CrossRef]
- Samal, S.; Blanco, I. Investigation of Dispersion, Interfacial Adhesion of Isoltropic and Anisotropic Filler in Polymer Composite. Appl. Sci. 2021, 11, 8561. [Google Scholar] [CrossRef]
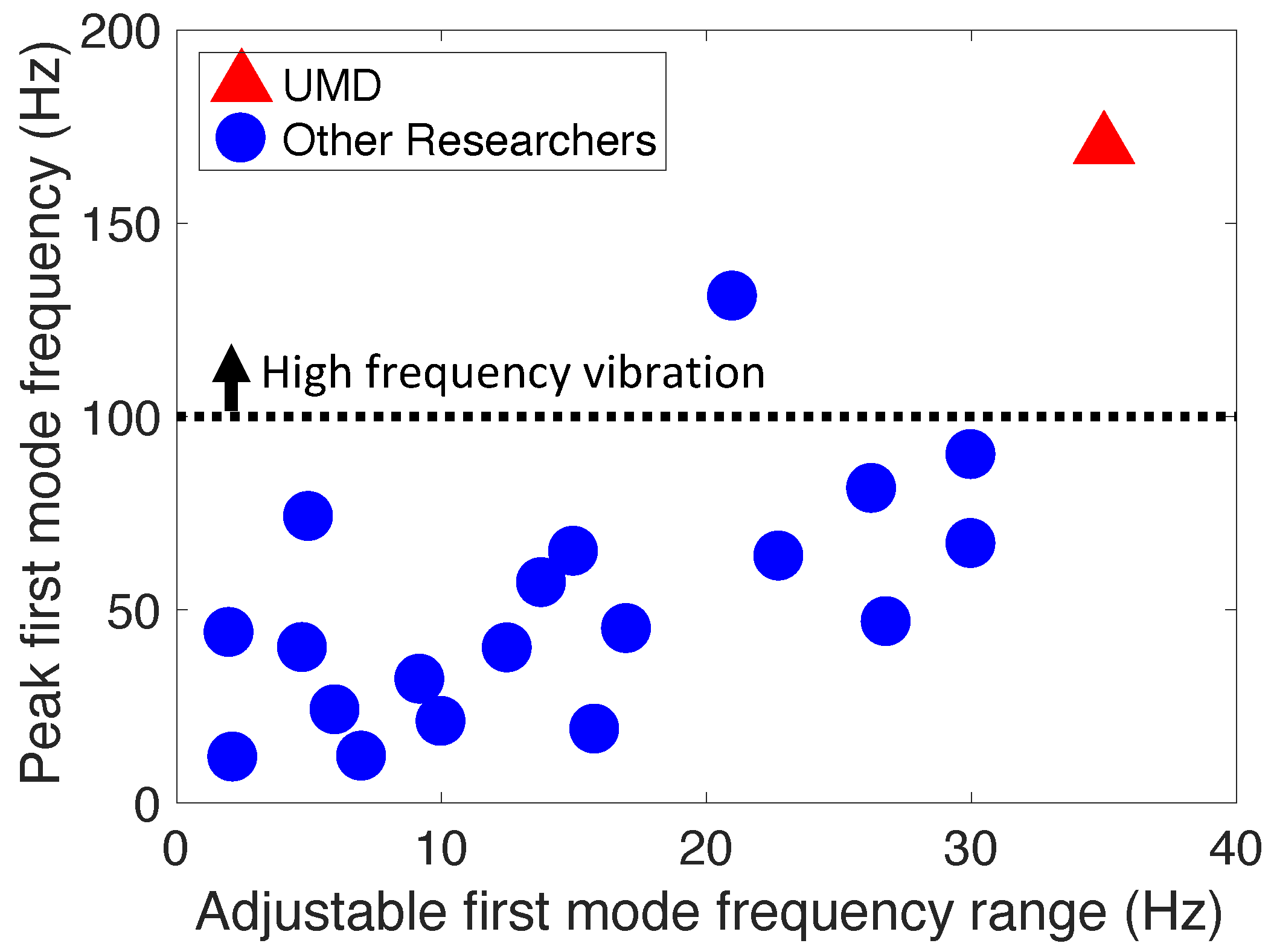

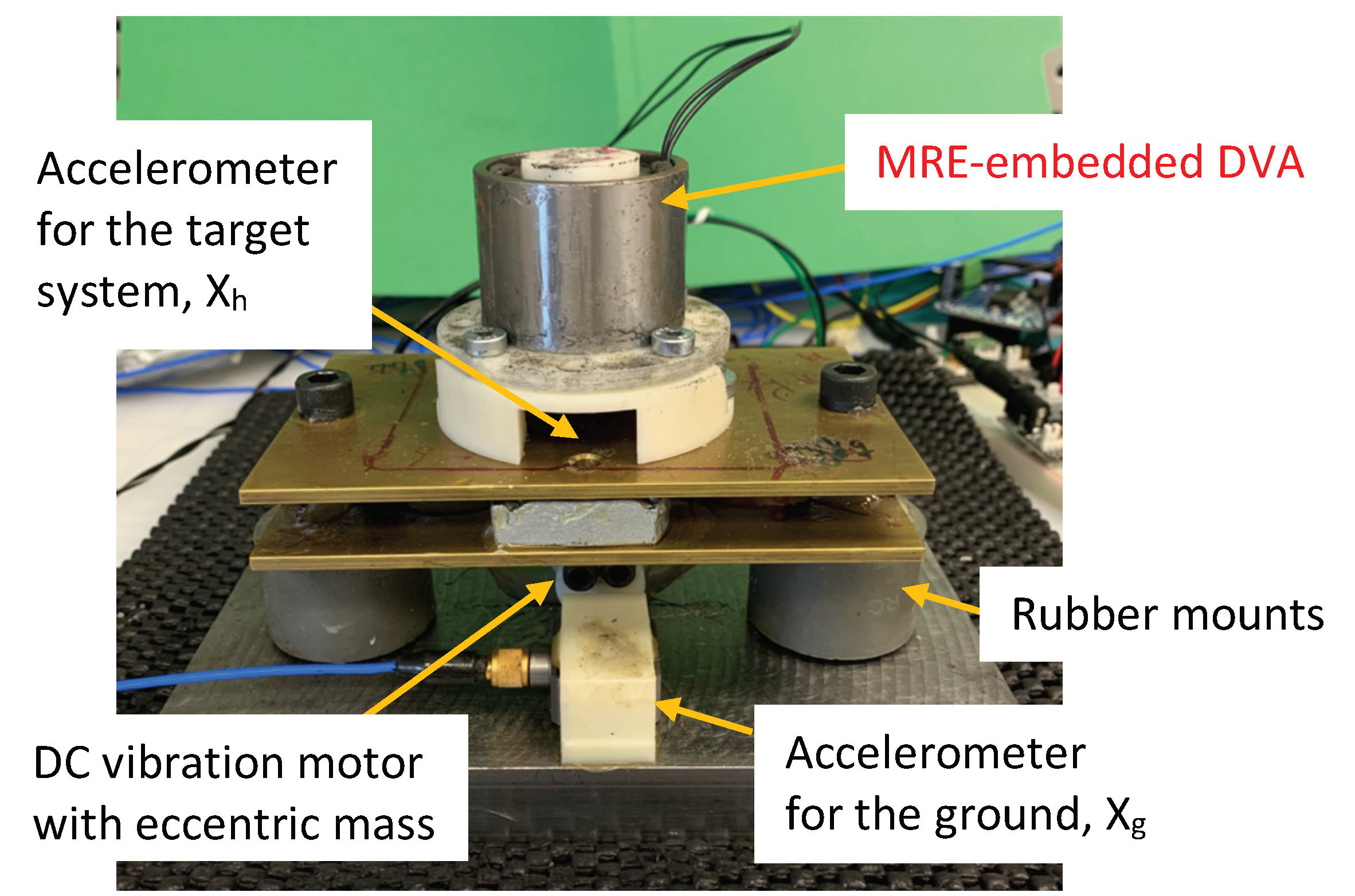
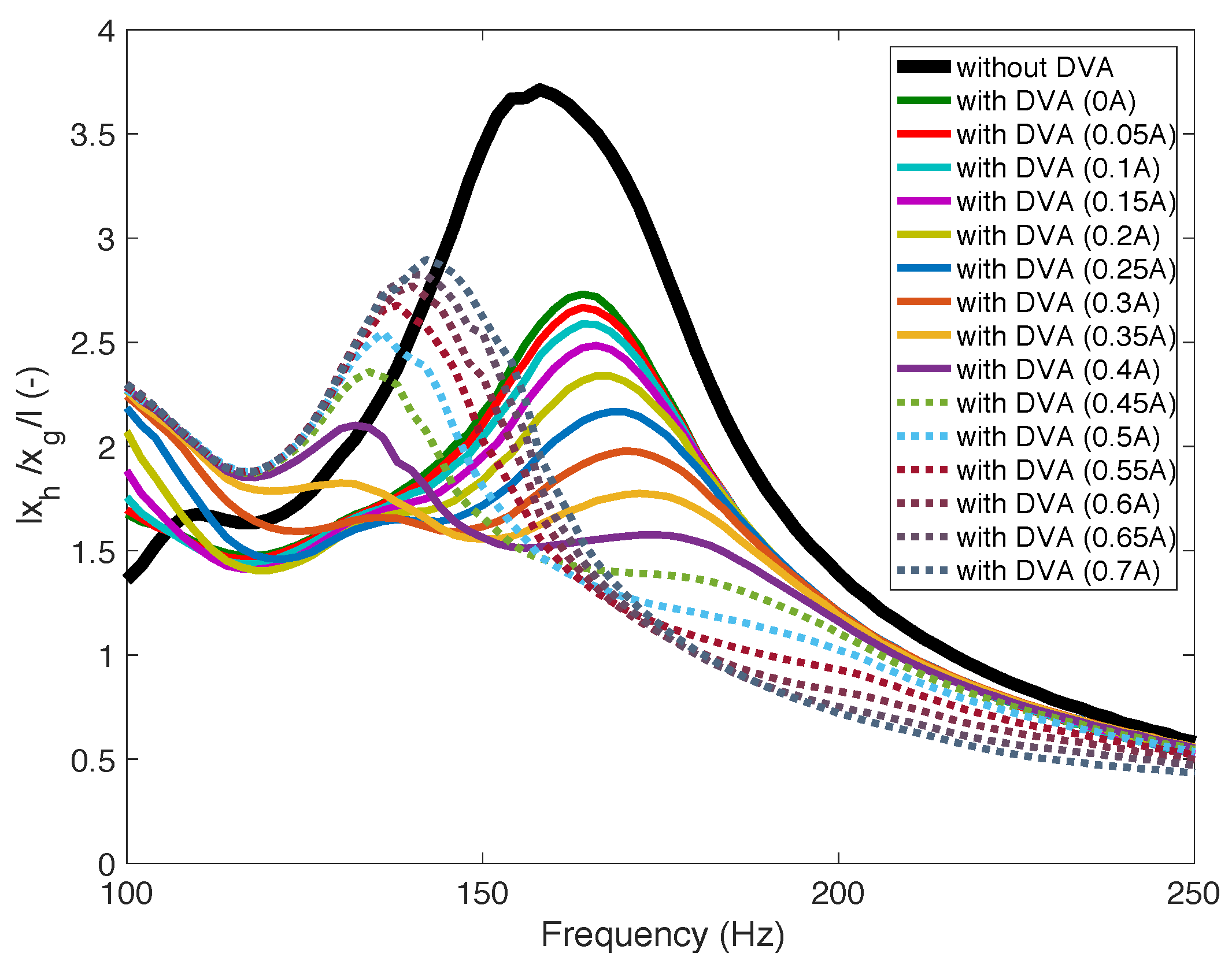
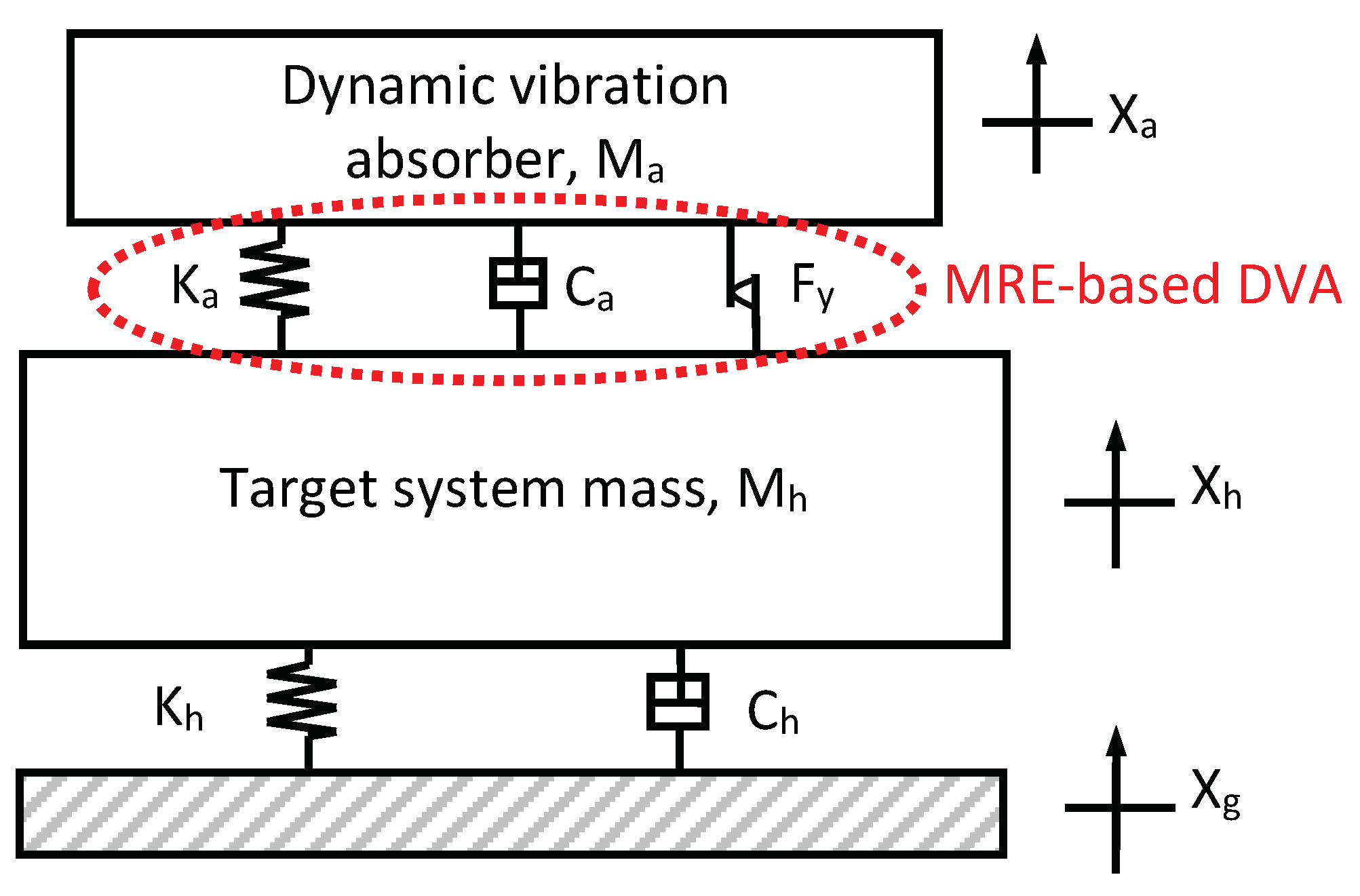
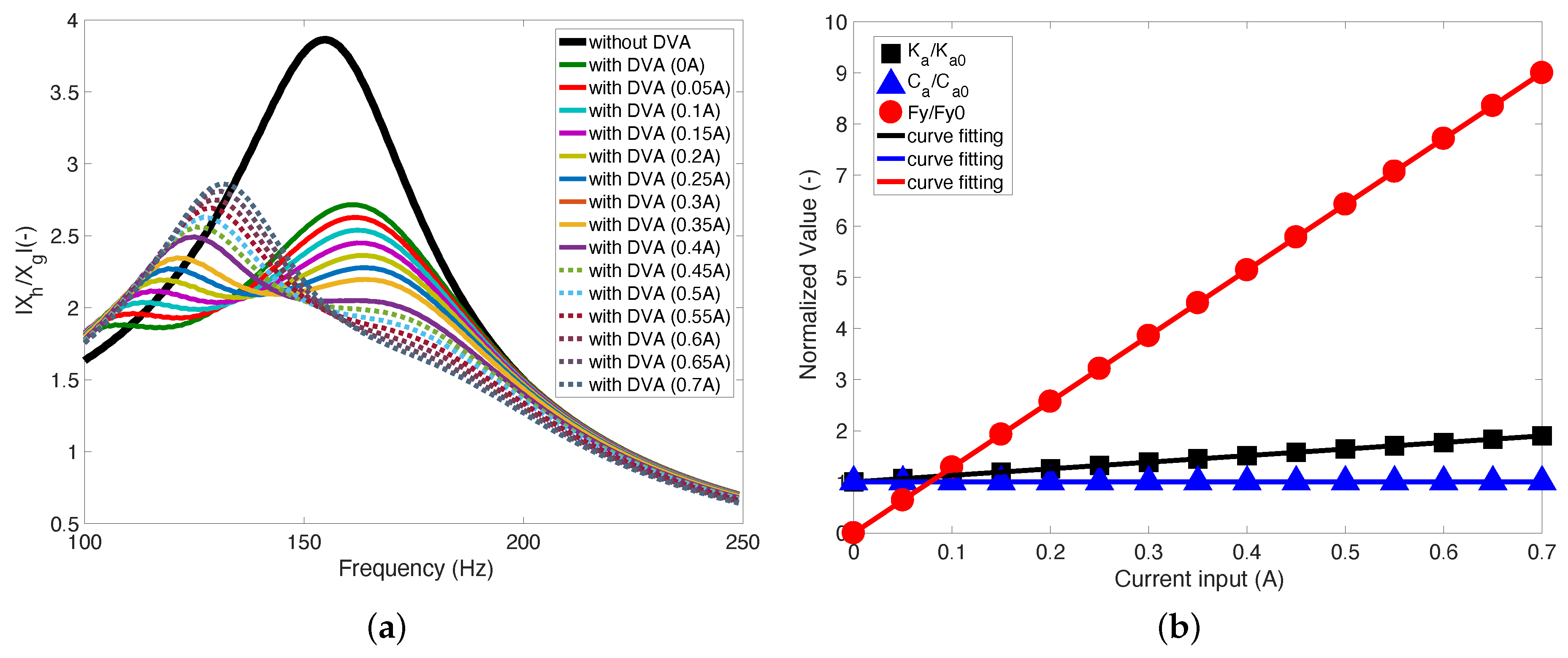


| Peak First Mode Frequency of a Target System (Hz) | Adjustable First Mode Frequency Range of a Target System (Hz) | References |
|---|---|---|
| 11.77 | 2.14 | [20] |
| 12 | 7 | [21] |
| 19 | 15.8 | [17] |
| 21 | 10 | [14] |
| 24 | 6 | [22] |
| 31.9 | 9.2 | [18] |
| 40 | 12.5 | [23] |
| 40.09 | 4.77 | [24] |
| 44 | 2 | [25] |
| 45 | 17 | [26] |
| 46.8 | 26.8 | [16] |
| 56.88 | 13.79 | [27] |
| 63.75 | 22.75 | [28] |
| 65 | 15 | [29] |
| 65 | 15 | [30] |
| 67 | 30 | [31] |
| 74 | 5 | [13] |
| 81.25 | 26.25 | [32] |
| 90 | 30 | [14] |
| 131 | 21 | [33] |
| Variables | Values |
|---|---|
| Mass of the target system, | 1027 g |
| Stiffness of the target system, | 1005 kN/m |
| Viscous damping of the target system, | 274.3 N · s/m |
| Moving mass of the MRE-DVA, | 122 g |
| Stiffness of the MRE-DVA at no current input, | 60.9 kN/m |
| Viscous damping of the MRE-DVA at no current input, | 34.5 N · s/m |
| Coulomb friction damping force (i.e., yield force), | 0.26 N |
| of the MRE-DVA at a current input of 0.05 A |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Choi, Y.; Wereley, N.M. Vibration Isolation Performance of an Adaptive Magnetorheological Elastomer-Based Dynamic Vibration Absorber. Actuators 2022, 11, 157. https://doi.org/10.3390/act11060157
Choi Y, Wereley NM. Vibration Isolation Performance of an Adaptive Magnetorheological Elastomer-Based Dynamic Vibration Absorber. Actuators. 2022; 11(6):157. https://doi.org/10.3390/act11060157
Chicago/Turabian StyleChoi, Young, and Norman M. Wereley. 2022. "Vibration Isolation Performance of an Adaptive Magnetorheological Elastomer-Based Dynamic Vibration Absorber" Actuators 11, no. 6: 157. https://doi.org/10.3390/act11060157
APA StyleChoi, Y., & Wereley, N. M. (2022). Vibration Isolation Performance of an Adaptive Magnetorheological Elastomer-Based Dynamic Vibration Absorber. Actuators, 11(6), 157. https://doi.org/10.3390/act11060157







