Design and Experiment Evaluation of Load Distribution on the Dual Motors in Cam-Based Variable Stiffness Actuator with Helping Mode
Abstract
:1. Introduction
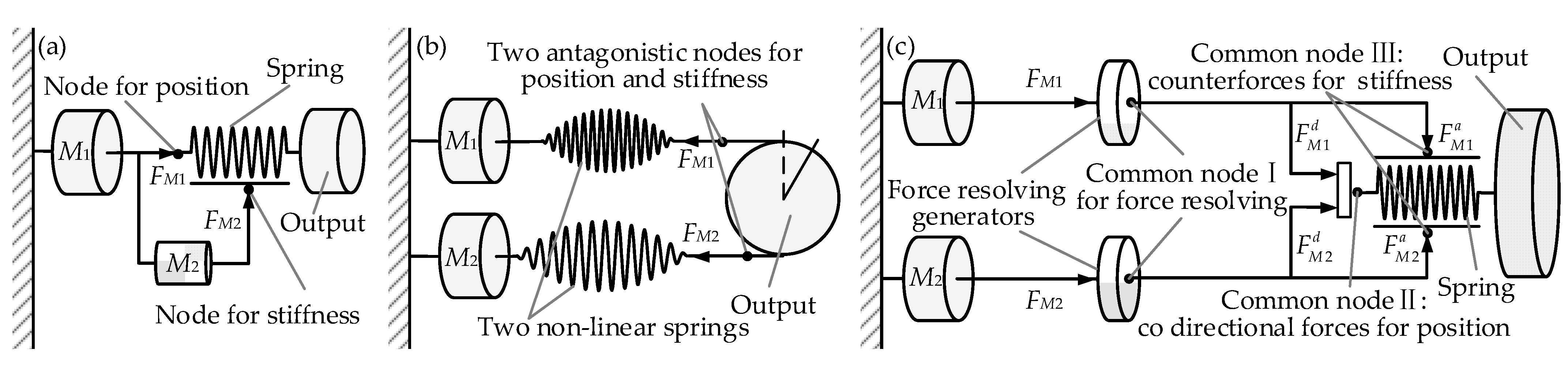
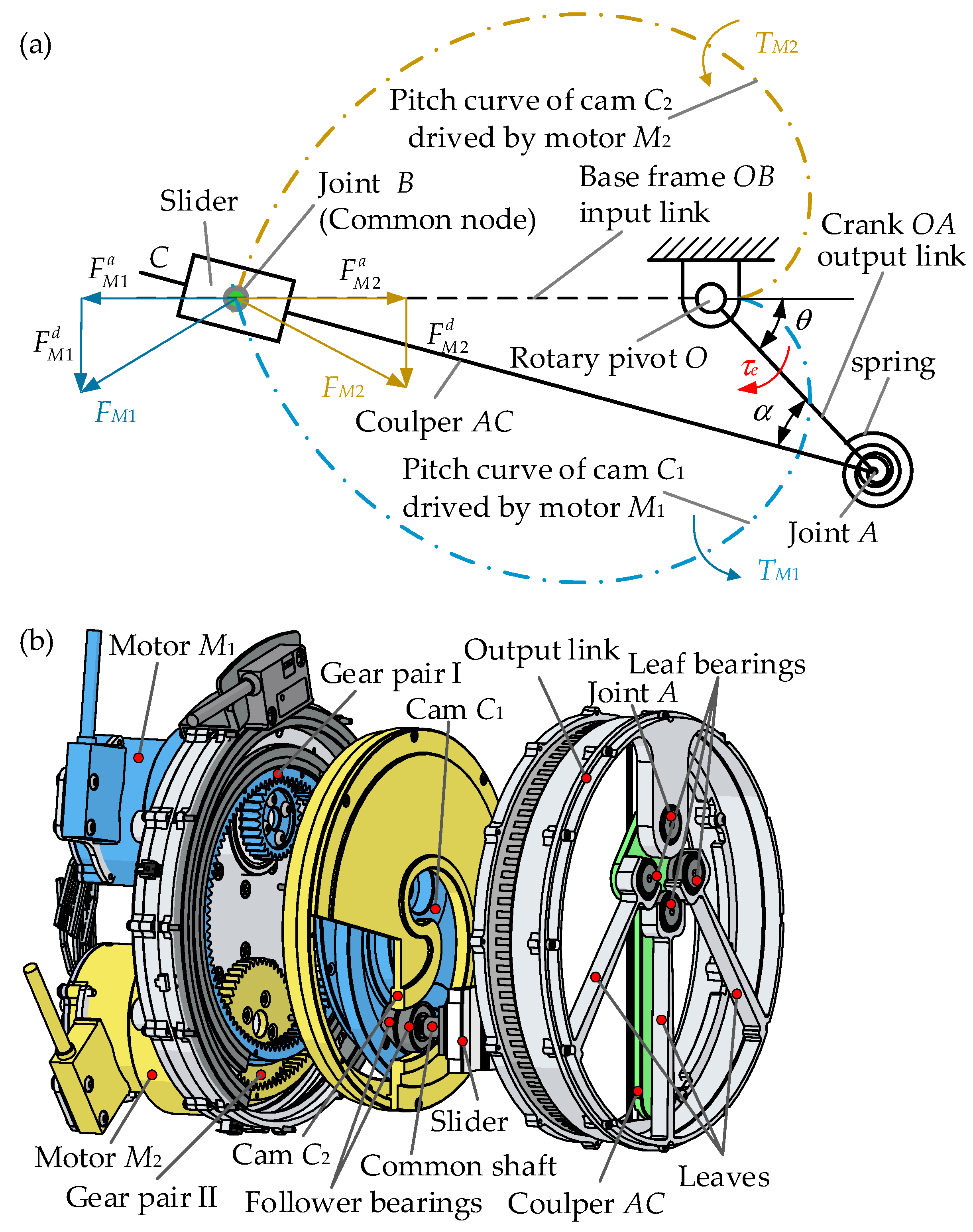
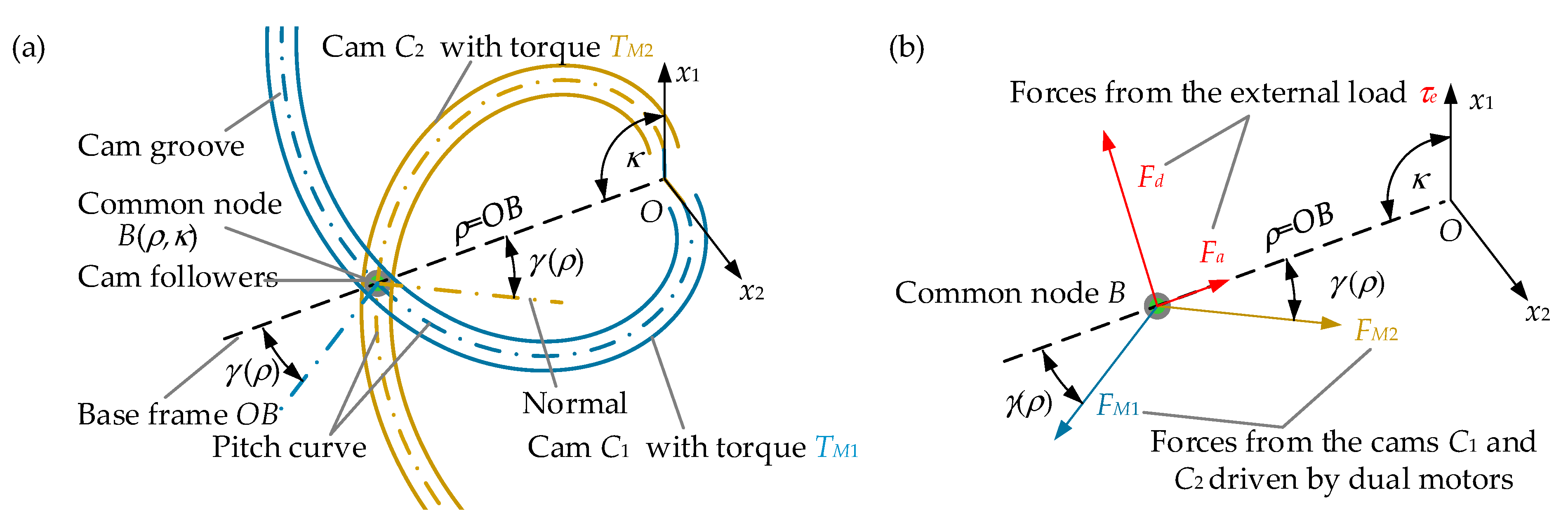
- Antagonistic action for stiffness adjustment, cooperative support for the external load. The VSA shares a common node, as shown in Figure 1c. The force resolving generators are two symmetrical differential cams. On this node, the counter-force of the stiffness adjustment is balanced by the difference of the dual cams’ force components in the joints’ radial direction; the external load is supported by the sum of the cams’ force components in the joints’ tangential direction.
- Load distribution with a small difference for a higher-level helping mode. The ratio between stiffness adjustment counter-force and output counter-force in the crank-slider mechanism can be analytically expressed. Regarding this ratio, the pressure angle of the cam pitch curve is minimized to reduce the load distribution difference on the dual motors.
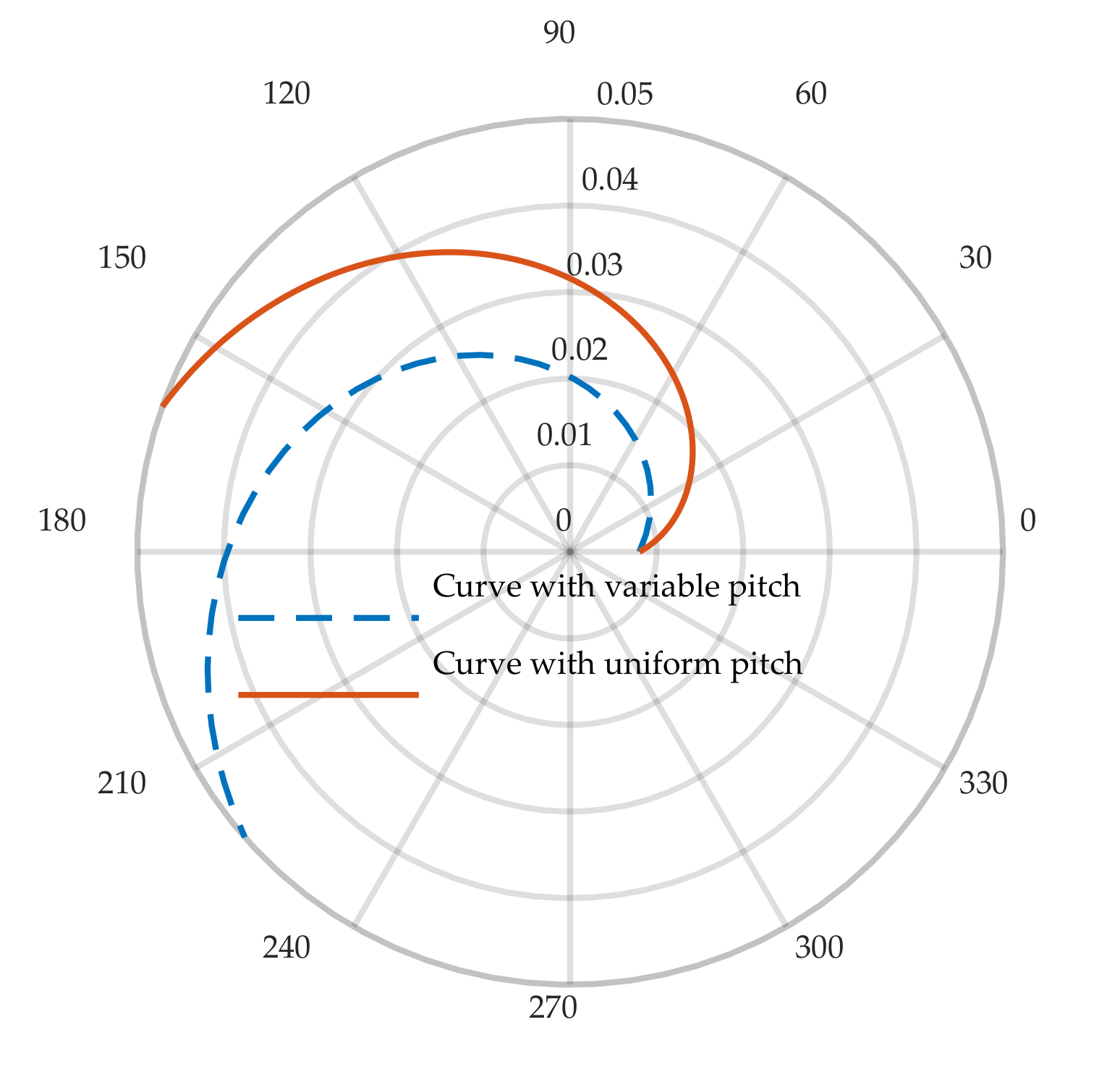
- Reasonable tradeoff design. Different from the strong constraint curve (such as Archimedes spiral), the pressure angle of the pitch curve can be adjusted arbitrarily to meet different design requirements and constraints, such as stiffness adjustment speed, cam local load, curvature radius of the pitch curve and load distribution. A well tradeoff can be made.
2. Principle and Mechanical Design of the Cam-Based VSA with Helping Mode
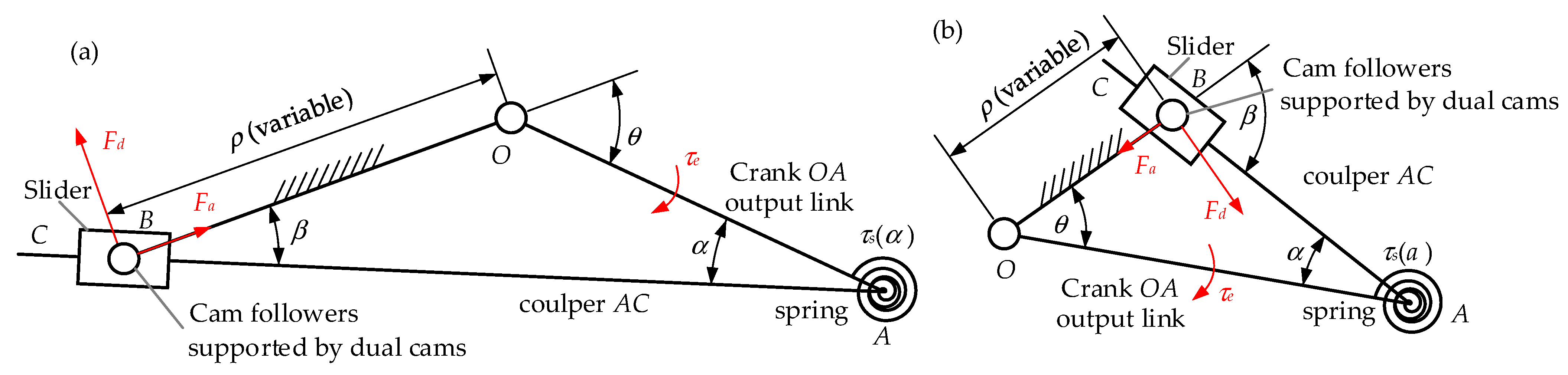
3. The Load Distribution Modeling in a Polar Coordinate System
4. Synthetic Method of the Cam Pitch Curve for an Optimal Load Distribution and Higher-Level Helping Mode
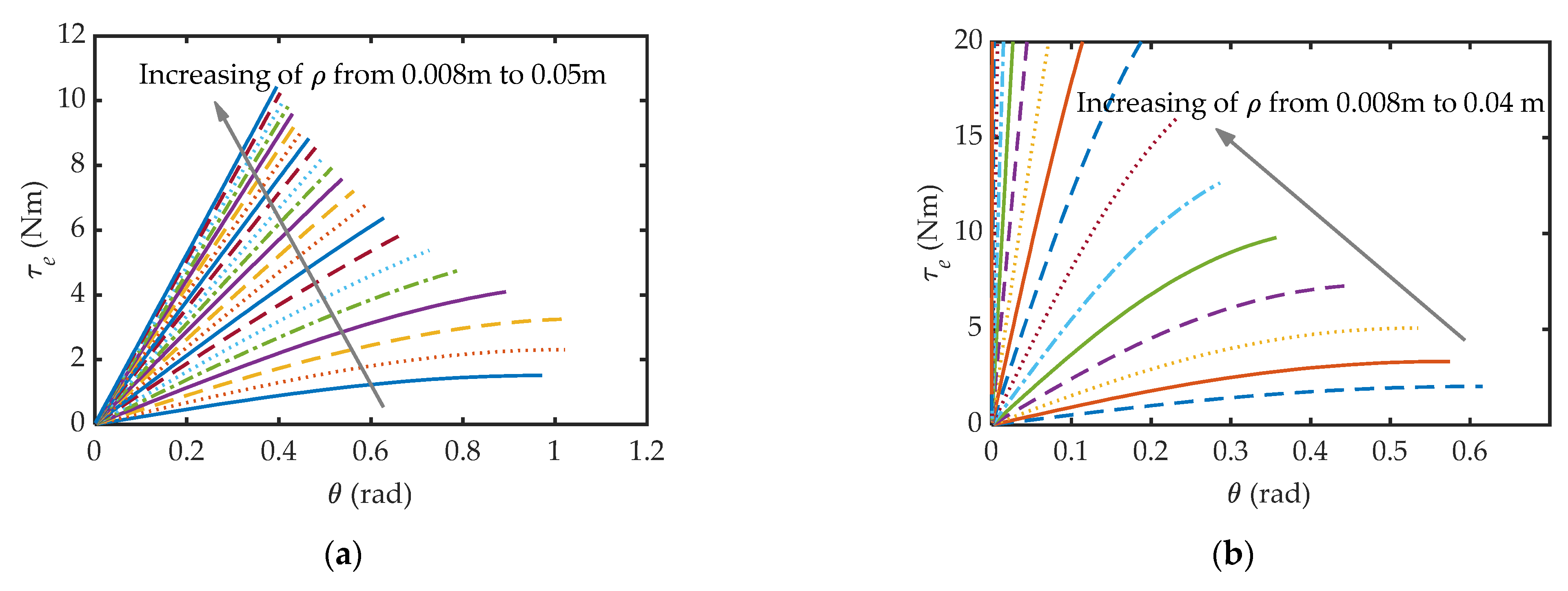
4.1. Characteristic Analysis of Stiffness Adjustment Module
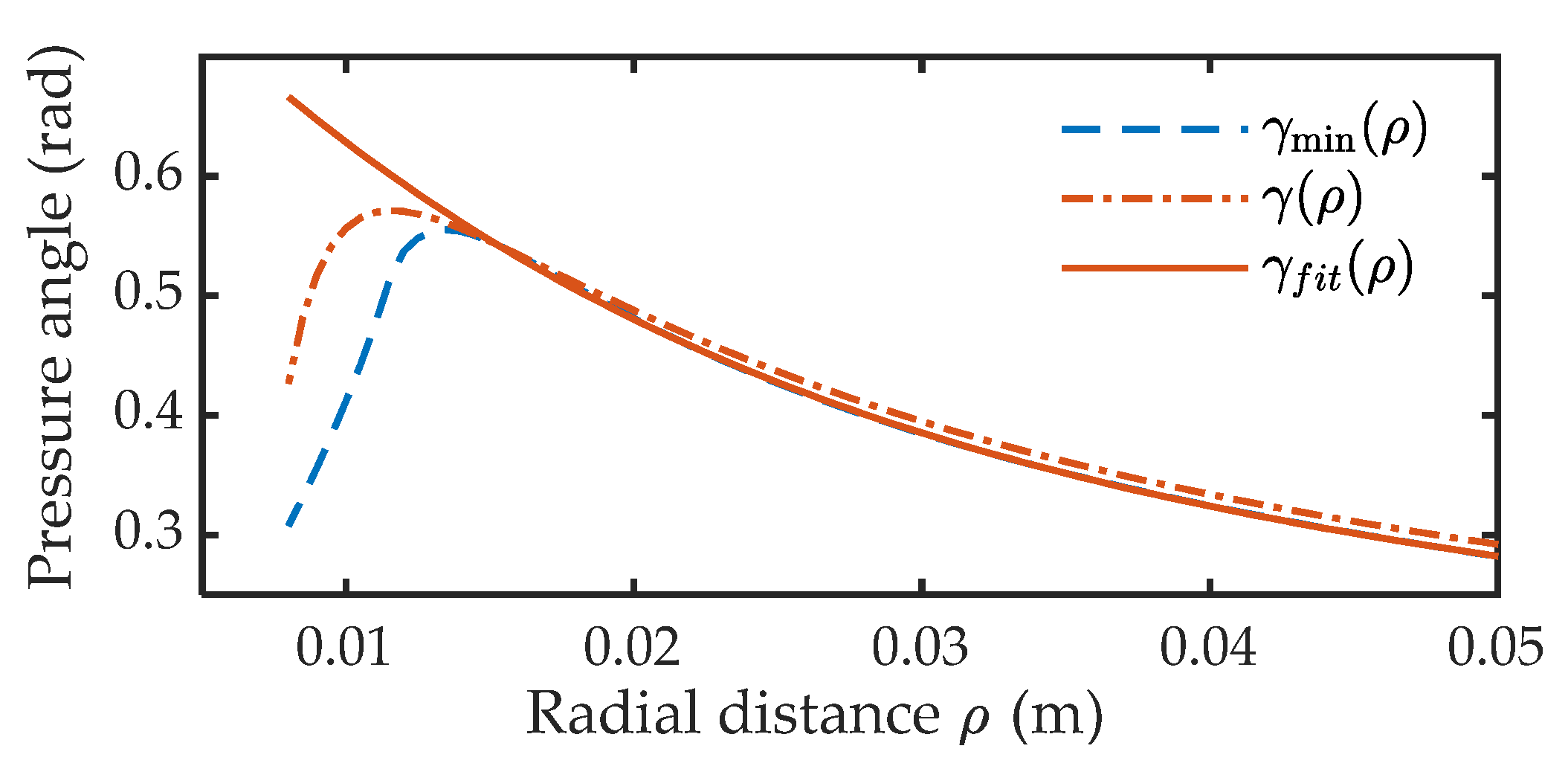
4.2. Numerical Synthesis of the Pressure Angle of the Cam Pitch Curve
4.2.1. Minimum Pressure Angle Constrained by Bearing’s Rated Load
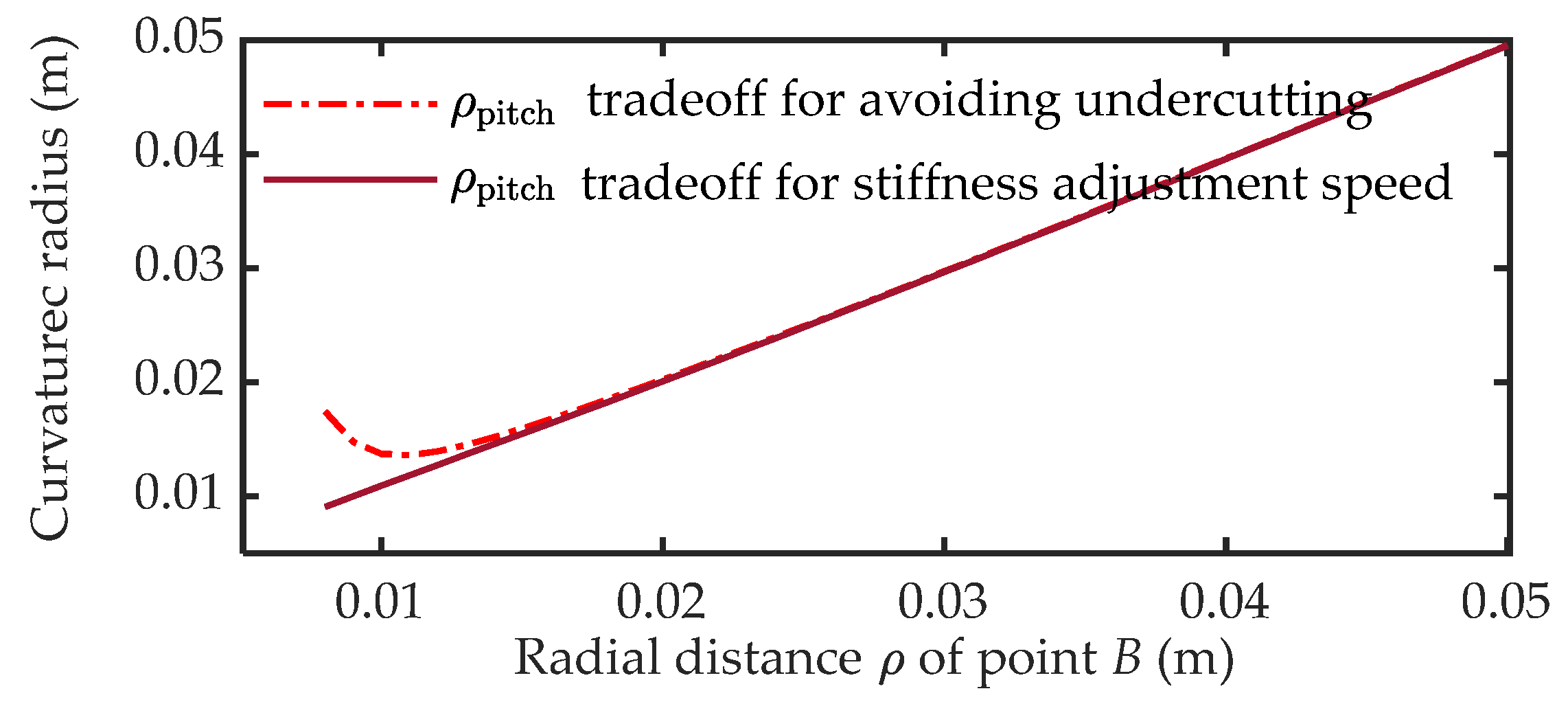
4.2.2. Tradeoff for Improving Stiffness Adjustment Speed Locally
4.2.3. Tradeoff for Avoiding Undercutting
4.3. Comparative Analysis of Design Result
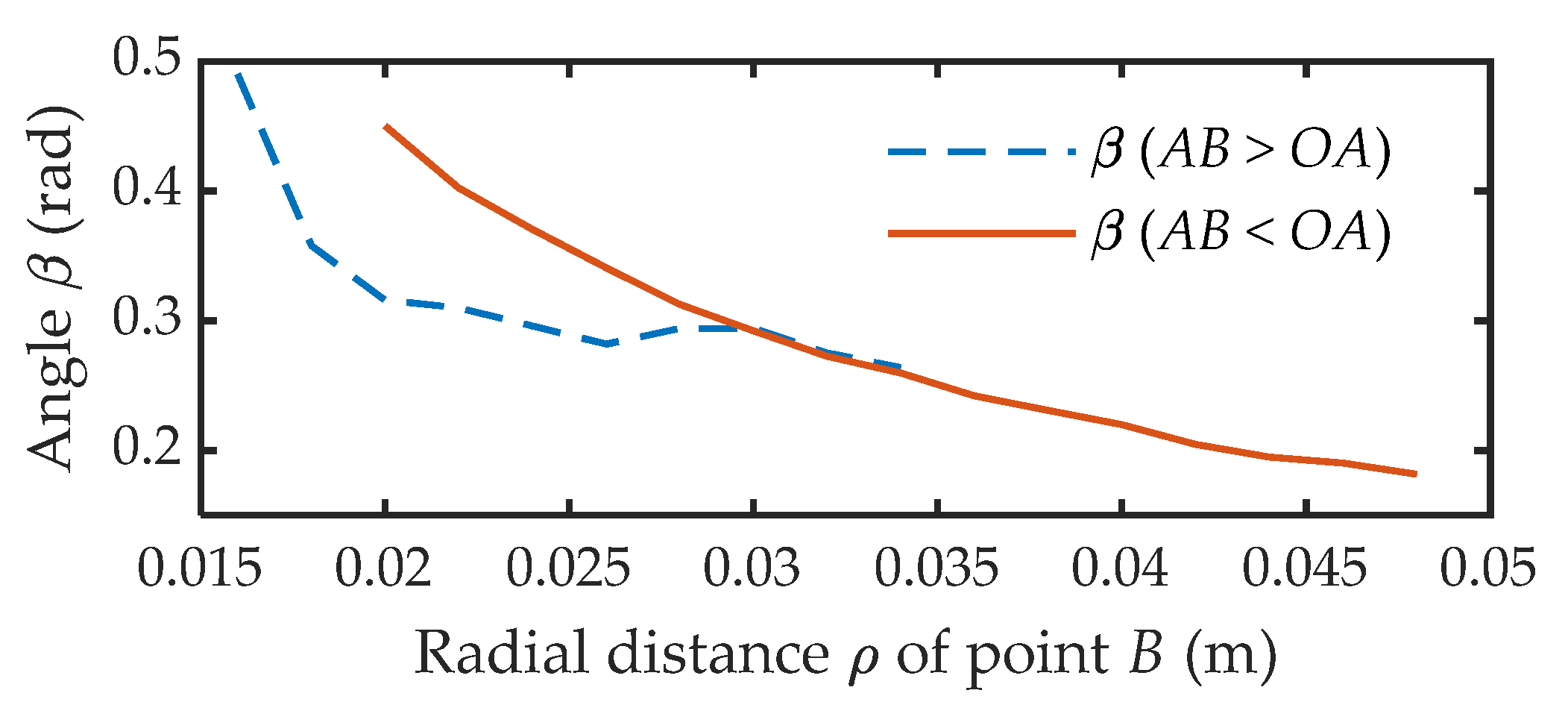
4.3.1. Analysis of Pressure Angle
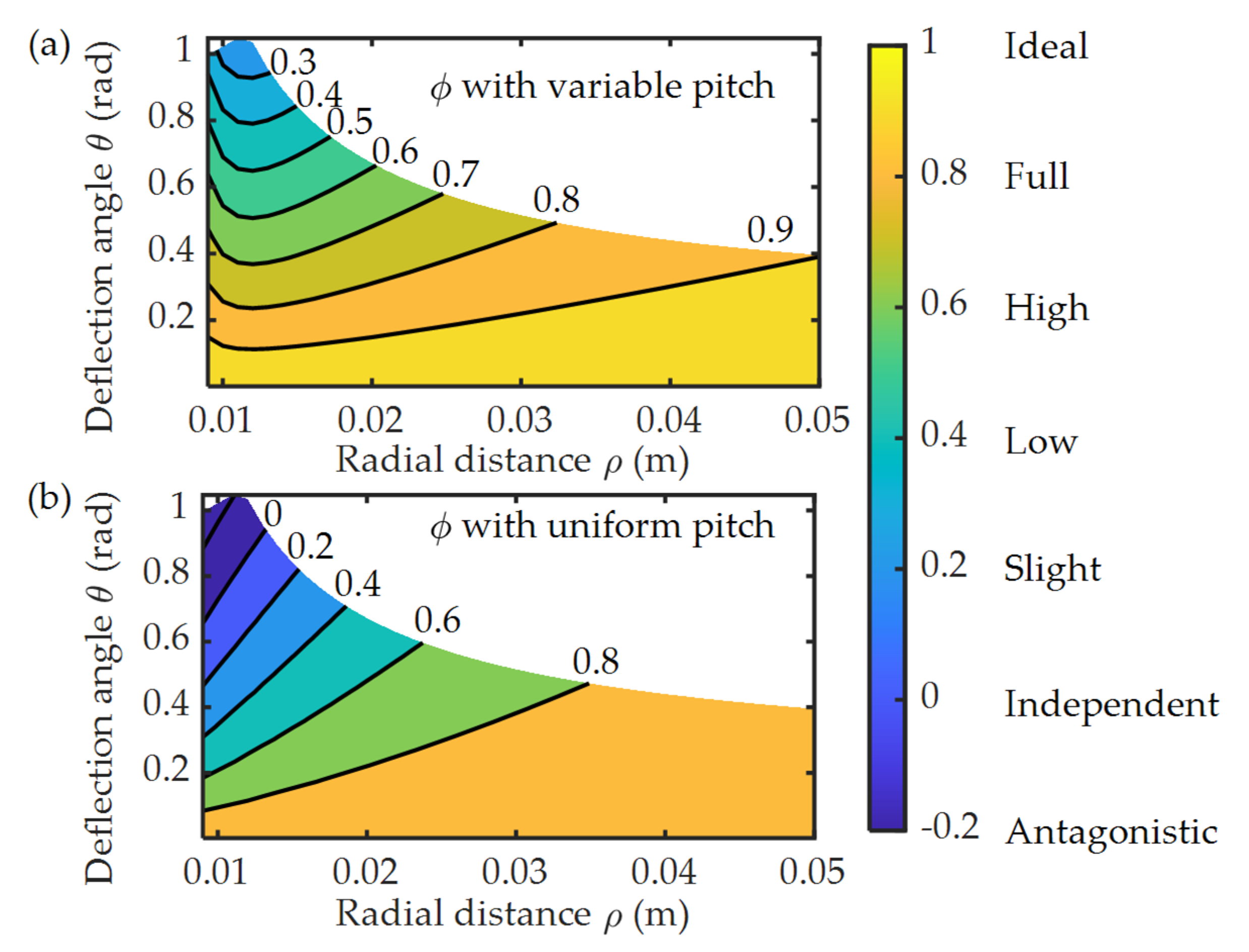
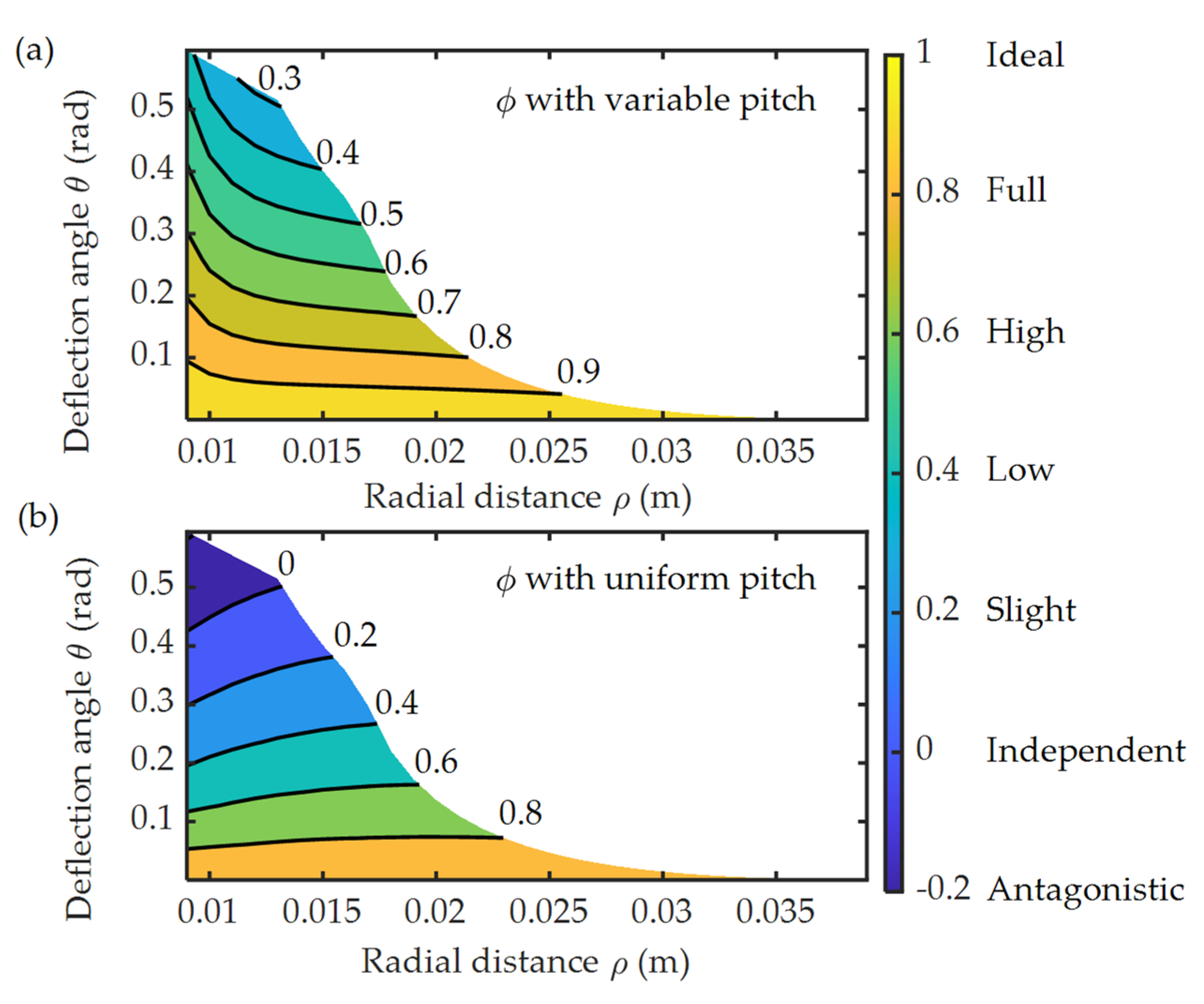
4.3.2. Design Results Analysis of the Load Ratio
- Changing cam followers with higher rated load [Fb]. With the same constraints in this paper, a smaller pressure angle γ (ρ) can be realized according to the rated load constraint equations in Equation (8).
5. Load Ratio Validation by Experiment
5.1. Reconfigurable Prototype and Test Platform
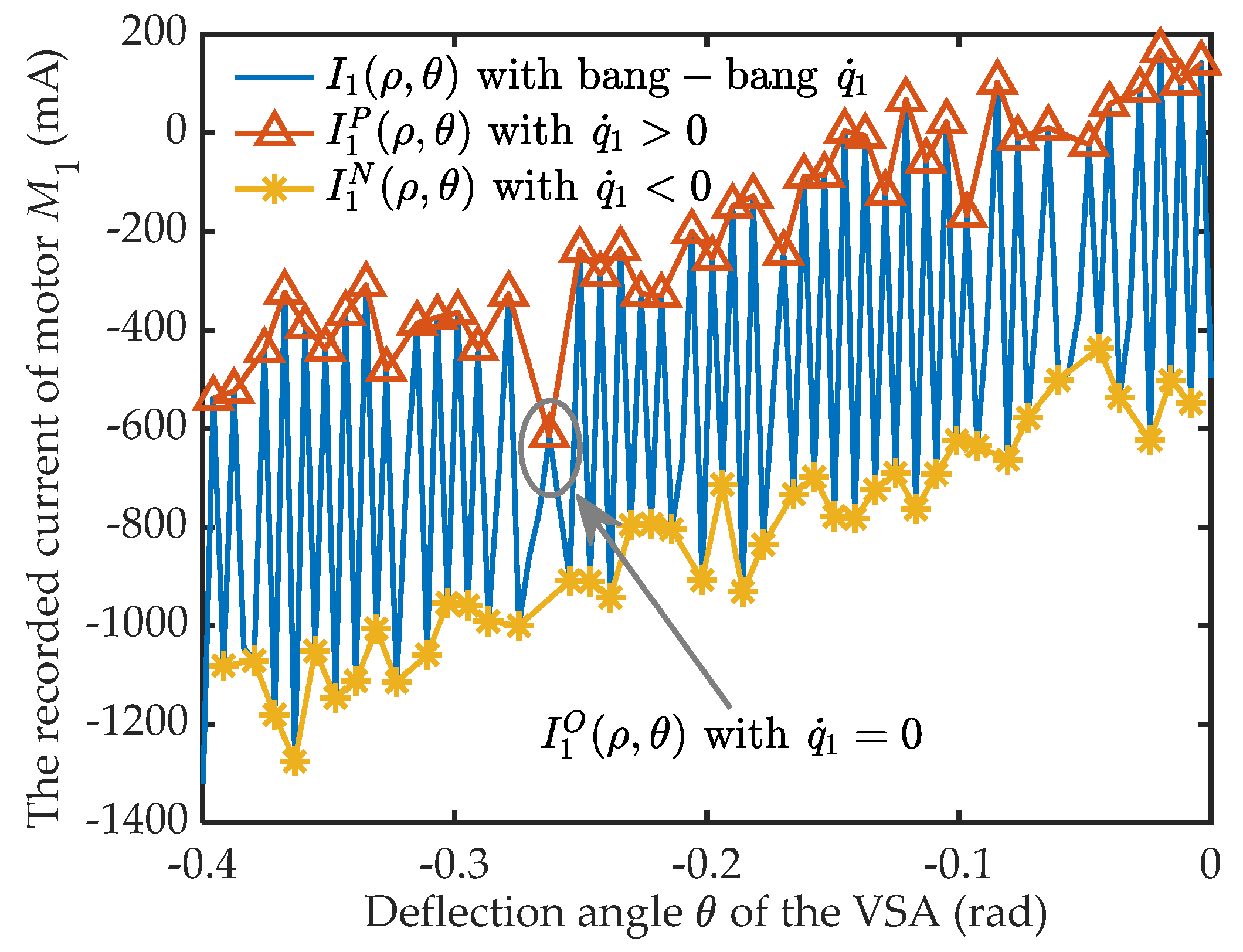
5.2. Dealing Method of Current Data with Bang-Bang Friction
5.3. Results of the Experiment
5.3.1. Measurement Range Choosing
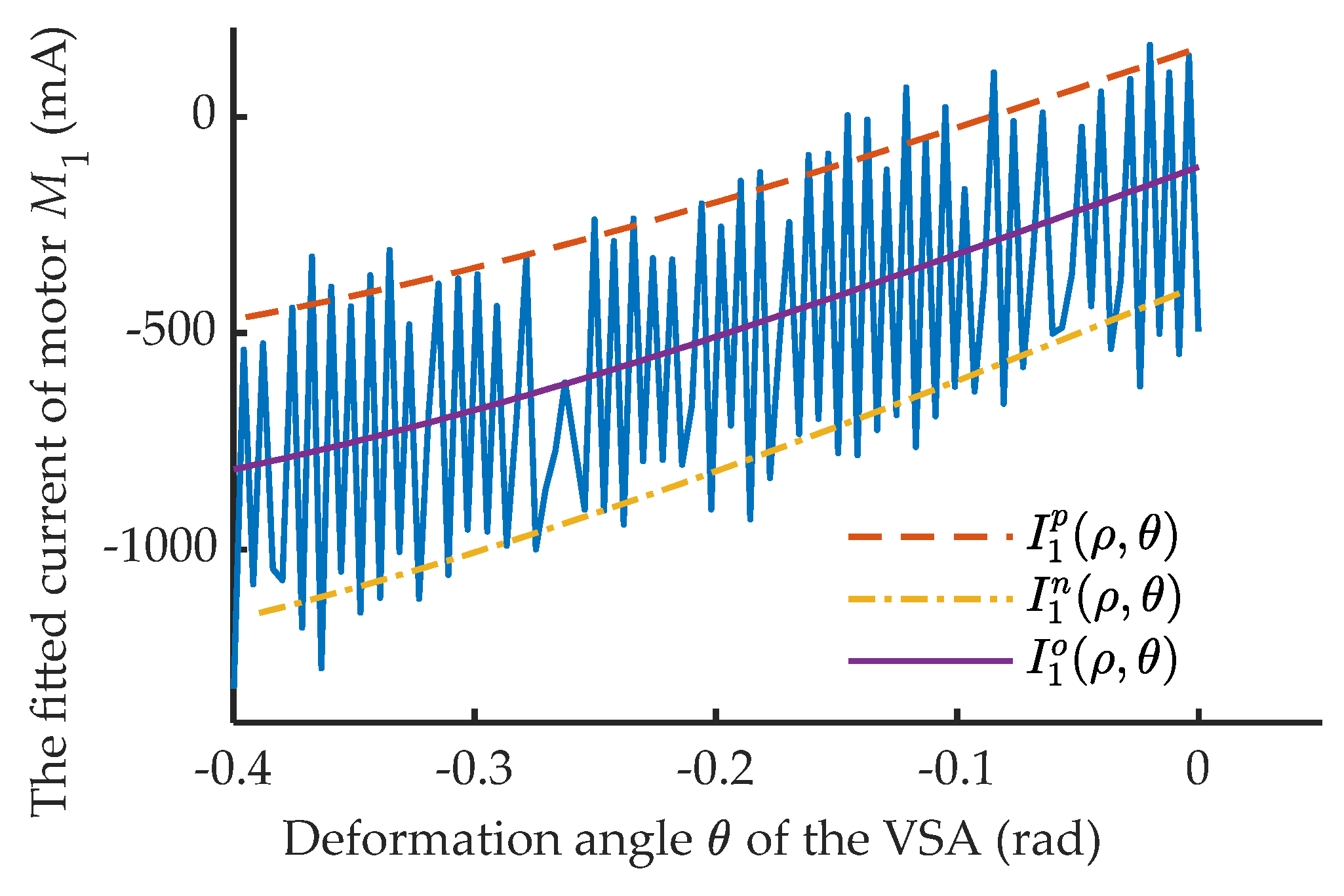
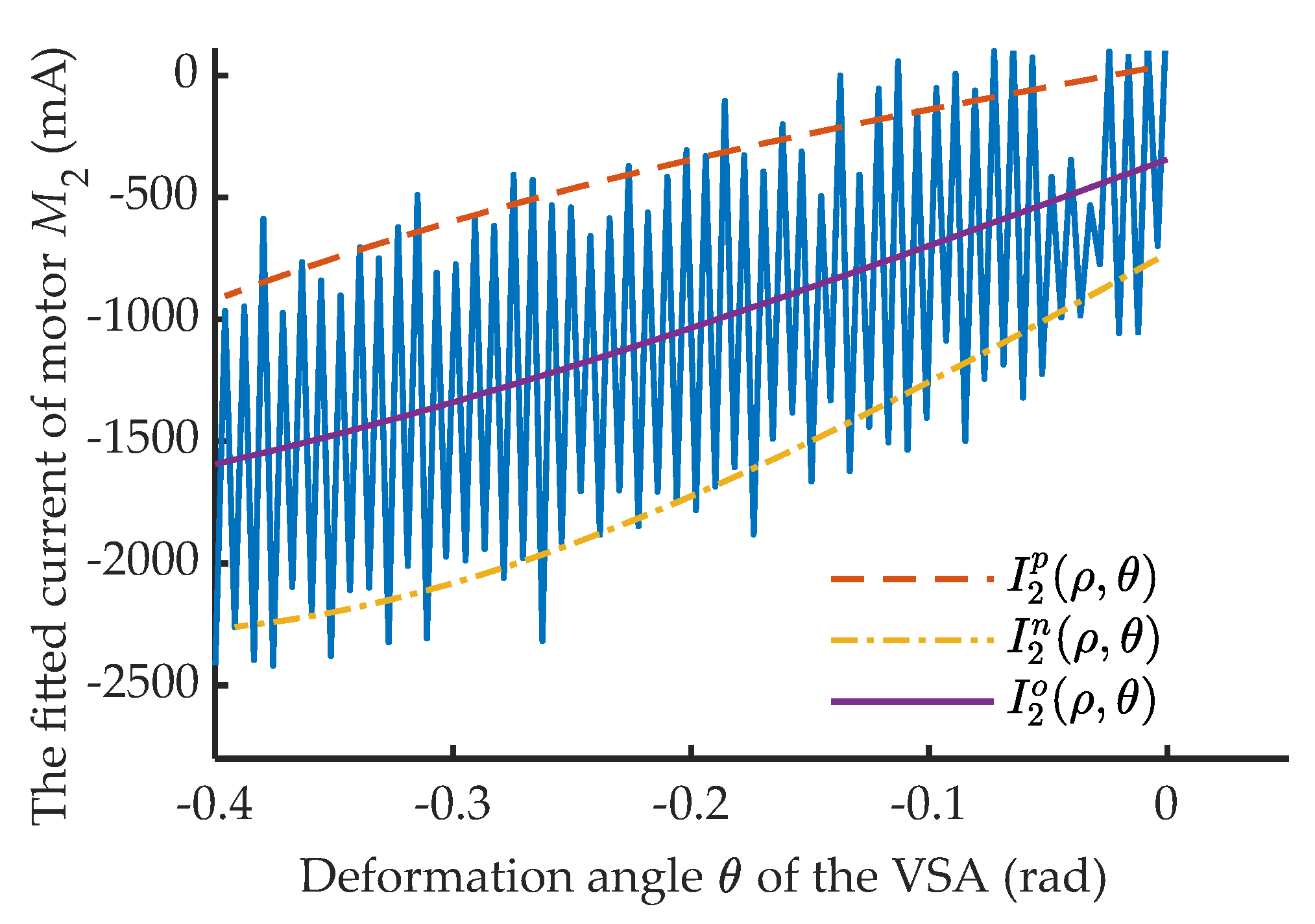
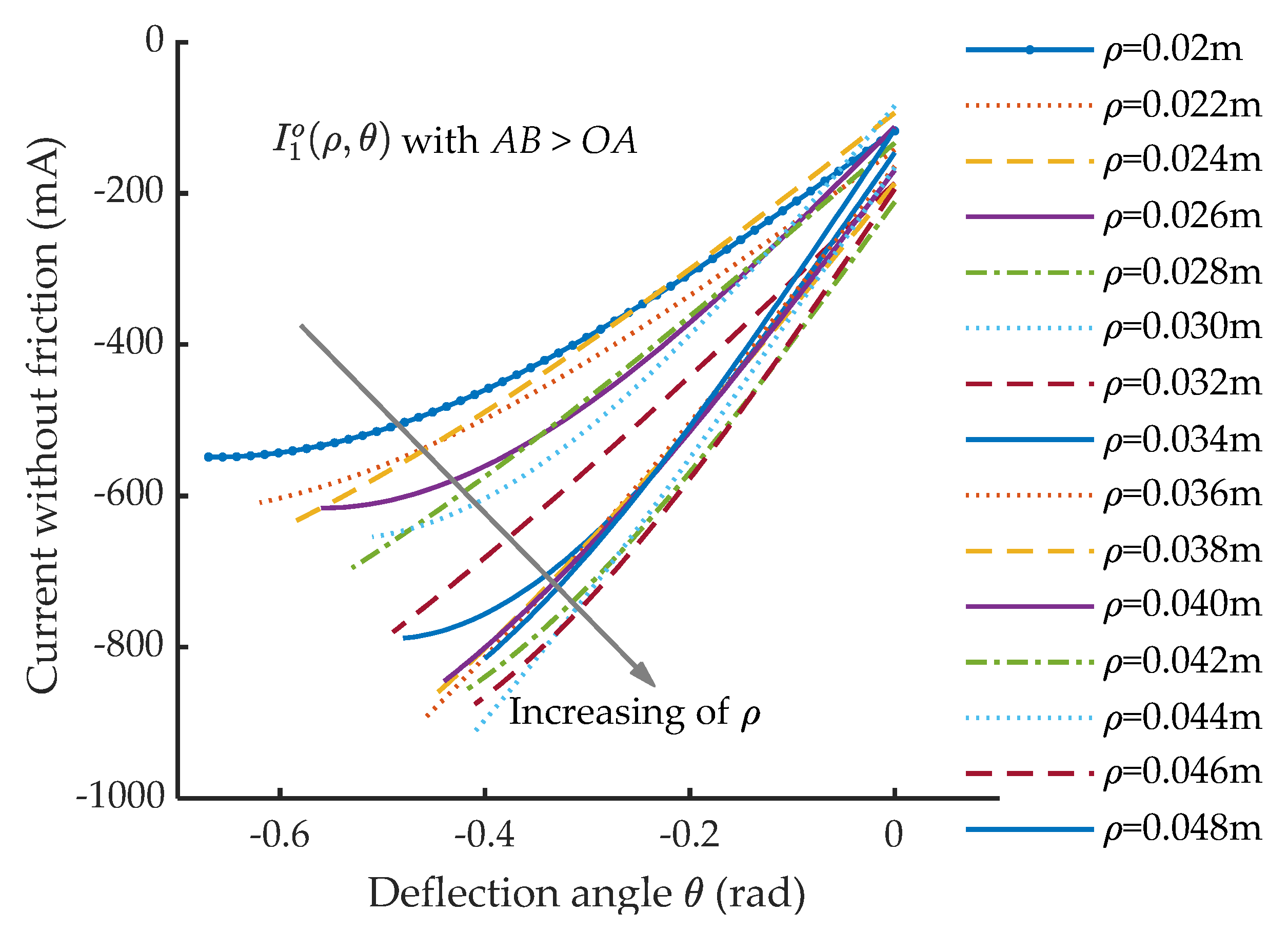
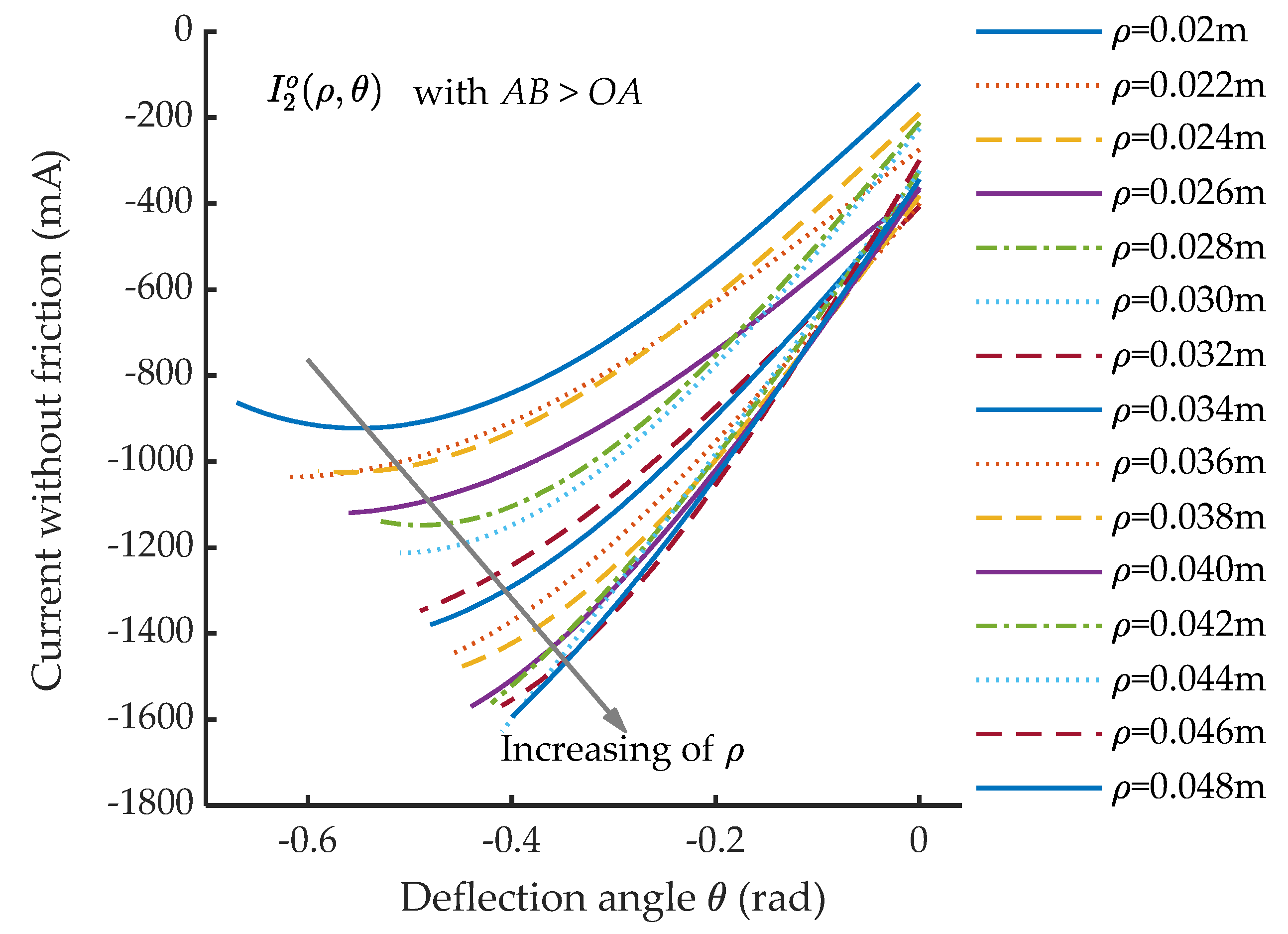
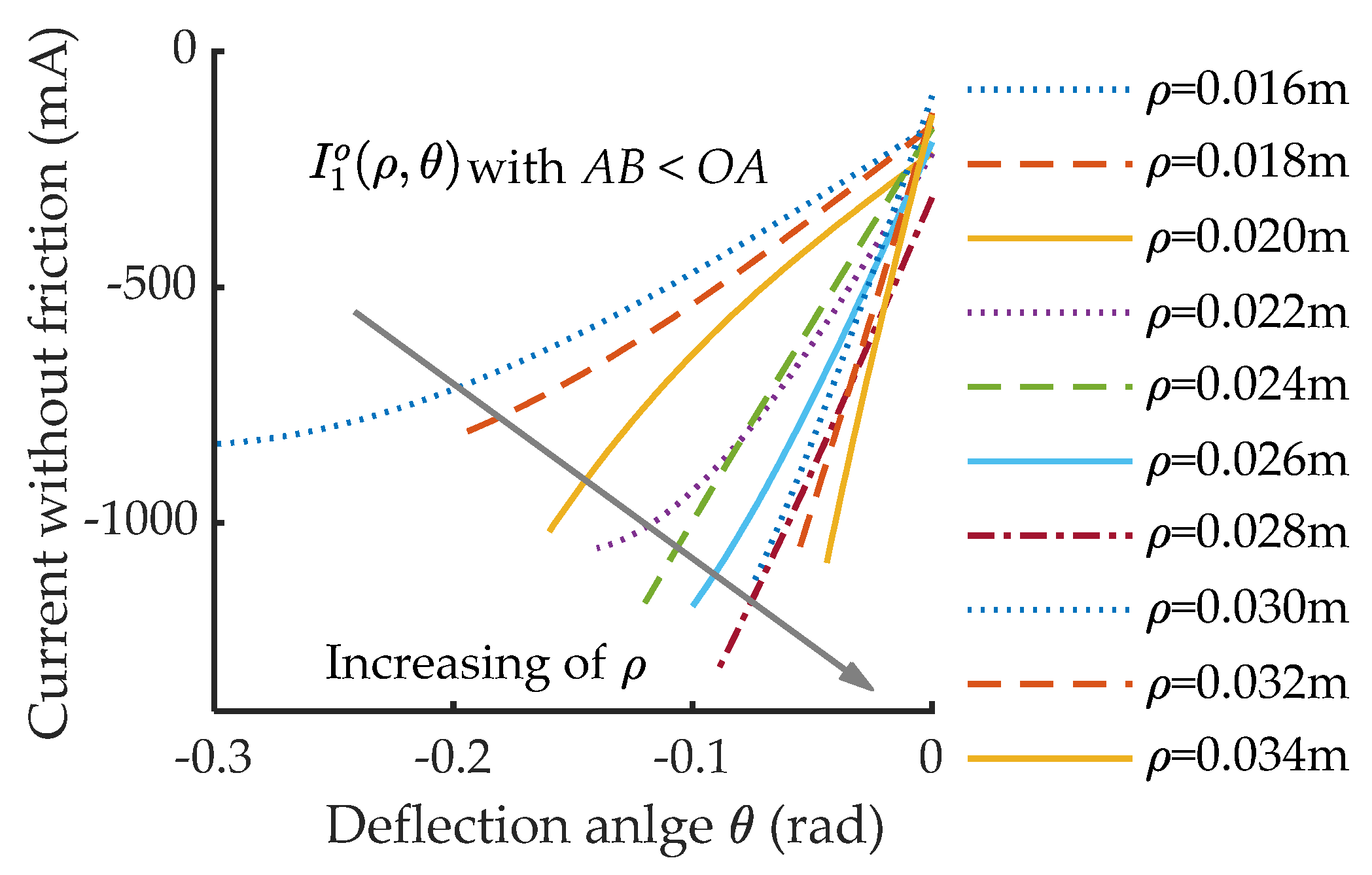
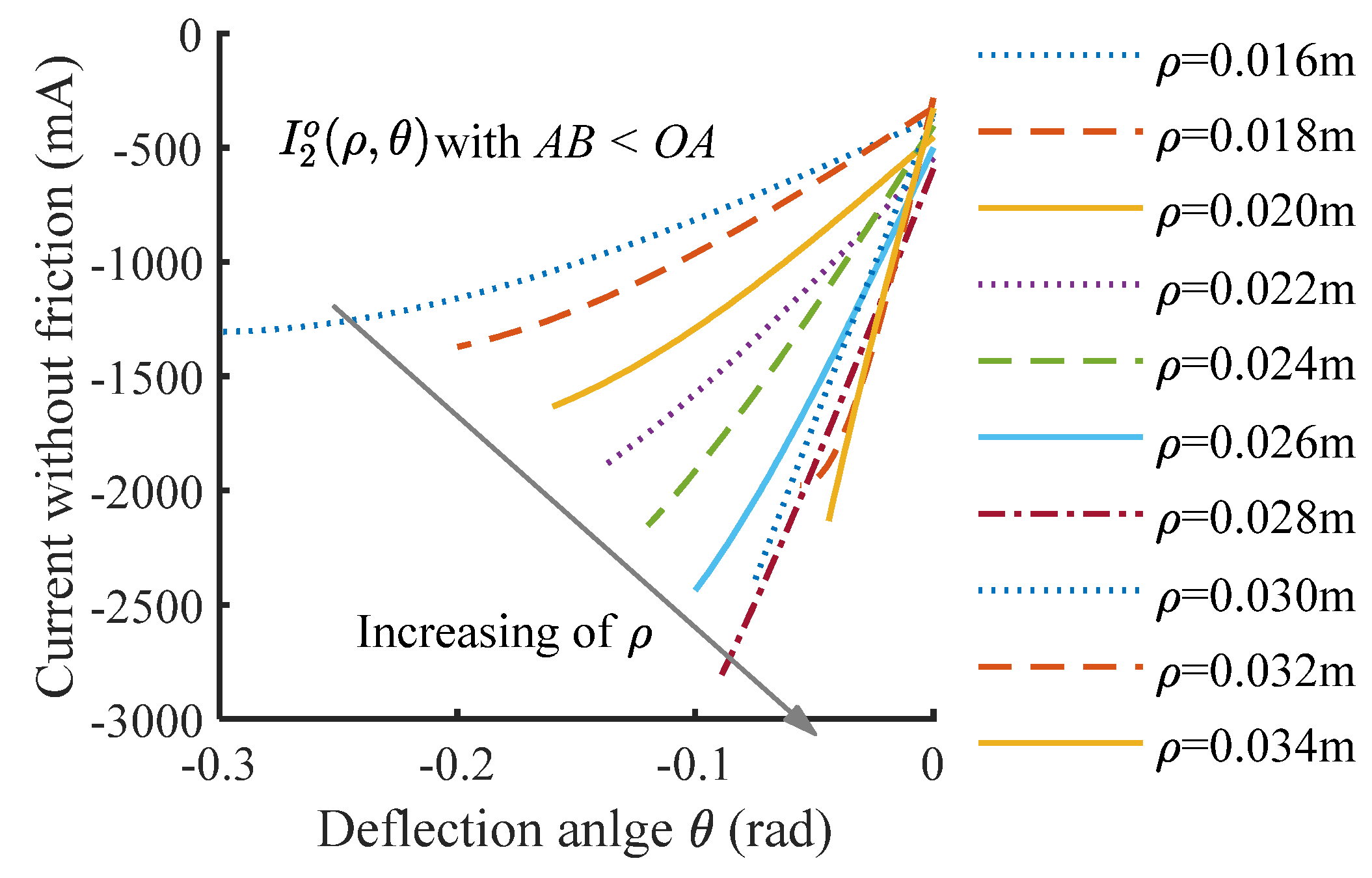
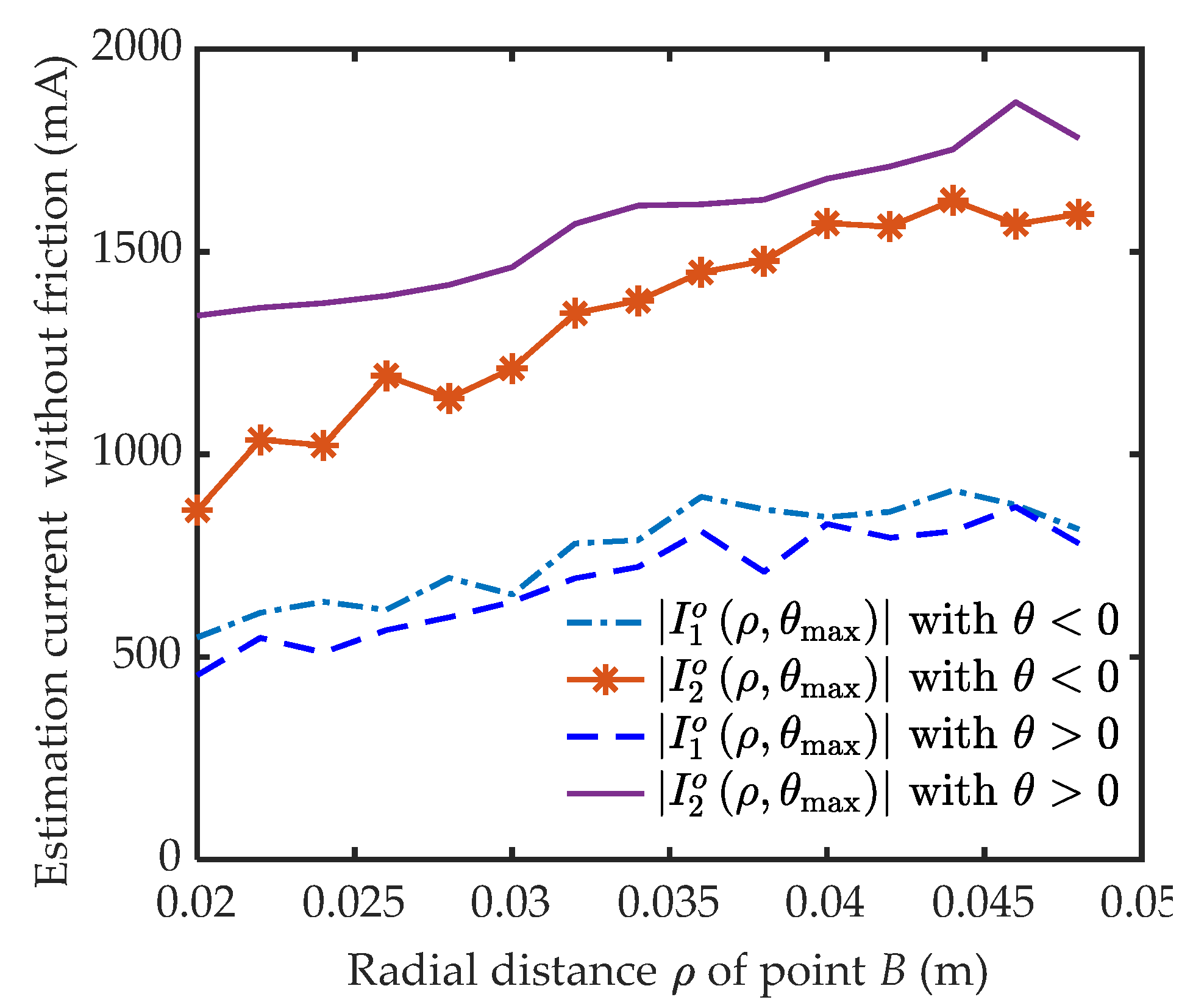
5.3.2. Frictionless Drive Current Estimation for the Dual Cams
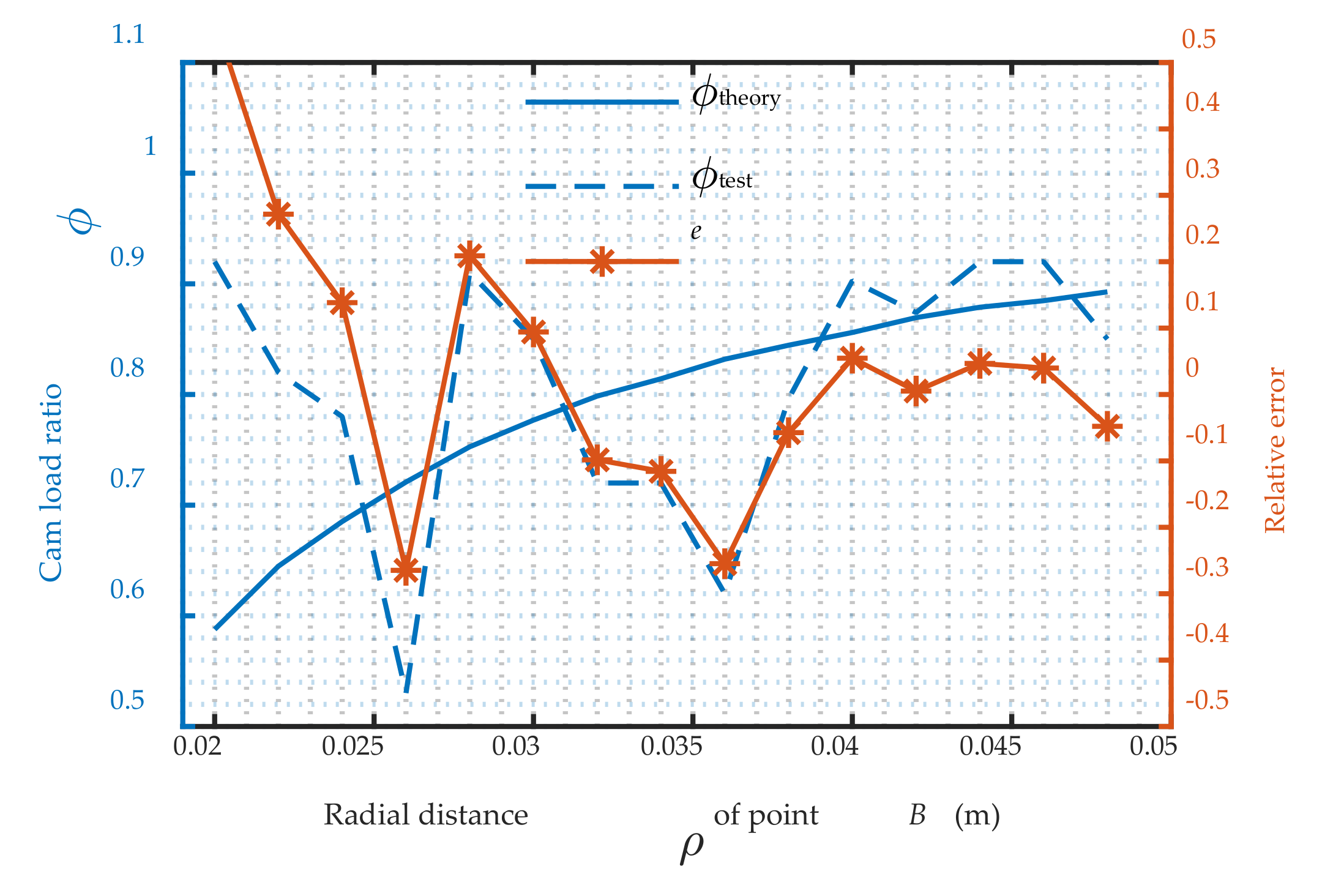
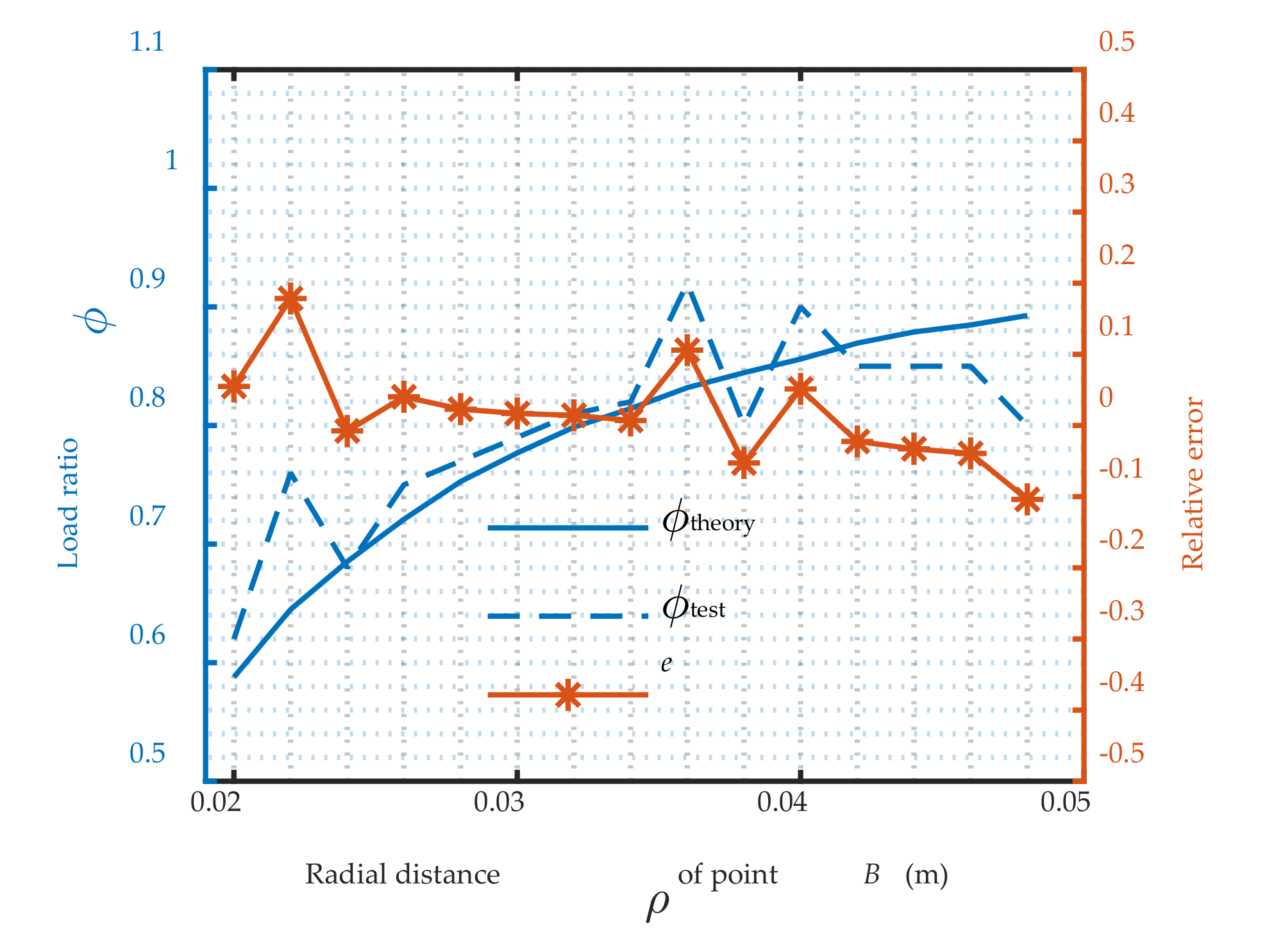
5.3.3. Estimation Results of Load Ratio
5.3.4. Error Analysis
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hong, C.; Tang, D.; Quan, Q.; Cao, Z.; Deng, Z. A combined series-elastic actuator & parallel-elastic leg no-latch bio-inspired jumping robot. Mech. Mach. Theory 2020, 149, 103814. [Google Scholar]
- Beckerle, P.; Stuhlenmiller, F.; Rinderknecht, S. Stiffness Control of Variable Serial Elastic Actuators: Energy Efficiency through Exploitation of Natural Dynamics. Actuators 2017, 6, 28. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.; Misgeld, B.J.; Pomprapa, A.; Leonhardt, S. A Testable Robust Stability Framework for the Variable Impedance Control of 1-DOF Exoskeleton with Variable Stiffness Actuator. IEEE Trans. Control. Syst. Technol. 2021, 29, 2728–2737. [Google Scholar] [CrossRef]
- Shao, Y.; Zhang, W.; Su, Y.; Ding, X. Design and optimisation of load-adaptive actuator with variable stiffness for compact ankle exoskeleton. Mech. Mach. Theory 2021, 161, 104323. [Google Scholar] [CrossRef]
- Moltedo, M.; Cavallo, G.; Baček, T.; Lataire, J.; Vanderborght, B.; Lefeber, D.; Rodriguez-Guerrero, C. Variable stiffness ankle actuator for use in robotic-assisted walking: Control strategy and experimental characterization. Mech. Mach. Theory 2019, 134, 604–624. [Google Scholar] [CrossRef]
- Gomez-Vargas, D.; Casas-Bocanegra, D.; Múnera, M.; Roberti, F.; Carelli, R.; Cifuentes, C.A. Variable Stiffness Actuators for Wearable Applications in Gait Rehabilitation. In Interfacing Humans and Robots for Gait Assistance and Rehabilitation; Springer: Cham, Switzerland, 2022; pp. 193–212. [Google Scholar]
- Li, Z.; Li, W.; Chen, W.H.; Zhang, J.; Wang, J.; Fang, Z.; Yang, G. Mechatronics design and testing of a cable-driven upper limb rehabilitation exoskeleton with variable stiffness. Rev. Sci. Instrum. 2021, 92, 024101. [Google Scholar] [CrossRef]
- Robinson, D.W.; Pratt, J.E.; Paluska, D.J.; Pratt, G.A. Series elastic actuator development for a biomimetic walking robot. In Proceedings of the 1999 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (Cat. No. 99TH8399), Atlanta, GA, USA, 19–23 September 1999; pp. 561–568. [Google Scholar]
- De Gaitani, F.H.M.; dos Santos, W.M.; Siqueira, A.A.G. Design and Performance Analysis of a Compact Series Elastic Actuator for Exoskeletons. J. Control Autom. Electr. Syst. 2022, 33, 1012–1021. [Google Scholar] [CrossRef]
- Yang, Z.; Li, X.; Xu, J.; Chen, R.; Yang, H. A new low-energy nonlinear variable stiffness actuator for the knee joint. Mech. Based Des. Struct. Mach. 2022, 1–15. [Google Scholar] [CrossRef]
- Christie, M.D.; Sun, S.; Ning, D.H.; Du, H.; Zhang, S.W.; Li, W.H. A highly stiffness-adjustable robot leg for enhancing locomotive performance. Mech. Syst. Signal Processing 2019, 126, 458–468. [Google Scholar] [CrossRef]
- Liu, H.; Zhu, D.; Xiao, J. Conceptual design and parameter optimization of a variable stiffness mechanism for producing constant output forces. Mech. Mach. Theory 2020, 154, 104033. [Google Scholar] [CrossRef]
- Lee, J.H.; Wahrmund, C.; Jafari, A. A Novel Mechanically Overdamped Actuator with Adjustable Stiffness (MOD-AwAS) for Safe Interaction and Accurate Positioning. Actuators 2017, 6, 22. [Google Scholar] [CrossRef]
- Albu-Schaffer, A.; Eiberger, O.; Grebenstein, M.; Haddadin, S.; Ott, C.; Wimbock, T.; Wolf, S.; Hirzinger, G. Soft robotics. IEEE Robot. Autom. Mag. 2008, 15, 20–30. [Google Scholar] [CrossRef]
- Li, Z.; Bai, S.; Madsen, O.; Chen, W.; Zhang, J. Design, modeling, and testing of a compact variable stiffness mechanism for exoskeletons. Mech. Mach. Theory 2020, 151, 103905. [Google Scholar] [CrossRef]
- Wolf, S.; Grioli, G.; Eiberger, O.; Friedl, W.; Grebenstein, M.; Höppner, H.; Burdet, E.; Caldwell, D.G.; Carloni, R.; Catalano, M.G.; et al. Variable stiffness actuators: Review on design and components. IEEE/ASME Trans. Mechatron. 2015, 21, 2418–2430. [Google Scholar] [CrossRef]
- Vanderborght, B.; Albu-Schäffer, A.; Bicchi, A.; Burdet, E.; Caldwell, D.G.; Carloni, R.; Catalano, M.; Eiberger, O.; Friedl, W.; Ganesh, G.; et al. Variable impedance actuators: A review. Robot. Auton. Syst. 2013, 61, 1601–1614. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Bai, S.; Chen, W.; Zhang, J. Nonlinear Stiffness Analysis of Spring-Loaded Inverted Slider Crank Mechanisms with a Unified Model. J. Mech. Robot. 2019, 12, 1–20. [Google Scholar] [CrossRef]
- Zhu, Y.; Wu, Q.; Chen, B.; Xu, D.; Shao, Z. Design and Evaluation of a Novel Torque-Controllable Variable Stiffness Actuator with Reconfigurability. IEEE/ASME Trans. Mechatron. 2021, 27, 99. [Google Scholar] [CrossRef]
- Jafari, A.; Tsagarakis, N.G.; Caldwell, D.G. A Novel Intrinsically Energy Efficient Actuator with Adjustable Stiffness (AwAS). IEEE/ASME Trans. Mechatron. 2013, 18, 355–365. [Google Scholar] [CrossRef]
- Sun, J.; Guo, Z.; Sun, D.; He, S.; Xiao, X. Design, modeling and control of a novel compact, energy-efficient, and rotational serial variable stiffness actuator (SVSA-II). Mech. Mach. Theory 2018, 130, 123–136. [Google Scholar] [CrossRef]
- Shao, Y.; Zhang, W.; Ding, X. Configuration synthesis of variable stiffness mechanisms based on guide-bar mechanisms with length-adjustable links. Mech. Mach. Theory 2021, 156, 104153. [Google Scholar] [CrossRef]
- Ning, Y.; Huang, H.; Xu, W.; Zhang, W.; Li, B. Design and implementation of a novel variable stiffness actuator with cam-based relocation mechanism. J. Mech. Robot. 2021, 13, 1–22. [Google Scholar] [CrossRef]
- Bi, S.S.; Liu, C.; Zhao, H.Z.; Wang, Y.L. Design and analysis of a novel variable stiffness actuator based on parallel-assembled-folded serial leaf springs. Adv. Robot. 2017, 31, 990–1001. [Google Scholar] [CrossRef]
- Xu, Y.; Guo, K.; Sun, J.; Li, J. Design, modeling and control of a reconfigurable variable stiffness actuator. Mech. Syst. Signal Processing 2021, 160, 107883. [Google Scholar] [CrossRef]
- Li, X.; Zhu, H.; Lin, W.; Chen, W.; Low, K.H. Structure-Controlled Variable Stiffness Robotic Joint Based on Multiple Rotary Flexure Hinges. IEEE Trans. Ind. Electron. 2020, 68, 12452–12461. [Google Scholar] [CrossRef]
- Petit, F.; Friedl, W.; Hoppner, H.; Grebenstein, M. Analysis and Synthesis of the Bidirectional Antagonistic Variable Stiffness Mechanism. IEEE/ASME Trans. Mechatron. 2014, 20, 684–695. [Google Scholar] [CrossRef]
- Moore, R.; Schimmels, J.M. Design of a Quadratic, Antagonistic, Cable-Driven, Variable Stiffness Actuator. J. Mech. Robot. 2021, 13, 1–20. [Google Scholar] [CrossRef]
- Lindenmann, A.; Heyden, E.; Mas, V.; Krause, D.; Matthiesen, S. Influence of friction bearings on the frequency response of a variable stiffness mechanism. Mech. Mach. Theory 2022, 168, 104588. [Google Scholar] [CrossRef]
- Zhang, M.; Ma, P.; Sun, F.; Sun, X.; Xu, F.; Jin, J.; Fang, L. Dynamic Modeling and Control of Antagonistic Variable Stiffness Joint Actuator. Actuators 2021, 10, 116. [Google Scholar] [CrossRef]
- Mengacci, R.; Garabini, M.; Grioli, G.; Catalano, M.G.; Bicchi, A. Overcoming the torque/stiffness range tradeoff in antagonistic variable stiffness actuators. IEEE/ASME Trans. Mechatron. 2021, 26, 3186–3197. [Google Scholar] [CrossRef]
- Yigit, C.B.; Bayraktar, E.; Boyraz, P. Low-cost variable stiffness joint design using translational variable radius pulleys. Mech. Mach. Theory 2018, 130, 203–219. [Google Scholar] [CrossRef]
- Malosio, M.; Spagnuolo, G.; Prini, A.; Tosatti, L.M.; Legnani, G. Principle of operation of RotWWC-VSA, a multi-turn rotational variable stiffness actuator. Mech. Mach. Theory 2017, 116, 34–49. [Google Scholar] [CrossRef]
- Li, Z.; Chen, W.; Zhang, J.; Li, Q.; Wang, J.; Fang, Z.; Yang, G. A novel cable-driven antagonistic joint designed with variable stiffness mechanisms. Mech. Mach. Theory 2022, 171, 104716. [Google Scholar] [CrossRef]
- Bilancia, P.; Berselli, G.; Palli, G. Virtual and Physical Prototyping of a Beam-Based Variable Stiffness Actuator for Safe Human-Machine Interaction. Robot. Comput. Integr. Manuf. 2020, 65, 101886. [Google Scholar] [CrossRef]
- Choi, J.; Hong, S.; Lee, W.; Kang, S.; Kim, M. A Robot Joint with Variable Stiffness Using Leaf Springs. IEEE Trans. Robot. 2011, 27, 229–238. [Google Scholar] [CrossRef]
- Mei, F.; Bi, S.; Liu, C.; Chang, Q. Optimal Design of Cam Curve Dedicated to Improving Load Uniformity of Bidirectional Antagonistic VSA. In International Conference on Intelligent Robotics and Applications; Springer: Cham, Switzerland, 2021; pp. 3–13. [Google Scholar]
- Mei, F.; Bi, S.; Chen, L.; Gao, H. A novel design of planar high-compliance joint in variable stiffness module with multiple uniform stress leaf branches on rigid-flexible integral linkage. Mech. Mach. Theory 2022, 174. [Google Scholar] [CrossRef]






| Description | Symbol | Value |
|---|---|---|
| Spring stiffness | ks | 85 Nm/rad |
| Angular deflection range of the spring | α | 0~0.22 rad |
| Range of the output load | τo | 0~20 Nm |
| Length of the crank OA | a | 0.04 m |
| Length range of the base frame OB | ρ | 0.008~0.05 m |
| Rated load of cam followers | [Fb] | 950 N |
| Diameter of cam followers | D | 0.019 m |
| Range of ϕ | ϕ = 1 | 0 < ϕ < 1 | ϕ = 0 | ϕ < 0 |
| Operation mode | Ideal helping mode | Normal helping mode | Independent mode | Antagonistic mode |
| Load distribution | TM1 = τe/2, TM2 = τe/2 | 0 < TM2 < τe/2, τe/2 < TM1 < te | TM2 = 0, TM 1 = τe | TM2 < 0, TM1 > τe |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mei, F.; Bi, S.; Cai, Y.; Gao, H. Design and Experiment Evaluation of Load Distribution on the Dual Motors in Cam-Based Variable Stiffness Actuator with Helping Mode. Actuators 2022, 11, 153. https://doi.org/10.3390/act11060153
Mei F, Bi S, Cai Y, Gao H. Design and Experiment Evaluation of Load Distribution on the Dual Motors in Cam-Based Variable Stiffness Actuator with Helping Mode. Actuators. 2022; 11(6):153. https://doi.org/10.3390/act11060153
Chicago/Turabian StyleMei, Fanghua, Shusheng Bi, Yueri Cai, and Hanjun Gao. 2022. "Design and Experiment Evaluation of Load Distribution on the Dual Motors in Cam-Based Variable Stiffness Actuator with Helping Mode" Actuators 11, no. 6: 153. https://doi.org/10.3390/act11060153
APA StyleMei, F., Bi, S., Cai, Y., & Gao, H. (2022). Design and Experiment Evaluation of Load Distribution on the Dual Motors in Cam-Based Variable Stiffness Actuator with Helping Mode. Actuators, 11(6), 153. https://doi.org/10.3390/act11060153






