Servo Robust Control of Uncertain Mechanical Systems: Application in a Compressor/PMSM System
Abstract
:1. Introduction
2. Servo Robust Control
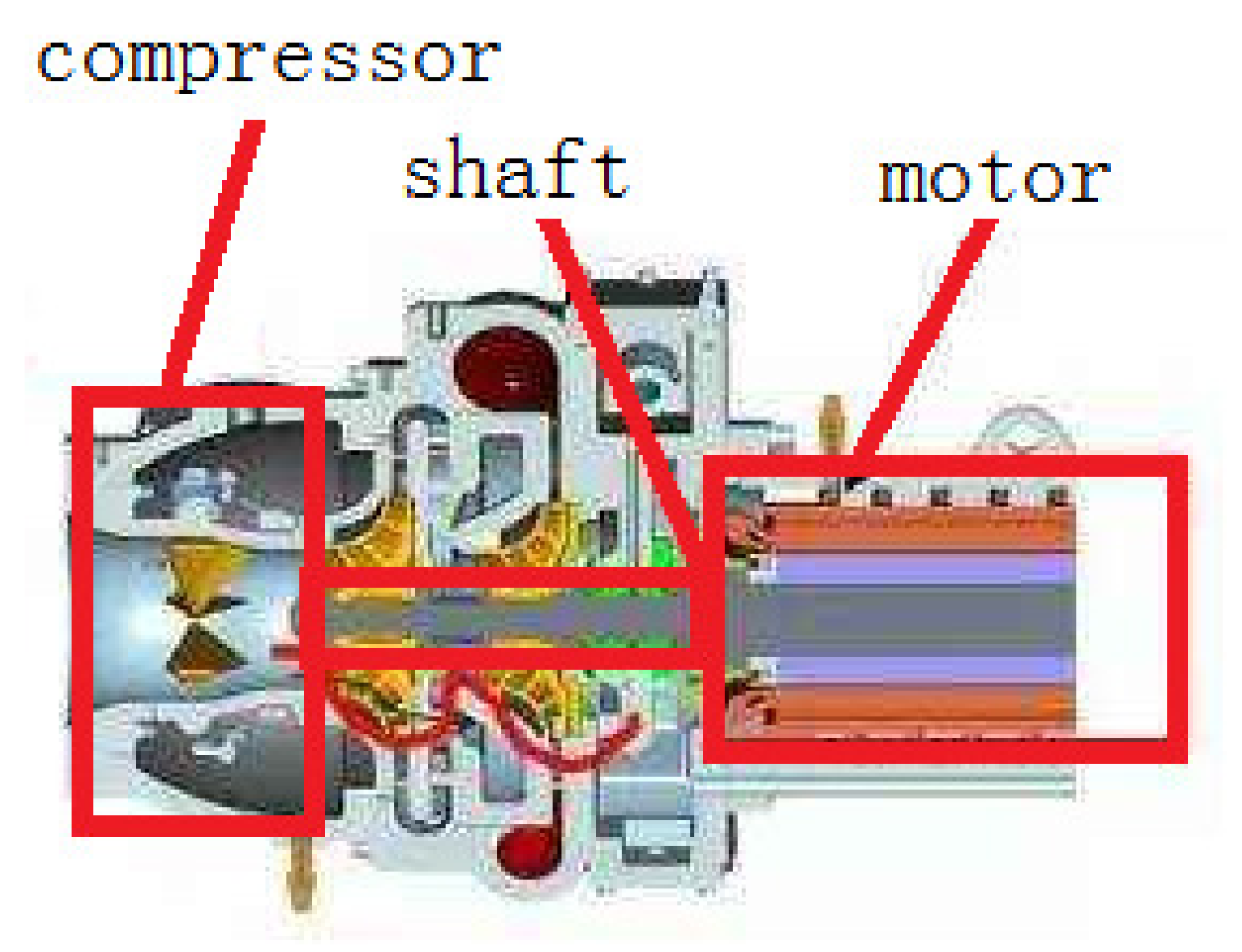
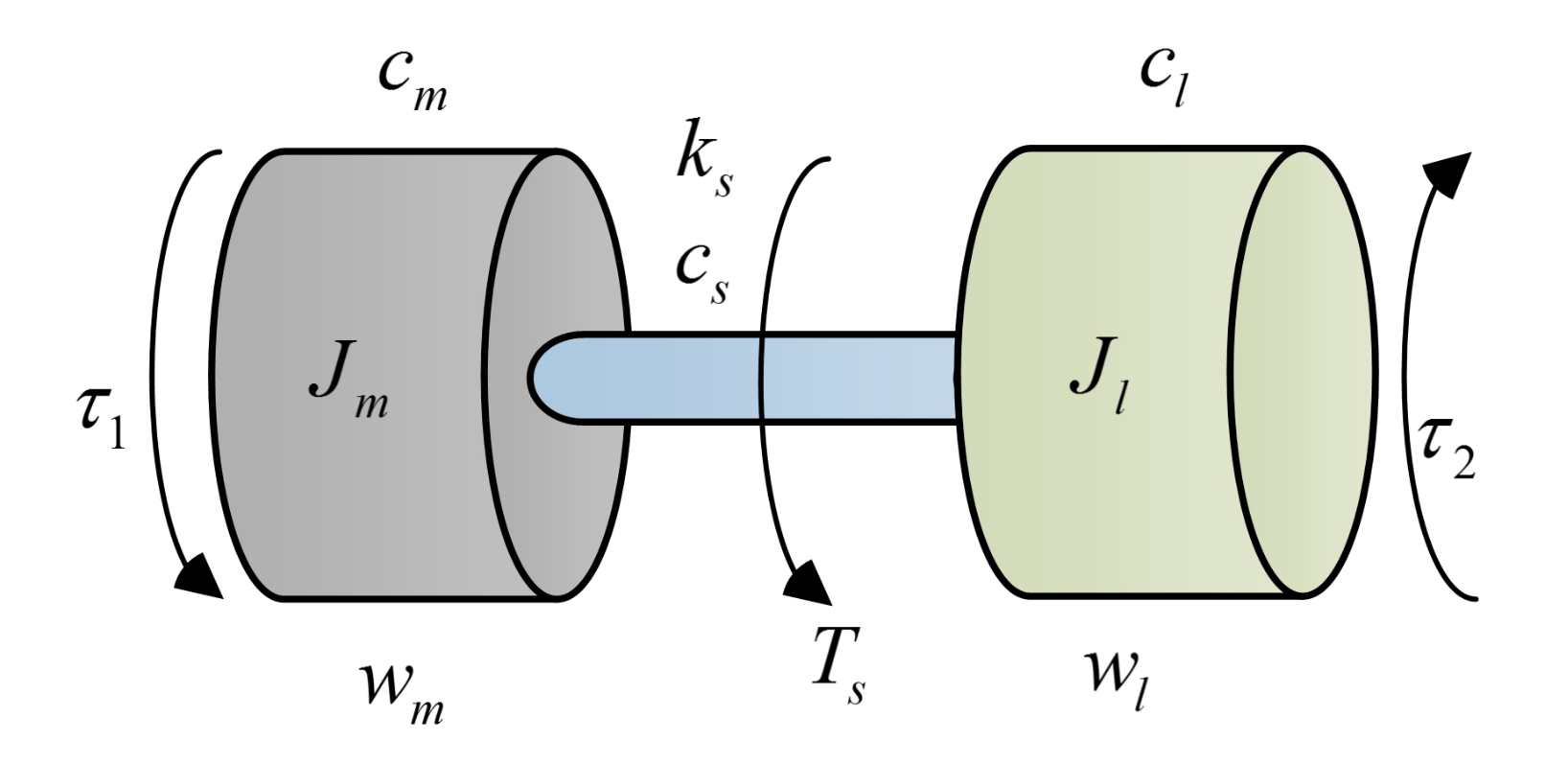
2.1. Dynamic Model of the Compressor/PMSM System
2.2. Problem Formulation
2.3. Servo Robust Control Design
2.4. Relationship between and
3. Optimal Parameter Design
3.1. Design of the Performance Index
3.2. Solution of the Optimization Problem
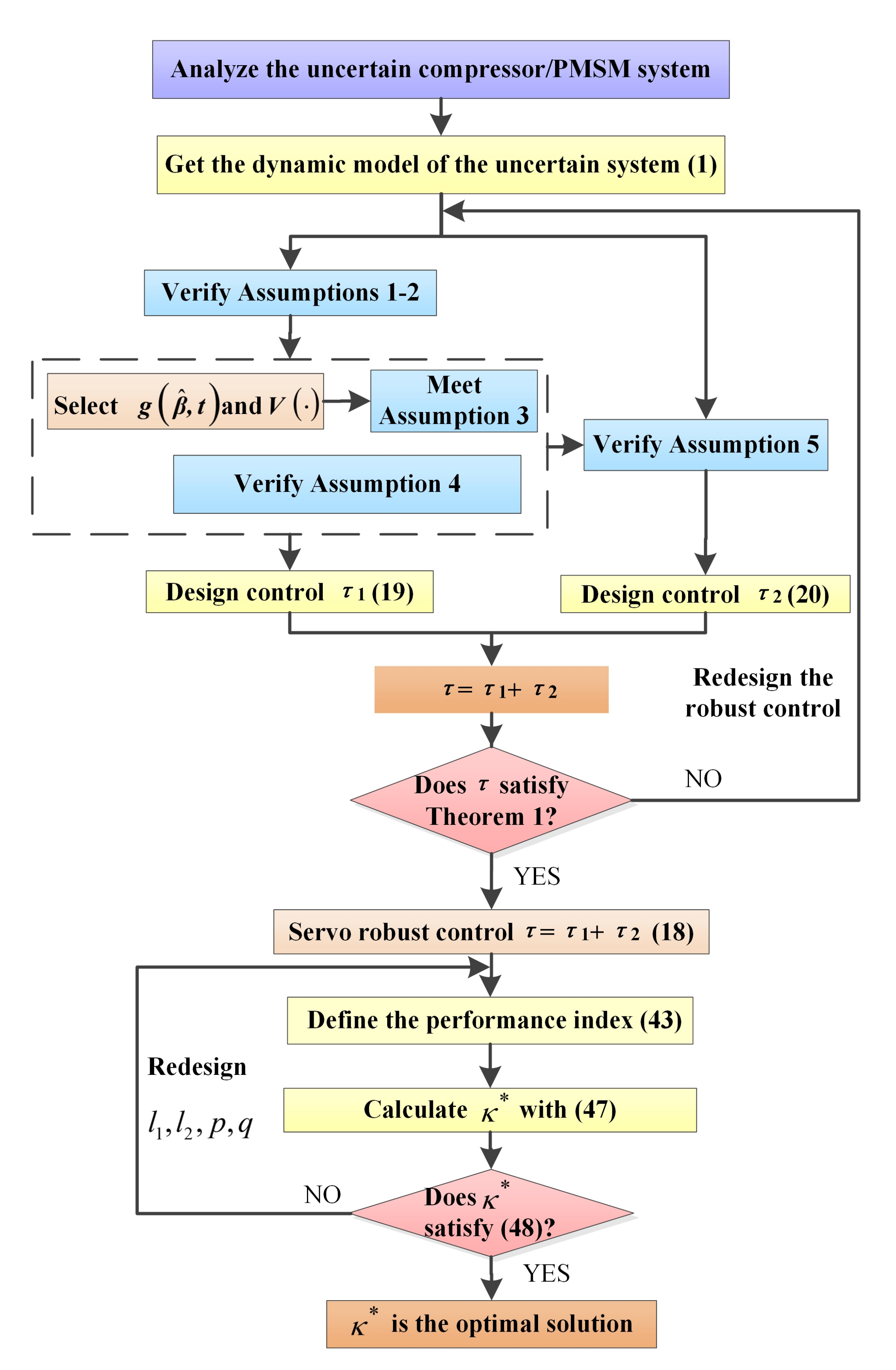
3.3. Design Procedure
4. Simulation and Discussion
4.1. Constraints and Assumptions Verification
4.2. Parameters Selection
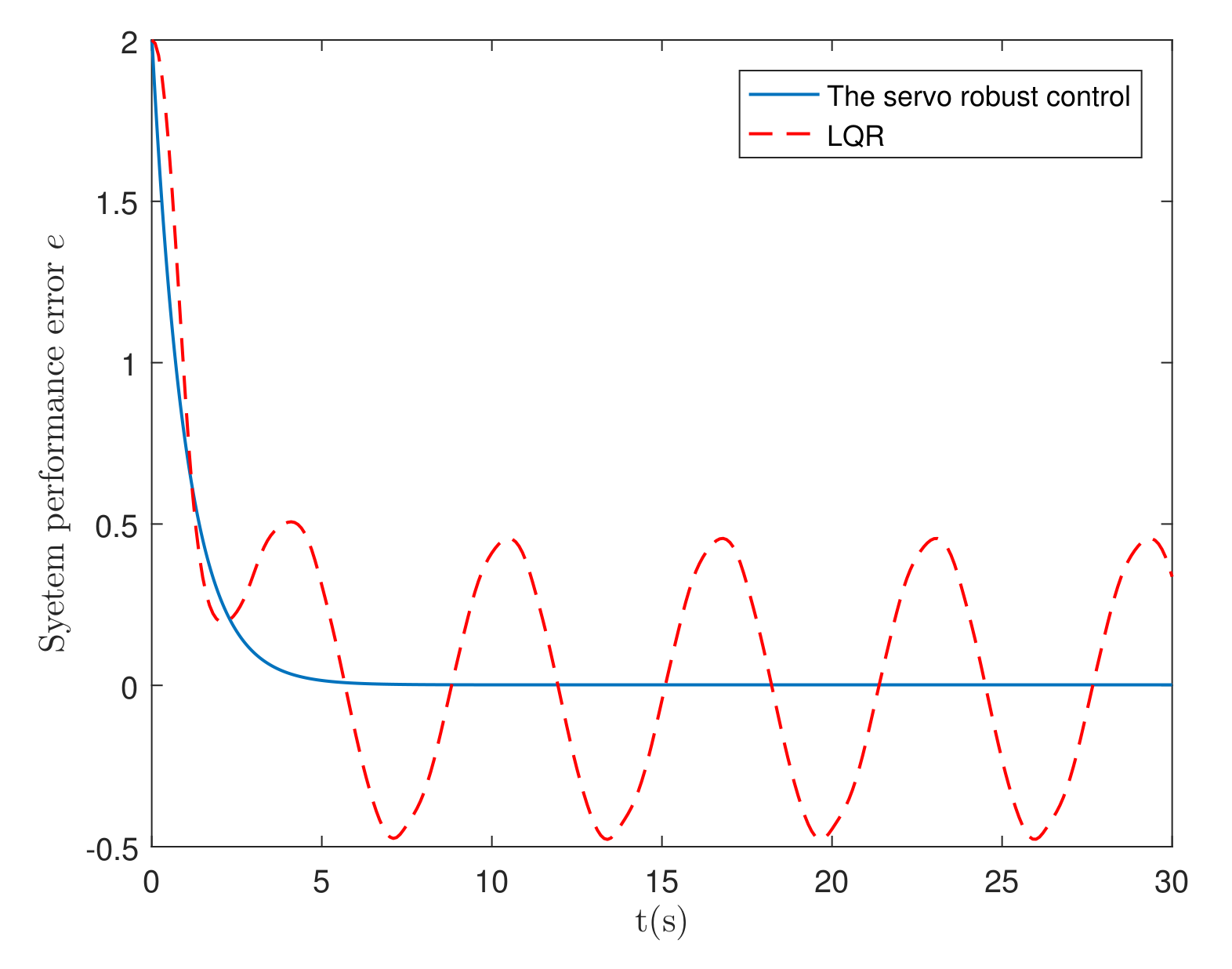
4.3. Alternative LQR Design: A Comparison
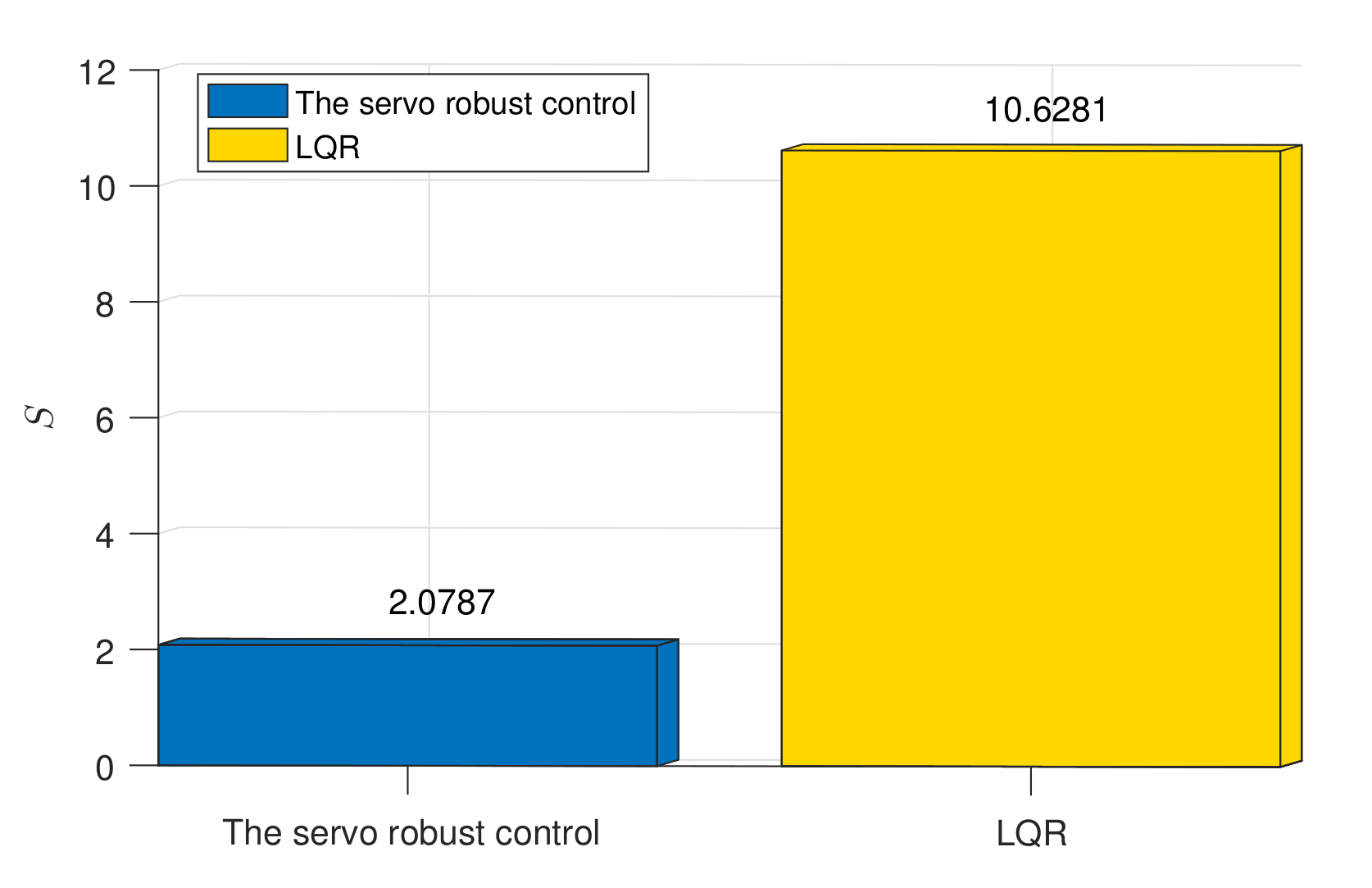
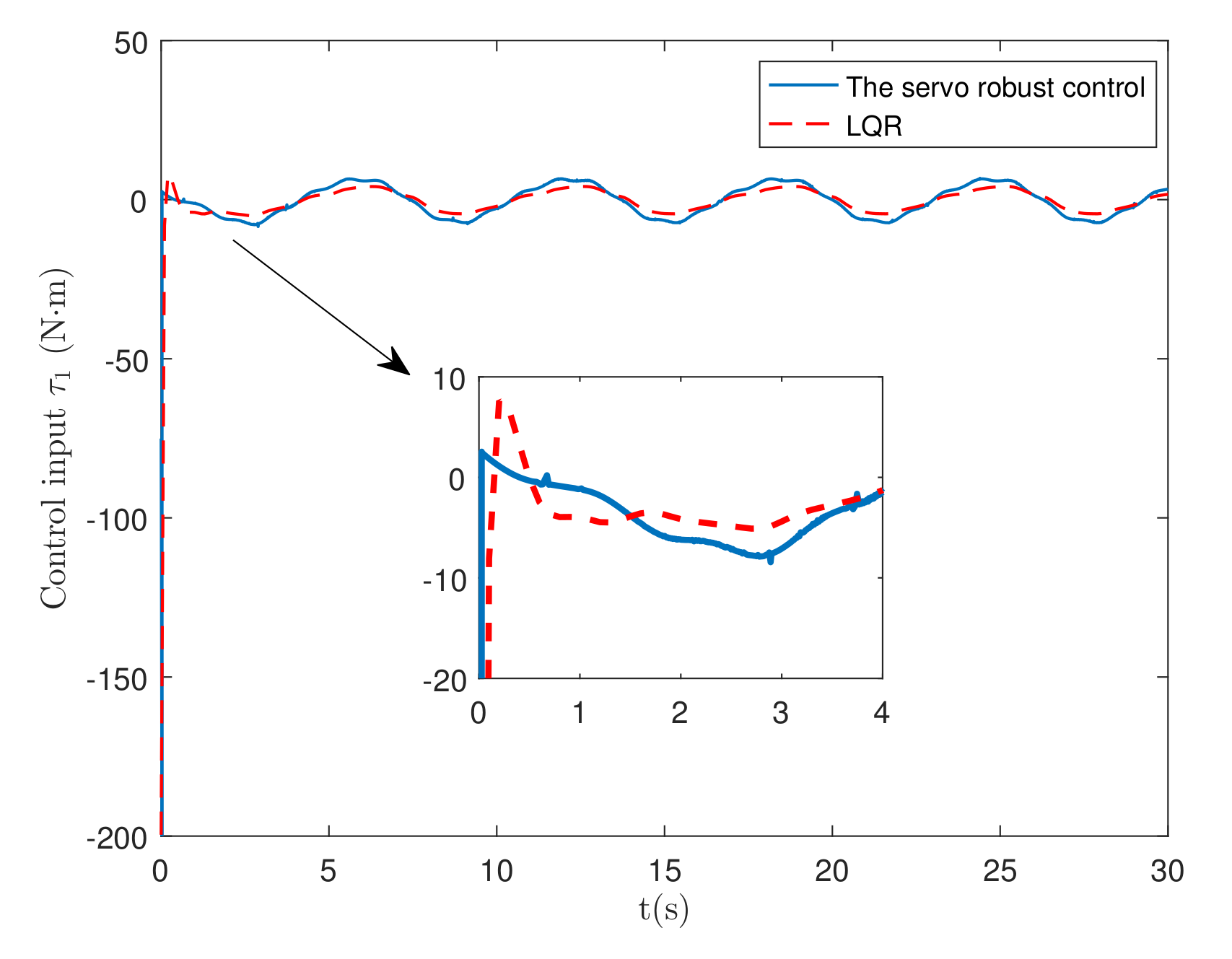
4.4. Simulations and Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, H.; Leng, J. Summary on development of permanent magnet synchronous motor. In Proceedings of the 2018 Chinese Control Furthermore, Decision Conference (CCDC), Shenyang, China, 9–11 June 2018; pp. 689–693. [Google Scholar] [CrossRef]
- Dhaouadi, R.; Hatab, A.A. Dynamic modelling of differential-drive mobile robots using Lagrange and Newton–Euler methodologies: A unified framework. Adv. Robot. Autom. 2013, 2, 1–7. [Google Scholar]
- Udwadia, F.E.; Kalaba, R.E. Analytical Dynamics: A New Approach; Cambridge University Press: New York, NY, USA, 1996. [Google Scholar]
- Udwadia, F.E.; Phohomsiri, P. Explicit equations of motion for constrained mechanical systems with singular mass matrices and applications to multi-body dynamics. Proc. R. Soc. Math. Phys. Eng. Sci. 2006, 462, 2097–2117. [Google Scholar] [CrossRef] [Green Version]
- Papastavridis, J.G. Analytical Mechanics: A Comprehensive Treatise on the Dynamics of Constrained Systems; Oxford University Press: New York, NY, USA, 2002. [Google Scholar]
- Udwadia, F.E. A new approach to stable optimal control of complex nonlinear dynamical systems. J. Appl. Mech. 2014, 81, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Vu, V.P.; Wang, W.J. Polynomial controller synthesis for uncertain large-scale polynomial T-S fuzzy systems. IEEE Trans. Cybern. 2021, 51, 1929–1942. [Google Scholar] [CrossRef] [PubMed]
- Zhen, S.; Peng, X.; Liu, X.; Li, H.; Chen, Y.H. A new PD based robust control method for the robot joint module. Mech. Syst. Signal Process. 2021, 161, 107958. [Google Scholar] [CrossRef]
- Lakhe, R.K.; Chaoui, H.; Alzayed, M.; Liu, S. Universal control of permanent magnet synchronous motors with uncertain dynamics. Actuators 2021, 10, 49. [Google Scholar] [CrossRef]
- Mani, P.; Rajan, R.; Shanmugam, L.; Joo, Y.H. Adaptive fractional fuzzy integral sliding mode control for PMSM model. IEEE Trans. Fuzzy Syst. 2019, 27, 1674–1686. [Google Scholar] [CrossRef]
- Chen, X.; Tong, W.; Mao, Y.; Zhao, T. Interval Type-2 Fuzzy Dynamic High Type Control of Permanent Magnet Synchronous Motor with Vector Decoupling Method. Actuators 2021, 10, 293. [Google Scholar] [CrossRef]
- Cruz, N.; Basin, M. Predefined-time control of full-scale 4D model of permanent-magnet synchronous motor with deterministic disturbances and stochastic noises. Actuators 2021, 10, 306. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhao, H.; Zhen, S.; Sun, H. Fuzzy-set theory based optimal robust constraint-following control for permanent magnet synchronous motor with uncertainties. Control Eng. Pract. 2021, 115, 104911. [Google Scholar] [CrossRef]
- Jin, M.; Kang, S.H.; Chang, P.H.; Lee, J. Robust control of robot manipulators using inclusive and enhanced time delay control. IEEE/ASME Trans. Mechatron. 2017, 22, 2141–2152. [Google Scholar] [CrossRef]
- Shao, X.; Liu, N.; Wang, Z.; Zhang, W.; Yang, W. Neuroadaptive integral robust control of visual quadrotor for tracking a moving object. Mech. Syst. Signal Process. 2020, 136, 106513. [Google Scholar] [CrossRef]
- Guo, H.; Xu, J.; Chen, Y.H. Robust control of fault-tolerant permanent-magnet synchronous motor for aerospace application with guaranteed fault switch process. IEEE Trans. Ind. Electron. 2015, 62, 7309–7321. [Google Scholar] [CrossRef]
- Li, C.; Zhao, H.; Sun, H.; Chen, Y.H. Robust bounded control for nonlinear uncertain systems with inequality constraints. Mech. Syst. Signal Process. 2020, 140, 106665. [Google Scholar] [CrossRef]
- Chen, Y.H.; Zhang, X. Adaptive robust approximate constraint-following control for mechanical systems. J. Frankl. Inst. 2010, 347, 69–86. [Google Scholar] [CrossRef]
- Zhao, R.; Li, M.; Niu, Q.; Chen, Y.H. Udwadia–Kalaba constraint-based tracking control for artificial swarm mechanical systems: Dynamic approach. Nonlinear Dyn. 2020, 100, 2381–2399. [Google Scholar] [CrossRef]
- Yu, R.; Chen, Y.H.; Han, B. Cooperative game approach to robust control design for fuzzy dynamical systems. IEEE Trans. Cybern. 2020. [Google Scholar] [CrossRef]
- Sun, Q.; Yang, G.; Wang, X.; Chen, Y.H. Designing robust control for mechanical systems: Constraint following and multivariable optimization. IEEE Trans. Ind. Inform. 2019, 16, 5267–5275. [Google Scholar] [CrossRef]
- Nordin, M.; Gutman, P.O. Controlling mechanical systems with backlash-A survey. Automatica 2002, 38, 1633–1649. [Google Scholar] [CrossRef]
- Chen, Y.H. Second-order constraints for equations of motion of constrained systems. IEEE/ASME Trans. Mechatron. 1998, 3, 240–248. [Google Scholar] [CrossRef]
- Khalil, H.K. Nonlinear Systems, 3rd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- Noble, B.; Daniel, J.W. Applied Linear Algebra, 2nd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 1977. [Google Scholar]
- Corless, M.J.; Leitmann, G. Continuous state feedback guaranteeing uniform ultimate boundedness for uncertain dynamic systems. IEEE Trans. Autom. Control 1981, 26, 1139–1144. [Google Scholar] [CrossRef] [Green Version]


| Parameter | Description | Units |
|---|---|---|
| moment of inertia of the motor | ||
| moment of inertia of the load | ||
| friction of viscous motor | ||
| friction of viscous load | () | |
| inner damping coefficient of the shaft | () | |
| elasticity coefficient of the shaft | ||
| control input | ||
| load torque disturbance |
| Parameter | Value |
|---|---|
| 0.4 | |
| 3 | |
| 0.7 | |
| 5 () | |
| 0.2 () | |
| 3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Q.; Yu, R.; Li, C.; Chen, Y.-H.; Gu, J. Servo Robust Control of Uncertain Mechanical Systems: Application in a Compressor/PMSM System. Actuators 2022, 11, 42. https://doi.org/10.3390/act11020042
Zhang Q, Yu R, Li C, Chen Y-H, Gu J. Servo Robust Control of Uncertain Mechanical Systems: Application in a Compressor/PMSM System. Actuators. 2022; 11(2):42. https://doi.org/10.3390/act11020042
Chicago/Turabian StyleZhang, Qiang, Rongrong Yu, Chenming Li, Ye-Hwa Chen, and Jieying Gu. 2022. "Servo Robust Control of Uncertain Mechanical Systems: Application in a Compressor/PMSM System" Actuators 11, no. 2: 42. https://doi.org/10.3390/act11020042
APA StyleZhang, Q., Yu, R., Li, C., Chen, Y.-H., & Gu, J. (2022). Servo Robust Control of Uncertain Mechanical Systems: Application in a Compressor/PMSM System. Actuators, 11(2), 42. https://doi.org/10.3390/act11020042








