Simulation of the Braking Effects of Permanent Magnet Eddy Current Brake and Its Effects on Levitation Characteristics of HTS Maglev Vehicles
Abstract
1. Introduction
2. The Detent Force Model
- (1)
- The magnetic induction intensity in the Z direction is 0.
- (2)
- The edge effect is ignored.
- (3)
- The frictional resistance and aerodynamic resistance are also ignored.
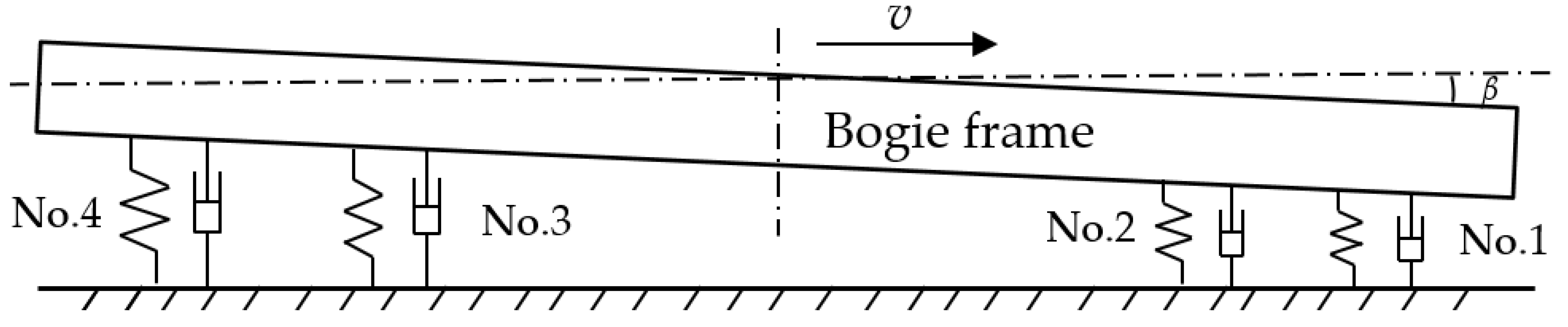
3. The HTS Maglev Test Vehicle Model
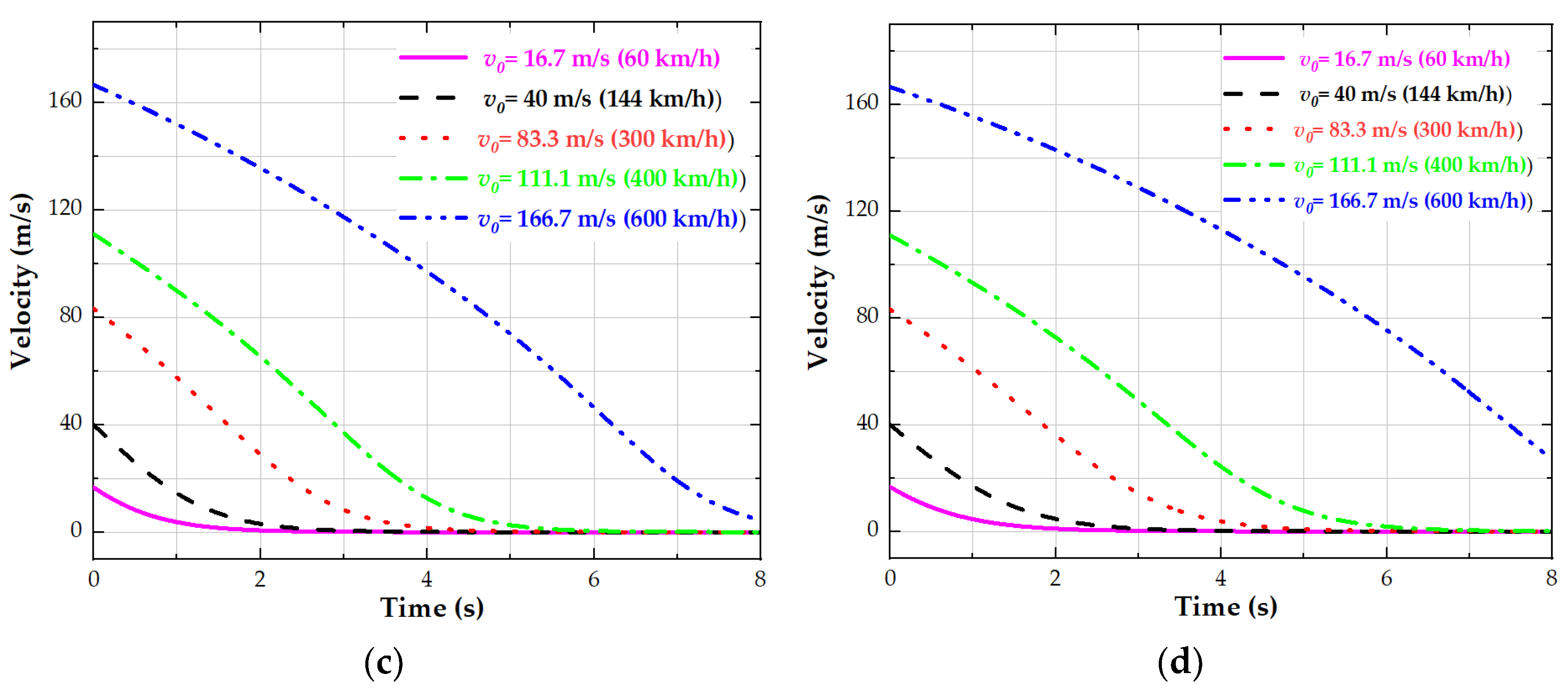
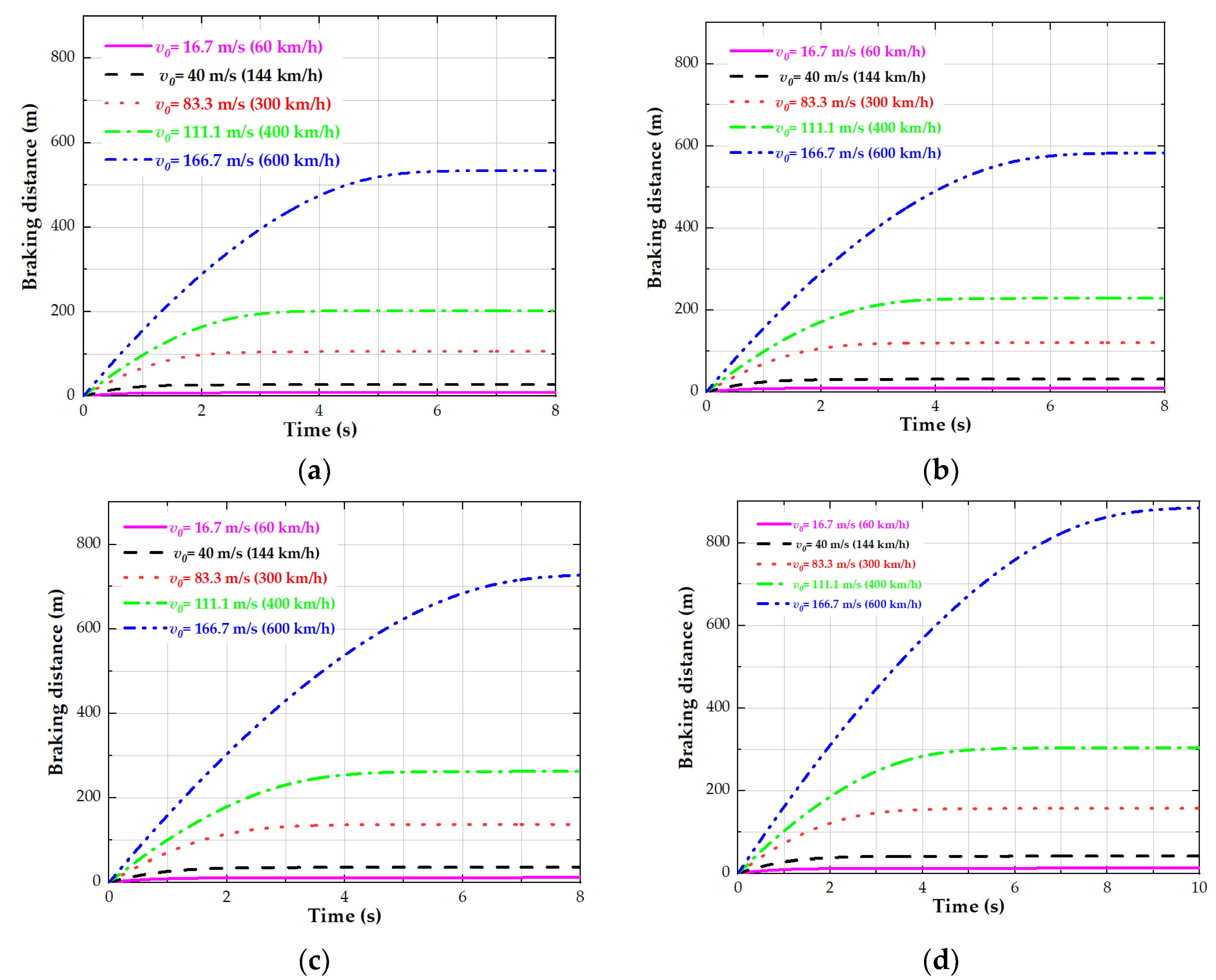
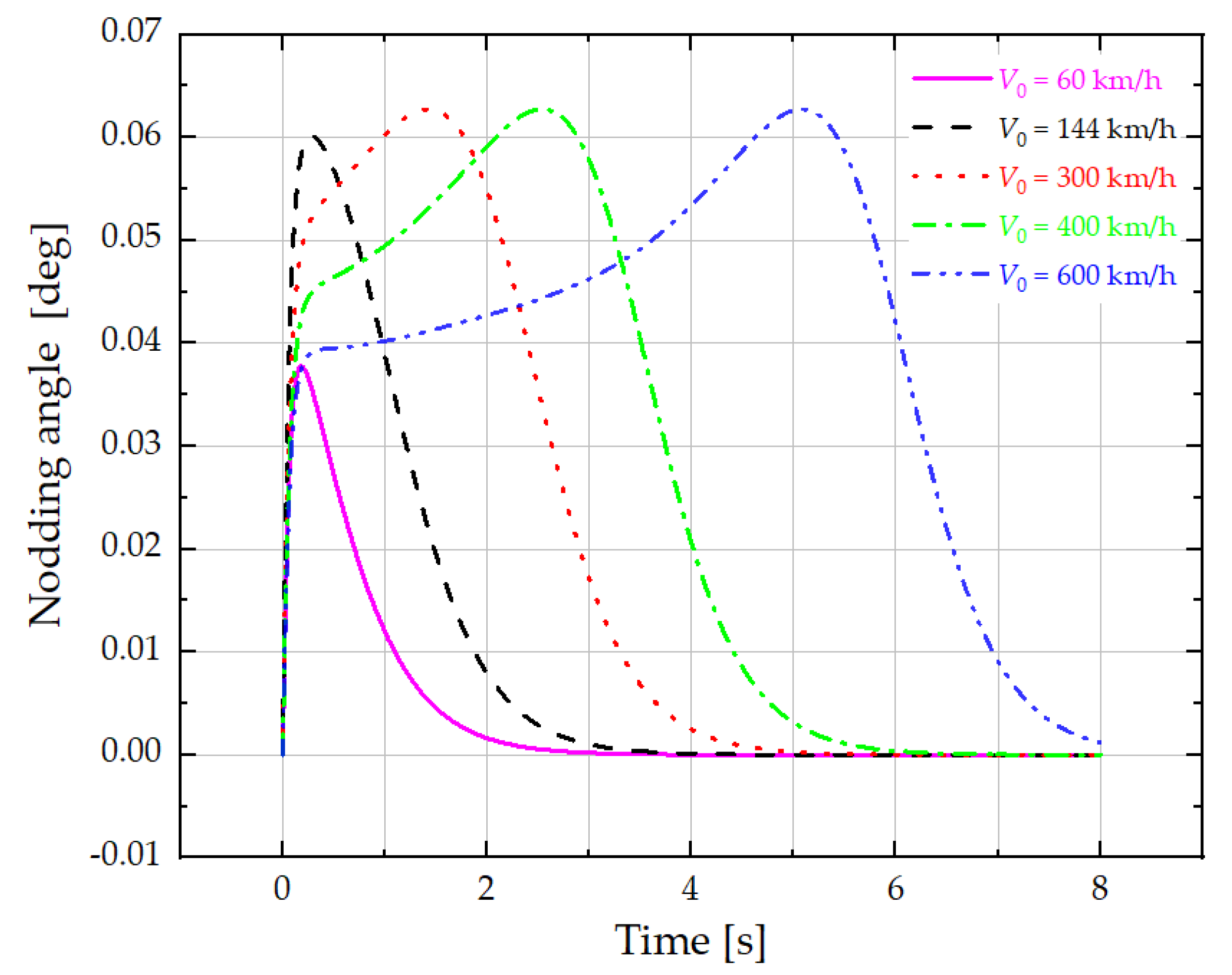
4. Results and Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ren, Q.; Zhang, J.; Luo, J. Characteristic Analysis and Control of a Rotary Electromagnetic Eddy Current Brake. Appl. Comput. Electrom. 2021, 36, 806–815. [Google Scholar]
- Valderas, D.; Mesa, I.; Adín, I.; Lehmann, H.; Lancaster, G.; Stark, O.; Baldauf, W.; del Portillo, J. 2014 Modeling Eddy Current Brake Emissions for Electromagnetic Compatibility with Signaling Devices in High-Speed Railways. IEEE Trans. Veh. Technol. 2017, 66, 9743–9752. [Google Scholar] [CrossRef]
- Zhang, B.; Peng, T.; Chen, Q.; Cao, Q.-L.; Ji, K.; Shuang, B.; Ye, J.-J.; Li, L. 3-D Nonlinear Transient Analysis and Design of Eddy Current Brake for High-speed Trains. Int. J. Appl. Electromagn. Mech. 2012, 40, 205–214. [Google Scholar] [CrossRef]
- Wang, P.; Chiueh, S. Analysis of Eddy-Current Brakes for High Speed Railway. IEEE Trans. Magn. 1998, 34, 1237–1239. [Google Scholar] [CrossRef]
- Ying, Z.; Chen, J. Efficiency Analysis of Eddy Current Braking Based on Electromagnetic Field Shape Adjustment. J. Tongji Univ. (Nat. Sci.) 2020, 48, 436–440. [Google Scholar]
- Zhao, X.; Zhang, Y. Braking Torque Analysis and Control Method of a New Motor with Eddy-Current Braking and Heating System for Electric Vehicle. Int. J. Autot. Tech-Kor. 2021, 22, 1159–1168. [Google Scholar] [CrossRef]
- Krishna, G.; Kumar, K. Experimental Investigation of Influence of Various Parameters on Permanent Magnet Eddy Current Braking System. Mater. Today Proc. 2018, 5, 2575–2581. [Google Scholar] [CrossRef]
- Fan, Y.; Yang, G. Design and Analysis of Magnetic Circuit of Permanent Magnet Eddy Current Brake. Vibroengineering Procedia 2019, 28, 111–117. [Google Scholar] [CrossRef]
- Li, J.; Yang, G.; Sun, Q. Characteristic and Thermal Analysis of Permanent Magnet Eddy Current Brake. CMES—Comp. Model. Eng. 2021, 126, 1011–1031. [Google Scholar] [CrossRef]
- Gulec, M.; Aydin, M.; Nerg, J. Analysis of an Axial-Flux Permanent-Magnet-Assisted Eddy-Current Brake at High-Temperature Working Conditions. IEEE Trans. 2021, 68, 5112–5121. [Google Scholar] [CrossRef]
- Guo, B.; Li, D.; Shi, J.; Gao, Z. A Performance Prediction Model for Permanent Magnet Eddy-Current Couplings Based on the Air-Gap Magnetic Field Distribution. IEEE. Trans. Magn. 2022, 58, 1–9. [Google Scholar] [CrossRef]
- Anantha, K.; Sathish, K. Investigation on Eddy Current Braking Systems-A Review. Appl. Mech. Mater. 2014, 592–594, 1089–1093. [Google Scholar] [CrossRef]
- Lee, C.-M.; Park, H.-J.; Cho, S.; Lee, J.; Lee, H.-W. Analysis of Multiple Factor of the Eddy Current Brake for Railway Application. Trans. Korean. Inst. Elect. Eng. 2015, 64, 1385–1390. [Google Scholar] [CrossRef]
- Jang, S. The Application of Linear Halbach Array to Eddy Current Rail Brake System. Magnetics 2001, 37, 2627–2629. [Google Scholar] [CrossRef]
- Song, N.; Zhu, M.; Zhou, G.; Guo, L.; Mu, Y.; Gao, J.; Ma, J.; Zhang, K. Design and Optimization of Halbach Permanent Magnet Array with Rectangle Section and Trapezoid Section. Int. J. Eng. 2021, 34, 184–191. [Google Scholar]
- Deng, Z.; Zhang, W.; Chen, Y.; Yang, X.; Xia, C.; Zheng, J. Optimization Study of the Halbach Permanent Magnetic Guideway for High Temperature Superconducting Magnetic Levitation. Supercond. Sci. Technol. 2020, 33, 034009. [Google Scholar] [CrossRef]
- Li, L.; Zhu, G.; Liu, X.; Chen, H.; Jiang, W.; Xue, M. Design and Optimization of a Novel HTS Flux-Modulated Linear Motor Using Halbach Permanent Magnet Arrays. IEEE. Trans. Appl. Supercond. 2021, 31, 1–4. [Google Scholar] [CrossRef]
- Choi, J.-S.; Yoo, J.-H. Optimal Array Design of the Permanent Magnet in an Eddy Current Brake. T. Kor. Soc. Mec. Eng. A 2009, 33, 658–663. [Google Scholar] [CrossRef]
- Jin, Y.; Kou, B.; Li, L.; Li, C.; Pan, D.; Song, K. Analytical Model for a Permanent Magnet Eddy-Current Brake with Transverse Edge Effect. IEEE Access 2019, 7, 61170–61179. [Google Scholar] [CrossRef]
- Chen, Q.; Tan, Y.; Li, G.; Li, J.; Mareels, I. Design of Double-Sided Linear Permanent Magnet Eddy Current Braking System. Prog. Electroma. Res. M 2017, 61, 61–73. [Google Scholar] [CrossRef]
- Wang, J.; Wang, S.; Zeng, Y.; Huang, H.; Luo, F.; Xu, Z.; Tang, Q.; Lin, G.; Zhang, C.; Ren, Z.; et al. The first man-loading high temperature superconducting Maglev test vehicle in the world. Phys. C 2002, 378, 809–814. [Google Scholar] [CrossRef]
- Deng, Z.; Zhang, W.; Zheng, J.; Wang, B.; Ren, Y.; Zheng, X.; Zhang, J. A High-temperature Superconducting Maglev-evacuated Tube Transport (HTS Maglev-ETT) Test System. IEEE. Trans. Appl. Supercond. 2017, 27, 3602008. [Google Scholar] [CrossRef]
- 600+ Per Hour! The World’s First HTS High Speed Maglev Engineering Prototype Vehicle Goes Offline. Available online: https://finance.sina.com.cn/tech/2021-01-14/doc-ikftpnnx6900907.shtml (accessed on 14 January 2021).
- Li, J.; Li, H.; Zheng, J.; Zheng, B.; Huang, H.; Deng, Z. Nonlinear vibration behaviors of high-Tc superconducting bulks in an applied permanent magnetic array field. J. Appl. Phys. 2017, 121, 243901. [Google Scholar] [CrossRef]
- Li, J.; Deng, Z.; Xia, C.; Gou, Y.; Wang, C.; Zheng, J. Subharmonic Resonance in Magnetic Levitation of the High-Temperature Superconducting Bulks YBa2Cu3O7-x Under Harmonic Excitation. IEEE. Trans. Appl. Supercond. 2019, 29, 3600908. [Google Scholar]
- Wang, H.; Deng, Z.; Ma, S.; Sun, R.; Li, H.; Li, J. Dynamic Simulation of the HTS Maglev Vehicle-Bridge Coupled System Based on Levitation Force Experiment. IEEE. Trans. Appl. Supercond. 2019, 29, 3601606. [Google Scholar] [CrossRef]


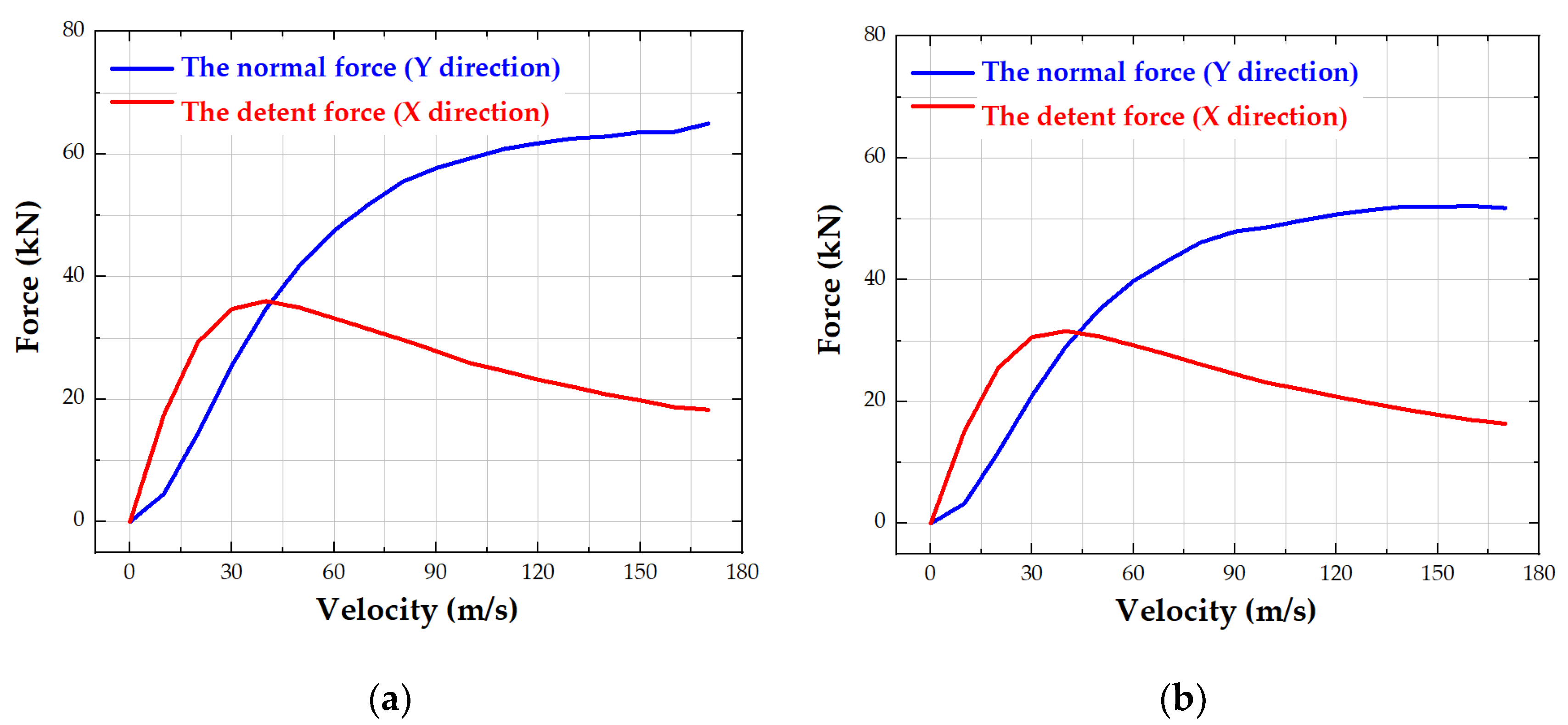

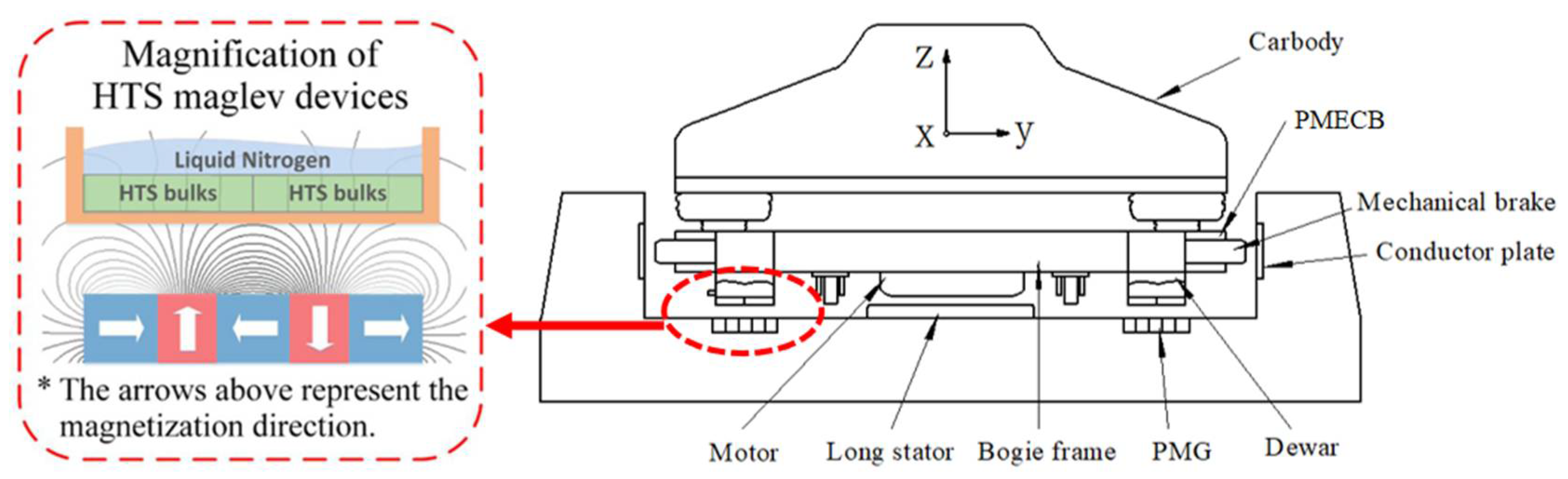
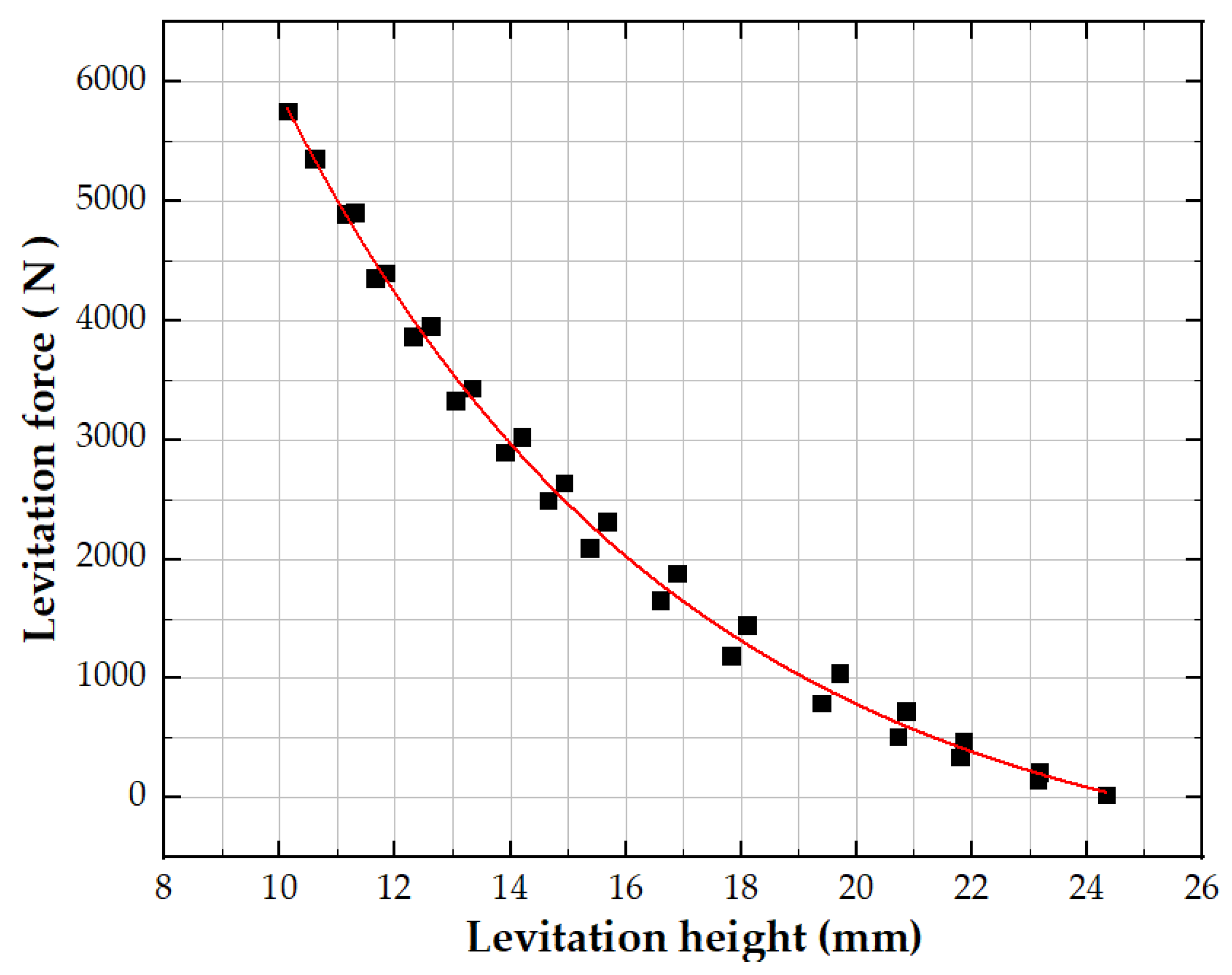
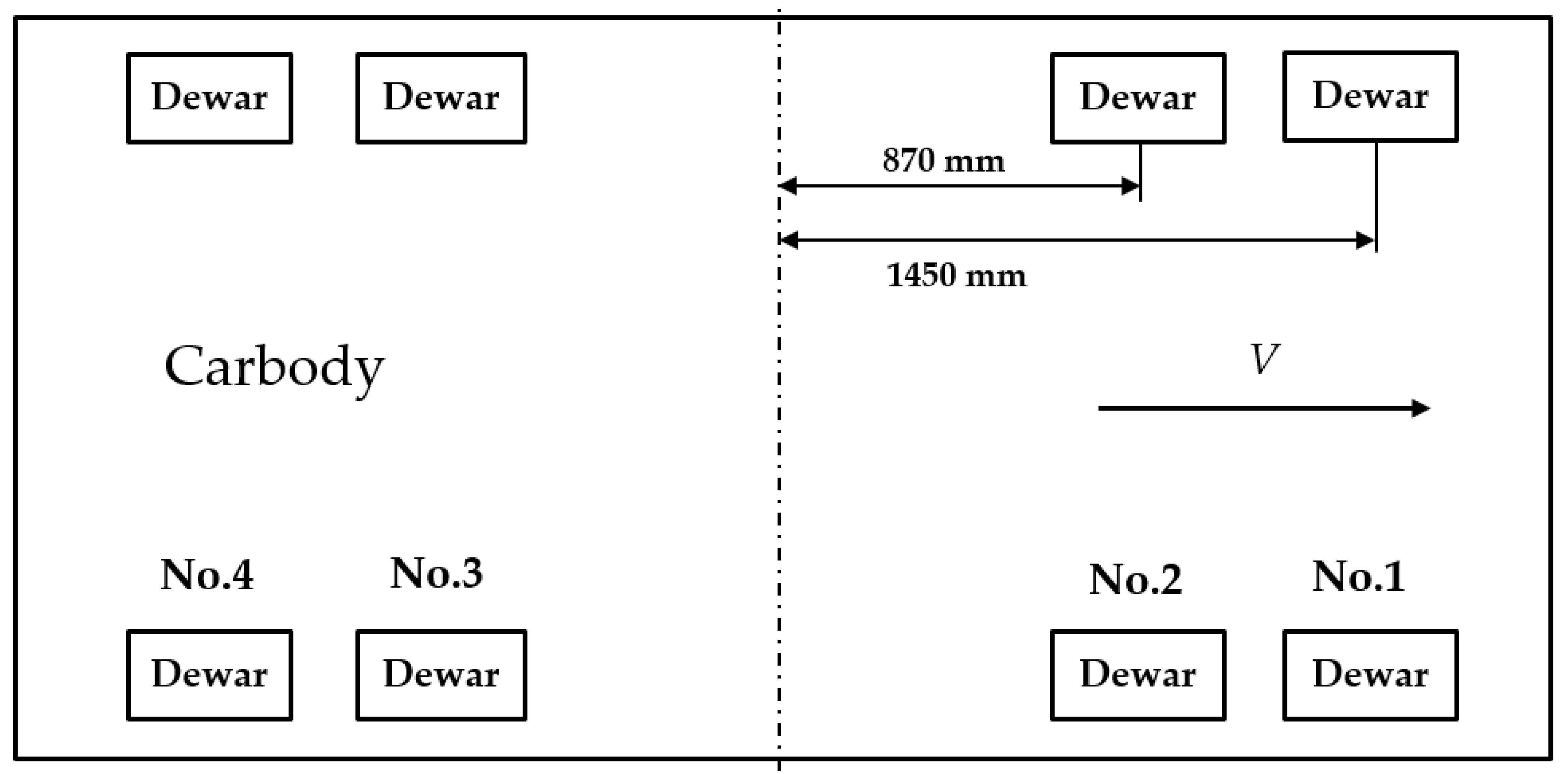
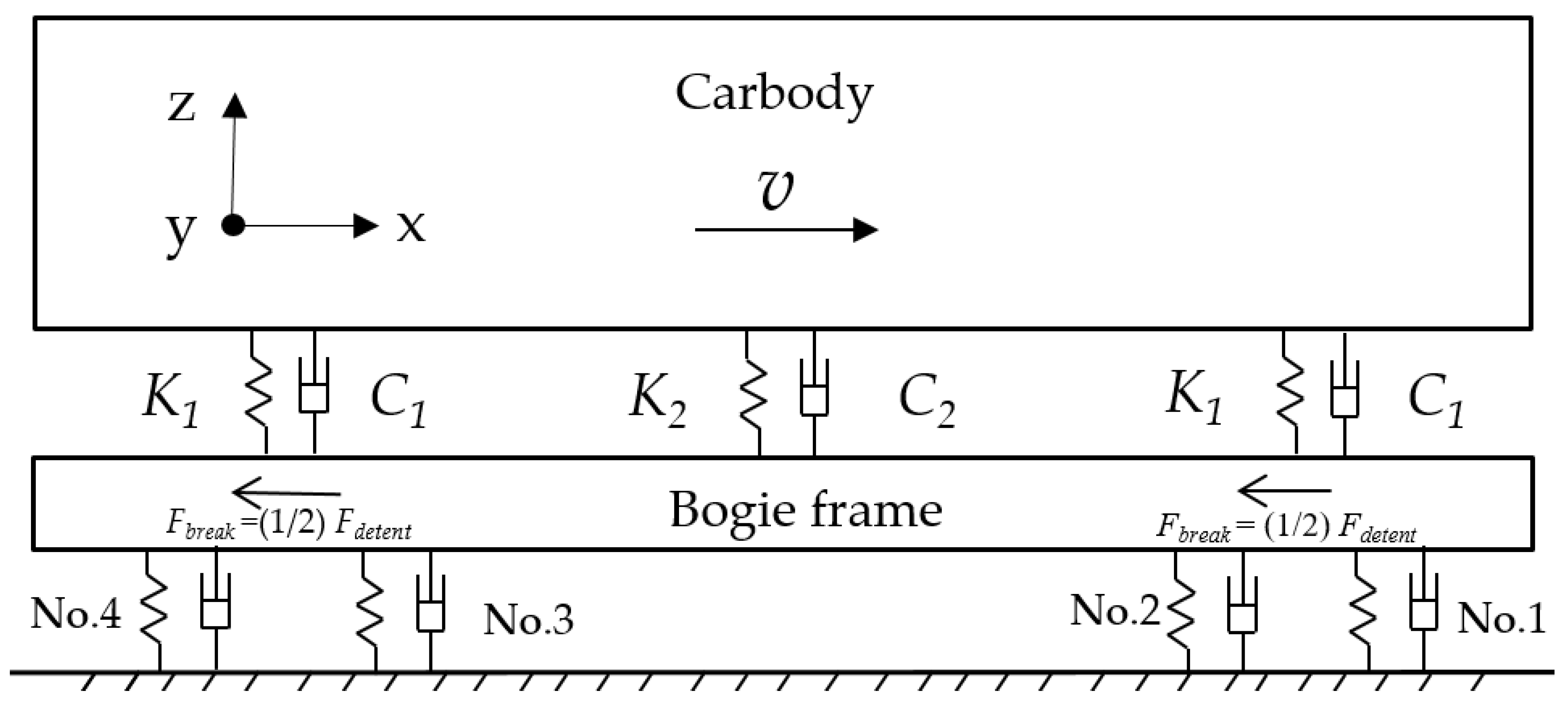
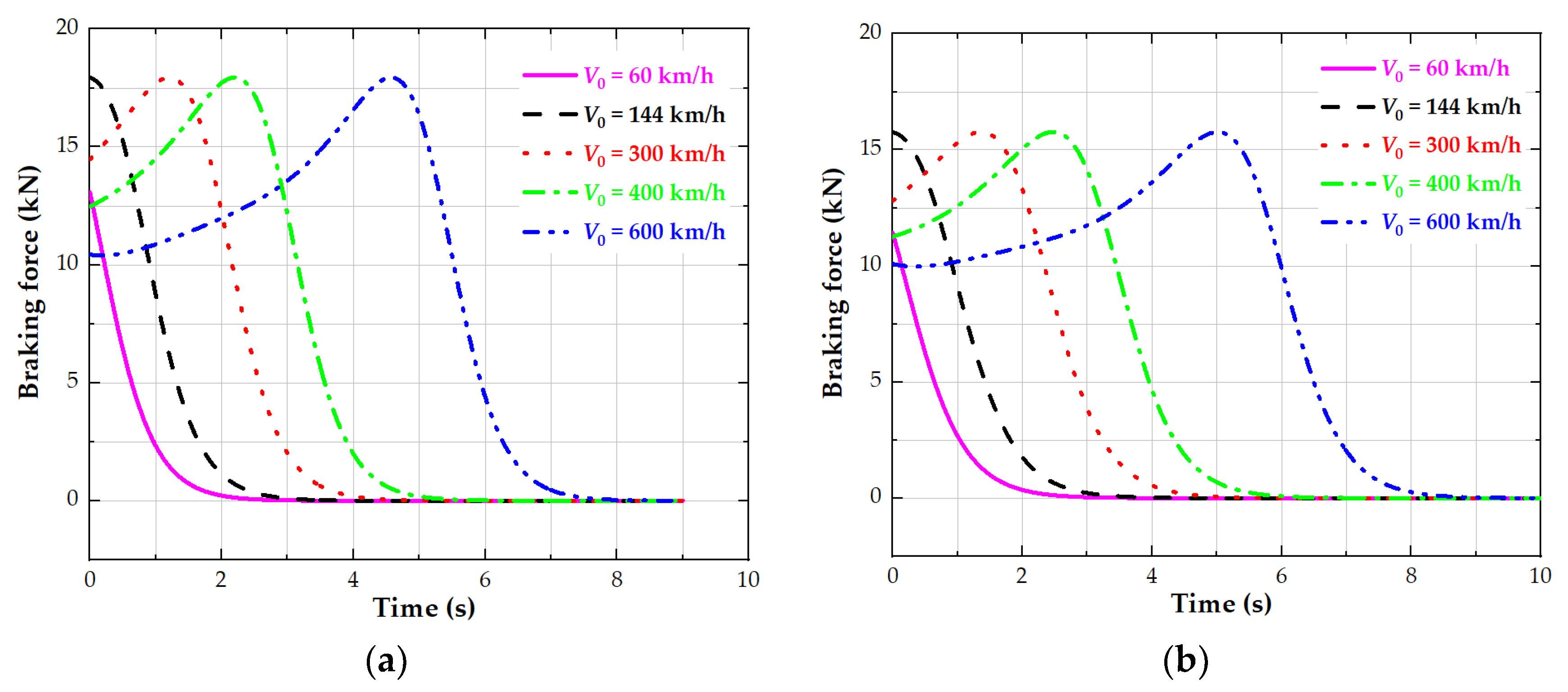
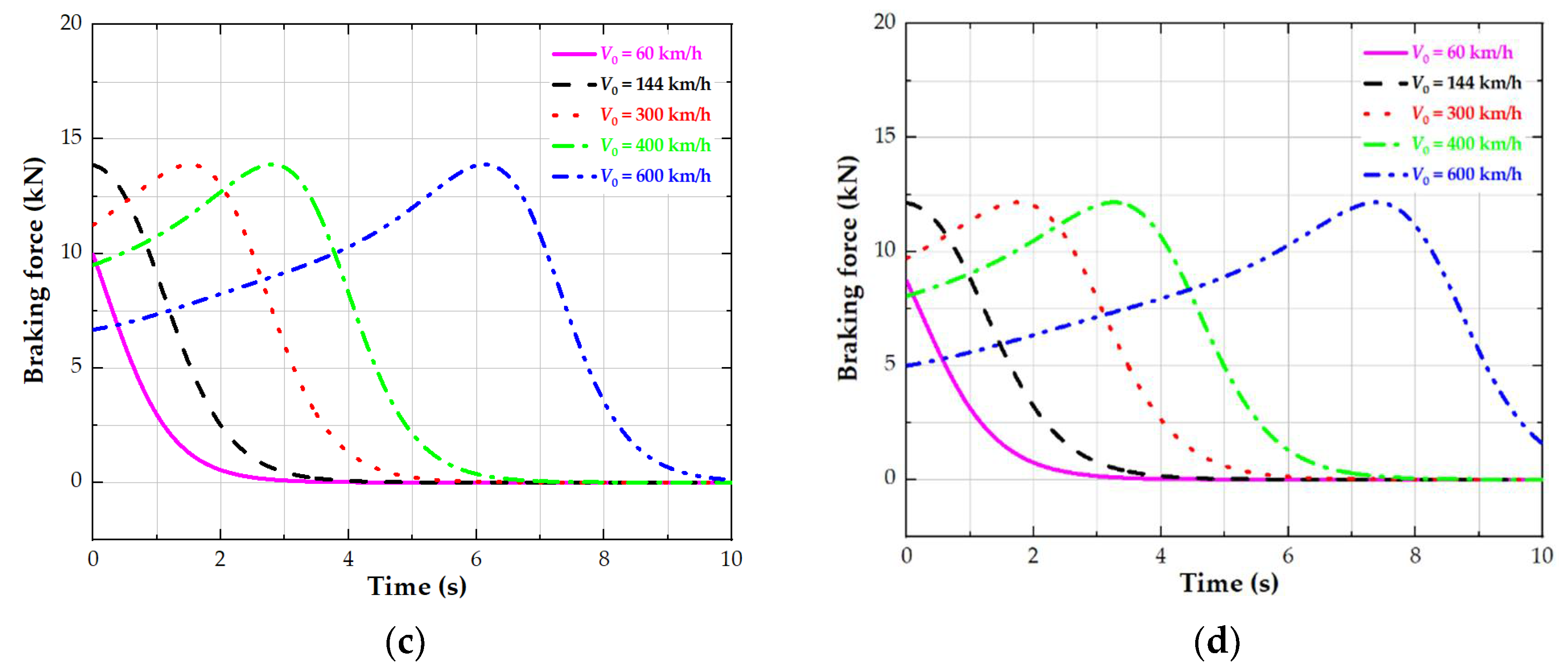
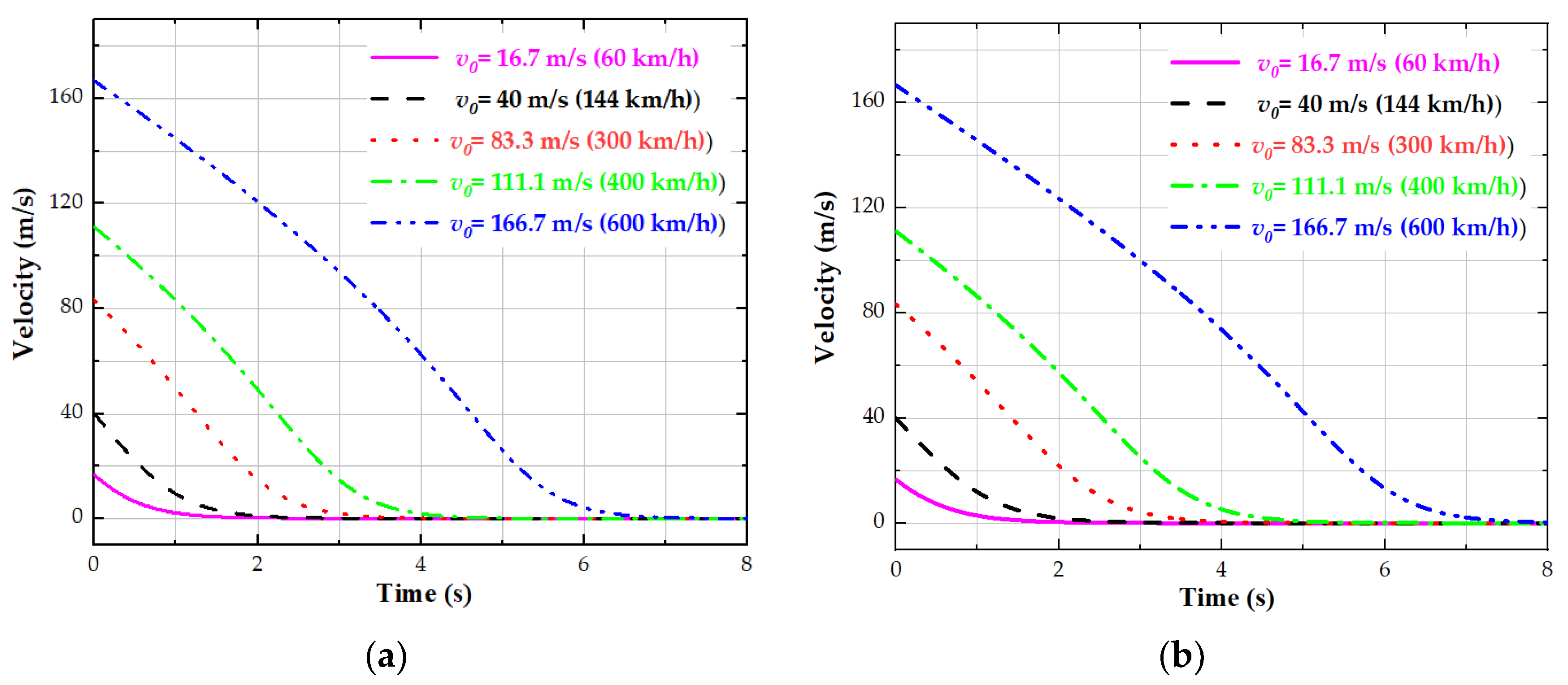
| Air Gap (mm) | 5 | 10 | 15 | 20 |
|---|---|---|---|---|
| Maximum detent force (kN) | 35.5 | 31 | 27.5 | 24.5 |
| Air Gap (mm) | a | b | c | d | e | s | t |
|---|---|---|---|---|---|---|---|
| 5 | −282.4 | 2342.4 | −53.81 | 0.5436 | −0.00265 | 5.34441 × 10−6 | −1.57078 × 10−9 |
| 10 | −264.82 | 2035.1 | −45.85 | 0.4446 | −0.00197 | 2.91624 × 10−6 | 1.92904 × 10−9 |
| 15 | −216.2 | 1754.05 | −38.67 | 0.3682 | −0.00162 | 2.44635 × 10−6 | 1.242 × 10−9 |
| 20 | −193.9 | 1531.10 | −33.40 | 0.3085 | −0.00126 | 1.32381 × 10−6 | 2.63298 × 10−9 |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Length of bogie | 3500 mm | Number of Dewars | 8 |
| Width of bogie | 2630 mm | Number of PMECBs | 4 |
| Bogie frame mass | 490 kg | Number of air springs | 2 |
| Carbody mass | 200 kg | Number of rubber springs | 4 |
| Motor mass | 700 kg | Initial speed | 60–600 km/h |
| Gauge | 2000 mm | Total weight | 1.91 t |
| Dewar mass | 33 kg | Levitation gap | 10~20 mm |
| Eddy current Brake mass | 63 kg | Length of the line | 165 m |
| Air Gap (mm) | 5 | 10 | 15 | 20 | ||||
|---|---|---|---|---|---|---|---|---|
| Velocity (km/h) | 60 | 600 | 60 | 600 | 60 | 600 | 60 | 600 |
| Braking time (s) | 1.5 | 7 | 1.0 | 8 | 2.0 | 9.5 | 3.0 | 11 |
| Braking distance (m) | 8 | 530 | 9 | 580 | 10 | 730 | 10 | 885 |
| Maximum braking force of the whole vehicle (kN) | 52 | 71 | 46 | 62 | 40 | 55 | 35 | 49 |
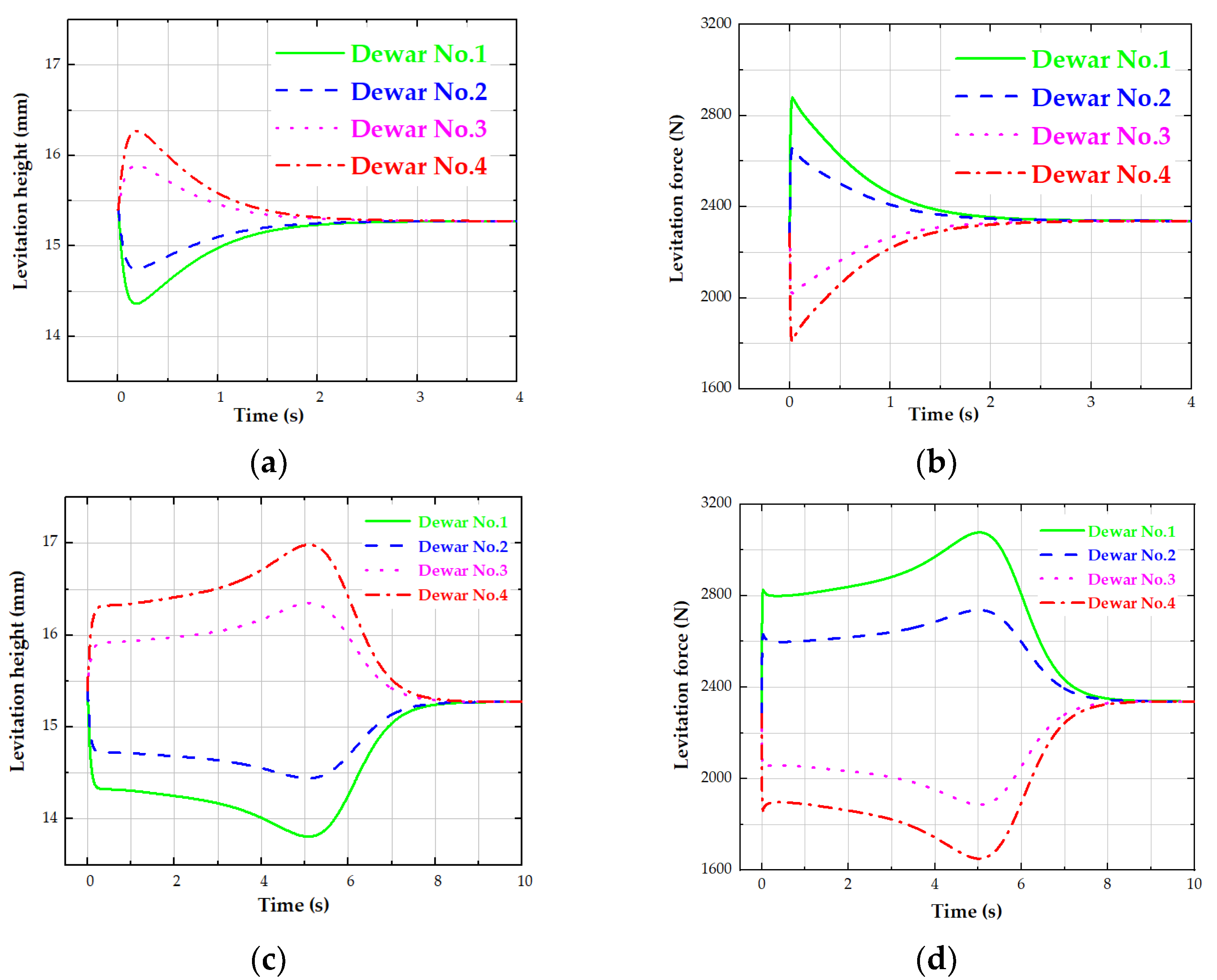
| Maglev Dewars | No. 1 | No. 2 | No. 3 | No. 4 |
|---|---|---|---|---|
| Stable levitation height (mm) | 15.3 | 15.3 | 15.3 | 15.3 |
| Stable levitation force (N) | 2340 | 2340 | 2340 | 2340 |
| Actual minimum levitation height (mm) | 14.3 | 14.8 | 15.8 | 16.3 |
| Actual minimum levitation force (N) | 2880 | 2650 | 2030 | 1820 |
| Levitation height change (mm) | −1.0 | −0.5 | +1.0 | +0.5 |
| Levitation force change (N) | +540 | +310 | −310 | −520 |
| Maglev Dewars | No. 1 | No. 2 | No. 3 | No. 4 |
|---|---|---|---|---|
| Stable levitation height (mm) | 15.3 | 15.3 | 15.3 | 15.3 |
| Stable levitation force (N) | 2340 | 2340 | 2340 | 2340 |
| Actual minimum levitation height (mm) | 13.8 | 14.5 | 16.1 | 16.8 |
| Actual minimum levitation force (N) | 3080 | 2750 | 1880 | 1640 |
| Levitation height change (mm) | −1.5 | −0.8 | +0.8 | +1.5 |
| Levitation force change (N) | +740 | +590 | −540 | −700 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, G.; Zhu, J.; Li, Y.; Yuan, Y.; Xiang, Y.; Lin, P.; Wang, L.; Liu, J.; Liang, L.; Deng, Z. Simulation of the Braking Effects of Permanent Magnet Eddy Current Brake and Its Effects on Levitation Characteristics of HTS Maglev Vehicles. Actuators 2022, 11, 295. https://doi.org/10.3390/act11100295
Zhang G, Zhu J, Li Y, Yuan Y, Xiang Y, Lin P, Wang L, Liu J, Liang L, Deng Z. Simulation of the Braking Effects of Permanent Magnet Eddy Current Brake and Its Effects on Levitation Characteristics of HTS Maglev Vehicles. Actuators. 2022; 11(10):295. https://doi.org/10.3390/act11100295
Chicago/Turabian StyleZhang, Gaowei, Jianmei Zhu, Yan Li, Yuhang Yuan, Yuqing Xiang, Peng Lin, Li Wang, Jianxin Liu, Le Liang, and Zigang Deng. 2022. "Simulation of the Braking Effects of Permanent Magnet Eddy Current Brake and Its Effects on Levitation Characteristics of HTS Maglev Vehicles" Actuators 11, no. 10: 295. https://doi.org/10.3390/act11100295
APA StyleZhang, G., Zhu, J., Li, Y., Yuan, Y., Xiang, Y., Lin, P., Wang, L., Liu, J., Liang, L., & Deng, Z. (2022). Simulation of the Braking Effects of Permanent Magnet Eddy Current Brake and Its Effects on Levitation Characteristics of HTS Maglev Vehicles. Actuators, 11(10), 295. https://doi.org/10.3390/act11100295








