The Effect of Spool Displacement Control to the Flow Rate in the Piezoelectric Stack-Based Valve System Subjected to High Operating Temperature
Abstract
1. Introduction
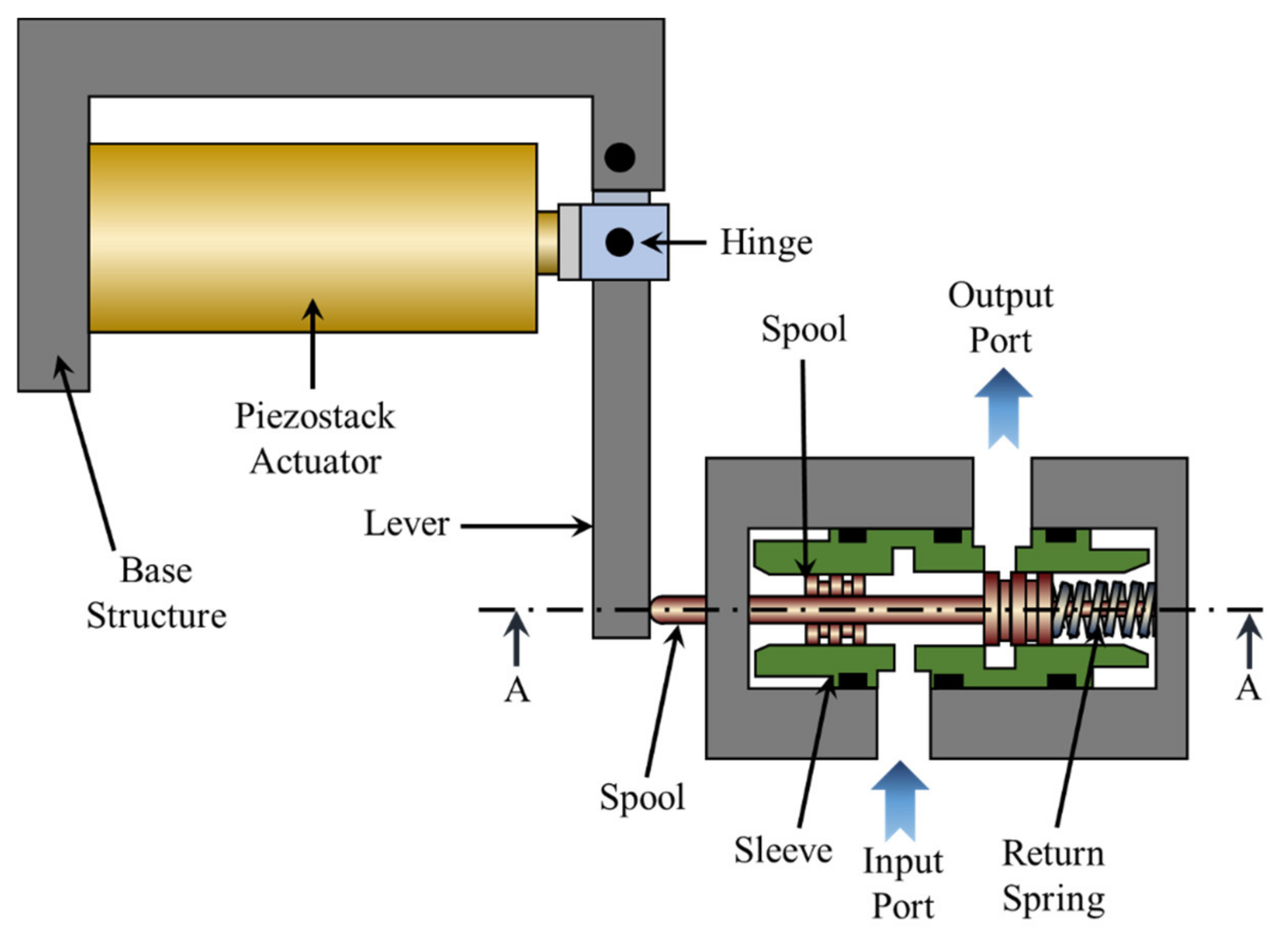
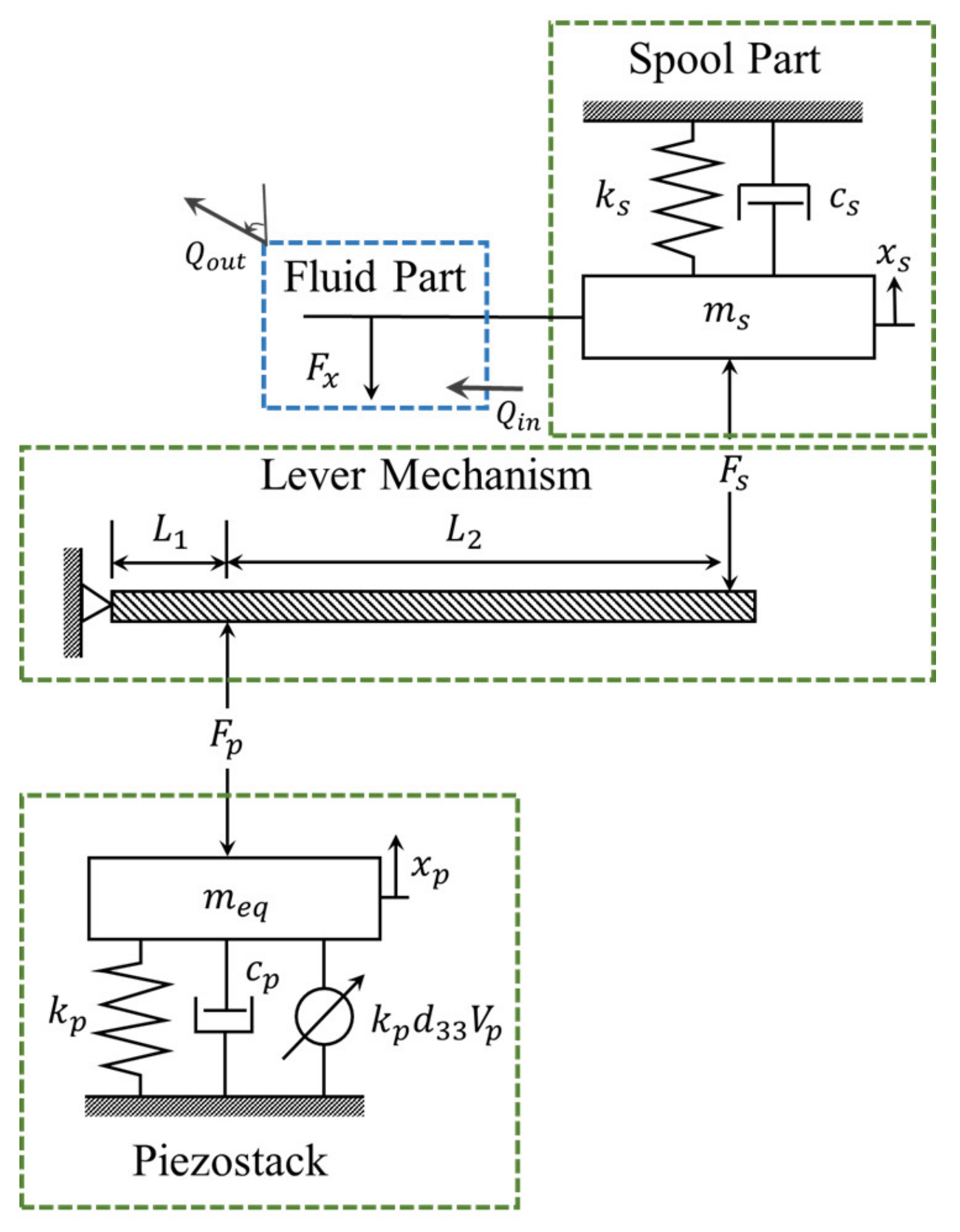
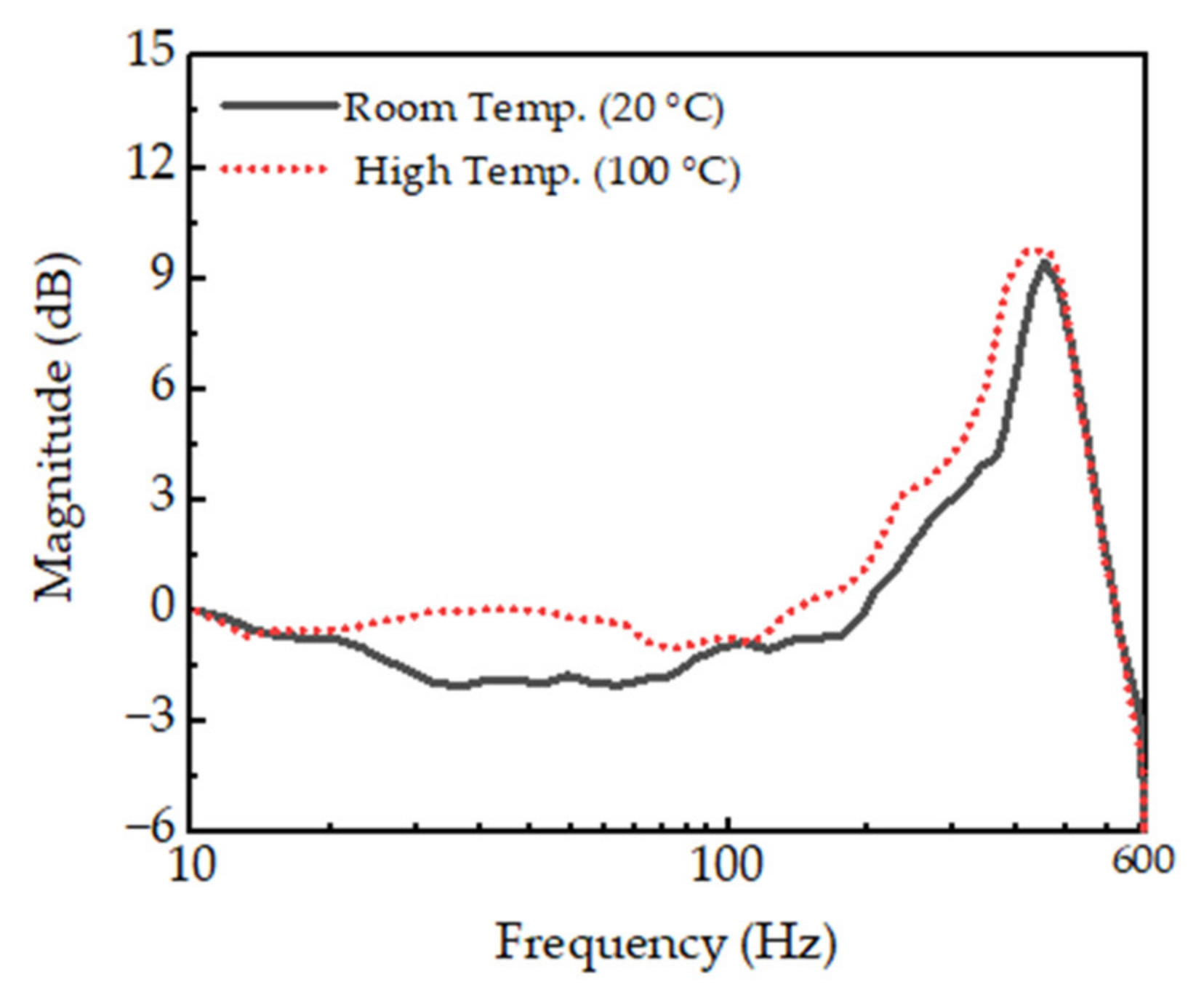
2. Modeling of Valve System
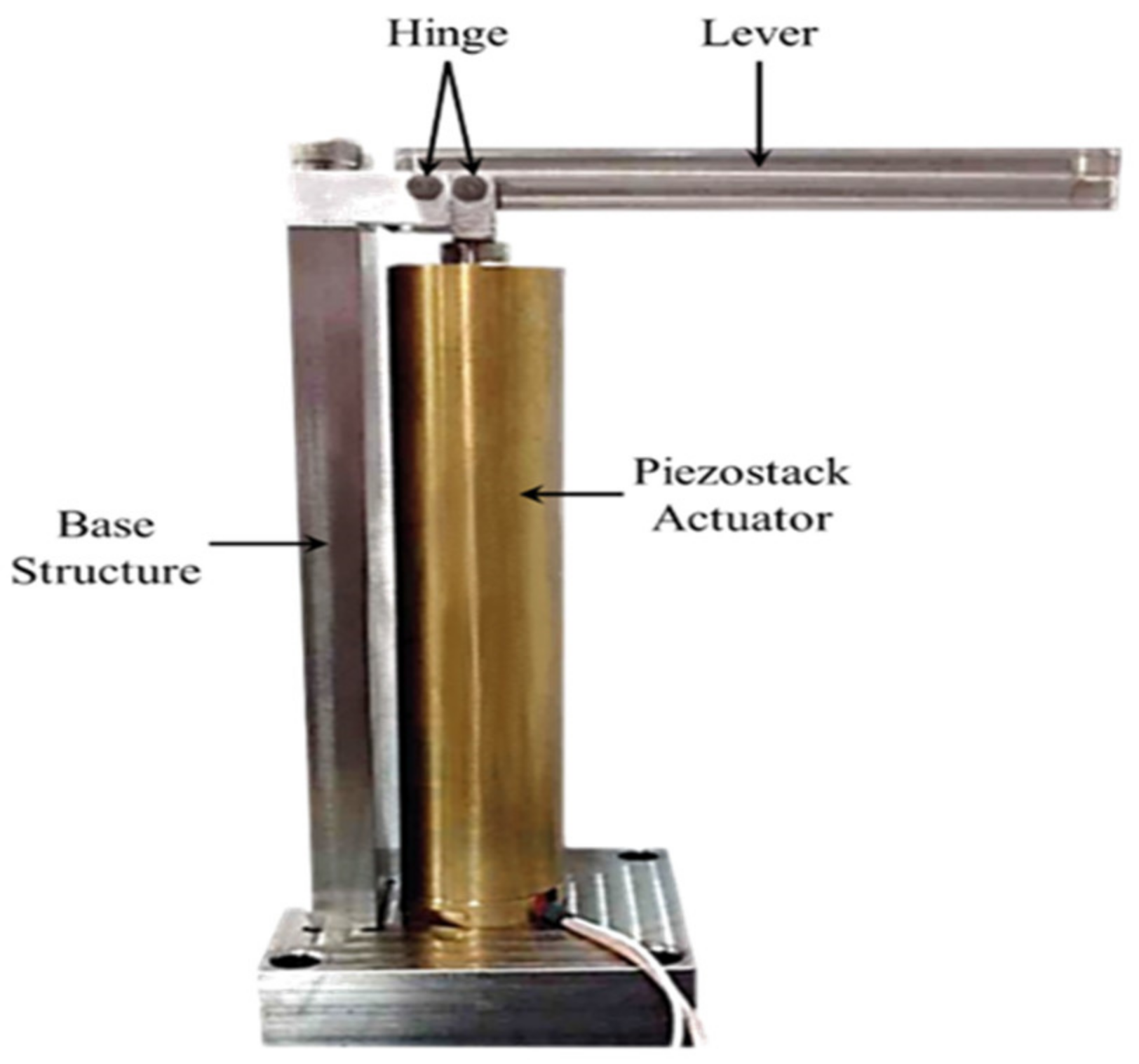
3. Design and Manufacturing of PSAVS
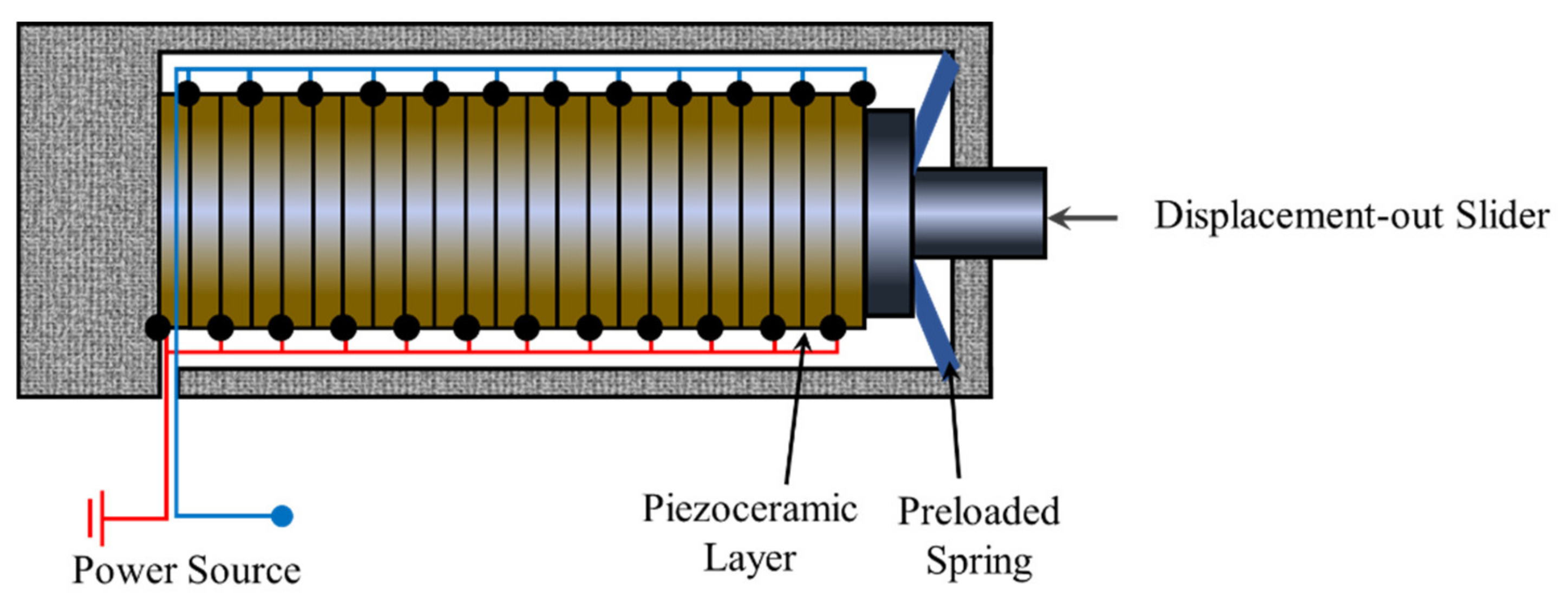
3.1. Piezostack Actuator
3.2. Lever Mechanism
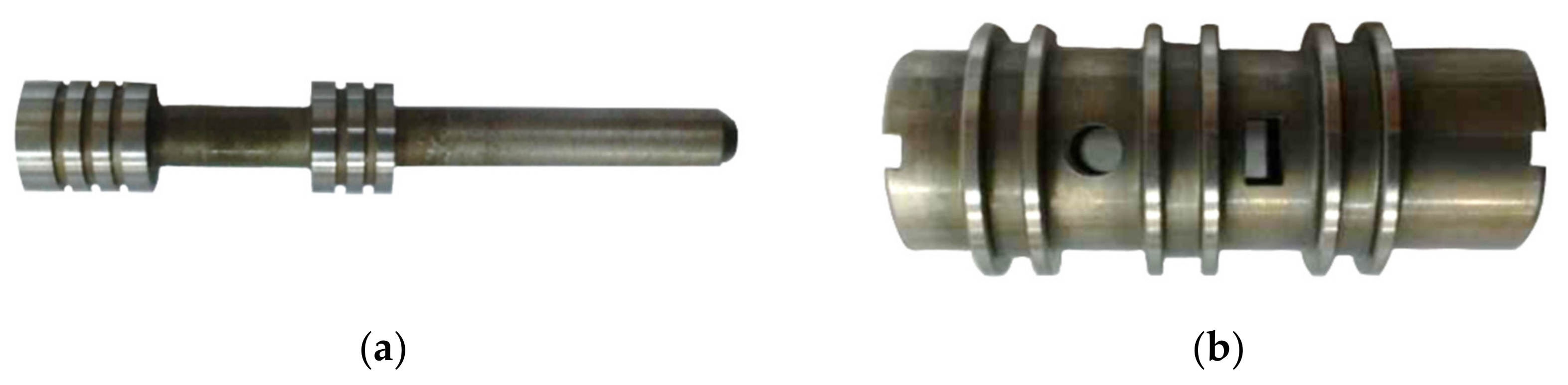
3.3. Spool Part
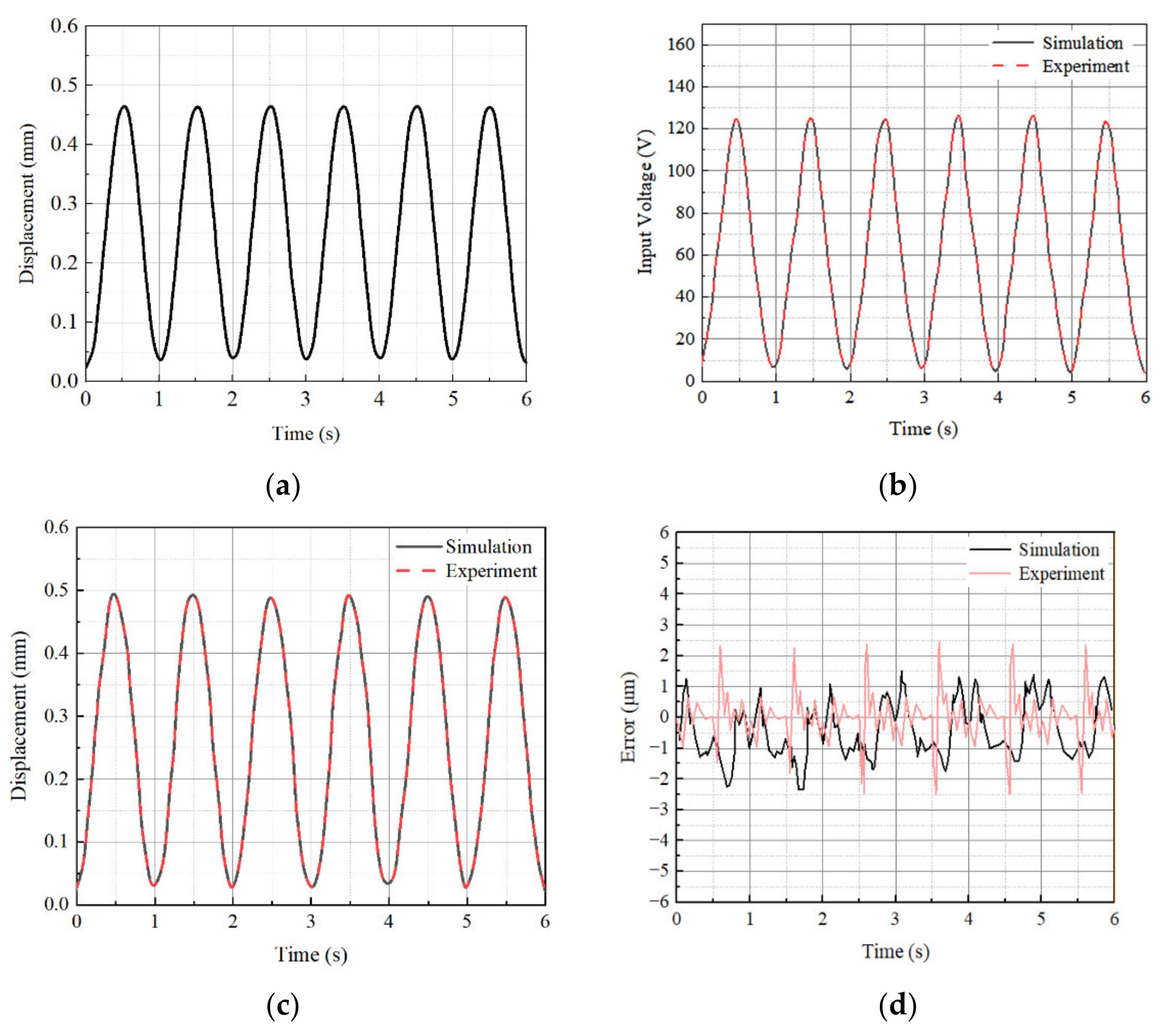
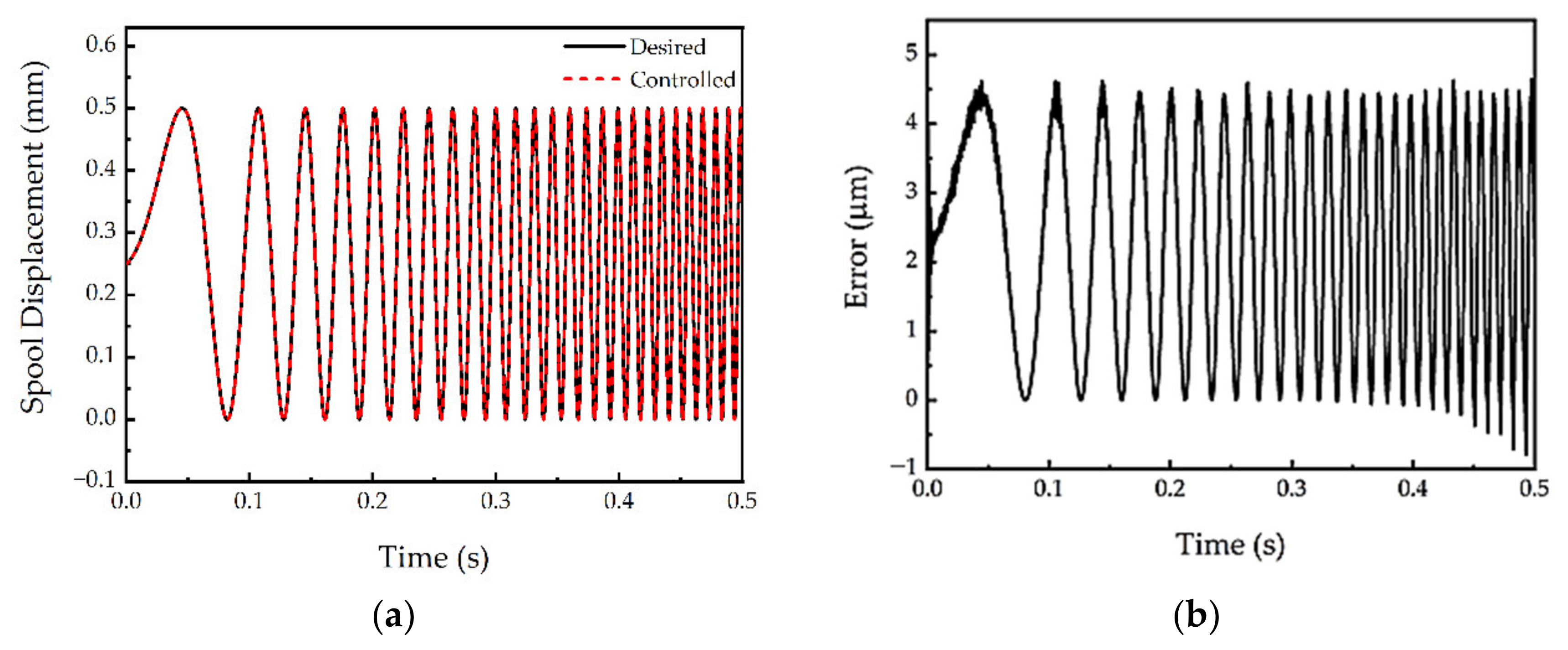
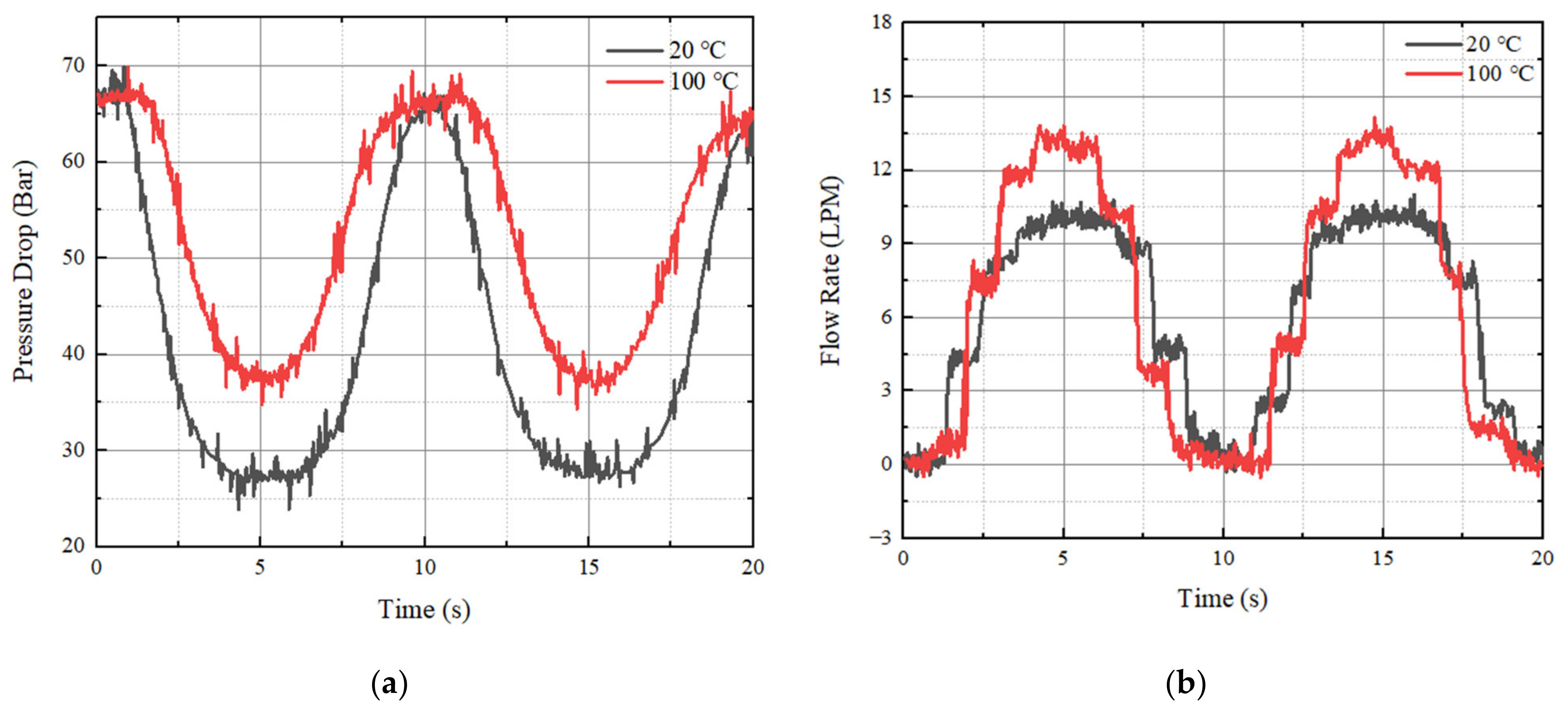
4. Control Performance
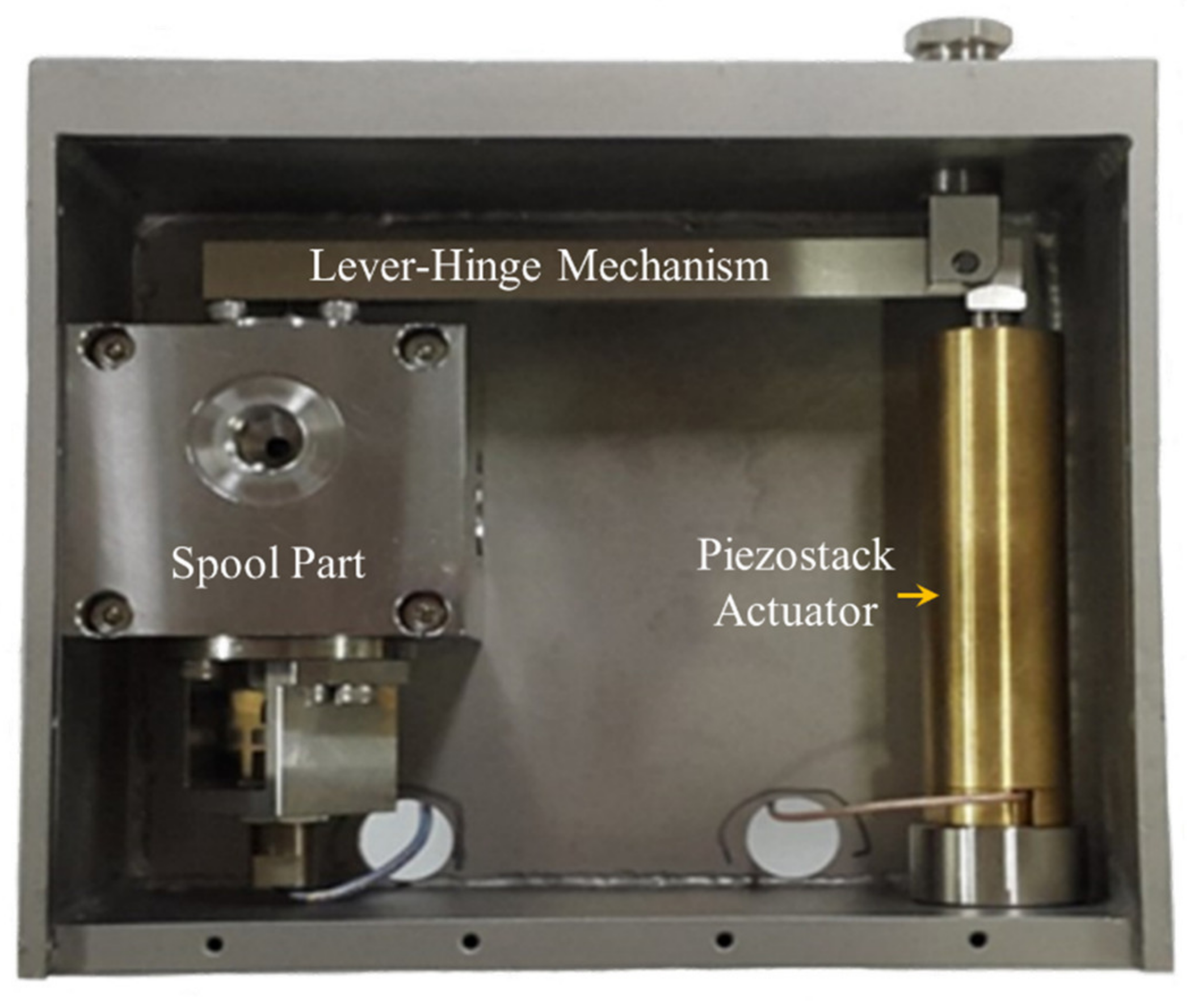
4.1. An Experimental Apparatus
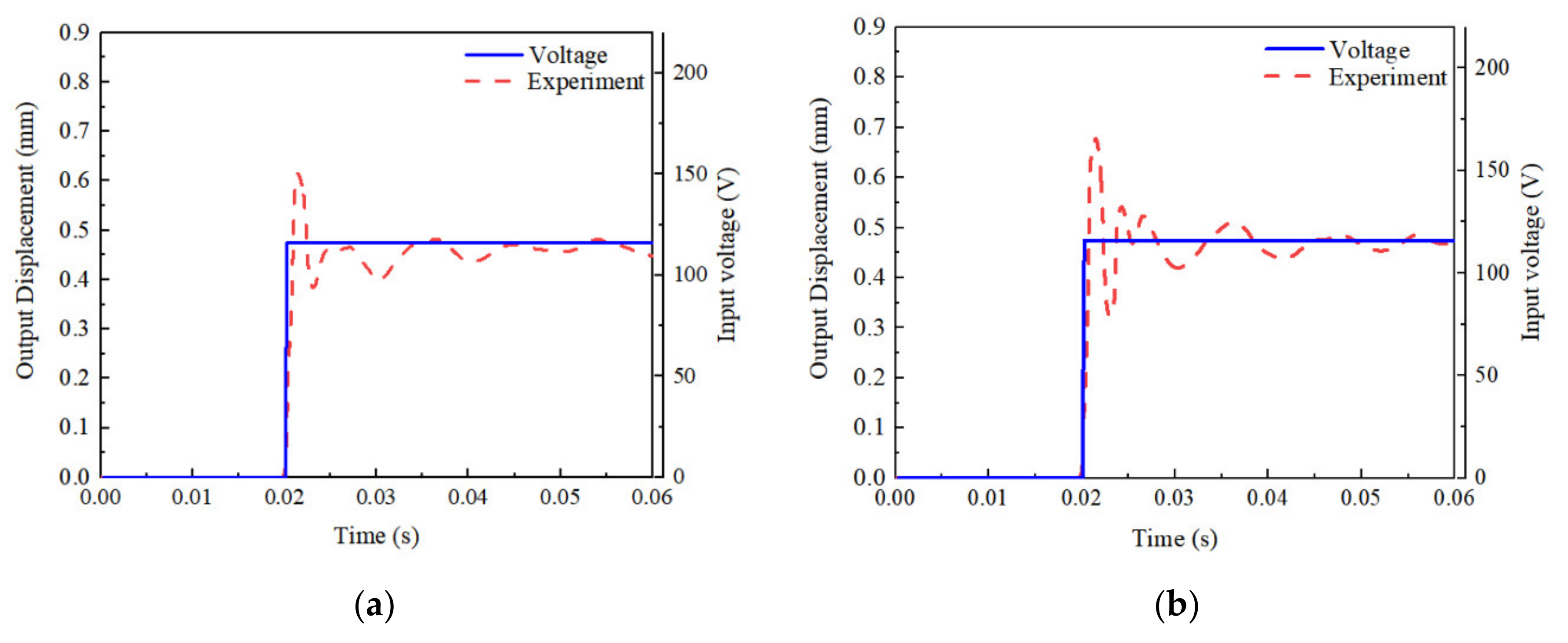
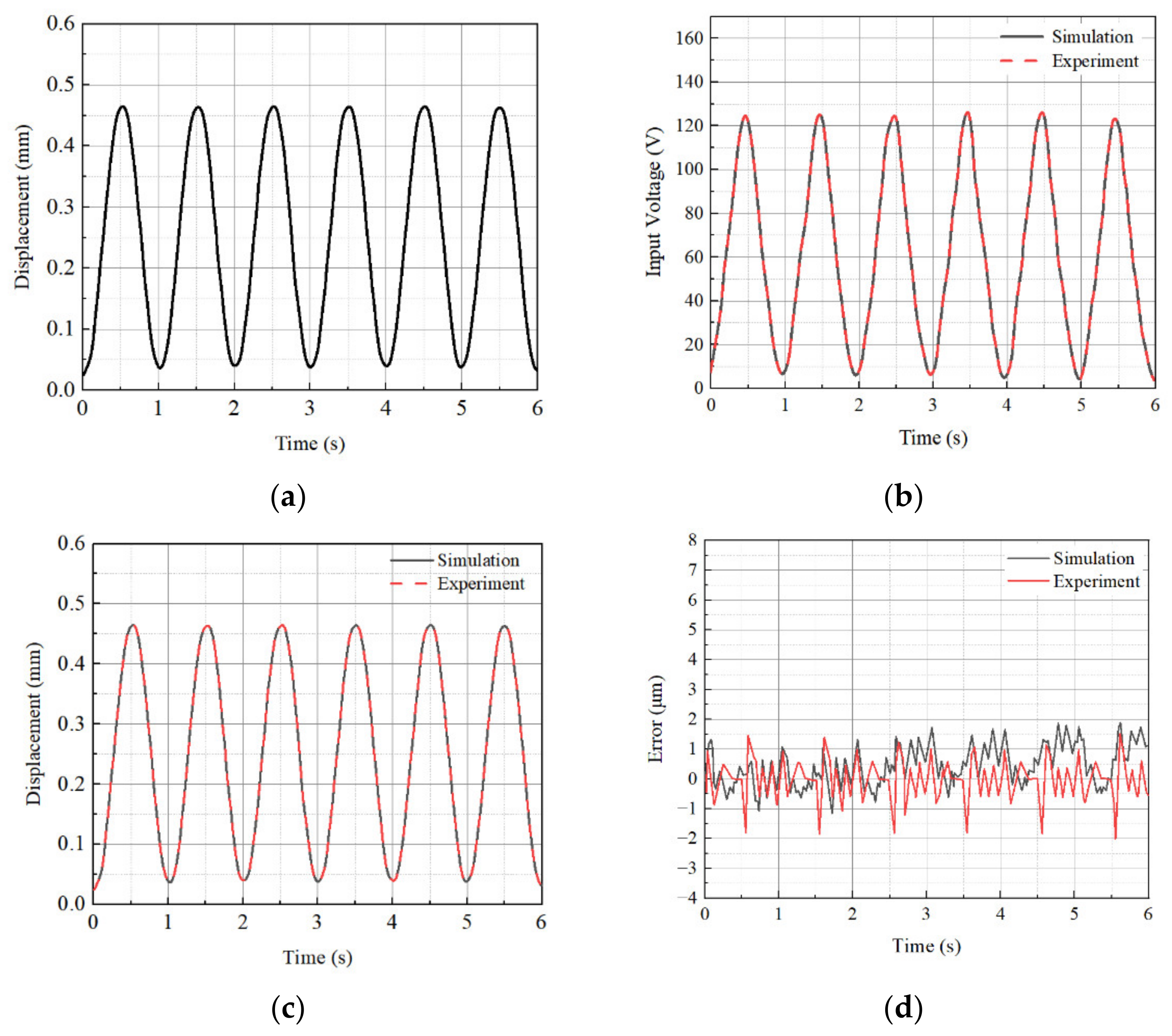
4.2. Spool Displacement Control
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Niezrecki, C.; Brei, D.; Balakrishnan, S.; Moskalik, A. Piezoelectric Actuation: State of the Art. Shock. Vib. Dig. 2001, 33, 269–280. [Google Scholar] [CrossRef]
- Kim, J.H.; Kim, S.H.; Kwak, Y.K. Development of a piezoelectric actuator using a three-dimensional bridge-type hinge mechanism. Rev. Sci. Instrum. 2003, 74, 2918–2924. [Google Scholar] [CrossRef]
- Juuti, J.; Kordás, K.; Lonnakko, R.; Moilanen, V.-P.; Leppävuori, S. Mechanically amplified large displacement piezoe-lectric actuators. Sens. Actuators A Phys. 2005, 120, 225–231. [Google Scholar] [CrossRef]
- Choi, S.; Han, S.; Lee, Y. Fine motion control of a moving stage using a piezoactuator associated with a displacement am-plifier. Smart Mater. Struct. 2004, 14, 222. [Google Scholar] [CrossRef]
- Nguyen, Q.-H.; Choi, S.-B.; Kim, J.-D. The design and control of a jetting dispenser for semiconductor electronic packaging driven by a piezostack and a flexible beam. Smart Mater. Struct. 2008, 17, 065028. [Google Scholar] [CrossRef]
- Lindler, J.E.; Anderson, E.H. Piezoelectric direct drive servovalve. In Proceedings of the Smart Structures and Materials 2002: Industrial and Commercial Applications of Smart Structures Technologies, San Diego, CA, USA, 9 July 2002; International Society for Optics and Photonics: Bellingham, WA, USA, 2002; pp. 488–496. [Google Scholar]
- Zhou, M.-L.; Yang, Z.-G.; Gao, W.; Tian, Y.-T.; Shen, C.-L.; Li, P. Fuzzy control of a new type of piezoelectric direct drive electro-hydraulic servo valve. In Proceedings of the 2005 International Conference on Machine Learning and Cybernetics, Guangzhou, China, 18–21 August 2005; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2005; Volume 2, pp. 819–824. [Google Scholar]
- Changbin, G.; Zongxia, J. A piezoelectric direct-drive servo valve with a novel multi-body contacting spool-driving mecha-nism: Design, modelling and experiment. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2014, 228, 169–185. [Google Scholar] [CrossRef]
- Jeon, J.; Nguyen, Q.-H.; Han, Y.-M.; Choi, S.-B. Design and Evaluation of a Direct Drive Valve Actuated by Piezostack Actuator. Adv. Mech. Eng. 2013, 5, 986812. [Google Scholar] [CrossRef]
- Niles, W.D.; Coassin, P.J. Piezo- and Solenoid Valve-Based Liquid Dispensing for Miniaturized Assays. ASSAY Drug Dev. Technol. 2005, 3, 189–202. [Google Scholar] [CrossRef]
- Sun, J.; Ng, J.H.; Fuh, Y.H.; Wong, Y.S.; Loh, H.T.; Xu, Q. Comparison of micro-dispensing performance between micro-valve and piezoelectric printhead. Microsyst. Technol. 2009, 15, 1437–1448. [Google Scholar] [CrossRef]
- Kamali, M.; Jazayeri, S.A.; Najafi, F.; Kawashima, K.; Kagawa, T. Study on the performance and control of a pie-zo-actuated nozzle-flapper valve with an isothermal chamber. Stroj. Vestn. J. Mech. Eng. 2016, 62, 318–328. [Google Scholar] [CrossRef][Green Version]
- Haus, B.; Aschemann, H.; Mercorelli, P.; Werner, N. Nonlinear modelling and sliding mode control of a pie-zo-hydraulic valve system. In Proceedings of the 2016 21st International Conference on Methods and Models in Automation and Robotics (MMAR), Miedzyzdroje, Poland, 29 August–1 September 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 442–447. [Google Scholar]
- Mohith, S.; Rao, M.; Karanth, N.; Kulkarni, S.; Upadhya, A.R. Development and assessment of large stroke pie-zo-hydraulic actuator for micro positioning applications. Precis. Eng. 2021, 67, 324–338. [Google Scholar]
- Poik, M.; Kohl, D.; Schitter, G. Similarity-based Feedback Control for Linear Operation of Piezoelectric Actuators. In Proceedings of the 2018 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Auckland, New Zealand, 9–12 July 2018; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2018; pp. 97–102. [Google Scholar]
- Bußmann, A.B.; Durasiewicz, C.P.; Kibler, S.H.A.; Wald, C.K. Piezoelectric titanium based microfluidic pump and valves for implantable medical applications. Sens. Actuators A Phys. 2021, 323, 112649. [Google Scholar] [CrossRef]
- Goettsche, T.; Kohnle, J.; Willmann, M.; Ernst, H.; Spieth, S.; Tischler, R.; Messner, S.; Zengerle, R.; Sandmaier, H. Novel approaches to particle tolerant valves for use in drug delivery systems. Sens. Actuators A Phys. 2005, 118, 70–77. [Google Scholar] [CrossRef]
- Zhao, Y.; Huang, X.; Liu, Y.; Wang, G.; Hong, K. Design and Control of a Piezoelectric-Driven Microgripper Perceiving Displacement and Gripping Force. Micromachines 2020, 11, 121. [Google Scholar] [CrossRef] [PubMed]
- Jang, S.; Oh, J.H. Rapid Fabrication of Microporous BaTiO3/PDMS Nanocomposites for Triboelectric Nanogenerators through One-step Microwave Irradiation. Sci. Rep. 2018, 8, 14287. [Google Scholar] [CrossRef]
- Persson, L.J.; Plummer, A.R.; Bowen, C.R.; Brooks, I. Design and modelling of a novel servovalve actuated by a pie-zoelectric ring bender. In Fluid Power Systems Technology; American Society of Mechanical Engineers: New York, NY, USA, 2015; p. V001T01A043. [Google Scholar]
- Stefanski, F.; Minorowicz, B.; Persson, J.; Plummer, A.; Bowen, C. Non-linear control of a hydraulic piezo-valve using a generalised Prandtl–Ishlinskii hysteresis model. Mech. Syst. Signal Process. 2017, 82, 412–431. [Google Scholar] [CrossRef]
- Wang, Y.; Guo, H.; Li, Y.; Liu, N.; Yang, C. Active control for vehicle interior noise based on DWT-FxLMS algorithm using a piezoelectric feedback system. Appl. Acoust. 2020, 167, 107409. [Google Scholar] [CrossRef]
- Ding, J.; Cao, Y.; Chen, Q.; Dong, J.; Xu, Z.; Tian, D.; Liu, C.; Zhang, B.; Zeng, P.; Wu, Y.; et al. Development of a novel piezoelectric-actuated inertial pump using bionic valve. J. Intell. Mater. Syst. Struct. 2021. [Google Scholar] [CrossRef]
- Simic, M.; Herakovic, N. Characterization of energy consumption of new piezo actuator system used for hydraulic on/off valves. J. Clean. Prod. 2021, 284, 124748. [Google Scholar] [CrossRef]
- Lu, X.; Gao, Q.; Li, Y.; Yu, Y.; Zhang, X.; Qiao, G.; Cheng, T. A Linear Piezoelectric Stick-Slip Actuator via Triangular Displacement Amplification Mechanism. IEEE Access 2020, 8, 6515–6522. [Google Scholar] [CrossRef]
- Li, Y.W.; Zhou, X.L.; Li, F. Temperature-dependent mechanical depolarization of ferroelectric ceramics. J. Phys. D Appl. Phys. 2010, 43, 1–8. [Google Scholar] [CrossRef]
- Ohuchi, H.; Nakano, K.; Uchino, K.; Endoh, H.; Fukumoto, H. High-Speed Electro-Hydraulic Servovalves Using Electro-strictive Ceramic PMN Actuators. In Proceedings of the Fluid Control and Measurement International Symposium, Tokyo, Japan, 2–6 September 1985; Harada, M., Ed.; Pergamon Press: Oxford, UK, 1985; pp. 415–420. [Google Scholar]
- Pikorea. Available online: https://www.pikorea.co.kr/ko/technology/piezo-technology/properties-piezo-actuators/dynamic-operation/ (accessed on 23 August 2021).
- McCloy, D.; Martin, H.R. Control of Fluid Power: Analysis and Design; Ellis Horwood, Ltd.: Chichester, UK; Halsted Press: New York, NY, USA, 1980. [Google Scholar]
- Manring, N.D.; Fales, R.C. Hydraulic Control Systems; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]




| Specification | Symbol | Value |
|---|---|---|
| Damping coefficient of the piezo stack actuator | ||
| Stiffness of the piezo stack actuator | ||
| Free displacement of the piezo stack due to a unit of the applied voltage | ||
| Mass of the displacement-out slider | ||
| Density of the piezoelectric ceramic | ||
| Section area of the piezoelectric ceramic | ||
| Length of the piezoelectric stack | ||
| Maximum operating temperature | 90 °C |
| Specification | Symbol | Value |
|---|---|---|
| Length (Between the tip point of the piezo stack actuator and the base part hinge) | ||
| Length (Between the tip point of the piezo stack actuator and spool contacting part) | ||
| Height of the lever | ||
| Density of the lever | ||
| Section area of the lever |
| Specification | Symbol | Value |
|---|---|---|
| Mass of the spool and spool rod | ||
| Damping coefficient of the spool | ||
| Spring coefficient of the return spring | ||
| Diameter of the spool | ||
| Diameter of the spool rod | ||
| Diameter of the inlet port | ||
| Height of the outlet port | ||
| Overlap length | ||
| Width of the outlet port | ||
| Discharge coefficient | ||
| Density of the fluid (Room temperature) | ||
| Density of the fluid (High temperature) | ||
| Length between inlet port and outlet port | ||
| Jet angle |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, Y.-J.; Kim, B.-G.; Jeon, J.-C.; Jung, D.; Choi, S.-B. The Effect of Spool Displacement Control to the Flow Rate in the Piezoelectric Stack-Based Valve System Subjected to High Operating Temperature. Actuators 2021, 10, 239. https://doi.org/10.3390/act10090239
Park Y-J, Kim B-G, Jeon J-C, Jung D, Choi S-B. The Effect of Spool Displacement Control to the Flow Rate in the Piezoelectric Stack-Based Valve System Subjected to High Operating Temperature. Actuators. 2021; 10(9):239. https://doi.org/10.3390/act10090239
Chicago/Turabian StylePark, Yu-Jin, Bo-Gyu Kim, Jun-Cheol Jeon, Dongsoo Jung, and Seung-Bok Choi. 2021. "The Effect of Spool Displacement Control to the Flow Rate in the Piezoelectric Stack-Based Valve System Subjected to High Operating Temperature" Actuators 10, no. 9: 239. https://doi.org/10.3390/act10090239
APA StylePark, Y.-J., Kim, B.-G., Jeon, J.-C., Jung, D., & Choi, S.-B. (2021). The Effect of Spool Displacement Control to the Flow Rate in the Piezoelectric Stack-Based Valve System Subjected to High Operating Temperature. Actuators, 10(9), 239. https://doi.org/10.3390/act10090239







