Characterization and Analysis of a Flexural Shape Memory Alloy Actuator
Abstract
:1. Introduction
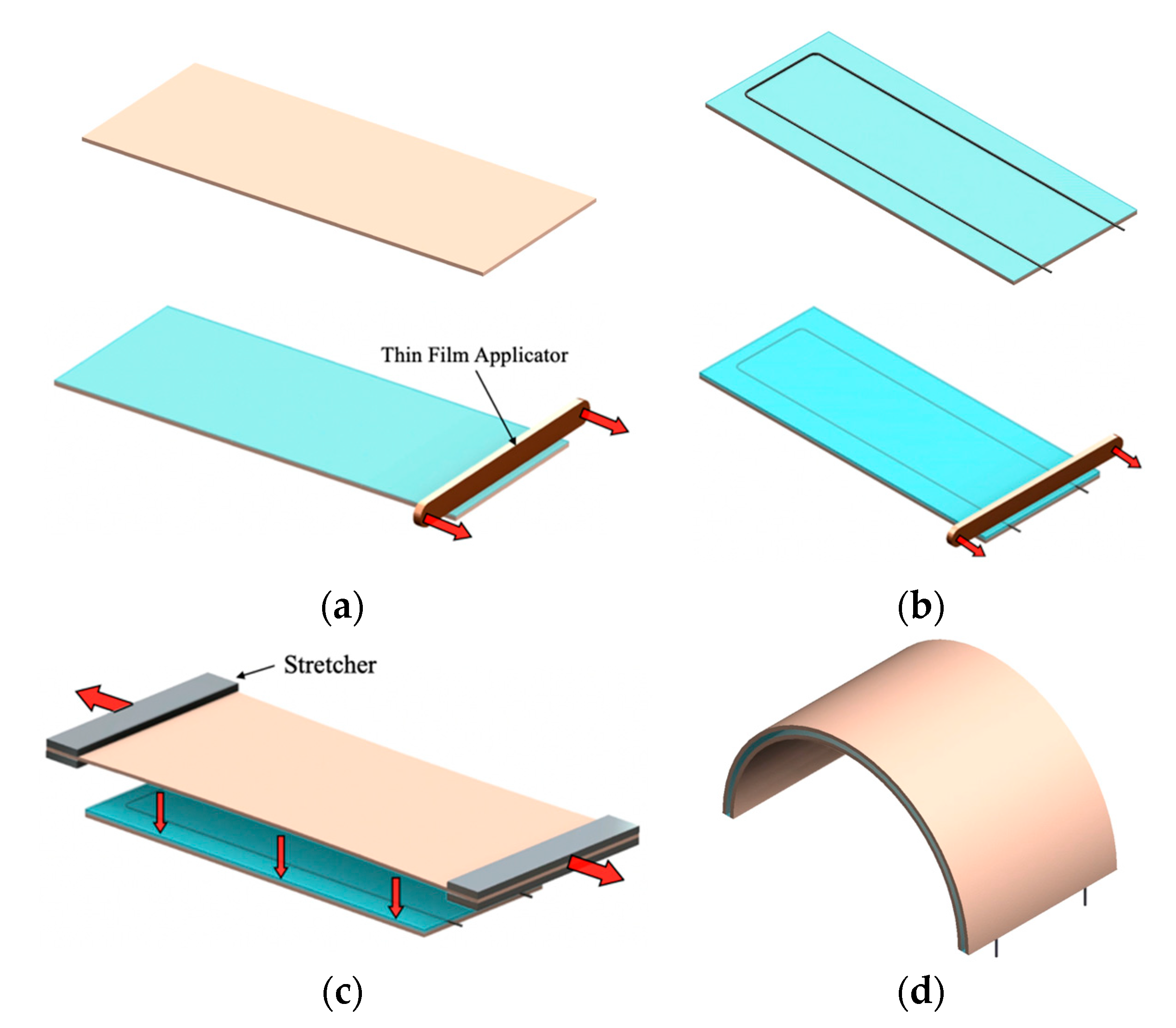
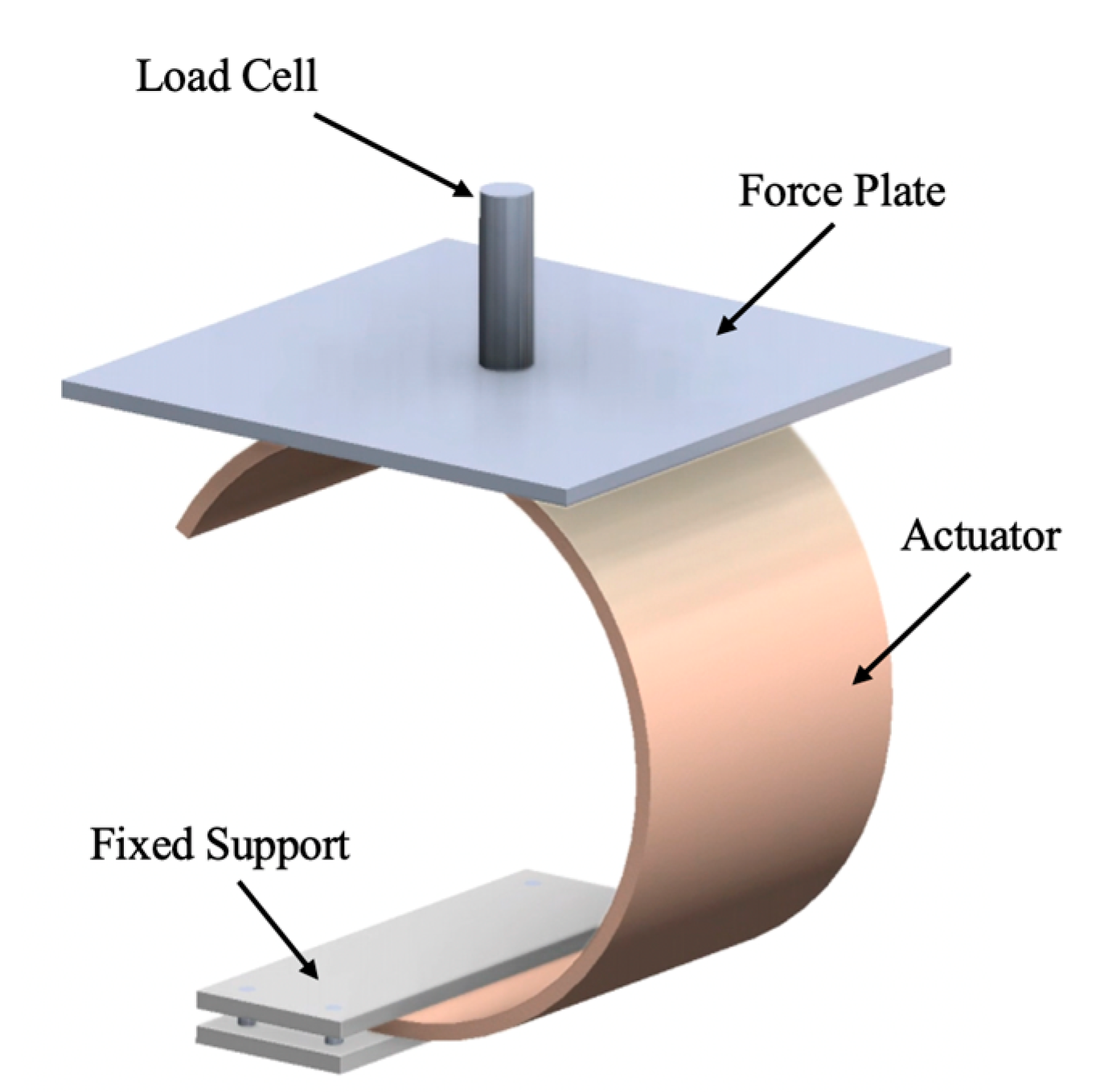
2. Actuator Design and Fabrication
3. Finite Element Analysis
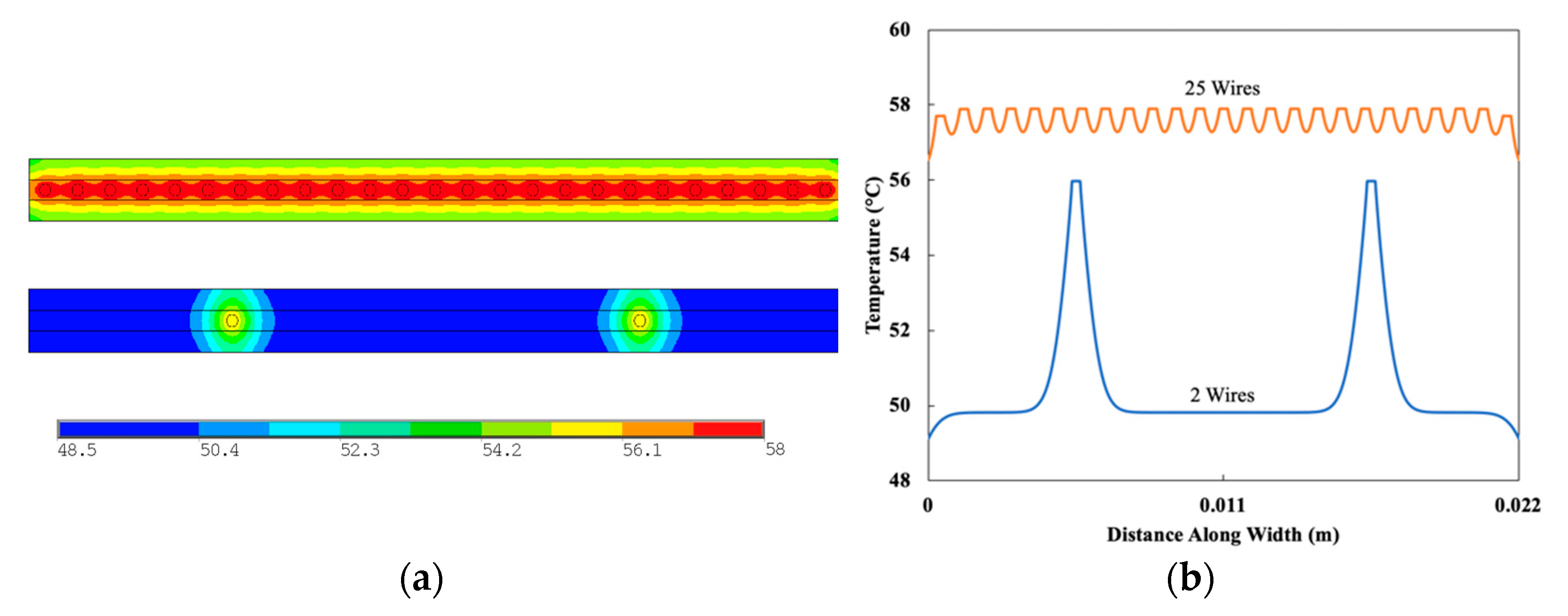
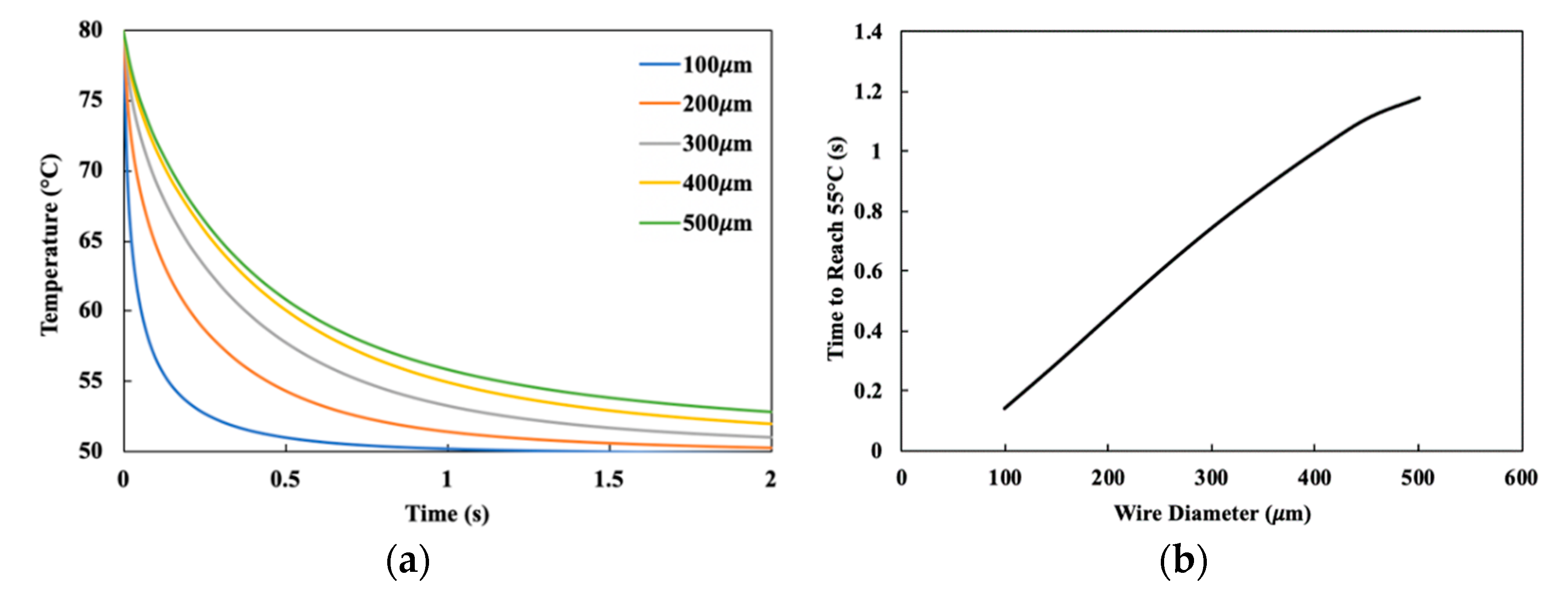
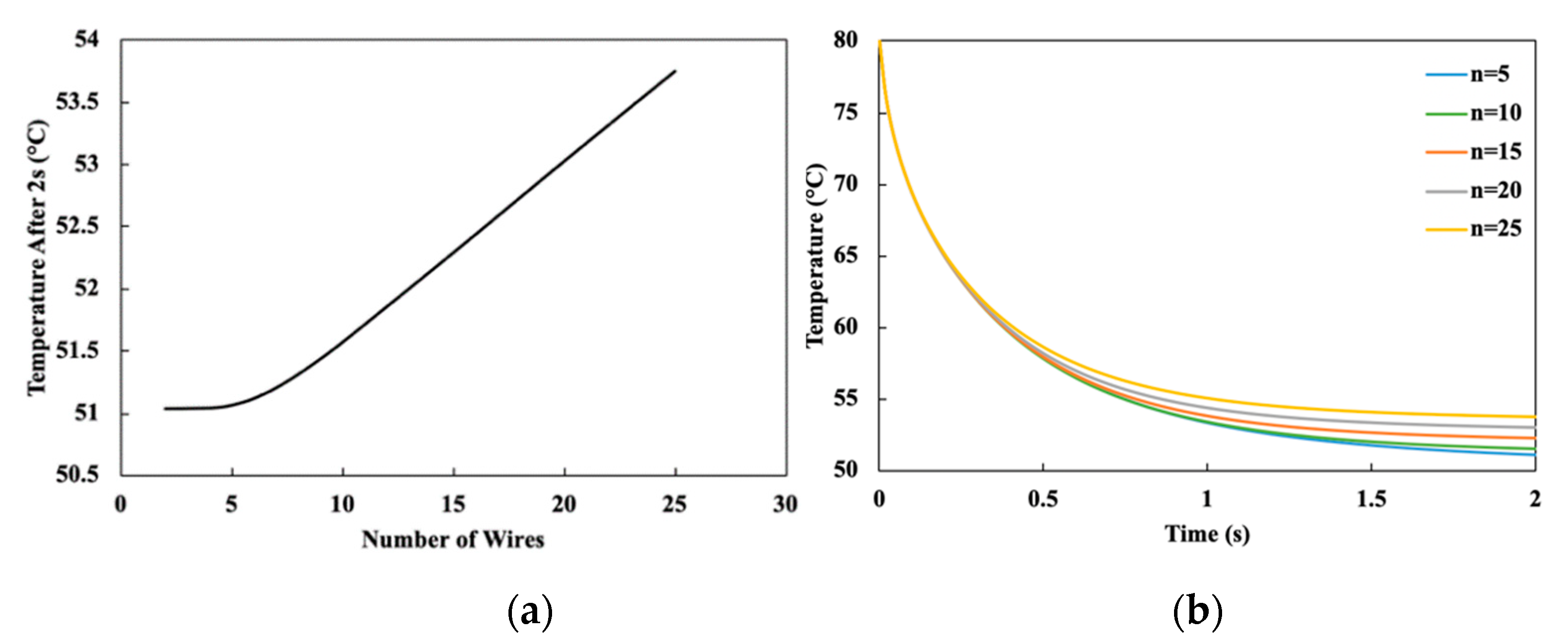
3.1. Thermal Analysis
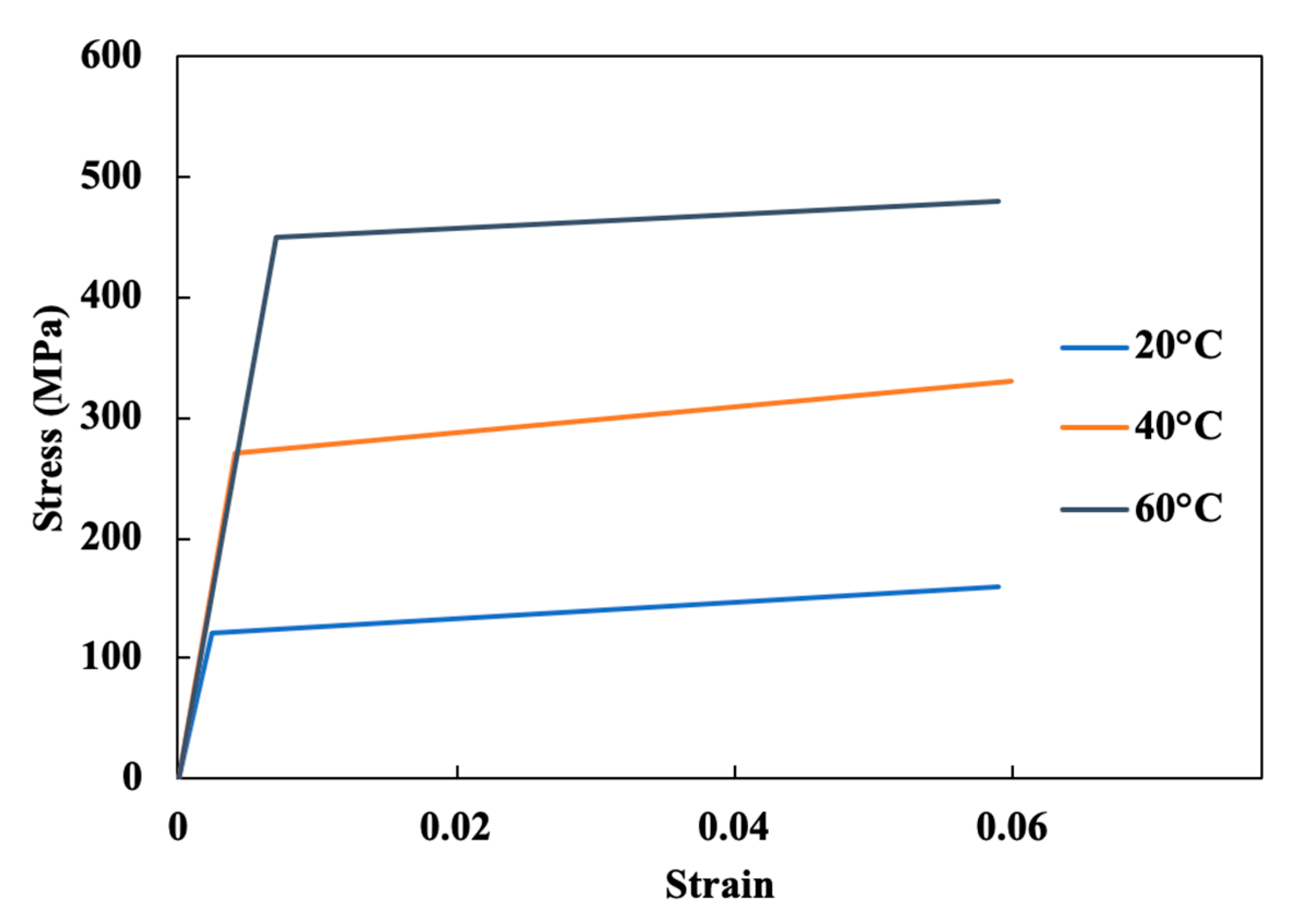
3.2. Mechanical Analysis
4. Experimental Testing
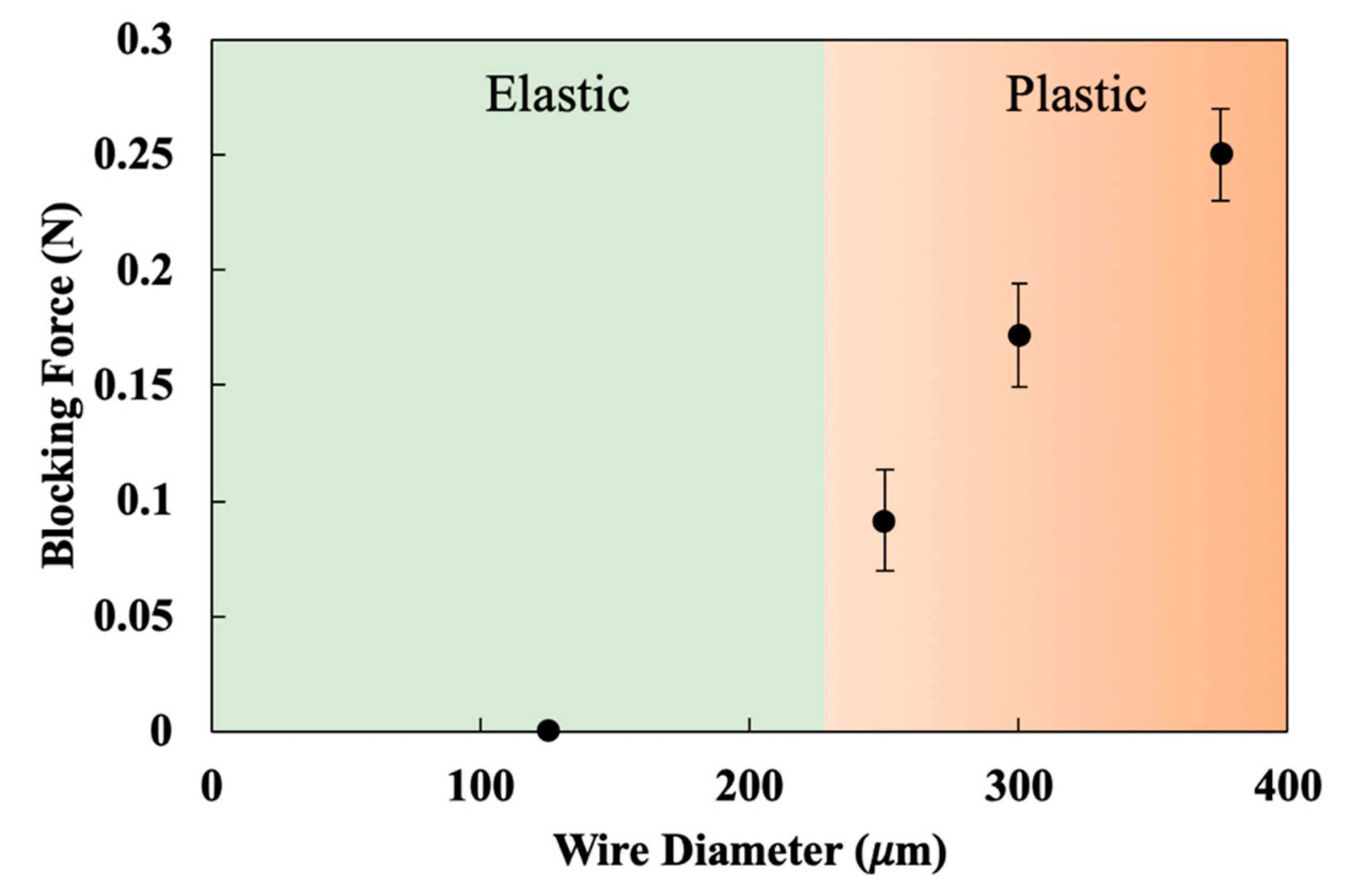
4.1. Blocking Force
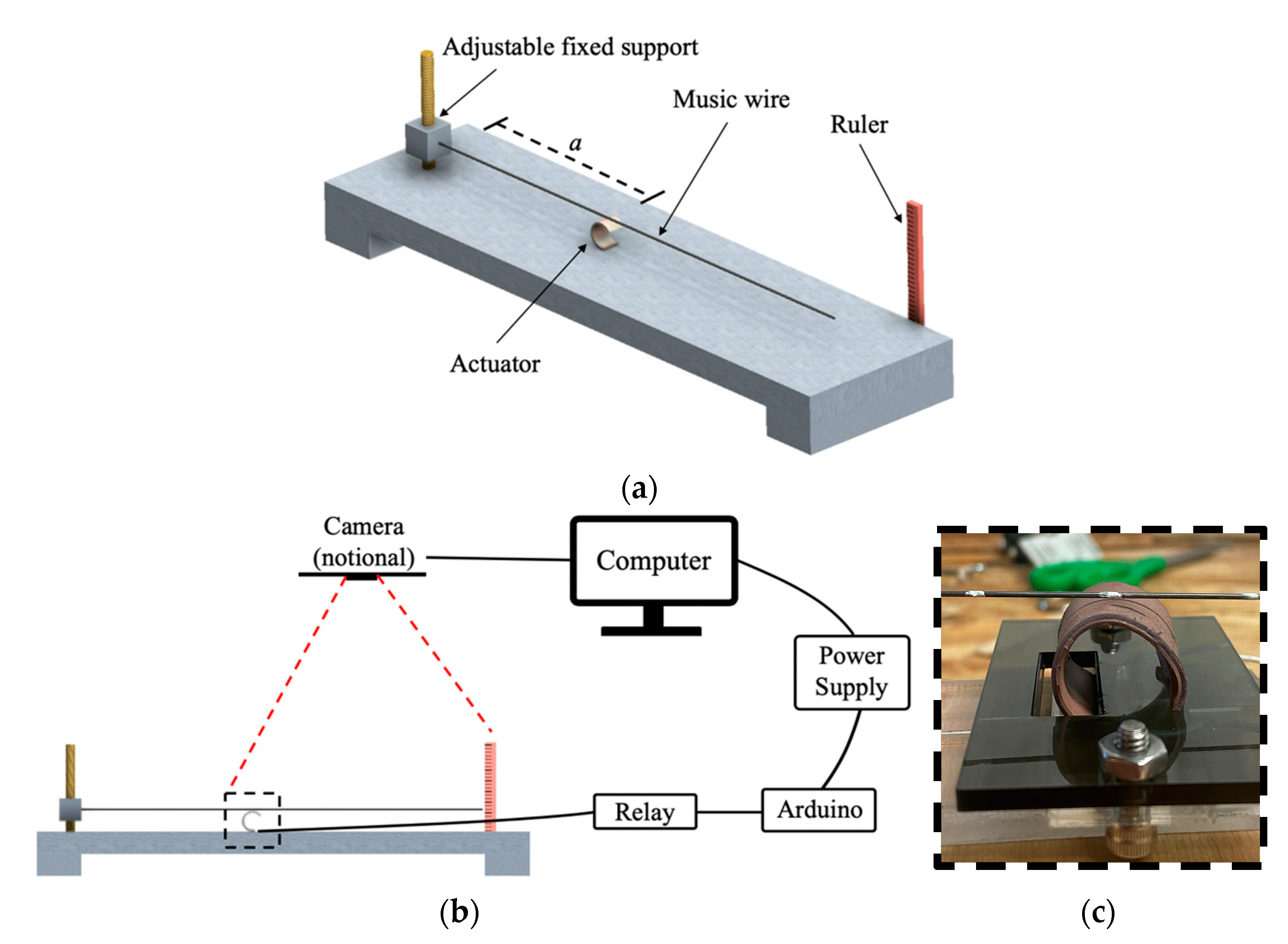
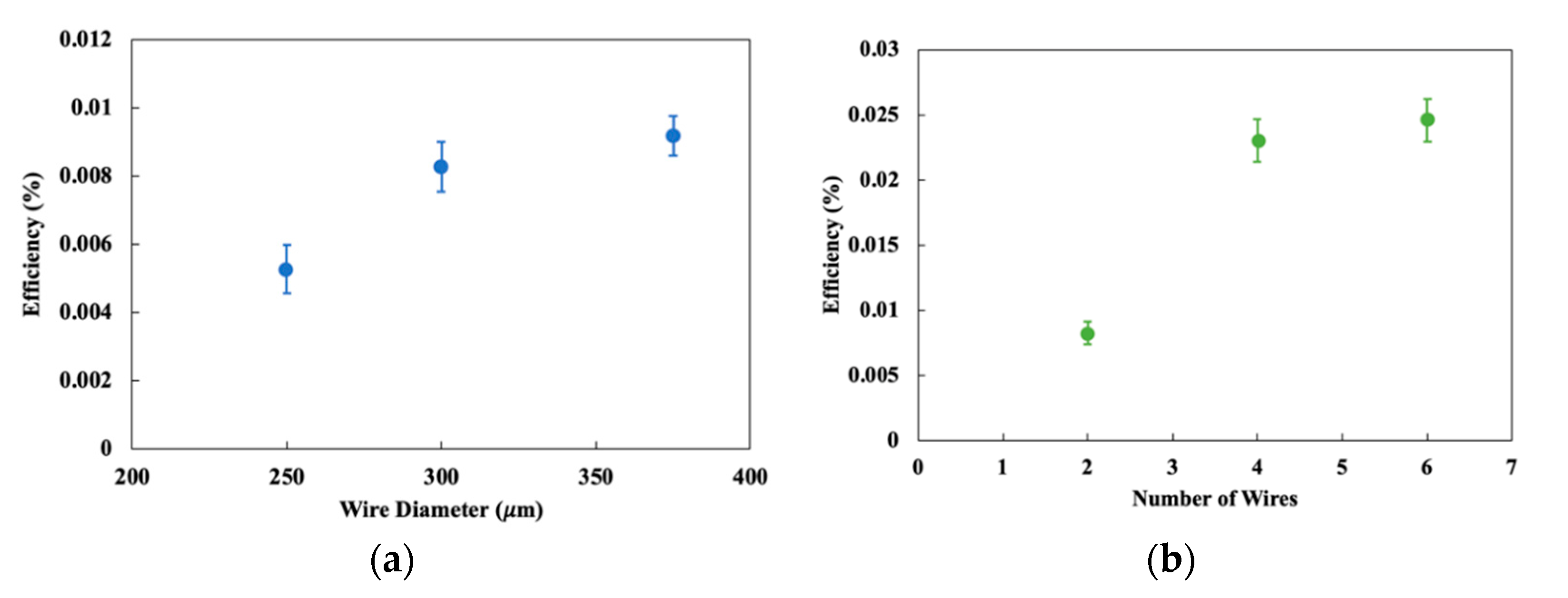
4.2. Efficiency Testing
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Majidi, C. Soft Robotics: A Perspective–Current Trends and Prospects for the Future. Soft Rob. 2014, 1, 5–11. [Google Scholar] [CrossRef]
- Kim, S.; Laschi, C.; Trimmer, B. Soft Robotics: A Bioinspired Evolution in Robotics. Trends Biotechnol. 2013, 31, 287–294. [Google Scholar] [CrossRef] [PubMed]
- Rus, D.; Tolley, M. Design, Fabrication and Control of Soft Robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef] [Green Version]
- Trivedi, D.; Rahn, C.D.; Kier, W.M.; Walker, I.D. Soft Robotics: Biological Inspiration, State of the Art, and Future Research. Appl. Bionics Biomech. 2008, 5, 99–117. [Google Scholar] [CrossRef]
- Laschi, C.; Mazzolai, B.; Cianchetti, M. Soft Robotics: Technologies and Systems Pushing the Boundaries of Robot Abilities. Sci. Rob. 2016, 1, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Miriyev, A. A Focus on Soft Actuation. Actuators 2019, 8, 74. [Google Scholar] [CrossRef] [Green Version]
- de Payrebrune, K.M.; O’Reilly, O.M. On Constitutive Relations for a Rod-Based Model of a Pneu-Net Bending Actuator. Extrem. Mech. Lett. 2016, 8, 38–46. [Google Scholar] [CrossRef] [Green Version]
- Rich, S.I.; Wood, R.J.; Majidi, C. Untethered Soft Robotics. Nat. Electron. 2018, 1, 102–112. [Google Scholar] [CrossRef]
- Tolley, M.T.; Shepherd, R.F.; Mosadegh, B.; Galloway, K.C.; Wehner, M.; Karpelson, M.; Wood, R.J.; Whitesides, G.M. A Resilient, Untethered Soft Robot. Soft Robot. 2014, 1, 213–223. [Google Scholar] [CrossRef]
- Youn, J.-H.; Jeong, S.M.; Hwang, G.; Kim, H.; Hyeon, K.; Park, J.; Kyung, K.-U. Dielectric Elastomer Actuator for Soft Robotics Applications and Challenges. Appl. Sci. 2020, 10, 640. [Google Scholar] [CrossRef] [Green Version]
- Derby, S.; Sreekumar, M.; Nagarajan, T.; Singaperumal, M.; Zoppi, M.; Molfino, R. Critical Review of Current Trends in Shape Memory Alloy Actuators for Intelligent Robots. Ind. Robot. Int. J. 2007, 34, 285–294. [Google Scholar] [CrossRef]
- Suman, A.; Fabbri, E.; Fortini, A.; Merlin, M.; Pinelli, M. On the Design Strategies for SMA-Based Morphing Actuators: State of the Art and Common Practices Applied to a Fascinating Case Study. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2020, 234, 2114–2130. [Google Scholar] [CrossRef]
- Bovesecchi, G.; Corasaniti, S.; Costanza, G.; Piferi, F.P.; Tata, M.E. Deployment of Solar Sails by Joule Effect: Thermal Analysis and Experimental Results. Aerospace 2020, 7, 180. [Google Scholar] [CrossRef]
- Rodrigue, H.; Wang, W.; Han, M.W.; Kim, T.J.; Ahn, S.H. An Overview of Shape Memory Alloy-Coupled Actuators and Robots. Soft Robot. 2017, 4, 3–15. [Google Scholar] [CrossRef] [PubMed]
- El-Atab, N.; Mishra, R.B.; Al-Modaf, F.; Joharji, L.; Alsharif, A.A.; Alamoudi, H.; Diaz, M.; Qaiser, N.; Hussain, M.M. Soft Actuators for Soft Robotic Applications: A Review. Adv. Intell. Syst. 2020, 2, 2000128. [Google Scholar] [CrossRef]
- Huang, X.; Ford, M.; Patterson, Z.J.; Zarepoor, M.; Pan, C.; Majidi, C. Shape Memory Materials for Electrically-Powered Soft Machines. J. Mater. Chem. B 2020, 8, 4539–4551. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Gu, X.; Xu, Y.; Zhu, J.; Zhang, W. Thermomechanical Modeling of Nonlinear Internal Hysteresis Due to Incomplete Phase Transformation in Pseudoelastic Shape Memory Alloys. Nonlinear Dyn. 2021, 103, 1393–1414. [Google Scholar] [CrossRef]
- Islam, A.B.M.R.; Karadoğan, E. Analysis of One-Dimensional Ivshin–Pence Shape Memory Alloy Constitutive Model for Sensitivity and Uncertainty. Materials 2020, 13, 1482. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mohd, J.; Leary, M.; Subic, A.; Gibson, M.A. A Review of Shape Memory Alloy Research, Applications and Opportunities. Mater. Des. 2014, 56, 1078–1113. [Google Scholar] [CrossRef]
- Lee, J.H.; Chung, Y.S.; Rodrigue, H. Long Shape Memory Alloy Tendon-Based Soft Robotic Actuators and Implementation as a Soft Gripper. Sci. Rep. 2019, 9, 1–12. [Google Scholar] [CrossRef]
- Wang, Z.; Hang, G.; Li, J.; Wang, Y.; Xiao, K. A Micro-Robot Fish with Embedded SMA Wire Actuated Flexible Biomimetic Fin. Sens. Actuators A Phys. 2008, 144, 354–360. [Google Scholar] [CrossRef]
- Patterson, Z.J.; Sabelhaus, A.P.; Chin, K.; Hellebrekers, T.; Majidi, C. An Untethered Brittle Star-Inspired Soft Robot for Closed-Loop Underwater Locomotion. In Proceedings of the 2020 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Las Vegas, NV, USA, 24 October–24 January 2021; pp. 8758–8764. [Google Scholar]
- Kim, H.S.; Heo, J.K.; Choi, I.G.; Ahn, S.H.; Chu, W.S. Shape Memory Alloy Driven Undulatory Locomotion of Soft Biomimetic Ray Robot. Bioinspiration Biomim. 2021, accepted. [Google Scholar] [CrossRef]
- Lin, H.-T.; Leisk, G.G.; Trimmer, B. GoQBot: A Caterpillar-Inspired Soft-Bodied Rolling Robot. Bioinspiration Biomim. 2011, 6, 026007. [Google Scholar] [CrossRef] [PubMed]
- Goldberg, N.N.; Huang, X.; Majidi, C.; Novelia, A.; O’Reilly, O.M.; Paley, D.A.; Scott, W.L. On Planar Discrete Elastic Rod Models for the Locomotion of Soft Robots. Soft Robot. 2019, 6, 595–610. [Google Scholar] [CrossRef]
- Seok, S.; Onal, C.D.; Cho, K.J.; Wood, R.J.; Rus, D.; Kim, S. Meshworm: A Peristaltic Soft Robot with Antagonistic Nickel Titanium Coil Actuators. IEEE/ASME Trans. Mechatron. 2012, 18, 1485–1497. [Google Scholar] [CrossRef]
- Lohse, F.; Wende, C.; Klass, K.D.; Hickmann, R.; Häntzsche, E.; Bollengier, Q.; Ashir, M.; Pöschel, R.; Bolk, N.; Trümper, W.; et al. Bio-inspired Semi-flexible Joint Based on Fibre-reinforced Composites with Shape Memory Alloys. J. Intell. Mater. Syst. Struct. 2021, 32, 462–472. [Google Scholar] [CrossRef]
- Huang, X.; Kumar, K.; Jawed, M.K.; Nasab, A.M.; Ye, Z.; Shan, W.; Majidi, C. Highly Dynamic Shape Memory Alloy Actuator for Fast Moving Soft Robots. Adv. Mater. Technol. 2019, 4, 1800540. [Google Scholar] [CrossRef]
- Mao, S.; Dong, E.; Jin, H.; Xu, M.; Zhang, S.; Yang, J.; Low, K.H. Gait Study and Pattern Generation of a Starfish-like Soft Robot with Flexible Rays Actuated by SMAs. J. Bionic Eng. 2014, 11, 400–411. [Google Scholar] [CrossRef]
- Bartlett, M.D.; Kazem, N.; Powell-Palm, M.J.; Huang, X.; Sun, W.; Malen, J.A.; Majidi, C. High Thermal Conductivity in Soft Elastomers with Elongated Liquid Metal Inclusions. Proc. Natl. Acad. Sci. USA 2017, 114, 2143–2148. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dynalloy. Available online: http://www.dynalloy.com/tech_data_wire.php (accessed on 12 August 2021).
- Brinson, L.C. One-Dimensional Constitutive Behavior of Shape Memory Alloys: Thermomechanical Derivation with Non-Constant Material Functions and Redefined Martensite Internal Variable. J. Intell. Mater. Syst. Struct. 1993, 4, 229–242. [Google Scholar] [CrossRef]
- Van Humbeeck, J. Non-Medical Applications of Shape Memory Alloys. Mater. Sci. Eng. A 1999, 273–275, 134–148. [Google Scholar] [CrossRef]
- Boyraz, P.; Runge, G.; Raatz, A. An Overview of Novel Actuators for Soft Robotics. Actuators 2018, 7, 48. [Google Scholar] [CrossRef] [Green Version]


| Component | w (kg/m3) | cp (J/kg-K) | k (W/m-K) | T(t = 0) (°C) |
|---|---|---|---|---|
| H48-2 | 2430 | 1000 | 2.2 | 50 |
| Ecoflex 30 | 1070 | 1050 | 0.2 | 50 |
| SMA Wire | 6450 | 837.4 | 18 | 80 |
| Position a from Fixed Support (mm) | (mm) | MW (N*mm) |
|---|---|---|
| 109.2 | 22 | 1.559 |
| 157.5 | 30 | 1.470 |
| 88.9 | 18 | 1.536 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dauksher, R.; Patterson, Z.; Majidi, C. Characterization and Analysis of a Flexural Shape Memory Alloy Actuator. Actuators 2021, 10, 202. https://doi.org/10.3390/act10080202
Dauksher R, Patterson Z, Majidi C. Characterization and Analysis of a Flexural Shape Memory Alloy Actuator. Actuators. 2021; 10(8):202. https://doi.org/10.3390/act10080202
Chicago/Turabian StyleDauksher, Richard, Zachary Patterson, and Carmel Majidi. 2021. "Characterization and Analysis of a Flexural Shape Memory Alloy Actuator" Actuators 10, no. 8: 202. https://doi.org/10.3390/act10080202
APA StyleDauksher, R., Patterson, Z., & Majidi, C. (2021). Characterization and Analysis of a Flexural Shape Memory Alloy Actuator. Actuators, 10(8), 202. https://doi.org/10.3390/act10080202






