Parameter Identification of the Nonlinear Piezoelectric Shear d15 Coefficient of a Smart Composite Actuator
Abstract
1. Introduction
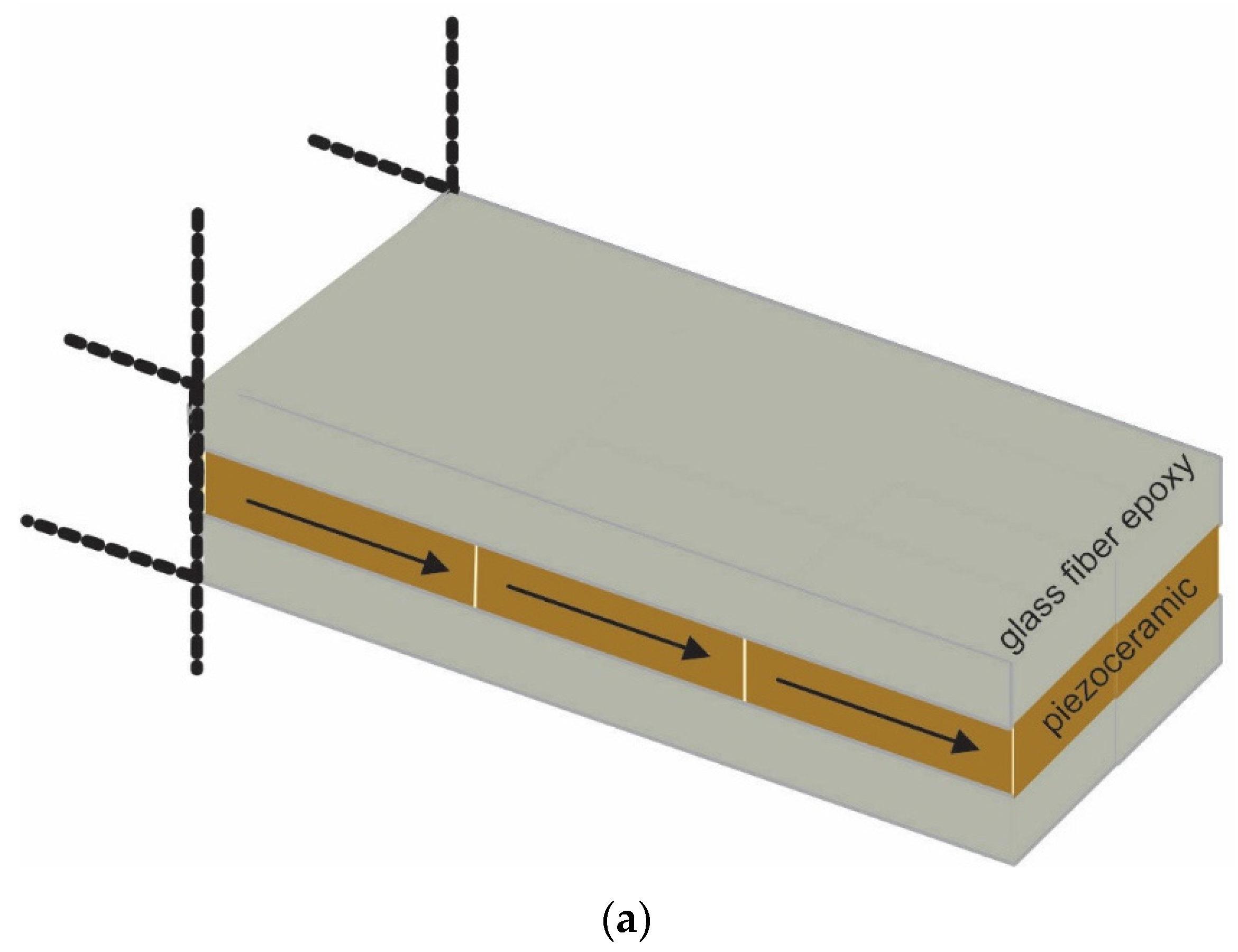
2. Estimation of Parameters of Piezoelectric Shear Coefficient
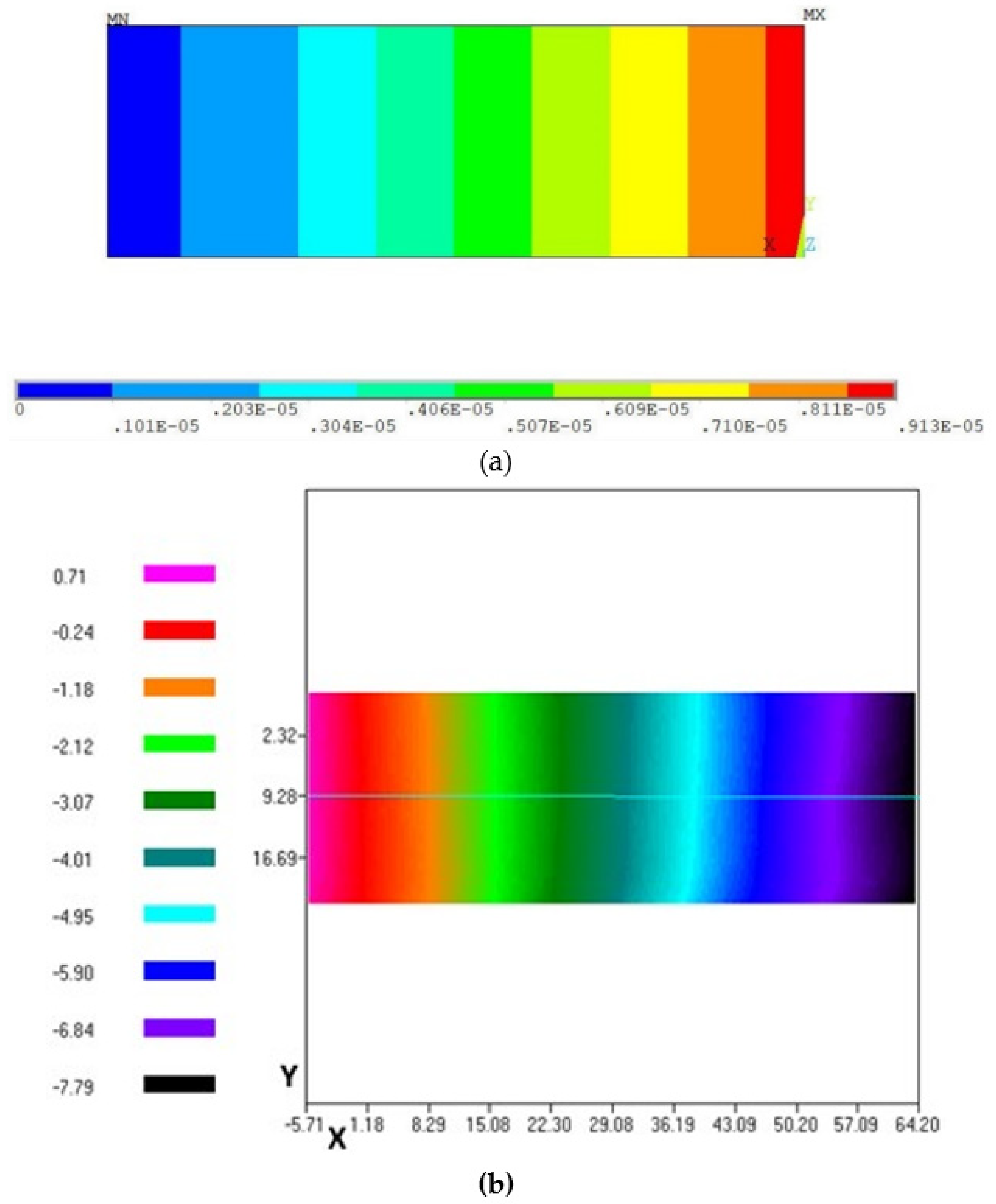
2.1. ESPI Measurements
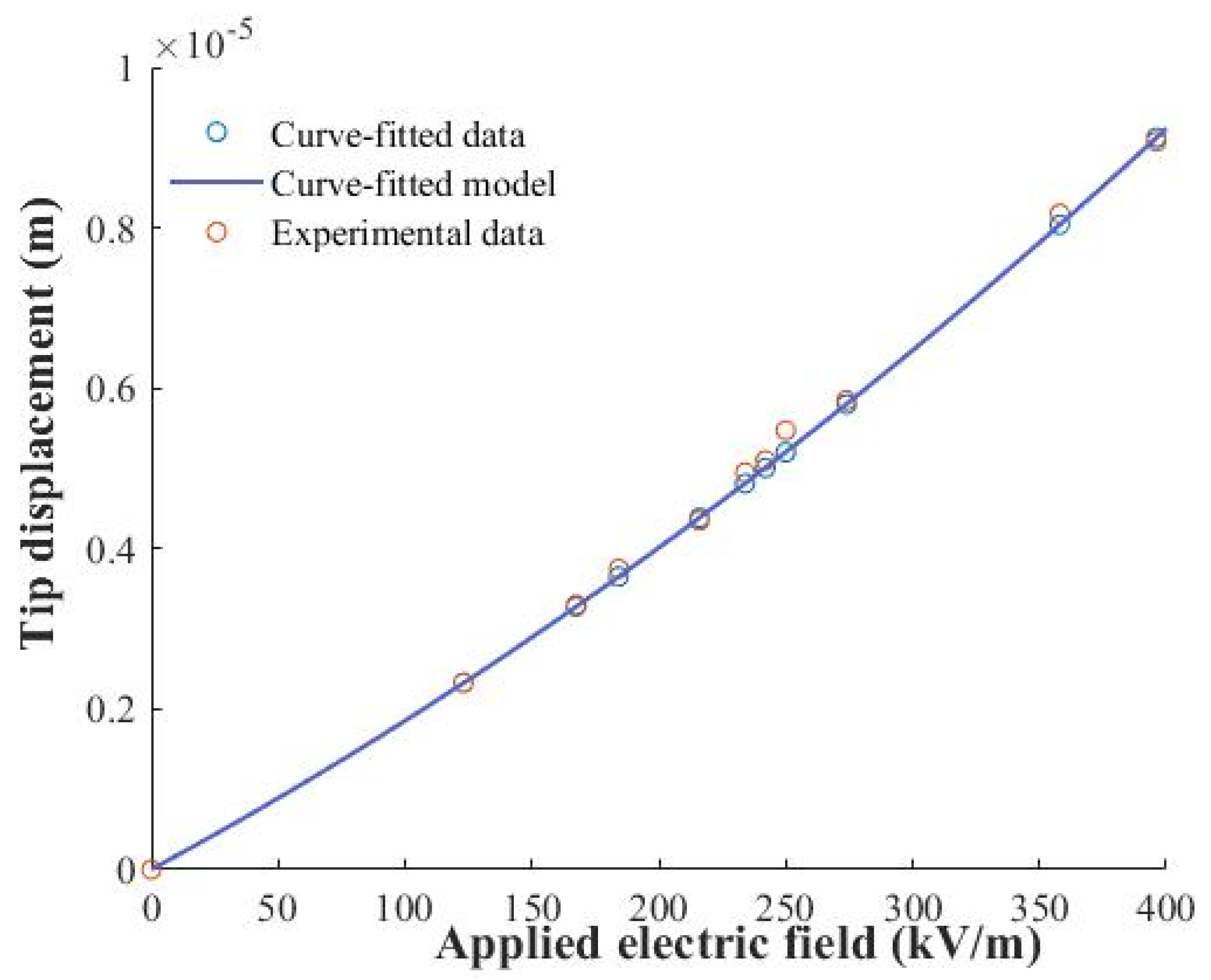
2.2. Parameter Identification of Piezoelectric Shear Coefficient
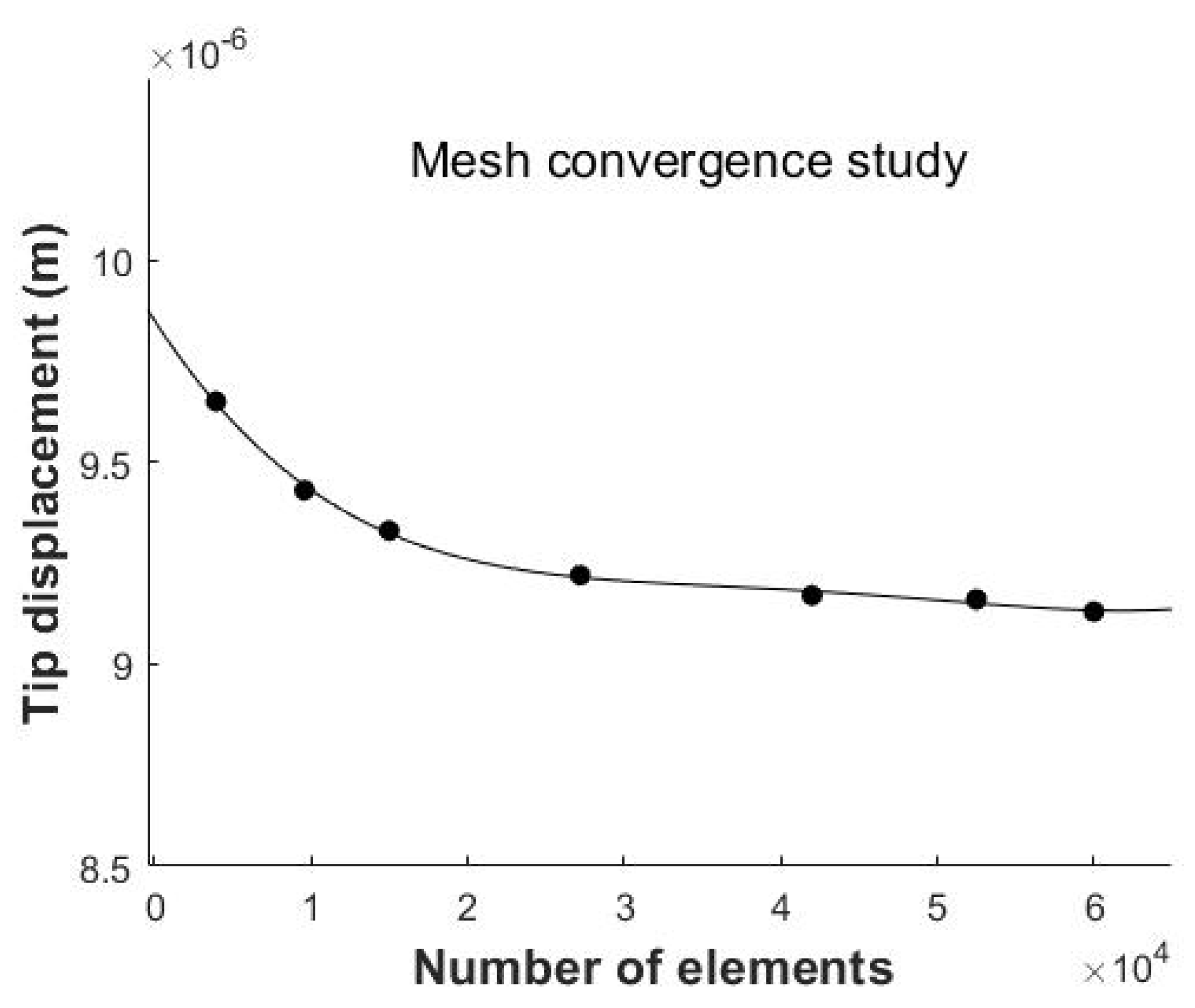
3. Finite Element (FE) Simulations Considering the Nonlinear Effect
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Materials | Constants | Notations | Values |
|---|---|---|---|
| PIC255 (axially poled) | piezoelectric coupling stress constant (Cm−2) | e15 = e24 | 11.9 |
| e31= e32 | –7.15 | ||
| e33 | 13.7 | ||
| permittivity constants at constant strain (nFm−1) | 8.234 | ||
| 7.588 | |||
| Young’s moduli (GNm−2) | E2 = E3 | 62.89 | |
| E1 | 47.69 | ||
| Shear Moduli (GNm−2) | G13= G12 | 22.26 | |
| G23 | 23.15 | ||
| Poisson’s ratios | v13= v12 | 0.46 | |
| v23 | 0.36 | ||
| Density (kgm−3) | 7800 | ||
| Glass fiber/epoxy | Young’s moduli (GNm−2) | E2 = E3 | 13.1 |
| E1 | 33.11 | ||
| Shear Moduli (GNm−2) | G13= G12 | 3 | |
| G23 | 2.3 | ||
| Poisson’s ratios | v13= v12 | 0.27 | |
| v23 | 0.40 | ||
| Adhesive | Young’s modulus (GNm−2) | E | 1.03 |
| Poisson’s ratio | v | 0.37 |
References
- Altammar, H.; Dhingra, A.; Salowitz, N. Damage Detection Using d15 Piezoelectric Sensors in a Laminate Beam Undergoing Three-Point Bending. Actuators 2019, 8, 70. [Google Scholar] [CrossRef]
- Niu, J.; Wu, J.; Liu, Q.; Chen, L.; Guo, S.A. Dumbbell Shaped Piezoelectric Motor Driven by the First-Order Torsional and the First-Order Flexural Vibrations. Actuators 2020, 9, 124. [Google Scholar] [CrossRef]
- Berik, P.; Maurya, D.; Kumar, P.; Kang, M.G.; Priya, S. Enhanced torsional actuation and stress coupling in Mn-modified 0.93(Na0.5Bi0.5TiO3)-0.07BaTiO3 lead-free piezoceramic system. Sci. Technol. Adv. Mater. 2017, 18, 51–59. [Google Scholar] [CrossRef] [PubMed]
- Malakooti, M.H.; Sodano, H.A. Electromechanical characterization of piezoelectric shear actuators. In Proceedings of the ASME Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Snowbird, UT, USA, 16–18 September 2013. [Google Scholar] [CrossRef]
- Malakooti, M.H.; Sodano, H.A.J. Direct measurement of piezoelectric shear coefficient. Appl. Phys. 2013, 113, 214106. [Google Scholar] [CrossRef]
- Hagiwara, M.; Takahashi, S.; Hoshina, T.; Takeda, H.; Sakurai, O.; Tsurumi, T. Nonlinear shear response in (K,Na)NbO3-based lead-free piezoelectric ceramics. Key Eng. Mater. 2010, 445, 47–50. [Google Scholar] [CrossRef]
- Li, S.; Cao, W.; Cross, L.E. The extrinsic nature of nonlinear behavior observed in lead zirconate titanate ferroelectric ceramic. J. Appl. Phys. 1991, 69, 7219–7224. [Google Scholar] [CrossRef]
- Hagiwara, M.; Hoshina, T.; Takeda, H.; Tsurumi, T. Nonlinear shear responses of lead zirconate titanate piezoelectric ceramics. Jpn. J. Appl. Phys. 2010, 49, 09MD04. [Google Scholar] [CrossRef]
- Berlincourt, D.A.; Curran, D.R.; Jaffe, H. Piezoelectric and Piezomagnetical Materials and Their Function in Transducers. Phys. Acoust. 1964, 1A, 169–270. [Google Scholar]
- Beigev, H.; Schmidtv, G. Electromechanical resonances for investigating linear and nonlinear properties of dielectrics. Ferroelectrics 1982, 41, 39–49. [Google Scholar] [CrossRef]
- Parashar, S.K.; DasGupta, A.; Wagner, U.; Hagedorn, P. Nonlinear shear vibrations of piezoceramic actuators. Int. J. Non-Linear Mech. 2005, 40, 429–443. [Google Scholar] [CrossRef]
- Parashar, S.K.; DasGupta, A.; Wagner, U.; Hagedorn, P. Investigation of nonlinear shear induced flexural vibrations of piezoceramic actuators. In Proceedings of the SPIE Smart Structures and Materials, San Diego, CA, USA, 26 July 2004; Volume 5383, pp. 71–81. [Google Scholar] [CrossRef]
- Parashar, S.K.; DasGupta, A.; Wagner, U.; Hagedorn, P. A modified Timoshenko beam theory for nonlinear shear-induced flexural vibrations of piezoceramic continua. Nonlinear Dyn. 2004, 37, 181–205. [Google Scholar] [CrossRef]
- Mueller, V.; Zhang, Q.M. Shear response of lead zirconate titanate piezoceramics. J. Appl. Phys. 1998, 83, 3754–3761. [Google Scholar] [CrossRef]
- Mueller, V.; Beige, H. Nonlinearity of soft PZT piezoceramic for shear and torsional actuator applications. In Proceedings of the ISAF 1998. Proceedings of the Eleventh IEEE International Symposium on Applications of Ferroelectrics (Cat.No.98CH36245), Montreux, Switzerland, 24–27 August 1998; pp. 459–462. [Google Scholar] [CrossRef]
- Berik, P.; Chang, W.; Jiang, X. Piezoelectric d36 in-plane shear-mode of lead-free BZT-BCT single crystals for torsion actuation. Appl. Phys. Lett. 2017, 110, 052902. [Google Scholar] [CrossRef]
- Benjeddou, A. Shear-Mode Piezoceramic Advanced Materials and Structures: A State of the Art. Mech. Adv. Mater. Struct. 2007, 14, 263–275. [Google Scholar] [CrossRef]
- Benjeddou, A. Field-dependent nonlinear piezoelectricity: A focused review. Int. J. Smart Nano Mater. 2018, 9, 68–84. [Google Scholar] [CrossRef]
- Trindade, M.A.; Kakazu, T.Y. Structural control of sandwich beams using shear piezoelectric actuators subjected to large electric fields. In Proceedings of the Conference IV Congresso Nacional de Engenharia Mecânica, Recife, Brazil, 20–25 August 2006. [Google Scholar]
- Berik, P.; Benjeddou, A. Static experimentations of the piezoceramic d15 shear actuation mechanism for sandwich structures with opposite or same poled patches-assembled core and composite faces. Int. J. Smart Nano Mater. 2011, 2, 230–244. [Google Scholar] [CrossRef][Green Version]
- Available online: https://www.piceramic.com/en/piezo-technology/properties-piezo-actuators/displacement-modes/ (accessed on 30 June 2021).
- Available online: https://www.thorlabs.com/NewGroupPage9_PF.cfm?Guide=10&Category_ID=220&ObjectGroup_ID=5030 (accessed on 30 June 2021).
- Gao, X.; Xin, X.; Wu, J.; Chu, Z.; Dong, S. A multilayered cylindrical piezoelectric shear actuator operating in shear (d15) mode. Appl. Phys. Lett. 2018, 112, 152902. [Google Scholar] [CrossRef]
- Available online: https://static.piceramic.com/fileadmin/user_upload/pi_ceramic/files/catalog_CAT/PI_CAT128E_R3_Piezoelectric_Actuators.pdf (accessed on 30 June 2021).


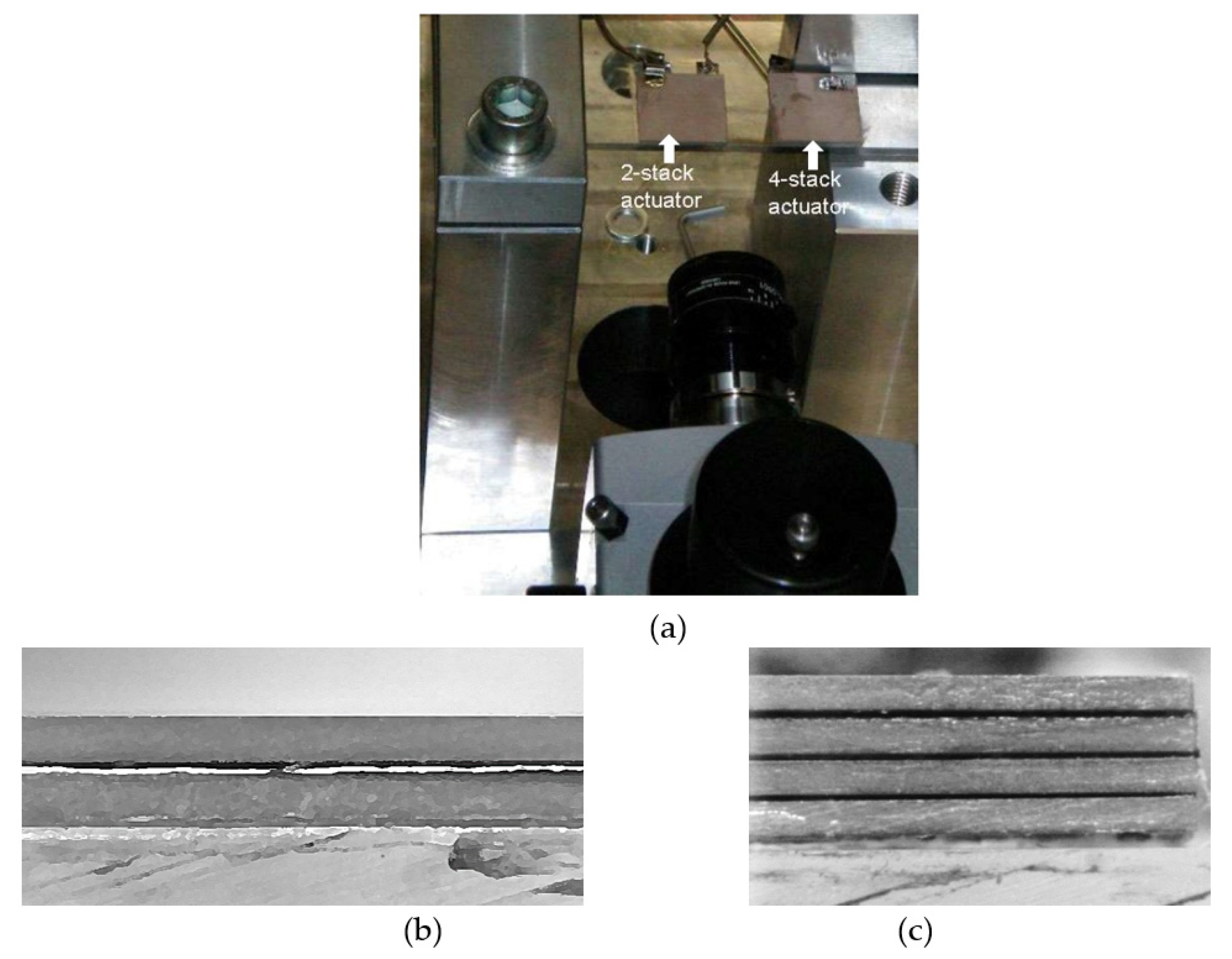

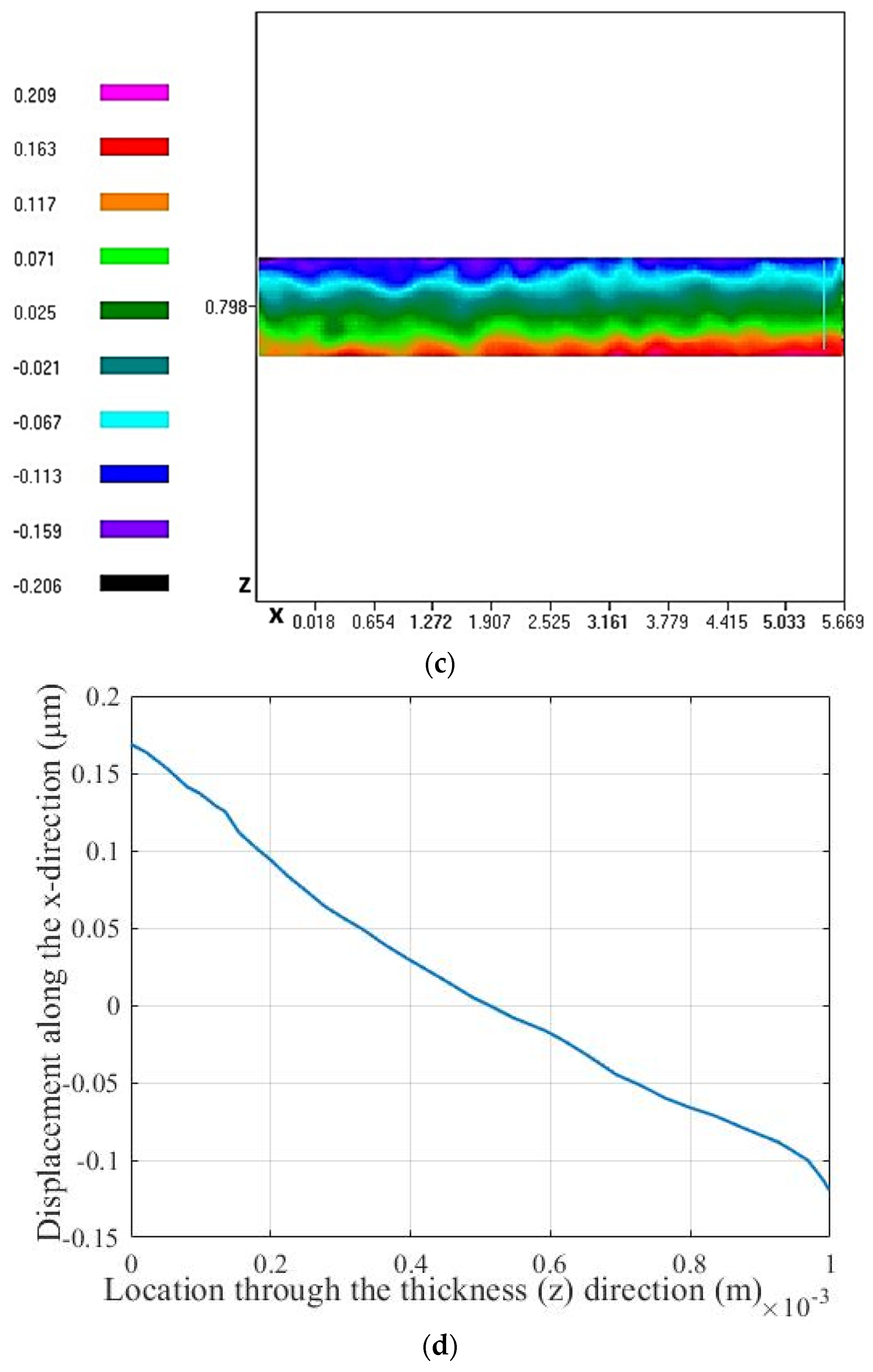
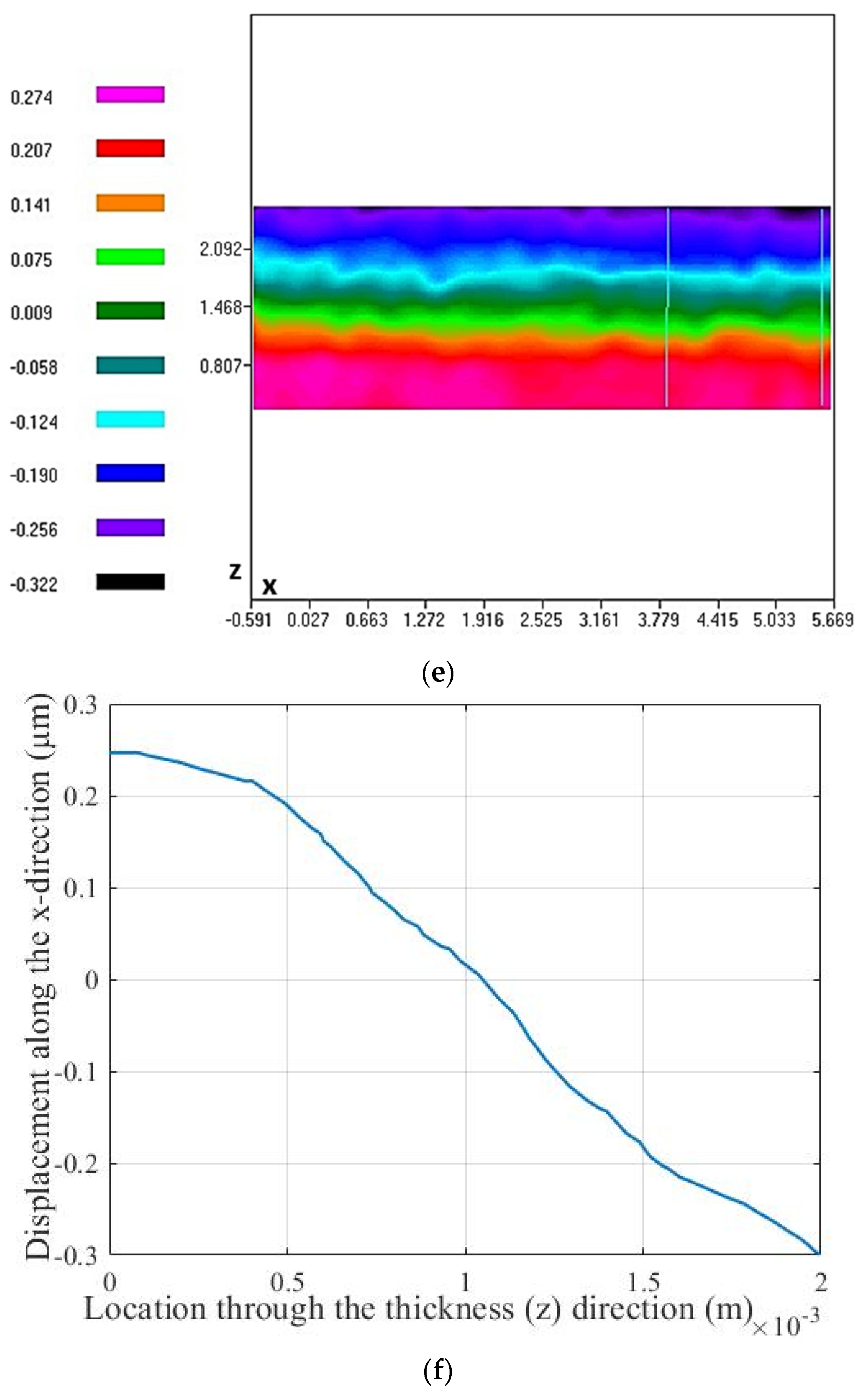
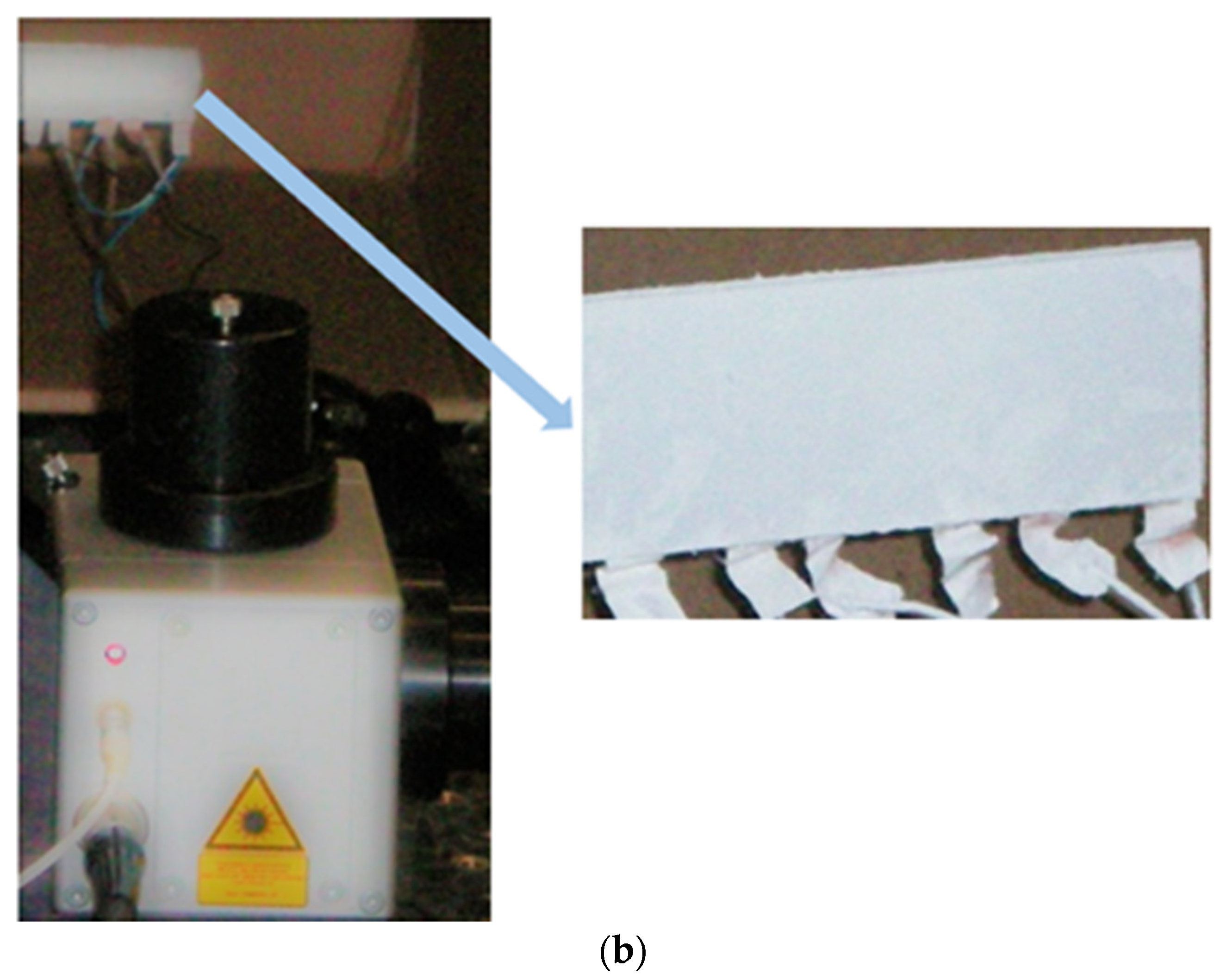



| Type | D, Displacement (µm) | |
|---|---|---|
| 1 layer | 0.156 | 783 |
| 2-stack | 0.288 | 723 |
| 4-stack | 0.546 | 686 |
| Applied Electric Field (kV/cm) | Experimental Displacement (µm) | Curve-Fitted Displacement (µm) | Deviation Percentage (%) | |
|---|---|---|---|---|
| 3.96 | 9.080 | 9.106 | 0.286 | 732 |
| 3.58 | 8.180 | 8.022 | 1.931 | 713 |
| 2.74 | 5.850 | 5.789 | 1.043 | 672 |
| Applied Electric Field (kV/cm) | Experimental Displacement (µm) | FE (Nonlinear) Displacement (µm) | FE (Linear) Displacement (µm) |
|---|---|---|---|
| 3.96 | 9.080 | 9.130 | 6.860 |
| 3.58 | 8.180 | 8.033 | 6.202 |
| 2.74 | 5.850 | 5.782 | 4.747 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berik, P.; Bishay, P.L. Parameter Identification of the Nonlinear Piezoelectric Shear d15 Coefficient of a Smart Composite Actuator. Actuators 2021, 10, 168. https://doi.org/10.3390/act10070168
Berik P, Bishay PL. Parameter Identification of the Nonlinear Piezoelectric Shear d15 Coefficient of a Smart Composite Actuator. Actuators. 2021; 10(7):168. https://doi.org/10.3390/act10070168
Chicago/Turabian StyleBerik, Pelin, and Peter L. Bishay. 2021. "Parameter Identification of the Nonlinear Piezoelectric Shear d15 Coefficient of a Smart Composite Actuator" Actuators 10, no. 7: 168. https://doi.org/10.3390/act10070168
APA StyleBerik, P., & Bishay, P. L. (2021). Parameter Identification of the Nonlinear Piezoelectric Shear d15 Coefficient of a Smart Composite Actuator. Actuators, 10(7), 168. https://doi.org/10.3390/act10070168







