Evaluation of Switching Power Amplifier Topology for Active Magnetic Bearings
Abstract
1. Introduction
2. Topologies of AMB Amplifiers
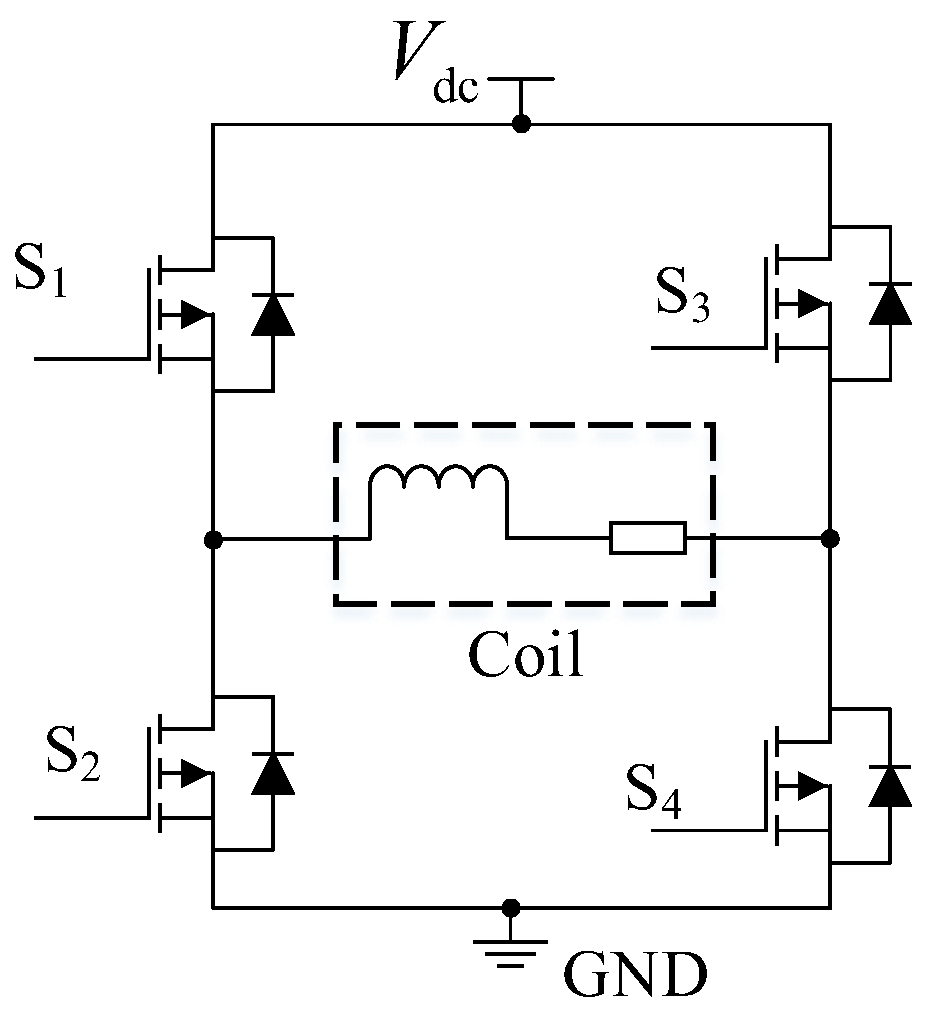
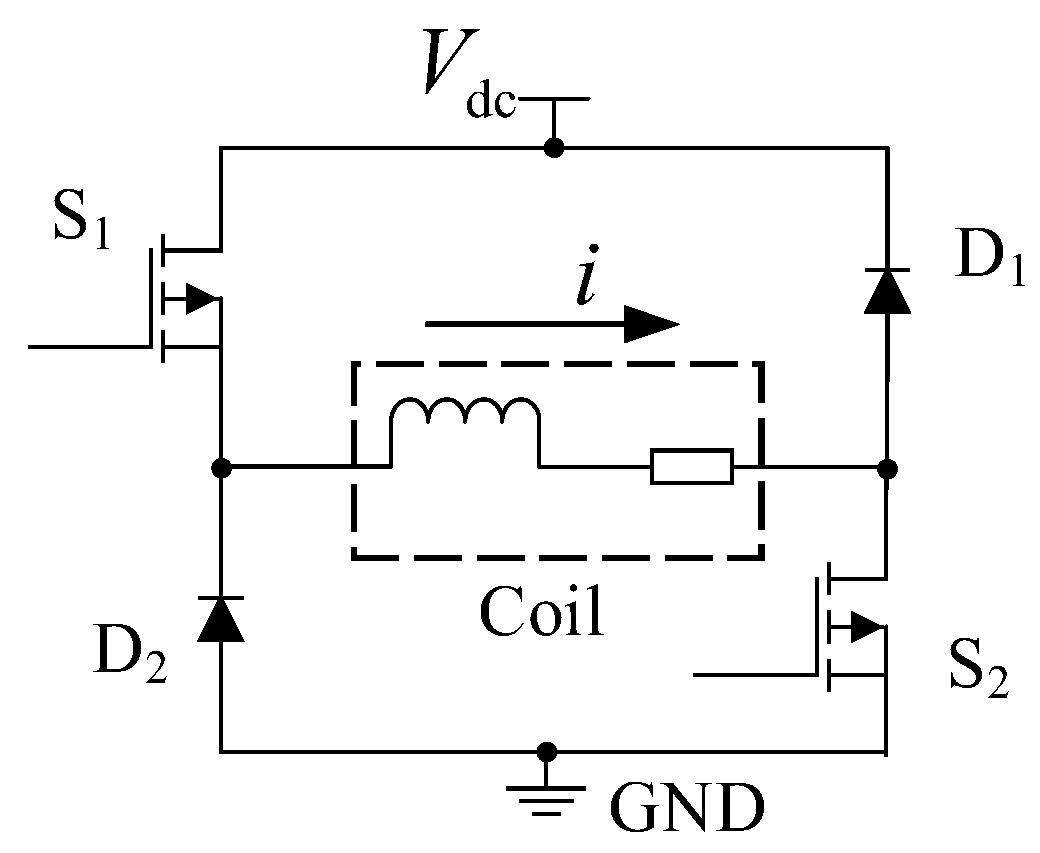
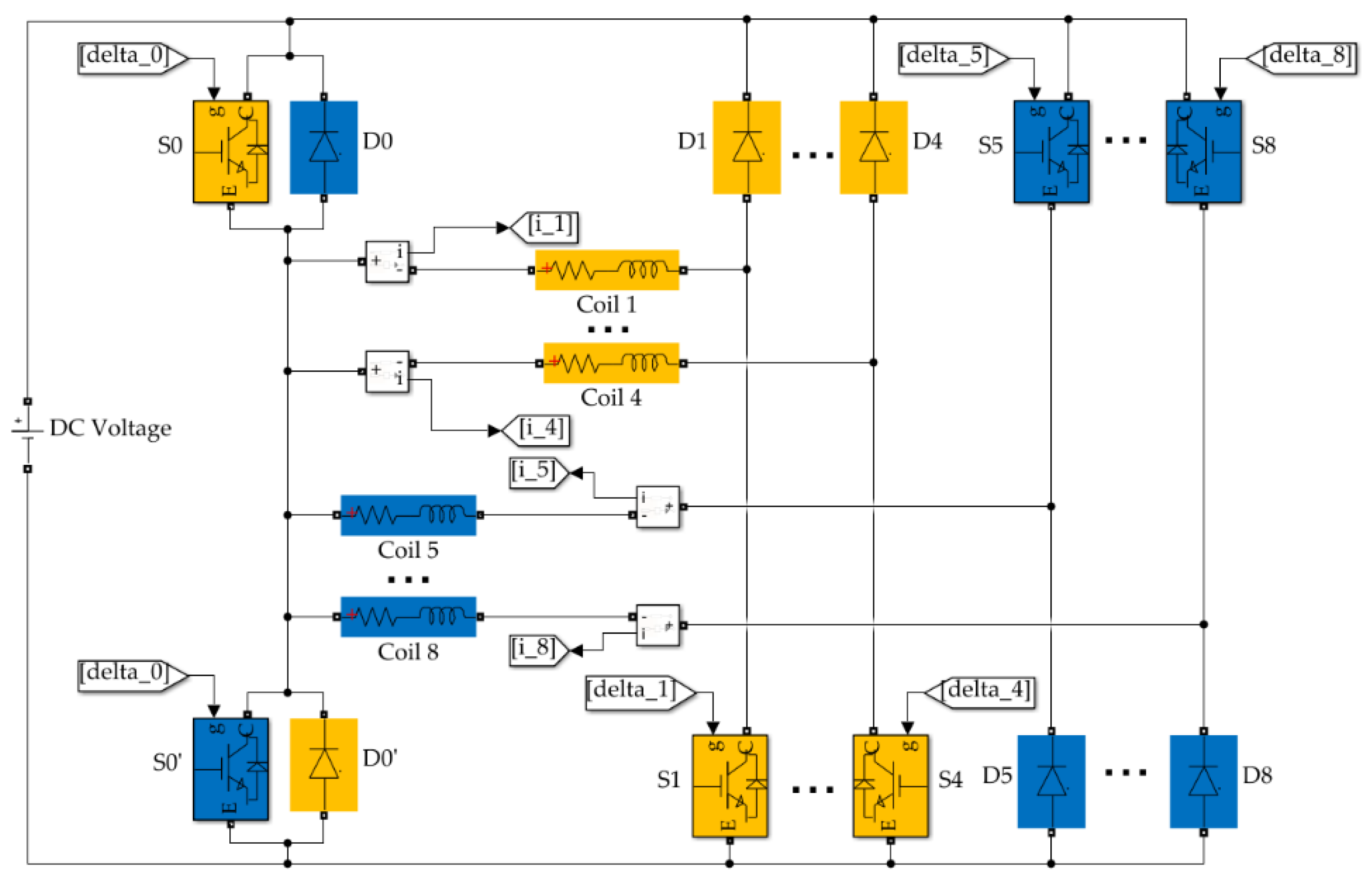
2.1. Half-Bridge Topology
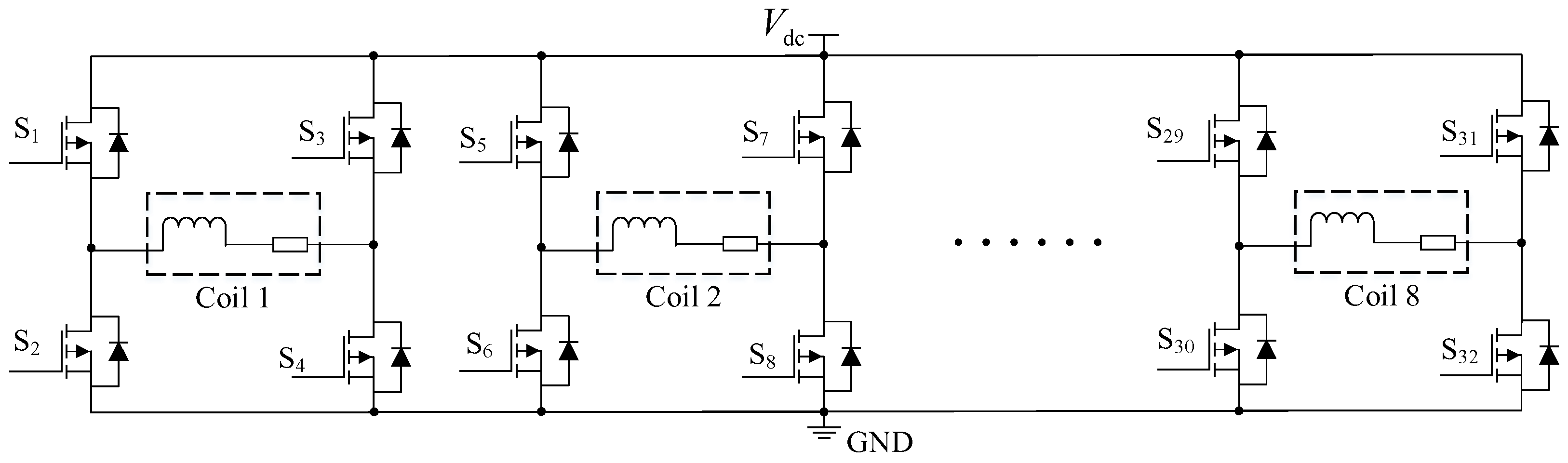
2.2. Three-Phase-Half-Bridge Topology
VC2 = (δ2 − 0.5) Vdc, 0 < δ2 < 1
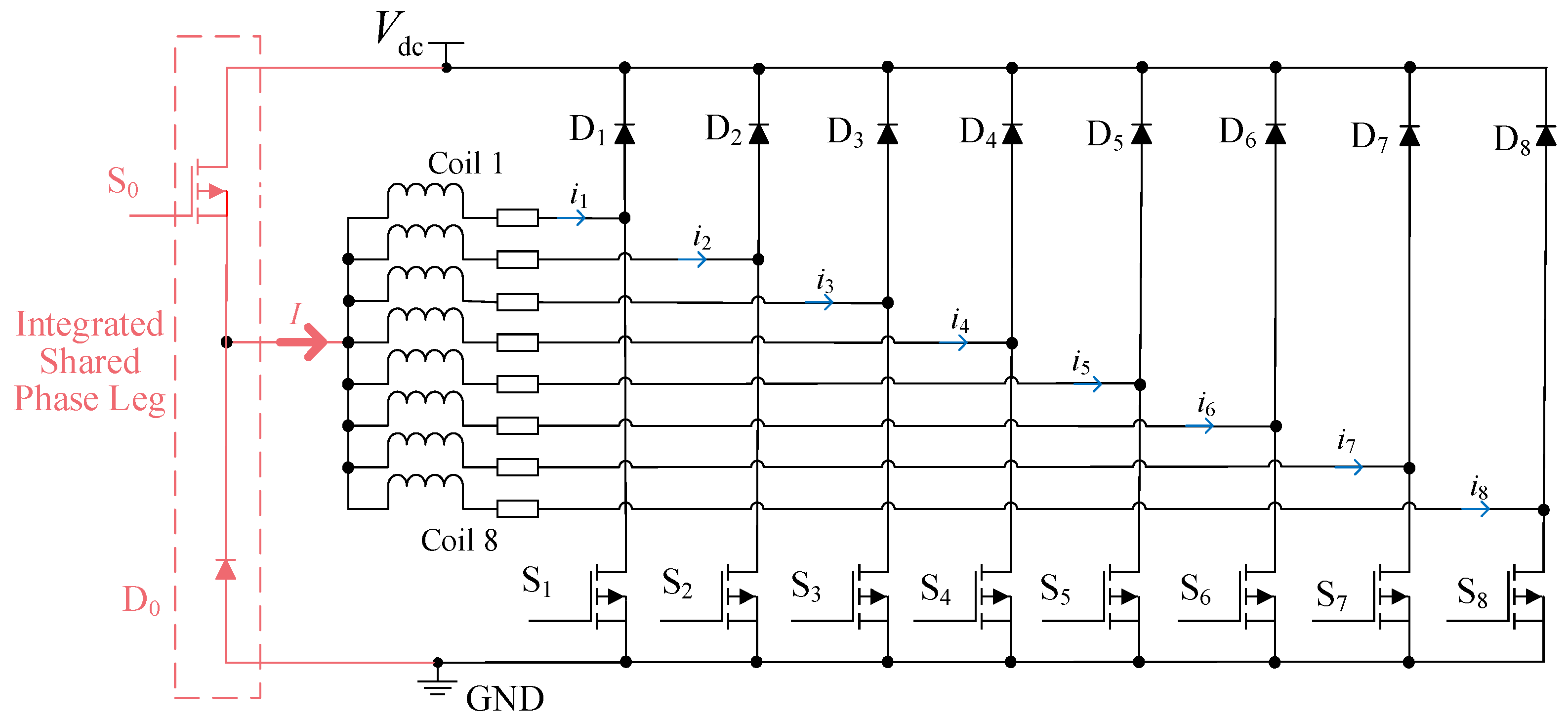
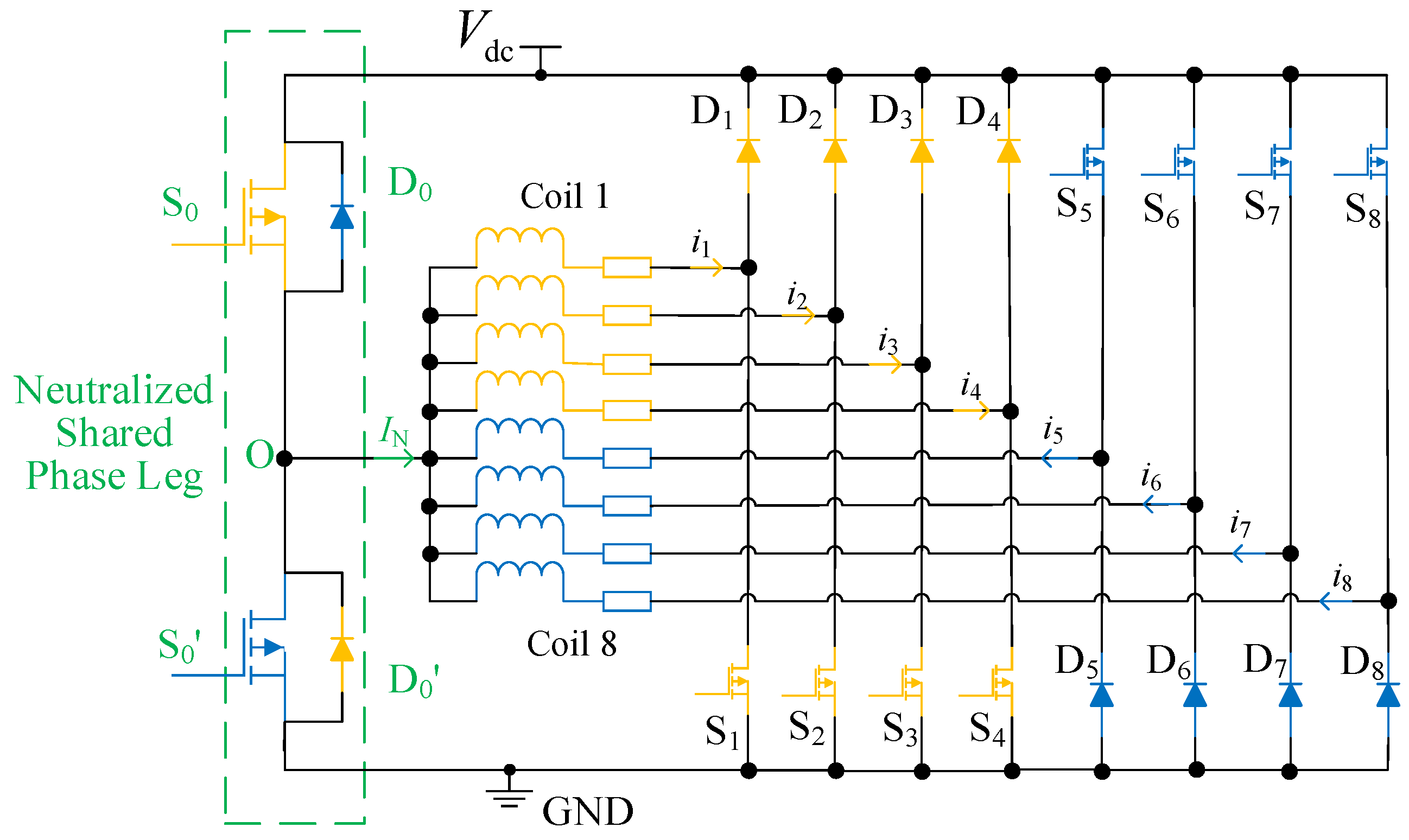
2.3. Neutralized-Sharing-Bridge Topology
3. Analysis and Comparisons of Topologies
3.1. Comparison of SPA Volume
3.2. Comparisons of Output Performance
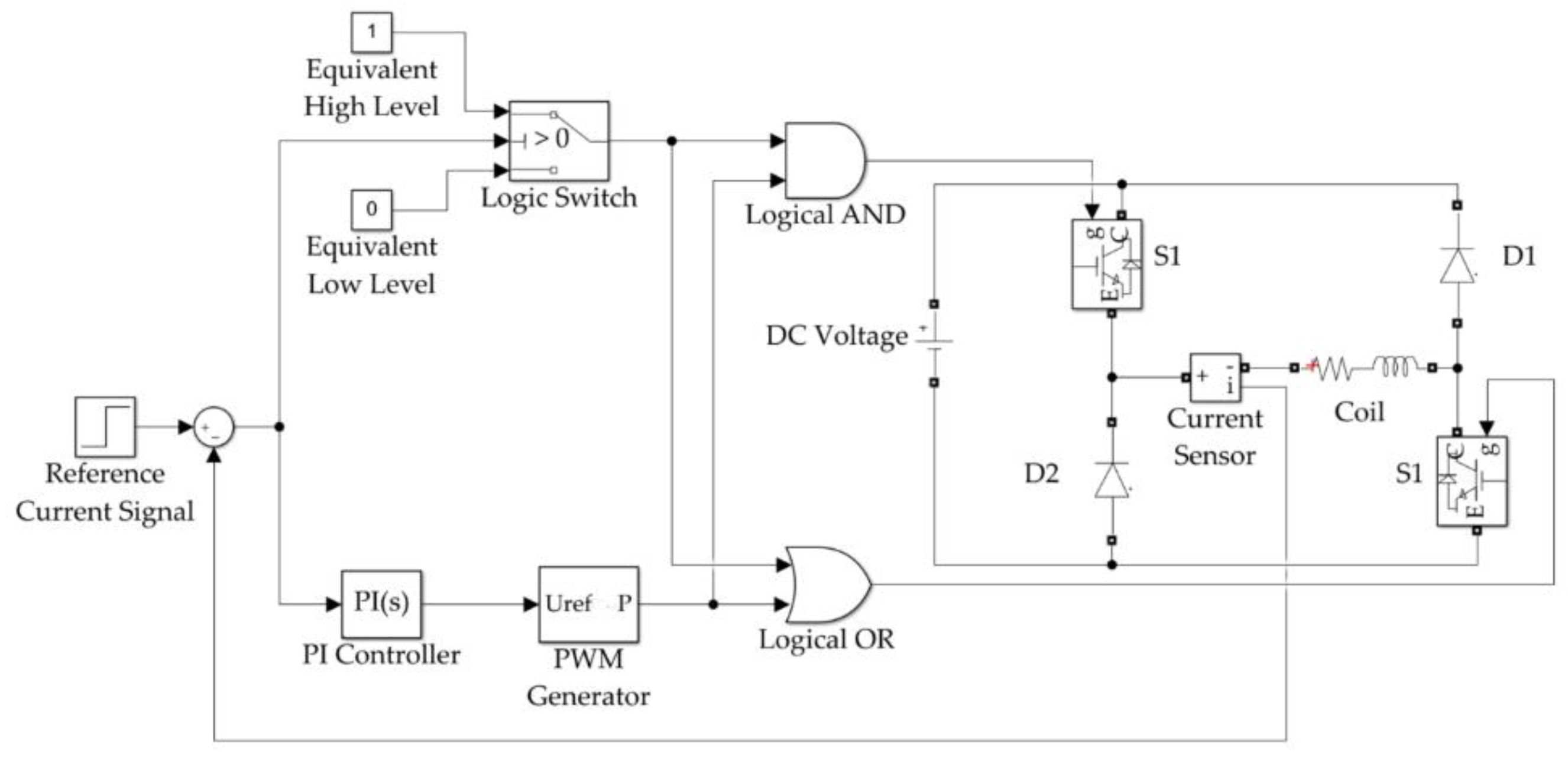
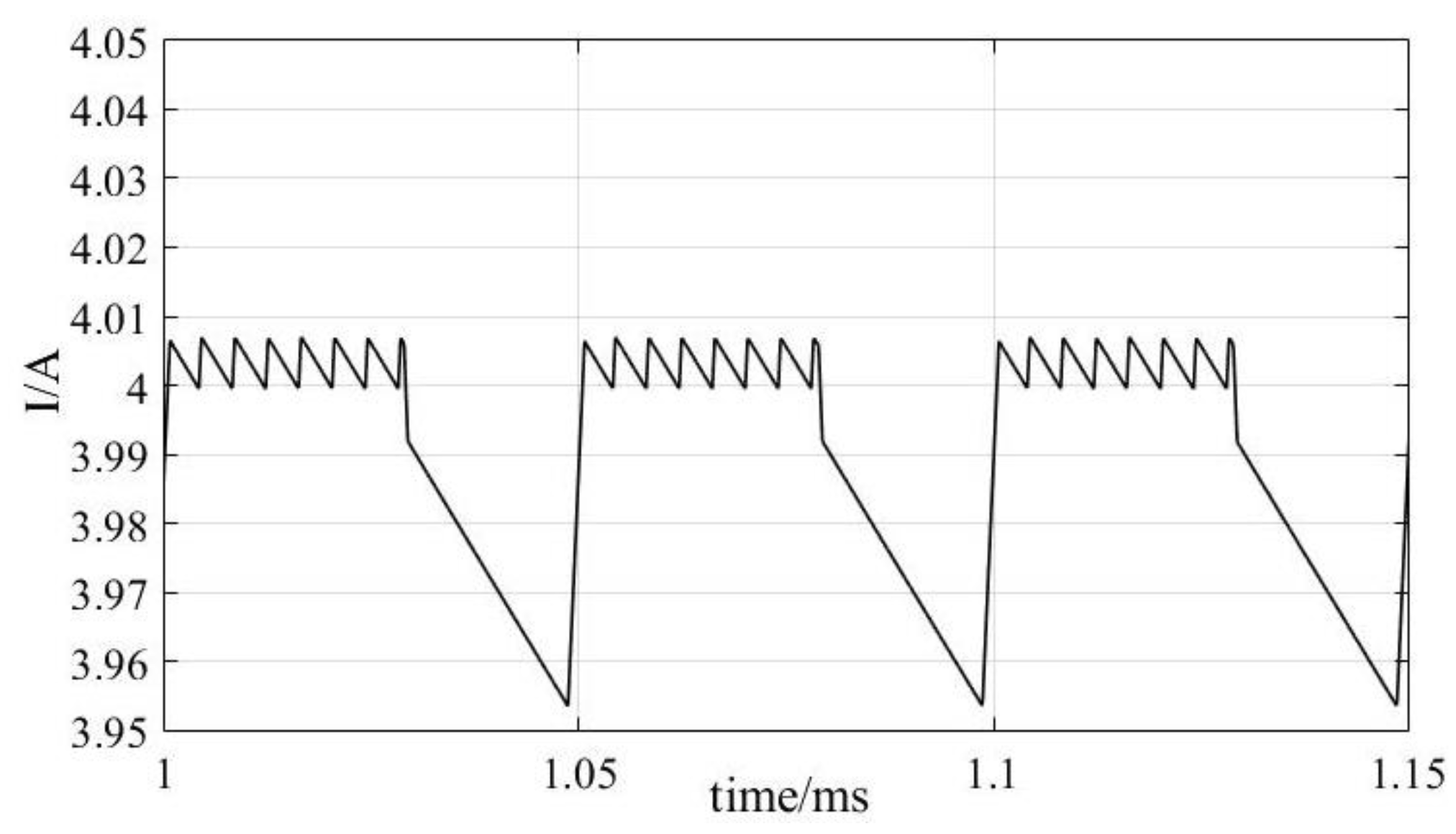
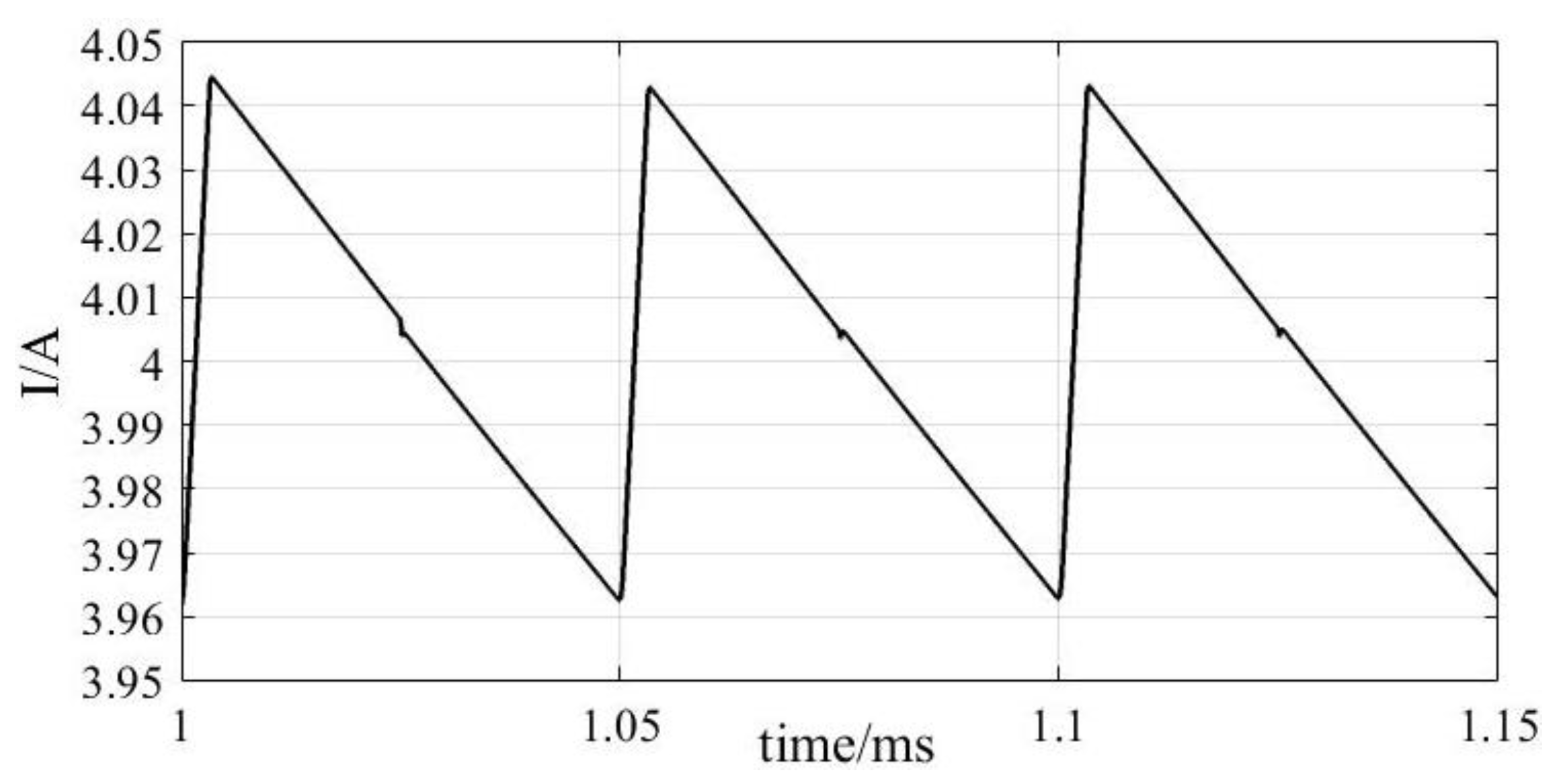
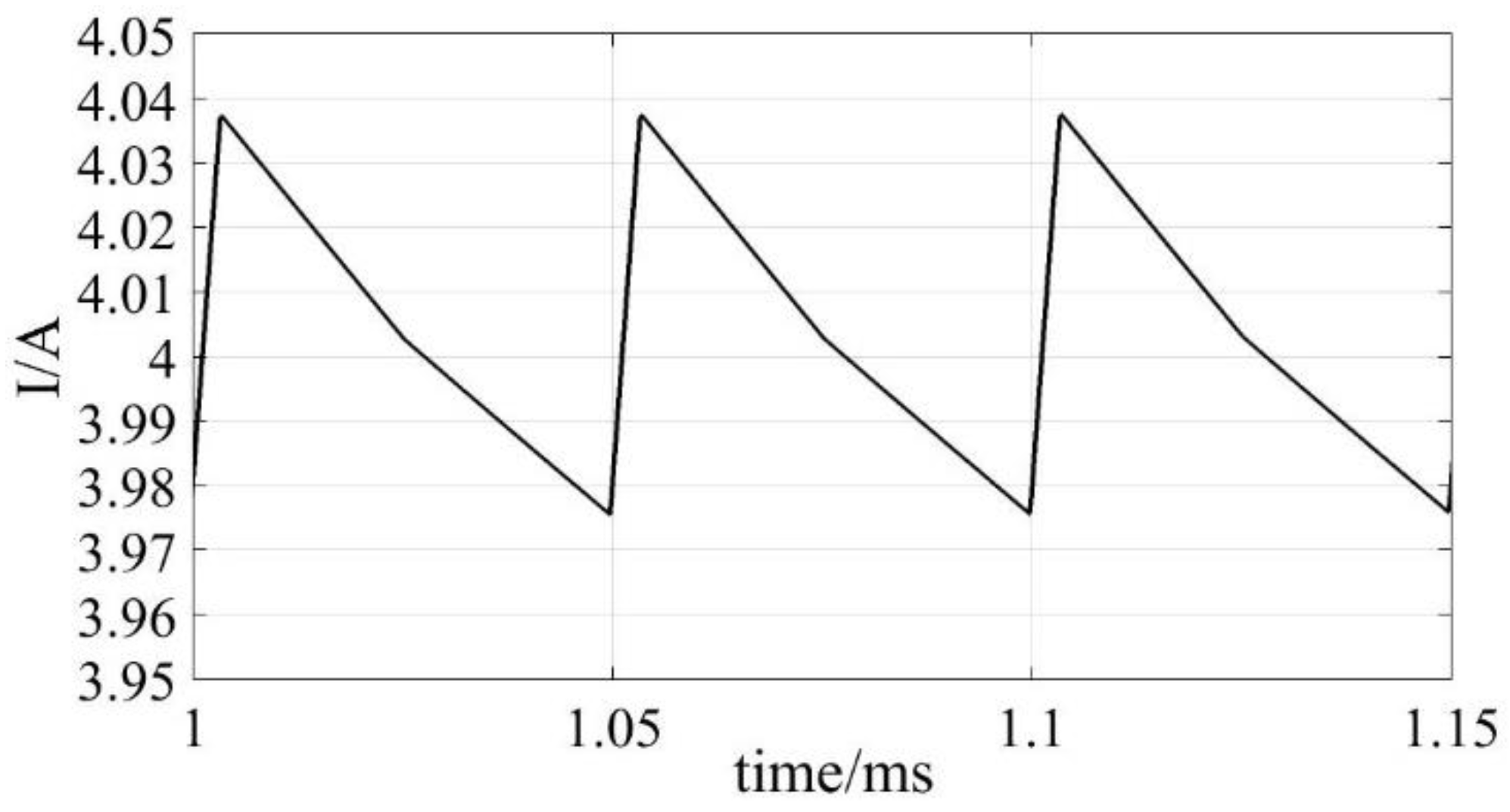
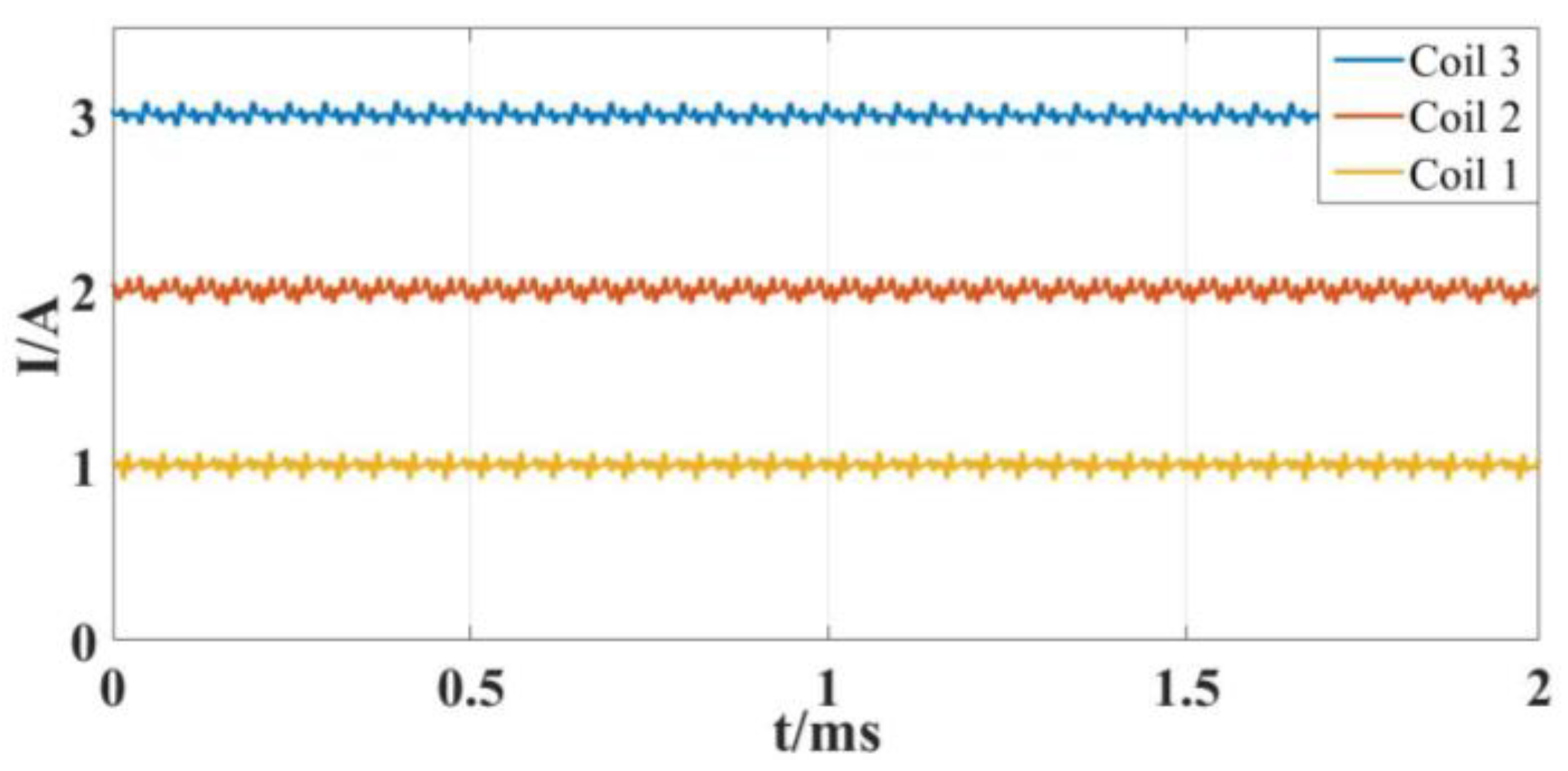
3.2.1. Comparison of Current Ripple
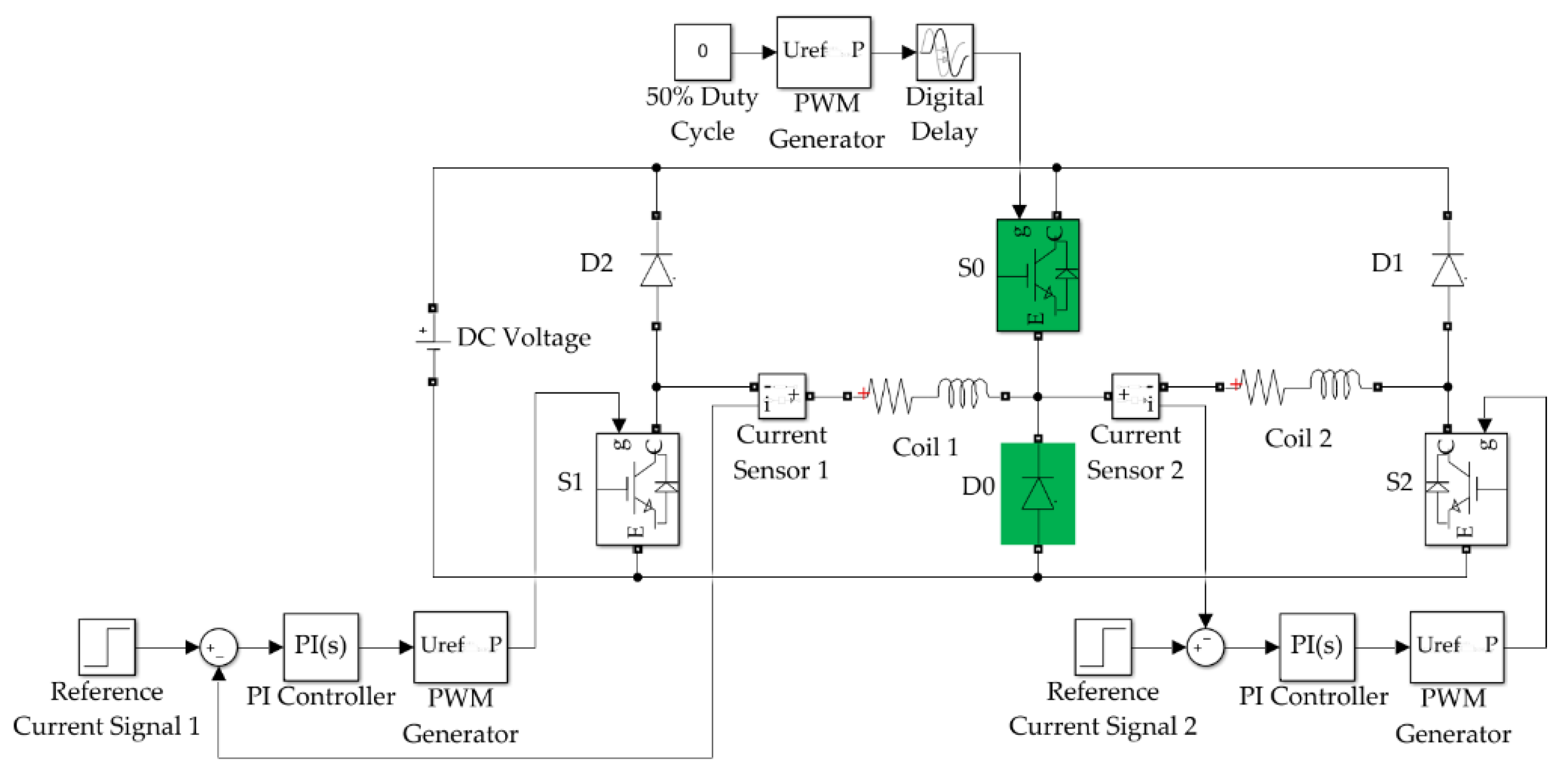
3.2.2. Comparison of Output Current Response Rate
3.3. Comparison of SPA Cost
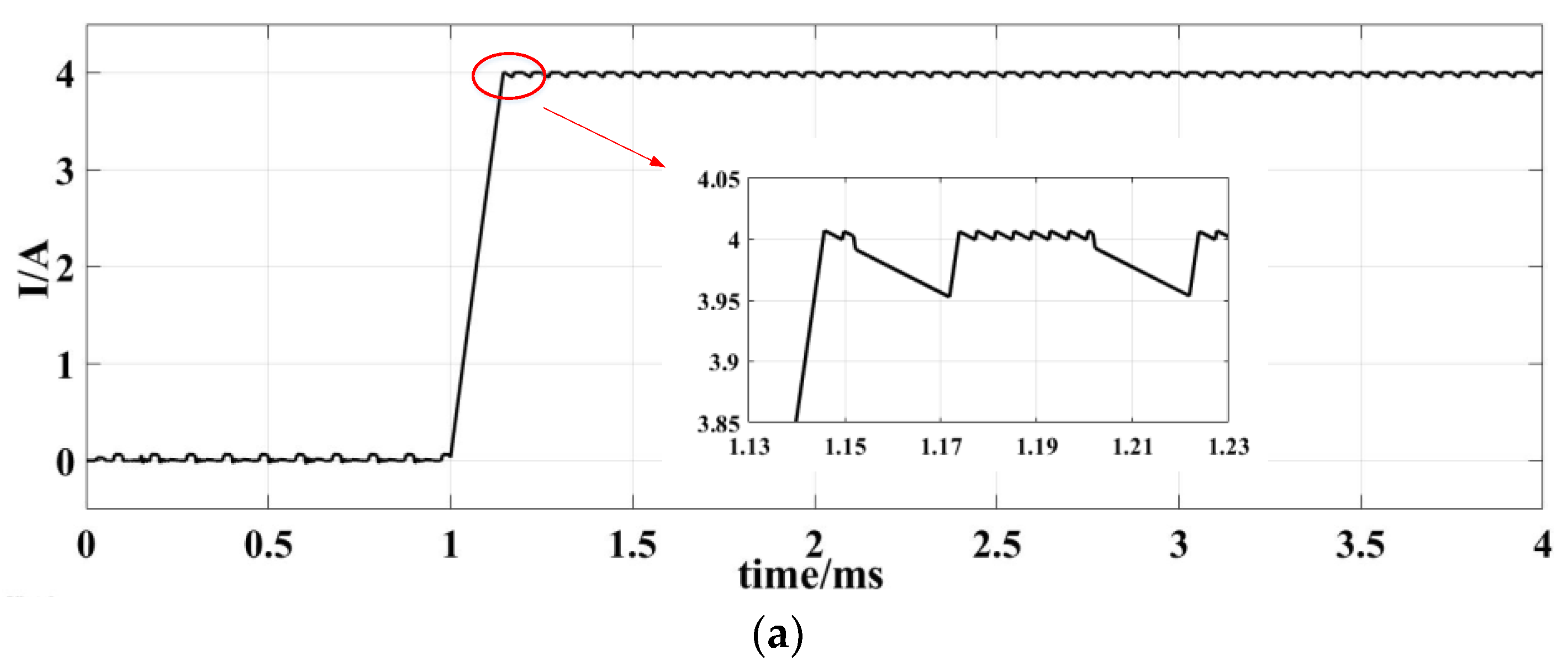
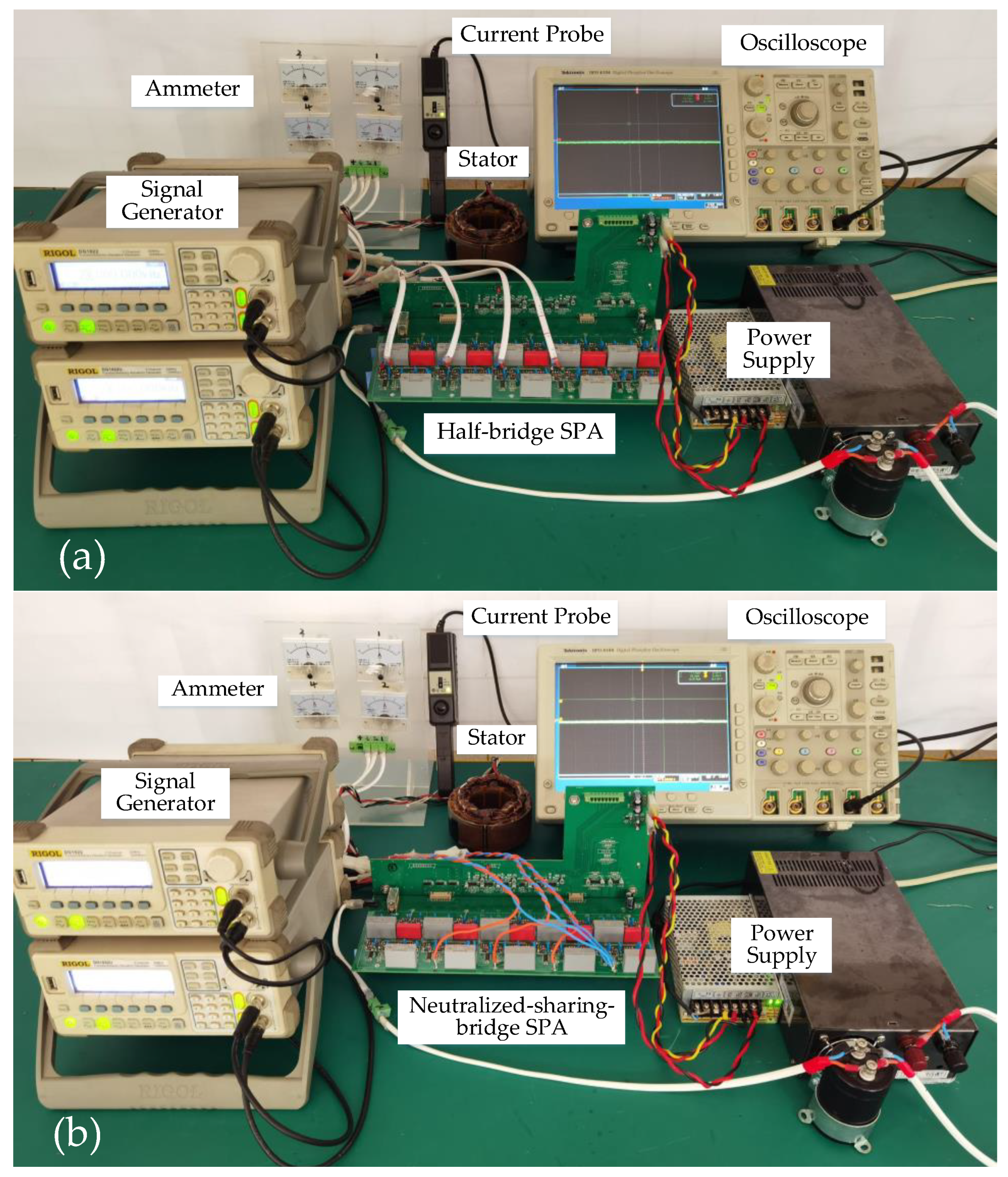
4. Experimental Verification
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Chamroon, C.; Cole, M.; Fakkaew, W. Linearizing control of a distributed actuation magnetic bearing for thin-walled rotor systems. Actuators 2020, 9, 99. [Google Scholar] [CrossRef]
- Maslen, E.H.; Schweitzer, G.; Bleuler, H.; Cole, M.; Traxler, A. Magnetic Bearings—Theory, Design, and Application to Rotating Machinery; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Xin, C.; Baixin, C.; Han, L.; Allen, G.M. An Accurate Linearization of Electromagnetic Force of Heteropolar Magnetic Bearings With Redundant Structures. J. Eng. Gas Turbines Power Trans. ASME 2020, 142. [Google Scholar] [CrossRef]
- ISO 14839-3. Mechanical Vibration—Vibration of Rotating Machinery Equipped with Active Magnetic Bearings—Part 3: Evaluation of Stability Margin. Available online: https://www.iso.org/standard/39057.html (accessed on 13 June 2021).
- Carabelli, S.; Maddaleno, F.; Muzzarelli, M. High-efficiency linear power amplifier for active magnetic bearings. IEEE Trans. Ind. Electron. 2000, 47, 17–24. [Google Scholar] [CrossRef]
- Cheng, X.; Zhang, L.; Zhou, R.G.; Song, S.; Wang, B.; Liu, H. Analysis of output precision characteristics of digital switching power amplifier in the active magnetic bearings system. Automatika 2017, 58, 205–215. [Google Scholar] [CrossRef]
- Chiu, H.-L.; Tsai, N.-C. Dual-mode power drive for active magnetic bearings. IET Electr. Power Appl. 2019, 13, 162–167. [Google Scholar] [CrossRef]
- Ren, Y.; Fang, J.C. Current-sensing resistor design to include current derivative in PWM H-bridge unipolar switching power amplifiers for magnetic bearings. IEEE Trans. Ind. Electron. 2012, 59, 4590–4600. [Google Scholar] [CrossRef]
- Fang, J.C.; Ren, Y. Self-adaptive phase-lead compensation based on unsymmetrical current sampling resistance network for magnetic bearing switching power amplifiers. IEEE Trans. Ind. Electron. 2012, 59, 1218–1227. [Google Scholar] [CrossRef]
- Jiang, D.; Kshirsagar, P. Analysis and control of a reduced switch converter for active magnetic bearings. In Proceedings of the IEEE Applied Power Electronics Conference & Exposition, Long Beach, CA, USA, 20–24 March 2016; pp. 3616–3622. [Google Scholar]
- Jiang, D.; Li, T.; Hu, Z.D.; Sun, H.B. Novel topologies of power electronics converter as active magnetic bearing drive. IEEE Trans. Ind. Electron. 2020, 67, 950–959. [Google Scholar] [CrossRef]
- Zhou, J.; Deng, Z.; Liu, C.; Li, K.; He, J. Current ripple analysis of five-phase six-leg switching power amplifiers for magnetic bearing with one-cycle control. In Proceedings of the International Conference on Electrical Machines & Systems, Chiba, Japan, 13–16 November 2016. [Google Scholar]
- Hu, Y.; Yang, K.; Guo, X.; Zhou, J.; Wu, H. Evaluation of the topology of switching power amplifiers for active magnetic bearings. Proceedings 2020, 64, 25. [Google Scholar]
- Ran, S.; Hu, Y.; Wu, H.; Cheng, X. Resonance vibration control for AMB flexible rotor system based on mu-synthesis controller. Math. Probl. Eng. 2018, 2018, 4362101. [Google Scholar] [CrossRef]
- Sun, J.; Zhang, Y. A novel integrated structure with a radial displacement sensor and a permanent magnet biased radial magnetic bearing. Sensors 2014, 14, 1950–1960. [Google Scholar] [CrossRef] [PubMed]
- Kshirsagar, P.; Yan, P. Multiple-Axis Magnetic Bearing and Control of The Magnetic Bearing with Active Switch Topologies. U.S. Patent US 2015/0068243 A1, 12 March 2015. [Google Scholar]
- Koehler, B.-U.; Denk, J.; Van Maanen, G.; Lang, M. Applying standard industrial components for active magnetic bearings. Actuators 2017, 6, 8. [Google Scholar] [CrossRef]








| Topology | Switches | Diodes | Isolated Power Suppliers |
|---|---|---|---|
| Half-bridge | 4 × 4 = 16 | 4 × 4 = 16 | 4 × 2 + 1 = 9 |
| Three-phase-half-bridge | 3 × 4 = 12 | 3 × 4 = 12 | 4 + 1 = 5 |
| Neutralized-sharing-bridge | 2 × 4 + 2 = 10 | 2 × 4 + 2 = 10 | 4 + 2 = 6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, K.; Hu, Y.; Guo, X.; Zhou, J.; Wu, H. Evaluation of Switching Power Amplifier Topology for Active Magnetic Bearings. Actuators 2021, 10, 131. https://doi.org/10.3390/act10060131
Yang K, Hu Y, Guo X, Zhou J, Wu H. Evaluation of Switching Power Amplifier Topology for Active Magnetic Bearings. Actuators. 2021; 10(6):131. https://doi.org/10.3390/act10060131
Chicago/Turabian StyleYang, Kezhen, Yefa Hu, Xinhua Guo, Jian Zhou, and Huachun Wu. 2021. "Evaluation of Switching Power Amplifier Topology for Active Magnetic Bearings" Actuators 10, no. 6: 131. https://doi.org/10.3390/act10060131
APA StyleYang, K., Hu, Y., Guo, X., Zhou, J., & Wu, H. (2021). Evaluation of Switching Power Amplifier Topology for Active Magnetic Bearings. Actuators, 10(6), 131. https://doi.org/10.3390/act10060131








