Design and Modeling of a Bio-Inspired Flexible Joint Actuator
Abstract
1. Introduction
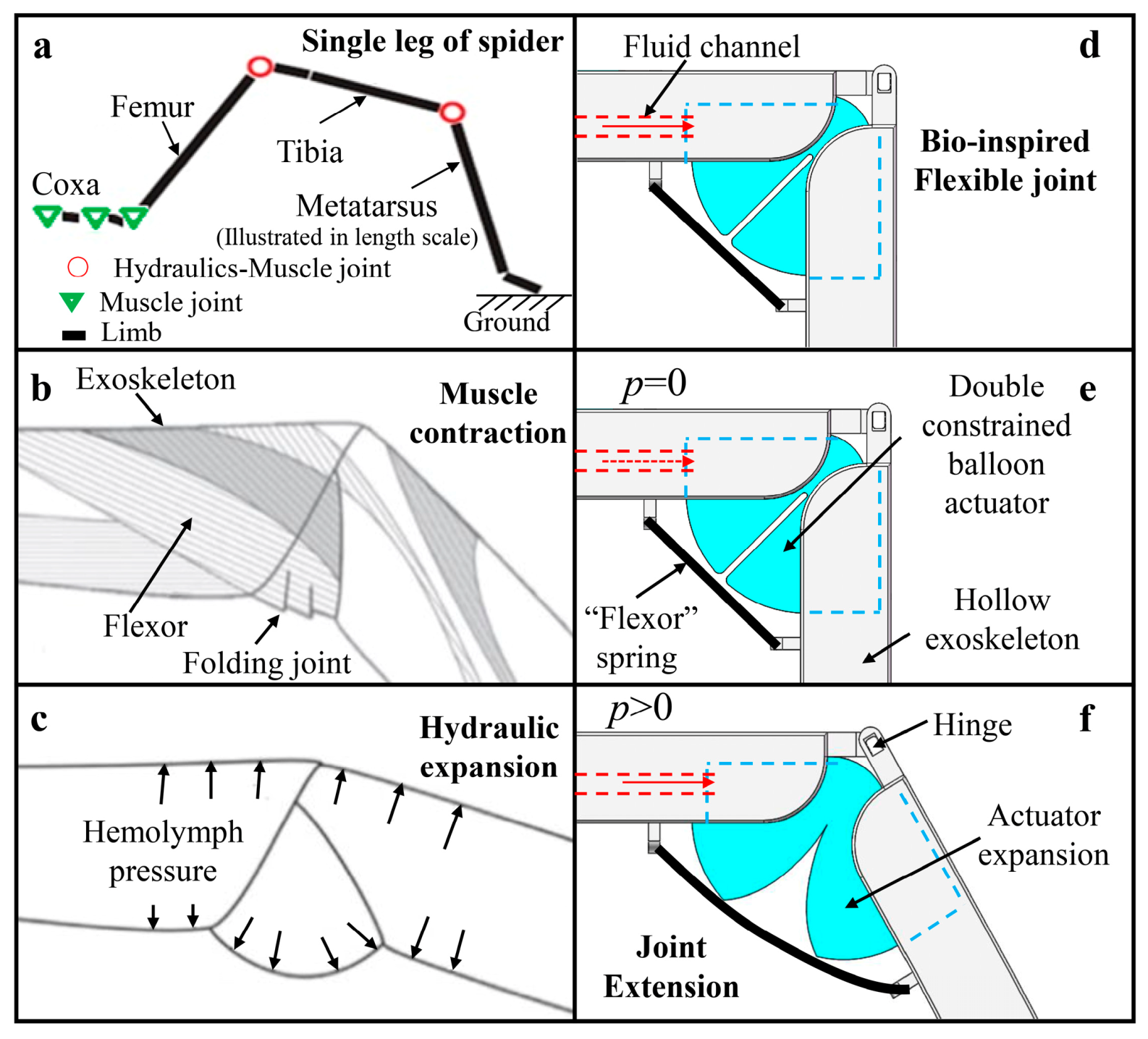
2. Bio-Inspired Flexible Joint
2.1. Double-Constrained Balloon Actuator
2.2. Soft Arthropod Robot with Two Joints
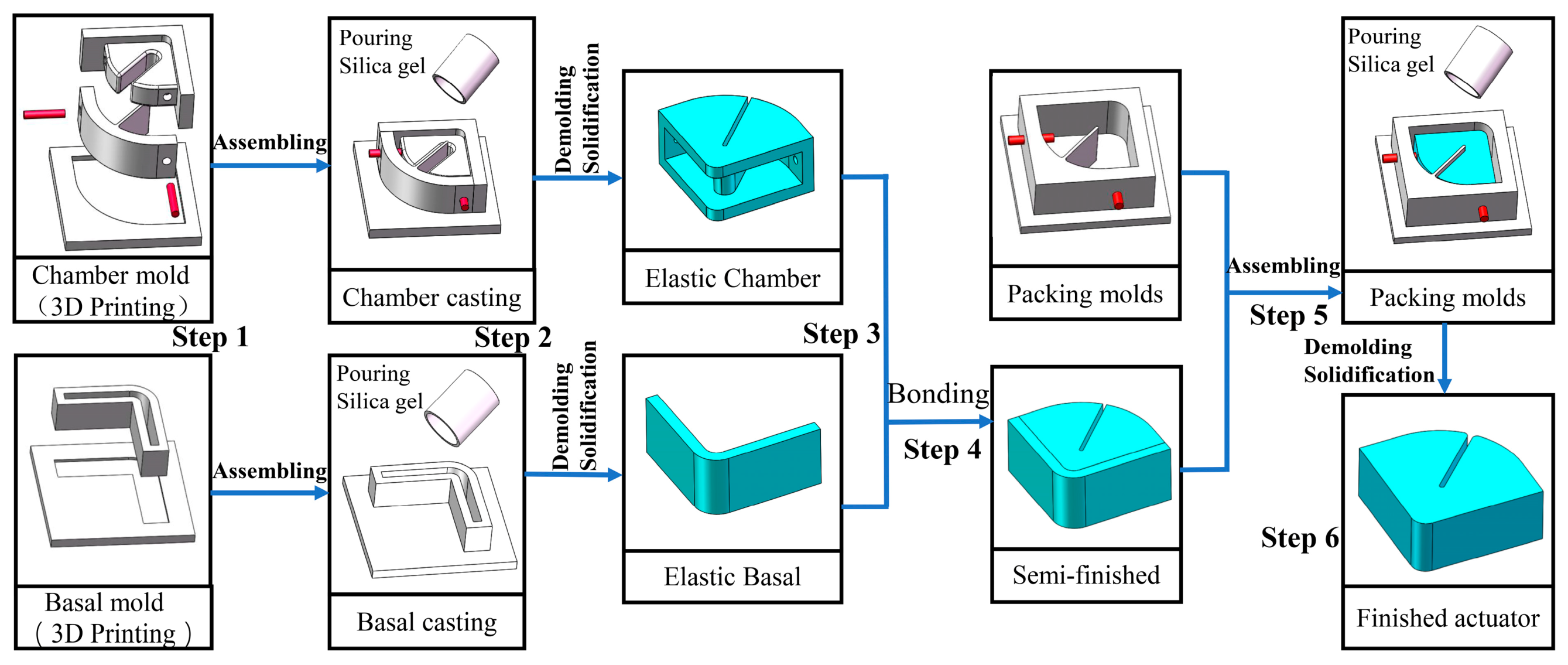
2.3. Fabrication of Flexible Joint
- Step 1: 3D printing molds. The pneumatic actuator can be divided into two parts, the chamber and the basal, all of them are made of casting. A stereolithography 3D printer EP-A650A Pro made by SHINNING 3D Co. Ltd. (Zhejiang, China) was used to make the chamber mold and basal mold. After printing, the molds were polished with 220 mesh to 5000 mesh sandpaper.
- Step 2: Preparing silica gel solution and casting. The silica gel solution (RTV-2) and curing agent (TEOS) were mixed in a container according to the weight ratio of 100:2, and the mixture was evenly stirred by an electromagnetic mixer. The prepared silicone gel solution was put into a vacuum tank, a negative pressure of—98 kpa was applied and left to stand for 5 min for defoaming. Then the silicone gel solution was slowly injected into the molds and cured at room temperature.
- Step 3: Demolding. To ensure the quality of solidification, demolding was carried out after casting for 24 h.
- Step 4: Bonding. The silica gel adhesive RTV-D06 was used to bond the chamber to the base layer.
- Step 5: Packaging molds. The chamber and base layer bonded in step 6 was put into the packaging mold. The semi-finished product was cast again and cured at room temperature.
- Step 6: Demolding. The fine silica gel gas tube was inserted into the chamber, and the socket was sealed with RTV D06 glue. The fabrication of flexible joint was now complete.
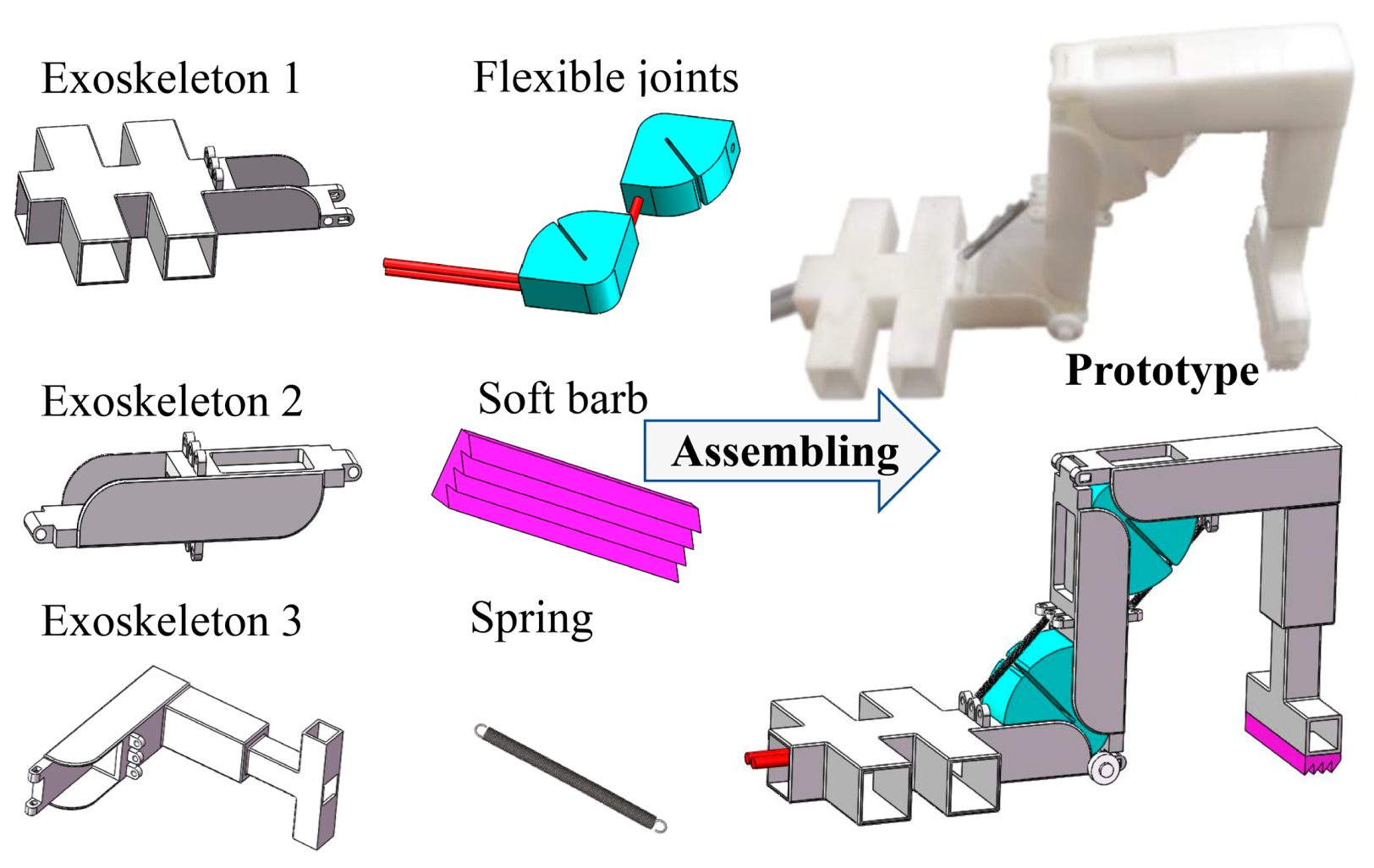
2.4. Fabrication of Soft Arthropod Robot
3. Modeling of Flexible Joint
3.1. Mathematical Model
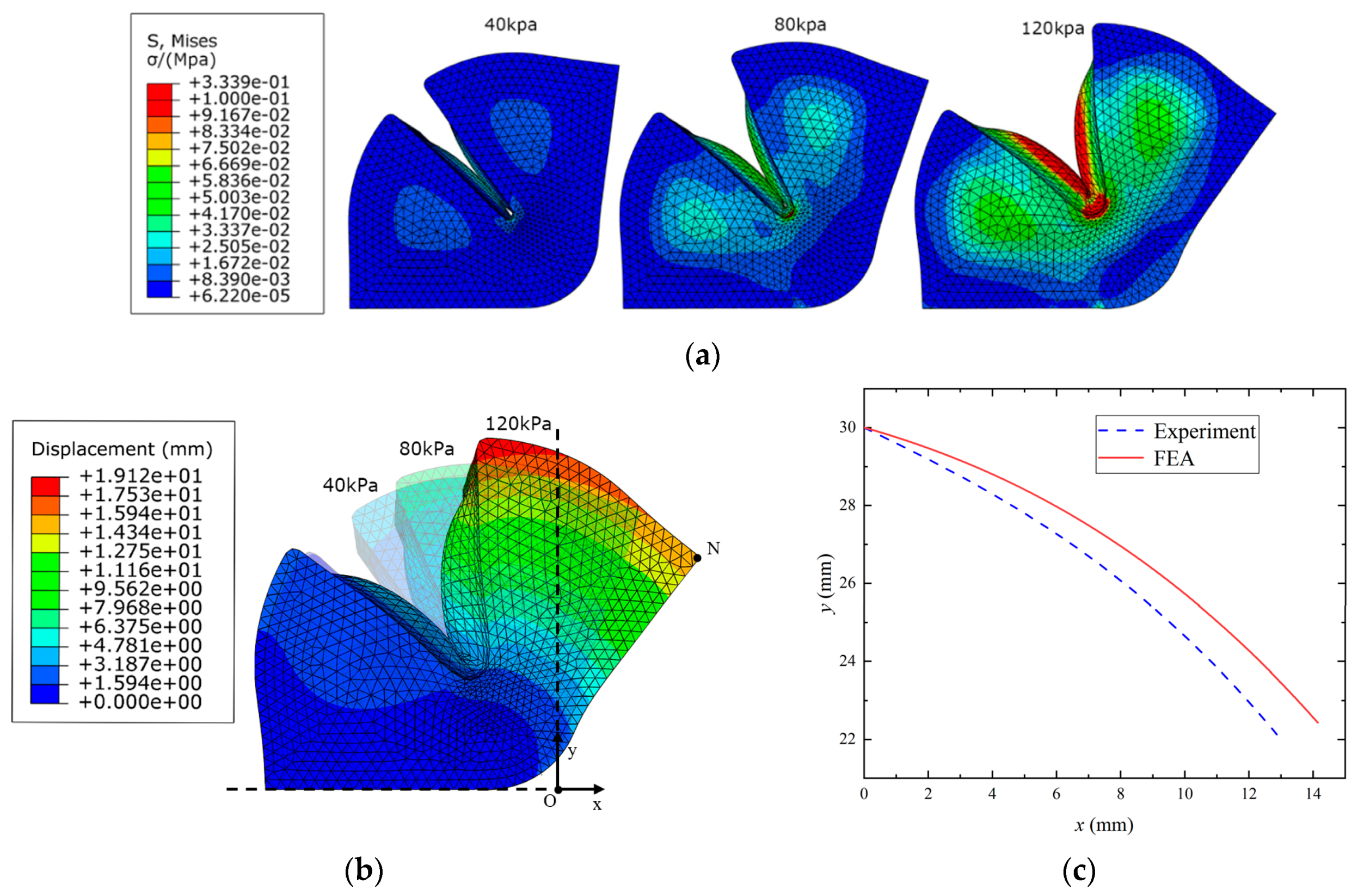
3.2. FEA Model
4. Experiments and Discussions
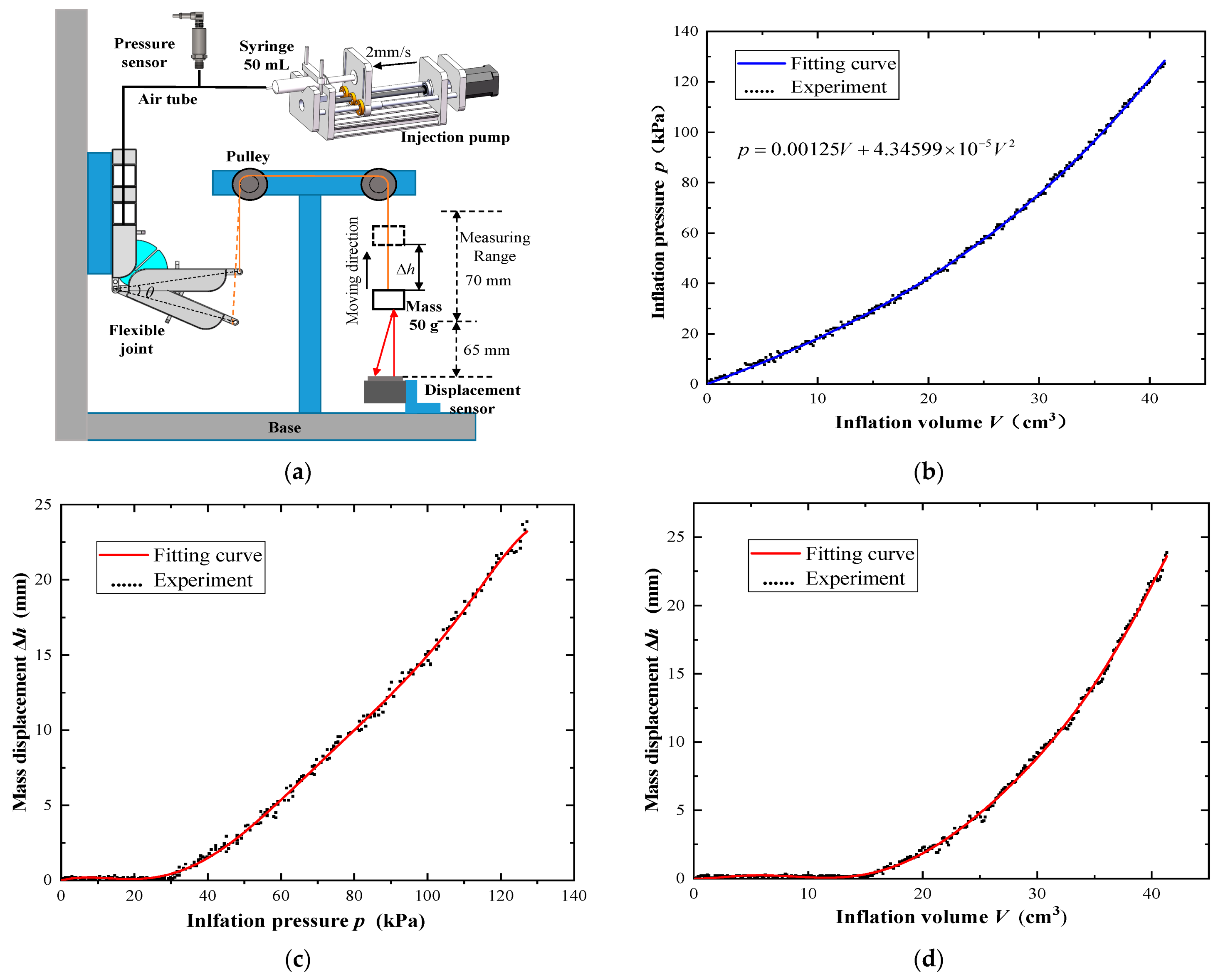
4.1. Rotation Angle
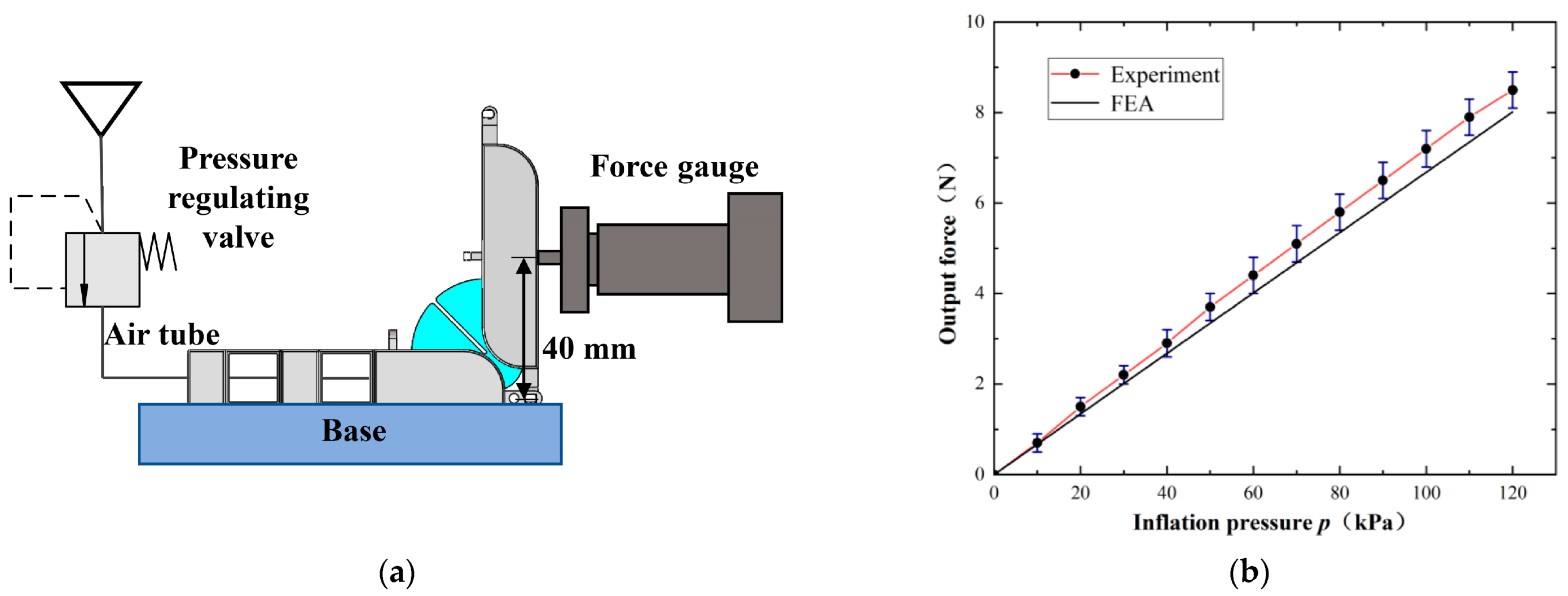
4.2. Output Force
4.3. Efficiency
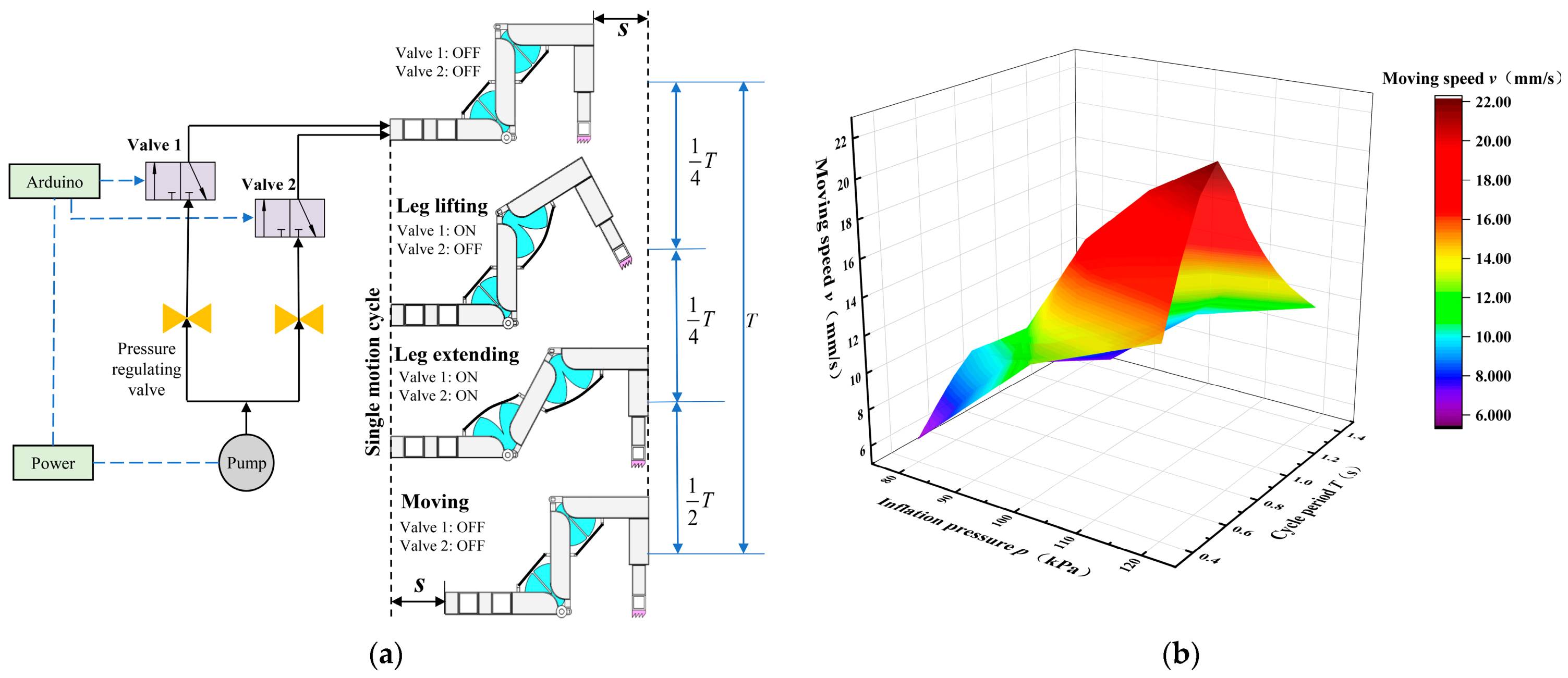
4.4. Locomotion of Single-Legged Soft Arthropod Robot
- When the inflation pressure is small (<90 kPa), the speed increases slowly due to the compressibility of the air;
- When the inflation pressure is in the range of 90 kPa to 110 kPa, the speed increases rapidly;
- When the inflation pressure is above 110 kPa, the speed increases slowly again.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Palagi, S.; Fischer, P. Bioinspired microrobots. Nat. Rev. Mater. 2018, 3, 113–124. [Google Scholar] [CrossRef]
- Rus, D.; Tolley, M.T. Design, fabrication and control of soft robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef] [PubMed]
- Hines, L.; Petersen, K.; Lum, G.Z.; Sitti, M. Soft Actuators for Small-Scale Robotics. Adv. Mater. 2017, 29, 1603483. [Google Scholar] [CrossRef] [PubMed]
- Wirekoh, J.; Parody, N.; Riviere, C.N.; Park, Y.-L. Design of fiber-reinforced soft bending pneumatic artificial muscles for wearable tremor suppression devices. Smart Mater. Struct. 2021, 30, 015013. [Google Scholar] [CrossRef]
- Manfredi, L.; Yue, L.; Zhang, J.; Cuschieri, A. A 4 DOFs variable stiffness soft module. In Proceedings of the 2018 IEEE International Conference on Soft Robotics (RoboSoft), Livorno, Italy, 24–28 April 2018; pp. 94–99. [Google Scholar]
- Gorissen, B.; Reynaerts, D.; Konishi, S.; Yoshida, K.; Kim, J.-W.; De Volder, M. Elastic Inflatable Actuators for Soft Robotic Applications. Adv. Mater. 2017, 29, 1604977. [Google Scholar] [CrossRef] [PubMed]
- Manfredi, L.; Putzu, F.; Guler, S.; Huan, Y.; Cuschieri, A. 4 DOFs hollow soft pneumatic actuator—HOSE. Mater. Res. Express 2018, 6, 045703. [Google Scholar] [CrossRef]
- Guan, Q.; Sun, J.; Liu, Y.; Leng, J. Status of and trends in soft pneumatic robotics. Sci. Sin. Technol. 2020, 50, 897–934. [Google Scholar]
- Ranzani, T.; Gerboni, G.; Cianchetti, M.; Menciassi, A. A bioinspired soft manipulator for minimally invasive surgery. Bioinspir. Biomimetics 2015, 10, 035008. [Google Scholar] [CrossRef] [PubMed]
- Ji, X.; Liu, X.; Cacucciolo, V.; Imboden, M.; Civet, Y.; El Haitami, A.; Cantin, S.; Perriard, Y.; Shea, H. An autonomous untethered fast soft robotic insect driven by low-voltage dielectric elastomer actuators. Sci. Robot. 2019, 4, eaaz6451. [Google Scholar] [CrossRef] [PubMed]
- Bernth, J.E.; Arezzo, A.; Liu, H. A Novel Robotic Meshworm with Segment-Bending Anchoring for Colonoscopy. IEEE Robot. Autom. Lett. 2017, 2, 1718–1724. [Google Scholar] [CrossRef]
- Wehner, M.; Truby, R.L.; Fitzgerald, D.J.; Mosadegh, B.; Whitesides, G.M.; Lewis, J.A.; Wood, R.J. An integrated design and fabrication strategy for entirely soft, autonomous robots. Nature 2016, 536, 451–455. [Google Scholar] [CrossRef] [PubMed]
- Manfredi, L.; Capoccia, E.; Ciuti, G.; Cuschieri, A. A Soft Pneumatic Inchworm Double balloon (SPID) for colonoscopy. Sci. Rep. 2019, 9, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Cianchetti, M.; Mattoli, V.; Mazzolai, B.; Laschi, C.; Dario, P. A new design methodology of electrostrictive actuators for bio-inspired robotics. Sens. Actuators B Chem. 2009, 142, 288–297. [Google Scholar] [CrossRef]
- Siebert, T.; Weihmann, T.; Rode, C.; Blickhan, R. Cupiennius salei: Biomechanical properties of the tibia–metatarsus joint and its flexing muscles. J. Comp. Physiol. B 2010, 180, 199–209. [Google Scholar] [CrossRef] [PubMed]
- Kropf, C. Hydraulic system of locomotion. In Spider Ecophysiology; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Foelix, R.F. Biology of Spiders; Oxford University Press: Oxford, UK, 2011. [Google Scholar]
- Zentner, L. Modelling and Application of the Hydraulic Spider Leg Mechanism. In Spider Ecophysiology; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Berring, J.; Kianfar, K.; Lira, C.; Menon, C.; Scarpa, F. A smart hydraulic joint for future implementation in robotic structures. Robot. 2010, 28, 1045–1056. [Google Scholar] [CrossRef]
- Liu, C.; Chen, S.; Sheng, C.; Ding, P.; Qian, Z.; Ren, L. The art of a hydraulic joint in a spider’s leg: Modelling, computational fluid dynamics (CFD) simulation, and bio-inspired design. J. Comp. Physiol. A 2019, 205, 491–504. [Google Scholar] [CrossRef] [PubMed]

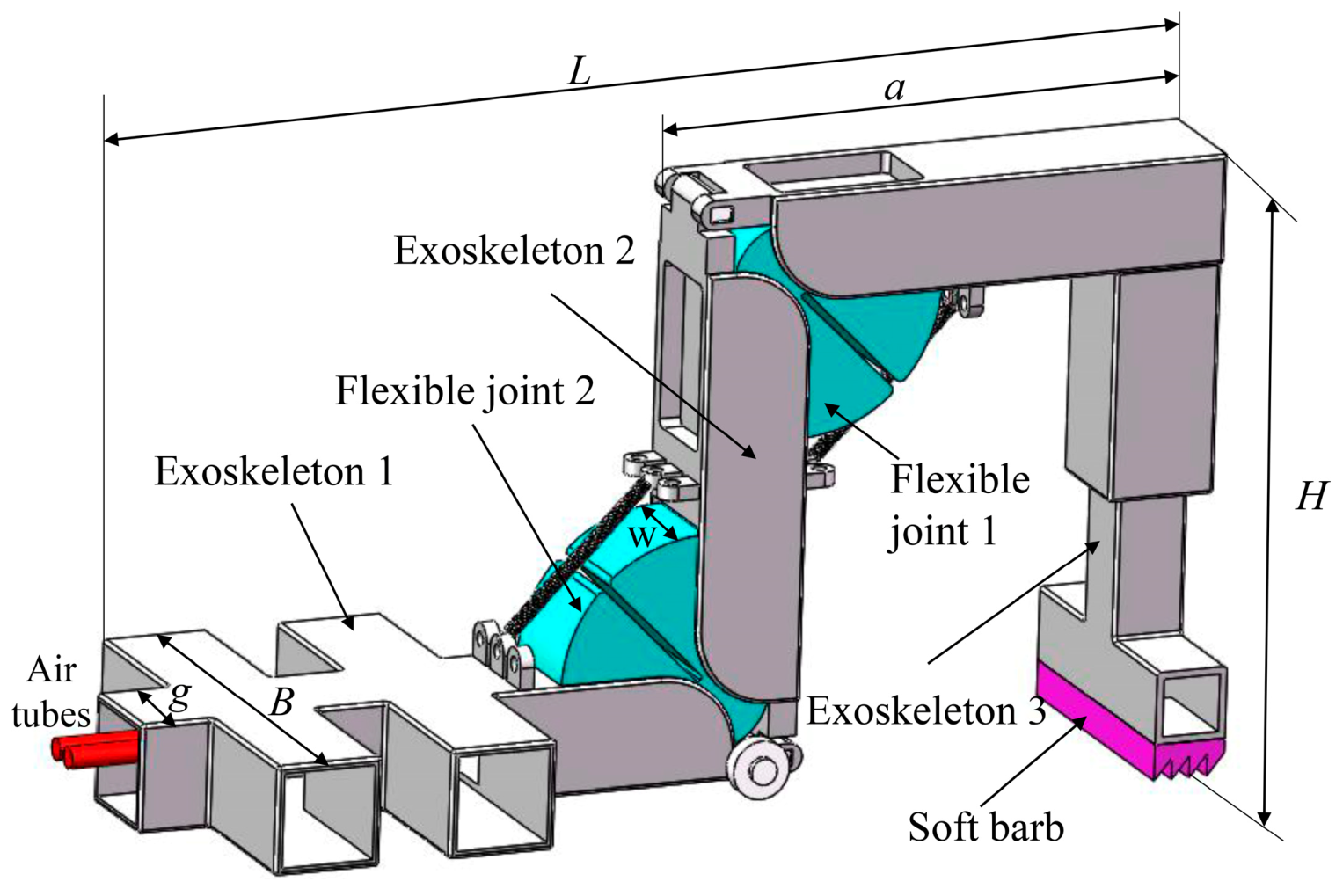


| Parameter | B | g | L | H | a | w | R | w1 |
| Value | 60 | 17 | 150 | 80 | 75 | 15 | 30 | 9 |
| Parameter | r | l1 | l2 | d | d1 | d2 | d3 | u |
| Value | 27 | 24 | 15 | 3 | 3 | 2 | 3 | 0.5 |
| Δh (mm) | 4.1 | 9.2 | 13.3 | 18.6 | 21.7 |
| Q (mJ) | 563 | 956 | 1294 | 1700 | 1928 |
| W (mJ) | 2.05 | 4.6 | 6.65 | 9.3 | 10.85 |
| η | 0.36% | 0. 48% | 0.51% | 0.54% | 0.56% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, M.; Rong, C.; He, L. Design and Modeling of a Bio-Inspired Flexible Joint Actuator. Actuators 2021, 10, 95. https://doi.org/10.3390/act10050095
Xu M, Rong C, He L. Design and Modeling of a Bio-Inspired Flexible Joint Actuator. Actuators. 2021; 10(5):95. https://doi.org/10.3390/act10050095
Chicago/Turabian StyleXu, Ming, Cheng Rong, and Long He. 2021. "Design and Modeling of a Bio-Inspired Flexible Joint Actuator" Actuators 10, no. 5: 95. https://doi.org/10.3390/act10050095
APA StyleXu, M., Rong, C., & He, L. (2021). Design and Modeling of a Bio-Inspired Flexible Joint Actuator. Actuators, 10(5), 95. https://doi.org/10.3390/act10050095





