Analytical and Experimental Investigations of Novel Maglev Coupling Based on Opposed Halbach Array for a 2D Valve
Abstract
1. Introduction
2. Configuration and Working Principle
3. Analytical Modelling
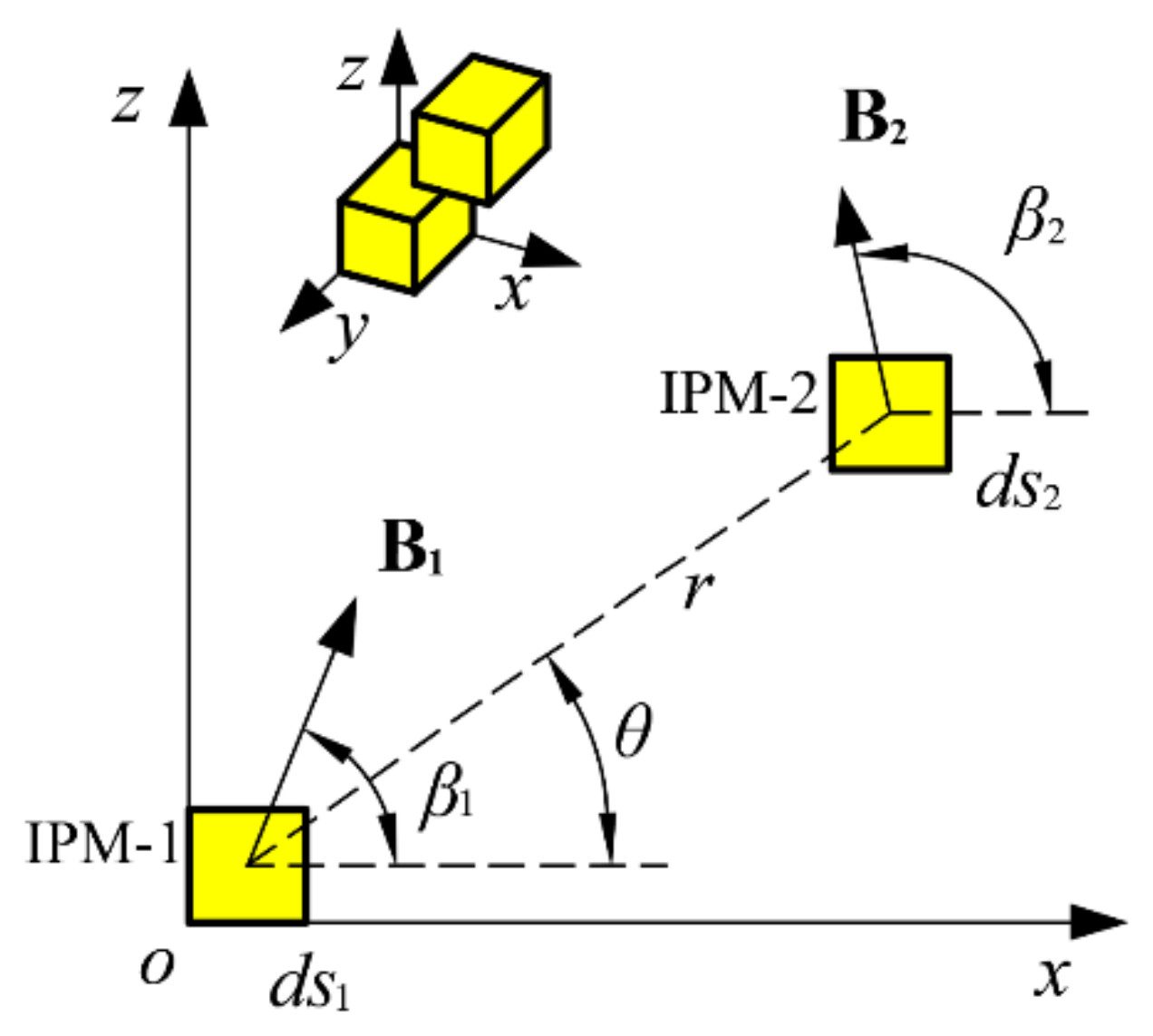
3.1. Analytical Modelling of Permanent Magnet Units
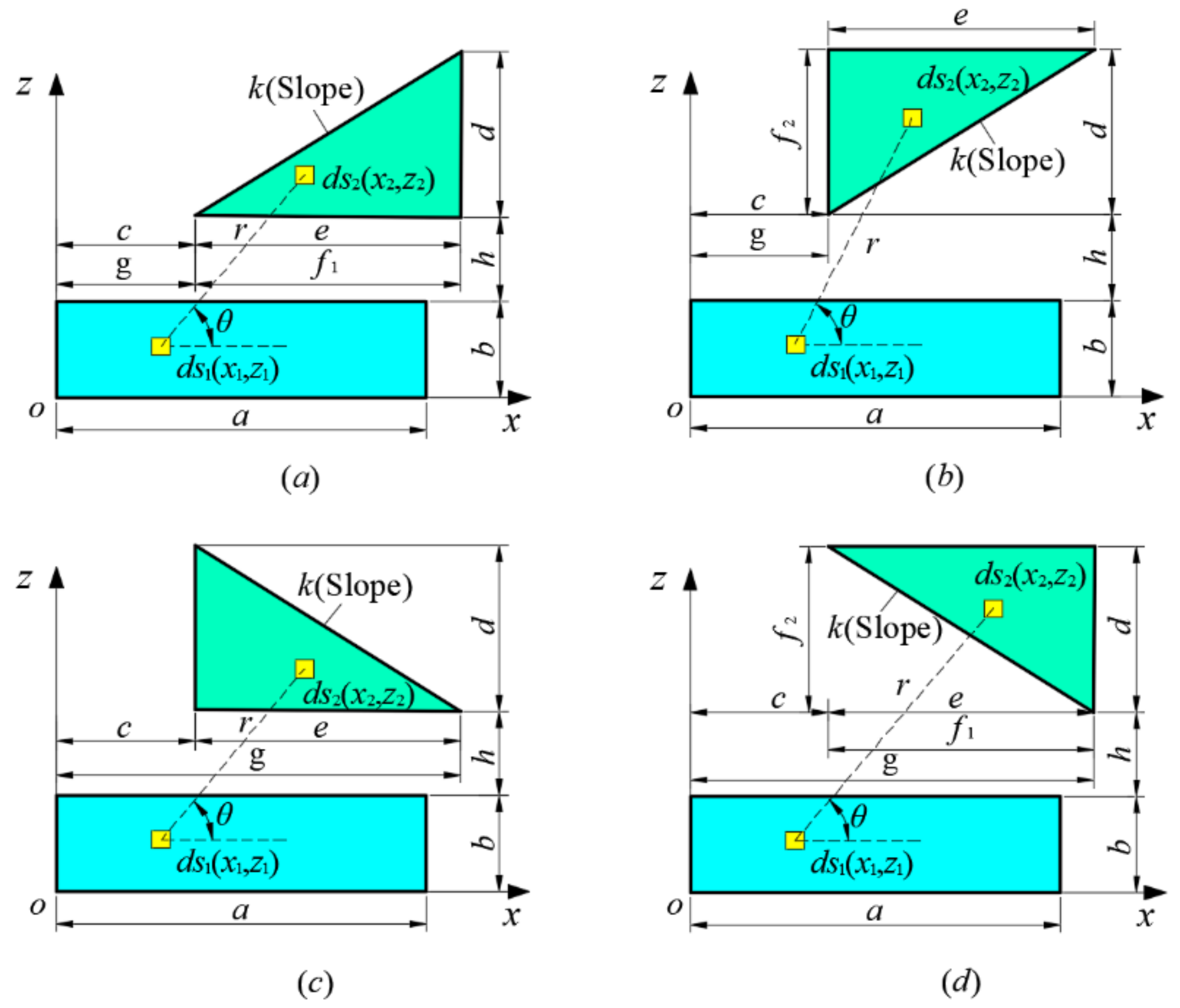
3.1.1. Rectangular–Rectangular CPMUs
3.1.2. Triangular–Rectangular CPMUs
3.1.3. Triangular–Triangular CPMUs
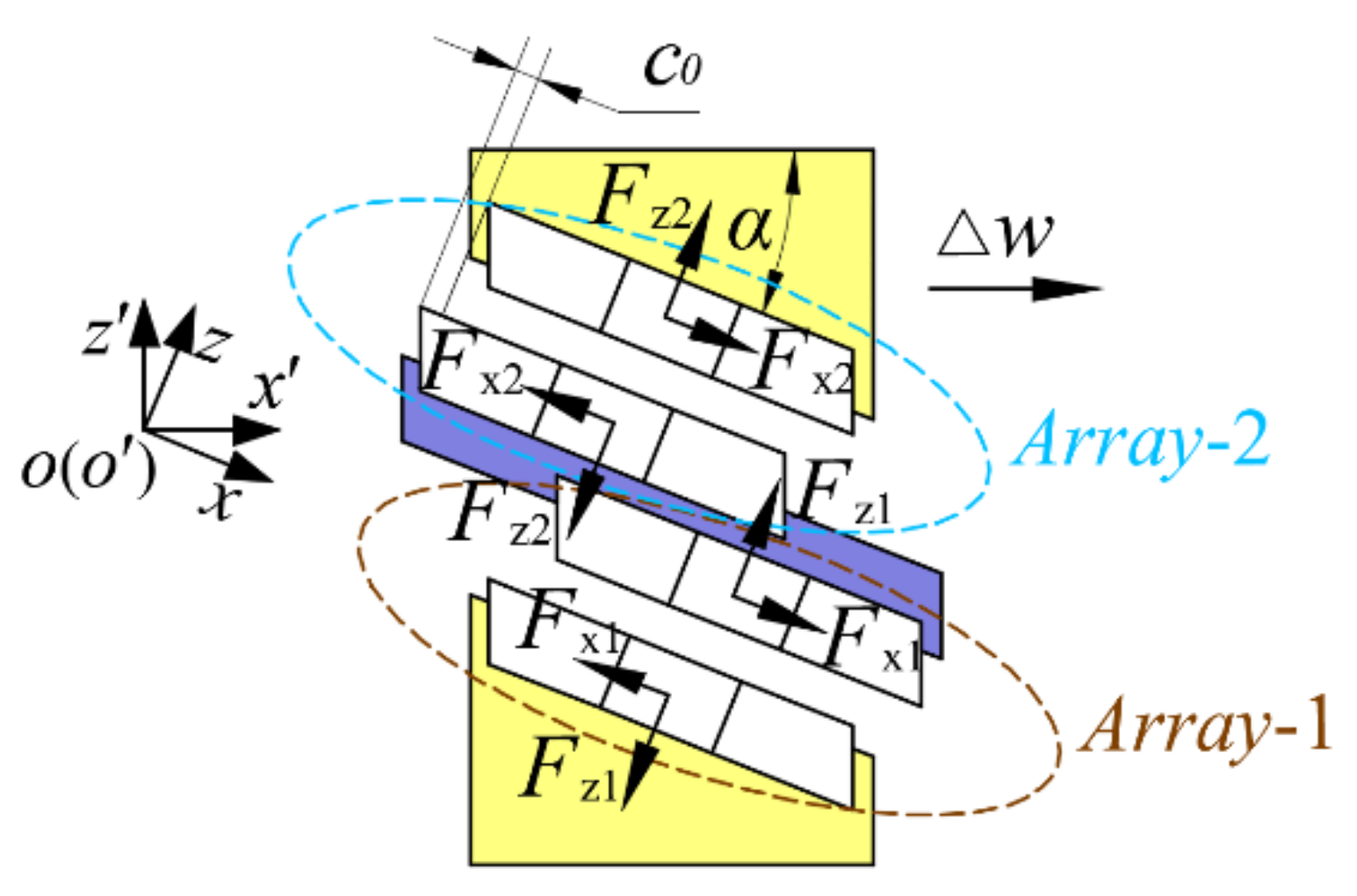
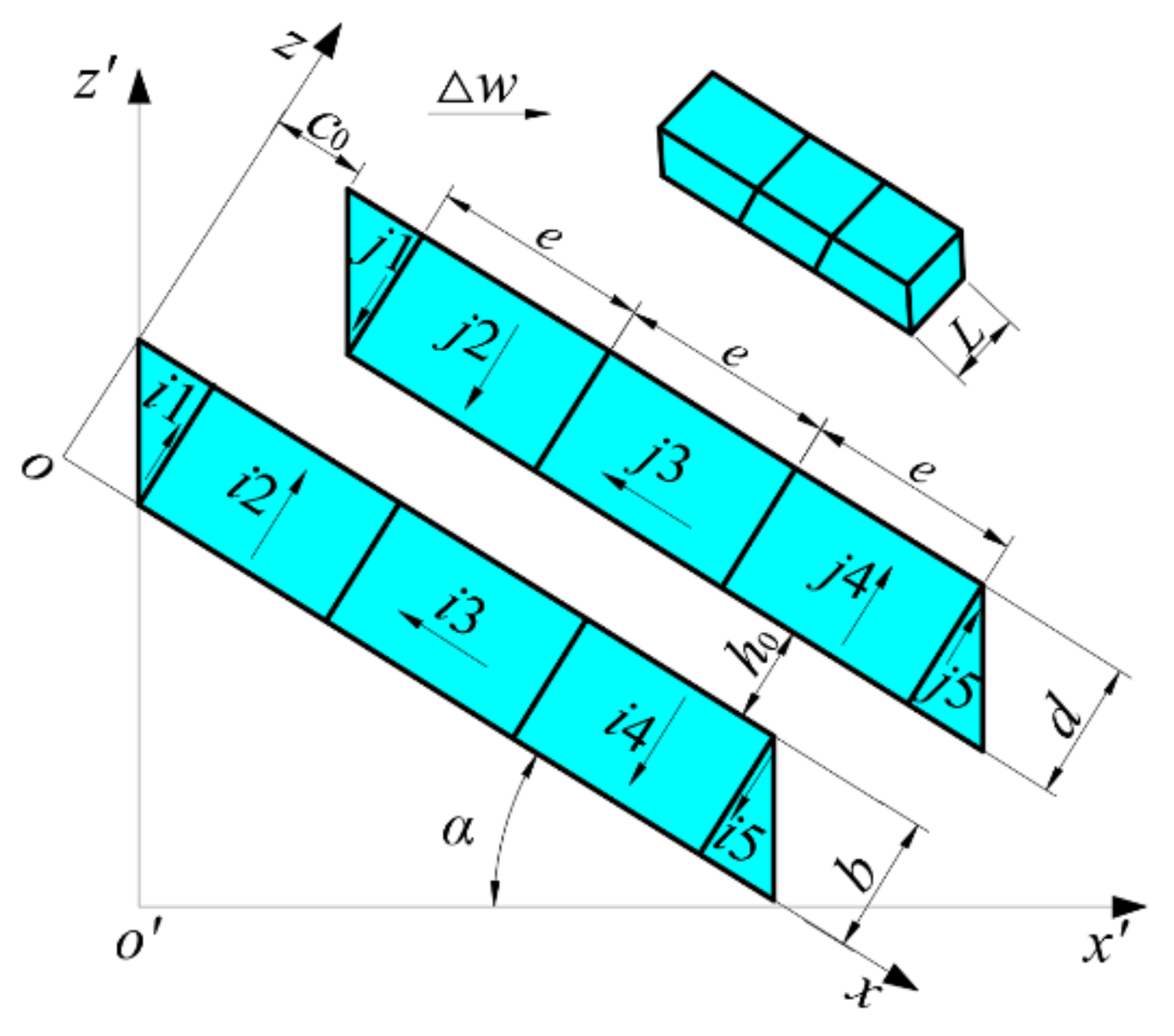
3.2. Superimposition of CPMUs
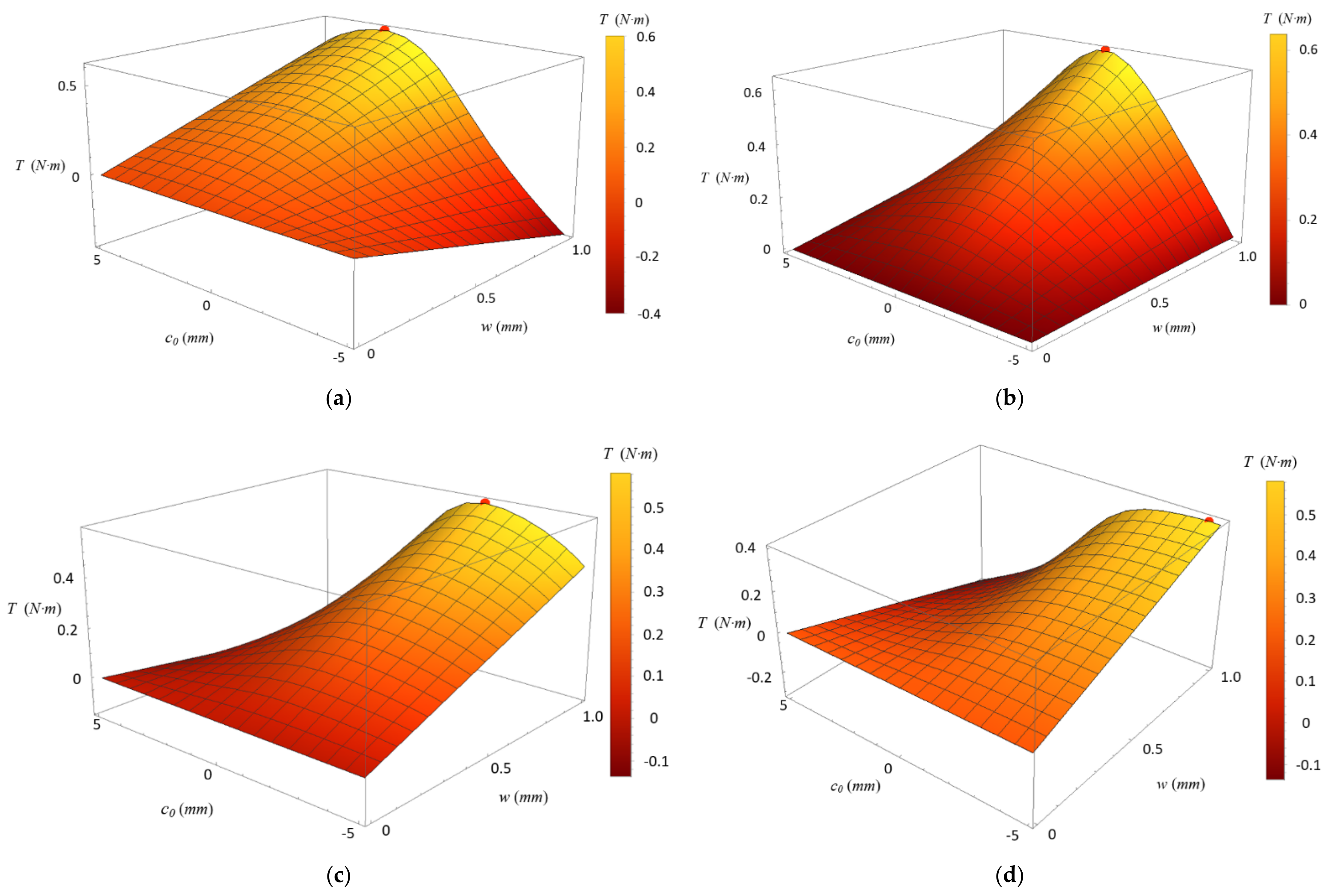
4. Parametric Analysis
5. Finite Element Simulation
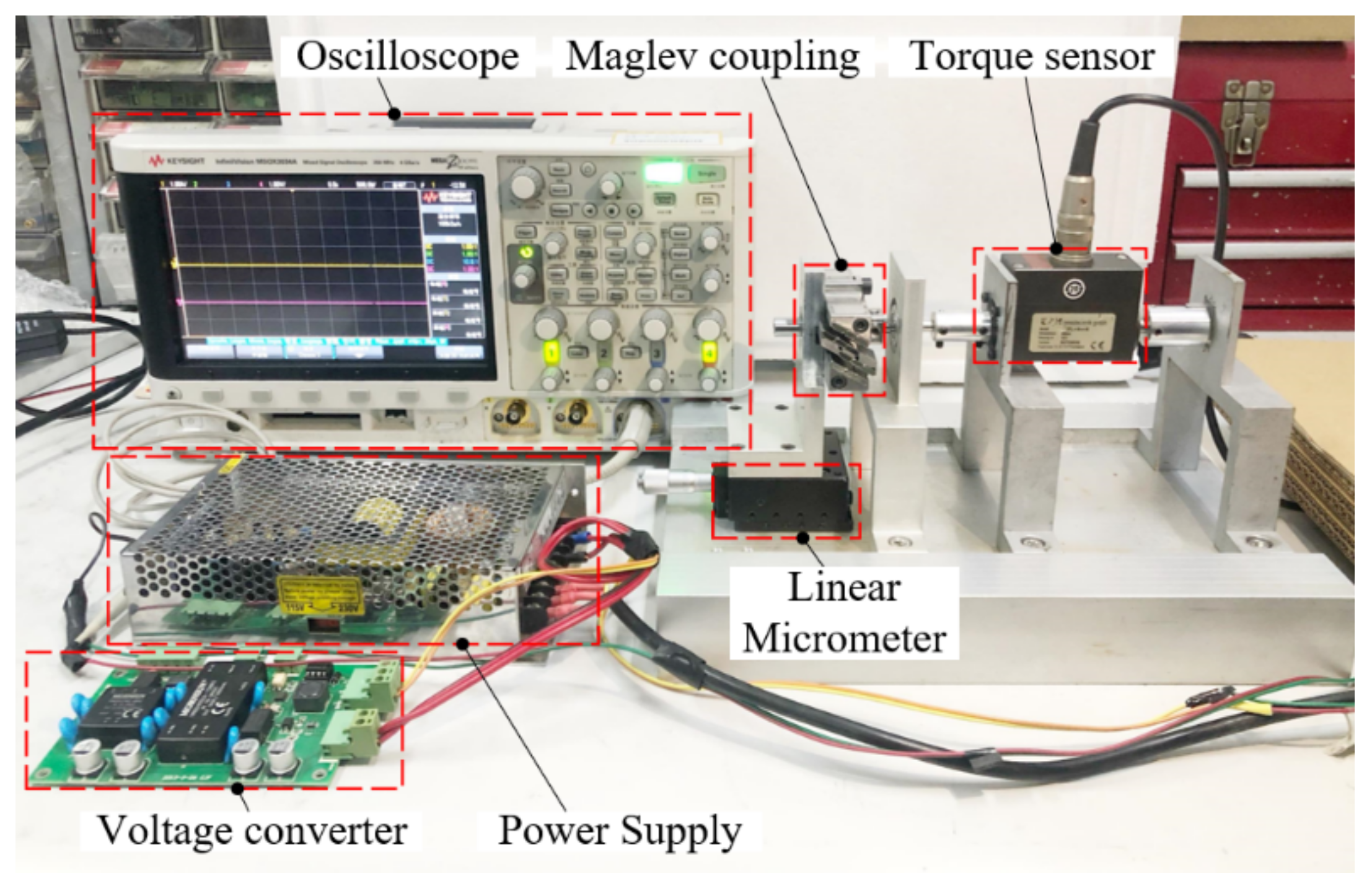
6. Experimental Study
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| 2D | Two-dimensional |
| EMC | Electro-mechanical converter |
| LVDT | Linear variable differential transformer |
| LEMC | Linear electro-mechanical converter |
| REMC | Rotary electro-mechanical converter |
| PM | Permanent magnet |
| IPM | Infinitesimals of permanent magnet |
| PMU | Permanent magnet unit |
| CPMU | Combination of permanent magnet unit |
| FEM | Finite element Method |
Nomenclature
| Bernoulli force | |
| Output torque | |
| Pitch angle of maglev coupling | |
| Magnetic common energy | |
| Residual magnetic induction intensity of IPM-1 | |
| Residual magnetic induction intensity of IPM-2 | |
| Magnetic field intensity generated by IPM-1 | |
| Magnetizing angle of IPM-1 | |
| Magnetizing angle of IPM-2 | |
| Angle between the point magnetic charges | |
| Distance between point magnetic charges | |
| Area of IPM-1 | |
| Area of IPM-2 | |
| Magnetic permeability of vacuum | |
| Length of PM (Y direction) | |
| distance between the left vertex of the hypotenuse and the vertical edge of the triangle | |
| distance between the lower vertex of the hypotenuse and the horizontal edge of the triangle | |
| distance between the lower vertex of triangle hypotenuse and z axis | |
| slope of triangle hypotenuse | |
| distance between the left vertex of the hypotenuse and the vertical edge of the green color triangle | |
| distance between the lower vertex of the hypotenuse and the horizontal edge of the green color triangle | |
| distance between the lower vertex of green color triangle hypotenuse and z axis | |
| slope of green color triangle hypotenuse | |
| Initial shifted distance of array-1 and array-2 | |
| Shifted distance of array-1 after moving | |
| Shifted distance of array-2 after moving | |
| Initial air gap height of array-1 and array-2 | |
| Air gap height of array-1 after moving | |
| Air gap height of array-2 after moving | |
| Moving displacement of external armature on axis direction | |
| Moving displacement of external armature on axis direction | |
| Moving displacement of external armature on axis direction | |
| X direction interaction force of array-1 | |
| Z direction interaction force of array-1 | |
| X direction interaction force of array-2 | |
| Z direction interaction force of array-2 | |
| Lever of force |
References
- Vyas, J.J.; Gopalsamy, B.; Joshi, H. Electro-Hydraulic Actuation Systems: Design, Testing, Identification and Validation; Springer Press: Singapore, 2018; ISBN 9789811325465. [Google Scholar]
- Xu, B.; Shen, J.; Liu, S.; Su, Q.; Zhang, J. Research and Development of Electro-hydraulic Control Valves Oriented to Industry 4.0: A Review. Chin. J. Mech. Eng. 2020, 33, 1–20. [Google Scholar] [CrossRef]
- Yang, H. Review of intelligent manufacturing and intelligent hydraulic components. Chin. Hydraul. Pneum. 2020, 1, 1–9. [Google Scholar]
- Tamburrano, P.; Plummer, A.R.; Distaso, E.; Amirante, R. A review of electro-hydraulic servovalve research and development. Int. J. Fluid. Power. 2018, 19, 1–23. [Google Scholar] [CrossRef]
- Tamburrano, P.; Plummer, A.R.; Distaso, E. A review of direct drive proportional electrohydraulic spool valves: Industrial state-of-the-art and research advancements. J. Dyn. Syst. Meas. Control 2019, 141, 020801. [Google Scholar] [CrossRef]
- Liu, X.; Shang, Y.; Jiao, Z.; Tang, H. Aircraft anti-skid braking control with flow servo-valve. In Proceedings of the 2015 International Conference on Fluid Power and Mechatronics (FPM), Harbin, China, 5 August 2015; pp. 536–541. [Google Scholar]
- Man, Z.; Ding, F.; Liu, S. Design and experiment on large-flow-rate electro-hydraulic control valve. Trans. Chin. Soc. Agric. Mach. 2015, 46, 345–351. [Google Scholar]
- Liu, W.; Wei, J.; Fang, J.; Li, S. Hydraulic-feedback proportional valve design for construction machinery. Proc. Inst. Mech. Eng. Part C J. Eng. Mech. Eng. Sci. 2015, 229, 3162–3178. [Google Scholar] [CrossRef]
- Zhang, J.; Lu, Z.; Xu, B.; Su, Q. Investigation on the dynamic characteristics and control accuracy of a novel proportional directional valve with independently controlled pilot stage. ISA Trans. 2019, 93, 218–230. [Google Scholar] [CrossRef]
- Wang, S.; Weng, Z.; Jin, B. A Performance Improvement Strategy for Solenoid Electromagnetic Actuator in Servo Proportional Valve. Appl. Sci. 2020, 10, 4352. [Google Scholar] [CrossRef]
- Li, C.; Yin, Y.; Wang, M. Influence of high temperature on couples matching and characteristics of jet pipe electrohydraulic servovalve. Chin. J. Mech. Eng. 2018, 54, 251–261. [Google Scholar] [CrossRef]
- Yan, H.; Wang, F.J.; Li, C.C. Research on the jet characteristics of the deflector–jet mechanism of the servo valve. Chin. Phys. B 2017, 26, 252–260. [Google Scholar] [CrossRef]
- Liu, C.; Jiang, H. A seventh-order model for dynamic response of an electro-hydraulic servo valve. Chin. J. Aeronaut. 2014, 27, 1605–1611. [Google Scholar] [CrossRef]
- Zhang, S.; Aung, N.Z.; Li, S. Reduction of undesired lateral forces acting on the flapper of a flapper–nozzle pilot valve by using an innovative flapper shape. Energy Conv. Manag. 2015, 106, 835–848. [Google Scholar] [CrossRef]
- Yin, Y. Electro Hydraulic Control Theory and Its Applications under Extreme Environment; Butterworth-Heinemann: Oxford, UK, 2019. [Google Scholar]
- Huang, L.; Ji, H.; Zhu, Y. Analysis of effective working characteristic of the proportional solenoid. In Proceedings of the 2017 International Conference on Green Energy and Applications (ICGEA), Singapore, 11 May 2017; pp. 35–38. [Google Scholar]
- Meng, B.; Lai, Y.J.; Qiu, X.G. Regulation Method for Torque–Angle Characteristics of Rotary Electric–Mechanical Converter Based on Hybrid Air Gap. Chin. J. Mech. Eng. 2020, 33, 1–11. [Google Scholar] [CrossRef]
- Li, Y.; Ding, F.; Cui, J. Low power linear actuator for direct drive electrohydraulic valves. J. Zhejiang Univ. SC A 2008, 9, 940–943. [Google Scholar] [CrossRef]
- Direct Drive Servo Valves D633/D634. Available online: https://www.heash-tech.com/uploads/59ba1fb5e65b8717954917.pdf (accessed on 27 January 2021).
- Han, C.; Choi, S.; Han, Y. A piezoelectric actuator-based direct-drive valve for fast motion control at high operating temperatures. Appl. Sci. 2018, 8, 1806. [Google Scholar] [CrossRef]
- Yang, Z.; He, Z.; Yang, F.; Rong, C.; Cui, X. Design and analysis of a voltage driving method for electro-hydraulic servo valve based on giant magnetostrictive actuator. Int. J. Appl. Electromagn. Mech. 2018, 57, 439–456. [Google Scholar] [CrossRef]
- Hu, G.; Zheng, K. Pressure drop and response time analysis of magnetorheological valve with mosquito-plate fluid flow channels. Trans. Chin. Soc. Agric. Mach. 2019, 50, 401–409. [Google Scholar]
- Shi, H. Magneto-mechanical behavior of magnetic shape memory alloy and its application in hydraulic valve actuator. J. Mech. Eng. 2018, 54, 235–244. [Google Scholar] [CrossRef]
- He, J.; Chen, X.; Lu, P. Theoretical analysis and experimental study on two-dimensional cartridge servo valve. Acta Aeronaut. Astronaut. Sin. 2019, 40, 282–292. [Google Scholar]
- Ren, Y.; Ruan, J. Theoretical and experimental investigations of vibration waveforms excited by an electro-hydraulic type exciter for fatigue with a two-dimensional rotary valve. Mechatronics 2016, 33, 161–172. [Google Scholar] [CrossRef]
- Li, S.; Ruan, J.; Meng, B. Two-dimensional electro-hydraulic proportional directional valve. Chin. J. Mech. Eng. 2016, 52, 202–212. [Google Scholar] [CrossRef]
- Zuo, X.; Ruan, J.; Liu, G. Characteristics of direct-acting airborne 2D electro-hydraulic pressure servo valve. Acta Aeronaut. Astronaut. Sin. 2017, 38, 421294. [Google Scholar]
- Jiang, W.; Chen, P.; Qiu, X.; Lai, Y.; Zhou, J. Research on static characteristics of a novel rotating proportional electro-mechanical converter. Chin. J. Sci. Instrum. 2018, 40, 107–114. [Google Scholar]
- Li, W. Design and Study of Large Flow 2D Electro-Hydraulic Proportional Directional Valves; Zhejiang University of Technology: Hangzhou, China, 2013; pp. 15–17. [Google Scholar]
- Zuo, Q. Principle, Theoretical Analysis and Experimental Study on Half-Bridge Pilot 2D Electro-Hydraulic Proportional Directional Valve; Zhejiang University of Technology: Hangzhou, China, 2014; pp. 16–20. [Google Scholar]
- Tian, L.; Yang, X.; Li, Y.; Tian, Q.; Li, H.; Zhang, X.W. Analytical magnetic force model for permanent magnetic Guide way and permanent magnetic bearings. Tribology 2008, 28, 73–77. [Google Scholar]
- Yonnet, J.P. Permanent magnet bearings and couplings. IEEE Trans. Magn. 1981, 17, 1169–1173. [Google Scholar] [CrossRef]
- Tian, L. Permanent Magnet Suspension Bearing and Track and Permanent Magnetic Analytical Model; Science Press: Beijing, China, 2018; pp. 92–214. [Google Scholar]
- Zhao, B.; Zhang, H.L. Application of Ansoft 12 in Engineering Electromagnetic Field; China Water & Power Press: Beijing, China, 2010; pp. 192–266. [Google Scholar]
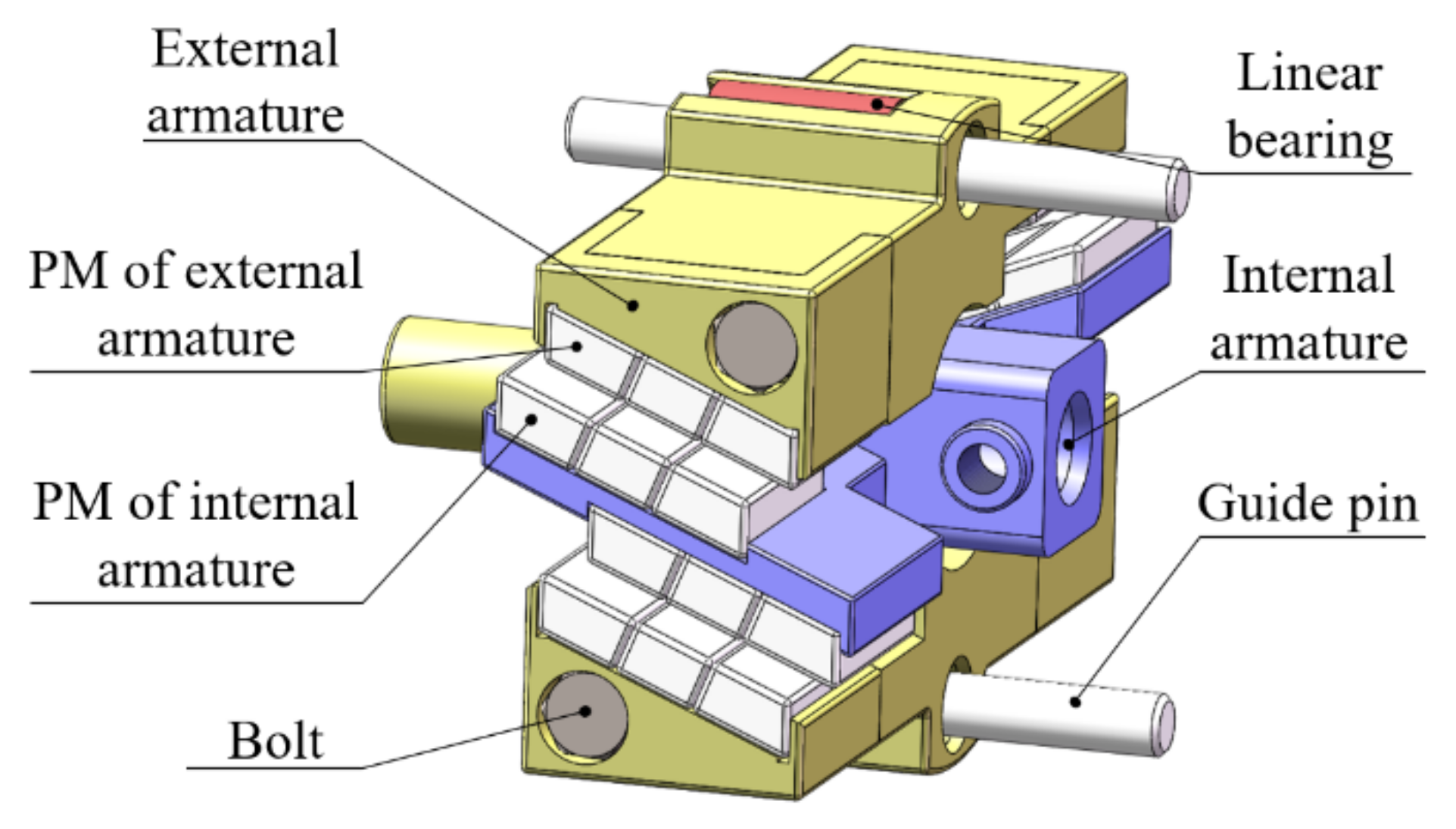
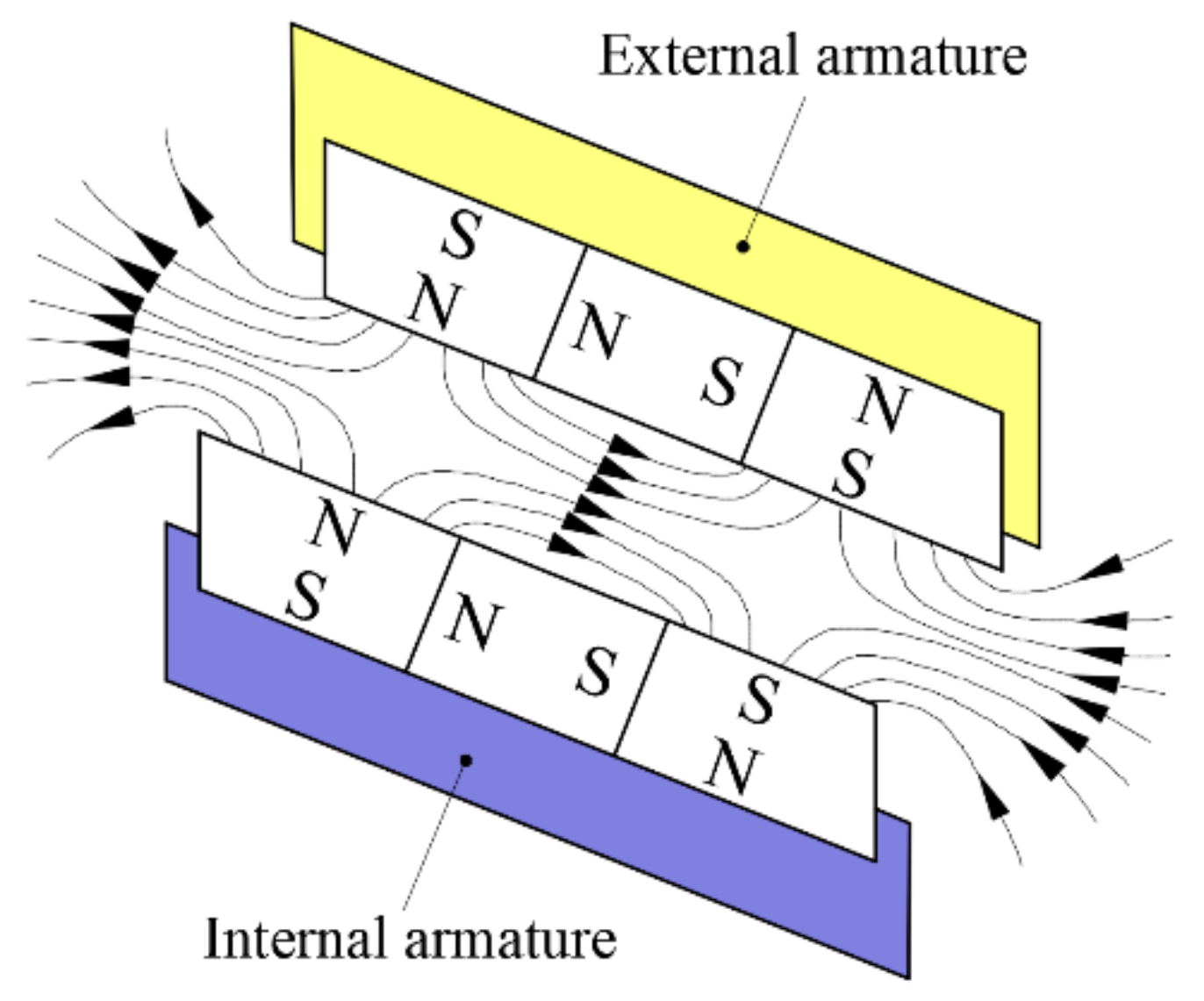
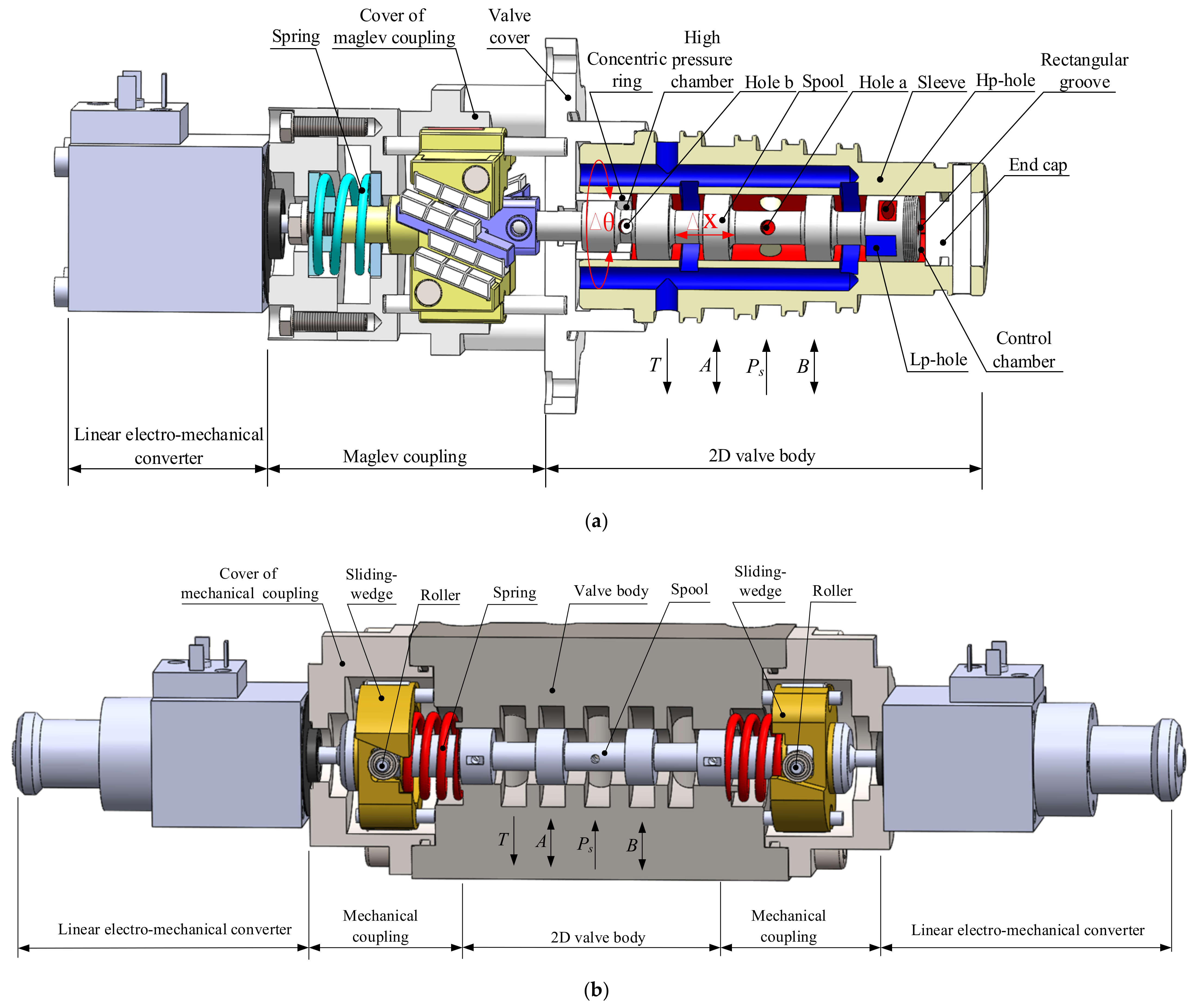




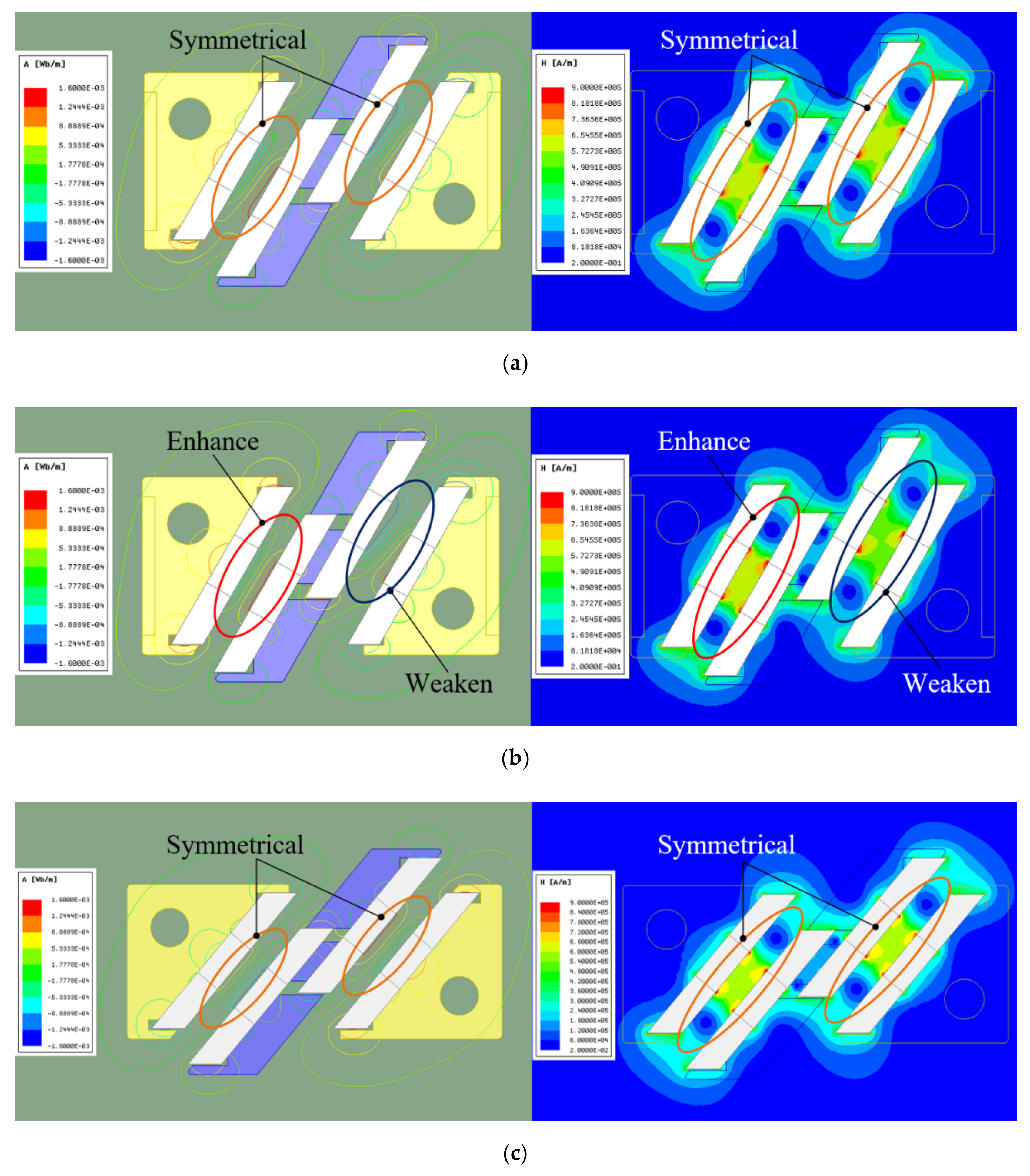


| Structure | ||||
|---|---|---|---|---|
| (a) | ||||
| (b) | ||||
| (c) | ||||
| (d) |
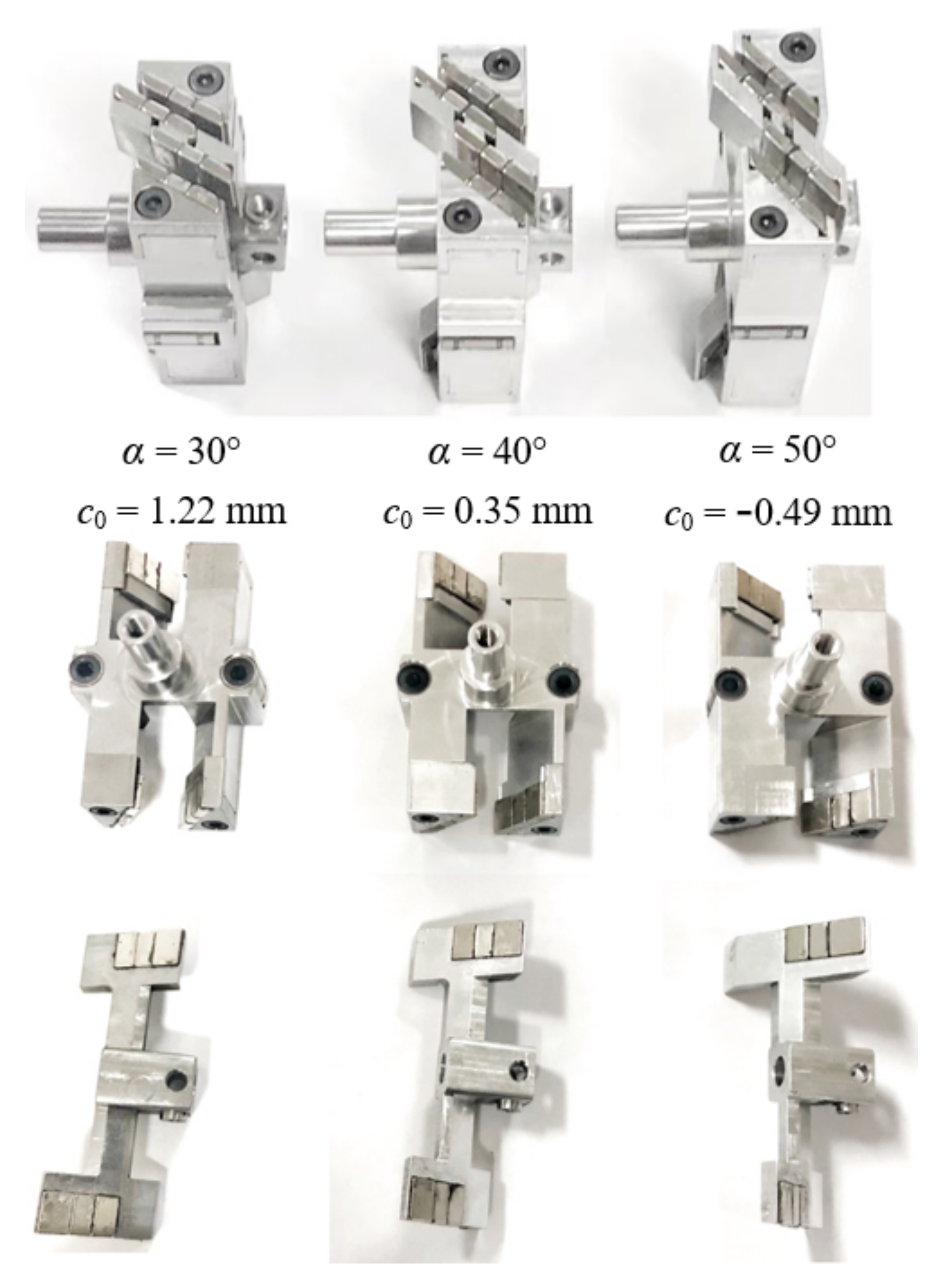
| Structure | ||||
|---|---|---|---|---|
| (a) | ||||
| (b) | ||||
| (c) | ||||
| (d) |
| Parameters | Value |
|---|---|
| /(°) | 0 to 90 |
| /(mm) | −5 to 5 |
| /(mm) | 3 |
| /(mm) | 0 to 1 |
| /(T) | 1.19 |
| Lever of force /(mm) | 35 |
| Width of PM /(mm) | 6 |
| Height of PM /(mm) | 3 |
| Length of PM /(mm) | 10 |
| Parameters | Value |
|---|---|
| Width × height × length of rectangular PM/(mm) | 6 × 3 × 10 |
| Long width × short width × height × length of 30° trapezoidal PM/(mm) | 7.73 × 6 × 3 × 10 |
| Long width × short width × height × length of 40° trapezoidal PM/(mm) | 8.52 × 6 × 3 × 10 |
| Long width × short width × height × length of 50° trapezoidal PM/(mm) | 9.58 × 6 × 3 × 10 |
| Optimal of 30° maglev coupling/(mm) | 1.22 |
| Optimal of 40° maglev coupling/(mm) | 0.35 |
| Optimal of 50° maglev coupling/(mm) | −0.49 |
| Height of air gap /(mm) | 3 |
| Residual magnetic induction intensity /(T) | 1.19 |
| Coercivity of magnet/(A/m) | 9.15 × 105 |
| Lever of force/(mm) | 35 |
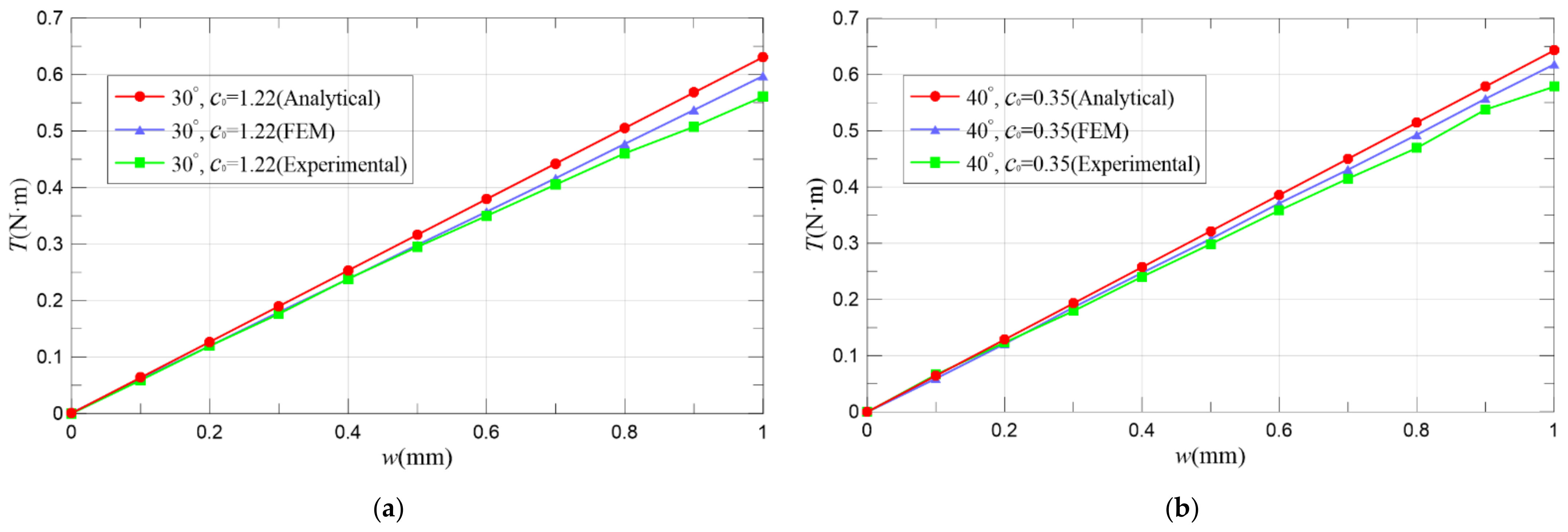
| 0.5 | 1 | ||
|---|---|---|---|
| Torque/(N·m) ( = 30°, = 1.22 mm) | Analytical | 0.316 | 0.631 |
| FEM | 0.298 | 0.597 | |
| Exp | 0.295 | 0.561 | |
| Difference | 6.65% | 11.09% | |
| Torque/(N·m) ( = 40°, = 0.35 mm) | Analytical | 0.322 | 0.643 |
| FEM | 0.308 | 0.618 | |
| Exp | 0.299 | 0.579 | |
| Difference | 7.14% | 9.95% | |
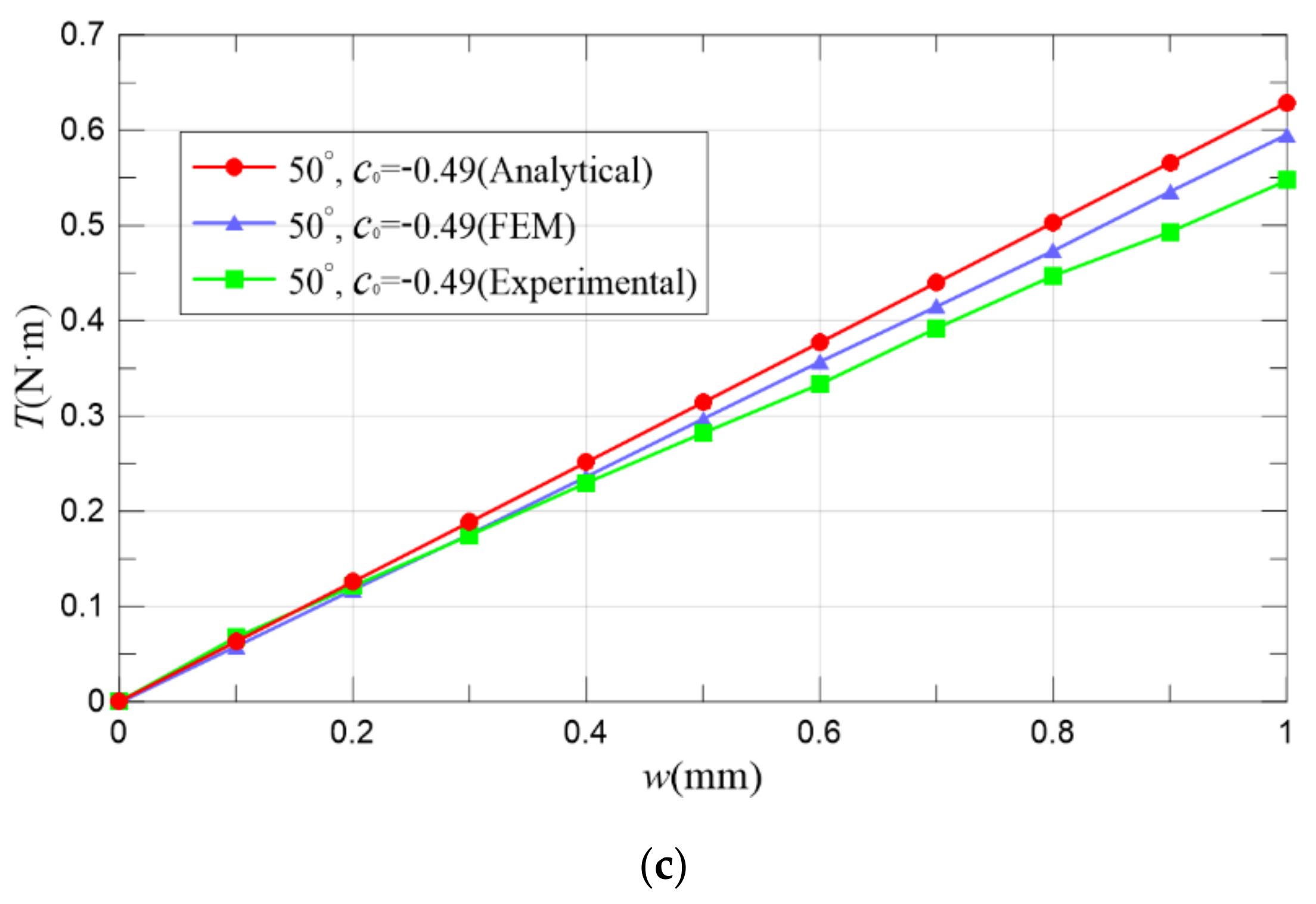
| Torque/(N·m) ( = 50°, = −0.49 mm) | Analytical | 0.314 | 0.629 |
| FEM | 0.297 | 0.595 | |
| Exp | 0.282 | 0.547 | |
| Difference | 10.19% | 13.04% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, B.; Zhu, C.; Xu, H.; Dai, M.; Li, S. Analytical and Experimental Investigations of Novel Maglev Coupling Based on Opposed Halbach Array for a 2D Valve. Actuators 2021, 10, 61. https://doi.org/10.3390/act10030061
Meng B, Zhu C, Xu H, Dai M, Li S. Analytical and Experimental Investigations of Novel Maglev Coupling Based on Opposed Halbach Array for a 2D Valve. Actuators. 2021; 10(3):61. https://doi.org/10.3390/act10030061
Chicago/Turabian StyleMeng, Bin, Chenhang Zhu, Hao Xu, Mingzhu Dai, and Sheng Li. 2021. "Analytical and Experimental Investigations of Novel Maglev Coupling Based on Opposed Halbach Array for a 2D Valve" Actuators 10, no. 3: 61. https://doi.org/10.3390/act10030061
APA StyleMeng, B., Zhu, C., Xu, H., Dai, M., & Li, S. (2021). Analytical and Experimental Investigations of Novel Maglev Coupling Based on Opposed Halbach Array for a 2D Valve. Actuators, 10(3), 61. https://doi.org/10.3390/act10030061






