A Low-Power High-Efficiency Adaptive Energy Harvesting Circuit for Broadband Piezoelectric Vibration Energy Harvester

Abstract

Existing piezoelectric vibration energy harvesting circuits require auxiliary power for the switch control module and are difficult to adapt to broadband piezoelectric vibration energy harvesters. This paper proposes a self-powered and low-power enhanced double synchronized switch harvesting (EDSSH) circuit. The proposed circuit consists of a low-power follow-up switch control circuit, reverse feedback blocking-up circuit, synchronous electric charge extraction circuit and buck-boost circuit. The EDSSH circuit can automatically adapt to the sinusoidal voltage signal with the frequency of 1 to 312.5 Hz that is output by the piezoelectric vibration energy harvester. The switch control circuit of the EDSSH circuit works intermittently for a very short time near the power extreme point and consumes a low amount of electric energy. The reverse feedback blocking-up circuit of the EDSSH circuit can keep the transmission efficiency at the optimal value. By using a charging capacitor of 1 mF, the charging efficiency of the proposed EDSSH circuit is 1.51 times that of the DSSH circuit.

1. Introduction

The self-powered wireless sensor which can automatically obtain electric energy from ambient vibration has great application prospects in the field of structural health detection [1,2,3]. It is important to develop an efficient self-powered system which can automatically convert environmental vibration energy into electric energy for the application of wireless sensors. Piezoelectric smart structures have been widely studied in the field of ambient vibration energy harvesting because of their sensitivity to vibration, high energy density [4,5] and high output voltage [6,7]. The research on piezoelectric vibration energy harvesting systems mainly includes three aspects: the modeling theory and method, the mechanical structure optimization design and the energy harvesting interface circuit.

Researchers have deeply investigated the modeling theory and methods of piezoelectric vibrators. Some analytical models are already available in the literature. Erturk and Inman [8,9] established an analytical distributed parameter model for cantilevered piezoelectric energy harvesters. Liao and Sodano [10,11] developed a theoretical model of a piezoelectric energy harvesting system to predict the power around a single vibration mode based on the Rayleigh–Ritz approach. Fakharian and Salmani [12] researched a lumped parameter model for an exponentially tapered piezoelectric beam in transverse vibration. Kärki [13], Baishya [14], Wang [15], Sriramdas [16] and Rammohan [17] studied a lumped-parameter transducer model for piezoelectric and ferroelectric polymers. Elvin [18,19] proposed a general equivalent circuit model for piezoelectric generators and a coupled finite element-circuit simulation model for analyzing a piezoelectric energy generator. Roundy [20] established a distributed parameter model for improving power output for vibration-based energy scavengers. Yang and Tang [21] established an equivalent circuit modeling of piezoelectric energy harvesters. Wang [22], Zhang [23], Ko [24] and Saleem [25] investigated modeling and parameter identification for equivalent circuit modeling of piezoelectric energy harvesters with theoretical analysis. Noël [26], He [27], Wang [28], Xie [29] and Billings [30] put forward a method of modeling and parameter identification for the circuit model of a nonlinear piezoelectric vibrator.

Several methods have been proposed by researchers to improve the energy conversion efficiency and expand the band of the operation frequency of VEHs. One of the approaches is frequency tuning, such as mechanical tuning [31,32,33,34,35], circuit tuning and magnetic tuning [36,37,38,39]. This strategy allows VEHs to adjust their resonant frequency according to the environmental vibration frequency. However, this increases the complexity or consume extra energy of the VEHs. For instance, Eichhorn [31] proposed a piezoelectric energy harvesting structure of a cantilever beam with additional mechanical mass, which changes the resonant frequency of the structure by applying pressure or tensile force to the cantilever beam. Fan [39] designed a vibration energy harvester that composed of a piezoelectric harvester, stopper and magnet. The system can tune the vibration energy harvester to a lower working frequency range by changing the gap between mass and magnets, so as to improve the efficiency of energy collection. Another approach is to use multi-modal energy capture technology with different resonant frequencies, including generator arrays [40,41,42,43] and coupled vibration [44,45,46,47,48,49]. Although coupled vibration is easy to achieve, the maximum output power of the VEH is consequently reduced. Nabavi [40] proposed a piezoelectric MEMS harvester with symmetrical and double clamping structure, which has multi-mode and nonlinear characteristics and effectively broadens the working bandwidth. Hu [44] proposed a broadband multi mass and multi spring piezoelectric vibration energy harvester based on a folded asymmetric gap cantilever beam, which can make each stage of pure bending form multiple resonance modes. The working frequency band of the structure is widened effectively. Recently, nonlinear vibration energy harvesting approaches, for instance, nonlinear magnetic coupling [50,51,52,53,54,55,56] and piecewise-linear structure [57,58,59,60], have attracted the interest of researchers. Although energy conversion efficiency of the unit volume material is decreased, the operation frequency band of the VEH could be widened.

There are special requirements for piezoelectric energy harvesters (PEHs) and their electric energy harvesting circuits due to the wide range of vibration frequency and large load range of actual working environment. Therefore, a low-power high-efficiency adaptive energy harvesting circuit which can match the PEH with wide operation frequency band and load range is the key to the practical application of the piezoelectric system.

The standard energy harvesting (SEH) [61,62] interface circuit was first reported as a power harvesting circuit of PEHs for its simple structures and needless of external control module, but the energy harvesting efficiency is low and obviously affected by load. Lefeuvre [63] proposed a two-stage interface circuit of the down-to-step voltage converter, which has low power and can charge a 4.8 V rechargeable battery efficiently. Garbuio and Guyomar [64,65,66] investigated the synchronized switch harvesting on inductor (SSHI) technique for vibration control of piezoelectric structures, which adds synchronous switching damping at both ends of piezoelectric elements to improve the efficiency of energy harvesting. The SSHI scheme uses an LC resonant circuit for capacitor charging to reduce energy loss. Liang and Badel [67,68] proposed a synchronous switch damping technology by adding series inductors in the circuit, in which the synchronous switch is used to quickly flip the piezoelectric voltage and improve the energy conversion efficiency. Shu, Ramadass, Lefeurve and Lallart [69,70,71,72] developed the Seris-SSHI (S-SSHI) and the parallel-SSHI (P-SSHI) technologies further, but the circuit scheme requires impedance matching to achieve maximum power transmission and can only match the load in a narrow range. Lefeuvre [73], Gasnier [74] and Dini [75] studied the SSHI technology and proposed synchronous electric charge extraction (SECE) technology. The energy harvesting efficiency of the SECE scheme is independent of the load, and the range of matching loads is wide for the use of the LC resonant circuit to transfer the power of the PEH to the inductor and then to the load through the DC-DC converter in the SECE scheme.

Recently, researchers proposed a class of double synchronized switch harvesting (DSSH) [76,77,78] circuits as shown in Figure 1 by optimizing the control of switches of SECE to make the circuit more efficient. Wang [79] analyzed a self-powered, ultra-low-power control circuit for weak coupling and heavy load. Wu [80] used two resonant rings to flip the capacitor voltage in each half cycle to improve the power harvesting efficiency of an SSHI circuit. Din [81] designed an energy harvesting circuit based on MOS devices to reduce power consumption. Chen [82] reported a fast self-starting and low-power management circuit for a micro piezoelectric energy harvester. Lallart [83] studied a nonlinear technology and self-powered circuit to harvest energy from PEHs under no-load conditions efficiently. The main shortcomings of the DSSH circuit are the complexity of the switch control, the need for an additional switch control circuit and auxiliary power supply, and the low conversion efficiency due to the inaccurate switch time control. To the best of our knowledge, existing solutions have either low energy harvesting efficiency such as the SEH circuit [61,62], are unable to sufficiently match loads such as P-SSHI and S-SSHI circuits [64,65,66,73,74,75] or are excessively complicated and require auxiliary power for the switch control circuit [76,77,78].

This paper proposes a low-power high-efficiency adaptive double synchronized switch harvesting circuit for broadband PEHs based on DSSH called an enhanced double synchronized switch harvesting (EDSSH) circuit. The schematic diagram is shown in Figure 2. The EDSSH circuit has four advantages. First, compared with DSSH, the EDSSH circuit simplifies the switching control circuit, reduces the power consumption of the switching control circuit and does not need a special auxiliary power supply. Moreover, in order to reduce the power consumption, the switch control circuit is in the sleep state most of the time and starts automatically only for a short time near the power extreme point. Secondly, the EDSSH circuit adds a power reverse feedback blocking-up circuit (RFBC) into DSSH to keep the energy harvesting efficiency optimal and avoid the problem of unstable energy harvesting efficiency of the DSSH circuit. Thirdly, the EDSSH circuit inherits the power extreme value tracking point technology of DSSH, which has stronger energy harvesting efficiency than SEH and SSHI circuits. Lastly, it can automatically adapt to input voltage signals with a wide frequency range.

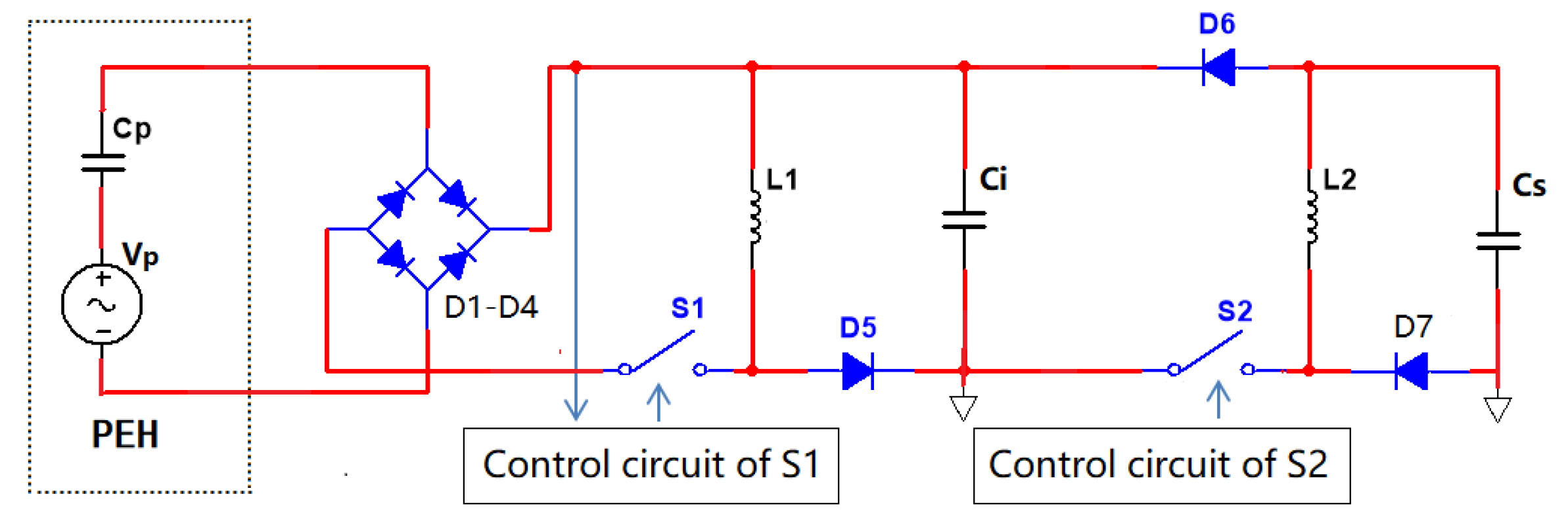
2. The Proposed EDSSH Circuit

2.1. Structure of EDSSH Circuit

The proposed EDSSH circuit is shown in Figure 2. The simulation circuit according to the schematic diagram of the EDSSH circuit is shown in Figure 3. The circuit includes a PEH, a rectifier bridge, an energy transfer circuit composed of S₁-L₁-D₅-C_i-S₂-D₆, a buck-boost circuit, the control circuit of switch S₁ and the control circuit of switch S₂. The PEH part is represented by an AC voltage source V_P (12.26 V, 50 Hz) and a capacitance C_P (62.36 nF).

The EDSSH circuit consists of four working circuits:

(1)

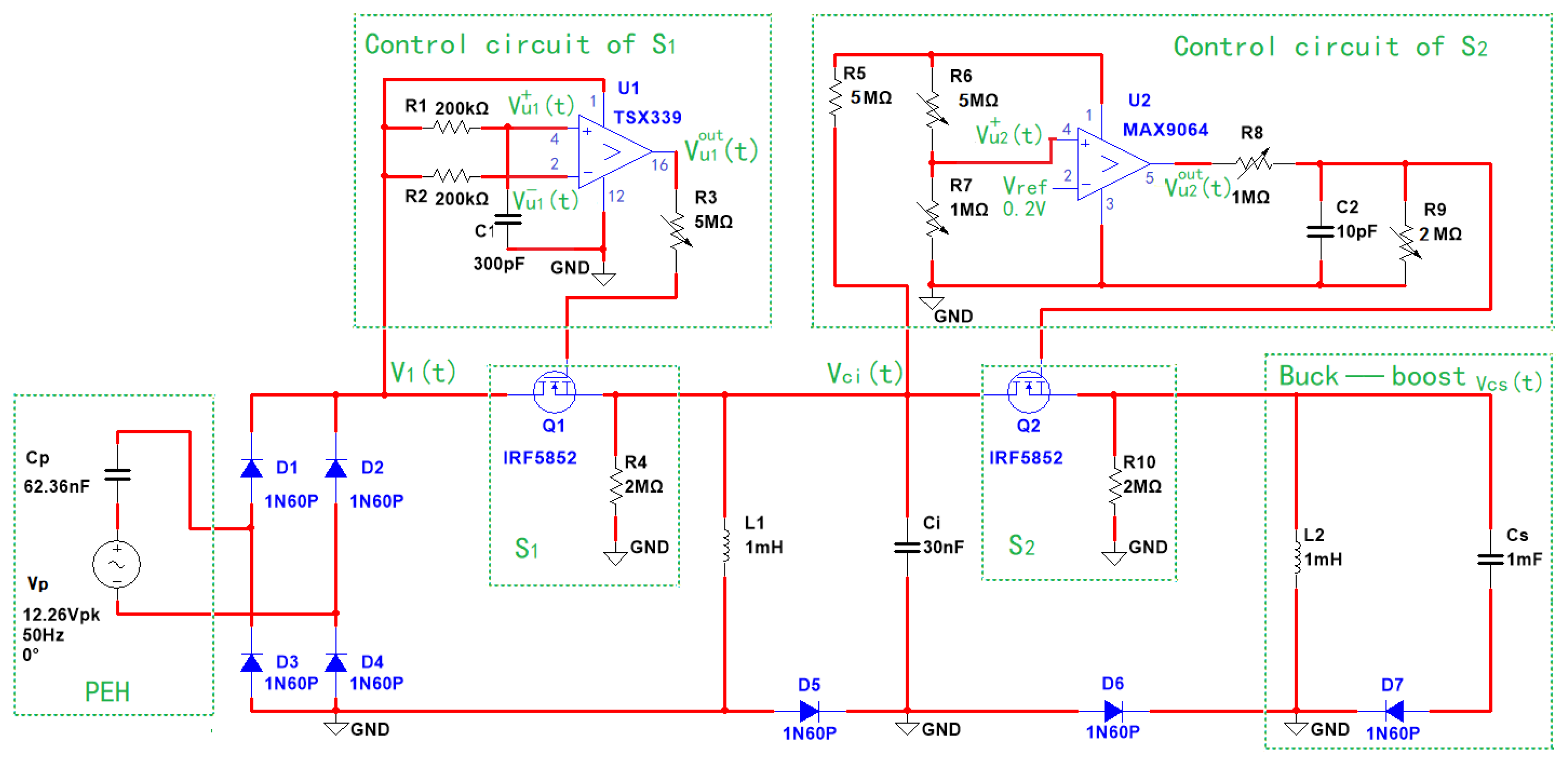
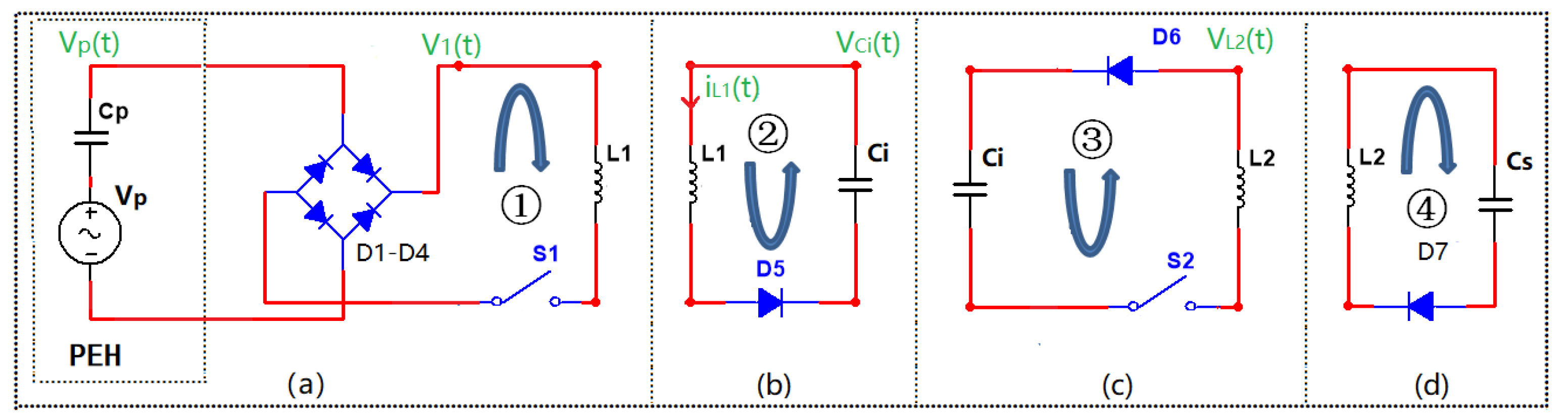
The first circuit loop is composed of the capacitor C_P, rectifier bridge D₁-D₄, inductor L₁, ultra-low on-resistance MOSFET Q₁ (representative switch S₁) and control circuit of S₁. This circuit is used to harvest the electric energy generated by the PEH. Compared with DSSH, the EDSSH circuit has three improvements. First, the EDSSH circuit simplifies the switching control circuit and uses a simple structure and low-power IC, a comparator TSX339, three resistors and one capacitor. The control circuit of S₁ is composed of the micro-power quad CMOS voltage comparator TSX339 and its peripheral circuit. The no-inverting input terminal of TSX339 is connected to $V_1\left(t\right)$ through the RC signal delay circuit (delay time T_C = R₁∗C₁). The inverting input terminal of TSX339 is connected to $V_1\left(t\right)$ through the current limiting resistor R₂. The terminal V_cc is connected to $V_1\left(t\right)$ directly. The ground terminal of TSX339 is grounded. The output terminal of TSX339 is connected to the gate terminal of Q₁ through an adjustable resistor R₃. Secondly, other passive components are also low power consumption. The rectifier bridge D₁-D₄ is composed of four ultra-low-power IN60P diodes with forward voltage of 0.24 V, reverse voltage of 40 V and maximum forward current of 50 mA. The controlled switch S₁ is represented by N-channel MOSFET IRF5852 with ultra-low static drain-to-source on-resistance $\left(R_{DS\left(on\right)}\right)$ of $0.09\mathsf{\Omega }$, switch frequency exceeding 10⁸/s and minimum drain-to-source breakdown voltage ($V_{BR\left(DSS\right)}$) of 20 V. Thirdly, the control circuit of S₁ with low power and low-cold-start threshold does not need additional power supply but is supplied by the output voltage $V_1\left(t\right)$ of the rectifier bridge. The control circuit of S₁ can automatically control S₁ turn on for a brief time when the voltage of $V_1\left(t\right)$ reaches the extreme value and consumes very little power. That is, TSX339 compares the voltage of the no-inverting input terminal and inverting input terminal in real time. When the voltage of the no-inverting input terminal is higher than that of the inverting input terminal at the peak value of $V_1\left(t\right)$, $V_1\left(t\right)$ is output directly through the output terminal of TSX339 to control Q₁ turn on for a short time. When the output voltage ($V_1\left(t\right)$) drops from a high value to the drain-source on-voltage of IRF5852, Q₁ turns off automatically. During this short time, electric energy is transferred from C_p to L₁.

(2)

The second circuit loop is composed of inductor L₁, capacitor C_i and diode D₅. Compared with DSSH, the EDSSH circuit sets a low-power diode D₅ in this circuit loop, which together with C_i and L₁ forms the reverse feedback blocking-up circuit (RFBC). It avoids the unstable power transfer efficiency of DSSH caused by L₁-C_i oscillation and ensures that power is only transferred from inductor L₁ to the capacitor C_i in one way.

(3)

The capacitor C_i, inductor L₂, diode D₆, ultra-low on-resistance MOSFET Q₂ (representative switch S₂) and the control circuit of S₂ constitute the third circuit loop. Compared with DSSH, the EDSSH circuit has three improvements. First, the EDSSH circuit simplifies the switching control circuit and uses a simple structure and low-power IC, a comparator MAX9064, five resistors and one capacitor. The control circuit of S₂ is composed of the low-power comparator MAX9064 and its peripheral circuit. The inverting input terminal does not need a reference voltage source due to its internal V_ref (0.2 V). Because the range of V_CC of MAX9064 is 0.9 to 5.5 V, but $V_{Ci}\left(t\right)$ reaches 14 V, $V_{Ci}\left(t\right)$ is connected to the no-inverting input of MAX9064 after depressurization through series resistance R₅, R₆ and R₇. Secondly, other passive components also have low power consumption, such as ultra-low on-resistance MOSFET Q₂ and diode D₆ (IN60P). Thirdly, the control circuit of switch S₂ with low power and does not need an additional power supply but is supplied by the output voltage $V_{Ci}\left(t\right)$, and it can control S₂ turn on when the voltage of $V_{Ci}\left(t\right)$ reaches the maximum after a brief time, and S₂ turns off when the current in the inductor L₂ reaches the maximum. That is, when the $V_{Ci}\left(t\right)$ reaches the maximum (at this time, the no-inverting input voltage of MAX9064 just exceeds its V_ref (0.2 V)), MAX9064 outputs high voltage for a short time (${\mathrm{t}}_{S2on}$) and controls S₂ turn on. The ${\mathrm{t}}_{S2on}$ is determined by the discharge time of the RC discharge circuit composed of R₉ and C₂, and it must be equal to one fourth of the oscillation period of the LC oscillation circuit composed of L₂ and C_i to ensure that the electric energy of C_i is transmitted to L₂ in one way automatically.

(4)

The inductor L₂, diode D₇ and capacitor C_S constitute the buck-boost circuit, which is used to charge C_S. It inherits from DSSH and can be used to broaden the load matching ability of the circuit.

2.2. Working Process and Control Strategy of EDSSH Circuit

Assume that the piezoelectric structure resonates with the sinusoidal excitation of frequency $\left(\omega \right)$, and the corresponding vibration period is $T_P$. The working process of the circuit is shown in Figure 4. The waveform of nodes of the EDSSH circuit in one power harvesting cycle is shown in Figure 5.

Take a vibration period in resonance as an example to illustrate the operation process and control strategy of the circuit. (1) The first stage (Figure 4a, 0–t₁ of Figure 5a): In the initial stage of system vibration, the switches S₁ and S₂ are turned off, and the PEH is in the vibration state without load coupling. Voltage is generated across the PEH due to structural bending. When the vibration amplitude and the voltage of the PEH ($V_p\left(t\right)$) reach the maximum at t₀ of the positive half period, the control circuit of S₁ controls S₁ turn on automatically until it detects the maximum of $V_1\left(t\right)$. The electric energy of the PEH is transferred from the C_p to L₁ in one way in the C_p-L₁ loop at this time. The electric energy transfer is complete when $V_1\left(t\right)$ drops to near zero; at this time the control circuit of S₁ turns off S₁ automatically. (2) The second stage (Figure 4b, t₁–t₂ of Figure 5a): L₁ transfers electric energy to C_i automatically due to LC oscillation. Due to the built-in diode D₅ in the L₁-C_i loop, the electric energy of L₁ can only be transferred from L₁ to C_i in one direction, and the loop does not have reverse feedback from C_i to L₁ caused by multiple LC oscillations. (3) The third stage (Figure 4c, t₂–t₃ of Figure 5a): The control circuit of S₂ controls S₂ turn on when the voltage of $V_{Ci}\left(t\right)$ reaches the maximum for a brief time, and S₂ turns off when the current in the inductor L₂ reaches the maximum. C_i transfers electric energy to L₂ automatically due to LC oscillation during this time. (4) The fourth stage (Figure 4d, t₃–t₄ of Figure 5a): Electric energy is stored on C_S of the buck-boost circuit.

3. Circuit Modeling

In the case of resonance of the PEH, suppose that the frequency of $V_P \left(t\right)$ is $\omega$ and the period is $T_p$. In $T_p$ time, the circuit harvests electric energy from PEH twice in a vibration cycle. For the convenience of introduction, the energy conversion relationship of the circuit in an energy extraction cycle is modeled.

3.1. The C_p-L₁ Loop

The main circuit of the Cp-L₁ loop. Combined with the research of Zhang [84], suppose the excitation signal is $f\left(t\right)=-F_{0}cos\left(\omega t\right)$, where $F_0$ and $\omega$ are the amplitude and frequency of the signal, respectively. The end displacement of the piezoelectric beam structure is $u\left(t\right)=-U_{P}cos\left(\omega t+\phi \right)$, where $U_P$ is the amplitude of the displacement signal and $\phi$ is the phase angle between $u\left(t\right)\mathrm{and}f\left(t\right)$. In the case of without circuit coupling, the voltage across the PEH is $V_P\left(t\right)=V_P\left[1-\mathit{cos}\left(\omega t+\phi \right)\right]$, where $V_P=\chi U_P/C_P$ is the voltage across the PEH when it reaches the extreme value of displacement, χ is the electromechanical coupling coefficient and $C_P$ is the piezoelectric clamping capacitance. There is an energy transfer in half a vibration period, and the electric energy generated by the PEH is $E_P=\frac{1}{2}{C}_P{V}_P^2$. Electric energy consumed by the rectifier bridge in this period is $E_{D1-4}=2C_P{V}_P{V}_d$, where $V_d$ is the forward voltage drop of a diode.

Regarding the power consumption of the control circuit of S₁$(E_{TSX})$, the control circuit of S₁ is dormant most of the time and does not consume electric energy. It only works for a very short time ($t_{S1on}$) when $V_P\left(t\right)$ reaches the peak. The $t_{S1on}$ is equal to 1/4 of the period ($T_{Cp-L1}=2\pi \sqrt{C_P{L}_1}$) of the LC oscillation circuit composed of C_P and L₁. The power loss of the control circuit of S₁ consists of four parts: (1) The power loss of input terminal of TSX339 ($E_{TSX-i}$) is mainly the electric energy consumption on resistor R₁ and R₂. The maximum power supply of TSX 339 is 18 V, the maximum input voltage is 16 V, the typical value of input resistance is $R_{TSXi}={10}^{12}\mathsf{\Omega }$ and the typical value of the current at the forward input terminal and reverse input terminal is $I_{TSXi}=1pA$. Therefore, the input terminal electrical power consumption of TSX339 is $E_{TSX-i}=\frac{1}{4}{T}_{Cp-L1}{I}_{TSXi}^2(R_1+R_2)$. (2) The power loss of output terminal of TSX339 ($E_{TSX-o}$) is mainly the electric energy consumption on resistor R₃ and R₄. Since the gating voltage of IRF5852 is 5.5 V and the voltage amplitude of $V_1\left(t\right)$ is 12.26 V, the resistance of variable resistor R₃ was set as 2.46 $\mathrm{M}\mathsf{\Omega }$. At this time the current flowing through R₃ and R₄ is $I_{TSXo}=V_1\left(t\right)/\left(R_3+R_4\right)$, and the power loss is $E_{TSX-o}={\int }_0^{t_{S1on}}{R}_3\ast I_{TSXo}^{2}dt+{\int }_0^{t_{S1on}}{R}_4\ast I_{TSXo}^{2}dt$ $={\int }_0^{t_{S1on}}{V}_1\left(t\right)I_{TSXo}dt$. (3) The power consumption of TSX339 itself ($E_{TSX-s}$): According to the electrical characteristics of TSX339, the typical current of its single comparator is 5µA, so the consumed electric energy is $E_{TSX-s}={\int }_0^{t_{S1on}}{V}_1\left(t\right)\ast 5\ast {10}^{-6}dt$. (4) The power loss on IRF5852 ($E_{TSX-Q1}$): The typical on-resistance of IRF5852 is 0.09 $\mathsf{\Omega }$, so the power loss on IRF5852 is $E_{TSX-Q1}={\int }_0^{t_{S1on}}0.09i_{L1}^2\left(t\right)dt$. Thus, the power consumption of the control circuit of S₁ is $E_{TSX}=E_{TSX-i}+E_{TSX-o}+E_{TSX-s}+E_{TSX-Q1}$.

Let the energy conversion coefficient of C_p-L₁ circuit loop be ${\gamma }_1$, then the electric energy transferred to the inductor L₁ at this time is:

$$\begin{array}{ll}\Delta E_{L1}& =\left({\gamma }_1{E}_P-E_{D1-4}\right)-E_{TSX}\\ & =C_P{V}_P\left(\frac{1}{2}{\gamma }_1{V}_P-2V_d\right)-E_{TSX}=\chi U_P\left(\frac{{\gamma }_1\chi U_P}{2C_P}-2V_d\right)-E_{TSX}\end{array}$$

(1)

3.2. The L₁-C_i Circuit

Only one charge occurs on C_i in a half cycle due to the influence of reverse feedback blocking-up caused by diode D₅. Assuming that the capacitance value of C_i is $C_i$, the initial voltage value of C_i is $V_{ci}$, and the voltage increase value of C_i after charging is $\Delta V_{Ci}$ because the positive pole of the negative capacitance C_i is grounded, so $C_i<0$, $V_{ci}<0$ and $\Delta V_{Ci}<0$, and the increased value of electric energy of C_i after the charging is completed is:

$$\Delta E_{Ci}=\frac{1}{2}{C}_i{\left(V_{Ci}+\Delta V_{Ci}\right)}^2-\frac{1}{2}{C}_i{V}_{Ci}{}^2=\frac{1}{2}{C}_i\Delta V_{Ci}{}^2+C_i{V}_{Ci}\Delta V_{Ci}$$

(2)

The charging current only flows through the diode D₅ once in half a cycle, so the energy consumed by the diode D₅ in this process is $E_{D5}={\int }_a^b{V}_d\ast i_{Ci}\left(t\right)dt=-{\int }_a^b{V}_d\ast C_{i}d{V}_{Ci}\left(t\right)=-C_i{V}_d\Delta V_{Ci}$, where a and b are the starting and ending time of charging of capacitor C_i, respectively. Assume that the efficiency of electric energy transfer of the loop is ${\gamma }_2$. According to the law of conservation of energy, we can obtain $\Delta E_{Ci}={\gamma }_2\Delta E_{L1}-E_{D5}$, and it can also be expressed in this way:

$$C_i\Delta V_{Ci}{}^2+2C_i\left(V_{Ci}-V_d\right)\Delta V_{Ci}-2{\mathsf{\gamma }}_2\Delta E_{L1}=0$$

(3)

By solving Equation (3), we can obtain $\Delta V_{Ci}$ as follows:

$$\Delta V_{Ci}=\sqrt{{\left(V_d-V_{Ci}\right)}^2+\frac{2{\mathsf{\gamma }}_2\Delta E_{L1}}{C_i}}+\left(V_d-V_{Ci}\right)$$

(4)

It can be seen from Formula (4) that $\Delta V_{Ci}$ is only related to $U_P$ when parameters of the circuit are determined because $\Delta E_{Ci}$ is only related to $\Delta V_{Ci}$, and $\Delta E_{Ci}$ is only related to $U_P$. According to Tang [32], the expression of $U_P$ is as follows:

$$U_P=\frac{F_0}{\sqrt{{\left(\eta \omega +\frac{4{\chi }^2}{\pi C_P}\right)}^2+{\left(K-M{\omega }^2+\frac{{\chi }^2}{C_P}\right)}^2}}$$

(5)

where$M$, $K$ and $\eta$ are the modal mass, modal stiffness and modal damping ratio of the PEH, respectively. By substituting Formulas (4) and (5) into Formula (2), we can obtain $\Delta E_{Ci}$.

3.3. The C_i-L₂ Loop

Regarding the power consumption of the control circuit of S₂$(E_{MAX})$, the control circuit of S₂ is dormant most of the time and does not consume electric energy. It only works for a very short time ($t_{S2on}$) when $V_{Ci}\left(t\right)$ reaches the peak. The $t_{S2on}$ is equal to 1/4 of the period ($T_{Ci-L2}=2\pi \sqrt{C_i{L}_2}$) of the LC oscillation circuit composed of C_i and L₂. The power loss of the control circuit of S₂ consists of four parts: (1) The power loss of the input terminal of MAX9064 ($E_{MAX-i}$) is mainly the electric energy consumption on resistors R₅, R₆ and R₇. The maximum voltage of $V_{Ci}\left(t\right)$ is 13.87 V. The input voltage range of MAX9064 is 0 to 5.5 V. In order to make the supply voltage and forward input voltage of MAX9064 reach 5.5 and 0.2 V, respectively, when $V_{Ci}\left(t\right)$ reaches 13.87 V, the resistance of R₅, R₆ and R₇ was set as $0.1,2.65\mathrm{and}4.18\mathrm{M}\mathsf{\Omega },$ respectively. At this time the current flowing through R₃ and R₄ is $I_{MAXi}=V_{Ci}\left(t\right)/\left(R_5+R_6+R_7\right)$. Therefore, the input terminal electrical power consumption of MAX9064 is $E_{MAX-i}={\int }_0^{t_{S2on}}\left(R_5+R_6+R_7\right)I_{MAXi}^{2}dt$. (2) The power loss of output terminal of MAX9064 ($E_{MAX-o}$) is mainly the electric energy consumption on resistors R₈, R₉ and R₁₀. To ensure that the electric energy of C_i is just transferred to L₂, the conduction time of switch S₂ must be just equal to $\frac{1}{4}{T}_{Ci-L2}$; that is, the discharge time $T_{C2-R9}$ of the discharge circuit composed of C₂ and R₉ is equal to $\frac{1}{4}{T}_{Ci-L2}$. According to this, R₈, R₉ and R₁₀ were set as $0.1,1\mathrm{and}2\mathrm{M}\mathsf{\Omega },$ respectively. Therefore, the power loss on R₈ and R₉ is $E_{R8}=\frac{1}{4}{T}_{Ci-L2}\ast R_8\ast {\left(\frac{5.5}{R_8+R_9}\right)}^2$ and $E_{R9}=\frac{1}{4}{T}_{Ci-L2}\ast R_9\ast {\left(\frac{5.5}{R_8+R_9}\right)}^2$, respectively, and the power loss on R₁₀ is equal to the electric energy on C₂ ($E_{C2}=\frac{1}{2}{C}_2\ast {\left(5.5\right)}^2$). Thus, $E_{MAX-o}=E_{R8}+E_{R9}+E_{C2}$. (3) The power consumption of MAX9064 itself ($E_{MAX-s}$): According to the electrical characteristics of MAX9064, the maximum value of operating current is 100 nA, so the consumed electric energy is $E_{MAX-s}={\int }_0^{t_{S2on}}{V}_{Ci}\left(t\right)\ast 0.1\ast {10}^{-6}dt$. (4) The power loss on IRF5852 ($E_{MAX-Q2}$): The typical on-resistance of IRF5852 is 0.09 $\mathsf{\Omega }$, so the power loss on IRF5852 is $E_{MAX-Q2}={\int }_0^{t_{S2on}}0.09i_{L2}^2\left(t\right)dt$. Therefore, the power consumption of the control circuit of S₁ is $E_{MAX}=E_{MAX-i}+E_{MAX-o}+E_{MAX-s}+E_{MAX-Q2}$.

Assuming that the residual electric energy of C_i is zero when the energy transfer is completed, since there is only one discharge process on C_i during this time, the electric energy consumed on D₆ is:

$$E_{D6}={\int }_a^b{V}_d\ast i_{L2}{C}_{Ci}\Delta V_{Ci}{}^2\left(t\right)dt=C_i{V}_d\Delta V_i$$

(6)

Suppose that the efficiency of electric energy transfer of the circuit is ${\gamma }_3$, then the electric energy transferred to L₂ is:

$$\Delta E_{L2}={\gamma }_3\Delta E_{Ci}-E_{D6}-E_{MAX}$$

(7)

3.4. The Buck-Boost Circuit

Let the capacitance, the initial voltage and the increased voltage after a single energy conversion of C_S be $C_S$, $V_{Cs0}$ and $\Delta V_{Cs}$, respectively. Suppose that the power transfer efficiency of the circuit is ${\gamma }_4$, then according to the law of conservation of energy, we have $\Delta E_{Cs}={\gamma }_4\Delta E_{L2}-E_{D7}$, and it can also be expressed as:

$$C_S\Delta V_{Cs}{}^2+2C_S\left(V_{Cs0}+V_d\right)\Delta V_{Cs}-2{\gamma }_4\Delta E_{L2}=0$$

(8)

By solving Formula (8), we can obtain $\Delta V_{Cs}$ as follows:

$$\Delta V_{Cs}=\sqrt{{\left(V_d+V_{Cs0}\right)}^2+\frac{2{\gamma }_4\Delta E_{L2}}{C_s}}-\left(V_d+V_{Cs0}\right)$$

(9)

We can obtain $\Delta V_{Cs}$ by substituting Formula (7) into Formula (9). Then the electric energy increment on the charging capacitor Cs can be obtained as follows:

$$\Delta E_{Cs}=\frac{1}{2}{C}_s{\left(V_{Cs}+\Delta V_{Cs}\right)}^2-\frac{1}{2}{C}_s{V}_{Cs}{}^2=\frac{1}{2}{C}_s\Delta V_{Cs}{}^2+C_s{V}_{Cs0}\Delta V_{Cs}.$$

(10)

According to the discussion above, the models of key parameters reflecting circuit performance are summarized as follows:

• Voltage on C_S (charging voltage of the circuit)

$$V_{Cs}={V_{Cs}}^{-}+\Delta V_{Cs}$$

(11)

2.

Electrical power of C_S (electric energy harvesting power of the circuit)

$$P_{Cs}=\frac{\Delta E_{Cs}}{\Delta t}=\frac{2\Delta E_{Cs}}{T_P}=\frac{C_s\Delta V_{Cs}{}^2+2C_s{V}_{Cs0}\Delta V_{Cs}}{T_P}$$

(12)

3.

Power Budget

The calculation formula of average power is $\overline{P}=\frac{E}{T}.$ During an energy extraction cycle with a duration of $\frac{1}{2}{T}_P$, the electric power generated by the piezoelectric vibrator is:

$${\overline{P}}_{in}=E_P/\left(0.5T_P\right)$$

(13)

The electric power losses of D₁–D₇, R₁–R₁₀, TSX339, MAX9064, Q₁ and Q₂ during an energy extraction cycle with a duration of $\frac{1}{2}{T}_P$ are shown in

Table 1. Power budget.

Object	Power Loss	Unit
D1 + D2 + D3 + D4	$\left(2C_P{V}_P{V}_d)/(0.5T_P\right)$	W
R1 + R2	[$\frac{1}{4}{T}_{Cp-L1}{I}_{TSXi}^2(R_1+R_2)$]$/(0.5T_P)$
R3 + R4	[${\int }_0^{t_{S1on}}(R_3+R_4)\ast I_{TSXo}^{2}dt$]$/(0.5T_P)$
TSX339	[${\int }_0^{t_{S1on}}{V}_1\left(t\right)\ast 5\ast {10}^{-6}dt$]$/(0.5T_P)$
D5	[$C_i{V}_{Ci}{V}_d$]$/(0.5T_P)$
Q1	[${\int }_0^{t_{S1on}}0.09i_{L1}^2\left(t\right)dt$]$/(0.5T_P)$
R5 + R6 + R7	[${\int }_0^{t_{S2on}}\left(R_5+R_6+R_7\right)I_{MAXi}^{2}dt$]$/(0.5T_P)$
R8 + R9	[$\frac{1}{4}{T}_{Ci-L2}*(R_8+R_9)\ast {(\frac{5.5}{R_8+R_9})}^2$]$/(0.5T_P)$
R10	[$\frac{1}{2}{C}_2\ast {\left(5.5\right)}^2$]$/(0.5T_P)$
MAX9064	[${\int }_0^{t_{S2on}}{V}_{Ci}\left(t\right)\ast 0.1\ast {10}^{-6}dt$]$/(0.5T_P)$
Q2	[${\int }_0^{t_{S2on}}0.09i_{L2}^2\left(t\right)dt$]$/(0.5T_P)$
D6	[$C_i{V}_{Ci}{V}_d$]$/(0.5T_P)$
D7	[$C_s{V}_{Cs}{V}_d$]$/(0.5T_P)$

.

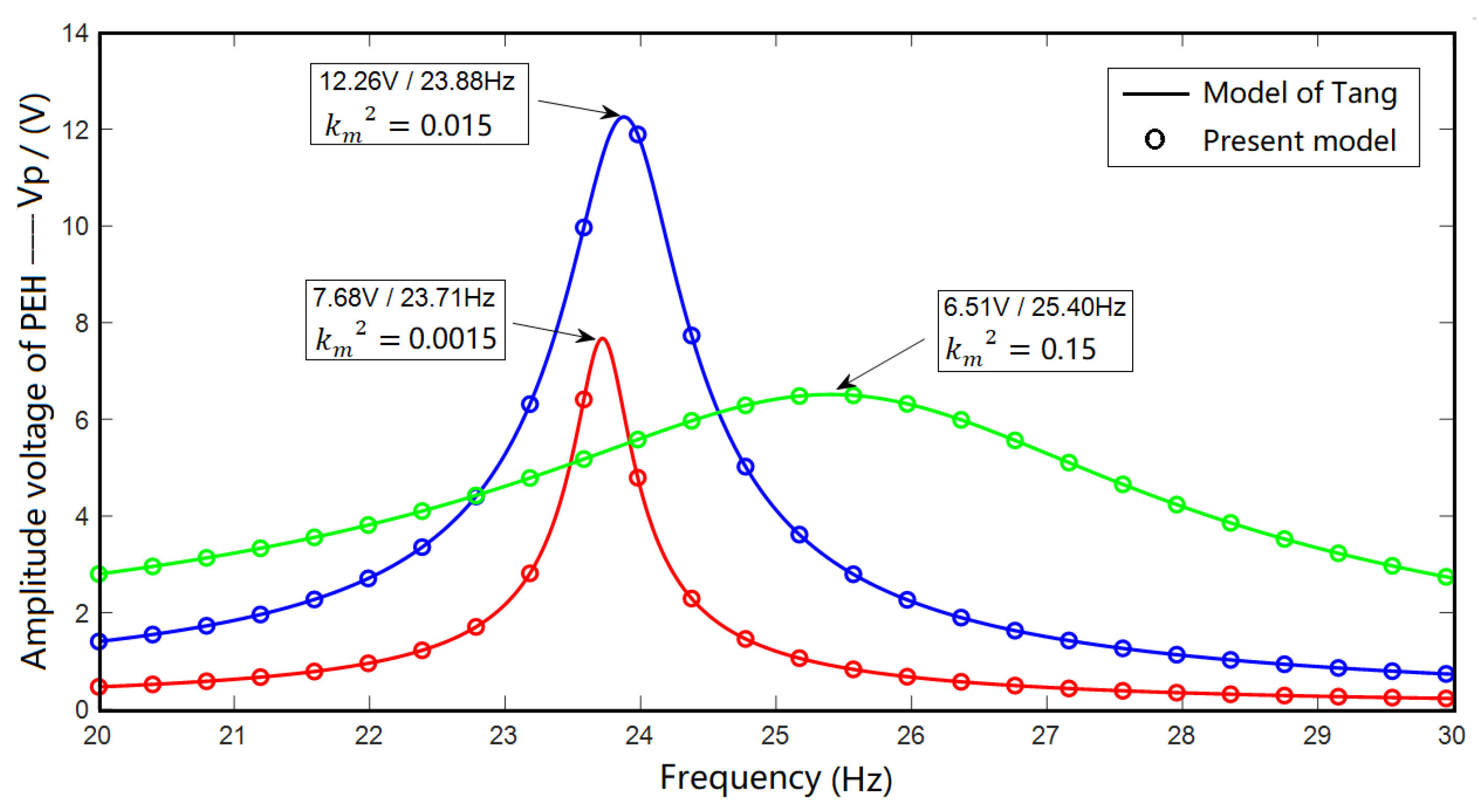
3.5. Validation of the Mode

In order to verify the accuracy of the model, the calculation results were compared with the existing literature and simulation results using the software MULTISIM. Parameters of the circuit are shown in

Table 2. Parameters of the circuit.

Object	Parameter	Value
PEH	Vp	12.26
	Capacitance, $C_P$(nF)	62.36
	Modal mass, $M$(kg)	1
	Modal stiffness, $K$(kN/m)	22.18
	Modal damping, $\eta$(N/m)	2.34
	Amplitude of modal force, $F_0$(N)	0.13
D1~D7		IN60P
	Forward voltage drop, $V_d$(V)	0.24
Resistor	$R_1,R_2$ ($\mathrm{K}\mathsf{\Omega }$)	200
	$R_3$ (M$\mathsf{\Omega }$)	2.46
	$R_4,R_{10}$ (M$\mathsf{\Omega }$)	2
	$R_5$ (M$\mathsf{\Omega }$)	4.09
	$R_6$ (M$\mathsf{\Omega }$)	2.75
	$R_7,R_8$ (M$\mathsf{\Omega }$)	0.1
	$R_9$ (M$\mathsf{\Omega }$)	1
Capacitor	$C_1$ (pF)	300
	$C_2$ (pF)	10
	$C_s$ (mF)	1
	$C_i$ (nF)	30
IC	U1	TSX339
	U2	MAX9064
Q1~Q2		IRF5852
Inductor	$L_1$,$L_2$, (mH)	1

.

• Comparison with the Existing Literature

Taking $k_m{}^2={\chi }^2/\left(K{C}_P\right)$ as the coupling factor of the PEH, the calculation results of present model were compared with Tang’s model [85] in terms of the influence of excitation frequency ($\omega$) on amplitude voltage of the PEH ($V_P$) in case $k_m{}^2$ = 0.0015 (weak coupling), 0.015 (medium coupling) and 0.15 (strong coupling). The results are shown in Figure 6, it can be seen that the error is less than 1%. Moreover, the strongest response of the EDSSH circuit to the PEH occurs in the case of medium coupling, and the corresponding output voltage amplitude and excitation frequency are 12.26 V and 23.88 Hz, respectively.

2.

Comparison with the Simulation Results

The calculation results of present model were compared with the simulation results of MULTISIM in terms of the voltage across C_S after charging for 60 s in three coupling cases. The results are shown in Figure 7. It can be seen that the voltage across C_S ($V_{Cs}$) calculated by present model in three coupling cases is 1.86, 4.48 and 1.59 V, and the simulation results are 1.58, 3.86 and 1.33 V with errors of 17.72%, 16.06% and 19.55%, respectively, which shows that the present model has good prediction ability. However, the simulation result is lower than the calculation result of present model. The main reason is that the loss of energy in each circuit is not fully considered in the model, but these factors are included in the electronic component parameter model of MULTISIM.

4. Simulation and Experiment

4.1. Simulation of Rectifier Bridge

MULTISIM software was used to simulate the circuit (Figure 3). Figure 8 shows the waveform of the output voltage ($V_P\left(t\right)$, red curve) generated by the PEH after being excited and the waveform of the output voltage ($V_1\left(t\right)$, blue curve) of the rectifier bridge. It can be seen that the rectifier bridge rectifies the AC sinusoidal signal of $V_P\left(t\right)$ into the DC signal ($(V_1\left(t\right)$).

4.2. Simulation of Control Circuit of S₁

The control circuit of S₁ consists of comparator TSX339, resistors R₁, R₂, R₃ and capacitor C₁. The no-inverting input voltage of TSX339 is represented by $V_{U1\left(t\right)}^{+}$, the inverting input voltage is represented by $V_{U1\left(t\right)}^{-}$ and the output voltage is represented by $V_{U1\left(t\right)}^{out}$. $V_{U1\left(t\right)}^{out}$ and $V_1\left(t\right)$ are the same. The main function of the circuit is to automatically make the $V_{U1\left(t\right)}^{out}$ high voltage when $V_1\left(t\right)$ reaches its peak value. $V_{U1\left(t\right)}^{out}$ controls the conduction of field-effect transistor Q1 (IRF5852) for a short duration at the same time. Electrical energy on C_P must be transmitted to L₁ in one way. The waveforms of $V_{U1\left(t\right)}^{+}$, $V_{U1\left(t\right)}^{-}$ and $V_{U1\left(t\right)}^{out}$ are shown in Figure 9. It can be seen that $V_{U1\left(t\right)}^{out}$ is low voltage due to $V_{U1\left(t\right)}^{+}$ being lower than $V_{U1\left(t\right)}^{-}$ in the period from 0 to t₀. During this period, IRF5852 is turned off. When $V_1\left(t\right)$ reaches its peak value at t₀, $V_{U1\left(t\right)}^{out}$ becomes higher than drain-source on-voltage of IRF5852 due to $V_{U1\left(t\right)}^{+}$ being higher than $V_{U1\left(t\right)}^{-}$. Then IRF5852 turns on automatically because $V_{U1\left(t\right)}^{out}$ is high voltage. When $V_1\left(t\right)\left(V_{U1\left(t\right)}^{out}\right)$ decreases to drain-source on-voltage of IRF5852 near t₁, IRF5852 turns off automatically.

4.3. Experiment

4.3.1. Experimental Platform

The experimental platform is shown in Figure 10. The function generator1 (AFG3021C) generates a sine wave function (acceleration 0.5 g) at the natural frequency of the PEH. The power amplifier amplifies the excitation signal generated by function generator1 to drive the exciter to vibrate, which makes the PEH produce resonance and output 12.26 V AC. Function generator2 simulates the control signal of switch S₂. The designed circuit board (the circuit diagram is shown in Figure 3) is connected with the output terminal of the PEH to harvest the electric energy generated by the PEH. The oscilloscope (TDS2024C) is used to measure the voltage signal of the corresponding measuring point on the circuit board.

4.3.2. Influence of RFBC on Electric Energy Harvesting Efficiency

In order to verify that the RFBC in the EDSSH circuit can make the circuit maintain the optimal electric energy harvesting efficiency, taking the L₁-C_i circuit as an example, the output voltage waveform of capacitor C_i was measured in one energy harvesting cycle (0.01 s) when DSSH circuit was not configured with D₅ (Figure 11a) and EDSSH circuit was configured with D₅ (Figure 11b).

It can be seen that $V_{Ci}\left(t\right)$ can reach the optimal value (V_max = −13.84 V) only when the duration of t₁-t₂ is equal to one fourth of the oscillation period (0.25∗T_ci-L1) of the L₁-C_i loop precisely. Otherwise, due to LC oscillation, the voltage values for C_i are different at different times of t₂. For example, the voltages of t₂ at $t_{2-1}$, $t_{2-2}$ and $t_{2-3}$ are V₁ (−7.86 V), V₂ (−3.74 V) and V₃ (−0.42 V), which are 56.79%, 26.98% and 3.03% of the optimal value (V_max), respectively. That is to say, the efficiency of energy transferring of L₁-C_i loop of DSSH circuit without D₅ cannot be kept at the optimal value. Figure 11b is the waveform of the output voltage $\left(V_{Ci}\left(t\right)\right)$ of the capacitor C_i when the EDSSH circuit is configured with D₅. It can be seen that only if the duration of t₁-t₂ is more than one fourth of the oscillation period of L₁-C_i loop, the L₁-C_i circuit only has one-way power transfer from L₁ to C_i; that is, after L₁ charges C_i, the voltage of C_i keeps the optimal value (V_max) unchanged.

4.3.3. Efficiency of EDSSH Circuit

The voltage $V_P\left(t\right)$ of the PEH and the increased voltage $\Delta V_{Ci}\left(t\right)$ of C_i were measured with the experimental platform shown in Figure 10, and their amplitudes are $V_P$ and $\Delta V_{Ci}$, respectively. The electric energy consumed by diode D₇ is $E_{D7}={\int }_{t_0}^{t_1}{V}_d\ast i_{Cs}\left(t\right)dt={\int }_{t_0}^{t_1}\ast C_{s}d{V}_{Cs}\left(t\right)=C_i{V}_d\Delta V_{Cs}$. It can be seen from Equation (9) that the value of $\Delta V_{Cs}$ in each charging cycle is related to the voltage of capacitor (C_S) and the charging time ($t$). Thus, $E_{D7}$ is not a constant but a variable related to $V_{Cs}$ and $t$. The $V_{Cs}$ of C_S was measured after 60 s charging, and the results are shown in

Table 3. Efficiency and nodal-voltage of EDSSH circuit.

${\mathit{V}}_{\mathit{P}} \left(\mathbf{V}\right)$	$\Delta {\mathit{V}}_{\mathit{C}\mathit{i}}\left(\mathbf{V}\right)$	${\mathbf{\eta }}_{\mathit{P}\mathit{E}\mathit{H}-{\mathit{C}}_{\mathit{i}}}(\%)$	${\mathit{V}}_{\mathit{C}\mathit{s}} \left(\mathbf{V}\right),60\mathbf{s}$	${\mathbf{\eta }}_{\mathit{D}6-{\mathit{C}}_{\mathit{s}}}(\%)$	$\mathbf{\eta }(\%)$
12.26	−13.84	73.29	3.85	74.81	54.83

, where ${\mathsf{\eta }}_{PEH-C_i}({\mathsf{\eta }}_{PEH-C_i}=\frac{\Delta E_{Ci}}{E_P}=\frac{\frac{1}{2}{C}_i\Delta V_{Ci}{}^2}{\frac{1}{2}{C}_P{V}_P^2}\ast 100\%)$is the efficiency of the circuit from the PEH to C_i, ${\mathsf{\eta }}_{D6-C_s}({\mathsf{\eta }}_{D6-C_s}=\frac{\Delta E_{Cs}}{{\sum }^{}\Delta E_{Ci}}\ast 100\%)$ is the charging efficiency within 60 s of the circuit from D₆ to C_s, $\sum \Delta E_{Ci}$ is the total electric energy accumulated on C_i within 60 s charging time and $\mathsf{\eta }\left(\mathsf{\eta }={\mathsf{\eta }}_{PEH-C_i}\ast {\mathsf{\eta }}_{D6-C_s}\right)$ is the total efficiency of the circuit.

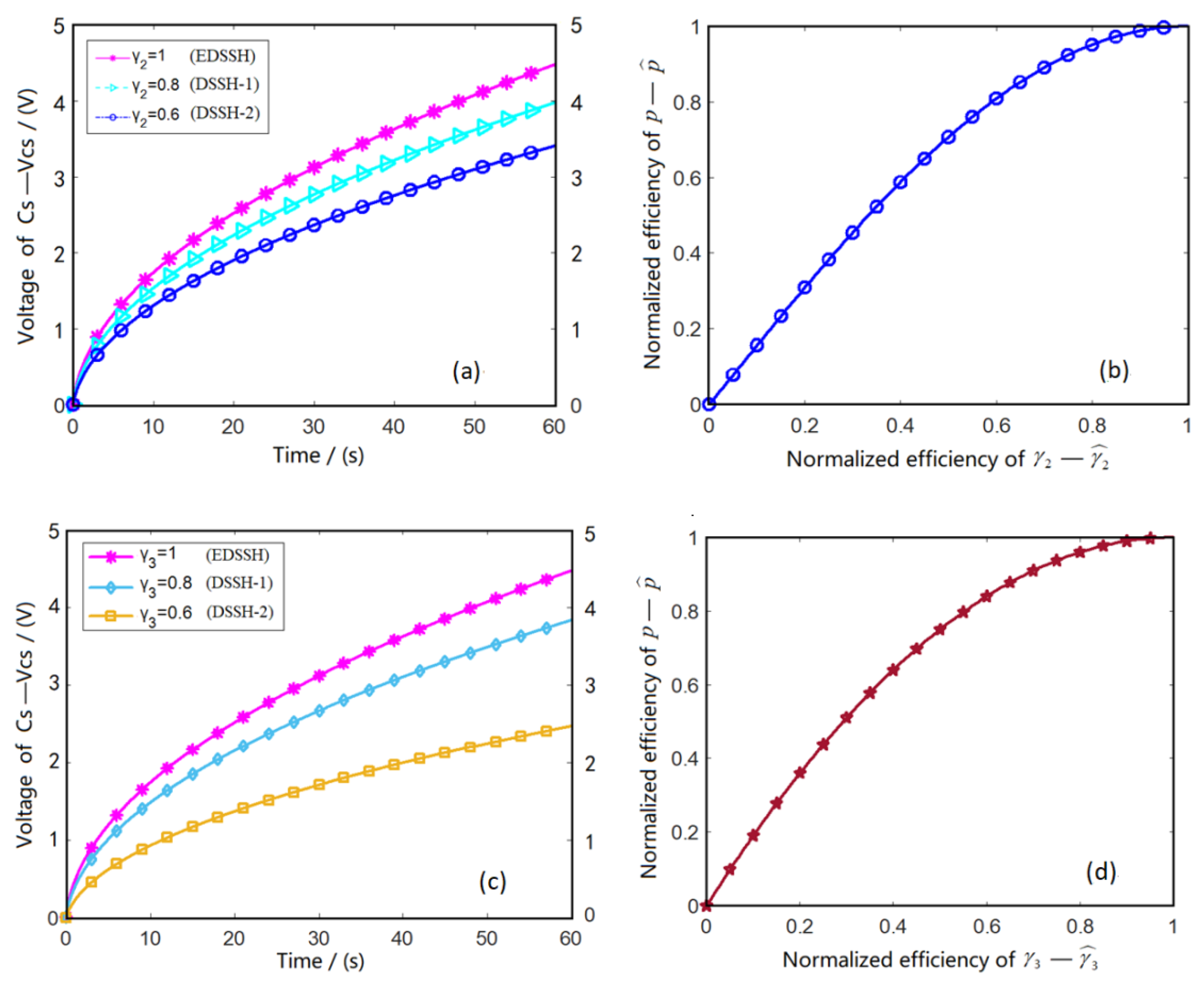
4.3.4. Efficiency Comparison between EDSSH and DSSH Circuits

It can be seen from Figure 11 that the output voltage of the DSSH circuit is uncertain. The voltage of C_S of EDSSH and DSSH circuits was measured after 60 s charging time five times, and the charging efficiency of each time was calculated, taking the average value. The results are shown in

Table 4. Efficiency comparison between EDSSH circuit and DSSH circuit.

	EDSSH			DSSH
Times	$V_{Cs} \left(\mathrm{V}\right)$	$\mathsf{\eta }$(%)	${\overline{\mathsf{\eta }}}_1(\%)$	$V_{Cs} \left(\mathrm{V}\right)$	$\mathsf{\eta }$(%)	${\overline{\mathsf{\eta }}}_2(\%)$
1	3.84	54.51	52.16	3.12	35.97	34.55
1	3.62	48.43		2.15	17.08
3	3.74	51.69		3.54	46.31
4	3.80	53.36		3.78	52.80
5	3.78	52.81		2.36	20.58

, where ${\overline{\mathsf{\eta }}}_1$ and ${\overline{\mathsf{\eta }}}_2$ are the average charging efficiency of EDSSH and DSSH circuits, respectively. It can be seen that the average charging efficiency of the EDSSH circuit is 1.51 times than that of DSSH.

5. Discussion

5.1. Advantages of EDSSH Circuit

(1)

The low-power design. The complex control module and special power module in the DSSH circuit are required. Compared with the control circuit of S₁ and S₂ in the DSSH circuit, the EDSSH circuit integrated a module of a control circuit of switch S₁ and S₂ with simple structure and low-power IC. The control circuit of S₁ only comprises a comparator TSX339, three resistors and one capacitor. The maximum average power consumption of TSX339 is 0.96 μW in one electrical energy extraction cycle when $V_p\left(t\right)$ reaches the maximum value of 12.26 V. In addition, the power supply and input signal of the comparator TSX339 are from the output terminal rectifier V₁. The control circuit of S₂ only comprises a comparator MAX9064, four resistors and one capacitor. The maximum average power consumption of MAX9064 is lower than 1 μW in one electrical energy extraction cycle when $V_{Ci}\left(t\right)$ reaches the maximum value. In addition, the power supply and input signal of the comparator MAX9064 are from the $V_{Ci}$. According to Section 4.3, the total average charging efficiency of the EDSSH circuit is 52.16% compared with 34.55% of that of the DSSH circuit.

(2)

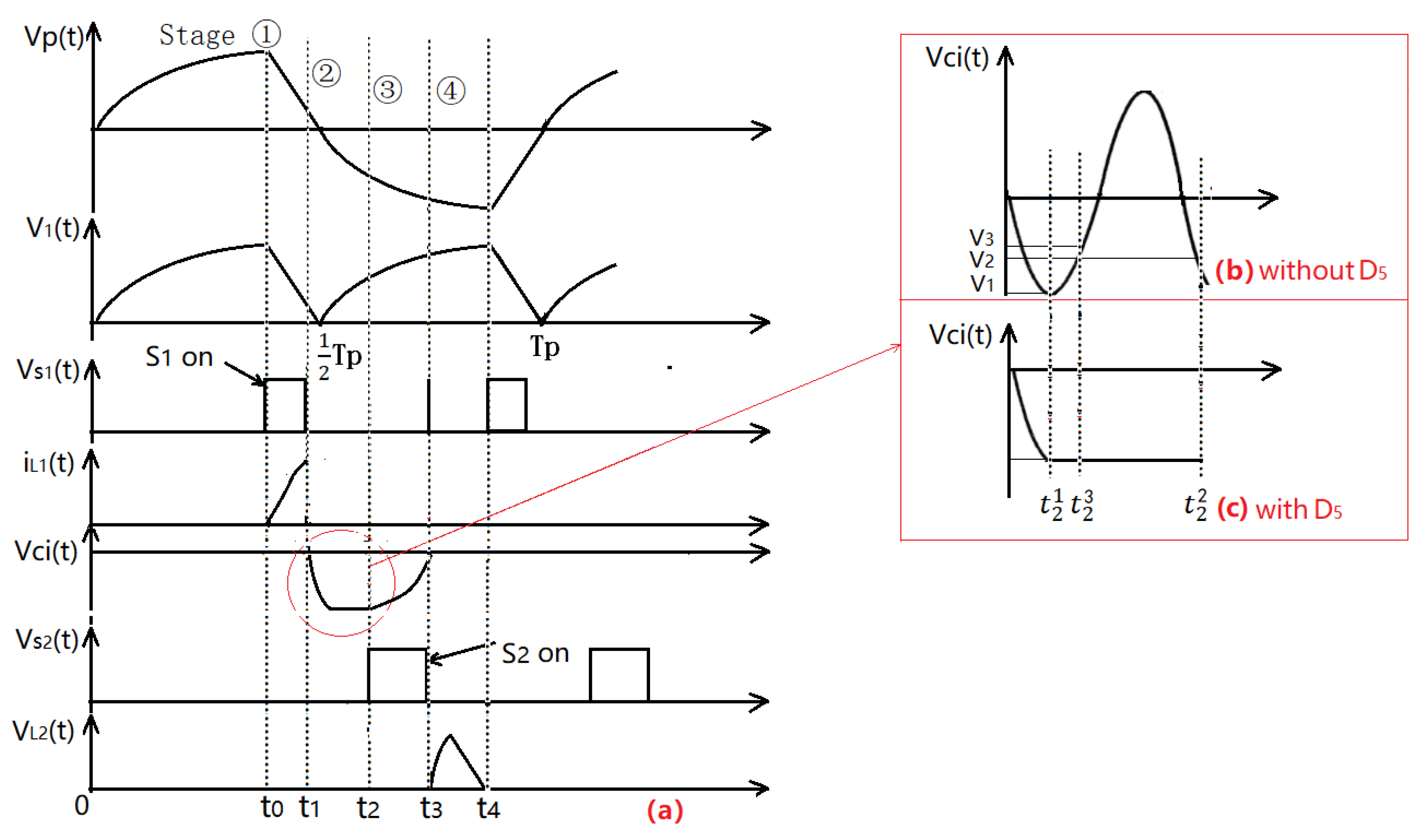
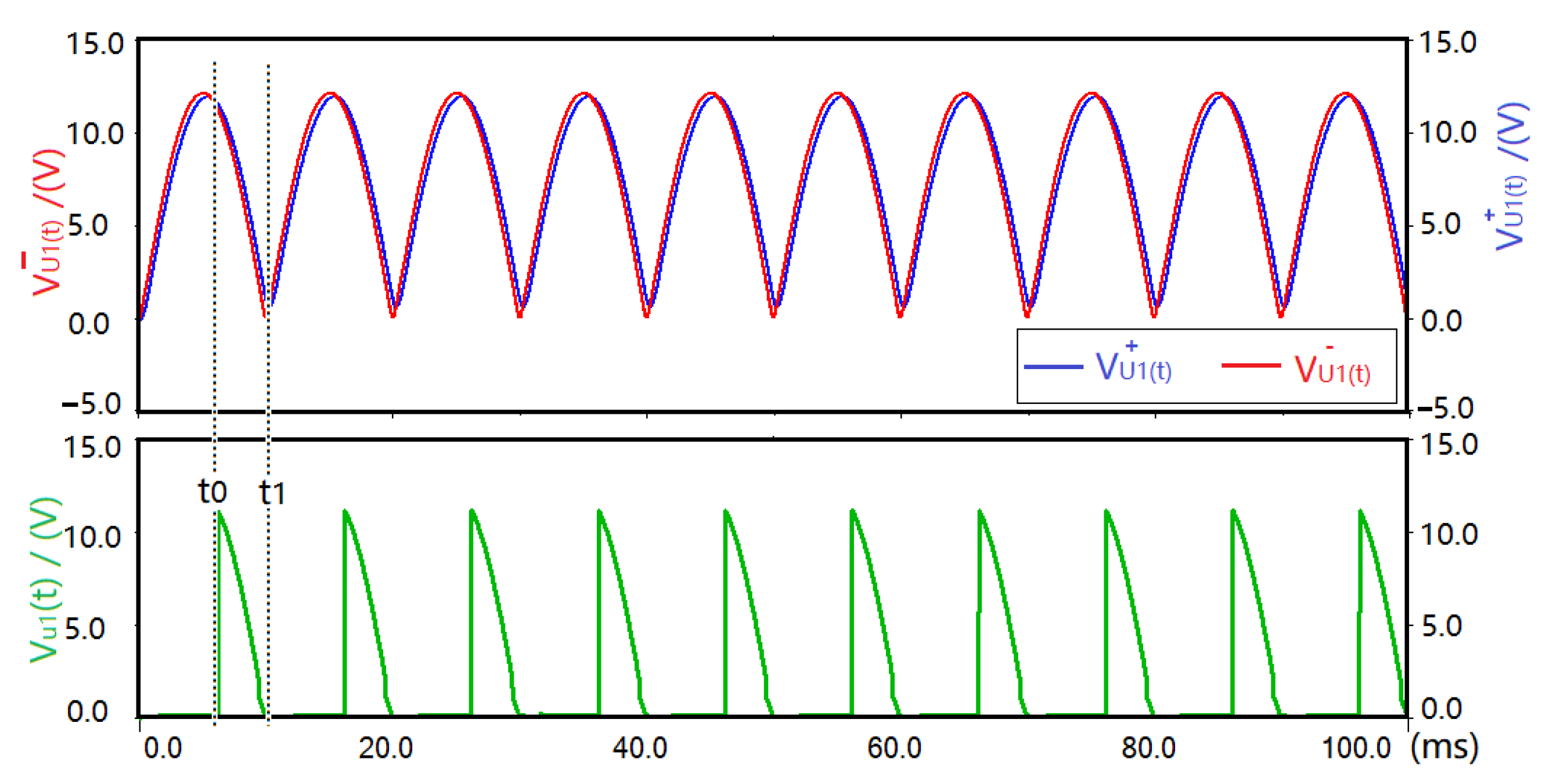
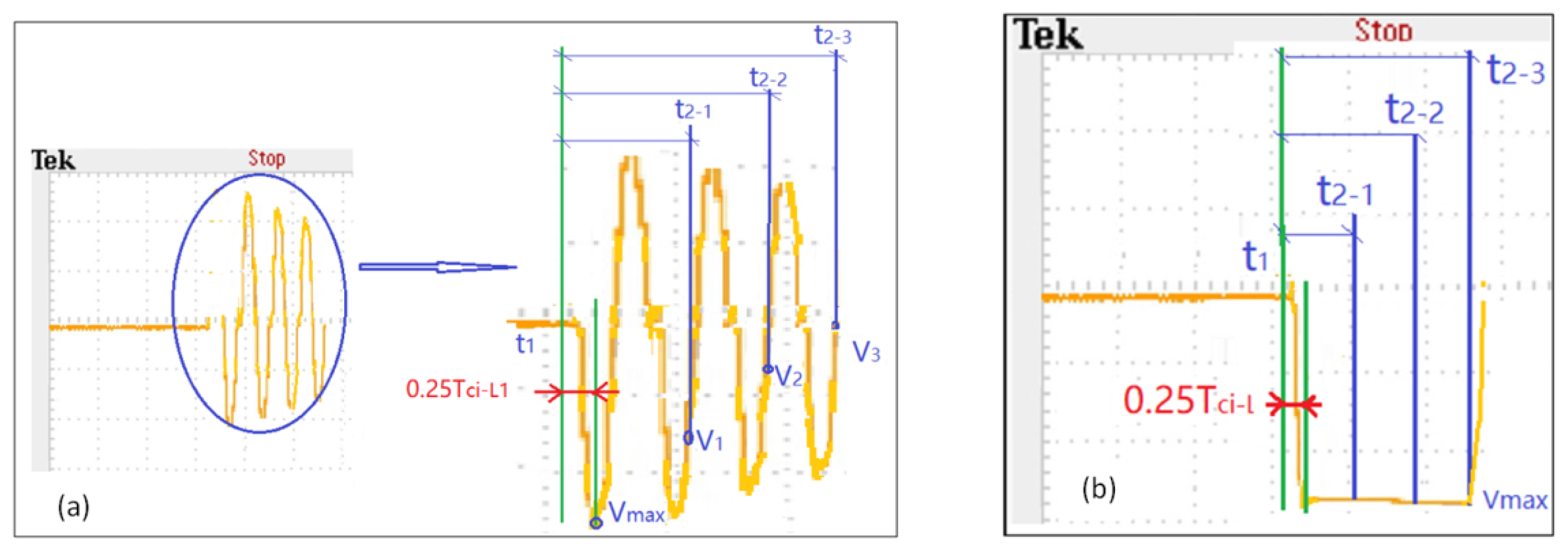
The high-efficiency design. The RFBC is used to keep the power harvesting efficiency at the optimal value. Diodes D₅ and D₆ are configured in the loop to avoid reverse feedback of electric energy caused by LC oscillations in one energy harvesting cycle. Taking the L₁-C_i circuit as an example, if D₅ is not configured in the DSSH circuit, the duration of t₁-t₂ should be precisely controlled to be equal to one fourth of the oscillation period of L₁-C_i. Otherwise, the voltage values of C_i are different according to different time of t₂ (Figure 5b, the voltage on C_i at $t_2^1$,$t_2^2$ and $t_2^3$ are V₁, V₂ and V₃, respectively), and the efficiency of electric energy transfer is uncertain. When the EDSSH circuit is configured with D₅, as long as the duration of t₁-t₂ is greater than one fourth of the oscillation period of L₁-C_i, the L₁-C_i loop only has one-way power transfer from L₁ to C_i; that is, the voltage of C_i remains unchanged after L₁ charging C_i (Figure 5c) so as to maintain the optimal efficiency.

(3)

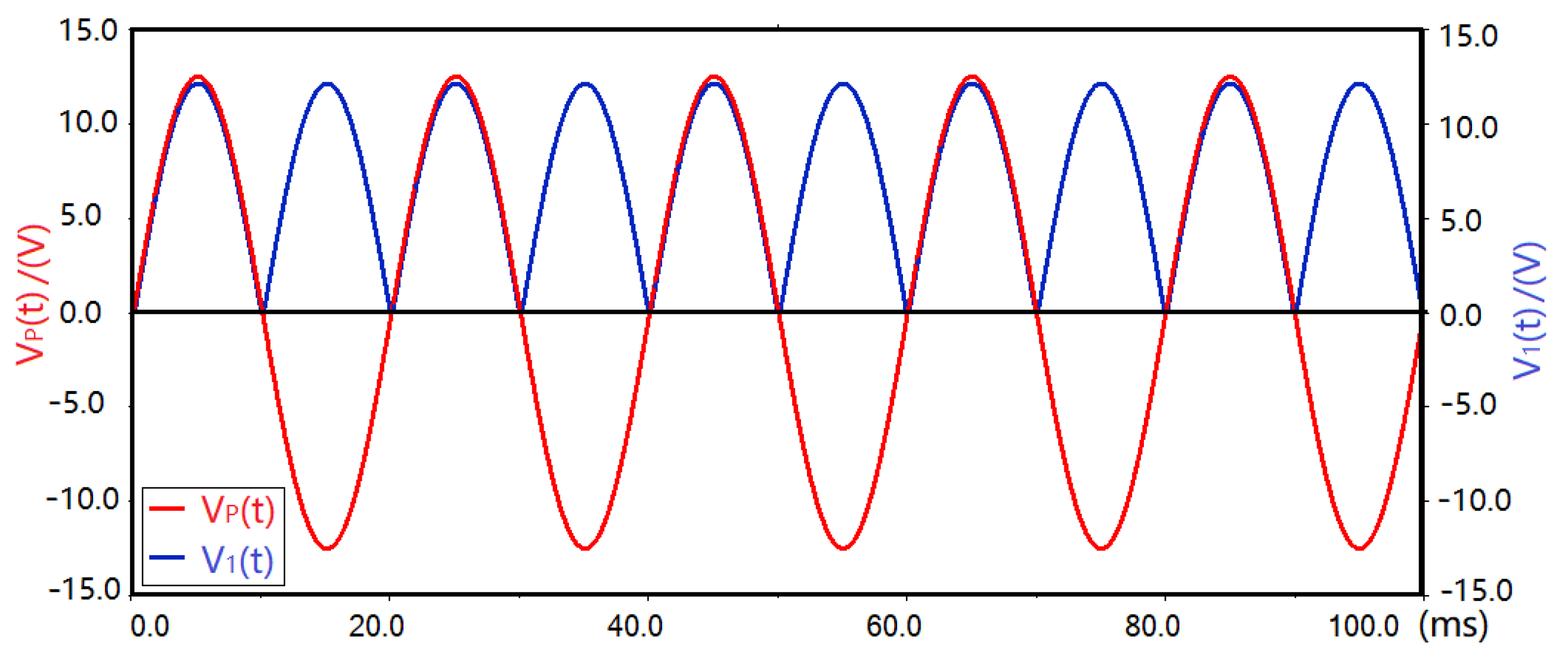
The adaptive design. Firstly, the control circuit of S₁ is in the sleep state most of the time and self-cold-starting and turns on for a short time when $V_1\left(t\right)$ reaches its peak value. The control circuit of S₂ is in the sleep state most of the time and self-cold-starting and turns on for a short time when $V_{Ci}\left(t\right)$ reaches its peak value. Secondly, it can adapt to the wide frequency range of input sinusoidal voltage signal. The main reason is that the single energy acquisition time $T_C$ of the circuit is only 1.6004 ms (including 0.0124 ms of C_P-L₁ loop, 0.0086 ms of L₁-C_i- loop, 0.0086 ms of C_i-L₂ loop and 1.5708 ms of L₂-C_s- loop). As long as half of the cycle of the input sinusoidal voltage signal is longer than $T_C$, the acquisition process can be completed automatically. Therefore, the circuit can automatically adapt to the input sinusoidal voltage signal of 1~312.5 Hz. Lastly, the circuit features a buck-boost structure, which can automatically match various loads.

5.2. Comparison of Charging Performance between EDSSH Circuit and DSSH Circuit

Because of the RFBC, the power conversion efficiency of the L₁-C_i loop and C_i-L₂ loop in the EDSSH circuit can be stabilized at the optimal value. The DSSH circuit, because it does not use this technology, exhibits loop reverse feedback, resulting in the reduction of power transfer efficiency. Here, we can compare the charging performance between the EDSSH circuit and DSSH circuit by using the established model (Formula (12) in this paper) and MULTISIM software. For example, the power transfer efficiency corresponding to $t_2^2$ and $t_2^3$ in Figure 5a is ${\mathsf{\gamma }}_2=$ 0.8 and 0.6. Set $C_s=$ 1 mF, $k_m{}^2=$ 0.015 and analyze the voltage of charging capacitor ($V_{Cs}$) after 60 s charging time under three conditions of ${\gamma }_2$ = 1.0, 0.8 and 0.6. The results are shown in Figure 12a, and it can be seen that $V_{Cs}$ increases with the increase in ${\gamma }_2$.The influence of the normalization efficiency (${\widehat{\gamma }}_2$(${\gamma }_2/{\gamma }_{2max}$)) of ${\gamma }_2$ on the normalized power ($\widehat{P}(P_2/P_{max}$)) was analyzed. The results are shown in Figure 12b, and it can be seen that $\widehat{P}$ increases with the increase in ${\widehat{\gamma }}_2$. Similar to ${\gamma }_2$, the influence of ${\gamma }_3$ on $V_{Cs}$ and the influence of the normalization efficiency (${\widehat{\gamma }}_3({\gamma }_3/{\gamma }_{3max}$)) of ${\gamma }_3$ on the normalized power ($\widehat{P}(P_3/P_{max}$)) are shown in Figure 12c,d, respectively. The results show that $V_{Cs}$ increases with the increase in ${\gamma }_3$ and $\widehat{P}$ increases with the increase in ${\widehat{\gamma }}_3$. This proves that the RFBC technology can significantly improve the amplitude of output voltage and keep the efficiency of each circuit at the optimal value.

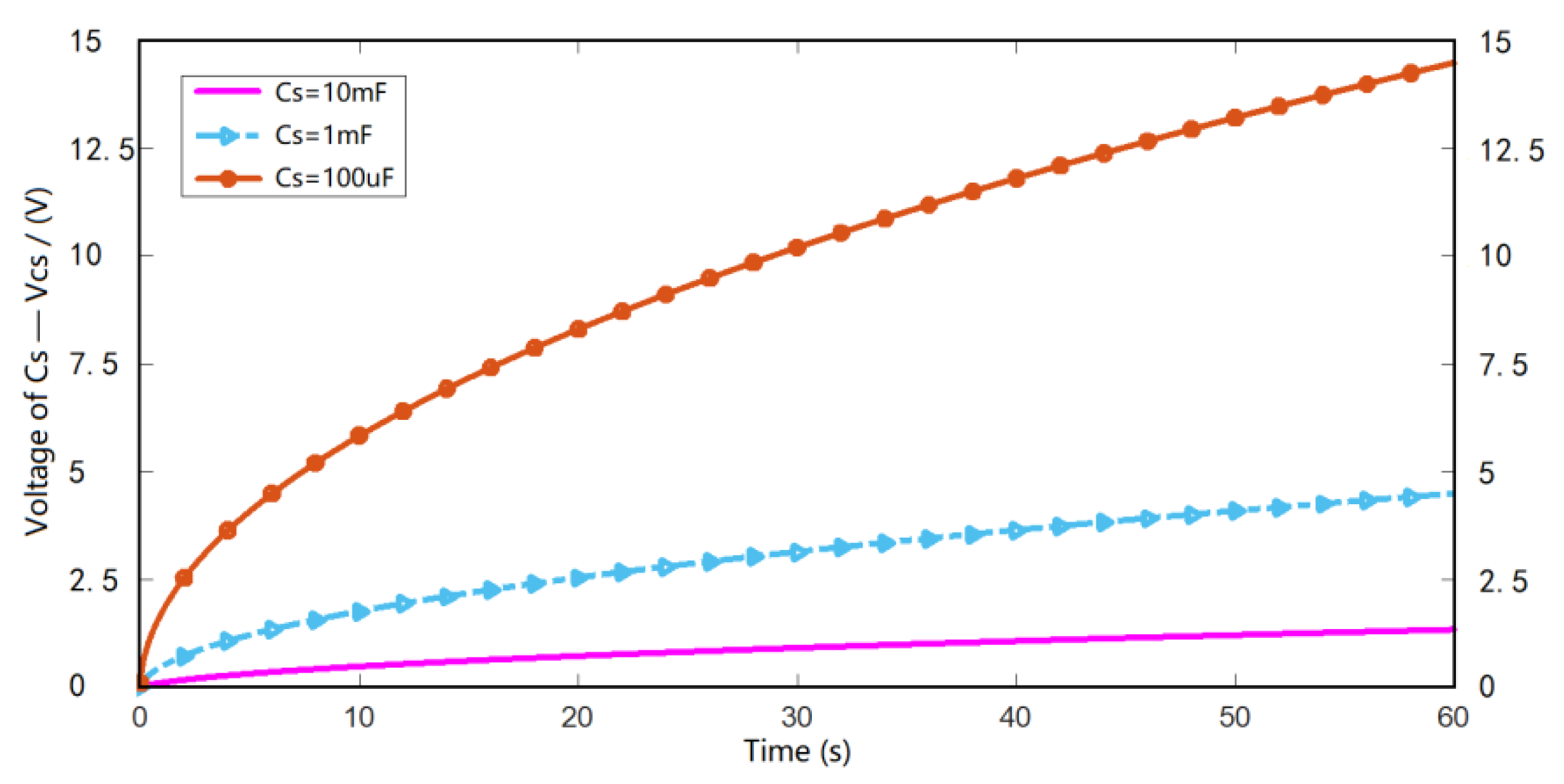
5.3. Influence of Capacitance of C_s on Charging Performance

The influence of capacitance of Cs on charging performance was analyzed based on the proposed circuit model (Formula (12)) and MULTISIM software. In the process of electric energy harvesting, the influence of the capacitance of C_s on its charging voltage $V_{Cs}$ is shown in Figure 13. It can be seen that when the capacitance $C_s=100\mathsf{\mu }\mathrm{F},1\mathrm{m}\mathrm{F}$ and $10\mathrm{m}\mathrm{F}$, $V_{Cs}$ reaches 14.76, 4.48 and 1.26 V, respectively, after 60 s charging time. This indicates that the voltage on the charging capacitor increases faster with smaller capacitor $C_s$.

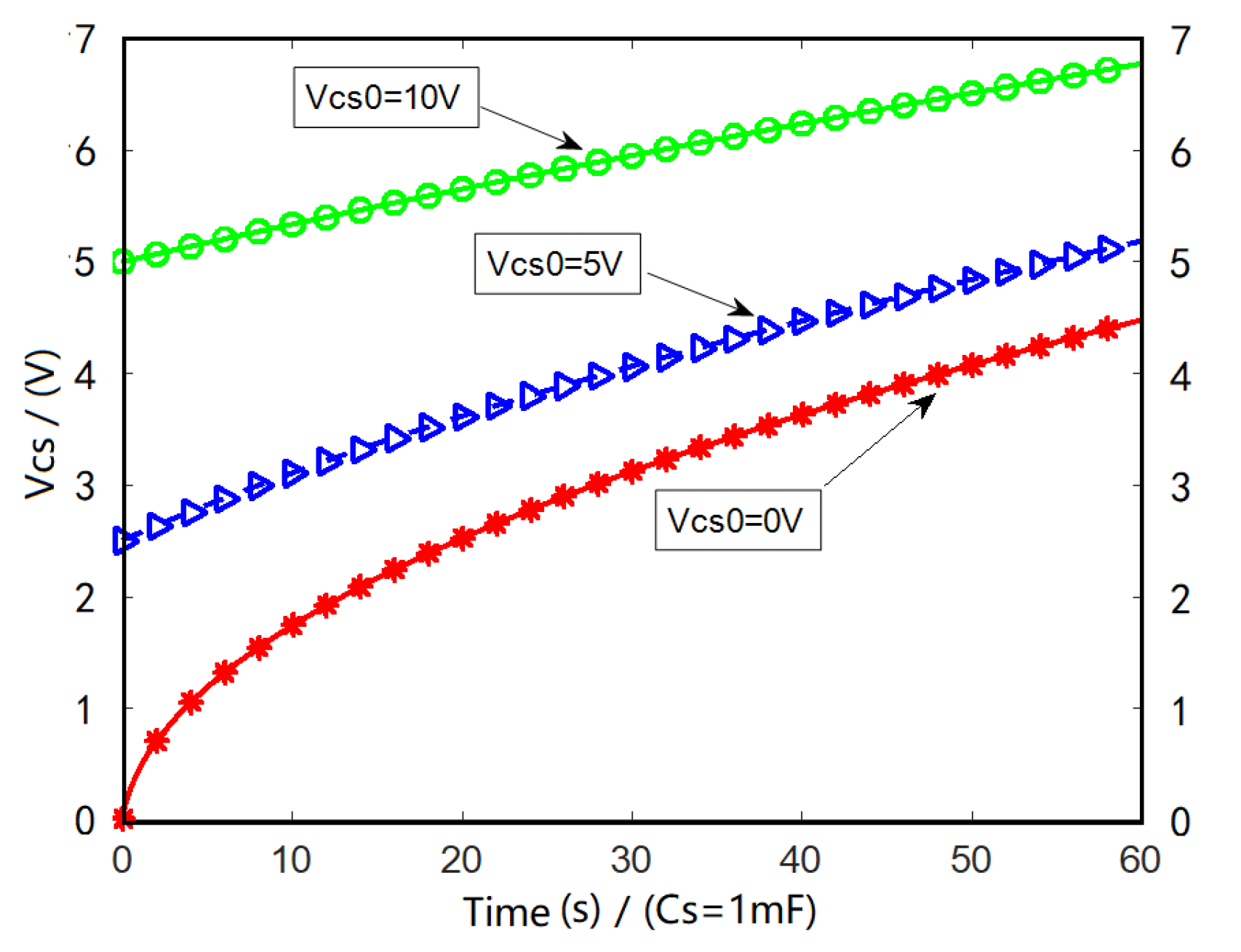
5.4. Influence of Initial Voltage of C_s on Charging Performance

In the process of power harvesting, the initial capacitance of C_s ($V_{Cs0}$) has a significantly influence on the increment of voltage ($\Delta V_{Cs}$) and voltage value ($V_{Cs}$) of the charging capacitor. Influence of initial voltage of Cs on charging performance can also be analyzed based on the proposed circuit model (Formula (12)) and MULTISIM software. As can be seen from Figure 14, when $V_{Cs0}$ is 0, 5 and 10 V, $V_{Cs}$ reaches 4.48, 5.16 and 6.75 V, respectively, after 60 s charging time. Figure 15 shows that when $V_{Cs0}$ increases from 0 to 10 V, the voltage increase value of charging capacitor decreases from 4.48 to 1.75 V after 60 s charging time.

5.5. Design Criteria for Parameters of EDSSH Circuit

Assuming that the piezoelectric structure resonates under the frequency of 50 Hz (period $T_P$ = 0.02 s), the longest time interval (t₀–t₃) between two power acquisitions is half of the resonance period ($\frac{1}{2}{T}_P$) according to Figure 5; that is to say, all the links of piezoelectric electric energy transfer to C_s are completed in this $\frac{1}{2}{T}_P$. Since the time required for each link is related to parameters of the circuit, it is necessary to discuss the design criteria of the circuit’s parameters of $C_P$, $L_1$, $C_i$, $L_2$, $C_s$ and control modules of S₁ which are related to power transfer efficiency.

1. Design of parameters of main circuit components: First, the clamping capacitor ($C_P$) of the piezoelectric structure was determined after the size design. In order to transfer the electric energy of C_P to L₁ to the greatest extent, the time (t₀-t₁) of electric energy transferred from the capacitor C_P to the inductor L₁ should be controlled at one eighth of $T_P$; that is, the oscillation period of C_p-L₁ loop should be less than $\frac{1}{8}{T}_P$. According to the calculation formula $T=2\mathsf{\pi }\sqrt{C_P\ast L_1}<\frac{1}{8}{T}_P$ of the LC oscillation period, the design range of $L_1$ is $L_1<\frac{{\left(\frac{1}{8}{T}_P\right)}^2}{4C_P{\mathsf{\pi }}^2}$. Then, the charging capacitor was selected according to the actual needs. Since the oscillation period of the L₂-C_S loop is generally less than $\frac{1}{8}{T}_P$, it is assumed that the capacitance value of the charging capacitor is $C_s$, and the design range of $L_2$ can be determined by $L_2<\frac{{\left(\frac{1}{8}{T}_P\right)}^2}{4C_s{\mathsf{\pi }}^2}$. Finally, the oscillation period of the L₁-C_i circuit is less than $\frac{1}{8}{T}_P$, so $C_i<\frac{{\left(\frac{1}{8}{T}_P\right)}^2}{4L_1{\mathsf{\pi }}^2}$. Of course, this is the basic principle and order. On the premise that the total time to complete a power transfer is less than $\frac{1}{2}{T}_P$, the time length of each circuit can be adjusted, and then the values of $L_1$, $C_i$, $L_2$ and $C_s$ can be determined.

2. Parameter design of switch control circuit of S₁: First, the power consumption of the circuit should be as small as possible; that is to say, the resistance value of $R_1$ and $R_2$ should be as large as possible. Then the delay time ($T_{RC}$) of the RC delay circuit composed of C₁ and R₂ should be controlled to be as short as possible, so that the power transfer can start at the amplitude of $V_1\left(t\right)$. The values of R₂ and C₁ were determined by $T_{RC}$ = $R_2\ast C_1$. Finally, the comparator should choose the components with the lowest power consumption.

6. Conclusions

(1)

A low-power high-efficiency adaptive electric energy harvesting circuit for broadband PEHs is proposed. The control circuit of switches is simpler, the electronic components used is fewer, and the power loss of switch control circuits is less than that of the DSSH circuit. It is self-cold-starting with a threshold voltage as low as 0.2 V.

(2)

Compared with the DSSH circuit, the electric energy harvesting efficiency of the EDSSH circuit with the proposed RFBC is stable at the optimal value. The average charging efficiency of the EDSSH circuit is 1.51 times than that of DSSH.

(3)

During the circuit test, the input voltage is an ideal sine wave signal, so the circuit can successfully capture the power extreme point of the signal. If there is noise interference in the input voltage, the circuit may operate incorrectly when capturing the power extreme point, which affects the power extraction efficiency of the circuit. Therefore, it is necessary to add circuit modules at the input of the circuit to remove noise interference.

(4)

According to the electric energy conversion time of each circuit of the circuit and the charging time of C_S, it can be calculated that the frequency range of the input voltage signal that the circuit can match is 1–312.5 Hz To broaden the frequency range of the input signal that the circuit can match, the relevant components must be recalculated and replaced.

(5)

The performance of the circuit matching nonlinear piezoelectric vibration energy harvester needs to be further investigated.