Planar Micro-Positioning Device Based on a 3D Digital Electromagnetic Actuator
Abstract
1. Introduction
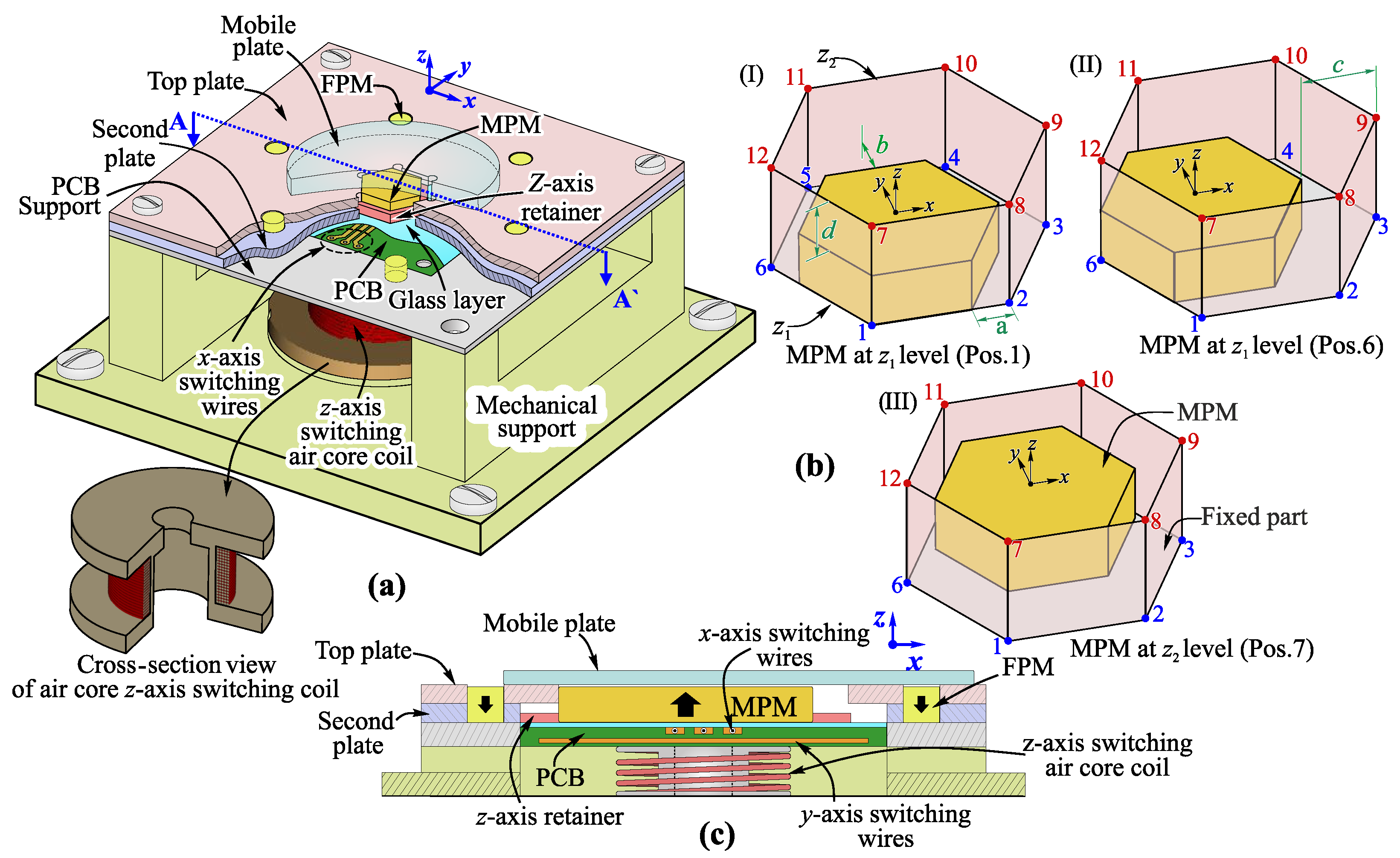
2. Design and Working Principle of the Positioning Device
2.1. Description of the Digital Actuator
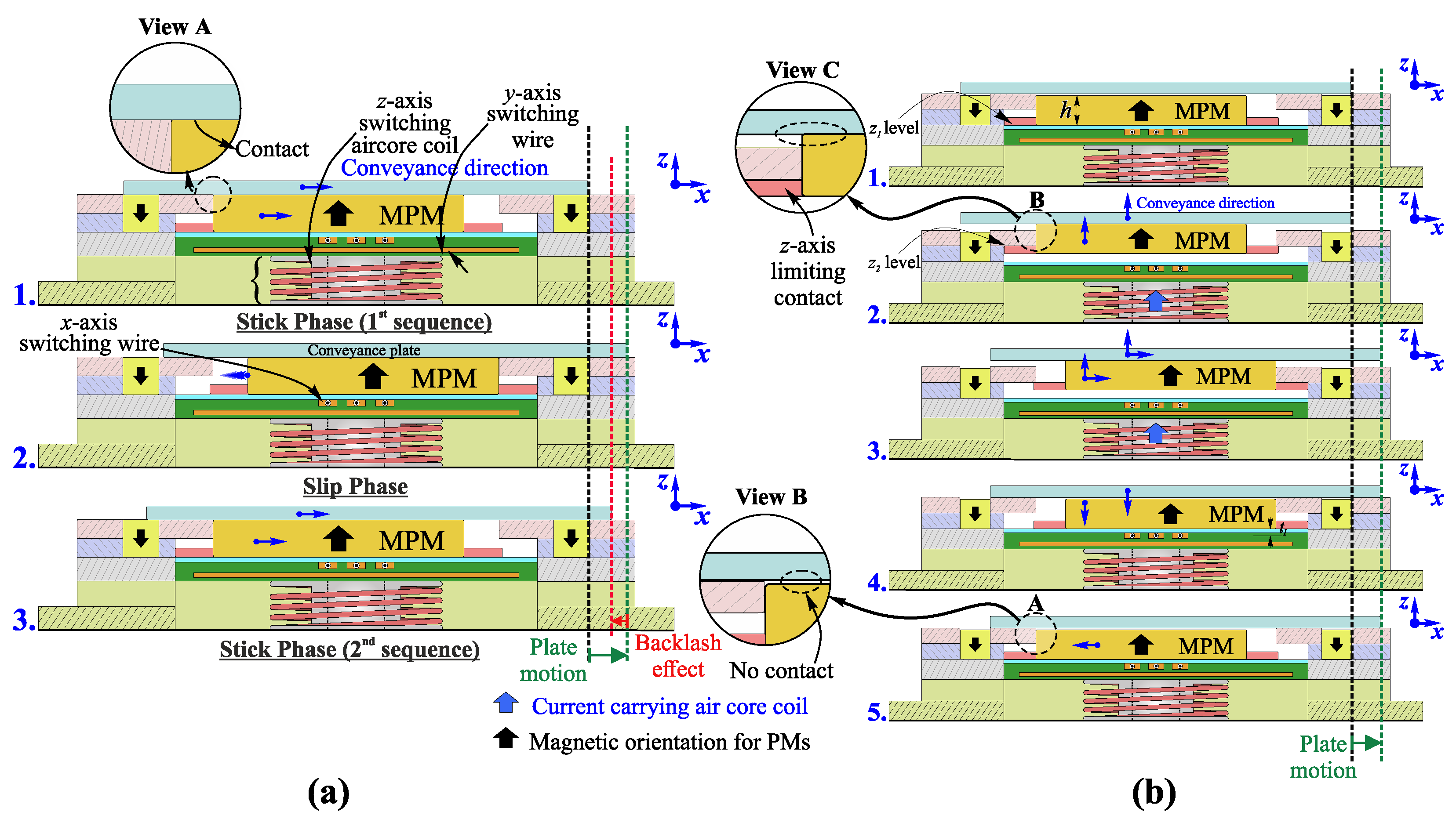
2.2. Displacement Approaches
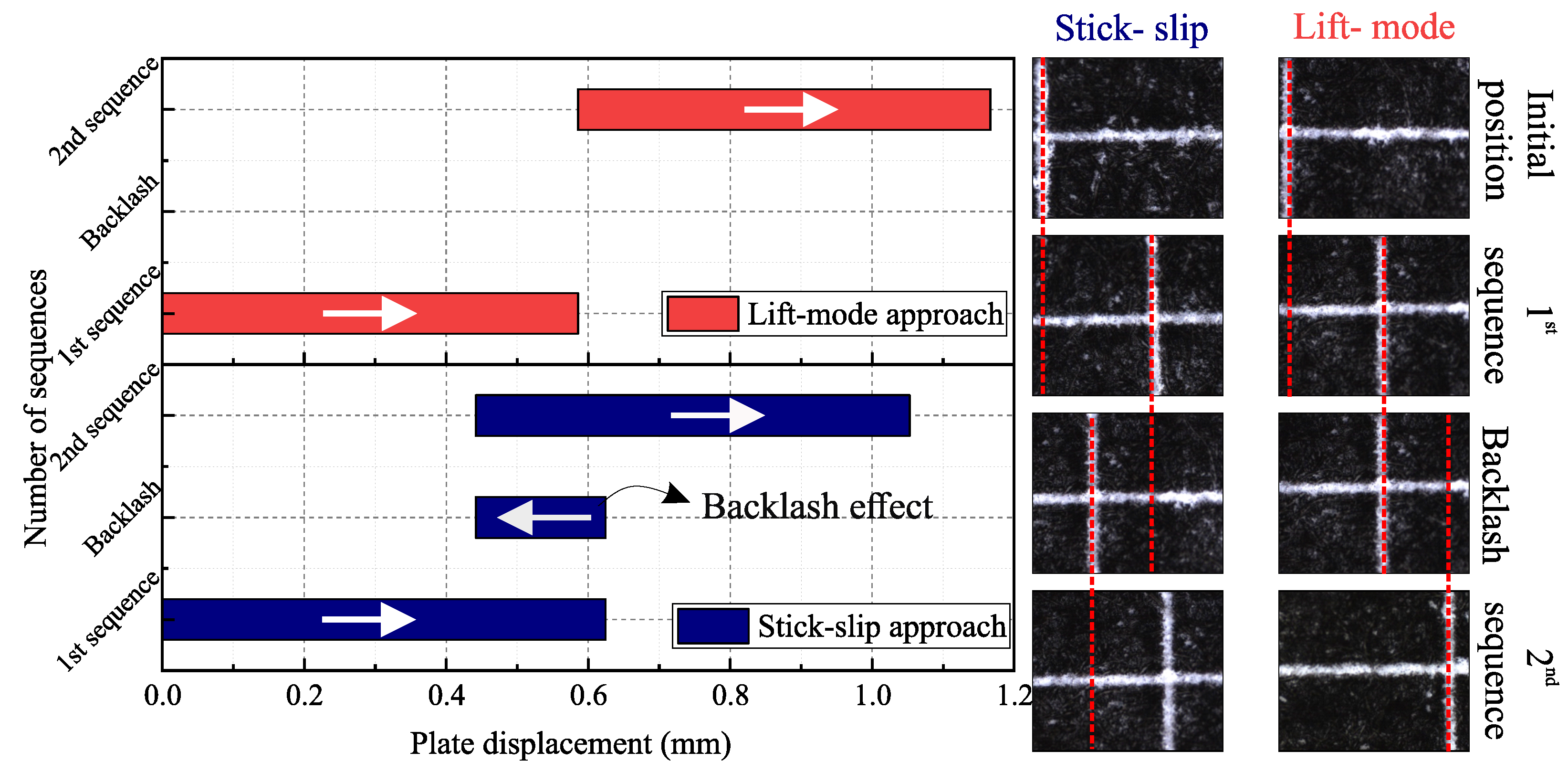
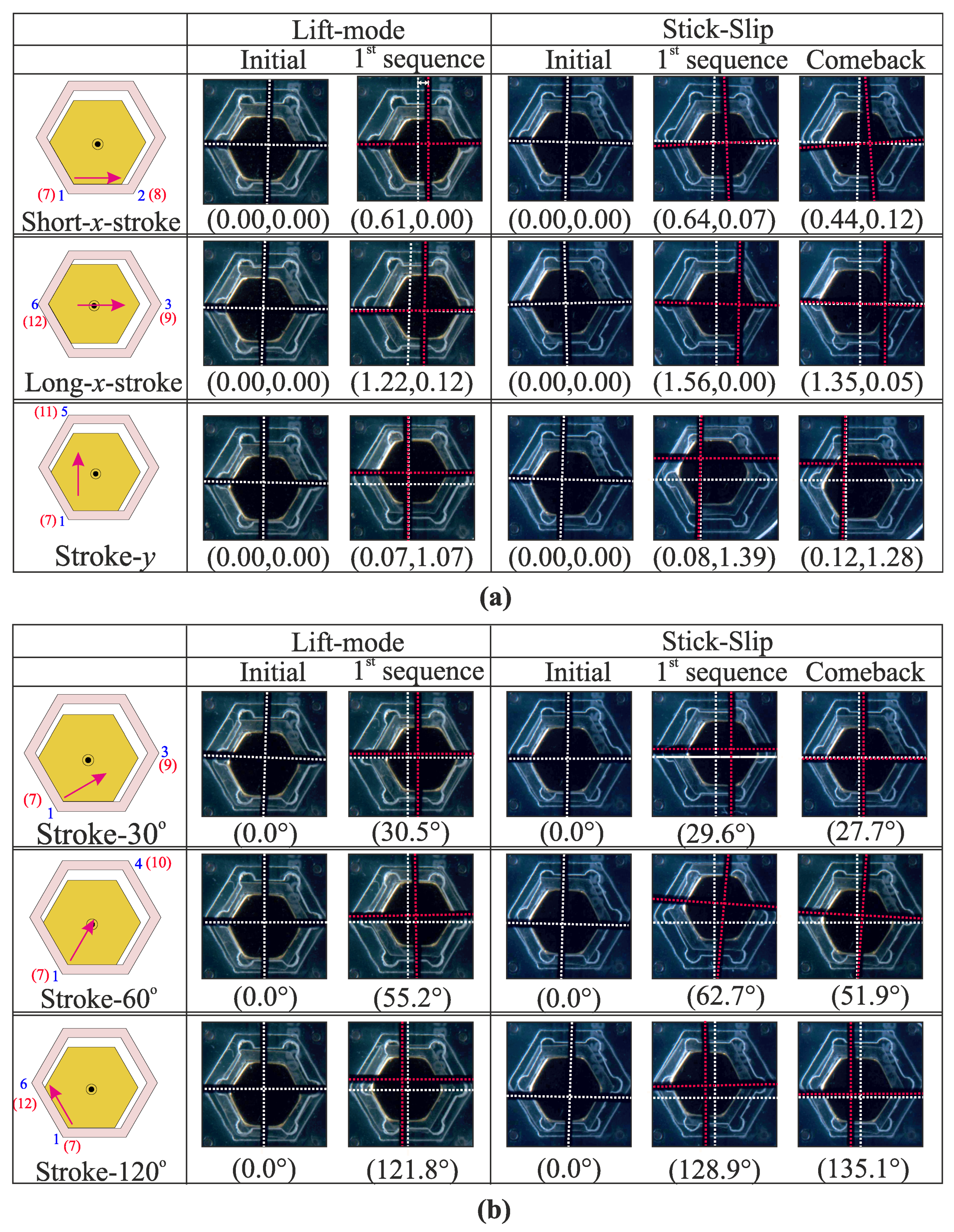
2.2.1. Stick-Slip Approach
2.2.2. Lift-Mode Approach
3. Modeling of the Positioning Device
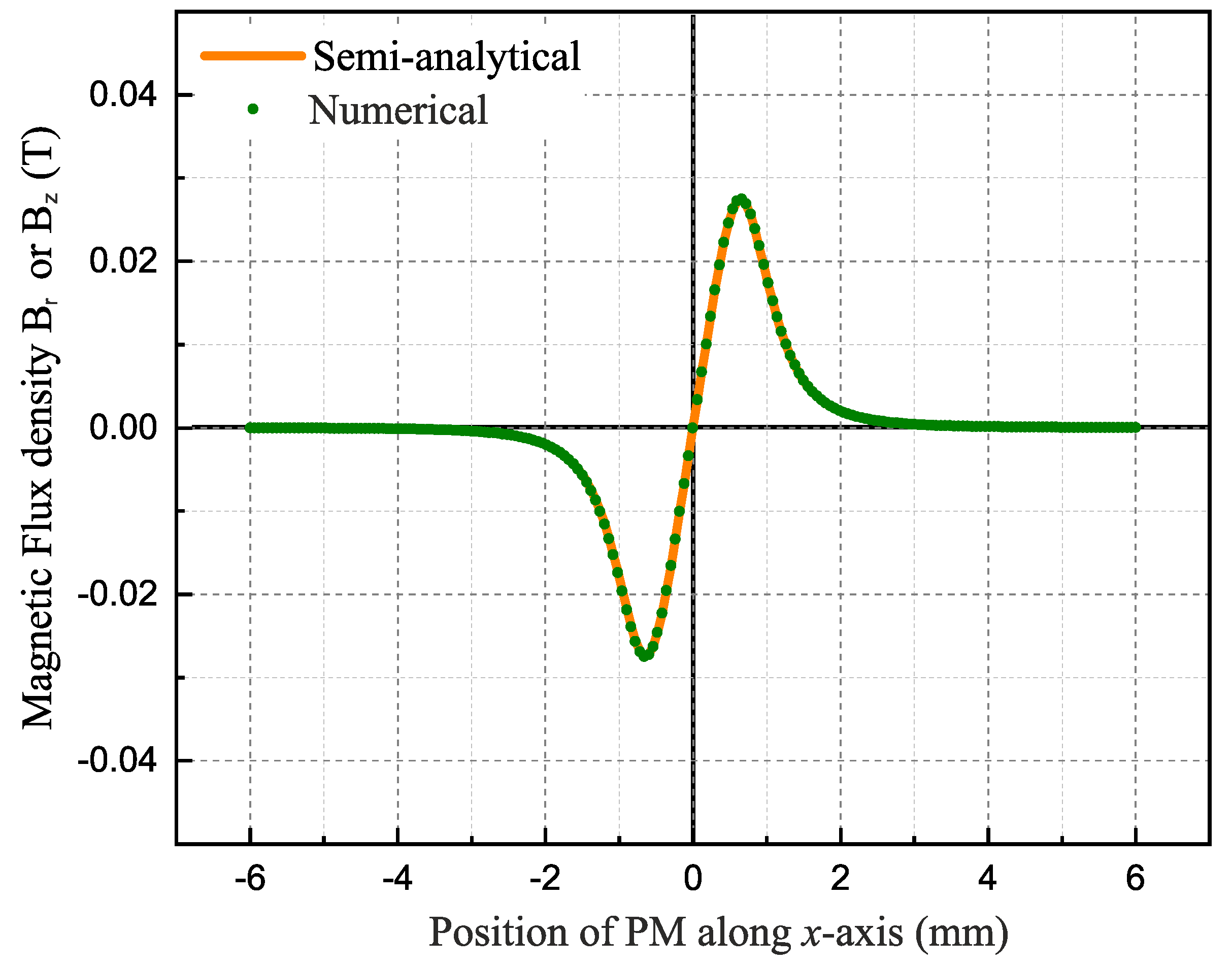
3.1. Magnetic Flux Density Calculation
3.2. Force Calculation
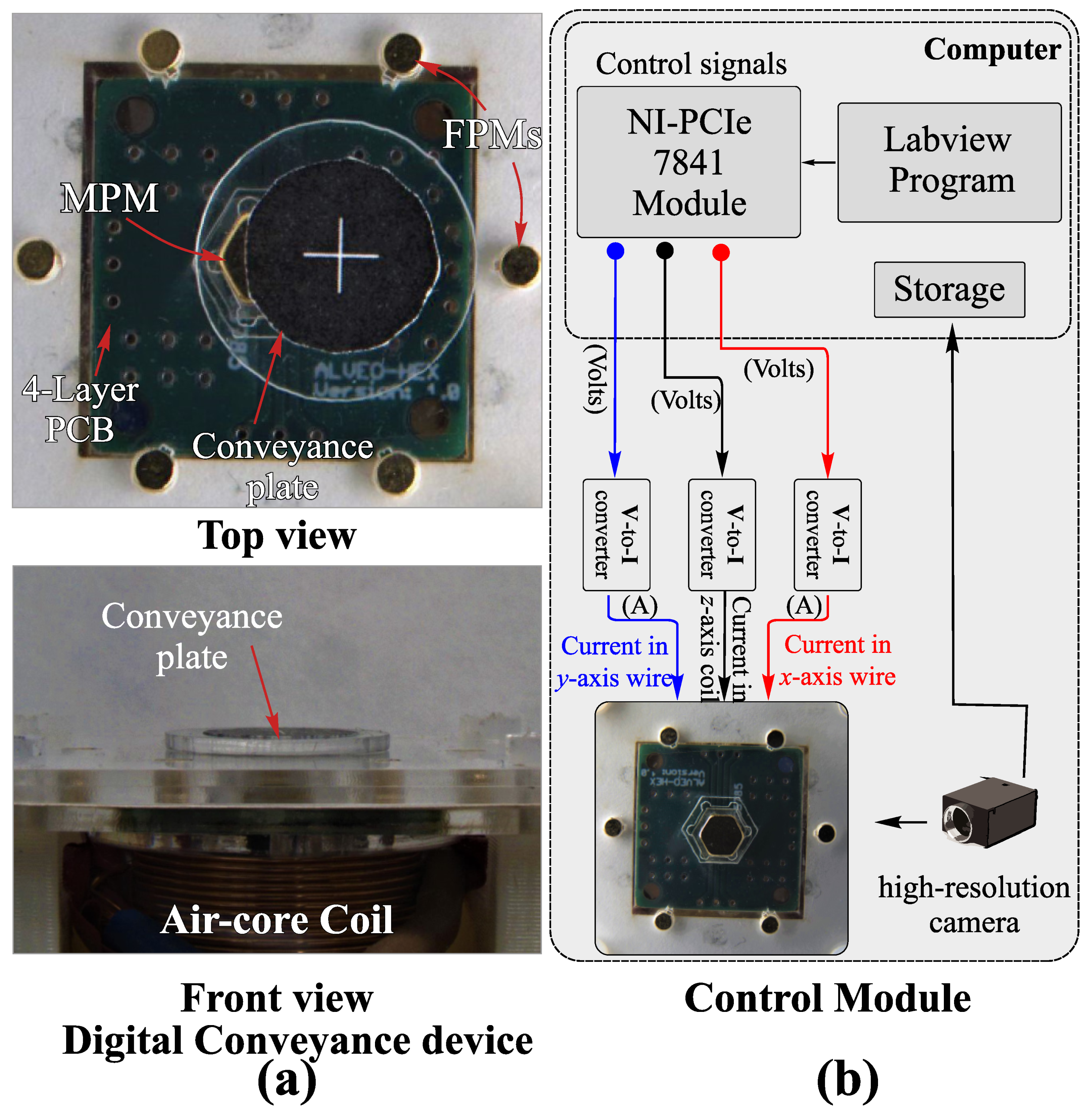
4. Experimental Setup and Results
4.1. Experimental Setup and Control Module
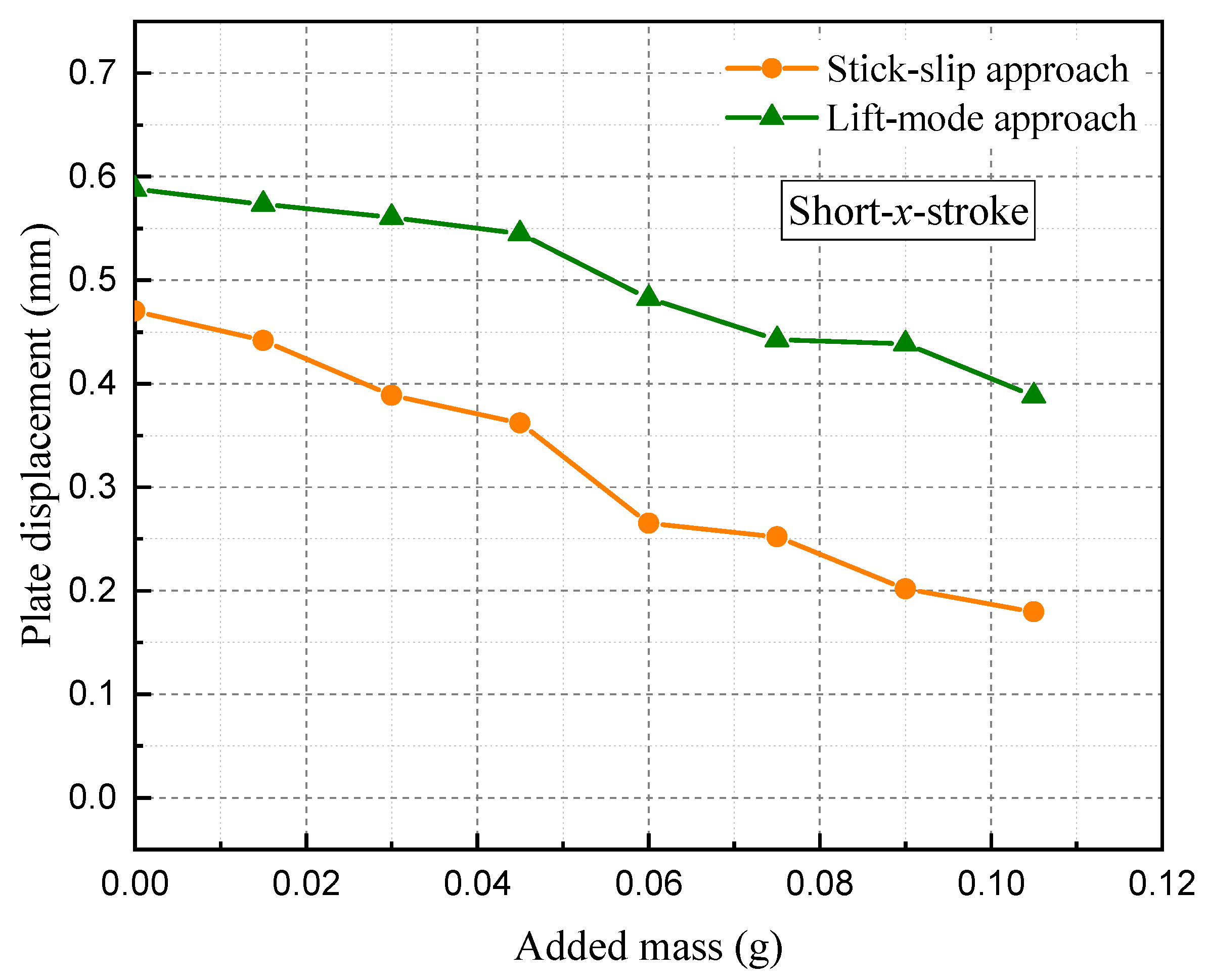
4.2. Results and Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Abbreviations
| MPM | Mobile Permanent Magnet |
| FPM | Fixed Permanent Magnet |
| PCB | Printed Circuit Board |
| NI | National Instruments |
References
- Arora, N.; Khan, M.U.; Petit, L.; Lamarque, F.; Prelle, C. Design and Development of a Planar Electromagnetic Conveyor for the Microfactory. IEEE/ASME Trans. Mechatron. 2019, 24, 1723–1731. [Google Scholar] [CrossRef]
- Ding, B.; Yang, Z.X.; Xiao, X.; Zhang, G. Design of Reconfigurable Planar Micro-Positioning Stages Based on Function Modules. IEEE Access 2019, 7, 15102–15112. [Google Scholar] [CrossRef]
- Wang, T.; Li, Y.; Zhang, Y.; Lin, R.; Qian, J.; Dou, Z. Design of a flexure-based parallel XY micropositioning stage with millimeter workspace and high bandwidth. Sens. Actuators A Phys. 2021, 331, 112899. [Google Scholar] [CrossRef]
- Singh, Y.; Shah, S.P.; Gandhi, P.S. High resolution flexible 4-PPR U-base planar parallel microstage robotic manipulator. IOP Conf. Ser. Mater. Sci. Eng. 2018, 402, 012034. [Google Scholar] [CrossRef]
- Lv, X.; Wei, W.; Mao, X.; Chen, Y.; Yang, J.; Yang, F. A novel MEMS electromagnetic actuator with large displacement. Sens. Actuators A Phys. 2015, 221, 22–28. [Google Scholar] [CrossRef]
- Henriksson, J.; Gullo, M.; Brugger, J. Integrated long-range thermal bimorph actuators for parallelizable Bio-AFM applications. IEEE Sens. J. 2013, 13, 2849–2856. [Google Scholar] [CrossRef][Green Version]
- Tellers, M.C.; Pulskamp, J.S.; Bedair, S.S.; Rudy, R.Q.; Kierzewski, I.M.; Polcawich, R.G.; Bergbreiter, S.E. Piezoelectric actuator array for motion-enabled reconfigurable RF circuits. In Proceedings of the 2015 Transducers—2015 18th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Anchorage, AK, USA, 21–25 June 2015; pp. 819–822. [Google Scholar] [CrossRef]
- Skandani, A.A.; Chatterjee, S.; Smith, M.L.; Baranski, J.; Wang, D.H.; Tan, L.S.; White, T.J.; Shankar, M.R. Discrete-state photomechanical actuators. Extrem. Mech. Lett. 2016, 9, 45–54. [Google Scholar] [CrossRef]
- Okyay, A.; Erkorkmaz, K.; Khamesee, M.B. Mechatronic design, actuator optimization, and control of a long stroke linear nano-positioner. Precis. Eng. 2018, 52, 308–322. [Google Scholar] [CrossRef]
- Wang, R.; Zhang, X. A planar 3-DOF nanopositioning platform with large magnification. Precis. Eng. 2016, 46, 221–231. [Google Scholar] [CrossRef]
- Shi, Z.; Bélier, B.; Martincic, E.; Petit, L.; Moulin, J.; Lefeuvre, E.; Terrien, J.; Prelle, C.; Lamarque, F. Development of a 2D array of micromachined electromagnetic digital actuators for micro-conveyance applications. Microsyst. Technol. 2018, 24, 411–417. [Google Scholar] [CrossRef]
- Deshmukh, A.A.; Petit, L.; Khan, M.U.; Lamarque, F.; Prelle, C. A novel 3D electromagnetic digital actuator with 12 discrete positions. IEEE/ASME Trans. Mechatron. 2018, 23, 1653–1661. [Google Scholar] [CrossRef]
- Tisnés, S.D.; Petit, L.; Prelle, C.; Lamarque, F. Modeling and Experimental Validation of a Planar Microconveyor Based on a 2 x 2 Array of Digital Electromagnetic Actuators. IEEE/ASME Trans. Mechatron. 2021, 26, 1422–1432. [Google Scholar] [CrossRef]
- Roemer, D.B.; Bech, M.M.; Johansen, P.; Pedersen, H.C. Optimum Design of a Moving Coil Actuator for Fast-Switching Valves in Digital Hydraulic Pumps and Motors. IEEE/ASME Trans. Mechatron. 2015, 20, 2761–2770. [Google Scholar] [CrossRef]
- Chin, C.S.; Wheeler, C. Sliding-Mode Control of an Electromagnetic Actuated Conveyance System Using Contactless Sensing. IEEE Trans. Ind. Electron. 2013, 60, 5315–5324. [Google Scholar] [CrossRef]
- Russo, M.; Barrientos-Diez, J.; Axinte, D. A kinematic coupling mechanism with binary electromagnetic actuators for high-precision positioning. IEEE/ASME Trans. Mechatron. 2021, 1. [Google Scholar] [CrossRef]
- Zhi, C.; Shinshi, T.; Saito, M.; Kato, K. Planar-type micro-electromagnetic actuators using patterned thin film permanent magnets and mesh type coils. Sens. Actuators A Phys. 2014, 220, 365–372. [Google Scholar] [CrossRef]
- Li, X.; Zhao, Y.; Hu, T. Design of a novel electrothermal actuator for integrated MEMS safety-and-arming devices. In Proceedings of the 10th IEEE International Conference on Nano/Micro Engineered and Molecular Systems, Xi’an, China, 7–11 April 2015; pp. 63–66. [Google Scholar] [CrossRef]
- Thachil, G.; Nair, D.R.; DasGupta, A. Design and Fabrication of Reliable Power Efficient Bistable MEMS Switch Using Single Mask Process. J. Microelectromech. Syst. 2020, 29, 1225–1233. [Google Scholar] [CrossRef]
- Cao, Y.; Dong, J. High-performance low-voltage soft electrothermal actuator with directly printed micro-heater. Sens. Actuators A Phys. 2019, 297, 111546. [Google Scholar] [CrossRef]
- Zeng, Z.; Jin, H.; Zhang, L.; Zhang, H.; Chen, Z.; Gao, F.; Zhang, Z. Low-voltage and high-performance electrothermal actuator based on multi-walled carbon nanotube/polymer composites. Carbon 2015, 84, 327–334. [Google Scholar] [CrossRef]
- Ogden, S.; Jonsson, J.; Thornell, G.; Hjort, K. A latchable high-pressure thermohydraulic valve actuator. Sens. Actuators 2012, 188, 292–297. [Google Scholar] [CrossRef]
- Cheng, T.; He, M.; Li, H.; Lu, X.; Zhao, H.; Gao, H. A Novel Trapezoid-Type Stick Slip Piezoelectric Linear Actuator Using Right Circular Flexure Hinge Mechanism. IEEE Trans. Ind. Electron. 2017, 64, 5545–5552. [Google Scholar] [CrossRef]
- Wang, G.; Chen, G.; Zhou, H.; Bai, F. Modeling and tracking control for piezoelectric actuator based on a new asymmetric hysteresis model. IEEE/CAA J. Autom. Sin. 2017, 4, 782–791. [Google Scholar] [CrossRef]
- Wallenhauer, C.; Kappel, A.; Gottlieb, B.; Schwebel, T.; Lüth, T. Efficient class-B analog amplifier for a piezoelectric actuator drive. Mechatronics 2009, 19, 56–64. [Google Scholar] [CrossRef]
- Abadie, J.; Chaillet, N.; Lexcellent, C. Modeling of a new SMA micro-actuator for active endoscopy applications. Mechatronics 2009, 19, 437–442. [Google Scholar] [CrossRef]
- Wang, S.; Rong, W.; Wang, L.; Xie, H.; Sun, L.; Mills, J.K. A novel linear-rotary piezoelectric positioning stage based on surface’s rectangular trajectory driving. Precis. Eng. 2019, 55, 376–380. [Google Scholar] [CrossRef]
- Liu, Y.T.; Li, B.J. A 3-axis precision positioning device using PZT actuators with low interference motions. Precis. Eng. 2016, 46, 118–128. [Google Scholar] [CrossRef]
- Dao, D.V.; Pham, P.H.; Senkawa, S.; Sugiyama, S. Tangential and perpendicular driving micro transmission systems based on ratchet mechanism and electrostatic actuator. In Proceedings of the TRANSDUCERS 2009—2009 International Solid-State Sensors, Actuators and Microsystems Conference, Denver, CO, USA, 21–25 June 2009; pp. 45–48. [Google Scholar] [CrossRef]
- Schmitt, L.; Schmitt, P.; Hoffmann, M. 3-Bit Digital-to-Analog Converter with Mechanical Amplifier for Binary Encoded Large Displacements. Actuators 2021, 10, 182. [Google Scholar] [CrossRef]
- Fukuta, Y.; Chapuis, Y.A.; Mita, Y.; Fujita, H. Design, fabrication, and control of MEMS-based actuator arrays for air-flow distributed micromanipulation. J. Microelectromech. Syst. 2006, 15, 912–926. [Google Scholar] [CrossRef]
- Conrad, H.; Kaiser, B.; Gaudet, M.; Langa, S.; Stolz, M.; Uhlig, S.; Schimmanz, K.; Schenk, H. A Novel Electrostatic Actuator Class. Precis. Eng. 2016, 168, 1533–1536. [Google Scholar] [CrossRef]
- Li, G.Y.; Li, X.Y.; Wang, H.; Yang, Z.Q.; Yao, J.Y.; Ding, G.F. Fabrication and characterization of superhydrophobic surface by electroplating regular rough micro-structures of metal nickel. Microelectron. Eng. 2012, 95, 130–134. [Google Scholar] [CrossRef]
- Uranga, A.; Verd, J.; Marigó, E.; Giner, J.; Muñóz-Gamarra, J.; Barniol, N. Exploitation of non-linearities in CMOS-NEMS electrostatic resonators for mechanical memories. Sens. Actuators A Phys. 2013, 197, 88–95. [Google Scholar] [CrossRef]
- Deshmukh, A.; Petit, L.; Khan, M.U.; Lamarque, F.; Prelle, C. Development of a six positions digital electromagnetic actuator. In Proceedings of the 2017 IEEE International Conference on Advanced Intelligent Mechatronics (AIM), Munich, Germany, 3–7 July 2017; pp. 975–980. [Google Scholar] [CrossRef]
- Petit, L.; Hajjar, H.A.; Prelle, C.; Lamarque, F. Design, Modeling, and Characterization of an Optical Switch Based on Four Positions Digital Actuator. IEEE/ASME Trans. Mechatron. 2016, 21, 1518–1527. [Google Scholar] [CrossRef]
- Flader, I.B.; Chen, Y.; Shin, D.D.; Heinz, D.B.; Ortiz, L.C.; Alter, A.L.; Park, W.; Goodson, K.E.; Kenny, T.W. Micro-tethering for in-process stiction mitigation of highly compliant structures. In Proceedings of the 2017 IEEE 30th International Conference on Micro Electro Mechanical Systems (MEMS), Las Vegas, NV, USA, 22–26 January 2017; pp. 675–678. [Google Scholar] [CrossRef]
- Kumar, B.V.R.; Sivakumar, K.; Rao, Y.S.; Karunanidhi, S. Design of a New Electromagnetic Brake for Actuator Locking Mechanism in Aerospace Vehcile. IEEE Trans. Magn. 2017, 53, 8002606. [Google Scholar] [CrossRef]
- Furlani, E.P. Permanent Magnet and Electromechanical Devices; Furlani, E.P., Ed.; Academic Press: San Diego, CA, USA, 2001. [Google Scholar]
- Xu, F.; Lv, Y.; Xu, X.; Dinavahi, V. FPGA-Based real-time wrench model of direct current driven magnetic levitation actuator. IEEE Trans. Ind. Electron. 2018, 65, 9635–9645. [Google Scholar] [CrossRef]
- Reza, M.M.; Ahmad, A.; Kumar, P.; Srivastava, R.K. Semi-analytical model for triangular skewed permanent magnet axial flux machine. In Proceedings of the 2017 IEEE Transportation Electrification Conference (ITEC-India), Pune, India, 13–15 December 2017. [Google Scholar] [CrossRef]
- Rubeck, C.; Yonnet, J.P.; Allag, H.; Delinchant, B.; Chadebec, O. Analytical Calculation of Magnet Systems: Magnetic Field Created by Charged Triangles and Polyhedra. IEEE Trans. Magn. 2013, 49, 144–147. [Google Scholar] [CrossRef]





| Permanent Magnets | ||
|---|---|---|
| MPM | FPM | |
| Shape | Hexagon | Cylinder |
| Dimensions | 5.5 mm (side) × 2 mm | Ø2.25 × 2 mm |
| Material | NdFeB | NdFeB |
| Magnetization | 1.40 T | 1.37 T |
| Mechanical structure and coil | ||
| Material | PMMA | |
| Top plate dimensions | 40 mm × 40 mm × 1 mm | |
| Second plate dimensions | 40 mm × 40 mm × 1 mm | |
| Coil | Ø 20 mm × 10 mm | |
| Ø 9 mm | ||
| Distances | ||
| FPM to cavity center | 12.88 mm | |
| x-switching wires to MPM | 0.2 mm | |
| y-switching wires to MPM | 0.3 mm | |
| Stroke values | ||
| Short-x-stroke | 0.6 mm | |
| Long-x-stroke | 1.20 mm | |
| y-Stroke | 1.04 mm | |
| z-Stroke | 0.5 mm | |
| Absolute Values of Magnetic Forces | ||||
|---|---|---|---|---|
| Short-x-Stroke | Long-x-Stroke | y-Stroke | z-Stroke | |
| z level | 0.95 mN | 1.91 mN | 1.64 mN | 0 mN |
| z level | 0.93 mN | 1.87 mN | 1.61 mN | 3.18 mN |
| Absolute Values of Electromagnetic Forces (For 1 A) | ||||
| Short-x-Stroke | Long-x-Stroke | y-Stroke | z-Stroke | |
| (F) | ||||
| z level | 4.58 mN | 4.58 mN | 4.27 mN | 0.72 mN |
| z level | 3.48 mN | 3.48 mN | 3.23 mN | 21.39 mN |
| Displacement Directions | Theoretical Stroke (mm) | Experimental Stroke (mm) |
|---|---|---|
| Short-x-stroke | 0.600 | 0.635 ± 0.004 |
| Long-x-stroke | 1.200 | 1.216 ± 0.003 |
| y-Stroke | 1.040 | 1.072 ± 0.006 |
| z-Stroke | 0.500 | 0.545 ± 0.002 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deshmukh, A.; Petit, L.; Khan, M.-u.; Lamarque, F.; Prelle, C. Planar Micro-Positioning Device Based on a 3D Digital Electromagnetic Actuator. Actuators 2021, 10, 310. https://doi.org/10.3390/act10120310
Deshmukh A, Petit L, Khan M-u, Lamarque F, Prelle C. Planar Micro-Positioning Device Based on a 3D Digital Electromagnetic Actuator. Actuators. 2021; 10(12):310. https://doi.org/10.3390/act10120310
Chicago/Turabian StyleDeshmukh, Ajinkya, Laurent Petit, Muneeb-ullah Khan, Frédéric Lamarque, and Christine Prelle. 2021. "Planar Micro-Positioning Device Based on a 3D Digital Electromagnetic Actuator" Actuators 10, no. 12: 310. https://doi.org/10.3390/act10120310
APA StyleDeshmukh, A., Petit, L., Khan, M.-u., Lamarque, F., & Prelle, C. (2021). Planar Micro-Positioning Device Based on a 3D Digital Electromagnetic Actuator. Actuators, 10(12), 310. https://doi.org/10.3390/act10120310






