Research on Low-Speed Driving Model of Ultrasonic Motor Based on Beat Traveling Wave Theory
Abstract
:1. Introduction
2. Structure of the Stator
2.1. Equivalent Model and Stator
2.2. Natural Frequency Analysis
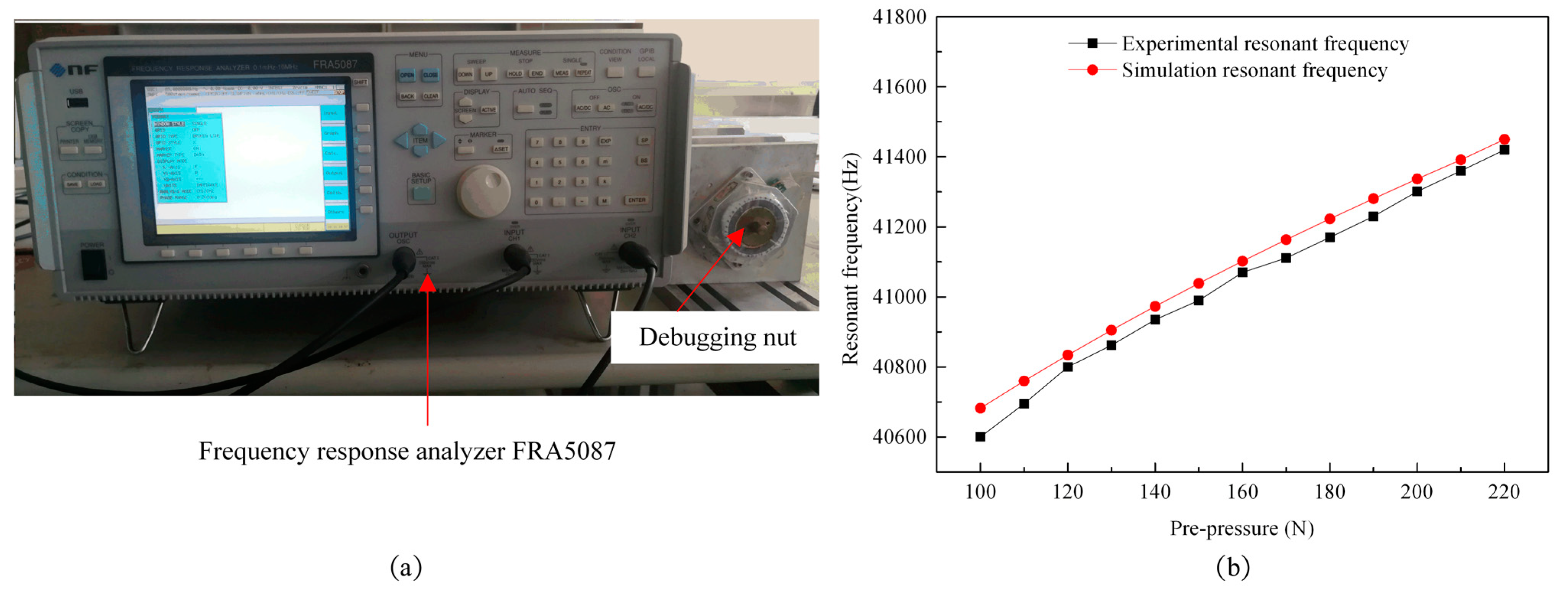
2.3. Amplitude Analysis under Prepressure
3. Theoretical Analysis of Ultrasonic Motor Driving Method
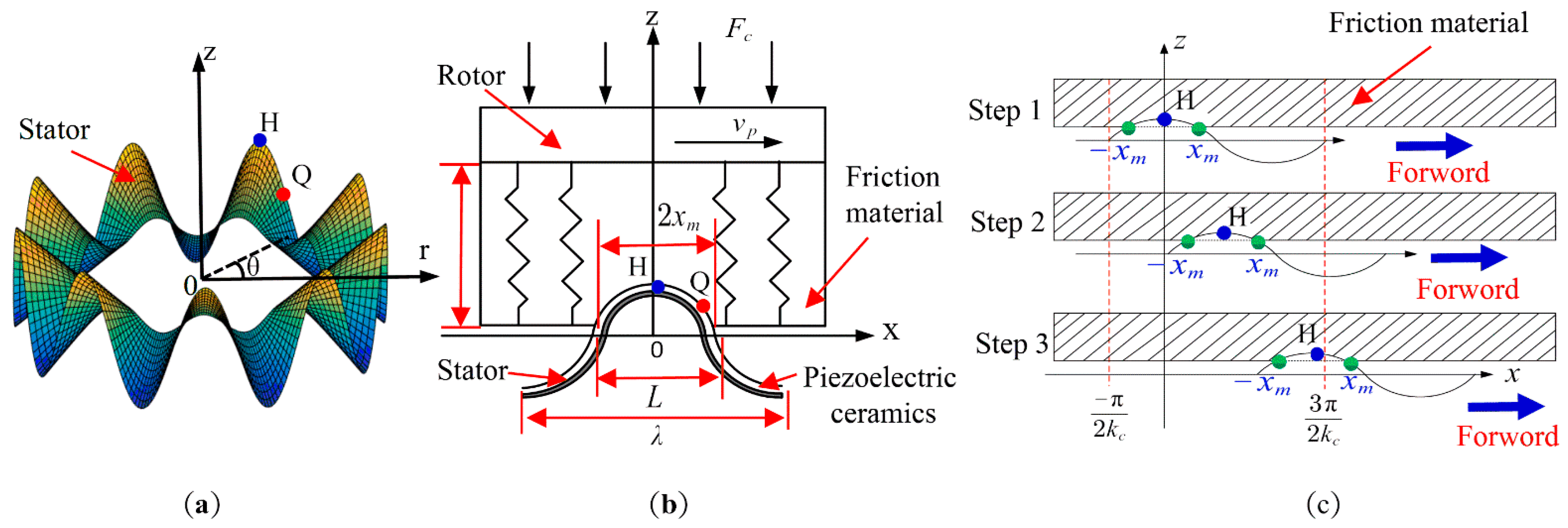
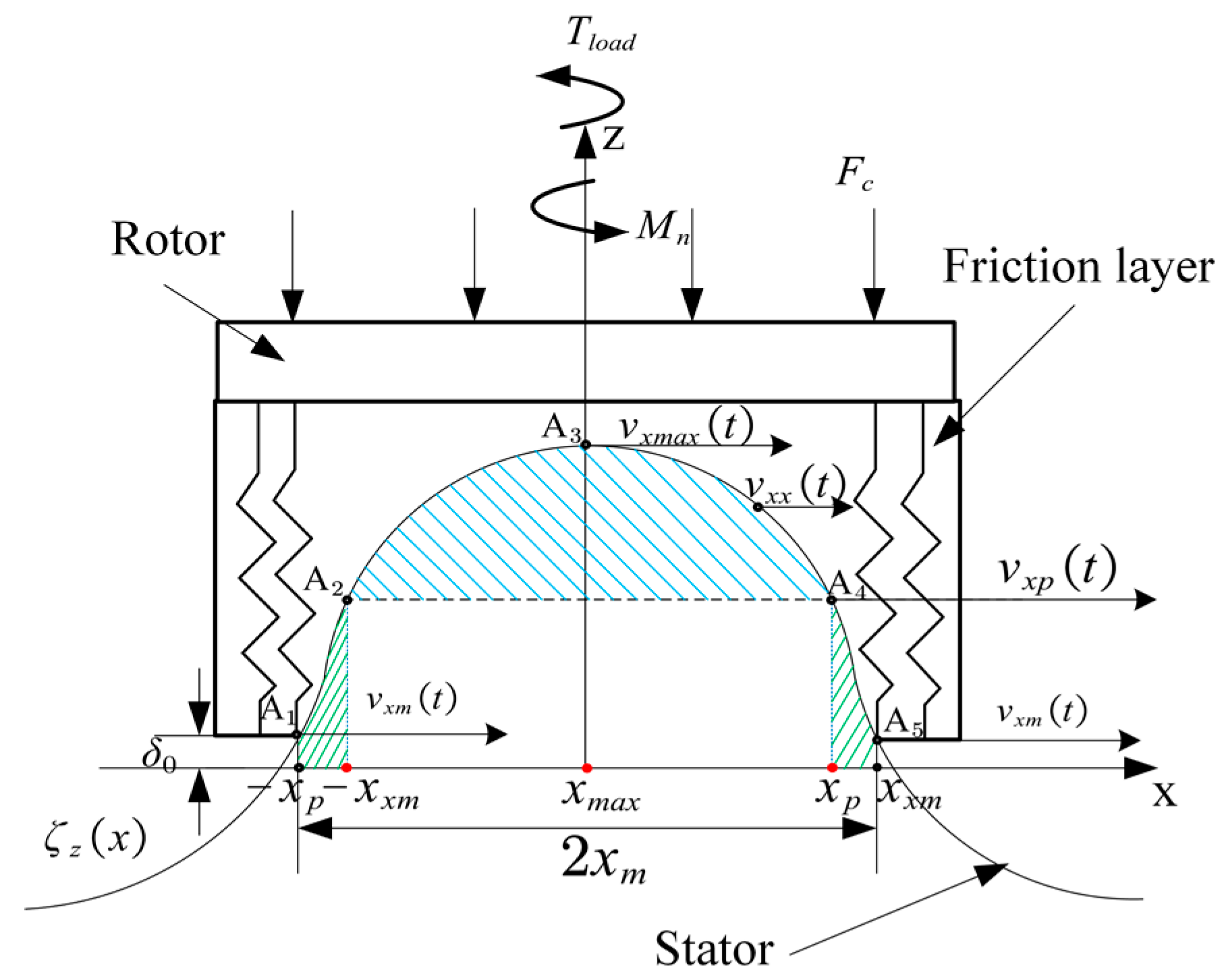
3.1. Stator Particle Point Setting
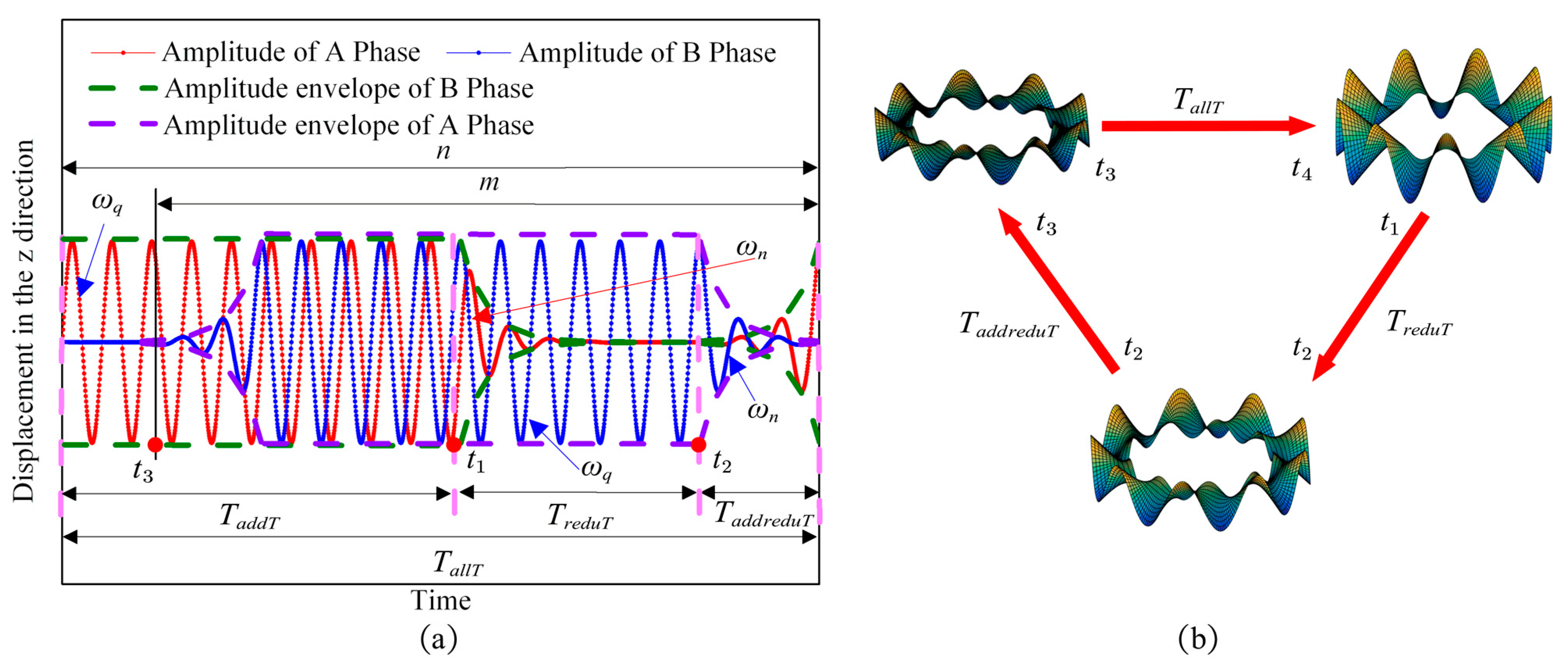
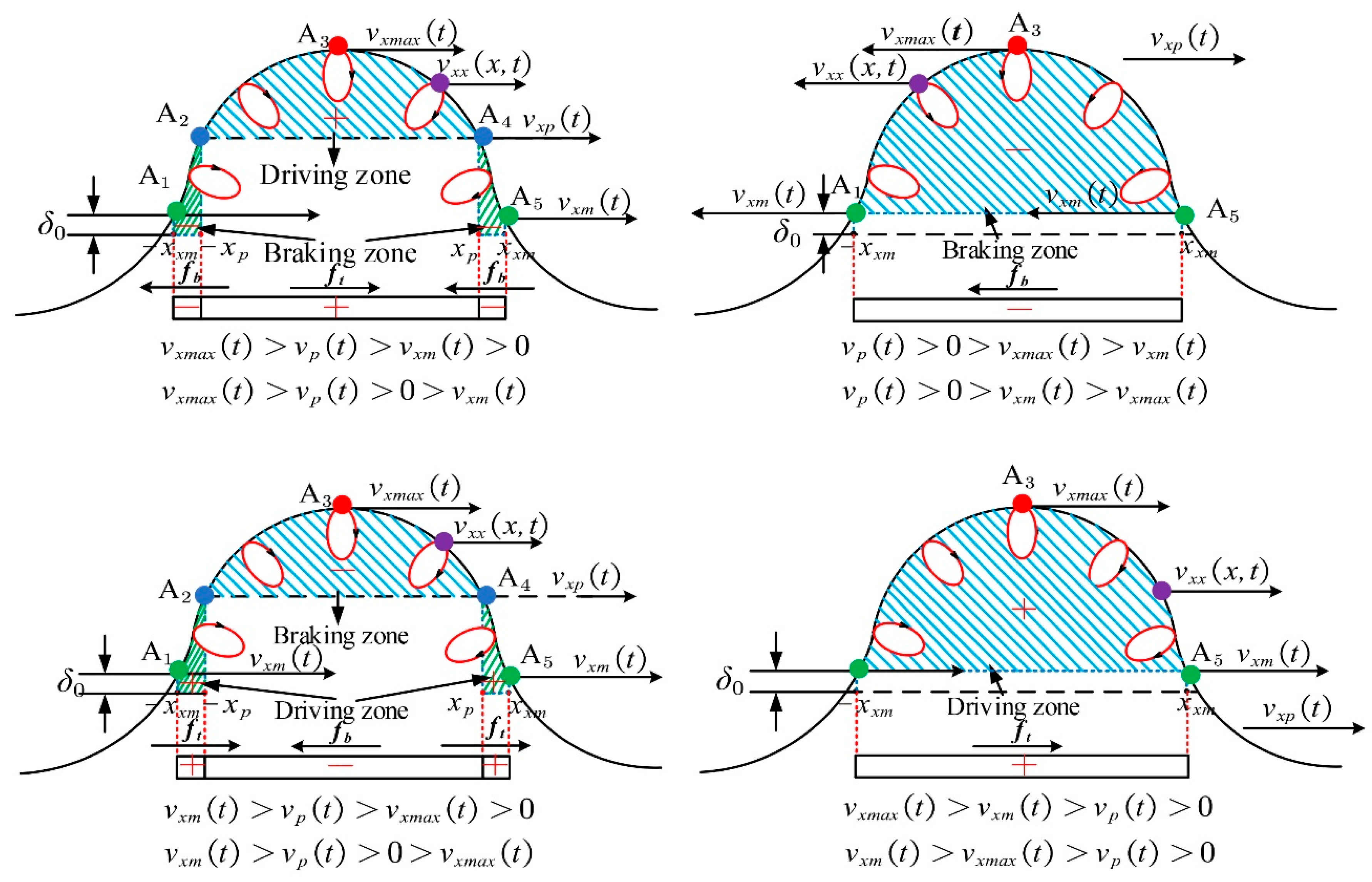
3.2. Partition Introduction to the Driving Method
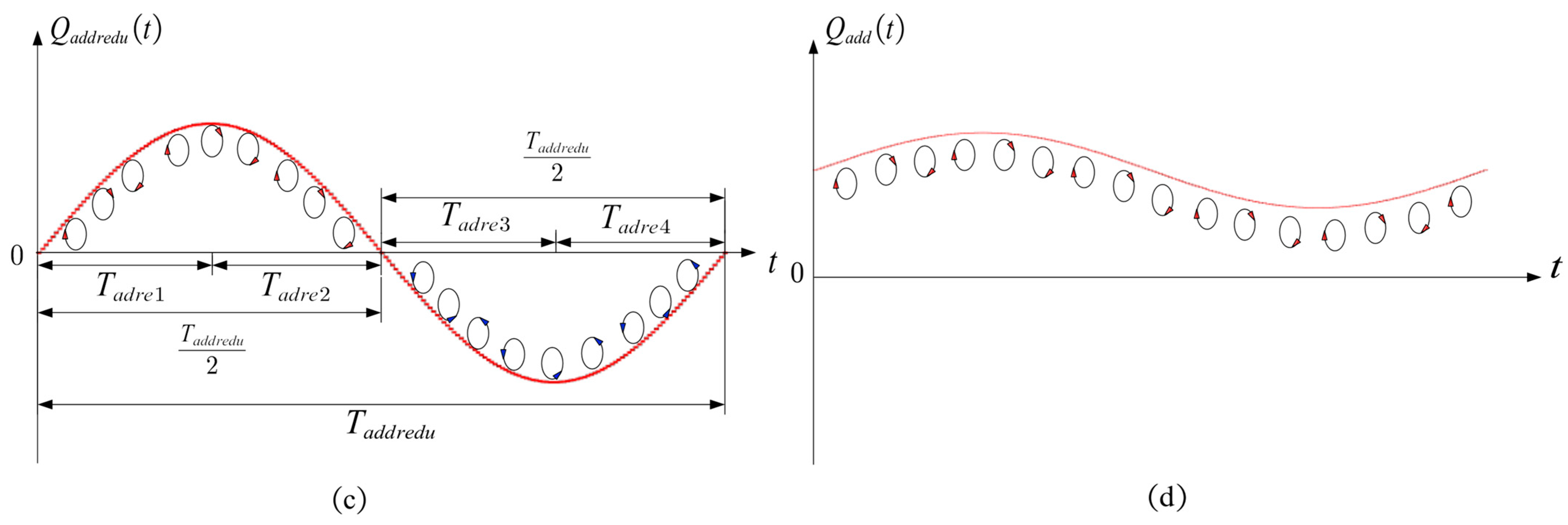
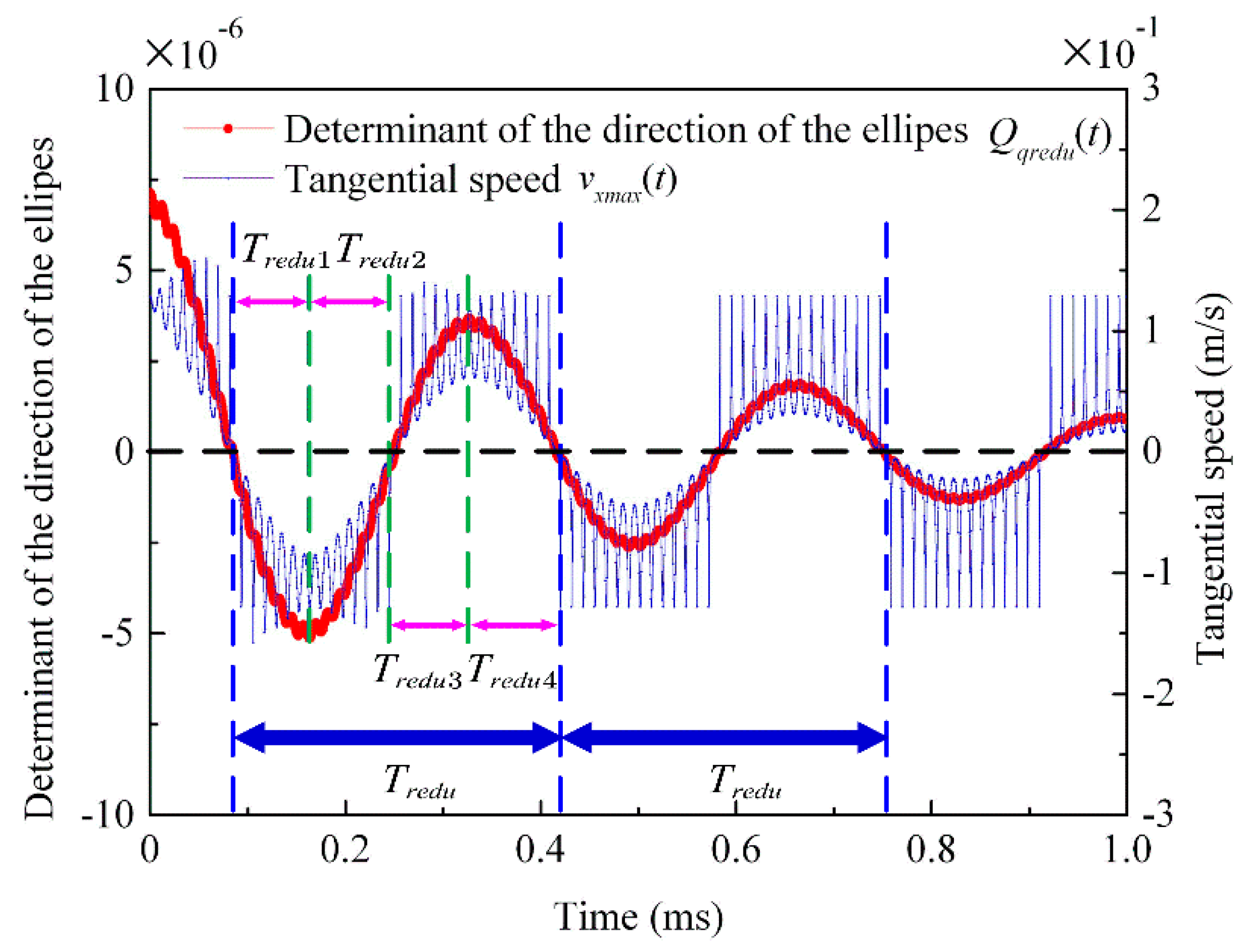
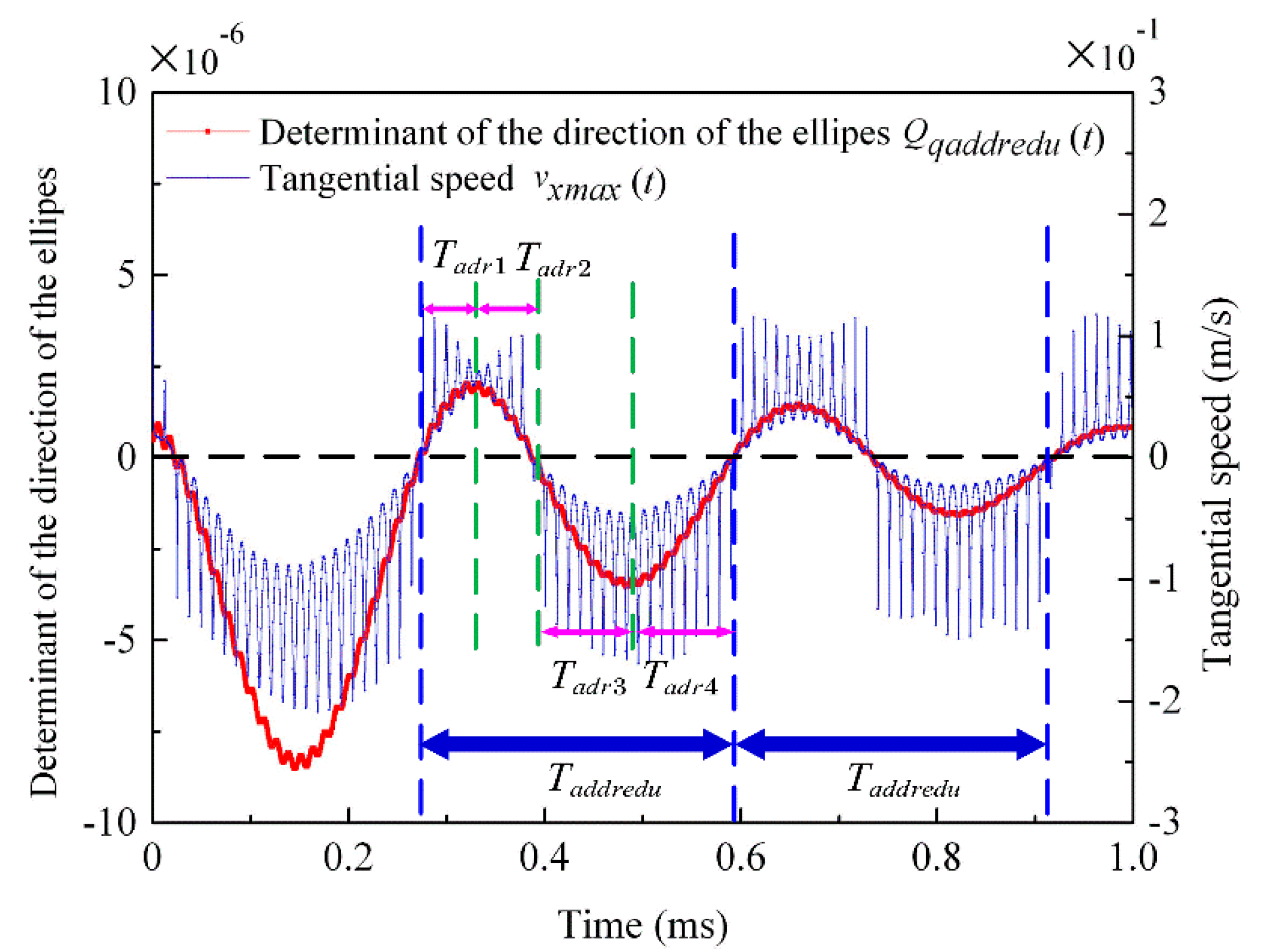
3.2.1. Traveling Standing Wave Amplitude Reduction Region ()
3.2.2. Traveling Standing Wave Amplitude Increasing and Decreasing Transition Region ()
3.2.3. The Traveling Standing Wave Increasing Region ()
3.3. Motion Characteristics of the Stator Particle Points in
3.4. Motion Characteristics of Particle Point H of the Stator Wave Crest
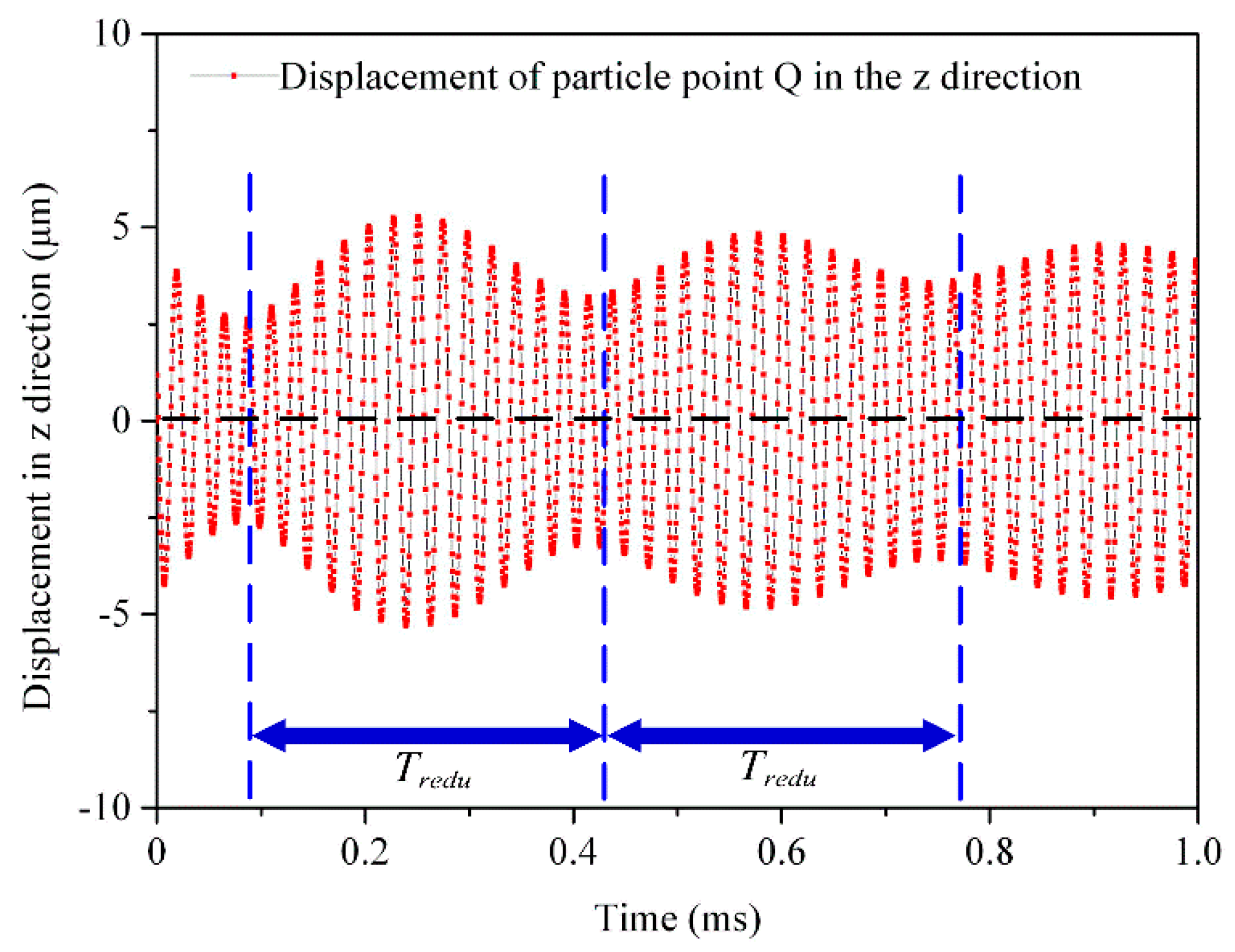
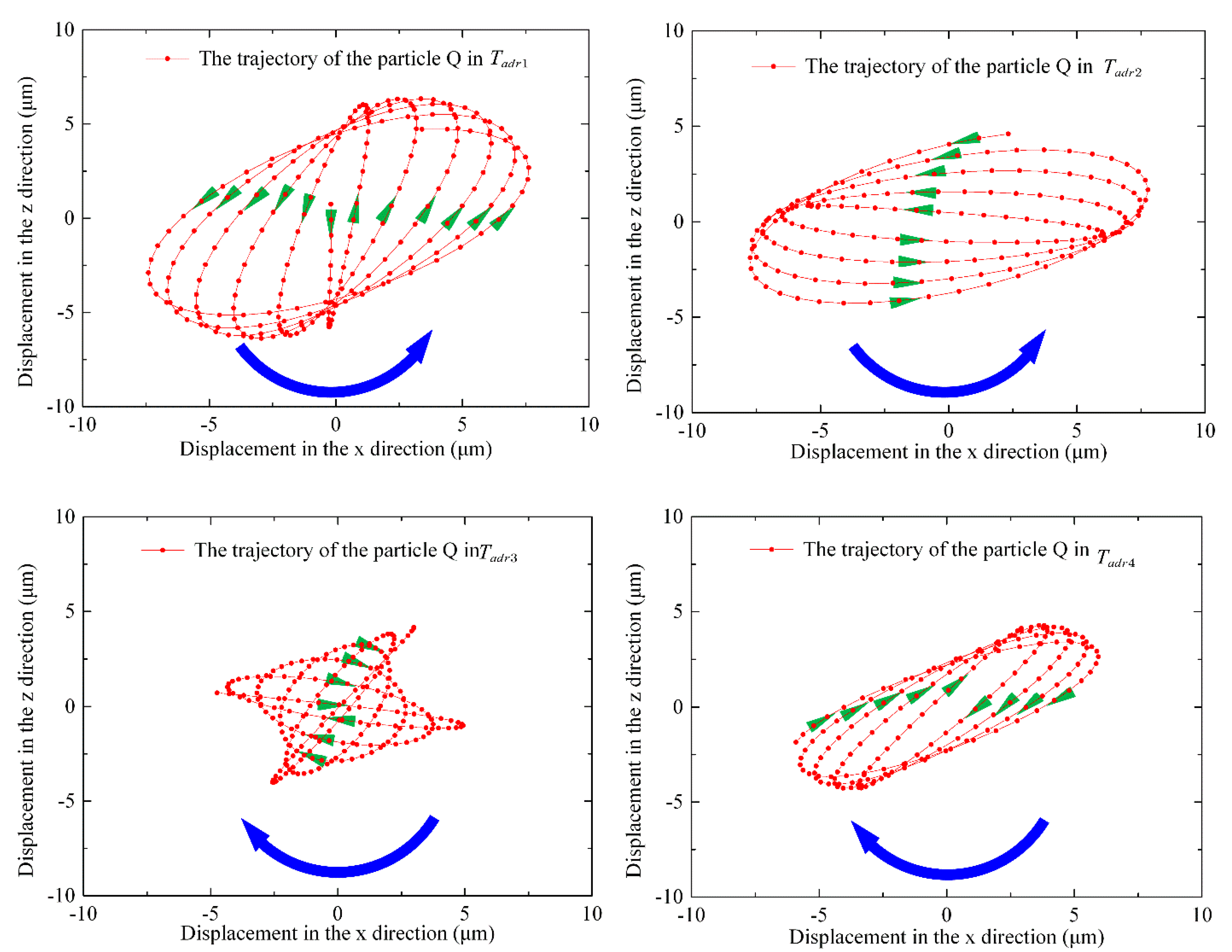
3.5. The Elliptical Trajectory of the Stator Particle Point Q
4. Stator and Rotor Contact Model and Transfer Model
4.1. Analysis of Stator and Rotor Contact Model
4.2. Transfer Model Analysis of the Stator and Rotor
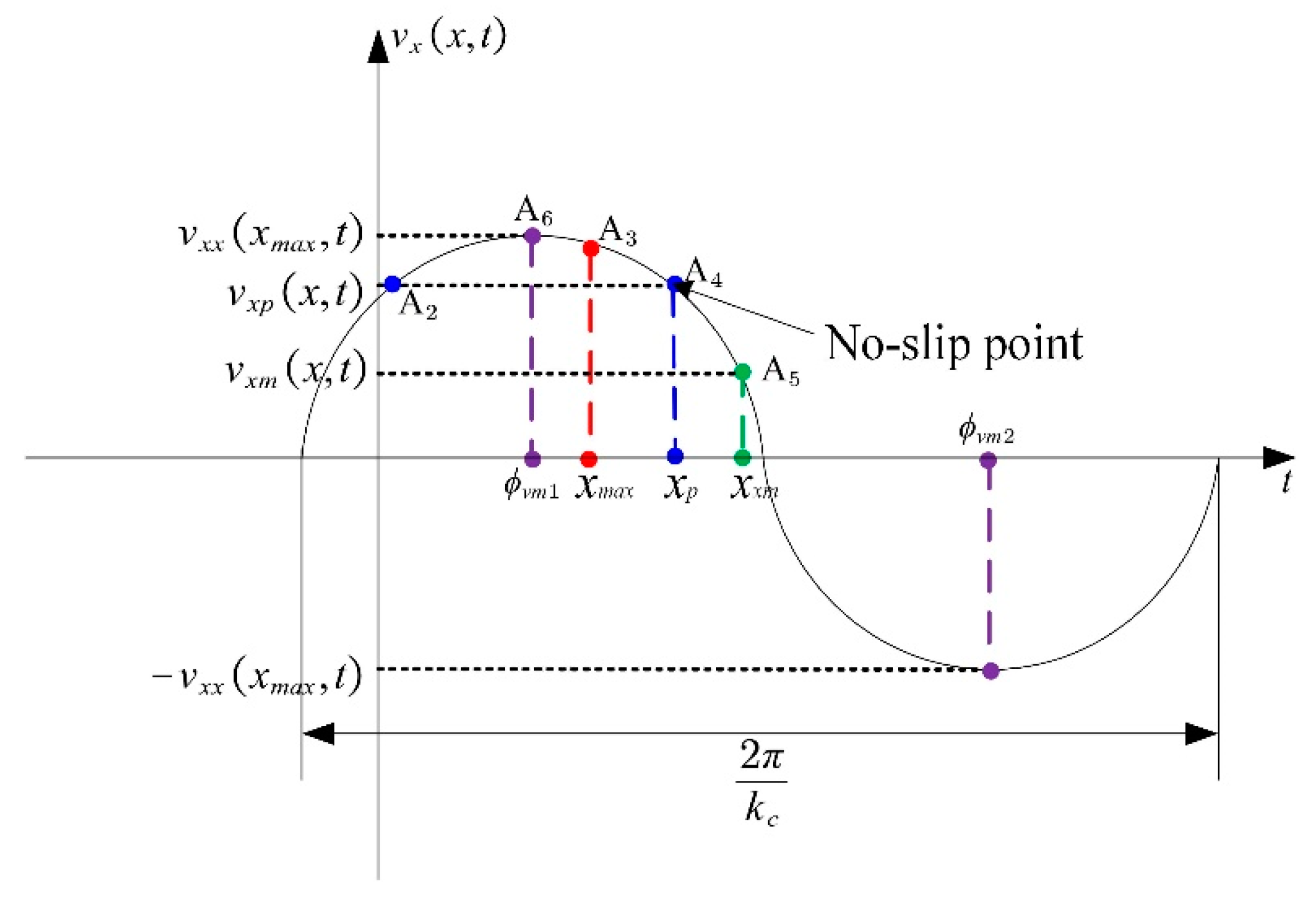
4.2.1. Analysis of the Nonslip Point
4.2.2. Position Value of Nonsliding Point
4.2.3. Analysis of Driving Torque
5. Simulation of Motion Characteristics of the Stator Particle Point
5.1. Motor Simulation Parameter Setting
5.2. Simulation of Particle Point Motion Characteristics
5.2.1. Simulation of Particle Point Motion Characteristics in
5.2.2. Simulation of the Particle Point Motion Characteristics in
5.2.3. Simulation of Particle Point Motion Characteristics in
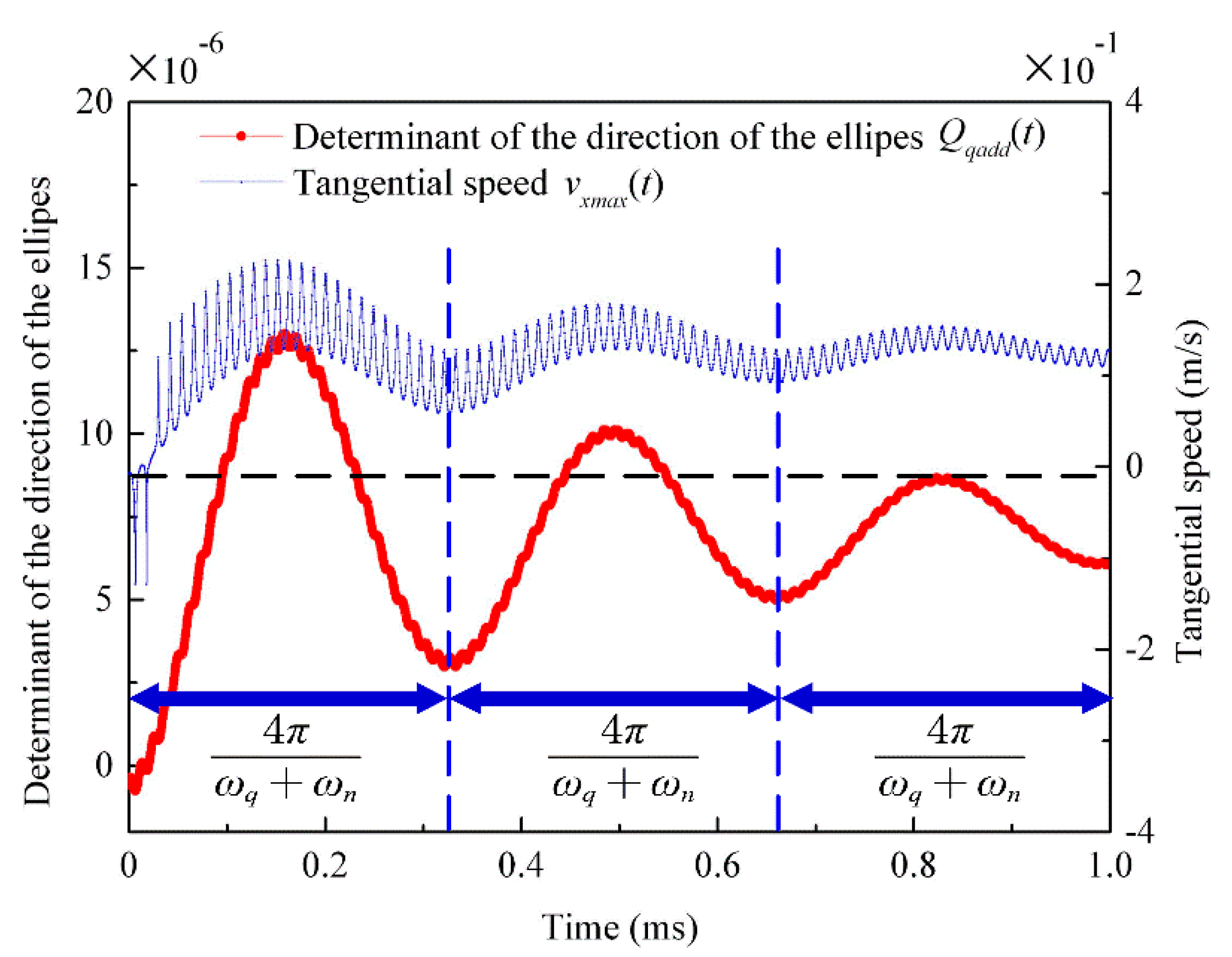
5.3. Simulation Characteristics of the Drive Method
6. Simulation of the Motor Output Characteristics
6.1. Setting of Characteristic Variables
6.2. Simulation of Motor Output Characteristics
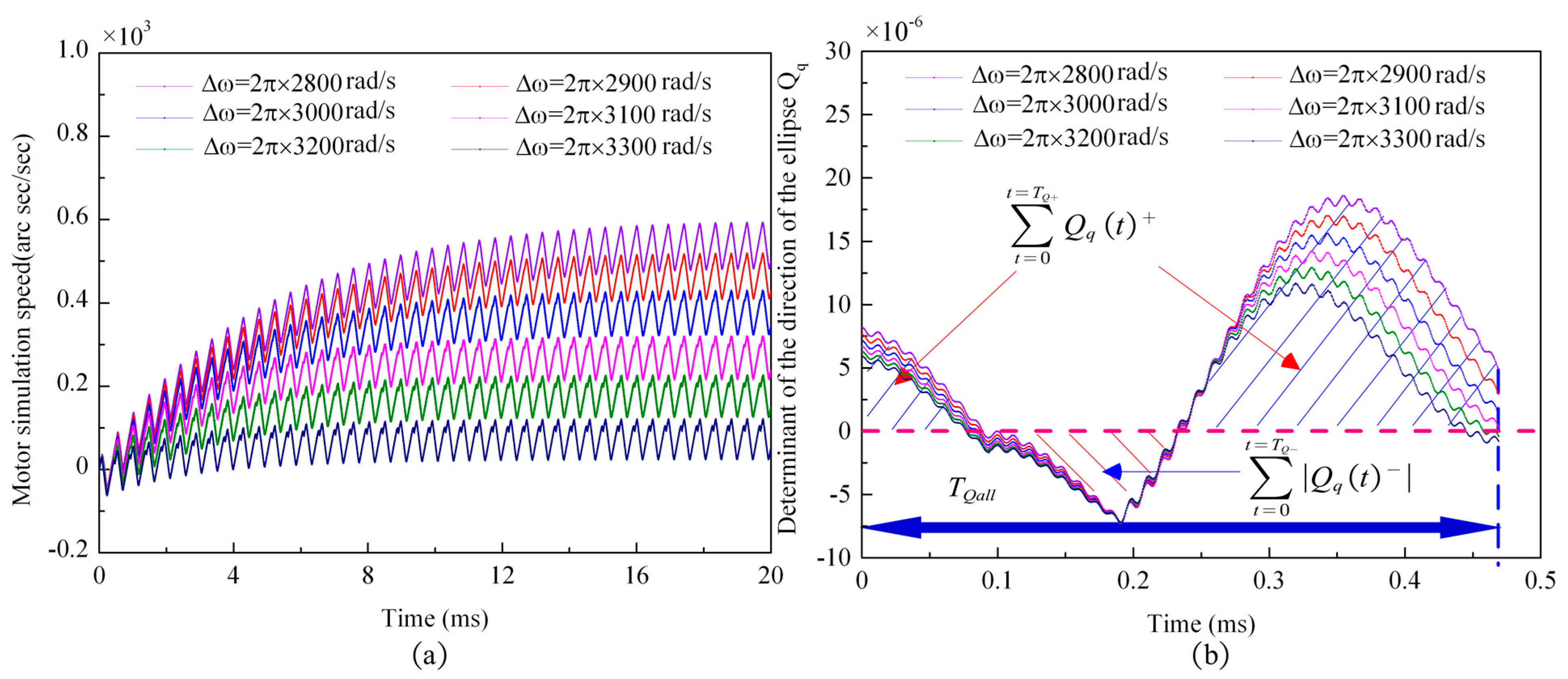
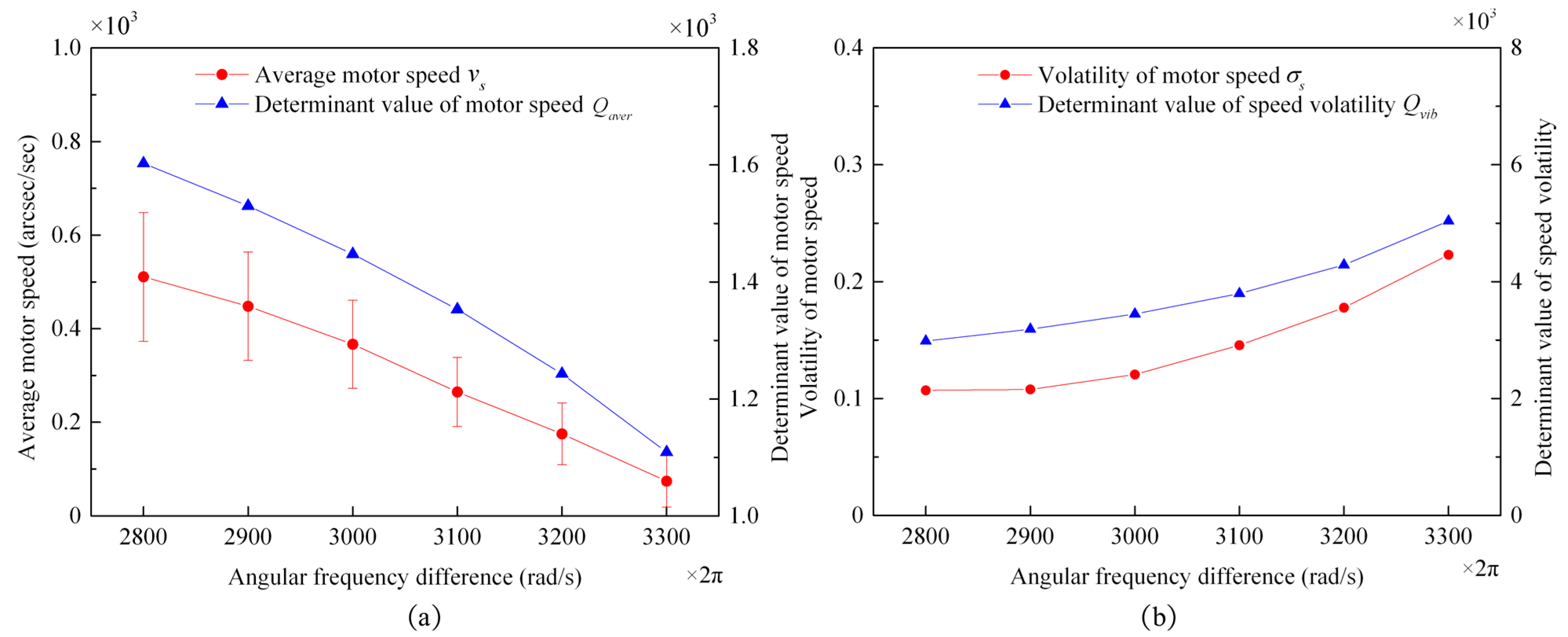
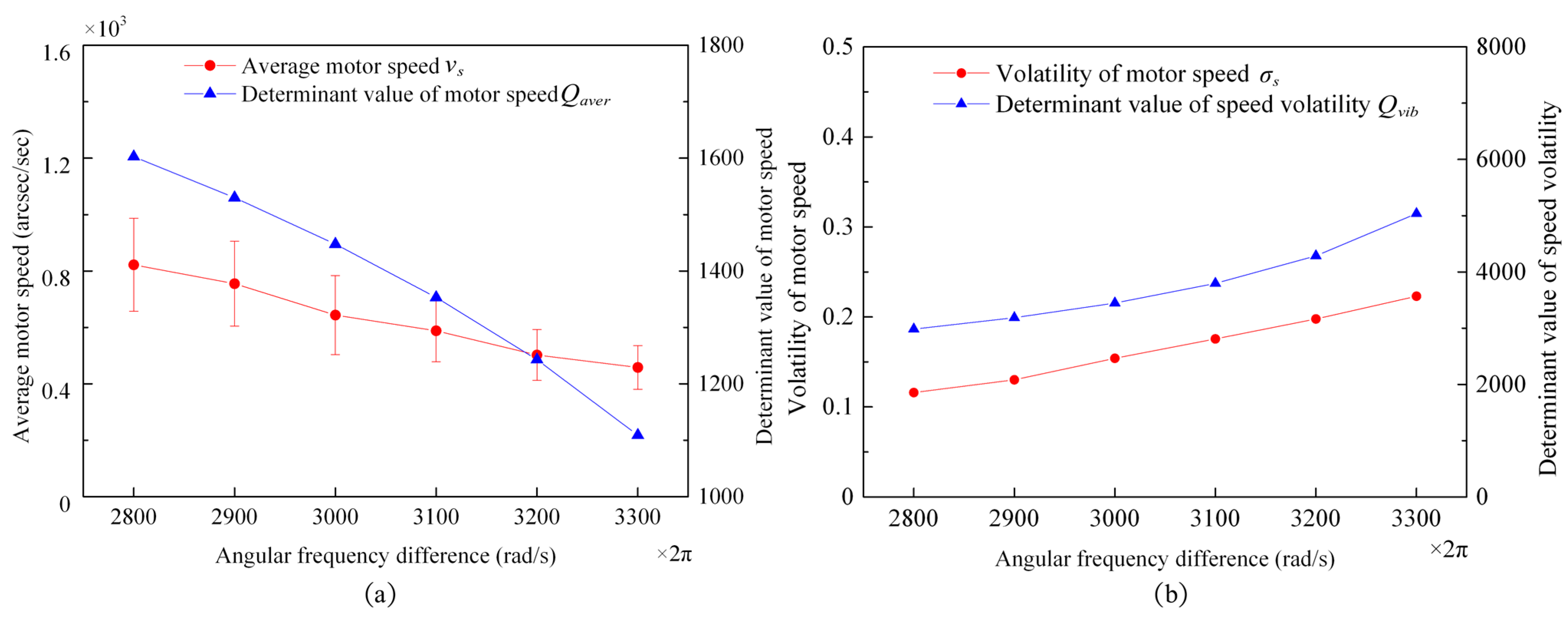
6.2.1. Simulation of Motor Speed and Determining Factor with Angular Frequency Difference
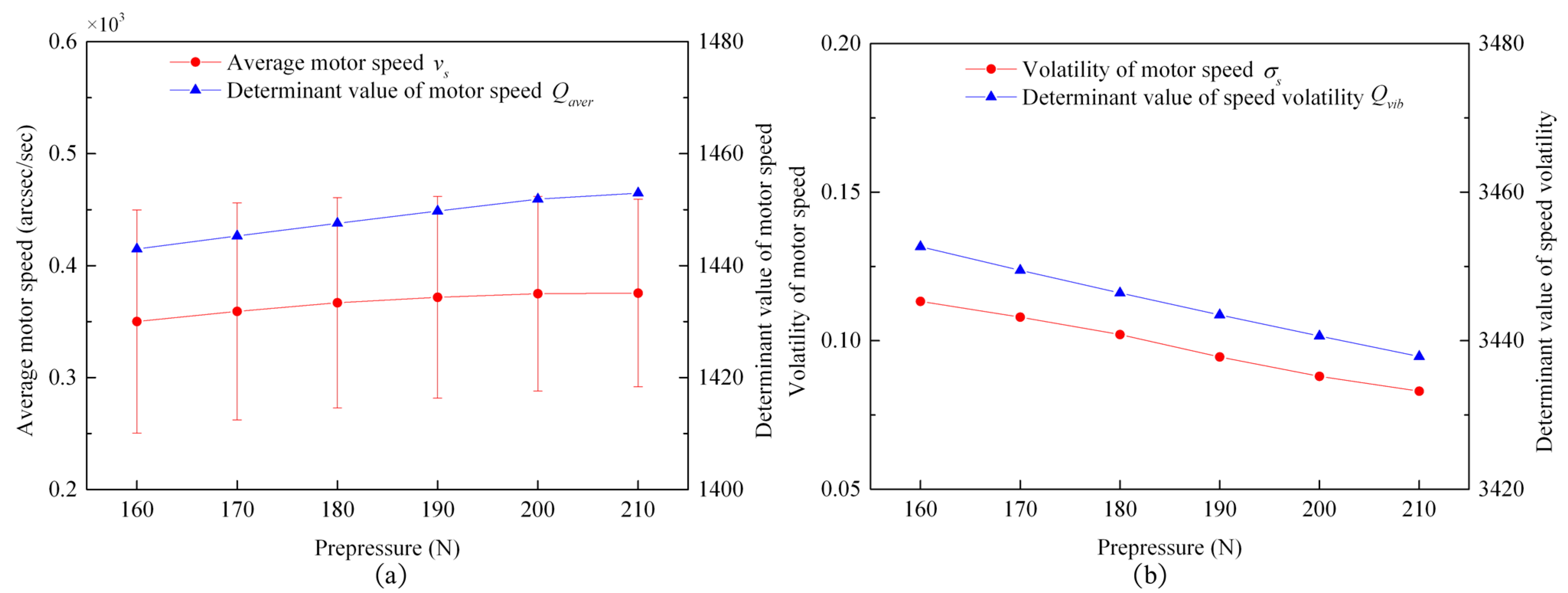
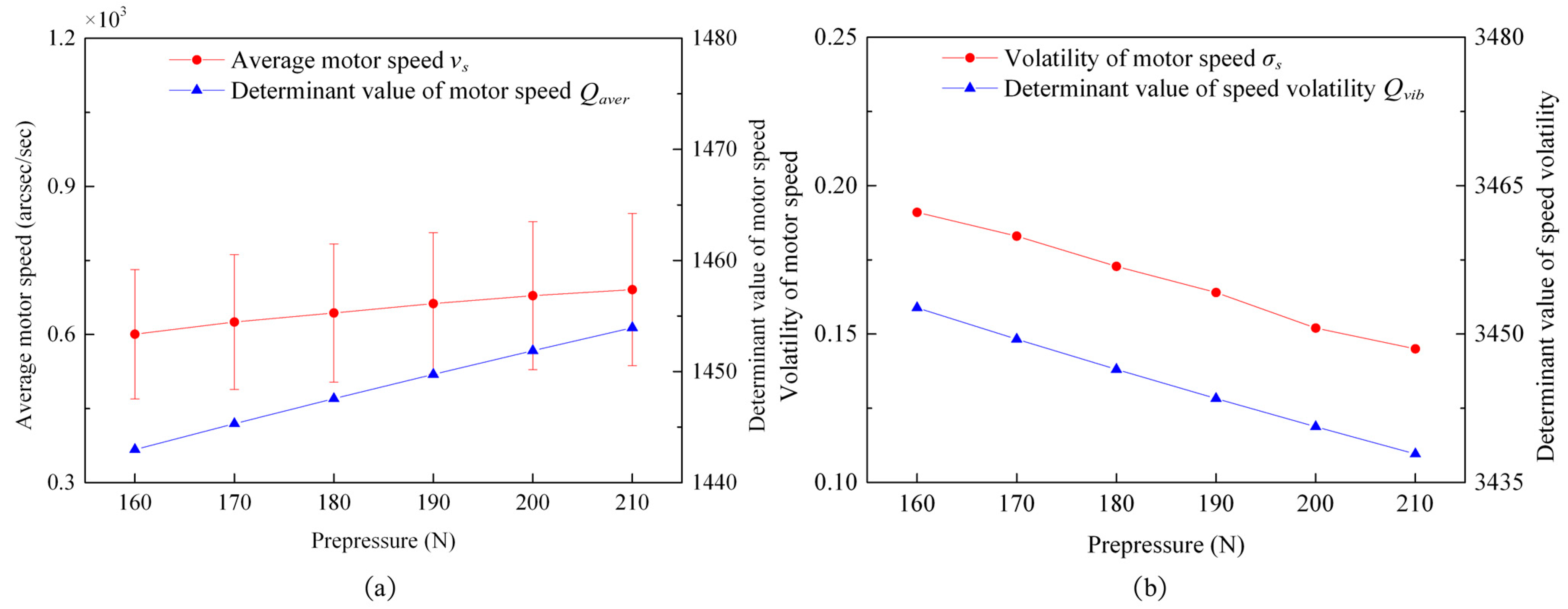
6.2.2. Simulation of Motor Speed and Determining Factor Change with Prepressure
7. Vibration Test of Motor
7.1. Amplitude Analysis Experiment
7.2. Prepressure Analysis Experiment
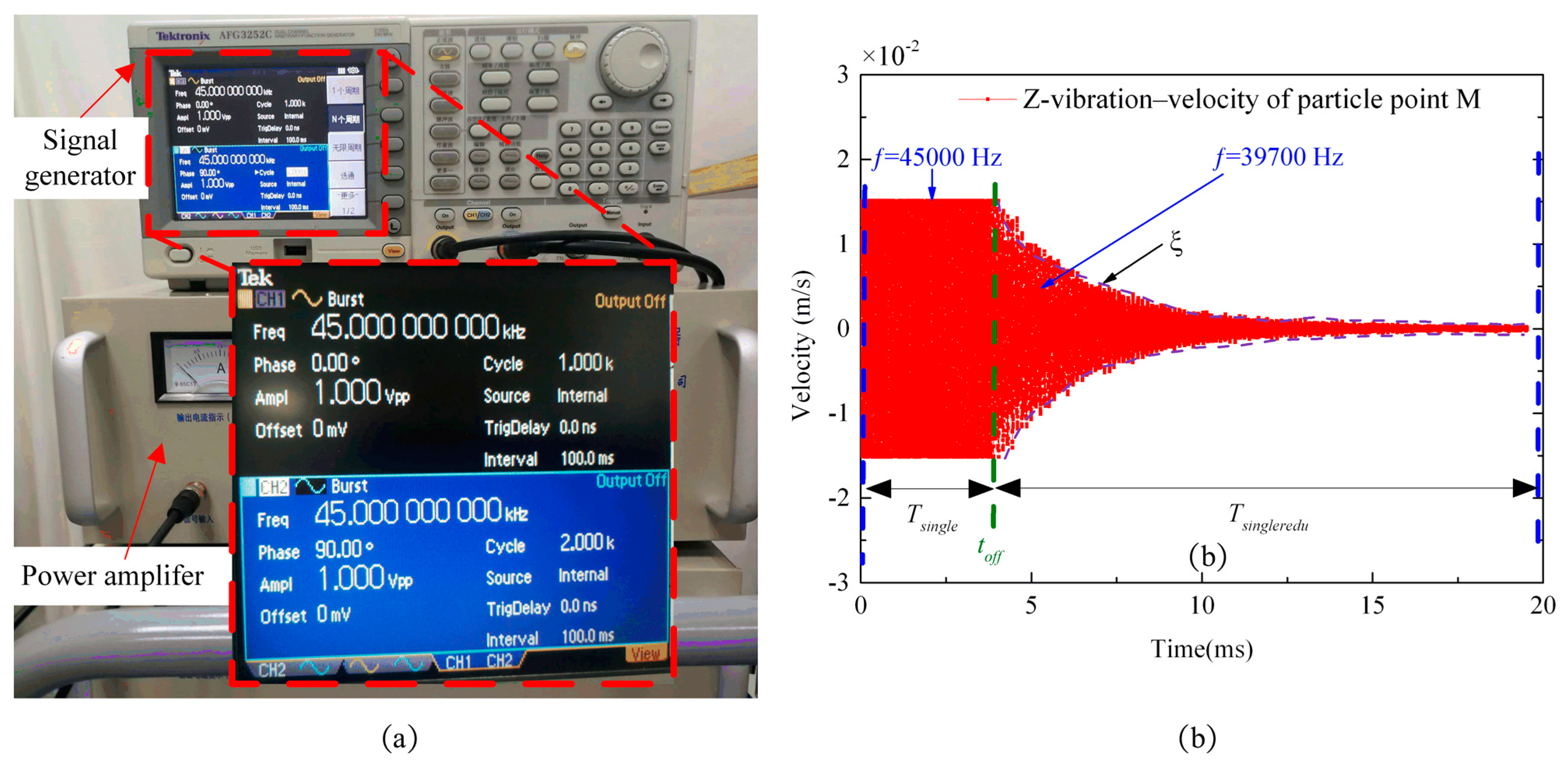
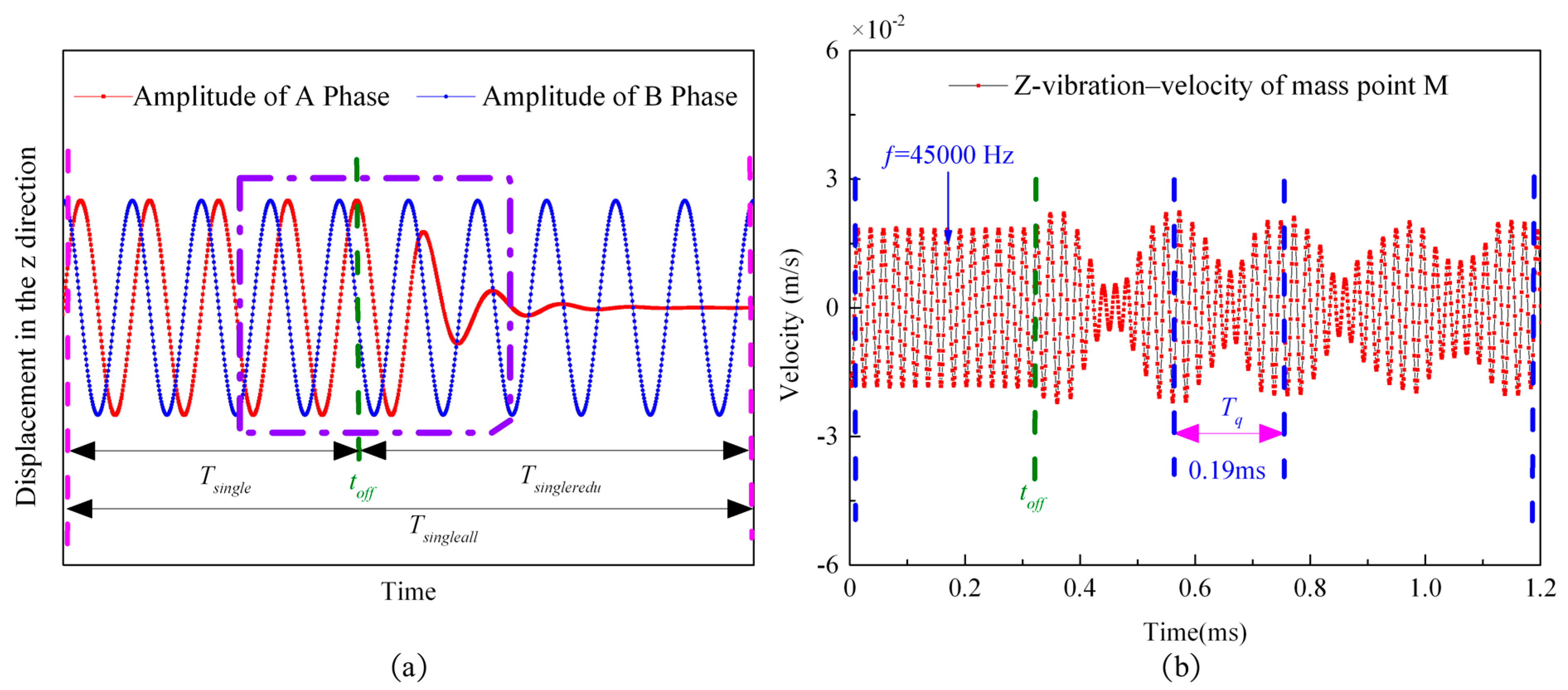
7.3. Single-Phase Power-Off Vibration Measurement Experiment
7.4. Two-Phase Power-Off Vibration Measurement Experiment
8. Experiment Research
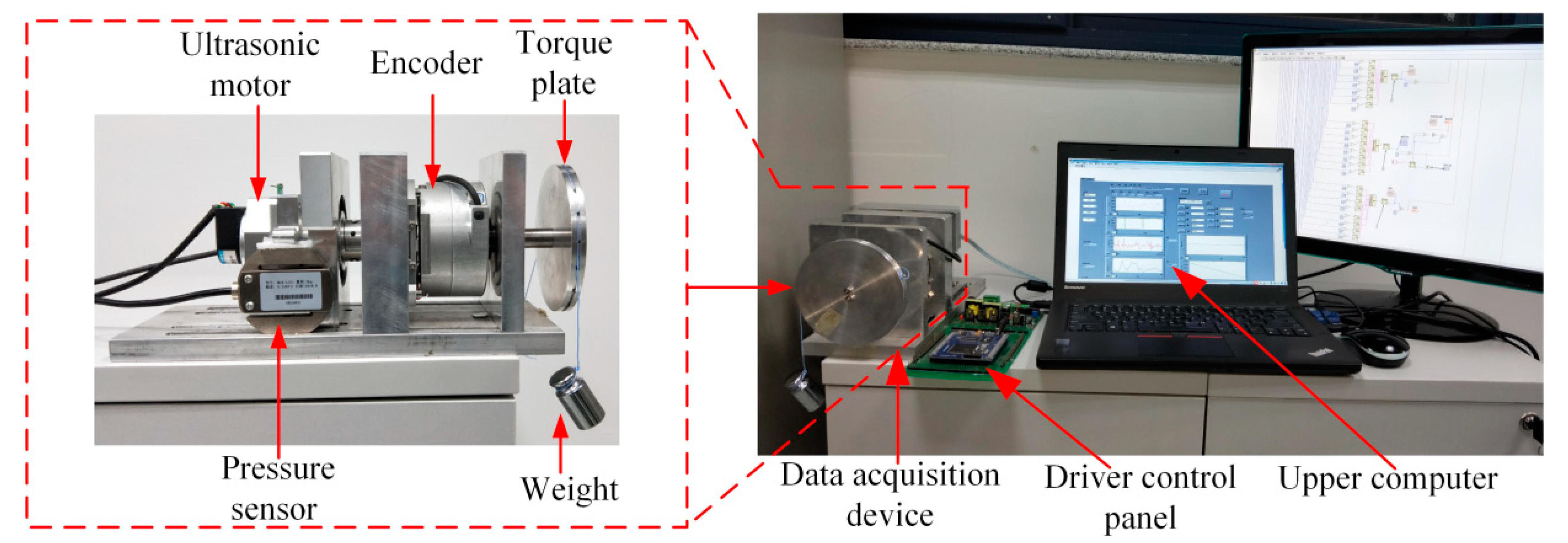
8.1. The Establishment of the Experimental Platform
8.2. Setting of Experimental Control Variables and Analysis of Experimental Results
8.3. Univariate and Multivariable Control Motor Experiments and Analysis of Experiments
8.3.1. Open-Loop Control of Single Variable Variation
8.3.2. Open-Loop Control of Multivariable Change
9. Conclusions
- According to the model of the stator structure with teeth, the relation of the stator resonant frequency with prepressure is obtained.
- By changing the control variable of the frequency difference, a theoretical analysis, simulation drawing, and vibration measurement experiment are carried out on the motion characteristics of the stator particle points, and, finally, the correctness of the theory of the beat traveling wave is proven, according to the conclusion.
- On the basis of the Hertz contact theory, to establish the contact model and transfer model of the stator and rotor, when the motor is driven by the superposition pulse drive method, the stator surface generates a traveling beat wave, which causes the driving end of the stator to experience an intermittent reciprocating vibration and drives the rotor rotation, which is the driving method when the drive motor realizes a low-speed running mechanism, and is the cause of motor speed cyclical fluctuations. To reduce the speed volatility, this paper changed the two control variables (prepressure and frequency difference), and when the angular frequency difference and prepressure changed in the range of and N, respectively—whether the motor speed was controlled by a single control variable or a multivariable control—the variation trend in the average speed, , and the speed volatility, , were consistent with the variation trend of the motor’s average speed determinant, , and thge speed volatility determinant, , respectively, which is verified by the velocity measurement experiment and the vibration measurement experiment, and lays a theoretical foundation for velocity adjustment and stability optimization.
- The significance of this study is that the researchers only needed to calculate the values of the two determinants to get the variation trend for the motor’s speed and volatility, and adjust the prepressure and driving frequency according to this trend to finally obtain the required results, thus avoiding tedious experiments and saving a lot of time for scientific research.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
Appendix C.1. Derivation of Equation (43) Monotonicity
Appendix C.2. Derivation of Equation (44) Monotonicity
References
- Zheng, S.; Han, B.; Guo, L. Composite hierarchical antidisturbance control for magnetic bearing system subject to multiple external disturbances. IEEE Trans. Ind. Electron. 2014, 61, 7004–7012. [Google Scholar] [CrossRef]
- Han, B.; Zheng, S.; Wang, Z.; Le, Y. Design, Modeling, Fabrication, Test of A Large Scale Single-Gimbal Magnetically Suspended Control Moment Gyro. IEEE Trans. Ind. Electron. 2015, 62, 7424–7435. [Google Scholar] [CrossRef]
- Pan, S.; Zhang, J.H.; Huang, W.Q. Robust controller design of SGCMG driven by hollow USM. Microsyst. Technol. 2016, 22, 741–746. [Google Scholar] [CrossRef]
- Wie, B. Singularity escape/avoidance steering logic for control moment gyro systems. Guid. Control Dyn. 2005, 28, 948–956. [Google Scholar] [CrossRef]
- Yu, L.H.; Fang, J.C.; Wu, C. Magnetically suspended control moment gyro gimbal servo-system using adaptive inverse control during disturbances. Electron. Lett. 2005, 41, 950–951. [Google Scholar] [CrossRef]
- Armstrong-Helouvry, B. Stick slip and control in low-speed motion. IEEE Trans. Autom. Control 1993, 38, 1483–1496. [Google Scholar] [CrossRef]
- Liu, J.; Liu, Y.; Zhao, L.; Xu, D.; Chen, W.; Deng, J. Design and Experiments of a Single-foot Linear Piezoelectric Actuator Operated in Stepping Mode. IEEE Trans. Ind. Electron. 2018, 65, 8063–8071. [Google Scholar] [CrossRef]
- Zhao, C.S. Ultrasonic Motors: Technologies and Applications; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Chen, N.; Zheng, J.; Fan, D. Pre-Pressure Optimization for Ultrasonic Motors Based on Multi-Sensor Fusion. Sensors 2020, 20, 2096. [Google Scholar] [CrossRef] [Green Version]
- Iv, N.; Mcfarland, A.J. Modeling of a piezoelectric rotary ultrasonic motor. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1995, 42, 210–224. [Google Scholar]
- Chau, K.T.; Chung, S.W.; Chan, C.C. Neuro-Fuzzy Speed Tracking Control of Traveling-Wave Ultrasonic Motor Drives Using Direct Pulsewidth Modulation. In Proceedings of the 2002 IEEE Industry Applications Conference, 37th IAS Annual Meeting (Cat. No. 02CH37344), Pittsburgh, PA, USA, 13–18 October 2002. [Google Scholar]
- Kobayashi, Y.; Hujioka, H.; Vongsaroj, T. A precise speed tracking control of ultrasonic motors via sampled-data H∞ control. SICE J. Control Meas. Syst. Integration. 2009, 2, 27–31. [Google Scholar]
- Shenoi, T.R.A. Gyrostabilizer Vehicular Technology. Appl. Mech. Rev. 2011, 64, 010801. [Google Scholar]
- Omura, T.; Tanabe, M.; Okubo, K.; Tagawa, N. Study on rotation speed control of coiled stator ultrasonic motor using pulse width modulation. In Proceedings of the 2011 IEEE International Ultrasonics Symposium, Orlando, FL, USA, 18–21 October 2011. [Google Scholar]
- Shi, Y.; Zhang, J.; Lin, Y.; Wu, W. Improvement of Low-Speed Precision Control of a Butterfly-Shaped Linear Ultrasonic Motor. IEEE Access 2020, 8, 135131–135137. [Google Scholar] [CrossRef]
- Senjyu, T.; Yokoda, S.; Uezato, K. A study on high efficiency drive of ultrasonic motors. In Proceedings of the Second International Conference on Power Electronics and Drive Systems, Singapore, 26–29 May 1997; Volume 1, pp. 365–370. [Google Scholar]
- Chen, N.; Zheng, J.; Jiang, X.; Fan, S.; Fan, D. Analysis and control of micro-stepping characteristics of ultrasonic motor. Front. Mech. Eng. 2020, 15, 585–899. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.; Liu, Y.; Li, K.; Chen, S.; Tian, X. Development of a resonant type piezoelectric stepping motor using longitudinal and bending hybrid bolt-clamped transducer. Sens. Actuators A 2019, 285, 182–189. [Google Scholar] [CrossRef]
- Zeng, W.; Pan, S.; Chen, L.; Xu, Z.; Xiao, Z.; Zhang, J. Research on Ultra-low Speed Driving Method of Traveling Wave Ultrasonic Motor for CMG. Ultrasonics 2020, 103, 106088. [Google Scholar] [CrossRef]
- Oh, J.H.; Yuk, H.S.; Lim, K.J. Study on the Ring Type Stator Design Technique for a Traveling Wave Rotary Type Ultrasonic Motor. Jpn. J. Appl. Phys. 2012, 51, 09MD13. [Google Scholar] [CrossRef]
- Achenbach, J.D. Reciprocity in Elastodynamics; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Majkut, L. Free and forced vibrations of timoshenko beams described by single difference equation. J. Teor. Appl. Mech. 2009, 47, 193–210. [Google Scholar]
- Mei, C.; Karpenko, Y.; Moody, S.; Allen, D. Analytical approach to free and forced vibrations of axially loaded cracked Timoshenko beams. J. Sound Vib. 2006, 291, 1041–1060. [Google Scholar] [CrossRef]
- Wang, H.; Pan, Z.; Zhu, H.; Guo, Y. Pre-pressure influences on the traveling wave ultrasonic motor performance: A theoretical analysis with experimental verification. AIP Adv. 2020, 10, 115211. [Google Scholar] [CrossRef]
- Lu, F.; Lee, H.P.; Lim, S.P. Modeling of contact with projections on rotor surfaces for ultrasonic traveling wave motors. Smart Mater. Struct. 2001, 10, 860. [Google Scholar] [CrossRef]
- Pirrotta, S.; Sinatra, R.; Meschini, A. A novel simulation model for ring type ultrasonic motor. Meccanica 2007, 42, 127–139. [Google Scholar] [CrossRef]
- Li, S.; Li, D.; Yang, M.; Cao, W. Parameters identification and contact analysis of traveling wave ultrasonic motor based on measured force and feedback voltage. Sens. Actuators A Phys. 2018, 284, 201–208. [Google Scholar] [CrossRef]
- Practical consideration of shear strain correction factor and Rayleigh damping in models of piezoelectric transducers. Sens. Actuators A Phys. 2004, 115, 202–208. [CrossRef]
- Djaghloul, M.; Boumous, Z.; Belkhiat, S. Driving Forces Study in the Ultrasonic Motor; American Institute of Physics: College Park, MD, USA, 2008. [Google Scholar]
- Wen, Z.; He, Q.; Qiao, G. An insight into the flexible drive mechanism in short cylinder ultrasonic piezoelectric vibrator. Rev. Sci. Instrum. 2020, 91, 055003. [Google Scholar] [CrossRef]
- Zhu, Y.; Yang, T.; Fang, Z.; Shiyang, L.; Cunyue, L.; Yang, M. Contact modeling for control design of traveling wave ultrasonic motors. Sens. Actuators A Phys. 2020, 310, 112037. [Google Scholar] [CrossRef]
- Boumous, Z.; Belkhiat, S.; Kebbab, F.Z. Effect of shearing deformation on the transient response of a traveling wave ultrasonic motor. Sens. Actuators A 2009, 150, 243–250. [Google Scholar] [CrossRef]
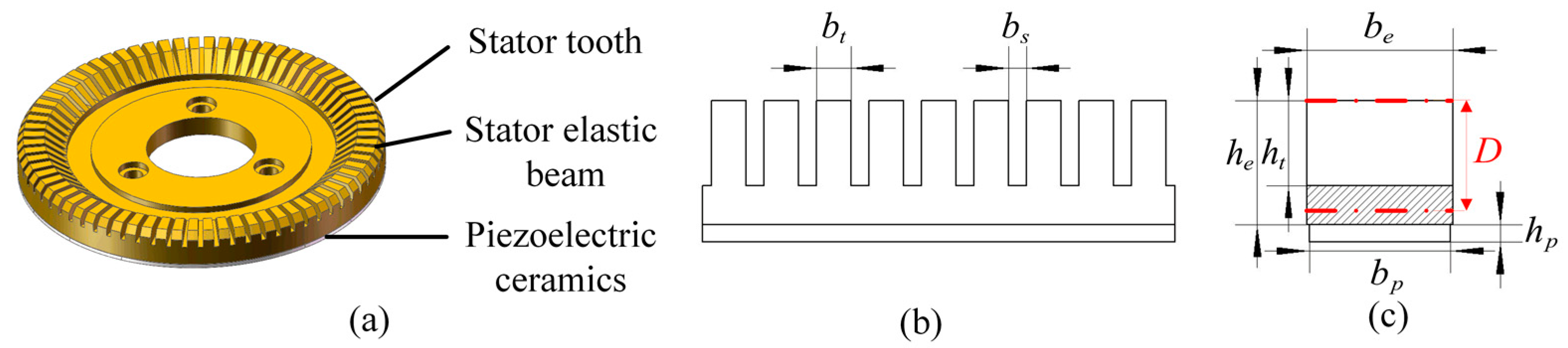












| Parameter | Description | Numerical Value (Unite) |
|---|---|---|
| Stator radius | ||
| Number of stator teeth | 72 | |
| Stator perimeter | ||
| Stator slot width | ||
| Stator elastic beam width | ||
| Friction material width | ||
| Stator elastic beam thickness | ||
| Stator teeth thickness | ||
| Piezoelectric material thickness | ||
| Friction material thickness | ||
| Stator elastic beam density | ||
| Piezoelectric material density | ||
| Friction material density | ||
| Stator elastic beam Young’s modulus | ||
| Piezoelectric material Young’s modulus | ||
| Friction material Young’s modulus | ||
| Stator elastic beam shear modulus | ||
| Piezoelectric material shear modulus | ||
| Stator elastic beam Poisson’s ratio | 0.33 | |
| Piezoelectric material Poisson’s ratio | 0.31 | |
| Friction material Poisson’s ratio | 0.3 | |
| Damping ratio | 0.006 | |
| Height-width ratio of the beam section | 0.9 | |
| Piezoelectric voltage | ||
| Mortor load | 0.3 (N·m) | |
| Moment of inertia of the rotor | 4.5 × 10−6 | |
| κ | Shear correction coefficient | 0.9 |
| (N) | 100 | 120 | 140 | 160 | 180 | 200 | 220 |
| (μm) | 8.3 | 7.3 | 6.6 | 6.0 | 5.5 | 5.2 | 4.8 |
| Parameters | Value |
|---|---|
| 14 | |
| 20 | |
| 0.3 (N·m) | |
| 180 (N) |
| Parameters | Value |
|---|---|
| 633 (acr s) | |
| 400 (arc s/s) | |
| 1447.5 | |
| 17.3% | |
| 3446.4 |
| Experimental Test Number | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| (N) | 180 | 170 | 160 | 190 | 200 | 210 |
| (rad/s) | 3300 | 3100 | 3000 | 2800 | 2900 | 3200 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeng, W.; Pan, S.; Chen, L.; Ren, W.; Hu, X. Research on Low-Speed Driving Model of Ultrasonic Motor Based on Beat Traveling Wave Theory. Actuators 2021, 10, 304. https://doi.org/10.3390/act10110304
Zeng W, Pan S, Chen L, Ren W, Hu X. Research on Low-Speed Driving Model of Ultrasonic Motor Based on Beat Traveling Wave Theory. Actuators. 2021; 10(11):304. https://doi.org/10.3390/act10110304
Chicago/Turabian StyleZeng, Weijun, Song Pan, Lei Chen, Weihao Ren, and Xiaobin Hu. 2021. "Research on Low-Speed Driving Model of Ultrasonic Motor Based on Beat Traveling Wave Theory" Actuators 10, no. 11: 304. https://doi.org/10.3390/act10110304






