Experimental Validation and Numerical Analysis of a High-Performance Blast Energy-Absorbing System for Building Structures
Abstract
1. Introduction
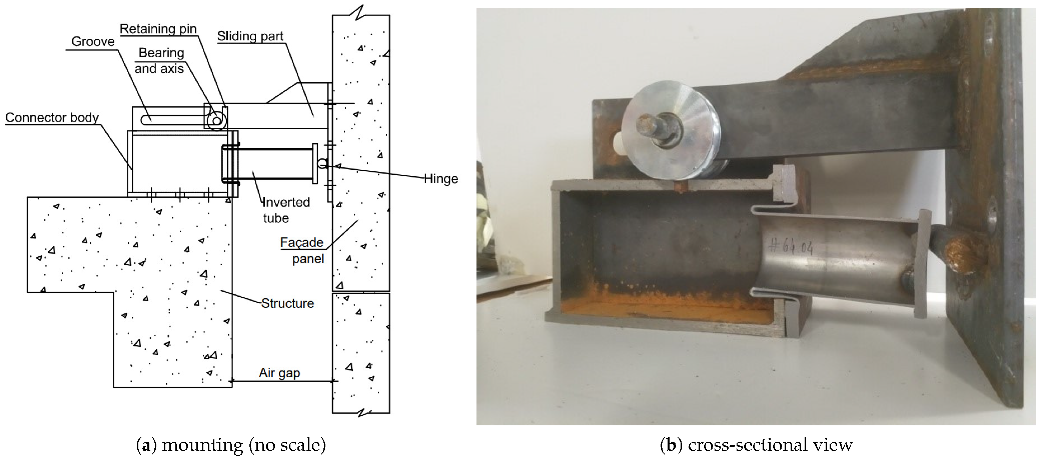
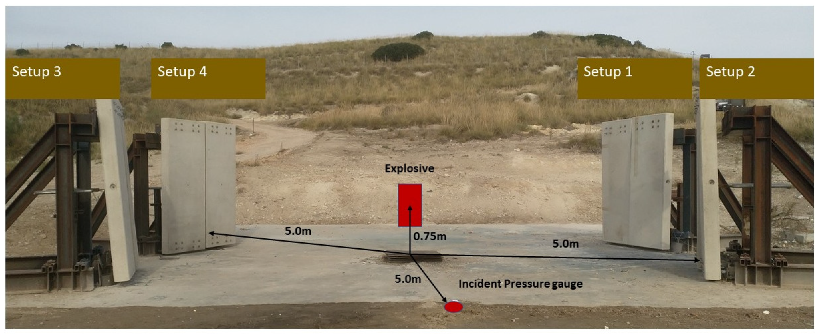
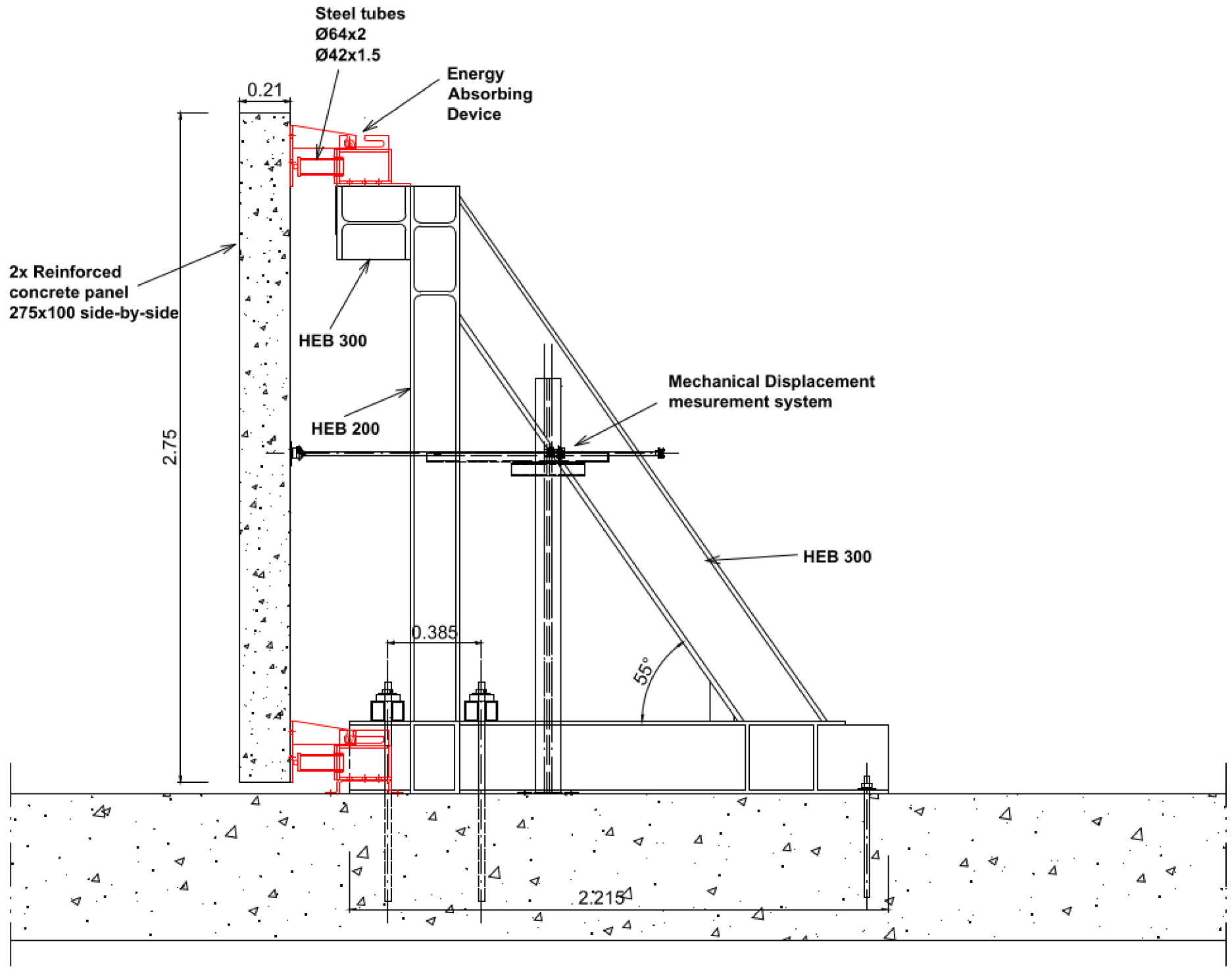
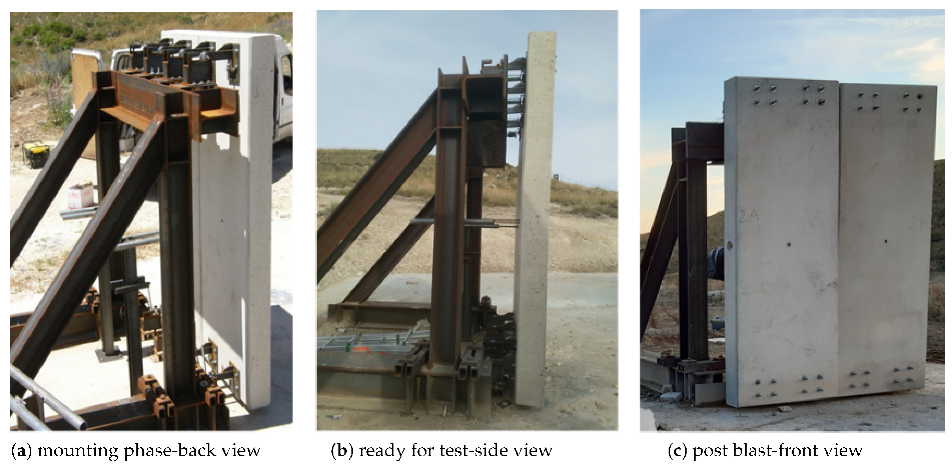
2. Experimental Campaign
2.1. Blast Test Setup
2.2. Materials
2.3. Explosive Charge
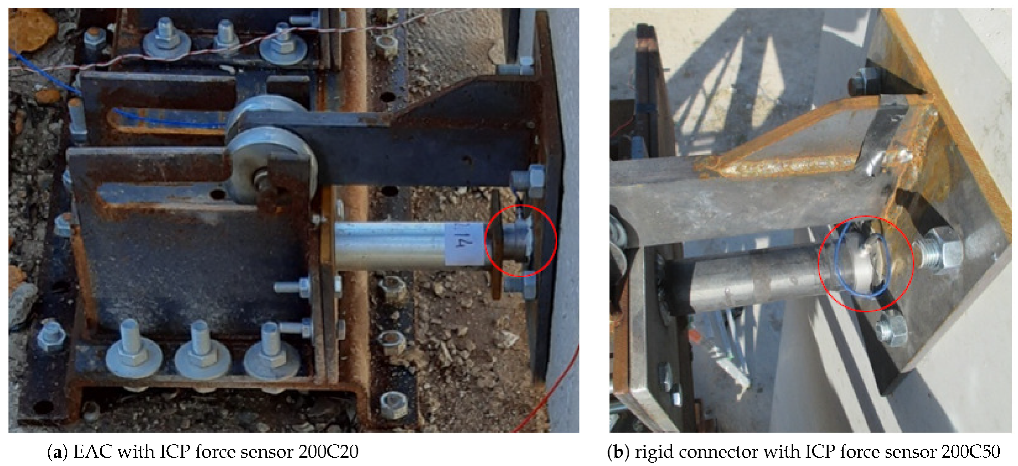
2.4. Instrumentation
2.5. Recorded Data
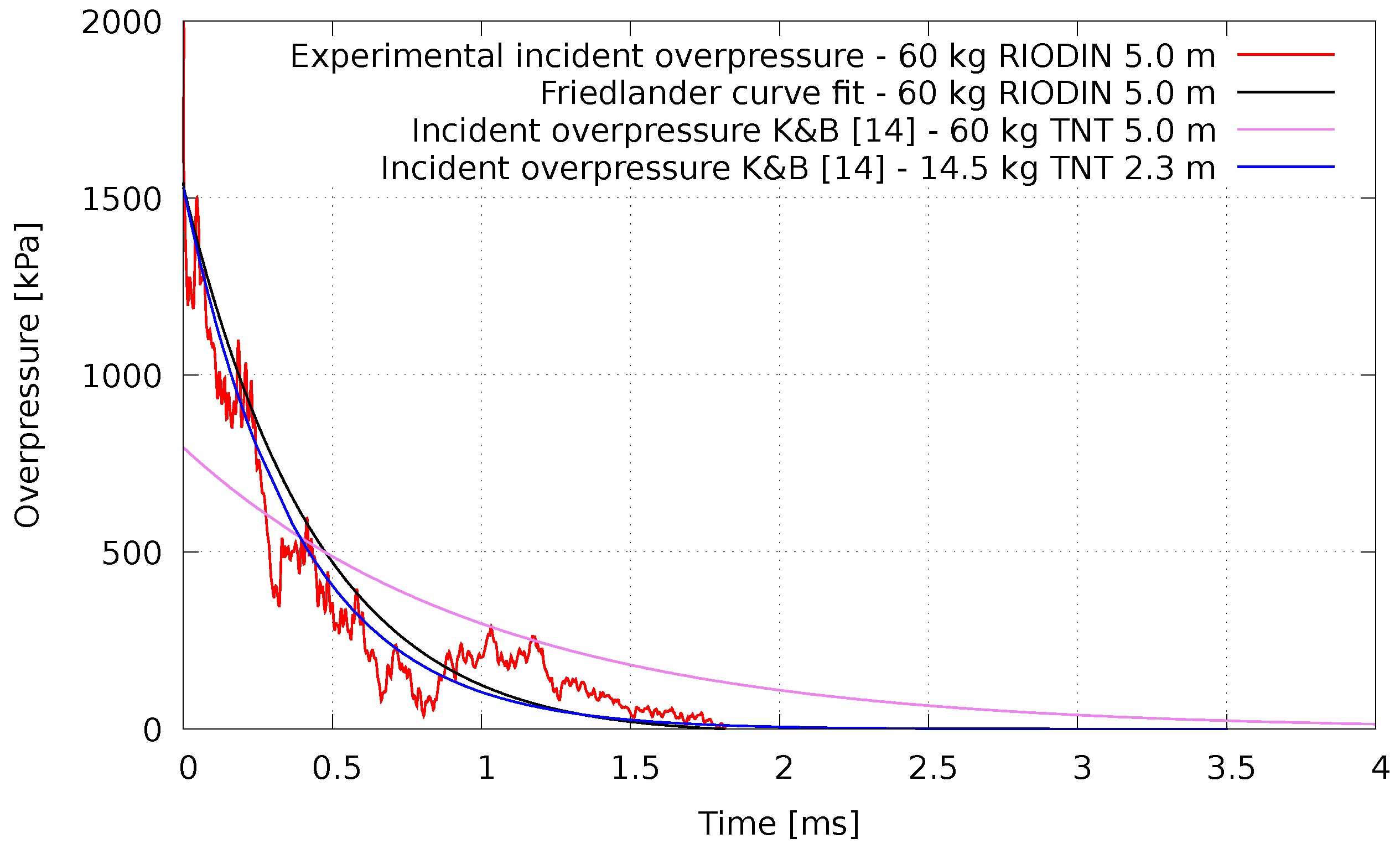
2.5.1. Blast Wave Overpressures
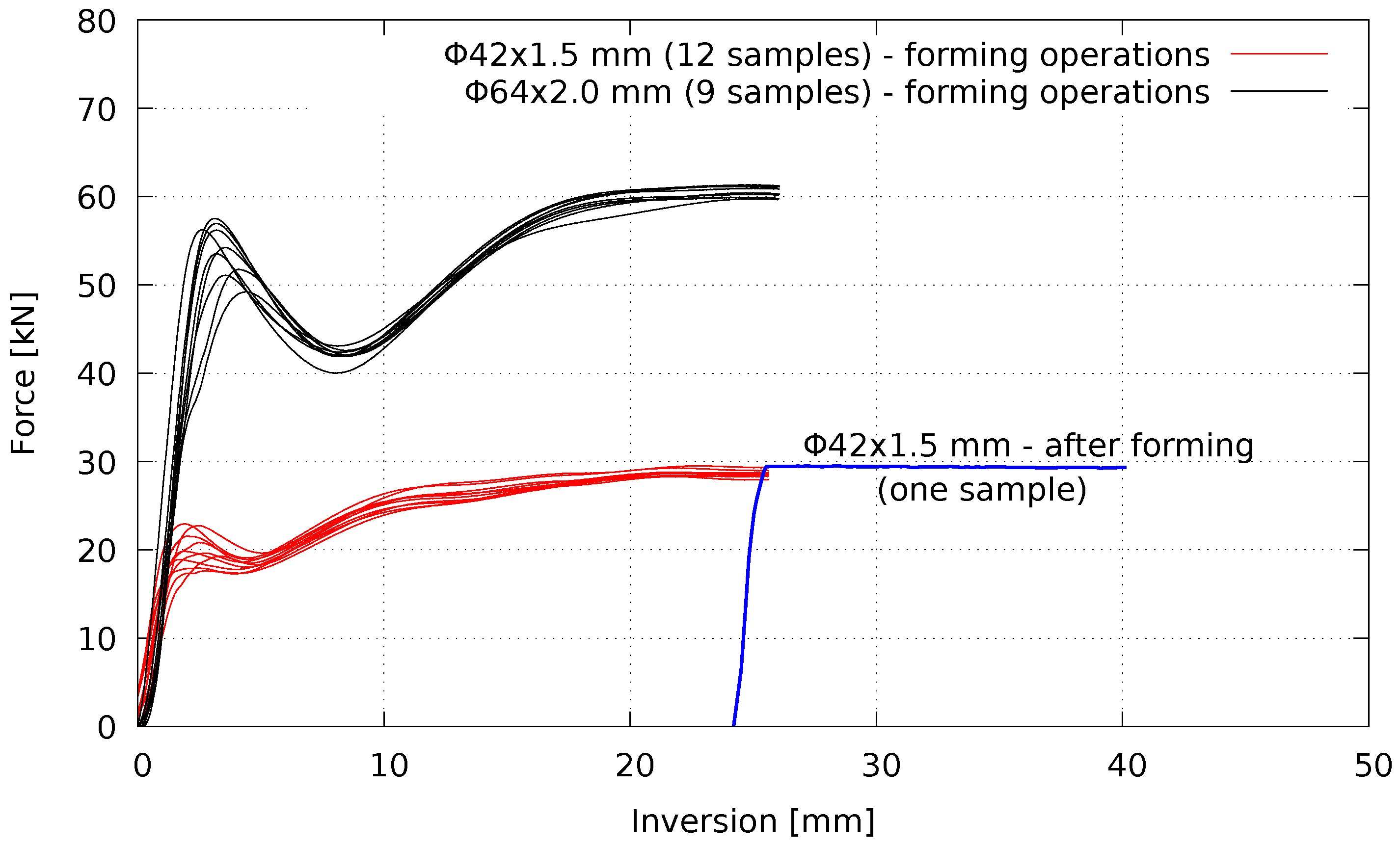
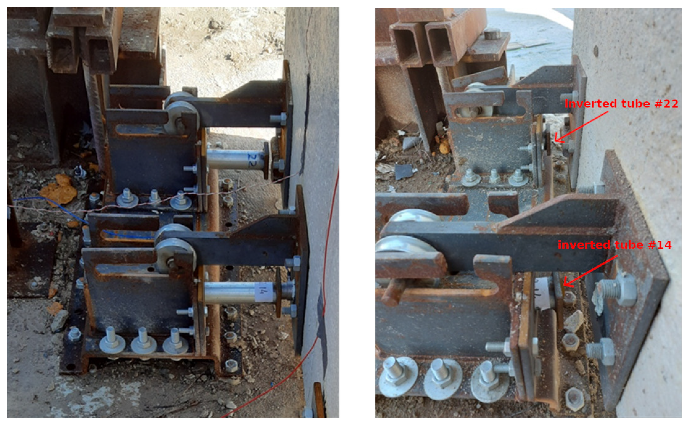
2.5.2. Shortening of the Inversion Tubes
2.5.3. Panel’s Midspan Displacements and Cracking
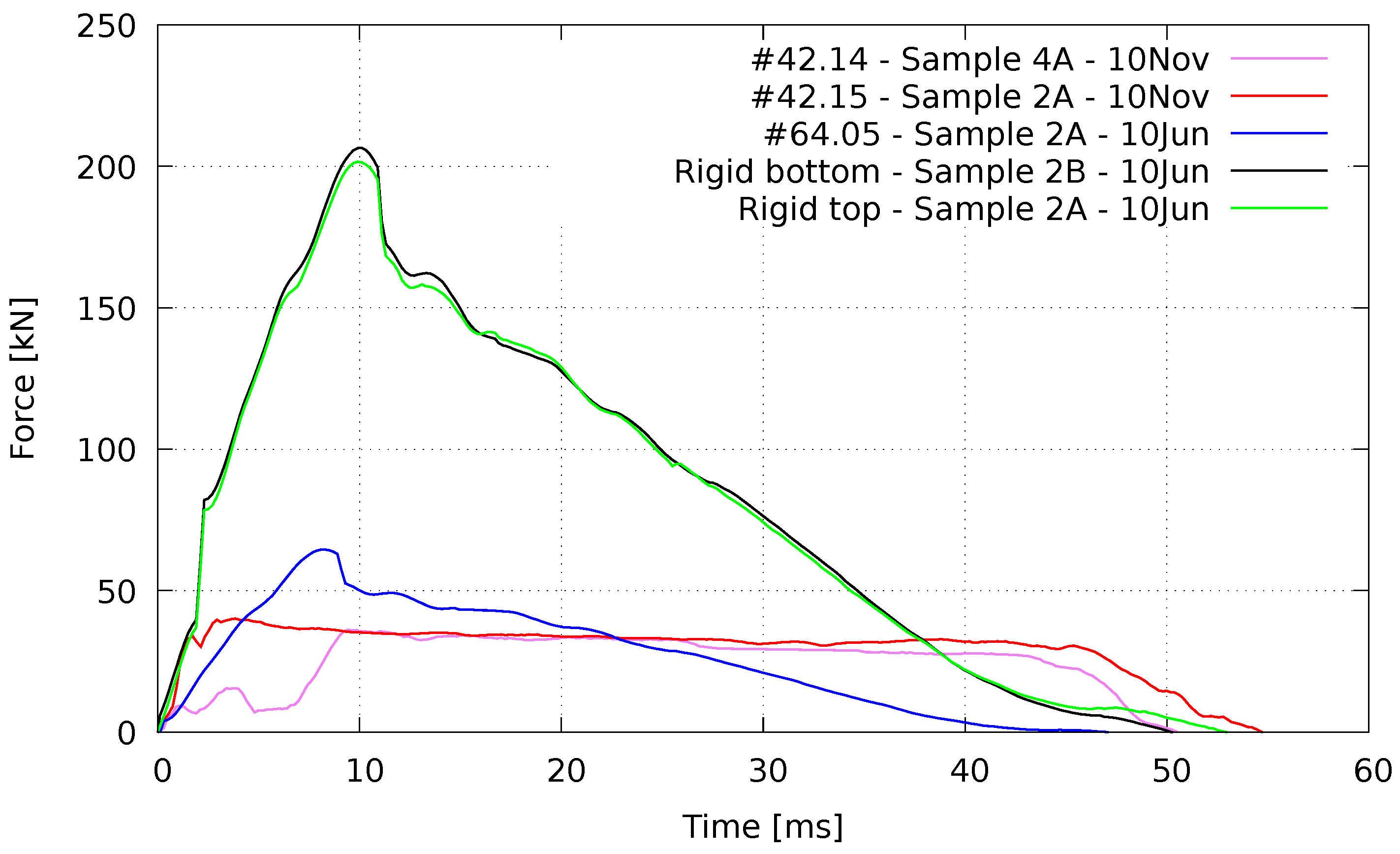
2.5.4. Transmitted Forces
3. FE Simulation
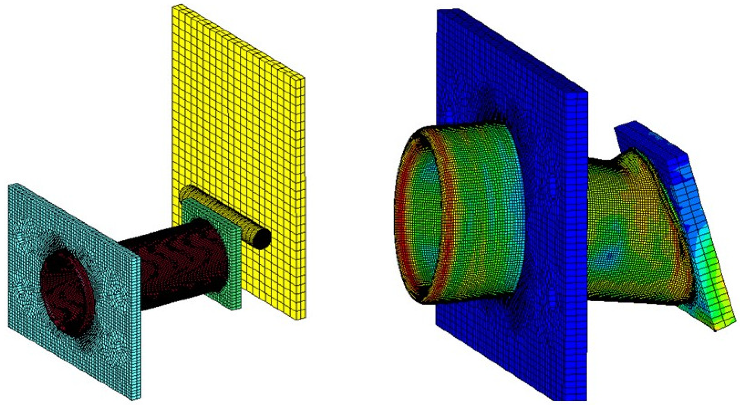
3.1. FE Numerical Model
3.2. Strain Rates
3.3. TNT Equivalent Curves
3.4. Validation of the FE Model
3.5. Prediction of the Blast Response of an Idealized RC Building Structure
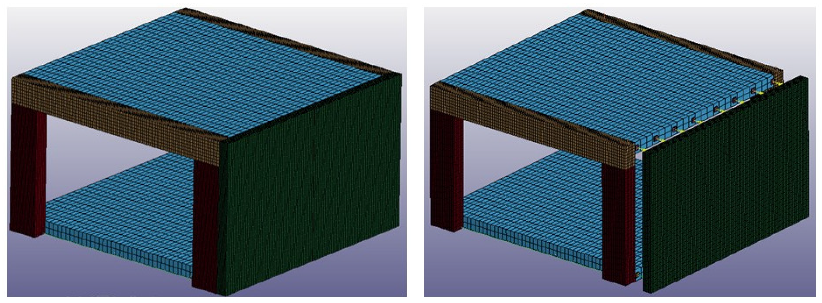
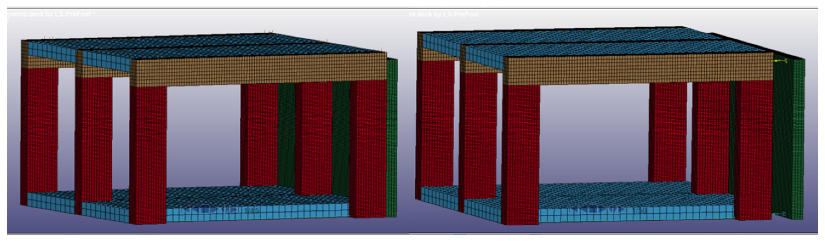
3.5.1. FE Model
3.5.2. Analysis of the Results
- (a)
- For a similar impulse magnitude, the longer duration of the loading tends to significantly worsen the damage to the structure (i.e., energy absorbed), in both structural systems (i.e., panel with rigid connectors and panel with EACs). From scenarios (i) to (ii), the maximum lateral displacement of the structure measured at the girder reduces 27% (rigid connection) and 31% ();
- (b)
- Introduction of EACs reduces the structure maximum displacement in 46% (scenario (i)) or 49% (scenario (ii));
- (c)
- In scenario (i) the introduction of EACs causes a reduction of 67% () of the energy absorbed by the building structure. The plots in Figure 19 illustrate the observations. On the other hand, the total internal energy of the overall system increases significantly (between 2.08 and 1.45 times), which means that the energy was mostly absorbed by the protective system (EACs and façade panel). In scenario (ii), reduction of the energy absorbed is 72% ();
- (d)
- The introduction of EACs also allows a significant reduction of the sacrificial façade panel damage and deflections, as observed in scenarios (i) and (ii). This fact is relevant, especially in very stiff structures where the deflections will concentrate in the façade elements.
- (e)
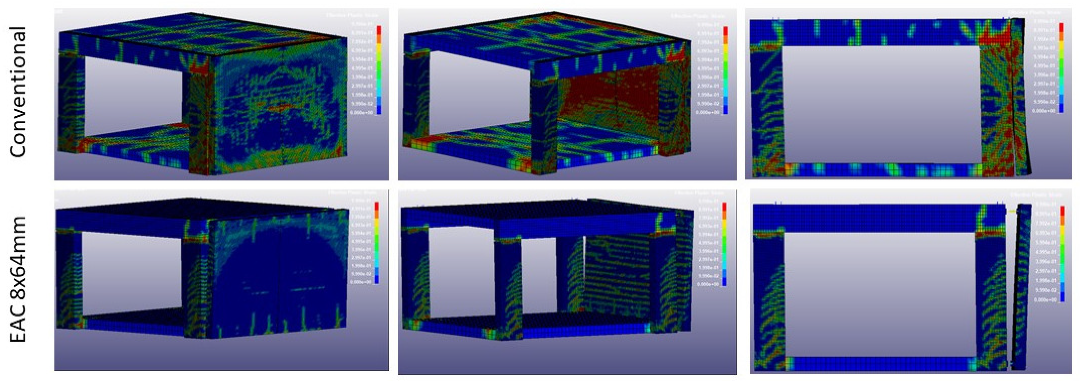
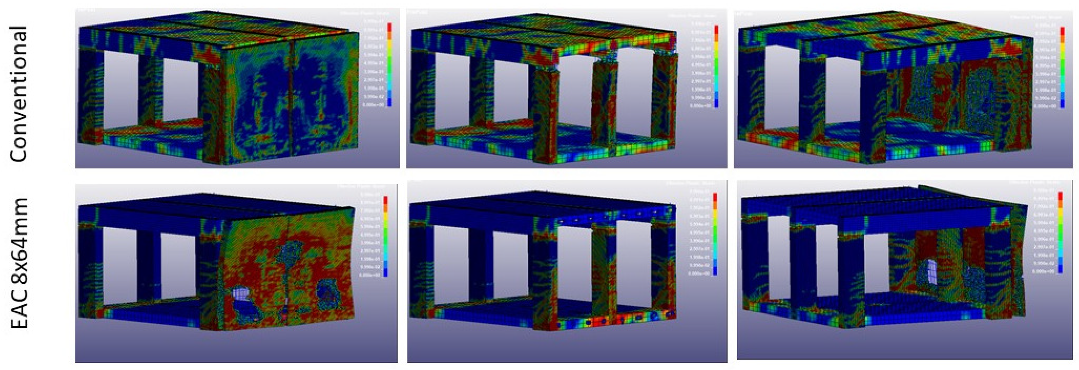
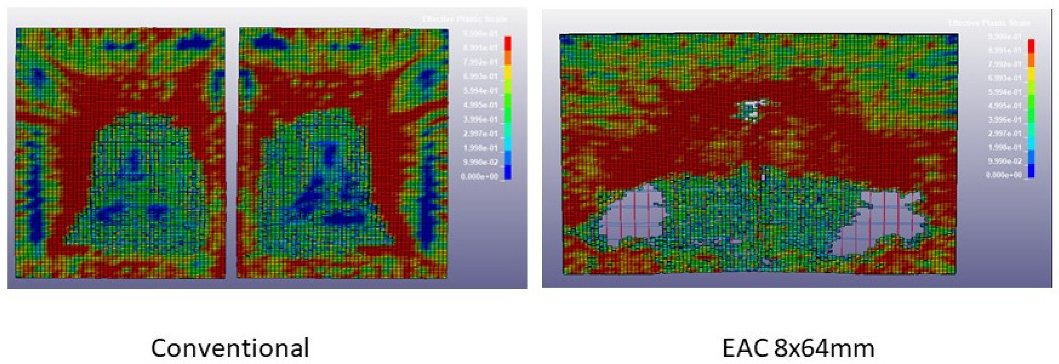
- Introduction of EACs reduces the damage in the supporting columns. This can be observed qualitatively by the amount and pattern of cracking lines and fully plasticized regions in the columns (see Figure 20).
- (f)
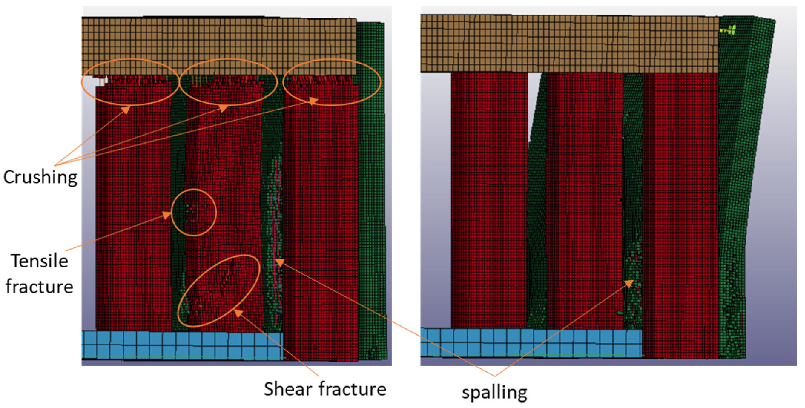
- In the conventional structure, the shear failure occurs by concrete crushing at the connection between the columns and the beams. This phenomenon is strongly reduced with the introduction of EACs. The lower the inversion force (i.e., the lateral force transmitted to the structure), the higher the reduction in energy absorbed by the structure.
4. Conclusions
- (i)
- Introduction of EACs strongly limits forces imparted to the protected structure, reducing drastically the energy absorbed;
- (ii)
- The comparison of the energies absorbed with and without a protective system demonstrates that the reductions can reach 72% in near-field explosions (when using connectors);
- (iii)
- In the case of far-field explosions, this reduction can reach 67%;
- (iv)
- In the explosions at the very near field, the reduction in energy absorbed was of the order of 68%, avoiding the loss or collapse of one main supporting element of the structure.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Barnett, S.; Millard, S.; Schleyer, G.; Soutsos, M.; Tyas, A. Explosion and Impact Resistance of Ultra High Performance Fibre Reinforced Concrete. Concrete 2007, 2, 32–33. [Google Scholar]
- Xu, J.; Wu, C.; Xiang, H.; Su, Y.; Li, Z.X.; Fang, Q.; Hao, H.; Liu, Z.; Zhang, Y.; Li, J. Behaviour of ultra high performance fibre reinforced concrete columns subjected to blast loading. Eng. Struct. 2016, 118, 97–107. [Google Scholar] [CrossRef]
- Bazan, M.; Oswald, C.J. Blast Design of Reinforced Concrete and Masonry Components Retrofitted with FRP; Protection Engineering Consultants LLC: San Antonio, TX, USA, 2010. [Google Scholar]
- ASCE. Design of Blast-Resistant Buildings in Petrochemical Facilities, 2nd ed.; American Society of Civil Engineers: Reston, VA, USA, 2010. [Google Scholar]
- Naito, C.; Dinan, R.; Bewick, B. Use of Precast Concrete Walls for Blast Protection of Steel Stud Construction. J. Perform. Constr. Facil. 2011, 25, 454–463. [Google Scholar] [CrossRef]
- Johnson, C.F. Concrete Masonry Wall Retrofit Systems for Blast Protection. Ph.D. Thesis, Texas A&M University, Corpus Christi, TX, USA, 2013. [Google Scholar]
- Nesterenko, V.F. Shock (Blast) Mitigation by “Soft” Condensed Matter. MRS Online Proc. Libr. 2003, 759, 43. [Google Scholar] [CrossRef]
- Alberdi, R.; Przywara, J.; Khandelwal, K. Performance evaluation of sandwich panel systems for blast mitigation. Eng. Struct. 2013, 56, 2119–2130. [Google Scholar] [CrossRef]
- Thompson, S.C.; Muchnick, H.; Choi, H.J.; McDowell, D.L.; Allen, J.K.; Mistree, F. Robust Materials Design of Blast Resistant Panels. In Proceedings of the 11th AIAA/ISSMO Multidisciplinary Analysis and Optimization Conference, Portsmouth, VA, USA, 6–8 September 2006. [Google Scholar]
- Zhu, F.; Zhao, L.; Lu, G.; Wang, Z. Deformation and failure of blast-loaded metallic sandwich panels—Experimental investigations. Int. J. Impact Eng. 2008, 35, 937–951. [Google Scholar] [CrossRef]
- Rebelo, H.; Lecompte, D.; Cismasiu, C.; Jonet, A.; Belkassem, B.; Maazoun, A. Experimental and numerical investigation on 3D printed PLA sacrificial honeycomb cladding. Int. J. Impact Eng. 2019, 131, 162–173. [Google Scholar] [CrossRef]
- Gomes, G.; Lúcio, V.J.G.; Cismasiu, C. Development of a high-performance blast energy-absorbing system for building structures. Int. J. Prot. Struct. 2022. submitted. [Google Scholar]
- Livermore Software Technology Corporation. LS-DYNA User Manual: Volume I (Version R10.1); Livermore Software Technology Corporation: Livermore, CA, USA, 2018. [Google Scholar]
- U.S. Department of Defense. UFC 3-340-02: Structures to Resist the Effects of Accidental Explosions; U.S. Department of Defense: Washington, DC, USA, 2014.
- Pires, L. Numerical Analysis of an Innovatiotive Blast Protective System for Buildings. Master’s Thesis, Instituto Superior Técnico, Lisbon University, Lisbon, Portugal, 2021. [Google Scholar]
- Cha, J.G.; Bae, C.H.; Han, B.K. Experimental Study of the Possibility of Using of External Inversion Crash Box on Sloped Crash Barrier. In Proceedings of the 23rd International Technical Conference on the Enhanced Safety of Vehicles (ESV), Seoul, Republic of Korea, 27–30 May 2013; pp. 69–79. [Google Scholar]
- Alañón, A.; Cerro-Prada, E.; Vázquez-Gallo, M.J.; Santos, A.P. Mesh size effect on finite-element modeling of blast-loaded reinforced concrete slab. Eng. Comput. 2018, 34, 649–658. [Google Scholar] [CrossRef]
- Schwer, L.E. Is Your Mesh Refined Enough? Estimating Discretization Error using GCI. In Proceedings of the LS-DYNA Anwenderforum, Bamberg, Germany, 30 September–1 October 2008. [Google Scholar]
- Symonds, P.; Jones, N. Impulsive loading of fully clamped beams with finite plastic deflections and strain-rate sensitivity. Int. J. Mech. Sci. 1972, 14, 49–69. [Google Scholar] [CrossRef]
- Marais, S.T.; Tait, R.B.; Cloete, T.J.; Nurick, G.N. Material testing at high strain rate using the split Hopkinson pressure bar. Lat. Am. J. Solids Struct. 2004, 1, 319–339. [Google Scholar]
- Szuladzinski, G. Formulas for Mechanical and Structural Shock and Impact, 1st ed.; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]






| Nominal Diameter and Thickness | |||||
|---|---|---|---|---|---|
| mm | GPa | MPa | MPa | kN | mm |
| 210.4 | 379 | 401 | 28.65 | 140 | |
| 210.6 | 371 | 423 | 60.45 | 140 |
| Test | Sensor | Date | Incident Pressure | Positive Phase | Incident Impulse | Decay Parameter |
|---|---|---|---|---|---|---|
| Serial | Height | 2021 | ||||
| - | m | - | kPa | ms | kPa·ms | - |
| #1 | 0.0 | 10 Jun | 1543.0 | 1.9 | 576.18 | 3.18 |
| #1 | 2.5 | 10 Jun | 765.7 | 1.6 | 410.05 | 1.34 |
| #2 | 0.0 | 10 Nov | 1133.9 | 1.7 | 658.5 | 1.24 |
| Blast | Sample | Position | Connector | Shortening | Rotation | Total Shortening |
|---|---|---|---|---|---|---|
| Test | Panel | mm | mm | |||
| #1 | #2A | BL BR TL TR | #64.05 #647 #64.06 #648 | 63.8 6.0 5.0 0.0 | 6.0 17.0 3.0 0.0 | 75 |
| #1 | #4B | BL BR TL TR | #64.09 #646 #64.11 #64.10 | 42.0 40.0 2.0 0.0 | 3.0 3.0 3.0 3.0 | 84 |
| #1 | #4A | BL BR TL TR | #64.04 #645 #64.08 #64.07 | 39.0 45.0 4.0 2.0 | 2.0 3.5 3.0 2.0 | 90 |
| #2 | #2A | BL BR TL TR | #42.23 #42.15 #42.07 #42.21 | 74.5 86.9 21.8 26.7 | 0.0 0.0 4.5 1.5 | 209.9 |
| #2 | #4B | BL BR TL TR | #42.13 #42.18 #42.10 #42.05 | 82.9 72.2 16.4 19.7 | 3.0 1.5 1.0 0.0 | 191.2 |
| #2 | #4A | BL BR TL TR | #42.22 #42.14 #42.08 #42.09 | 107.9 102.4 36.1 28.3 | 0.0 0.0 0.0 0.0 | 274.7 |
| Average Displacement | Panel Deflection | ||
|---|---|---|---|
| Sample Panel | Bottom | Top | Midspan |
| mm | mm | mm | |
| #2B * #2A #4B #4A | 0.0 80.7 77.5 105.2 | 0.0 24.3 18.1 32.2 | 29.0 14.8 13.3 9.5 |
| EAC | Panel | Position | Peak Force/Plateau | Duration |
|---|---|---|---|---|
| Id | kN | ms | ||
| Rigid | 2B | BL | 206.6 | 51 |
| Rigid | 2B | TR | 201.7 | 53 |
| #64.05 | 2A | BL | 64.6 | 42 |
| #42.15 | 2A | BL | 33.5 | 55 |
| #42.14 | 4A | BL | 31.9 | 52 |
| Experimental Load | Equivalent TNT Load | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Sample | Load | Load | ||||||||
| kg@m | kPa | kPa·ms | kg@m | kPa | kPa·ms | kPa | kPa·ms | ms | - | |
| 64 | 60@5 | 1543 | 576 | 14.5 | 1529 | 573 | 9478 | 2335 | 3.5 | 13.17 |
| 42 | 60@5 | 1133 | 658 | 21.0 | 1132 | 629 | 6461 | 2182 | 5.6 | 15.54 |
| Equivalent | Panel Midspan Deflection | EAC Deformation | Total EAC Deformation | |||||
|---|---|---|---|---|---|---|---|---|
| Sample | Load | Rigid Connectors | EAC | Top | Bottom | Numerical | Experimental | |
| kg@m | ms | mm | mm | mm | mm | mm | mm | |
| 64 | 14.3@2.5 | 20 | 17 | 8 | 12 | 27 | 78 | 83 |
| 42 | 21.0@3.0 | 45 | 26 | 4 | 42 | 65 | 214 | 200 * |
| Max. Displacement | Max. Inversion | Internal Energy | |||||
|---|---|---|---|---|---|---|---|
| Case—Scenario | Top Girder | Panel Midspan | Top | Bottom | Structure | Panel | Inverter |
| mm | mm | mm | mm | kJ | kJ | kJ | |
| Rigid attachment—(i) | 89 | 135 | NA | NA | 48.1 | 103.2 | NA |
| EAC —(i) | 48 | 59 | 295 | 300 | 15.8 | 17.3 | 282.0 |
| Rigid attachment—(ii) | 65 | 139 | NA | NA | 36.3 | 111.0 | NA |
| EAC —(ii) | 33 | 57 | 165 | 293 | 10.3 | 9.7 | 200.0 |
| Rigid attachment—(iii) | 64 | 195 | NA | NA | 120.0 | 199.8 | NA |
| EAC —(iii) | 59 | 232 | 152 | 330 | 38.6 | 241.0 | 175.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gomes, G.d.J.; Lúcio, V.J.d.G.; Cismaşiu, C.; Mingote, J.L. Experimental Validation and Numerical Analysis of a High-Performance Blast Energy-Absorbing System for Building Structures. Buildings 2023, 13, 601. https://doi.org/10.3390/buildings13030601
Gomes GdJ, Lúcio VJdG, Cismaşiu C, Mingote JL. Experimental Validation and Numerical Analysis of a High-Performance Blast Energy-Absorbing System for Building Structures. Buildings. 2023; 13(3):601. https://doi.org/10.3390/buildings13030601
Chicago/Turabian StyleGomes, Gabriel de Jesus, Valter José da Guia Lúcio, Corneliu Cismaşiu, and José Luis Mingote. 2023. "Experimental Validation and Numerical Analysis of a High-Performance Blast Energy-Absorbing System for Building Structures" Buildings 13, no. 3: 601. https://doi.org/10.3390/buildings13030601
APA StyleGomes, G. d. J., Lúcio, V. J. d. G., Cismaşiu, C., & Mingote, J. L. (2023). Experimental Validation and Numerical Analysis of a High-Performance Blast Energy-Absorbing System for Building Structures. Buildings, 13(3), 601. https://doi.org/10.3390/buildings13030601







