Shear Strength of HVFA-SCC Beams without Stirrups
Abstract
1. Introduction
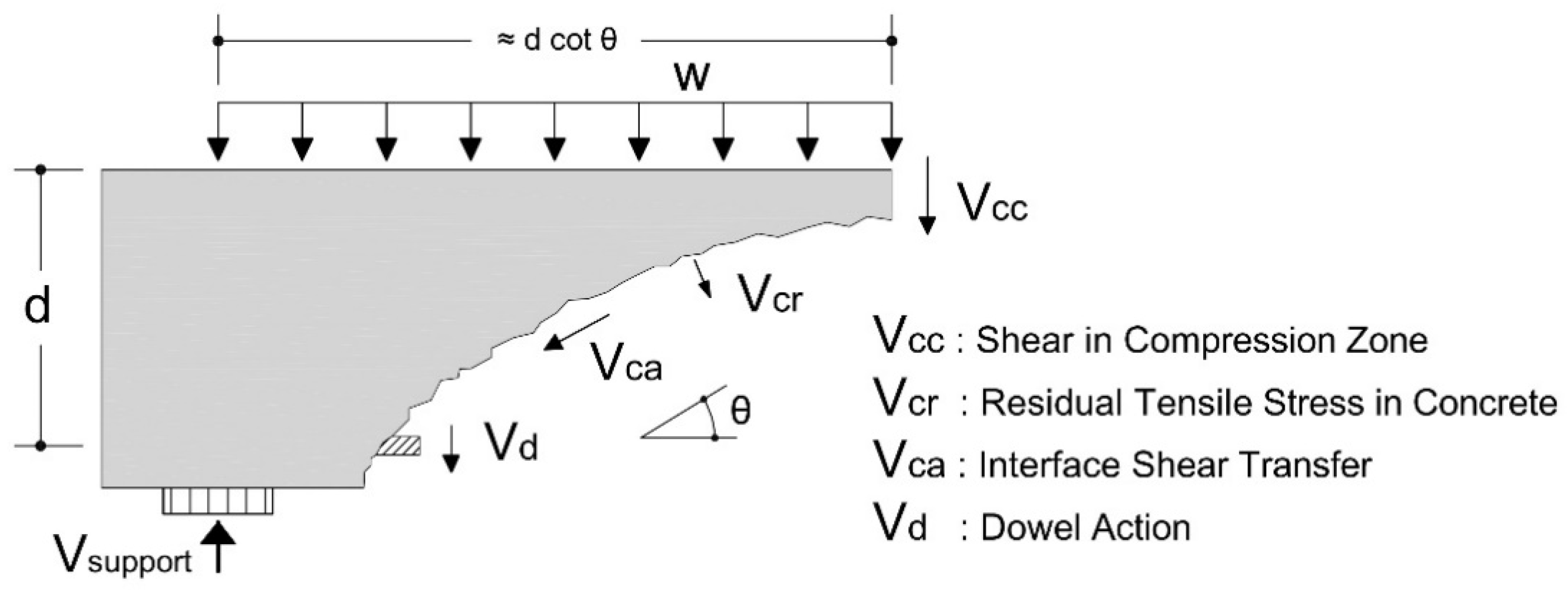
2. Parameters Affecting Shear
3. Methods
3.1. Experimental Investigation
3.1.1. Materials and Properties
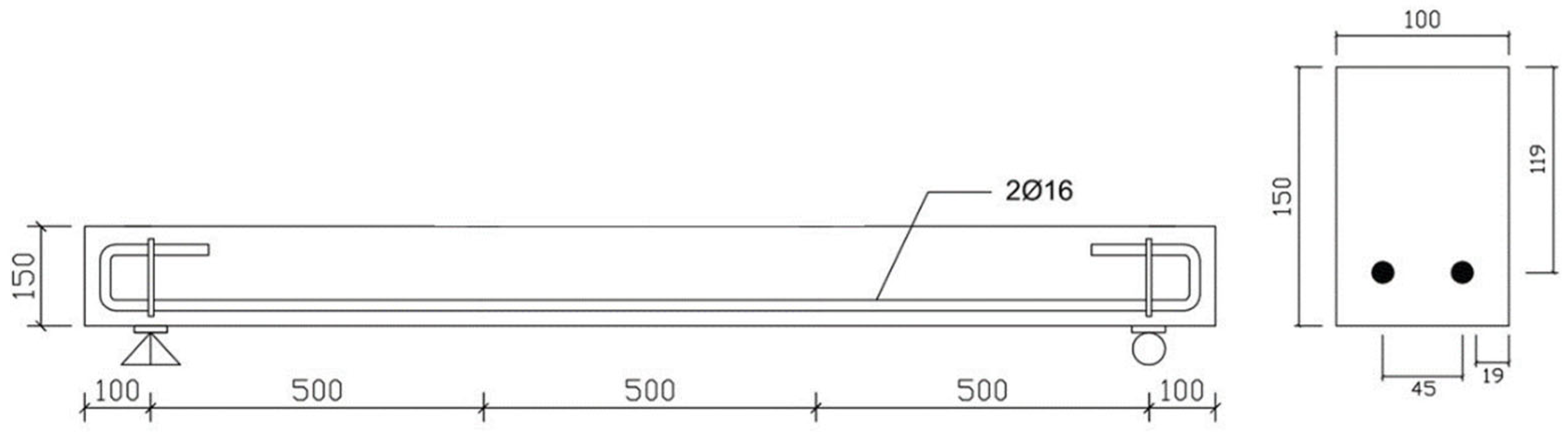
3.1.2. Beam Specimens
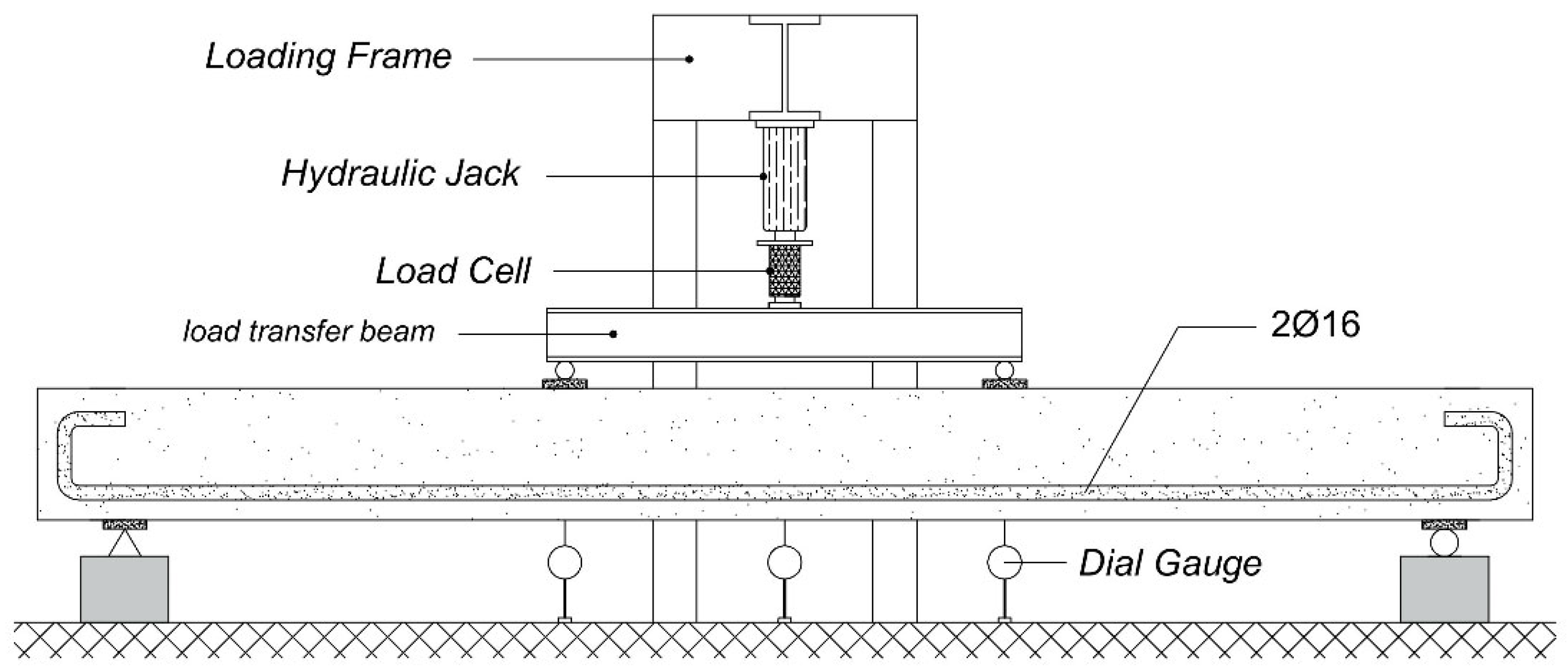
3.1.3. Testing Beam Specimens
3.2. Numerical Modeling
3.2.1. Materials Model
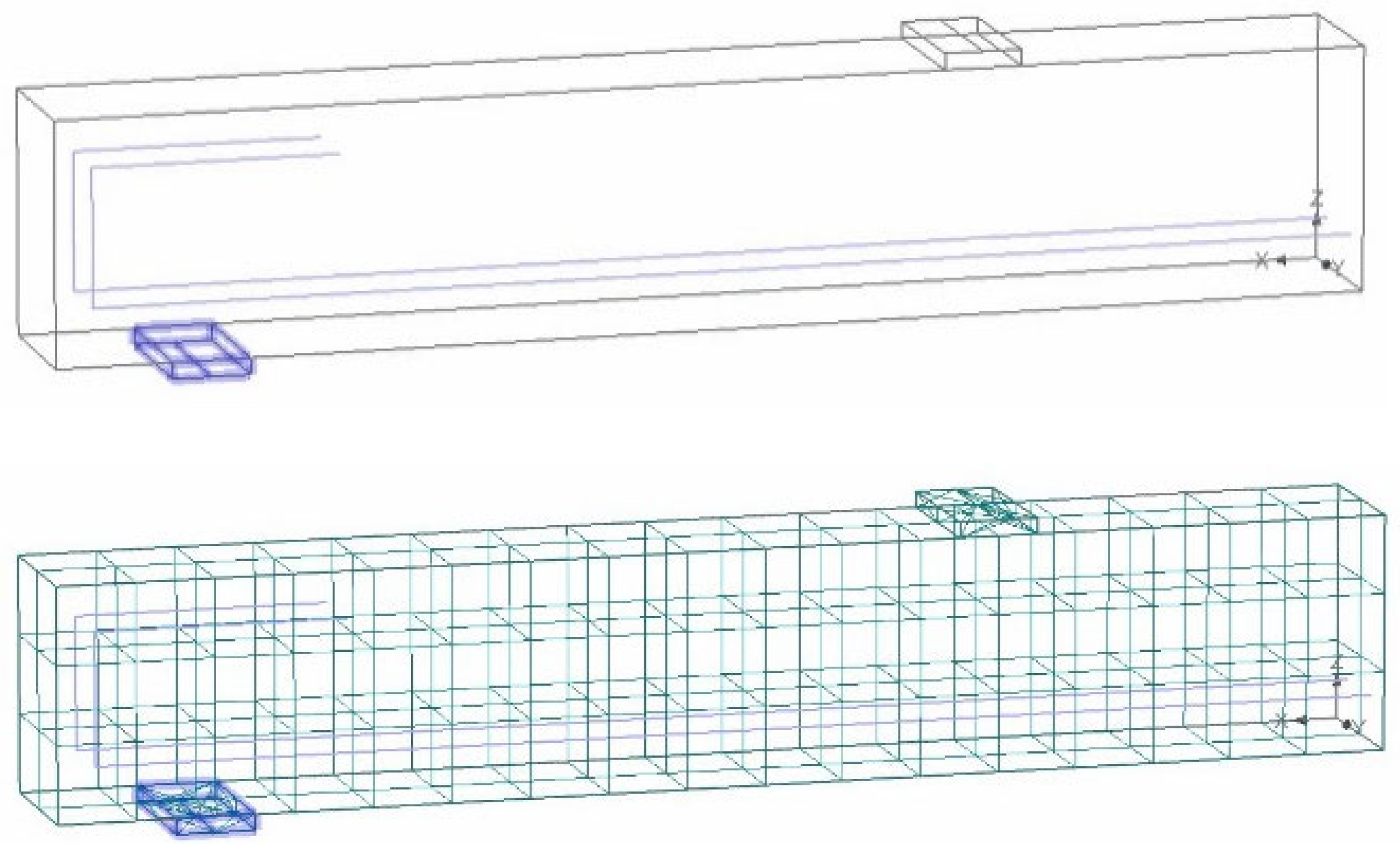
3.2.2. Beam Model and Finite Element Meshing
3.2.3. Loading
4. Results and Discussion
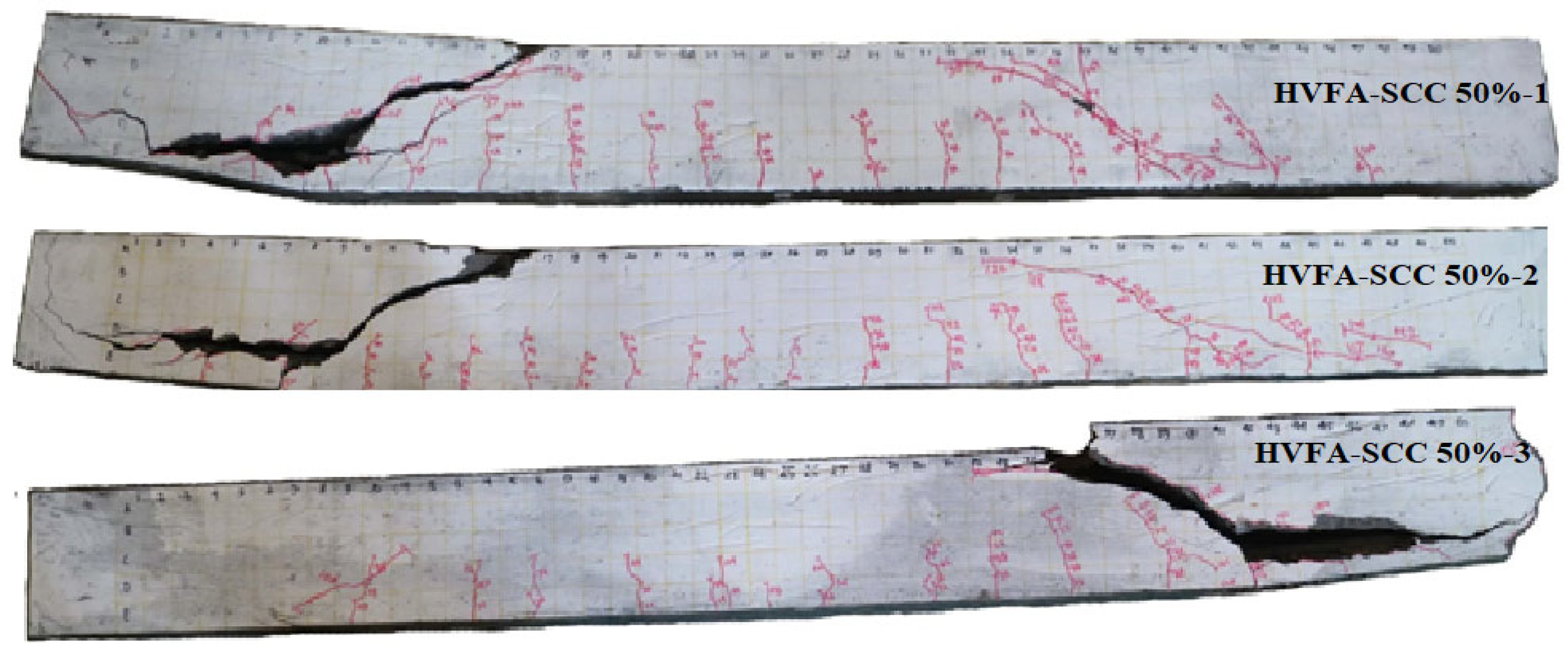
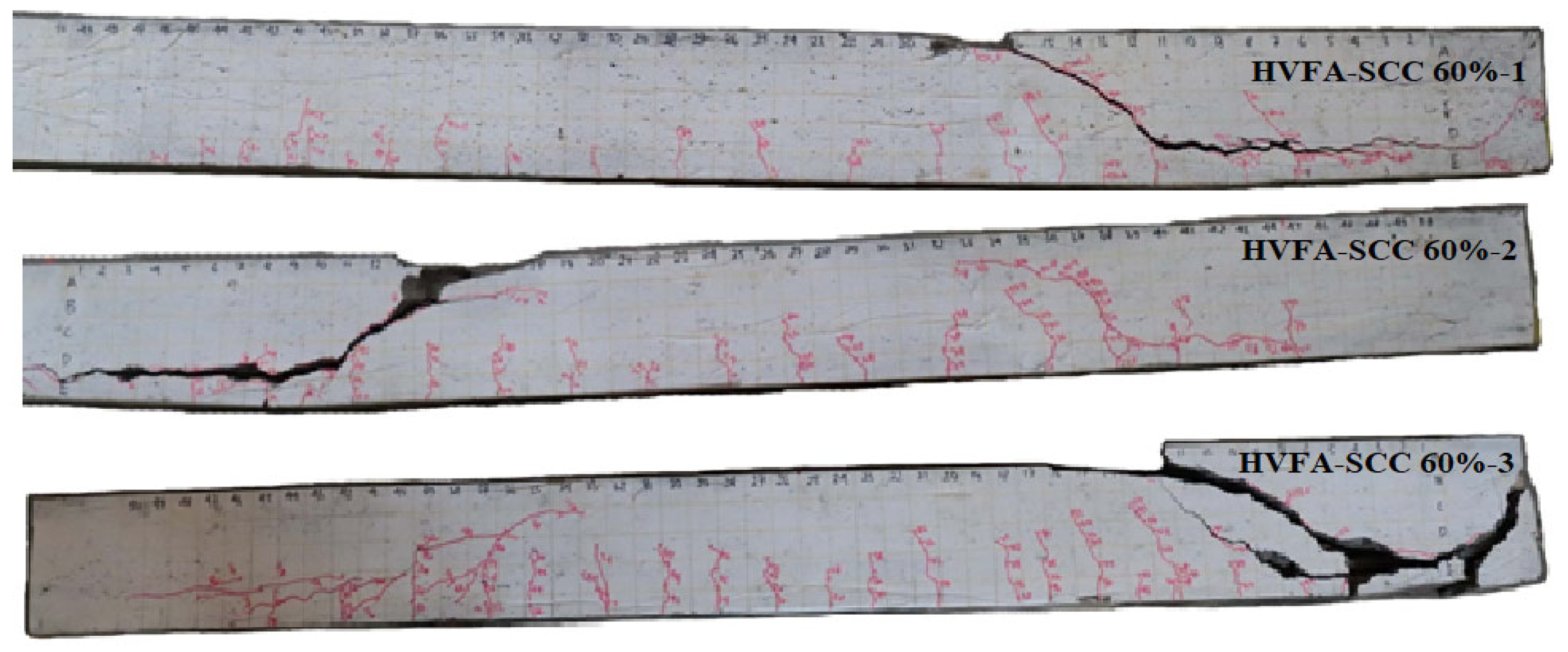
4.1. Cracking Failure Modes
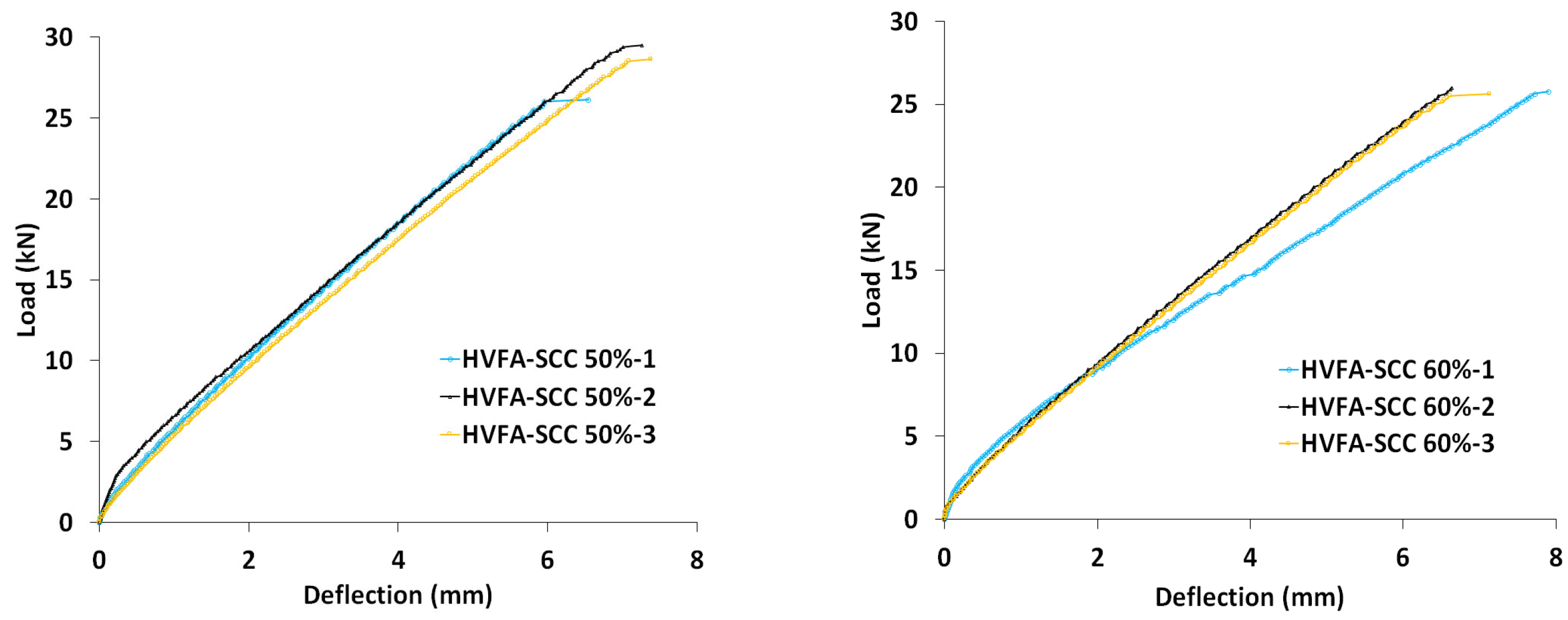
4.2. Load-Deflection
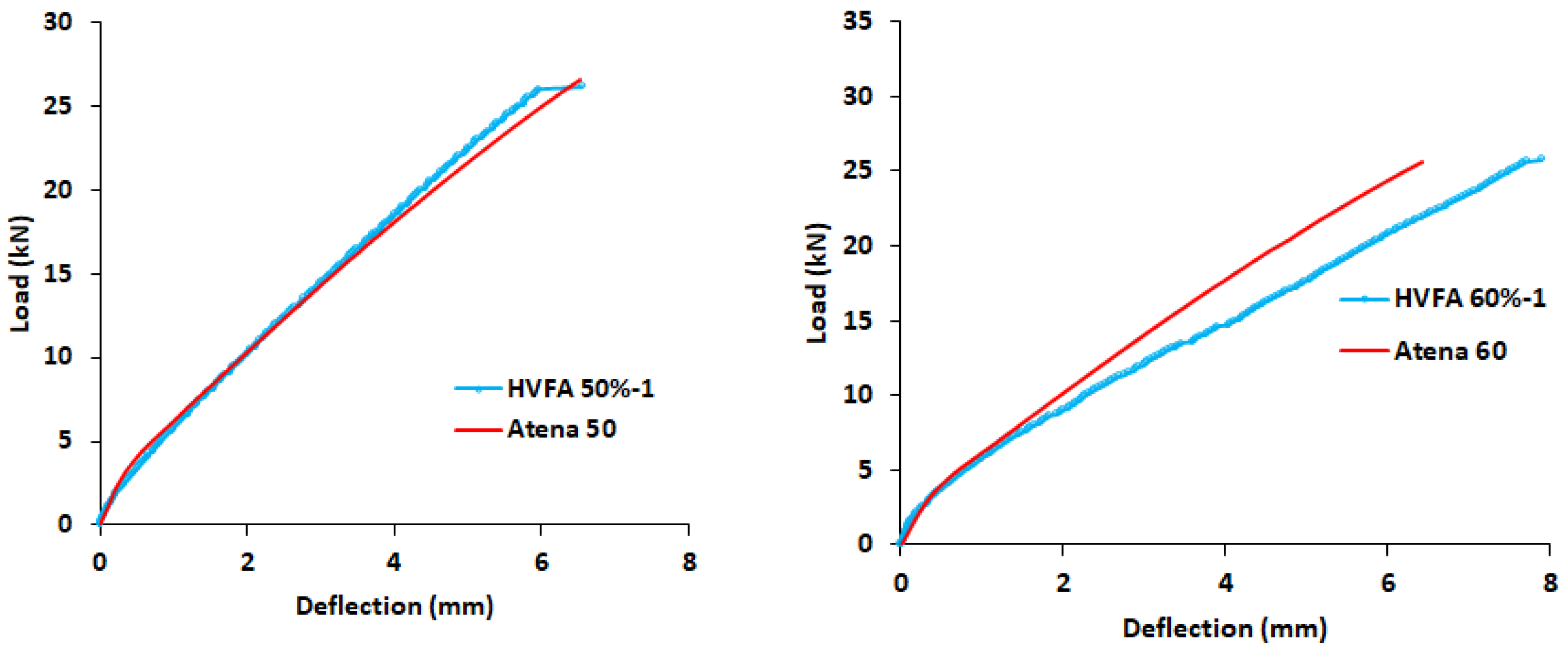
4.3. Experimental Results vs. Numerical Simulation
4.4. Parametric Studies
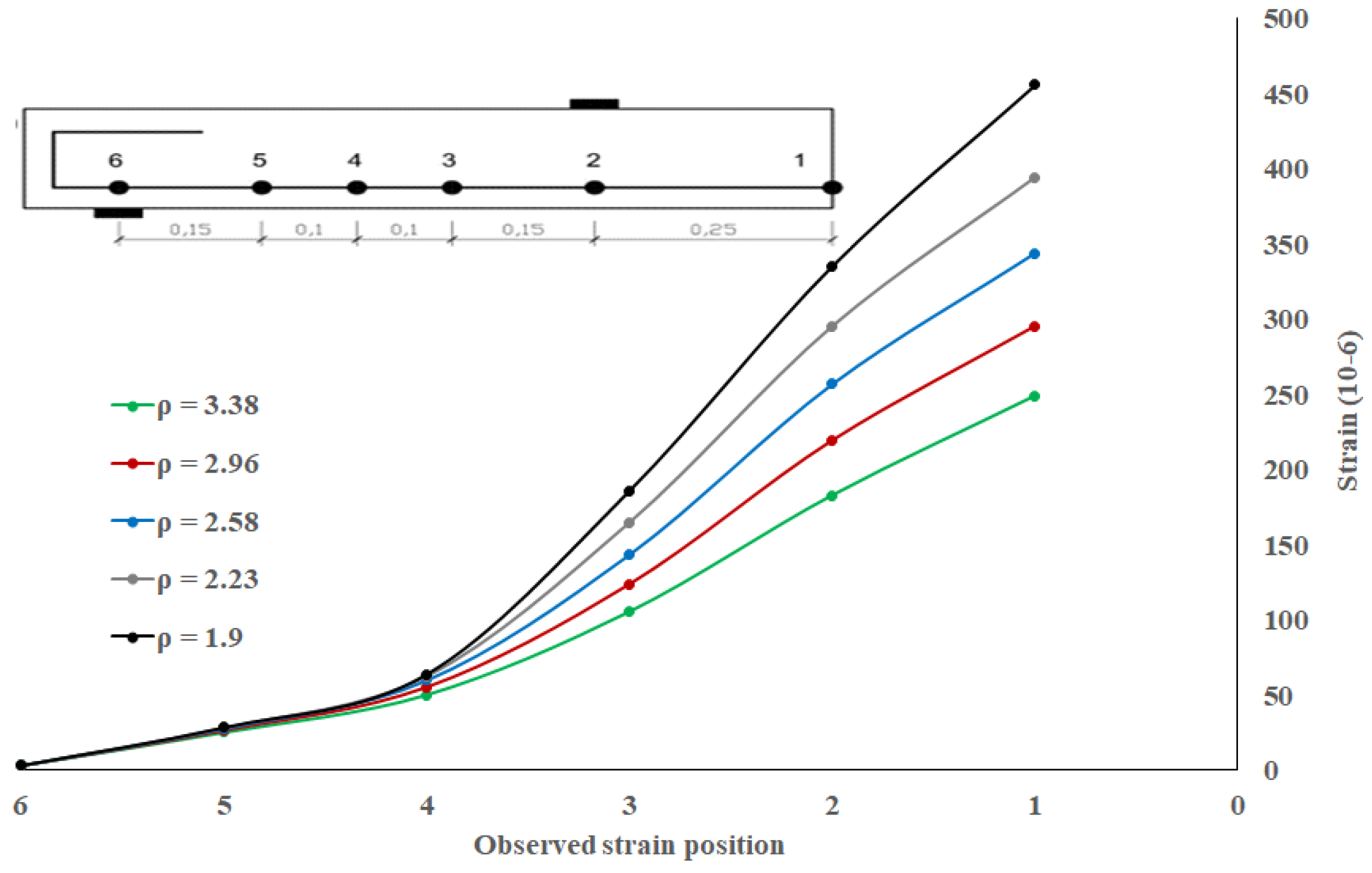
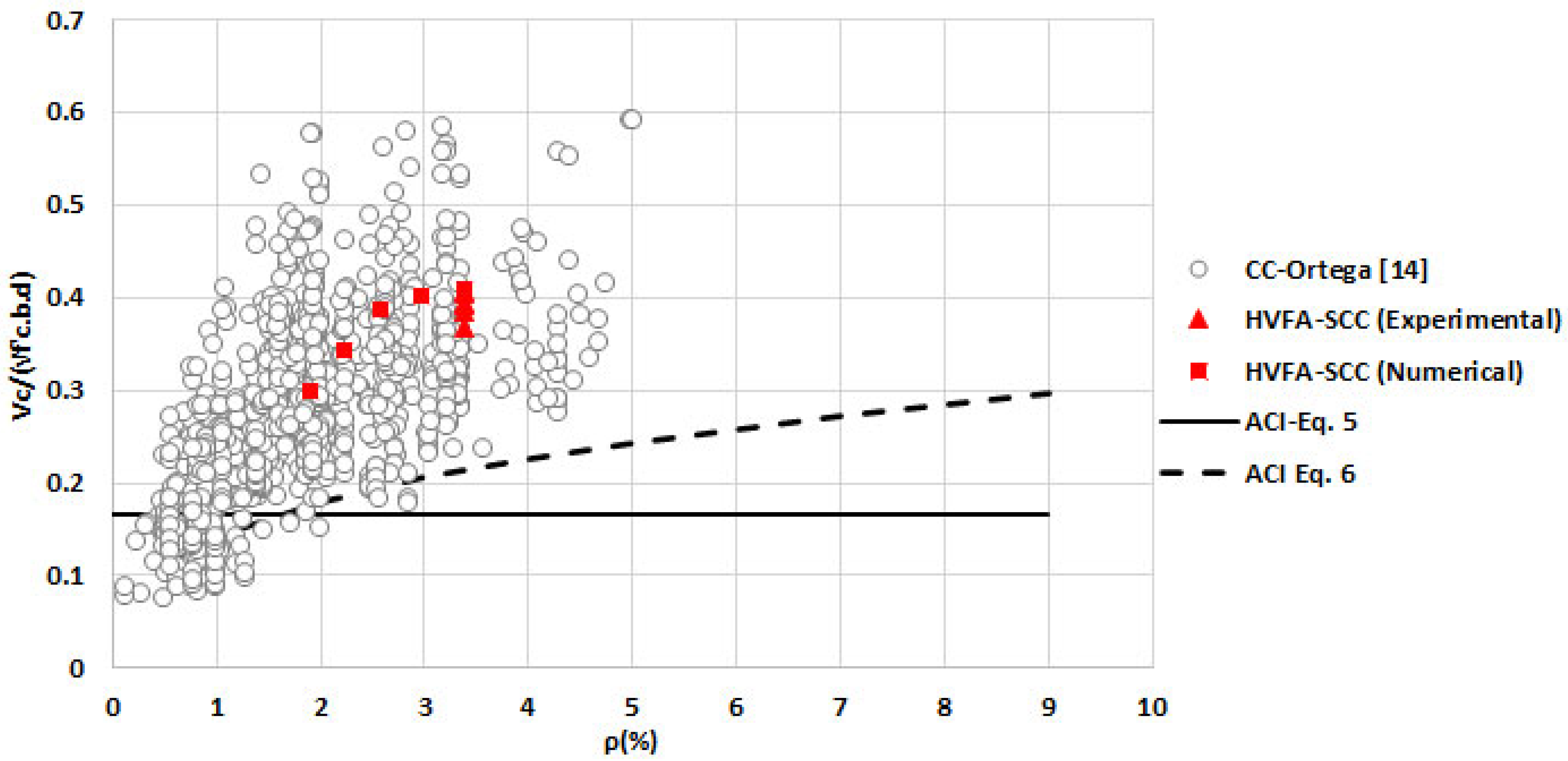
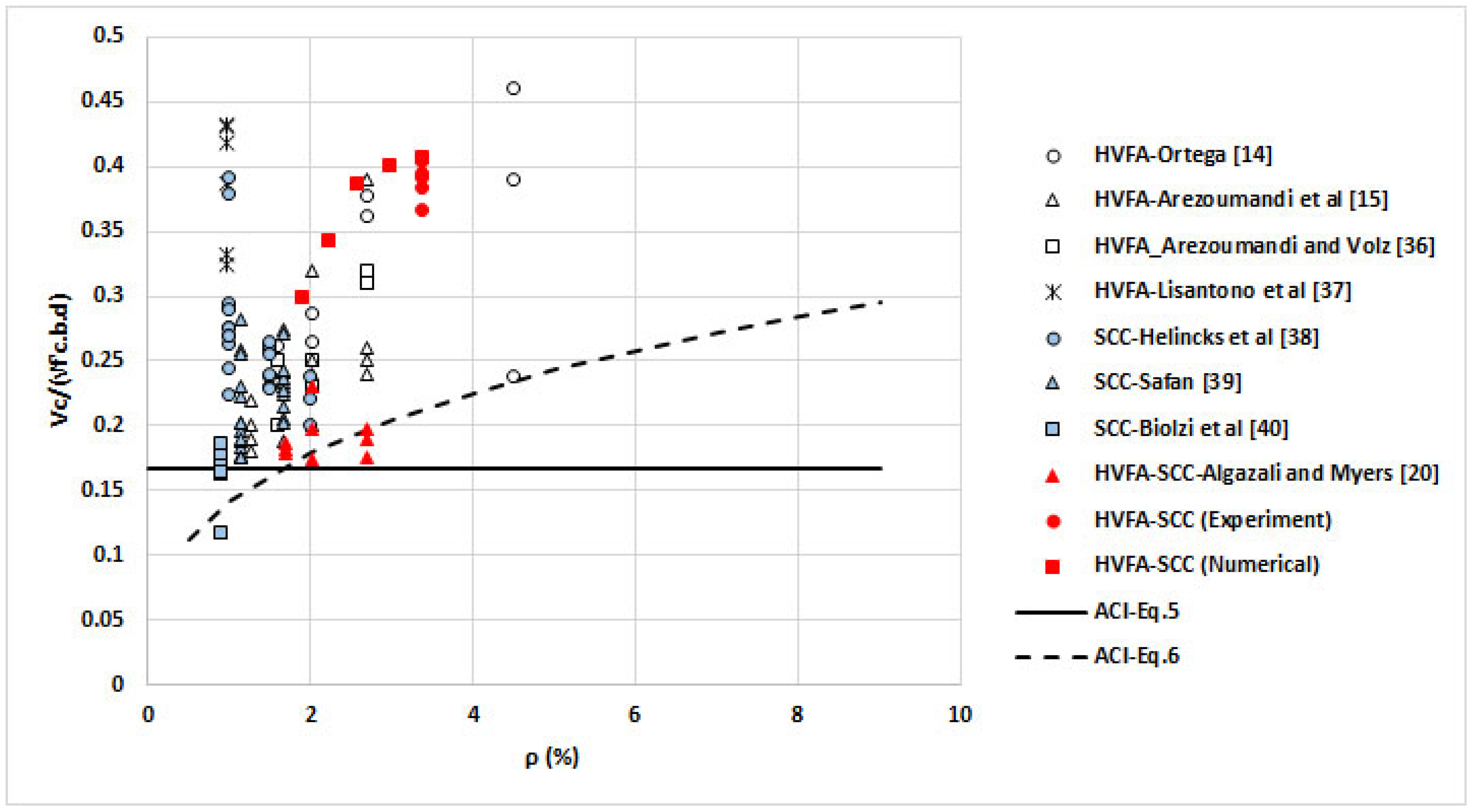
4.4.1. The Effect of the Reinforcement Ratio (ρ)
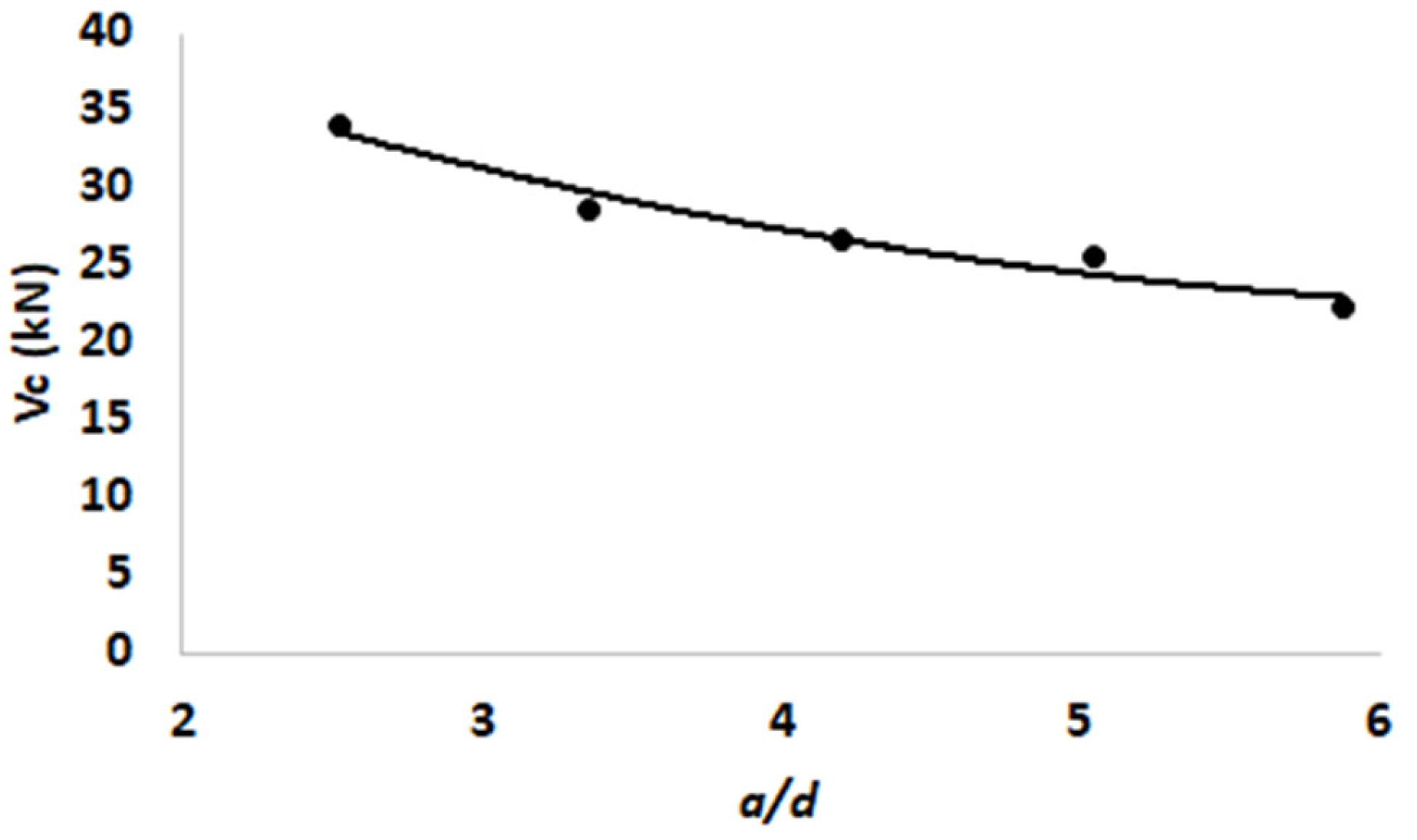
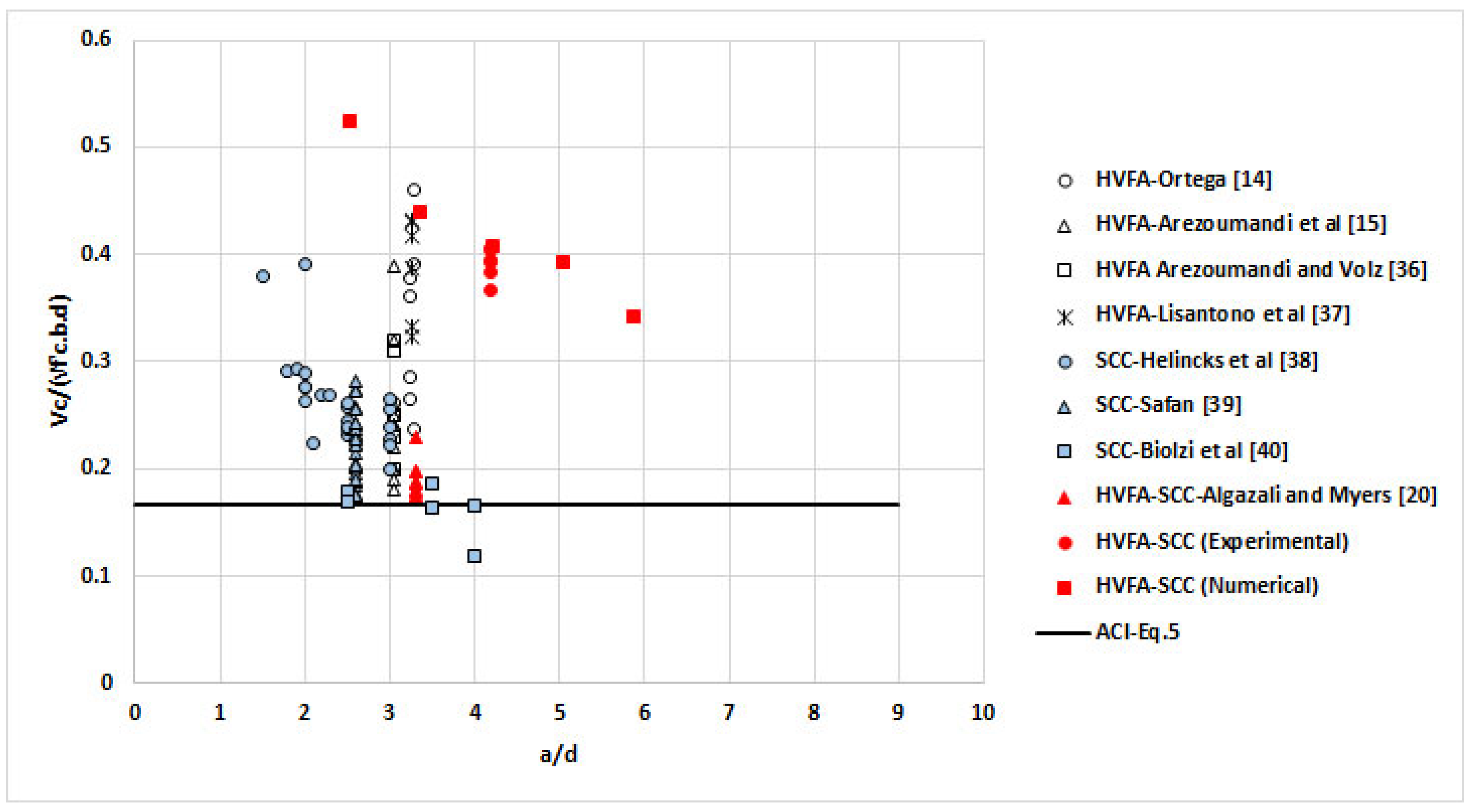
4.4.2. The Effect of Shear Span to Beam Effective Depth Ratio (a/d)
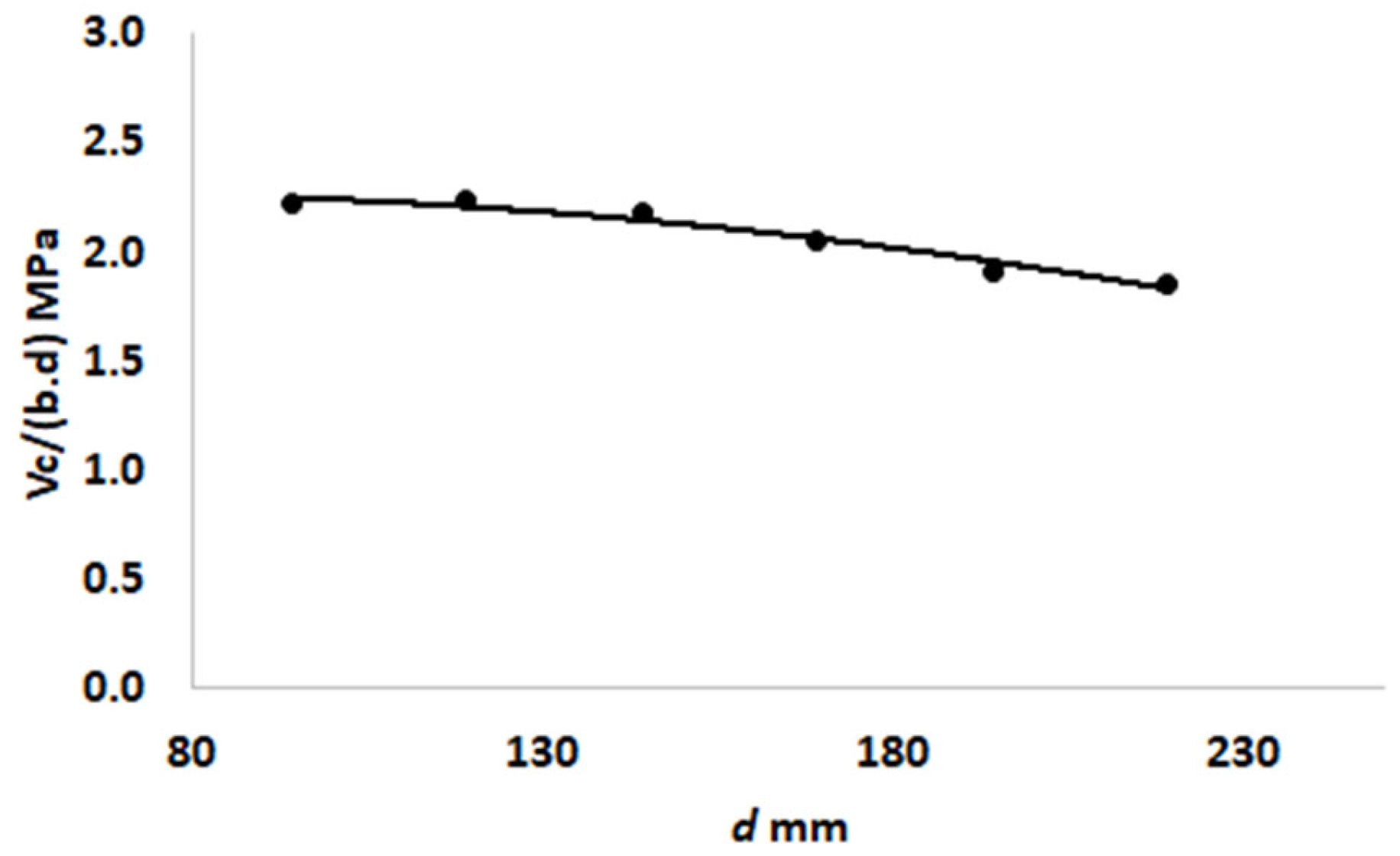
4.4.3. The Effect of Effective Beam Depth (d)
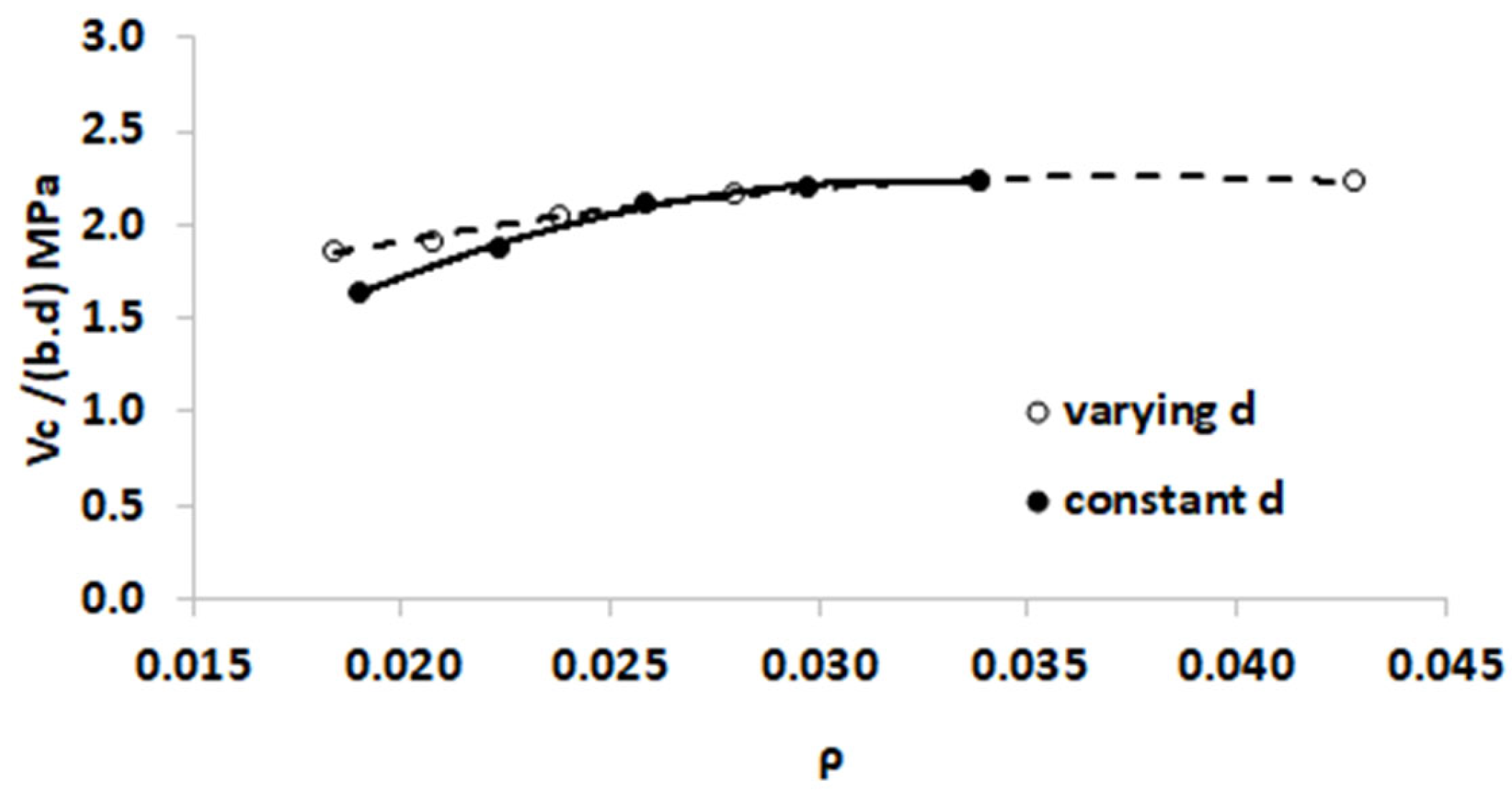
4.5. Shear Strength of HVFA-SCC
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mehta, P.K. High-performance, high-volume fly ash concrete for sustainable development. In Proceedings of the International Workshop on Sustainable Development and Concrete Technology, Beijing, China, 20–21 May 2004. [Google Scholar]
- Bilodeau, A.; Malhotra, V.M. High-volume fly ash system: Concrete solution for sustainable development. ACI Struct. J. 2000, 97, 41–48. [Google Scholar] [CrossRef]
- Malhotra, V.M. Superplasticized Fly Ash Concrete for Structural Applications. Concr. Int. 1979, 8, 28–31. [Google Scholar]
- Okamura, H.; Ouchi, M. Self-compacting concrete. J. Adv. Concr. Technol. 2003, 1, 5–15. [Google Scholar] [CrossRef]
- Sahmaran, M.; Yurtseven, A.; Yaman, I.O. Workability of hybrid fiber reinforced self-compacting concrete. Build. Environ. 2005, 40, 1672–1677. [Google Scholar] [CrossRef]
- Kristiawan, S.A.; Budi, A.S.; Hadi, A.N. Uniaxial compressive stress-strain behavior of self-compacting concrete with high-volume fly ash. Int. J. GEOMATE 2018, 14, 77–85. [Google Scholar] [CrossRef]
- Kristiawan, S.A.; Sunarmasto; Tyas, G.P. Degradation of self-compacting concrete (SCC) due to sulfuric acid attack: Experiment investigation on the effect of high volume fly ash content. IOP Conf. Ser. Mater. Sci. Eng. 2016, 107, 1. [Google Scholar] [CrossRef]
- Kristiawan, S.A.; Sunarmasto; Ridlo, M.M. Sorptivity of self-compacting concrete with high volume fly ash and its eco-mechanical-durability performance. IOP Conf. Ser. Mater. Sci. Eng. 2018, 442, 1. [Google Scholar] [CrossRef]
- Kristiawan, S.A.; Sangadji, S.; Sunarmasto. Eco-durability index of self-compacting concrete incorporating high volume fly ash. IOP Conf. Ser. Mater. Sci. Eng. 2019, 615, 1. [Google Scholar] [CrossRef]
- Bouzoubaâ, N.; Lachemi, M. Self-compacting concrete incorporating high volumes of class F fly ash: Preliminary results. Cem. Concr. Res. 2001, 31, 413–420. [Google Scholar] [CrossRef]
- Nehdi, M.; Pardhan, M.; Koshowski, S. Durability of self-consolidating concrete incorporating high-volume replacement composite cements. Cem. Concr. Res. 2004, 34, 2103–2112. [Google Scholar] [CrossRef]
- Chen, S.; Li, J. Shear behavior of reinforced high-strength concrete beams. ACI Struct. J. 2013, 110, 1112–1114. [Google Scholar]
- Ahmad, S.; Inaam, Q. Shear strength of self-compacting concrete and recycled aggregate concrete beams: An appraisal of design codes. Asian J. Civ. Eng. 2019, 20, 327–340. [Google Scholar] [CrossRef]
- Ordonez, C.A.O. Shear and Fracture Behavior of High-Volume Fly Ash Reinforced Concrete for Sustainable Construction. Doctoral Dissertation, Missouri University of Science and Technology, Rolla, MO, USA, 2012. [Google Scholar]
- Arezoumandi, M.; Volz, J.S.; Ortega, C.A.; Myers, J.J. Shear Behavior of High-Volume Fly Ash Concrete versus Conventional Concrete: Experimental Study. J. Struct. Eng. 2015, 141, 1506–1513. [Google Scholar] [CrossRef]
- Darmawan, M.S.; Bayuaji, R.; Sugiardjo, H.; Husin, N.A.; Affandhie, R.B.A. Shear Strength of Geopolymer Concrete Beams Using High Calcium Content Fly Ash in a Marine Environment. Buildings 2019, 9, 98. [Google Scholar] [CrossRef]
- Cladera, A. Shear design procedure for reinforced normal and high-strength concrete beams using artificial neural networks. Part I: Beams without stirrups. Eng. Struct. 2004, 26, 917–926. [Google Scholar] [CrossRef]
- Sadati, S.; Arezoumandi, M.; Khayat, K.H.; Volz, J.S. Shear performance of reinforced concrete beams incorporating recycled concrete aggregate and high-volume fl y ash. J. Clean. Prod. 2016, 115, 284–293. [Google Scholar] [CrossRef]
- Choulli, Y.; Marı, A.R.; Cladera, A. Shear behaviour of full-scale prestressed i-beams made with self compacting concrete. Mater. Struct. 2008, 41, 131–141. [Google Scholar] [CrossRef]
- Alghazali, H.H.; Myers, J.J. Shear behavior of full-scale high volume fly ash-self consolidating concrete (HVFA-SCC) beams. Constr. Build. Mater. 2017, 157, 161–171. [Google Scholar] [CrossRef]
- Joint ASCE-ACI Task Committee 426 on Shear and Diagonal Tension of the Committee on Masonry and Reinforced Concrete of the Structural Division. The Shear Strength of Reinforced Concrete Materials—Slabs. J. Struct. Div. 1974, 100, 1543–1591. [Google Scholar] [CrossRef]
- Joint ASCE-ACI Committee 445 on Shear and Torsion. Recent approaches to shear design of structural concrete on shear and torsion. J. Struct. Eng.-ASCE 1999, 124, 1375–1417. [Google Scholar]
- Jung, S.; Kim, K.S. Knowledge-based prediction of shear strength of concrete beams without shear reinforcement. Eng. Struct. 2008, 30, 1515–1525. [Google Scholar] [CrossRef]
- El-Sayed, A.K.; El-Salakawy, E.F.; Benmokrane, B. Shear capacity of high-strength concrete beams reinforced with FRP bars. ACI Struct. J. 2006, 103, 383–389. [Google Scholar] [CrossRef]
- Russo, G.; Somma, G.; Angeli, P. Design shear strength formula for high strength concrete beams. Mater. Struct. Constr. 2004, 37, 680–688. [Google Scholar] [CrossRef]
- Elsanadedy, H.M.; Abbas, H.; Almusallam, T.H. Shear strength prediction of HSC slender beams without web reinforcement. Mater. Struct. 2016, 49, 3749–3772. [Google Scholar] [CrossRef]
- MacGregor, J.G. Reinforced Concrete Mechanics & Design, 3rd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 1997. [Google Scholar]
- Park, R.; Paulay, T. Reinforced Concrete Strutures; John Wiley & Sons: New York, NY, USA, 1975; pp. 127–128. [Google Scholar]
- Collins, M.P.; Bentz, E.C.; Sherwood, E.G.; Xie, L. Adequate theory for the shear strength of reinforced concrete structures. In Morley Symposium on Concrete Plasticity and its Application; University of Cambridge, Department of Engineering: Cambridge, UK, 2007; pp. 75–94. [Google Scholar]
- Brown, M.D.; Bayrak, O.; Jirsa, J.O. Design for shear based on loading conditions. ACI Struct. J. 2006, 103, 541–550. [Google Scholar]
- Nilson, A.; Darwin, D.; Dolan, C. Design of Concrete Structures, 14th ed.; McGraw-Hill: New York, NY, USA, 2010. [Google Scholar]
- Bazant, Z.P.; Kim, J. Size Effect in Shear Failure of Longitudinally Reinforced Beams. Int. Concr. Abstr. Portal 1984, 81, 456–468. [Google Scholar]
- ACI Committee. ACI 318-19. Building Code Requirements for Structural Concrete; American Concrete Institute: Farmington Hills, MI, USA, 2019. [Google Scholar]
- Alhussainy, F.; Hasan, H.A.; Rogic, S.; Sheikh, M.N.; Hadi, M.N.S. Direct tensile testing of Self-Compacting Concrete. Constr. Build. Mater. 2016, 112, 903–906. [Google Scholar] [CrossRef]
- Syroka-Korol, E.; Tejchman, J. Experimental investigations of size effect in reinforced concrete beams failing by shear. Eng. Struct. 2014, 58, 63–78. [Google Scholar] [CrossRef]
- Arezoumandi, M.; Volz, J.S. Effect of fl y ash replacement level on the shear strength of high-volume fl y ash concrete beams. J. Clean. Prod. 2013, 59, 120–130. [Google Scholar] [CrossRef]
- Lisantono, A.; Wigroho, H.Y.; Purba, R.A. Shear behaviour of high volume fly ash concrete as replacement of Portland cement in RC beam. Proc. Eng. 2017, 171, 80–87. [Google Scholar] [CrossRef]
- Helincks, P.; Boel, V.; de Corte, W.; de Schutter, G.; Desnerck, P. Structural behaviour of powder-type self-compacting concrete: Bond performance and shear capacity. Eng. Struct. 2013, 48, 121–132. [Google Scholar] [CrossRef]
- Safan, M. Shear Strength of Self-compacting Concrete Containing Different Fillers and Coarse Aggregates. Can. J. Civ. Eng. 2012, 39, 760–770. [Google Scholar] [CrossRef]
- Biolzi, L.; Cattaneo, S.; Mola, F. Bending-shear response of self-consolidating and high-performance reinforced concrete beams. Eng. Struct. 2014, 59, 399–410. [Google Scholar] [CrossRef]






| Oxide | SiO2 | Fe2O3 | Al2O3 | CaO | MgO | K2O | SO3 | TiO2 | P2O5 | Cl | MnO | SrO | BaO | Nd2O3 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Concentration (%) | 31.76 | 21.12 | 11.29 | 15.02 | 3.85 | 2.35 | 1.67 | 1.59 | 1.01 | 0.56 | 0.27 | 0.14 | 0.15 | 0.12 |
| Composition | Type | Unit | Mixtures | |
|---|---|---|---|---|
| HVFA-SCC 50% | HVFA-SCC 60% | |||
| Cement | type 1 | kg/m3 | 250 | 200 |
| Fly Ash | type C | kg/m3 | 250 | 300 |
| Fine Aggregate | river sand | kg/m3 | 870.3 | 877.6 |
| Coarse Aggregate | crushed stone | kg/m3 | 787.7 | 781.3 |
| Water | NA | L/m3 | 150 | 150 |
| HRWR | m-glenium sky 8614 | L/m3 | 10 | 10 |
| % of fly ash replacement | 50 | 60 | ||
| Property | Unit | Mix ID | Target Value | |
|---|---|---|---|---|
| HVFA-SCC 50% | HVFA-SCC 60% | |||
| Slump flow | mm | 700 | 700 | 650–800 |
| T50 | s | 3.16 | 3.11 | 2–5 |
| L-Box | mm/mm | 0.90 | 0.94 | 0.8–1.0 |
| V funnel | s | 9.34 | 9.50 | 6–12 |
| Material | Cylinder Compressive Strength * (MPa) | Cube Compressive Strength ** (MPa) | Tensile Strength * (MPa) | Yield Strength * (MPa) | Modulus of Elasticity *** (MPa) | Material Model |
|---|---|---|---|---|---|---|
| HVFA-SCC 50% | 35.18 (2.35) | 41.38 | 2.06 (0.05) | NA | 34,470 | 3D NonLinear Cementitious Material 2 |
| HVFA-SCC 60% | 31.84 (2.32) | 37.46 | 2.21 (0.11) | NA | 33,170 | |
| Reinforcement φ 16 | NA | NA | NA | 402.9 (10.3) | 200,000 | Reinforcement-Bilinear |
| Steel Plate | NA | NA | NA | NA | 200,000 | 3D Elastic Isotropic |
| Beam ID | fc’ (MPa) | * First Flexural Crack (kN) | * First Primary Diagonal Crack (kN) | Ultimate Shear (kN) | Maximum Deflection (mm) |
|---|---|---|---|---|---|
| HVFA-SCC 50%-1 | 32.68 | 3.00 | 23.13 | 26.13 | 6.55 |
| HVFA-SCC 50%-2 | 37.34 | 4.00 | 25.25 | 29.50 | 7.27 |
| HVFA-SCC 50%-3 | 35.51 | 4.25 | 25.75 | 28.63 | 7.39 |
| HVFA-SCC 60%-1 | 30.45 | 4.00 | 22.50 | 25.75 | 7.90 |
| HVFA-SCC 60%-2 | 30.56 | 4.50 | 23.50 | 26.00 | 6.64 |
| HVFA-SCC 60%-3 | 34.52 | 3.50 | 21.88 | 25.63 | 7.13 |
| Beam ID | ft (MPa) | ATENA | Vtest/Vatena | δtest/δatena | |
|---|---|---|---|---|---|
| Vult (kN) | δ (mm) | ||||
| HVFA-SCC 50% | 2.06 | 26.58 | 6.63 | 1.06 | 1.08 |
| HVFA-SCC 60% | 2.21 | 25.66 | 6.42 | 1.01 | 1.13 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Budi, A.S.; Safitri, E.; Sangadji, S.; Kristiawan, S.A. Shear Strength of HVFA-SCC Beams without Stirrups. Buildings 2021, 11, 177. https://doi.org/10.3390/buildings11040177
Budi AS, Safitri E, Sangadji S, Kristiawan SA. Shear Strength of HVFA-SCC Beams without Stirrups. Buildings. 2021; 11(4):177. https://doi.org/10.3390/buildings11040177
Chicago/Turabian StyleBudi, Agus Setiya, Endah Safitri, Senot Sangadji, and Stefanus Adi Kristiawan. 2021. "Shear Strength of HVFA-SCC Beams without Stirrups" Buildings 11, no. 4: 177. https://doi.org/10.3390/buildings11040177
APA StyleBudi, A. S., Safitri, E., Sangadji, S., & Kristiawan, S. A. (2021). Shear Strength of HVFA-SCC Beams without Stirrups. Buildings, 11(4), 177. https://doi.org/10.3390/buildings11040177





