Upward Unsteady-State Solidification of Dilute Al–Nb Alloys: Microstructure Characterization, Microhardness, Dynamic Modulus of Elasticity, Damping, and XRD Analyses
Abstract
1. Introduction
2. Experimental Procedure
3. Results and Discussions
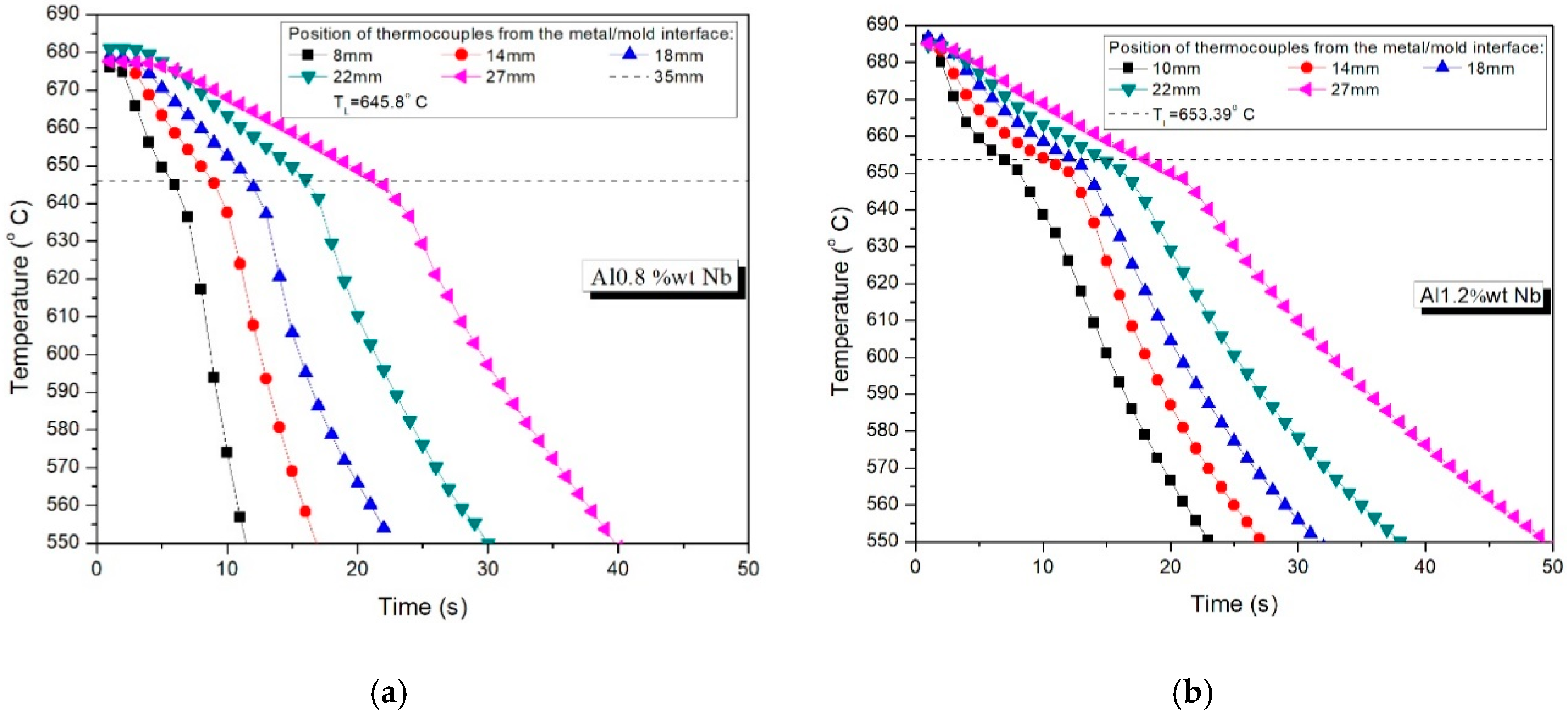
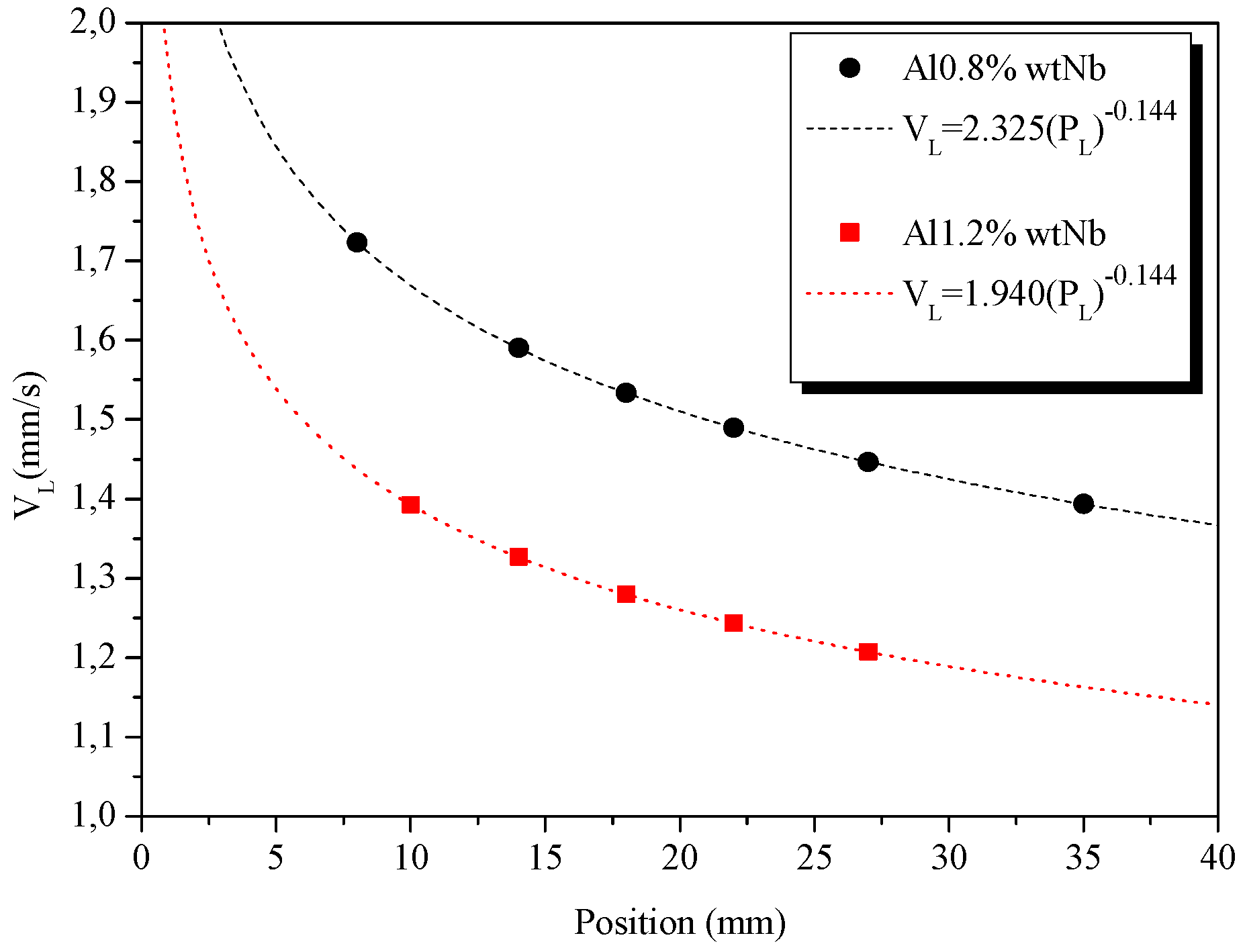
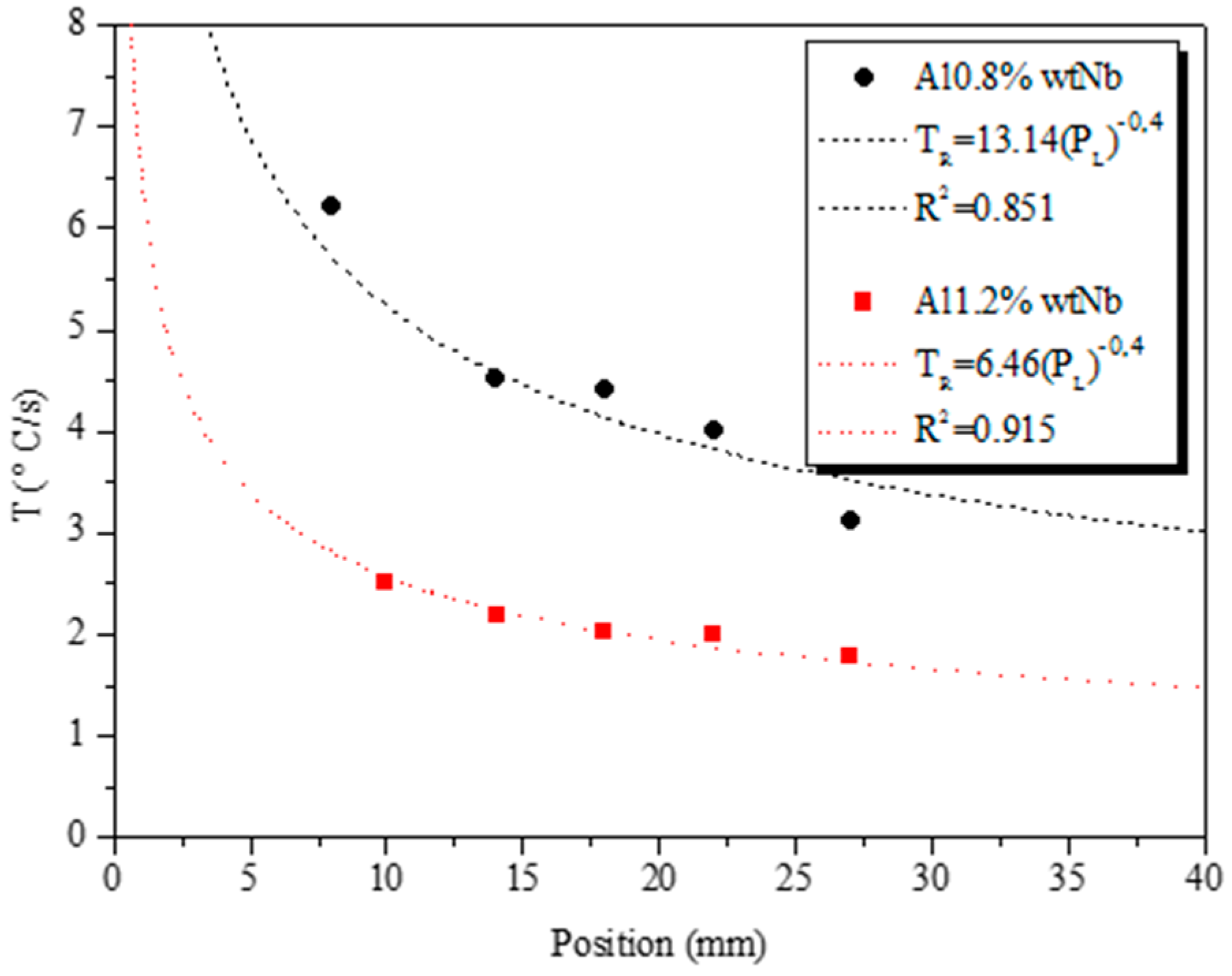
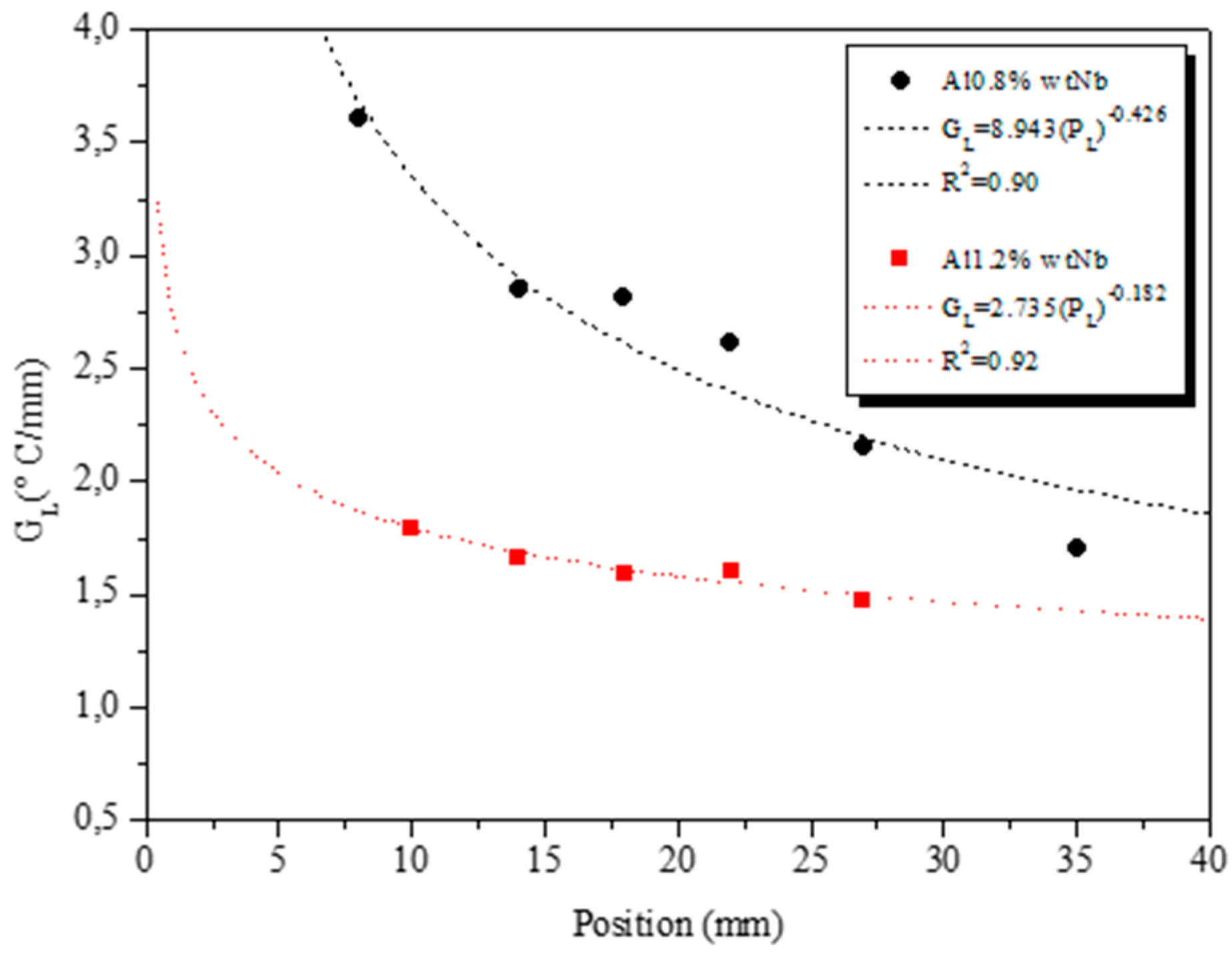
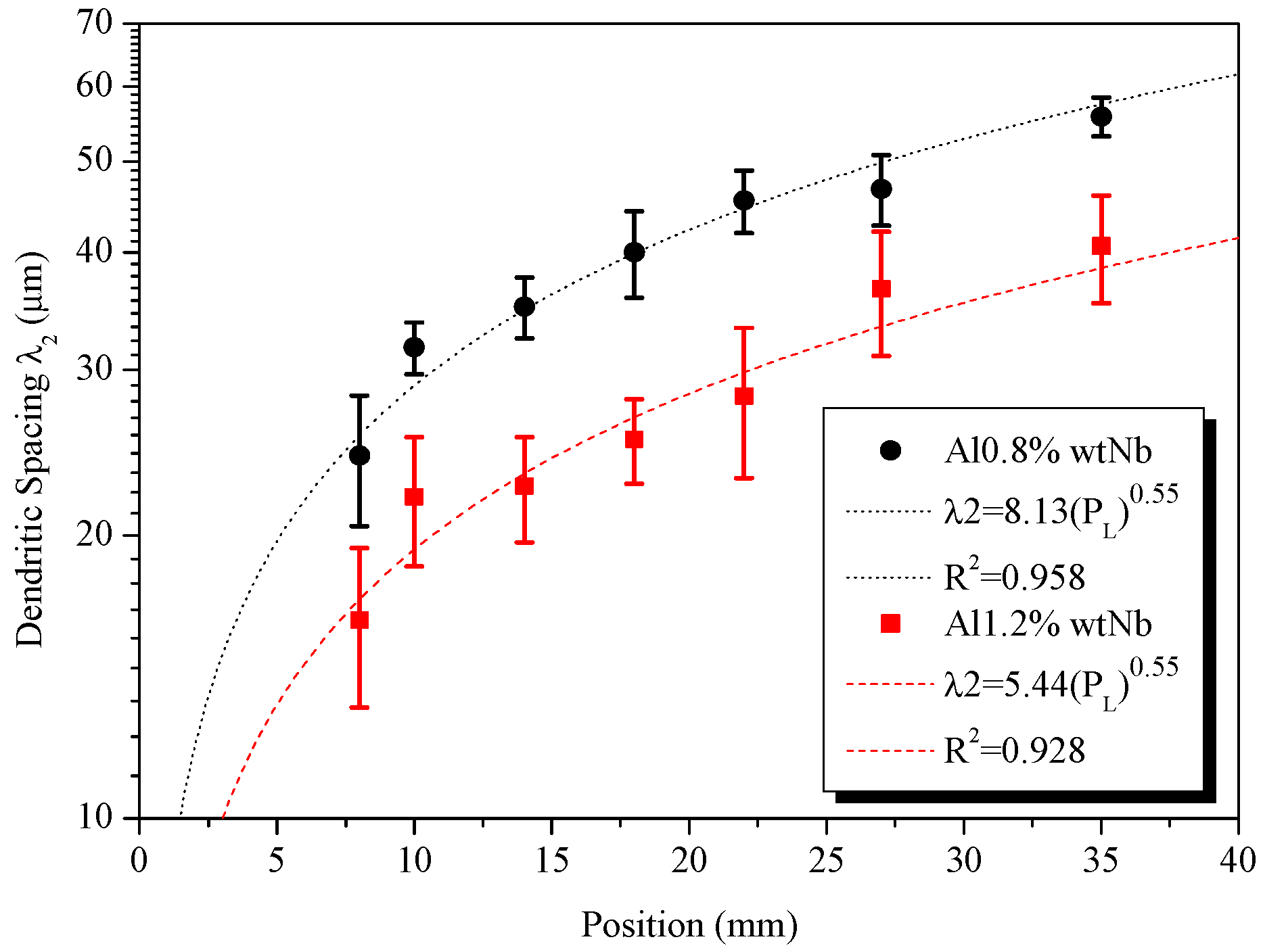
3.1. Thermal Variables
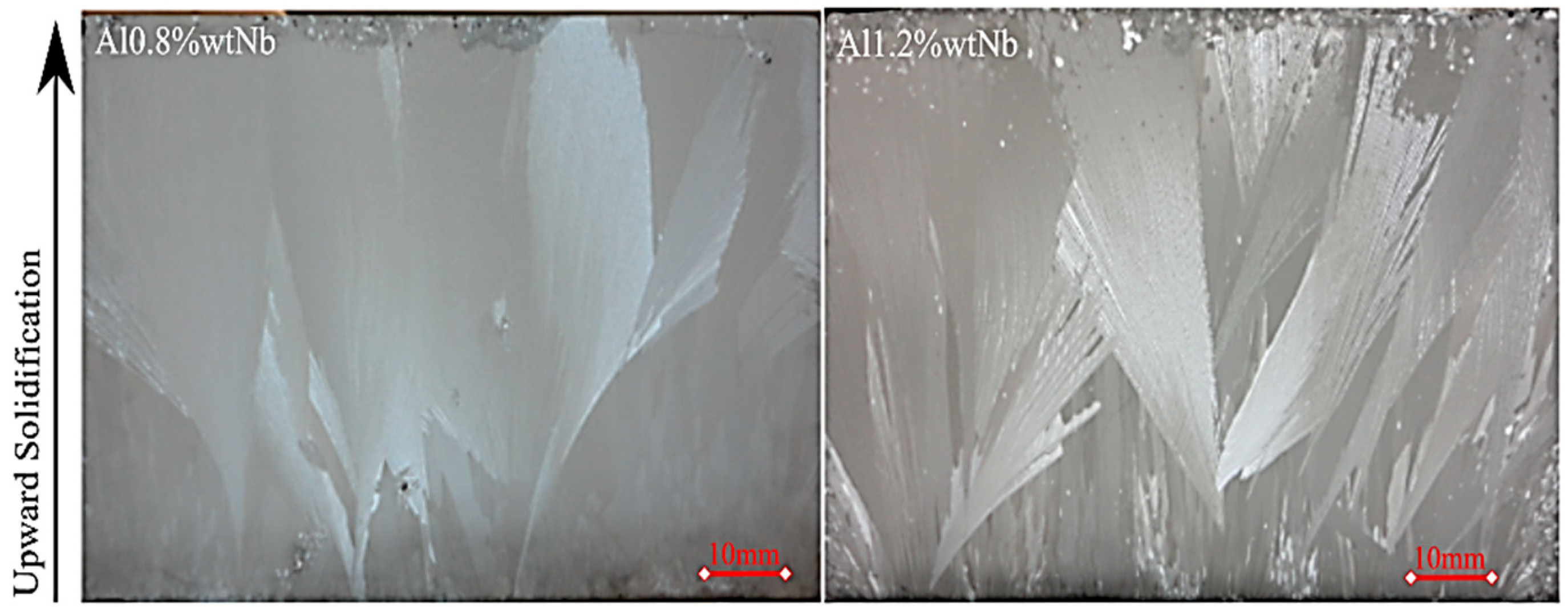
3.2. Macrostructure
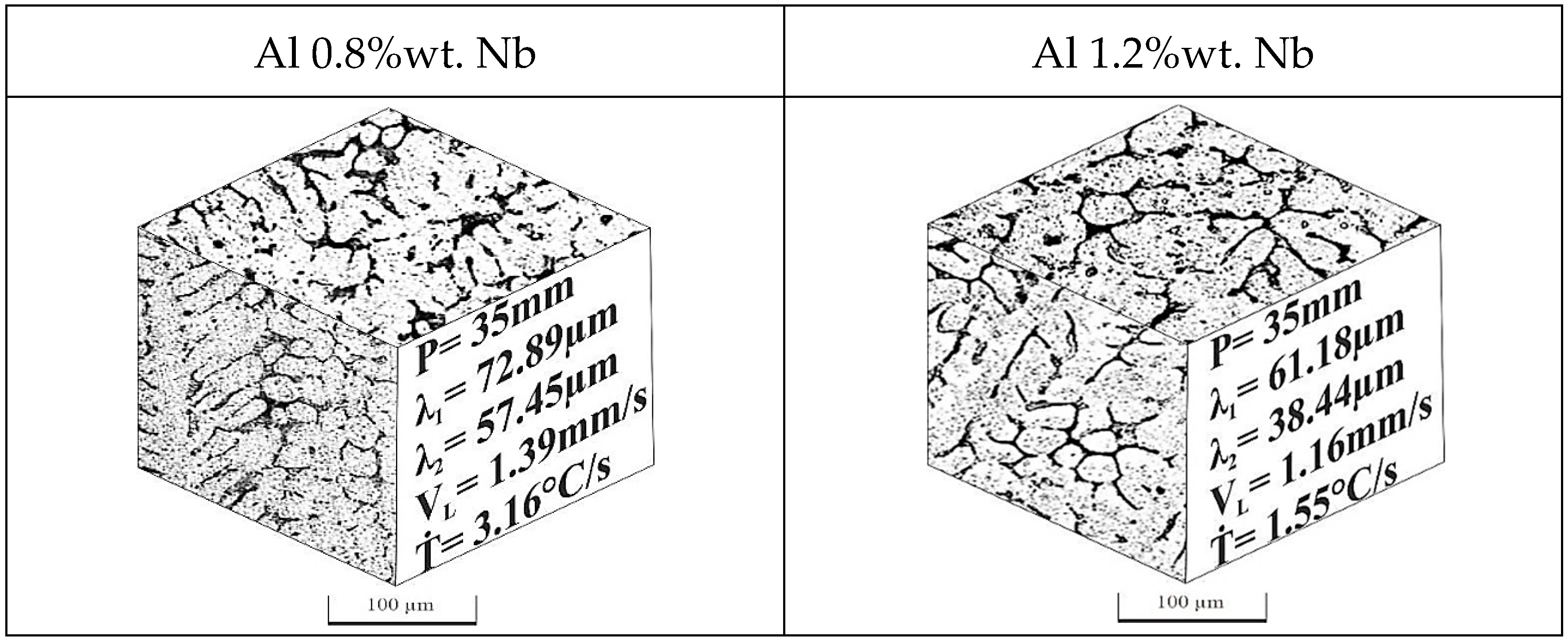
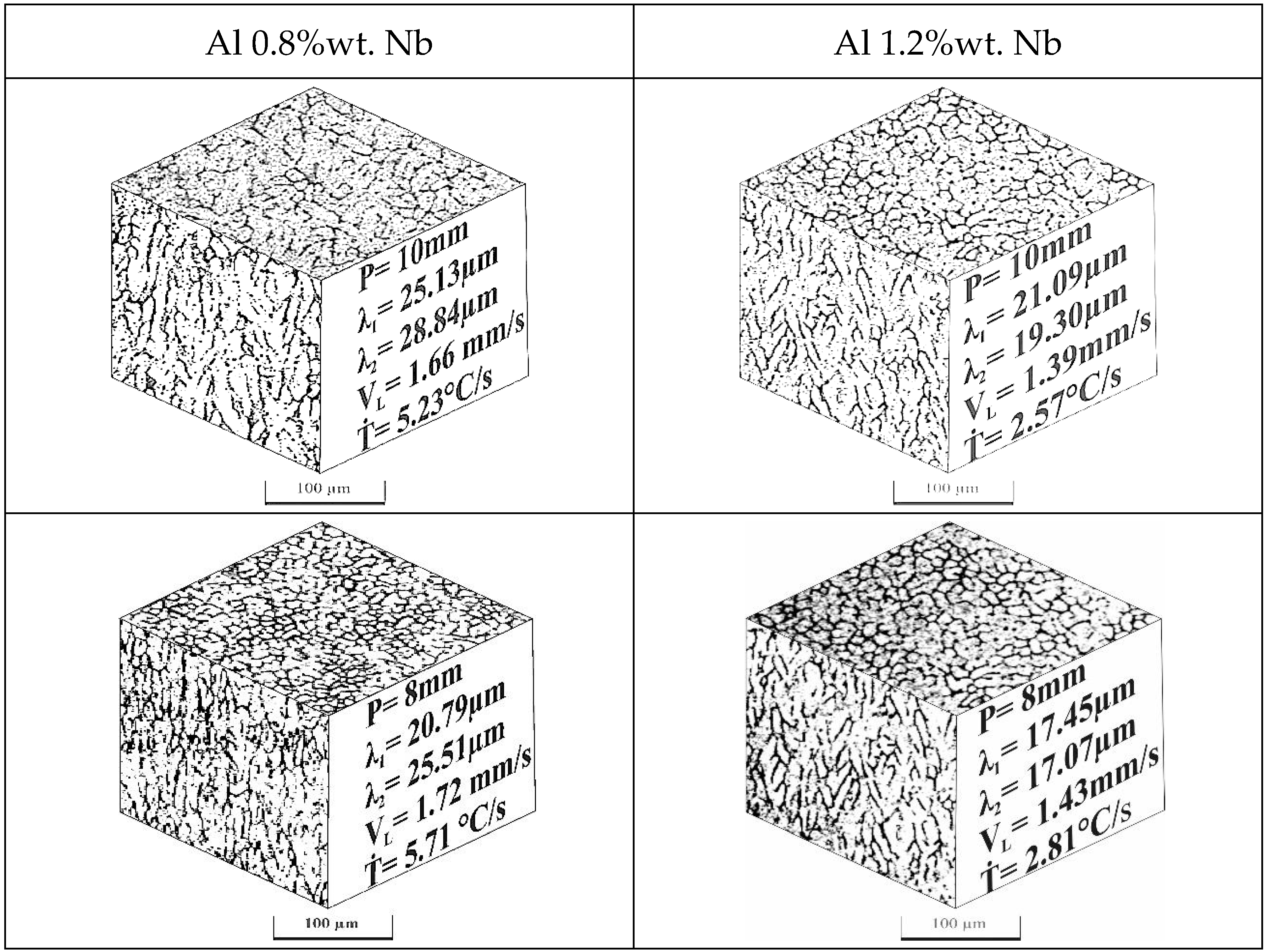
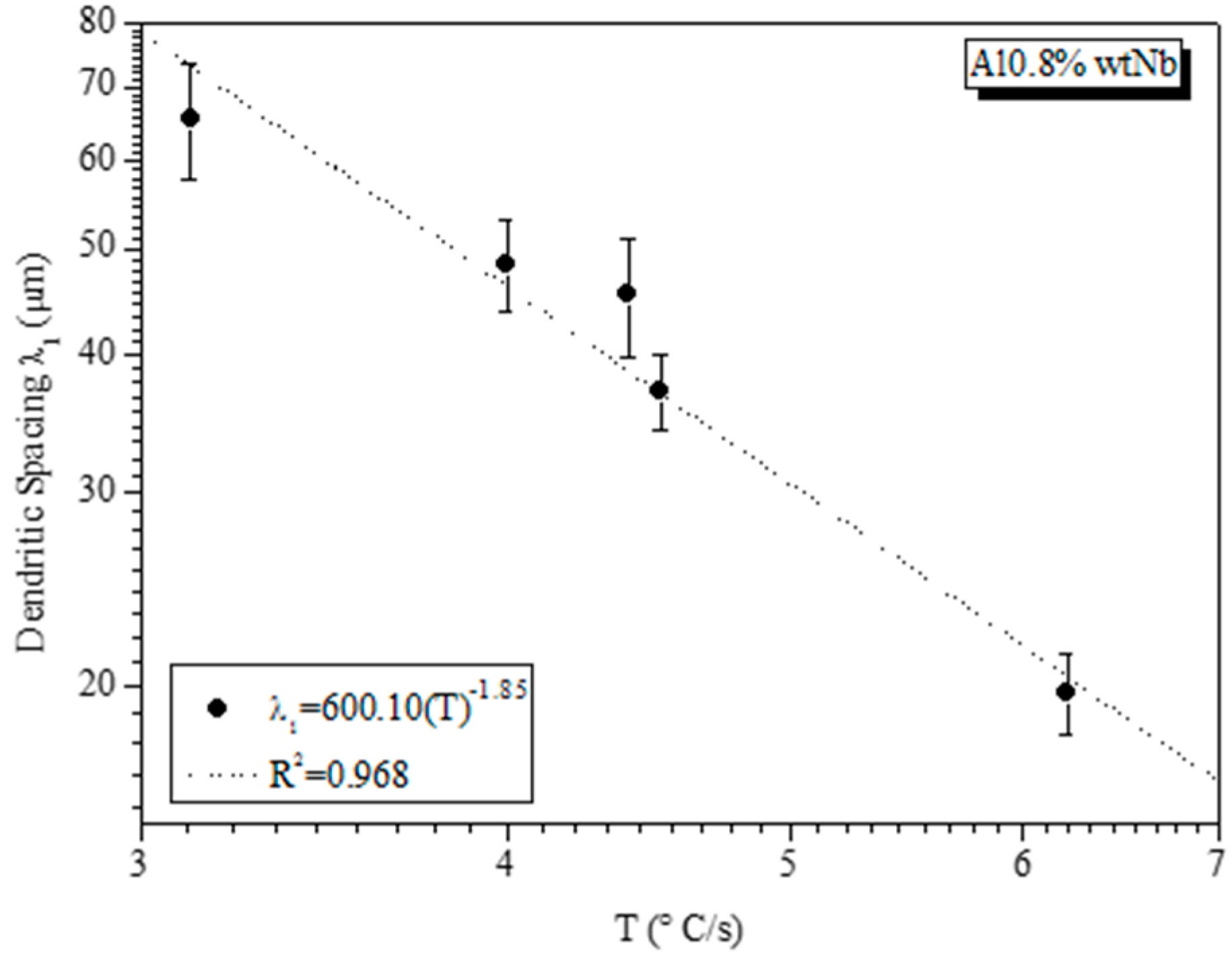
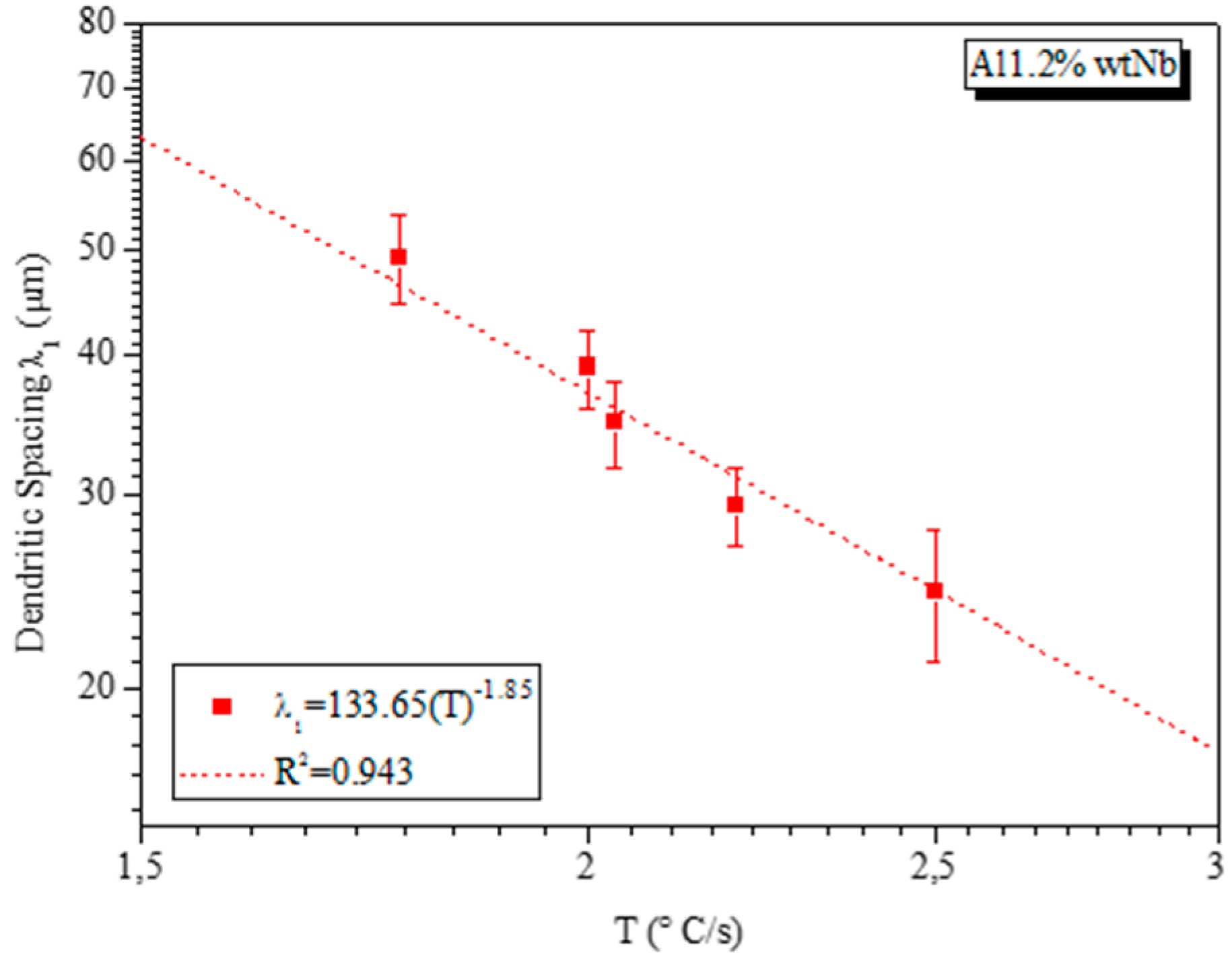
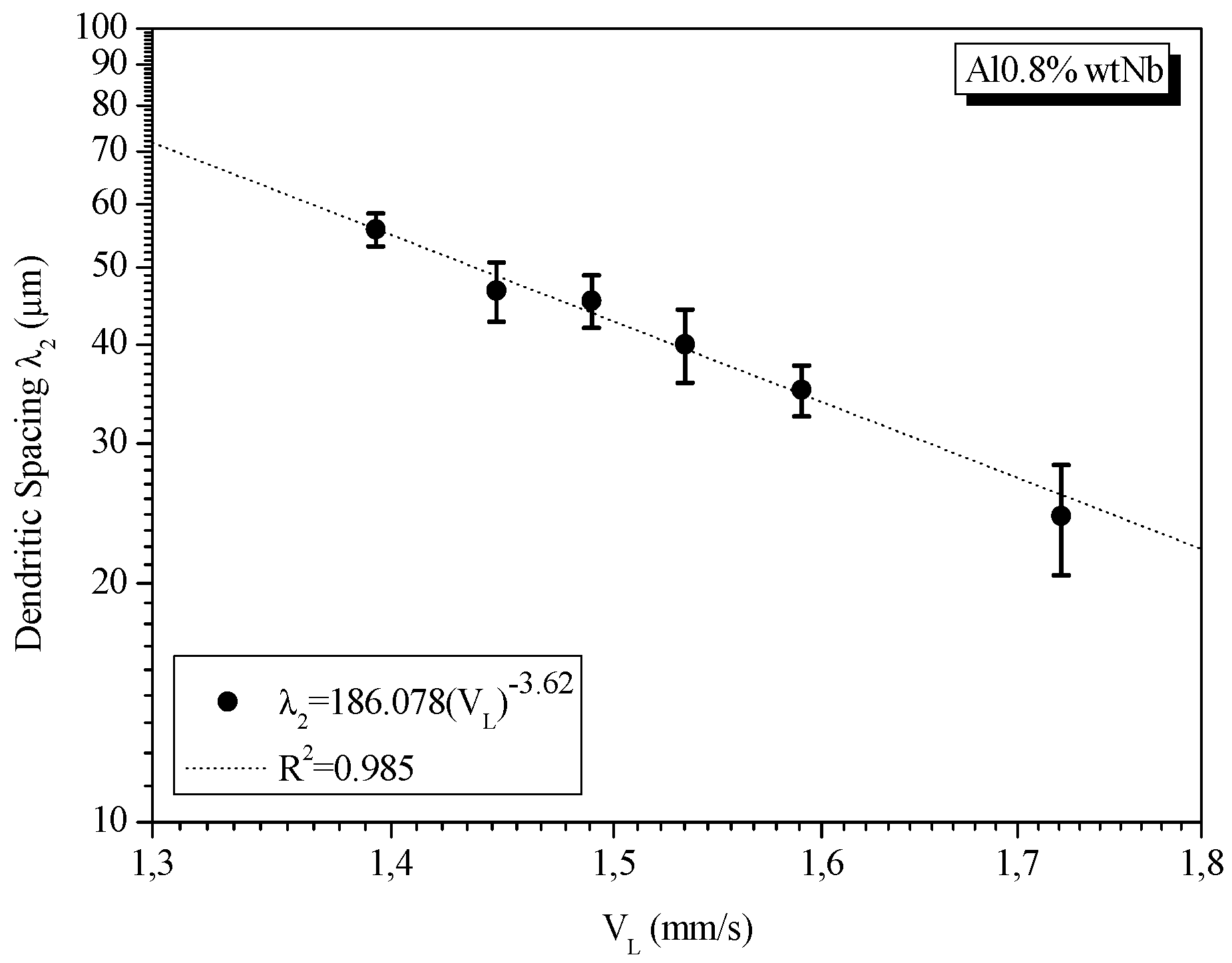
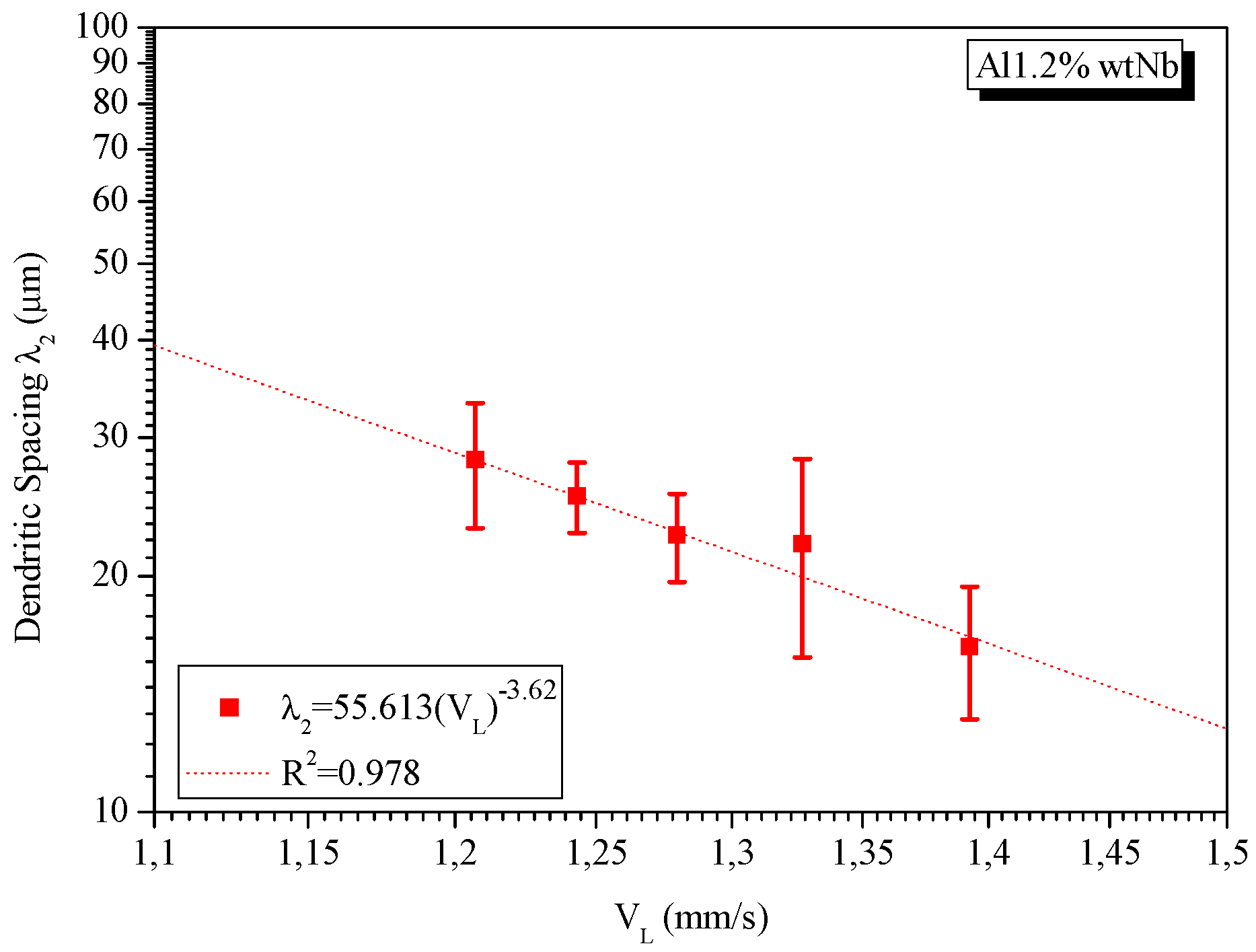
3.3. Microstructure
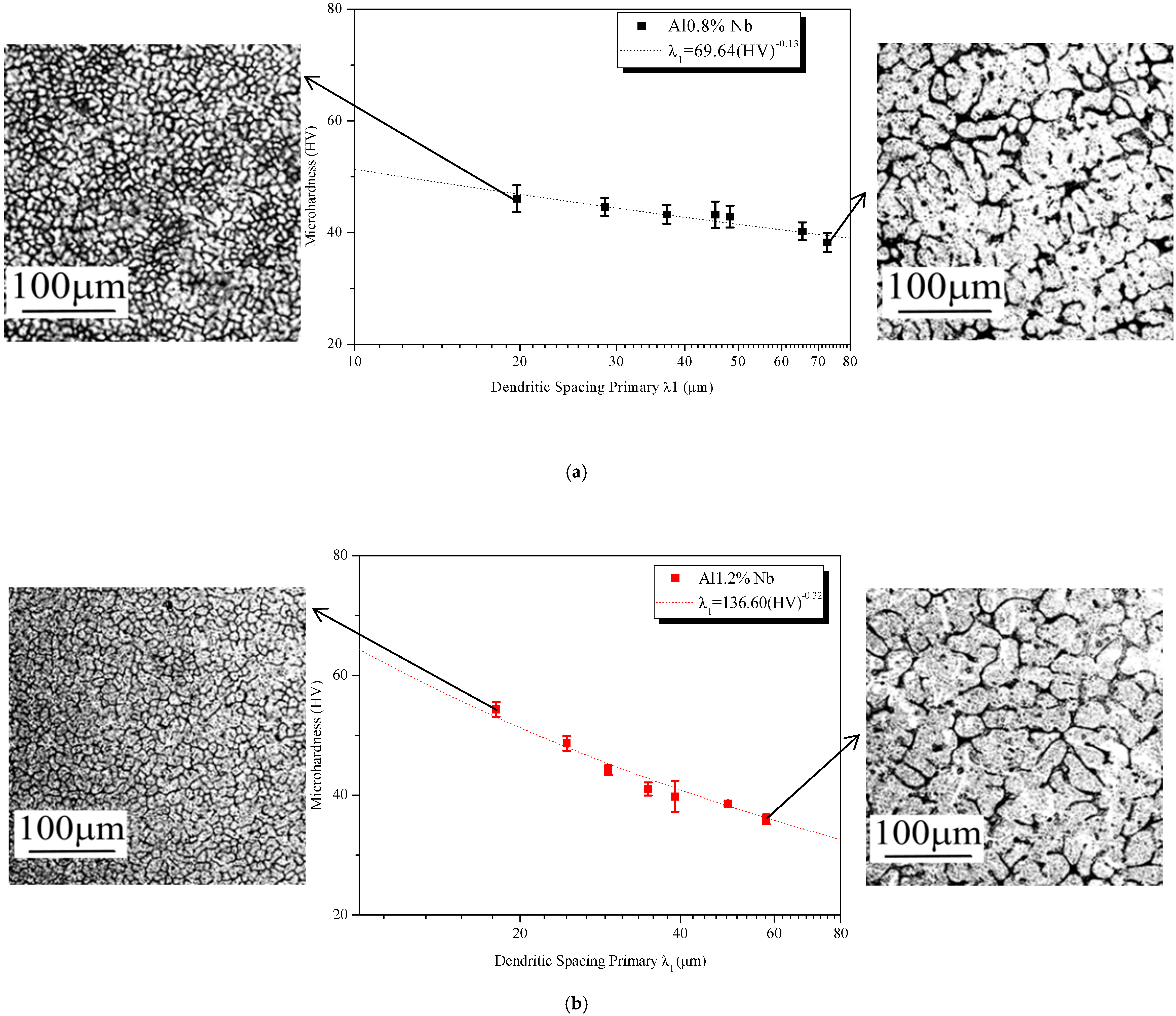
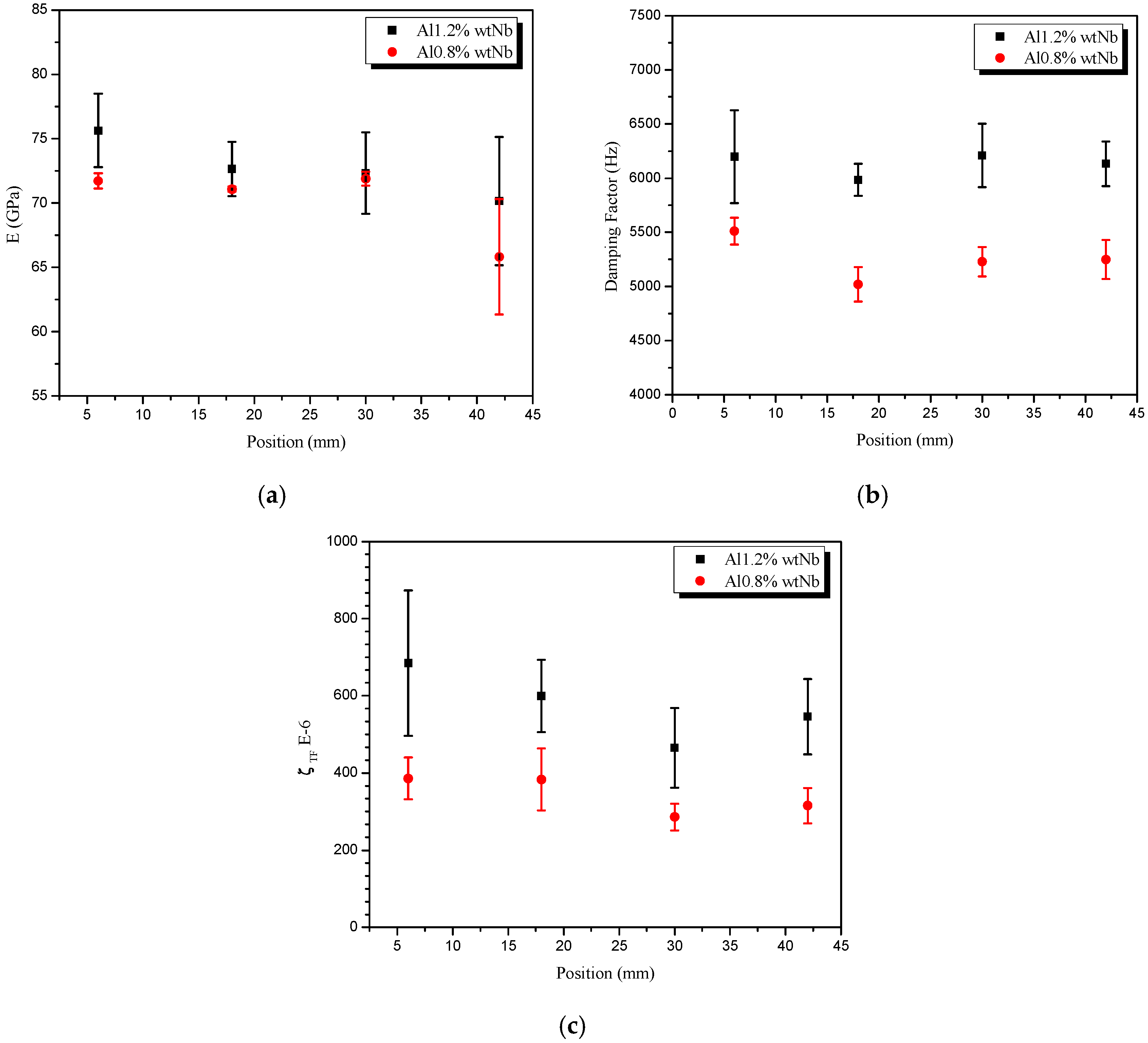
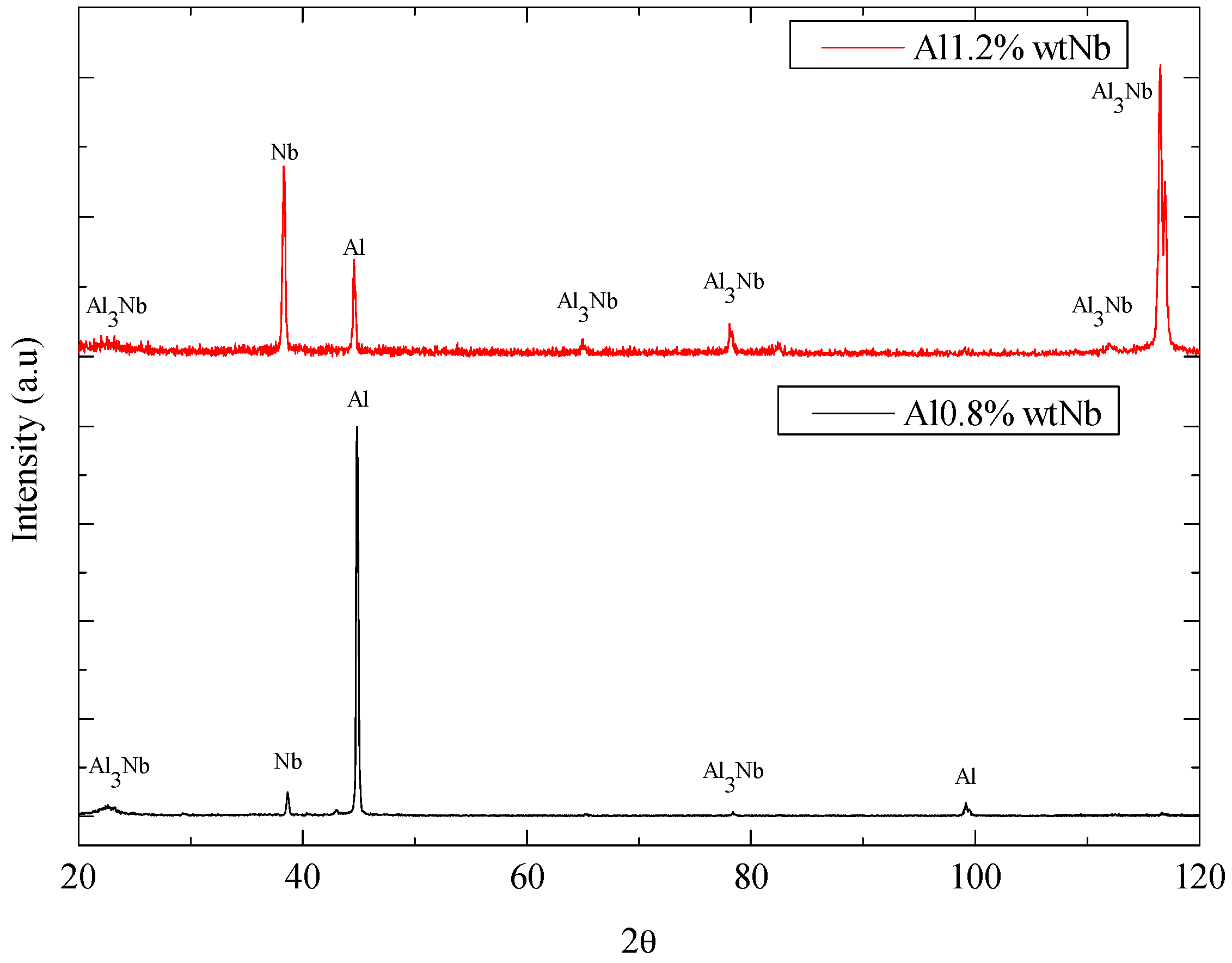
3.4. Characterisation: Microhardness, Dynamic Modulus of Elasticity, Damping Frequency, and XRD
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Zhang, J.; Cui, C.; Han, M.; Chen, J.; Xu, N.; Liu, L.; Fu, H. Microstructure and property of Czochralski-grown Si–TaSi2 eutectic in situ composite for field emission. J. Cryst. Growth 2005, 276, 92–96. [Google Scholar] [CrossRef]
- Shafii, M.B.; Dehkordi, E.A.; Moghadam, M.E.; Koochesfahani, M.M. Koochesfahani. Using nucleators to control freckles in unidirectional solidification. Exp. Therm. Fluid Sci. 2009, 33, 1209–1215. [Google Scholar] [CrossRef]
- Bergeon, N.; Trivedi, R.; Billia, B.; Echebarria, B.; Karma, A.; Liu, S.; Weiss, C.; Mangelinck, N. Necessity of investigating microstructure formation during directional solidification of transparent alloys in 3D. Adv. Sp. Res. 2005, 36, 80–85. [Google Scholar] [CrossRef]
- Fadel, A.A.; Rosa, D.; Murça, L.B.; Fereira, J.L.A.; Araújo, J.A. Effect of high mean tensile stress on the fretting fatigue life of an Ibis steel reinforced aluminium conductor. Int. J. Fatigue 2012, 42, 24–34. [Google Scholar] [CrossRef]
- Cesnik, M. Uninterrupted and accelerated vibrational fatigue testing with simultaneous monitoring of the natural frequency and damping. J. Sound Vib. 2012, 331, 5370–5382. [Google Scholar] [CrossRef]
- Dieter, G.E. Mechanical metallurgy, 1st ed.; Mehl, R.F., Bver, M., Eds.; McGraw—Hill Book Company: New York City, NY, USA, 1961; ISBN 07-016890-3. [Google Scholar]
- Alves, A.R.; Coutinho, A.D.R. The evolution of the niobium production in Brazil. Mater. Res. 2015, 18, 106–112. [Google Scholar] [CrossRef]
- Heisterkamp, F.; Carneiro, T. Niobium: Future Possibilities–Technology and the Market Place. In Proceedings of the International Symposium, Niobium, Orlando, FL, USA, 2–5 December 2001; Science & Tecnology; pp. 1109–1160. [Google Scholar]
- Li, C.Y.; Huang, J.F.; Lu, J.; Cao, L.Y.; Fei, J. Effect of Nb coating on oxidation behavior of C/C composites. Corros. Sci. 2012, 63, 182–186. [Google Scholar] [CrossRef]
- Car, T.; Salamon, K.; Radić, N.; Ivkov, J. Elastic Energy Fraction as the Phenomenological Connection Between Electrical, Mechanical and Thermal Properties of the Al–(Nb, Mo, Ta, W) Amorphous Thin Films. Met. Mater. Int. 2019, 1–8. [Google Scholar] [CrossRef]
- Zhu, Z.; Du, Y.; Zhang, L.; Chen, H.; Xu, H.; Tang, C. Experimental identification of the degenerated equilibrium and thermodynamic modeling in the Al-Nb system. J. Alloy. Compd. 2008, 460, 632–638. [Google Scholar] [CrossRef]
- Pisch, A.; Pasturel, A. On the partial enthalpy of mixing of Nb in liquid Al. Thermochim. Acta 2019, 671, 103–109. [Google Scholar] [CrossRef]
- Hutchinson, C.R.; Fan, X.; Pennycook, S.J.; Shiflet, G.J. On the origin of the high coarsening resistance of Ω plates in Al-Cu-Mg-Ag alloys. Acta Mater. 2001, 49, 2827–2841. [Google Scholar] [CrossRef]
- Wang, C.; Gao, Q.; Yuan, Y.; Zhang, H.; Zhang, J.; Wang, Q.; Qu, F. Microstructure evolutions of Ni-Ti-Nb-Al alloys with different Al addition. J. Alloy. Compd. 2017, 695, 2923–2929. [Google Scholar] [CrossRef]
- Takeyama, M.B.; Noya, A. Application of Al–Nb alloy film to metal capping layer on Cu Application of Al–Nb alloy fi lm to metal capping layer on Cu. Jpn. J. Appl. Phys. 2016, 55, 02BC22. [Google Scholar] [CrossRef]
- Munitz, A.; Gokhale, A.B.; Abbaschian, R. Effect of supercooling on the microstructure of Al-Nb alloys. J. Mater. Sci. 2000, 35, 2263–2271. [Google Scholar] [CrossRef]
- Koutná, N.; Erdely, P.; Zöhrer, S.; Franz, R.; Du, Y.; Liu, S.; Mayrhofer, P.H.; Holec, D. Experimental Chemistry and Structural Stability of AlNb 3 Enabled by Antisite Defects Formation. Materials 2019, 12, 1104. [Google Scholar] [CrossRef] [PubMed]
- Jorda, J.L.; Flükiger, R.; Muller, J. A new metallurgical aluminium system investigation of the niobium. J. Less Common Met. 1980, 75, 227–239. [Google Scholar] [CrossRef]
- He, C.; Stein, F.; Palm, M. Thermodynamic description of the systems Co-Nb, Al-Nb and Co-Al-Nb. J. Alloy. Compd. 2015, 637, 361–375. [Google Scholar] [CrossRef]
- Wang, X.L.; Zhang, K.F. Mechanical alloying, microstructure and properties of Nb-16Si alloy. J. Alloy. Compd. 2010, 490, 677–683. [Google Scholar] [CrossRef]
- Stefan-Kharicha, M.; Kharicha, A.; Wu, M.; Ludwig, A. Observation of flow regimes and transitions during a columnar solidification experiment. Fluid Dyn. Res. 2014, 46, 4. [Google Scholar] [CrossRef]
- Rosa, D.M.; Spinelli, J.E.; Ferreira, I.L.; Garcia, A. Cellular/dendritic transition and microstructure evolution during transient directional solidification of Pb-Sb alloys. Metall. Mater. Trans. A 2008, 39, 2161–2174. [Google Scholar] [CrossRef]
- Yu, L.; Ding, G.L.; Reye, J.; Tewari, S.N.; Ojha, S.N. Cellular/dendritic array tip morphology during directional solidification of Pb-5.8 Wt Pct Sb alloy. Metall. Mater. Trans. A 1999, 30, 2463–2472. [Google Scholar] [CrossRef]
- Ding, G.L.; Huang, W.D.; Huang, X.; Lin, X.; Zhou, Y.H. On primary dendritic spacing during unidirectional solidification. Acta Mater. 1996, 44, 3705–3709. [Google Scholar] [CrossRef]
- Spinelli, J.E.; Rosa, D.M.; Ferreira, I.L.; Garcia, A. Influence of melt convection on dendritic spacings of downward unsteady-state directionally solidified Al-Cu alloys. Mater. Sci. Eng. A 2004, 383, 271–282. [Google Scholar] [CrossRef]
- Miller, J.D.; Pollock, T.M. Stability of dendrite growth during directional solidification in the presence of a non-axial thermal field. Acta Mater. 2014, 78, 23–36. [Google Scholar] [CrossRef]
- Bertelli, F.; Freitas, E.S.; Cheung, N.; Arenas, M.A.; Conde, A.; de Damborenea, J.; Garcia, A. Microstructure, tensile properties and wear resistance correlations on directionally solidified Al-Sn-(Cu; Si) alloys. J. Alloy. Compd. 2016, 695, 3621–3631. [Google Scholar] [CrossRef]
- Vida, T.A.; Conde, A.; Freitas, E.S.; Arenas, M.A.; Cheung, N.; Brito, C.; de Damborenea, J.; Garcia, A. Directionally solidified dilute Zn-Mg alloys: Correlation between microstructure and corrosion properties. J. Alloy. Compd. 2017, 723, 536–547. [Google Scholar] [CrossRef]
- Reis, B.P.; França, R.P.; Spim, J.A.; Garcia, A.; Costa, E.M.; Santos, C.A. The effects of dendritic arm spacing (as-cast) and aging time (solution heat-treated) of Al–Cu alloy on hardness. J. Alloy. Compd. 2013, 549, 324–335. [Google Scholar] [CrossRef]
- Reis, B.P.; Lopes, M.M.; Garcia, A.; Santos, C.A. The correlation of microstructure features, dry sliding wear behavior, hardness and tensile properties of Al-2wt%Mg-Zn alloys. J. Alloy. Compd. 2018, 764, 267–278. [Google Scholar] [CrossRef]
- Kakitani, R.; Reyes, R.V.; Garcia, A.; Spinelli, J.E.; Cheung, N. Relationship between spacing of eutectic colonies and tensile properties of transient directionally solidified Al-Ni eutectic alloy. J. Alloy. Compd. 2018, 733, 59–68. [Google Scholar] [CrossRef]
- Xavier, M.G.; Cruz, C.B.; Kakitani, R.; Silva, B.L.; Garcia, A.; Cheung, N.; Spinelli, J.E. Directional solidification of a Sn-0.2Ni solder alloy in water-cooled copper and steel molds: Related effects on the matrix micromorphology, nature of intermetallics and tensile properties. J. Alloy. Compd. 2017, 723, 1039–1052. [Google Scholar] [CrossRef]
- Garcia, A. The effects of Zn segregation and microstructure length scale on the corrosion behavior of a directionally solidi fi ed Mg-25 wt.% Zn alloy. J. Alloy. Compd A 2017, 723, 649–660. [Google Scholar] [CrossRef]
- Quaresma, M.V. The effect of solidification thermal variables on surface quality of Al–Cu ingots. J. Alloy. Compd. 2007, 428, 130–138. [Google Scholar] [CrossRef]
- Silva, B.L.; Garcia, A.; Spinelli, J.E. Complex eutectic growth and Bi precipitation in ternary Sn-Bi-Cu and Sn-Bi-Ag alloys. J. Alloy. Compd. 2016, 691, 600–605. [Google Scholar] [CrossRef]
- Santos, C.A.; Garcia, A. Determination of transient interfacial heat transfer coefficients in chill mold. Castings 2001, 319, 174–186. [Google Scholar] [CrossRef]
- ASTM E3-11. Standard Guide for Preparation of Metallographic Specimens 1; ASTM International: West Conshohocken, PA, USA, 2011; pp. 1–12. [Google Scholar] [CrossRef]
- Gündüz, M.; Çadirli, E. Directional solidification of aluminium-copper alloys. Mater. Sci. Eng. A 2002, 327, 167–185. [Google Scholar] [CrossRef]
- ASTM E1876-15. Standard Test Method for Dynamic Young’s Modulus, Shear Modulus, and Poisson’s Ratio by Impulse Excitation of Vibration; ASTM International: West Conshohocken, PA, USA, 2015. [Google Scholar] [CrossRef]
- ASTM International. ASTM E384: Standard Test Method for Knoop and Vickers Hardness of Materials; ASTM International: West Conshohocken, PA, USA, 2012; pp. 1–43. [Google Scholar] [CrossRef]
- Okamoto, T.; Kishitake, K. Dendritic structure in unidirectionally solidified aluminum, tin, and zinc base binary alloys. J. Cryst. Growth 1975, 29, 137–146. [Google Scholar] [CrossRef]
- Wang, F.; Ma, D.X.; Zhang, J.; Bogner, S.; Bührig-Polaczek, A. A high thermal gradient directional solidification method for growing superalloy single crystals. J. Mater. Process. Technol. 2014, 214, 3112–3121. [Google Scholar] [CrossRef]
- Brundidge, C.L.; Miller, J.D.; Pollock, T.M. Development of dendritic structure in the liquid-metal-cooled, directional-solidification process. Metall. Mater. Trans. A 2011, 42, 2723–2732. [Google Scholar] [CrossRef]
- Cai, W.; Lu, X.L.; Zhao, L.C. Damping behavior of TiNi-based shape memory alloys. Mater. Sci. Eng. A 2005, 394, 78–82. [Google Scholar] [CrossRef]
- Chen, Y.; Jiang, H.C.; Liu, S.W.; Rong, L.J.; Zhao, X.Q. Damping capacity of TiNi-based shape memory alloys. J. Alloy. Compd. 2009, 482, 151–154. [Google Scholar] [CrossRef]
- Van Humbeeck, J. Damping capacity of thermoelastic martensite in shape memory alloys. J. Alloy. Compd. 2003, 355, 58–64. [Google Scholar] [CrossRef]
- Elliott, R.; Shunk, F. The Al-Nb system (Aluminium-Niobium). Bull. Alloy Ph. Diagr. 1981, 2, 75–81. [Google Scholar] [CrossRef]



| Metals | Al | Nb | Fe | Si | S | Ca | Ho | Ni | Others |
|---|---|---|---|---|---|---|---|---|---|
| Aluminium | balance | - | 0.23 | 0.3 | 0.03 | - | - | - | 0.04 |
| Niobium | - | balance | - | - | - | 0.31 | 0.17 | 0.06 | - |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moreira Coutinho, M.; Saraiva Silva, J.I.; Primo Sousa, T.; Monteiro Rosa, D. Upward Unsteady-State Solidification of Dilute Al–Nb Alloys: Microstructure Characterization, Microhardness, Dynamic Modulus of Elasticity, Damping, and XRD Analyses. Metals 2019, 9, 713. https://doi.org/10.3390/met9060713
Moreira Coutinho M, Saraiva Silva JI, Primo Sousa T, Monteiro Rosa D. Upward Unsteady-State Solidification of Dilute Al–Nb Alloys: Microstructure Characterization, Microhardness, Dynamic Modulus of Elasticity, Damping, and XRD Analyses. Metals. 2019; 9(6):713. https://doi.org/10.3390/met9060713
Chicago/Turabian StyleMoreira Coutinho, Maycol, José Ildon Saraiva Silva, Thiago Primo Sousa, and Daniel Monteiro Rosa. 2019. "Upward Unsteady-State Solidification of Dilute Al–Nb Alloys: Microstructure Characterization, Microhardness, Dynamic Modulus of Elasticity, Damping, and XRD Analyses" Metals 9, no. 6: 713. https://doi.org/10.3390/met9060713
APA StyleMoreira Coutinho, M., Saraiva Silva, J. I., Primo Sousa, T., & Monteiro Rosa, D. (2019). Upward Unsteady-State Solidification of Dilute Al–Nb Alloys: Microstructure Characterization, Microhardness, Dynamic Modulus of Elasticity, Damping, and XRD Analyses. Metals, 9(6), 713. https://doi.org/10.3390/met9060713





