A Proposal for the Application of Failure Assessment Diagrams to Subcritical Hydrogen Induced Cracking Propagation Processes
Abstract
:1. Introduction
2. Background
2.1. Behaviour in HIC Conditions
2.2. Crack Propagaion Model in HIC
2.3. FAD Implementation of the Model
3. Materials and Methods
3.1. Materials
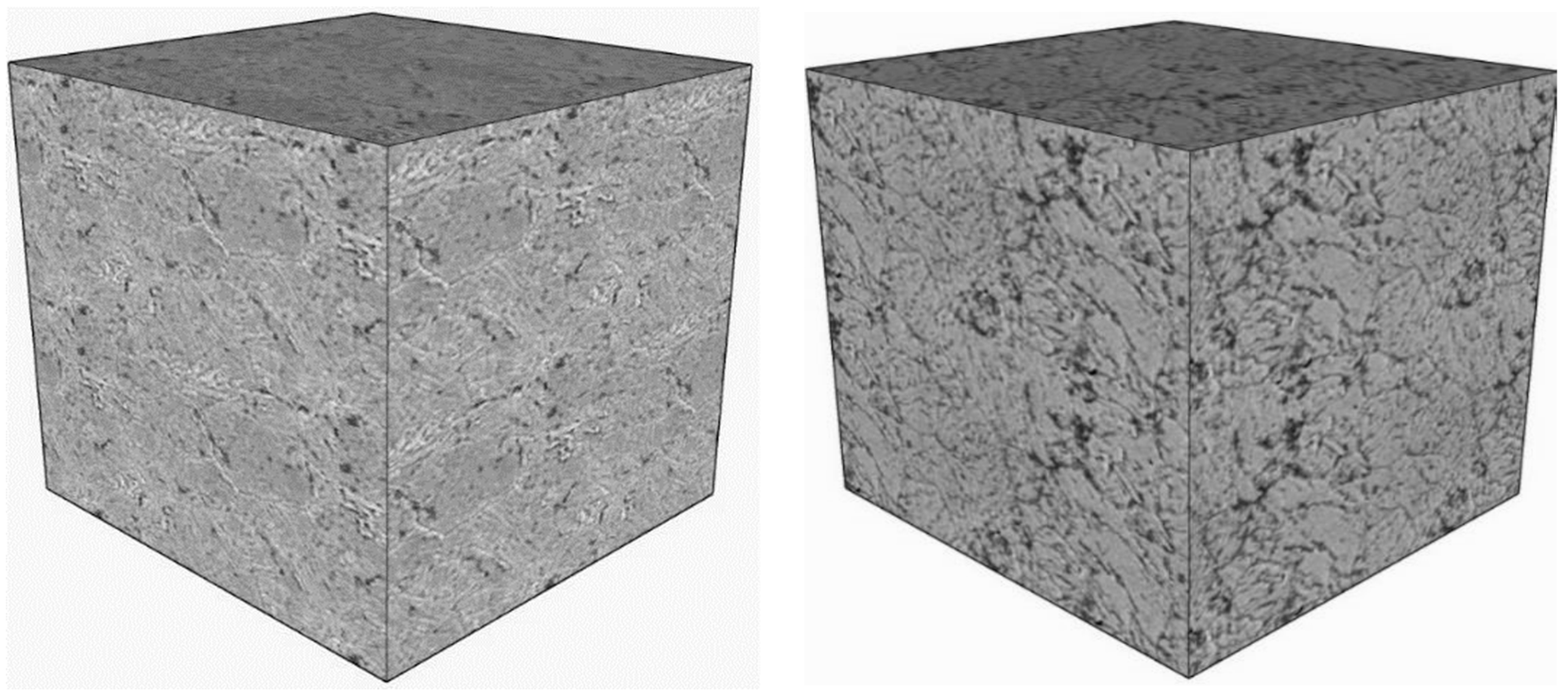
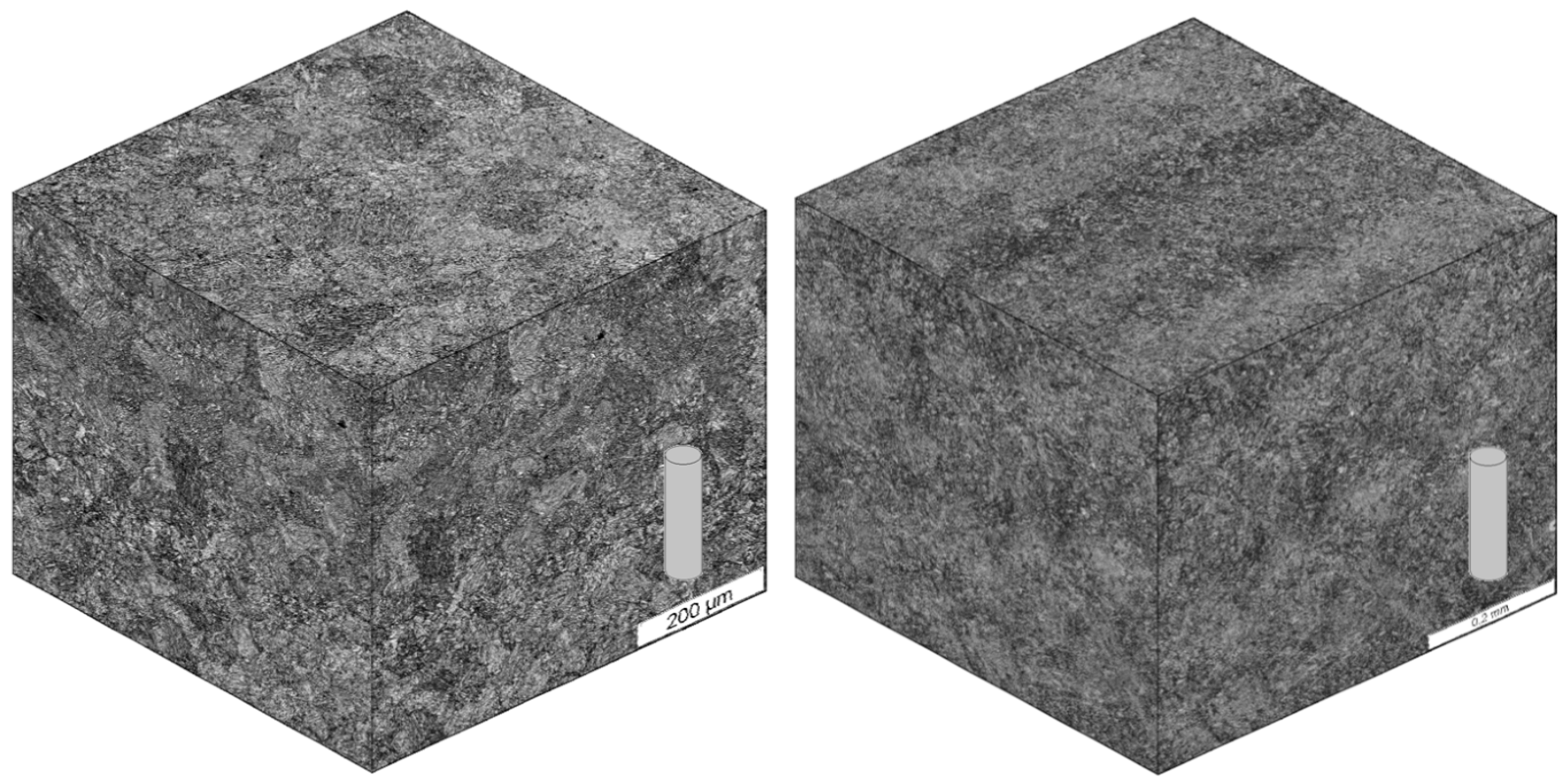
- Mid-strength low alloy 2.25Cr-1Mo and 3Cr-1Mo-0.25V steels: Designed for working in harsh conditions, commonly used in offshore facilities and water treating reactors. In Figure 6, an image of their bainitic microstructure is presented, showing a relatively small grain size homogeneously distributed.
- High-strength 1Cr-1Ni-1.25Mn and 1Cr-1Ni-0.5Mo steels: Grades R5S and R6, respectively according to [23], obtained by quenching and tempering processes and then forged, employed in the manufacturing of offshore links of chains for mooring lines. In Figure 7, an image of its martensitic and bainitic microstructure is presented, also showing a relatively small grain size homogeneously distributed.
3.2. Environments Applied for Hydrogen Embrittlement
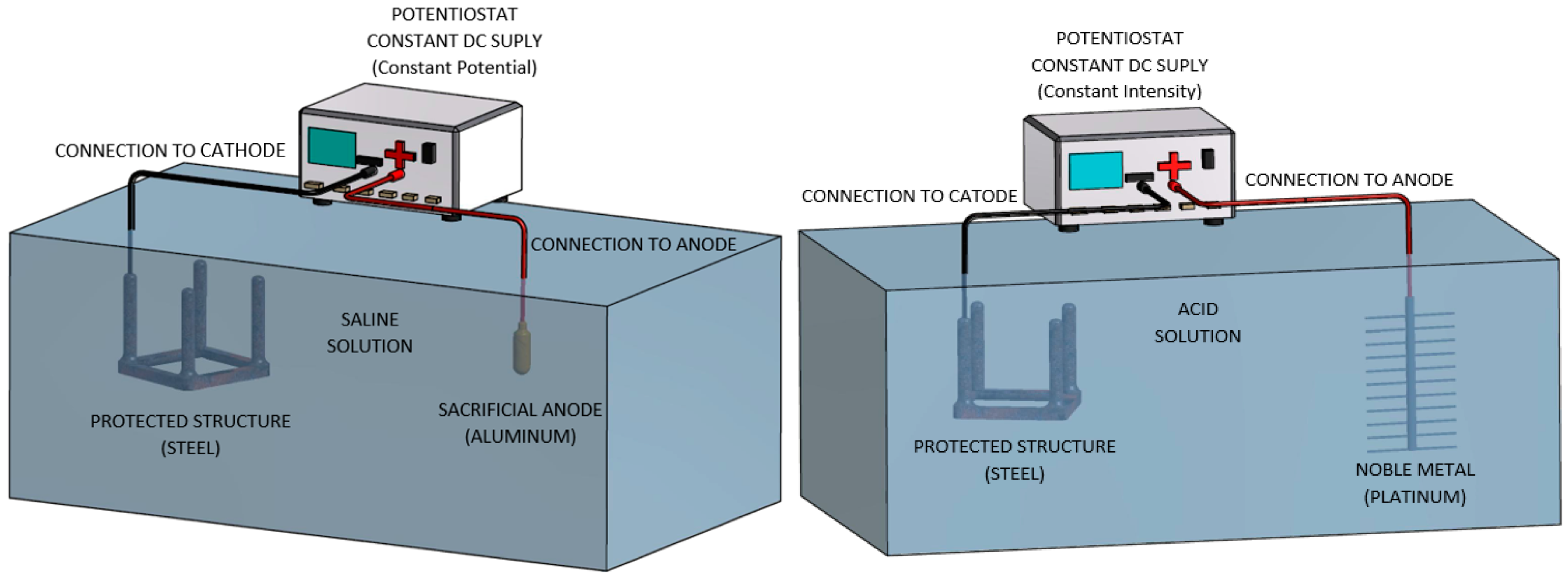
- Cathodic protection (CP) is used to avoid the corrosion phenomena in marine water environments. It involves the use of a sacrificial anode of aluminum (more active than steel), which, in the presence of seawater, is connected to the steel structure. This is the cathode that will be protected from corrosion [24] due to the imposition of a fixed potential between the two, which will maintain the stability of the process.In this study, an aggressive environment of marine water was simulated, consisting of a 3.5% in weight dissolution of NaCl in distilled H2O [11]. An aluminum anode was employed. The pH was controlled at the range 5.5–5.7 [11] during the whole duration of the tests at room temperature 20–25 °C. The level of cathodic protection (aggressiveness) employed was 1050 mV of fixed potential imposed.
- Cathodic charge (CC), or cathodic polarization, is used to reproduce situations where a huge amount of hydrogen is present, such as acid environments or local situations of hydrogen concentration. It consists in the interconnection, via an acid electrolyte, of a noble material (platinum in this case) and the steel, which will passivate and receive protection due to the fixed current interposed between the two [24].In this work, the levels of current interposed were of 5 mA/cm2 (of submerged sample). The aqueous environment employed was an acid electrolyte consisting of a 1N H2SO4 solution in distilled H2O, with 10 mg of an As2O3 solution and 10 drops of CS2 per liter of dissolution. The As2O3 solution was prepared following the Pressouyre′s method [4,10,25]. The pH was measured in the range 0.65–0.80 during the tests and at room temperature (20–25 °C). The resulting protection causes the hydrogen atoms to be absorbed on the host lattice [26,27], producing local or global embrittlement.
3.3. Optimization Proposals for the Model Presented in Epigraph 2
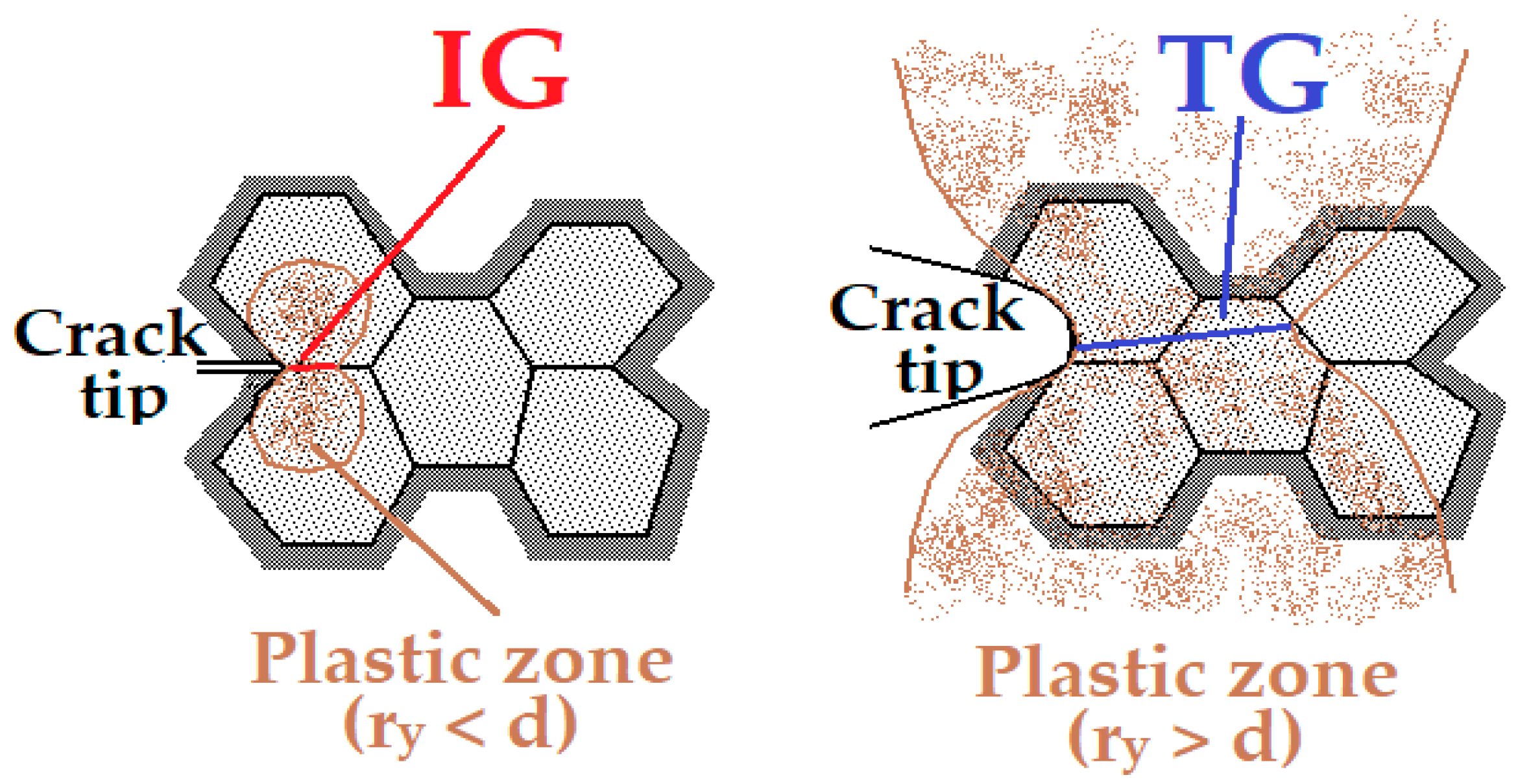
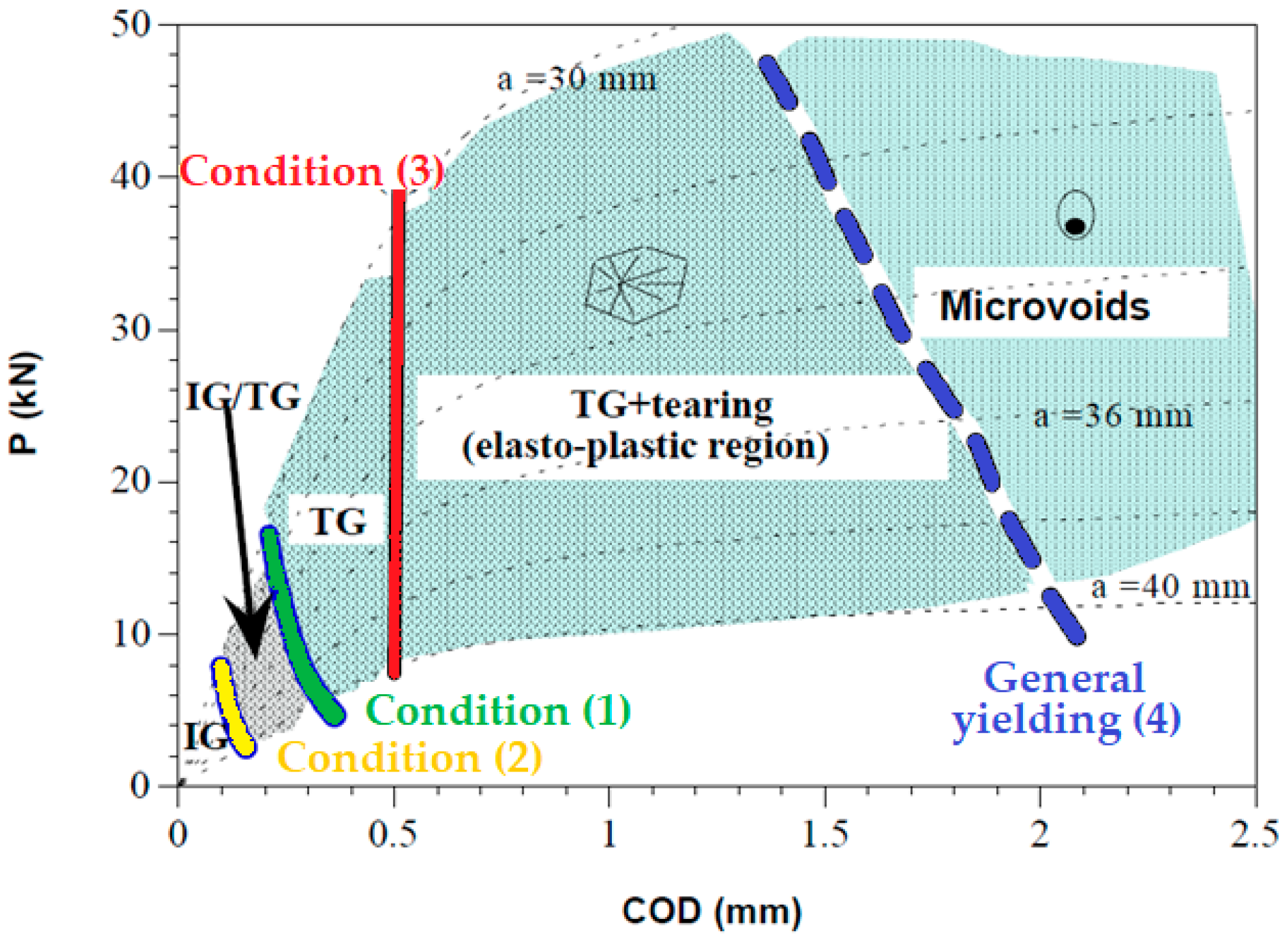
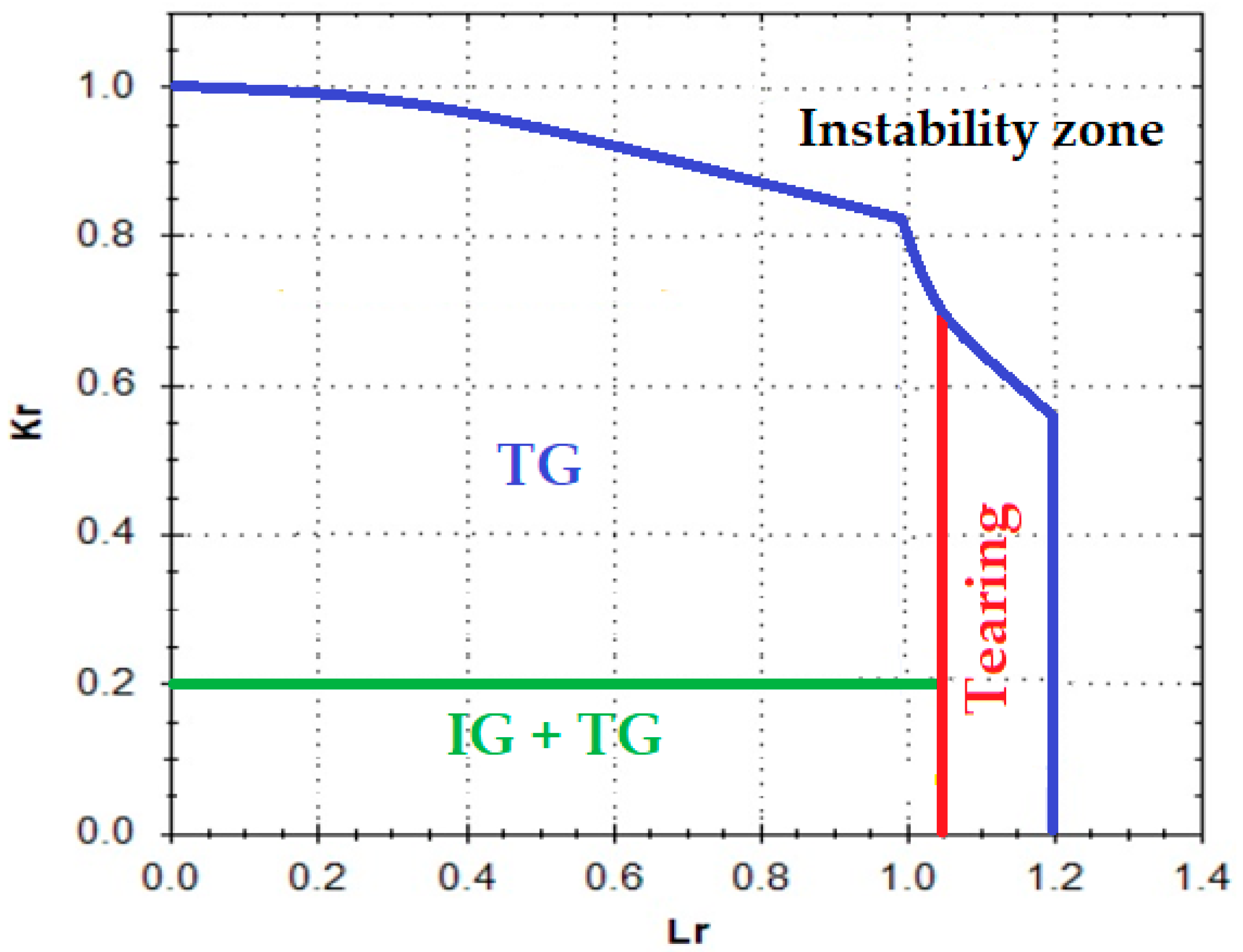
- Slight modification of the zones in which the FAD is divided for HIC conditions. As previously outlined in Section 2 and shown in Figure 5, the FAD diagram is divided into different zones for the different HIC mechanisms taking place (IG, IG/TG, TG, Tearing). On most occasions, depending on the material and the environment, a pure IG zone is very limited; in practice there is a mixed IG/TG mode from the beginning of the propagation. For this reason, in this work, a modification to merge the zones of pure IG and IG + TG into a single one is proposed, modifying the FAD model zones to the one presented in Figure 9 (comparison of Figure 5 and Figure 9).
- Definition of the crack propagation initiation in the elastic-plastic range. For this purpose, the concept of the iso-a slope in its straight initial part is employed. Crack initiation is marked at the point in which the iso-aini curve intersects the P-COD register, the iso-ain being the one that has a slope at the initial straight part equal to 95% of the iso-a0 (iso-a corresponding to the crack length prior to the test, a0). By using this approach, it is possible to define the crack initiation in an elastic-plastic field, rather than in the linear elastic fracture mechanics (LEFM) one that, for example, ASTM standards [9] recommend. In Figure 10, a graphical representation of the new approach, as well as the LEFM one, is given.
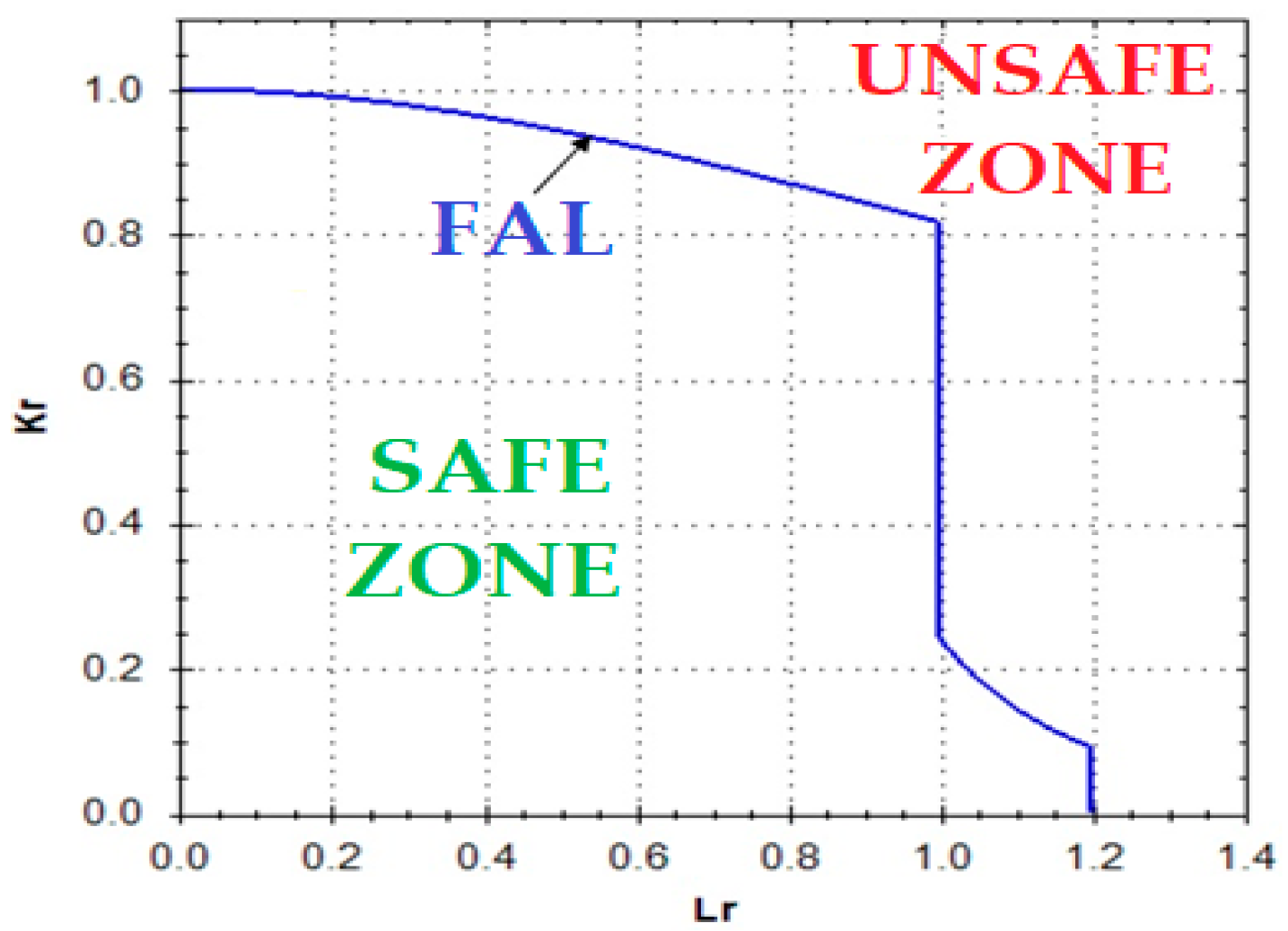
- The last proposed correction is focused on the crack propagation path representation in the FAD. It consists in introducing a modification in the definition of the Kr coordinate (Kr = KI/Kmat) in each of the points that define the crack path propagation in the FAD. It allows the fracture toughness reduction caused by an aggressive environment to be taken into account, without the need to determine KIHIC. The FAD application to environmentally assisted processes leads to predictions far from real behavior, due to the fact that it applies for its calculation a fracture toughness value obtained in non-aggressive scenarios. Applying the aforementioned model, described in Section 2.2, on FAD diagrams and superposing crack path propagations, which is a current trend for predicting propagation and failure in high-plasticity conditions, gave results that did not match with real behavior, as shown in Figure 11. The proposed correction (Figure 11) consists in obtaining an approximate Kmat value in environment, Kmat-env, directly from the load-displacement (P-COD) curve obtained in the test. This is done by applying a proportionality between the values of the test in environment and their equivalent values from a fracture toughness test in air, performed on the same material. This correction can be expressed as follows, Kr then being:
3.4. Test Plan
4. Results
5. Discussion
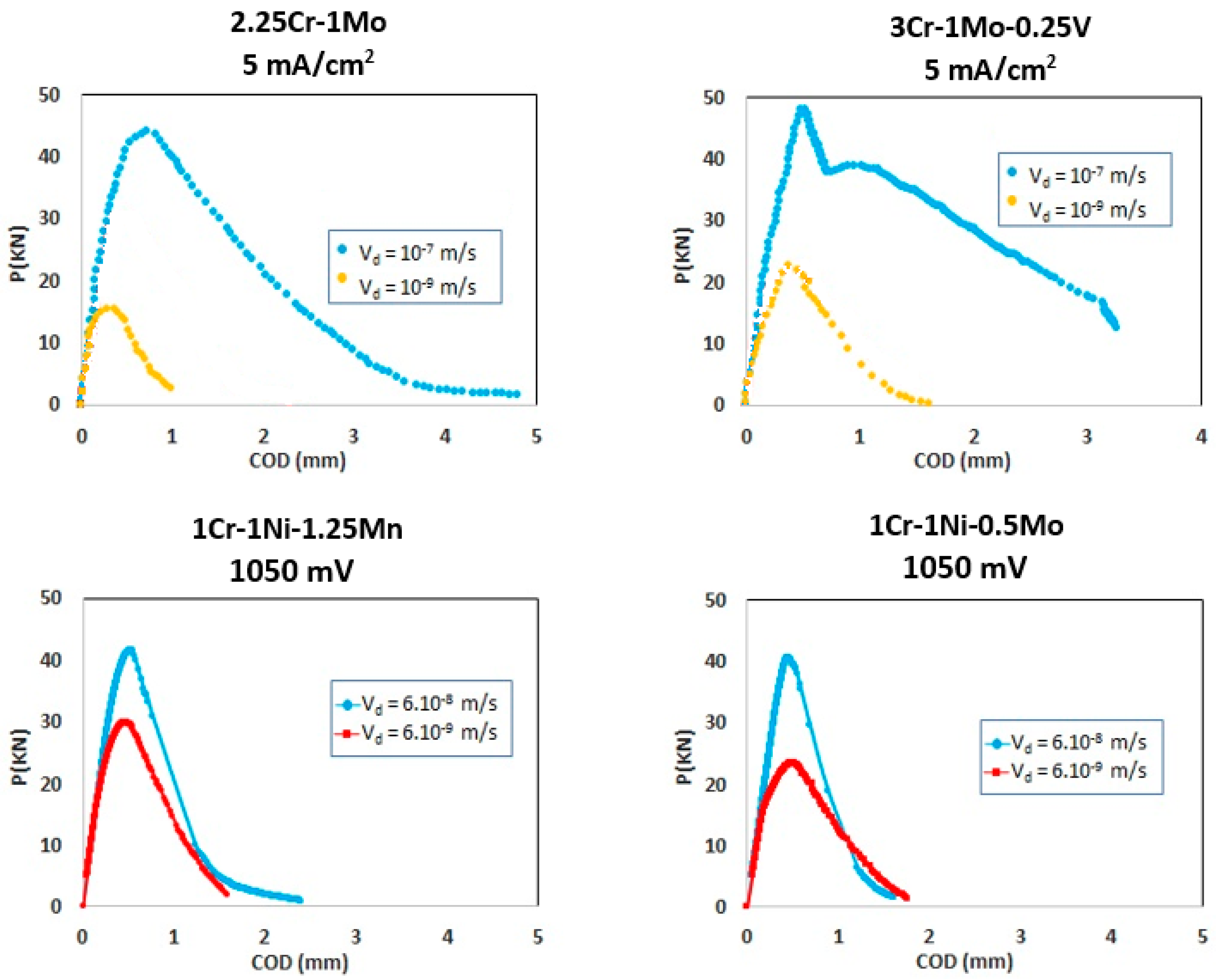
- 2.25Cr-1Mo steel:
- 10−7 m/s: The initial subcritical process started in the cleavage domain (pure TG, but close to the mixed IG + TG), and the subsequent propagation ran under the cleavage domain (TG) and the plastic domain (Tearing) prior to crossing the failure line (FAL) to the instability zone.
- 10−9 m/s: The process started at the mixed cleavages domain (IG + TG, but very close to the pure TG), and propagated across the TG zone until crossing FAL to the instability zone. No tearing was shown.
- 3Cr-1Mo-0.25V steel:
- 10−7 m/s: The process started at the mixed cleavages domain (IG + TG) and propagated across the TG zone until crossing FAL to the instability zone. No tearing was shown.
- 10−9 m/s: The propagation started at the cleavages domain (IG + TG), and followed across the TG zone until crossing the FAL. No tearing was shown.
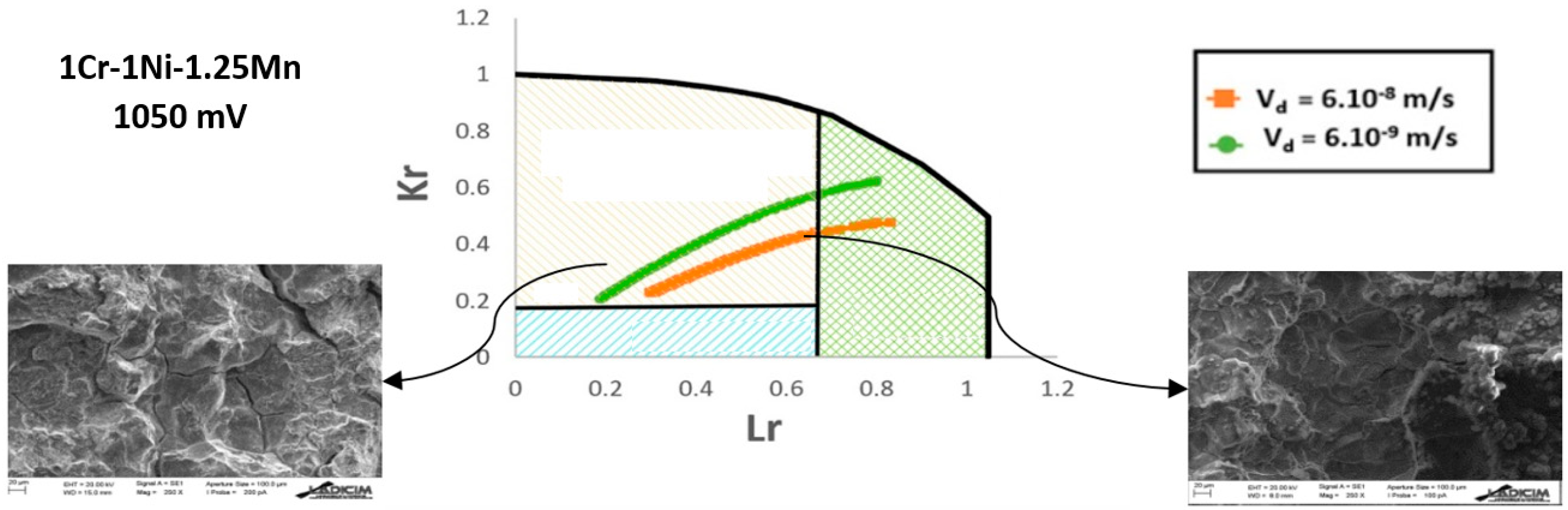
- 1Cr-1Ni-1.25Mn steel:
- 6 × 10−8 m/s: The process started at the TG domain (but close to the mixed IG + TG) and propagated across it up to the plastic domain (Tearing), where the test was stopped (it would have crossed the FAL to the instability zone if continued).
- 6 × 10−9 m/s: The process started at the TG zone (but very close to the mixed IG + TG) and propagated across the TG zone up to the plastic domain (Tearing), where the test was stopped (it would have crossed the FAL to the instability zone if continued).
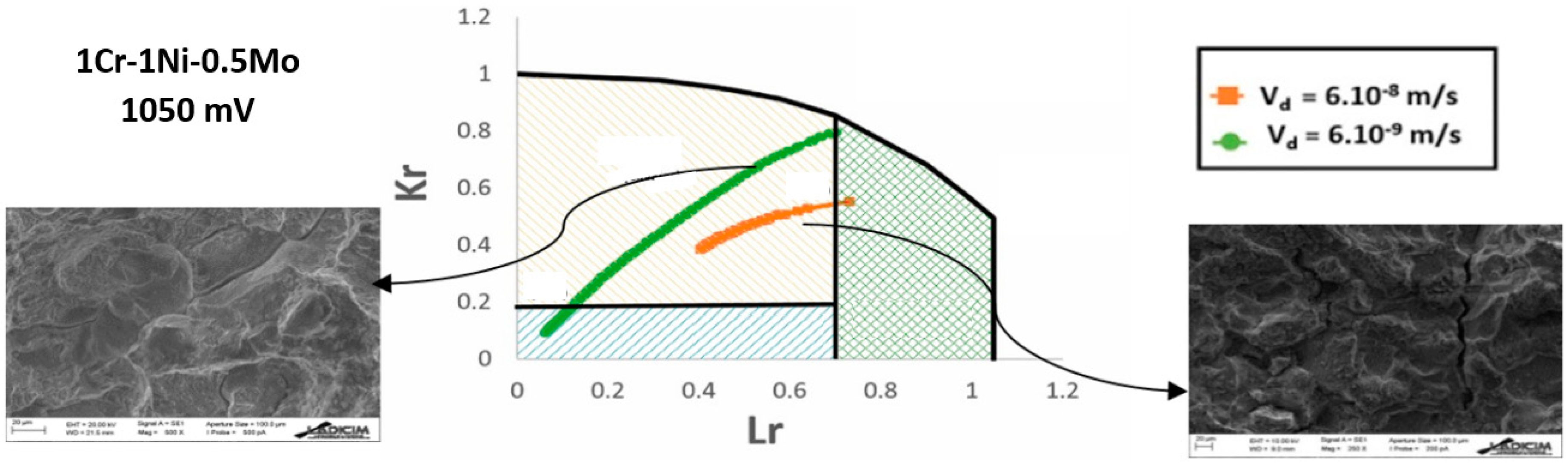
- 1Cr-1Ni-0.5Mo steel:
- 6 × 10−8 m/s: The process started at the TG domain and propagated across it up to the plastic domain (Tearing), where the test was stopped.
- 6 × 10−9 m/s: The process started at the mixed cleavages domain (IG + TG) and propagated across the TG zone up to the plastic domain (Tearing) very close to the triple point of coincidence of TG-Tearing-FAL (see the green curve on Figure 21), where the test was stopped (it would have crossed the FAL to the instability zone if continued).
6. Conclusions and Future Work
Author Contributions
Funding
Conflicts of Interest
References
- Navas, J. Diseño y PWHT de aceros avanzados de alta Resistencia para cadenas de líneas de fondeo. Ph.D. Thesis, University of the Basque Country, Bilbao, Spain, 2015. [Google Scholar]
- Gabetta, G.; Cole, I. A summary of report for an ESIS working party on fracture control guidelines for environmentally assisted cracking of low alloy steels. Fatigue Fract. Eng. Mater. Struct. 1993, 16, 603. [Google Scholar] [CrossRef]
- Kumar, V.; German, M.; Shih, C.F. An Engineering Approach to Elastic-Plastic Solid; Research project EPRI-1931; General Electric Company: Schenectady, NY, USA, 1981. [Google Scholar]
- Álvarez, J.A.; Gutiérrez-Solana, F. An elastic-plastic fracture mechanics based methodology to characterize cracking behaviour and its applications to environmental assisted processes. Nucl. Eng. Des. 1998, 188, 185–202. [Google Scholar] [CrossRef]
- Álvarez, J.A.; Gutiérrez-Solana, F. The application of FAD in the assessment of environmental assisted cracking and fracture conditions. In Proceedings of the 13th European Conference on Fractue (ECF 13), San Sebastian, Spain, 8 September 2000. [Google Scholar]
- Zhuang, C.; Feng, Y.; Huo, C. Study of the mismatched girth welds of X80 line pipes. In Proceedings of the 2006 International Pipeline Conference, Calgary, AB, Canada, 25–29 September 2006; pp. 187–192. [Google Scholar]
- Hasan, S.M.; Khan, F.; Kenny, S. Probabilistic transgranular stress corrosion cracking analysis for oil and gas pipelines. J. Press. Vessel. Technol. Trans. 2012, 134, 51701. [Google Scholar] [CrossRef]
- BS 7910:2013+A1:2015. Guide to Methods for Assessing the Acceptability of Flaw in Metallic Structures; BSI: London, UK, 2013. [Google Scholar]
- ASTM Standard E-1820-18ae1. Standard Test Method for Measurement of Fracture Toughness; ASTM International: West Conshohocken, PA, USA, 2018. [Google Scholar]
- Arroyo, B.; Álvarez, J.A.; Lacalle, R.; Uribe, C.; García, T.E.; Rodríguez, C. Analysis of key factors of hydrogen environmental assisted cracking evaluation by small punch test on medium and high strength steels. Mater. Sci. Eng. 2017, 691, 180–194. [Google Scholar] [CrossRef]
- Arroyo, B.; Álvarez, J.A.; Lacalle, R. Study of the Energy for Embrittlement Damage Initiation by SPT Means. Estimation of KIEAC in Aggressive Environments and Rate Considerations. Theor. Appl. Fract. Mech. 2016, 86, 61–68. [Google Scholar] [CrossRef]
- ISO 7539. Corrosion of Metals and Alloys (Parts 1 to 9); ISO: Geneva, Switzerland, different dates for each part.
- ASTM E-1681. Standard Test Method for Determining Threshold Stress Intensity Factor for Environment-Assisted Cracking of Metallic Materials; ASM International: West Conshohocken, PA, USA, 2013. [Google Scholar]
- Sedriks, A.J. Stress Corrosion Cracking Test Methods. Natl. Asoc. Corros. Eng. 1974, 543. [Google Scholar]
- Arroyo, B. Caracteización mecáncia de aceros de alta y media resistencia en condiciones de fragilización por hidrógeno mediante ensayos Small Punch. Ph.D. Thesis, University of Cantabria, Santander, Spain, 2017. [Google Scholar]
- González, P.; Cicero, S.; Arroyo, B.; Álvarez, J.A. A Theory of Critical Distances based methodology for the analysis of environmentally assisted cracking in steels. Eng. Fract. Mech. 2019, 214, 134–148. [Google Scholar] [CrossRef]
- Thompsonk, A.J.; Bemstein, I.M. Advances in Corrosion Science and Technology; Fontana, M.G., Staettle, R.W., Eds.; Springer US: Plenum, NY, USA, 1980; Volume 7. [Google Scholar]
- Gutiérrez-Solana, F.; Valiente, A.; González, J.; Varona, J.M. A strain-based fracture model for stress corrosion cracking of low-alloy-steels. Metall. Mater. Trans. 1996, 27A, 291–304. [Google Scholar] [CrossRef]
- Bernstein, I.M.; Thompson, A.W. Effect on metallurgical variables on environmental fracture of steels. Int. Met. Rev. 1976, 21, 269–290. [Google Scholar]
- Álvarez, J.A. Fisuración inducida por hidrógeno en aceros soldables microaleados. Caracterización y modelo de comportamiento. Ph.D. Thesis, University of Cantabria, Santander, Spain, 1999. [Google Scholar]
- SINTAP. Structural Integrity Assesment Procedures for European Industry; Final Procedure, British Steel Report: Sheffield, UK, 1999. [Google Scholar]
- “Vindio 1.0”, Software for Structural Integrity Powered by Inesco Ingenieros. Available online: www.inescoingenieros.com (accessed on 6 June 2019).
- Offshore Standard DNV-OS-E302. Offshore Mooring Chain; Det Norske Veritas (DNV): Akershus, Norway, 2008. [Google Scholar]
- Hamilton, J.M. The challenges of Deep-Water Artic Development. Int. J. Offshore Polar Eng. 2011, 21, 241–247. [Google Scholar]
- Bernstein, I.M.; Pressouyre, G.M. Role of Traps in the Microstructural Control of Hydrogen Embrittlement of Steels; Noyes Publ: Park Ridge, NJ, USA, 1988. [Google Scholar]
- Fukai, Y. The Metal-Hydrogen System: Basic Bulk Properties; Springer Science & Business Media: Berlin, Germany, 2006. [Google Scholar]
- Kirchheim, R.; Pundt, A. Hydrogen in Metals. In Physical Metallurgy, 5th ed.; Elsevier: Amsterdam, The Netherlands, 2014; pp. 2597–2705. [Google Scholar] [CrossRef]
- ASTM Standard E-399-17. Standard Test Method for Linear-Elastic Plane-Strain Fracture Toughness KIc of Metallic Materials; ASTM International: West Conshohocken, PA, USA, 2017. [Google Scholar]





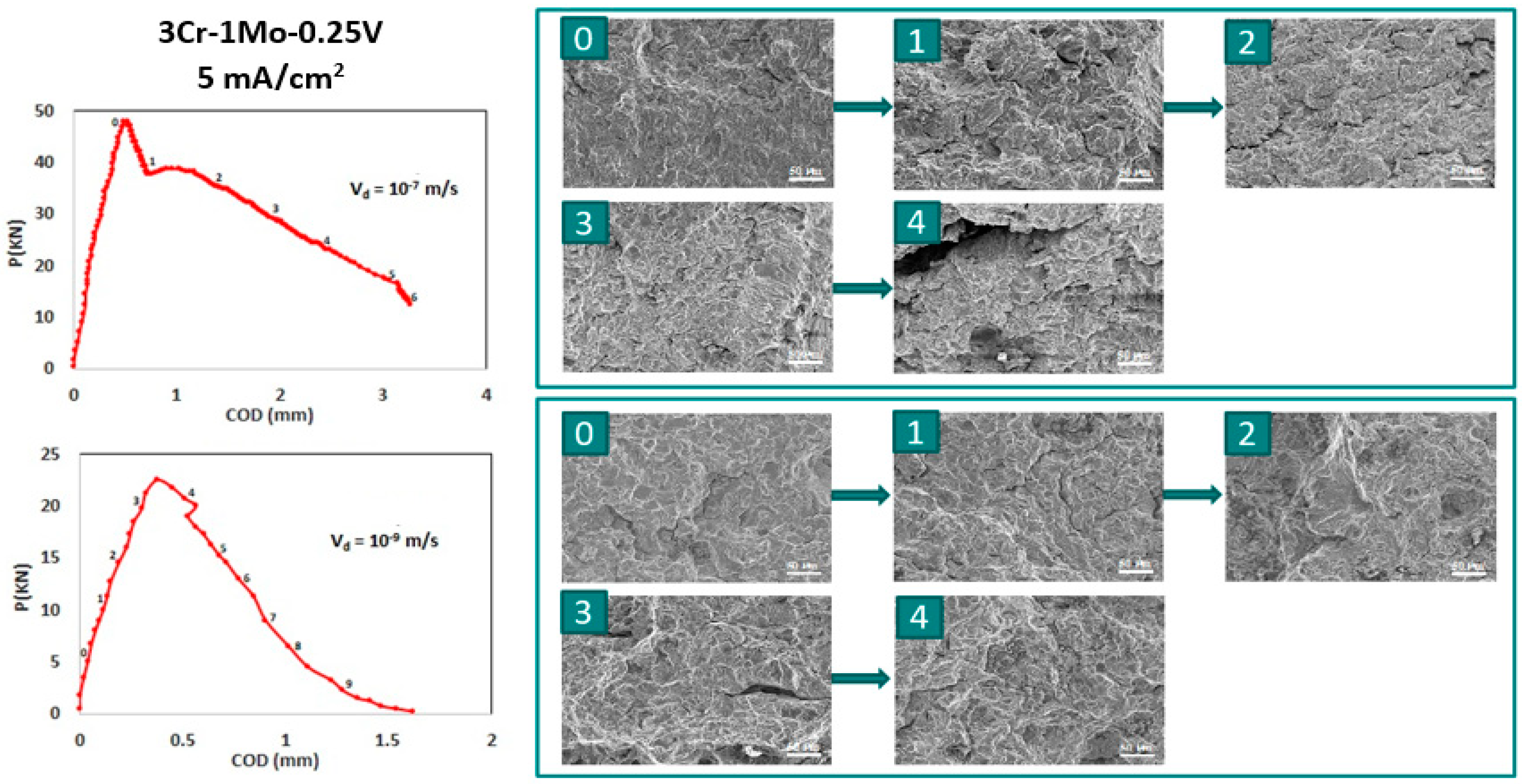

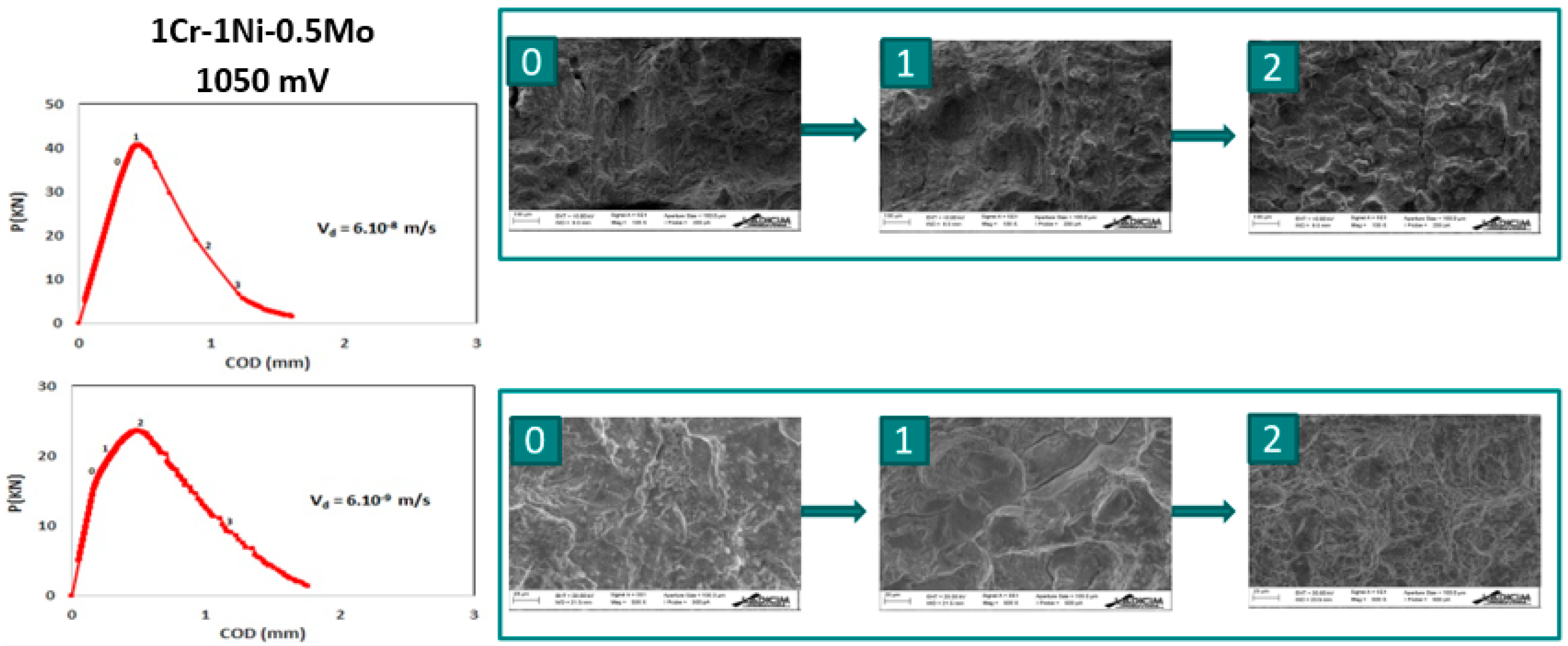
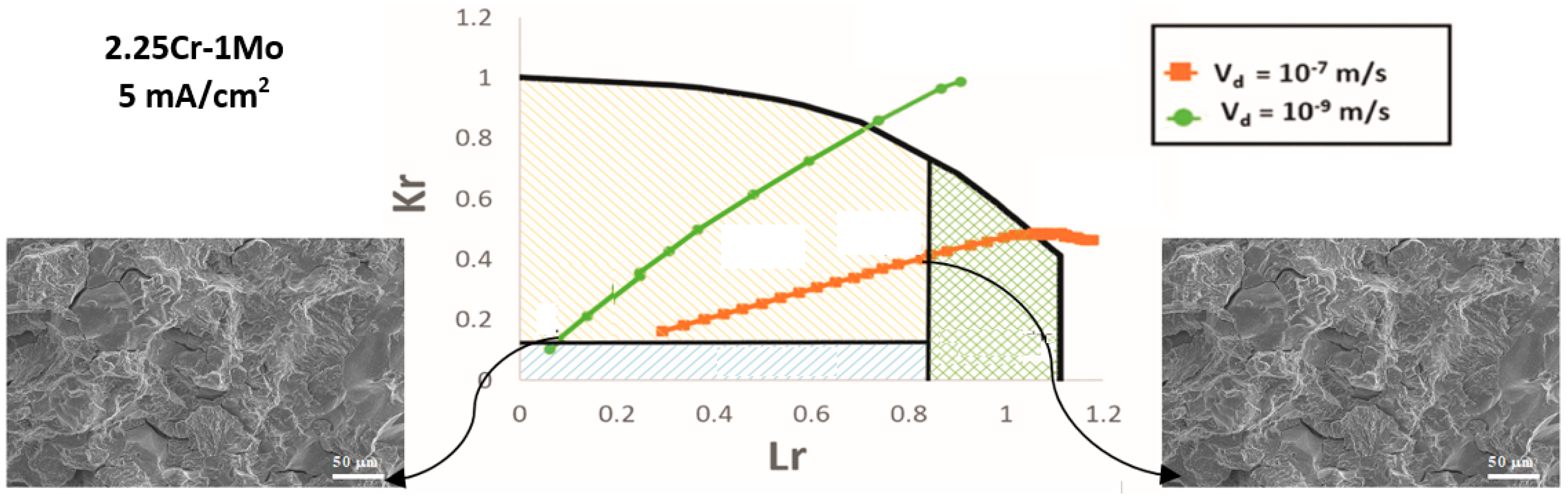
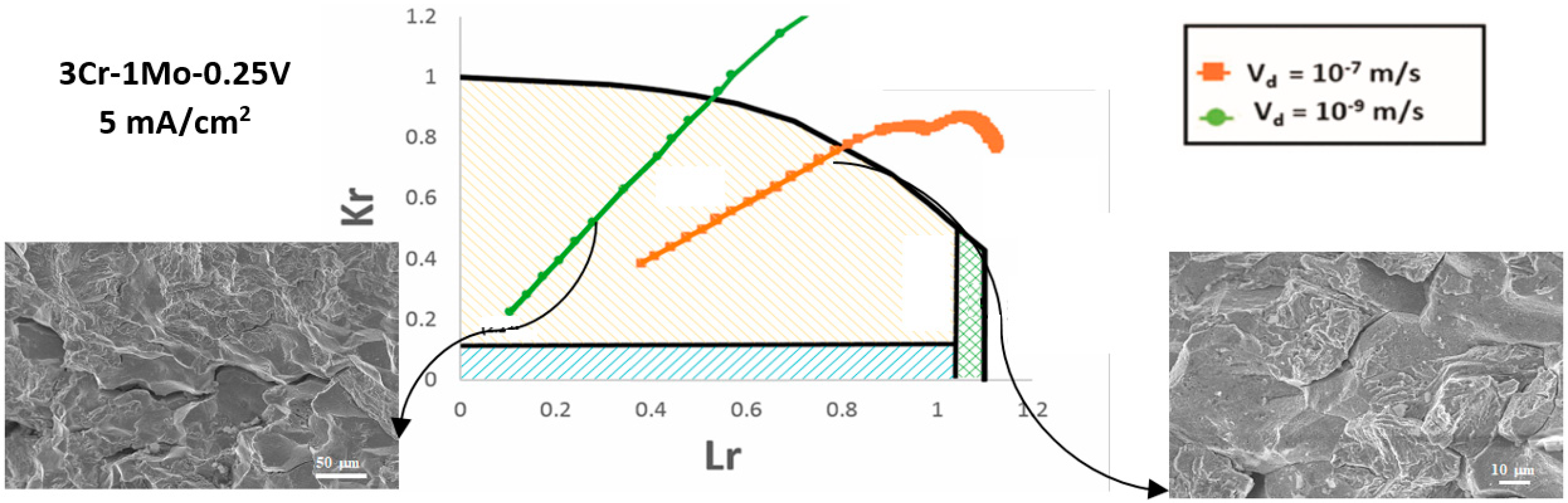

| Parameter | 2.25Cr-1Mo | 3Cr-1Mo-0.25V | 1Cr-1Ni-1.25Mn | 1Cr-1Ni-0.5Mo |
|---|---|---|---|---|
| Sy (MPa) | 584 | 642 | 1024 | 1056 |
| Su (MPa) | 715 | 768 | 1120 | 1159 |
| E (GPa) | 206 | 211 | 219 | 209 |
| εmax (%) | 7.3 | 5.8 | 6.0 | 6.2 |
| Ramberg Osgood α | 0.592 | 0.354 | 0.622 | 0.485 |
| Ramberg Osgood n | 9.4 | 18.5 | 17.0 | 18.0 |
| J0.2 (KJ/m2) | 662 | 241 | 821 | 760 |
| KJ0.2 (MPa·m1/2) | 387 | 236 | 445 | 418 |
| Grain size (µm) | 49 | 17 | 48 | 47 |
| Material | Environment (i,E) | Rate (Vd) | |
|---|---|---|---|
| 2.25Cr-1Mo | Cathodic Charge | 5 mA/cm2 | 10−7 m/s |
| 10−9 m/s | |||
| 3Cr-1Mo-0.25V | Cathodic Charge | 5 mA/cm2 | 10−7 m/s |
| 10−9 m/s | |||
| 1Cr-1Ni-1.25Mn | Cathodic Protection | 1050 mV | 6 × 10−8 m/s |
| 6 × 10−9 m/s | |||
| 1Cr-1Ni-0.5Mo | Cathodic Protection | 1050 mV | 6 × 10−8 m/s |
| 6 × 10−9 m/s | |||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arroyo Martínez, B.; Álvarez Laso, J.A.; Gutiérrez-Solana, F.; Cayón Martínez, A.; Jirón Martínez, Y.J.; Seco Aparicio, A.R. A Proposal for the Application of Failure Assessment Diagrams to Subcritical Hydrogen Induced Cracking Propagation Processes. Metals 2019, 9, 670. https://doi.org/10.3390/met9060670
Arroyo Martínez B, Álvarez Laso JA, Gutiérrez-Solana F, Cayón Martínez A, Jirón Martínez YJ, Seco Aparicio AR. A Proposal for the Application of Failure Assessment Diagrams to Subcritical Hydrogen Induced Cracking Propagation Processes. Metals. 2019; 9(6):670. https://doi.org/10.3390/met9060670
Chicago/Turabian StyleArroyo Martínez, Borja, José Alberto Álvarez Laso, Federico Gutiérrez-Solana, Alberto Cayón Martínez, Yahoska Julieth Jirón Martínez, and Ana Ruht Seco Aparicio. 2019. "A Proposal for the Application of Failure Assessment Diagrams to Subcritical Hydrogen Induced Cracking Propagation Processes" Metals 9, no. 6: 670. https://doi.org/10.3390/met9060670
APA StyleArroyo Martínez, B., Álvarez Laso, J. A., Gutiérrez-Solana, F., Cayón Martínez, A., Jirón Martínez, Y. J., & Seco Aparicio, A. R. (2019). A Proposal for the Application of Failure Assessment Diagrams to Subcritical Hydrogen Induced Cracking Propagation Processes. Metals, 9(6), 670. https://doi.org/10.3390/met9060670






