The Coupled Temperature Field Model of Difficult-to-Deform Mg Alloy Foil High-Efficiency Electro-Rolling and Experimental Study
Abstract
1. Introduction
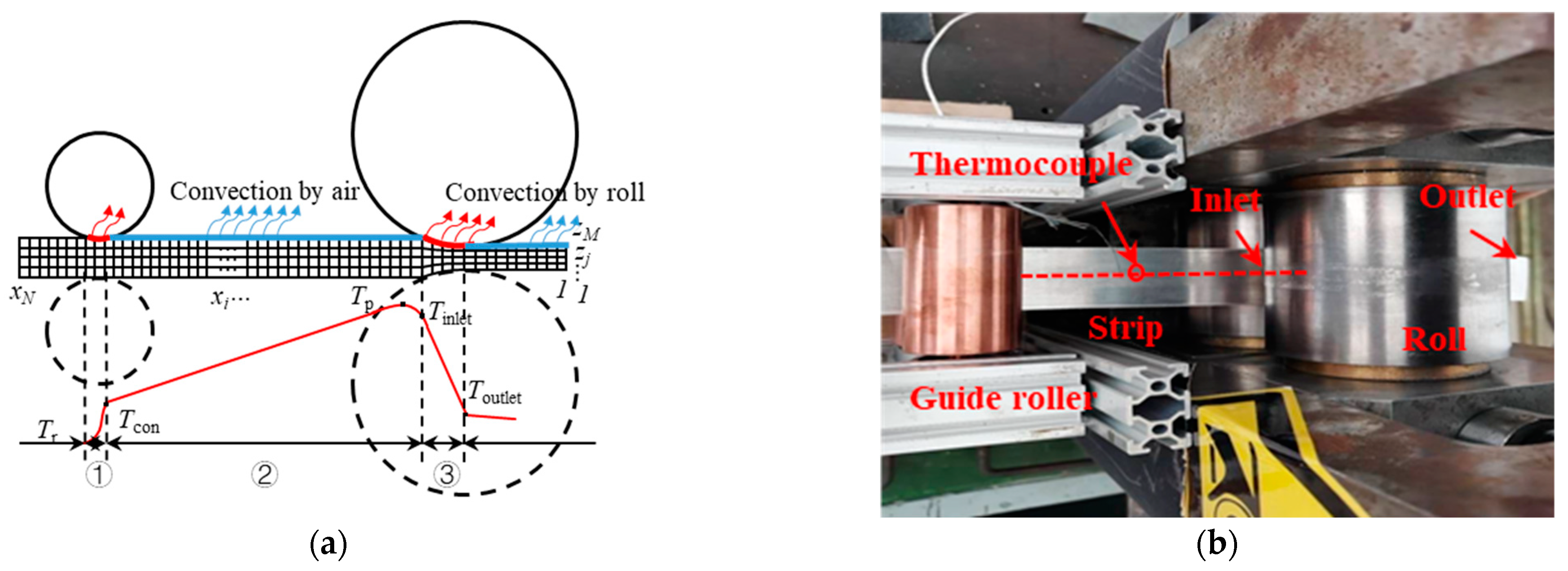
2. The NAER Coupled Temperature Field Model
2.1. Basic Assumptions and Meshing
- (1)
- The material is isotropic and homogeneous, and the material density is constant.
- (2)
- During the rolling process, the contact resistance between the guide roller and the foil is constant.
- (3)
- The current density in the guide roller contact zone and the rolling deformation zone is equal to the current density of the transition zone of Mg foils and remains unchanged along the rolling direction.
- (4)
- The rolling temperature and reduction rate do not affect the contact heat transfer coefficient, and the heat transfer coefficient between the roll and the magnesium foil is constant.
2.2. Temperature Field Model
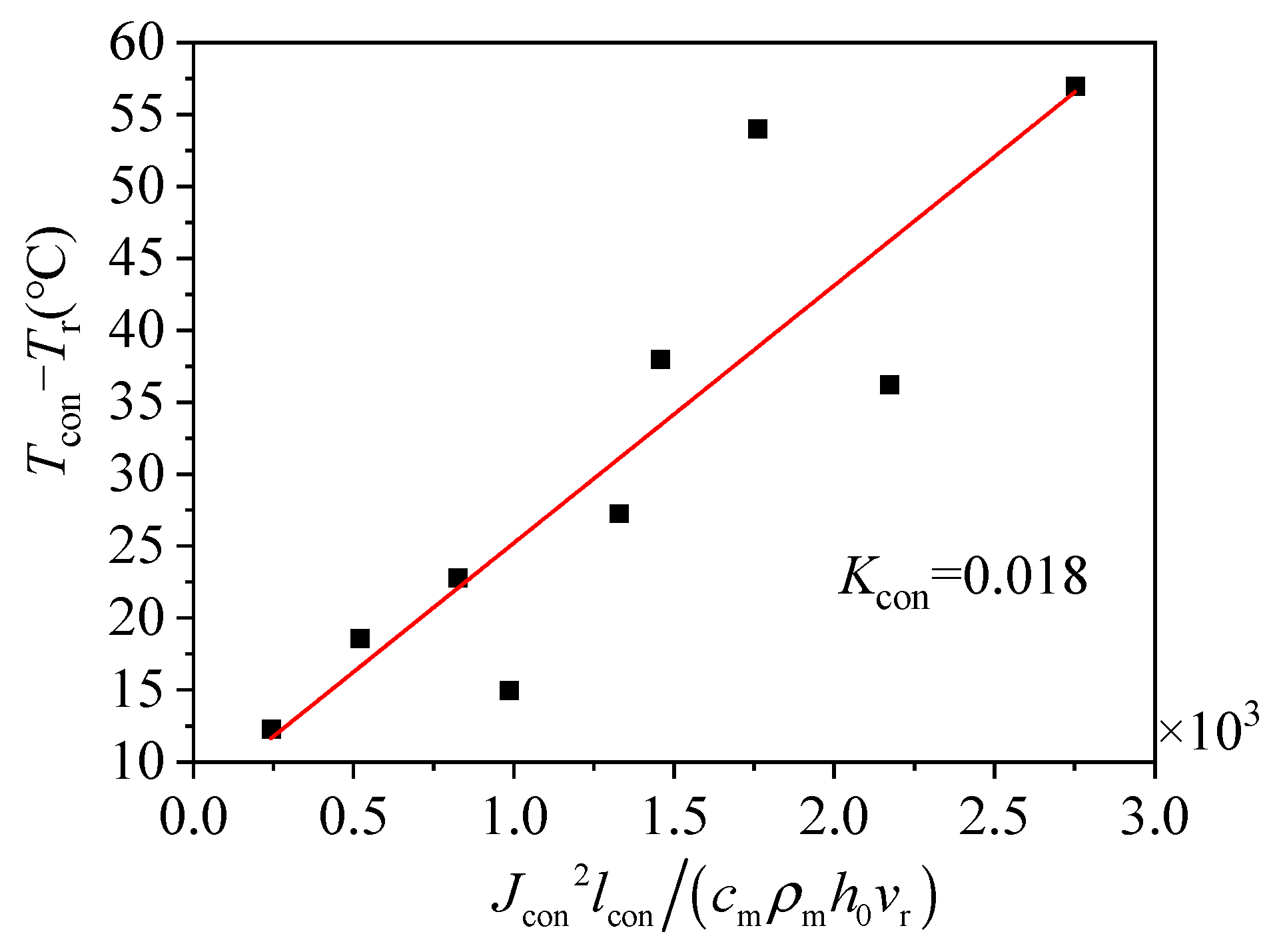
2.3. Boundary Conditions
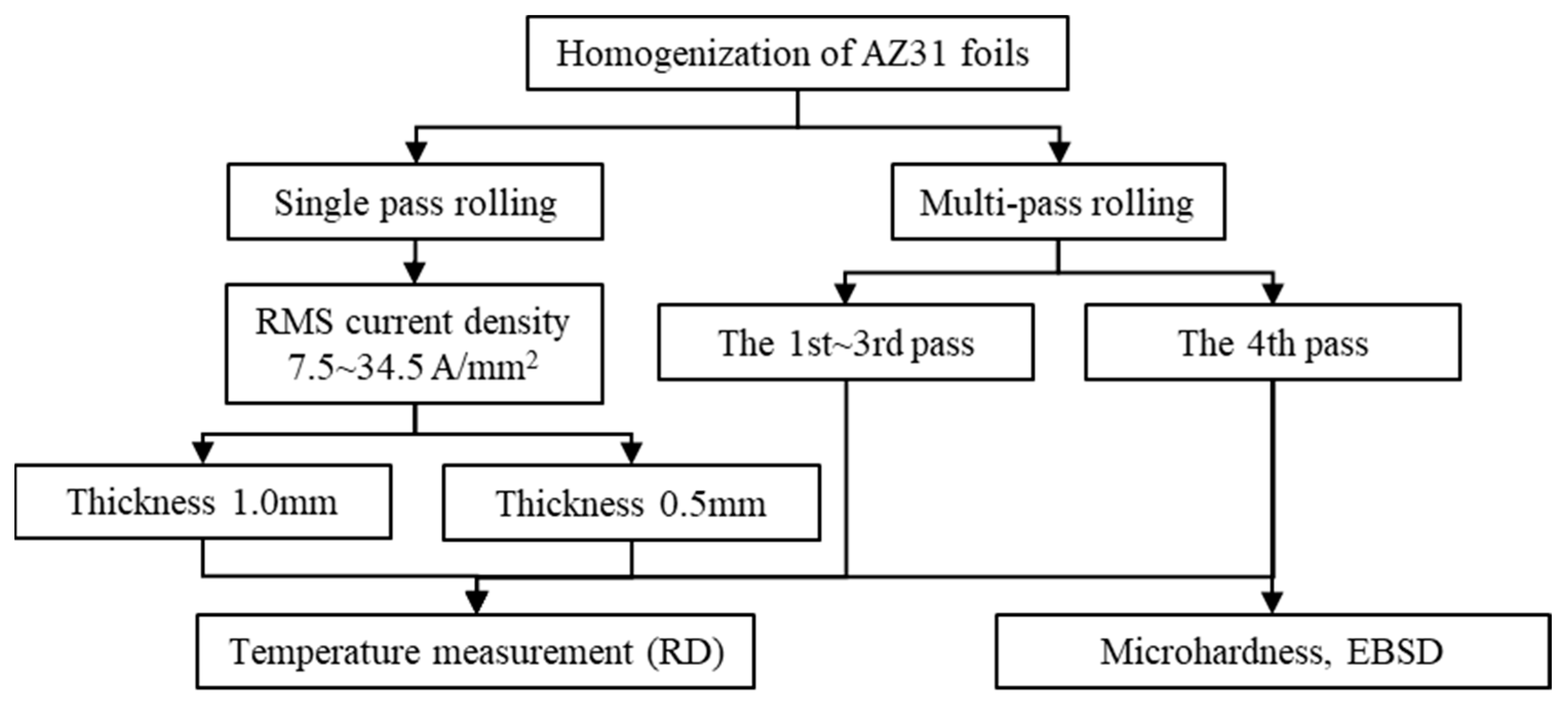
3. Experimental
4. Example Verification
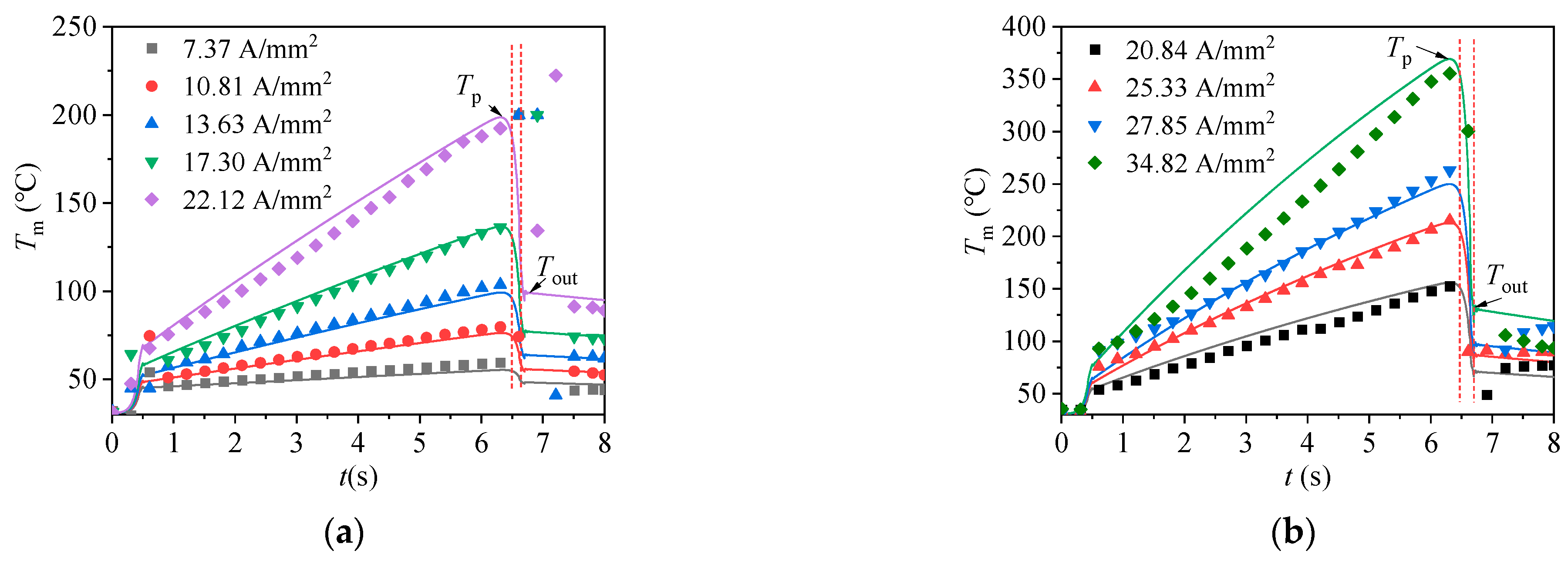
4.1. Temperature Field Simulation of the NAER
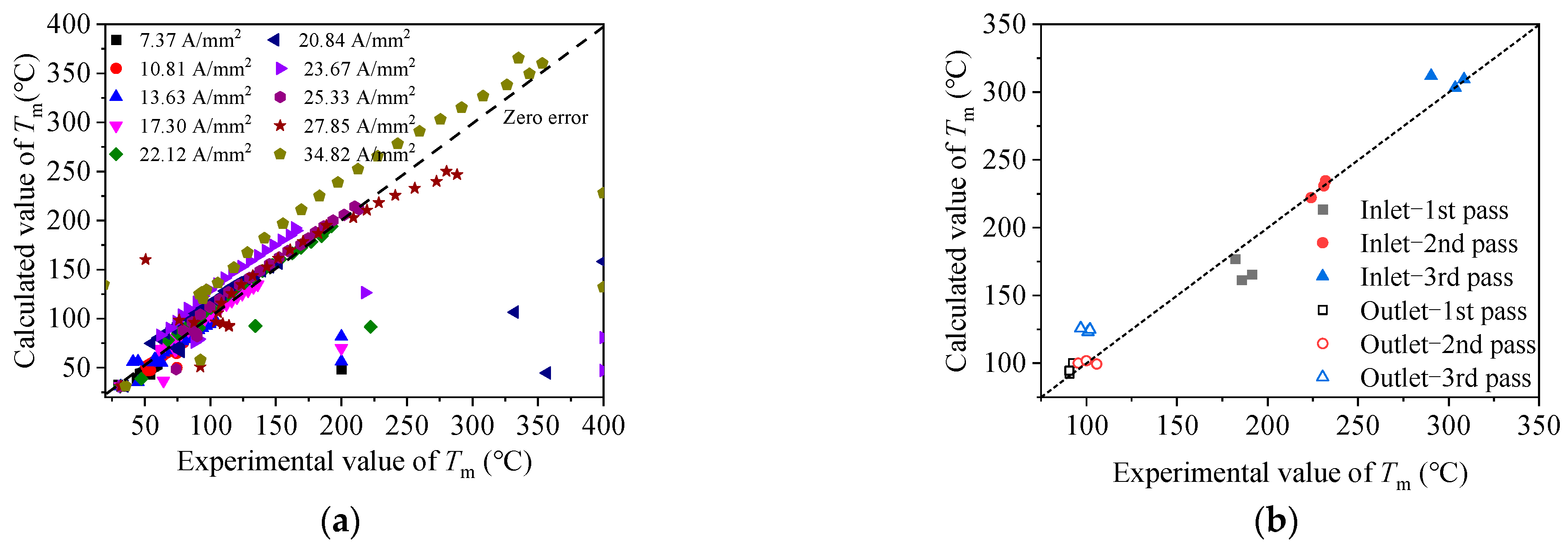
4.2. Accuracy Evaluation of the Temperature Field Model
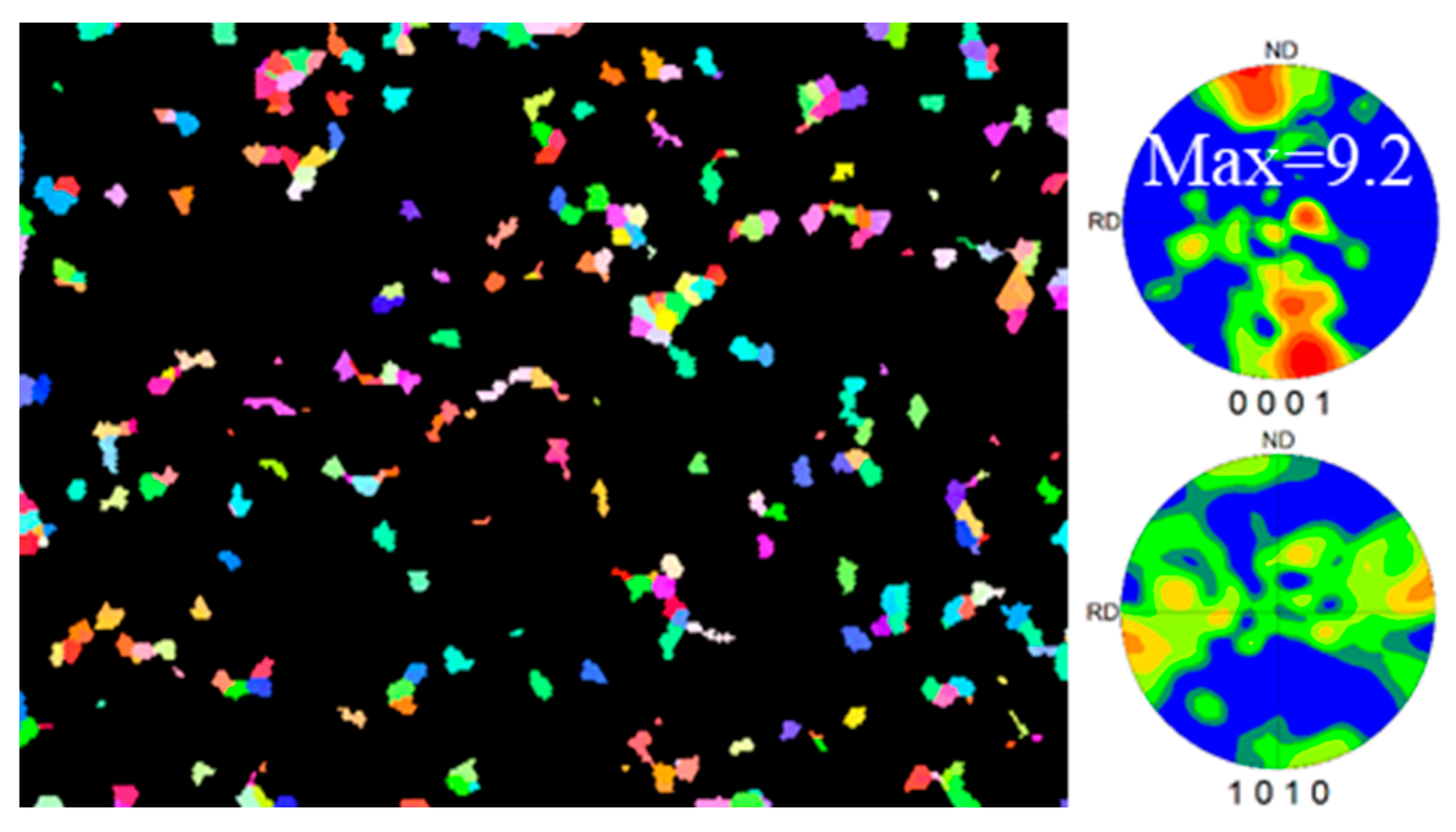
4.3. Microstructure
5. Conclusions
- (1)
- A coupled temperature field model of the NAER was established by considering the mill device and electrification process, and the Joule heat, deformation heat, and friction heat in the NAER process were accurately considered. The NAER temperature field model could accurately predict the peak temperature at the inlet and the outlet temperature of the multi-pass NAER of different thicknesses and different current densities. The average relative error of the prediction was about 7.1%, and the maximum relative error was about 23.0%.
- (2)
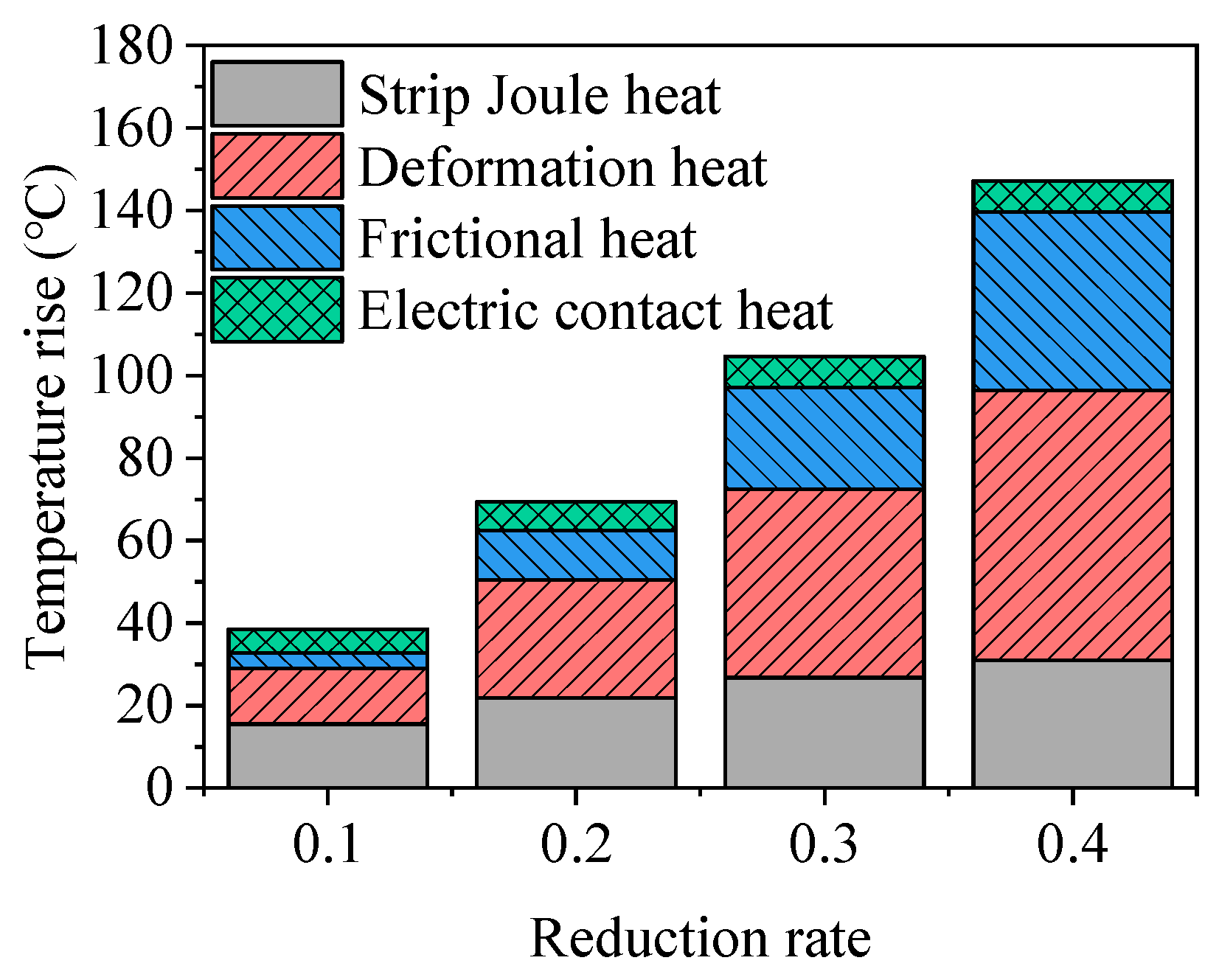
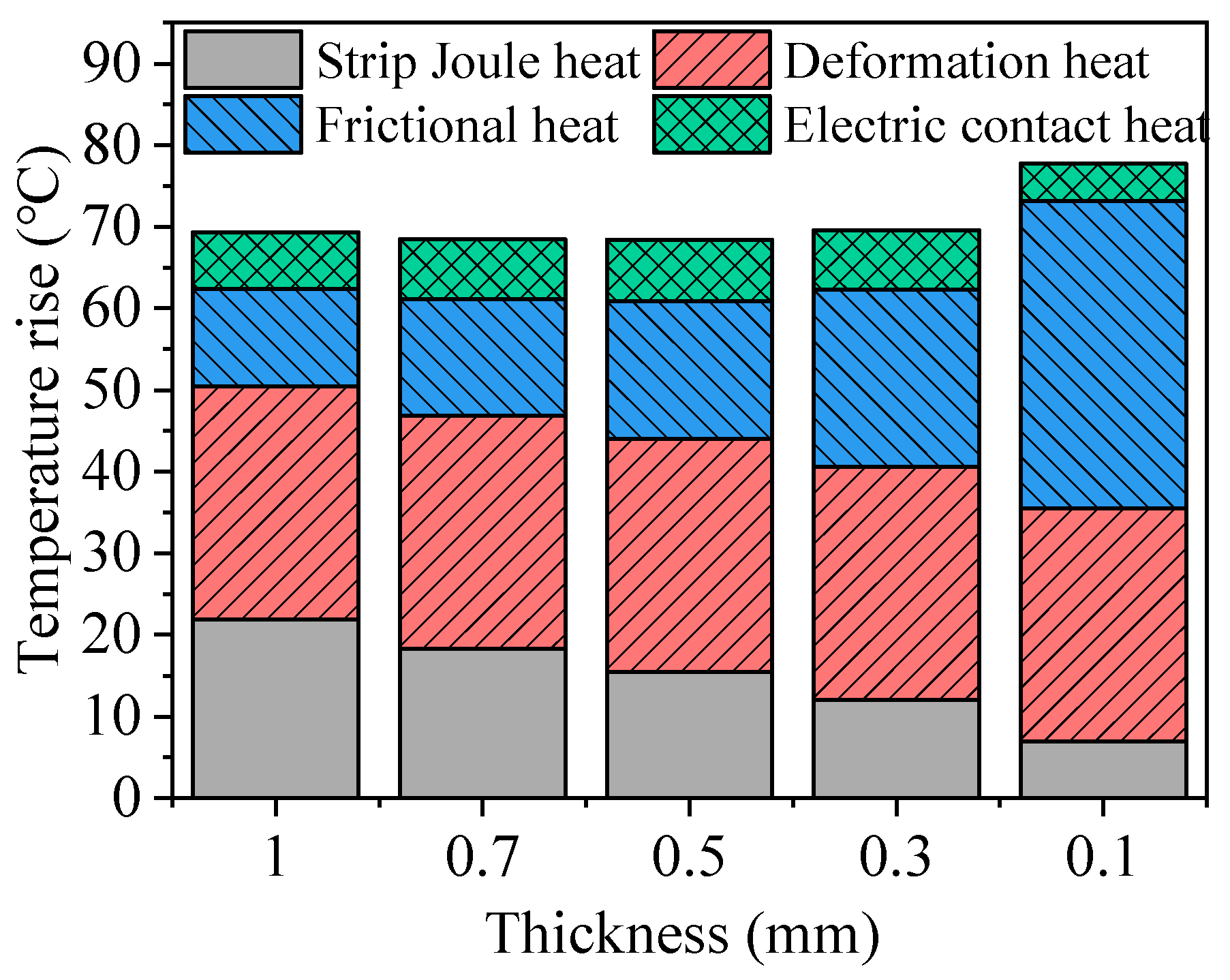
- A thorough analysis of the effect of dynamical boundary conditions on the transient temperature in the transition zone and deformation zone of the AZ31 foil in the presence of a loaded pulse current is presented, taking into account the particularities of the NAER heat source and heat exchange mechanism. Both the experimental results and the simulation results show that the temperature in the deformation zone decreases significantly with the decrease in inlet thickness. Compared with the temperature rise of deformation heat, friction heat, and Joule heat in the deformation zone, the influence of Joule heat (the material of Joule heat and electric contact heat) on the temperature rise of the deformation zone is gradually reduced to 8.9%, and the influence of Joule heat on the temperature rise of the deformation zone can be almost ignored. Therefore, the current parameters must be calculated accurately according to the NAER temperature field model to ensure the stability of temperature in the deformation zone.
- (3)
- Combining the simulation of the AZ31 foil temperature field with the experimental results of the NAER showed that the electric pulse rolling temperature field model could accurately set the rolling entrance temperature, ensuring that there would be no high-temperature melting and low-temperature brittle fracture and greatly improving the surface quality of the rolled Mg foils. The microstructural analysis showed that the wrought Mg alloy initial recrystallization at 200 °C and the texture strength of the alloy turned into a turning point at the entrance temperature of 200~320 °C. In particular, at a current density of 28.5 A/mm2, the grain size is 4.61 μm and the texture intensity is 11.3 at the inlet of the mill.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, Q.; Liu, Y.; Luo, Q.; Song, J.; Xiao, B.; Jiang, B.; Wu, L.; Zhao, H.; Shen, Q.; Pan, F. Ameliorating the edge cracking behavior of Mg-Mn-Al alloy sheets prepared by multi-pass online heating rolling. J. Manuf. Process. 2023, 85, 977–986. [Google Scholar] [CrossRef]
- Chen, Q.; Chen, R.; Su, J.; He, Q.; Tan, B.; Xu, C.; Huang, X.; Dai, Q.; Lu, J. The mechanisms of grain growth of Mg alloys: A review. J. Magnes. Alloys 2022, 10, 2384–2397. [Google Scholar] [CrossRef]
- Ren, X.; Huang, Y.; Zhang, X.; Li, H.; Zhao, Y. Influence of shear deformation during asymmetric rolling on the microstructure, texture, and mechanical properties of the AZ31B magnesium alloy sheet. Mater. Sci. Eng. A-Struct. Mater. Prop. Microstruct. Process. 2021, 800, 140306. [Google Scholar] [CrossRef]
- Tian, J.; Lu, H.; Zhang, W.; Nie, H.; Shi, Q.; Deng, J.; Liang, W.; Wang, L. An effective rolling process of magnesium alloys for suppressing edge cracks: Width-limited rolling. J. Magnes. Alloys 2022, 10, 2193–2207. [Google Scholar] [CrossRef]
- Kuang, J.; Li, X.; Zhang, R.; Ye, Y.; Luo, A.A.; Tang, G. Enhanced rollability of Mg-3Al-1Zn alloy by pulsed electric current: A comparative study. Mater. Des. 2016, 100, 204–216. [Google Scholar] [CrossRef]
- Liu, Q.; Song, J.; Zhao, H.; Xiao, B.; Zheng, X.; Pan, F. Improved edge quality for AZ31 sheets using online heating rolling technique. J. Mater. Eng. Perform. 2020, 29, 4212–4221. [Google Scholar] [CrossRef]
- Huang, Y.; Xiao, B.; Song, J.; Zhao, H.; Liu, Q.; Jiang, B.; Pan, F. Effect of tension on edge crack of on-line heating rolled AZ31B magnesium alloy sheet. J. Mater. Res. Technol.-JmrT 2020, 9, 1988–1997. [Google Scholar] [CrossRef]
- Liu, Q.; Song, J.; Pan, F.; She, J.; Zhang, S.; Peng, P. The edge crack, texture evolution, and mechanical properties of Mg-1Al-1Sn-Mn alloy sheets prepared using on-line heating rolling. Metals 2018, 8, 860. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, H.; Liu, G. Performance analysis of wide magnesium alloy foil rolled by multi-pass electric plastic rolling. Met. Mater. Int. 2023, 29, 2783–2794. [Google Scholar] [CrossRef]
- Xu, Z.; Tang, G.; Tian, S.; Ding, F.; Tian, H. Research of electroplastic rolling of AZ31 Mg alloy strip. J. Mater. Process. Technol. 2006, 182, 128–133. [Google Scholar] [CrossRef]
- Wang, R.; Xu, Z.; Jiang, Y.; Tang, G.; Wan, J.; Li, Q. Design high-performance AZ31 ultrathin strip through multi-pass electroplastic rolling without off-line annealing. Mater. Sci. Eng. A-Struct. Mater. Prop. Microstruct. Process. 2023, 862, 144510. [Google Scholar] [CrossRef]
- Chai, X.; Li, H.; Zhang, J.; Zhou, Y.; Ma, H.; Zhang, P.; Tian, J. A highly adaptable temperature field calculation model for work roll in hot tandem rolling. J. Plast. Eng. 2017, 24, 36–41. [Google Scholar]
- Wu, H.; Sun, J.; Lu, X.; Peng, W.; Wang, Q.; Zhang, D. Predicting stress and flatness in hot-rolled strips during run-out table cooling. J. Manuf. Process. 2022, 84, 815–831. [Google Scholar] [CrossRef]
- Jiang, Y.; Tang, G.; Shek, C.; Xie, J.; Xu, Z.; Zhang, Z. Mechanism of electropulsing induced recrystallization in a cold-rolled Mg-9Al-1Zn alloy. J. Alloys Compd. 2012, 536, 94–105. [Google Scholar] [CrossRef]
- Liu, G.; Yang, L.; Zhang, H. The electric-thermal uneven characteristics simulation of wide Mg alloy strip and electroplastic rolling experiment. Appl. Sci. 2023, 13, 2772. [Google Scholar] [CrossRef]
- Liu, X.; Fu, L.; Lu, Y.; Wang, T.; Xiao, H. Theory and numerical simulation of minimum rolling thickness for thin strip rolling. Iron Steel 2021, 56, 87–95. [Google Scholar]
- Yang, L.; Liu, S.; Liu, G. Dynamic contact heat transfer mechanism of magnesium alloy strip by rolling process simulation. Rare Met. Mater. Eng. 2023, 52, 890–898. [Google Scholar]
- Wang, X.; Xu, J.; Shan, D.; Guo, B.; Cao, J. Modeling of thermal and mechanical behavior of a magnesium alloy AZ31 during electrically-assisted micro-tension. Int. J. Plast. 2016, 85, 230–257. [Google Scholar] [CrossRef]
- Li, Y.; Ma, L.; Jiang, Z.; Huang, Z.; Lin, J.; Ji, Y. Numerical simulation and experimental verification of temperature field in medium plate rolling of AZ31 magnesium alloy. Rare Met. Mater. Eng. 2019, 48, 2185–2192. [Google Scholar]
- Liao, H.; Tang, G.; Jiang, Y.; Xu, Q.; Sun, S.; Liu, J. Effect of thermo-electropulsing rolling on mechanical properties and microstructure of AZ31 magnesium alloy. Mater. Sci. Eng. A-Struct. Mater. Prop. Microstruct. Process. 2011, 529, 138–142. [Google Scholar] [CrossRef]
- Guan, D.; Rainforth, W.M.; Ma, L.; Wynne, B.; Gao, J. Twin recrystallization mechanisms and exceptional contribution to texture evolution during annealing in a magnesium alloy. Acta Mater. 2017, 126, 132–144. [Google Scholar] [CrossRef]





| Rolling Pass | RMS Current Density Je/(A/mm2) | Inlet Thickness h0/mm | Outlet Thickness h1/mm | Rolling Force/kN |
|---|---|---|---|---|
| 1 | 21.7 | 1.050 | 0.90 | 56.24 |
| 2 | 24.0 | 0.901 | 0.77 | 62.05 |
| 3 | 28.5 | 0.772 | 0.62 | 77.7 |
| 4-1 | 25.0 | 0.601 | 0.512 | 70.5 |
| 4-2 | 28.5 | 0.599 | 0.491 | 65.4 |
| 4-3 | 32.1 | 0.601 | 0.472 | 60.2 |
| Mass Density ρm/kg·m−3 | Electrical Resistivity Rm/nΩ·m | Temperature Tm/°C | Specific Heat Capacity cm/J·kg−1·°C−1 | Thermal Conductivity km/W·m−1·°C−1 |
|---|---|---|---|---|
| 1.754 × 103 | 91.405 | 25 | 1005 | 84.7 |
| 100 | 1032 | 90.5 | ||
| 200 | 1049 | 95.8 | ||
| 300 | 1069 | 98.4 | ||
| 400 | 1092 | 98.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, G.; Yang, J.; Shan, T.; Li, H.; Wang, D.; Yang, L. The Coupled Temperature Field Model of Difficult-to-Deform Mg Alloy Foil High-Efficiency Electro-Rolling and Experimental Study. Metals 2024, 14, 343. https://doi.org/10.3390/met14030343
Liu G, Yang J, Shan T, Li H, Wang D, Yang L. The Coupled Temperature Field Model of Difficult-to-Deform Mg Alloy Foil High-Efficiency Electro-Rolling and Experimental Study. Metals. 2024; 14(3):343. https://doi.org/10.3390/met14030343
Chicago/Turabian StyleLiu, Gengliang, Jiaxuan Yang, Tianren Shan, Huaimei Li, Dianlong Wang, and Lipo Yang. 2024. "The Coupled Temperature Field Model of Difficult-to-Deform Mg Alloy Foil High-Efficiency Electro-Rolling and Experimental Study" Metals 14, no. 3: 343. https://doi.org/10.3390/met14030343
APA StyleLiu, G., Yang, J., Shan, T., Li, H., Wang, D., & Yang, L. (2024). The Coupled Temperature Field Model of Difficult-to-Deform Mg Alloy Foil High-Efficiency Electro-Rolling and Experimental Study. Metals, 14(3), 343. https://doi.org/10.3390/met14030343







