Damage Analysis of Third-Generation Advanced High-Strength Steel Based on the Gurson–Tvergaard–Needleman (GTN) Model
Abstract
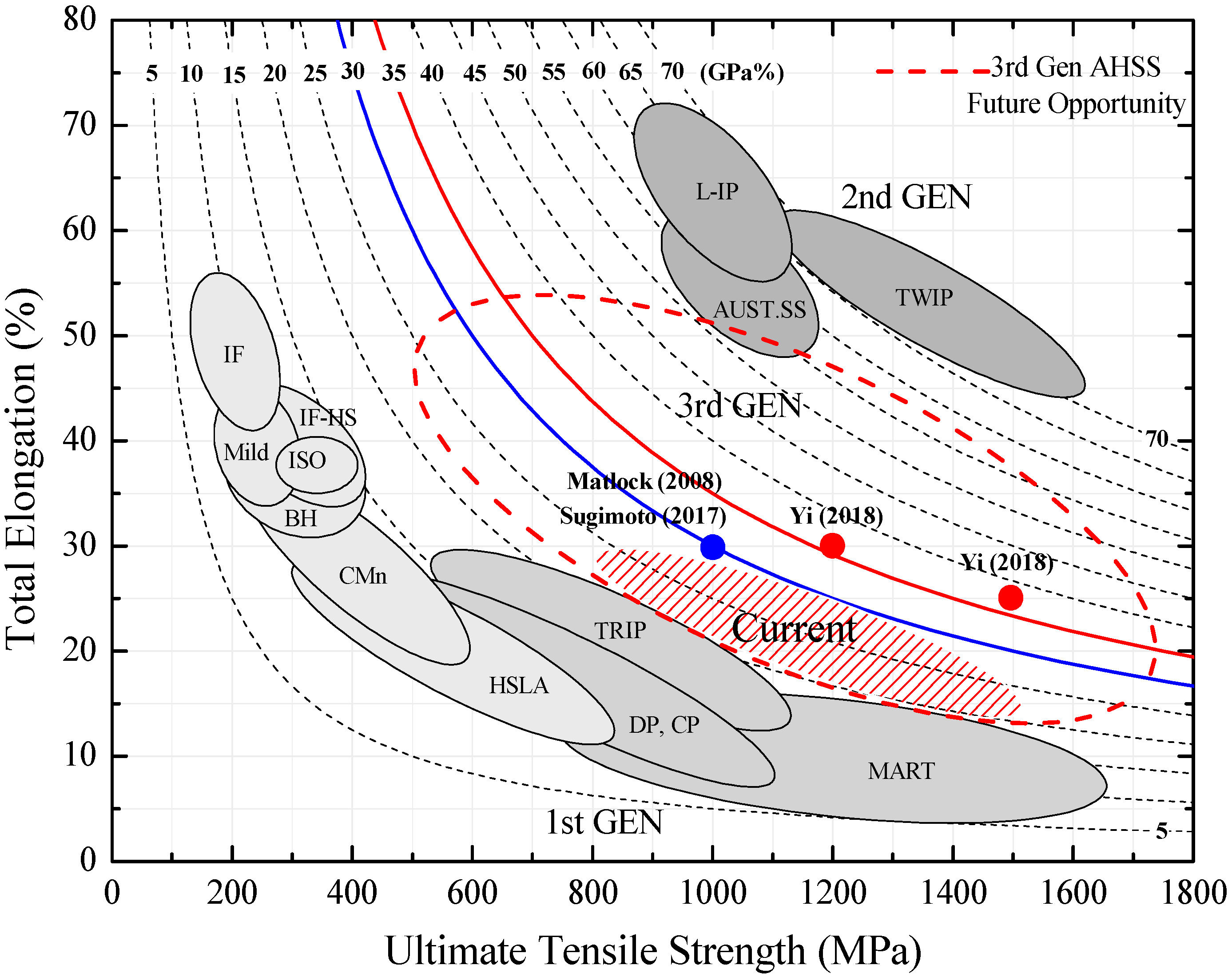
:1. Introduction
2. Materials and Methods
2.1. Microstructural Analysis
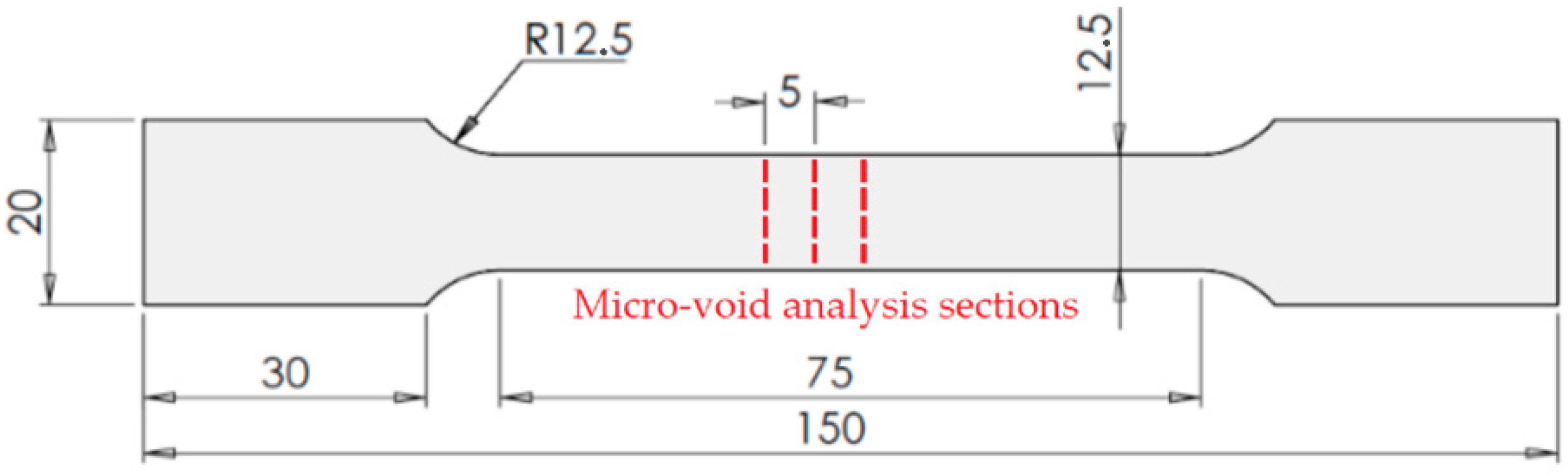
2.2. Mechanical Testing
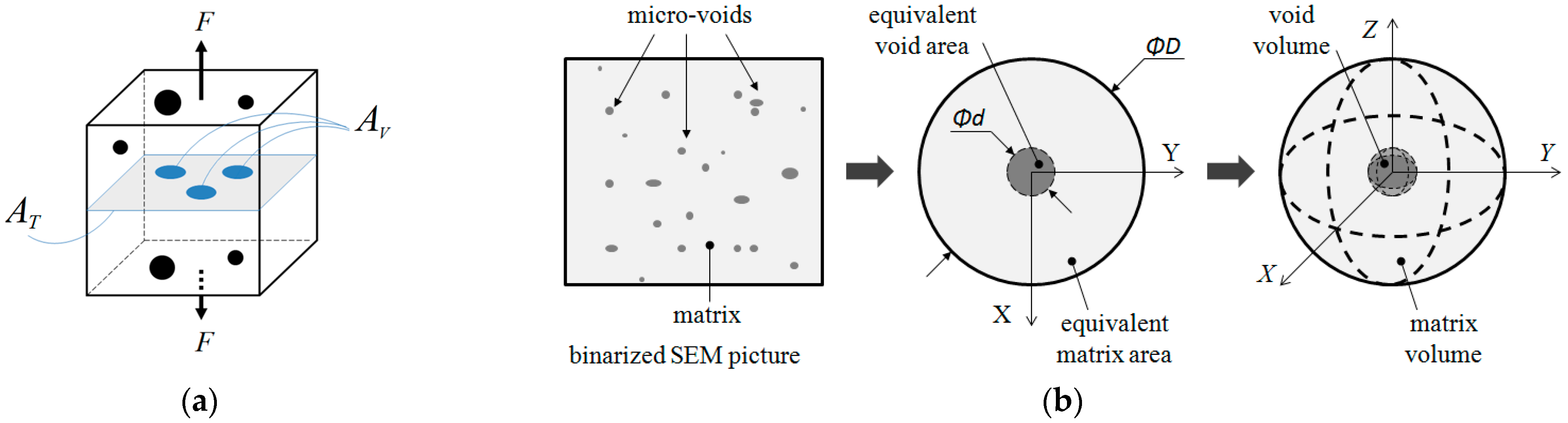
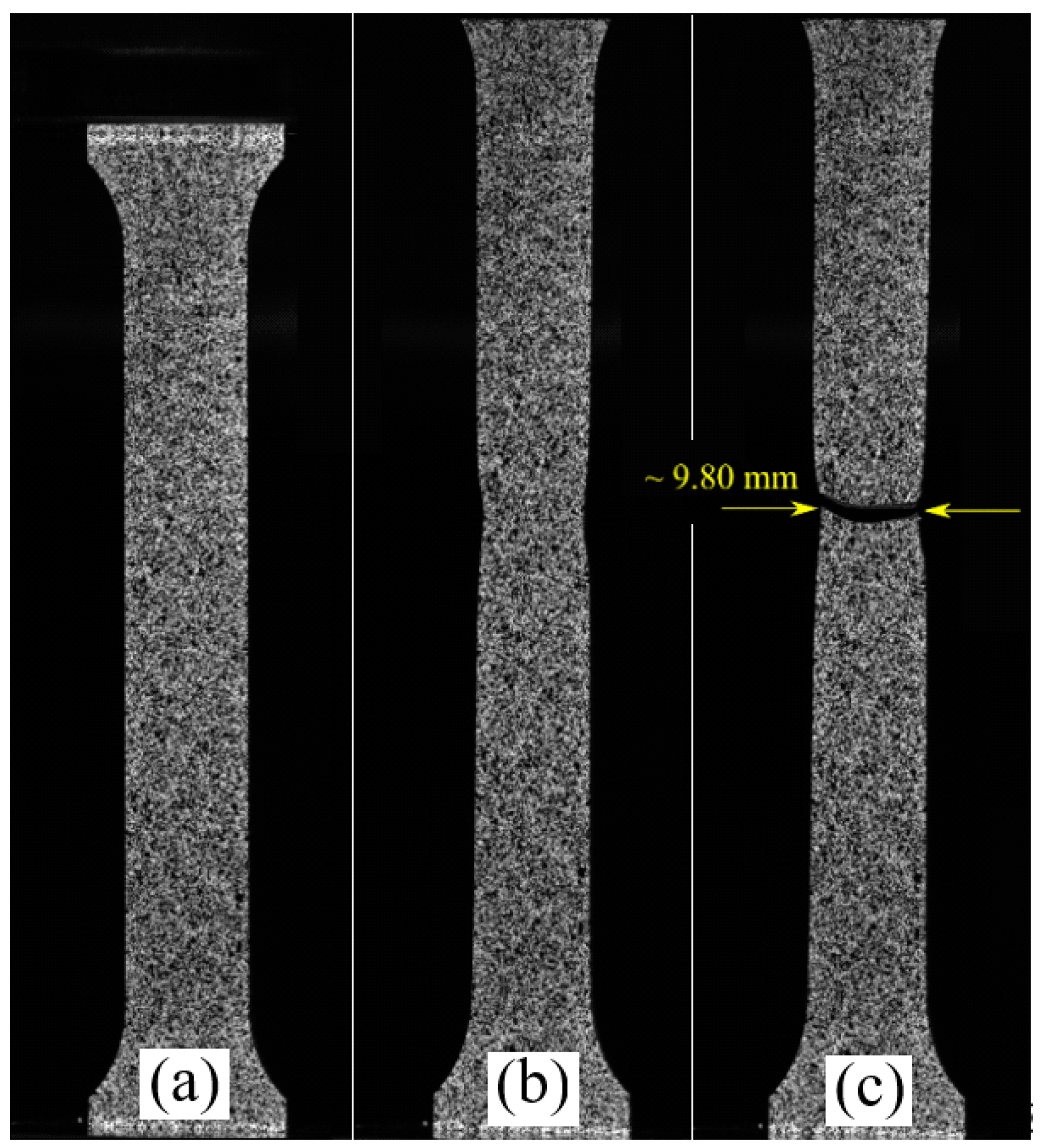
2.3. Microvoid Analysis
2.4. GTN Damage Model Parameters Calibration
3. Results
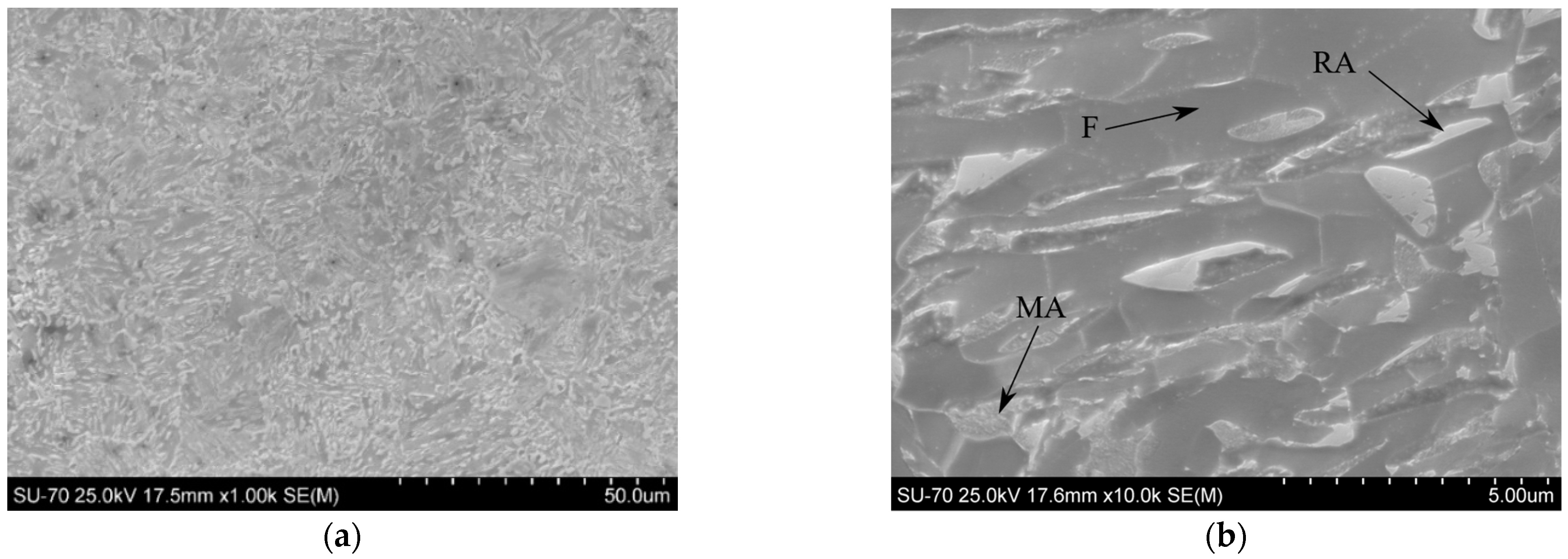
3.1. As-Received Microstructure
3.2. Mechanical Properties
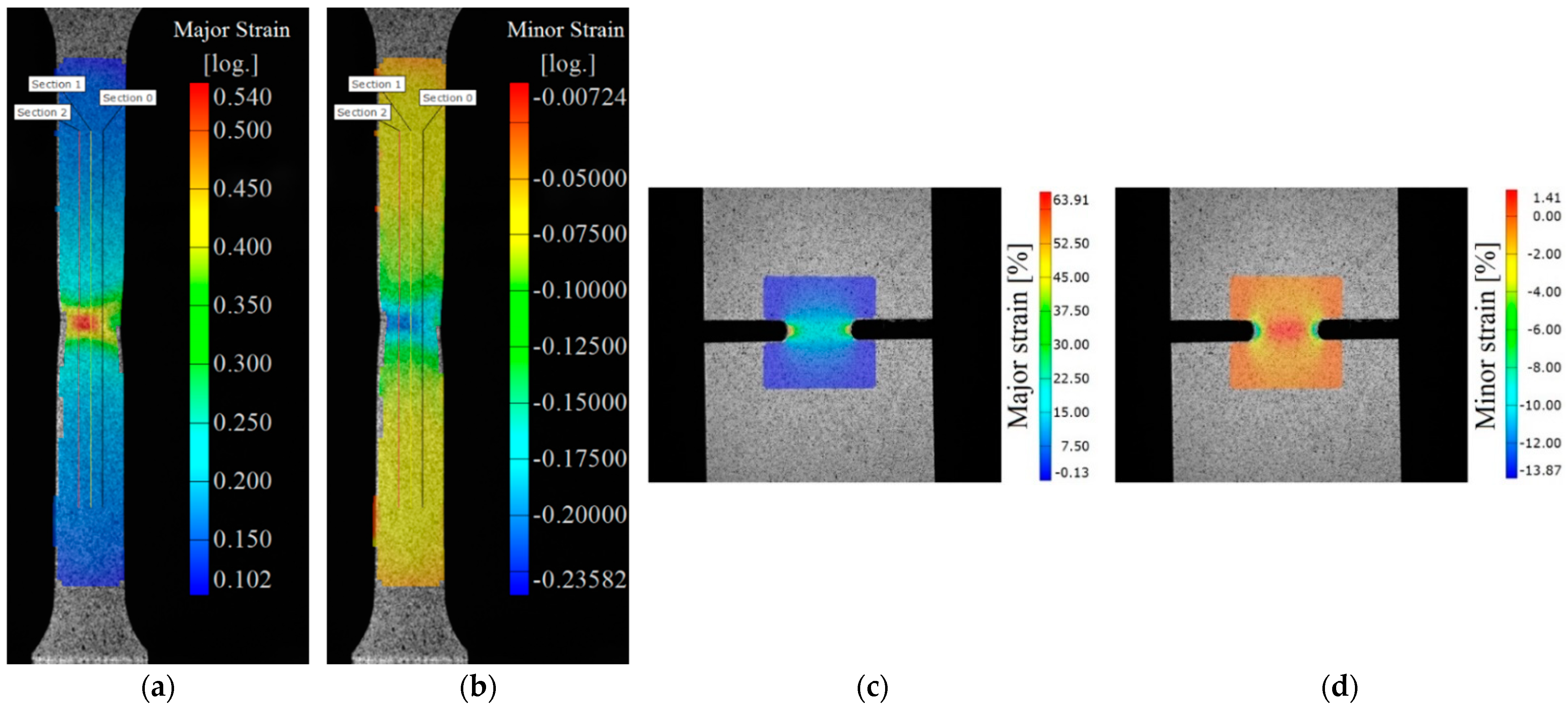
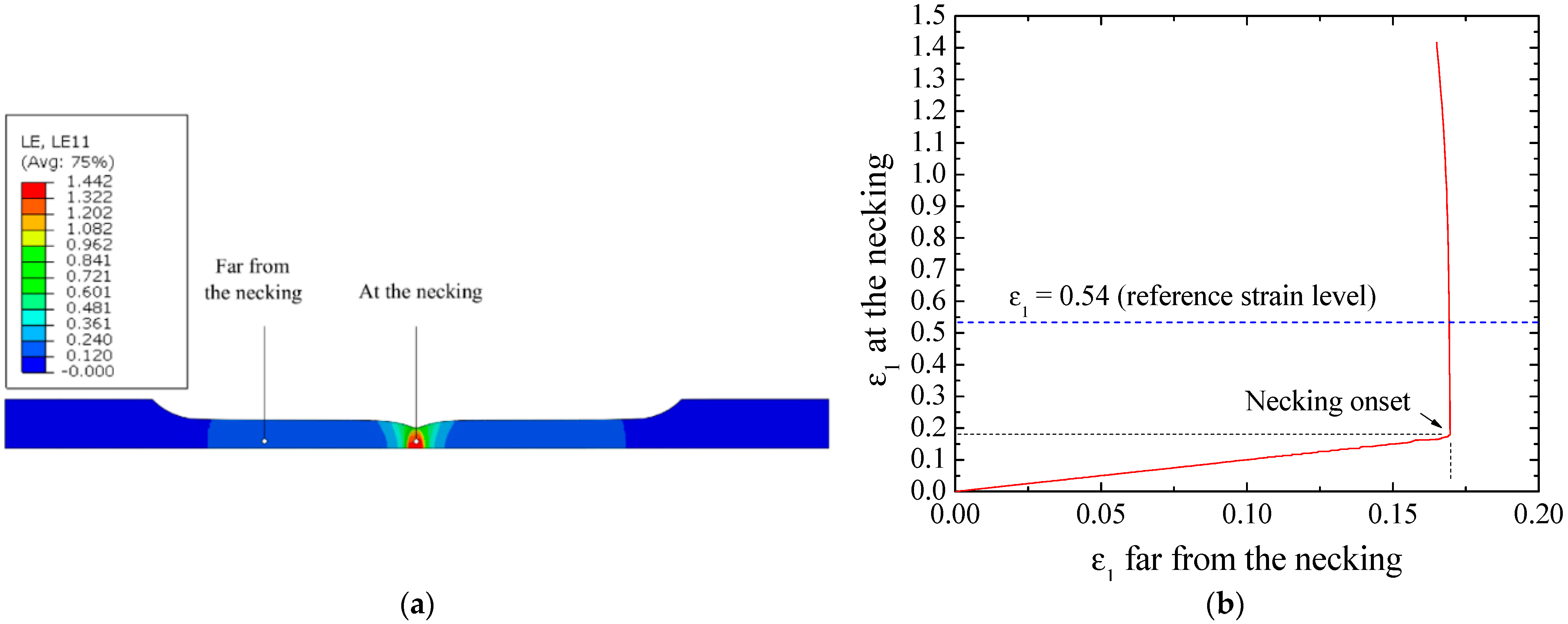
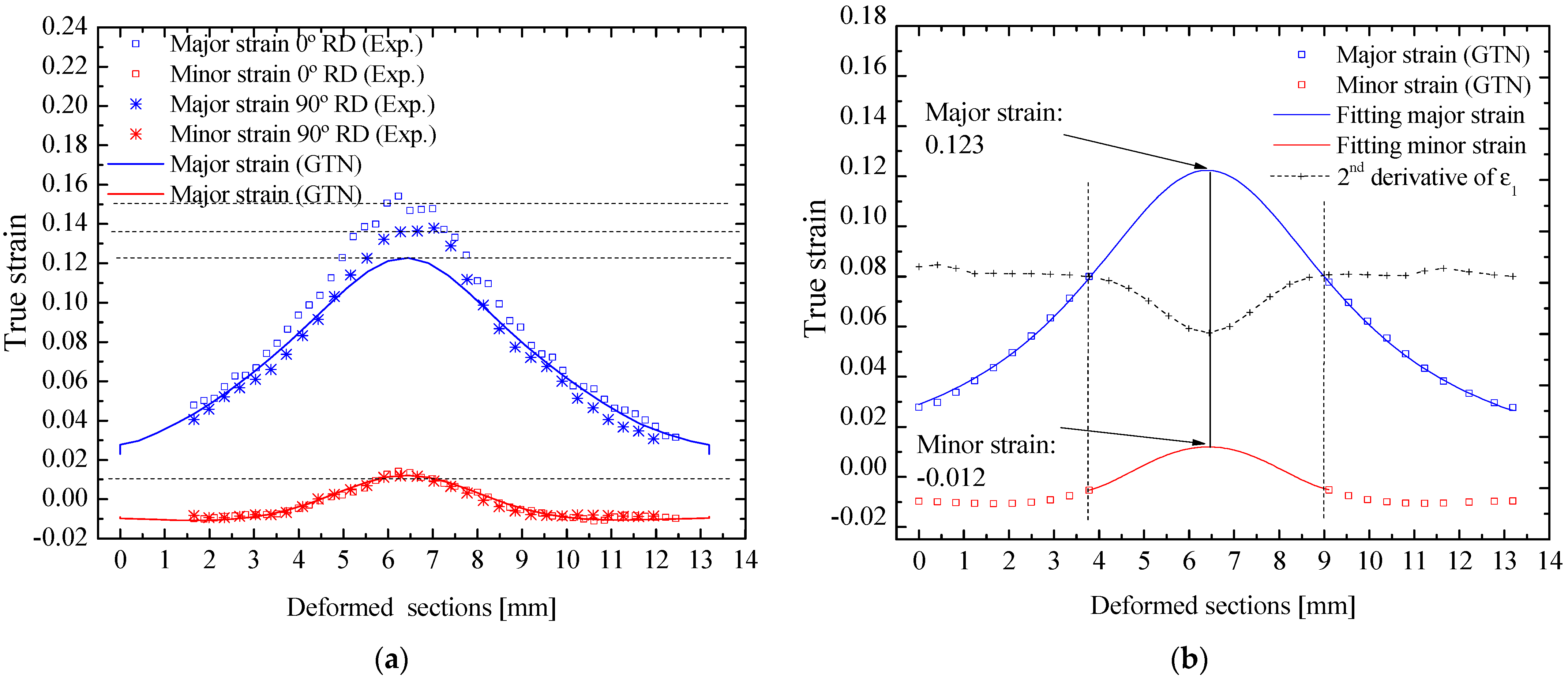
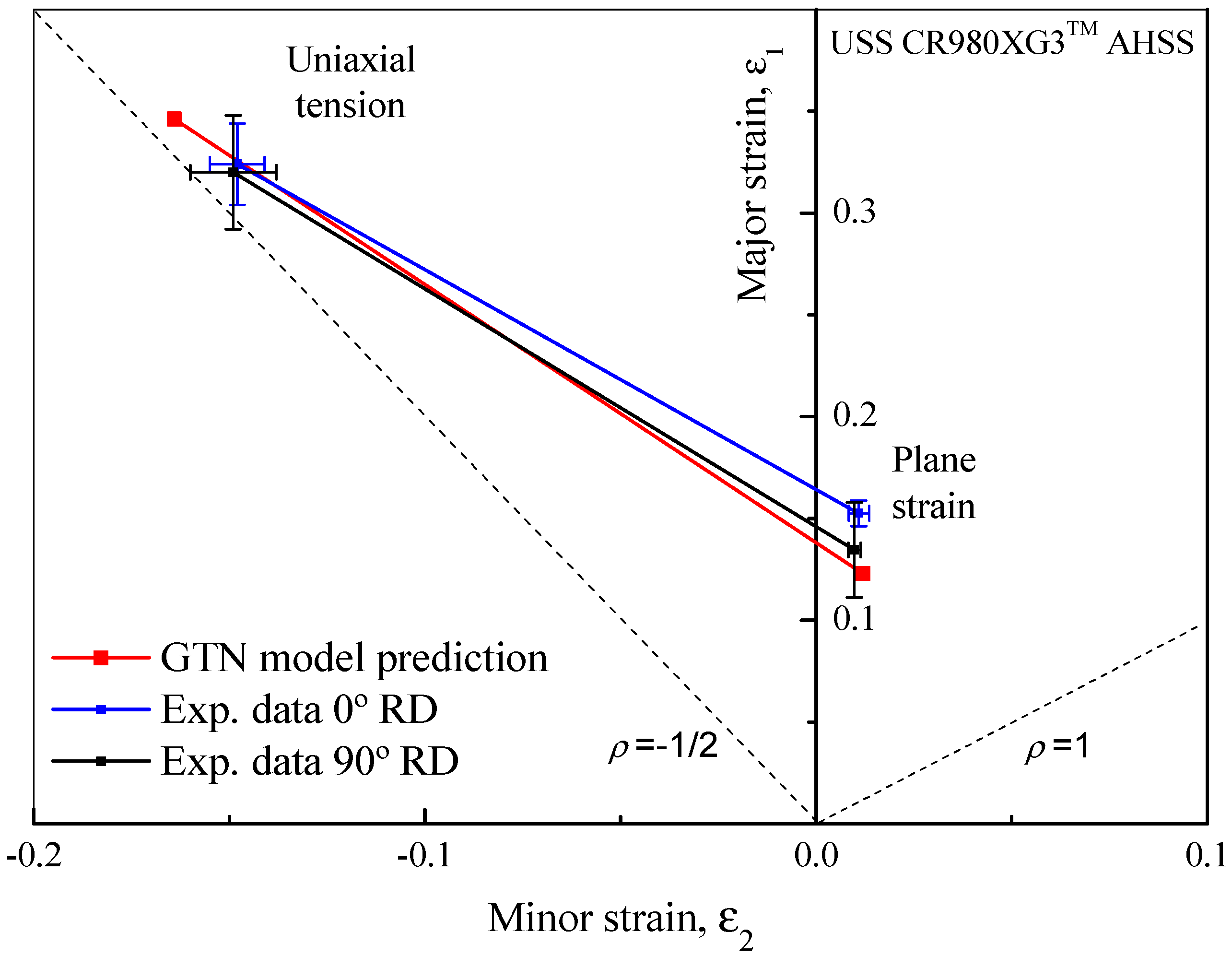
3.3. Limit Strains
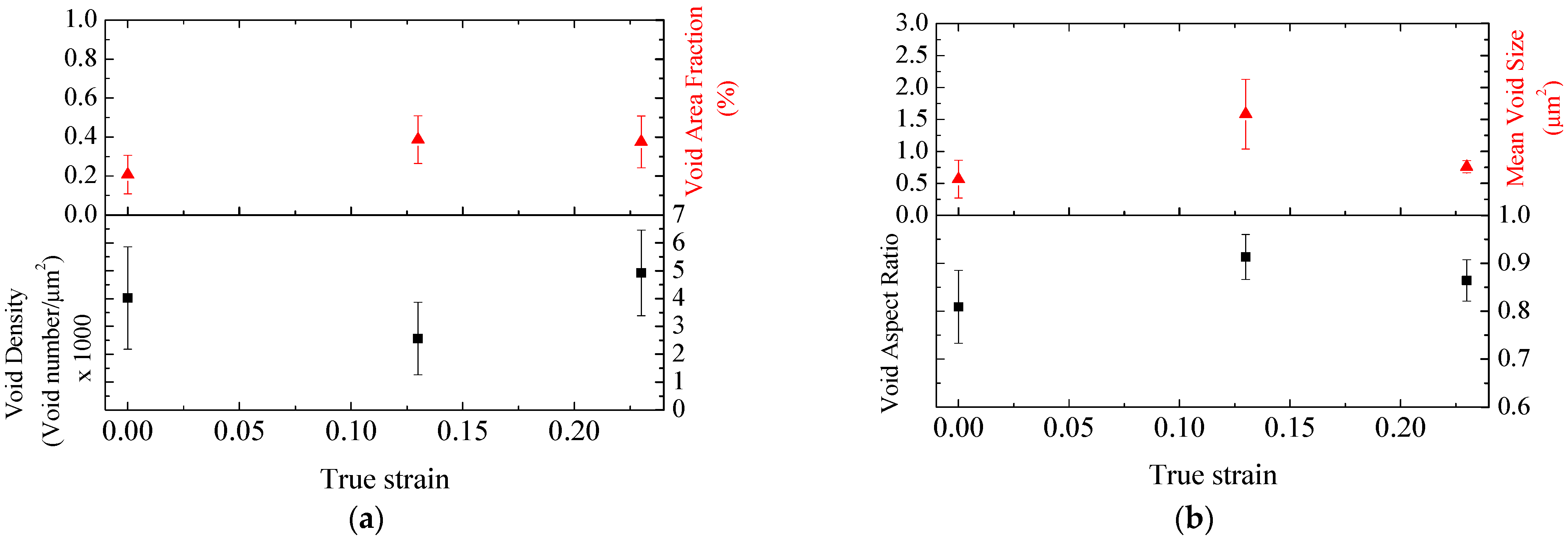
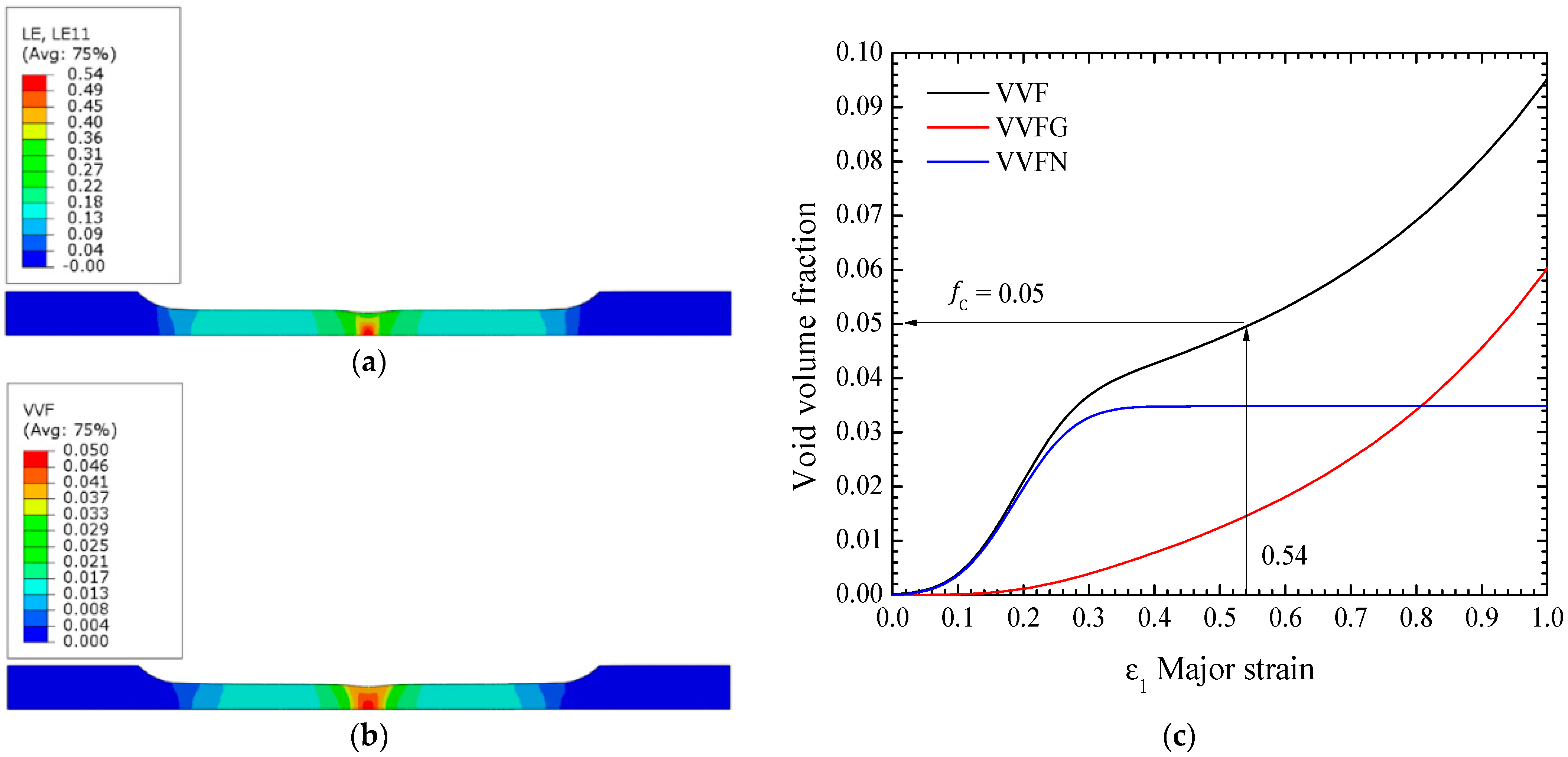
3.4. Void Results
3.5. Full Set of GTN Damage Model Parameters
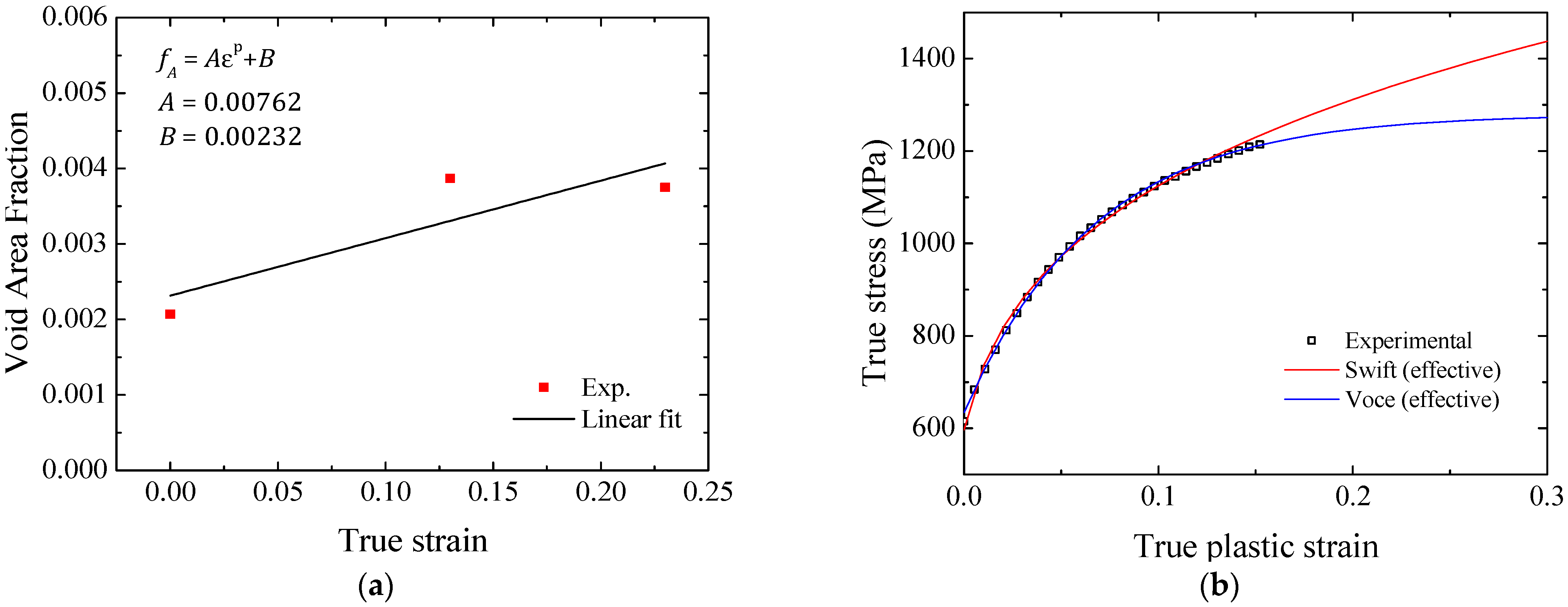
3.5.1. Effective Work Hardening and Initial Porosity
3.5.2. Yield Locus Parameters (, , )
3.5.3. Void Nucleation Parameters (, , )
3.5.4. Failure Parameters (, )
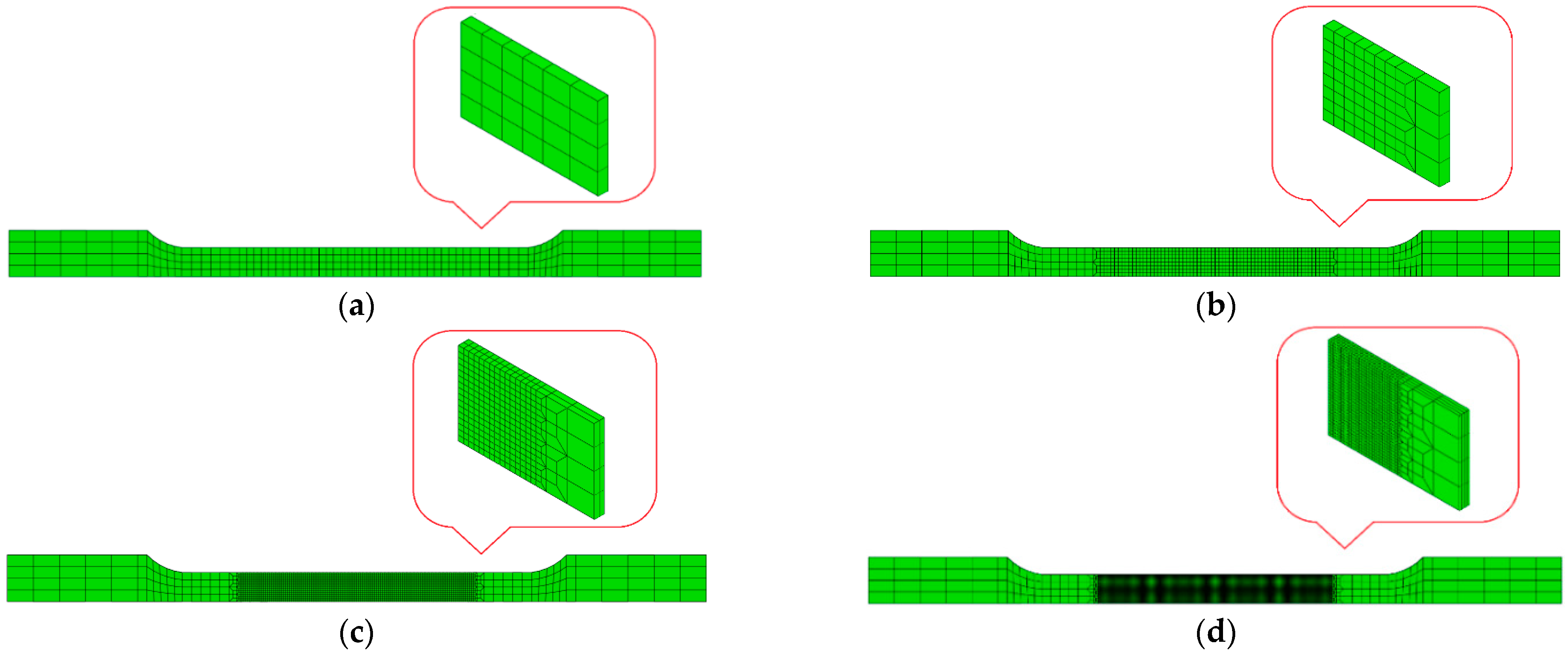
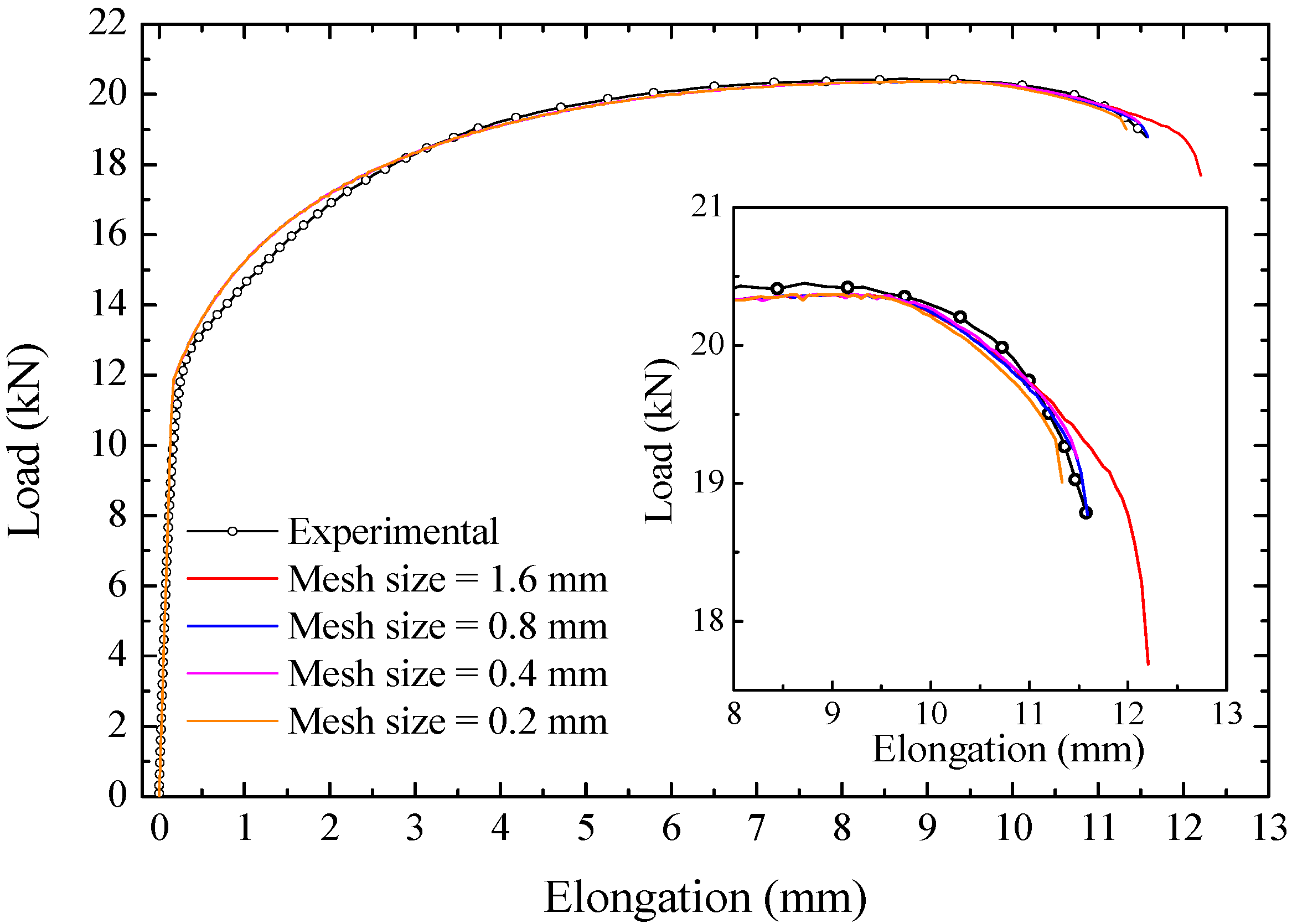
3.5.5. Mesh Sensitivity
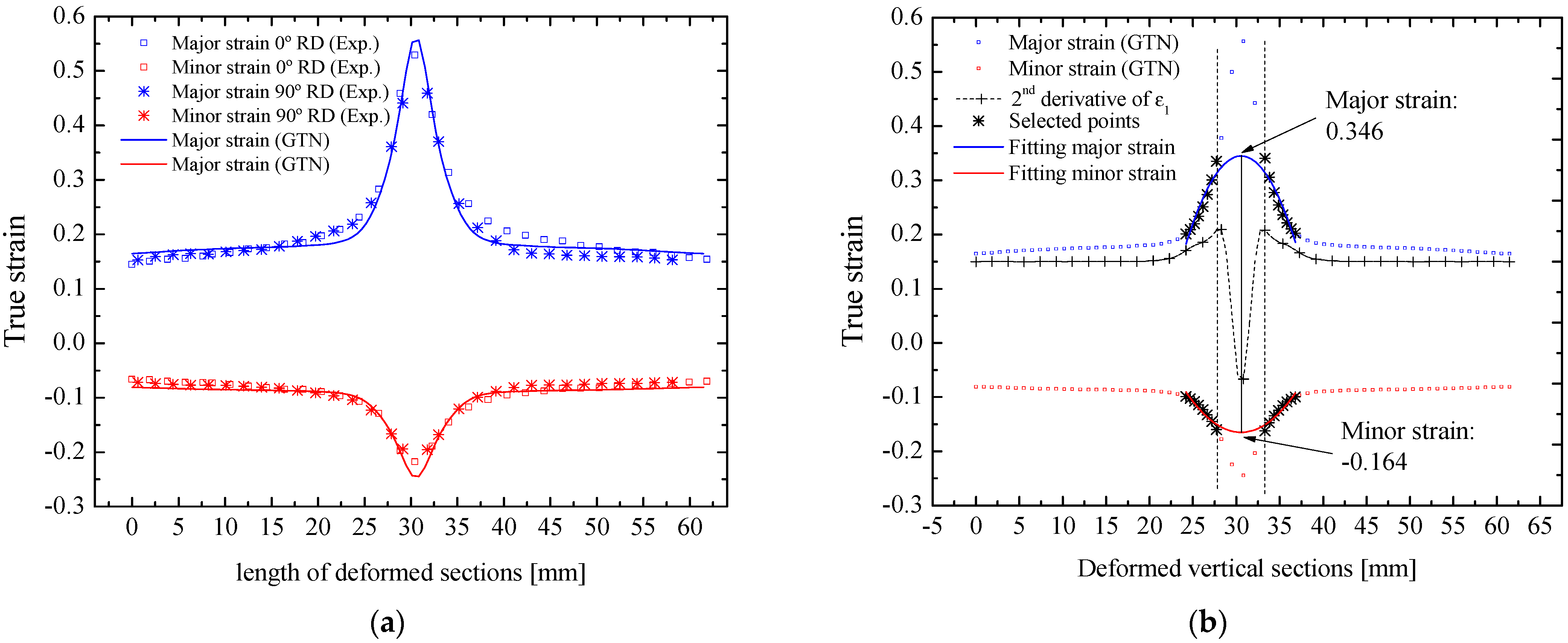
3.6. Numerical Predictions of the In-Plane Limit Strains
4. Discussion
5. Conclusions
- The standard mechanical testing provided the average mechanical properties, namely the yield stress (604 MPa), ultimate tensile strength (1040 MPa), and total elongation (23.4%). The tested material presents a weak initial plastic anisotropy, with a planar anisotropy close to zero (−0.079) along with the normal anisotropy coefficient close to unity (0.917).
- In the as-received state, the XRD analysis provided a retained austenite volume fraction of 12.2%, which, in turn, is prone to transform into martensite during the early stages of plastic straining.
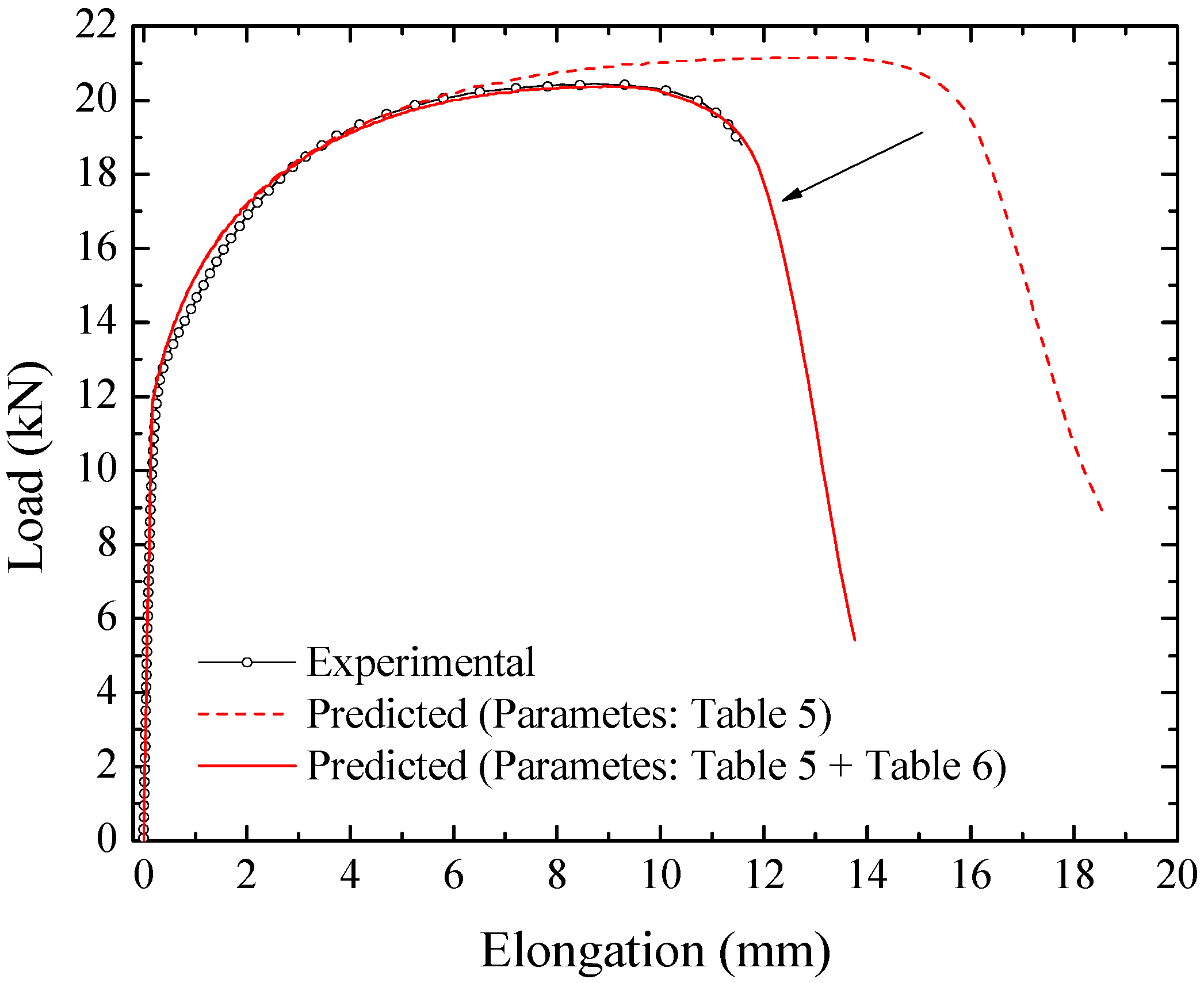
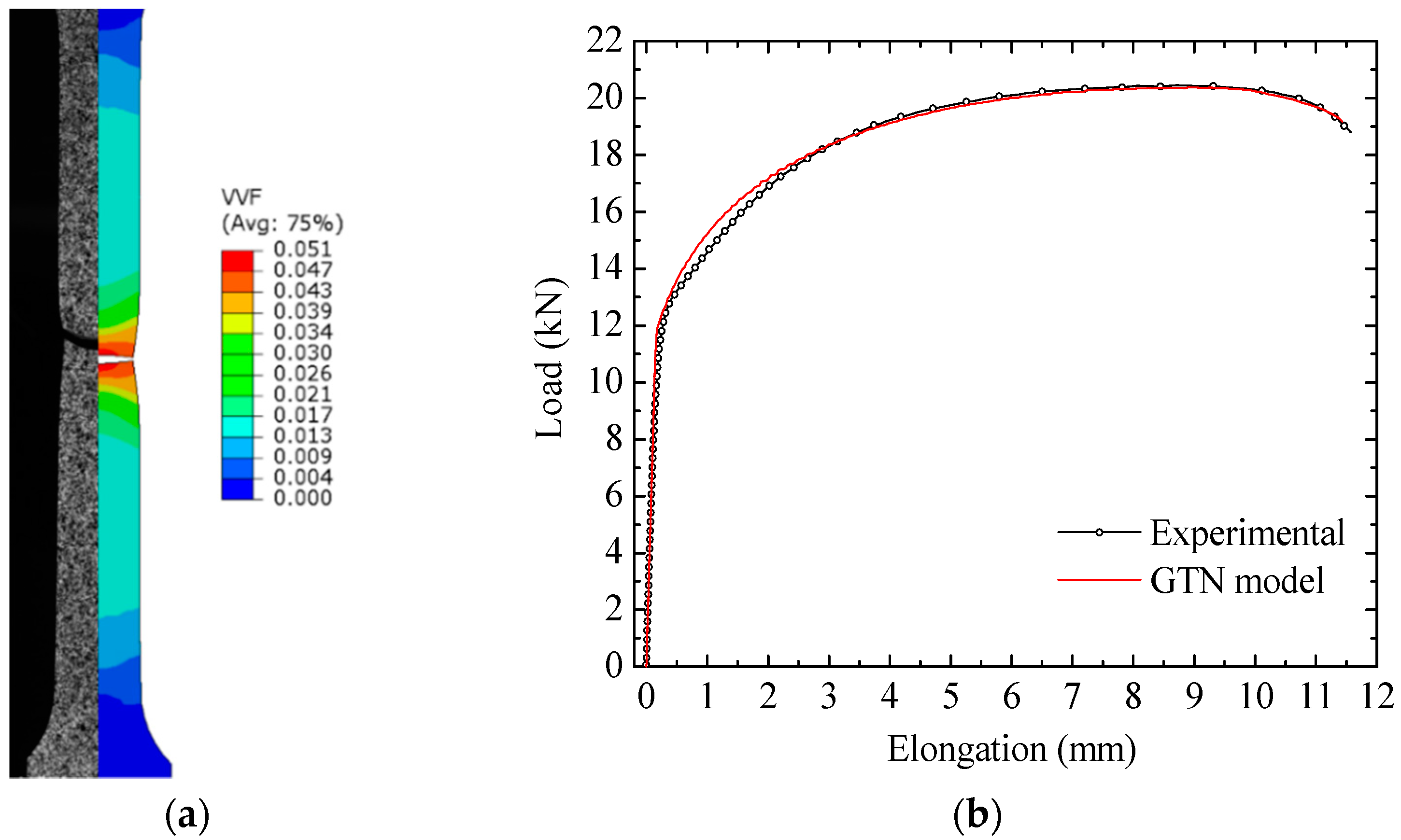
- The CR980XG3TM steel provided an experimental Lankford r-value close to the unity, and thus isotropic plasticity could be assumed as a first approximation in the modeling. The identified damage parameters of the GTN model were able to reproduce the experimental load-elongation obtained from the uniaxial tensile test. The mesh sensitivity analysis also showed that the mesh size does not influence the finite element predictions in the uniform elongation domain. However, as the necking appears, the smaller the mesh size, and hence the deformation is more localized. A mesh size of 0.4 mm in the gauge length zone was enough to fit the experimental data.
- A simple methodology for calibrating the parameters of the GTN model was performed based on the adopted mechanical testing and finite element simulations. The calibration method provided the complete set of the GTN damage model parameters for the CR980XG3TM steel, namely = 0.99988, = 1.74, = 0.83, = 0.18, = 0.07, = 0.035, = 0.05, and = 0.095. Moreover, the calibrated GTN parameters provided an excellent forecast for the experimental limit strains located on the left-hand side of the forming limit curve.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Council of European Union–European Parliament. Regulation (EU) Nº 333/2014 of European Parliament of the Council of 11 March 2014 amending Regulation (EC) Nº 443/2009 to define the modalities for reaching the 2020 target to reduce CO2 emissions from new passenger cars. Off. J. Eur. Union 2014, 103, 15–21. [Google Scholar]
- Mock, P. CO2 Emission Standards for Passenger Cars and Light Commercial Vehicles in European Union. ICCT—The International Council on Clean Transportation. 2019. Available online: https://theicct.org/publication/co2-emission-standards-for-passenger-cars-and-light-commercial-vehicles-in-the-european-union/ (accessed on 9 December 2021).
- ACEA—European Automobile Manufacturers Association. The European Commission’s Proposal on Post-2021 CO2 Targets for Cars and Vans. ACEA European Automobile Manufacturers Association, March 2018. Available online: https://www.acea.auto/publication/position-paper-european-commission-proposal-on-post-2021-co2-targets-for-cars-and-vans/ (accessed on 9 December 2021).
- Matlock, D.K.; Speer, J.G. Chapter 11—Third Generation of AHSS: Microstructure Design Concepts. In Proceedings of the International Conference on Microstructure and Texture in Steel and Other Materials, Jamshedpur, India, 5–7 February 2008; Haldar, A., Suwas, S., Bhattacharjee, D., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; pp. 185–205. [Google Scholar]
- Grajcar, A.; Kuziak, R.; Zalecki, W. Third generation of AHSS with increased fraction of retained austenite for the automotive industry. Arch. Civ. Mech. Eng. 2012, 12, 334–341. [Google Scholar] [CrossRef]
- Aydin, H.; Essadiqi, E.; Jung, I.-H.; Yue, S. Development of 3rd generation AHSS with medium Mn content alloying compositions. Mater. Sci. Eng. A 2013, 564, 501–508. [Google Scholar] [CrossRef]
- Speer, J.; Matlock, D.K.; De Cooman, B.C.; Schroth, J.G. Carbon partitioning into austenite after martensite transformation. Acta Mater. 2003, 51, 2611–2622. [Google Scholar] [CrossRef]
- Yi, H.L.; Sun, L.; Xiong, X.C. Challenges in the formability of the next generation of automotive steel sheets. Mater. Sci. Technol. 2018, 34, 1112–1117. [Google Scholar] [CrossRef]
- Sugimoto, K.-I.; Hojo, T.; Kobayashi, J. Critical assessment 29: TRIP-aided bainitic ferrite steels. Mater. Sci. Technol. 2017, 33, 2005–2009. [Google Scholar] [CrossRef]
- Branagan, D.; Parsons, C.; Machrowicz, T.; Cischke, J.; Frerichs, A.; Meacham, B.; Cheng, S.; Justice, G.; Sergueeva, A. Effect of Deformation during Stamping on Structure and Property Evolution in 3rd Generation AHSS. Open J. Met. 2018, 8, 15–33. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.; Speer, J.G. Quenching and Partitioning Steel Heat Treatment. Met. Microstruct. Anal. 2013, 2, 268–281. [Google Scholar] [CrossRef] [Green Version]
- Shen, I. General Motors Applies Third-Generation Advanced High-Strength Steel in New Vehicles for China—Open Innovation Enables Fast Adoption of Lightweight Technology. GM Corporate Newsroom, Shanghai, 1 January 2015. Available online: https://media.gm.com/media/cn/en/gm/news.detail.html/content/Pages/news/cn/en/2015/dec/1201_advanced-steel.html (accessed on 12 October 2021).
- Hance, B.M.; Link, T.M. Effects of fracture area measurement method and tension test specimen type on fracture strain values of 980 class AHSS. In Proceedings of the IOP Conference Series: Materials Science and Engineering, 38th International Deep Drawing Research Group Annual Conference, Enschede, The Netherlands, 3–7 June 2019; Volume 651, p. 012061. [Google Scholar] [CrossRef]
- Macek, B.; Lutz, J. Virtual and Physical Testing of Third-Generation High Strength Steel—Evaluating a New High-Strength Steel’s Ability to Improve on an Existing Stamped-Steel Production Part. SAE International—Automotive Engineering. 18 August 2020. Available online: https://www.sae.org/news/2020/08/stamping-ahss-tech-paper (accessed on 12 October 2021).
- Kempken, J. Chapter 16—Technological challenges and solutions for the production of state-of-the-art second-and third-generation AHSS grades. In Advanced High Strength Steel, Lecture Notes in Mechanical Engineering; Roy, T.K., Ed.; Springer: Dusseldorf, Germany, 2018; pp. 143–148. [Google Scholar]
- Paykani, M.A.; Shahverdi, H.R.; Merismaeilli, R. First and third generation of advanced high strength steel in FeCrNiBSi system. J. Mater. Process. Technol. 2016, 238, 256–265. [Google Scholar]
- Hance, B. Advanced High Strength Steel (AHSS) performance level definitions and targets. SAE Int. J. Mater. Manf. 2018, 11, 505–516. [Google Scholar] [CrossRef]
- Noder, J.; Gutierrez, J.E.; Zhumagulov, A.; Dykeman, J.; Ezzat, H.; Butcher, C. A Comparative Evaluation of Third-Generation Advanced High-Strength Steels for Automotive Forming and Crash Applications. Materails 2021, 14, 4970. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Ma, L.; Peng, P.; Jia, Q.; Wan, Z.; Zhu, Y.; Guo, W. Microstructural evolution and deformation behavior of fiber laser welded QP980 steel joint. Mater. Sci. Eng. A 2018, 717, 124–133. [Google Scholar] [CrossRef]
- Guo, W.; Wan, Z.; Peng, P.; Jia, Q.; Zou, G.; Peng, Y. Microstructure and mechanical properties of fiber laser welded QP980 steel. J. Mater. Process. Technol. 2018, 256, 229–238. [Google Scholar] [CrossRef]
- Guo, W.; Wan, Z.; Jia, Q.; Ma, L.; Zhang, H.; Tan, C.; Peng, P. Laser weldability of TWIP980 with DP980/B1500HS/QP980 steels: Microstructure and mechanical properties. Opt. Laser Technol. 2020, 124, 105961. [Google Scholar] [CrossRef]
- Pereira, A.B.; Santos, R.O.; Carvalho, B.S.; Butuc, M.C.; Vincze, G.; Moreira, L.P. The Evaluation of Laser Weldability of the Third-Generation Advanced High Strength Steel. Metals 2019, 9, 1051. [Google Scholar] [CrossRef] [Green Version]
- Zhao, H.; Huang, R.; Sun, Y.; Tan, C.; Wu, L.; Chen, B.; Song, X.; Li, G. Microstructure and mechanical properties of fiber laser welded QP980/press-hardened 22MnB5 steel joint. J. Mater. Res. Technol. 2020, 9, 10079–10090. [Google Scholar] [CrossRef]
- Sun, Q.; Lu, Y.; Chen, J. Identification of material parameters of a shear modified GTN damage model by small punch test. Int. J. Fract. 2020, 222, 25–35. [Google Scholar] [CrossRef]
- Gurson, A. Continuum theory of ductile rupture by void nucleation and growth. Part I. Yield criteria and flow rules for porous ductile media. J. Eng. Mater. Technol. 1977, 99, 2–15. [Google Scholar] [CrossRef]
- Tvergaard, V.; Needleman, A. Analysis of the cup-cone fracture in a round tensile bar. Acta Metall. 1984, 32, 157–169. [Google Scholar] [CrossRef]
- Li, G.; Cui, S. A review on theory and application of plastic meso-damage mechanics. Theor. Appl. Fract. Mech. 2020, 109, 102686. [Google Scholar] [CrossRef]
- Gholipour, H.; Biglari, F.; Nikbin, K. Experimental and numerical investigation of ductile fracture using GTN damage model on in-situ tensile tests. Int. J. Mech. Sci. 2019, 164, 105170. [Google Scholar] [CrossRef]
- Abbasi, M.; Ketabchi, M.; Izadkhah, H.; Fatmehsaria, D.; Aghbash, A. Identification of GTN model parameters by application of response surface methodology. Procedia Eng. 2011, 10, 415–420. [Google Scholar] [CrossRef] [Green Version]
- Kami, A.; Dariani, B.M.; Vanini, A.S.; Comsa, D.S.; Banabic, D. Numerical determination of the forming limit curves of anisotropic sheet metals using GTN damage model. J. Mater. Process. Technol. 2015, 216, 472–483. [Google Scholar] [CrossRef]
- ASTM. Standard E975-13; Standard Practice for X-ray determination of retained austenite in steel with Near-Random Crystallographic Orientation. American Society for Testing and Materials; ASTM: West Conshohocken, PA, USA, 2013.
- ISO 12004-2:2007; Metallic Materials—Sheet and Strip—Determination of Forming Limit Curves—Part 2: Determination of Forming-Limit Curves in Laboratory. International Organization for Standardization: Geneva, Switzerland, 2007.
- Schwindt, C.D.; Stout, M.; Iurman, L.; Signorelli, J.W. Forming Limit Curve Determination of a DP-780 Steel Sheet. Procedia Mater. Sci. 2015, 8, 978–985. [Google Scholar] [CrossRef] [Green Version]
- Samei, J.; Green, D.E.; Cheng, J.; Lima, M.S.D.C. Influence of strain path on nucleation and growth of voids in dual phase steel sheets. Mater. Des. 2016, 92, 1028–1037. [Google Scholar] [CrossRef]
- Chaboche, J. Continuum damage mechanics: Present state and future trends. Nucl. Eng. Des. 1987, 105, 19–33. [Google Scholar] [CrossRef]
- Santos, R.O.; da Silveira, L.B.; Moreira, L.P.; Cardoso, M.C.; da Silva, F.R.F.; Paula, A.D.S.; Albertacci, D.A. Damage identification parameters of dual-phase 600–800 steels based on experimental void analysis and finite element simulations. J. Mater. Res. Technol. 2019, 8, 644–659. [Google Scholar] [CrossRef]
- Faleskog, J.; Gao, X.; Shih, C.F. Cell model for nonlinear fracture analysis—I. Micromechanics calibration. Int. J. Fract. 1998, 89, 355–373. [Google Scholar] [CrossRef]
- Abaqus User’S Manual; Version 6.9; Dassault Systemes Simulia Corp.: Providence, RI, USA, 2009.
- Finfrock, C.B.; Clarke, A.J.; Thomas, G.A.; Clarke, K.D. Austenite Stability and Strain Hardening in C-Mn-Si Quenching and Partitioning Steels. Met. Mater. Trans. A 2020, 51, 2025–2034. [Google Scholar] [CrossRef]
- Navarro-López, A.; Hidalgo, J.; Sietsma, J.; Santofimia, M.J. Characterization of bainitic/martensitic structures formed in isothermal treatments below the Ms temperature. Mater. Charact. 2017, 128, 111–127. [Google Scholar] [CrossRef]
- Jatczak, C.F. Retained Austenite and Its Measurement by X-ray Diffraction. In SAE Technical Paper Series; SAE: Warrendale, PA, USA, 1980. [Google Scholar]
- Pantalé, O.; Gueye, B. Influence of the constitutive flow law in FEM simulation of the radial forming process. J. Eng. 2013, 2013, 231847. [Google Scholar] [CrossRef] [Green Version]
- Slimane, A.; Bouchouicha, B.; Benguediab, M.; Slimane, S.-A. Parametric study of the ductile damage by the Gurson–Tvergaard–Needleman model of structures in carbon steel A48-AP. J. Mater. Res. Technol. 2015, 4, 217–223. [Google Scholar] [CrossRef] [Green Version]
- Bergo, S.; Morin, D.; Hopperstad, O.S. Numerical implementation of a non-local GTN model for explicit FE simulation of ductile damage and fracture. Int. J. Solids Struct. 2021, 219–220, 134–150. [Google Scholar] [CrossRef]
- Cao, T.-S.; Maire, E.; Verdu, C.; Bobadilla, C.; Lasne, P.; Montmitonnet, P.; Bouchard, P.-O. Characterization of ductile damage for a high carbon steel using 3D X-ray micro-tomography and mechanical tests—Application to the identification of a shear modified GTN model. Comput. Mater. Sci. 2014, 84, 175–187. [Google Scholar] [CrossRef]
- Requena, G.; Maire, E.; Leguen, C.; Thuillier, S. Separation of nucleation and growth of voids during tensile deformation of a dual phase steel using synchrotron microtomography. Mater. Sci. Eng. A 2014, 589, 242–251. [Google Scholar] [CrossRef]
- Maire, E.; Bouaziz, O.; Di Michiel, M.; Verdu, C. Initiation and growth of damage in a dual-phase steel observed by X-ray microtomography. Acta Mater. 2008, 56, 4954–4964. [Google Scholar] [CrossRef]
- Chen, D.; Li, Y.; Yang, X.; Jiang, W.; Guan, L. Efficient parameters identification of a modified GTN model of ductile fracture using machine learning. Eng. Fract. Mech. 2021, 245, 107535. [Google Scholar] [CrossRef]
- Saeidi, N.; Ashrafizadeh, F.; Niroumand, B.; Forouzan, M.; Barlat, F. Damage mechanism and modeling of void nucleation process in a ferrite–martensite dual phase steel. Eng. Fract. Mech. 2014, 127, 97–103. [Google Scholar] [CrossRef]
- Morin, L.; Leblond, J.-B.; Kondo, D. A Gurson-type criterion for plastically anisotropic solids containing arbitrary ellipsoidal voids. Int. J. Solids Struct. 2015, 77, 86–101. [Google Scholar] [CrossRef]


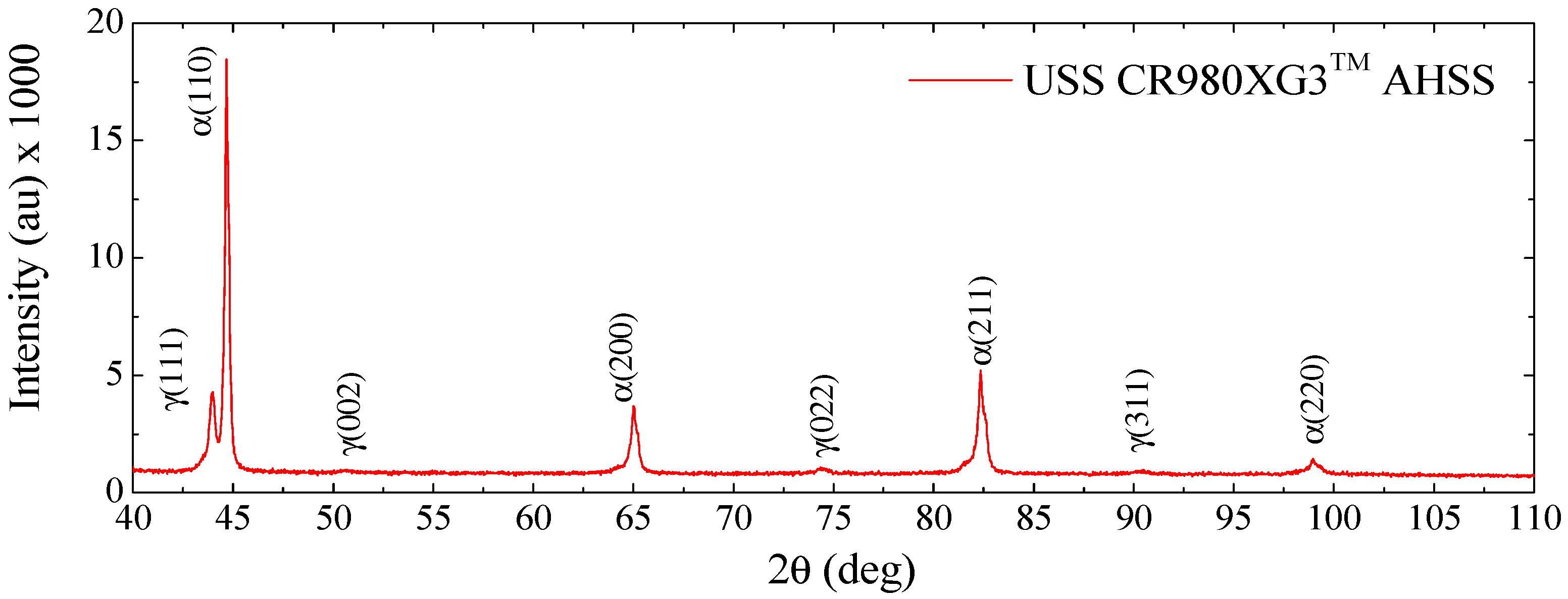




| Phase Index | hkl | 2θ | Rindex | Iindex |
|---|---|---|---|---|
| α | 110 | 44.6 | 233.8 | 6100.6 |
| 200 | 65.0 | 31.9 | 1615.1 | |
| 211 | 82.2 | 60.9 | 2643.9 | |
| 220 | 98.9 | 20.6 | 573.0 | |
| γ | 111 | 43.6 | 182.8 | 2079.5 |
| 200 | 50.8 | 81.6 | 105.8 | |
| 220 | 74.6 | 44.4 | 214.1 | |
| 311 | 90.6 | 51.3 | 159.2 |
| Orientation (θ) | r-Value | ||||
|---|---|---|---|---|---|
| 0° | 604 ± 7 | 1040 ± 9 | 18.0 ± 0.5 | 23.4 ± 0.2 | 0.861 ± 0.003 |
| 45° | 643 ± 4 | 1015 ± 8 | 17.9 ± 0.2 | 23.0 ± 0.6 | 0.957 ± 0.004 |
| 90° | 668 ± 7 | 1023 ± 8 | 16.9 ± 0.6 | 21.9 ± 1.3 | 0.895 ± 0.005 |
| Work-Hardening Equation | (MPa) | R2 | ||||
|---|---|---|---|---|---|---|
| Hollomon | 1723 ± 9 | 0.187 ± 0.002 | - | - | 0.977 | |
| Ludwik | 1569 ± 11 | 0.386 ± 0.009 | 474 ± 11 | - | 0.993 | |
| Swift | 1880 ± 7 | 0.231 ± 0.002 | - | 0.69 ± 0.03 | 0.997 | |
| Voce | 643 ± 1 | 14.914 ± 0.065 | 634 ± 1 | - | 0.999 | |
| (deg) | Uniaxial Tension | Plane Strain | ||
|---|---|---|---|---|
| 0 | −0.1480 ± 0.0070 | 0.3240 ± 0.0200 | 0.0109 ± 0.0026 | 0.1525 ± 0.0062 |
| 90 | −0.1490 ± 0.0110 | 0.3200 ± 0.0280 | 0.0098 ± 0.0016 | 0.1345 ± 0.0234 |
| Elastic Properties | Isotropic Effective Work Hardening (Swift) | |||||
|---|---|---|---|---|---|---|
| (MPa) | ν | (MPa) | A | B | ||
| 192,000 | 0.289 | 1880 | 0.0069 | 0.231 | 0.00762 | 0.00232 |
| 0.99988 | 1.74 | 0.83 | 3.03 | 0.18 | 0.07 | 0.035 |
| Element Deletion | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.99988 | 1.74 | 0.83 | 3.03 | 0.18 | 0.07 | 0.035 | 0.050 | 0.095 | yes |
| Mesh Size: | 1.6 mm | 0.8 mm | 0.4 mm | 0.2 mm |
|---|---|---|---|---|
| ) | 262.2 N | 261.9 N | 260.8 N | 265.4 N |
| Normalized CPU time | 1.0× | 2.6× | 9.4× | 50.7× |
| Normalized Nº of elements | 1.0× | 2.5× | 16.8× | 126.7× |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santos, R.O.; Moreira, L.P.; Butuc, M.C.; Vincze, G.; Pereira, A.B. Damage Analysis of Third-Generation Advanced High-Strength Steel Based on the Gurson–Tvergaard–Needleman (GTN) Model. Metals 2022, 12, 214. https://doi.org/10.3390/met12020214
Santos RO, Moreira LP, Butuc MC, Vincze G, Pereira AB. Damage Analysis of Third-Generation Advanced High-Strength Steel Based on the Gurson–Tvergaard–Needleman (GTN) Model. Metals. 2022; 12(2):214. https://doi.org/10.3390/met12020214
Chicago/Turabian StyleSantos, Rafael O., Luciano P. Moreira, Marilena C. Butuc, Gabriela Vincze, and António B. Pereira. 2022. "Damage Analysis of Third-Generation Advanced High-Strength Steel Based on the Gurson–Tvergaard–Needleman (GTN) Model" Metals 12, no. 2: 214. https://doi.org/10.3390/met12020214
APA StyleSantos, R. O., Moreira, L. P., Butuc, M. C., Vincze, G., & Pereira, A. B. (2022). Damage Analysis of Third-Generation Advanced High-Strength Steel Based on the Gurson–Tvergaard–Needleman (GTN) Model. Metals, 12(2), 214. https://doi.org/10.3390/met12020214








