Strength Calculation and Equal Load-Carrying-Capacity Design of an Undermatched HSLA Lap Joint under Out-of-Plane Bending
Abstract
1. Introduction
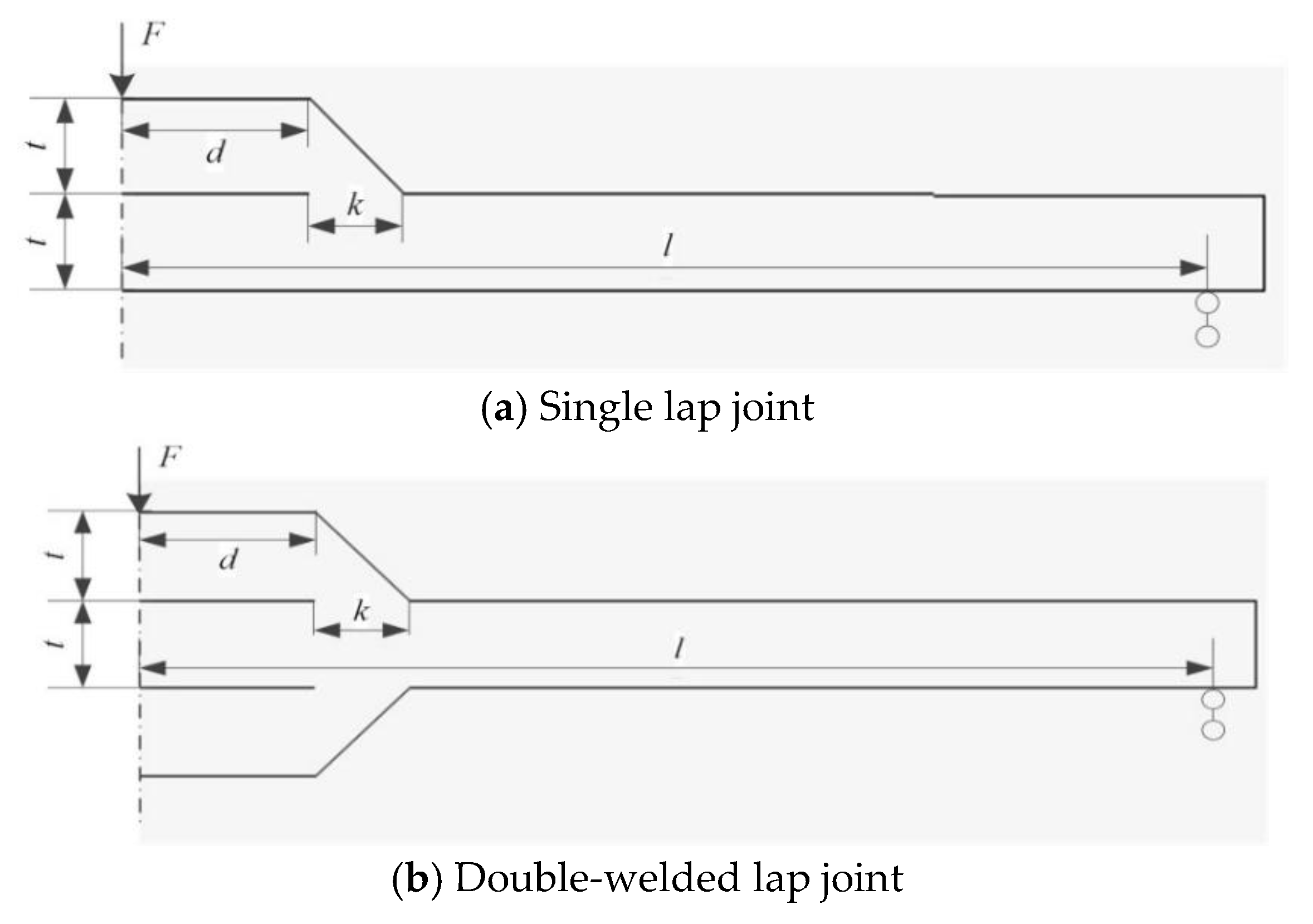
2. Methods
2.1. FE Modeling
2.2. Welding Procedure
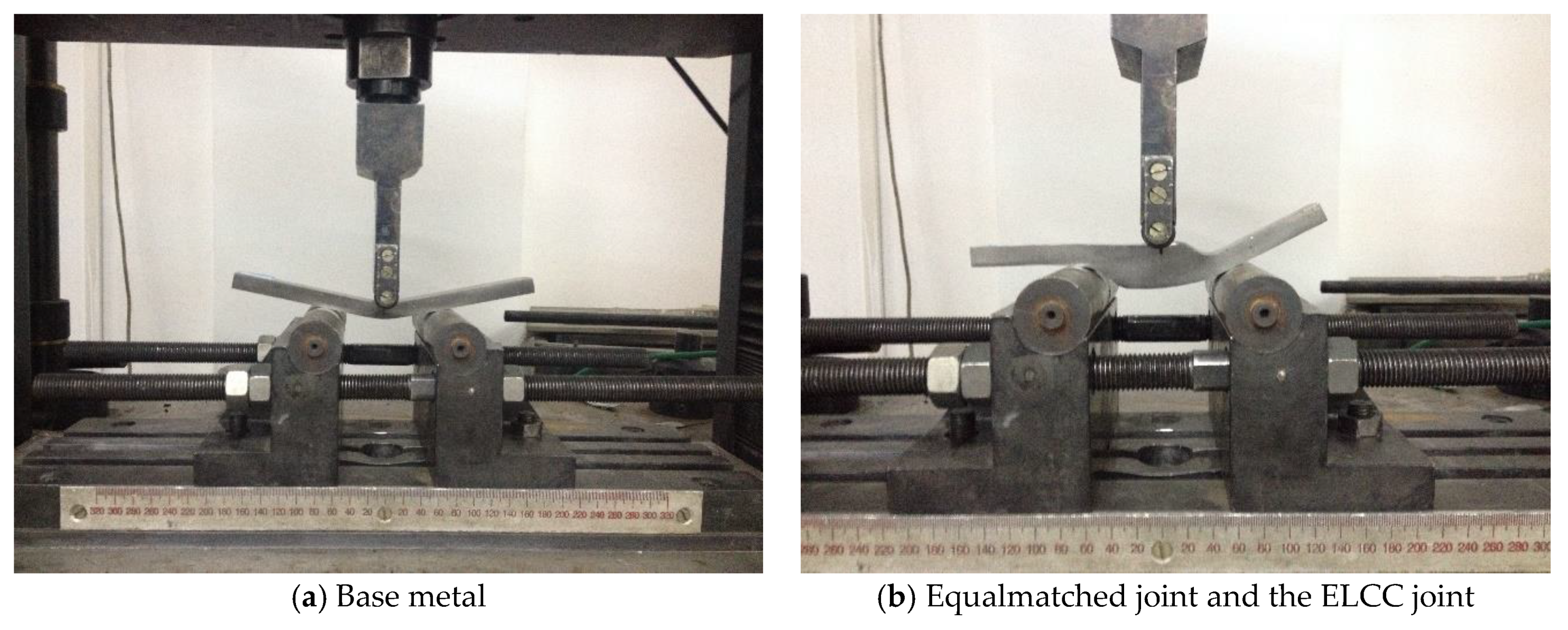
2.3. Experimental Testing
3. Results and Discussion
3.1. Establishing the Strength Calculation Method of a Lap Joint under Out-of-Plane Bending
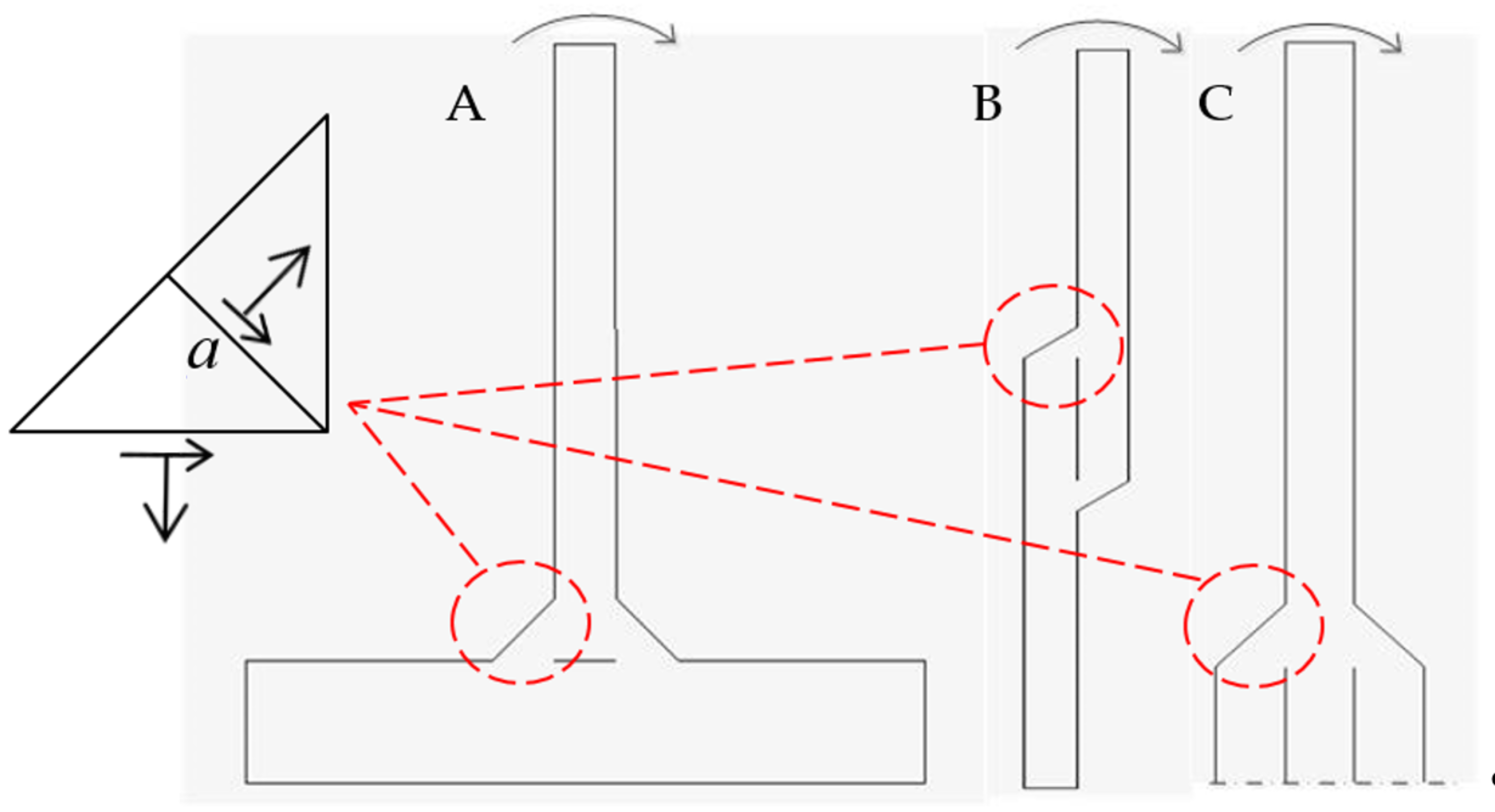
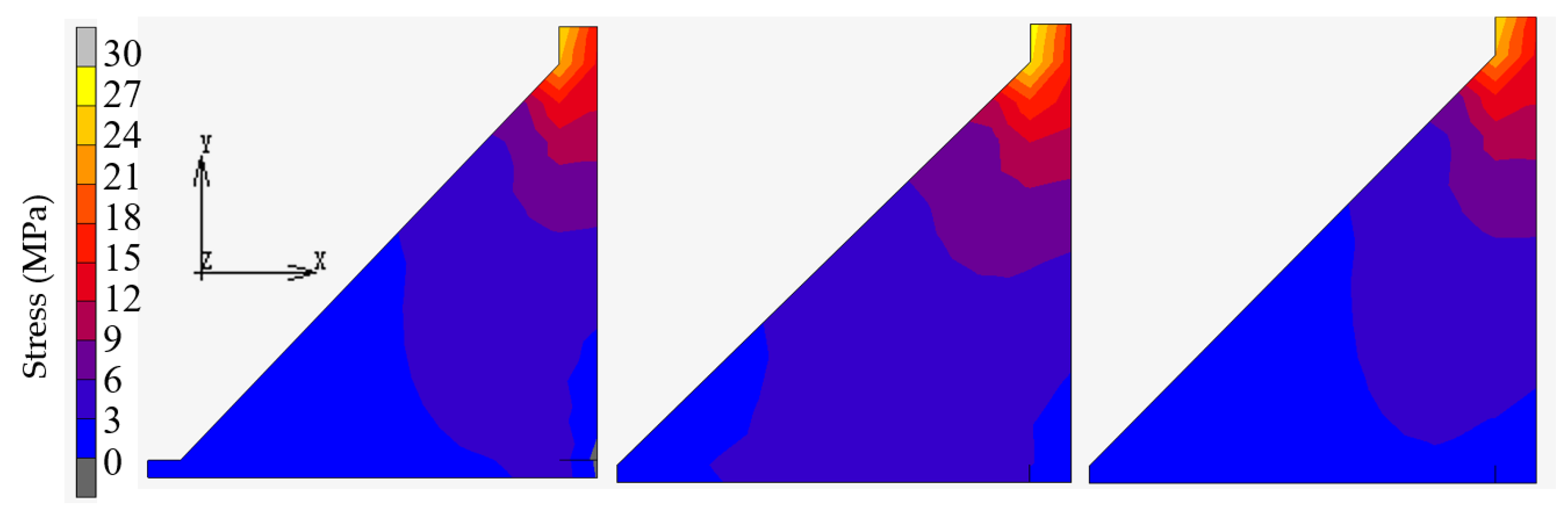
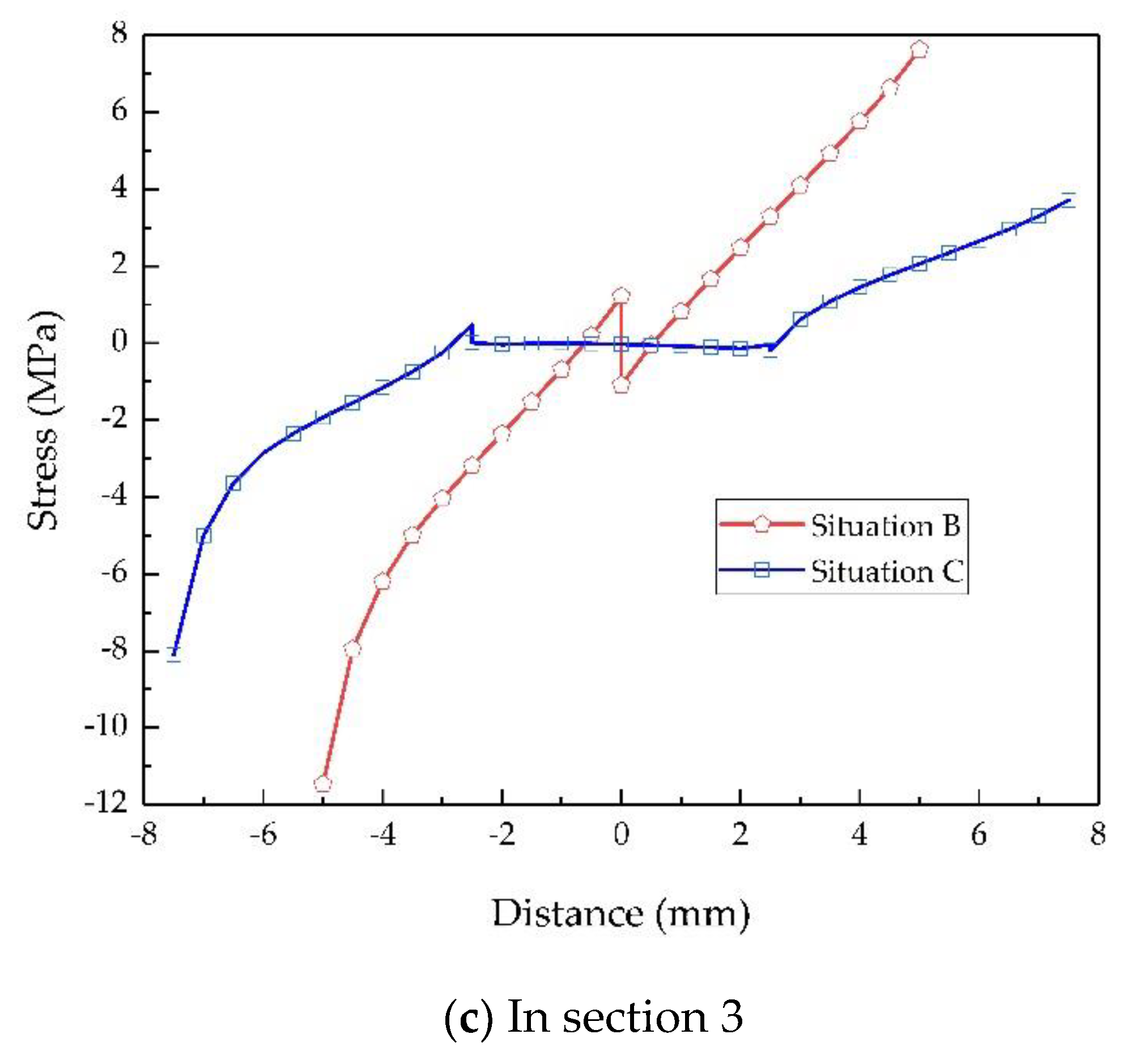
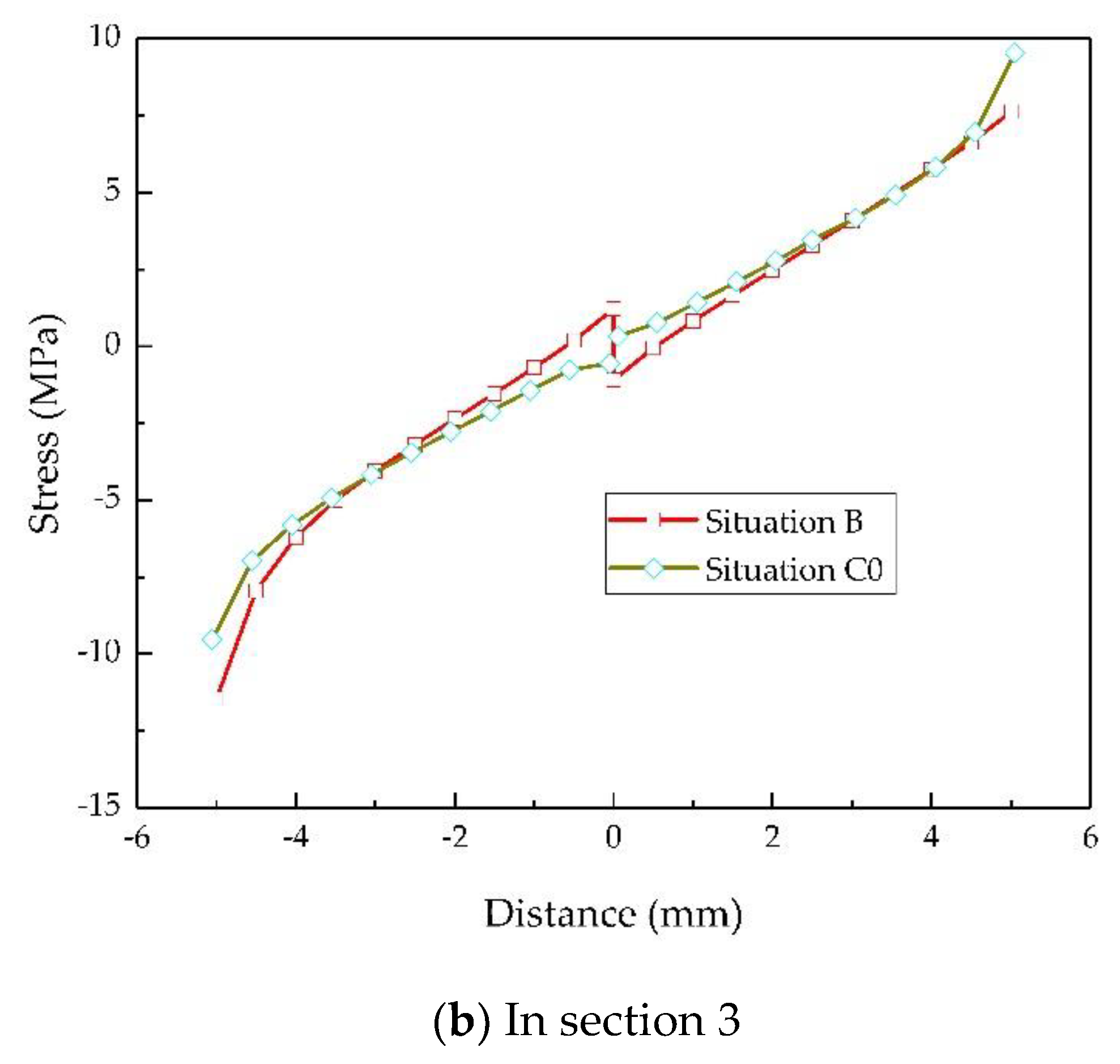
3.1.1. The Mechanical Similarity Shown by FE Results
3.1.2. The Strength Calculation Method
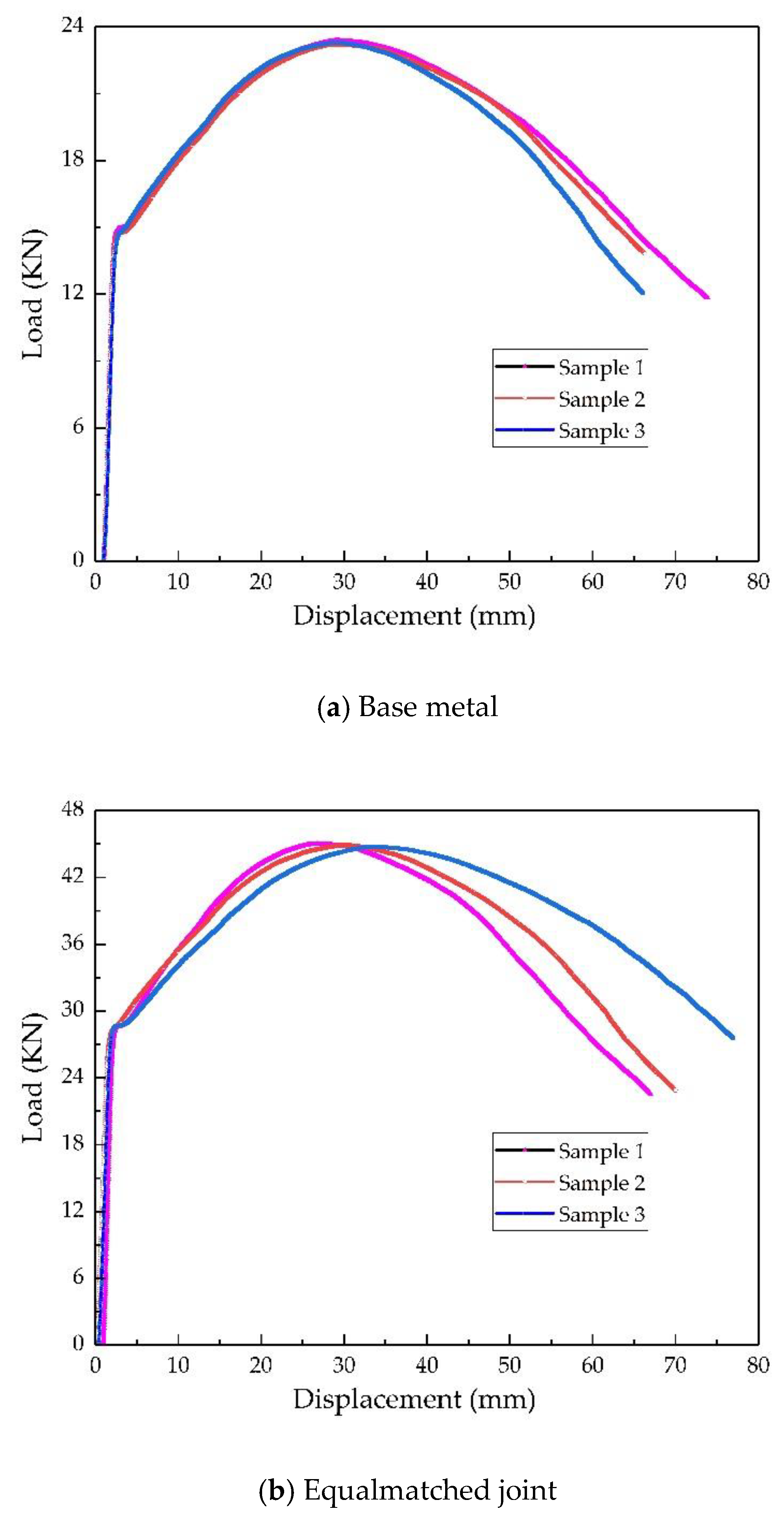
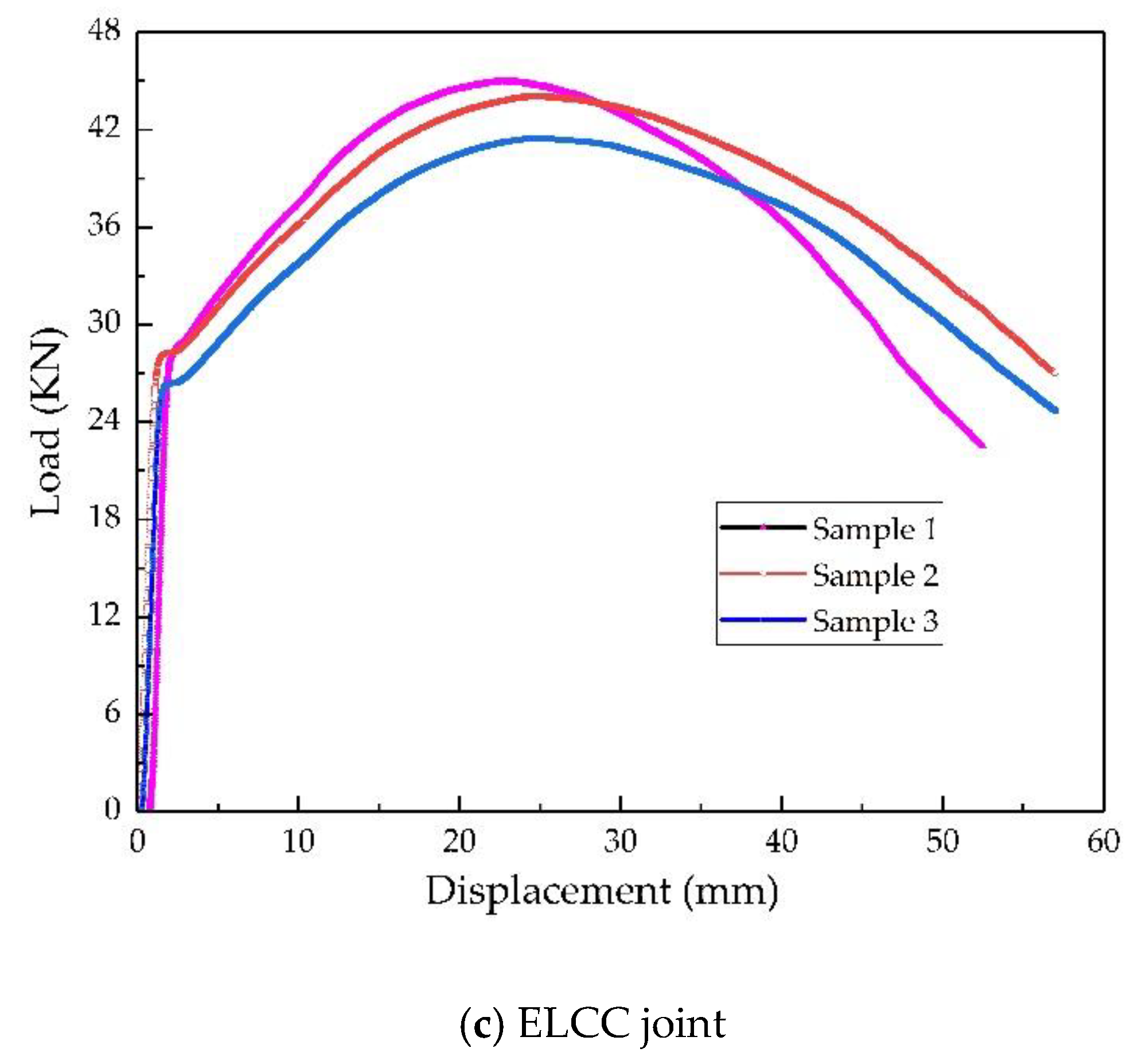
3.2. Experimental Results
3.3. Application in the Prediction of Failure and the LCC of a Lap Joint
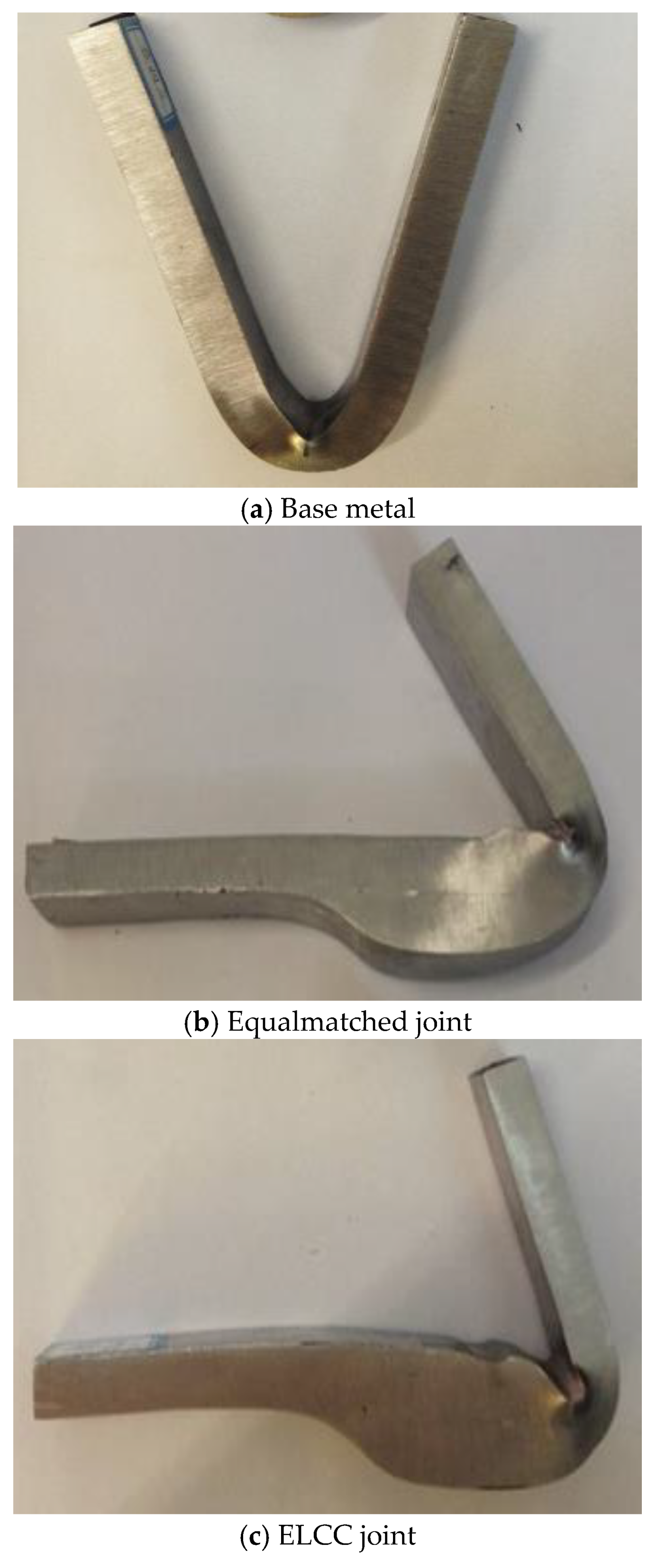
3.4. Application in the ELCC Design of an Undermatched Lap Joint
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gao, B.; Lai, Q.; Cao, Y.; Hu, R.; Xiao, L.; Pan, Z.; Liang, N.; Li, Y.; Sha, G.; Liu, M.; et al. Ultrastrong low-carbon nanosteel produced by heterostructure and interstitial mediated warm rolling. Sci. Adv. 2020, 6. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.W.; Jiang, Z.Y. Thermomechanical processing of advanced high strength steels. Prog. Mater. Sci. 2018, 94, 174–242. [Google Scholar] [CrossRef]
- Ding, R.; Yao, Y.J.; Sun, B.H.; Liu, G.; He, J.G.; Li, T.; Wan, X.H.; Dai, Z.B.; Ponge, D.; Raabe, D.; et al. Chemical boundary engineering: A new route toward lean, ultrastrong yet ductile steels. Sci. Adv. 2020, 6. [Google Scholar] [CrossRef]
- Ran, M.M.; Sun, F.F.; Li, G.Q.; Wang, Y.B. Mechanical properties of mismatched high strength steel butt joints with three softened/hardened strength distribution patterns. Thin Wall. Struct. 2020, 146. [Google Scholar] [CrossRef]
- Shi, R.; Ma, Y.; Wang, Z.; Gao, L.; Yang, X.-S.; Qiao, L.; Pang, X. Atomic-scale investigation of deep hydrogen trapping in NbC/α-Fe semi-coherent interfaces. Acta Mater. 2020, 200, 686–698. [Google Scholar] [CrossRef]
- Breen, A.J.; Stephenson, L.T.; Sun, B.H.; Li, Y.J.; Kasian, O.; Raabe, D.; Herbig, M.; Gault, B. Solute hydrogen and deuterium observed at the near atomic scale in high-strength steel. Acta Mater. 2020, 188, 108–120. [Google Scholar] [CrossRef]
- Robertson, I.M.; Sofronis, P.; Nagao, A.; Martin, M.L.; Wang, S.; Gross, D.W.; Nygren, K.E. Hydrogen Embrittlement Understood. Metall. Mater. Trans. A 2015, 46, 2323–2341. [Google Scholar] [CrossRef]
- Świerczyńska, A.; Landowski, M. Plasticity of Bead-on-Plate Welds Made with the Use of Stored Flux-Cored Wires for Offshore Applications. Materials 2020, 13, 3888. [Google Scholar] [CrossRef]
- Tomków, J.; Fydrych, D.; Rogalski, G. Role of Bead Sequence in Underwater Welding. Materials 2019, 12, 3372. [Google Scholar] [CrossRef]
- Gáspár, M.; Sisodia, R. Improving the HAZ toughness of Q plus T high strength steels by post weld heat treatment. Iop Conf. Ser. Mater. Sci. 2018, 426. [Google Scholar] [CrossRef]
- Loureiro, A.J.R. Effect of heat input on plastic deformation of undermatched welds. J. Mater. Process. Tech. 2002, 128, 240–249. [Google Scholar] [CrossRef]
- Heinz, D.; Richter, B.; Weber, S. Application of advanced materials for ship construction—Experiences and problems. Mater. Corros. 2000, 51, 407–412. [Google Scholar] [CrossRef]
- Magudeeswaran, G.; Balasubramanian, V.; Reddy, G.M. Effect of welding processes and consumables on high cycle fatigue life of high strength, quenched and tempered steel joints. Mater. Des. 2008, 29, 1821–1827. [Google Scholar] [CrossRef]
- Hao, S.; Schwalbe, K.H.; Cornec, A. The effect of yield strength mis-match on the fracture analysis of welded joints: Slip-line field solutions for pure bending. Int. J. Solids Struct. 2000, 37, 5385–5411. [Google Scholar] [CrossRef]
- Tuma, J.V.; Sedmak, A. Analysis of the unstable fracture behaviour of a high strength low alloy steel weldment. Eng. Fract. Mech. 2004, 71, 1435–1451. [Google Scholar] [CrossRef]
- Lukács, J.; Dobosy, A. Matching effect on fatigue crack growth behaviour of high-strength steels GMA welded joints. Weld. World 2019, 63, 1315–1327. [Google Scholar] [CrossRef]
- Peter, C.; Mikael, M.; Mattias, N.; Svante, T. Undermatching Butt Welds in High Strength Steel, IABSE annual meeting and symposium. In Proceedings of the IABSE Symposium, Bangkok, Thailand, 9–11 September 2009. [Google Scholar]
- Rodrigues, D.M.; Menezes, L.F.; Loureiro, A. Modelling the effect of HAZ undermatching on the crack-tip stress distribution in idealized welds. Int. J. Mech.Sci. 2004, 46, 1481–1488. [Google Scholar] [CrossRef][Green Version]
- Baud, R.V. Fillet profiles for constant stress. Prod. Eng. 1934, 5, 133–134. [Google Scholar]
- Neuber, H. The flat bar subjected to tensile loading with an optimum transition in section. Forsch Ing. 1969, 35, 29–30. [Google Scholar] [CrossRef]
- Schnack, E.; Weikl, W. Shape optimization under fatigue using continuum damage mechanics. Comput. Aided Des. 2002, 34, 929–938. [Google Scholar] [CrossRef]
- Mattheck, C. Teacher tree: The evolution of notch shape optimization from complex to simple. Eng. Fract. Mech. 2006, 73, 1732–1742. [Google Scholar] [CrossRef]
- Baptista, R.; Infante, V.; Branco, C.M. Study of the fatigue behavior in welded joints of stainless steels treated by weld toe grinding and subjected to salt water corrosion. Int. J. Fatigue 2008, 30, 453–462. [Google Scholar] [CrossRef]
- Yi, H.J.; Lee, Y.J.; Lee, K.O. TIG Dressing Effects on Weld Pores and Pore Cracking of Titanium Weldments. Metals 2016, 6, 243. [Google Scholar] [CrossRef]
- Mettanen, H.; Nykanen, T.; Skriko, T.; Ahola, A.; Bjork, T. Fatigue strength assessment of TIG-dressed ultra-high-strength steel fillet weld joints using the 4R method. Int. J. Fatigue 2020, 139. [Google Scholar] [CrossRef]
- Yaowu, S. Welding Structure, 3rd ed.; China Machine Press: Beijing, China, 2008; Volume 3. [Google Scholar]
- Wen, X.; Wang, P.; Dong, Z.B.; Liu, Y.; Fang, H.Y. Nominal Stress-Based Equal-Fatigue-Bearing-Capacity Design of under-matched HSLA Steel Butt-welded Joints. Metals 2018, 8, 880. [Google Scholar] [CrossRef]
- Wen, X.; Wang, P.; Dong, Z.B.; Fang, H.Y. A Fracture Mechanics-Based Optimal Fatigue Design Method of Under-Matched HSLA Steel Butt-Welded Joints with Imperfections. Appl. Sci. Basel 2019, 9, 3609. [Google Scholar] [CrossRef]
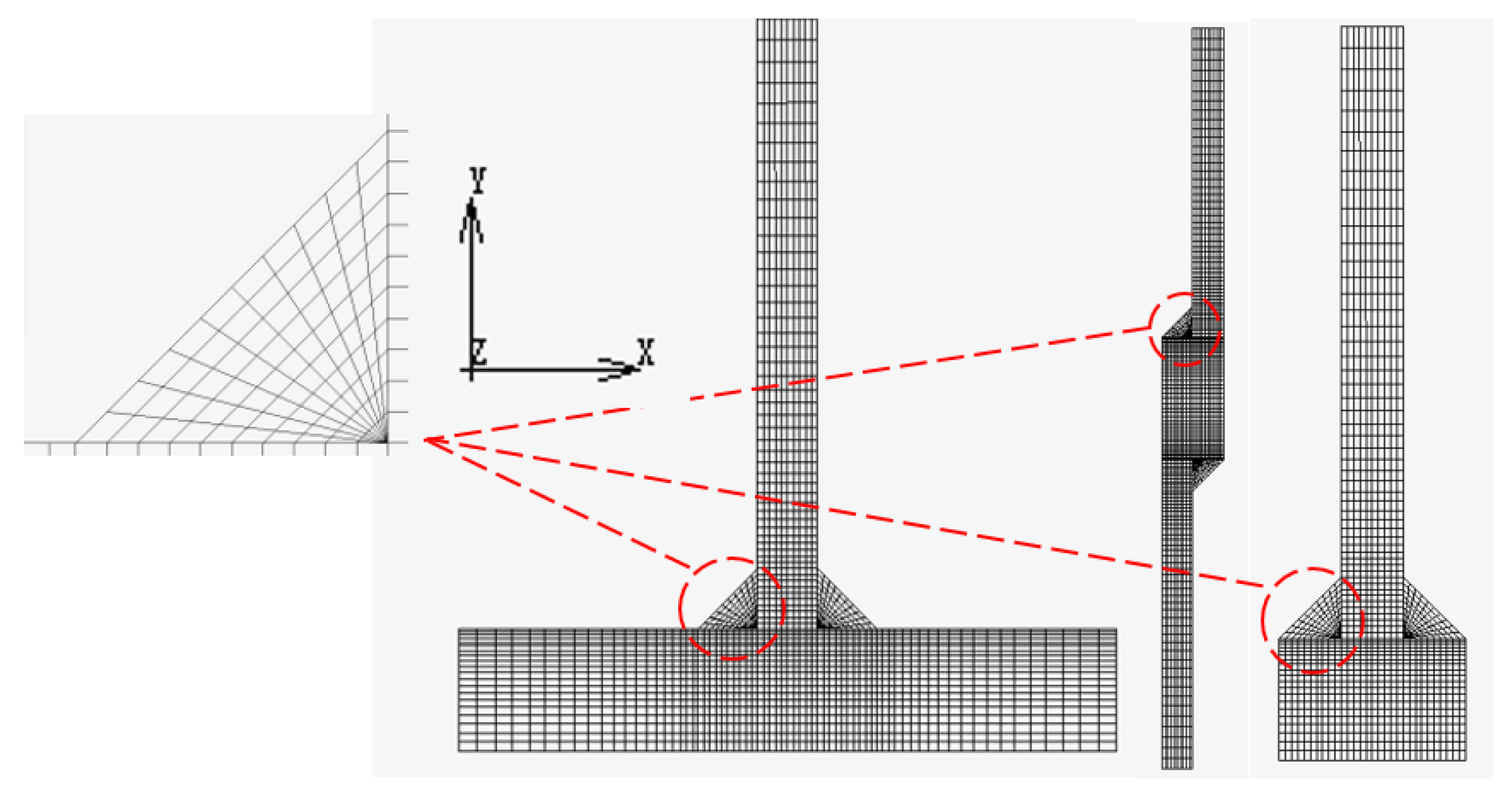
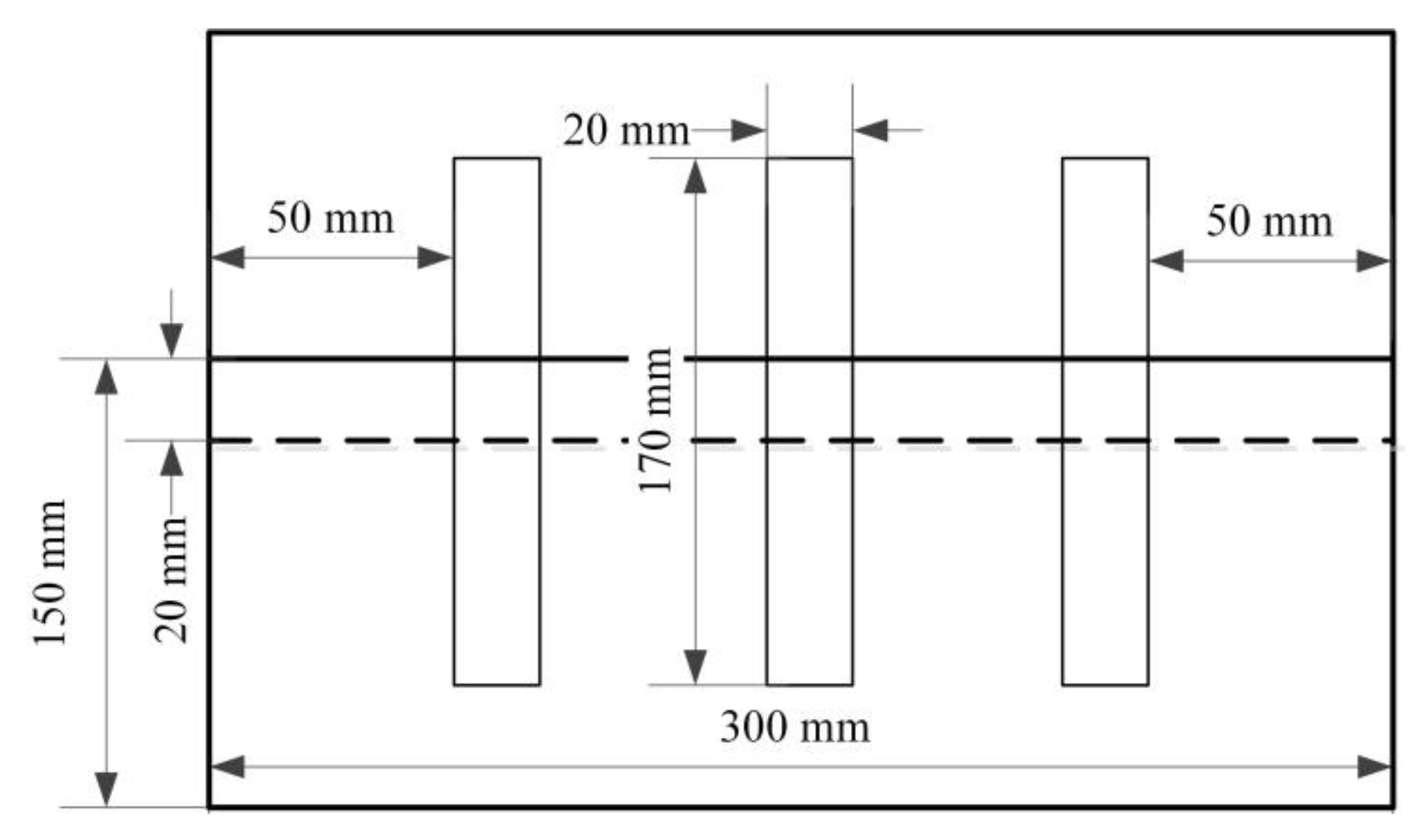
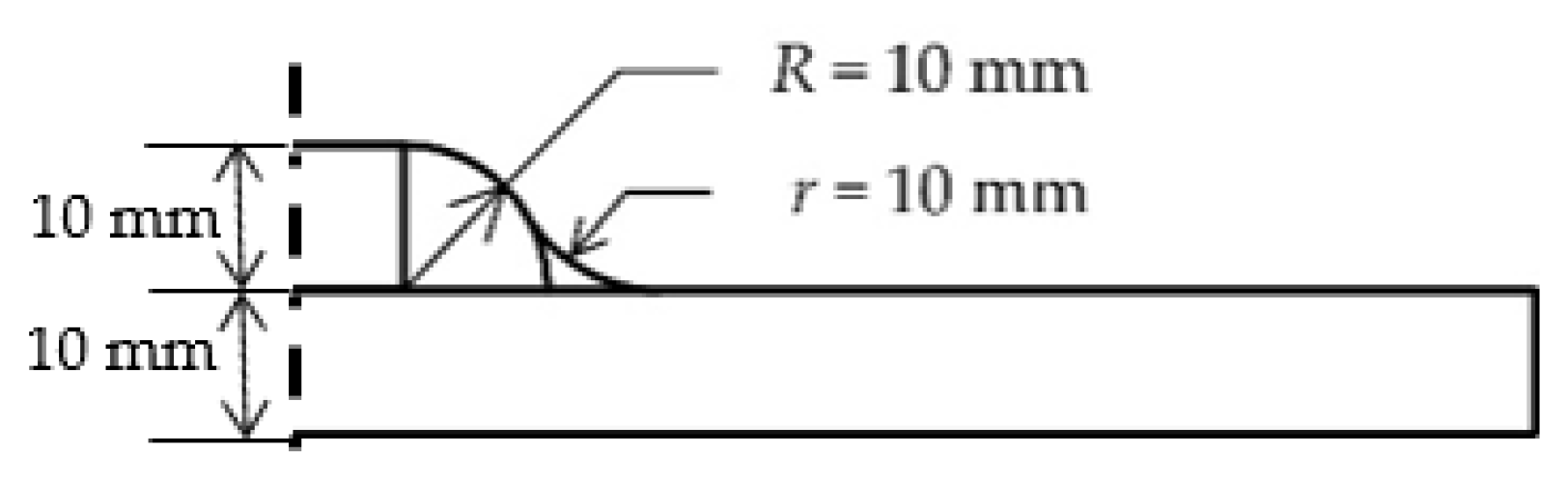
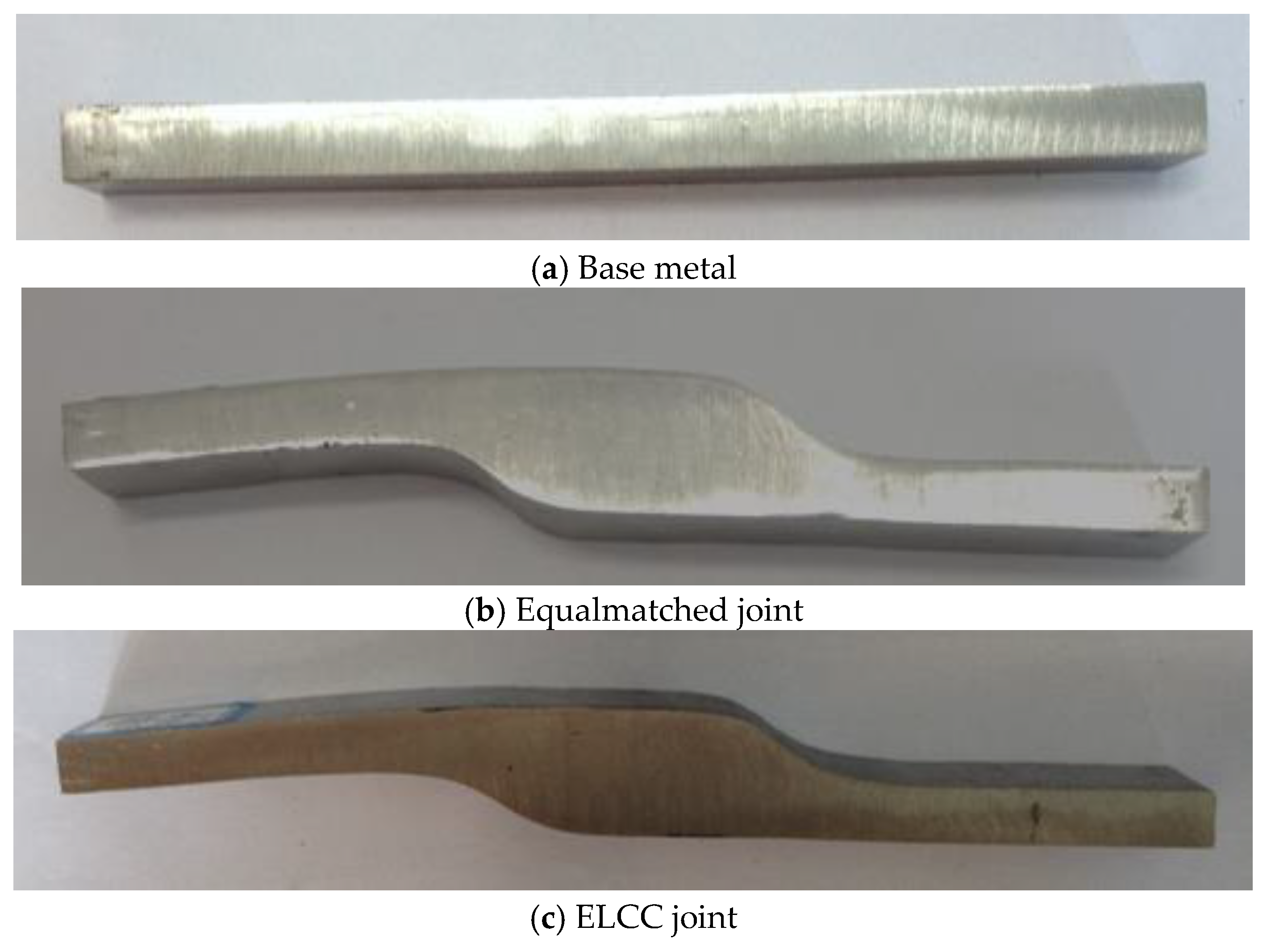



| Materials | C | Si | Mn | Cr | Mo | Ni | Tensile Strength σu (MPa) | Yield Strength σy (MPa) | Elongation Rate A (%) |
|---|---|---|---|---|---|---|---|---|---|
| HQ785 | 0.10 | 0.20 | 1.41 | 0.56 | 0.33 | - | 840 | 790 | 18 |
| ER110S-G | 0.09 | 0.55 | 1.68 | - | 0.43 | 1.02 | 835 | 760 | 20 |
| ER70S-6 | 0.10 | 0.90 | 1.65 | - | - | - | 560 | 405 | 32 |
| Joint Type | Welding Process | Current Type/Polarity | Welding Current (A) | Arc Voltage (V) | Welding Speed (mm/s) | Shielding Gas/Flow Rate (L/min) | Preheating Temperature (°C) |
|---|---|---|---|---|---|---|---|
| Equalmatched | MAG | DC/− | 270 | 30 | 5.5 | CO2/5 Ar/18 | 80 ± 5 |
| Undermatched | MIG | DC/− | 200 | 19 | 2 | Ar/13 | - |
| Sample Type | Base Metal | Equalmatched Joint | ELCC Joint | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Sample No. | 1 | 2 | 3 | 1 | 2 | 3 | 1 | 2 | 3 |
| F (kN) | 23.38 | 23.22 | 23.29 | 45.02 | 44.88 | 44.77 | 44.96 | 44.06 | 41.48 |
| Average of F (kN) | 23.30 | 44.89 | 43.50 | ||||||
| Bending Angle (°) | 125 | 121 | 108 | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, J.; Dong, Z.; Fang, H.; Wang, J. Strength Calculation and Equal Load-Carrying-Capacity Design of an Undermatched HSLA Lap Joint under Out-of-Plane Bending. Metals 2021, 11, 161. https://doi.org/10.3390/met11010161
Guo J, Dong Z, Fang H, Wang J. Strength Calculation and Equal Load-Carrying-Capacity Design of an Undermatched HSLA Lap Joint under Out-of-Plane Bending. Metals. 2021; 11(1):161. https://doi.org/10.3390/met11010161
Chicago/Turabian StyleGuo, Junli, Zhibo Dong, Hongyuan Fang, and Jiajie Wang. 2021. "Strength Calculation and Equal Load-Carrying-Capacity Design of an Undermatched HSLA Lap Joint under Out-of-Plane Bending" Metals 11, no. 1: 161. https://doi.org/10.3390/met11010161
APA StyleGuo, J., Dong, Z., Fang, H., & Wang, J. (2021). Strength Calculation and Equal Load-Carrying-Capacity Design of an Undermatched HSLA Lap Joint under Out-of-Plane Bending. Metals, 11(1), 161. https://doi.org/10.3390/met11010161






