Segregation of P and S Impurities to A Σ9 Grain Boundary in Cu

Abstract

The segregation of P and S to grain boundaries (GBs) in fcc Cu has implications in diverse physical-chemical properties of the material and this can be of particular high relevance when the material is employed in high performance applications. Here, we studied the segregation of P and S to the symmetric tilt Σ9 ($2\overline{2}\overline{1}$) [110], 38.9° GB of fcc Cu. This GB is characterized by a variety of segregation sites within and near the GB plane, with considerable differences in both atomic site volume and coordination number and geometry. We found that the segregation energies of P and S vary considerably both with distance from the GB plane and sites within the GB plane. The segregation energy is significantly large at the GB plane but drops to almost zero at a distance of only ≈3.5 Å from this. Additionally, for each impurity there are considerable variations in energy (up to 0.6 eV) between segregation sites in the GB plane. These variations have origins both in differences in coordination number and atomic site volume with the effect of coordination number dominating. For sites with the same coordination number, up to a certain atomic site volume, a larger atomic site volume leads to a stronger segregation. After that limit in volume has been reached, a larger volume leads to weaker segregation. The fact that the segregation energy varies with such magnitude within the Σ9 GB plane may have implications in the accumulation of these impurities at these GBs in the material. Because of this, atomic-scale variations of concentration of P and S are expected to occur at the Σ9 GB center and in other GBs with similar features.

1. Introduction

The binding energy of substitutional and interstitial impurities to grain boundaries (GBs) is highly dependent of the local structure of the GBs [1,2]. This is because at many GB the local atomic environment differs considerably when compared to the bulk [3]. These features have an important direct impact on several local properties of the material at the GBs, such as segregation of impurities, defect mobility and grain boundary sink efficiency [1,4,5]. For some materials, such local GB features can affect the microcrystallinity [6] and other global properties, such as creep [7], plasticity, strength and can also affect hydrogen embrittlement and stress corrosion cracking mechanisms [8,9,10]. These phenomena have additional relevance when the material is used in high performance applications, such as in long-term repositories for spent nuclear fuel.

For a specific GB, the local atomic environment can also vary considerably. This happens both within the GB plane and along the direction perpendicular to the GB plane. We have shown previously that for fcc Cu the Σ5 and Σ11 GBs can increase the strength of the segregation of substitutional impurities at certain atomic sites while the Σ3 GB has no ability to trigger the segregation of impurities [7]. The dominating effect is the volume expansion at certain GB sites of the Σ5 and Σ11 and because the Σ3 has no sites with considerable volume expansion, the segregation of impurities from the bulk is not favorable [1,7].

For oxygen free Cu (Cu-OF) it has been shown that the presence of phosphorus improves the creep properties at near ambient temperatures [11,12]. The underlying mechanisms involve the formation of cavities and the presence of P is thought to largely influence those mechanisms [13,14]. Previous studies suggested that the presence of S could also affect those mechanisms but a recent study did not find convincing correlations between the amounts of S present in the GBs and the creep ductility of Cu [13]. In previous studies it was also found that S segregates at Cu GBs [2,15], and the effects of the presence of S on the mechanisms of segregation of P at those GBs are currently not understood. Because these two impurities share many similarities in terms of segregation mechanisms and energies [7], it is important to understand their tendencies for segregation at GBs in Cu in order to have a more detailed picture of the local GB chemistry, because this can affect the global, macroscopic properties of the material. Our previous investigations on the segregation of P and S at low index symmetric tilt GBs of Cu—Σ3, Σ5 and Σ11—have shown a large variation in the segregation energies (ΔE_seg) for these elements both as a function of GB type and as a function of the distance from the GB plane [7]. However, the Σ3, Σ5 and Σ11 GBs have as a common feature, the fact that within their GB planes, the coordination, geometry and local volume for copper atoms and interstitial sites is fairly homogeneous and considerable variations in these features occur only as a function of distance from the GB plane [1]. In order to understand if the ΔE_seg can also vary considerably within GB planes it is important to study other GBs with a more diverse local atomic environment within GB plane. The Σ9 GB has a variety of atomic positions within the GB plane where half of the GB resembles the Σ3 and the remaining half is dominated by a cavity with a larger volume expansion than the Σ5 [16]. Because the Σ9 GB can occur with considerable frequency in Cu [16,17,18], the goal of this study is to investigate the segregation of P and S to the Σ9 GB with density functional theory (DFT) calculations and compare how it differs from the previously studied GBs. We found large variations in ΔE_seg obtained for Σ9 for both P and S within the GB plane, which is a unique feature among the Σ9, Σ3, Σ5 and Σ11 GBs. Similarly to the other GBs previously studied, ΔE_seg varies significantly with distance from the GB plane. Overall, we found that for the Σ9 GB, the segregation tendency for the substitutional impurities P and S has larger variations than for the Σ3, Σ5 and Σ11 GBs. This happens both within the GB plane and as a function of distance from the GB plane.

2. Materials and Methods

Density functional theory (DFT) calculations of the segregation or absorption of S and P to the Σ9 GB of fcc Cu were performed with the Vienna ab initio simulation package (VASP 5.4.1) [19] with the Perdew–Burke–Ernzerhof [20] (PBE) exchange–correlation functional with pseudopotentials of the projector augmented wave [21,22] (PAW) type. The PBE functional showed good accuracy for describing the interactions between p-block elements with the bulk and surfaces of Cu [2,23,24,25,26]. For all calculations, a plane wave cutoff of 460 eV and a k-point mesh of (7 × 9 × 3) in the Monkhorst–Pack sampling scheme were employed [27]. The energies herein reported are electronic energies at 0 K which allow accurate comparisons between binding energies at sites with similar chemical environment in the solid [28,29,30]. The segregation energy (ΔE_seg) of an element X at a GB site is defined as

$$\mathsf{\Delta }{E}_{\mathrm{seg}}=E_{\left(X\_\mathrm{GB}\right)}- E_{\left(X\_\mathrm{Bulk}\right)}$$

(1)

where E_{(nX_GB)} is the energy of a supercell of Cu containing a GB model with the dopant X placed in a substitutional site at the GB plane or in its close-vicinity, and E_{(nX_Bulk)} is the reference energy of the same supercell with the dopant placed far from the GB plane at a bulk site. A more negative value for ΔE_seg implies stronger bonding and a value equal to zero implies no preference for bonding to the respective site compared to the bulk. The GB energy is here defined as

γ_GB = (E_GB – E_bulk)/2A

(2)

where the electronic energies are: E_GB, the energy of the supercell that contains the GB; E_bulk, the total energy of a supercell of Cu single crystal that contains the same number of atoms as those present in the supercell used for modelling the GB and A, area of the GB in the supercell. The quantity is divided by two to account for the fact that there are two identical GBs in the supercell. Lower values of γ_GB imply stronger cohesive bonding between the two grains. The GB model Σ9 ($2\overline{2}\overline{1}$) [110], 38.9° consisting of a supercell with dimensions: x = 7.67 Å, y = 5.11 Å and z = 21.13 Å is shown in Figure 1.

We have chosen this GB because it occurs frequently in fcc Cu [16,17,18] and it has a geometry with a diverse openness due to sites with different coordination numbers and local volume at the GB plane. In a previous work we have shown that the volume expansion is a key parameter for driving the segregation of dopants to GBs [1,7]. The GB model of Figure 1 was constructed using the coincidence-site lattice (CSL) method and a periodic supercell that contains two oppositely oriented Σ9 GBs. The CSL allows the accurate construction of periodic GB models of single crystals for GBs that have a high density of coincidence sites at the GB plane [31,32]. After the construction of the model with the CLS method, a first optimization of the GB supercell was performed. In this optimization step the supercell containing the GB was relaxed along the [2$\overline{2}\overline{1}]$ direction. After this optimization was completed, an optimization of the internal coordinates of all atoms was carried out. Additional optimizations of the supercell and of the internal coordinates were iteratively carried out until the optimized geometry shown in Figure 1 was found. The self-consistent field (SCF) electronic energies were considered converged when their change between two cycles was smaller than 1 × 10⁻⁵ eV and the forces acting on each of the atoms smaller than 0.002 eV∙Å⁻¹ for the ionic relaxations and additionally zero Pulay stress for the supercell optimization. For the determination of the segregation energies, the internal coordinates of all atoms of the supercell were optimized. The GB model employed, shown in Figure 1, has been optimized and then benchmarked against a supercell of single crystal fcc Cu. In the GB model, the distance from the GB plane to a parallel plane where we consider that the binding energy of a dopant is equal to that of bulk Cu was validated by comparison with the case where the dopant is at a substitutional site in a supercell of single crystal fcc Cu with symmetry (3 × 3 × 3) containing 108 Cu-atoms. The difference between both values is in the order of 0.003 eV. This value is considered as the limit of error of the computational method.

The coordination numbers and atomic site volumes were determined using Voronoi tessellation of the supercell. The volume expansion (%) of a given atomic site is here defined relative to the volume at the bulk fcc site as

$$V_e= \frac{\left(V_s- V_b\right)\times 100}{V_b}$$

(3)

where V_e is the volume expansion (%), V_s is the volume of the atomic site s and V_b is the volume at the bulk fcc site.

3. Results and Discussion

The obtained GB energy for the Σ9 GB model shown in Figure 1 is 689 mJ/m² which is in good agreement with the literature data: 1200 mJ/m², 859 mJ/m² and 850 mJ/m² [16,33,34]. The study of the segregation of P and S as substitutional impurities was done by sequentially placing one dopant at a time on the sites labelled in Figure 1 to replace the corresponding Cu atom. The obtained ΔE_seg are given in

Table 1. Segregation energies ΔE_seg (eV) for P and S for the atomic sites labelled in Figure 1, the respective distances (d) from the GB plane, coordination number of the site and volume expansion relative to the fcc single crystal (%).

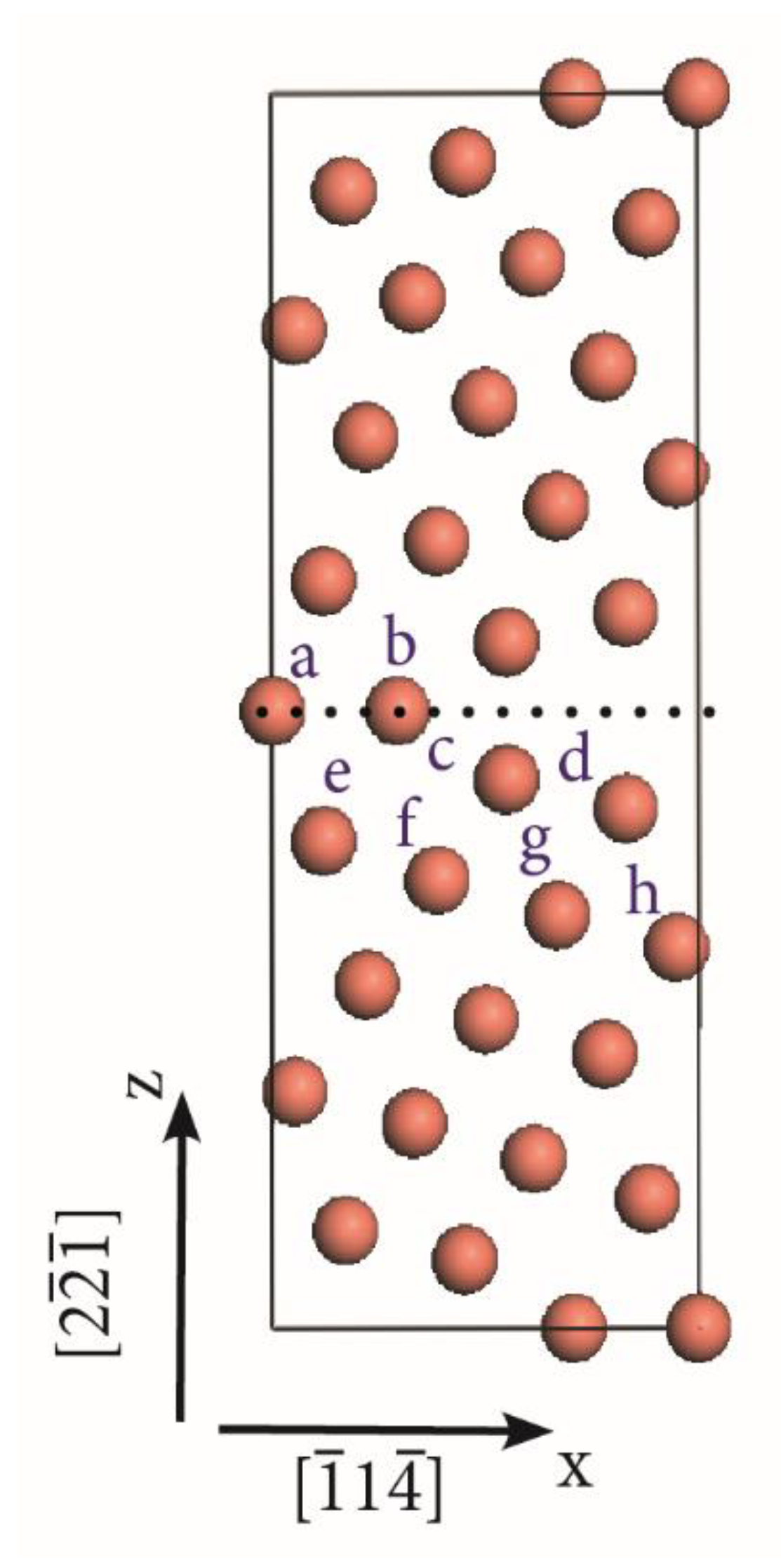
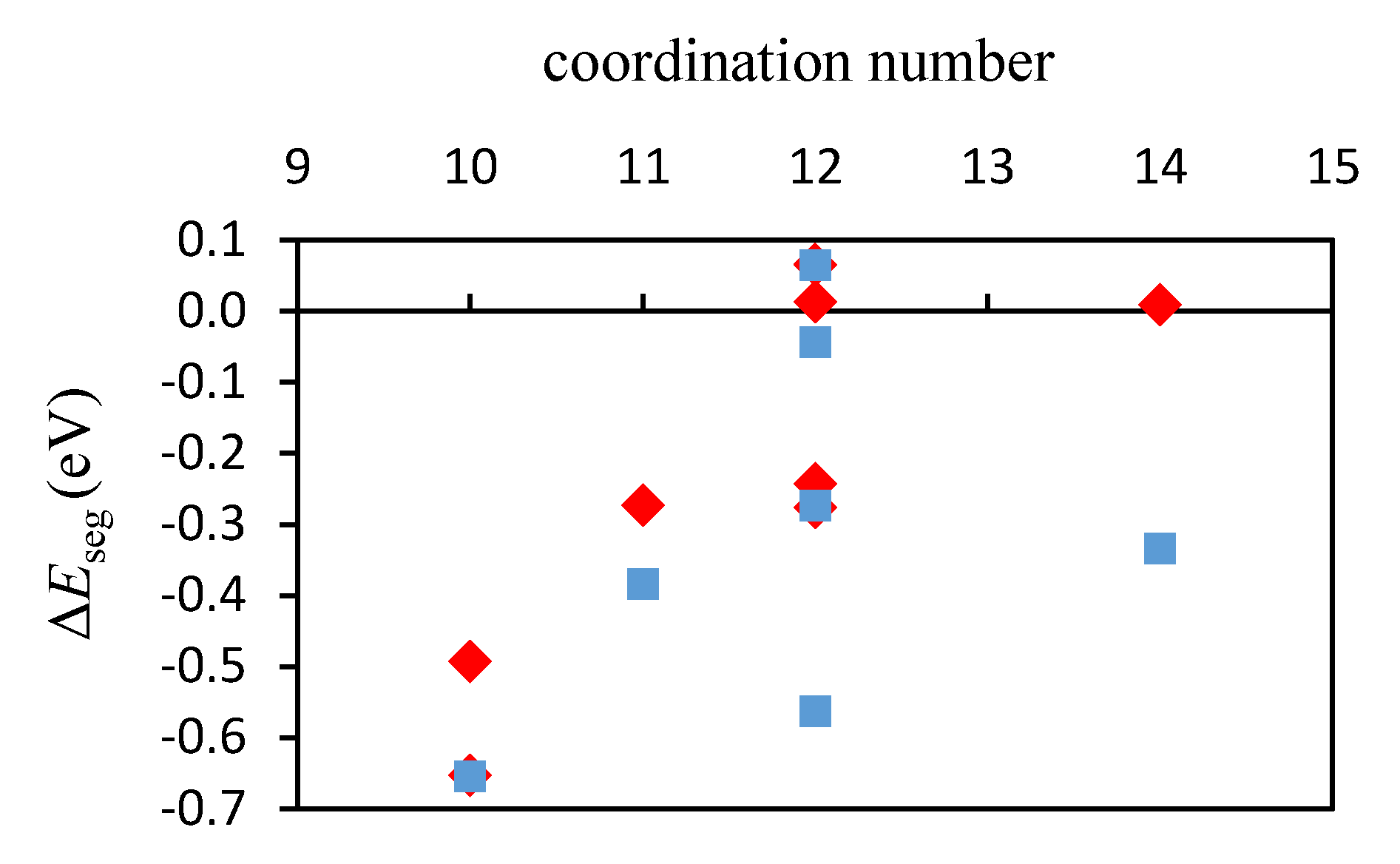
Atomic Position	Impurity		z Coordinate (fractional)	z Coordinate (Å)	d (Å)	Coordination Number	Volume Expansion (%)
	P (ΔEseg)	S (ΔEseg)
a	−0.653	−0.724	0.500	10.853	0.000	10	2.3
b	0.009	−0.335	0.500	10.853	0.000	14	14.0
c	−0.493	−0.655	0.443	9.675	1.248	10	6.7
d	−0.274	−0.384	0.421	9.167	1.716	11	6.6
e	−0.276	−0.562	0.392	8.636	2.360	12	5.5
f	−0.243	−0.274	0.363	7.956	2.972	12	0.5
g	0.065	0.000	0.340	7.343	3.488	12	−0.9
h	0.013	0.000	0.315	6.808	4.015	12	0.8

and the plot of the ΔE_seg as a function of the distance from the GB plane for both P and S are shown in Figure 2.

The ΔE_seg vary significantly as a function of the distance from the GB center due to changes in coordination number and volume expansion, as will be shown below. This is in line with previous findings for Σ3, Σ5 and Σ11 GBs [7]. From these three GBs, the Σ5 is that where P and S have the largest ΔE_seg and additionally, from these three GBs the change in ΔE_seg as a function of d is largest for Σ5. For the current case of the Σ9 GB, it can be seen however that the dependency of ΔE_seg on d is even stronger than what was previously found for Σ5 [7]. While for the Σ9 the ΔE_seg is of considerable magnitude in the GB plane for sites a and c, it can be seen that already at a distance of only ≈3.5 Å there is no driving force for segregation because ΔE_seg = 0. Additionally, a unique feature of the Σ9 GB is that at the GB plane there is a considerable difference in ΔE_seg, as shown by the values obtained for the sites a and b.

The difference between ΔE_seg for sites a and b is of around 0.6 eV for P and of around 0.4 eV for S, although both sites lay in the GB plane. This has not been previously observed for the Σ3, Σ5 and Σ11 GBs. As can be seen in the data of Table 1, site b has a considerably larger volume expansion than site a, simultaneously the coordination number for site b is of 14 when compared to a coordination number of 10 for site a. The coordination number and volume expansion for sites a and c show that even though site c has a larger volume expansion than site a, it leads to similarly large values of ΔE_seg for both P and S which indicates that the same coordination number for site c as for site a is the factor that has the largest effect in the ΔE_seg. A plot of the coordination number of the different sites vs. the respective ΔE_seg are shown in Figure 3.

In this figure it is visible that lower coordination numbers lead to a larger magnitude of ΔE_seg. The magnitude of ΔE_seg decreases with increasing coordination number up to a coordination number of 12 which is the coordination number of the fcc single crystal. For the sites with coordination number 12, the differences in ΔE_seg are due to effects of volume expansion. For the sites with coordination number 12, larger volume expansion leads to larger magnitude of ΔE_seg and consequently stronger segregation of impurities. This effect has been previously observed for a single crystal of fcc Cu [1]. It can be seen additionally that the atomic sites c and d produce considerably different values of ΔE_seg. Both sites have the same volume expansion, but site c has a coordination number of 10 while site d has a coordination number of 11. This highlights again the importance of the coordination number in driving the segregation of impurities. It has been observed previously that the volume expansion and the coordination: bond number and geometry, affect the ΔE_seg of impurities at the GBs and at the bulk [1,7]. Moving away from the GB plane towards the bulk decreases the volume expansion which together with an increase in the coordination number causes the ΔE_seg to become smaller.

The importance of rationalizing the ΔE_seg with GB structure can be seen in the non-linear effects of the changes in coordination number and volume on the values of ΔE_seg. For example, sites a and b both have different coordination numbers and atomic site volumes. Site b has a considerably higher coordination number (CN = 14) and volume expansion (14.0%) when compared to CN = 10 and volume expansion of 2% for site a, respectively. Site a is a near neighbor to the large cavity located at the GB center which leads to a lower coordination number and a not too large volume expansion. This results in an additional driving force for bonding the impurities to site a, which is reflected in the ΔE_seg obtained for this site when compared to site b. A similar phenomenon occurs for sites c and d. Site c is located near the central GB cavity and has a volume expansion of 6.7% when compared to the bulk. The volume expansion at site d is similar, 6.6%, but the coordination number of site c is 10 while that of site d is 11. This leads to a smaller magnitude of ΔE_seg for site d when compared to site c. These effects that determine the ΔE_seg are the result of the balance between volume expansion and coordination. The values of ΔE_seg for sites a and c are larger than for sites b and d, respectively, because in the former pair of sites the coordination numbers are smaller. The effect of the volume expansion can be clearly seen when comparing sites with the same coordination number. The volume expansion can have a favorable effect in driving the segregation if the volume expansion is within the limits that favor the bonding of the impurities with neighboring Cu atoms.

Both P and S have similar ΔE_seg and the variation in the values with d and coordination number follows a similar trend for both cases. For the same sites, S tends to have a larger magnitude of ΔE_seg than P and we can conclude that if both impurities compete for the same atomic site the segregation of S is favored over that of P. Overall, we also found that the coordinates of the Cu atoms that are first neighbors to the impurities suffer slight changes due to the presence of the impurities. The Cu-P or Cu-S bond distances became shorter by up to 0.1 Å when compared to the Cu-Cu distances for the same atomic positions without the presence of the impurities. The occurrence of such contractions, even if small, is also favored by volume expansion and lower coordination number at those sites.

4. Conclusions

The Σ9 grain boundary (GB) has a varied coordination environment and contains atomic sites with considerable differences in atomic site volume within the GB plane. Among the low index GBs this is a unique feature and provides good ground for understanding the geometric effects that are at the origin of the differences in segregation energies for different GB sites. Our data show that the segregation energies of the substitutional impurities P and S vary considerably with distance from the GB plane. The distance from the GB center in turn affects considerably the coordination number and volume expansion of the sites. While the segregation energies are considerably large at the GB, at only ≈3.5 Å the segregation is not favorable. At the GB plane there are significant differences in the segregation energies for each impurity with differences in the order of ≈0.6 eV. At the origin of these effects is a combination of coordination number and volume expansion. We find that the coordination number is a dominating effect but for sites with the same coordination number a larger volume expansion leads to a stronger segregation.

The large differences in segregation energies at the GB plane for the Σ9 GB is a unique feature among lower index symmetric tilt GBs where the segregation of these impurities has been previously studied: Σ3, Σ5 and Σ11. The fact that the segregation energies vary with such magnitudes within the Σ9 GB plane may have implications in the accumulation of these impurities at GBs in the material. In this sense, atomic-scale variations of concentration of these impurities are expected to occur at the Σ9 GB and other GBs with similar features.