Particle Entrapment in Line Elastohydrodynamic Contacts and the Influence of Intermolecular (van der Waals) Forces
Abstract
1. Introduction
2. Mathematical Analysis
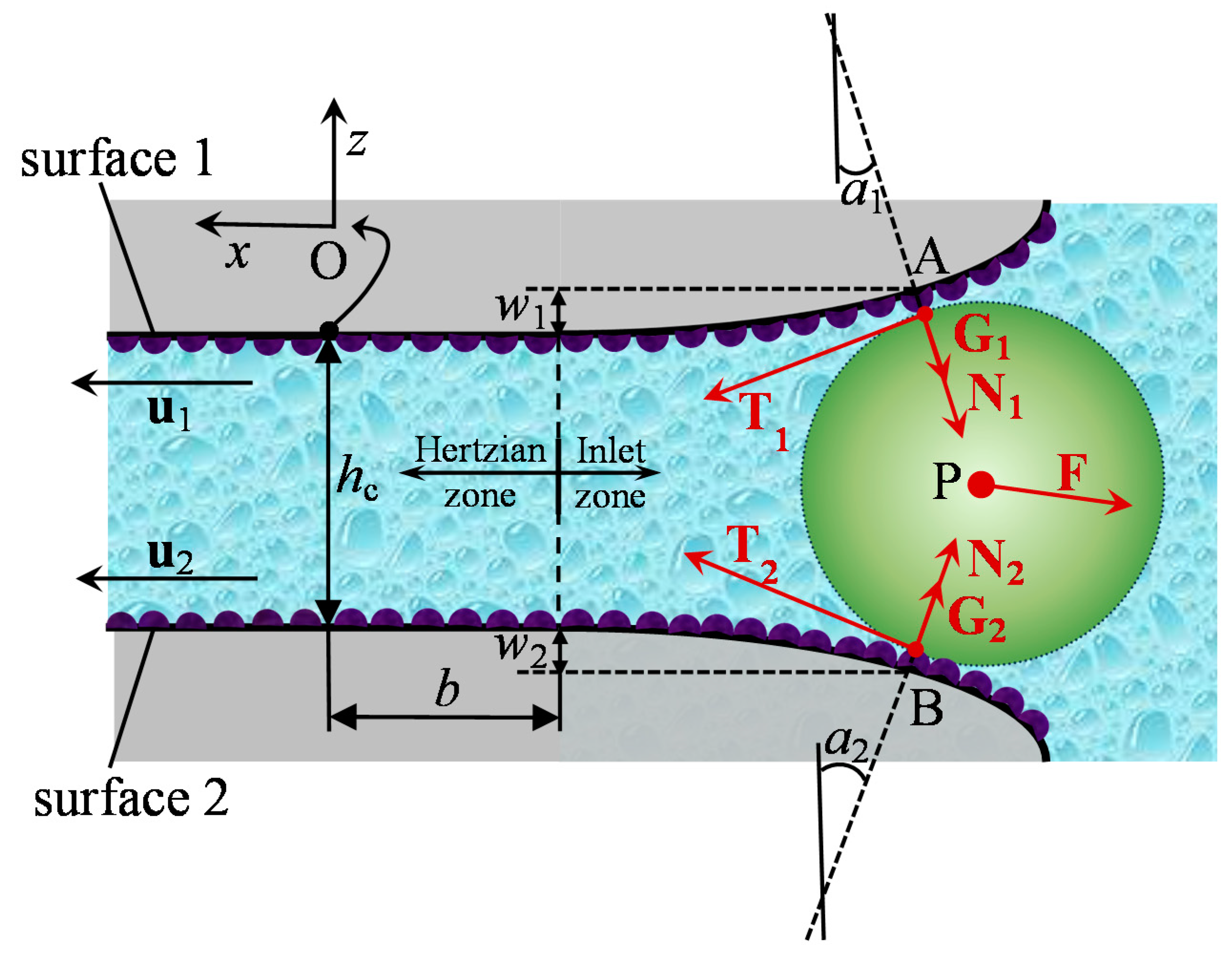
2.1. Contact Geometry
2.2. Fluid Forces
2.3. Intermolecular Forces
2.4. Mechanical Forces
2.5. Force Balance
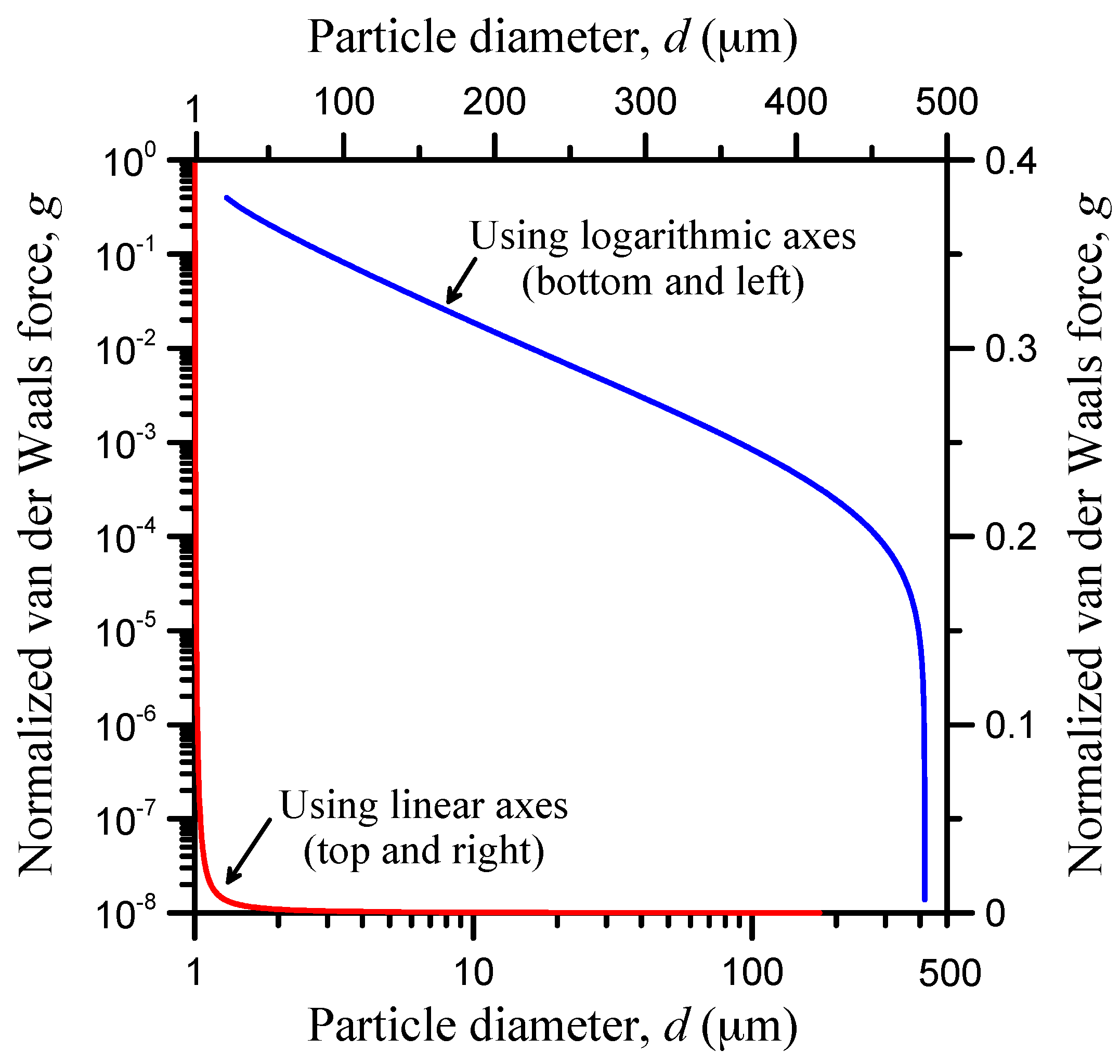
2.6. Entrapment Criterion
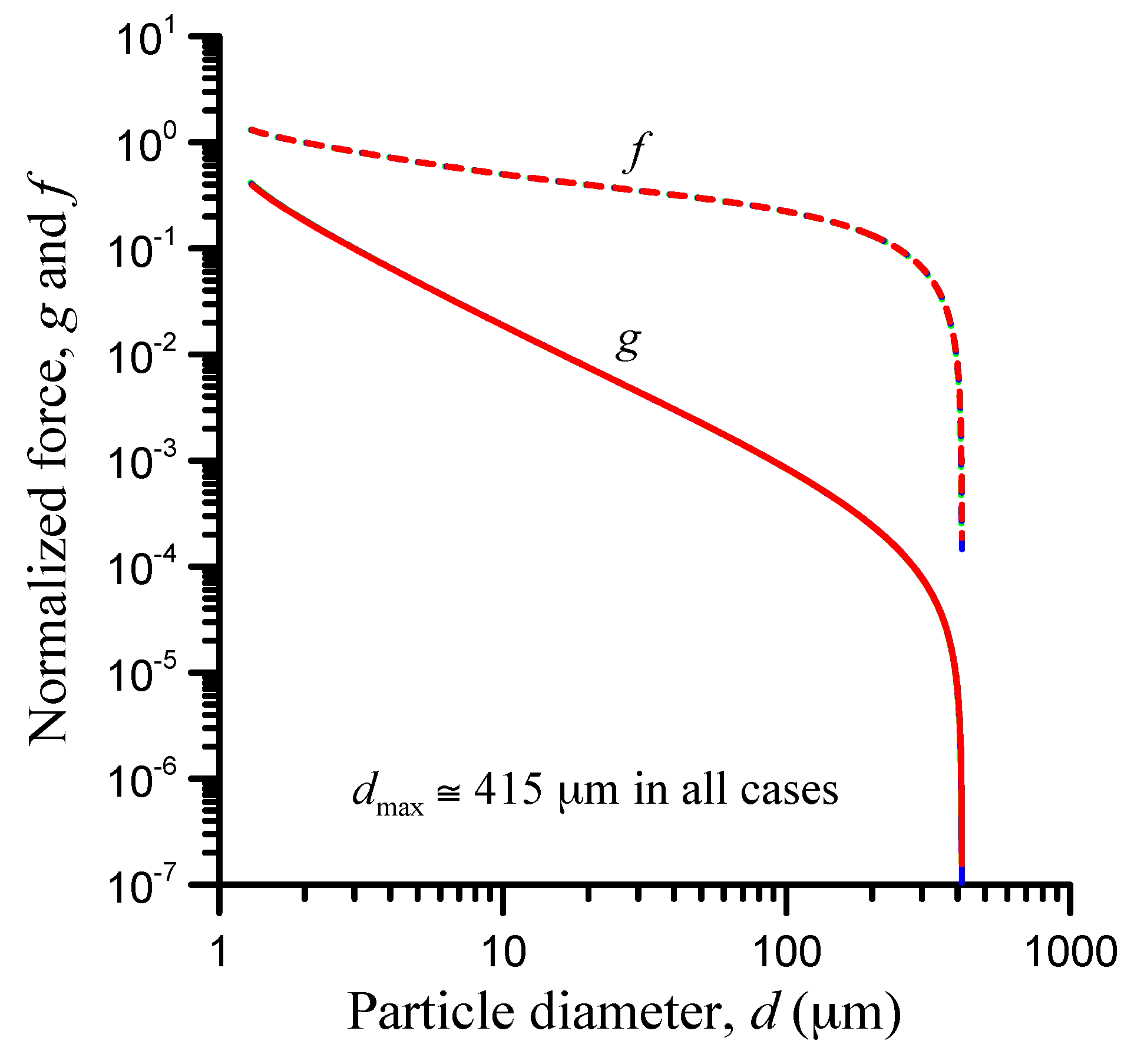
3. Application and Parametric Analysis
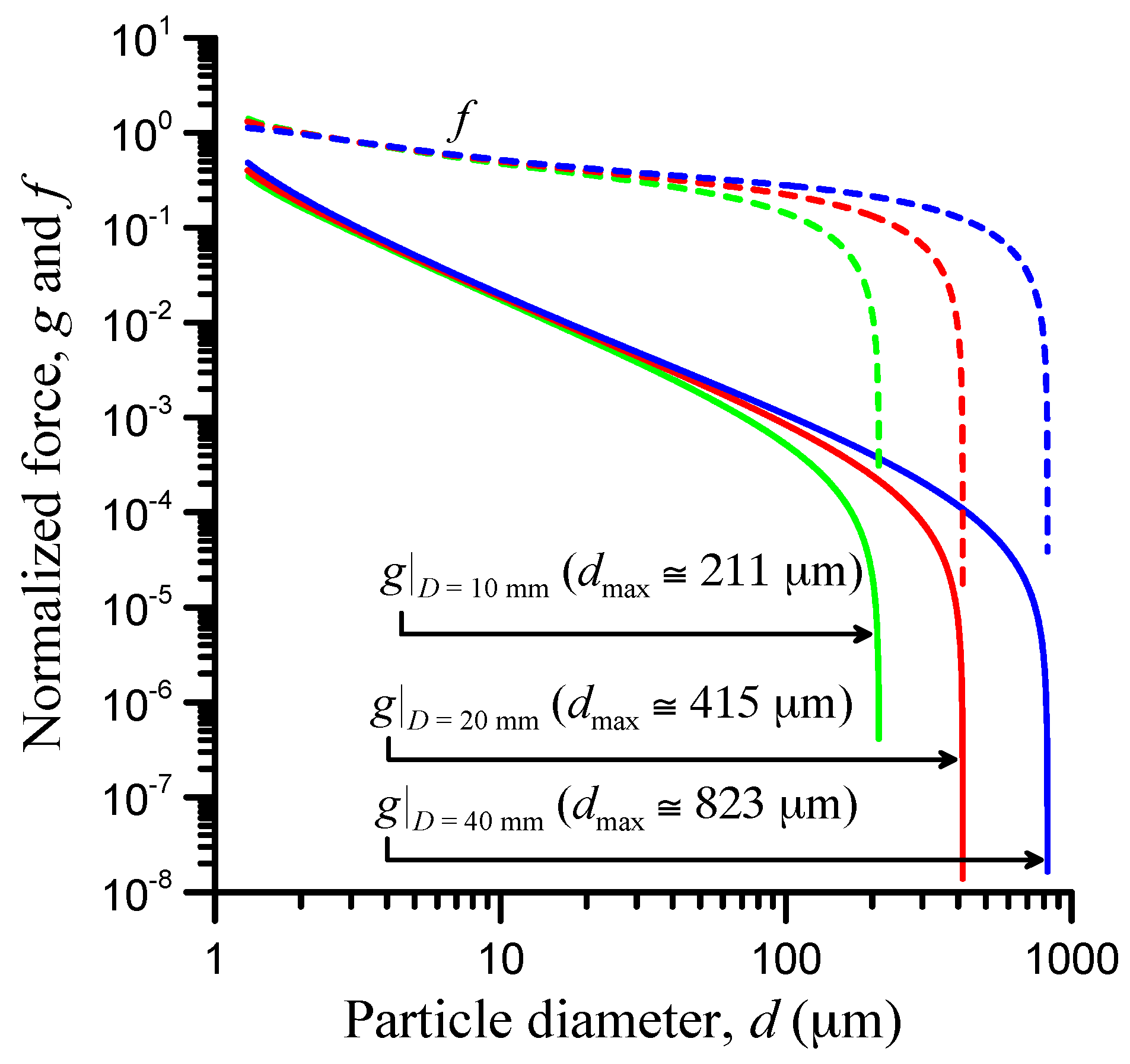
3.1. Contact-Load Effect
3.2. Contact-Width Effect
3.3. Elastic-Modulus Effect
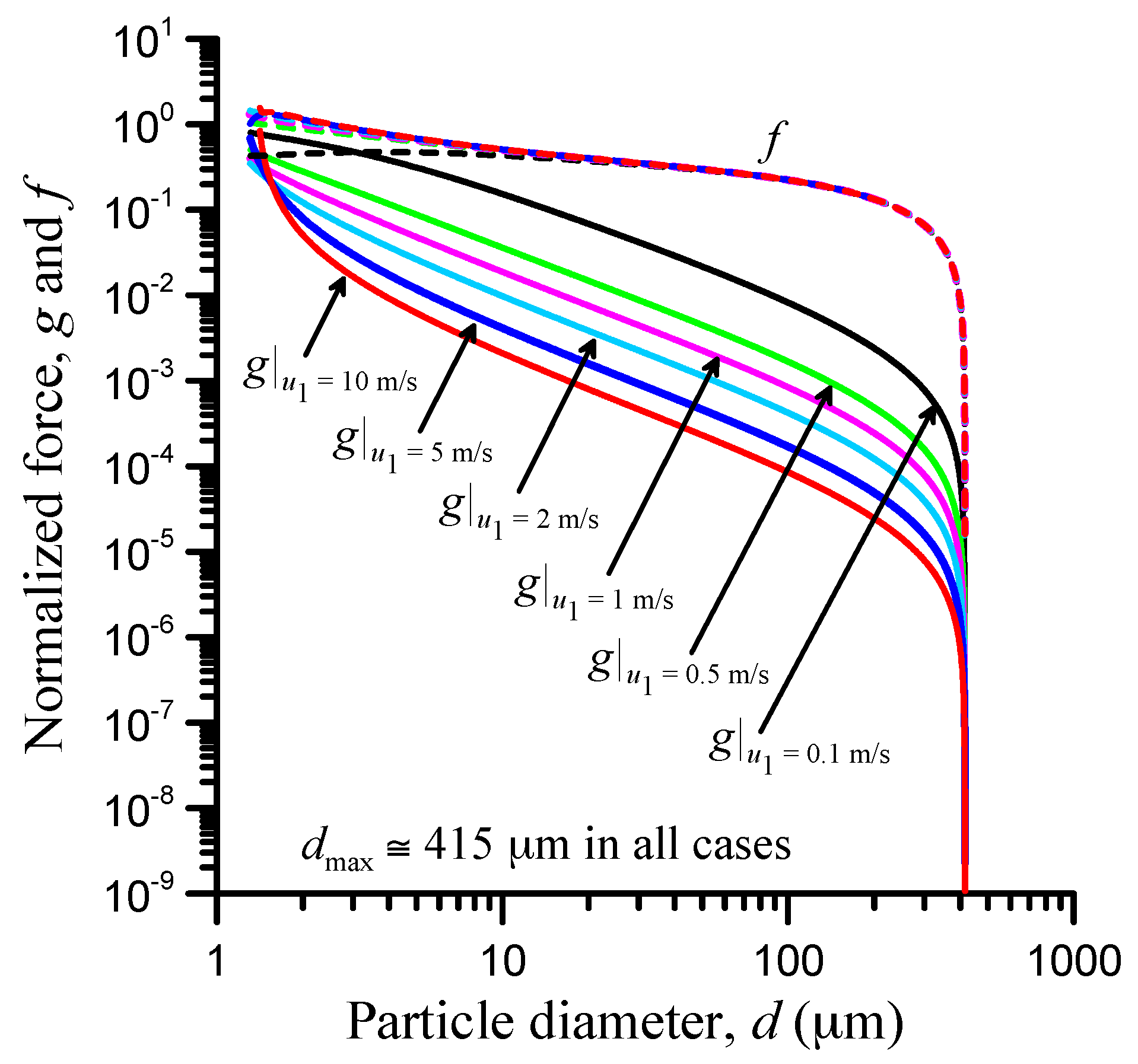
3.4. Speed Effect
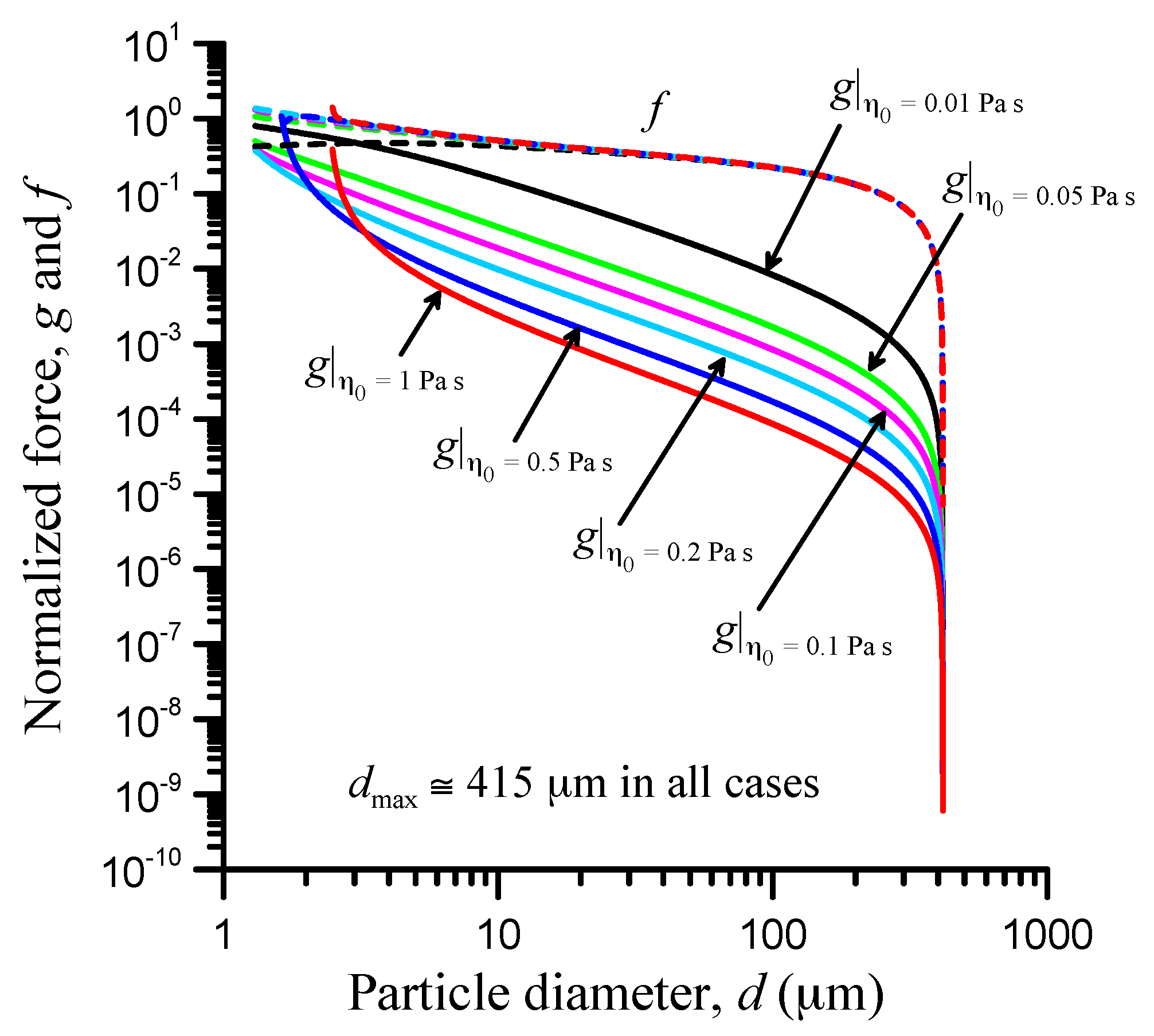
3.5. Viscosity Effect
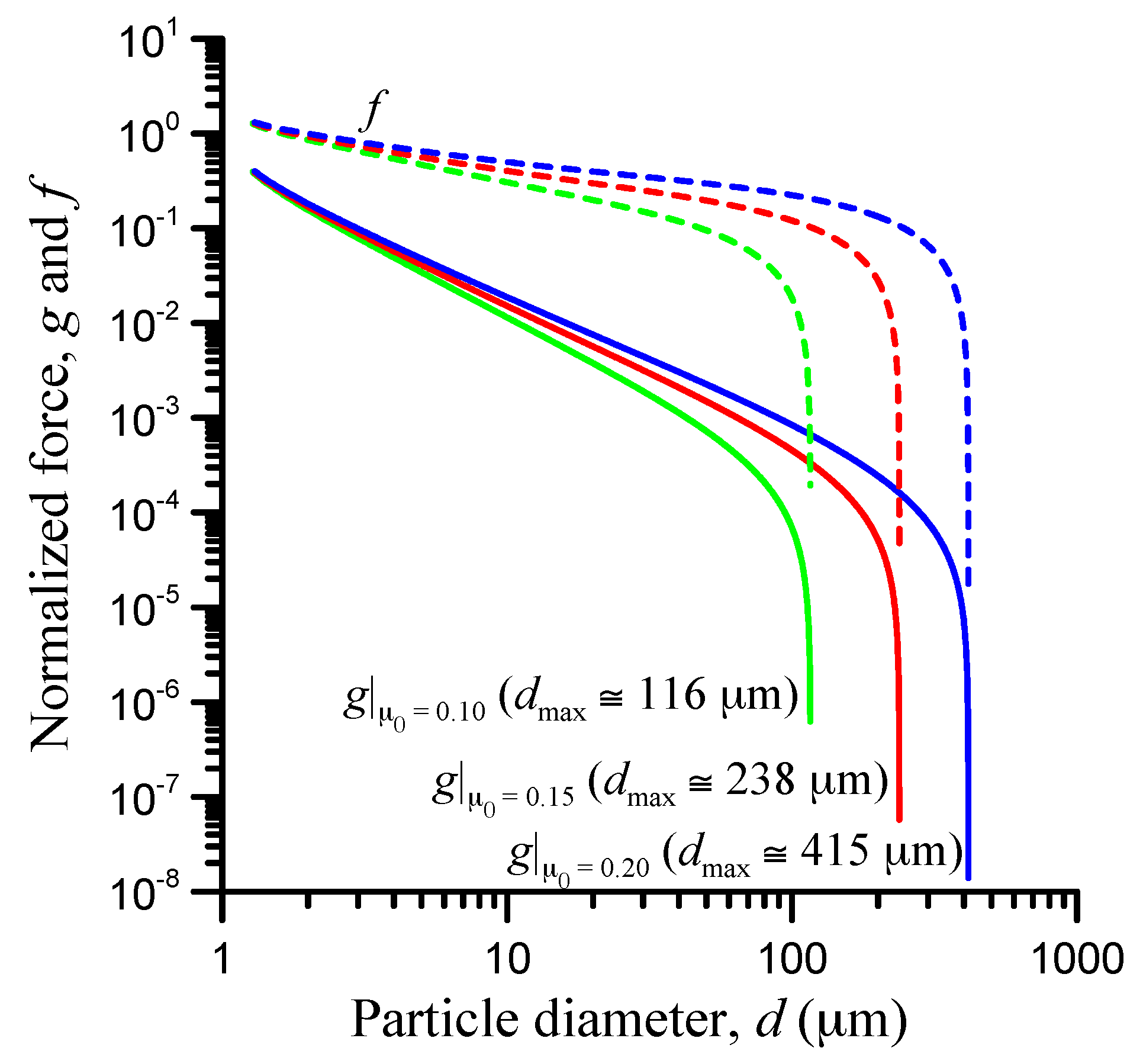
3.6. Friction-Coefficient Effect
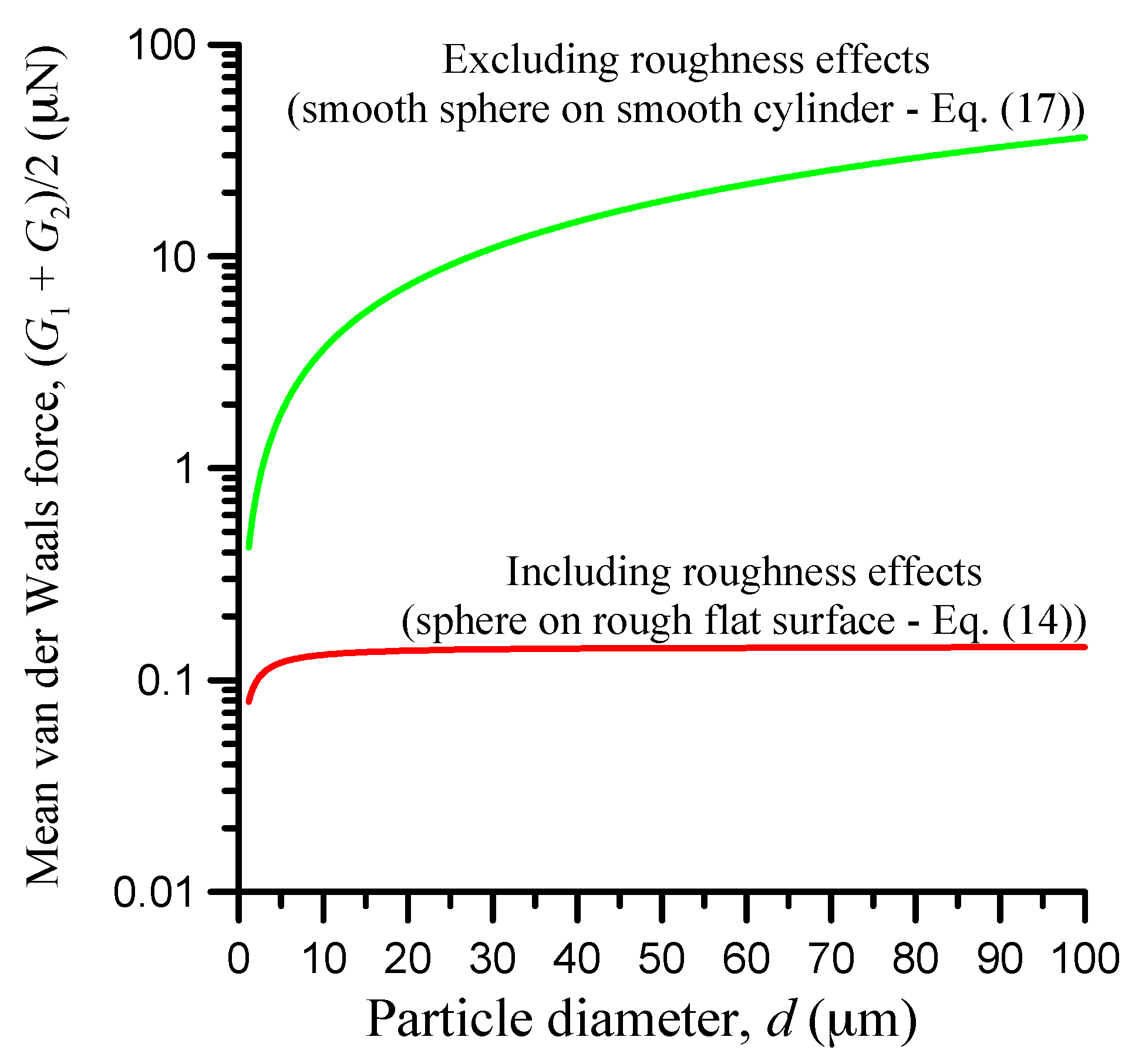
3.7. Roughness Effect
4. Discussion and Conclusions
- (a)
- It is almost certain that a particle collides with at least one surface and at least one time prior to the point of pinching. The probability of a particle simultaneously colliding with both surfaces 1 and 2 without prior collisions in the inlet zone is close to zero. Considering material elasticity and the fluid drag dissipating particle restitution, any particle inertia is quickly lost in such collisions.
- (b)
- For the reason just explained, namely particle collisions to the contact surfaces prior to pinching, any residual electrostatic charge of a (metallic) particle prior to the pinching point is reduced. However, friction during particle collisions may cause tribocharging in the case of dissimilar materials. Such effects are difficult to predict given the uncertainties in the motion of micro-particles, particularly in particle suspensions with inter-particle collisions. It should also be recalled that liquid lubricants are not insulators (their electric conductance depends on many factors such as the base oil and additives used, temperature, and others) and that surfaces are never free of some tribofilm, be it in the form of an engineered coating, an oxide layer, or an adhered contaminant [30]. This reduces the chance of electrostatic effects. Interested readers are referred to Israelachvili [15] for in-depth reading of related phenomena.
Funding
Conflicts of Interest
Nomenclature
| a1, a2 | angles (Figure 1; Equation (10), (11)) |
| b | half-width of the Hertzian contact zone (Figure 1; Equation (3)) |
| b1, b2 | coefficients; (i = 1, 2) |
| ct | thermal correction factor (Equation (A4)) |
| c1, c2 | constants (Equation (2)) |
| d | particle diameter |
| dmax | maximum particle diameter for entrapment (Equation (29)) |
| d0 | auxiliary variable in Equation (29) |
| D | roller diameter |
| Ee | effective modulus of elasticity (Equation (5)) |
| E1, E2 | elastic moduli of surfaces 1 and 2 |
| f | normalised fluid force (Equation (31)) |
| F | total fluid force (Figure 1) |
| Fp | particle load (Figure 2) |
| Fx, Fz | magnitude of the components of F in Ox and Oz (Equations (8) and (9)) |
| g | normalised van der Waals force (Equation (30)) |
| G | signed value of the van der Waals force between a sphere and a cylinder (Equation (17)) |
| G, G1, G2 | van der Waals forces on the particle (Figure 1 and Figure 2) |
| G1, G2 | signed values of van der Waals forces (Equation (14)) |
| hc | central film thickness (Figure 1; Equation (A1)) |
| h1, h2, hf, hp | nonretarded Hamaker constants of surfaces 1 and 2, the lubricant, and the particle |
| H | Hamaker constant |
| H1, H2 | combined, nonretarded Hamaker constants (Equation (15)) |
| k | lubricant thermal conductivity at operating temperature |
| L | load per unit length of the contact |
| m1, m2 | coefficients; when ; otherwise, (i = 1, 2) |
| N, N1, N2 | contact reaction forces (Figure 1 and Figure 2) |
| N1, N2 | magnitudes of N1 and N2 (Equation (27)) |
| p0 | maximum pressure of the line contact (Equation (A5)) |
| P | particle-asperity normal reaction force in Figure 2 |
| P | magnitude of P |
| q | system determinant (Equation (28)) |
| R | equivalent radius of curvature (Equation (4)) |
| R1, R2 | radii of curvature of surfaces 1 and 2 |
| s | separation between sphere (particle) and cylinder (roller) |
| s1, s2 | separation between particle and asperity of surface 1 or 2 |
| S0 | temperature exponent of the Roelands viscosity formula |
| S02 | ASTM slope of the lubricant between 40 and 100 °C, divided by 0.2 (Equation (A3)) |
| T, T1, T2 | frictional forces (Figure 1 and Figure 2) |
| T, T1, T2 | magnitudes of T, T1, and T2, respectively (Equations (20) and (24)) |
| u1, u2 | tangential velocities (Figure 1) |
| u1, u2 | magnitudes of the tangential velocities (Figure 1) |
| w1, w2 | elastic normal surface displacements (Figure 1; Equation (1)) |
| W | van der Waals interaction energy (Equation (16)) |
| x | coordinate (Figure 1) |
| xA, xB, xP | x-coordinates of points A, B, and the particle centre P |
| z | coordinate (Figure 1) |
| zP | ordinate of particle’s centre |
| Greek Symbols | |
| α | pressure-viscosity coefficient of the lubricant at operating temperature (Equation (A2)) |
| η0 | lubricant dynamic viscosity at operating temperature |
| ϑ | angle (Figure 2) |
| θ | operating temperature |
| θ0 | ambient temperature |
| λ | parameter (Equation (A6)) |
| μ | “local” coefficient of friction (Equation (21)) |
| μmax | maximum permissible value of μt |
| , , | total friction coefficients (Equation (22) or (25)) |
| , , | “macroscopic” (Coulomb) coefficients of friction |
| ν1, ν2 | Poisson’s ratios of surfaces 1 and 2 |
| ν40, ν100 | kinematic viscosity at 40 and 100 °C, respectively |
| σ1, σ2, σP | RMS roughness of surface 1, 2, and the particle |
| Abbreviations | |
| ASTM | American Society for Testing and Materials |
| EHL | elastohydrodynamically lubricated |
| EHD | elastohydrodynamic |
| RMS | root mean square |
Appendix A
References
- Nikas, G.K. A state-of-the-art review on the effects of particulate contamination and related topics in machine-element contacts. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2010, 224, 453–479. [Google Scholar] [CrossRef]
- Nikas, G.K. Review of studies on the detrimental effects of solid contaminants in lubricated machine element contacts. In Reliability Engineering Advances; Hayworth, G.I., Ed.; Nova Science Publishers: New York, NY, USA, 2009; pp. 1–44. ISBN 9781606923290. [Google Scholar]
- Dwyer-Joyce, R.S. The Effects of Lubricant Contamination on Rolling Bearing Performance. Ph.D. Thesis, Imperial College London, Department of Mechanical Engineering, London, UK, 1993. [Google Scholar]
- Nikas, G.K. Theoretical Modelling of the Entrainment and Thermomechanical Effects of Contamination Particles in Elastohydrodynamic Contacts. Ph.D. Thesis, Imperial College London, Department of Mechanical Engineering, London, UK, 1999. [Google Scholar]
- Nikas, G.K. Particle entrainment in elastohydrodynamic point contacts and related risks of oil starvation and surface indentation. ASME J. Tribol. 2002, 124, 461–467. [Google Scholar] [CrossRef]
- Kang, Y.S.; Sadeghi, F.; Ai, X. Debris effects on EHL contact. ASME J. Tribol. 2000, 122, 711–720. [Google Scholar] [CrossRef]
- Han, H.; Zhang, Y. Theoretical effects of contaminant particles on the lubrication considering particle rotation. In Proceedings of the STLE/ASME International Joint Tribology Conference IJTC2008, Miami, FL, USA, 20–22 October 2008; pp. 303–305. [Google Scholar] [CrossRef]
- Han, H.; Zhang, Y.; Zhong, Z. Effect of particle transient motion on lubrication. Ind. Lubr. Tribol. 2010, 62, 126–135. [Google Scholar] [CrossRef]
- Dwyer-Joyce, R.S. Predicting the abrasive wear of ball bearings by lubricant debris. Wear 1999, 233–235, 692–701. [Google Scholar] [CrossRef]
- Dwyer-Joyce, R.S.; Hamer, J.C.; Sayles, R.S.; Ioannides, E. Lubricant screening for debris effects to improve fatigue and wear life. In Proceedings of 18th Leeds–Lyon Symposium on Tribology; Elsevier Tribology and Interface Engineering Series; Elsevier: Lyon, France, 1991; Volume 21, pp. 57–63. [Google Scholar] [CrossRef]
- Kusano, Y.; Hutchings, I.M. Modelling the entrainment and motion of particles in a gap: Application to abrasive wear. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2003, 217, 427–433. [Google Scholar] [CrossRef]
- Zhong, Z.; Zhang, Y.; Han, H. A simple model of the entrainment of particles in a gap considering elasticity. In Proceedings of the STLE/ASME International Joint Tribology Conference IJTC2008, Miami, FL, USA, 20–22 October 2008; pp. 461–463. [Google Scholar] [CrossRef]
- Morales Espejel, G.M.; Gabelli, A. Particle entrapment and indentation process in rolling bearings. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2016, 230, 1572–1587. [Google Scholar] [CrossRef]
- Nikas, G.K. Mathematical analysis of the entrapment of solid spherical particles in non-conformal contacts. ASME J. Tribol. 2001, 123, 83–93. [Google Scholar] [CrossRef]
- Israelachvili, J.N. Intermolecular and Surface Forces, 3rd ed.; Academic Press (Elsevier): Oxford, UK, 2011; ISBN 9780123919274. [Google Scholar]
- Johnson, K.L. Contact Mechanics; Section 10.4; Cambridge University Press: Cambridge, UK, 1985; ISBN 0521347963. [Google Scholar]
- Khonsari, M.M.; Booser, E.R. Applied Tribology, 3rd ed.; Table 3.1.; Wiley: Hoboken, NJ, USA, 2017; ISBN 9781118700259. [Google Scholar]
- Nikas, G.K.; Ioannides, E.; Sayles, R.S. Thermal modeling and effects from debris particles in sliding/rolling EHD line contacts—A possible local scuffing mode. ASME J. Tribol. 1999, 121, 272–281. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Schmid, S.R.; Jacobson, B.O. Fundamentals of Fluid Film Lubrication, 2nd ed.; Marcel Dekker, Inc.: New York, NY, USA, 2004; ISBN 0824753712. [Google Scholar]
- Parsegian, V.A. Van der Waals Forces: A Handbook for Biologists, Chemists, Engineers and Physicists; Cambridge University Press: Cambridge, UK, 2006; ISBN 9780521839068. [Google Scholar]
- Rumpf, H. Particle Technology; Chapman and Hall: London, UK, 1990; ISBN 9789401179461. [Google Scholar]
- Rabinovich, Y.I.; Adler, J.J.; Ata, A.; Singh, R.K.; Moudgil, B.M. Adhesion between nanoscale rough surfaces: I. Role of asperity geometry. J. Colloid Interf. Sci. 2000, 232, 10–16. [Google Scholar] [CrossRef] [PubMed]
- Montgomery, S.W.; Franchek, M.A.; Goldschmidt, V.W. Analytical dispersion force calculations for nontraditional geometries. J. Colloid Interf. Sci. 2000, 227, 567–584. [Google Scholar] [CrossRef] [PubMed]
- Berman, A.D.; Israelachvili, J.N. Microtribology and microrheology of molecularly thin liquid films. In Modern Tribology Handbook; vol. 1, chapter 16; Bhushan, B., Ed.; CRC Press LLC: London, UK, 2001; ISBN 0849384036. [Google Scholar]
- Mo, Y.; Turner, K.T.; Szlufarska, I. Friction laws at the nanoscale. Nature 2009, 457, 1116–1119. [Google Scholar] [CrossRef] [PubMed]
- Meyer, J.; Fuchs, R.; Staedler, T.; Jiang, X. Effect of surface roughness on sliding friction of micron-sized glass beads. Friction 2014, 2, 255–263. [Google Scholar] [CrossRef]
- Nikas, G.K. Particle extrusion in elastohydrodynamic line contacts: Dynamic forces and energy consumption. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2017, 231, 1320–1340. [Google Scholar] [CrossRef]
- ISO 4287:1997 Standard. Geometrical Product Specifications (GPS)—Surface Texture: Profile Method—Terms, Definitions and Surface Texture Parameters; International Organization for Standardization: Geneva, Switzerland, 1997.
- Rabinovich, Y.I.; Adler, J.J.; Ata, A.; Singh, R.K.; Moudgil, B.M. Adhesion between nanoscale rough surfaces: II. Measurement and comparison with theory. J. Colloid Interf. Sci. 2000, 232, 17–24. [Google Scholar] [CrossRef] [PubMed]
- Jacobson, S.; Hogmark, S. Tribofilms—On the Crucial Importance of Tribologically Induced Surface Modifications. In Recent Developments in Wear Prevention, Friction and Lubrication; Chapter 5; Nikas, G.K., Ed.; Research Signpost: Kerala, India, 2010; ISBN 9788130803777. [Google Scholar]
- Cusano, C.; Sliney, H.E. Dynamics of solid dispersions in oil during the lubrication of point contacts: Part I—Graphite. ASLE Trans. 1982, 25, 183–189. [Google Scholar] [CrossRef]
- Wan, G.T.Y.; Spikes, H.A. The behavior of suspended solid particles in rolling and sliding elastohydrodynamic contacts. Tribol. Trans. 1988, 31, 12–21. [Google Scholar] [CrossRef]
- Pan, P.; Hamrock, B.J. Simple formulas for performance parameters used in elastohydrodynamically lubricated line contacts. ASME J. Tribol. 1989, 111, 246–251. [Google Scholar] [CrossRef]
- Gupta, P.K.; Cheng, H.S.; Zhu, D.; Forster, N.H.; Schrand, J.B. Viscoelastic effects in MIL-L-7808-type lubricant, part I: Analytical formulation. Tribol. Trans. 1992, 35, 269–274. [Google Scholar] [CrossRef]
- Wu, C.S.; Klaus, E.E.; Duda, J.L. Development of a method for the prediction of pressure-viscosity coefficients of lubricating oils based on free-volume theory. ASME J. Tribol. 1989, 111, 121–128. [Google Scholar] [CrossRef]
- Denis, J.; Briant, J.; Hipeaux, J.C. Lubricant Properties, Analysis and Testing; Section 2.5.5.1; Editions Technip: Paris, France, 2000; ISBN 2710807467. [Google Scholar]



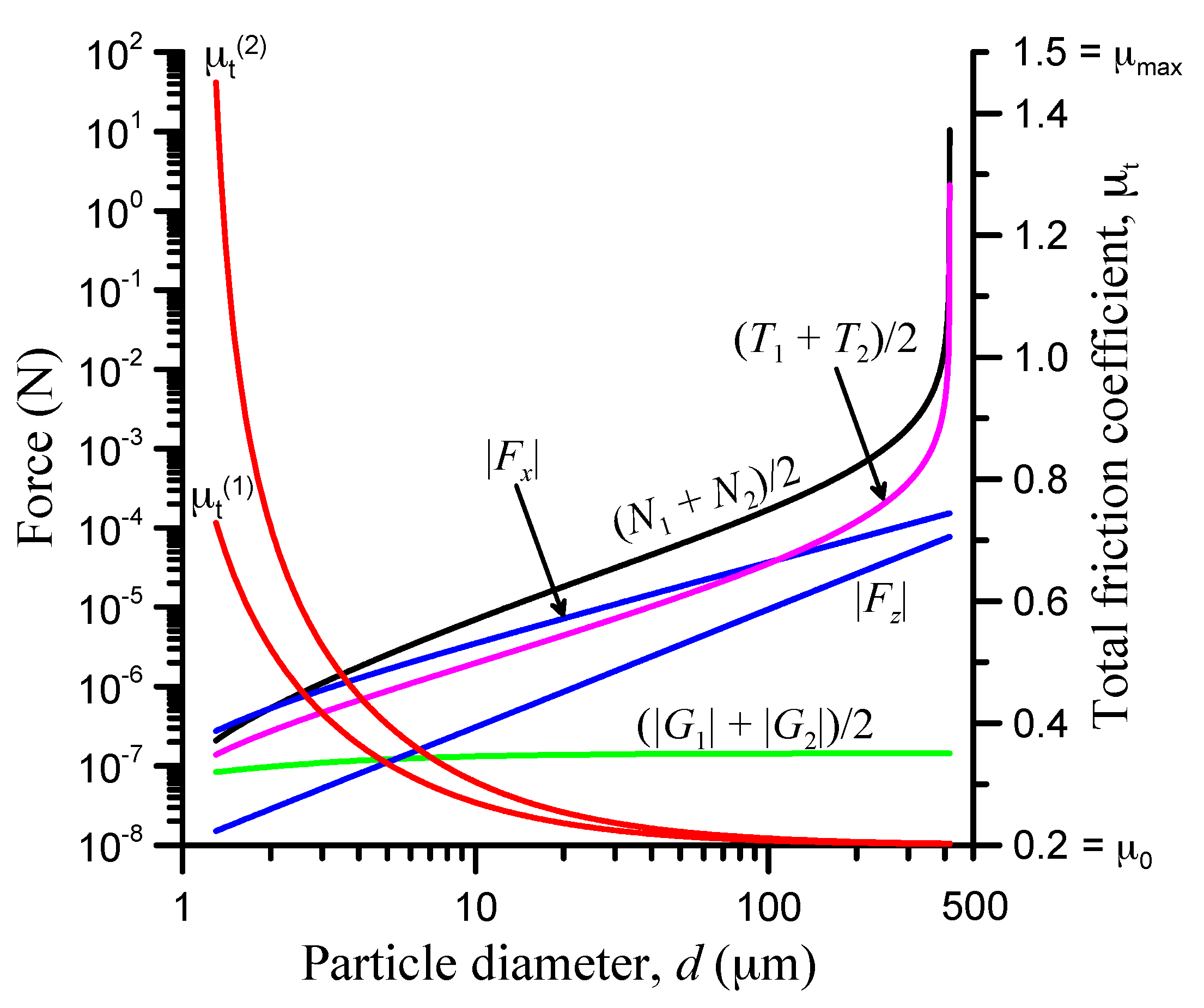
| Parameter | Value |
|---|---|
| Elastic modulus: E1; E2 (GPa) | 207; 207 |
| Poisson’s ratio: ν1; ν2 | 0.3; 0.3 |
| Diameter of rollers 1 and 2: D (mm) | 20 |
| Load per unit length of the contact: L (N/mm) | 100 |
| Surface speed: u1; u2 (m/s) | 1; 0.001 |
| Ambient temperature: θ0 (°C) | 23 |
| Operating temperature: θ (°C) | 23 |
| Lubricant mass density at operating temperature: ρ0 (kg/m3) | 708 |
| Lubricant dynamic viscosity at operating temperature: η0 (Pa s) | 0.1 |
| Lubricant kinematic viscosities: ν40; ν100 (mm2/s (cSt)) | 68; 8.8 |
| Lubricant thermal conductivity at operating temperature: k (W/(m K)) | 0.14 |
| Temperature exponent of the Roelands viscosity formula: S0 | 1.1 (Hamrock et al. [19]) |
| “Macroscopic” coefficient of friction at particle pinching: ; | 0.2; 0.2 (Nikas [27]) |
| Maximum permissible coefficient of friction: μmax | 1.5 |
| Nonretarded Hamaker constant: h1; h2; hp; hf (10–20 J) | 40; 40; 40; 5 (Israelachvili [15]) |
| Separation between particle and surface: s1; s2 (nm) | 0.3; 0.3 (Rabinovich et al. [22]) |
| RMS surface roughness: σ1; σ2 (nm) | 250; 380 (Khonsari and Booser [17]) |
| η0 (Pa s) | ν40 (mm2/s) | ν100 (mm2/s) |
|---|---|---|
| 0.01 | 6.8 | 0.88 |
| 0.05 | 34 | 4.4 |
| 0.1 | 68 | 8.8 |
| 0.2 | 136 | 17.6 |
| 0.5 | 340 | 44 |
| 1 | 680 | 88 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nikas, G.K. Particle Entrapment in Line Elastohydrodynamic Contacts and the Influence of Intermolecular (van der Waals) Forces. Lubricants 2020, 8, 60. https://doi.org/10.3390/lubricants8050060
Nikas GK. Particle Entrapment in Line Elastohydrodynamic Contacts and the Influence of Intermolecular (van der Waals) Forces. Lubricants. 2020; 8(5):60. https://doi.org/10.3390/lubricants8050060
Chicago/Turabian StyleNikas, George K. 2020. "Particle Entrapment in Line Elastohydrodynamic Contacts and the Influence of Intermolecular (van der Waals) Forces" Lubricants 8, no. 5: 60. https://doi.org/10.3390/lubricants8050060
APA StyleNikas, G. K. (2020). Particle Entrapment in Line Elastohydrodynamic Contacts and the Influence of Intermolecular (van der Waals) Forces. Lubricants, 8(5), 60. https://doi.org/10.3390/lubricants8050060






