Aspects of Self-Organization of Tribological Stressed Lubricating Greases
Abstract
1. Introduction
2. Fundamental Definitions
3. The General Grease Behaviour
4. A Thermodynamic Point of View
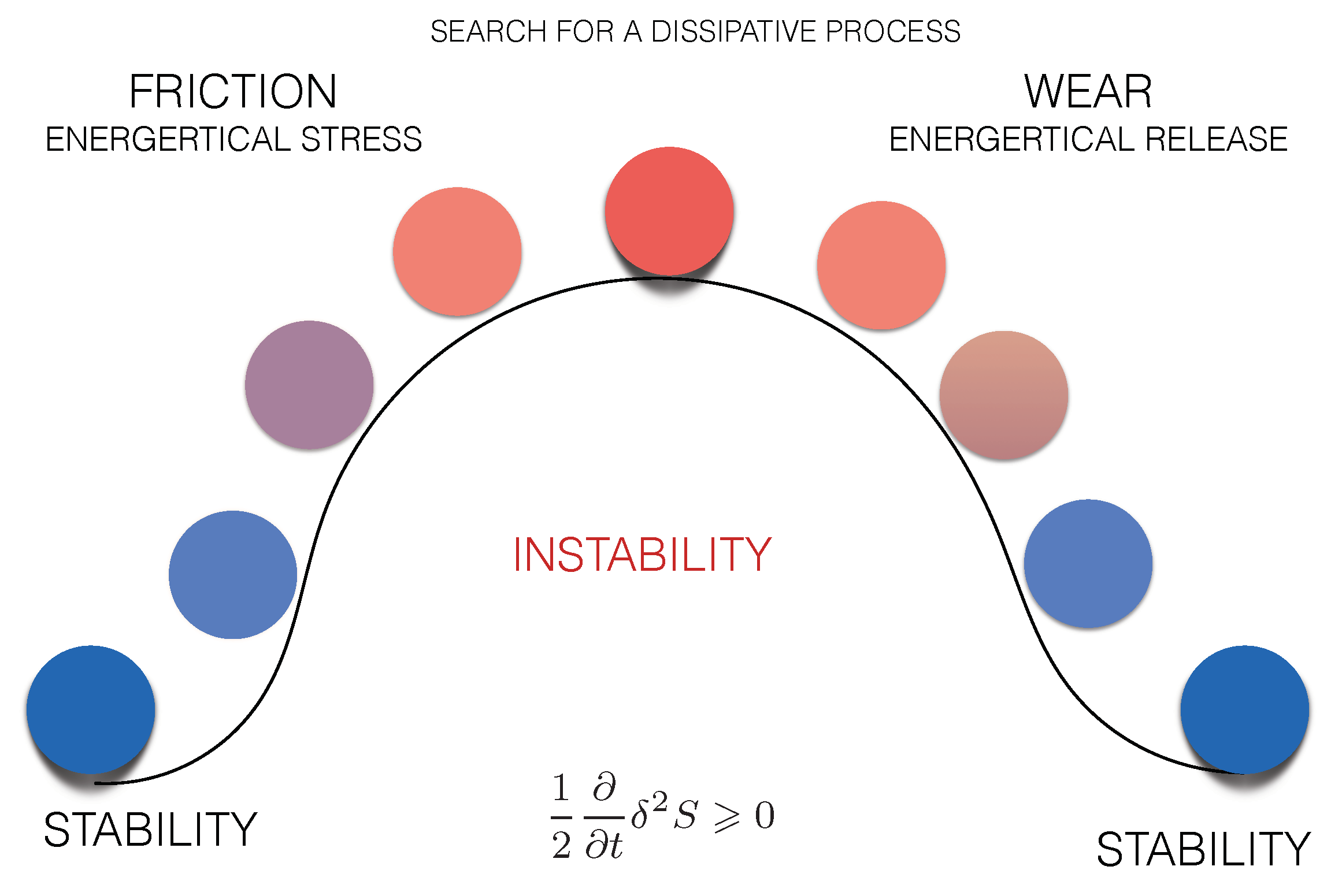
5. Criterion for Instability
- shear process of the base oil
- the process of fragmentation of the solid agglomerates
- process of coagulation or agglomeration caused by collision of particles.
- the first addend is always negative because of the sign and the square
- in order for the second addend to become negative, it would be necessary to meet the following assumption
- and for the third addend to become negative
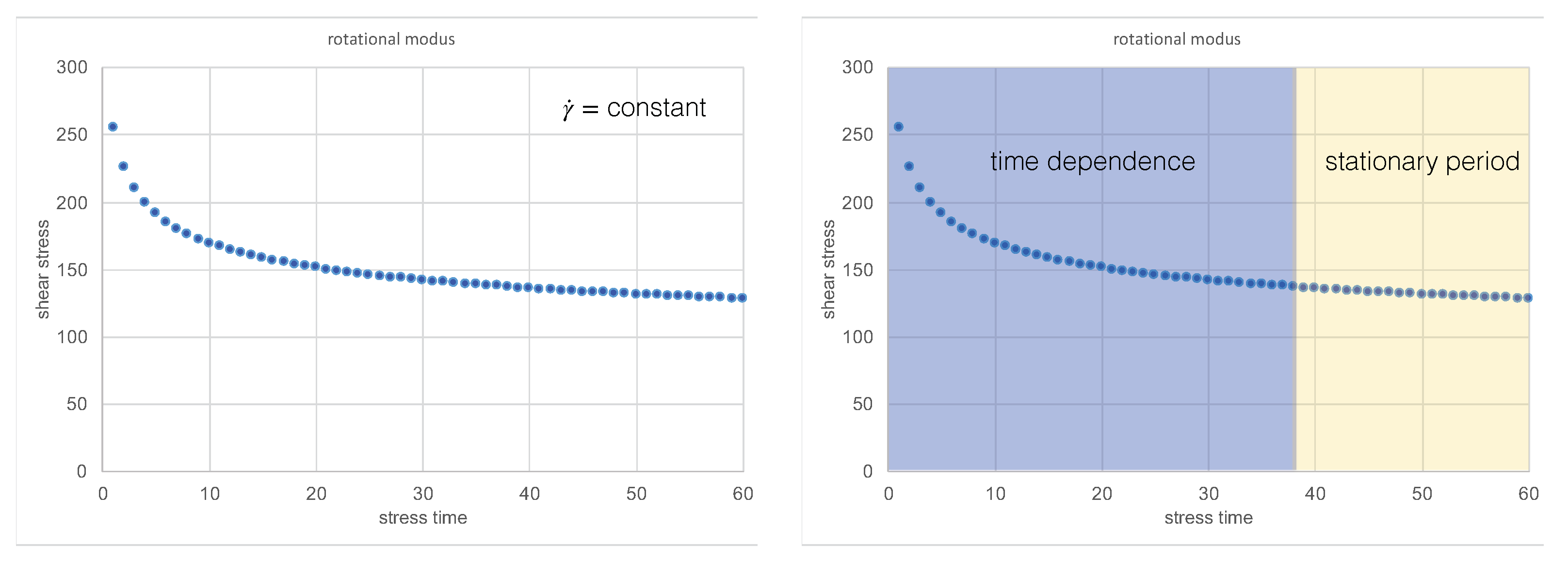
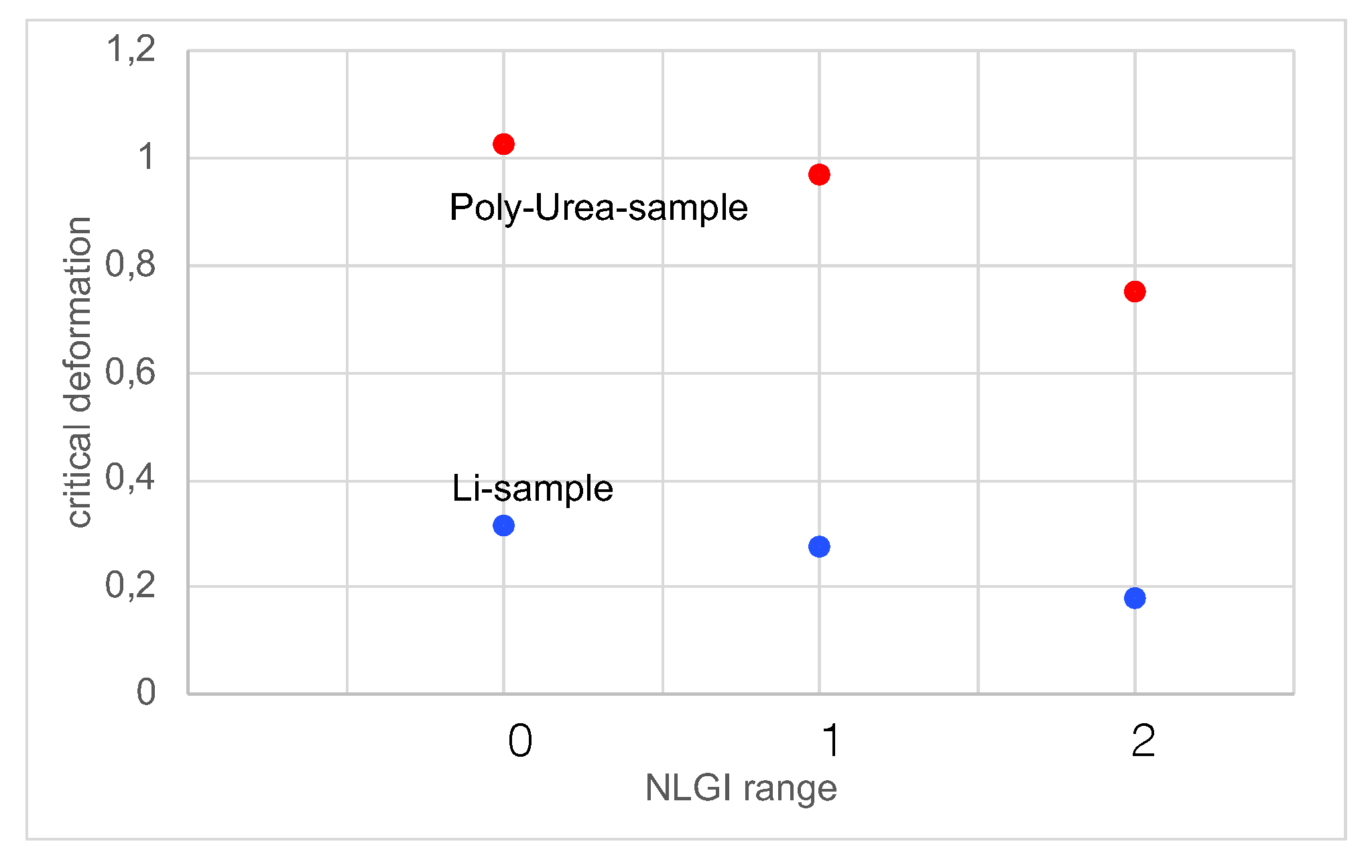
6. Experimental Work
7. Some Thoughts About the Stationary Period
8. Conclusions
Acknowledgments
Conflicts of Interest
Abbreviations
| G’ | storage modulus [Pa] |
| S | entropy [J/K] |
| T | temperature [K] |
| a,b | constants of integration |
| e | energy density [J/m] |
| deformation [−] | |
| shear rate [1/s] | |
| heat conductivity [W/mK] | |
| shear stress [Pa] |
References
- Nosonovsky, M.; Bushan, B. Thermodynamics of surface degradation, self-organization and self-healing for biomimetic surfaces. Philos. Trans. R. Soc. A 2009, 367. [Google Scholar] [CrossRef] [PubMed]
- Abdel-Aal, H. Concerning the Thermo-Thermodynamical aspects of wear regime transition in dry tribosystems. Ann. Univ. Dunaries Jos Galati Fascicle VIII 2015, 8, 41–47. [Google Scholar]
- Gershman, I.S.; Mironov, A.E.; Gershman, E.I.; Fox-Rabinovich, G.S.; Veldhuis, C. Self-organisation during friction of sliding bearing antifriction materials. Entropy 2015, 17, 7967–7978. [Google Scholar] [CrossRef]
- Rezasoltani, A.; Khonsari, M.M. On the correlation between mechanical degradation of lubricating greases and entropy. Tribol. Lett. 2014, 56, 197–204. [Google Scholar] [CrossRef]
- Bryant, M.D.; Khonsari, M.M.; Ling, F.F. On the thermodynamics of degradation. Proc. R. Soc. A 2008, 464, 2001–2014. [Google Scholar] [CrossRef]
- Kuhn, E. Stability of the wear process of lubricating greases. In Proceedings of the 15th Arnold Tross Colloquium, Hamburg, Germany, 17 May 2019. [Google Scholar]
- Czarny, R. Einfluss der Thixotropie auf die rheologischen Eigenschaften der Schmierfette. Tribol. Und Schmier. 1989, 36, 134–140. [Google Scholar]
- Bartz, W.J. u.a. Schmierfette, 2nd ed.; Expert Verlag: Renningen, Germany, 2015. [Google Scholar]
- Paszkowski, M. Effect of grease thickener and surface material on rheological properties of boundary layer. Colloids Surf. A Physicochem. Eng. Aspects 2014, 480, 462–467. [Google Scholar] [CrossRef]
- Nunez, N.; Masrtin-Alfonso, J.E.; Valencia, C.; Sanchez, M.C.; Franco, J.M. Rheology of new green lubricating grease formulations conmtaining cellulose pulp and its methylated derivative as thickener agents. Ind. Crop. Prod. 2012, 37, 500–507. [Google Scholar] [CrossRef]
- Sanchez, R.; Valencia, C.; Franco, J.M. Rheological and tribological characterization of a new acylated chitosan-based biodegradable lubricating graease: A comparaitve study with tradtional lithium and calcium greases. Tribol. Trans. 2014, 57, 445–454. [Google Scholar] [CrossRef]
- Sanchez, M.C.; Franco, J.M.; Valencia, C.; Gallegos, C.; Urquiola, F.; Urchegui, R. Atomic force microscopy and thermorheological characterisation of lubricating greases. Tribol. Lett. 2011, 41, 463–470. [Google Scholar] [CrossRef]
- Gonçalves, D.E.P.; Campos, A.V.; Seabra, J.H. An experimentsal study on starved grease lubricated contacts. Lubricants 2018, 6, 82. [Google Scholar] [CrossRef]
- Paszkowski, M.; Olsztynska-Janus, S. Grease thixotropy: Evaluation of grease microstructure change due to shear relaxation. Ind. Lubr. Tribol. 2014, 66, 223–237. [Google Scholar] [CrossRef]
- Kuhn, E. Energy investigations of the rheological wear of lubricating greases. Rheology 1992, 2, 252. [Google Scholar] [CrossRef]
- Kuhn, E. Investigation into the degradation of the structure of lubricating greases. Tribol. Trans. 1998, 41, 247–250. [Google Scholar] [CrossRef]
- Zhou, Y.; Bosman, R.; Lught, P.M. A model for shear degradation of lithium soap grease at ambient temperature. Tribol. Trans. 2018, 61, 61–78. [Google Scholar] [CrossRef]
- Osara, J.A.; Bryant, M.D. Thermodynamics of grease degradation. Tribol. Int. 2019, 137, 433–445. [Google Scholar] [CrossRef]
- Gurt, A.; Khonsari, M. The use of entropy in modeling the mechanical degradation of grease. Lubricants 2019, 7, 82. [Google Scholar] [CrossRef]
- Lijesh, K.P.; Khonsari, M. On the assessment of mechanical degradation of grease using entropy generation rate. Tribol. Lett. 2019. [Google Scholar] [CrossRef]
- Kuhn, E. Tribological stress of lubricating greases in the light of system entropy. Lubricants 2016, 4, 37. [Google Scholar] [CrossRef]
- Prigogine, I. Vom Sein zum Werden- Zeit und Komplexitaet in den Naturwissenschaften; Piper-Verlag: Munich, Germany, 1980. [Google Scholar]
- Nosonovsky, M. Entropy in tribology: In the search for application. Entropy 2010, 12, 1345–1390. [Google Scholar] [CrossRef]
- Gershman, I.; Gershman, E.I.; Mironov, A.E.; Fox-Rabinovich, G.; Veldhuis, S. Application of the self-organization phenomenon in the development of wear resistsant materials—A review. Entropy 2016, 18, 385. [Google Scholar] [CrossRef]
- Gershamn, I.S.; Gershman, E.I.; Fox-Rabinovich, G.S.; Veldhius, S.C. Description of seizure process for gas dynamic spray of metal powders from non-equilibrium thermodynamics standpoint. Entropy 2016, 18, 315. [Google Scholar] [CrossRef]
- Fox-Rabinovich, G.; Veldhuis, S.C.; Kovalev, A.I.; Wainstein, D.L.; Gershman, I.S.; Korshunov, S.; Shuster, L.S.; Endrino, J.L. Features of self-organization in ion modified nanocrystalline plasma vapor deposited AlTiN coatings under serve tribological conditions. J. Appl. Phys. 2007, 102, 074305. [Google Scholar] [CrossRef]
- De Groot, S.R.; Mazur, P. Basics of Thermodynamic of Irreversible Processes; Bibliograph. Institut: Mannheim, Germany, 1984; ISBN 978-341001620. [Google Scholar]
- Acar, N.; Franco, J.M.; Kuhn, E. On the shear-induced structural degradation of lubricating greases and associated activation energy: An experimental rheological study. Tribol. Int. 2020, 144, 106105. [Google Scholar] [CrossRef]
- Nosonovsky, M.; Bhushan, B. Surface-self-organization: From wear to self-healing in biological and technical surfaces. Appl. Surf. Sience 2010, 256, 3982–3987. [Google Scholar] [CrossRef]

| Effect | Driving Force | Condition to Initiate | Final Configuration |
|---|---|---|---|
| stationary microtopography after running in | feedback due to coupling of friction and wear | wear affects microtopography until it reaches the stationary value | minimum friction and wear [1] |
| in situ tribofilm formation | chemical reaction leads to film growth | wear decreases with increasing film thickness | minimum friction and wear at stationary film thickness [1] |
| stationary grease structure after time dependence | involved fragmentation and coagulation | thermodynamic criteria | minimum entropy production |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuhn, E. Aspects of Self-Organization of Tribological Stressed Lubricating Greases. Lubricants 2020, 8, 28. https://doi.org/10.3390/lubricants8030028
Kuhn E. Aspects of Self-Organization of Tribological Stressed Lubricating Greases. Lubricants. 2020; 8(3):28. https://doi.org/10.3390/lubricants8030028
Chicago/Turabian StyleKuhn, Erik. 2020. "Aspects of Self-Organization of Tribological Stressed Lubricating Greases" Lubricants 8, no. 3: 28. https://doi.org/10.3390/lubricants8030028
APA StyleKuhn, E. (2020). Aspects of Self-Organization of Tribological Stressed Lubricating Greases. Lubricants, 8(3), 28. https://doi.org/10.3390/lubricants8030028





