Simulation of a Steel-Aluminum Composite Material Subjected to Rolling Contact Fatigue
Abstract
1. Introduction
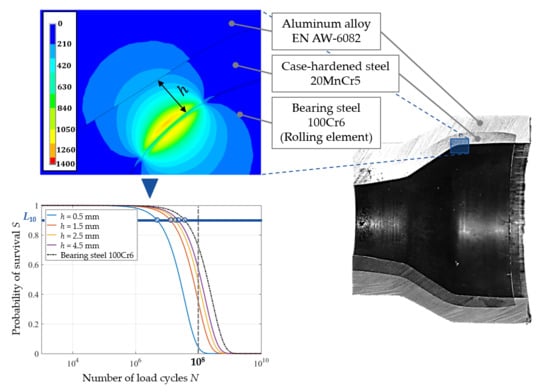
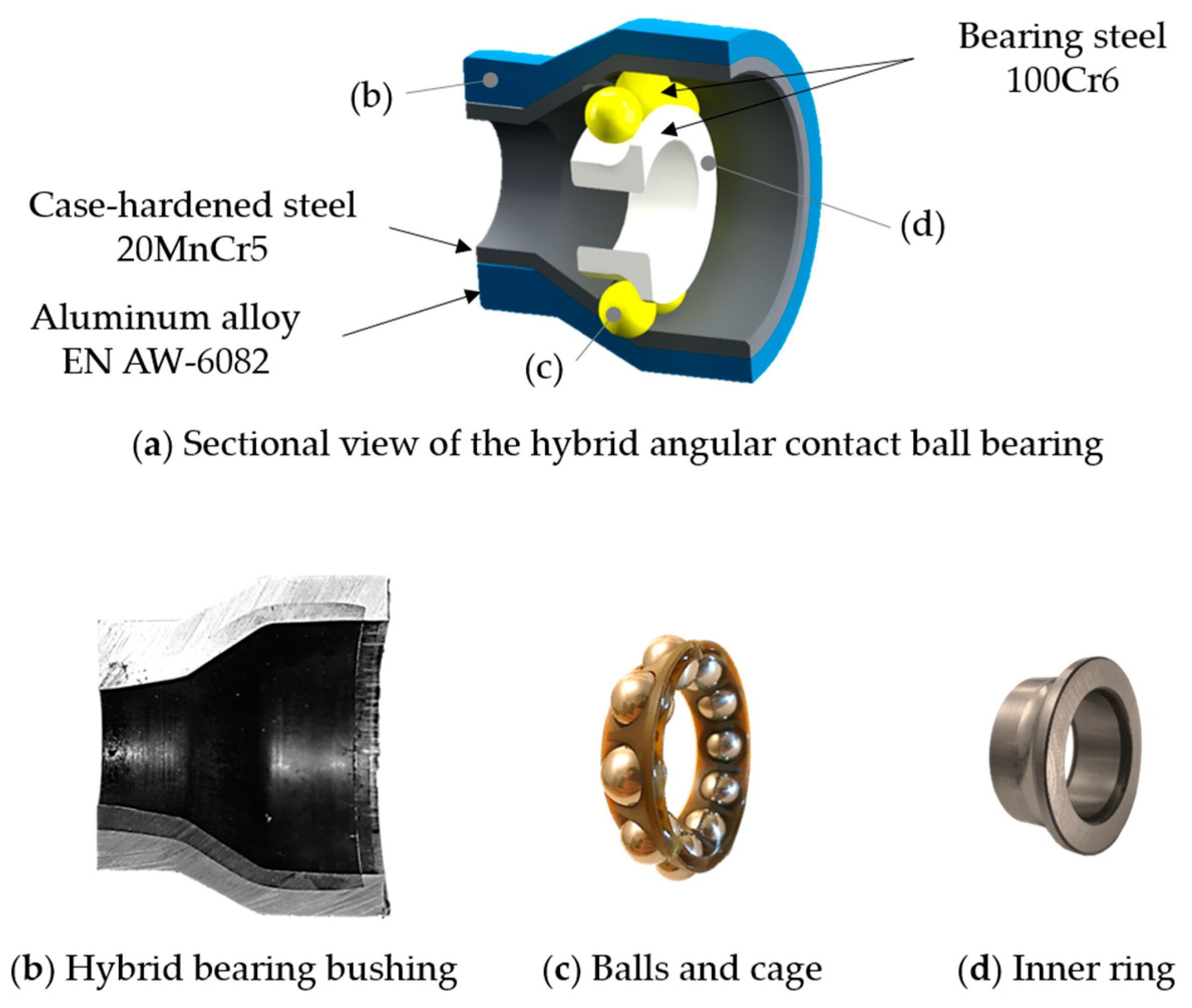
2. A hybrid Angular Contact Ball Bearing Bushing
3. Fatigue Life Calculation Based on the Ioannides–Harris Fatigue Life Model and the Dang Van Criterion
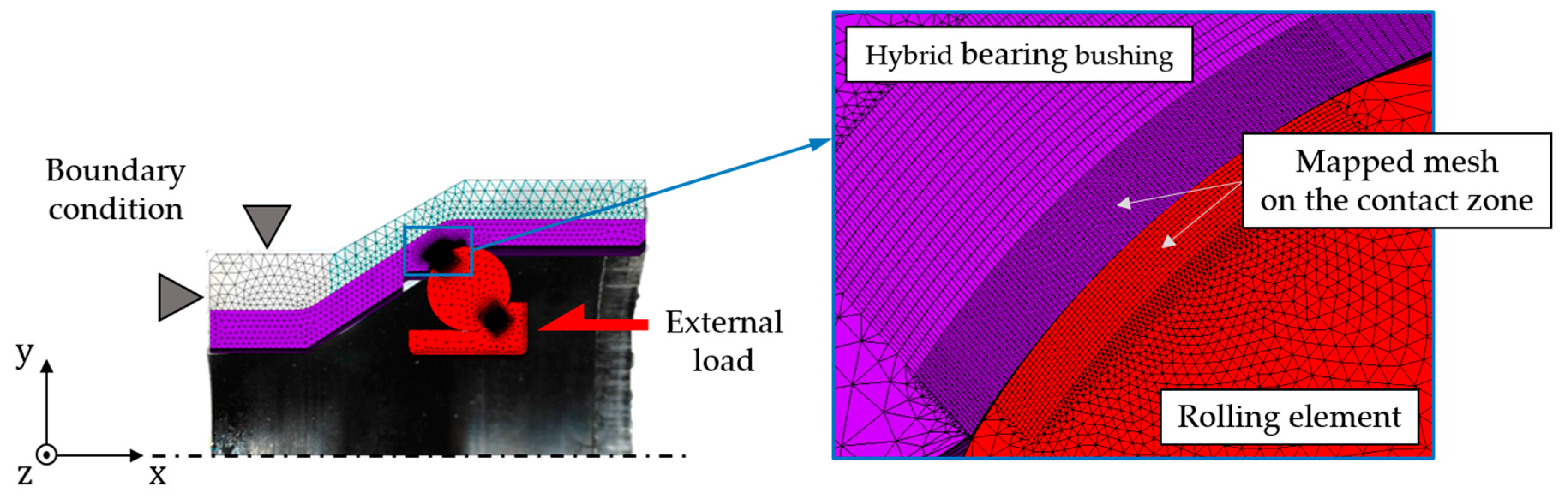
4. Modeling of the Hybrid Angular Contact Ball Bearing Bushing Based on Finite Element Method
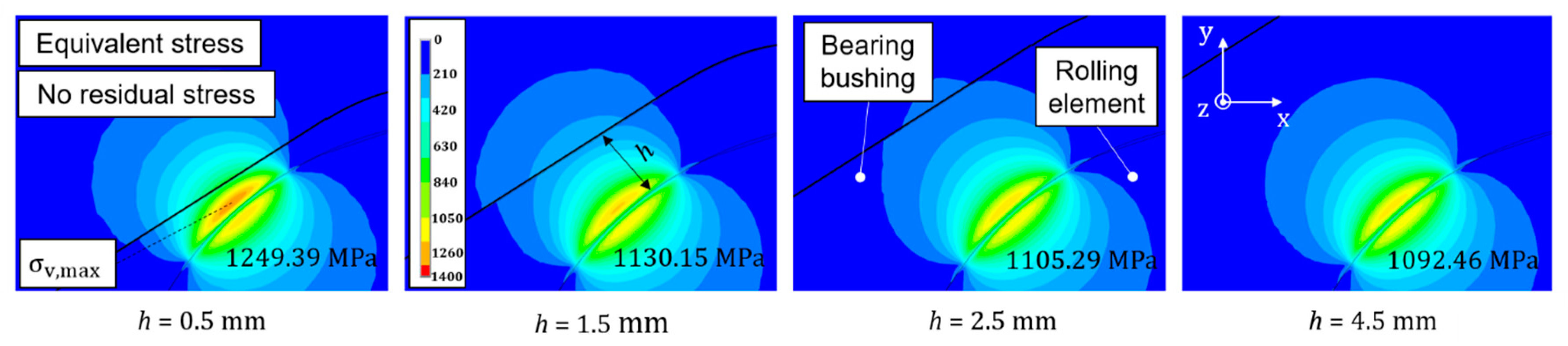
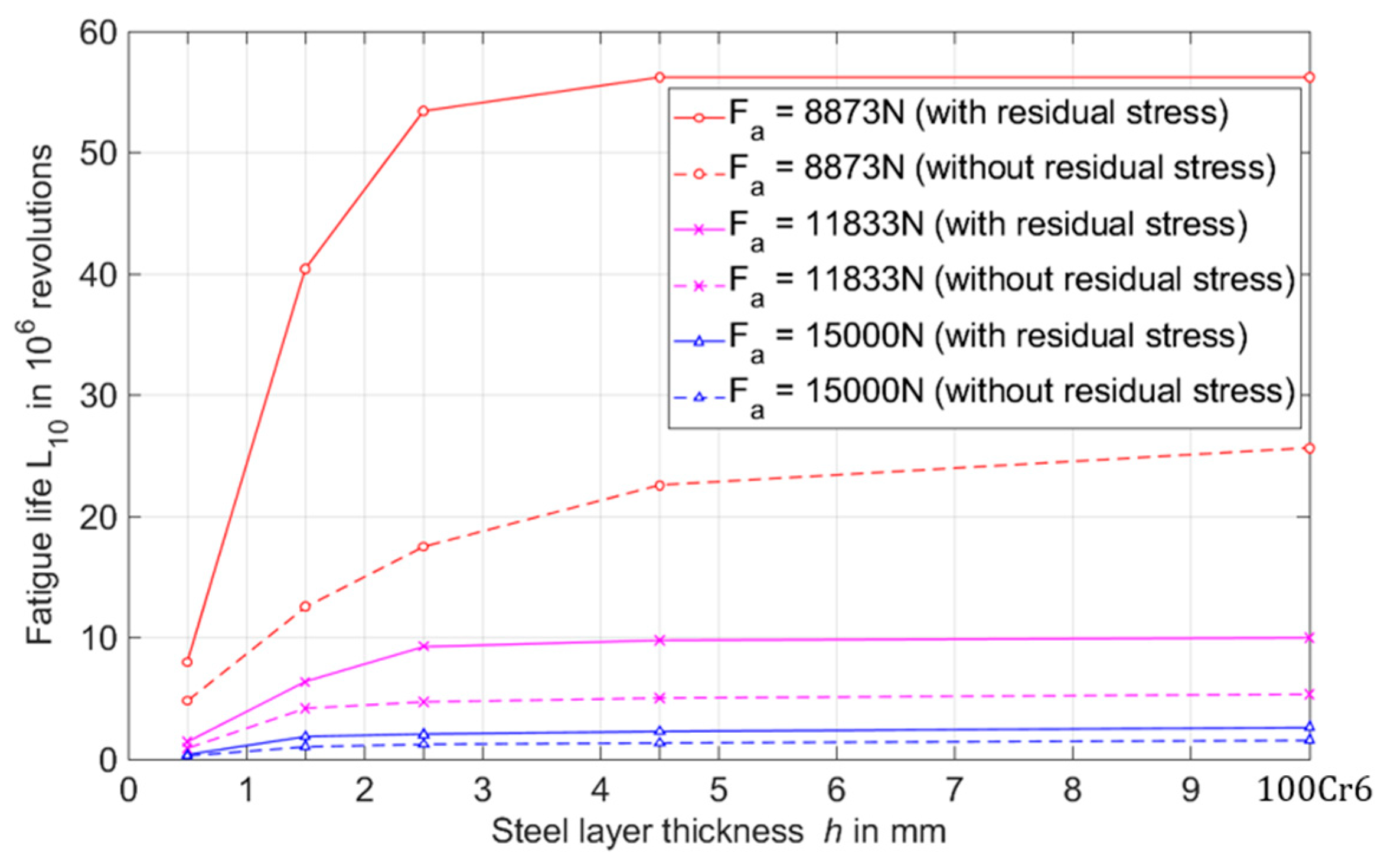
5. Results
6. Conclusions and Future Work
- steel layer thickness (for low values);
- external load (for low F values);
- compressive residual stresses (if they were considered).
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sadeghi, F.; Jalalahmadi, B.; Slack, T.S.; Raje, N.; Arakere, N.K. A Review of Rolling Contact Fatigue. ASME J. Tribol. 2009, 131, 041403. [Google Scholar] [CrossRef]
- Ringsberg, J.W. Life prediction of rolling contact fatigue crack initiation. Int. J. Fatigue 2001, 23, 575–586. [Google Scholar] [CrossRef]
- Franklin, F.J.; Widiyarta, I.; Kapoor, A. Computer simulation of wear and rolling contact fatigue. Wear 2001, 251, 949–955. [Google Scholar] [CrossRef]
- Radscheit, R.R. Laserstrahlfügen Von Aluminium Mit Stahl. Ph.D. Thesis, University of Bremen, Bremen, Germany, 1996. [Google Scholar]
- Bach, F.-W.; Beniyash, A.; Lau, K.; Versemann, R. Joining of steel aluminium hybrid structures with electron beam on atmosphere. Adv. Mater. Res. 2005, 6, 143–150. [Google Scholar] [CrossRef]
- Merklein, M.; Giera, A. Laser assisted Friction Stir Welding of drawable steel-aluminium tailored hybrids. Int. J. Mater. Form. 2008, 1, 1299–1302. [Google Scholar] [CrossRef]
- Merklein, M.; Johannes, M.; Lechner, M.; Kuppert, A. A review on tailored blanks production. J. Mater. Process. Technol. 2014, 214, 151–164. [Google Scholar] [CrossRef]
- Harris, T.A.; Kotzalas, M.N. Rolling Bearing Analysis, 5th ed.; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar] [CrossRef]
- Grittner, N.; von Senden gen, H.; Stelling, O.; Bormann, D.; Schimanski, K.; Nikolaus, M.; von Hehl, A.; Bach, F.-W.; Zoch, H.-W. Verbundstrangpressen von Titan-Aluminium-Verbindungen-Rod Extrusion of Titanium-Aluminium composites. Materialwissenschaft und Werkstofftechechnik 2009, 40, 901–906. [Google Scholar] [CrossRef]
- Coors, T.; Hwang, J.; Pape, F.; Poll, G. Theoretical investigations on the fatigue behavior of a tailored forming steel-aluminium bearing component. In Proceedings of the AIP Conference Proceedings 2113, Vitoria-Gasteiz, Spain, 8–10 May 2019. [Google Scholar]
- Thürer, S.E.; Uhe, J.; Golovko, O.; Bonk, C.; Bouguecha, A.; Klose, C.; Behrens, B.-A.; Maier, H.J. Co-extrusion of semi-finished aluminium-steel compounds. In Proceedings of the AIP Conference Proceedings 1896, Dublin, Ireland, 16 October 2017. [Google Scholar]
- Behrens, B.-A.; Bouguecha, A.; Frischkorn, C.; Huskic, A.; Chugreeva, A. Process routes for die forging of hybrid bevel gears and bearing bushings. In Proceedings of the AIP Conference Proceedings 1896, Dublin, Ireland, 16 October 2017. [Google Scholar]
- Shaw, L.L. Thermal residual stresses in plates and coatings composed of multi-layered and functionally graded materials. Compos. Part B Eng. 1998, 29, 199–210. [Google Scholar] [CrossRef]
- Dahlman, P.; Grunberg, F.; Jacobson, M. The influence of rake angle, cutting feed and cutting depth on residual stresses in hard turning. J. Mater. Process.Technol. 2004, 147, 181–184. [Google Scholar] [CrossRef]
- Ioannides, E.; Harris, T.A. A new fatigue life model for rolling bearings. J. Tribol. 1985, 107, 367–378. [Google Scholar] [CrossRef]
- Rolling Bearings—Dynamic Load Ratings and Rating Life; Deutsches Institut für Normung e.V.: Berlin, Germany, 2010.
- Lundberg, G.; Palmgren, A. Dynamic Capacity of Rolling Bearings; Acta Polytechnic Mechanical Engineering Series; Generalstabens Litografiska Anstalts Förlag: Stockholm, Sweden, 1947. [Google Scholar]
- Weibull, W. A Statistical Theory of the Strength of Materials; Ingeniörsvetenskapsakademiens handlingar; Generalstabens Litografiska Anstalts Förlag: Stockholm, Sweden, 1939. [Google Scholar]
- Ioannides, E.; Bergling, G.; Gabelli, A. An Analytical Formulation for the Life of Rolling Bearings. Mech. Eng. Acta Polytech. Scand. 1999, 137, 1–80. [Google Scholar]
- Dang Van, K. Sur la resistance a la fatigue des metaux. Sci. Tech. l’Armement 1973, 47, 479–496. [Google Scholar]
- Cerullo, M. Application of Dang Van criterion to rolling contact fatigue in wind turbine roller bearings. In Proceedings of the 13th International Conference on Fracture on Proceeding, Beijing, China, 16–21 June 2013; pp. 16–21. [Google Scholar]
- Gabelli, A.; Lai, J.; Lund, T.; Ryden, K.; Strandell, I.; Morales-Espejel, G.E. The fatigue limit of bearing steels–Part II: Characterization for life rating standards. Int. J. Fatigue 2012, 38, 169–180. [Google Scholar] [CrossRef]
- Lai, J.; Lund, T.; Ryden, K.; Gabelli, A.; Strandell, I. The fatigue limit of bearing steels–Part I: A pragmatic approach to predict very high cycle fatigue strength. Int. J. Fatigue 2012, 28, 155–168. [Google Scholar] [CrossRef]
- Santos, E.T.C.; Kida, K.; Rozwadowska, J. Fatigue Strength Improvement of AISI E52100 Bearing Steel by Induction Heating and Repeated Quenching. Mater. Sci. 2012, 47, 677–682. [Google Scholar] [CrossRef]
- Schwerdt, D. Schwingfestigkeit und Schädigungsmechanismen der Aluminium-legierungen EN AW-6056 und EN AW-6082 Sowie des Vergütungsstahls 42CrMo4 bei Sehr Hohen Schwingspielzahlen. Ph.D. Thesis, Darmstadt University of Technology, Darmstadt, Germany, 2011. [Google Scholar]
- Wusatowski, Z. Fundamentals of Rolling; Pergamon: Oxford, UK, 1969. [Google Scholar] [CrossRef]
- Chunjiang, Z.; Xiaokai, Y.; Qingxue, H.; Shidong, G.; Xin, G. Analysis on the load characteristics and coefficient of friction of angular contact ball bearing at high speed. Tribol. Int. 2015, 87, 50–56. [Google Scholar] [CrossRef]
- Karolczuk, A.; Macha, E. A review of critical plane orientations in multiaxial fatigue failure criteria of metallic materials. Int. J. Fracture 2005, 134, 267–304. [Google Scholar] [CrossRef]
- Pabst, A.; Tremmel, S.; Wartzack, S. Investigation of Residual Compressive Stresses in Rolling Bearing Components and their Impact on the Rating Life. In Proceedings of the STLE Annual Meeting & Exhibition, Lake Buena Vista, FL, USA, 18–24 May 2014. [Google Scholar]
- Voskamp, A. Microstructural Changes During Rolling Contact Fatigue-Metal Fatigue in the Subsurface Region of Deep Groove Ball Bearing Inner Rings. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1996. [Google Scholar]
- Pape, F.; Neubauer, T.; Maiß, O.; Denkena, B.; Poll, G. Influence of Residual Stresses Introduced by Manufacturing Processes on Bearing Endurance Time. Tribol. Lett. 2017, 65, 70. [Google Scholar] [CrossRef]




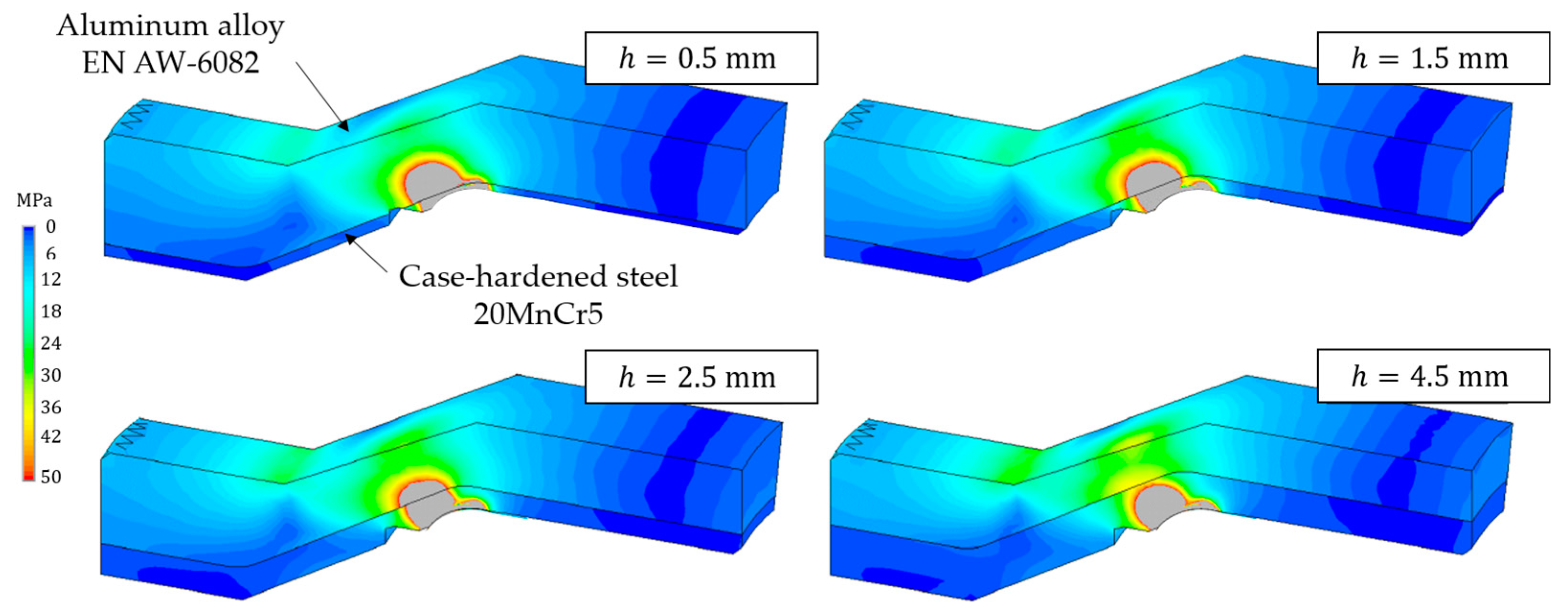

| Material | Young’s Modulus | Poisson’s Ratio | Density | |
|---|---|---|---|---|
| 100Cr6 | 210 GPa | 0.28 | 7.85 | 350 MPa |
| 20MnCr5 | 210 GPa | 0.3 | 7.75 | 290 MPa |
| EN AW-6082 | 69 GPa | 0.33 | 2.7 | 50 MPa |
| Steel Layer Thickness h in mm | 0.5 | 1.5 | 2.5 | 4.5 | 100Cr6 |
|---|---|---|---|---|---|
| Axial load in N ( value) | 8873 (4) | 8873 (4) | 8873 (4) | 8873 (4) | 8873 (4) |
| 11833 (3) | 11833 (3) | 11833 (3) | 11833 (3) | 11833 (3) | |
| 15000 (2.5) | 15000 (2.5) | 15000 (2.5) | 15000 (2.5) | 15000 (2.5) |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, J.-I.; Coors, T.; Pape, F.; Poll, G. Simulation of a Steel-Aluminum Composite Material Subjected to Rolling Contact Fatigue. Lubricants 2019, 7, 109. https://doi.org/10.3390/lubricants7120109
Hwang J-I, Coors T, Pape F, Poll G. Simulation of a Steel-Aluminum Composite Material Subjected to Rolling Contact Fatigue. Lubricants. 2019; 7(12):109. https://doi.org/10.3390/lubricants7120109
Chicago/Turabian StyleHwang, Jae-Il, Timm Coors, Florian Pape, and Gerhard Poll. 2019. "Simulation of a Steel-Aluminum Composite Material Subjected to Rolling Contact Fatigue" Lubricants 7, no. 12: 109. https://doi.org/10.3390/lubricants7120109
APA StyleHwang, J.-I., Coors, T., Pape, F., & Poll, G. (2019). Simulation of a Steel-Aluminum Composite Material Subjected to Rolling Contact Fatigue. Lubricants, 7(12), 109. https://doi.org/10.3390/lubricants7120109