A Fast Numerical Approach for Investigating Adhesion Strength in Fibrillar Structures: Impact of Buckling and Roughness
Abstract
1. Introduction
2. Materials and Methods
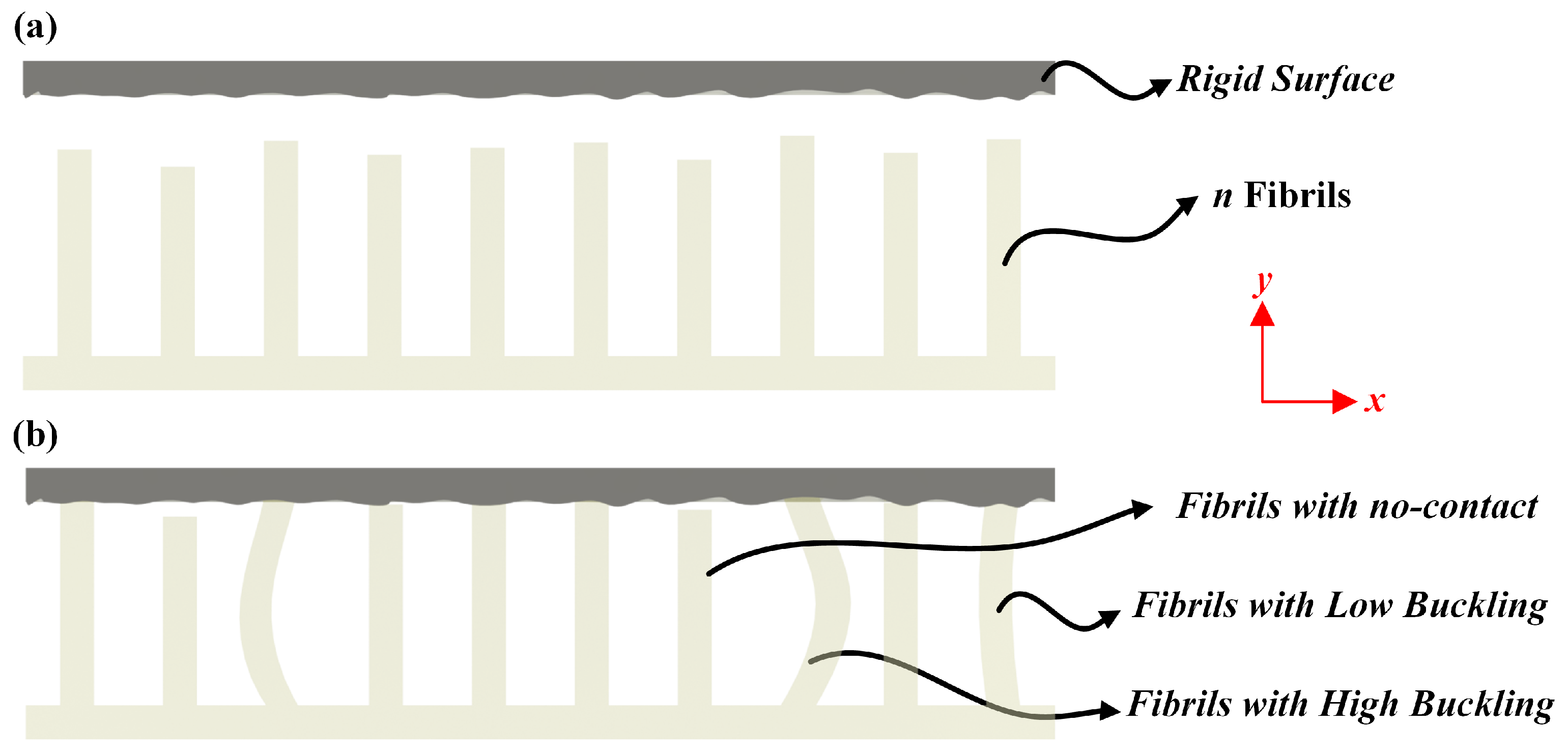
2.1. Problem Statement and Theory
2.1.1. The Loading Process
2.1.2. The Unloading Process
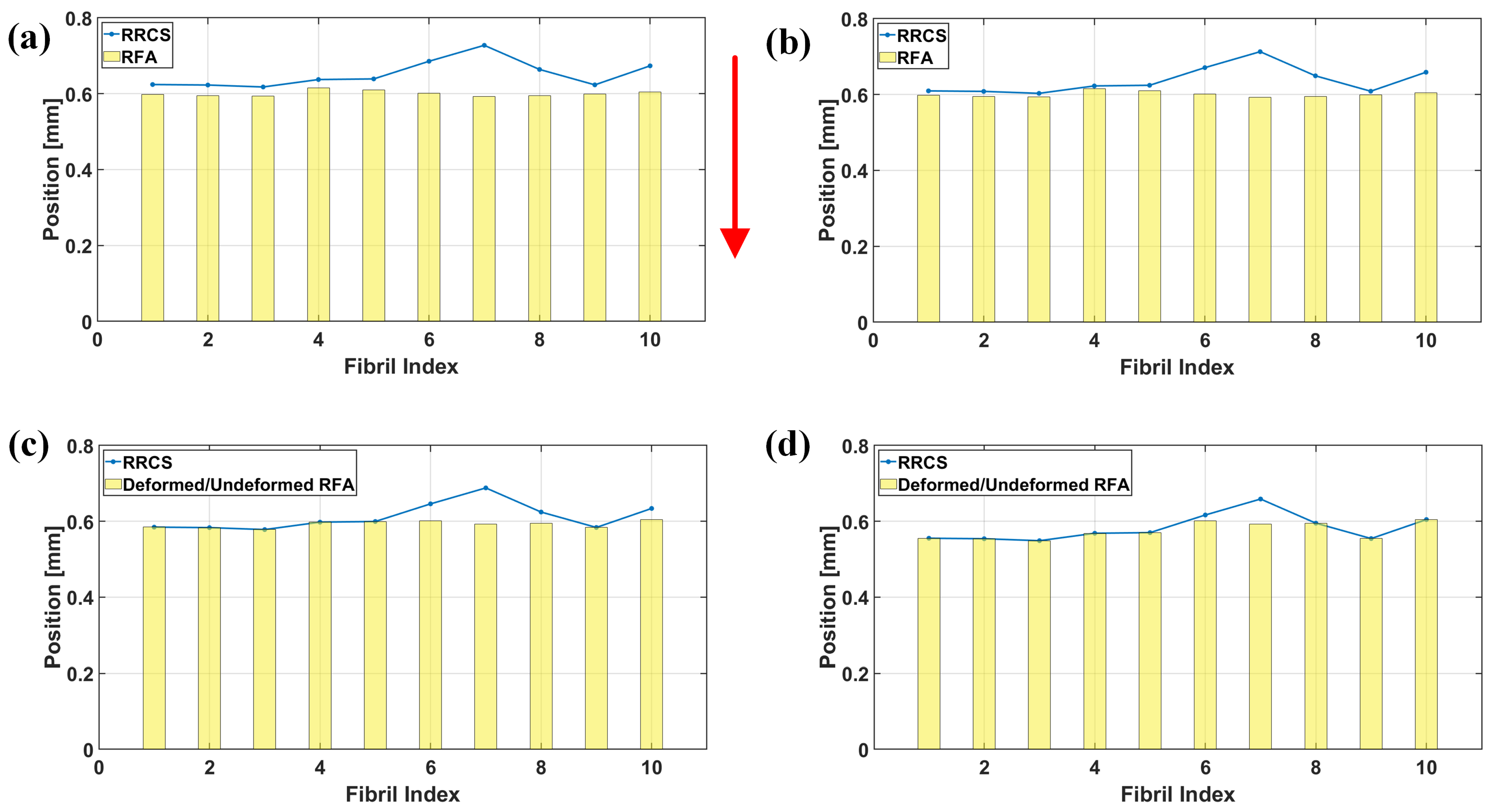
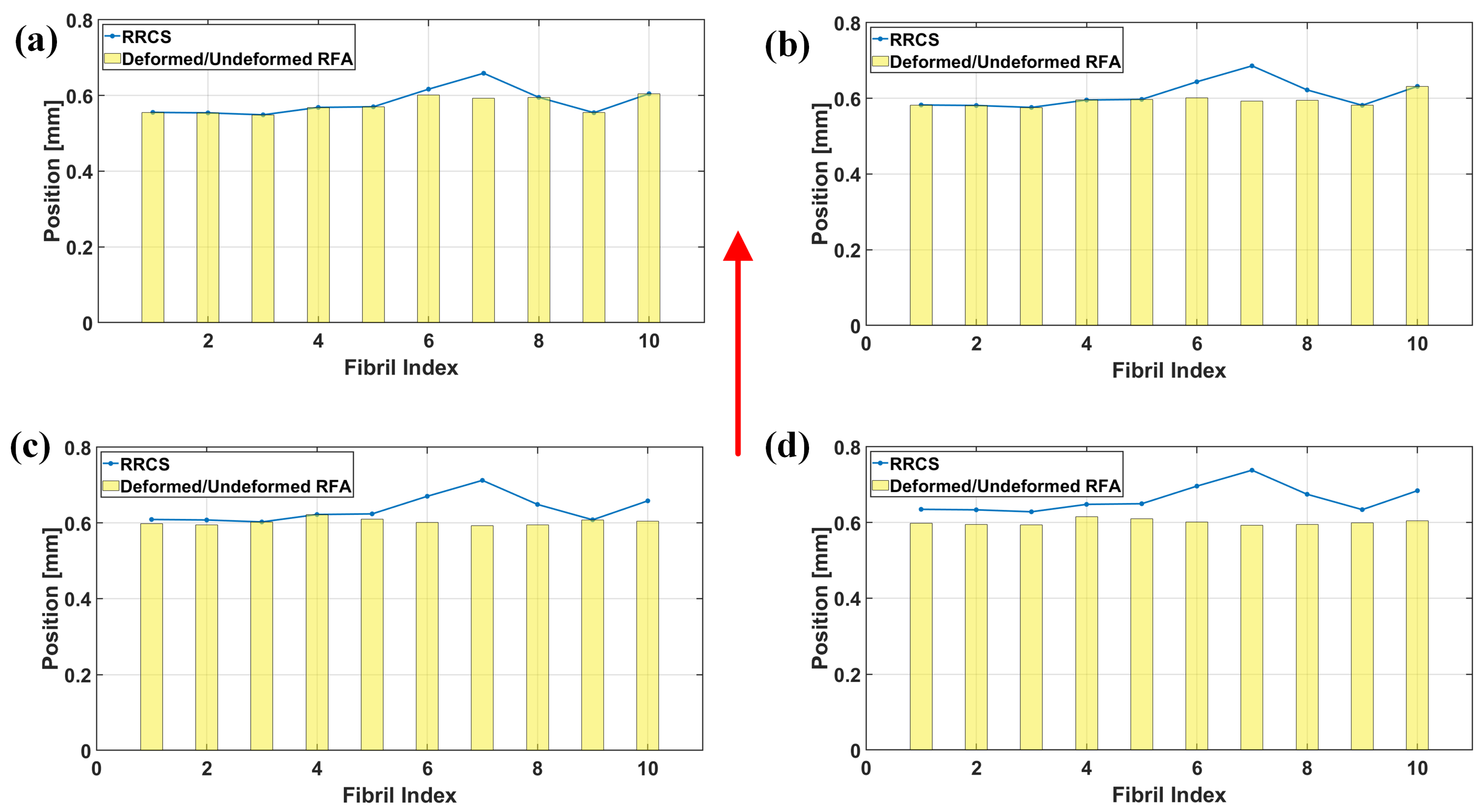
2.1.3. Roughness
2.2. Numerical Computation
| Algorithm 1 Loading Phase |
|
- No Buckling Condition: In this state, a fibril is not buckled. Under this condition, the adhesion force remains as described in Equation (4).
- Low Buckling Condition: In the low buckling state, the adhesion force of the ith fibril decreases to 75% of its value in the unbuckled state. This reduction is based on experimental observations of fibril adhesion forces [26].
- High Buckling Condition: In contrast, under high buckling conditions, the adhesion force of the fibril is reduced to 25% of its unbuckled value. This adjustment reflects experimental findings that indicate significant decreases in adhesion forces under severe buckling conditions, with values approximating 30% in some studies [28,29]. For the purposes of simulation, a conservative estimate lower than the observed value is used.
| Algorithm 2 Unloading Phase |
|
Multiple Simulations and Normalization
- Maximum Adhesion Force Normalization: The maximum adhesion force is normalized against the adhesion force of a fibril array with no variance in fibril height or surface roughness of the counter surface. This normalization is performed using the single fibril adhesion value given by Equation (4) () and the number of fibrils (n) in the array. This approach allows for a clear distinction of the effects of height variance in the fibril array or roughness in the rigid counter surface on the adhesion strength.
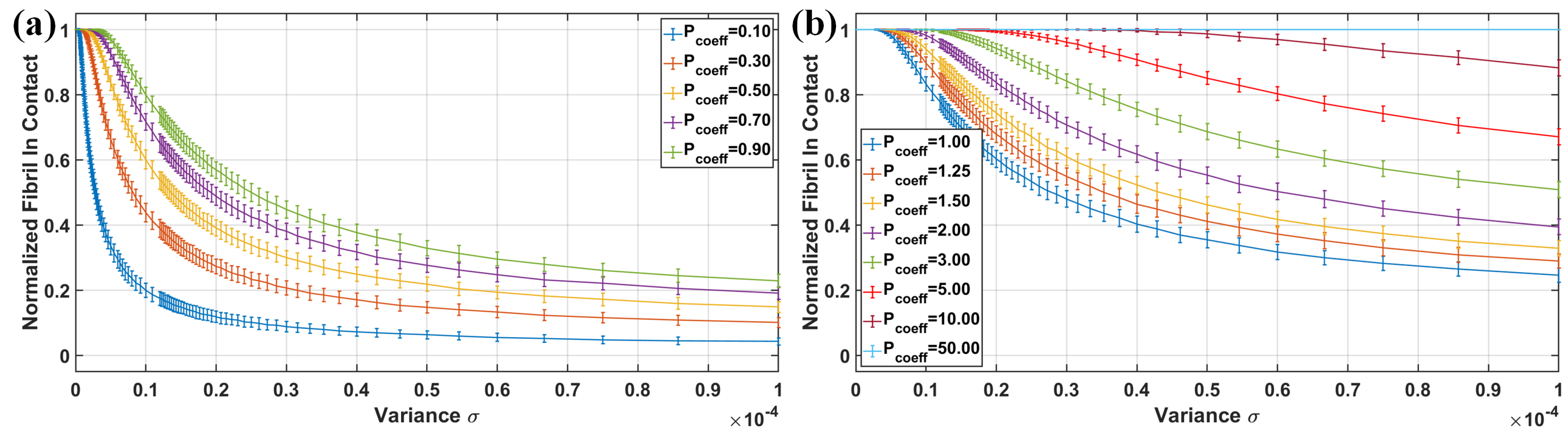
- Number of Fibrils in Contact () and Number of Buckled Fibrils () Normalization: These metrics are normalized to the total number of fibrils in the array (n). This normalization enables clear observation of the impact of the pre–load factor on the number of fibrils in contact and buckled fibrils.
- Relative Displacement Normalization: The relative displacement of the counter surface () is normalized to the maximum elongation of the fibril, which is calculated assuming no variation in fibril height or counter surface roughness before contact loss. This maximum elongation occurs during the unloading phase due to the adhesive interaction between the fibril and the counter surface. The maximum elongation is determined using Equation (4) and the stiffness of the fibril () under ideal conditions, where the pre–load is below the critical value and there are no height variations or surface roughness. This normalization procedure ensures that the elongation of a rough fibril array is accurately related to the maximum deformation experienced by a smooth fibril array in contact with a smooth, rigid surface.
3. Results
3.1. Adhesion between a Rigid Surface and a Rough Fibril Array
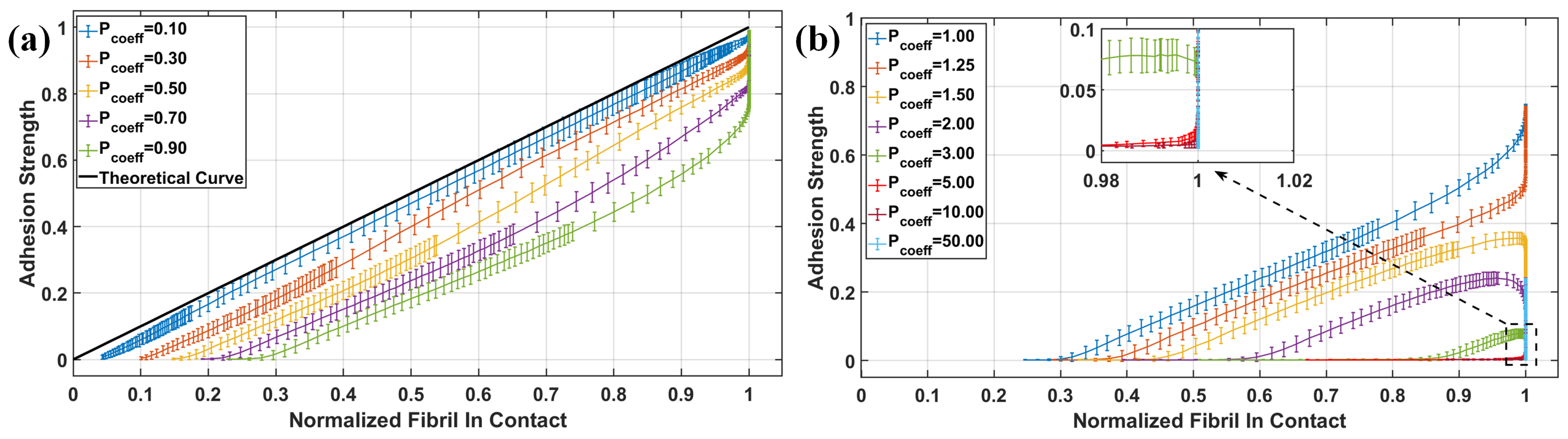
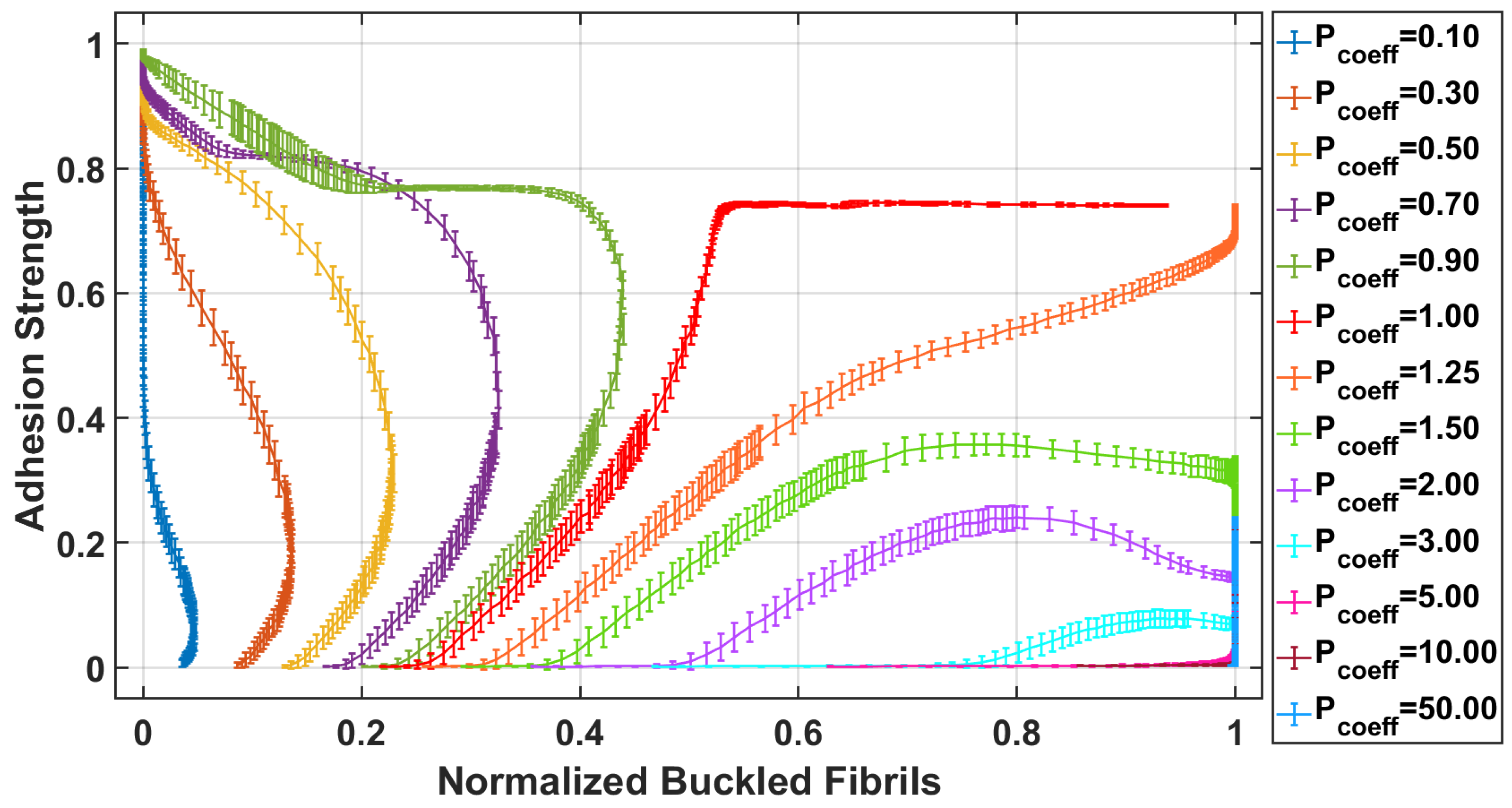
3.1.1. Effect of Number of Fibrils on the Adhesive Strength
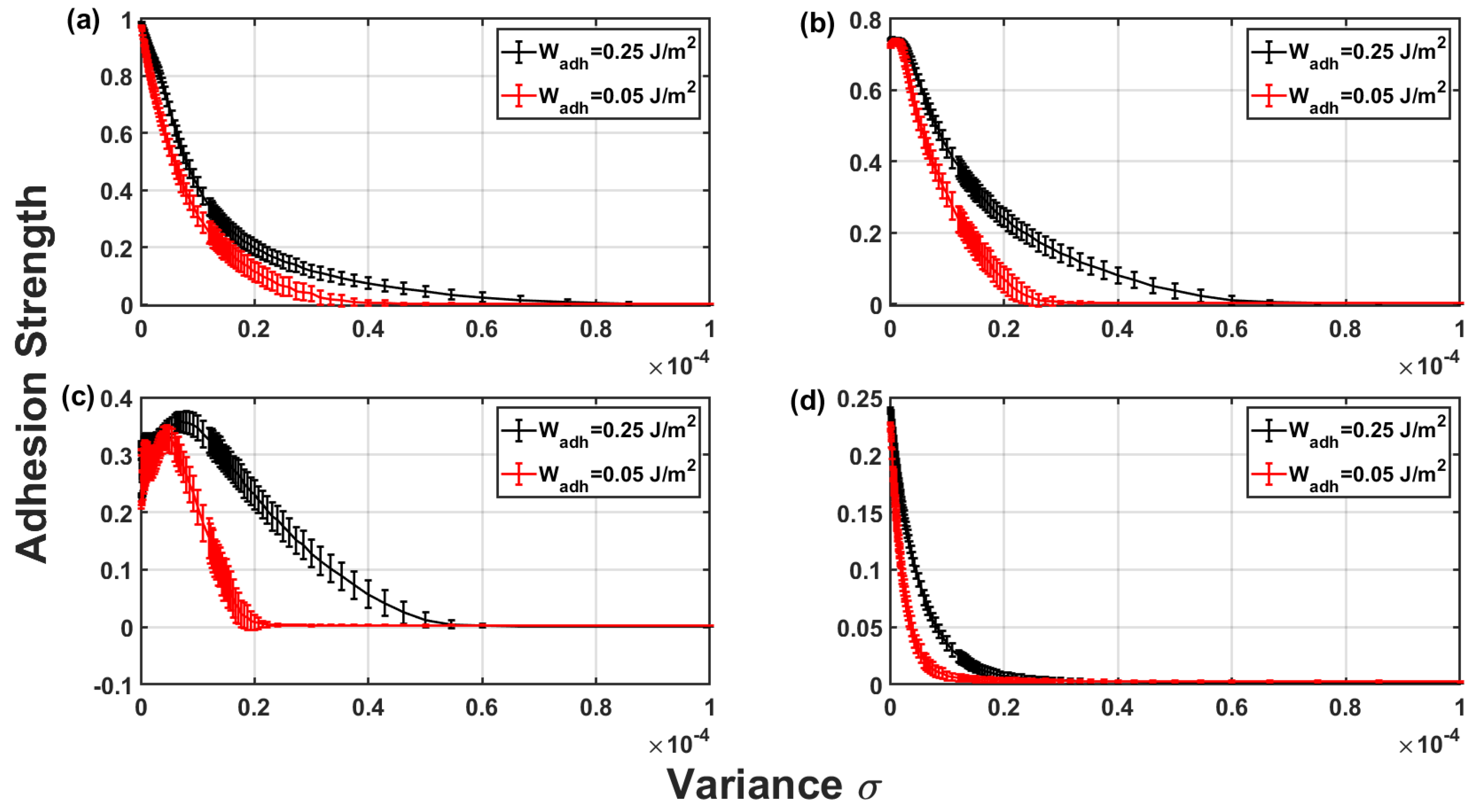
3.1.2. Effect of Work of Adhesion on the Adhesive Strength
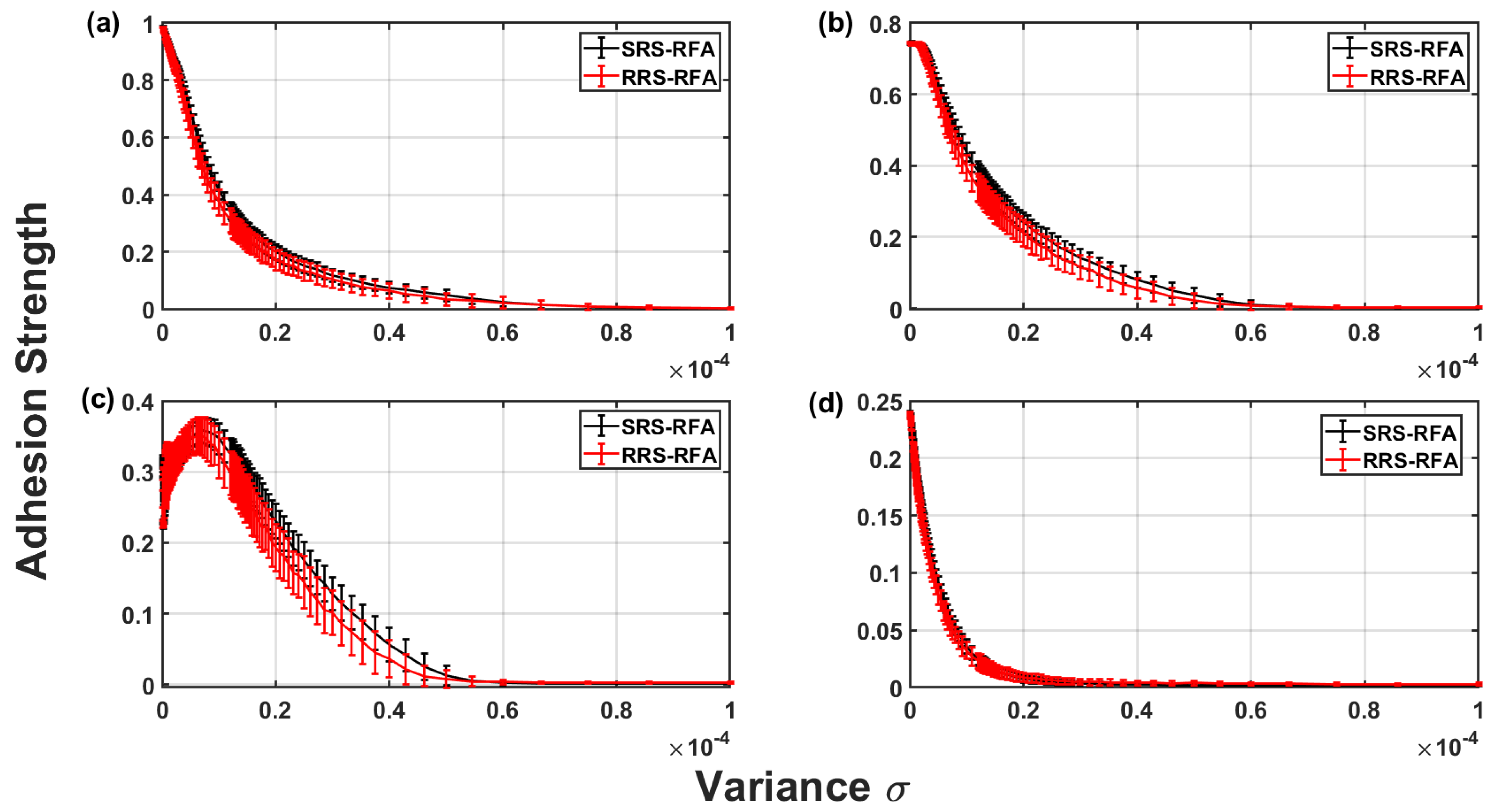
3.1.3. Effect of Both Surfaces Being Rough on the Adhesive Strength
4. Discussion
5. Conclusions
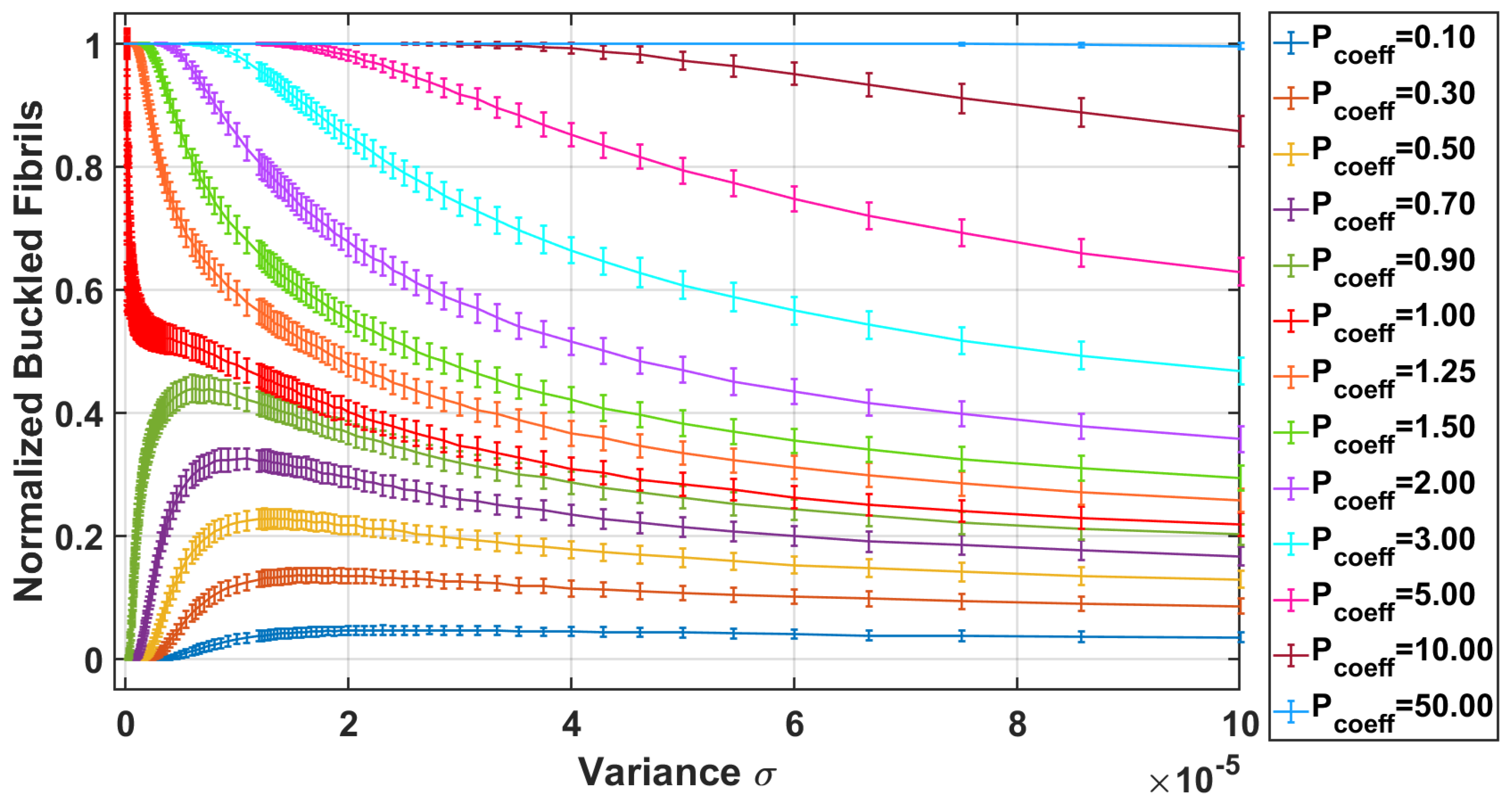
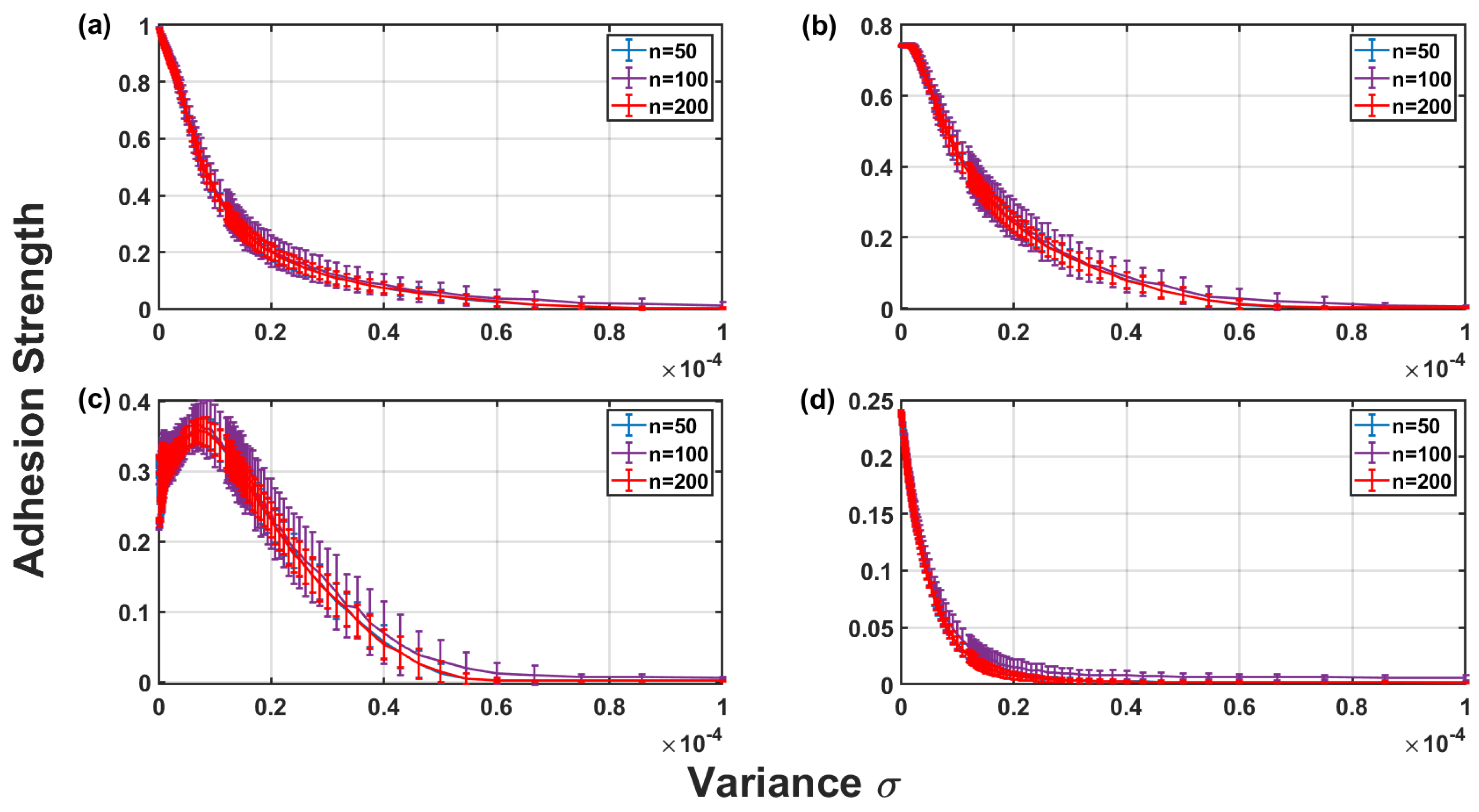
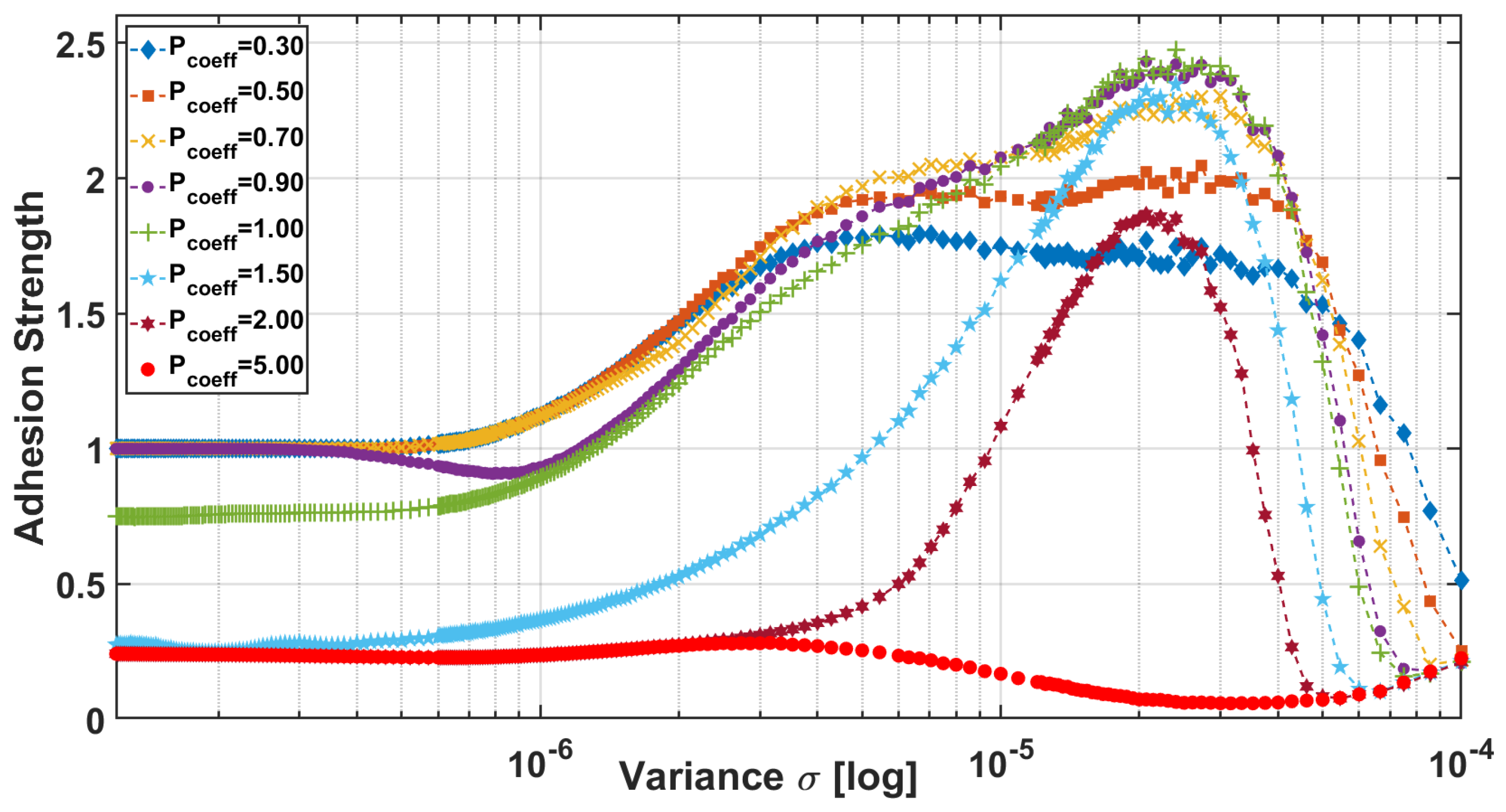
- Effect of Roughness on Adhesion: The adhesion strength exhibits an exponential decay with increasing roughness parameters. This relationship is consistent across various pre–loads. This finding aligns with existing studies which show that the adhesion strength decreases in a similar manner with respect to roughness. The numerical model effectively captured the influence of surface roughness on adhesion strength.
- Role of Fibril Number: The number of fibrils in the array has a negligible effect on normalized adhesion strength when considering a normal distribution of fibril heights. This finding contrasts with previous studies that incorporated backing layer effects and structural coupling [33,42,47], indicating the importance of model assumptions in predicting adhesion behavior.
- Impact of Pre–Load on Adhesion Strength: Increasing the pre–load enhances the adhesion strength up to a certain threshold, beyond which the fibrils begin to buckle. The critical pre–load is directly related to the onset of buckling, and exceeding this value significantly reduces adhesion due to the instability introduced by buckling.
Supplementary Materials
Funding
Data Availability Statement
Conflicts of Interest
References
- Persson, B.; Tosatti, E. The effect of surface roughness on the adhesion of elastic solids. J. Chem. Phys. 2001, 115, 5597–5610. [Google Scholar] [CrossRef]
- Persson, B. Adhesion between an elastic body and a randomly rough hard surface. Eur. Phys. J. E 2002, 8, 385–401. [Google Scholar] [CrossRef] [PubMed]
- Peressadko, A.; Hosoda, N.; Persson, B. Influence of surface roughness on adhesion between elastic bodies. Phys. Rev. Lett. 2005, 95, 124301. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Williamson, J.P. Contact of nominally flat surfaces. Proc. R. Soc. Lond. Ser. Math. Phys. Sci. 1966, 295, 300–319. [Google Scholar]
- Zhang, S.; Li, D.; Liu, Y. Friction behavior of rough surfaces on the basis of contact mechanics: A review and prospects. Micromachines 2022, 13, 1907. [Google Scholar] [CrossRef]
- Johnson, K.L.; Kendall, K.; Roberts, A. Surface energy and the contact of elastic solids. Proc. R. Soc. Lond. A Math. Phys. Sci. 1971, 324, 301–313. [Google Scholar]
- Greenwood, J.A. Contact of Rough Surfaces: The Greenwood and Williamson/Tripp, Fuller and Tabor Theories. In Encyclopedia of Tribology; Wang, Q.J., Chung, Y.W., Eds.; Springer: Boston, MA, USA, 2013; pp. 517–522. [Google Scholar]
- Kim, J.K.; Varenberg, M. Contact splitting in dry adhesion and friction: Reducing the influence of roughness. Beilstein J. Nanotechnol. 2019, 10, 1–8. [Google Scholar] [CrossRef]
- Kamperman, M.; Kroner, E.; del Campo, A.; McMeeking, R.M.; Arzt, E. Functional adhesive surfaces with “gecko” effect: The concept of contact splitting. Adv. Eng. Mater. 2010, 12, 335–348. [Google Scholar] [CrossRef]
- He, X.; Li, Q.; Popov, V.L. Simulation of adhesive contact of soft microfibrils. Lubricants 2020, 8, 94. [Google Scholar] [CrossRef]
- Boesel, L.F.; Greiner, C.; Arzt, E.; Del Campo, A. Gecko-inspired surfaces: A path to strong and reversible dry adhesives. Adv. Mater. 2010, 22, 2125–2137. [Google Scholar] [CrossRef]
- Kasem, H.; Varenberg, M. Effect of counterface roughness on adhesion of mushroom-shaped microstructure. J. R. Soc. Interface 2013, 10, 20130620. [Google Scholar] [CrossRef] [PubMed]
- Barreau, V.; Hensel, R.; Guimard, N.K.; Ghatak, A.; McMeeking, R.M.; Arzt, E. Fibrillar elastomeric micropatterns create tunable adhesion even to rough surfaces. Adv. Funct. Mater. 2016, 26, 4687–4694. [Google Scholar] [CrossRef]
- Autumn, K.; Gravish, N. Gecko adhesion: Evolutionary nanotechnology. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2008, 366, 1575–1590. [Google Scholar] [CrossRef] [PubMed]
- Eisenhaure, J.; Kim, S. A review of the state of dry adhesives: Biomimetic structures and the alternative designs they inspire. Micromachines 2017, 8, 125. [Google Scholar] [CrossRef]
- He, B.; Chen, W.; Jane Wang, Q. Surface texture effect on friction of a microtextured poly(dimethylsiloxane) (PDMS). Tribol. Lett. 2008, 31, 187–197. [Google Scholar] [CrossRef]
- Greiner, C.; del Campo, A.; Arzt, E. Adhesion of bioinspired micropatterned surfaces: Effects of pillar radius, aspect ratio, and preload. Langmuir 2007, 23, 3495–3502. [Google Scholar] [CrossRef] [PubMed]
- Micciché, M.; Arzt, E.; Kroner, E. Single macroscopic pillars as model system for bioinspired adhesives: Influence of tip dimension, aspect ratio, and tilt angle. ACS Appl. Mater. Interfaces 2014, 6, 7076–7083. [Google Scholar] [CrossRef] [PubMed]
- Del Campo, A.; Greiner, C.; Arzt, E. Contact shape controls adhesion of bioinspired fibrillar surfaces. Langmuir 2007, 23, 10235–10243. [Google Scholar] [CrossRef] [PubMed]
- Porwal, P.K.; Hui, C.Y. Strength statistics of adhesive contact between a fibrillar structure and a rough substrate. J. R. Soc. Interface 2008, 5, 441–448. [Google Scholar] [CrossRef]
- Li, Q.; Popov, V.L. Adhesive contact of rough brushes. Beilstein J. Nanotechnol. 2018, 9, 2405–2412. [Google Scholar] [CrossRef] [PubMed]
- He, X.; Li, Q.; Popov, V.L. Strength of adhesive contact between a rough fibrillar structure and an elastic body: Influence of fibrillar stiffness. J. Adhes. 2022, 98, 1820–1833. [Google Scholar] [CrossRef]
- Zhang, Y.; He, L. Preload-responsive adhesion of microfibre arrays to rough surfaces. Appl. Math. Mech. 2017, 38, 155–160. [Google Scholar] [CrossRef]
- Hu, H.; Tian, H.; Gao, Y.; Wan, Z.; Wang, L.; Xu, H.; Wang, C.; Shao, J.; Zheng, Z. Revisiting the contact splitting hypothesis: An effective route for enhancing adhesion on rough surface. J. Mech. Phys. Solids 2023, 170, 105121. [Google Scholar] [CrossRef]
- Kendall, K. The adhesion and surface energy of elastic solids. J. Phys. D Appl. Phys. 1971, 4, 1186. [Google Scholar] [CrossRef]
- Paretkar, D.R.; Bartlett, M.D.; McMeeking, R.; Crosby, A.J.; Arzt, E. Buckling of an adhesive polymeric micropillar. J. Adhes. 2013, 89, 140–158. [Google Scholar] [CrossRef]
- Long, R.; Hui, C.Y. The effect of preload on the pull-off force in indentation tests of microfibre arrays. Proc. R. Soc. A Math. Phys. Eng. Sci. 2009, 465, 961–981. [Google Scholar] [CrossRef]
- Purtov, J.; Frensemeier, M.; Kroner, E. Switchable adhesion in vacuum using bio-inspired dry adhesives. ACS Appl. Mater. Interfaces 2015, 7, 24127–24135. [Google Scholar] [CrossRef] [PubMed]
- Isla, P.Y.; Kroner, E. A novel bioinspired switchable adhesive with three distinct adhesive states. Adv. Funct. Mater. 2015, 25, 2444–2450. [Google Scholar] [CrossRef] [PubMed]
- Tinnemann, V.; Arzt, E.; Hensel, R. Switchable double-sided micropatterned adhesives for selective fixation and detachment. J. Mech. Phys. Solids 2019, 123, 20–27. [Google Scholar] [CrossRef]
- Timoshenko, S.; Goodier, J.N. Theoy of Elasticity; McGraw Hill Education: New York, NY, USA, 1986. [Google Scholar]
- Eray, T.; Sümer, B.; Koc, I.M. Analytical and experimental analysis on frictional dynamics of a single elastomeric pillar. Tribol. Int. 2016, 100, 293–305. [Google Scholar] [CrossRef]
- Koç, I.M.; Eray, T. Modeling frictional dynamics of a visco-elastic pillar rubbed on a smooth surface. Tribol. Int. 2018, 127, 187–199. [Google Scholar] [CrossRef]
- Maugis, D. Contact, Adhesion and Rupture of Elastic Solids; Springer Science & Business Media: Berlin, Germany, 2013; Volume 130. [Google Scholar]
- Aksak, B.; Murphy, M.P.; Sitti, M. Adhesion of biologically inspired vertical and angled polymer microfiber arrays. Langmuir 2007, 23, 3322–3332. [Google Scholar] [CrossRef] [PubMed]
- Ciavarella, M.; Papangelo, A. On the sensitivity of adhesion between rough surfaces to asperity height distribution. Phys. Mesomech. 2018, 21, 59–66. [Google Scholar] [CrossRef]
- Wei, Z.; He, M.F.; Zhao, Y.P. The effects of roughness on adhesion hysteresis. J. Adhes. Sci. Technol. 2010, 24, 1045–1054. [Google Scholar] [CrossRef]
- Eray, T. Tunable adhesion of an elastic pillar by pressurizing inner cavity. Proc. Inst. Mech. Eng. Part J Eng. Tribol. 2022, 236, 541–551. [Google Scholar] [CrossRef]
- Yu, Y.; Sanchez, D.; Lu, N. Work of adhesion/separation between soft elastomers of different mixing ratios. J. Mater. Res. 2015, 30, 2702–2712. [Google Scholar] [CrossRef]
- Ciavarella, M.; Papangelo, A. A generalized Johnson parameter for pull-off decay in the adhesion of rough surfaces. Phys. Mesomech. 2018, 21, 67–75. [Google Scholar] [CrossRef]
- Guidoni, G.; Schillo, D.; Hangen, U.; Castellanos, G.; Arzt, E.; McMeeking, R.; Bennewitz, R. Discrete contact mechanics of a fibrillar surface with backing layer interactions. J. Mech. Phys. Solids 2010, 58, 1571–1581. [Google Scholar] [CrossRef]
- Bacca, M.; Booth, J.A.; Turner, K.L.; McMeeking, R.M. Load sharing in bioinspired fibrillar adhesives with backing layer interactions and interfacial misalignment. J. Mech. Phys. Solids 2016, 96, 428–444. [Google Scholar] [CrossRef]
- Amato, L.; Keller, S.S.; Heiskanen, A.; Dimaki, M.; Emnéus, J.; Boisen, A.; Tenje, M. Fabrication of high-aspect ratio SU-8 micropillar arrays. Microelectron. Eng. 2012, 98, 483–487. [Google Scholar] [CrossRef]
- Spolenak, R.; Gorb, S.; Arzt, E. Adhesion design maps for bio-inspired attachment systems. Acta Biomater. 2005, 1, 5–13. [Google Scholar] [CrossRef] [PubMed]
- Long, R.; Hui, C.Y.; Kim, S.; Sitti, M. Modeling the soft backing layer thickness effect on adhesion of elastic microfiber arrays. J. Appl. Phys. 2008, 104. [Google Scholar] [CrossRef]
- Aksak, B.; Hui, C.Y.; Sitti, M. The effect of aspect ratio on adhesion and stiffness for soft elastic fibres. J. R. Soc. Interface 2011, 8, 1166–1175. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.; Sitti, M.; Hui, C.Y.; Long, R.; Jagota, A. Effect of backing layer thickness on adhesion of single-level elastomer fiber arrays. Appl. Phys. Lett. 2007, 91. [Google Scholar] [CrossRef]
- Greiner, C.; Buhl, S.; del Campo, A.; Arzt, E. Experimental parameters controlling adhesion of biomimetic fibrillar surfaces. J. Adhes. 2009, 85, 646–661. [Google Scholar] [CrossRef]
- Cheng, Y.; Peng, Z.; Chen, S. A Theoretical Model of Enhanced Adhesion of Bioinspired Micropillar Arrayed Surfaces. J. Mech. Phys. Solids 2024, 186, 105592. [Google Scholar] [CrossRef]
- Argatov, I.; Li, Q.; Popov, V.L. Cluster of the Kendall-type adhesive microcontacts as a simple model for load sharing in bioinspired fibrillar adhesives. Arch. Appl. Mech. 2019, 89, 1447–1472. [Google Scholar] [CrossRef]
- Booth, J.A.; Bacca, M.; McMeeking, R.M.; Foster, K.L. Benefit of backing-layer compliance in fibrillar adhesive patches—Resistance to peel propagation in the presence of interfacial misalignment. Adv. Mater. Interfaces 2018, 5, 1800272. [Google Scholar] [CrossRef]
- Jiang, Y.; Grierson, D.S.; Turner, K.T. Flat punch adhesion: Transition from fracture-based to strength-limited pull-off. J. Phys. D Appl. Phys. 2014, 47, 325301. [Google Scholar] [CrossRef]
- Linghu, C.; Yang, X.; Liu, Y.; Li, D.; Gao, H.; Hsia, K.J. Mechanics of shape-locking-governed R2G adhesion with shape memory polymers. J. Mech. Phys. Solids 2023, 170, 105091. [Google Scholar] [CrossRef]
- Hui, C.Y.; Glassmaker, N.; Tang, T.; Jagota, A. Design of biomimetic fibrillar interfaces: 2. Mechanics of enhanced adhesion. J. R. Soc. Interface 2004, 1, 35–48. [Google Scholar] [CrossRef]
- Linghu, C.; Liu, Y.; Yang, X.; Li, D.; Tan, Y.Y.; Mohamed Hafiz, M.H.B.; Rohani, M.F.B.; Du, Z.; Su, J.; Li, Y.; et al. Fibrillar adhesives with unprecedented adhesion strength, switchability and scalability. Natl. Sci. Rev. 2024, nwae106. [Google Scholar] [CrossRef]
- Yao, H.; Gao, H. Mechanics of robust and releasable adhesion in biology: Bottom–up designed hierarchical structures of gecko. J. Mech. Phys. Solids 2006, 54, 1120–1146. [Google Scholar] [CrossRef]
- Tang, T.; Hui, C.Y.; Glassmaker, N.J. Can a fibrillar interface be stronger and tougher than a non-fibrillar one? J. R. Soc. Interface 2005, 2, 505–516. [Google Scholar] [CrossRef]
- Varenberg, M.; Gorb, S. Shearing of fibrillar adhesive microstructure: Friction and shear-related changes in pull-off force. J. R. Soc. Interface 2007, 4, 721–725. [Google Scholar] [CrossRef]
- Linghu, C.; Du, Z.; Sun, Y.; Chen, W.; Hsia, K.J. On shear adhesion of adhesive fibrils. Extrem. Mech. Lett. 2023, 64, 102092. [Google Scholar] [CrossRef]
- Abusomwan, U.; Sitti, M. Effect of retracton speed on adhesion of elastomer fibrillar structures. Appl. Phys. Lett. 2012, 101, 211907. [Google Scholar] [CrossRef]
- Afferrante, L.; Violano, G.; Carbone, G. Exploring the dynamics of viscoelastic adhesion in rough line contacts. Sci. Rep. 2023, 13, 15060. [Google Scholar] [CrossRef] [PubMed]
- Papangelo, A.; Ciavarella, M. Detachment of a rigid flat punch from a viscoelastic material. Tribol. Lett. 2023, 71, 48. [Google Scholar] [CrossRef]
- Tan, D.; Wang, X.; Liu, Q.; Shi, K.; Yang, B.; Liu, S.; Wu, Z.S.; Xue, L. Switchable adhesion of micropillar adhesive on rough surfaces. Small 2019, 15, 1904248. [Google Scholar] [CrossRef]
- He, Q.; Zhao, Z.; Zhong, Q.; Liu, S.; Deng, K.; Liu, Y.; Zhang, N.; Zhao, Z.; Zhan, F.; Zhao, J. Switchable shape memory polymer bio-inspired adhesive and its application for unmanned aerial vehicle landing. Chin. J. Aeronaut. 2024, 37, 380–390. [Google Scholar] [CrossRef]
- Linghu, C.; Liu, Y.; Tan, Y.Y.; Sing, J.H.M.; Tang, Y.; Zhou, A.; Wang, X.; Li, D.; Gao, H.; Hsia, K.J. Overcoming the adhesion paradox and switchability conflict on rough surfaces with shape-memory polymers. Proc. Natl. Acad. Sci. USA 2023, 120, e2221049120. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.; Xia, N.; Zhang, L. A review of bioinspired dry adhesives: From achieving strong adhesion to realizing switchable adhesion. Bioinspir. Biomimetics 2024, 19, 051003. [Google Scholar] [CrossRef] [PubMed]
- Aksak, B.; Sahin, K.; Sitti, M. The optimal shape of elastomer mushroom-like fibers for high and robust adhesion. Beilstein J. Nanotechnol. 2014, 5, 630–638. [Google Scholar] [CrossRef]


| Physical Parameter | Value |
|---|---|
| Young’s modulus of fibril, : | MPa |
| Young’s modulus of rigid surface, : | 100 GPa |
| Poisson ratio of fibril, : | 0.5 |
| Poisson ratio of rigid surface, : | 0.3 |
| The radius of fibril, R: | 100 m |
| The height of fibril, : | 600 m |
| The number of fibrils, n: | 50, 100, 2000 |
| Work of adhesion, W: | 0.05, 0.25 J/m2 [38] |
| Pre–load factor, : | 0.1:50 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eray, T. A Fast Numerical Approach for Investigating Adhesion Strength in Fibrillar Structures: Impact of Buckling and Roughness. Lubricants 2024, 12, 294. https://doi.org/10.3390/lubricants12080294
Eray T. A Fast Numerical Approach for Investigating Adhesion Strength in Fibrillar Structures: Impact of Buckling and Roughness. Lubricants. 2024; 12(8):294. https://doi.org/10.3390/lubricants12080294
Chicago/Turabian StyleEray, Turgay. 2024. "A Fast Numerical Approach for Investigating Adhesion Strength in Fibrillar Structures: Impact of Buckling and Roughness" Lubricants 12, no. 8: 294. https://doi.org/10.3390/lubricants12080294
APA StyleEray, T. (2024). A Fast Numerical Approach for Investigating Adhesion Strength in Fibrillar Structures: Impact of Buckling and Roughness. Lubricants, 12(8), 294. https://doi.org/10.3390/lubricants12080294





