Investigation on the Static Performance of Surface-Throttling Frictionless Pneumatic Cylinder through Finite Element Method
Abstract
1. Introduction
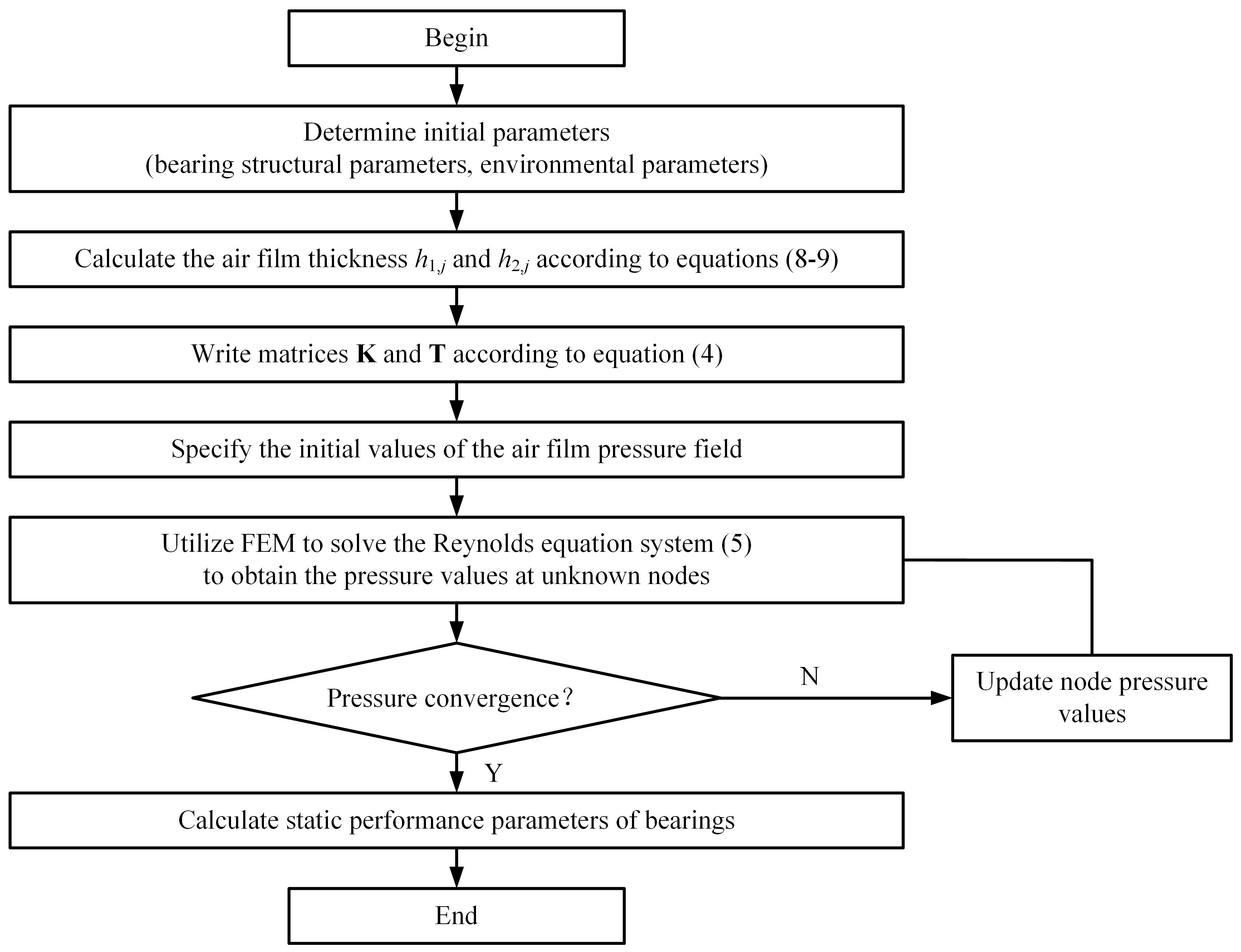
2. The FEM for Surface-Throttling Aerostatic Bearings
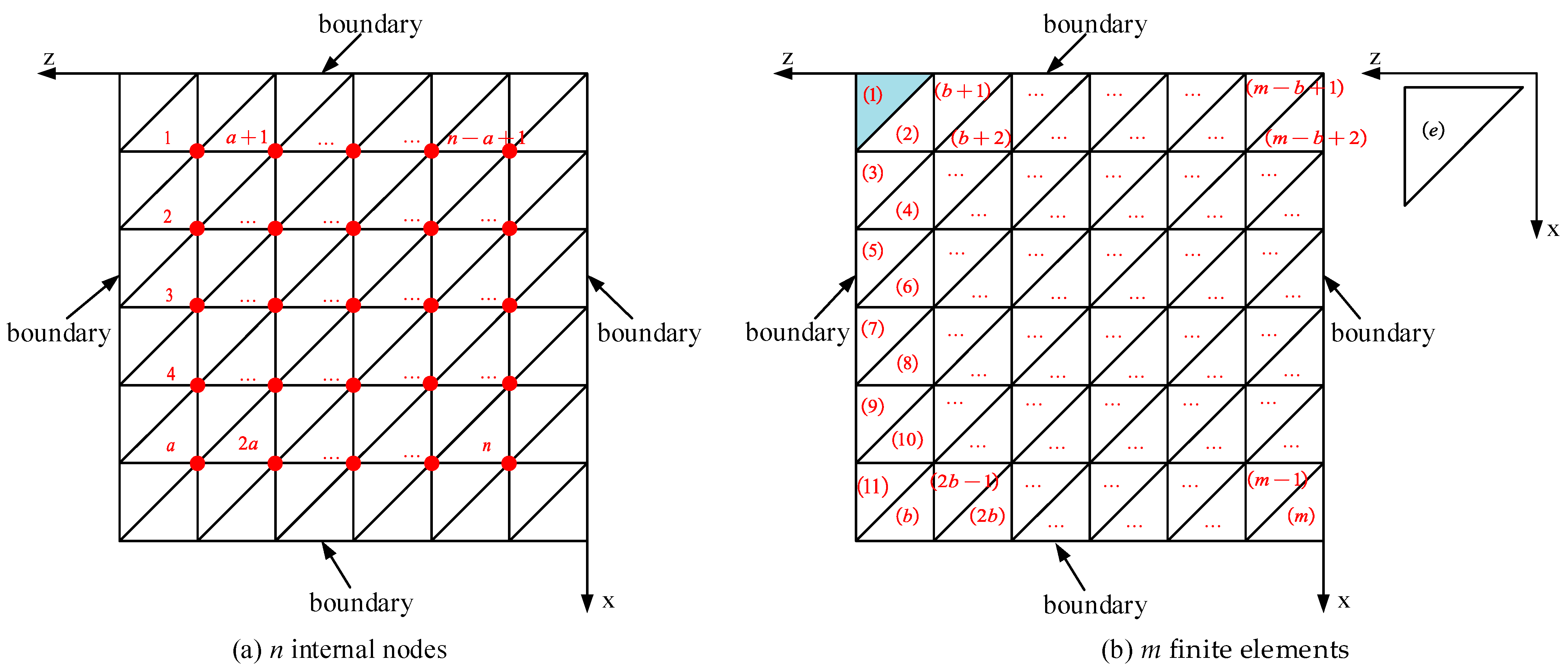
2.1. The Finite Element Formulation of the Reynolds Equation
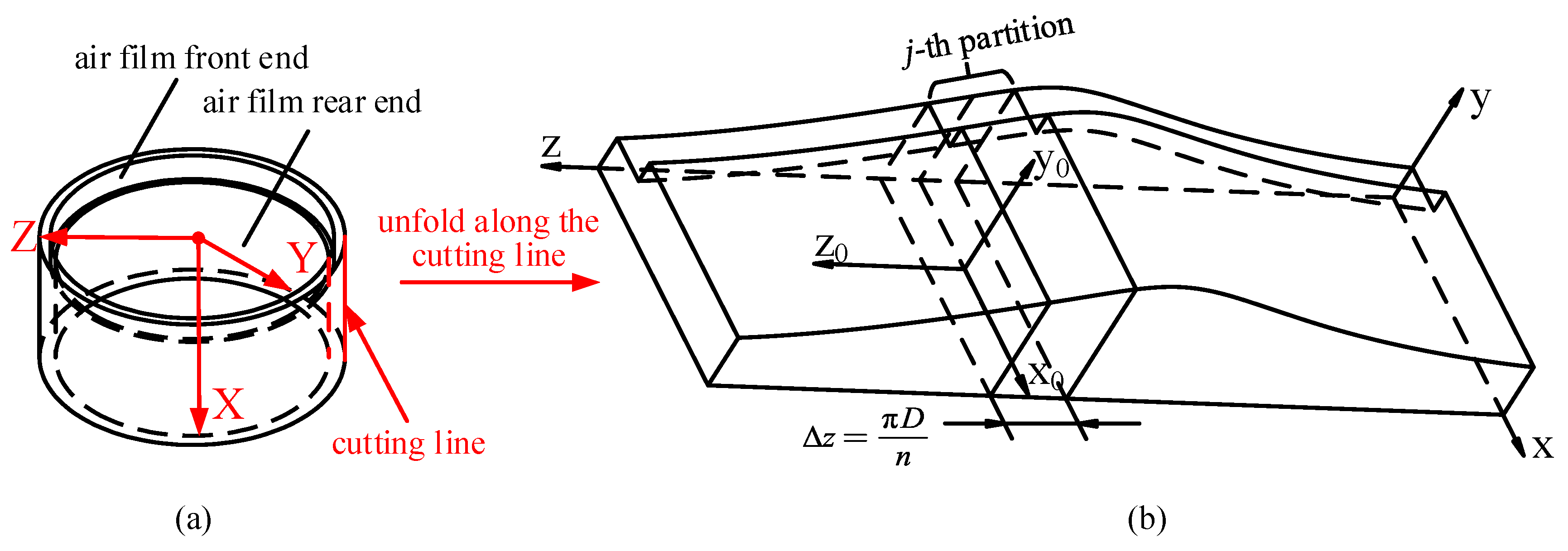
2.2. FEM Calculation for Surface-Throttling Aerostatic Bearings
- ➀
- Partitioning of the air film calculation domain
- ➁
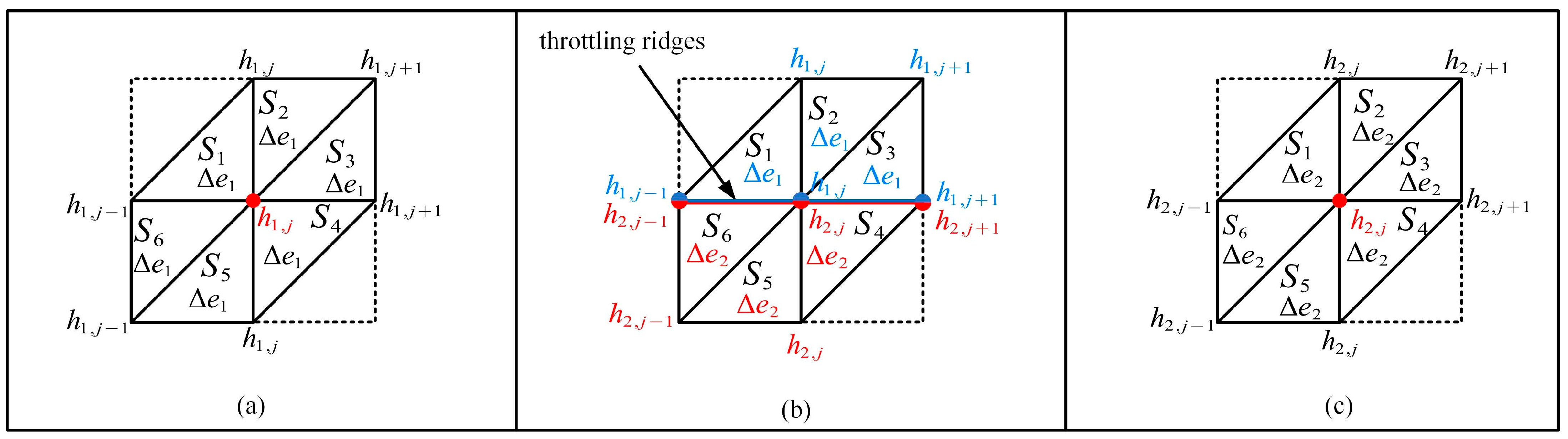
- Specification of node air film thickness
- ➂
- Calculation of flow rate for surface-throttling aerostatic bearings
3. Static Performance Calculation of Surface-Throttling Aerostatic Bearings
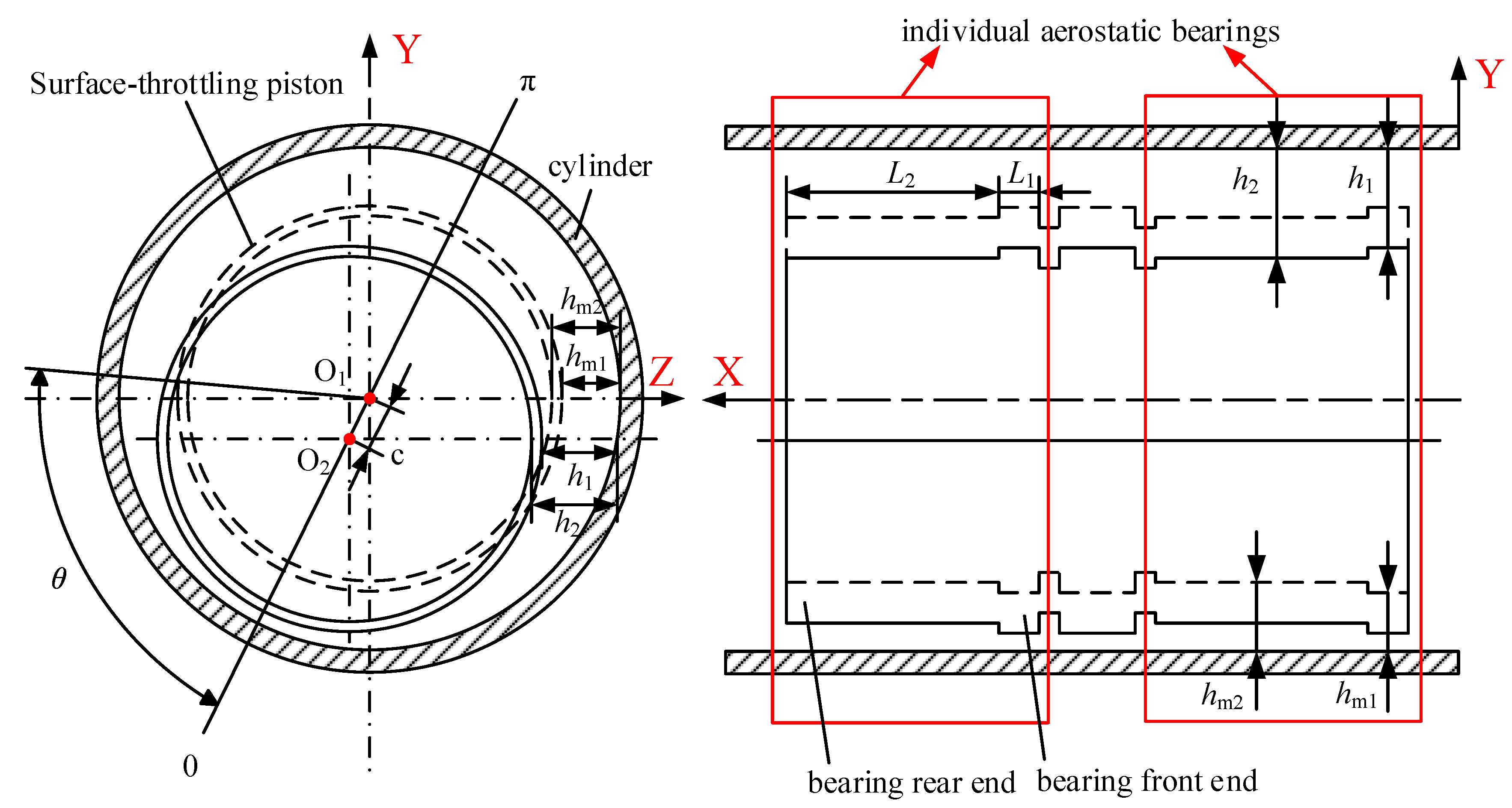
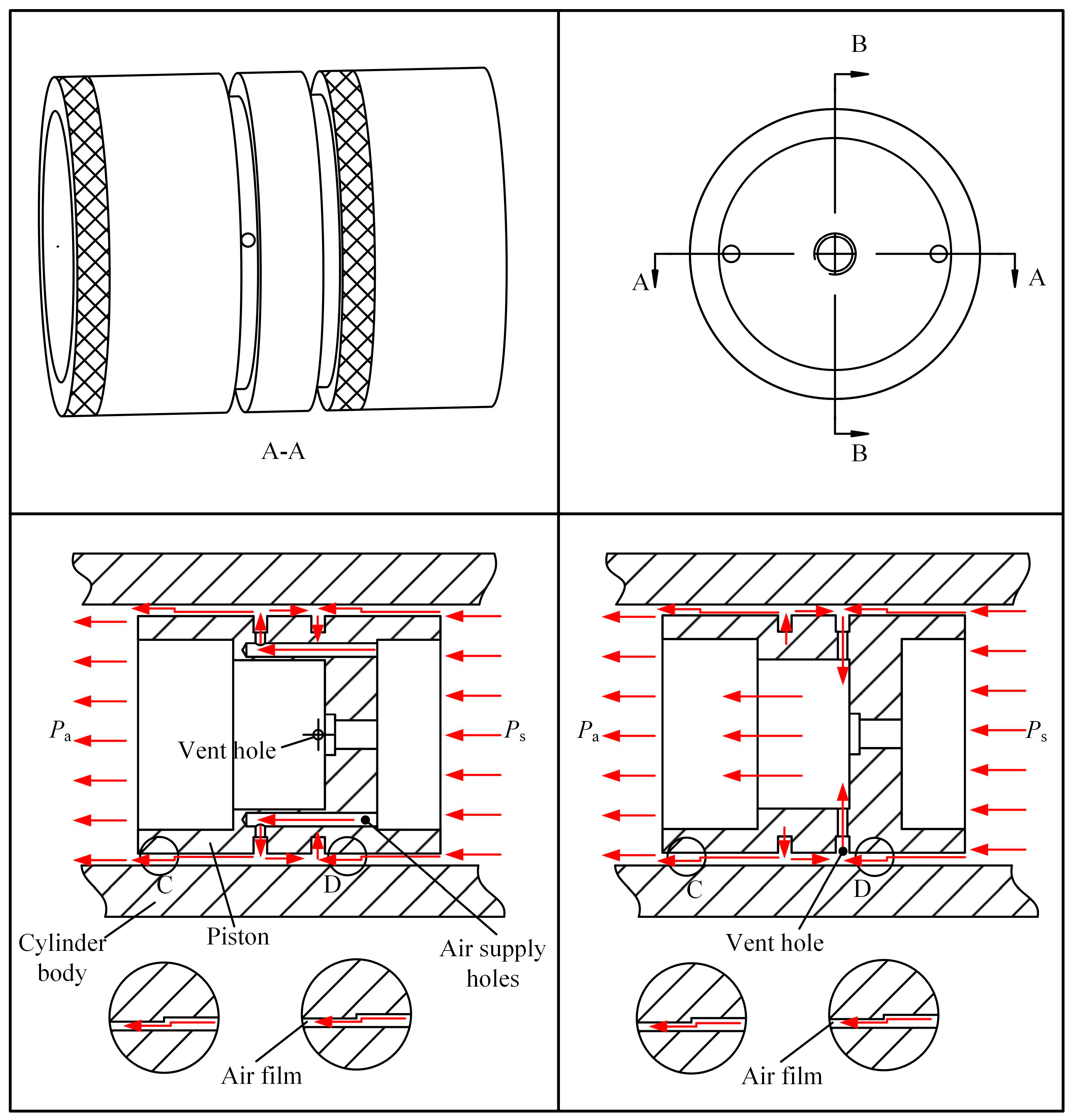
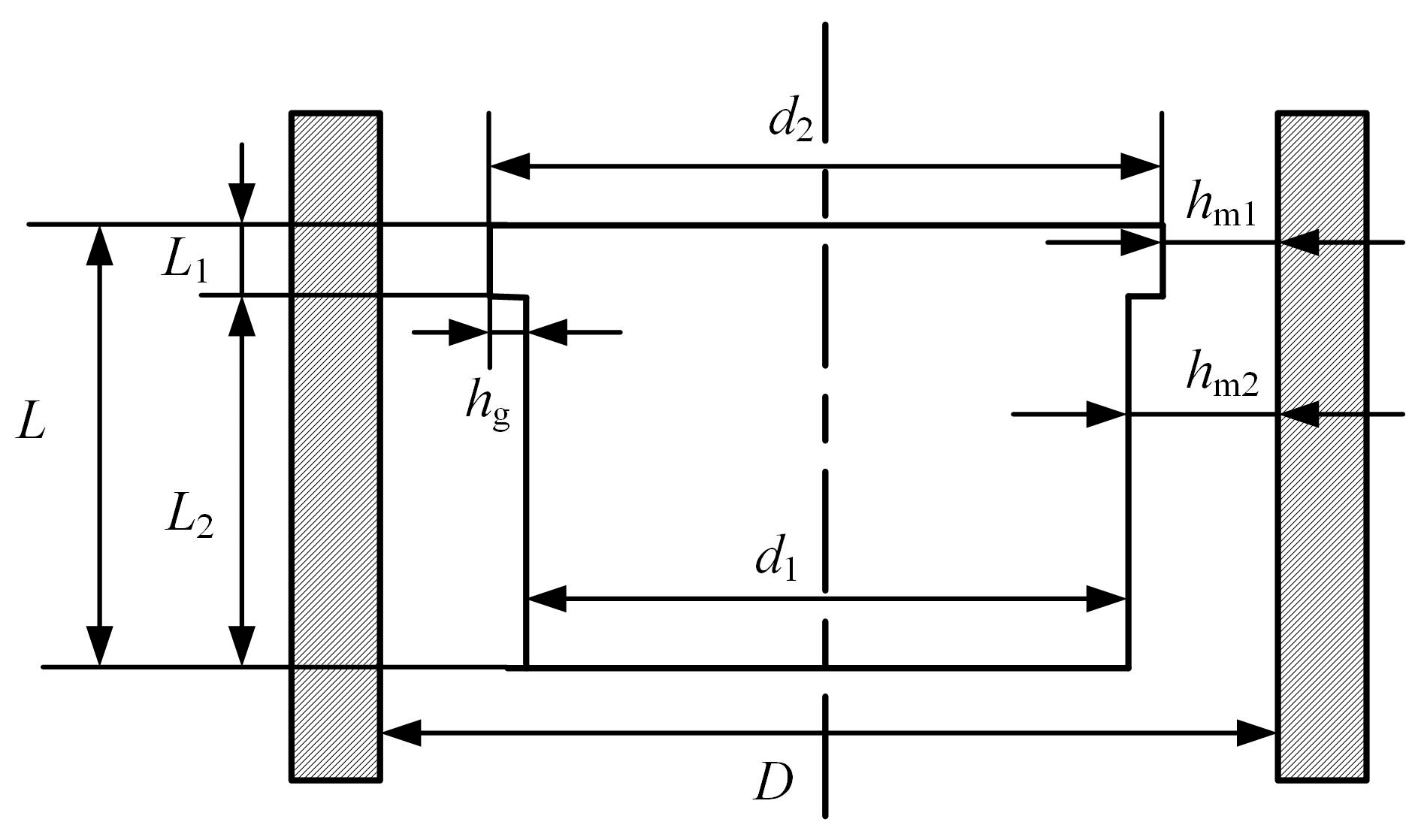
3.1. Structural Design and Operating Principles of Surface-Throttling Frictionless Pneumatic Cylinder
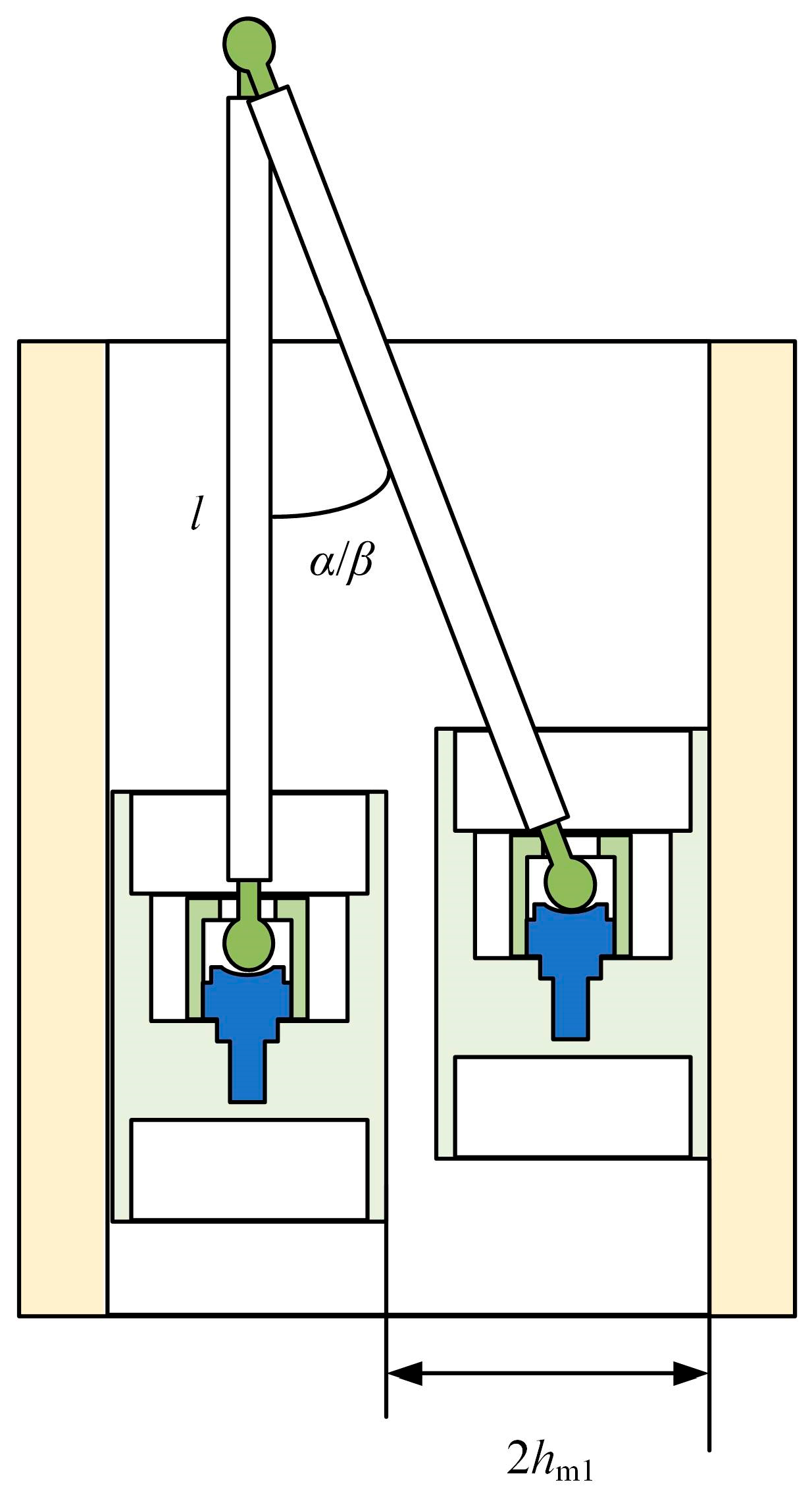
3.2. Static Equilibrium Calculation of Surface-Throttling Aerostatic Bearings
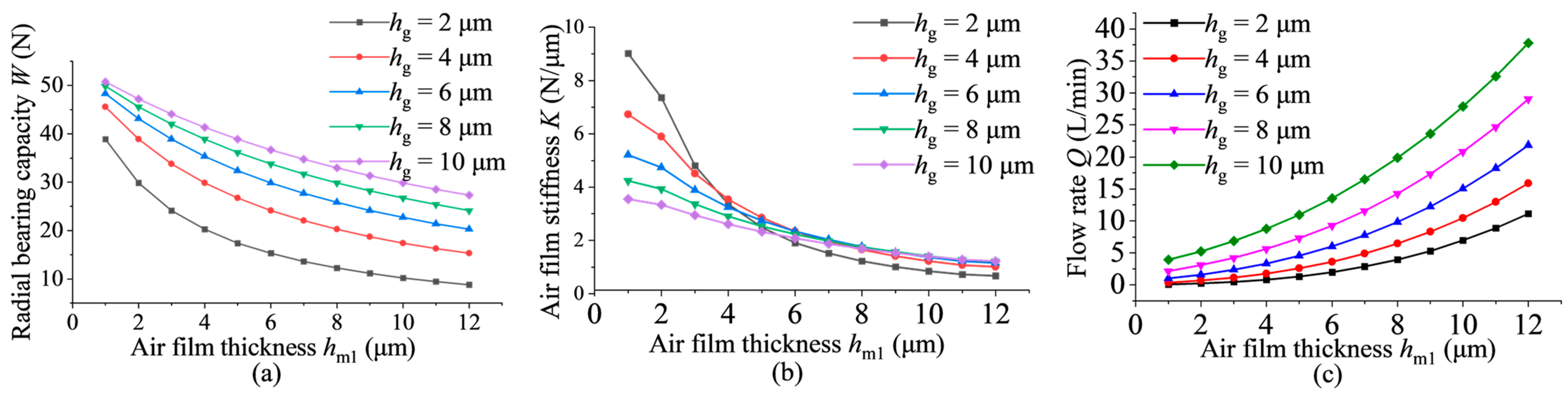
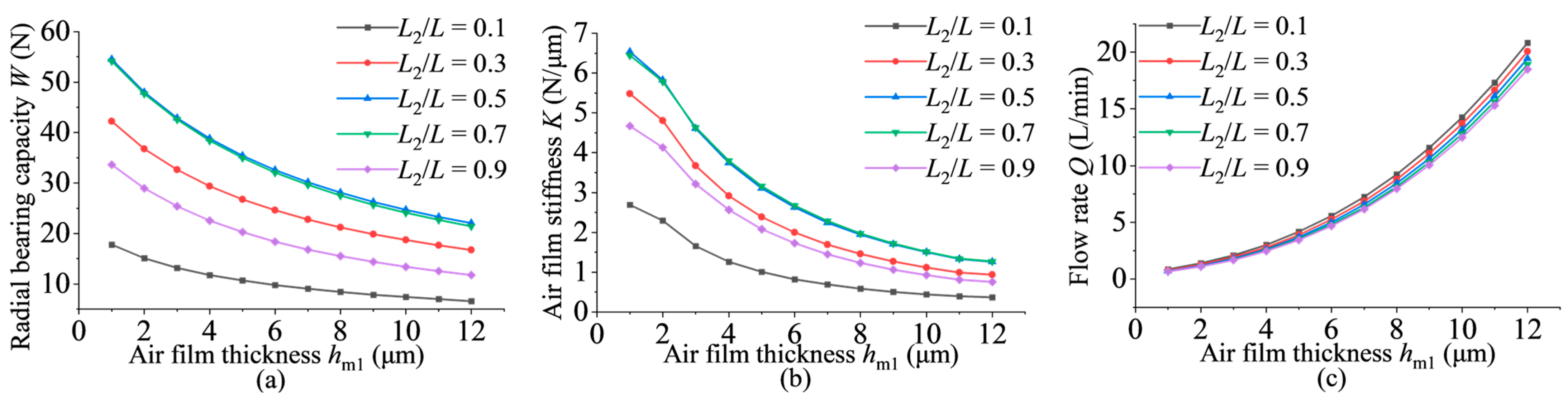
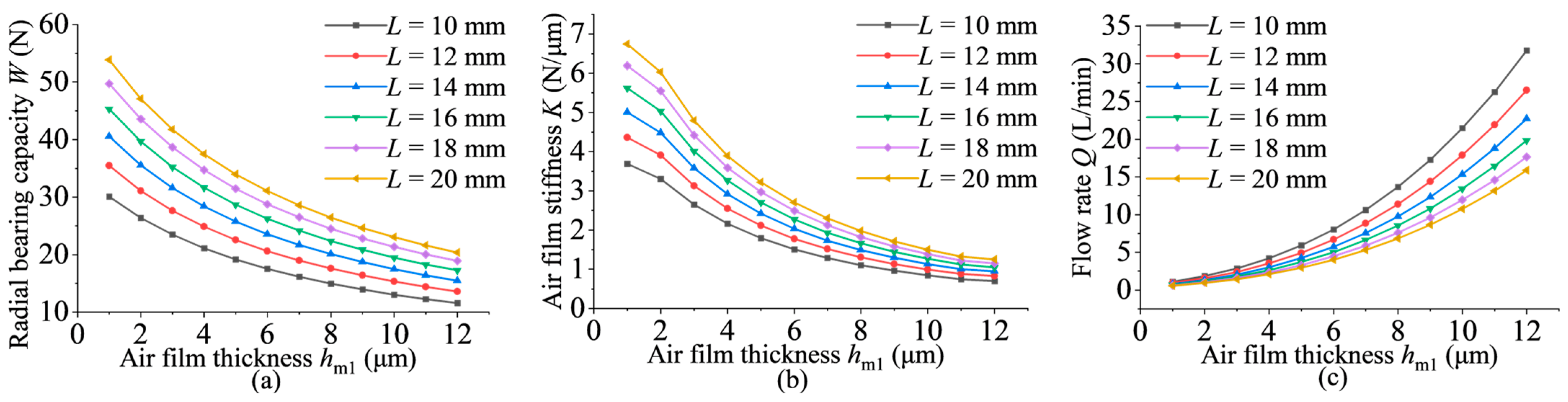
3.3. Static Performance Calculation of Surface-Throttling Aerostatic Bearings Using FEM
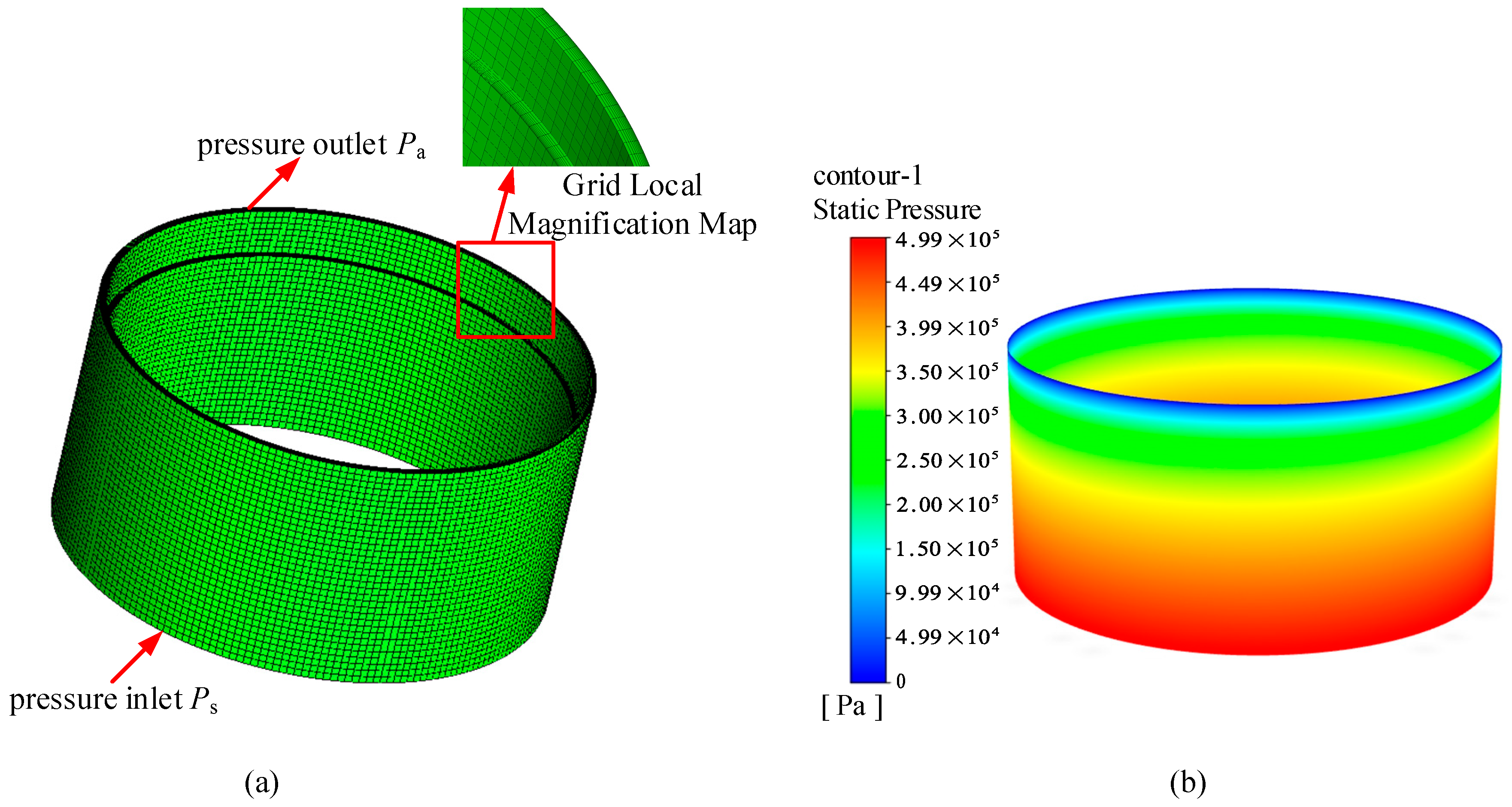
4. Verification and Discussion
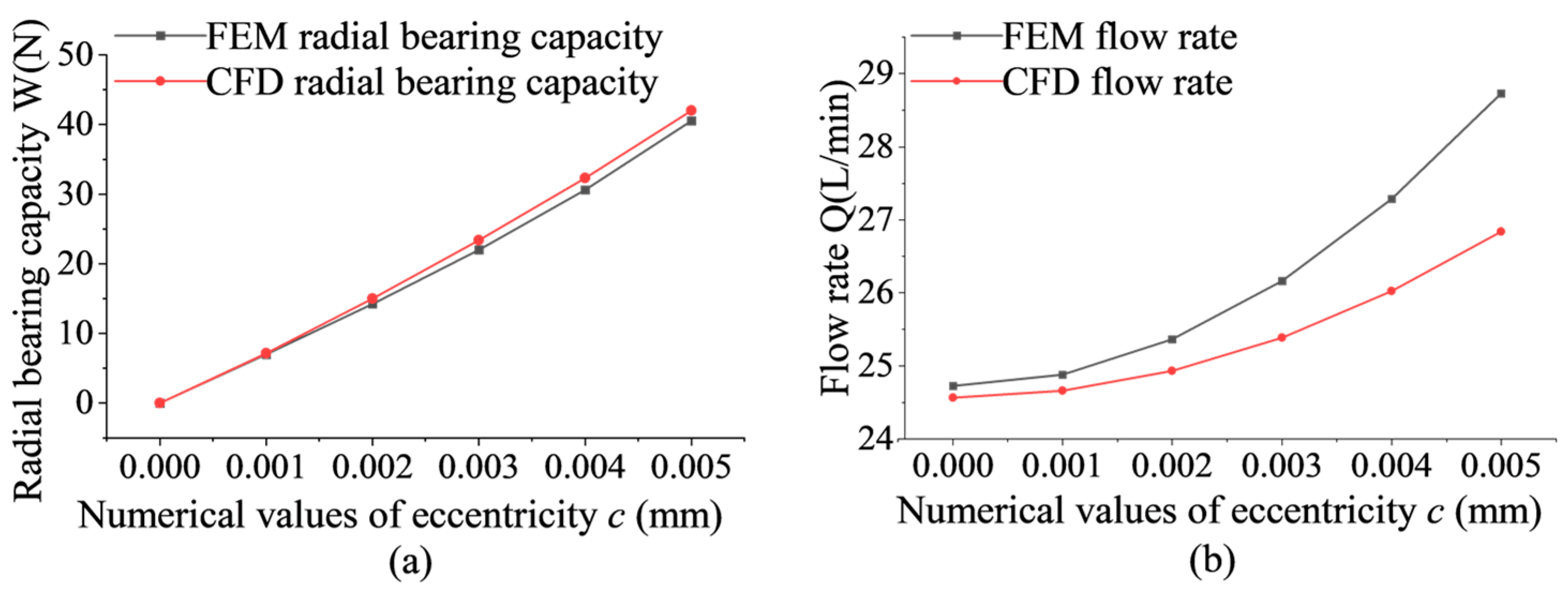
4.1. Verification of CFD Calculation Results
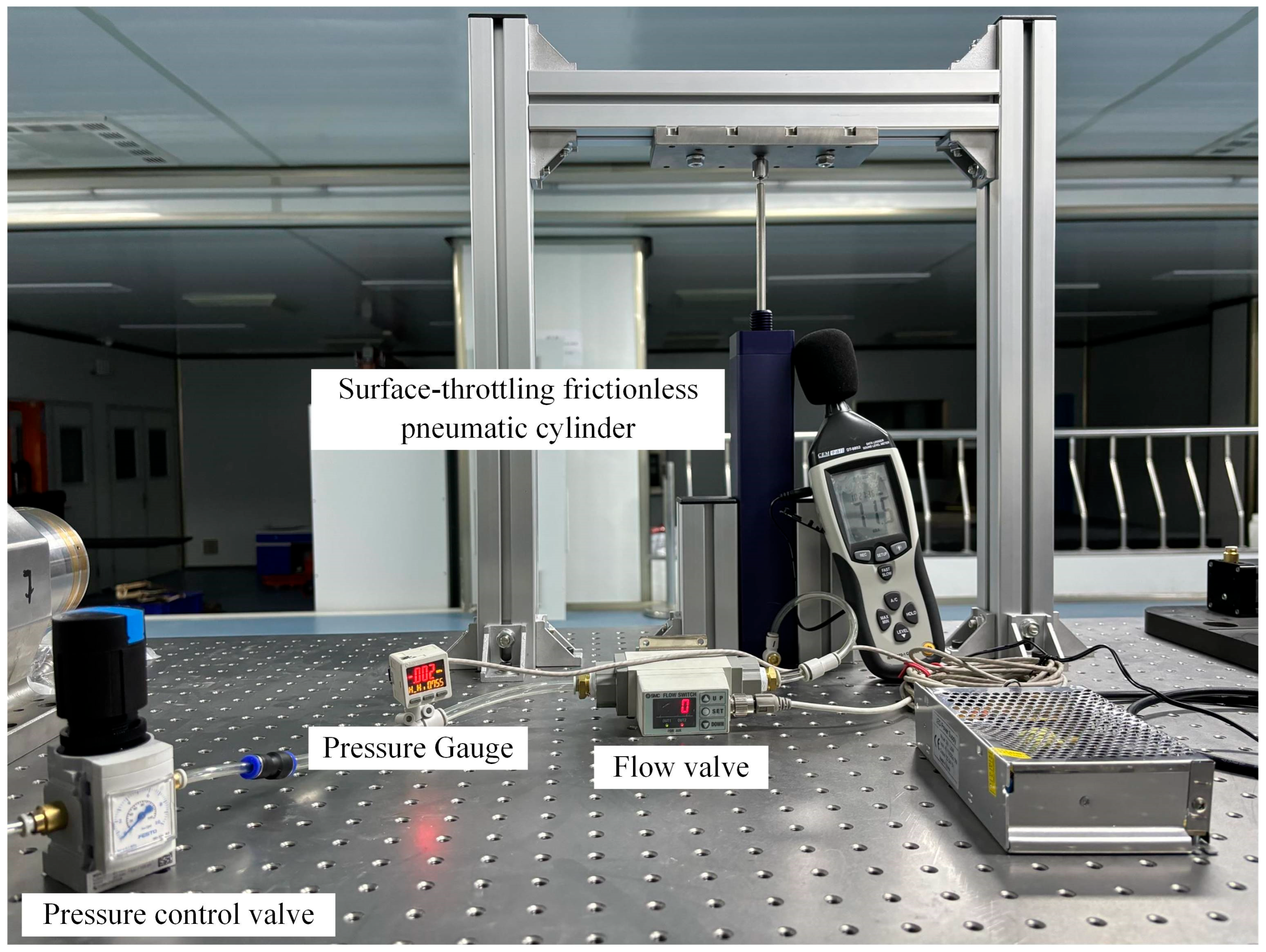
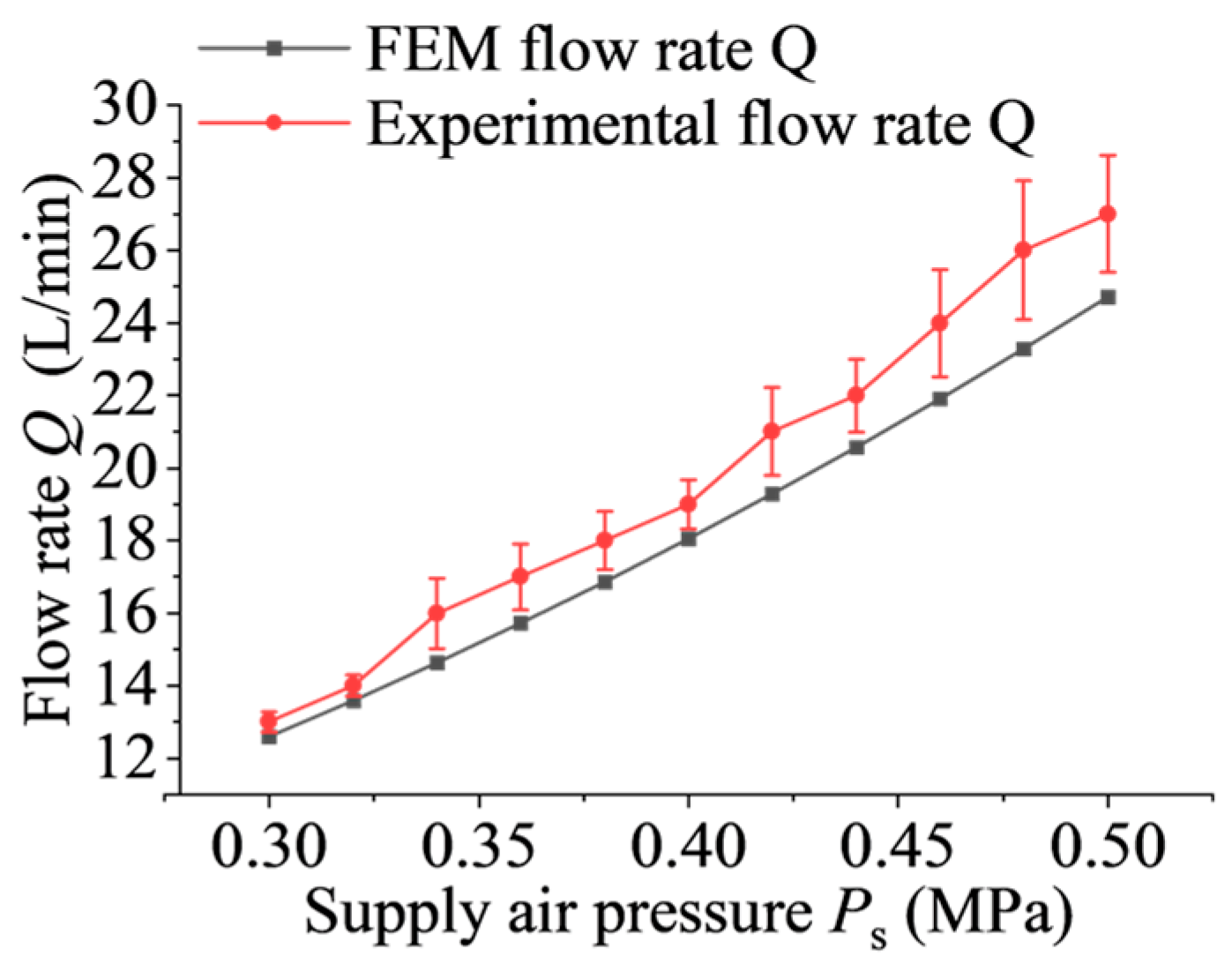
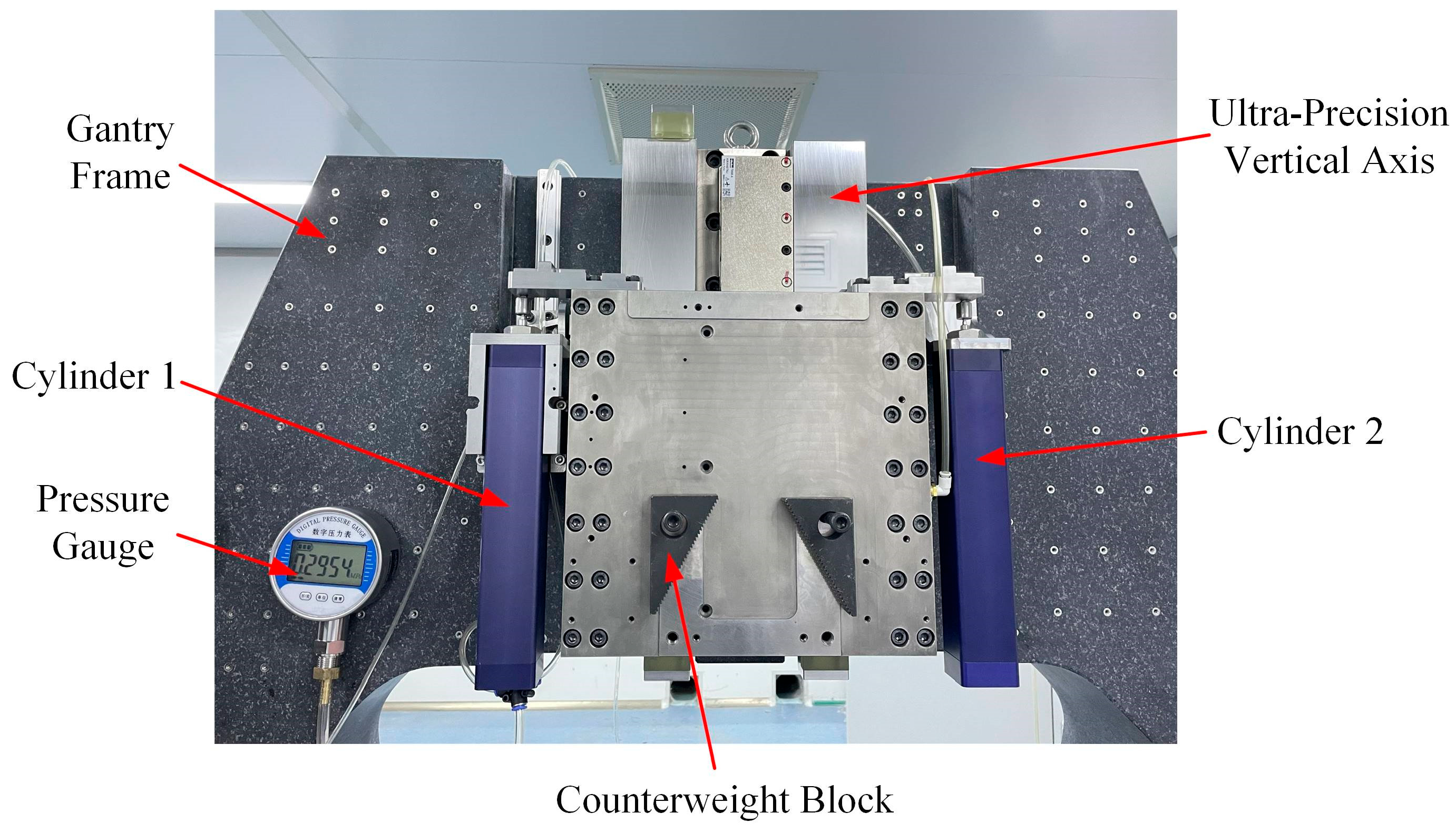
4.2. Experimental Verification
4.3. Comparison of Computational Efficiency between the Improved FEM and CFD Calculation
5. Conclusions
- The improved FEM proposed in this paper addresses the computational challenge of varying air film thickness at different locations within surface-throttling aerostatic bearings. It overcomes the difficulties associated with variations in element air film thickness at different bearing positions. Additionally, it offers insights into the calculation of flow rate for surface-throttling aerostatic bearings.
- Static equilibrium calculations were performed for the dual-cylinder system, considering the inherent errors in the ultra-precision machine tool’s vertical axis. This process yielded a range of radial bearing capacity and support force values for the frictionless pneumatic cylinders, offering theoretical guidance for selecting cylinder parameters.
- The improved FEM proposed in this paper has been validated through comparisons with the CFD calculation and experimental data. For the cylinders in this paper, the errors between the improved FEM and the CFD calculation are6% for radial bearing capacity and 7% for flow rate, respectively. The error between the calculated flow rate and the experimental data is 10%. While there are slight discrepancies in local numerical values, the overall trends in the computed results closely match.
- The computational efficiency of the proposed FEM model is significantly improved compared with the CFD calculation. For the cylinders in this paper, the average computation time decreased from 8.329 h to 51.392 s.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Xing, T.; Zhao, X.; Song, L.; Cui, Z.; Zou, X.; Sun, T. On-Machine Measurement Method and Geometrical Error Analysis in a Multi-Step Processing System of an Ultra-Precision Complex Spherical Surface. J. Manuf. Process. 2022, 80, 161–177. [Google Scholar] [CrossRef]
- Hatefi, S.; Abou-El-Hossein, K. Review of Single-Point Diamond Turning Process in Terms of Ultra-Precision Optical Surface Roughness. Int. J. Adv. Manuf. Technol. 2020, 106, 2167–2187. [Google Scholar] [CrossRef]
- Pu, X.; Xu, J.; Huang, P.; Du, H.; Zhu, Z. Fast Tool Servo-Based Ultra-Precision Diamond Sculpturing for Fabricating Micro-Structured Surfaces. Int. J. Mech. Sci. 2024, 263, 108790. [Google Scholar] [CrossRef]
- Zhang, H.; Xiang, S.; Wu, C.; Yang, J. Optimal Proportion Compensation Method of Key Geometric Errors for Five-Axis Machine Tools Considering Multiple-Direction Coupling Effects. J. Manuf. Process. 2024, 110, 447–461. [Google Scholar] [CrossRef]
- Shi, W.; Chunqiang, Y.; Tai, Y.; Yupeng, W.; Yong, Z. Comprehensive Analysis and Evaluation of the Geometric Errors of the Rotating Axis of Five-Axis Double-Pendulum Machine Tools Based on S-Shaped Samples. Int. J. Adv. Manuf. Technol. 2023, 129, 5135–5148. [Google Scholar] [CrossRef]
- Wang, J.; Guo, J. The Identification Method of the Relative Position Relationship between the Rotary and Linear Axis of Multi-Axis Numerical Control Machine Tool by Laser Tracker. Measurement 2019, 132, 369–376. [Google Scholar] [CrossRef]
- Takahashi, M.; Yoshioka, H.; Shinno, H. A Newly Developed Long-Stroke Vertical Nano-Motion Platform with Gravity Compensator. J. Adv. Mech. Des. Syst. Manuf. 2008, 2, 356–365. [Google Scholar] [CrossRef]
- Park, C.H.; Song, C.K.; Hwang, J.; Kim, B.-S. Development of an Ultra Precision Machine Tool for Micromachining on Large Surfaces. Int. J. Precis. Eng. Manuf. 2009, 10, 85–91. [Google Scholar] [CrossRef]
- Hsieh, M.-F.; Tung, C.-J.; Yao, W.-S.; Wu, M.-C.; Liao, Y.-S. Servo Design of a Vertical Axis Drive Using Dual Linear Motors for High Speed Electric Discharge Machining. Int. J. Mach. Tools Manuf. 2007, 47, 546–554. [Google Scholar] [CrossRef]
- Raparelli, T.; Mazza, L.; Trivella, A. Experimental Analysis and Preliminary Model of Non-Conventional Lip Seals. Tribol. Int. 2023, 181, 108311. [Google Scholar] [CrossRef]
- Xu, X.; Zhang, Y. Effect of Different Chemical Conversion Coatings on the Tribological Performance of Cylindrical Thrust Roller Bearings under Conditions of Dry Friction and Solid Lubrication. Wear 2024, 548, 205351. [Google Scholar] [CrossRef]
- Zhang, B.; Ma, X.; Liu, L.; Wang, Y.; Yu, H.; Morina, A.; Lu, X. Reciprocating Sliding Friction Behavior and Wear State Transition Mechanism of Cylinder Liner and Piston Ring. Wear 2024, 546, 205293. [Google Scholar] [CrossRef]
- Wang, G.; Li, W.; Liu, G.; Feng, K. A Novel Optimization Design Method for Obtaining High-Performance Micro-Hole Aerostatic Bearings with Experimental Validation. Tribol. Int. 2023, 185, 108542. [Google Scholar] [CrossRef]
- Gao, Q.; Chen, W.; Lu, L.; Huo, D.; Cheng, K. Aerostatic Bearings Design and Analysis with the Application to Precision Engineering: State-of-the-Art and Future Perspectives. Tribol. Int. 2019, 135, 1–17. [Google Scholar] [CrossRef]
- Yao, Y.; Zhang, K.; Yu, Z.; Mei, T. Research on a New Frictionless Air Cylinder. In Advanced Design Technology, PTS 1–3; Gao, J., Ed.; Trans Tech Publications Ltd.: Zurich, Switzerland, 2011; Volume 308–310, pp. 859–863. [Google Scholar]
- Zhu, X.; Jin, X.; Yao, B.; Cao, J. Modeling and Design of a Frictionless Pneumatic Cylinder System with Air Bearings. In Proceedings of the 2015 IEEE International Conference on Cyber Technology in Automation, Control, and Intelligent Systems (Cyber), Shenyang, China, 8–12 June 2015; pp. 1192–1197. [Google Scholar]
- Qian, P.; Liu, L.; Wu, J.; Pu, C.; Luo, H.; Fu, Y.; Zuo, Q. A Novel Double-Acting, Air-Floating, Frictionless Pneumatic Actuator. Sens. Actuators A Phys. 2023, 362, 114674. [Google Scholar] [CrossRef]
- Yu, P.; Huang, L.; Li, S.; Guo, L.; Zhong, M.; Zhang, L. Theoretical Predictions and Experimental Measurements of Novel Aerostatic Bearing with Multi-Inclined-Orifice Restrictors for the Improvement of Stability. Precis. Eng.-J. Int. Soc. Precis. Eng. Nanotechnol. 2024, 88, 266–278. [Google Scholar] [CrossRef]
- Kwan, Y.B.P.; Post, J.B. A Tolerancing Procedure for Inherently Compensated, Rectangular Aerostatic Thrust Bearings. Tribol. Int. 2000, 33, 581–585. [Google Scholar] [CrossRef]
- Talukder, H.M.; Stowell, T.B. Pneumatic Hammer in an Externally Pressurized Orifice-Compensated Air Journal Bearing. Tribol. Int. 2003, 36, 585–591. [Google Scholar] [CrossRef]
- Li, Y.F.; Yin, Y.H.; Yang, H.; Liu, X.E.; Mo, J.; Cui, H.L. Micro-Vibration Analysis and Optimization of Aerostatic Bearing with Pocketed Orifice-Type Restrictor. J. Appl. Fluid Mech. 2018, 11, 1115–1124. [Google Scholar] [CrossRef]
- Yoshimura, T.; Hanafusa, T.; Kitagawa, T.; Hirayama, T.; Matsuoka, T.; Yabe, H. Clarifications of the Mechanism of Nano-Fluctuation of Aerostatic Thrust Bearing with Surface Restriction. Tribol. Int. 2012, 48, 29–34. [Google Scholar] [CrossRef]
- Qi, L.; Liu, L.; Gao, Q.; Yao, Y.; Lu, L.; Gao, S. Investigation on the Influence of Structural Rigidity on the Stiffness of Aerostatic Guideway Considering Fluid-Structure Interaction. Tribol. Int. 2022, 173, 107658. [Google Scholar] [CrossRef]
- Belforte, G.; Raparelli, T.; Trivella, A.; Viktorov, V.; Visconte, C. CFD Analysis of a Simple Orifice-Type Feeding System for Aerostatic Bearings. Tribol. Lett. 2015, 58, 25. [Google Scholar] [CrossRef]
- Gao, S.; Cheng, K.; Chen, S.; Ding, H.; Fu, H. CFD Based Investigation on Influence of Orifice Chamber Shapes for the Design of Aerostatic Thrust Bearings at Ultra-High Speed Spindles. Tribol. Int. 2015, 92, 211–221. [Google Scholar] [CrossRef]
- Chen, Y.S.; Chiu, C.C.; Cheng, Y.D. Influences of Operational Conditions and Geometric Parameters on the Stiffness of Aerostatic Journal Bearings. Precis. Eng.-J. Int. Soc. Precis. Eng. Nanotechnol. 2010, 34, 722–734. [Google Scholar] [CrossRef]
- Yoshimoto, S.; Yamamoto, M.; Toda, K. Numerical Calculations of Pressure Distribution in the Bearing Clearance of Circular Aerostatic Thrust Bearings with a Single Air Supply Inlet. J. Tribol.-Trans. ASME 2007, 129, 384–390. [Google Scholar] [CrossRef]




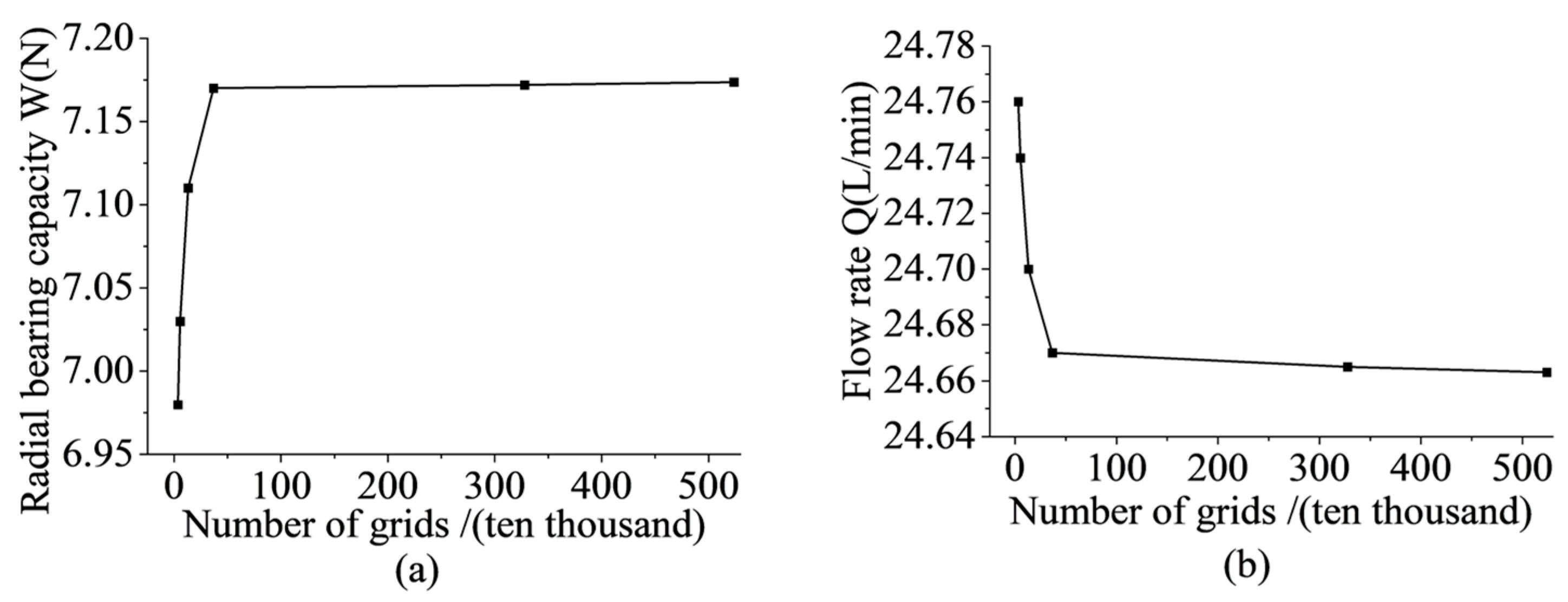
| Mesh | (m1 + m2) × n | Radial Bearing Capacity W (N) | Flow Rate Q (L/min) |
|---|---|---|---|
| 1 | 15 × 20 | 6.800885638 | 24.88392443 |
| 2 | 30 × 40 | 6.949990431 | 24.88348226 |
| 3 | 60 × 80 | 6.987733346 | 24.88336807 |
| 4 | 90 × 120 | 6.99474121 | 24.88334682 |
| Cylinder 1 | Cylinder 2 | |||||
|---|---|---|---|---|---|---|
| Total Load (F + G)/kg | Pressure during Slow Ascension /MPa | Pressure during Slow Descent /MPa | Pressure Differential/MPa | Pressure during Slow Ascension /MPa | Pressure during Slow Descent /MPa | Pressure Differential/MPa |
| 66.508 | 0.3353 | 0.3342 | 0.0011 | 0.335 | 0.3345 | 0.0005 |
| 67.333 | 0.3398 | 0.3369 | 0.0029 | 0.3394 | 0.337 | 0.0024 |
| 68.158 | 0.3426 | 0.3399 | 0.0027 | 0.3422 | 0.3393 | 0.0029 |
| 68.983 | 0.3461 | 0.3441 | 0.002 | 0.346 | 0.345 | 0.001 |
| 69.808 | 0.3505 | 0.3479 | 0.0026 | 0.3508 | 0.3479 | 0.0029 |
| Different Eccentricities (μm) | CFD Calculation/(h) | FEM/(s) | ||
|---|---|---|---|---|
| CFD Model Setup /(h) | Mesh Generation /(h) | Post-Processing /(h) | ||
| 0 | 0.45 | 3.15 | 4.25 | 50.49 |
| 0.001 | 0.56 | 3.41 | 4.26 | 50.70 |
| 0.002 | 0.53 | 3.45 | 4.27 | 51.16 |
| 0.003 | 0.62 | 3.48 | 4.22 | 51.55 |
| 0.004 | 0.61 | 3.52 | 4.49 | 52.09 |
| 0.005 | 0.58 | 3.68 | 4.45 | 52.36 |
| Average | 0.558 | 3.448 | 4.323 | 51.392 |
| Total | 8.329 | 51.392 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, J.; Gao, S.; Qi, L.; Gao, Q.; Zhu, M.; Yang, H.; Li, Y.; Wei, W.; Lu, L. Investigation on the Static Performance of Surface-Throttling Frictionless Pneumatic Cylinder through Finite Element Method. Lubricants 2024, 12, 254. https://doi.org/10.3390/lubricants12070254
Xu J, Gao S, Qi L, Gao Q, Zhu M, Yang H, Li Y, Wei W, Lu L. Investigation on the Static Performance of Surface-Throttling Frictionless Pneumatic Cylinder through Finite Element Method. Lubricants. 2024; 12(7):254. https://doi.org/10.3390/lubricants12070254
Chicago/Turabian StyleXu, Jingfeng, Siyu Gao, Lizi Qi, Qiang Gao, Min Zhu, Hongbin Yang, Yinze Li, Wenyuan Wei, and Lihua Lu. 2024. "Investigation on the Static Performance of Surface-Throttling Frictionless Pneumatic Cylinder through Finite Element Method" Lubricants 12, no. 7: 254. https://doi.org/10.3390/lubricants12070254
APA StyleXu, J., Gao, S., Qi, L., Gao, Q., Zhu, M., Yang, H., Li, Y., Wei, W., & Lu, L. (2024). Investigation on the Static Performance of Surface-Throttling Frictionless Pneumatic Cylinder through Finite Element Method. Lubricants, 12(7), 254. https://doi.org/10.3390/lubricants12070254




