Experimental Research on Dynamic Characteristics of a Multi-Disc Rotor System Supported by Aerostatic Bearings
Abstract
1. Introduction
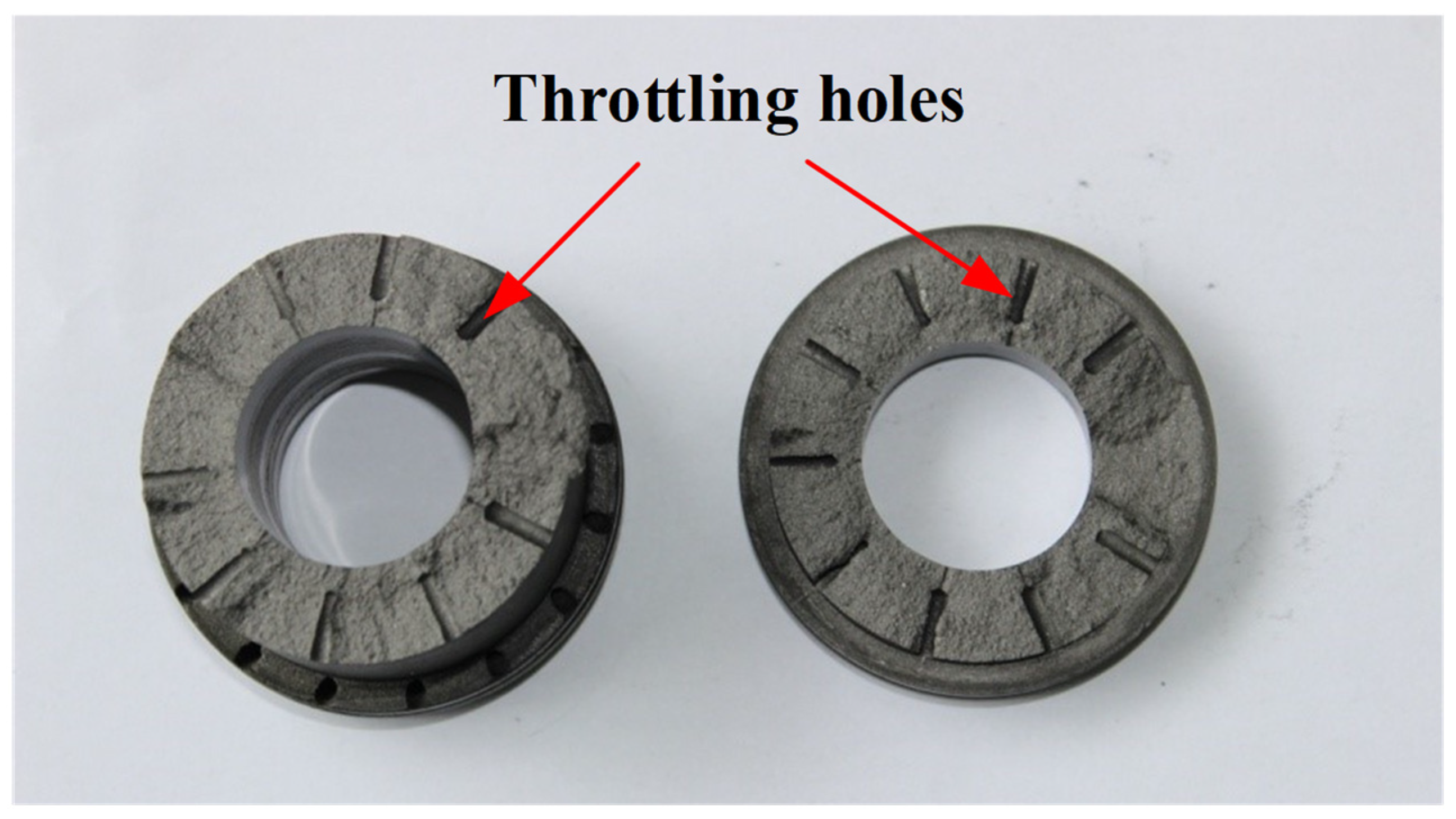
2. Test Bench for Multi-Disc Rotor Systems Supported by Aerostatic Bearings
3. Experimental Schemes
4. Discussion
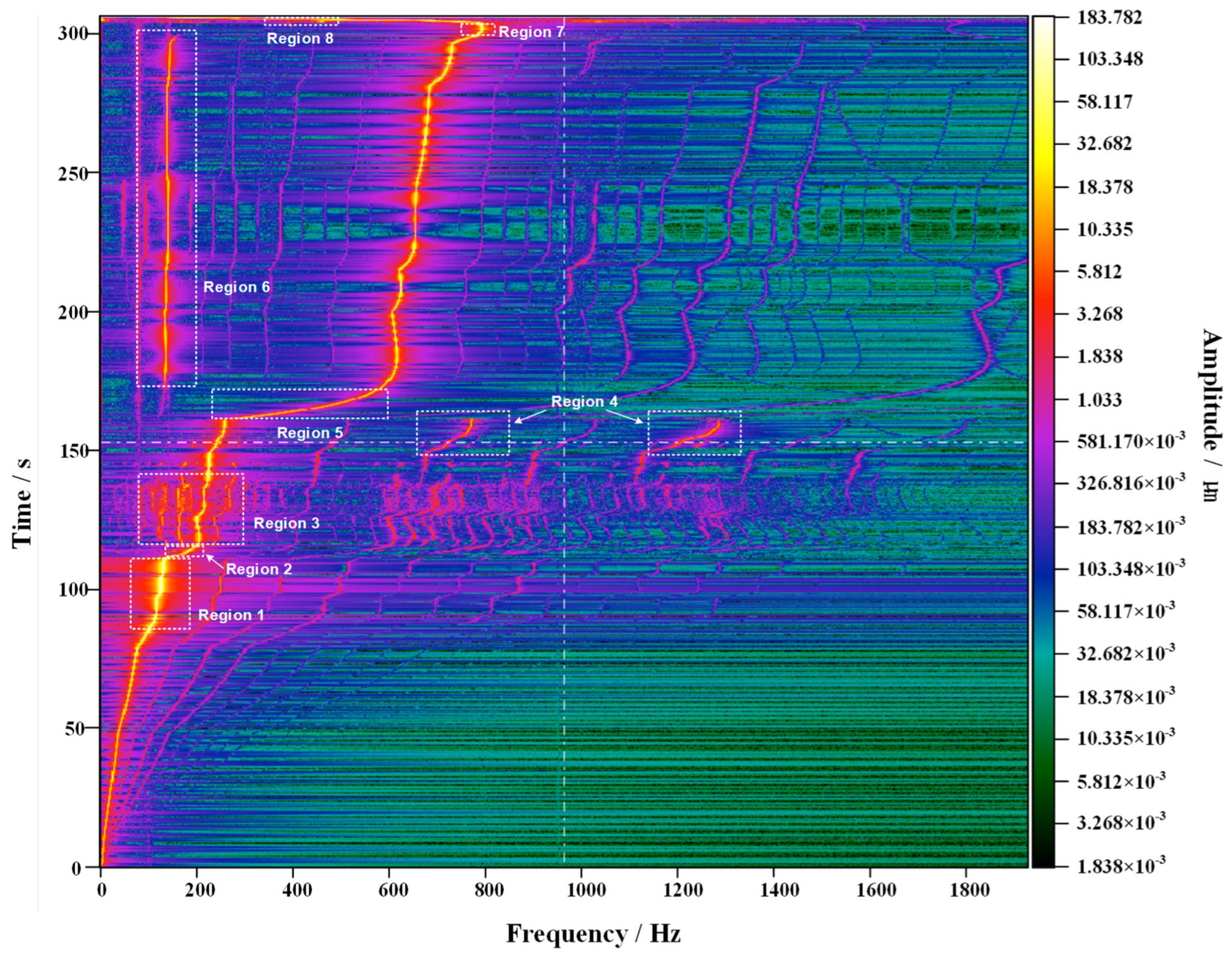
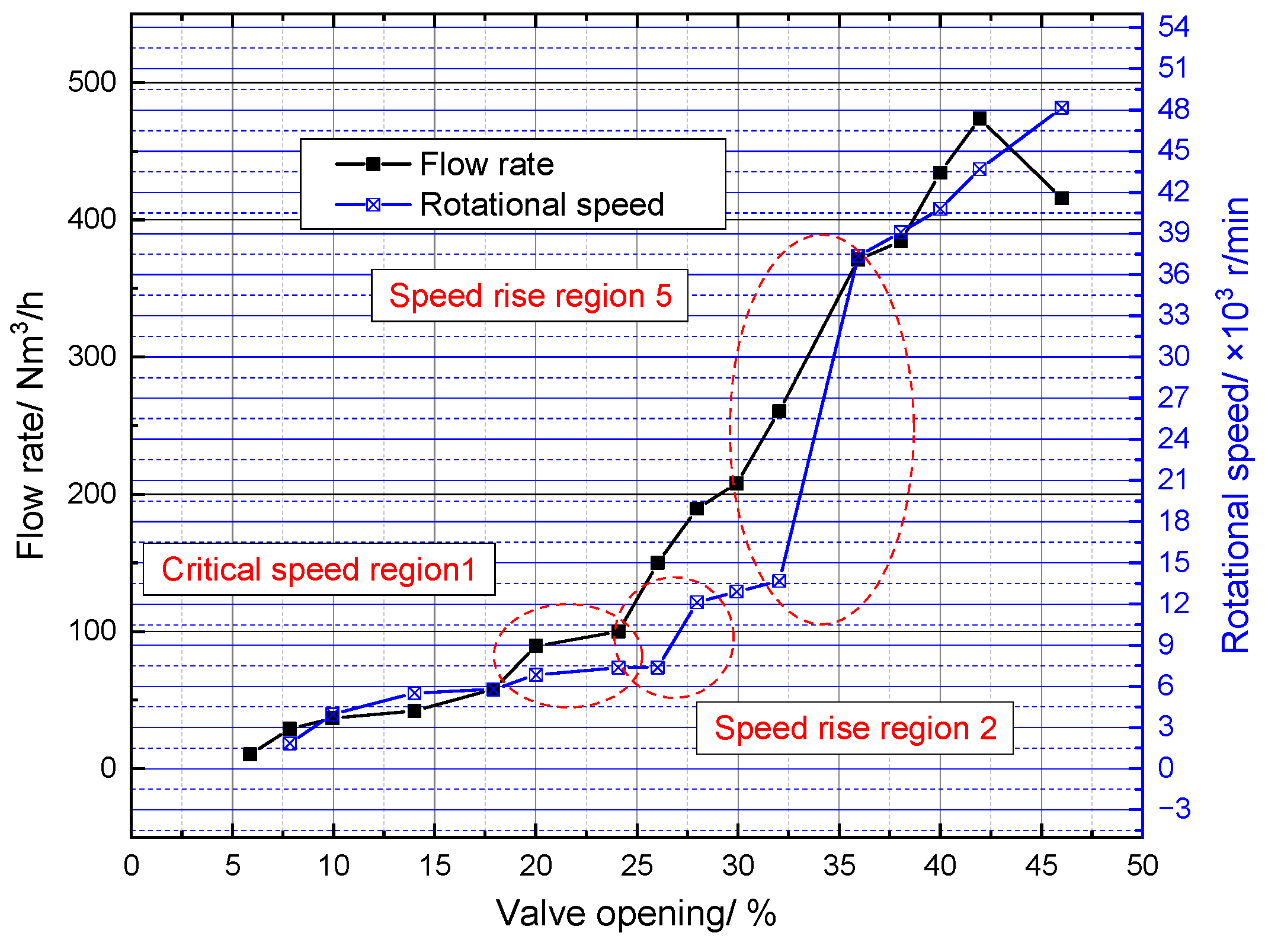
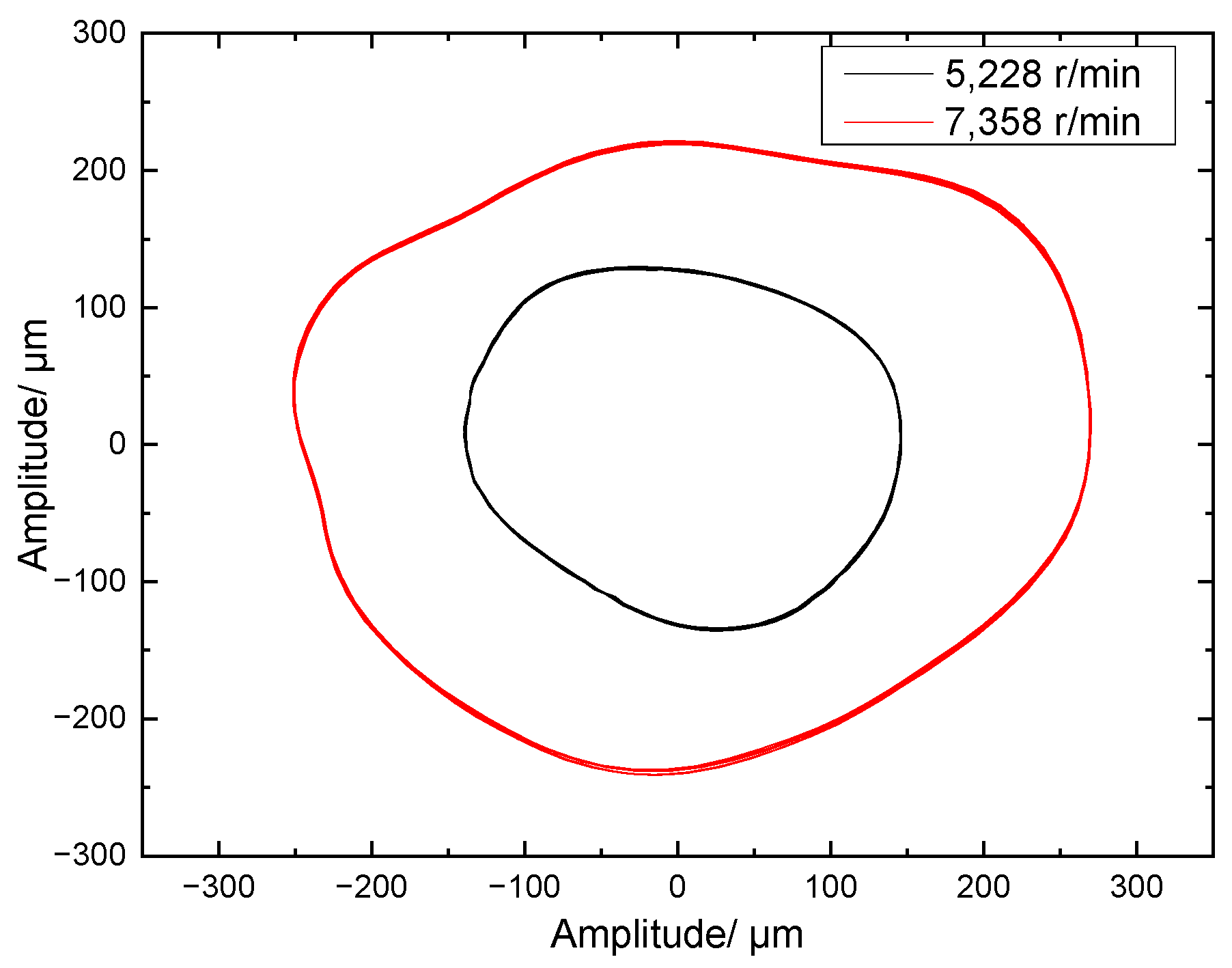
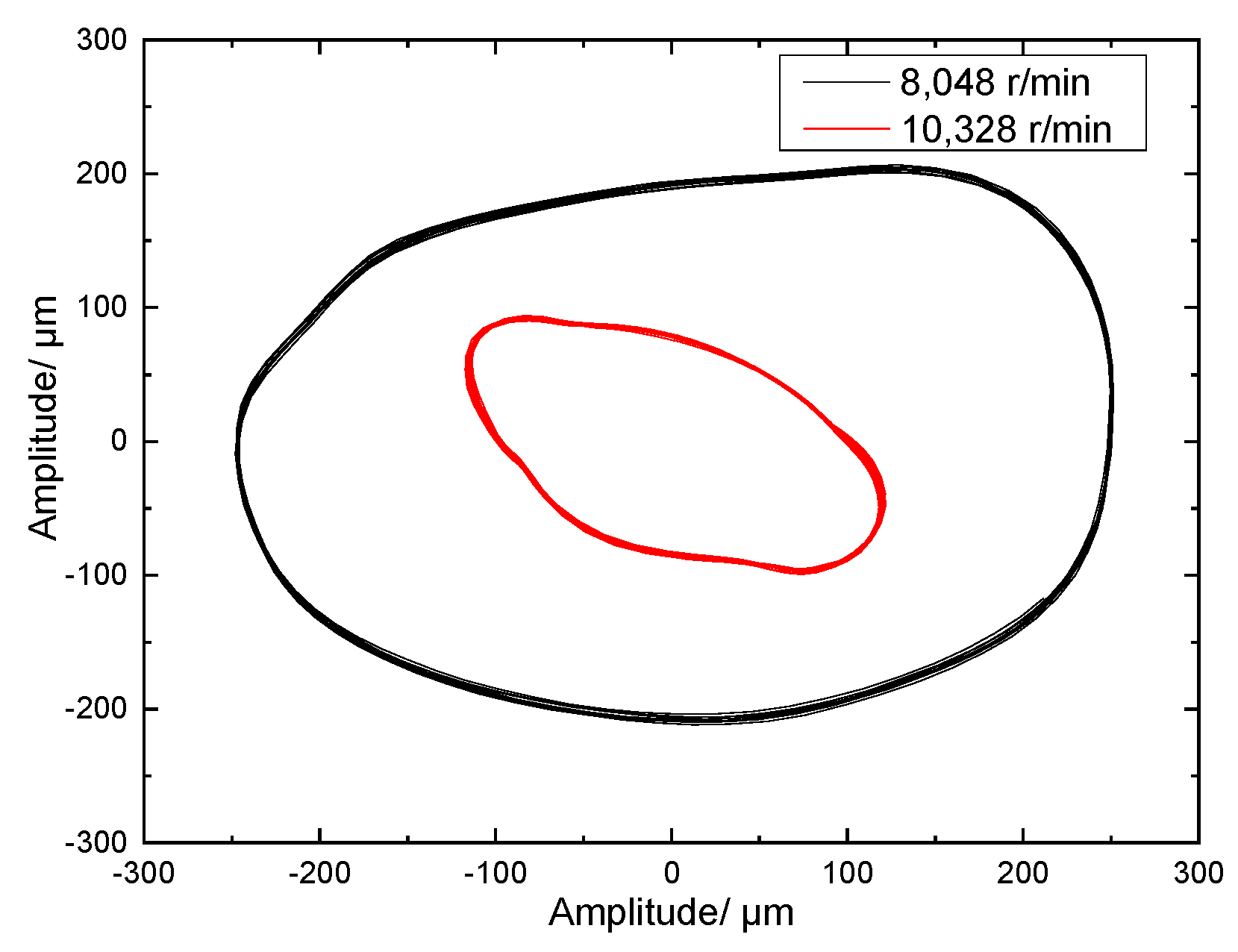
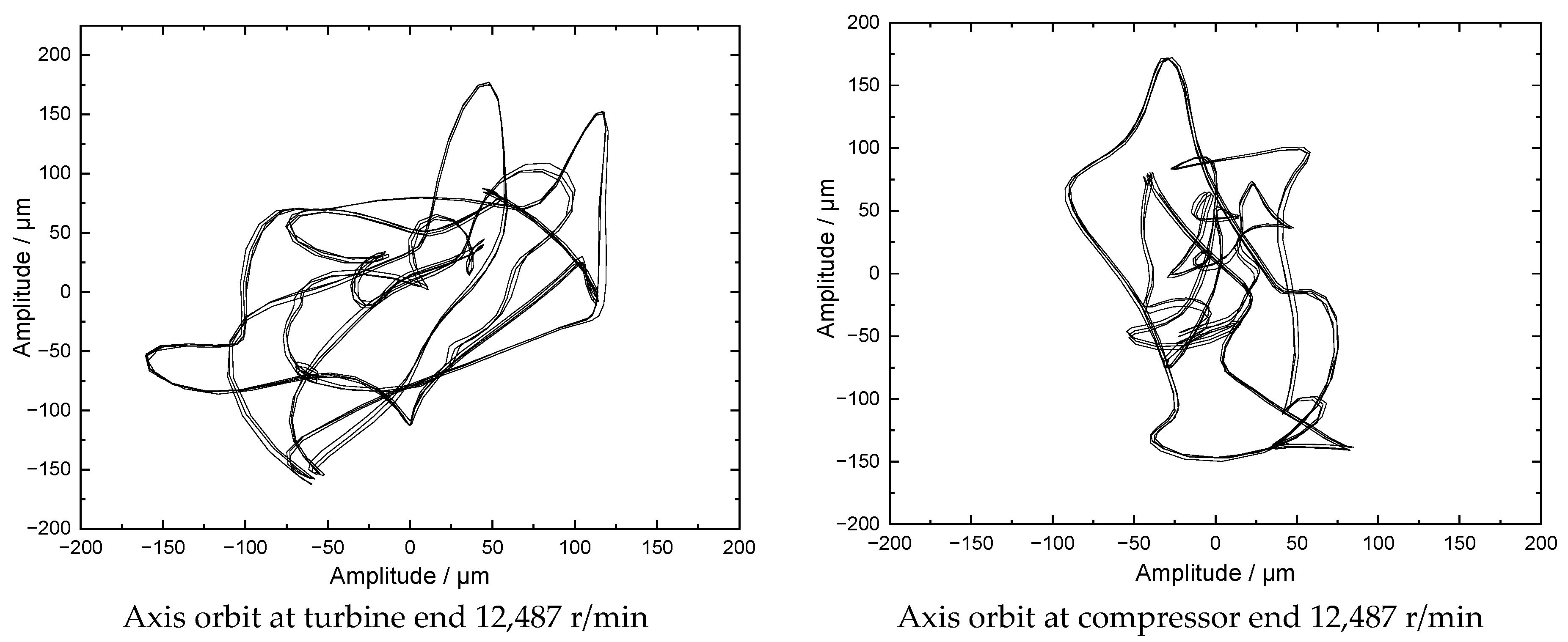
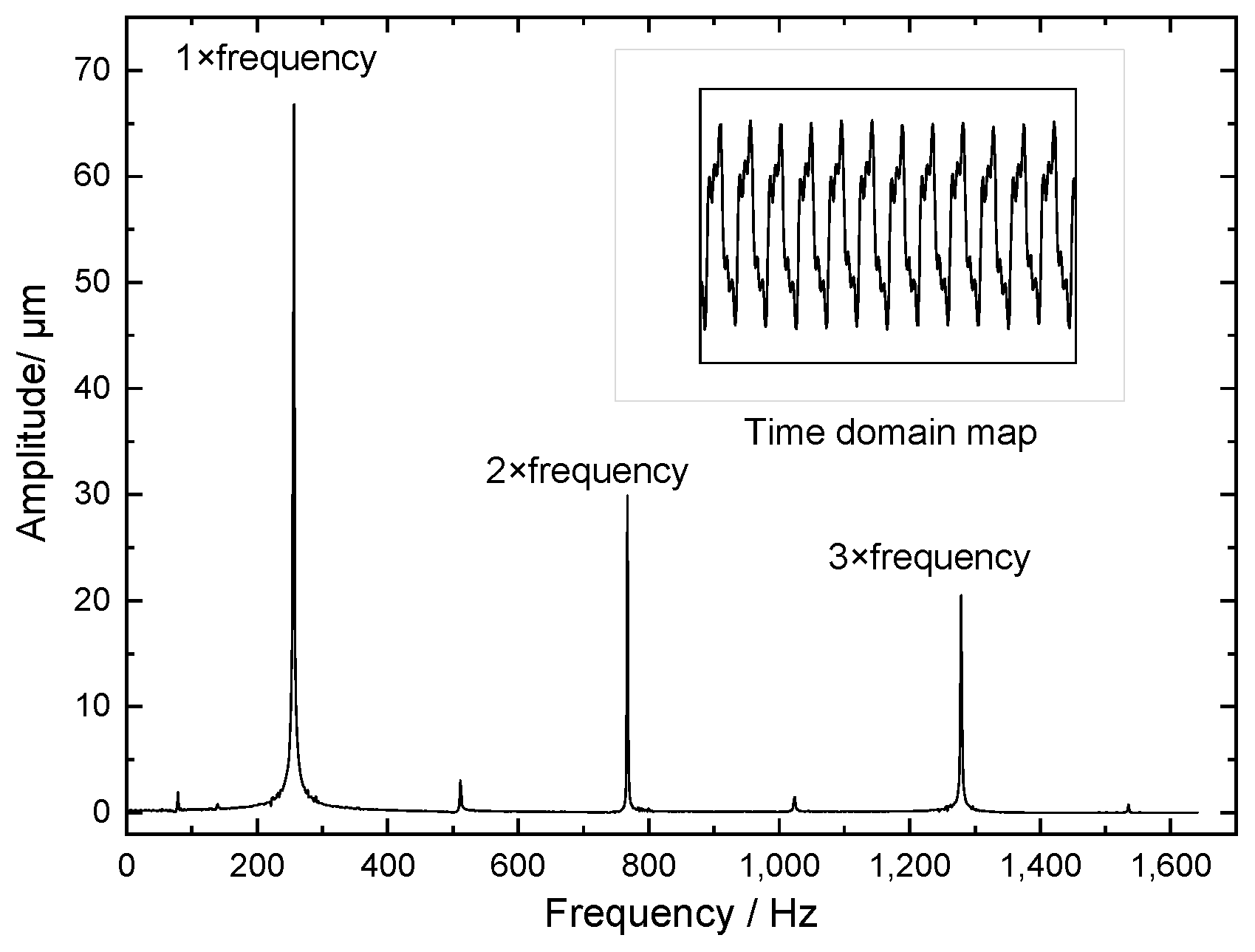
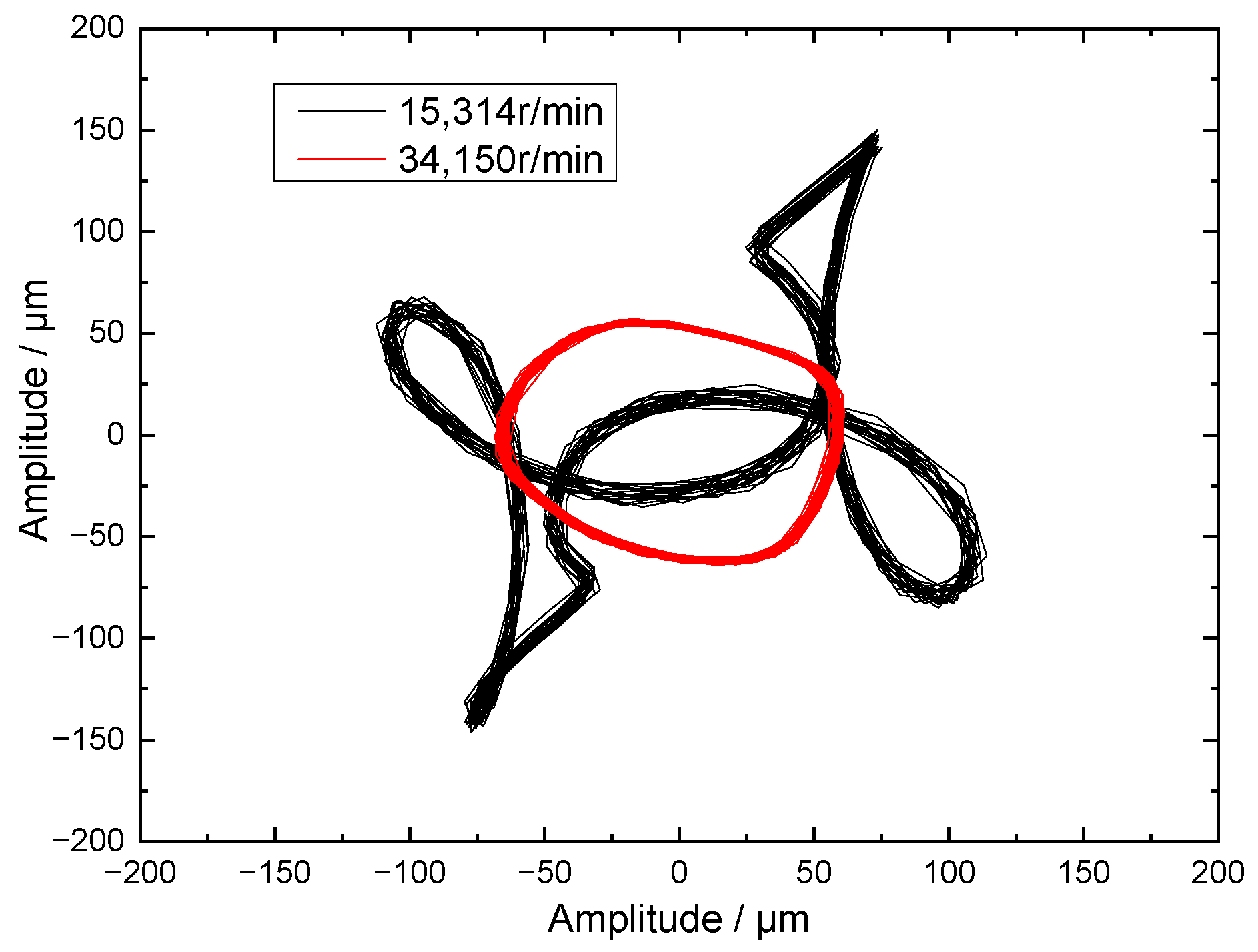
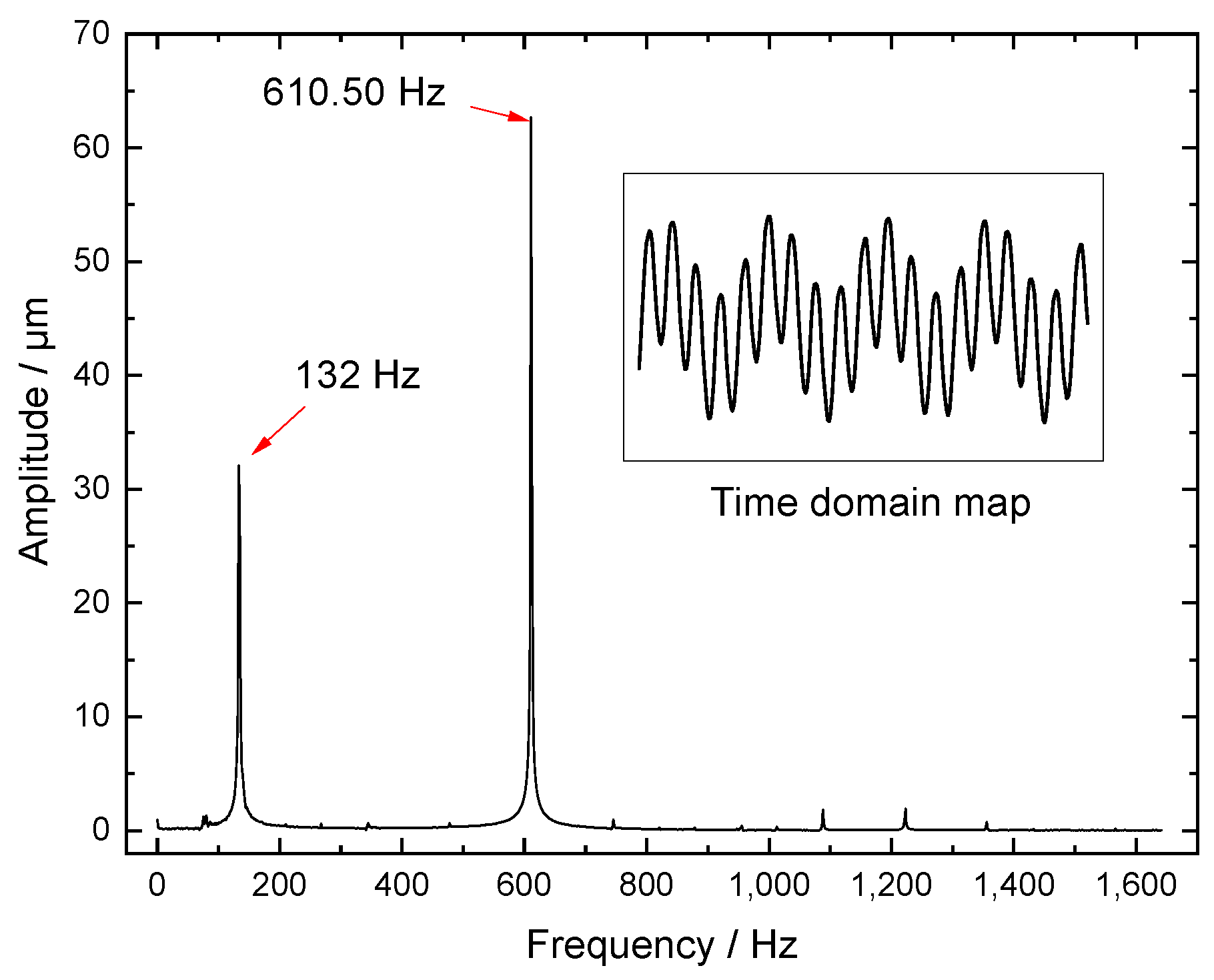
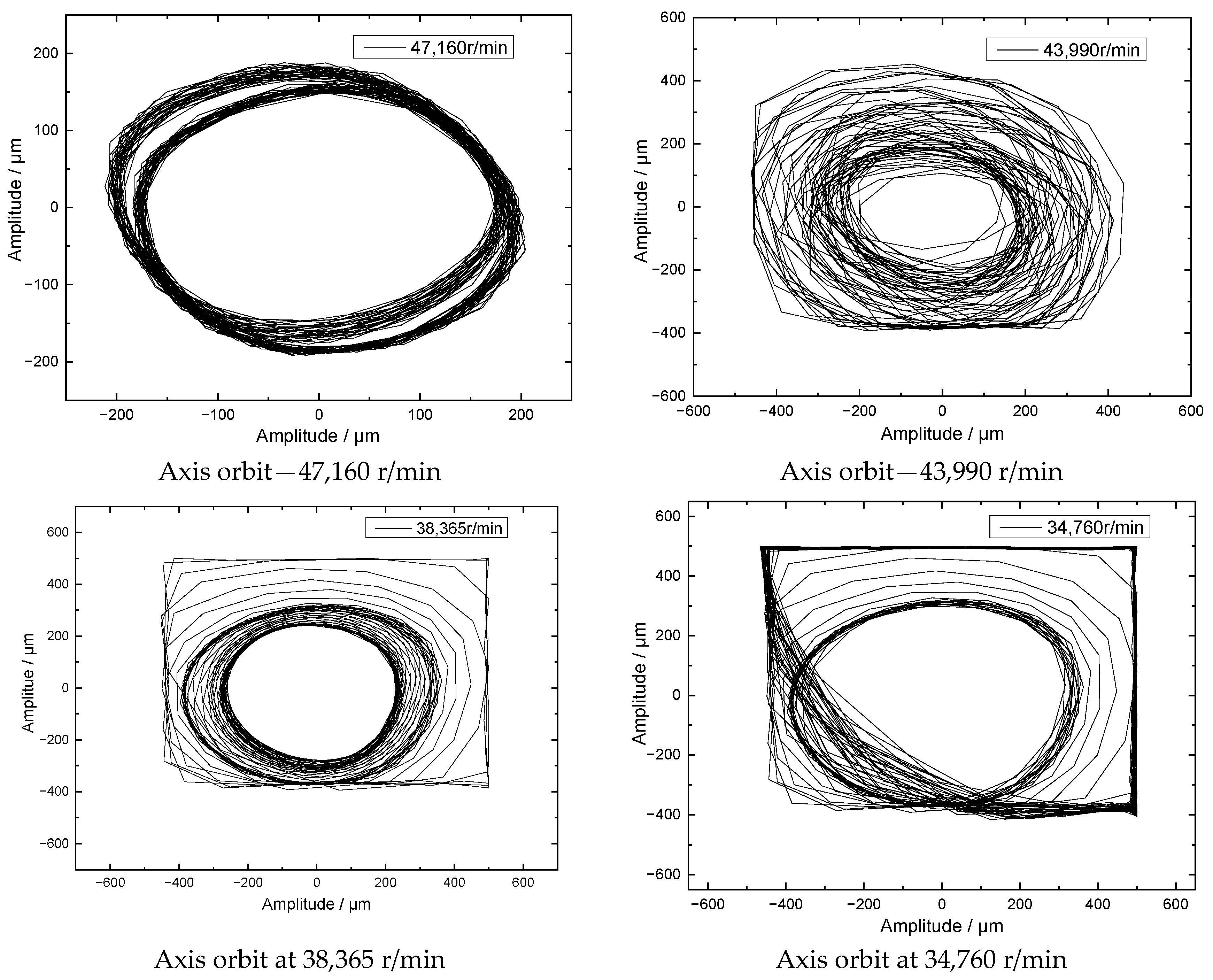
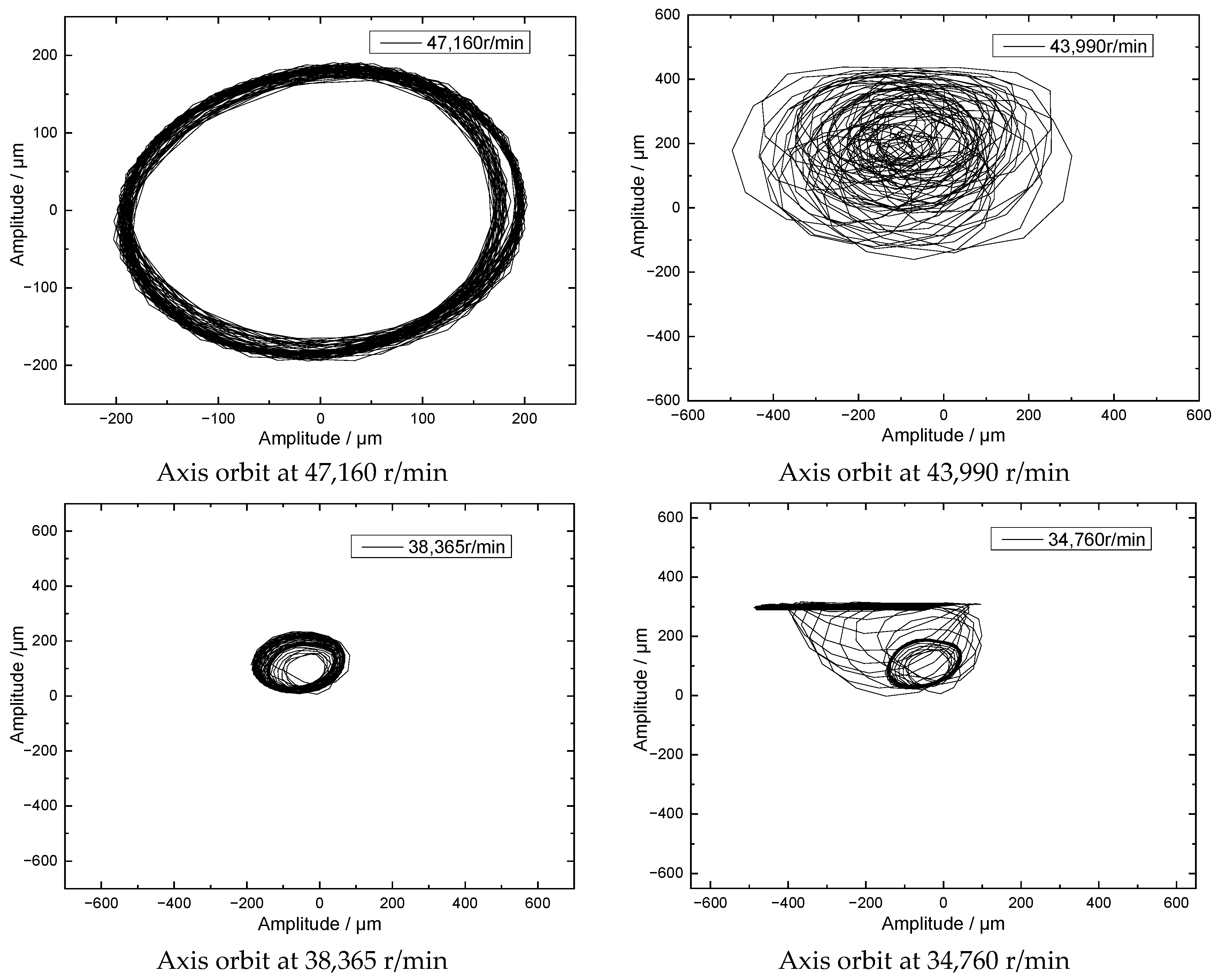
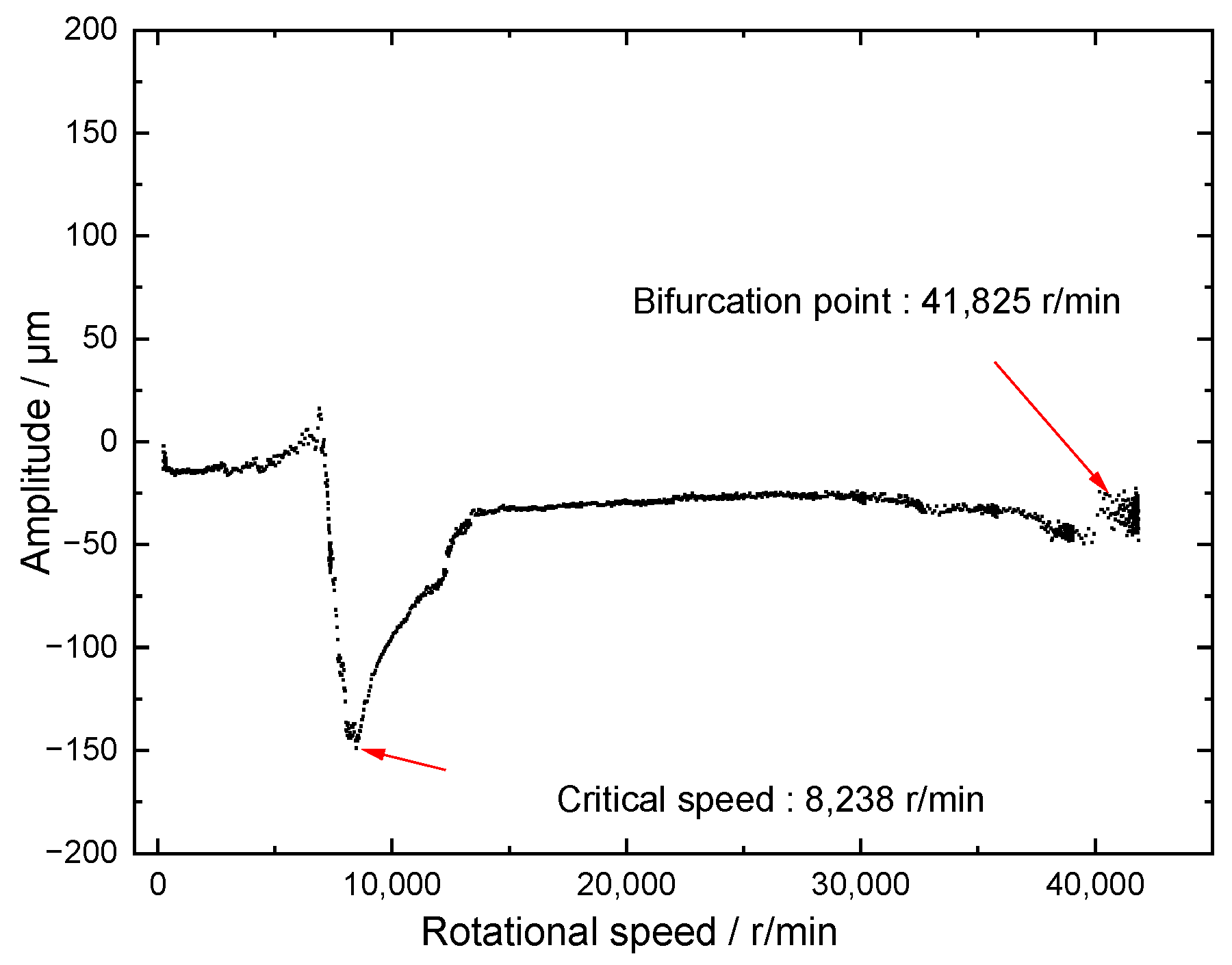
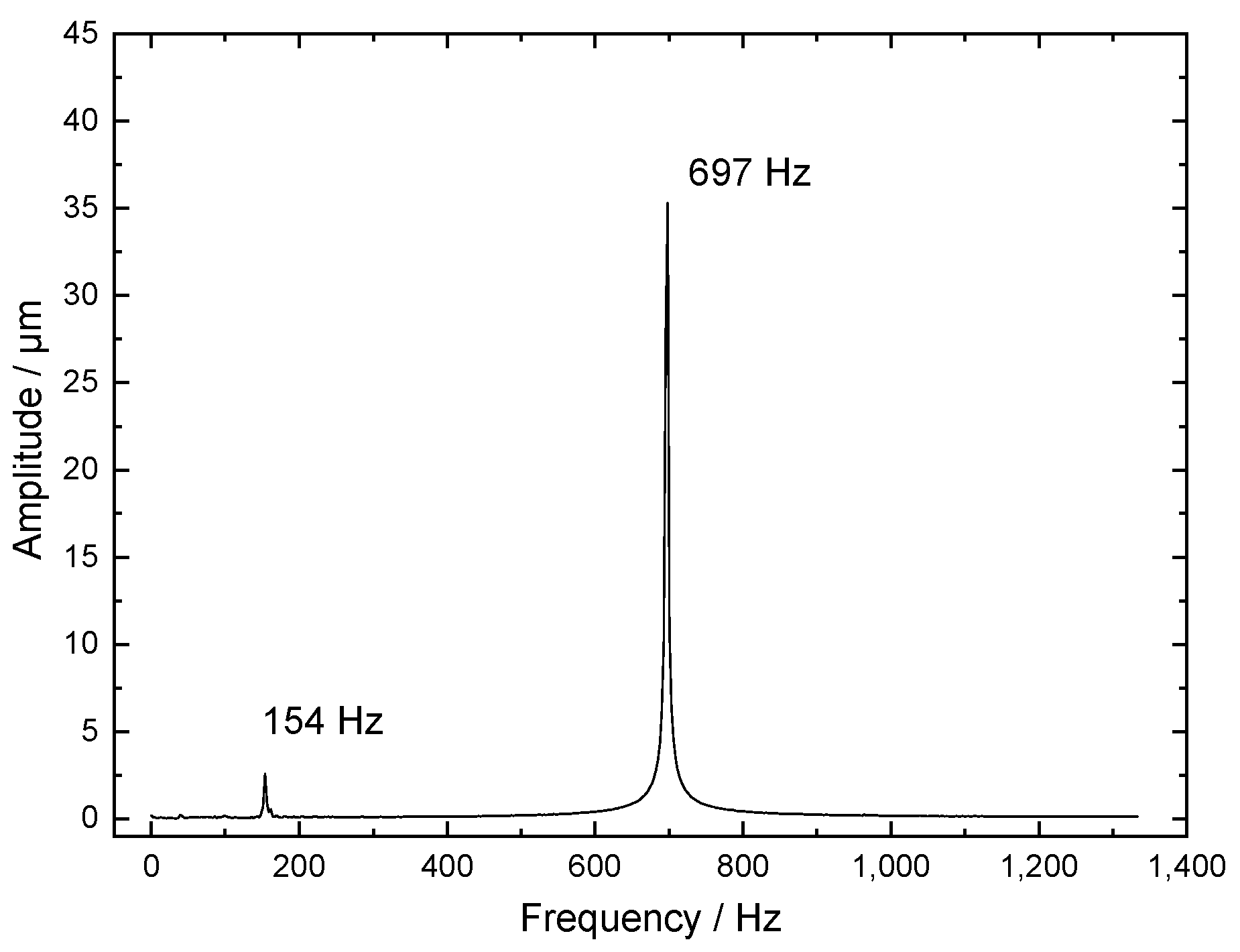
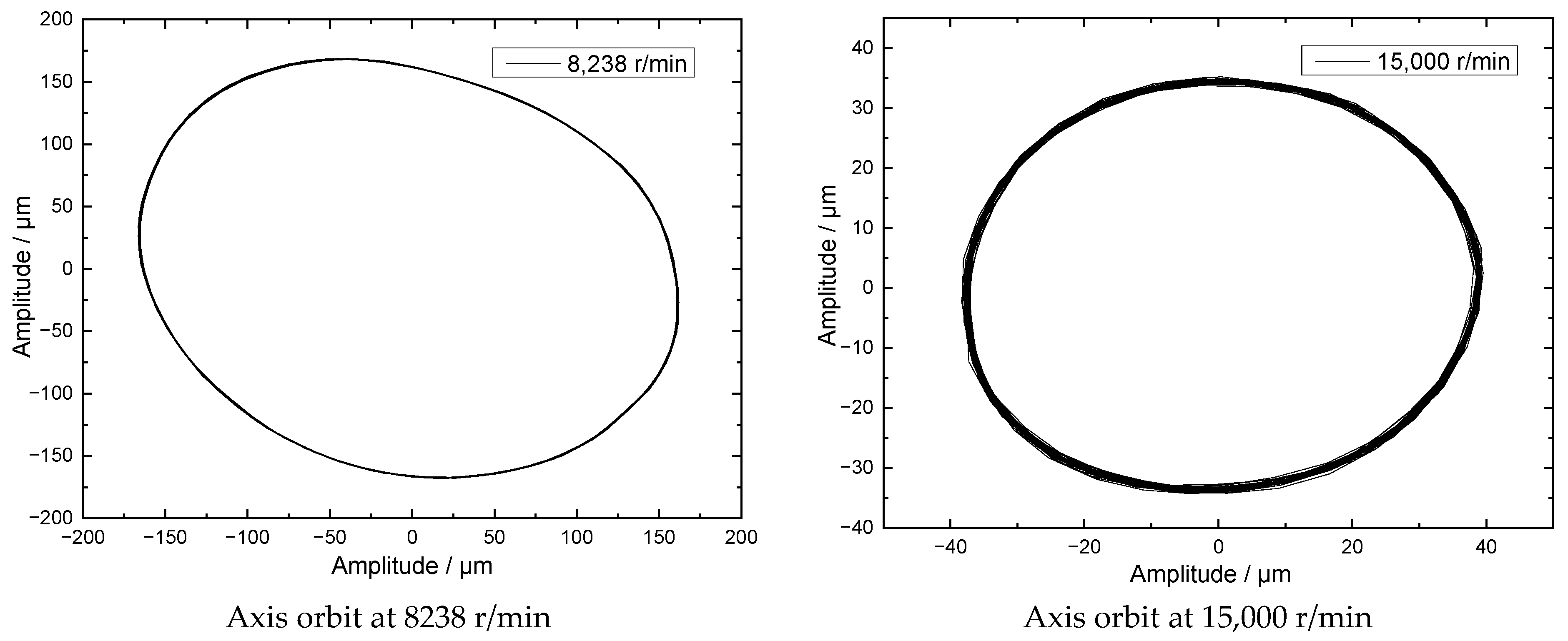
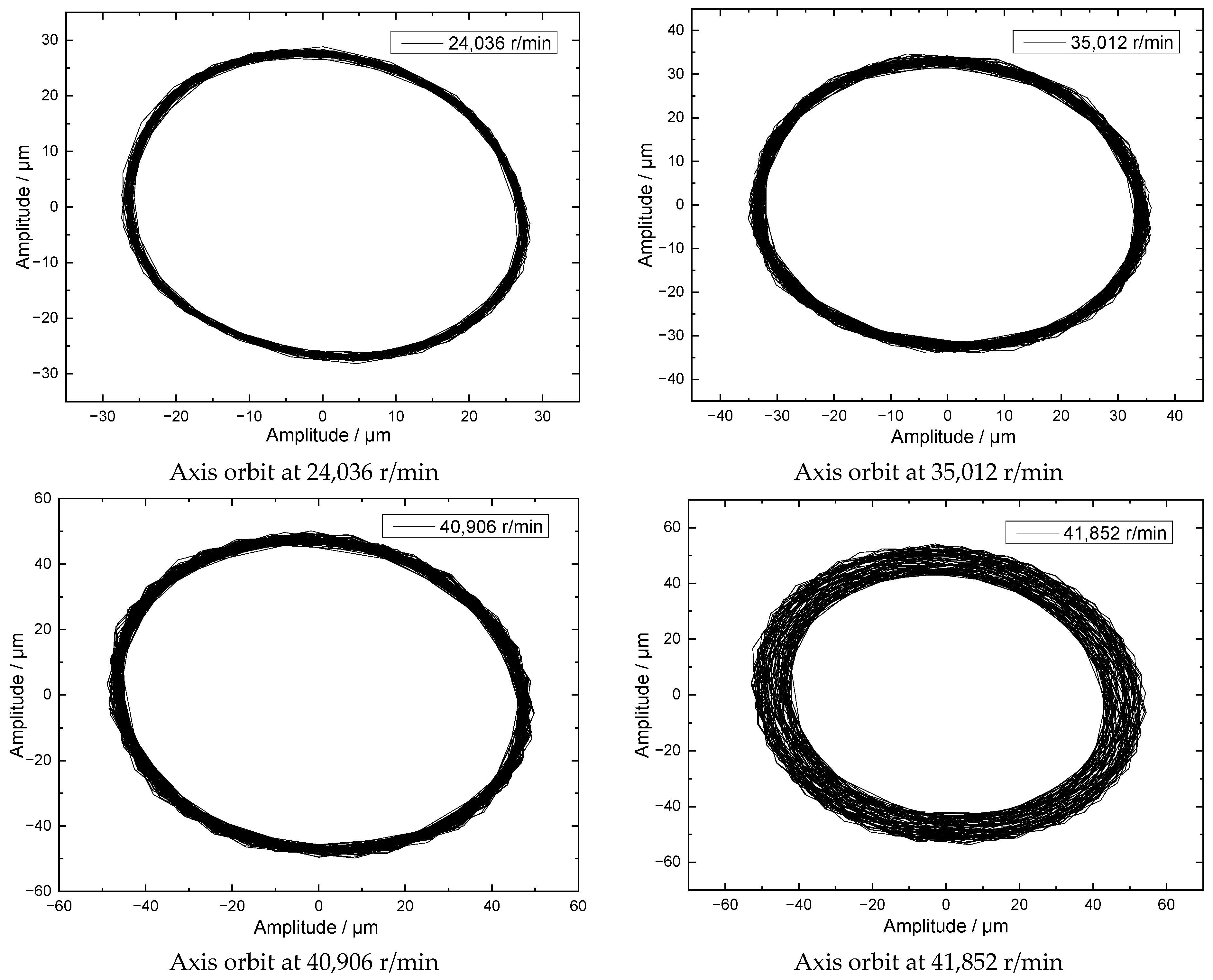
4.1. Experimental Results of No. 1
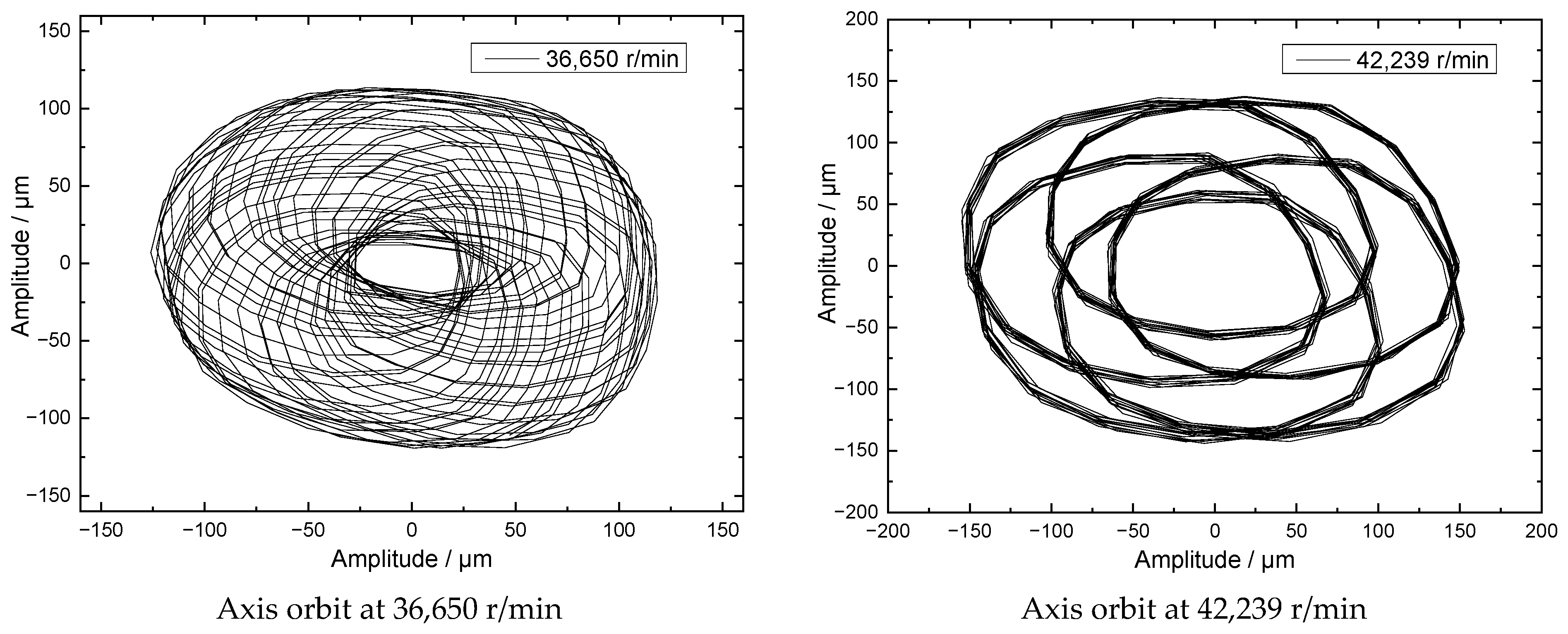
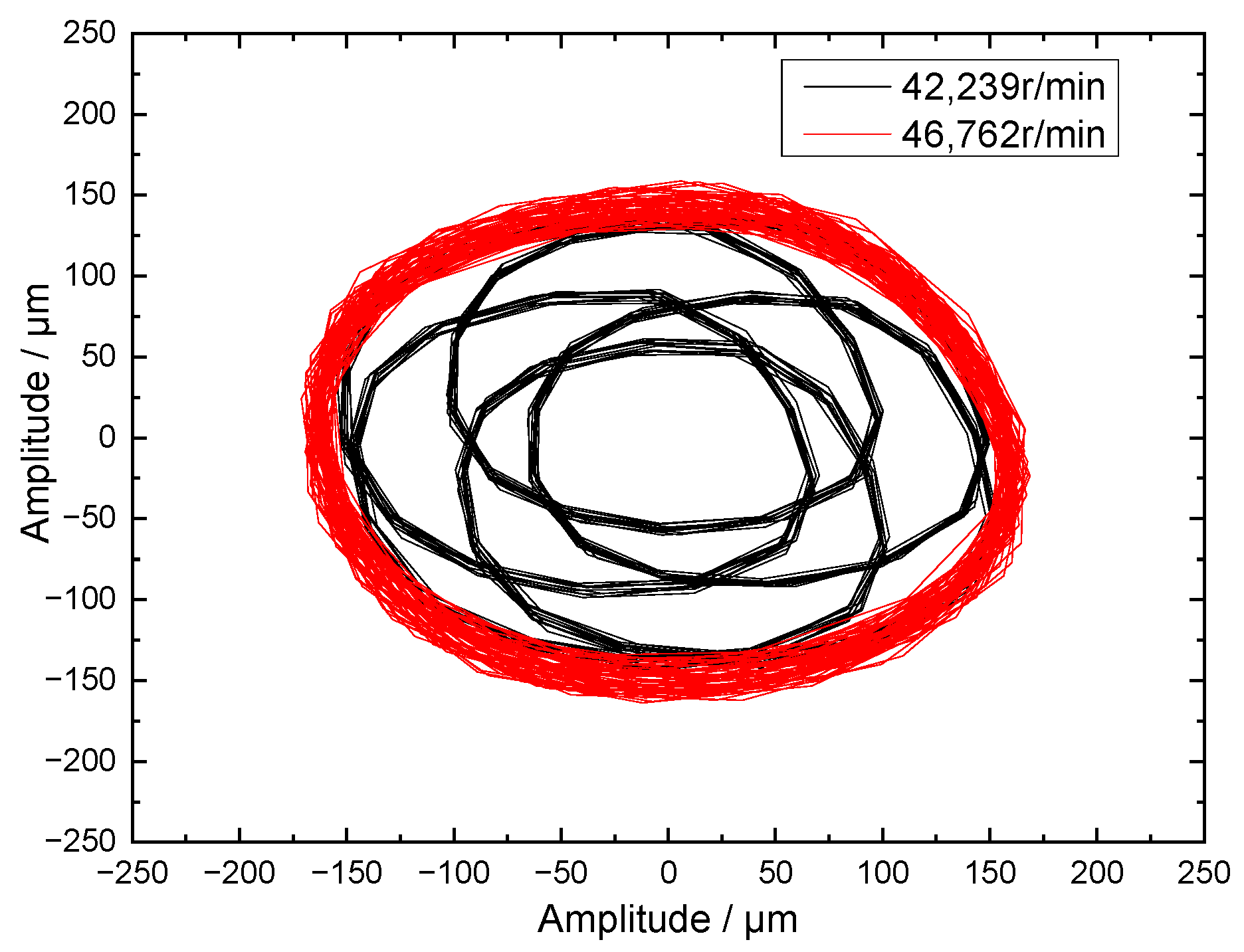
4.2. Experimental Results of No. 2
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gao, Q.; Chen, W.; Lu, L.; Huo, D.; Cheng, K. Aerostatic bearings design and analysis with the application to precision engineering: State of the art and future perspectives. Tribol. Int. 2019, 135, 1–17. [Google Scholar] [CrossRef]
- Shinnoh, Y.H. Design concept and structural configuration of advanced nano-pattren generator with large work area “Angel”. Int. J. Autom. 2011, 5, 38–44. [Google Scholar]
- Xu, F.; Dong, Z.; Zhang, H.; Xie, Z. Vibration characteristics control of hybrid radial gas foil bearing-rotor system: Simulation and experiment. Mech. Syst. Signal Process. 2023, 198, 110402. [Google Scholar] [CrossRef]
- Wang, P.; Chen, B.; Li, J.; Wang, J.; Zhang, Y.; Feng, K. Novel aerostatic bearings with hermetically squeeze film dampers for the improvement of stability: Theoretical and experimental investigations. Precis. Eng. 2024, 85, 263–278. [Google Scholar] [CrossRef]
- Wang, G.; Li, W.; Liu, G.; Feng, K. A novel optimization design method for obtaining high-performance micro-hole aerostatic bearings with experimental validation. Tribol. Int. 2023, 185, 108542. [Google Scholar] [CrossRef]
- Wang, P.; Zhang, Y.; Feng, L.; Hou, W.; Wang, J.; Li, W.; Feng, K. Study on the pneumatic hammer phenomenon of aerostatic bearings based on the empirical mode method: Numerical and experimental analysis. Tribol. Int. 2023, 181, 108305. [Google Scholar] [CrossRef]
- Feng, K.; Li, J.; Li, W.; Huang, J.; Gao, F.; Sun, J. A novel parallel capillary-cavity model for the analysis of pneumatic hammer vibration in porous aerostatic bearings. Tribol. Int. 2023, 189, 108993. [Google Scholar] [CrossRef]
- Gao, Q.; Qi, L.; Gao, S.; Lu, L.; Song, L.; Zhang, F. A FEM based modeling method for analyzing the static performance of aerostatic thrust bearings considering the fluid-structure interaction. Tribol. Int. 2021, 156, 106849. [Google Scholar] [CrossRef]
- Yu, P.; Huang, L.; Li, S.; Guo, L.; Zhong, M.; Zhang, L. Theoretical predictions and experimental measurements of novel aerostatic bearing with multi-inclined-orifice restrictors for the improvement of stability. Precis. Eng. 2024, 88, 266–278. [Google Scholar] [CrossRef]
- Wu, Y.; Li, C.; Li, J.; Du, J. Lubrication mechanism and characteristics of aerostatic bearing with close-spaced micro holes. Tribol. Int. 2024, 192, 109278. [Google Scholar] [CrossRef]
- Maamari, N.; Krebs, A.; Weikert, S.; Wild, H.; Wegener, K. Stability and dynamics of an orifice based aerostatic bearing with a compliant back plate. Tribol. Int. 2019, 138, 279–296. [Google Scholar] [CrossRef]
- An, L.; Wang, W.; Wang, C. Dynamic modeling and analysis of high-speed aerostatic journal bearing-rotor system with recess. Tribol. Int. 2023, 187, 108686. [Google Scholar] [CrossRef]
- Yang, P.; Zhu, K.; Wang, X. On the non-linear stability of self-acting gas journal bearings. Tribol. Int. 2009, 42, 71–76. [Google Scholar] [CrossRef]
- Zhang, J.; Zou, D.; Ta, N.; Rao, Z. Numerical research of pressure depression in aerostatic thrust bearing with inherent orifice. Tribol. Int. 2018, 123, 385–396. [Google Scholar] [CrossRef]
- Zhang, J.; Zou, D.; Ta, N.; Rao, Z.; Ding, B. A numerical method for solution of the discharge coefficients in externally pressurized gas bearings with inherent orifice restrictors. Tribol. Int. 2018, 125, 156–168. [Google Scholar] [CrossRef]
- Zhang, J.; Yu, F.; Zou, D.; Ta, N.; Rao, Z. Comparison of the characteristics of aerostatic journal bearings considering misalignment under pure-static and hybrid condition. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2019, 233, 769–781. [Google Scholar] [CrossRef]
- Shi, J.; Cao, H.; Jin, X. Dynamics of 5-DOF aerostatic spindle with time-varying coefficients of air bearing. Mech. Syst. Signal Process. 2022, 172, 109005. [Google Scholar] [CrossRef]
- Shi, J.; Jin, X.; Cao, H. Chatter stability analysis in Micro-milling with aerostatic spindle considering speed effect. Mech. Syst. Signal Process. 2022, 169, 108620. [Google Scholar] [CrossRef]
- Shi, J.; Cao, H.; Jian, X. Investigation on the static and dynamic characteristics of 3-DOF aerostatic thrust bearings with orifice restrictor. Tribol. Int. 2019, 138, 435–449. [Google Scholar] [CrossRef]
- Chen, C.; Yang, J.; Nie, C. Experimental research on nonlinear dynamic characteristic of gas lubrication bearings and high speed rotors. J. Aerosp. Power 2008, 23, 1413–1419. [Google Scholar]
- Bian, S.; Wang, Z.; Yang, J.; Yu, M.; Bian, J. Experiment on axial clearance on high-speed dual-turbine rotor stability. J. Aerosp. Power 2017, 32, 1091–1098. [Google Scholar]
- Yang, J.; Chen, C.; Liu, Y.; Han, D. An engineering stability criterion and its experimental validation of frequency couple modulation for sliding rotor-bearing system. J. Vib. Shock 2010, 29, 97–104. [Google Scholar]
- Chen, Y.; Liu, Y.; Yang, W.; Wang, Y.; Zhang, L.; Wu, Y. Research on optimization and verification of the number of stator blades of kW ammonia working medium radial flow turbine in ocean thermal energy conversion. J. Mar. Sci. Eng. 2021, 9, 901. [Google Scholar] [CrossRef]
- Bischoff, S.; Decker, L. First operating results of a dynamic gas bearing turbine in an industrial hydrogen liquefier. AIP Conf. Proc. 2010, 1218, 887. [Google Scholar]
- Ohlig, K.; Bischoff, S. Dynamic gas bearing turbine technology in hydrogen plants. In Advances in Cryogenic Engineering. Transactions of the Cryogenic Engineering Conference—CEC; American Institute of Physics AIP: College Park, MD, USA, 2012; Volume 57. [Google Scholar]
- Shi, T.; Xiong, W.; Peng, X.; Feng, J.; Guo, Y. Experimental investigation on the start-stop performance of gas foil bearing-rotor system in the centrifugal air compressor for hydrogen fuel cell vehicles. Int. J. Hydrogen Energy 2023, 48, 34501–34519. [Google Scholar] [CrossRef]
- Shi, T.; Wang, H.; Yang, W.; Peng, X. Mathematical modeling and optimization of gas foil bearings-rotor system in hydrogen fuel cell vehicles. Energy 2024, 290, 130129. [Google Scholar] [CrossRef]
- Stoop, F.; Meier, S.; Civelli, P.; Mayr, J.; Wegener, K. Multi-variable rotor dynamics optimization of an aerostatic spindle. CIRP J. Manuf. Sci. Technol. 2023, 42, 12–23. [Google Scholar] [CrossRef]
- Belforte, G.; Colombo, F.; Raparelli, T.; Trivella, A.; Viktorov, V. High-speed electrospindle running on air bearing: Design and experimental verification. Meccanica 2008, 43, 591–600. [Google Scholar] [CrossRef]
- Han, D.; Tang, C.; Hao, L.; Yang, J. Experimental studies on the effects of bearing supply gas pressure on the response of a permanent magnet disk-type motor rotor. J. Mech. Sci. Technol. 2016, 30, 4887–4892. [Google Scholar] [CrossRef]
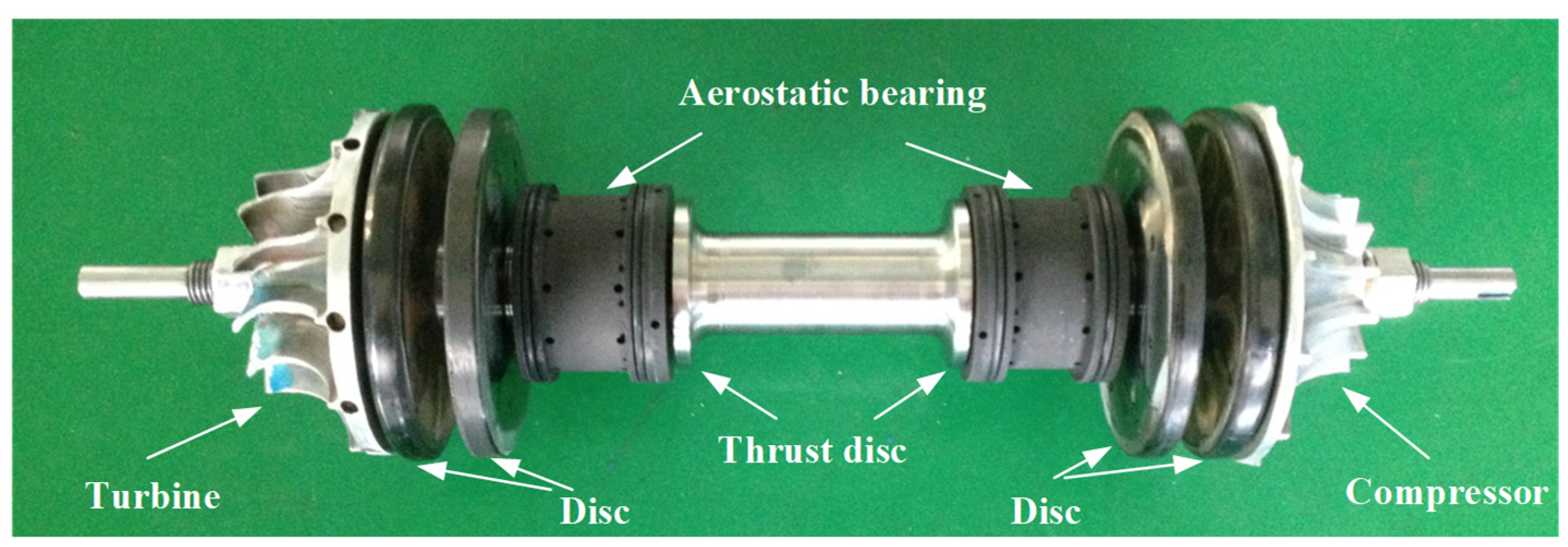
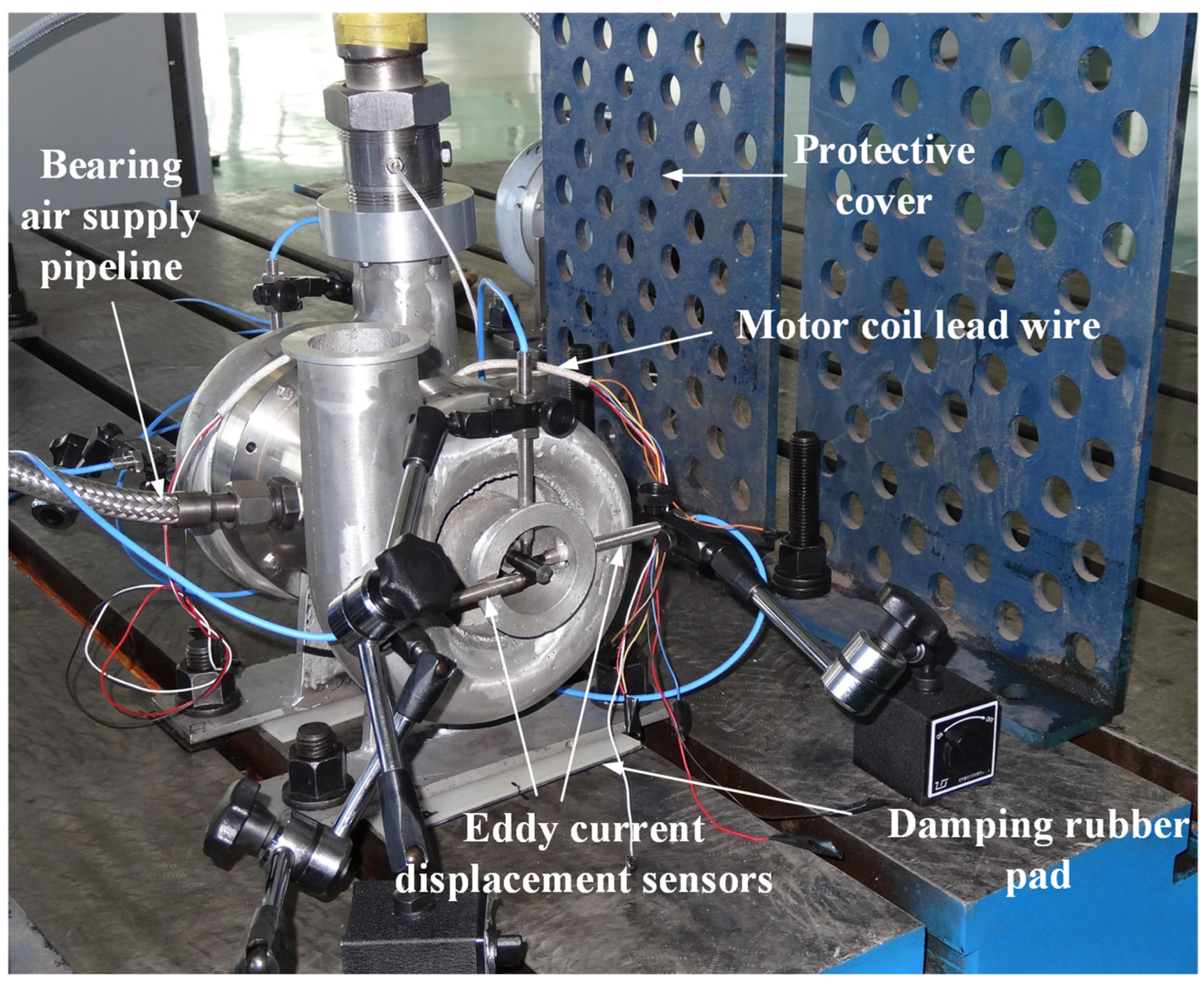






| Parameters | Value | Unit |
|---|---|---|
| Shaft mass | 896.0 | g |
| Disc mass | 310.0 | g |
| Turbine mass | 169.0 | g |
| Compressor mass | 136.0 | g |
| Total rotor mass | 2602.0 | g |
| Bearing span | 112.7 | mm |
| Medial disc span | 169.0 | mm |
| Outer disc span | 195.3 | mm |
| Number of magnets | 8 | / |
| Impeller mass center distance | 249.80 | mm |
| Bearing inner diameter | 25.0 | mm |
| Bearing length | 40.0 | mm |
| Number of throttle rows | 2 | / |
| Number of throttling holes per row | 10 | / |
| Throttle hole diameter | 0.30 | mm |
| O-ring diameter | 3.10 | mm |
| Experimental Scheme | Bearing Supply Pressure/MPa | |
|---|---|---|
| Aerostatic Bearing at Compressor End (Set Value/Actual Value) | Aerostatic Bearing at Turbine End (Set Value/Actual Value) | |
| No. 1 | 0.60 MPa/0.61 MPa | 0.60 MPa/0.60 MPa |
| No. 2 | 0.75 MPa/0.76 MPa | 0.75 MPa/0.76 MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, Z.; Zhang, J.; Cai, Y.; Han, D. Experimental Research on Dynamic Characteristics of a Multi-Disc Rotor System Supported by Aerostatic Bearings. Lubricants 2024, 12, 151. https://doi.org/10.3390/lubricants12050151
Su Z, Zhang J, Cai Y, Han D. Experimental Research on Dynamic Characteristics of a Multi-Disc Rotor System Supported by Aerostatic Bearings. Lubricants. 2024; 12(5):151. https://doi.org/10.3390/lubricants12050151
Chicago/Turabian StyleSu, Zhimin, Jianbo Zhang, Yimou Cai, and Dongjiang Han. 2024. "Experimental Research on Dynamic Characteristics of a Multi-Disc Rotor System Supported by Aerostatic Bearings" Lubricants 12, no. 5: 151. https://doi.org/10.3390/lubricants12050151
APA StyleSu, Z., Zhang, J., Cai, Y., & Han, D. (2024). Experimental Research on Dynamic Characteristics of a Multi-Disc Rotor System Supported by Aerostatic Bearings. Lubricants, 12(5), 151. https://doi.org/10.3390/lubricants12050151







