Parametric Optimization of Surface Textures in Oil-Lubricated Long-Life Aircraft Valves
Abstract
1. Introduction
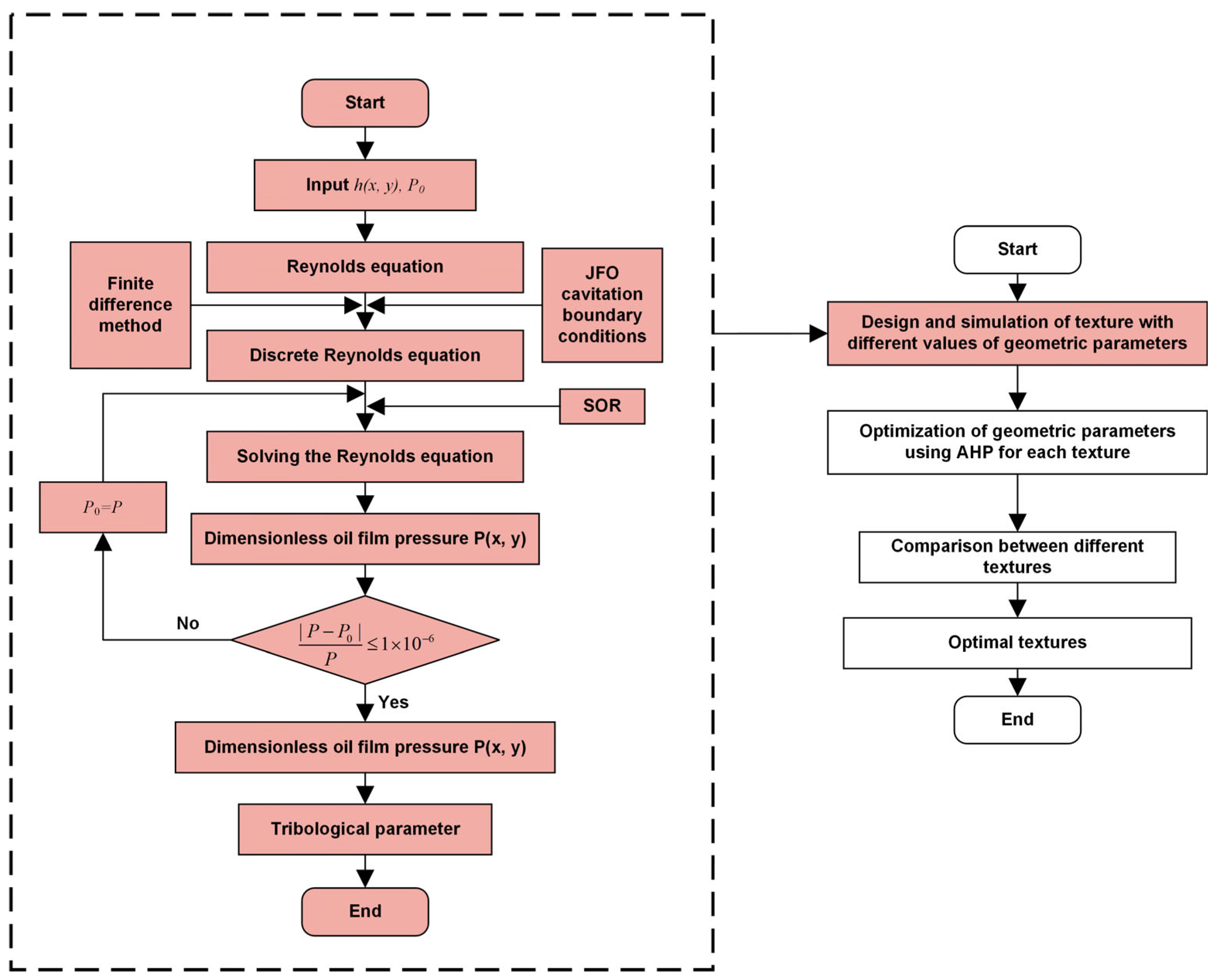
2. Lubrication Model
2.1. Reynolds Equation with JFO Boundary Condition
2.2. Parameters Evaluating Tribological Performance
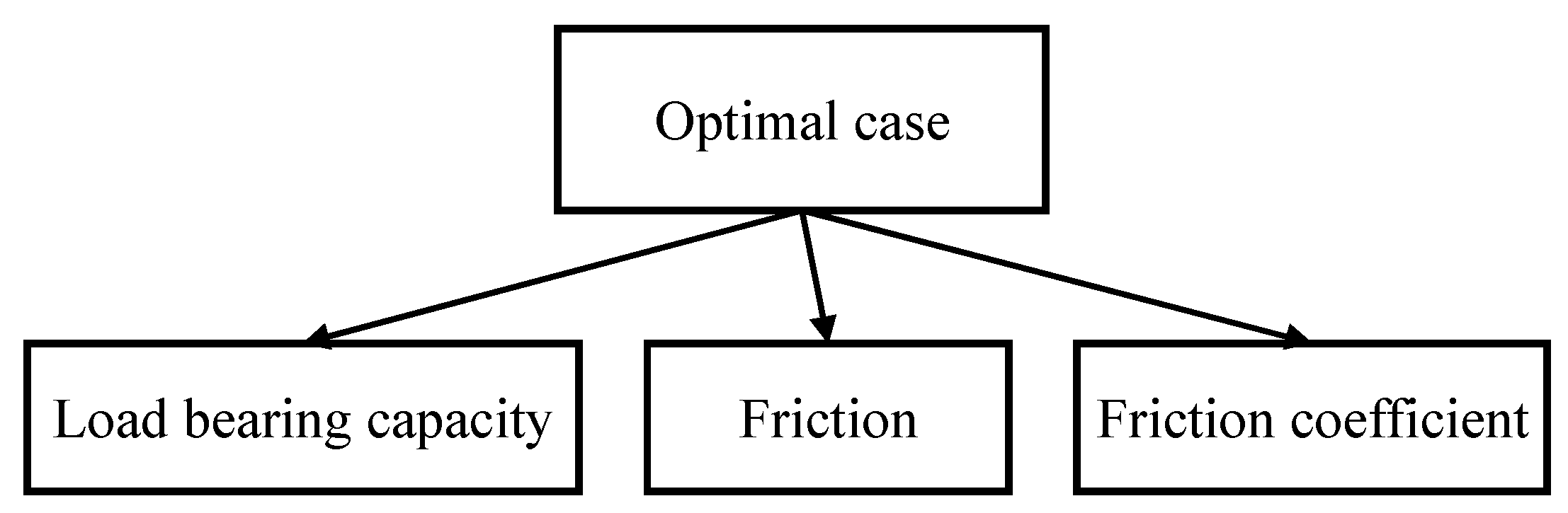
2.3. Optimization Using AHP
- (1)
- Definition of degrees of importance
- The to-be-optimized system is generally affected by more than one factor, while some factors may affect the system more severely compared to other factors. Hence, AHP requires definition of degrees of importance to characterize the significance of each factor in affecting the system.
- (2)
- Construction of judgement matrix
- The judgement matrix facilitates pairwise comparison between any two studied factors, and each value in it is calculated based on the degrees of importance and defines the relative importance between two factors.
- (3)
- Consistency check
- Consistency check is used to ensure logical consistency in the pairwise comparison between any two factors, by calculating the consistency index (CI) and consistency ratio (CR) based on the judgement matrix. If the CR is less than the commonly accepted threshold of 0.1, the judgment matrix is considered to have acceptable consistency, indicating that the pairwise comparisons are logically coherent.
- (4)
- Optimization using a coupled factor
- A coupled factor can then be defined using weighted sum of all factors, whereby the weight of each factor takes the corresponding value in the normalized eigen factor of the judgement matrix. Parametric optimization accounting for multiple factors is then transformed to be maximizing or minimizing the coupled factor.
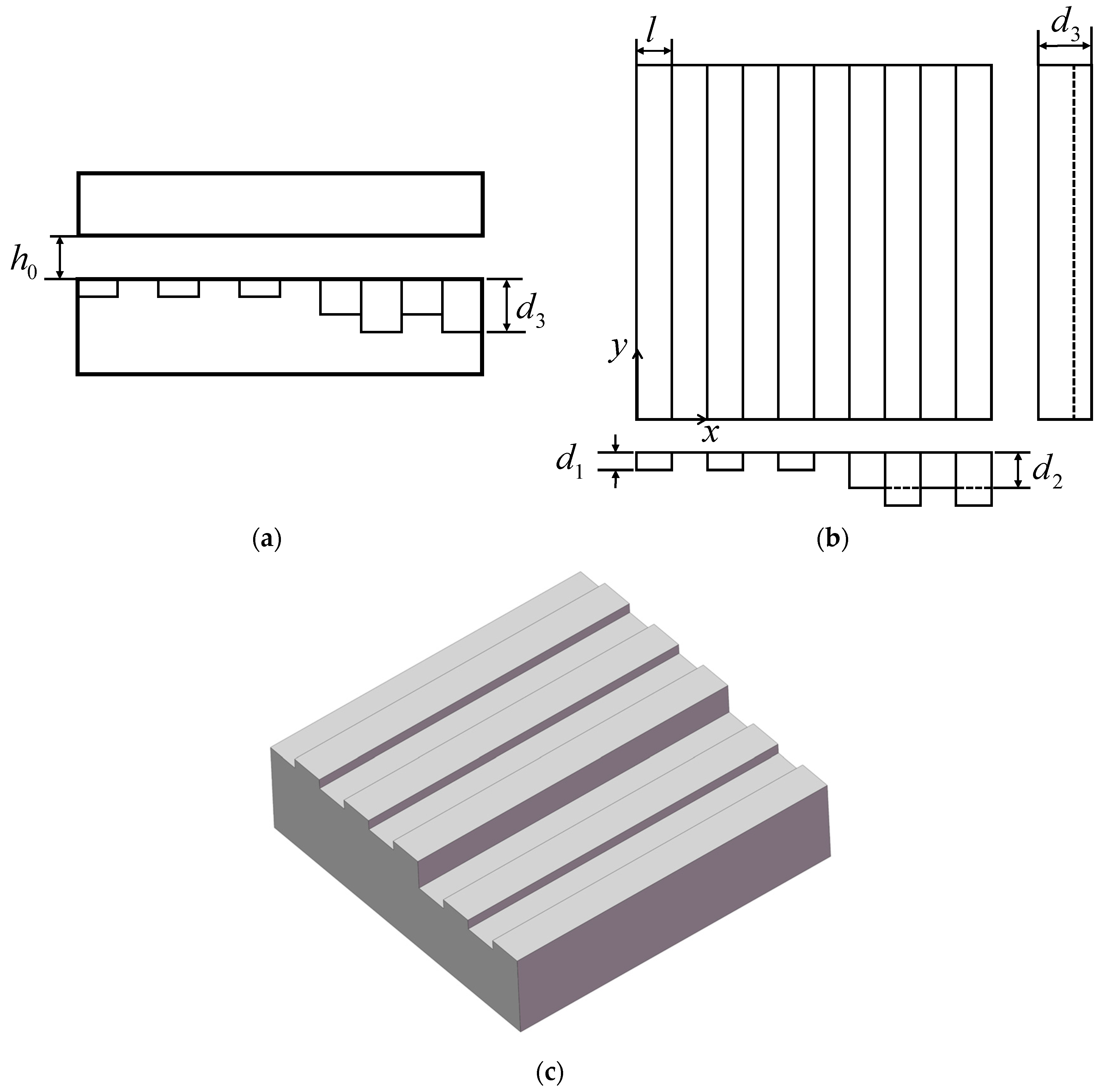
3. Modeling of Surface Textures
3.1. Geometric Models of Surface Textures
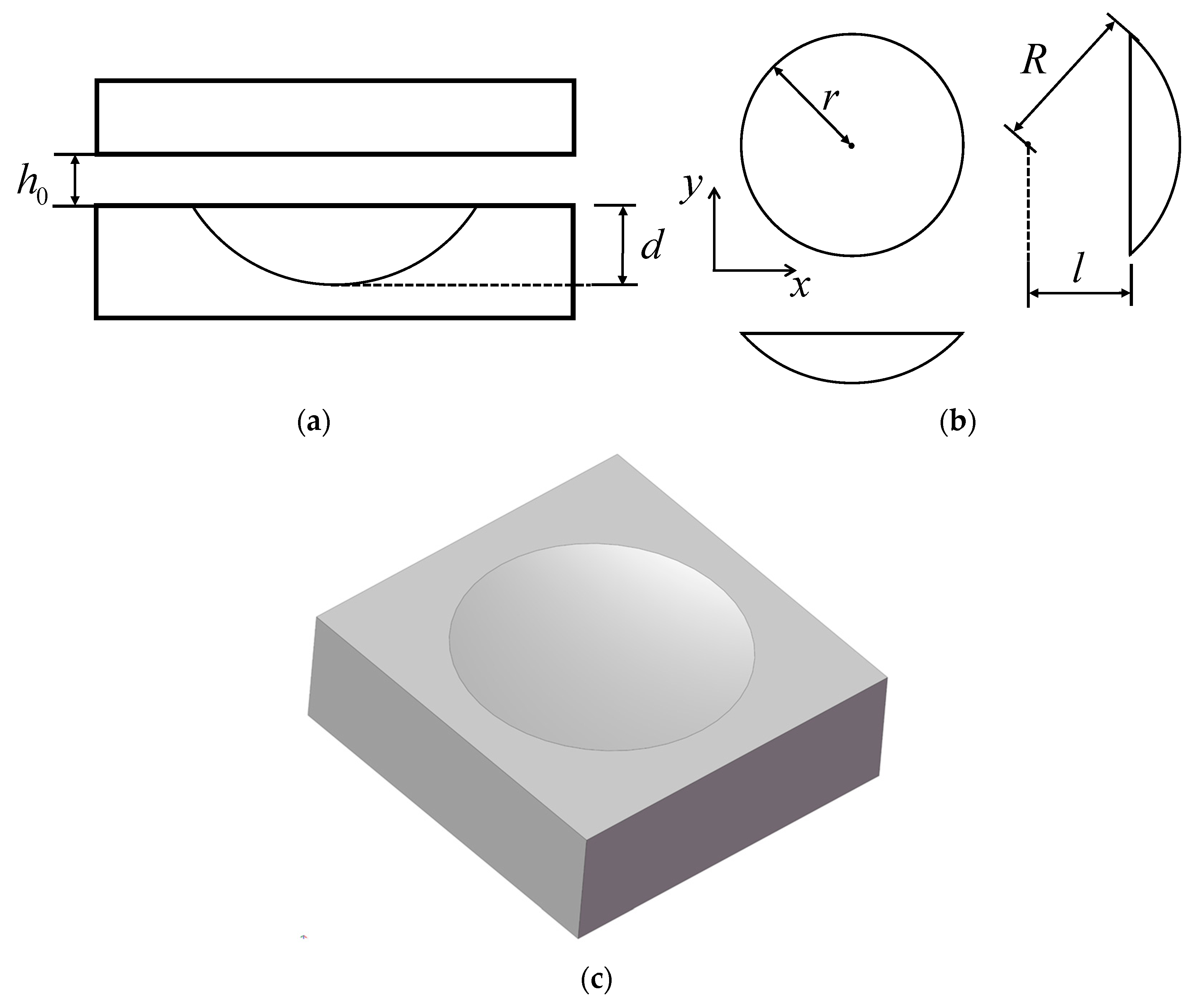
3.1.1. Spherical Cap
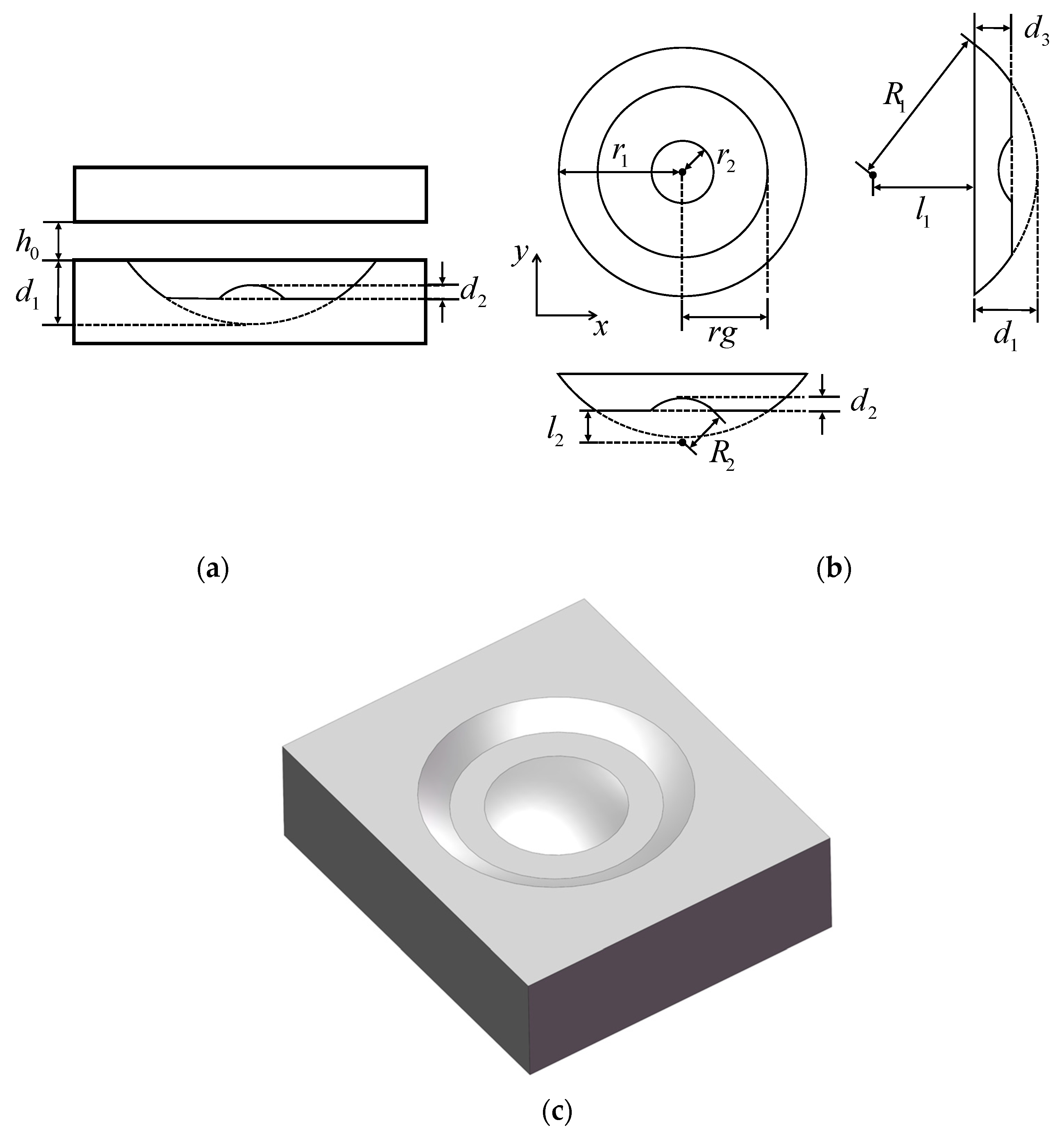
3.1.2. Ellipsoidal Cap
3.1.3. Tree-Frog
3.1.4. Grass-Lip
3.1.5. Nepenthes
3.2. Arrangements for Parametric Optimization
4. Parametric Optimization of Surface Textures: Results and Discussion
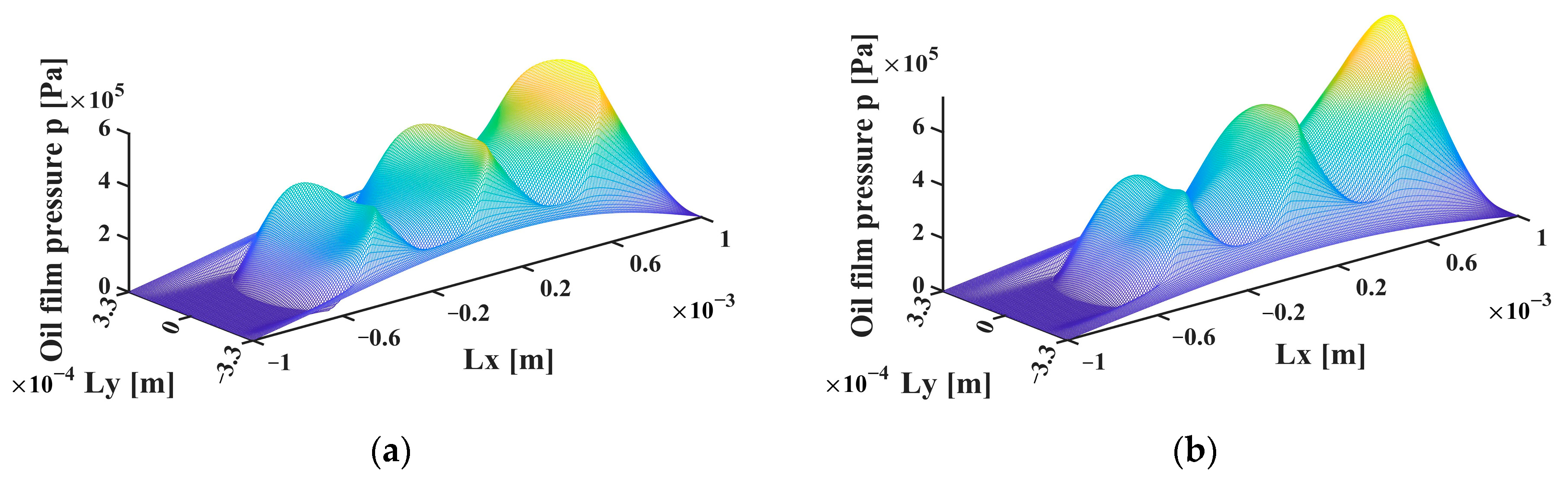
4.1. Convergence of Numerical Simulation Results
4.2. Optimization of Geometric Parameters
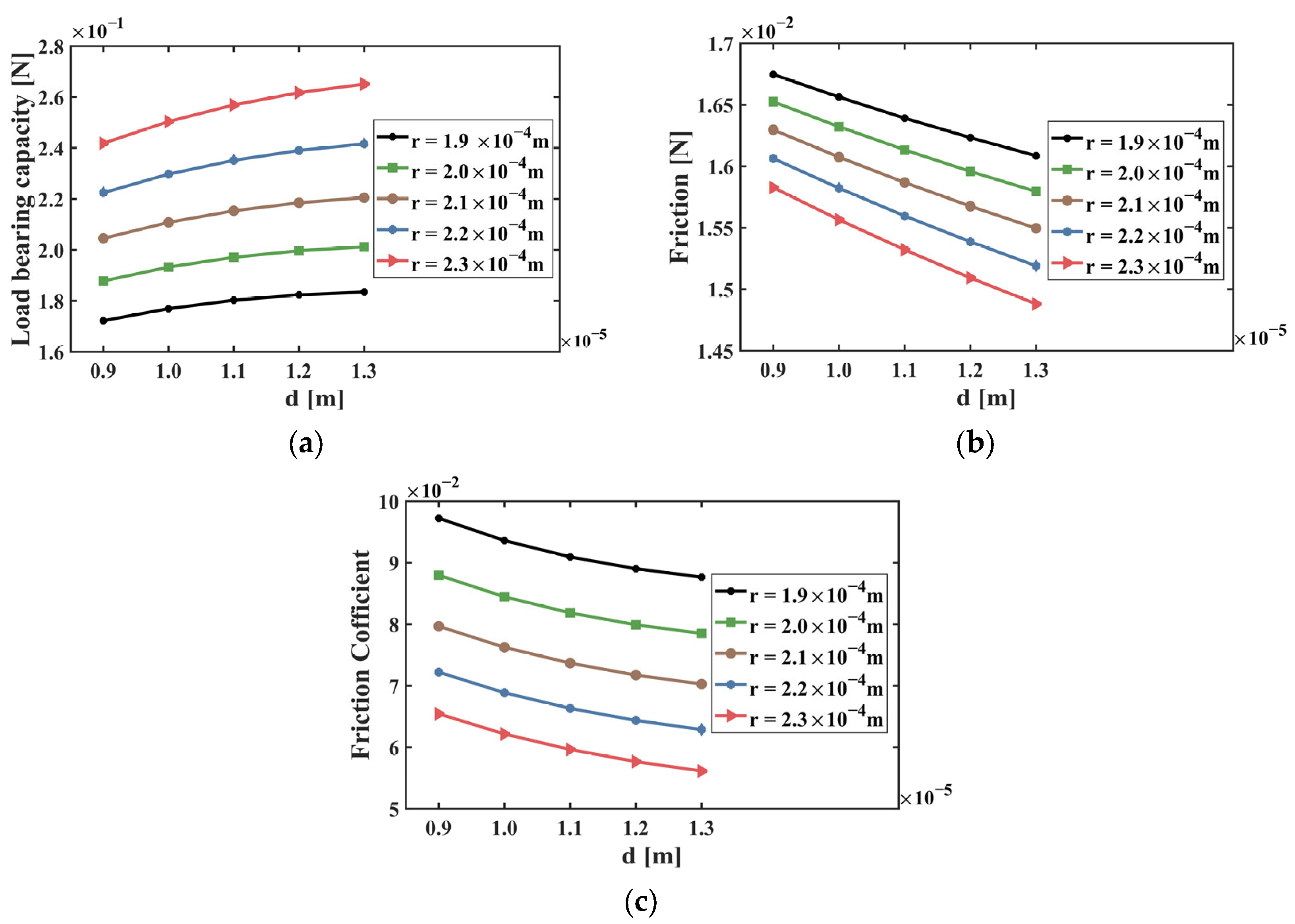
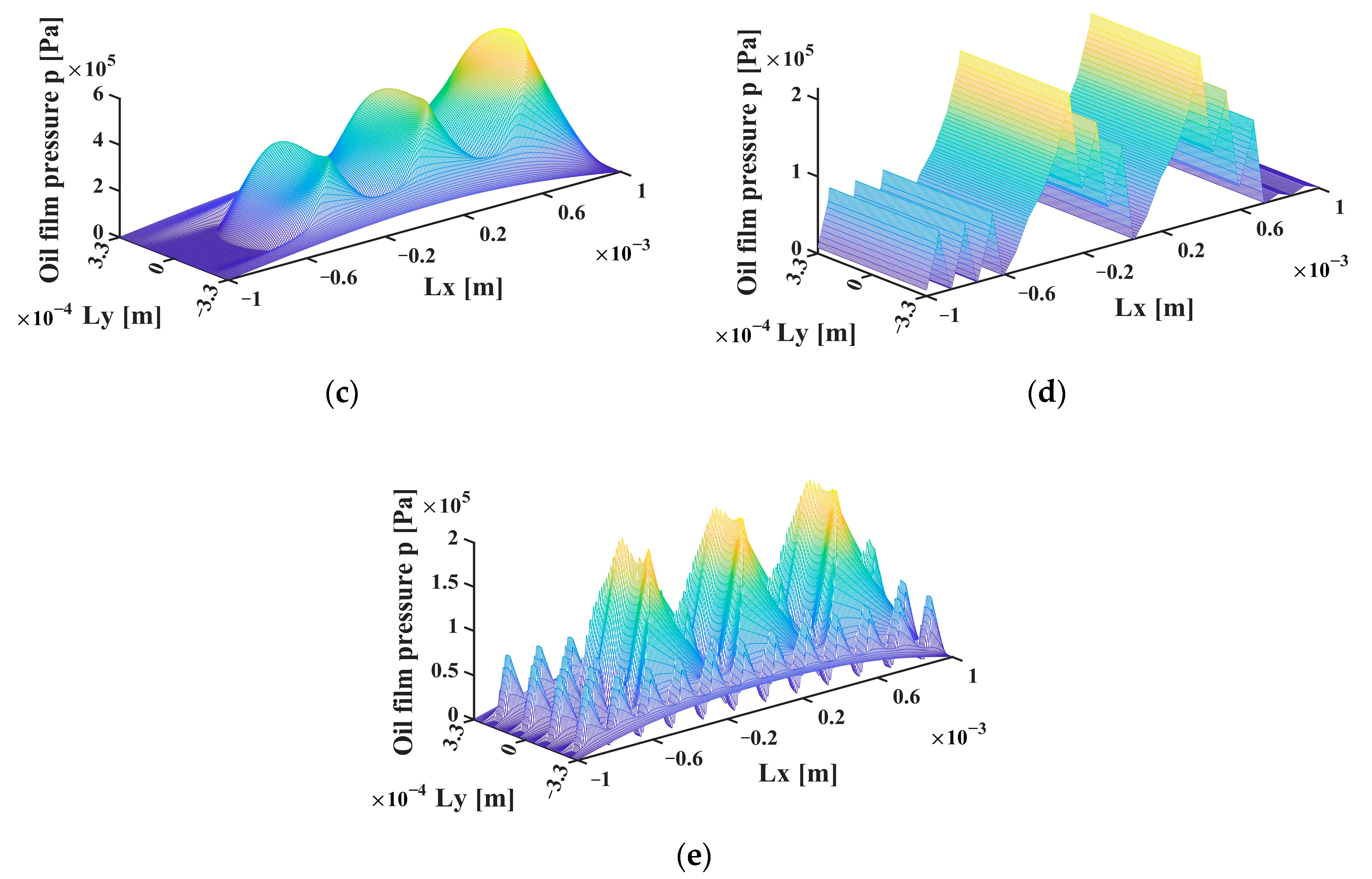
4.2.1. Effects of Geometric Parameters on Tribological Performance
4.2.2. Optimal Results of Geometric Parameters
4.2.3. Comparison of Tribological Performances Between Different Textures
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, H.; Liu, Y.; Wang, W.; Qin, L.; Dong, G. Surface texture design and its tribological application. J. Mech. Eng. 2019, 55, 85–93. [Google Scholar]
- Khatri, C.B.; Sharma, S.C. Influence of textured surface on the performance of non-recessed hybrid journal bearing operating with non-Newtonian lubricant. Tribol. Int. 2016, 95, 221–235. [Google Scholar] [CrossRef]
- Costa, H.; Hutchings, I. Hydrodynamic lubrication of textured steel surfaces under reciprocating sliding conditions. Tribol. Int. 2007, 40, 1227–1238. [Google Scholar] [CrossRef]
- Greiner, C.; Merz, T.; Braun, D.; Codrignani, A.; Magagnato, F. Optimum dimple diameter for friction reduction with laser surface texturing: The effect of velocity gradient. Surf. Topogr. Metrol. Prop. 2015, 3, 044001. [Google Scholar] [CrossRef]
- Vrbka, M.; Šamánek, O.; Šperka, P.; Navrat, T.; Křupka, I.; Hartl, M. Effect of surface texturing on rolling contact fatigue within mixed lubricated non-conformal rolling/sliding contacts. Tribol. Int. 2010, 43, 1457–1465. [Google Scholar] [CrossRef]
- Tang, W.; Zhou, Y.; Zhu, H.; Yang, H. The effect of surface texturing on reducing the friction and wear of steel under lubricated sliding contact. Appl. Surf. Sci. 2013, 273, 199–204. [Google Scholar] [CrossRef]
- Sung, I.-H.; Lee, H.-S.; Kim, D.-E. Effect of surface topography on the frictional behavior at the micro/nano-scale. Wear 2003, 254, 1019–1031. [Google Scholar] [CrossRef]
- Qiu, Y.; Khonsari, M. Experimental investigation of tribological performance of laser textured stainless steel rings. Tribol. Int. 2011, 44, 635–644. [Google Scholar] [CrossRef]
- Ronen, A.; Etsion, I.; Kligerman, Y. Friction-reducing surface-texturing in reciprocating automotive components. Tribol. Trans. 2001, 44, 359–366. [Google Scholar] [CrossRef]
- Lu, P.; Wood, R.J.; Gee, M.G.; Wang, L.; Pfleging, W. The friction reducing effect of square-shaped surface textures under lubricated line-contacts—An experimental study. Lubricants 2016, 4, 26. [Google Scholar] [CrossRef]
- Gachot, C.; Rosenkranz, A.; Hsu, S.; Costa, H. A critical assessment of surface texturing for friction and wear improvement. Wear 2017, 372, 21–41. [Google Scholar] [CrossRef]
- Gropper, D.; Wang, L.; Harvey, T.J. Hydrodynamic lubrication of textured surfaces: A review of modeling techniques and key findings. Tribol. Int. 2016, 94, 509–529. [Google Scholar] [CrossRef]
- Wang, T.; Huang, W.; Liu, X.; Li, Y.; Wang, Y. Experimental study of two-phase mechanical face seals with laser surface texturing. Tribol. Int. 2014, 72, 90–97. [Google Scholar] [CrossRef]
- Kovalchenko, A.; Ajayi, O.; Erdemir, A.; Fenske, G.; Etsion, I. The effect of laser texturing of steel surfaces and speed-load parameters on the transition of lubrication regime from boundary to hydrodynamic. Tribol. Trans. 2004, 47, 299–307. [Google Scholar] [CrossRef]
- Pettersson, U.; Jacobson, S. Influence of surface texture on boundary lubricated sliding contacts. Tribol. Int. 2003, 36, 857–864. [Google Scholar] [CrossRef]
- Ryk, G.; Etsion, I. Testing piston rings with partial laser surface texturing for friction reduction. Wear 2006, 261, 792–796. [Google Scholar] [CrossRef]
- Wang, Z.; Hu, S.; Zhang, H.; Ji, H.; Yang, J.; Liang, W. Effect of surface texturing parameters on the lubrication characteristics of an axial piston pump valve plate. Lubricants 2018, 6, 49. [Google Scholar] [CrossRef]
- Qiu, M.; Delic, A.; Raeymaekers, B. The effect of texture shape on the load-carrying capacity of gas-lubricated parallel slider bearings. Tribol. Lett. 2012, 48, 315–327. [Google Scholar] [CrossRef]
- Yu, H.; Wang, X.; Zhou, F. Geometric shape effects of surface texture on the generation of hydrodynamic pressure between conformal contacting surfaces. Tribol. Lett. 2010, 37, 123–130. [Google Scholar] [CrossRef]
- Shen, C.; Khonsari, M. Numerical optimization of texture shape for parallel surfaces under unidirectional and bidirectional sliding. Tribol. Int. 2015, 82, 1–11. [Google Scholar] [CrossRef]
- Papadopoulos, C.; Efstathiou, E.; Nikolakopoulos, P.; Kaiktsis, L. Geometry optimization of textured three-dimensional micro-thrust bearings. J. Tribol. Oct 2011, 133, 041702. [Google Scholar] [CrossRef]
- Zhang, H.; Dong, G.-N.; Hua, M.; Chin, K.-S. Improvement of tribological behaviors by optimizing concave texture shape under reciprocating sliding motion. J. Tribol. 2017, 139, 011701. [Google Scholar] [CrossRef]
- Shen, C.; Khonsari, M. Texture shape optimization for seal-like parallel surfaces: Theory and experiment. Tribol. Trans. 2016, 59, 698–706. [Google Scholar] [CrossRef]
- Chen, C.; Liu, C.; Li, Y.; Mou, S. Geometry optimization for asymmetrical herringbone grooves of miniature hydrodynamic journal bearings by using Taguchi technique. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2015, 229, 196–206. [Google Scholar] [CrossRef]
- Wang, W.; He, Y.; Zhao, J.; Mao, J.; Hu, Y.; Luo, J. Optimization of groove texture profile to improve hydrodynamic lubrication performance: Theory and experiments. Friction 2020, 8, 83–94. [Google Scholar] [CrossRef]
- Huang, Q.; Shi, X.; Xue, Y.; Zhang, K.; Wu, C. Assessment and Optimization of Tribological Parameters for Bionic Textured AISI 4140-SnAgCu Self-Lubricating Composite Under Dry Sliding Conditions Using AHP and RSM. Tribol. Trans. 2022, 65, 479–491. [Google Scholar] [CrossRef]
- Sloane, E.B.; Liberatore, M.J.; Nydick, R.L.; Luo, W.; Chung, Q. Using the analytic hierarchy process as a clinical engineering tool to facilitate an iterative, multidisciplinary, microeconomic health technology assessment. Comput. Oper. Res. 2003, 30, 1447–1465. [Google Scholar] [CrossRef]
- Chatburn, R.L.; Primiano Jr, F.P. Decision analysis for large capital purchases: How to buy a ventilator. Respir. Care 2001, 46, 1038–1053. [Google Scholar]
- Turri, J. Program eases decision making. Health Prog. 1988, 69, 40–44. [Google Scholar]
- Kaplan, B.; Shaw, N. Future directions in evaluation research: People, organizational, and social issues. Methods Inf. Med. 2004, 43, 215–231. [Google Scholar]
- Xu, J.; Zhang, G.; Fan, S.; Ni, J.; Lian, J. Research on the Controllable Interface Response Enhancement of the Textured Pilot Valve. Machines 2022, 10, 357. [Google Scholar] [CrossRef]
- Tao, H.; Cao-feng, Y.; Chuan-li, W.; Hai-shun, D.; Hui, Z. Analyzing hydrodynamic bearing capacity and lubrication charac-teristics of micro-texture valve core friction pair. Mech. Sci. Technol. Aerosp. Eng. 2016, 35, 1829–1833. [Google Scholar]
- Chen, X.; Zeng, L.; Zheng, F. Friction performance and optimisation of diamond-like texture on hydraulic cylinder surface. Micro Nano Lett. 2018, 13, 1001–1006. [Google Scholar] [CrossRef]
- Wang, C.; Zhou, D.; He, T.; Hao, F.; MA, D.; Yang, L. Interactive Test Analysis of Dynamic Pressure Bearing Force for Valve Core Texturing. Chin. Hydraul. Pneum. 2019, 3, 8–13. [Google Scholar]
- Syed, N.R.; Kakoty, S.K. Influence of spherical protruded and dimple texture on the journal bearing performance: A comparative theoretical analysis implementing JFO boundary conditions. Ind. Lubr. Tribol. 2022, 74, 788–795. [Google Scholar] [CrossRef]
- Wang, L.; Wang, W.; Wang, H.; Ma, T.; Hu, Y. Numerical analysis on the factors affecting the hydrodynamic performance for the parallel surfaces with microtextures. J. Tribol. 2014, 136, 021702. [Google Scholar] [CrossRef]
- Zhang, K.; Yagi, K. Refined Hydrodynamic Lubrication Model with a High-Accuracy Numerical Algorithm for Starved Lubrication with Free Surfaces. Tribol. Lett. 2023, 71, 122. [Google Scholar] [CrossRef]
- Wen, S.; Huang, P. Principles of Tribology; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Wang, W.; He, Y.; Zhao, J.; Li, Y.; Luo, J. Numerical optimization of the groove texture bottom profile for thrust bearings. Tribol. Int. 2017, 109, 69–77. [Google Scholar] [CrossRef]
- Gherca, A.; Maspeyrot, P.; Hajjam, M.; Fatu, A. Influence of texture geometry on the hydrodynamic performances of parallel bearings. Tribol. Trans. 2013, 56, 321–332. [Google Scholar] [CrossRef]
- Uddin, M.; Ibatan, T.; Shankar, S. Influence of surface texture shape, geometry and orientation on hydrodynamic lubrication performance of plane-to-plane slider surfaces. Lubr. Sci. 2017, 29, 153–181. [Google Scholar] [CrossRef]
- Ünlü, B.S.; Atik, E. Determination of friction coefficient in journal bearings. Mater. Des. 2007, 28, 973–977. [Google Scholar] [CrossRef]
- Yang, S.; Wang, H.; Zhang, Y.; Zhang, L. Cutting performance evaluation of surface micro-texture ball end milling based on multi-objective decision making. J. Harbin Univ. Sci. Technol. 2016, 21, 1–5. [Google Scholar]
- Franek, J.; Kresta, A. Judgment Scales and Consistency Measure in AHP. Procedia Econ. Financ. 2014, 12, 164–173. [Google Scholar] [CrossRef]
- He, T.; Wang, C.L.; Deng, H.S.; Huang, Y.L.; Yu, C.F. Hydrodynamic Lubrication and Load Carrying Capacity Analysis of Laser Surface Texturing Valve Core. Key Eng. Mater. 2016, 693, 348–355. [Google Scholar] [CrossRef]
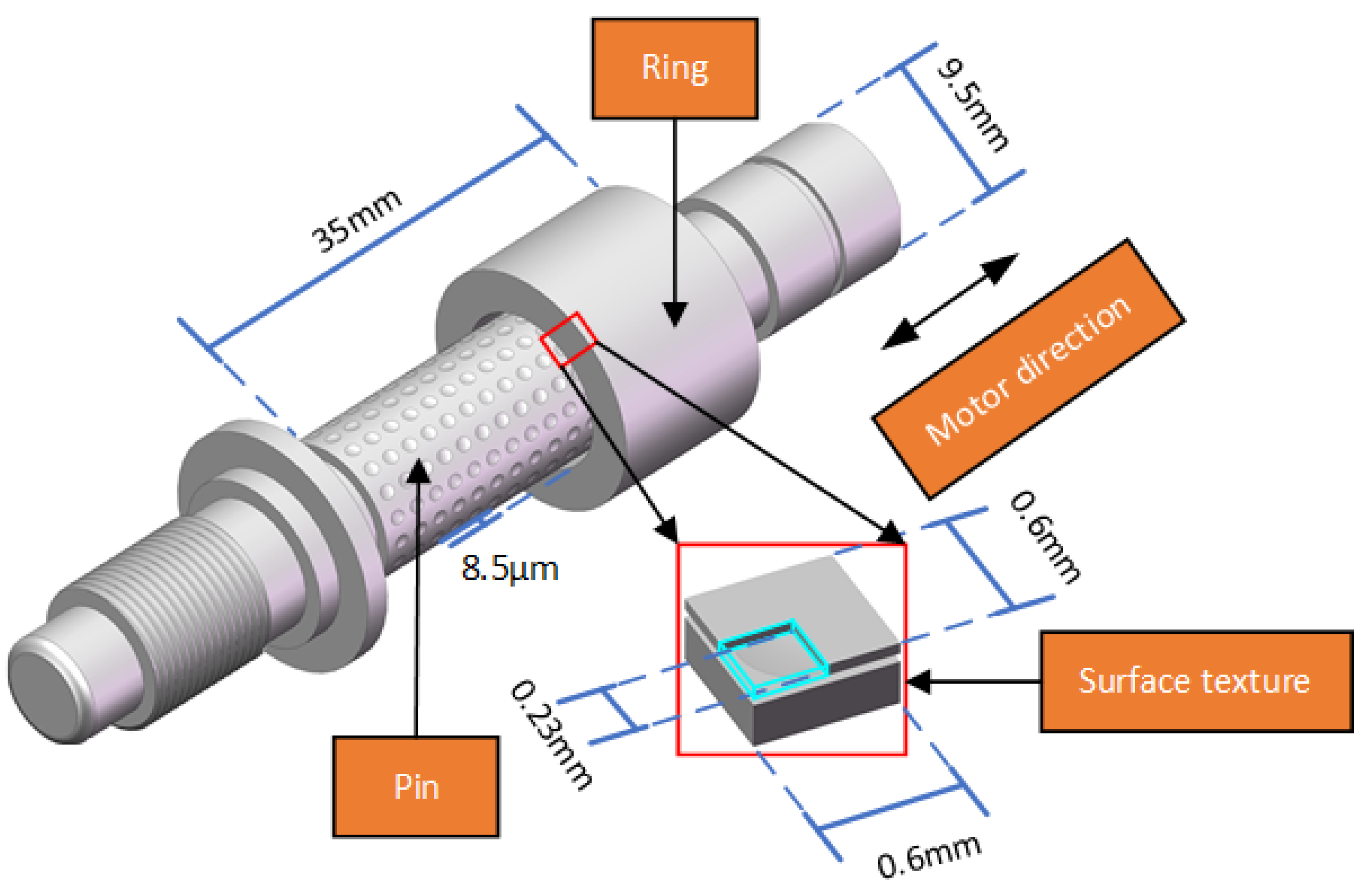






| Ring and Pin | ||
|---|---|---|
| Materials | 1Cr18Ni10Ti Stainless Steel | |
| Mechanical properties | 520 | |
| 206 | ||
| 187 | ||
| Texture Parameters | Unit | Parameter Value |
|---|---|---|
| 0.3 | ||
| Lubricant viscosity η0 | 0.4 | |
| 8.5 | ||
| 700 | ||
| 700 | ||
| 230 | ||
| 20 |
| Texture Type | Value | |
|---|---|---|
| Spherical cap | 190, 200, 210, 220, 230 | |
| 9, 10, 11, 12, 13 | ||
| Ellipsoidal cap | 200, 220, 240, 260, 280 | |
| 190, 200, 210, 220, 230 | ||
| 600, 700, 800, 900, 1000 | ||
| 9, 10, 11, 12, 13 | ||
| Tree-frog | 220, 230, 240 | |
| 70, 80, 90 | ||
| 11, 12, 13 | ||
| 3, 4, 5 | ||
| 6, 7, 8 | ||
| Grass-lip | 3, 6, 9, 12, 15, 18, 21, 24, 27, 30 | |
| 66 | ||
| Nepenthes | 300 | |
| 200 | ||
| 30 | ||
| 20 | ||
| 6, 7, 8, 9, 10 | ||
| 3, 4 |
| Tribological Results for Spherical Texture (Initial Designs Generated in Table 3) | ||||||||
| Geometric Parameters | ||||||||
| 190 | 9 | 0.17221 | 0.01675 | 0.09725 | 0.64607 | 0.73638 | 0.54172 | 0.62823 |
| 10 | 0.17694 | 0.01656 | 0.09361 | 0.66381 | 0.74455 | 0.56277 | 0.64438 | |
| 11 | 0.18023 | 0.01639 | 0.09095 | 0.67618 | 0.75232 | 0.57923 | 0.65710 | |
| 12 | 0.18233 | 0.01623 | 0.08903 | 0.68404 | 0.75968 | 0.59170 | 0.66687 | |
| 13 | 0.18348 | 0.01609 | 0.08767 | 0.68836 | 0.76665 | 0.60090 | 0.67423 | |
| 200 | 9 | 0.18782 | 0.01652 | 0.08798 | 0.70463 | 0.74626 | 0.59875 | 0.67046 |
| 10 | 0.19325 | 0.01632 | 0.08446 | 0.72502 | 0.75552 | 0.62372 | 0.68932 | |
| 11 | 0.19711 | 0.01613 | 0.08185 | 0.73948 | 0.76436 | 0.64360 | 0.70441 | |
| 12 | 0.19968 | 0.01596 | 0.07992 | 0.74914 | 0.77276 | 0.65918 | 0.71632 | |
| 13 | 0.20119 | 0.01580 | 0.07851 | 0.75478 | 0.78074 | 0.67100 | 0.72548 | |
| 210 | 9 | 0.20454 | 0.01630 | 0.07968 | 0.76736 | 0.75671 | 0.66118 | 0.71625 |
| 10 | 0.21083 | 0.01607 | 0.07625 | 0.79095 | 0.76715 | 0.69090 | 0.73836 | |
| 11 | 0.21537 | 0.01587 | 0.07368 | 0.80799 | 0.77715 | 0.71499 | 0.75630 | |
| 12 | 0.21852 | 0.01568 | 0.07173 | 0.81982 | 0.78670 | 0.73438 | 0.77078 | |
| 13 | 0.22050 | 0.01550 | 0.07028 | 0.82724 | 0.79580 | 0.74959 | 0.78224 | |
| 220 | 9 | 0.22245 | 0.01606 | 0.07221 | 0.83456 | 0.76768 | 0.72951 | 0.76588 |
| 10 | 0.22976 | 0.01582 | 0.06886 | 0.86199 | 0.77940 | 0.76499 | 0.79187 | |
| 11 | 0.23518 | 0.01560 | 0.06632 | 0.88231 | 0.79067 | 0.79434 | 0.81333 | |
| 12 | 0.23905 | 0.01539 | 0.06436 | 0.89684 | 0.80148 | 0.81846 | 0.83096 | |
| 13 | 0.24160 | 0.01519 | 0.06288 | 0.90638 | 0.81181 | 0.83784 | 0.84517 | |
| 230 | 9 | 0.24188 | 0.01583 | 0.06543 | 0.90744 | 0.77916 | 0.80508 | 0.82024 |
| 10 | 0.25042 | 0.01557 | 0.06216 | 0.93950 | 0.79225 | 0.84752 | 0.85085 | |
| 11 | 0.25693 | 0.01532 | 0.05963 | 0.96392 | 0.80488 | 0.88342 | 0.87663 | |
| 12 | 0.26175 | 0.01509 | 0.05766 | 0.98200 | 0.81705 | 0.91359 | 0.89823 | |
| 13 | 0.26511 | 0.01488 | 0.05613 | 0.99461 | 0.82874 | 0.93856 | 0.91608 | |
| Tribological Results for Spherical Texture (Additional Designs Obtained by Expanding the Value Range of d) | ||||||||
| Geometric parameters | ||||||||
| 230 | 14 | 0.26545 | 0.01468 | 0.05531 | 0.99588 | 0.83987 | 0.95238 | 0.92615 |
| 15 | 0.26643 | 0.01450 | 0.05442 | 0.99956 | 0.85057 | 0.96808 | 0.93747 | |
| 16 | 0.26655 | 0.01433 | 0.05375 | 1.00000 | 0.86079 | 0.98015 | 0.94627 | |
| 17 | 0.26593 | 0.01417 | 0.05327 | 0.99767 | 0.87054 | 0.98894 | 0.95281 | |
| 18 | 0.26473 | 0.01402 | 0.05294 | 0.99319 | 0.87983 | 0.99500 | 0.95748 | |
| 19 | 0.26305 | 0.01388 | 0.05275 | 0.98687 | 0.88868 | 0.99862 | 0.96049 | |
| 20 | 0.26094 | 0.01375 | 0.05268 | 0.97897 | 0.89709 | 1.00000 | 0.96200 | |
| 21 | 0.25849 | 0.01362 | 0.05271 | 0.96975 | 0.90509 | 0.99942 | 0.96218 | |
| 22 | 0.25574 | 0.01351 | 0.05283 | 0.95946 | 0.91270 | 0.99711 | 0.96122 | |
| 23 | 0.25281 | 0.01341 | 0.05303 | 0.94845 | 0.91992 | 0.99347 | 0.95938 | |
| 24 | 0.24971 | 0.01331 | 0.05329 | 0.93682 | 0.92678 | 0.98861 | 0.95672 | |
| 25 | 0.24648 | 0.01321 | 0.05361 | 0.92472 | 0.93330 | 0.98271 | 0.95339 | |
| 26 | 0.24314 | 0.01313 | 0.05399 | 0.91219 | 0.93949 | 0.97581 | 0.94940 | |
| 27 | 0.23971 | 0.01304 | 0.05442 | 0.89932 | 0.94537 | 0.96807 | 0.94485 | |
| 28 | 0.23621 | 0.01297 | 0.05490 | 0.88618 | 0.95095 | 0.95957 | 0.93981 | |
| 29 | 0.23267 | 0.01290 | 0.05542 | 0.87292 | 0.95626 | 0.95047 | 0.93438 | |
| 30 | 0.22911 | 0.01283 | 0.05599 | 0.85954 | 0.96130 | 0.94085 | 0.92861 | |
| 31 | 0.22556 | 0.01276 | 0.05659 | 0.84624 | 0.96609 | 0.93090 | 0.92262 | |
| 32 | 0.22202 | 0.01270 | 0.05722 | 0.83295 | 0.97065 | 0.92061 | 0.91641 | |
| 33 | 0.21849 | 0.01265 | 0.05789 | 0.81970 | 0.97498 | 0.91000 | 0.91000 | |
| 34 | 0.21497 | 0.01260 | 0.05859 | 0.80649 | 0.97910 | 0.89912 | 0.90340 | |
| 35 | 0.21149 | 0.01254 | 0.05932 | 0.79346 | 0.98302 | 0.88813 | 0.89674 | |
| 36 | 0.20805 | 0.01250 | 0.06007 | 0.78052 | 0.98675 | 0.87697 | 0.88995 | |
| 37 | 0.20463 | 0.01245 | 0.06085 | 0.76771 | 0.99031 | 0.86568 | 0.88308 | |
| 38 | 0.20125 | 0.01241 | 0.06166 | 0.75503 | 0.99370 | 0.85429 | 0.87615 | |
| 39 | 0.19791 | 0.01237 | 0.06250 | 0.74251 | 0.99692 | 0.84286 | 0.86917 | |
| 40 | 0.19461 | 0.01233 | 0.06337 | 0.73013 | 1.00000 | 0.83136 | 0.86216 | |
| Texture | Scheme | Tribological Parameter | |||
|---|---|---|---|---|---|
| Value | |||||
| Spherical cap | 230 | 0.25849 | 0.01362 | 0.05271 | |
| 21 | |||||
| Ellipsoidal cap | 280 | 0.25530 | 0.01129 | 0.04423 | |
| 230 | |||||
| 600 | |||||
| 25 | |||||
| Tree-frog | 240 | 0.29503 | 0.01501 | 0.05089 | |
| 70 | |||||
| 13 | |||||
| 3 | |||||
| 8 | |||||
| Grass-lip | 9 | 0.12709 | 0.00584 | 0.04596 | |
| 18 | |||||
| 27 | |||||
| 66 | |||||
| Nepenthes | 300 | 0.07877 | 0.01639 | 0.20802 | |
| 200 | |||||
| 30 | |||||
| 20 | |||||
| 7 | |||||
| 4 | |||||
| Texture | ||
|---|---|---|
| Conventional texture | Spherical cap | 0.7096 |
| Ellipsoidal cap | 0.8064 | |
| Biomimetic texture | Tree-frog | 0.7555 |
| Grass-lip | 0.8521 | |
| Nepenthes | 0.2736 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Pei, Q.; Liu, Z.; Luo, S.; Zhou, L.; Li, J.; Chen, L. Parametric Optimization of Surface Textures in Oil-Lubricated Long-Life Aircraft Valves. Lubricants 2024, 12, 405. https://doi.org/10.3390/lubricants12120405
Li P, Pei Q, Liu Z, Luo S, Zhou L, Li J, Chen L. Parametric Optimization of Surface Textures in Oil-Lubricated Long-Life Aircraft Valves. Lubricants. 2024; 12(12):405. https://doi.org/10.3390/lubricants12120405
Chicago/Turabian StyleLi, Pei, Qingxiang Pei, Zhe Liu, Sihai Luo, Liucheng Zhou, Junning Li, and Leilei Chen. 2024. "Parametric Optimization of Surface Textures in Oil-Lubricated Long-Life Aircraft Valves" Lubricants 12, no. 12: 405. https://doi.org/10.3390/lubricants12120405
APA StyleLi, P., Pei, Q., Liu, Z., Luo, S., Zhou, L., Li, J., & Chen, L. (2024). Parametric Optimization of Surface Textures in Oil-Lubricated Long-Life Aircraft Valves. Lubricants, 12(12), 405. https://doi.org/10.3390/lubricants12120405









