Influencing Factors on the Fluting in an Axial Ball Bearing at DC Bearing Currents
Abstract
1. Introduction
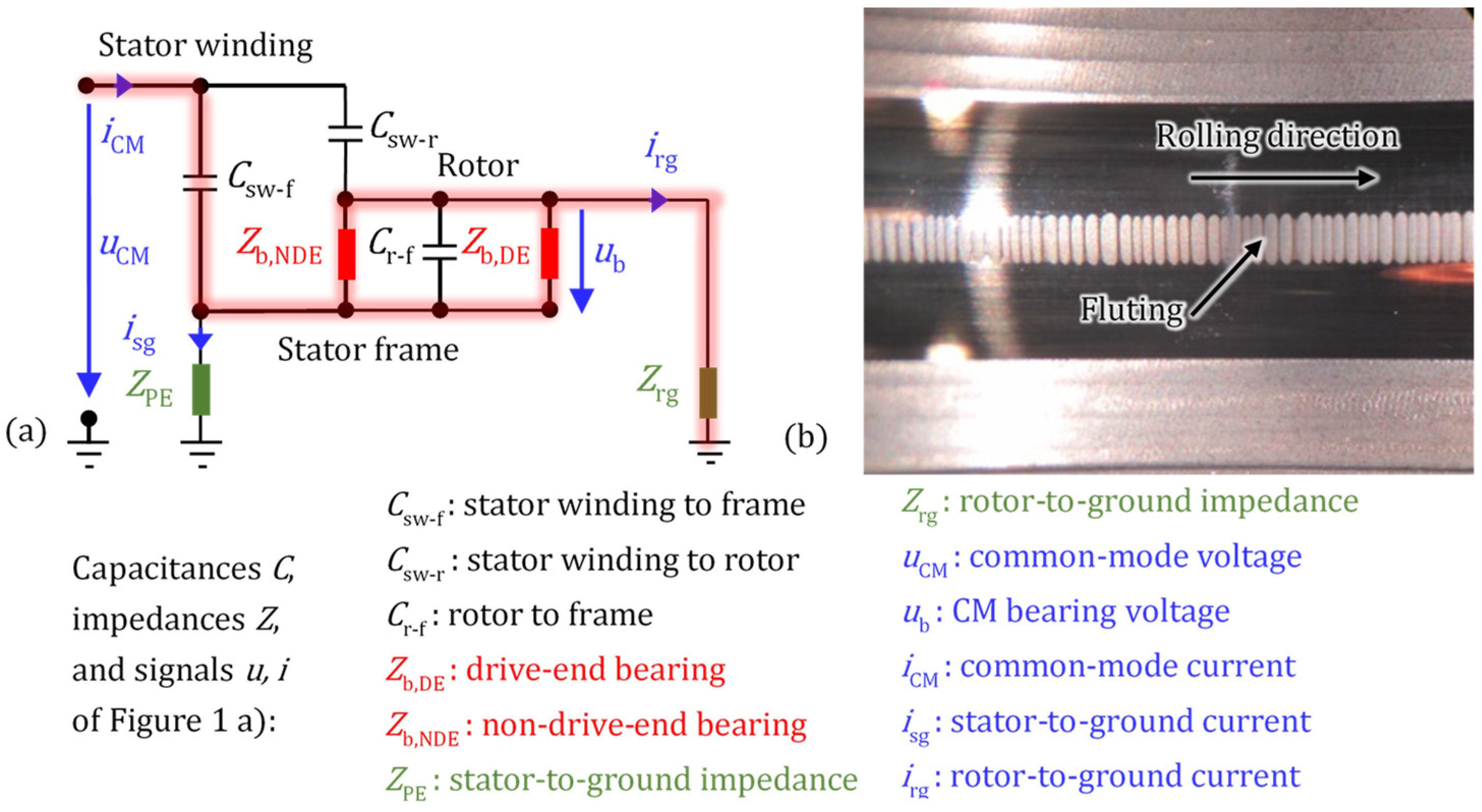
2. Parasitic Currents in Bearings of Inverter-Fed Electrical Machines
3. Axial Ball Bearing Test Rigs
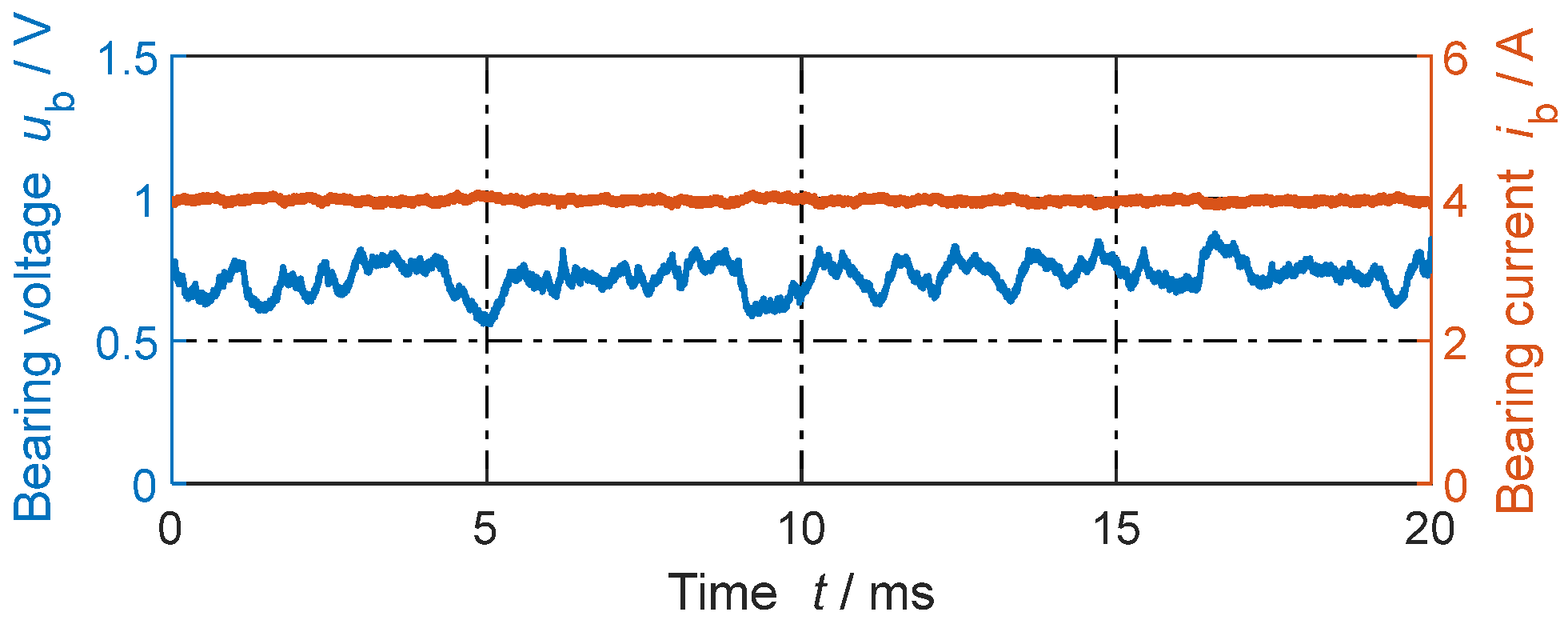
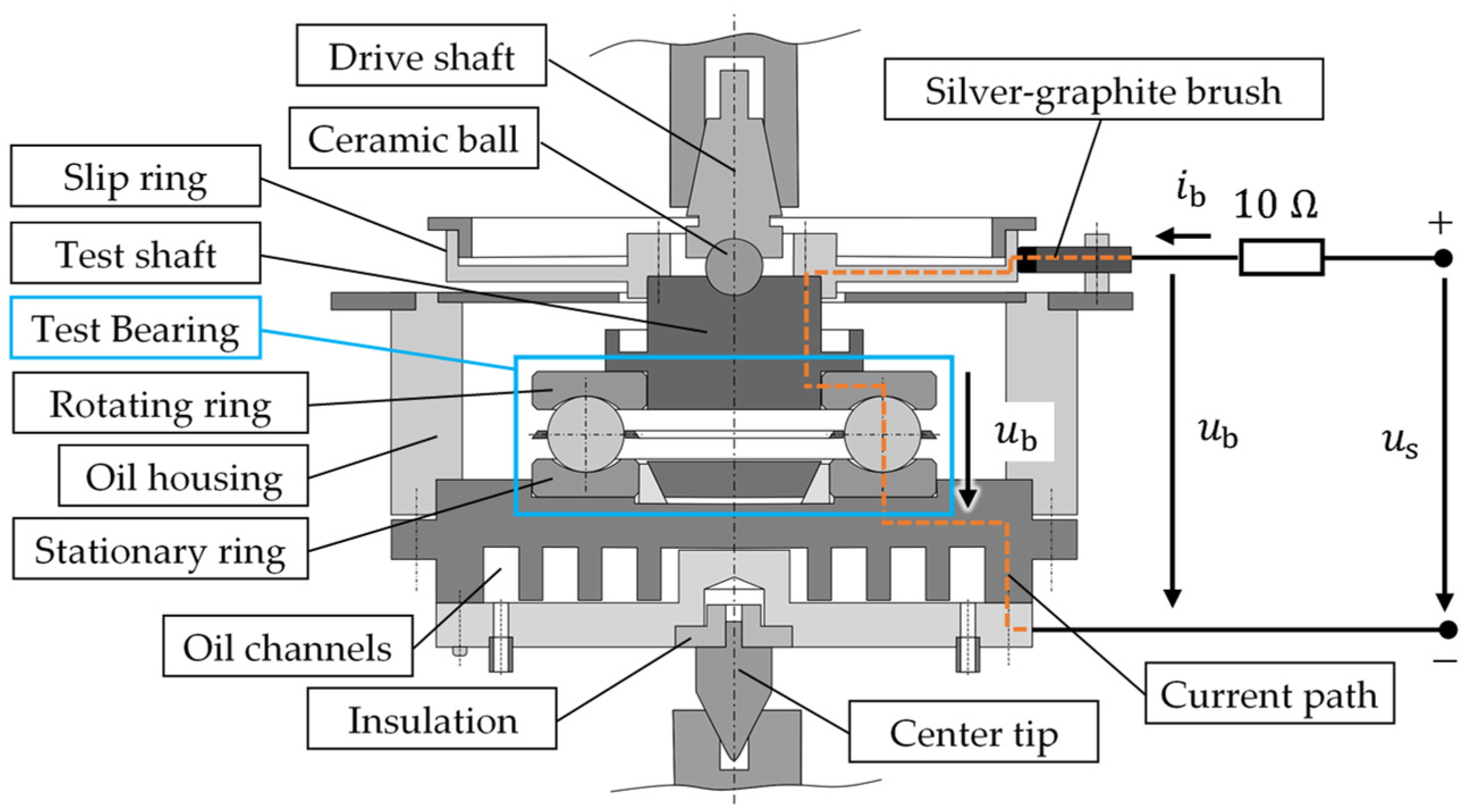
3.1. Test Rig #1

3.2. Test Rig #2
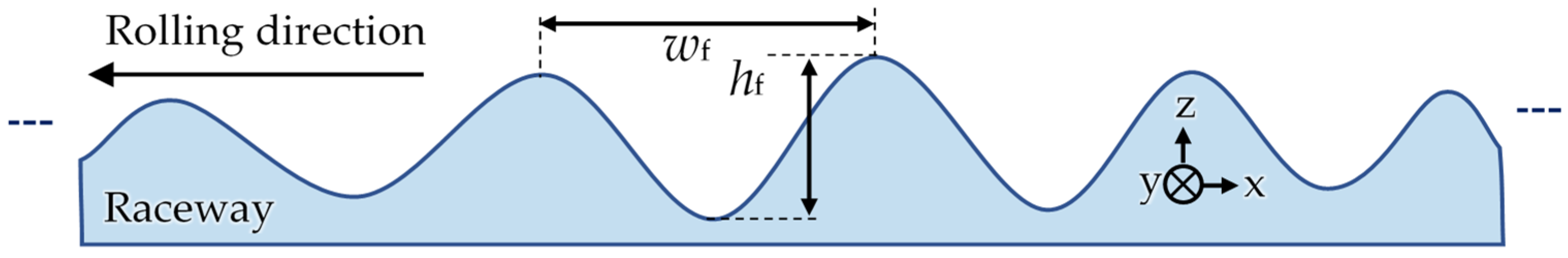
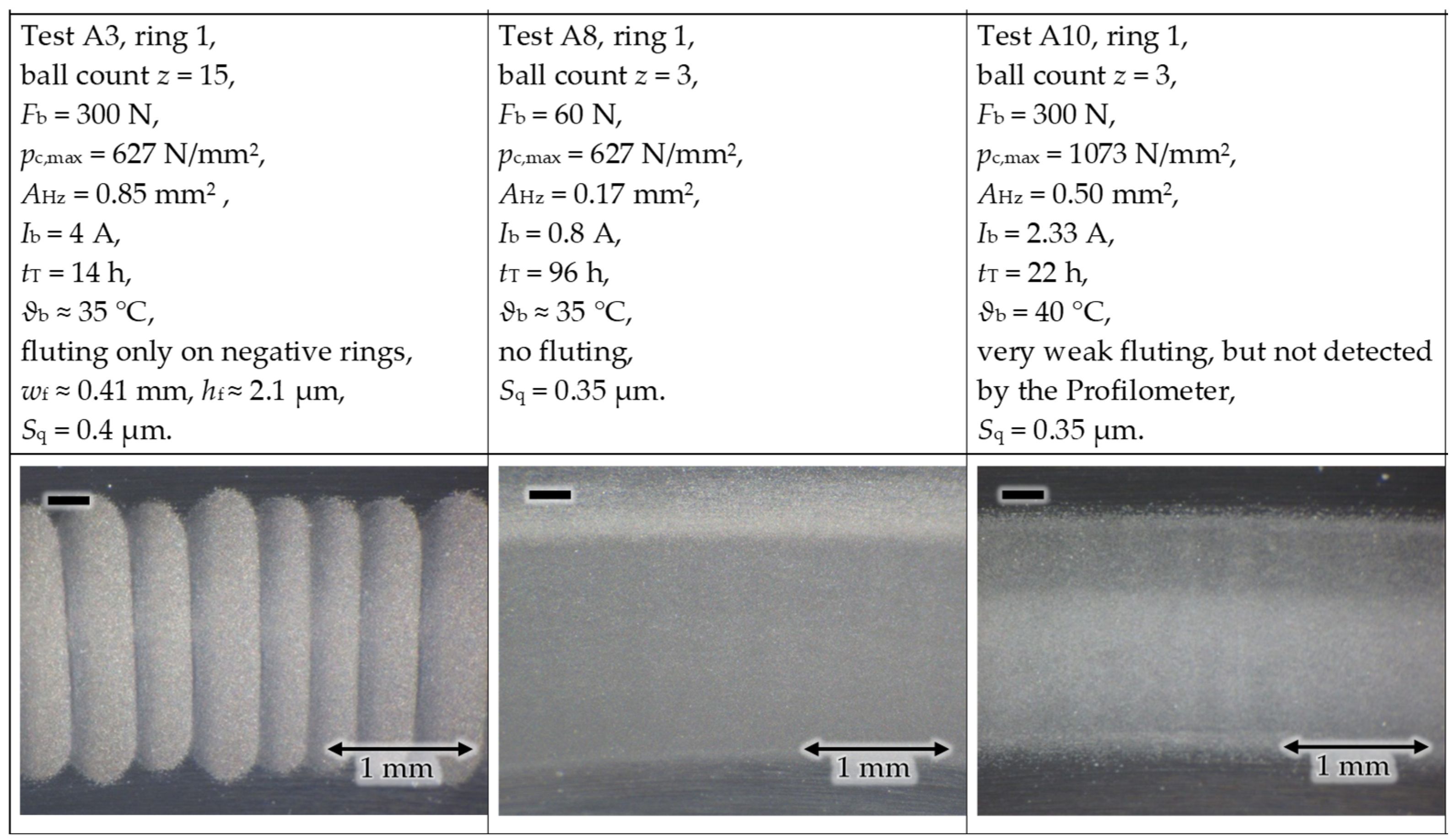
4. Influencing Parameters on Fluting
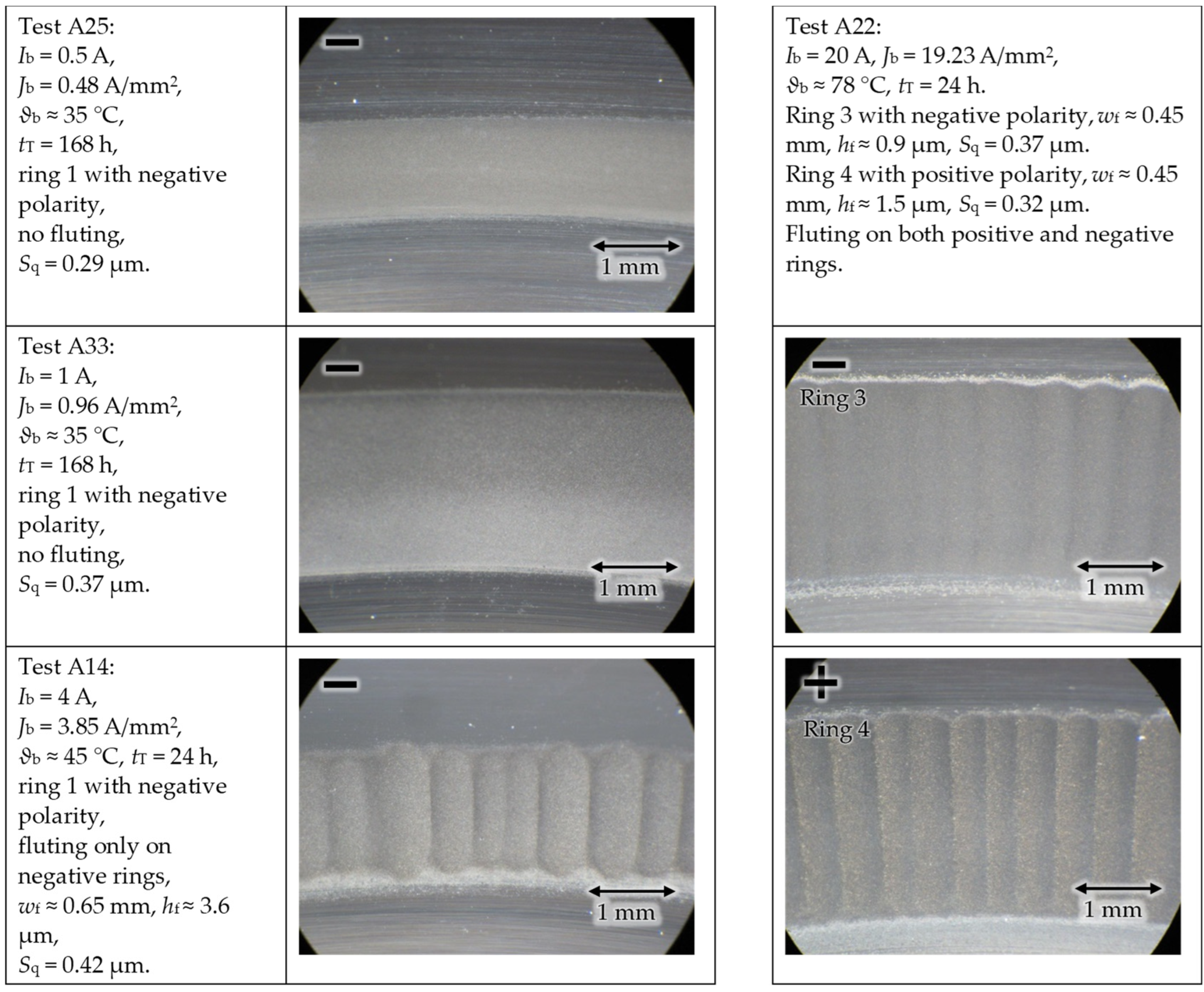
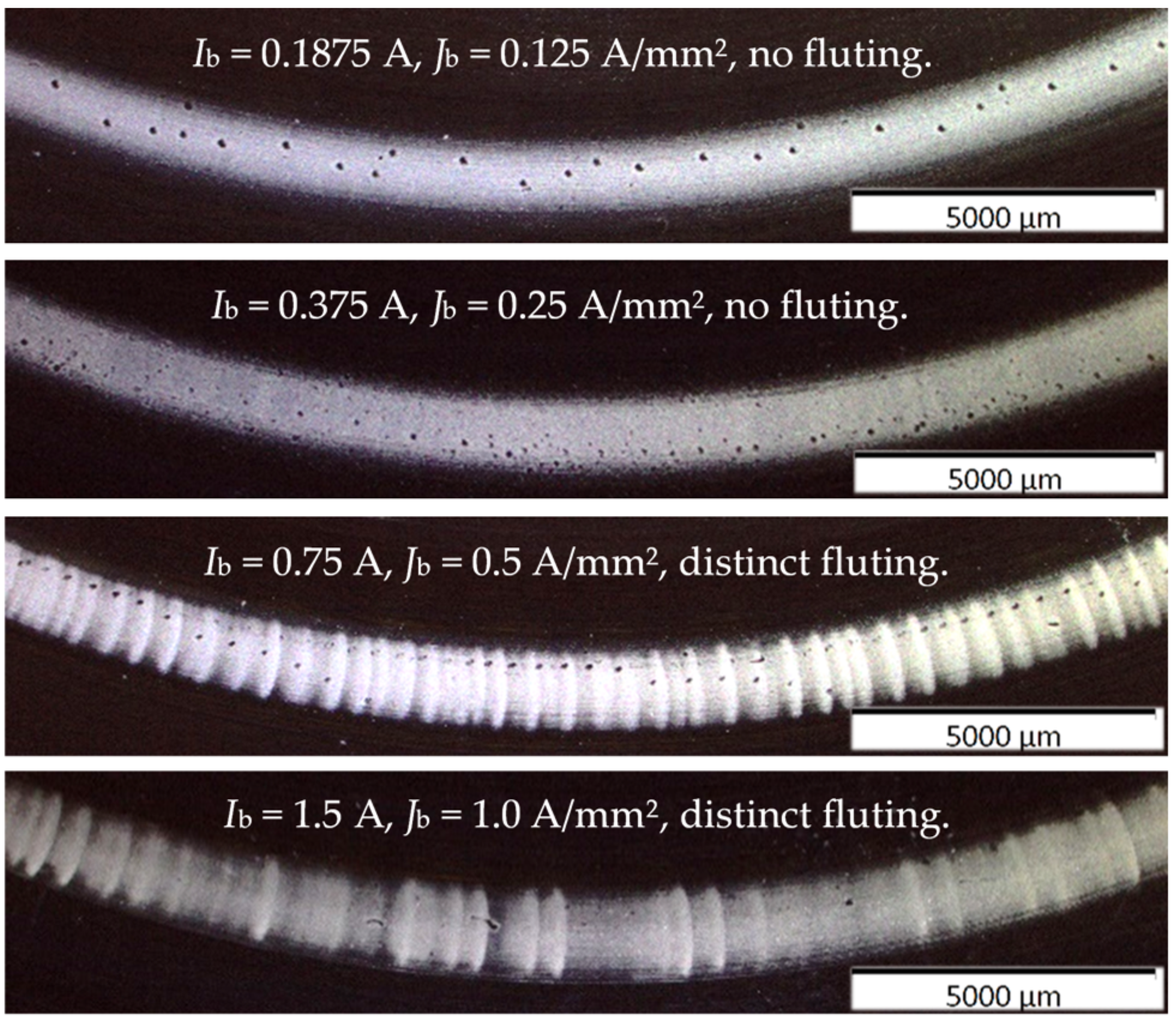
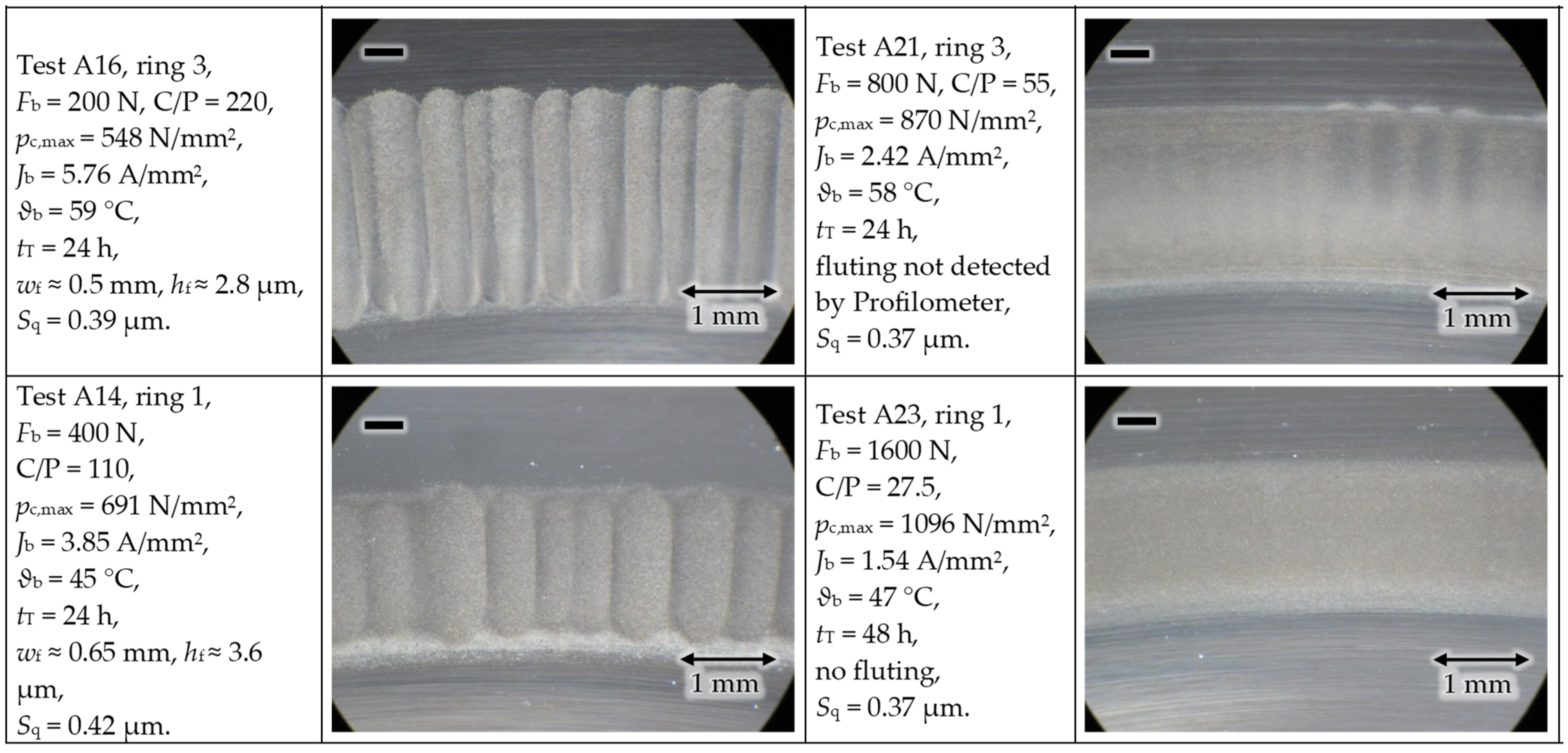
4.1. DC Bearing Current Amplitude
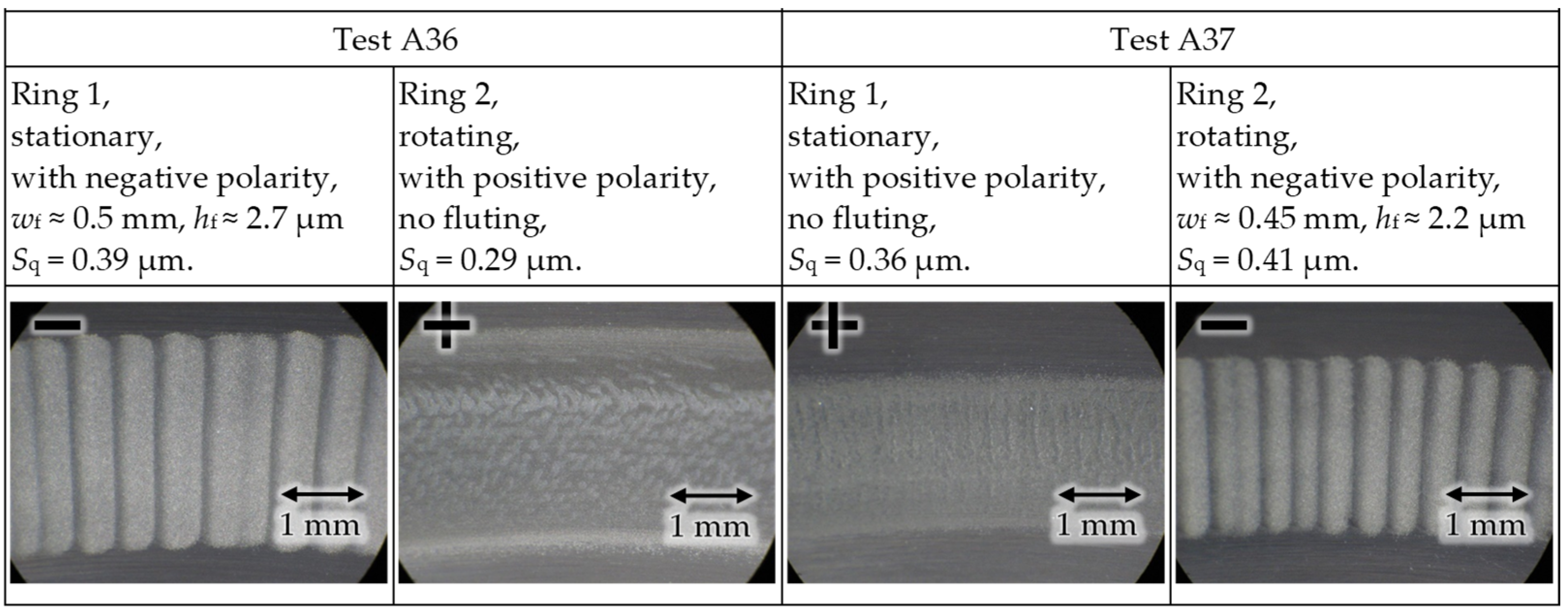
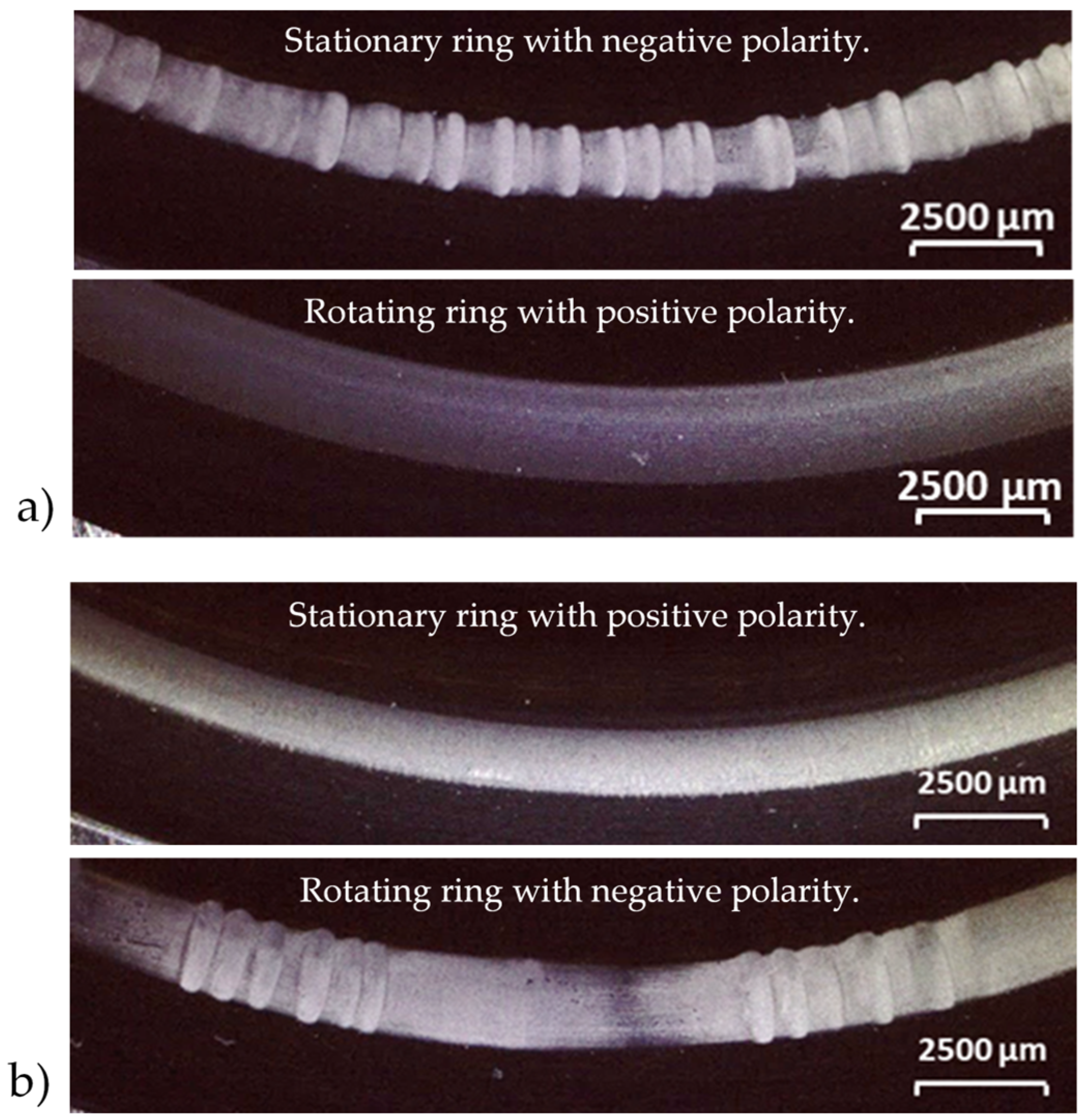
4.2. DC Bearing Current Polarity
4.3. Mechanical Force
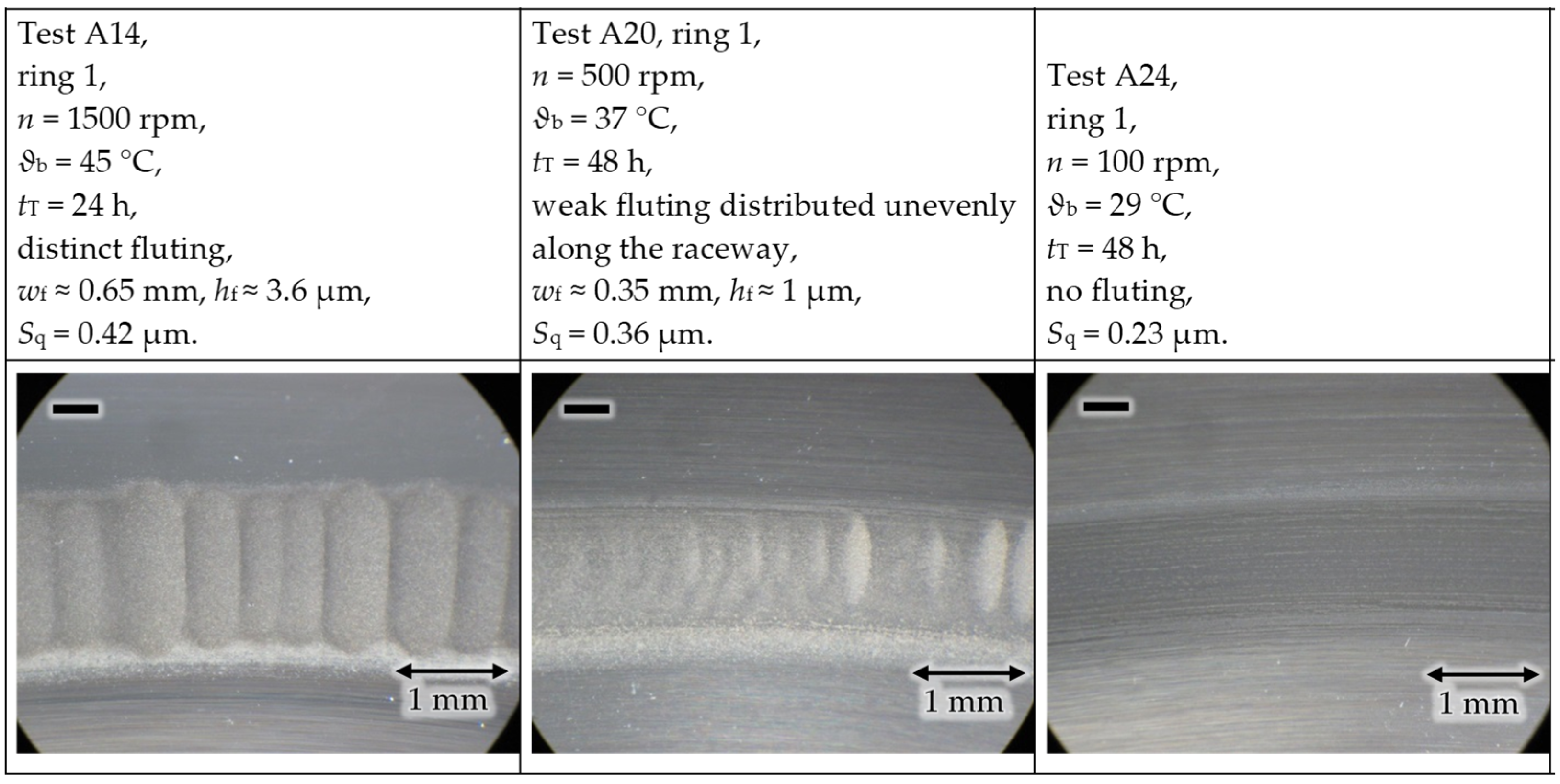
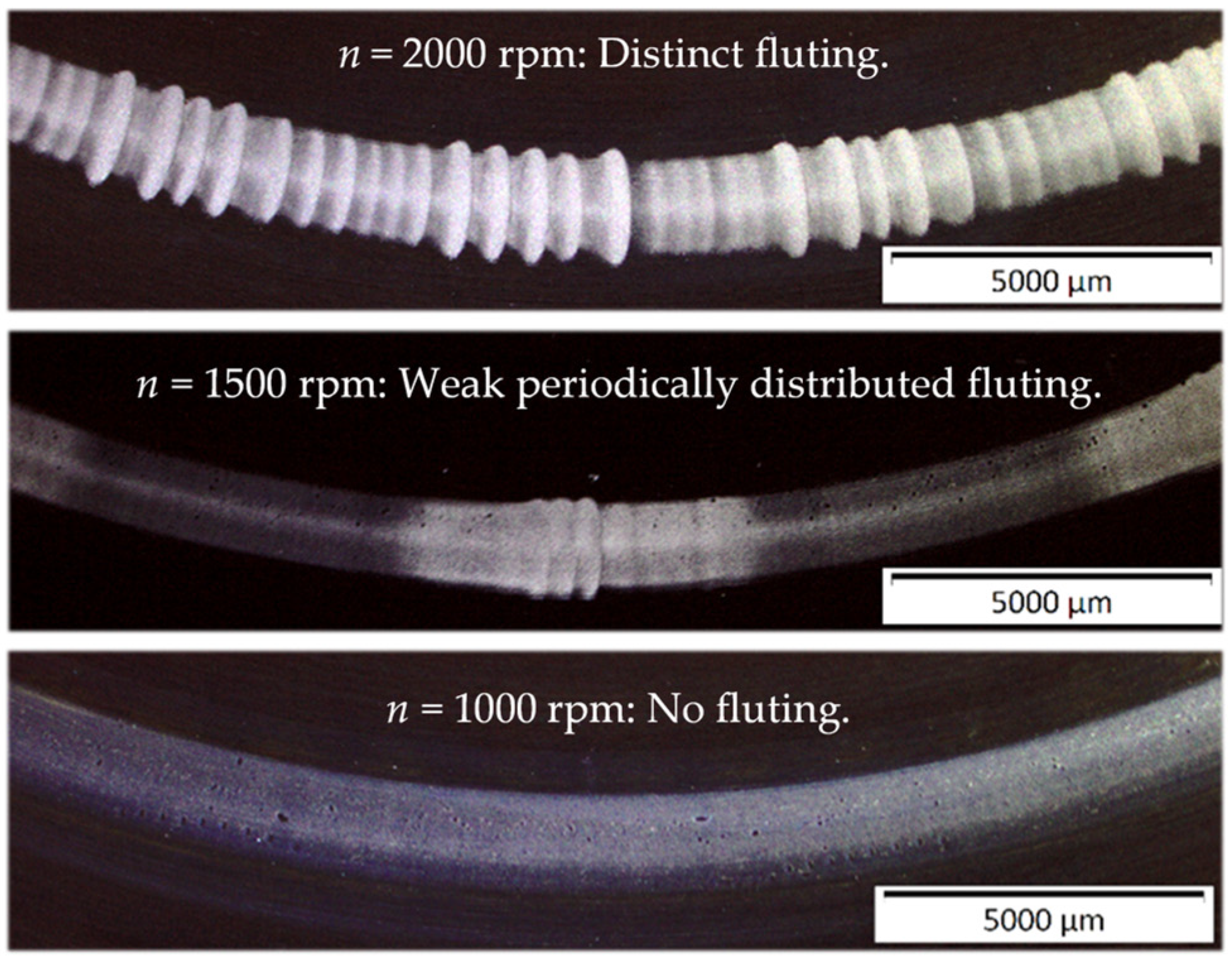
4.4. Bearing Rotational Speed
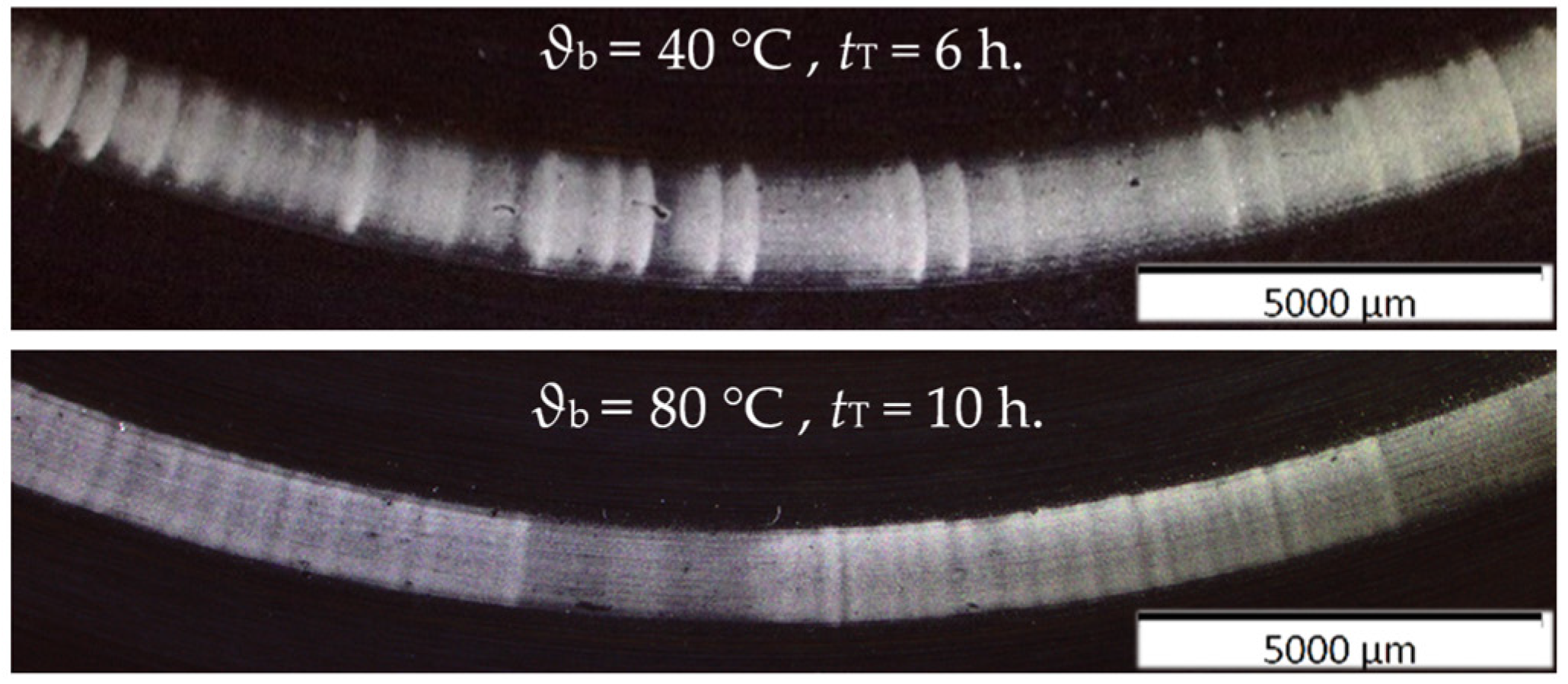
4.5. Average Bearing Temperature
4.6. Number of Balls per Bearing
4.7. Overview of the Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
Nomenclature
| A | Area of contacting a-spots | n | Shaft rotational speed |
| AHz | Total Hertz’ian contact area per raceway | P | Equivalent dynamic load of the bearing |
| Momentary transversal ball acceleration | P | Electrical power | |
| Bearing width | pc,max | Maximum ball–raceway contact pressure | |
| Bearing voltage ratio | Rq1 | Surface profile roughness of a raceway, rms | |
| C | Dynamic load capacity of the bearing | Rq2 | Surface profile roughness of a ball, rms |
| C0 | Static load rating of the bearing | Rvar | Electrical variable resistance of test setup |
| Cb | Bearing capacitance | Sq | Surface profile roughness, rms |
| Cr-f | Rotor-to-frame capacitance | Momentary ball position | |
| Csw-f | Stator winding-to-frame capacitance | Amplitude of | |
| Csw-r | Stator winding-to-rotor capacitance | t | Time |
| D | Bearing outer diameter | tT | Test duration |
| d | Bearing inner diameter | Time-averaged bearing voltage | |
| Eb | Electric field strength in the lubricant | Time-averaged source voltage | |
| ED | Electrical breakdown field strength | Voltage | |
| F | Ball axial force | Momentary bearing voltage | |
| Fb | Bearing force | Common-mode motor terminal voltage | |
| f | Frequency | Momentary source voltage | |
| h | Central lubrication film thickness | wf | Fluting width |
| hf | Fluting height between peak and valley | Zb,DE | Drive-end bearing impedance |
| Minimum oil film thickness | Zb,NDE | Non-drive-end bearing impedance | |
| Ib | Time-averaged bearing current | ZPE | Stator-to-ground impedance |
| ib | Momentary bearing current | Zrg | Rotor-to-ground impedance |
| Bearing current amplitude | Number of the balls per bearing | ||
| iCM | Momentary common-mode current | Wave count of raceway fluting | |
| irg | Momentary rotor-to-ground current | ϑb | Average bearing temperature |
| isg | Momentary stator-to-ground current | λ | Lambda ratio of roughness h/σ |
| Jb | Apparent bearing current density | σ | Composite surface roughness of Rq1 and Rq2 , rms |
References
- Furtmann, A.; Poll, G. Electrical stress and parasitic currents in machine elements of drivetrains with voltage source inverters. Power Transm. Eng. 2018, 2018, 48–53. [Google Scholar]
- Schneider, V.; Behrendt, C.; Hoeltje, P.; Cornel, D.; Becker-Dombrowsky, F.M.; Puchtler, S.; Guitierrez Guzman, F.; Ponick, B.; Jacobs, G.; Kirchner, E. Electrical bearing damage, a problem in the nano- and macro-range. Lubricants 2022, 10, 194–214. [Google Scholar] [CrossRef]
- Graf, S.; Sauer, B. Surface mutation of the bearing raceway during electrical current passage in mixed friction operation. Bear. World J. 2020, 5, 137–147. [Google Scholar]
- Tischmacher, H. Systemanalysen zur Elektrischen Belastung von Wälzlagern bei Umrichtergespeisten Elektromotoren (System Analysis Regarding Electrical Stress in Rolling Element Bearings of Inverter-Fed Electrical Machines, in German). Ph.D. Thesis, Leibniz University Hannover, Hanover, Germany, 2017. [Google Scholar]
- Muetze, A. Thousands of hits: On inverter-induced bearing currents, related work, and the literature. E&I Elektrotechnik Informationstechnik 2011, 128, 382–388. [Google Scholar]
- Puchtler, S.; van der Kuip, J.; Kirchner, E. Analyzing ball bearing capacitance using single steel ball bearings. Tribol. Lett. 2023, 71, 38. [Google Scholar] [CrossRef]
- Scheuermann, S.; Hagemann, B.; Brodatzki, M.; Doppelbauer, M. Influence analysis on the bearings’ impedance behavior of inverter-fed motor drives. In Proceedings of the International Conference Elektrisch-Mechanische Antriebssysteme, Vienna, Austria, 8–9 November 2023; VDE: Hessen, Germany, 2023. [Google Scholar]
- Nagata, Y.; Glovnea, R. Dielectric properties of grease lubricants. Acta Tribol. 2010, 18, 34–41. [Google Scholar]
- Bechev, D.; Kiekbusch, T.; Sauer, B. Characterization of electrical lubricant properties for modelling of electrical drive systems with rolling bearings. Bear. World J. 2018, 3, 93–106. [Google Scholar]
- Raadnui, S.; Kleesuwan, S. Electrical pitting wear debris analysis of grease-lubricated rolling element bearings. Wear 2011, 271, 1707–1718. [Google Scholar] [CrossRef]
- Romanenko, A. Study of Inverter-Induced Bearing Damage Monitoring in Variable-Speed-Driven Motor Systems. Ph.D. Thesis, Lappeenranta University, Lappeenranta, Finland, 2017. [Google Scholar]
- Plazenet, T.; Boileau, T.; Caironi, C.C.; Nahid-Mobarakeh, B. Influencing parameters on discharge bearing currents in inverter-fed induction motors. IEEE Trans. Energy Convers. 2021, 36, 940–949. [Google Scholar] [CrossRef]
- Kohaut, A. Riffelbildung in Waelzlagern infolge elektrischer Korrosion (Fluting in roller bearings due to electrical erosion, in German). Z. Fuer Angew. Phys. 1948, 1, 197–211. [Google Scholar]
- Ortegel, F. Waelzlager in Elektromaschinen (Roller bearings in electrical machines, in German). In Proceedings of the Conference Waelzlager in Elektromaschinen und in der Buerotechnik, Schweinfurt, Germany; 1989. [Google Scholar]
- Harris, T.A. Rolling Bearing Analysis, 4th ed.; John Wiley & Sons: New York, NY, USA, 2001; ISBN 978-0-471-35457-4. [Google Scholar]
- Hamrock, B.J.; Dowson, D. Ball Bearing Lubrication, the Elastohydrodynamics of Elliptical Contacts; John Wiley and Sons: New York, NY, USA, 1981; ISBN 978-0-471-03553-4. [Google Scholar]
- Weicker, M.; Gemeinder, Y. Lagerimpedanz-Berechnungsprogramm V3-0, AxRiKuLa (Bearing Impedance Calculation Program), In-house calculator, Institute for Electrical Energy Conversion; TU Darmstadt: Darmstadt, Germany, 2018. [Google Scholar]
- Andreason, S. Stromdurchgang Durch Waelzlager (Passage of Electric Current through Rolling Bearings, in German). Die Kugellagerzeitschrift (SKF Co.) 1968, 153, 6–12. [Google Scholar]
- Holm, R. Electric Contacts, 4th ed.; Springer: Berlin/Heidelberg, Germany, 2013; ISBN 978-3-642-05708-3. [Google Scholar]
- Pittroff, H. Waelzlager im Elektrischen Stromkreis: Riffelbildung Infolge von Stromdurchgang (Roller Bearings in Electrical Circuits: Fluting Due to Current Flow, in German). Elektr. Bahnen 1968, 39, 54–61. [Google Scholar]
- Radnai, B.; Gemeinder, Y.; Sauer, B.; Binder, A. Schaedlicher Stromdurchgang (Harmful Bearing Currents, in German); Research Report Part I, Report no. 1127; FVA Forschungsvereinigung Antriebstechnik e.V.: Frankfurt, Germany, 2015. [Google Scholar]
- Safdarzadeh, O.; Binder, A.; Weicker, M. Measuring Electric Contact in an Axial Ball Bearing at DC Current Flow. IEEE Trans. Ind. Appl. 2023, 59, 3341–3352. [Google Scholar]
- Kuechler, A. High Voltage Engineering: Fundamentals-Technology-Applications; Springer: Berlin/Heidelberg, Germany, 2017; ISBN 978-3-642-11992-7. [Google Scholar]
- Safdarzadeh, O.; Weicker, M.; Binder, A. Transient Thermal Analysis of the Contact in Bearings Exposed to Electrical Currents. In Proceedings of the 4th International FVA-Conference: “The Expert Forum for Bearings”, Wuerzburg, Germany, 5–6 July 2022; pp. 24–32. [Google Scholar]
- Prashad, H. Tribology in Electrical Enviroments; Elsevier: Hyderabad, India, 2005; ISBN 978-0-444-51880-4. [Google Scholar]
- ISO 25178; Geometrical Product Specifications (GPS)—Surface Texture: Areal. ISO: Geneva, Switzerland, 2016.
- ISO 16610-61; Geometrical Product Specification (GPS)—Filtration—Part 61: Linear Areal Filters—Gaussian Filters. ISO: Geneva, Switzerland, 2015.
- Mikami, H.; Kawamura, T. Influence of Electrical Current on Bearing Flaking Life; Technical Paper no. 2007-01-0113; SAE World Congress & Exhibition: Detroit, MI, USA, 2007. [Google Scholar]
- Capan, R.; Safdarzadeh, O.; Graf, S.; Weicker, M.; Sauer, B.; Binder, A.; Koch, O. Schädlicher Stromdurchgang (Harmful Bearing Currents), in German; Research Report, Part III; FVA Forschungsvereinigung Antriebstechnik e.V.: Frankfurt, Germany, 2023. [Google Scholar]


| Term | Description |
|---|---|
| Bearing lubricant | Mineral-oil-based grease from the company Schaeffler, named Arcanol Multi 3. Thickener: lithium soap. Base oil viscosity at 40 °C 110 mm2/s, at 100 °C 12 mm2/s. Operating temperature −20 °C to 120 °C. |
| Axial bearing | . Company Schaeffler, static load capacity C0 = 97 kN, dynamic load capacity C = 44 kN. |
| Digital oscilloscope | Model Waverunner LT364L, 500 MHz, accuracy 2 mV, vertical resolution 8 bits, 1 M samples capture memory. |
| Current clamp | Model IWATSU SS-250, 100 MHz, max. 30 A, accuracy ±1.0% or ±10 mA. |
| Voltage probe | Model TT-SI200, 200 MHz, ±60 V, accuracy ±1.0% or ±20 mV. |
| DC motor | 12 V, 120 W, 1500 rpm. |
| DC voltage source | Model K3010D, 30 V, 10 A. |
| Fb = 400 N, pc,max = 691 N/mm2 | Fb = 1600 N, pc,max = 1096 N/mm2 | |||
|---|---|---|---|---|
| 100 rpm | h = 0.11 μm | λ = h/σ = 1 < 3 | h = 0.10 μm | λ = h/σ = 0.9 < 3 |
| 1500 rpm | h = 0.62 μm | λ = h/σ = 5.6 > 5 | h = 0.57 μm | λ = h/σ = 5.2 > 5 |
| Term | Description |
|---|---|
| Bearing lubricant | Polyglycol oil, company Klueber, named internally OF4.1, water content 321 ppm, kinematic viscosity at 40 °C: 100.2 mm2/s *, at 30 °C: 153.8 mm2/s *, relative permittivity at 40 °C: 6.14 **. |
| Axial bearing | Type 51208, same as in test rig #1. |
| Heater–cooler system | Model RP 1845, Company Lauda, operating temperature between -40 °C and 200 °C. |
| Digital oscilloscope | Model NI USB-5133, Company National Instruments, bandwidth 50 MHz, vertical resolution 8 bits, 100 MS/s, 32 MB memory per channel (2 channels). |
| Current clamp | Model N2782B, Company Agilent, max. 30 A DC, bandwith 50 MHz. |
| Voltage probe | Model TT-LF 312, bandwidth 150 MHz (10:1) and 15 MHz (1:1), max. 600 V, Company Testec. |
| Voltage source | Model HCS-3304, Company Manson, 60 V, 8 A. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Safdarzadeh, O.; Capan, R.; Werner, M.; Binder, A.; Koch, O. Influencing Factors on the Fluting in an Axial Ball Bearing at DC Bearing Currents. Lubricants 2023, 11, 455. https://doi.org/10.3390/lubricants11100455
Safdarzadeh O, Capan R, Werner M, Binder A, Koch O. Influencing Factors on the Fluting in an Axial Ball Bearing at DC Bearing Currents. Lubricants. 2023; 11(10):455. https://doi.org/10.3390/lubricants11100455
Chicago/Turabian StyleSafdarzadeh, Omid, Resat Capan, Michel Werner, Andreas Binder, and Oliver Koch. 2023. "Influencing Factors on the Fluting in an Axial Ball Bearing at DC Bearing Currents" Lubricants 11, no. 10: 455. https://doi.org/10.3390/lubricants11100455
APA StyleSafdarzadeh, O., Capan, R., Werner, M., Binder, A., & Koch, O. (2023). Influencing Factors on the Fluting in an Axial Ball Bearing at DC Bearing Currents. Lubricants, 11(10), 455. https://doi.org/10.3390/lubricants11100455






