Friction Issues over the Railway Wheels-Axis Assembly Motion
Abstract
:1. Introduction
2. Friction in Wheel–Rail Contact
3. Bearings Utilized in Railway Applications and Vehicles Equipped with Bogies
3.1. Types of Bearings for Railway Applications and Vehicles Equipped with Bogies
- -
- Tapered roller bearing units;
- -
- Cylindrical roller bearings and cylindrical roller bearing units;
- -
- Spherical roller bearings.
3.2. Lubrication of Bearings for Railway Applications and Vehicles Equipped with Bogies
3.3. Frictional Torque and Temperature in Roller Bearing
- -
- Rolling friction between the raceway surfaces of inner and outer rings and rolling surfaces;
- -
- Sliding friction between the inner ring rib and roller end surface;
- -
- Churning resistance of lubricating oil;
- -
- Sliding friction between the rollers and cage.
3.4. Failures in Roller Bearing
- -
- Improper and/or excessive/inadequate grease;
- -
- Bearing clearance not within prescribed limits;
- -
- Journal finish and diameter not in accordance with the OEM;
- -
- Excessive or inadequate lateral clearance between axle box covers and bearings;
- -
- Too little or too much gap between rollers and roller rings.
- -
- Loose bearing failure 23%;
- -
- Water etch 20%;
- -
- Wheel tread defect 13%;
- -
- Fatigue spalling 13%;
- -
- Bearing destruction 12%;
- -
- Mechanical problems 6%;
- -
- Lubrication problems 4%;
- -
- Adapter (displaced, worn, wrong size or broken) 4%;
- -
- Displaced seal 3%;
- -
- Truck related failure 2%;
- -
- Application defects about 0%;
- -
- Manufacturer, remanufacturer or reconditioner defect about 0%.
4. Materials and Methods
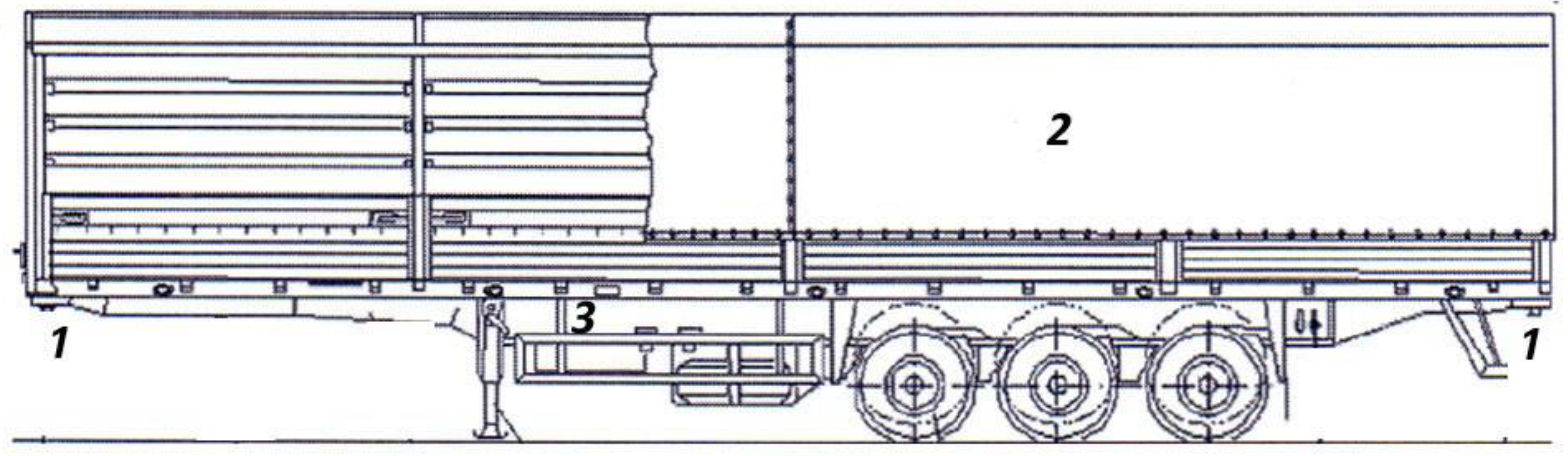
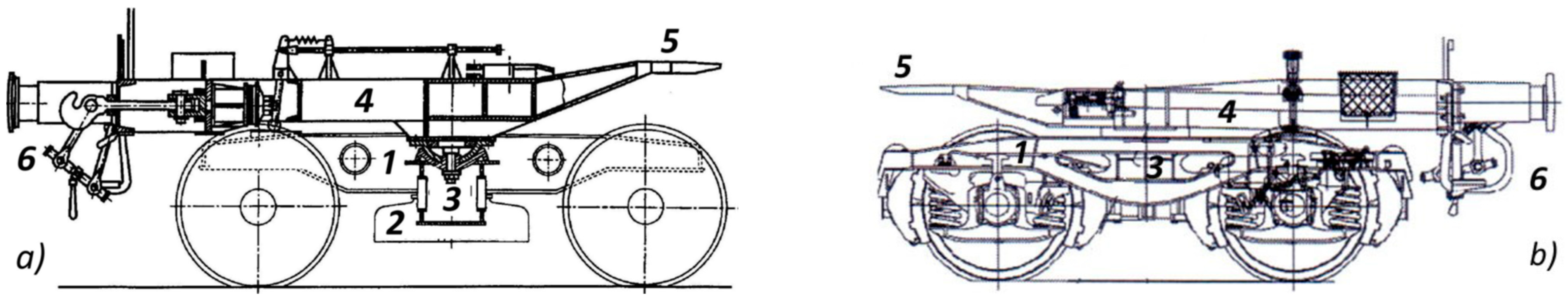
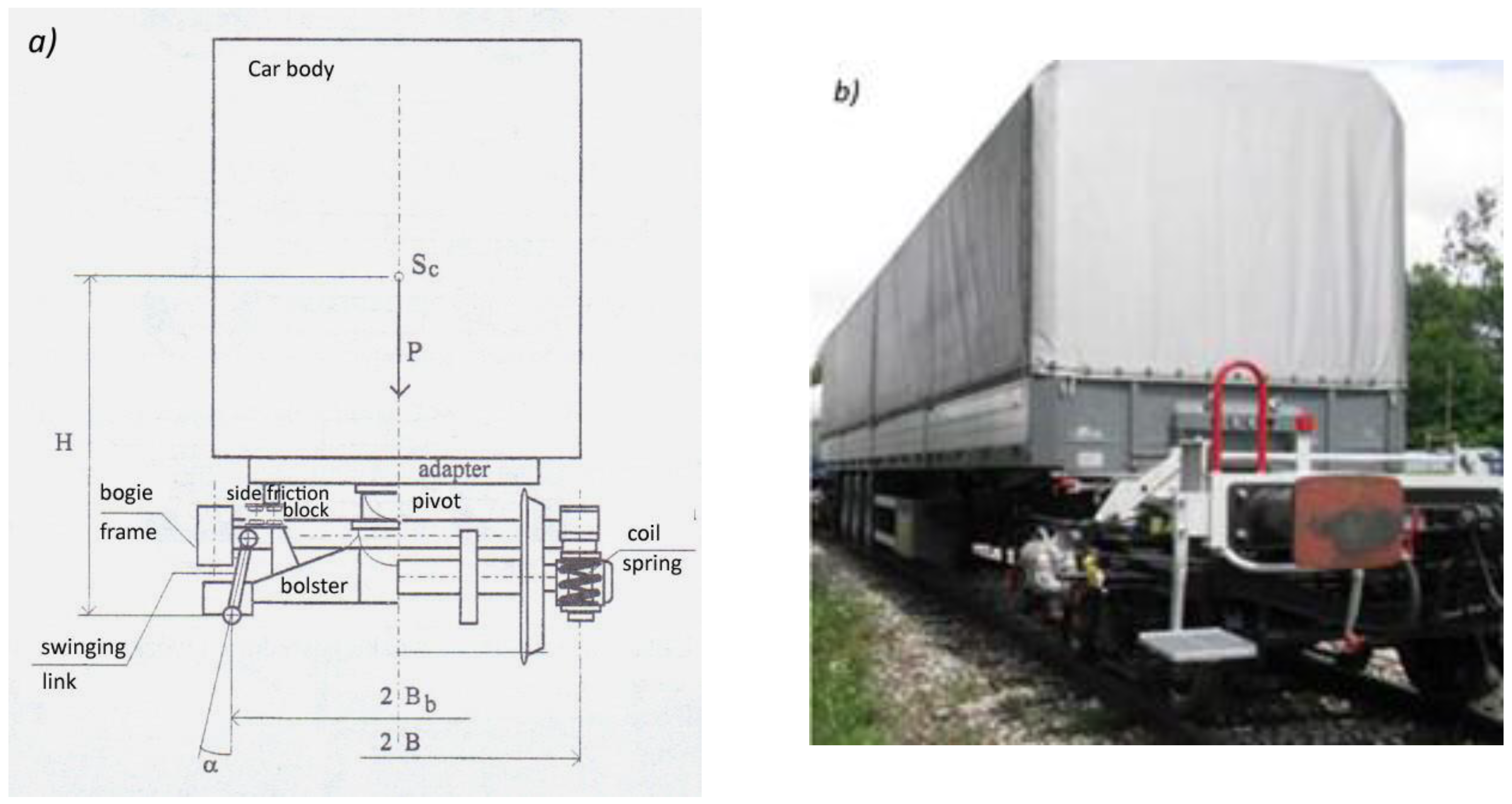
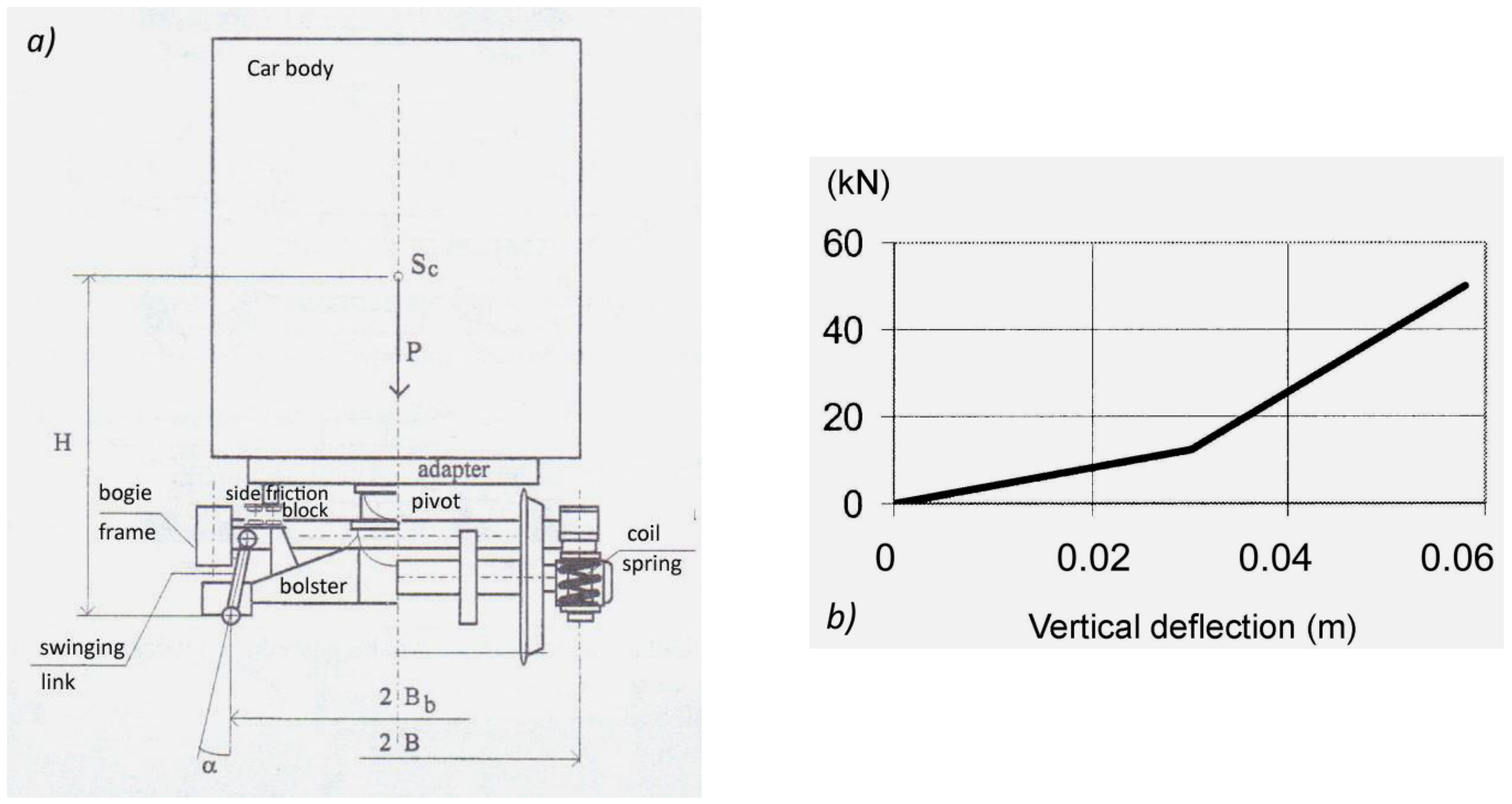
4.1. Structural Elements of a Freight Wagon in the Bimodal System
4.2. Simulation Model of the Freight Wagon
4.3. Wheel–Rail Contact Model
4.4. Computational Parameters of the Simulation Model
- -
- wagon base was equal to 14 m,
- -
- bogie base was equal to 2.3 m,
- -
- total mass of empty wagon was equal to 25,070 kg, and that of the loaded one was equal to 48,070 kg,
- -
- mass of empty wagon body was equal to 11,000 kg and that of the fully loaded one was equal to 34,000 kg,
- -
- wheelset mass was equal to 1700 kg.
- -
- nominal wheel diameter was equal to 0.92 m.
- -
- lateral stiffness of primary suspension spring was equal to 3890 kN/m for the empty wagon and to 5560 kN/m for the loaded one;
- -
- longitudinal stiffness of primary suspension spring was equal to 1000 kN/m;
- -
- lateral stiffness of secondary suspension spring was equal to 113 kN/m for the emp-ty wagon and to 351 kN/m for the loaded one;
- -
- vertical stiffness of secondary suspension spring was equal to 100,000 kN/m;
- -
- damping coefficient of primary suspension in longitudinal, lateral and vertical directions was equal to 0 kNs/m, 7 kNs/m and 7 kNs/m, respectively, for the case of the empty wagon, and to 0 kNs/m, 12 kNs/m and 12 kNs/m, respectively, for the case of the loaded one;
- -
- damping coefficient of secondary suspension in lateral direction was equal to 0 kNs/m;
- -
- vertical stiffness of outer spring in primary suspension was equal to 1548 kN/m;
- -
- vertical stiffness of inner spring in primary suspension was equal to 3748 kN/m;
- -
- vertical stiffness of side friction block spring was equal to 2740 kN/m;
- -
- damping coefficient of side friction block in longitudinal and lateral directions was equal to 0.1 kNs/m;
- -
- damping coefficient on the central pivot surface was equal to 0.1 kNs/m.
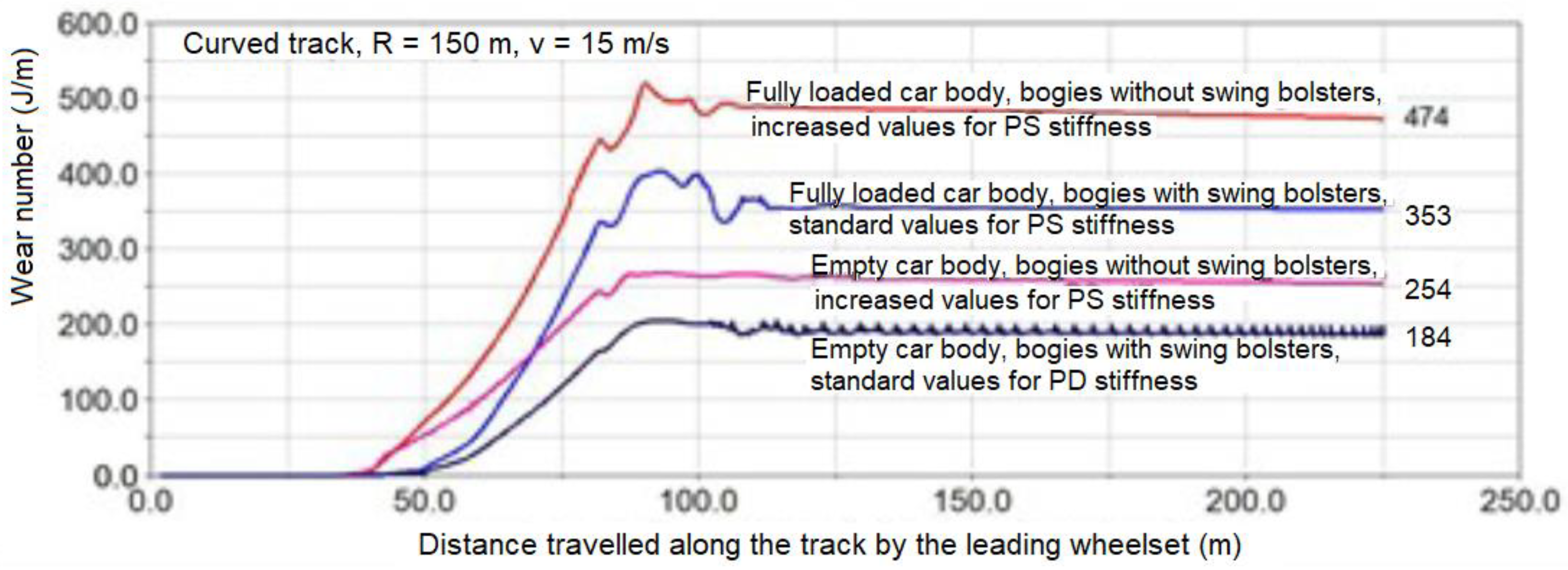
4.5. Wear Numbers for Wheels and Rails on a Curved Track with a Small Curve Radius
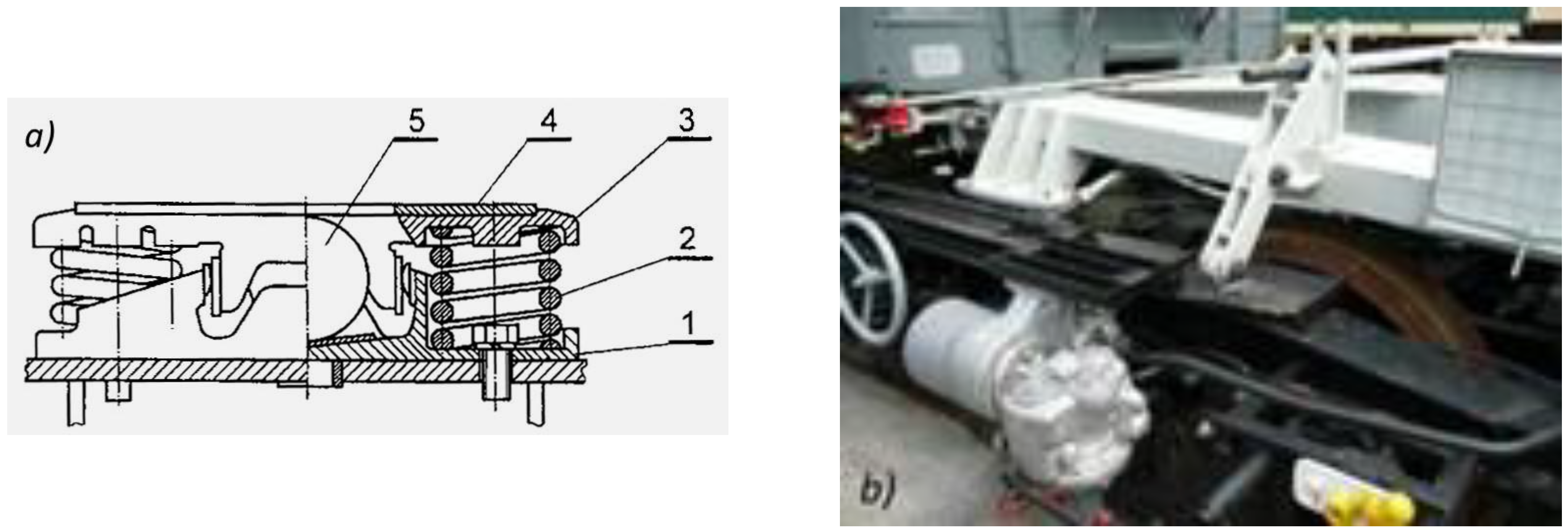
4.6. Friction in the Set of Bearings in the Wheels-Axis Assembly in Bogie
- -
- for a wheel under overloading (running on the inside of the curve);
- -
- for a wheel under underloading (running on the outer side of the curve).
- —the non-dimensional ratio of the peripheral friction force and the total friction forces in the wheel–rail contact [45]. Its values can be in range <0,1>;
- —the non-dimensional ratio of transverse friction force and the total friction force in the wheel–rail contact [45]. Its values can also be in range <0,1>;
- —the total friction force calculated from Equation (8) [45].
- —an angle of the anticipation of wheel slip on the rail. Its value was assumed to be equal to 0, for each case at present study;
- —the anticipation of wheel slip on the rail;
- —radius of the wheel;
- Ψ—an angle of wheel slip on the rail. Its values were assumed to be in range <0,0.5> mrad.
- G—maximum static axlebox load [kN], —maximum static axleload [kN], —weight of the wheelset [kN]
- Kr = mean radial load [kN], = 1—assumed payload factor, = 1.3—assumed dynamic radial factor, = 1—assumed dynamic traction factor.
- —mean axial load [kN], = 0.1—assumed dynamic axial factor.
- —factor calculated from Equation (17) [12]:
- = 130 mm—shaft diameter, = 150 mm—distance between two load centres, = 0.1—factor, which value related to the case of the load acting near to the middle plane of the bearing.
- —the rolling frictional torque affected by lubricant starvation and inlet shear heating [Nmm], which is calculated from Equation (19) [51]:
- —rotational speed [rpm], 50 kph—assumed wagon speed, 130 mm2/s—kinematic viscosity of the base oil of the grease at temperature of 20 °C, —the inlet shear heating reduction factor calculated from Equation (20) [51]:
- —bearing mean diameter [mm], = 130 mminner diameter of bearing, = 230 mm—outer diameter of bearing
- —the kinematic replenishment/starvation reduction factor calculated from Equation (21) [51]:
- —the replenishment/starvation constant for grease lubrication, —bearing type related geometric constant, for tapered roller bearings.
- —variable determined from Equation (22) [51]:
- , —geometrical constants for tapered roller bearings.
- —the axial load factor calculated from Formula (23) [51].
- —the assumed parameter for tapered roller bearing.
- —the sliding frictional torque affected by the quality of lubrication conditions [Nmm], which is calculated from Equation (24) [51]:
- —variable determined from Equation (25) [51]:
- , —geometrical constants for tapered roller bearings.
- —sliding friction coefficient calculated from Equation (26) [51]:
- —constant during wagon motion, reflecting effect of boundary lubrication, —constant for tapered roller bearing, reflecting effect of EHL conditions.
- —weighting factor for the sliding friction coefficient calculated from Equation (27) [51]:
- —the frictional torque from integral seals [Nmm], calculated from Equation (28) [51]:
- mm—seal counterface diameter, , , —constants assumed for tapered roller bearing.
- —the frictional torque from drag losses, churning, splashing, etc. [Nmm]. It was assumed that
5. Results and Discussion
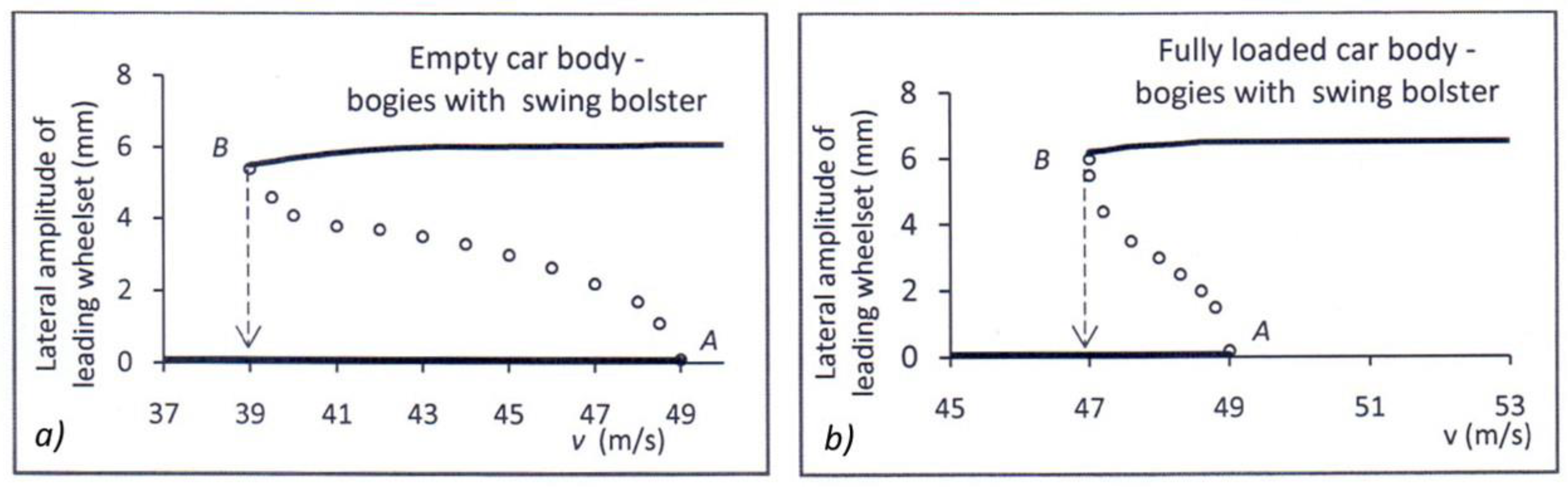
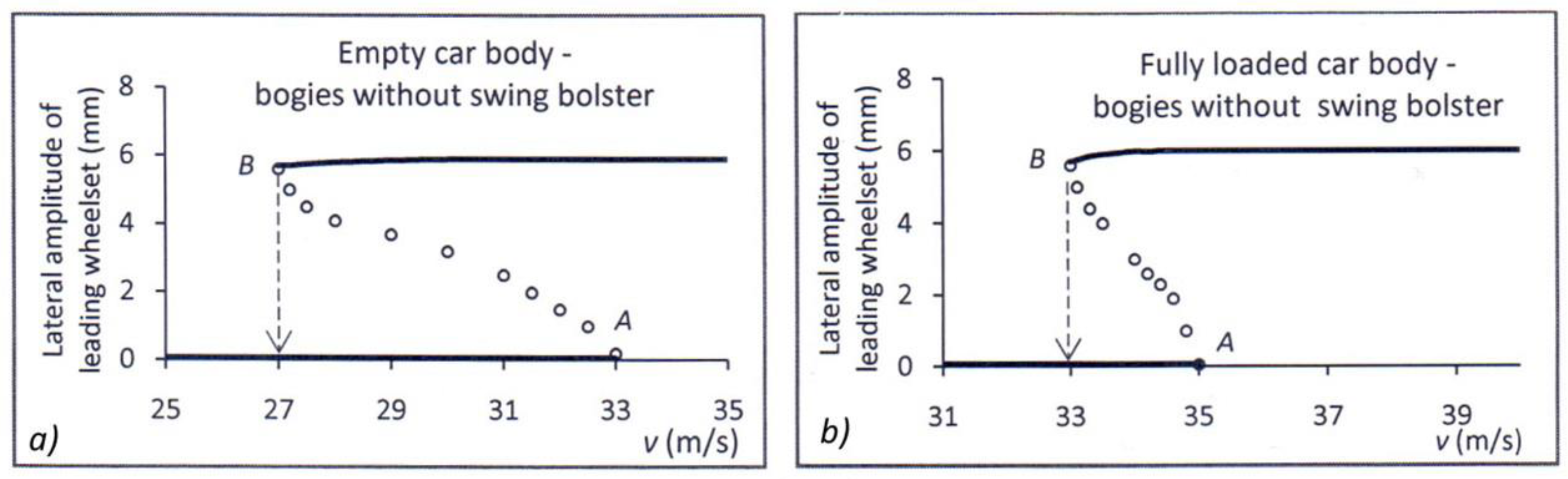
5.1. Results of Numerical Simulations on Straight Track
5.2. Wear Numbers for Wheels and Rails Obtained on a Curved Track with a Small Curve Radius
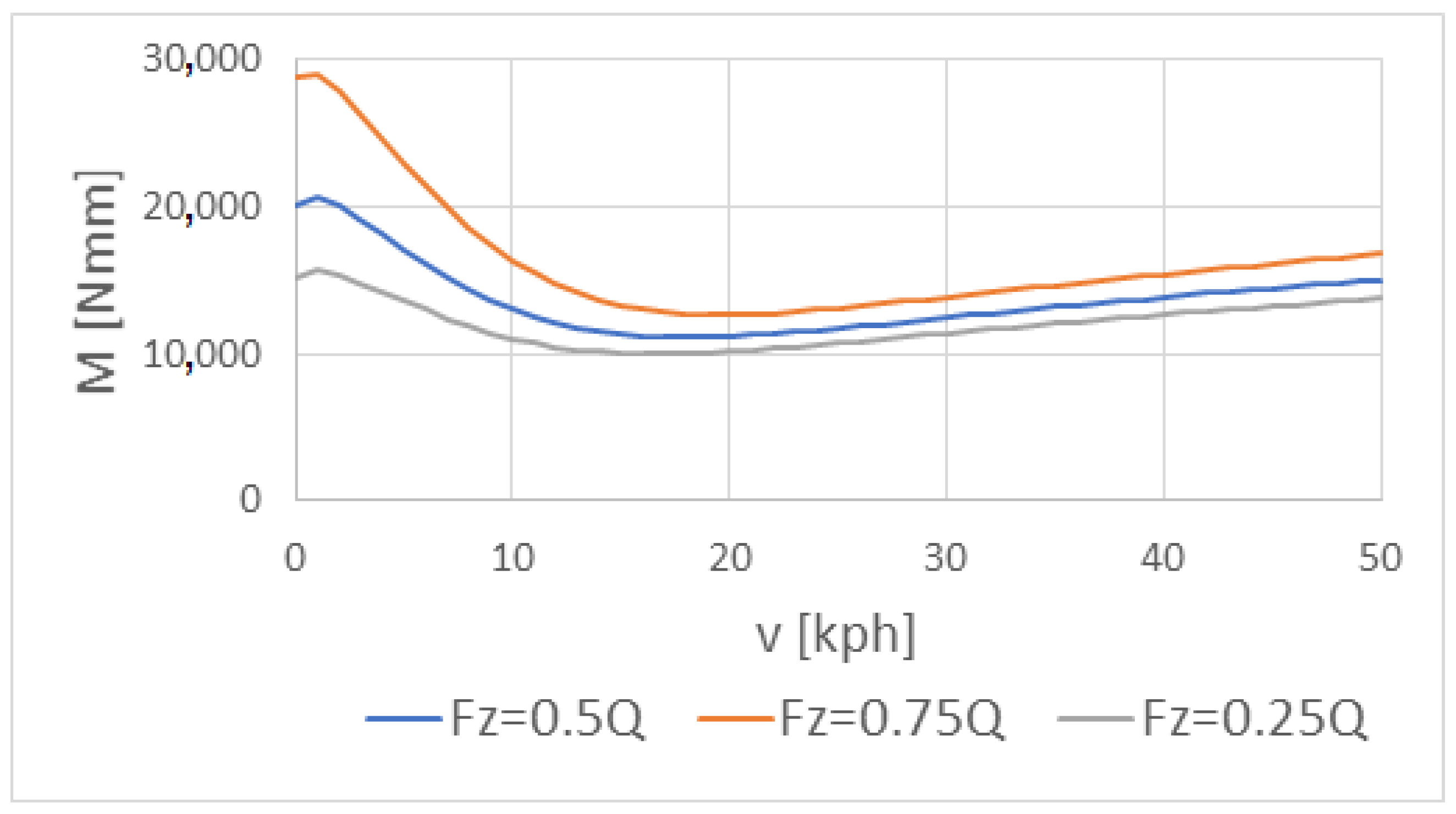
5.3. Results of Numerical Calculations of Friction in Bearing
- —minimum value of the resistive torque in taped roller bearing
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Medwid, M.; Stawecki, W.; Czerwinsk, J. Bimodal rolling stock as the economically justified alternative to the existing combined transport systems. Pojazdy Szyn. 2016, 2, 1–8. [Google Scholar]
- Reddy, V.; Chattopadhyay, G.; Hargreaves, D. Analysis of Rail Wear Data for Evaluation of Lubrication Performance. Cquniversity. Conference Contribution. 2006. Available online: https://hdl.handle.net/10018/42614 (accessed on 2 December 2021).
- Chen, G. Friction-Induced Vibration of a Railway Wheelset-Track System and Its Effect on Rail Corrugation. Lubricants 2020, 8, 18. [Google Scholar] [CrossRef] [Green Version]
- Khan, S.A.; Persson, I.; Lundberg, J.; Stenström, C. Prediction of the effects of friction control on top-of-rail cracks. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2016, 232, 484–494. [Google Scholar] [CrossRef] [Green Version]
- Trummer, G.; Lee, Z.S.; Lewis, R.; Six, K. Modelling of Frictional Conditions in the Wheel–Rail Interface Due to Application of Top-of-Rail Products. Lubricants 2021, 9, 100. [Google Scholar] [CrossRef]
- Eadie, D.T.; Vidler, B.; Hooper, N.E.; Makowsky, T. Top of rail friction control: Lateral force and rail wear reduction in a freight application. In Proceedings of the International Heavy Haul Association Conference, Fort Worth, TX, USA, 5–9 May 2003; pp. 573–581. [Google Scholar]
- Lundberg, J.; Rantatalo, M.; Wanhainen, C.; Casselgren, J. Measurements of friction coefficients be-tween rails lubricated with a friction modifier and the wheels of an IORE locomotive during real working conditions. Wear 2015, 324–325, 109–117. [Google Scholar] [CrossRef] [Green Version]
- Gallardo-Hernandez, E.A.; Lewis, R. Twin disc assessment of wheel/rail adhesion. Wear 2008, 265, 1309–1316. [Google Scholar] [CrossRef] [Green Version]
- Lemma, Y.; Rantatalo, M.; Lundberg, J. Top-of-Rail Friction Measurements of the Swedish Iron Ore Line. In Proceedings of the 3rd international workshop and Congress on eMaintenance, Lulea, Sweden, 17–18 June 2014. [Google Scholar]
- Products for Railway Applications. Schaeffler Group Industrial. 2010. Available online: https://www.schaeffler.com/remotemedien/media/_shared_media/08_media_library/01_publications/schaeffler_2/tpi/downloads_8/tpi_158_de_en.pdf (accessed on 2 December 2021).
- Railways. NSK Ltd. 2021. Available online: https://www.nsk.com/industries/railways.html (accessed on 2 December 2021).
- Railway Technical Handbook. Vol. 1. Axleboxes, Wheelset Bearings, Sensors, Condition Monitoring, Subsystems and Services. SKF Group. 2011. Available online: https://www.skf.com/binaries/pub12/Images/0901d196801410ca-10987_2-EN_tcm_12-503040.pdf (accessed on 2 December 2021).
- Iwnicki, S. Handbook of Railway Vehicle Dynamics; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Fernandes, H.M.G. Analysis of Failures of Rolling Stock Railways Rolling Bearings. Master’s Thesis, Faculty of Engineering of the University of Porto, Porto, Portugal, 2017. [Google Scholar]
- EN 12081:2017 Railway Applications—Axleboxes—Lubricating Greases. Available online: https://www.nlfnorm.cz/en/ehn/5841 (accessed on 2 December 2021).
- Cyriac, F.; Lugt, P.M.; Bosman, R. Yield Stress and Low-Temperature Start-Up Torque of Lubricating Greases. Tribol. Lett. 2016, 63, 6. [Google Scholar] [CrossRef] [Green Version]
- Grease Lubrication for Railway Axlebox Bearings. Evol. SKF Technol. Mag. 2007, 7. Available online: https://evolution.skf.com/grease-lubrication-for-railway-axlebox-bearings/ (accessed on 2 December 2021).
- Lundberg, J.; Aditya, P.; Söderholm, P. Running temperature and mechanical stability of grease as maintenance parameters of railway bearings. Int. J. Autom. Comput. 2010, 7, 160–166. [Google Scholar] [CrossRef]
- Bosman, R.; Lugt, P.M. The Microstructure of Calcium Sulfonate Complex Lubricating Grease and Its Change in the Presence of Water. Tribol. Trans. 2018, 61, 842–849. [Google Scholar] [CrossRef] [Green Version]
- He, Q.; Li, A.; Guo, Y.; Liu, S.; Kong, L.-H. Effect of nanometer silicon dioxide on the frictional behavior of lubricating grease. Nanomater. Nanotechnol. 2017, 7, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Hu, E.Z.; Xu, Y.; Hu, K.H.; Hu, X.G. Tribological properties of 3 types of MoS2 additives in different base greases. Lubr. Sci. 2017, 29, 541–555. [Google Scholar] [CrossRef]
- Zheng, B.; Zhou, J.; Jia, X.; He, Q. Friction and wear property of lithium grease contained with copper oxide nanoparticles. Appl. Nanosci. 2019, 10, 1355–1367. [Google Scholar] [CrossRef]
- Mohamed, A.; Ali, S.; Osman, T.A.; Kamel, B.M. Development and manufacturing an automated lubrication machine test for nano grease. J. Mater. Res. Technol. 2020, 9, 2054–2062. [Google Scholar] [CrossRef]
- Casado, J.E.; González, A.F.; Huerga, Á.J.D.R.; Rodríguez-Solla, H.; Díaz-García, M.E.; Badía-Laíño, R. Unctuous ZrO2 nanoparticles with improved functional attributes as lubricant additives. IOP Publ. Nanotechnol. 2017, 28, 495704. [Google Scholar] [CrossRef] [PubMed]
- Rylski, A.; Siczek, K. The Effect of Addition of Nanoparticles, Especially ZrO2-Based, on Tribological Behavior of Lubricants. Lubricants 2020, 8, 23. [Google Scholar] [CrossRef] [Green Version]
- Pape, F.; Poll, G. Investigations on Graphene Platelets as Dry Lubricant and as Grease Additive for Sliding Contacts and Rolling Bearing Application. Lubricants 2020, 8, 3. [Google Scholar] [CrossRef] [Green Version]
- Yamanaka, H.; Zenbutsu, M. Development of Tapered Roller Bearings for Electric/Hybrid Vehicles, NSK Technical Journal Motion & Control 32, 2021, 1–6. Available online: https://www.nsk.com/rd/pdf/techJournal/etj-0032.pdf (accessed on 2 December 2021).
- Zhang, C.; Gu, L.; Mao, Y.; Wang, L. Modeling the frictional torque of a dry-lubricated tapered roller bearing considering the roller skewing. Friction 2019, 7, 551–563. [Google Scholar] [CrossRef] [Green Version]
- Gupta, P.K. On the Dynamics of a Tapered Roller Bearing. ASME J. Tribol. 1989, 111, 278–287. [Google Scholar] [CrossRef]
- Schwarz, V.A. Experimental studies of the thermal behaviour of a tapered roller bearing assembly. In Proceedings of the 11th Brazilian Congress of Thermal Sciences and Engineering ENCIT, Curitiba, Brazil, 5–8 December 2006. [Google Scholar]
- Witte, D.C. Operating torque of tapered roller bearings. ASLE Trans. 1973, 16, 61–67. [Google Scholar] [CrossRef]
- Aihara, S. A new running torque formula for tapered roller bearings under axial load. J. Tribol. 1987, 109, 471–477. [Google Scholar] [CrossRef]
- Zhou, R.S.; Hoeprich, M.R. Torque of tapered roller bearings. J. Tribol. 1991, 113, 590–597. [Google Scholar] [CrossRef]
- Yan, K.; Wang, N.; Zhai, Q.; Zhu, Y.; Zhang, J.; Niu, Q. Theoretical and experimental investigation on the thermal characteristics of double-row tapered roller bearings of high speed locomotive. Int. J. Heat Mass Transf. 2015, 84, 1119–1130. [Google Scholar] [CrossRef]
- Monograph on ICF. All-Coil Coaches. Indian Railw. Inst. Civ. Eng. (IRICEN) Monogr. 2016, 1, 411001. Available online: https://www.iricen.gov.in/iricen/books_jquery/Monograph%20on%20ICF.pdf (accessed on 2 December 2021).
- MAXBE_D2.1a) Axle Bearings Failure Modes and Degradation Process. Available online: https://web.fe.up.pt/~maxbe/maxbe_wps.html (accessed on 9 February 2022).
- VI-Grade Engineering Software and Services. VI Rail 15.0 Documentation. 2019. Available online: https://www.vi-grade.com/dynatc/releasenotes-0189-4a93/VI_Automotive_19_0_Release_Notes.pdf (accessed on 2 December 2021).
- Kalker, J.J. Three-Dimensional Elastic Bodies in Rolling Contact. In Solid Mechanics and Its Applications; Springer: Dordrecht, The Netherlands; Boston, MA, USA; London, UK, 1990. [Google Scholar]
- Piotrowski, J.; Kik, W. A simplified model of wheel/rail contact mechanics for non-hertzian problems and its application in rail vehicle dynamic simulations. Veh. Syst. Dyn. 2008, 46, 27–48. [Google Scholar] [CrossRef]
- Kisilowski, J.; Knothe, K. Advanced Railway Vehicle System Dynamics; WNT: Warszawa, Poland, 1991. [Google Scholar]
- Iwnicki, S. Manchester Benchmarks for Rail Vehicle Simulation. Veh. Syst. Dyn. 1998, 30, 295–313. [Google Scholar] [CrossRef]
- EN 14363:2016; Railway Applications. Testing and Simulation for the Acceptance of Running Characteristics of Railway Vehicles. Running Behaviour and Stationary Tests. Comite Europeen de Normalisation: Brussels, Belgium, 2016.
- Jendel, T.; Berg, M. Prediction of Wheel Profile Wear. Veh. Syst. Dyn. 2002, 37 (Suppl. 1), 502–513. [Google Scholar] [CrossRef]
- Matej, J. Modeling and computer simulations of bimodal cars from the point of view of their proneness to derailment. Pr. Nauk. Politech. Warszawskiej. Mech. 2010, 234, 3–132. [Google Scholar]
- Elkins, J.A.; Carter, A. Testing and Analysis Techniques for Safety Assessment of Rail Vehicles. Veh. Syst. Dyn. 1993, 2, 185–208. [Google Scholar] [CrossRef]
- Nagase, K.; Wakabayashi, Y.; Sakahara, H. A study of the phenomenon of wheel climb derailment: Results of basic experiments using model bogies. IMechE Part F J. Rail Rapid Transit 2002, 216, 237–247. [Google Scholar] [CrossRef]
- Shu, X.; Wilson, N.; Wu, H.; Tunna, J. A bi-parameter distance criterion for flange climb derailment. In Proceedings of the ASME/IEEE Joint Rail Conference, Pueblo, CO, USA, 16–18 March 2005; pp. 9–17. [Google Scholar]
- Wu, H.; Shu, X.; Wilson, N. Flange Climb Derailment Criteria and Wheel/Rail Profile Management and Maintenance Guidelines for Transit Operations; TCRP Report 71; Transportation Technology Center, Inc.: Pueblo, CO, USA, 2005. [Google Scholar]
- Zeng, J.; Guan, Q.H. Study on flange climb derailment criteria of a railway wheelset. Veh. Syst. Dyn. 2008, 46, 239–251. [Google Scholar] [CrossRef]
- The SKF Model for Calculating the Frictional Moment. SKF, 2021, 1–15. Available online: https://www.skf.com/binaries/pub12/Images/0901d1968065e9e7-The-SKF-model-for-calculating-the-frictional-moment_tcm_12-299767.pdf (accessed on 2 December 2021).
- Kanazawa, Y.; de Laurentis, N.; Kadiric, A. Studies of Friction in Grease-Lubricated Rolling Bearings Using Ball-on-Disc and Full Bearing Tests. Tribol. Trans. 2020, 63, 77–89. [Google Scholar]
- Bercea, I.; Mita, N.; Damian, I.; Bercea, M.; Cretu, S. A Theoretical and Experimental Study on Friction Losses in a Tapered Roller Bearing. Tibology Ind. 1996, 18, 19–23. [Google Scholar]

| Case | Critical Speeds (m/s) |
|---|---|
| Empty car body, bogies without swing bolster | 27/33 |
| Fully loaded wagon, bogie without swing bolster | 33/35 |
| Empty car body, bogies with swing bolster | 39/49 |
| Fully loaded wagon, bogies with swing bolster | 47/49 |
| Bogie with Swing Bolster | Bogie without Swing Bolster | ||
|---|---|---|---|
| Standard stiffness for primary suspension data | Increased stiffness for primary suspension | ||
| Empty wagon body | Fully loaded wagon body | Empty wagon body | Fully loaded wagon body |
| Wear numbers (J/m) | Wear numbers (J/m) | ||
| 184 | 353 | 254 | 474 |
| Wheel Loading Case | [–] | |
|---|---|---|
| 0.0018 | ||
| 0.0014 | ||
| Nadal criterion | 0.0029 | |
| Weinstock criterion | 0.0024 | |
| Matej criterion | 0.0027 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Derbiszewski, B.; Obraniak, A.; Wozniak, M.; Rylski, A.; Siczek, K.; Kubiak, P. Friction Issues over the Railway Wheels-Axis Assembly Motion. Lubricants 2022, 10, 26. https://doi.org/10.3390/lubricants10020026
Derbiszewski B, Obraniak A, Wozniak M, Rylski A, Siczek K, Kubiak P. Friction Issues over the Railway Wheels-Axis Assembly Motion. Lubricants. 2022; 10(2):26. https://doi.org/10.3390/lubricants10020026
Chicago/Turabian StyleDerbiszewski, Bogdan, Andrzej Obraniak, Marek Wozniak, Adam Rylski, Krzysztof Siczek, and Przemyslaw Kubiak. 2022. "Friction Issues over the Railway Wheels-Axis Assembly Motion" Lubricants 10, no. 2: 26. https://doi.org/10.3390/lubricants10020026
APA StyleDerbiszewski, B., Obraniak, A., Wozniak, M., Rylski, A., Siczek, K., & Kubiak, P. (2022). Friction Issues over the Railway Wheels-Axis Assembly Motion. Lubricants, 10(2), 26. https://doi.org/10.3390/lubricants10020026






