Probing the Dynamic Progression of Erosion–Corrosion of X65 Pipeline Steel Using the Electrical Resistance Method in Conjunction with Galvanostatic Polarization
Abstract
1. Introduction
2. Materials and Methods
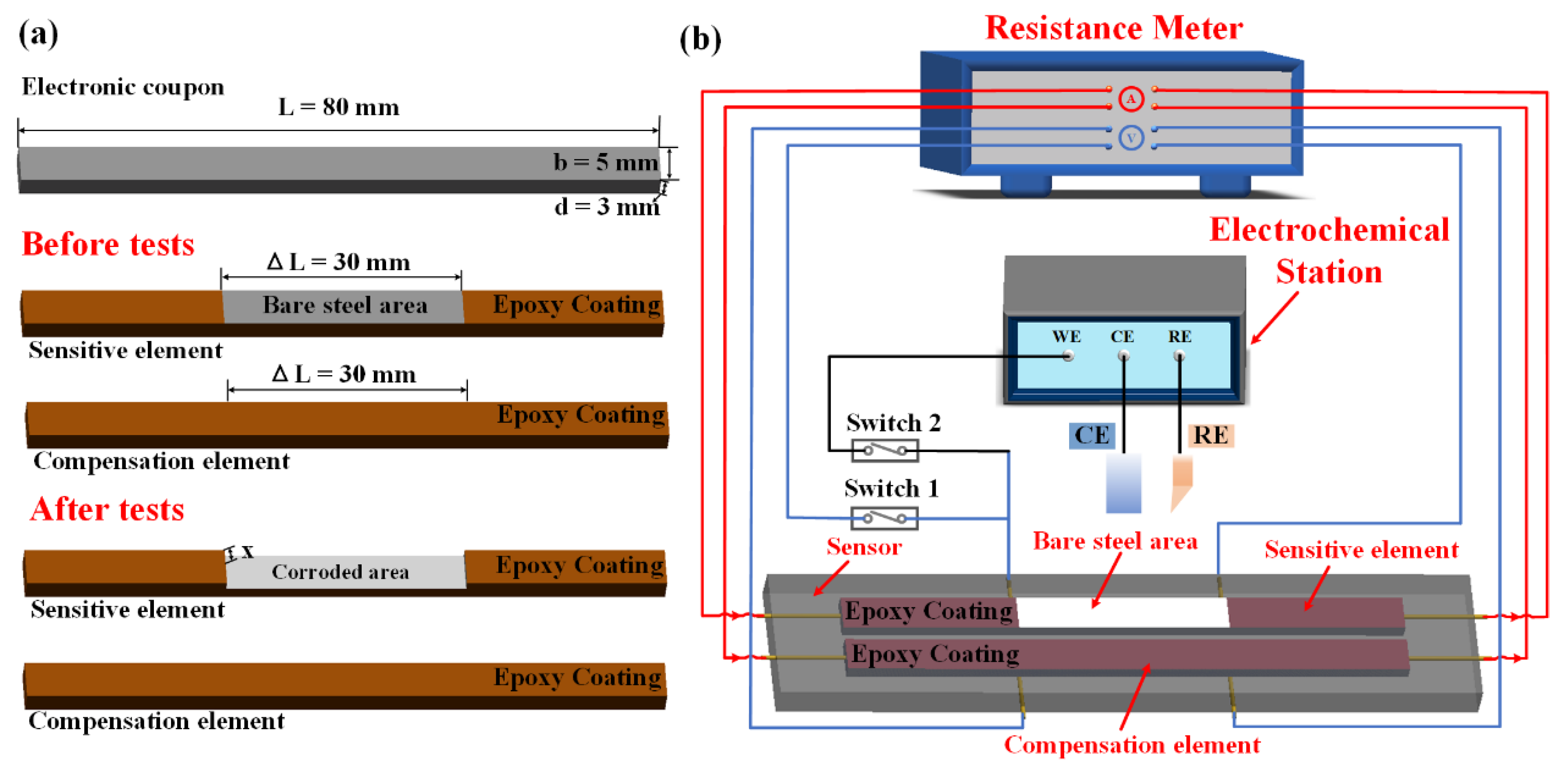
2.1. The Sensor System and Measurement Principle
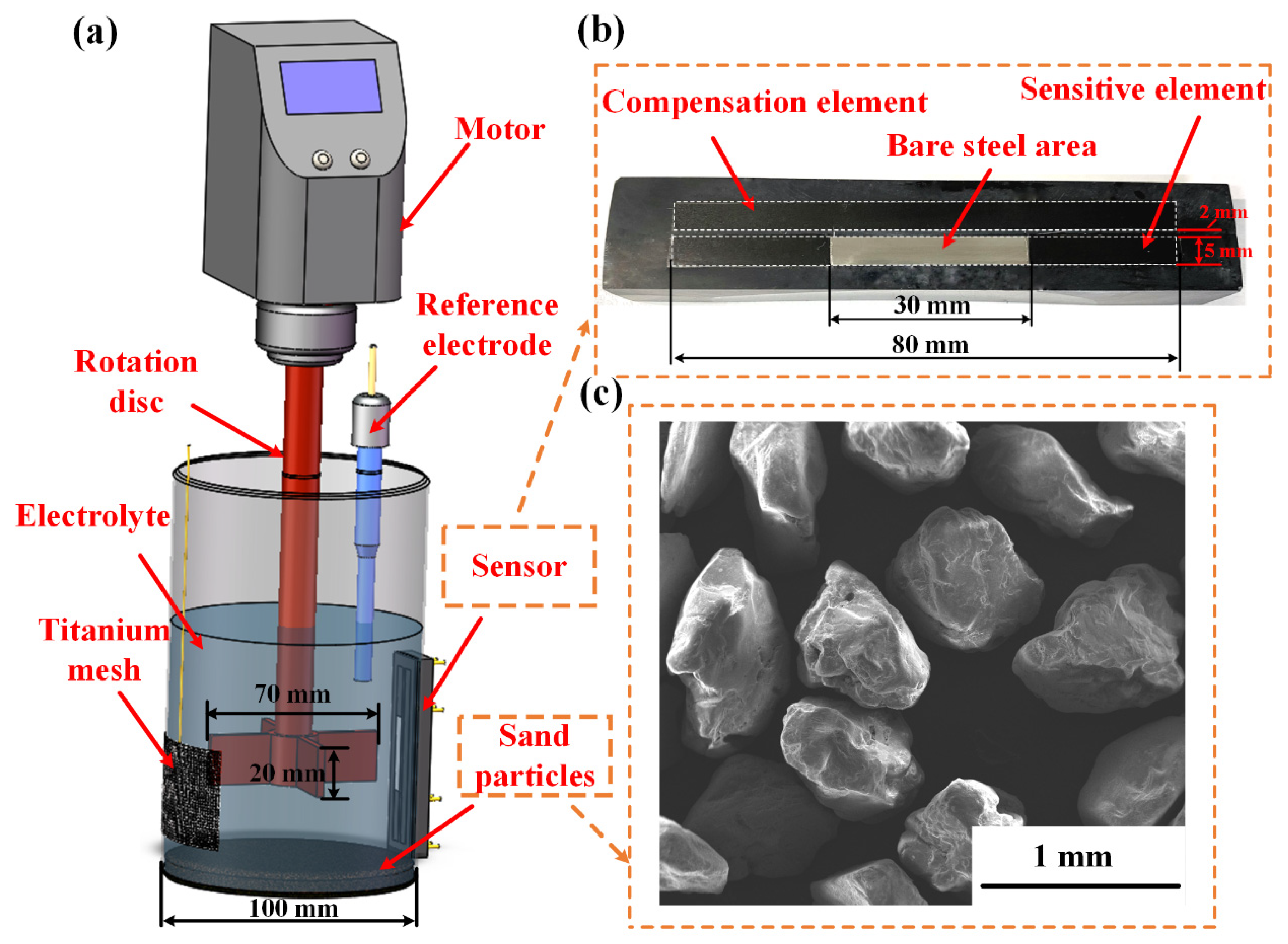
2.2. Test Setup and Materials
2.3. Test Procedure
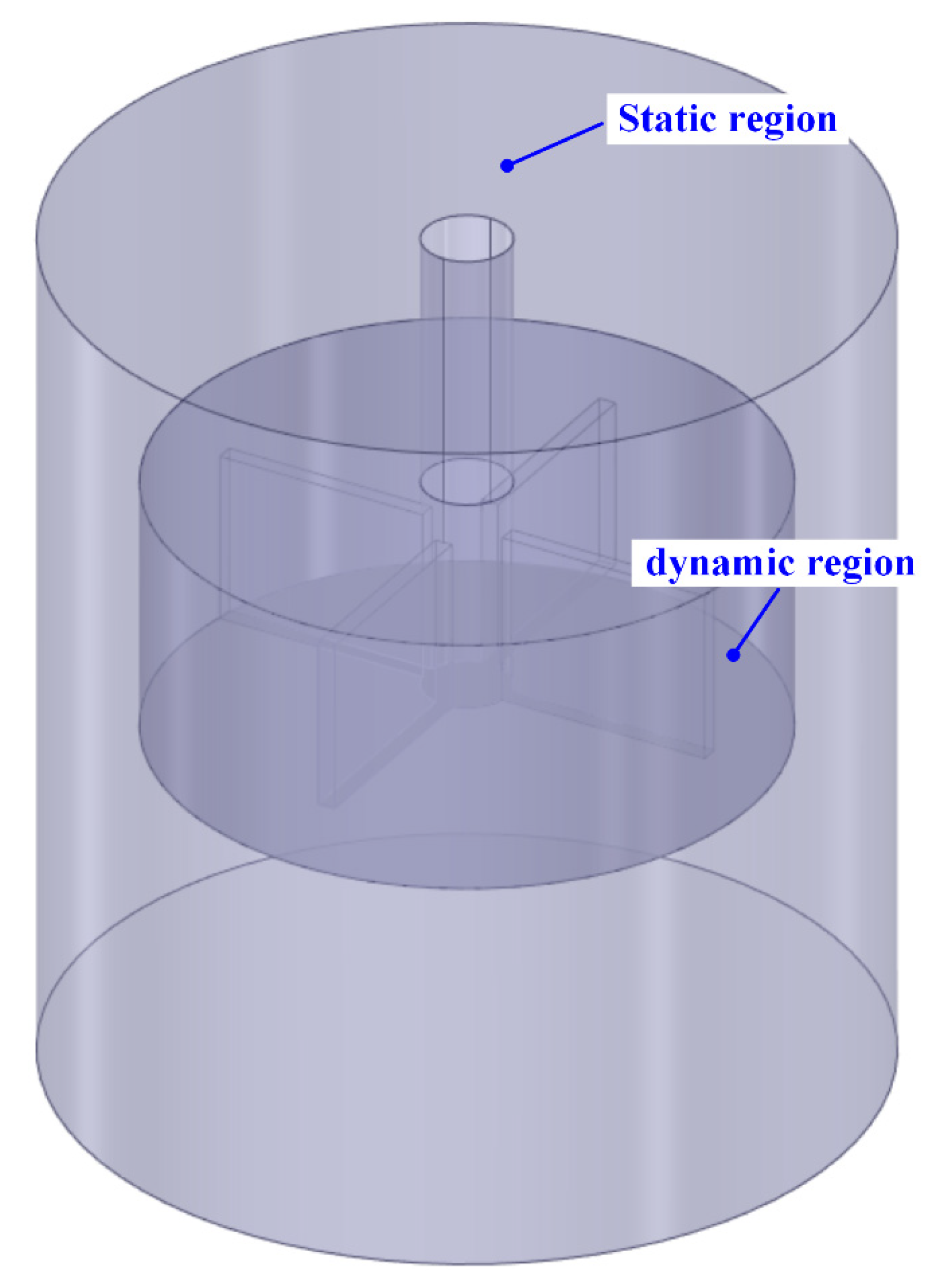
2.4. CFD Simulation
3. Results
3.1. CFD Simulation Results
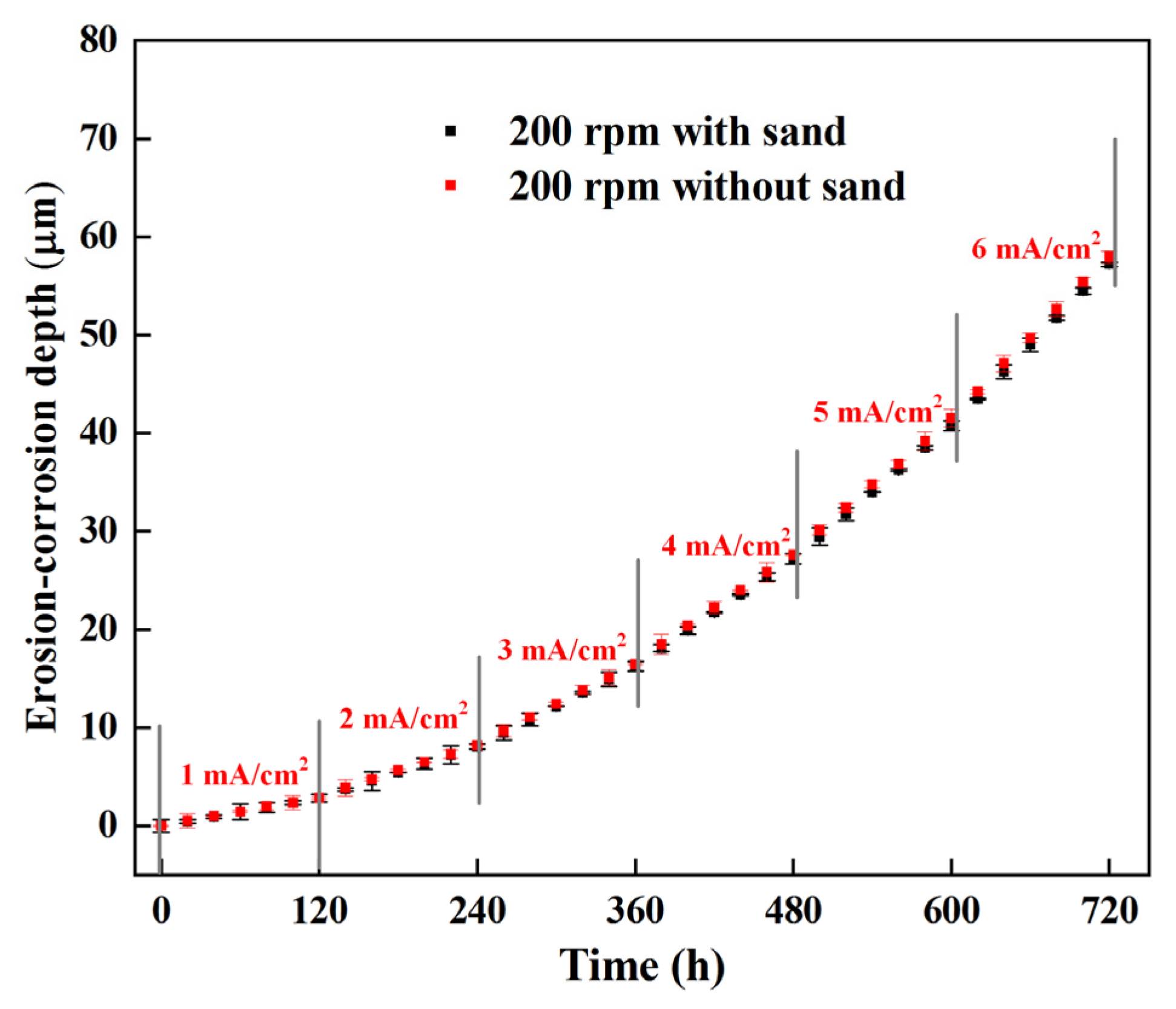
3.2. The Erosion–Corrosion Depth and Total Metal Loss Rate Measured by ER Method
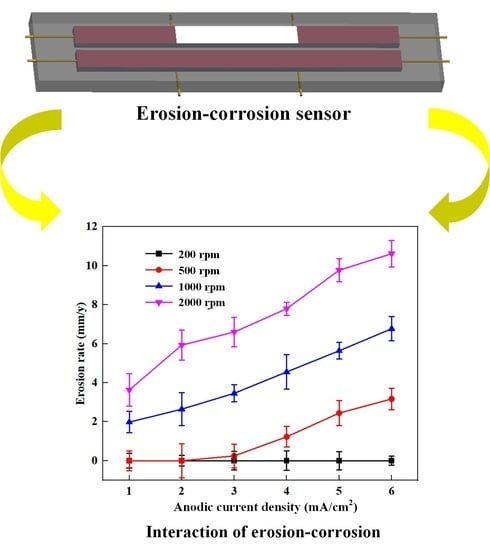
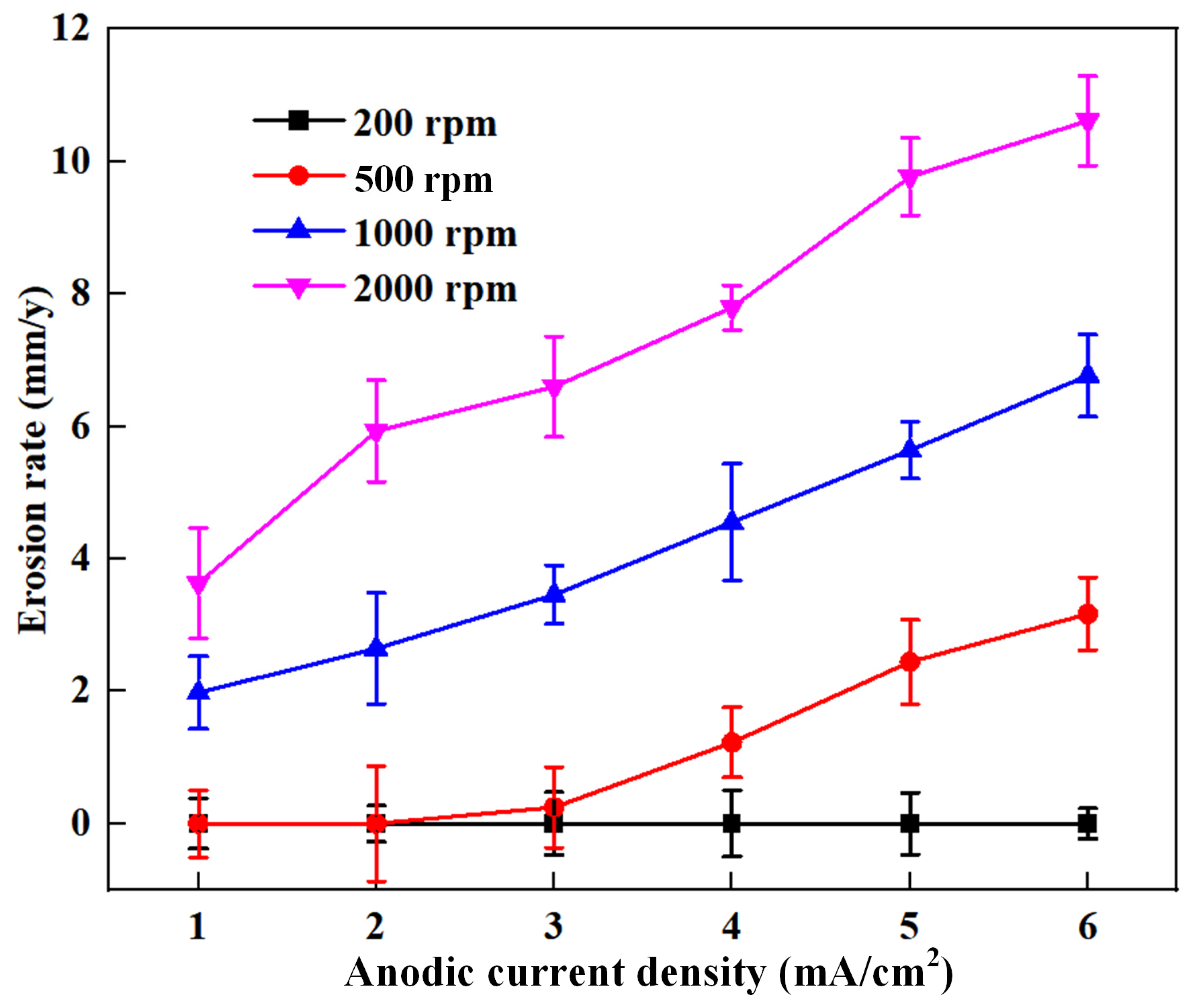
3.3. Dynamically Changed Erosion and Corrosion Rates Measured by the Erosion–Corrosion Sensor
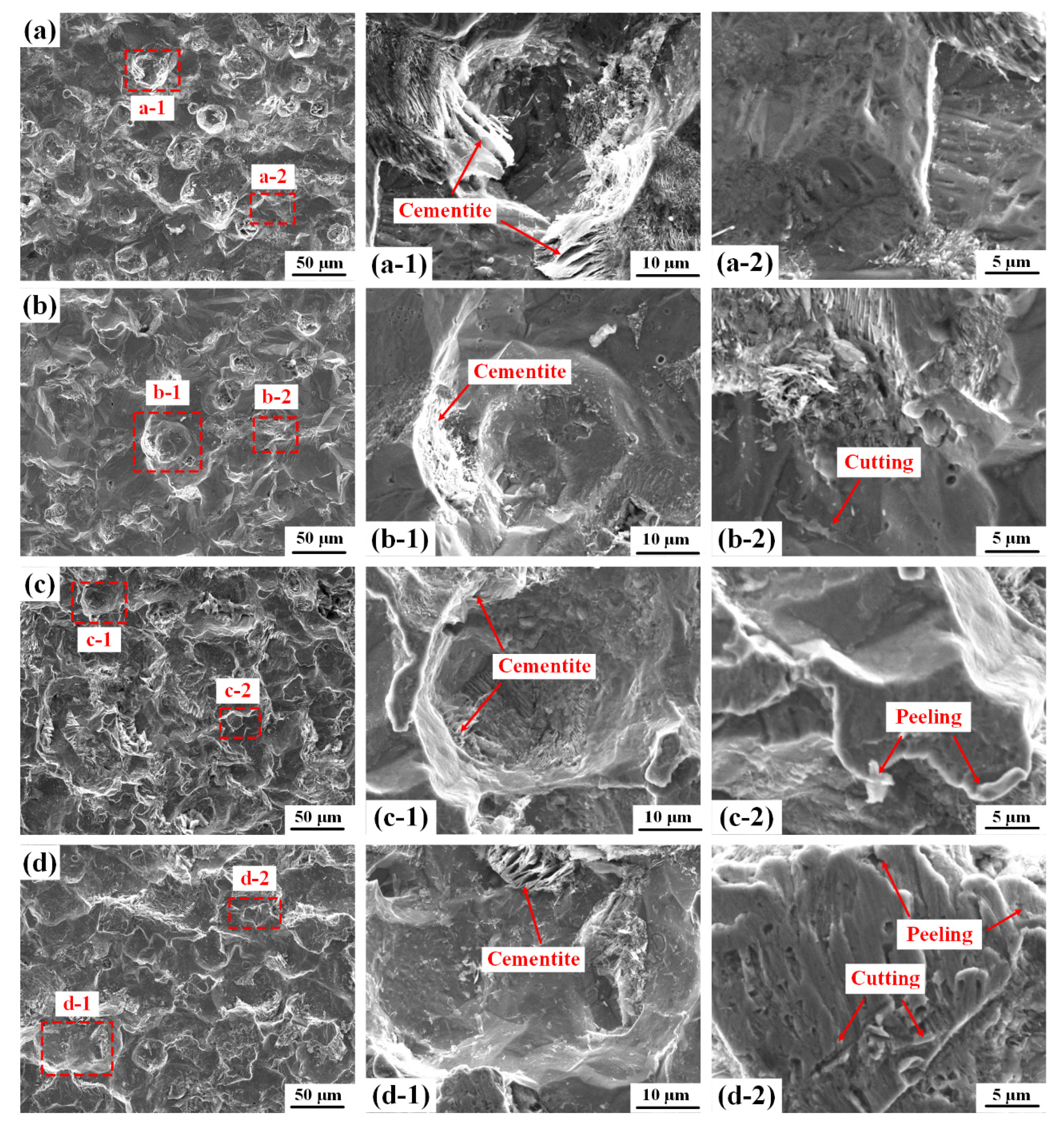
3.4. The Surface Morphologies under Different Test Conditions
4. Discussion
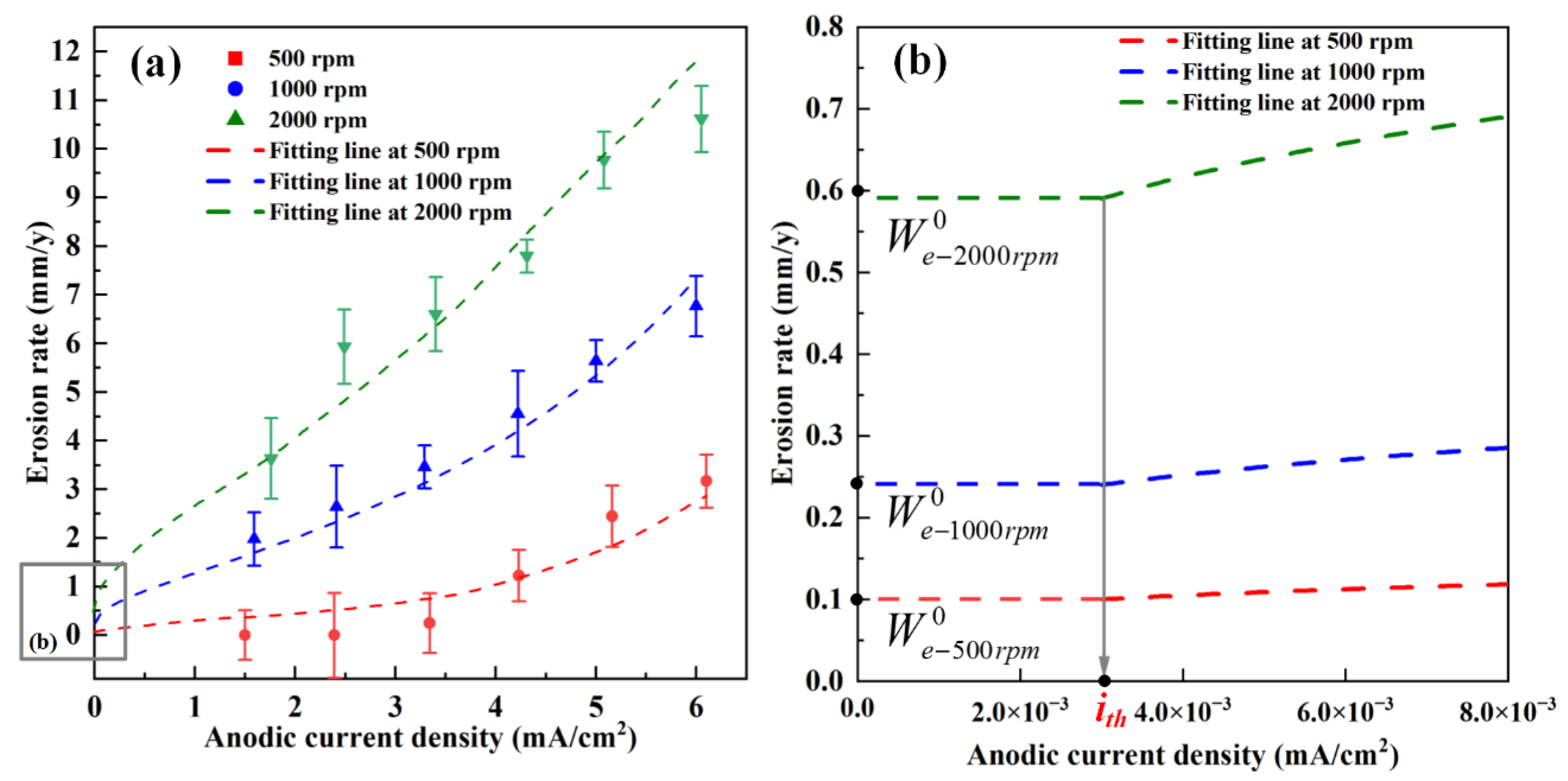
4.1. The Critical Impact Energy Required to Induce Initiation of Erosion
4.2. Interaction of Mechanical and Electrochemical Factors in Erosion–Corrosion of X65 Steel
5. Conclusions
- The combination of microelectrical resistance measurement and anodic polarization tests is a highly effective approach to study the interactive effects of mechanical and electrochemical factors on erosion–corrosion of X65 pipeline steel. The synchronously changed erosion and corrosion rates at various rotation speeds can be probed online using a small number of test samples.
- There is a critical impact energy at which particles begin to induce erosion damage on the surface of X65 pipeline steel in flowing slurry. When the kinetic energy of sand particles is higher than the critical impact energy, the impact of sand particles can remove the flaky cementite skeletons at the edge of the pits and cause the initiation of erosion.
- The threshold anodic current density that can induce a chemomechanical effect on erosion–corrosion of X65 steel can be quickly fitted based on the probing results of the erosion–corrosion sensor. The quantitative relationship between erosion and corrosion components, which can be used for erosion–corrosion simulation by numerical method, is established.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- El-Sherik, A. Trends in Oil and Gas Corrosion Research and Technologies; Woodhead Publishing: Sawston, UK, 2017. [Google Scholar]
- Xu, Y.; Liu, L.; Xu, C.; Wang, X.; Huang, Y. Electrochemical characteristics of the dynamic progression of erosion-corrosion under different flow conditions and their effects on corrosion rate calculation. J. Solid State Electrochem. 2020, 24, 2511–2524. [Google Scholar] [CrossRef]
- Xu, Y.; Liu, L.; Zhou, Q.; Wang, X.; Huang, Y. An Overview of Major Experimental Methods and Apparatus for Measuring and Investigating Erosion-Corrosion of Ferrous-Based Steels. Metals 2020, 10, 180. [Google Scholar] [CrossRef]
- Maher, M.; Iraola-Arregui, I.; Ben, Y.; Rhouta, B.; Trabadelo, V. The synergistic effect of wear-corrosion in stainless steels: A review. Mater. Today Proc. 2022, 51, 1975–1990. [Google Scholar] [CrossRef]
- Chung, R.J.; Jiang, J.; Pang, C.; Yu, B.; Eadie, R.; Li, D. Erosion-corrosion behaviour of steels used in slurry pipelines. Wear 2021, 477, 203771. [Google Scholar] [CrossRef]
- Liu, Y.; Zhao, Y.; Yao, J. Synergistic erosion–corrosion behavior of X80 pipeline steel at various impingement angles in two-phase flow impingement. Wear 2021, 466–467, 203572. [Google Scholar] [CrossRef]
- Lu, B.; Xia, D.; Luo, J. Mechanism of Corrosion-Enhanced Erosion of Steel in Oil and Gas Production; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar]
- Lu, B.; Luo, J. Correlation between surface-hardness degradation and erosion resistance of carbon steel-Effects of slurry chemistry. Tribol. Int. 2015, 83, 146–155. [Google Scholar] [CrossRef]
- Stack, M.; Stott, F.H. An approach to modeling erosion-corrosion of alloys using erosion-corrosion maps. Corros. Sci. 1993, 35, 1027–1034. [Google Scholar] [CrossRef]
- Malka, R.; Nešić, S.; Gulino, D. Erosion-corrosion and synergistic effects in disturbed liquid-particle flow. Wear 2007, 262, 791–799. [Google Scholar] [CrossRef]
- Guo, H.; Lu, B.; Luo, J. Interaction of mechanical and electrochemical factors in erosion-corrosion of carbon steel. Electrochim. Acta 2005, 51, 315–323. [Google Scholar] [CrossRef]
- Guo, H.; Lu, B.; Luo, J. Response of surface mechanical properties to electrochemical dissolution determined by in situ nano-indentation technique. Electrochem. Commun. 2006, 8, 1092–1098. [Google Scholar] [CrossRef]
- Liu, L.; Xu, Y.; Zhu, Y.; Wang, X.; Huang, Y. The Roles of Fluid Hydrodynamics, Mass Transfer, Rust Layer and Macro-Cell Current on Flow Accelerated Corrosion of Carbon Steel in Oxygen Containing Electrolyte. J. Electrochem. Soc. 2020, 167, 141510. [Google Scholar] [CrossRef]
- Fujiwara, K.; Domae, M.; Yoneda, K.; Inada, F.; Ohira, T.; Hisamune, K. Correlation of flow accelerated corrosion rate with iron solubility. Nucl. Eng. Des. 2011, 241, 4482–4486. [Google Scholar] [CrossRef]
- Uchida, S.; Naitoh, M.; Okada, H.; Uehara, Y.; Koshizuka, S. Evaluation of flow accelerated corrosion by coupled analysis of corrosion and flow dynamics. Relationship of oxide film thickness, hematite/magnetite ratio, ECP and wall thinning rate. Nucl. Eng. Des. 2011, 241, 4585–4593. [Google Scholar] [CrossRef]
- Liu, L.; Xu, Y.; Xu, C.; Wang, X.; Huang, Y. Detecting and monitoring erosion-corrosion using ring pair electrical resistance sensor in conjunction with electrochemical measurements. Wear 2019, 428–429, 328–339. [Google Scholar] [CrossRef]
- Zeng, L.; Zhang, G.; Guo, X. Erosion–corrosion at different locations of X65 carbon steel elbow. Corros. Sci. 2014, 85, 318–330. [Google Scholar] [CrossRef]
- Owen, J.; Ramsey, C.; Barker, R.; Neville, A. Erosion-corrosion interactions of X65 carbon steel in aqueous CO2 environments. Wear 2018, 414–415, 376–389.
- Wood, R. Erosion–corrosion interactions and their effect on marine and offshore materials. Wear 2006, 261, 1012–1023. [Google Scholar] [CrossRef]
- Barik, R.; Wharton, J.; Wood, R.; Stokes, K. Electro-mechanical interactions during erosion–corrosion. Wear 2009, 267, 1900–1908. [Google Scholar] [CrossRef]
- Zeng, L.; Shuang, S.; Guo, X.; Zhang, G. Erosion-corrosion of stainless steel at different locations of a 90° elbow. Corros. Sci. 2016, 111, 72–83. [Google Scholar] [CrossRef]
- Xu, Y.; Liu, L.; Zhou, Q.; Wang, X.; Huang, Y. Understanding the influences of pre-corrosion on the erosion-corrosion performance of pipeline steel. Wear 2020, 442–443, 203151. [Google Scholar] [CrossRef]
- Xu, Y.; Tan, M.Y. Probing the initiation and propagation processes of flow accelerated corrosion and erosion corrosion under simulated turbulent flow conditions. Corros. Sci. 2019, 151, 163–174. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, Q.; Gao, S.; Wang, X.; Huang, Y. Exploring the effects of sand impacts and anodic dissolution on localized erosion-corrosion in sand entraining electrolyte. Wear 2021, 478–479, 203907. [Google Scholar] [CrossRef]
- Guo, H.; Lu, B.; Luo, J. Non-Faraday material loss in flowing corrosive solution. Electrochim. Acta 2006, 51, 5341–5348. [Google Scholar] [CrossRef]
- Liu, L.; Xu, Y.; Wang, Z.; Wang, X.; Huang, Y. Probing and separating corrosion and erosion of pipeline steel using electrical resistance method in conjunction with electrochemical measurements. Measurement 2021, 183, 109797. [Google Scholar] [CrossRef]
- Vieira, R.; Parsi, M.; Zahedi, P.; McLaury, B.; Shirazi, S. Electrical resistance probe measurements of solid particle erosion in multiphase annular flow. Wear 2017, 382–383, 15–28. [Google Scholar] [CrossRef]
- Xu, Y.; Huang, Y.; Wang, X.; Lin, X. Experimental study on pipeline internal corrosion based on a new kind of electrical resistance sensor. Sens. Actuators B Chem. 2016, 224, 37–47. [Google Scholar] [CrossRef]
- Orlikowski, J.; Darowicki, K.; Mikołajski, S. Multi-sensor monitoring of the corrosion rate and the assessment of the efficiency of a corrosion inhibitor in utility water installations. Sens. Actuators B Chem. 2013, 181, 22–28. [Google Scholar] [CrossRef]
- Xia, D.; Deng, C.; Macdnald, D.; Jamali, S.; Mills, D.; Luo, J.L.; Strebl, M.G.; Amiri, M.; Jin, W.; Song, S.; et al. Electrochemical measurements used for assessment of corrosion and protection of metallic materials in the field: A critical review. J. Mater. Sci. Technol. 2022, 112, 151–183. [Google Scholar] [CrossRef]
- Zheng, Z.B.; Zheng, Y.G. Erosion-enhanced corrosion of stainless steel and carbon steel. Corros. Sci. 2016, 102, 259–268. [Google Scholar] [CrossRef]
- Maria, D.; Bruce, B.; Srdjan, N.; Marc, S. Effect of Flow and Steel Microstructure on the Formation of Iron Carbonate. Corros. J. Sci. Eng. 2019, 10, 75. [Google Scholar]
- Md. Aminul, I.; Zoheir, N. Mechanical and Electrochemical Synergism of API X42 Pipeline Steel during Erosion-Corrosion. J. Bio Tribo Corros. 2015, 1, 26. [Google Scholar] [CrossRef]
- Zhu, Y.; Xu, Y.; Wang, M.; Wang, X.; Liu, G.; Huang, Y. Understanding the influences of temperature and microstructure on localized corrosion of subsea pipeline weldment using an integrated multi-electrode array. Ocean. Eng. 2019, 189, 106351. [Google Scholar] [CrossRef]
- Farelas, F.; Galicia, M.; Brown, B.; Nesic, S.; Castaneda, H. Evolution of dissolution processes at the interface of carbon steel corroding in a CO2 environment studied by EIS. Corros. Sci. 2010, 52, 509–517. [Google Scholar] [CrossRef]

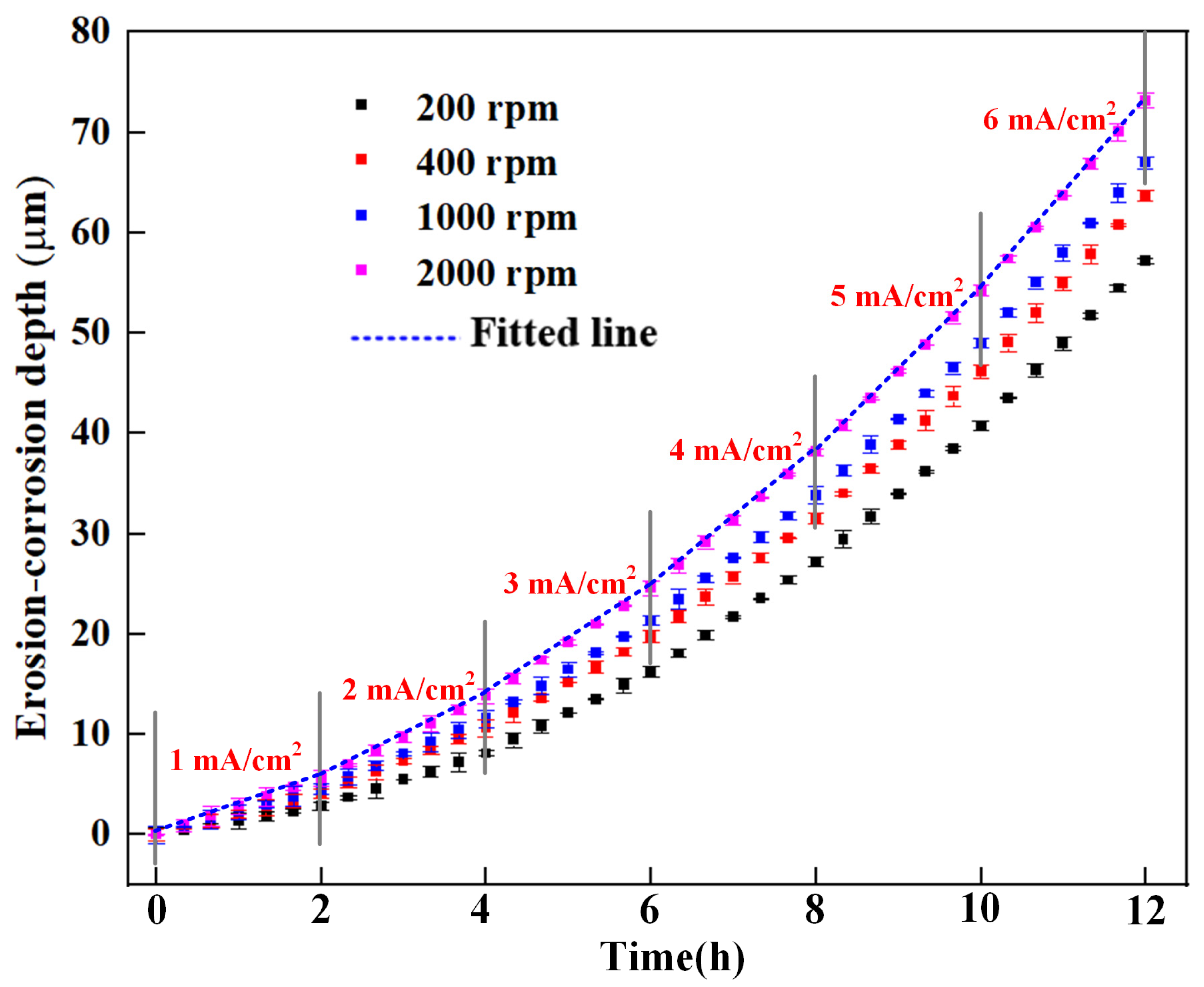
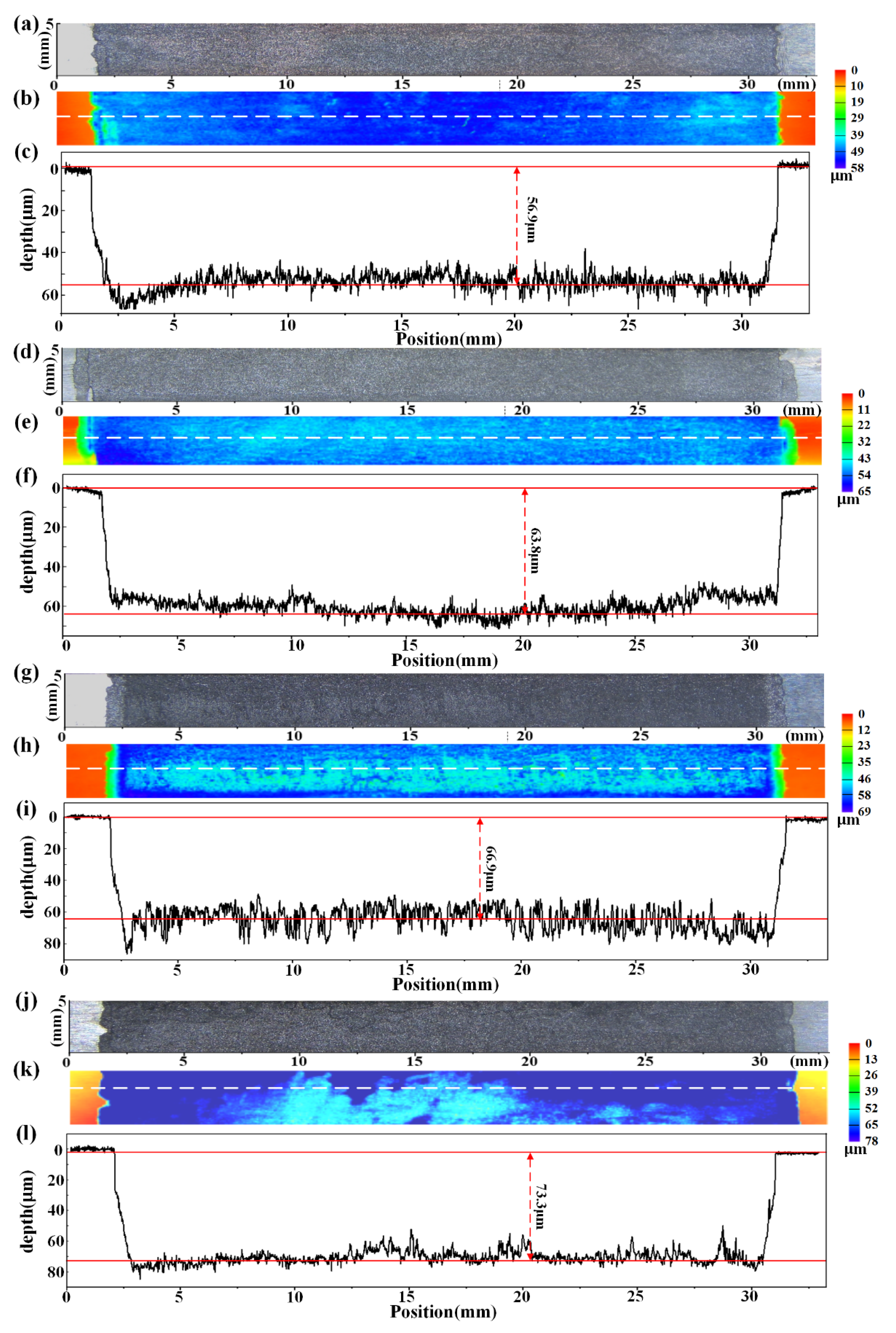
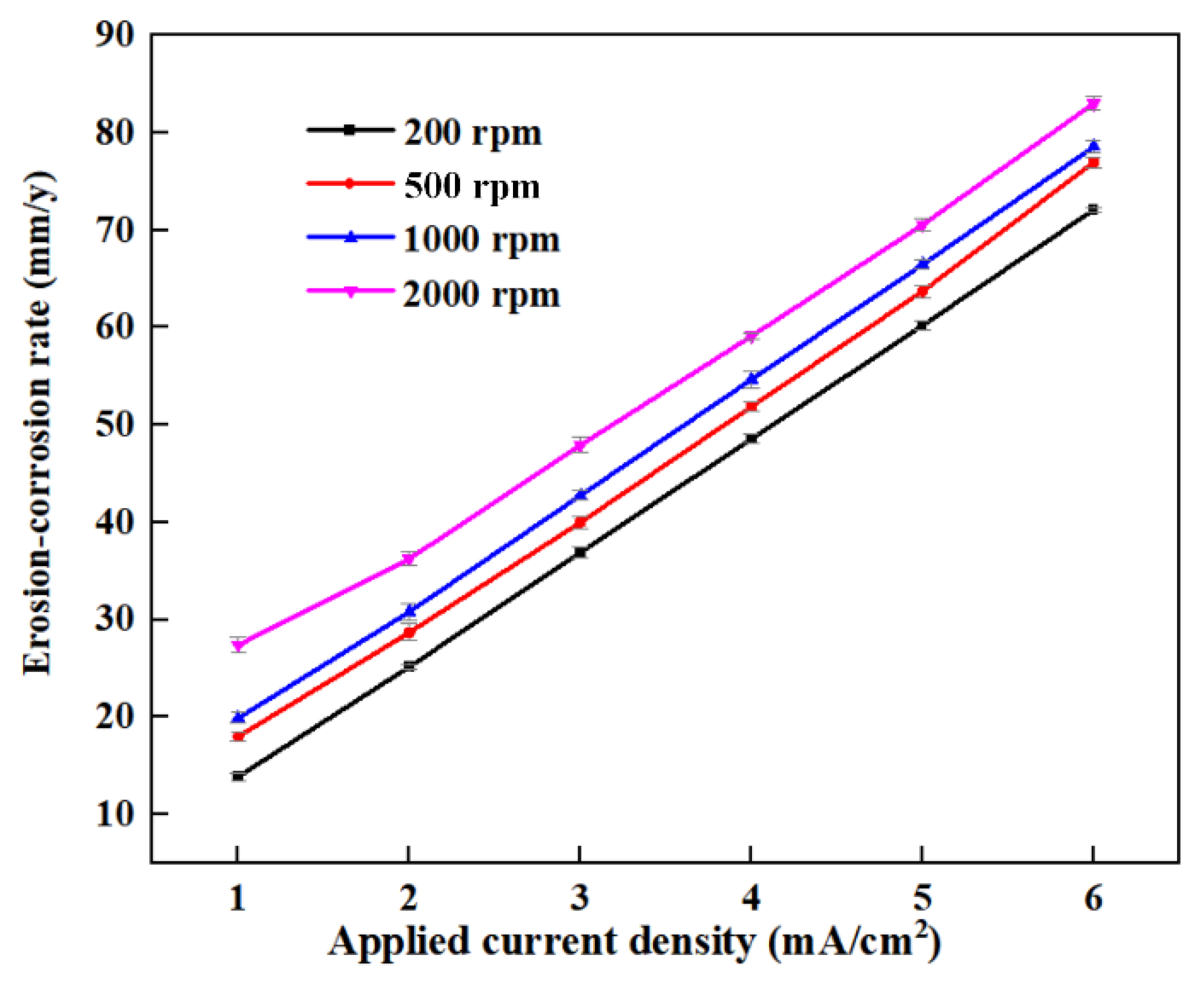
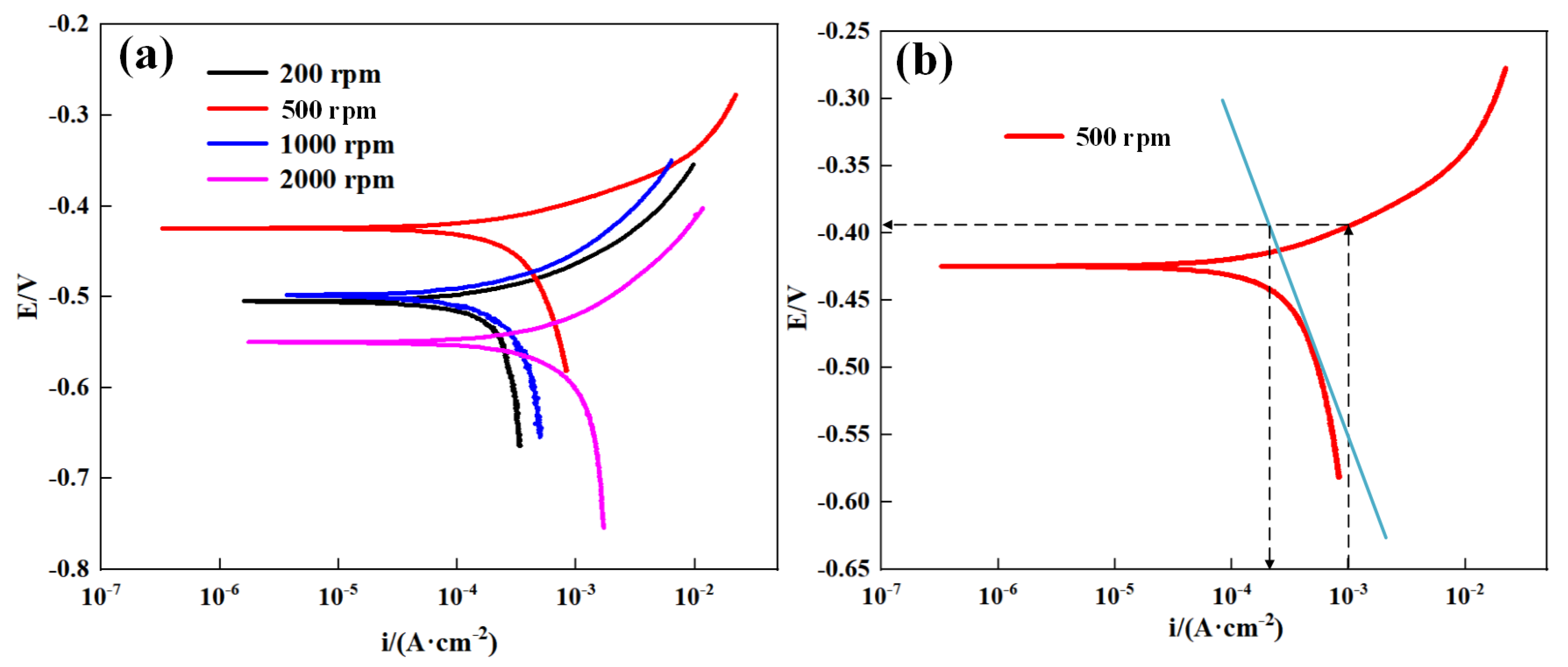
| Rotation Speed | Applied Current Density (mA/cm2) | ||||||
|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | ||
| ia (mA/cm2) | 200 rpm | 1.19 | 2.11 | 3.09 | 4.06 | 5.04 | 6.03 |
| 500 rpm | 1.50 | 2.39 | 3.34 | 4.23 | 5.16 | 6.10 | |
| 1000 rpm | 1.59 | 2.41 | 3.29 | 4.22 | 5.01 | 6.04 | |
| 2000 rpm | 1.76 | 2.49 | 3.40 | 4.31 | 5.08 | 6.05 | |
| (mm/y) | 200 rpm | 14.2 | 25.3 | 36.9 | 48.6 | 60.3 | 72.2 |
| 500 rpm | 17.9 | 28.6 | 39.9 | 50.6 | 61.8 | 73.0 | |
| 1000 rpm | 19.0 | 28.8 | 39.4 | 50.5 | 59.9 | 71.8 | |
| 2000 rpm | 21.1 | 29.8 | 40.7 | 51.6 | 60.8 | 72.4 | |
| Rotation Speed | ith (mA/cm2) | n | B | R2 |
|---|---|---|---|---|
| 500 rpm | 0.0033 | 1.25 | 0.13 | 0.83 |
| 1000 rpm | 0.0027 | 1.32 | 0.12 | 0.92 |
| 2000 rpm | 0.0032 | 1.31 | 0.12 | 0.93 |
| Average | 0.0031 | 1.29 | 0.12 | - |
| Data from the Literature [2] | Erosion Rate Predicted Using Equation (13) (mm/y) | ||
|---|---|---|---|
| Anodic Current Density (mA/cm2) | Pure Erosion Rate | Experimental Erosion Rate (mm/y) | |
| 0.34 | 0.12 | 1.0 ± 0.4 | 0.32 |
| 0.38 | 0.24 | 1.1 ± 0.3 | 0.73 |
| 0.43 | 0.41 | 1.4 ± 0.2 | 1.31 |
| 0.51 | 0.59 | 2.0 ± 0.2 | 2.01 |
| 0.68 | 0.91 | 3.8 ± 0.3 | 3.49 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, K.; Jiang, W.; Chen, W.; Liu, L.; Xu, Y.; Huang, Y. Probing the Dynamic Progression of Erosion–Corrosion of X65 Pipeline Steel Using the Electrical Resistance Method in Conjunction with Galvanostatic Polarization. Lubricants 2022, 10, 345. https://doi.org/10.3390/lubricants10120345
Liu K, Jiang W, Chen W, Liu L, Xu Y, Huang Y. Probing the Dynamic Progression of Erosion–Corrosion of X65 Pipeline Steel Using the Electrical Resistance Method in Conjunction with Galvanostatic Polarization. Lubricants. 2022; 10(12):345. https://doi.org/10.3390/lubricants10120345
Chicago/Turabian StyleLiu, Kongzhong, Wanheng Jiang, Wanbin Chen, Liang Liu, Yunze Xu, and Yi Huang. 2022. "Probing the Dynamic Progression of Erosion–Corrosion of X65 Pipeline Steel Using the Electrical Resistance Method in Conjunction with Galvanostatic Polarization" Lubricants 10, no. 12: 345. https://doi.org/10.3390/lubricants10120345
APA StyleLiu, K., Jiang, W., Chen, W., Liu, L., Xu, Y., & Huang, Y. (2022). Probing the Dynamic Progression of Erosion–Corrosion of X65 Pipeline Steel Using the Electrical Resistance Method in Conjunction with Galvanostatic Polarization. Lubricants, 10(12), 345. https://doi.org/10.3390/lubricants10120345