Understanding the Mechanism of Load-Carrying Capacity between Parallel Rough Surfaces through a Deterministic Mixed Lubrication Model
Abstract
:1. Introduction
2. Materials and Methods
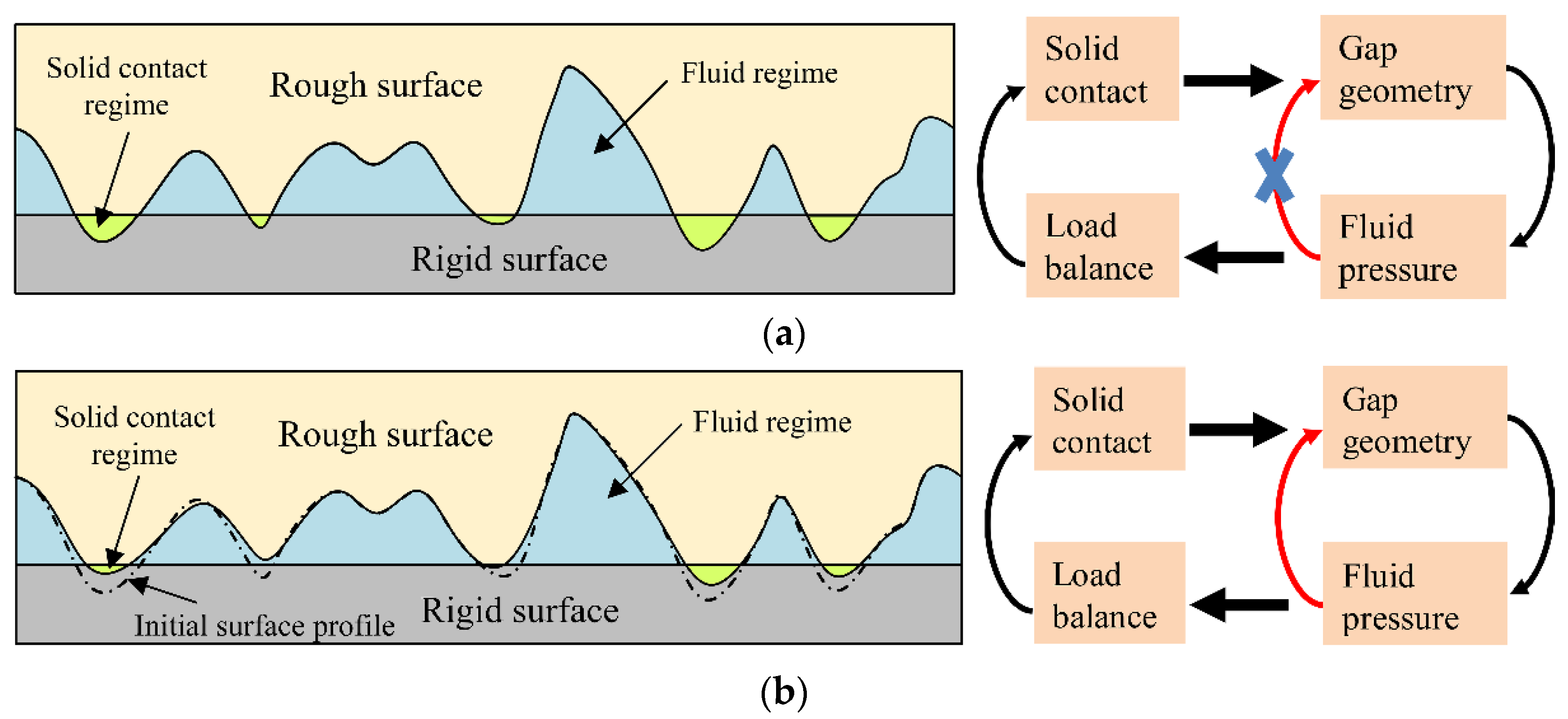
2.1. The Proposed Deterministic Model
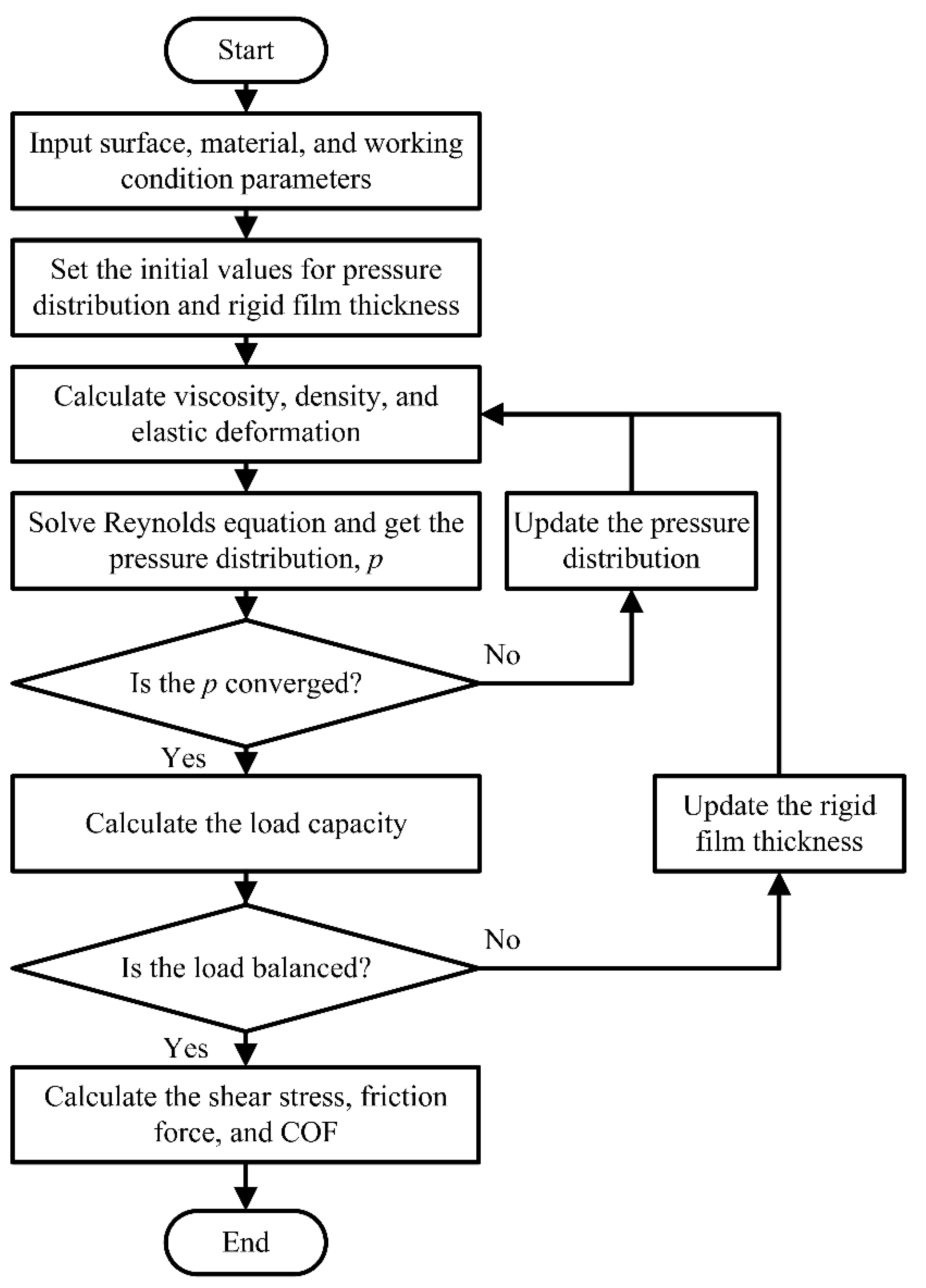
2.2. Numerical Methods
2.2.1. Obtaining the Initial Pressure Distribution
2.2.2. Solving Reynolds Equation with Film Thickness Approaching Zero
2.2.3. Updating the Rigid Film Thickness Value
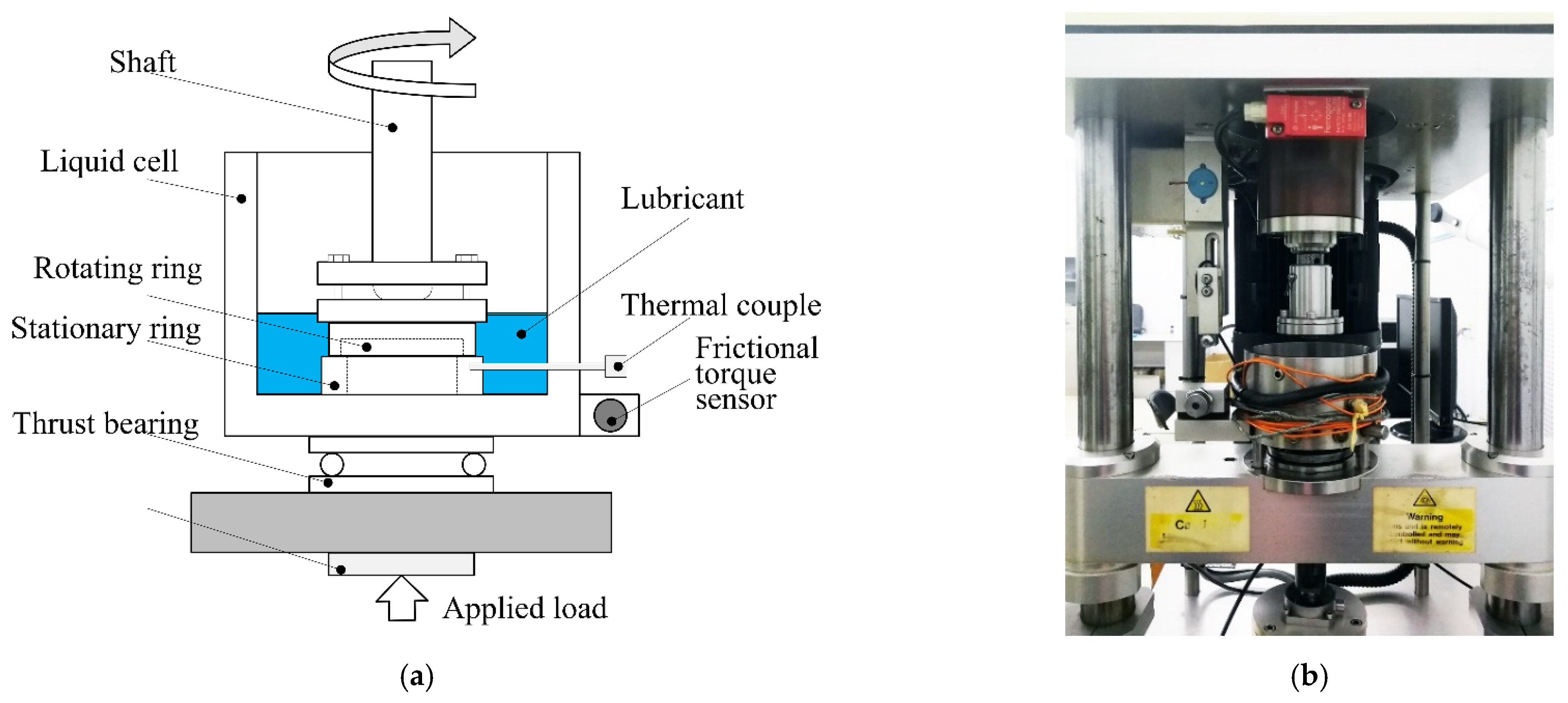
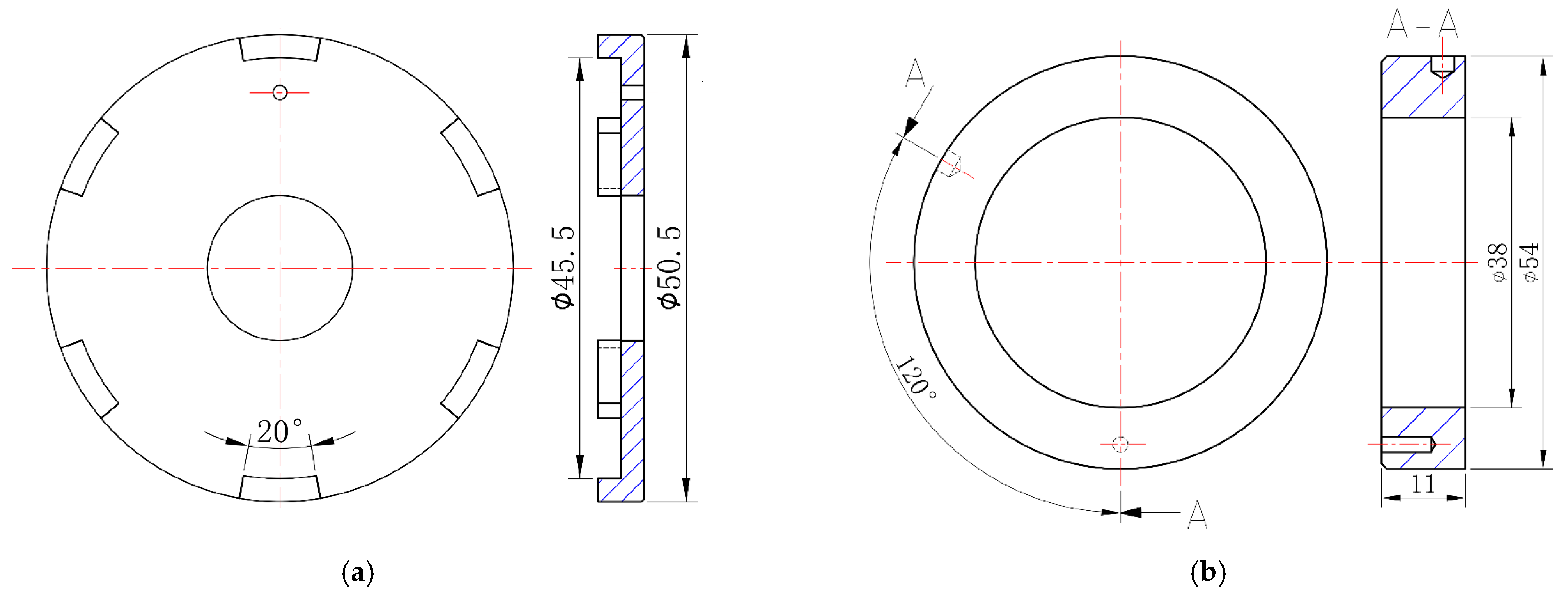
2.3. Experimental Details
2.3.1. Experimental Materials
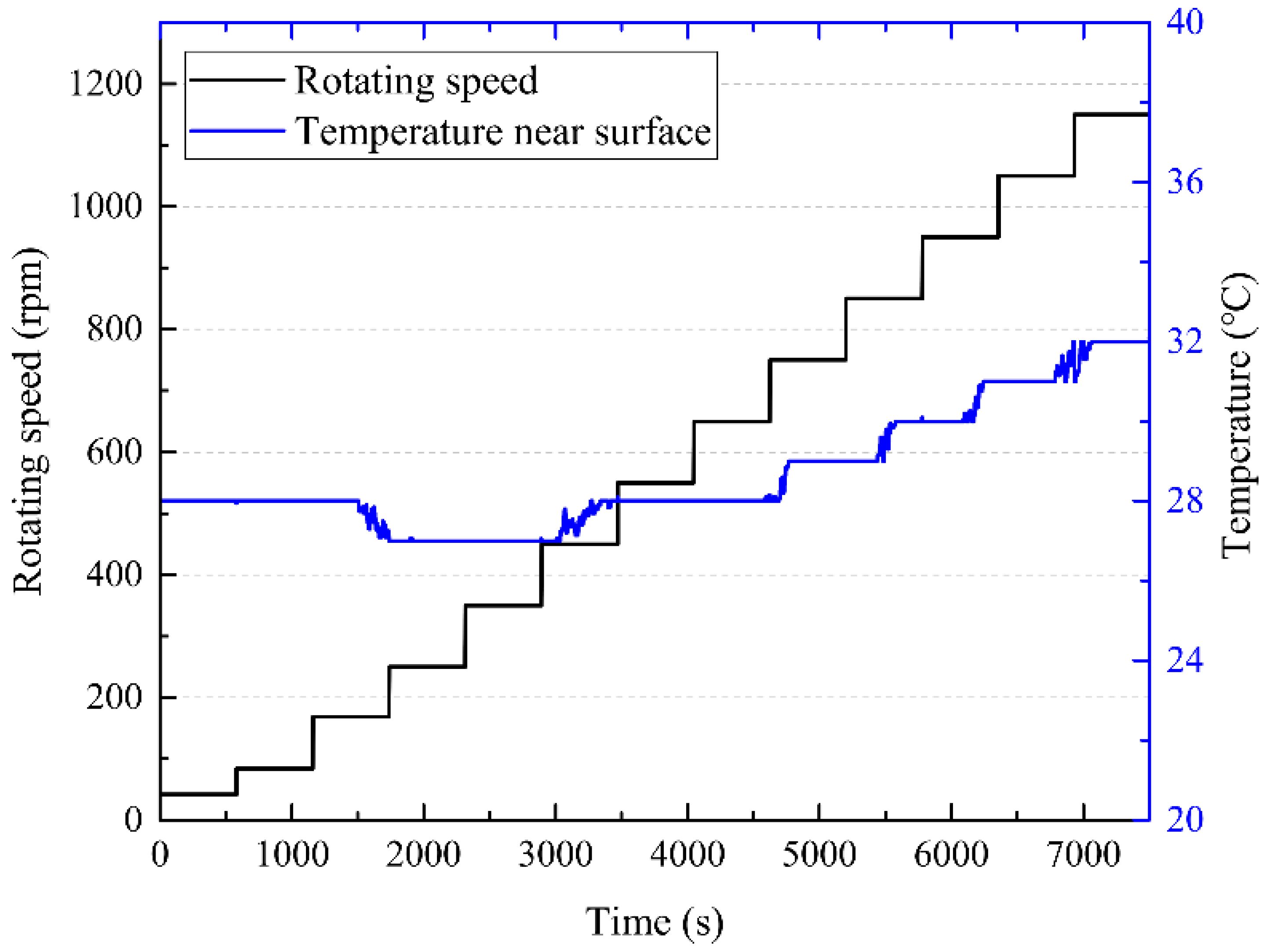
2.3.2. Tribological Test
3. Results and Discussion
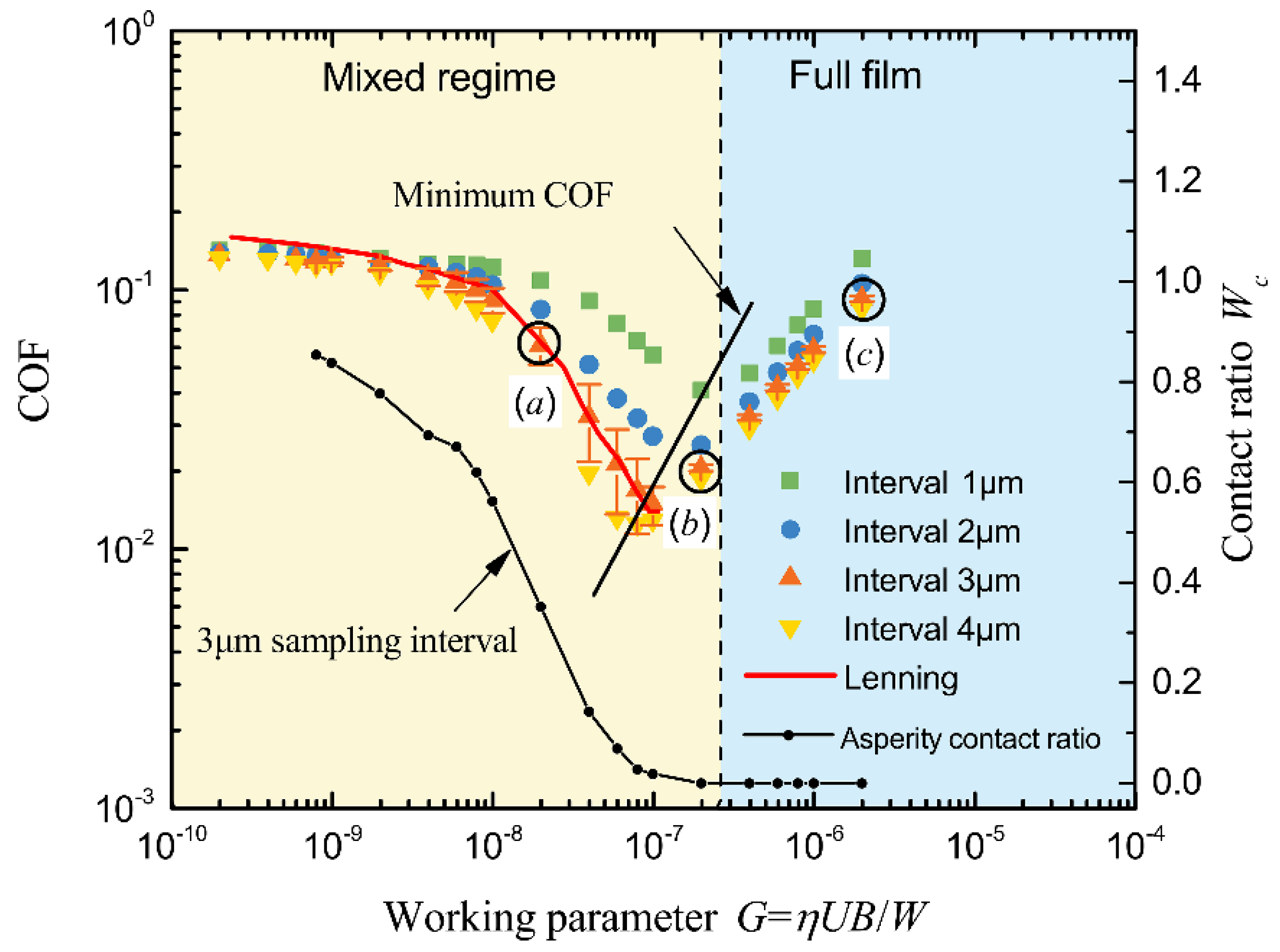
3.1. Verification Based on Lenning’s Results
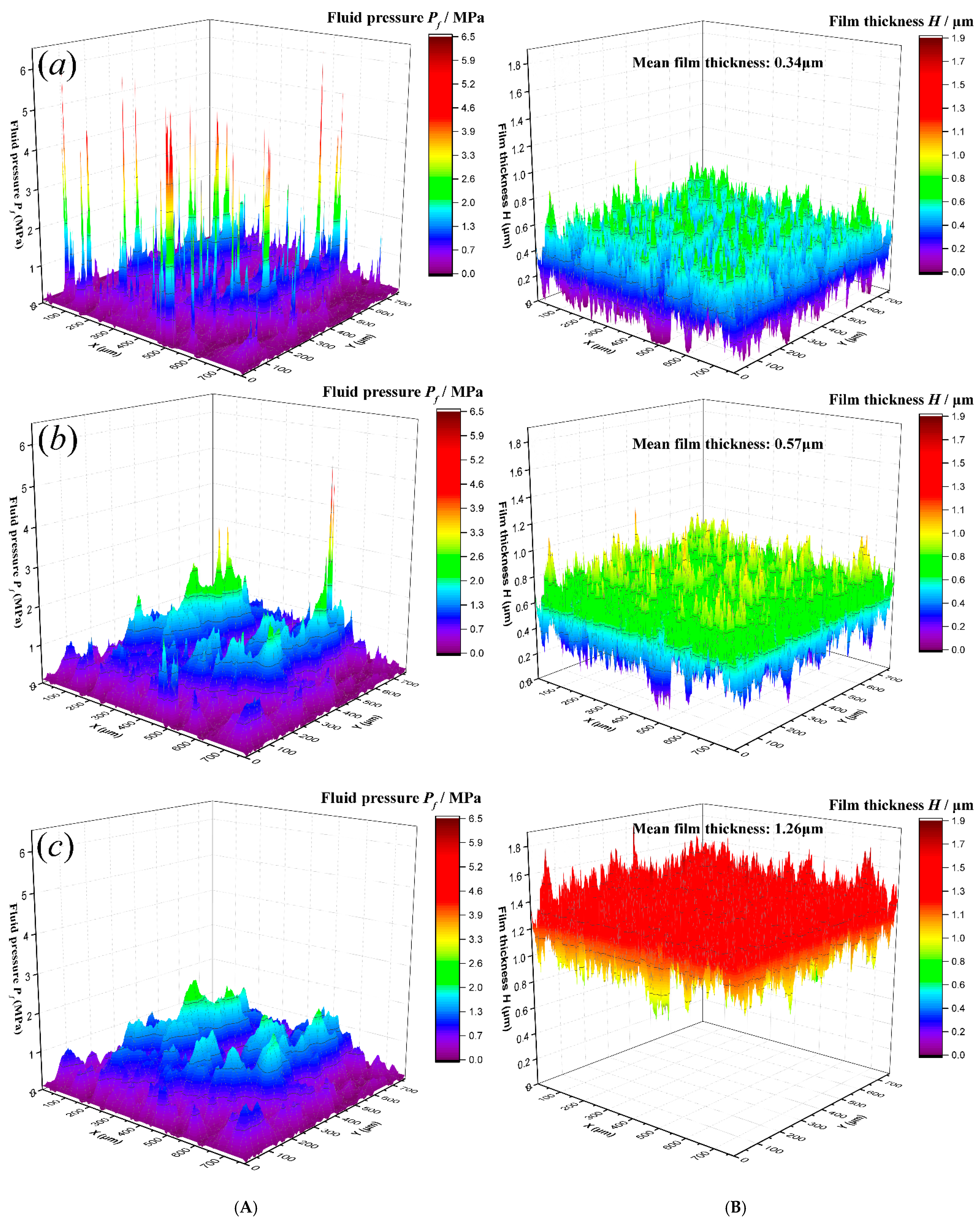
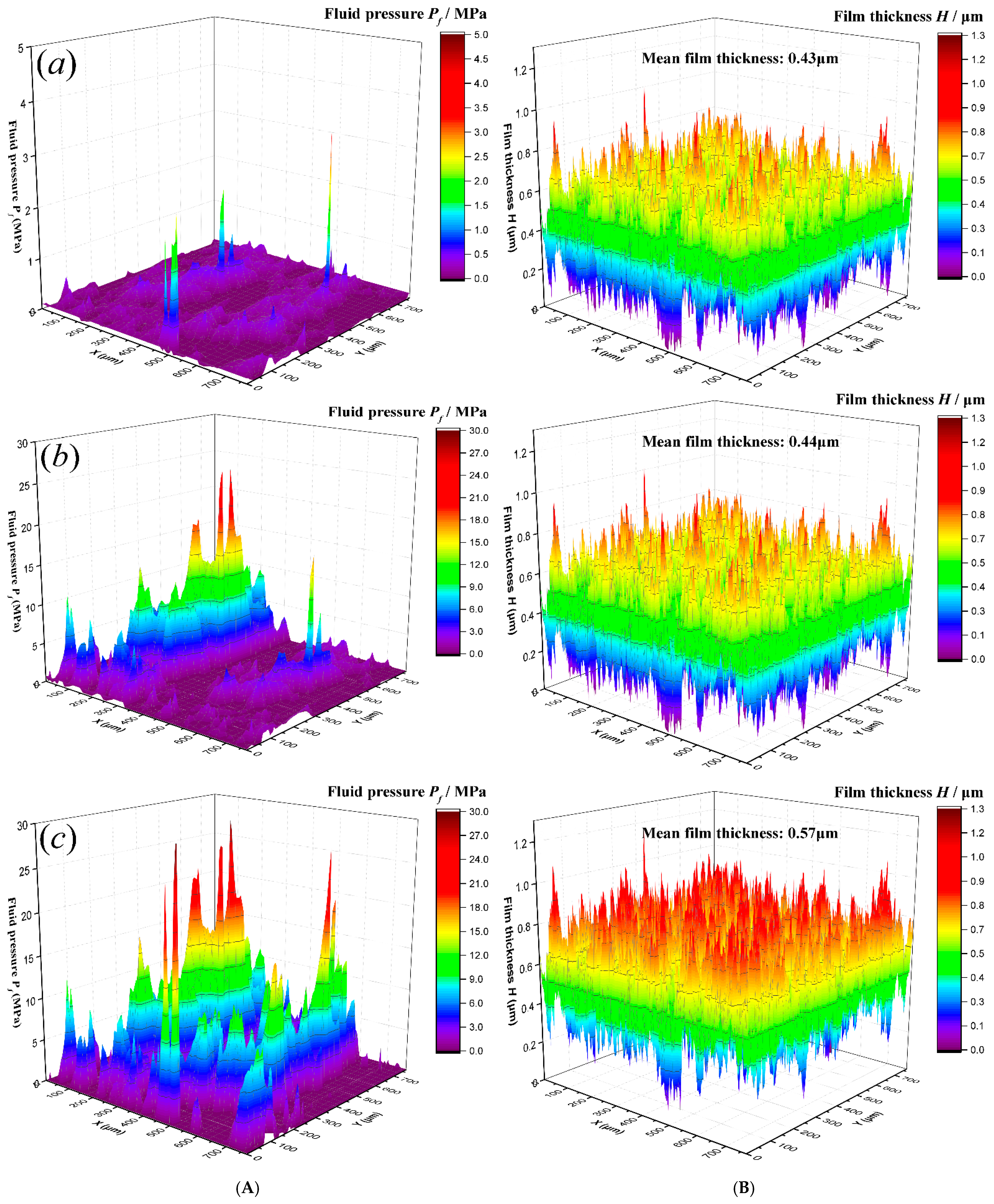
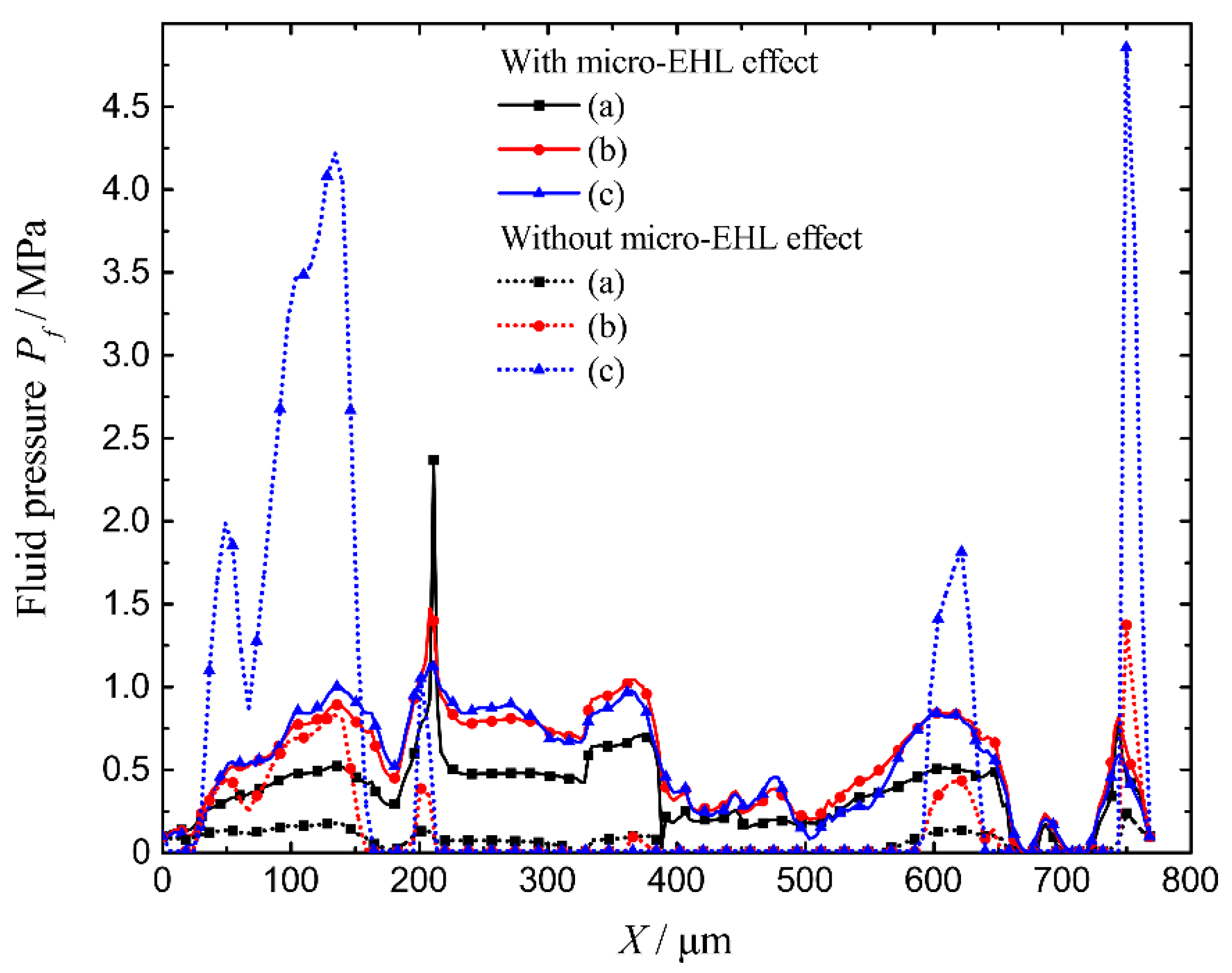
3.2. The Role of the Micro-EHL on Generating Load-Carrying Capacity
3.3. Experimental Results
4. Drawbacks and Outlooks
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| E′ | effective Young’s modulus |
| F | total friction force |
| Fh | friction force caused by fluid shear |
| Fc | friction force caused by asperity contact |
| f | coefficient of friction |
| fc | dry friction coefficient |
| G | duty parameter |
| h | film thickness |
| Kp, Ki, Kd | coefficients in PID controller |
| p | pressure |
| p0, pl, pc, ph | ambient pressure, lubricant pressure, contact pressure, fluid pressure |
| Sq | root-mean-square roughness |
| Sal | autocorrelation length of roughness |
| Ssk | skewness of roughness |
| Sku | kurtosis of roughness |
| Sdq | root mean square gradient of roughness |
| U | entrainment velocity |
| v | elastic deformation |
| w | load |
| wc | load shared by asperity contact |
| x | x-coordinate |
| y | y-coordinate |
| x, xe | start and end coordinates of solution domain in the x-direction |
| y0, ye | start and end coordinates of solution domain in the y-direction |
| α | pressure-viscosity exponent |
| δ1 | roughness data |
| η | viscosity |
| η0 | viscosity under ambient condition |
| ρ | density |
| ρ0 | density under ambient condition |
| τ | shearing stress |
| τL | limiting shear stress |
| τL0 | initial shear stress of lubricant |
| shearing rate | |
| γL | pressure coefficient |
References
- Reynolds, O., IV. On the theory of lubrication and its application to Mr. Beauchamp tower’s experiments, including an experimental determination of the viscosity of olive oil. Philos. Trans. R. Soc. Lond. 1886, 177, 157–234. [Google Scholar] [CrossRef]
- Fogg, A. Fluid Film Lubrication of Parallel Thrust Surfaces. Proc. Inst. Mech. Eng. 1946, 155, 49–67. [Google Scholar] [CrossRef]
- Lebeck, A.O. Parallel Sliding Load Support in the Mixed Friction Regime. Part 1—The Experimental Data. J. Tribol. 1987, 109, 189–195. [Google Scholar] [CrossRef]
- Ayadi, K.; Brunetiere, N.; Tournerie, B.; Maoui, A. Experimental and numerical study of the lubrication regimes of a liquid mechanical seal. Tribol. Int. 2015, 92, 96–108. [Google Scholar] [CrossRef]
- Denny, D. Some measurements of fluid pressures between plane parallel thrust surfaces with special reference to radial-face seals. Wear 1961, 4, 64–83. [Google Scholar] [CrossRef]
- Pape, J.G. Fundamental Research on a Radial Face Seal. A S L E Trans. 1968, 11, 302–309. [Google Scholar] [CrossRef]
- So, H.; Chen, C. Effects of Micro-Wedges Formed Between Parallel Surfaces on Mixed Lubrication—Part I: Experimental Evidence. Tribol. Lett. 2004, 17, 513–520. [Google Scholar] [CrossRef]
- Lebeck, A.O. Parallel Sliding Load Support in the Mixed Friction Regime. Part 2—Evaluation of the Mechanisms. J. Tribol. 1987, 109, 196–205. [Google Scholar] [CrossRef]
- Cameron, A.; Wood, W.L. Parallel Surface Thrust Bearing. A S L E Trans. 1958, 1, 254–258. [Google Scholar] [CrossRef]
- Dobry, A. The transitions between boundary, mixed and hydrodynamic lubrication. Wear 1964, 7, 290–297. [Google Scholar] [CrossRef]
- Fowles, P.E. The Application of Elastohydrodynamic Lubrication Theory to Individual Asperity-Asperity Collisions. J. Lubr. Technol. 1969, 91, 464–475. [Google Scholar] [CrossRef]
- Kuzma, D.C. Fluid inertia effects in squeeze films. Flow Turbul. Combust. 1968, 18, 15–20. [Google Scholar] [CrossRef]
- Lebeck, A.O. Mixed lubrication in mechanical face seals with plain faces. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 1999, 213, 163–175. [Google Scholar] [CrossRef]
- Stanghan-Batch, B.; Iny, E.H. A Hydrodynamic Theory of Radial-Face Mechanical Seals. J. Mech. Eng. Sci. 1973, 15, 17–24. [Google Scholar] [CrossRef]
- So, H.; Chen, C. Effects of micro-wedges formed between parallel surfaces on mixed lubrication–Part II: Modeling. Tribol. Lett. 2005, 19, 83–91. [Google Scholar] [CrossRef]
- Brunetière, N.; Francisco, A. Lubrication Mechanisms Between Parallel Rough Surfaces. Tribol. Lett. 2019, 67, 116. [Google Scholar] [CrossRef] [Green Version]
- Minet, C.; Brunetière, N.; Tournerie, B. A Deterministic Mixed Lubrication Model for Mechanical Seals. J. Tribol. 2011, 133, 042203. [Google Scholar] [CrossRef]
- Greenwood, J.; Wu, J. Surface Roughness and Contact: An Apology. Meccanica 2001, 36, 617–630. [Google Scholar] [CrossRef]
- Lorenz, B.; Carbone, G.; Schulze, C. Average separation between a rough surface and a rubber block: Comparison between theories and experiments. Wear 2010, 268, 984–990. [Google Scholar] [CrossRef]
- Ciavarella, M.; Greenwood, J.; Paggi, M. Inclusion of “interaction” in the Greenwood and Williamson contact theory. Wear 2008, 265, 729–734. [Google Scholar] [CrossRef]
- Yastrebov, V.; Durand, J.; Proudhon, H.; Cailletaud, G. Rough surface contact analysis by means of the Finite Element Method and of a new reduced model. Comptes Rendus Mécanique 2011, 339, 473–490. [Google Scholar] [CrossRef]
- Yastrebov, V.; Anciaux, G.; Molinari, J.-F. From infinitesimal to full contact between rough surfaces: Evolution of the contact area. Int. J. Solids Struct. 2015, 52, 83–102. [Google Scholar] [CrossRef]
- Waddad, Y.; Magnier, V.; Dufrénoy, P.; De Saxcé, G. A multiscale method for frictionless contact mechanics of rough surfaces. Tribol. Int. 2016, 96, 109–121. [Google Scholar] [CrossRef]
- Fowles, P.E. Extension of the Elastohydrodynamic Theory of Individual Asperity-Asperity Collisions to the Second Half of the Collision. J. Lubr. Technol. 1971, 93, 213–215. [Google Scholar] [CrossRef]
- Fowles, P.E. A Thermal Elastohydrodynamic Theory for Individual Asperity-Asperity Collisions. J. Lubr. Technol. 1971, 93, 383–394. [Google Scholar] [CrossRef]
- Fowles, P.E. The Statistical Application of a Thermal EHL Theory for Individual Asperity-Asperity Collisions to the Sliding Contact of Rough Surfaces. J. Lubr. Technol. 1975, 97, 311–318. [Google Scholar] [CrossRef]
- Ai, X.; Cheng, H.S. A Transient EHL Analysis for Line Contacts With Measured Surface Roughness Using Multigrid Technique. J. Tribol. 1994, 116, 549–556. [Google Scholar] [CrossRef]
- Ai, X.; Cheng, H.S. The Effects of Surface Texture on EHL Point Contacts. J. Tribol. 1996, 118, 59–66. [Google Scholar] [CrossRef]
- Venner, C.H.; Lubrecht, A.A. Transient Analysis of Surface Features in an EHL Line Contact in the Case of Sliding. J. Tribol. 1994, 116, 186–193. [Google Scholar] [CrossRef]
- Venner, C.H.; Lubrecht, A.A. Numerical Simulation of a Transverse Ridge in a Circular EHL Contact under Rolling/Sliding. J. Tribol. 1994, 116, 751–761. [Google Scholar] [CrossRef]
- Venner, C.; Lubrecht, A. Numerical Simulation of Waviness in A Circular Ehl Contact, under Rolling/Sliding. Vehicle Tribol. 1995, 30, 259–272. [Google Scholar]
- Venner, C.H.; Lubrecht, A.A. Numerical Analysis of the Influence of Waviness on the Film Thickness of a Circular EHL Contact. J. Tribol. 1996, 118, 153–161. [Google Scholar] [CrossRef]
- Chang, L.; Cusano, C.; Conry, T.F. Effects of Lubricant Rheology and Kinematic Conditions on Micro-Elastohydrodynamic Lubrication. J. Tribol. 1989, 111, 344–351. [Google Scholar] [CrossRef]
- Lee, R.-T.; Hamrock, B.J. A Circular Non-Newtonian Fluid Model: Part II—Used in Microelastohydrodynamic Lubrication. J. Tribol. 1990, 112, 497–505. [Google Scholar] [CrossRef]
- Kweh, C.C.; Patching, M.J.; Evans, H.P.; Snidle, R.W. Simulation of Elastohydrodynamic Contacts Between Rough Surfaces. J. Tribol. 1992, 114, 412–419. [Google Scholar] [CrossRef]
- Chang, L.; Jackson, A.; Webster, M.N. Effects of 3-D Surface Topography on the EHL Film Thickness and Film Breakdown. Tribol. Trans. 1994, 37, 435–444. [Google Scholar] [CrossRef]
- Chang, L. Deterministic modeling and numerical-simulation of lubrication between rough surfaces—A review of recent developments. Wear 1995, 184, 155–160. [Google Scholar] [CrossRef]
- Chang, L. A deterministic model for line-contact partial elastohydrodynamic lubrication. Tribol. Int. 1995, 28, 75–84. [Google Scholar] [CrossRef]
- Jiang, X.; Hua, D.Y.; Cheng, H.S.; Ai, X.; Lee, S.C. A Mixed Elastohydrodynamic Lubrication Model With Asperity Contact. J. Tribol. 1999, 121, 481–491. [Google Scholar] [CrossRef]
- Zhu, D.; Hu, Y.-Z. The Study of Transition from Elastohydrodynamic to Mixed and Boundary Lubrication. The Advancing Frontier of Engineering Tribology, Proceedings of the 1999 STLE/ASME H.S. Cheng Tribology Surveillance. 1999, pp. 150–156. Available online: https://www.researchgate.net/profile/Dong-Zhu-7/publication/283921871_The_study_of_transition_from_full_film_elastohydrodynamic_to_mixed_and_boundary_lubrication/links/56c3bb2308aee3dcd4167a77/The-study-of-transition-from-full-film-elastohydrodynamic-to-mixed-and-boundary-lubrication.pdf (accessed on 1 December 2021).
- Hu, Y.-Z.; Zhu, D. A Full Numerical Solution to the Mixed Lubrication in Point Contacts. J. Tribol. 1999, 122, 1–9. [Google Scholar] [CrossRef]
- Venner, C.H. EHL Film Thickness Computations at Low Speeds: Risk of Artificial Trends as a Result of Poor Accuracy and Implications for Mixed Lubrication Modelling. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2005, 219, 285–290. [Google Scholar] [CrossRef]
- Zhu, D. On some aspects of numerical solutions of thin-film and mixed elastohydrodynamic lubrication. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2007, 221, 561–579. [Google Scholar] [CrossRef]
- Zhu, D.; Liu, Y.; Wang, Q. On the Numerical Accuracy of Rough Surface EHL Solution. Tribol. Trans. 2014, 57, 570–580. [Google Scholar] [CrossRef]
- Wang, Y.; Dorgham, A.; Liu, Y.; Wang, C.; Wilson, M.C.T.; Neville, A.; Azam, A. An Assessment of Quantitative Predictions of Deterministic Mixed Lubrication Solvers. J. Tribol. 2020, 143, 1–42. [Google Scholar] [CrossRef]
- Wang, W.-Z.; Hu, Y.-Z.; Liu, Y.-C.; Zhu, D. Solution agreement between dry contacts and lubrication system at ultra-low speed. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2010, 224, 1049–1060. [Google Scholar] [CrossRef]
- He, T.; Zhu, D.; Wang, J.; Wang, Q.J. Experimental and Numerical Investigations of the Stribeck Curves for Lubricated Counterformal Contacts. J. Tribol. 2016, 139, 021505. [Google Scholar] [CrossRef]
- Li, S.; Kahraman, A. A fatigue model for contacts under mixed elastohydrodynamic lubrication condition. Int. J. Fatigue 2011, 33, 427–436. [Google Scholar] [CrossRef]
- Yan, X.-L.; Wang, X.-L.; Zhang, Y.-Y. A Parametric Study on Fatigue Life for Mixed Elastohydrodynamic Lubrication Point Contacts. J. Tribol. 2013, 135, 041501. [Google Scholar] [CrossRef]
- Elrod, H.G. A cavitation algorithm. Transactions of the ASME. J. Lubr. Technol. 1981, 103, 350–354. [Google Scholar] [CrossRef]
- Pu, W.; Zhu, D.; Wang, J. A Starved Mixed Elastohydrodynamic Lubrication Model for the Prediction of Lubrication Performance, Friction and Flash Temperature With Arbitrary Entrainment Angle. J. Tribol. 2018, 140, 031501. [Google Scholar] [CrossRef]
- Stanley, H.M.; Kato, T. An FFT-Based Method for Rough Surface Contact. J. Tribol. 1997, 119, 481–485. [Google Scholar] [CrossRef]
- Almqvist, A.; Sahlin, F.; Larsson, R.; Glavatskih, S. On the dry elasto-plastic contact of nominally flat surfaces. Tribol. Int. 2007, 40, 574–579. [Google Scholar] [CrossRef]
- Barber, J.R. Contact Mechanics; Springer: Berlin/Heidelberg, Germany, 2018; Volume 250. [Google Scholar]
- Barus, C. ART. X.—Isothermals, Isopiestics and Isometrics relative to Viscosity. Am. J. Sci. (1880–1910) 1893, 45, 87. [Google Scholar] [CrossRef]
- Dowson, D. Elasto-Hydrodynamic Lubrication/by D. Dowson and G. R. Higginson; Chapters 9 and 10 by J.F. Archard and A.W. Crook; International Series on Materials Science and Technology; Oxford [Eng.]; Higginson, G.R., Ed.; Pergamon Press: New York, NY, USA, 1977; Volume 23. [Google Scholar]
- Bair, S.; Winer, W.O. The High Pressure High Shear Stress Rheology of Liquid Lubricants. J. Tribol. 1992, 114, 1–9. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Y.; Wang, Y. A Method for Improving the Capability of Convergence of Numerical Lubrication Simulation by Using the PID Controller, in Advances in Mechanism and Machine Science; Springer: Berlin/Heidelberg, Germany, 2019; pp. 3845–3854. [Google Scholar]
- Lenning, R.L. The transition from boundary to mixed friction. Lubr. Eng. 1960, 16, 575–582. [Google Scholar]
- Wu, J.-J. Simulation of rough surfaces with FFT. Tribol. Int. 2000, 33, 47–58. [Google Scholar] [CrossRef]
- Reizer, R.; Pawlus, P.; Galda, L.; Grabon, W.; Dzierwa, A. Modeling of worn surface topography formed in a low wear process. Wear 2012, 278-279, 94–100. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Y.; Zhang, G.; Wang, Y. A Simulation Method for Non-Gaussian Rough Surfaces Using Fast Fourier Transform and Translation Process Theory. J. Tribol. 2018, 140, 021403. [Google Scholar] [CrossRef]
- Brunetiere, N.; Wang, Q.J. Large-Scale Simulation of Fluid Flows for Lubrication of Rough Surfaces. J. Tribol. 2013, 136, 011701. [Google Scholar] [CrossRef]
- Qiu, Y.; Khonsari, M.M. On the Prediction of Cavitation in Dimples Using a Mass-Conservative Algorithm. J. Tribol. 2009, 131, 041702. [Google Scholar] [CrossRef]



| Parameters | Value |
|---|---|
| Young’s modulus of WC-Ni, Es | 550 GPa |
| Hardness of WC-Ni, Hs | 1500 HV |
| Young’s modulus of GCr15, Er | 220 GPa |
| Hardness of GCr15, Hr | 200 HV |
| Viscosity of PAO02, ηP | 8.4 × 10−3 Pa∙s |
| Viscosity-pressure index, α | 1.95 × 10−8 Pa−1 |
| Dry friction coefficient, fc | 0.15 |
| Parameters | Value |
|---|---|
| Inlet viscosity, η | 17.4 × 10−3 Pa∙s |
| Viscosity-pressure index, α | 1.95 × 10−8 Pa−1 |
| Applied load on unit area, W | 0.69 MPa |
| Width of bearing, B | 6.35 mm |
| Sliding speed, U | 0.001~6 m/s |
| Root mean square roughness, Sq | 0.137 μm |
| Dry friction coefficient, fc | 0.15 |
| Sq (μm) | Ssk | Sku | Sal (μm) | ||
|---|---|---|---|---|---|
| Sample 1 | Test area 1 | 0.493 | −0.52 | 5.39 | 6.45 |
| Test area 2 | 0.510 | −0.55 | 4.81 | 6.12 | |
| Sample 2 | Test area 1 | 0.494 | −0.57 | 5.04 | 6.19 |
| Test area 2 | 0.504 | −0.46 | 4.58 | 6.52 | |
| Average | 0.500 | −0.53 | 4.96 | 6.32 | |
| Sq (μm) | Ssk | Sku | Sal (μm) | ||
|---|---|---|---|---|---|
| Sample 1 | Test area 1 | 0.032 | −11.63 | 141 | 7.108 |
| Test area 2 | 0.025 | −7.68 | 178 | 9.656 | |
| Sample 2 | Test area 1 | 0.034 | −9.01 | 110 | 8.305 |
| Test area 2 | 0.045 | −9.33 | 102 | 7.451 | |
| Average | 0.500 | 0.034 | −9.41 | 133 | |
| Sq (μm) | Ssk | Sku | Sal (μm) | |
|---|---|---|---|---|
| Before test | 0.5 | −0.53 | 4.96 | 7 |
| AS1 | 0.4 | −0.8 | 6 | 7 |
| AS2 | 0.3 | −1.5 | 15 | 7 |
| AS3 | 0.2 | −3 | 30 | 7 |
| AS4 | 0.2 | −3.5 | 30 | 7 |
| AS5 | 0.2 | −4 | 30 | 7 |
| AS6 | 0.1 | −6 | 90 | 7 |
| After test | 0.034 | −9.41 | 133 | 7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Azam, A.; Zhang, G.; Dorgham, A.; Liu, Y.; Wilson, M.C.T.; Neville, A. Understanding the Mechanism of Load-Carrying Capacity between Parallel Rough Surfaces through a Deterministic Mixed Lubrication Model. Lubricants 2022, 10, 12. https://doi.org/10.3390/lubricants10010012
Wang Y, Azam A, Zhang G, Dorgham A, Liu Y, Wilson MCT, Neville A. Understanding the Mechanism of Load-Carrying Capacity between Parallel Rough Surfaces through a Deterministic Mixed Lubrication Model. Lubricants. 2022; 10(1):12. https://doi.org/10.3390/lubricants10010012
Chicago/Turabian StyleWang, Yuechang, Abdullah Azam, Gaolong Zhang, Abdel Dorgham, Ying Liu, Mark C. T. Wilson, and Anne Neville. 2022. "Understanding the Mechanism of Load-Carrying Capacity between Parallel Rough Surfaces through a Deterministic Mixed Lubrication Model" Lubricants 10, no. 1: 12. https://doi.org/10.3390/lubricants10010012
APA StyleWang, Y., Azam, A., Zhang, G., Dorgham, A., Liu, Y., Wilson, M. C. T., & Neville, A. (2022). Understanding the Mechanism of Load-Carrying Capacity between Parallel Rough Surfaces through a Deterministic Mixed Lubrication Model. Lubricants, 10(1), 12. https://doi.org/10.3390/lubricants10010012





