Quantum Cosmology in the Light of Quantum Mechanics
Abstract
:1. Introduction
2. Quantum Cosmology: A (Very) Brief Review
2.1. Classical Constraints
2.2. Canonical Quantisation
2.3. Minisuperspace
3. Classical Analogy: The Geometric Minisuperspace
4. Quantum Picture
4.1. Quantum Field Theory in the Spacetime
4.2. Quantum Field Theory in the Minisuperspace
5. Particles and Universes Propagating in Their Spaces
5.1. Semiclassical Universes: Classical Spacetime and Quantum Matter Fields
5.2. Semiclassical Particles: Geodesics and Uncertainties in the Position
6. Conclusions and Further Comments
Funding
Acknowledgments
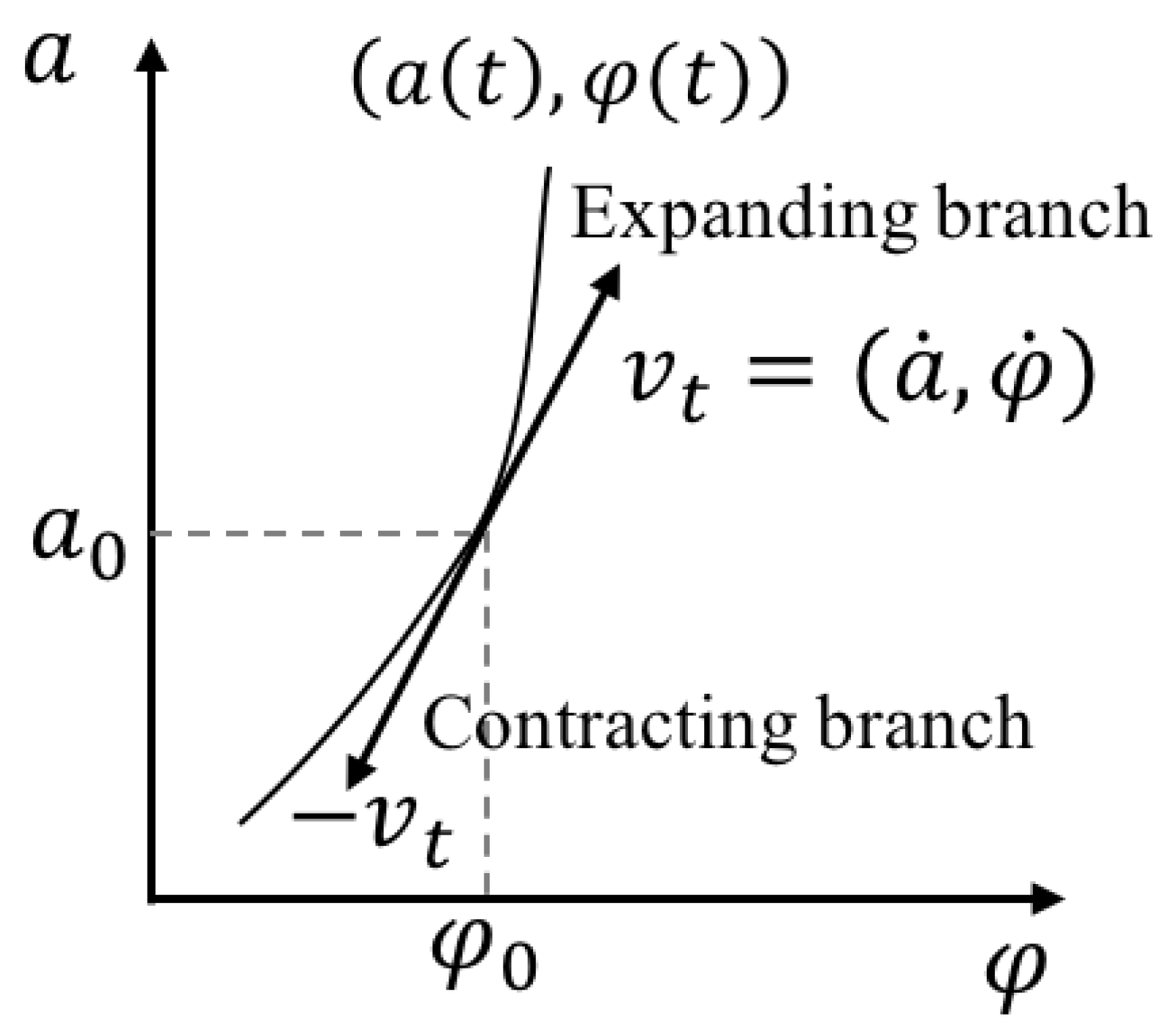
Conflicts of Interest
References
- Gell-Mann, M.; Hartle, J.B. Quantum mechanics in the light of quantum cosmology. In Complexity, Entropy and the Physics of Information; Zurek, W.H., Ed.; Addison-Wesley: Reading, PA, USA, 1990. [Google Scholar]
- Kiefer, C. Quantum Cosmology and the Emergence of a Classical World. In Philosophy, Mathematics and Modern Physics: A Dialogue; Rudolph, E., Stamatescu, I.O., Eds.; Springer: Berlin/Heidelberg, Germany, 1994; pp. 104–119. [Google Scholar] [CrossRef]
- Everett, H. “Relative State” Formulation of Quantum Mechanics. Rev. Mod. Phys. 1957, 29, 454. [Google Scholar] [CrossRef]
- Gell-Mann, M.; Hartle, J.B. Classical equations for quantum systems. Phys. Rev. D 1993, 47, 3345–3382. [Google Scholar] [CrossRef]
- Hartle, J.B. Spacetime quantum mechanics and the quantum mechanics of spacetime. In Gravitation and Quantization: Proceedings of the 1992 Les Houches Summer School; Julia, B., Zinn-Justin, J., Eds.; North Holland: Amsterdam, The Netherlands, 1995. [Google Scholar]
- Rovelli, C. Notes for a brief history of quantum gravity. In Proceedings of the 9th Marcel Grossmann Meeting, Rome, Italy, 2–8 July 2000. [Google Scholar]
- Kiefer, C.; Singh, T.P. Quantum gravitational corrections to the functional Schrödinger equation. Phys. Rev. D 1991, 44, 1067. [Google Scholar] [CrossRef]
- Kiefer, C.; Krämer, M. Quantum gravitational contributions to the CMB anisotropy spectrum. Phys. Rev. Lett. 2012, 108, 021301. [Google Scholar] [CrossRef]
- Brizuela, D.; Kiefer, C.; Krämer, M. Quantum-gravitational effects on gauge-invariant scalar and tensor perturbations during inflation: The de Sitter case. Phys. Rev. D 2016, 93, 104035. [Google Scholar] [CrossRef]
- Brizuela, D.; Kiefer, C.; Krämer, M. Quantum-gravitational effects on gauge-invariant scalar and tensor perturbations during inflation: The slow-roll approximation. Phys. Rev. D 2016, 94, 123527. [Google Scholar] [CrossRef]
- Wheeler, J.A. On the nature of quantum geometrodynamics. Ann. Phys. 1957, 2, 604–614. [Google Scholar] [CrossRef]
- De Witt, B.S. Quantum Theory of Gravity. I. The Canonical Theory. Phys. Rev. 1967, 160, 1113–1148. [Google Scholar] [CrossRef]
- Hawking, S.W. Spacetime foam. Nucl. Phys. B 1978, 144, 349–362. [Google Scholar] [CrossRef]
- Hawking, S.W. The boundary conditions of the universe. In Astrophysical Cosmology, Proceedings of the Study Week on Cosmology and Fundamental Physics, Rome, Italy, 28 September–2 October 1981; Pontifical Academy of Sciences: Vatican City, Italy, 1982; pp. 563–572. [Google Scholar]
- Hawking, S.W. Quantum cosmology. In Relativity, Groups and Topology II, Les Houches, Session XL, 1983; De Witt, B.S., Stora, R., Eds.; Elsevier Science Publishers B. V.: Amsterdam, The Netherlands, 1984. [Google Scholar]
- Hawking, S.W. The quantum state of the universe. Nucl. Phys. B 1984, 239, 257–276. [Google Scholar] [CrossRef]
- Hawking, S.W. The Density Matrix of the Universe. Phys. Scr. 1987, T15, 151–153. [Google Scholar] [CrossRef]
- Vilenkin, A. Creation of universes from nothing. Phys. Lett. B 1982, 117, 25–28. [Google Scholar] [CrossRef]
- Vilenkin, A. Quantum creation of universes. Phys. Rev. D 1984, 30, 509–511. [Google Scholar] [CrossRef]
- Vilenkin, A. Boundary conditions in quantum cosmology. Phys. Rev. D 1986, 33, 3560–3569. [Google Scholar] [CrossRef]
- Vilenkin, A. Quantum cosmology and the initial state of the Universe. Phys. Rev. D 1988, 37, 888–897. [Google Scholar] [CrossRef]
- Vilenkin, A. Approaches to quantum cosmology. Phys. Rev. D 1994, 50, 2581–2594. [Google Scholar] [CrossRef]
- Vilenkin, A. Predictions from Quantum Cosmology. Phys. Rev. Lett. 1995, 74, 846–849. [Google Scholar] [CrossRef] [PubMed]
- Hartle, J.B.; Hawking, S.W. Wave function of the Universe. Phys. Rev. D 1983, 28, 2960. [Google Scholar] [CrossRef]
- Hartle, J.B. The quantum mechanics of cosmology. In Quantum Cosmology and Baby Universes; Coleman, S., Hartle, J.B., Piran, T., Weinberg, S., Eds.; World Scientific: London, UK, 1990; Volume 7. [Google Scholar]
- Halliwell, J.J.; Hawking, S.W. Origin of structure in the Universe. Phys. Rev. D 1985, 31, 1777–1791. [Google Scholar] [CrossRef]
- Halliwell, J.J. Correlations in the wave function of the Universe. Phys. Rev. D 1987, 36, 3626–3640. [Google Scholar] [CrossRef]
- Halliwell, J.J. Decoherence in quantum cosmology. Phys. Rev. D 1989, 39, 2912–2923. [Google Scholar] [CrossRef]
- Halliwell, J.J. Introductory lectures on quantum cosmology. In Quantum Cosmology and Baby Universes; Coleman, S., Hartle, J.B., Piran, T., Weinberg, S., Eds.; World Scientific: London, UK, 1990; Volume 7. [Google Scholar]
- Kiefer, C. Continuous measurement of mini-superspace variables by higher multipoles. Class. Quant. Grav. 1987, 4, 1369–1382. [Google Scholar] [CrossRef]
- Kiefer, C. Decoherence in quantum electrodynamics and quantum gravity. Phys. Rev. D 1992, 46, 1658–1670. [Google Scholar] [CrossRef]
- Kiefer, C. Quantum Gravity; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Wiltshire, D.L. An Introduction to Quantum Cosmology. In Cosmology: The Physics of the Universe; Robson, B., Visvanathan, N., Woolcock, W., Eds.; World Scientific: Singapore, 1996; pp. 473–531. [Google Scholar]
- Arnowitt, R.; Deser, S.; Misner, C.W. Dynamical structure and definition of energy in General Relativity. Phys. Rev. 1959, 116, 1322–1330. [Google Scholar] [CrossRef]
- Linde, A. Particle Physics and Inflationary Cosmology; Contemporary Concepts in Physics; Harwood Academic Publishers: Chur, Switzerland, 1993; Volume 5. [Google Scholar]
- Joos, E.; Zeh, H.D.; Kiefer, C.; Giulini, D.J.; Kupsch, J.; Stamatescu, I.O. Decoherence and the Appearance of a Classical World in Quantum Theory; Springer: Berlin, Germany, 2003. [Google Scholar]
- Schlosshauer, M. Decoherence and the Quantum-To-Classical Transition; Springer: Berlin, Germany, 2007. [Google Scholar]
- Robles-Pérez, S.; González-Díaz, P.F. Quantum entanglement in the multiverse. J. Exp. Theor. Phys. 2014, 118, 34. [Google Scholar] [CrossRef]
- Robles-Pérez, S.J. Creation of entangled universes avoids the Big Bang singularity. J. Grav. 2014, 2014, 382675. [Google Scholar] [CrossRef]
- Robles-Pérez, S. Effects of inter-universal entanglement on the state of the early universe. In Cosmology on Small Scales 2018. Dark Matter Problem and Selected Controversies in Cosmology; Krizek, M., Dumin, Y.V., Eds.; Institute of Mathematics, Czech Academy of Sciences: Žitná, Czech Republic, 2018. [Google Scholar]
- Garay, I.; Robles-Pérez, S. Classical geodesics from the canonical quantisation of spacetime coordinates. arXiv 2019, arXiv:1901.05171. [Google Scholar]
- Christodoulakis, T.; Dimakis, N.; Terzis, P.A.; Doulis, G.; Grammenos, T.; Melas, E.; Spanaou, A. Conditional symmetries and the canonical quantization of constrained minisuperspace actions: The Schwarzschild case. J. Geom. Phys. 2013, 71, 127–138. [Google Scholar] [CrossRef]
- Karagiorgos, A.; Pailas, T.; Dimakis, N.; Terzis, P.A.; Christodoulakis, T. Quantum cosmology of a Bianchi III LRS goemetry coupled to a source free electromagnetic field. J. Cosmol. Astropart. Phys. 2018, 2018, 030. [Google Scholar] [CrossRef]
- Chernikov, N.A.; Tagirov, E.A. Quantum theory of scalar field in de Sitter space-time. Annales de l’IHP Physique Théorique 1968, 9, 109–141. [Google Scholar]
- Birrell, N.D.; Davies, P.C.W. Quantum Fields in Curved Space; Cambridge University Press: Cambridge, UK, 1982. [Google Scholar]
- Mukhanov, V.F.; Winitzki, S. Quantum Effects in Gravity; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Schrödinger, E. Discussion of Probability relations between separated systems. Math. Proc. Camb. Philos. Soc. 1936, 31, 555–563. [Google Scholar] [CrossRef]
- Bell, J.S. Speakable and Unspeakable in Quantum Mechanics; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- Robles-Pérez, S.J. Cosmological perturbations in the entangled inflationary universe. Phys. Rev. D 2018, 97, 066018. [Google Scholar] [CrossRef]
- Caderni, N.; Martellini, M. Third quantization formalism for Hamiltonian cosmologies. Int. J. Theor. Phys. 1984, 23, 233. [Google Scholar] [CrossRef]
- McGuigan, M. Third quantization and the Wheeler-DeWitt equation. Phys. Rev. D 1988, 38, 3031. [Google Scholar] [CrossRef]
- Rubakov, V.A. On third quantization and the cosmological constant. Phys. Lett. B 1988, 214, 503–507. [Google Scholar] [CrossRef]
- Strominger, A. Baby Universes. In Quantum Cosmology and Baby Universes; Coleman, S., Hartle, J.B., Piran, T., Weinberg, S., Eds.; World Scientific: London, UK, 1990; Volume 7. [Google Scholar]
- Robles-Pérez, S.; González-Díaz, P.F. Quantum state of the multiverse. Phys. Rev. D 2010, 81, 083529. [Google Scholar] [CrossRef]
- Robles-Pérez, S. Invariant vacuum. Phys. Lett. B 2017, 774, 608–615. [Google Scholar] [CrossRef]
- Robles-Pérez, S.J. Time reversal symmetry in cosmology and the quantum creation of universes. arXiv 2019, arXiv:1901.03387. [Google Scholar]
- Robles-Pérez, S.J. Restoration of matter-antimatter symmetry in the multiverse. arXiv 2017, arXiv:1706.06304. [Google Scholar]
- Boyle, L.; Finn, K.; Turok, N. CPT-Symmetric universe. Phys. Rev. Lett. 2018, 121, 251301. [Google Scholar] [CrossRef]
- Robles-Pérez, S. Quantum cosmology of a conformal multiverse. Phys. Rev. D 2017, 96, 063511. [Google Scholar] [CrossRef]
- Feynman, R.P. The theory of positrons. Phys. Rev. 1949, 76, 749. [Google Scholar] [CrossRef]
- Kiefer, C. The semiclassical approximation to quantum gravity. In Canonical Gravity: From Classical to Quantum; Ehlers, J., Friedrich, H., Eds.; Springer: Berlin, Germany, 1994. [Google Scholar]
- Sharma, L.K.; Sharma, G.S. Quark potentials for mesons in the Klein-Gordon equation. Pramana 1984, 22, 539–547. [Google Scholar] [CrossRef]
- Littlejohn, R.G. Introduction to Relativistic Quantum Mechanics and the Klein-Gordon Equation. Available online: http://bohr.physics.berkeley.edu/classes/221/1112/notes/kleing.pdf (accessed on 24 April 2019).
- Manarse, F.K.; Misner, C.W. Fermi normal coordinates and some basic concepts in differential geometry. J. Math. Phys. 1963, 4, 735. [Google Scholar] [CrossRef]
- Podolsky, J.; Svarc, R. Interpreting spacetimes of any dimension using geodesic deviation. Phys. Rev. D 2012, 85, 044057. [Google Scholar] [CrossRef]
- Kiefer, C. Wave packets in minisuperspace. Phys. Rev. D 1988, 38, 1761. [Google Scholar] [CrossRef]
- Kiefer, C.; Zeh, H.D. Arrow of time in a recollapsing quantum universe. Phys. Rev. D 1995, 51, 4145–4153. [Google Scholar] [CrossRef]
- Bezrukov, F.L.; Shaposhnikov, M.E. The Standard Model Higgs boson as the inflation. Phys. Lett. B 2008, 659, 703. [Google Scholar] [CrossRef]
| 1 | Time is created at the onset of the universe and thus the wave function of the universe cannot be a time-dependent function, so we cannot apply an initial condition on the state of the universe. However, the universe may have a boundary where to impose the conditions that eventually would determine everything else in the whole history of the universe. |
| 2 | These are essentially the relative states of Everett’s formulation of quantum mechanics [3]. However, Everett did not provide an explanation of why some states and no others are selected from the whole set of possible states. To explain it, Hartle needed to add, besides the boundary condition of the state of the universe and the equations of quantum mechanics, a new ingredient: the coarse-graining process that makes some states emerge from the decoherence process. These are the selected states of the Everett’s formulation. |
| 3 | The outcomes of a classical experiment are exclusive, i.e., the cat is either dead or alive but not both. |
| 4 | The existence of a semiclassical domain in the universe, and actually our own existence, can be seen as two possible outcomes of the cosmological experiment. As Hartle stated [5], we live in the middle of this particular experiment. |
| 5 | |
| 6 | Note, however, that this space can still be infinite dimensional. |
| 7 | It turns out that the quantum behaviour of a system is not a matter of its smallness, at least in principle. The analysis of the decoherence processes (see, for instance, Refs. [36,37]) shows that the quantum-to-classical transition essentially depends on the complexity of the system, i.e., on the number of constituents and the quantum coherence of their correlations. A macroscopic system typically contains a huge number of constituents so that the quantum correlations rapidly disappear. However, the application of the quantum theory to a model of the universe with a small number of degrees of freedom, e.g., a minisuperspace model of the universe, shows that the quantum effects might be important as well in the universe [38,39,40]. For instance, the quantum state of the universe could be given by a squeezed state, which is usually dubbed a quantum state without classical analogue [38]; or the composite quantum state of two otherwise semiclassical universes could be entangled [38,39,40]. Incidentally, it also reveals that the concept of classicality has different acceptations that must be taken with care in the context of quantum cosmology. As stated in Section 1, the application of the quantum theory to the universe challenges some of its fundamentals. |
| 8 | Eventually, these inhomogeneous modes are interpreted as particles and gravitons propagating in the homogeneous and isotropic background spacetime. |
| 9 | Spinorial and vector fields can be considered as well. |
| 10 | For convenience, the scalar field has been rescaled according to . |
| 11 | Unless otherwise indicated, we always consider cosmic time, i.e., . |
| 12 | Recall that the scalar field has been rescaled according to , see f.n. 10. |
| 13 | |
| 14 | Let us bear in mind, however, that it is only an analogy. |
| 15 | This way of obtaining the Klein–Gordon equation from the Hamiltonian constraint of a test particle that propagates in the spacetime has been well-known for a long time. It can be seen, for instance, in Ref. [44]. However, it is not customarily used in quantum field theory. |
| 16 | The exact meaning of “sufficiently localised” is specified in Section 5. |
| 17 | By a future directed vector in the minisuperspace, we mean a vector positively oriented with respect to the scale factor component, which is the time-like variable of the minisuperspace. |
| 18 | We do not call it second quantisation of the universe because in this formalism the field can represent many universes. |
| 19 | This can explicitly be seen in a very simplified cosmological model [38]. As we show below, it might have consequences in the quantum creation of universes. |
| 20 | In the quantisation of a complex scalar field, it would be full of particle–antiparticle pairs. |
| 21 | From now on, we omit the hats on top of the operators to ease the notation. |
| 22 | Recall that the field was rescaled according to , see f.n. 10. |
| 23 | The Euclidean gap also prevent matter and antimatter from collapse |
| 24 | Classically, the changes, and , transform the action in Equation (21) [41] into
|
| 25 | For instance, in the case of a flat DeSitter spacetime, the modes with physical wavelengths much smaller than the cosmological horizon, , can be interpreted in terms of particles with highly definite trajectories [41]. |
| 26 | This will be done elsewhere. |

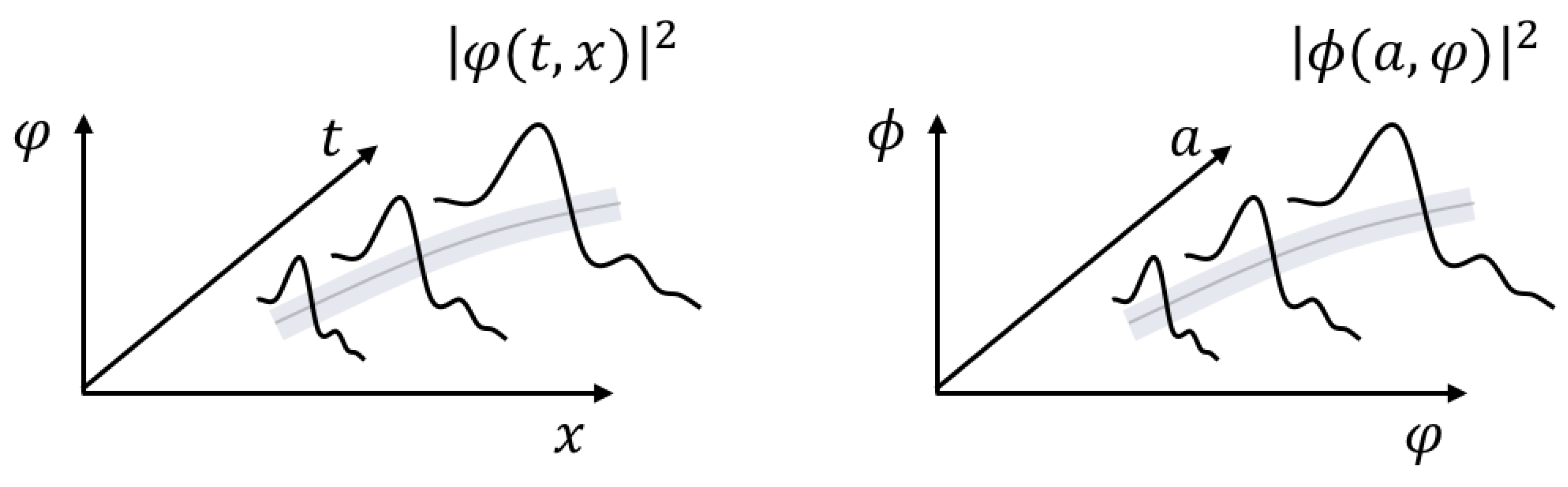

© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Robles-Pérez, S.J. Quantum Cosmology in the Light of Quantum Mechanics. Galaxies 2019, 7, 50. https://doi.org/10.3390/galaxies7020050
Robles-Pérez SJ. Quantum Cosmology in the Light of Quantum Mechanics. Galaxies. 2019; 7(2):50. https://doi.org/10.3390/galaxies7020050
Chicago/Turabian StyleRobles-Pérez, Salvador J. 2019. "Quantum Cosmology in the Light of Quantum Mechanics" Galaxies 7, no. 2: 50. https://doi.org/10.3390/galaxies7020050
APA StyleRobles-Pérez, S. J. (2019). Quantum Cosmology in the Light of Quantum Mechanics. Galaxies, 7(2), 50. https://doi.org/10.3390/galaxies7020050




