Tracing Hot Spot Motion in Sagittarius A* Using the Next-Generation Event Horizon Telescope (ngEHT)
Abstract
1. Modellng Flares in Sgr A* with Hot Spots
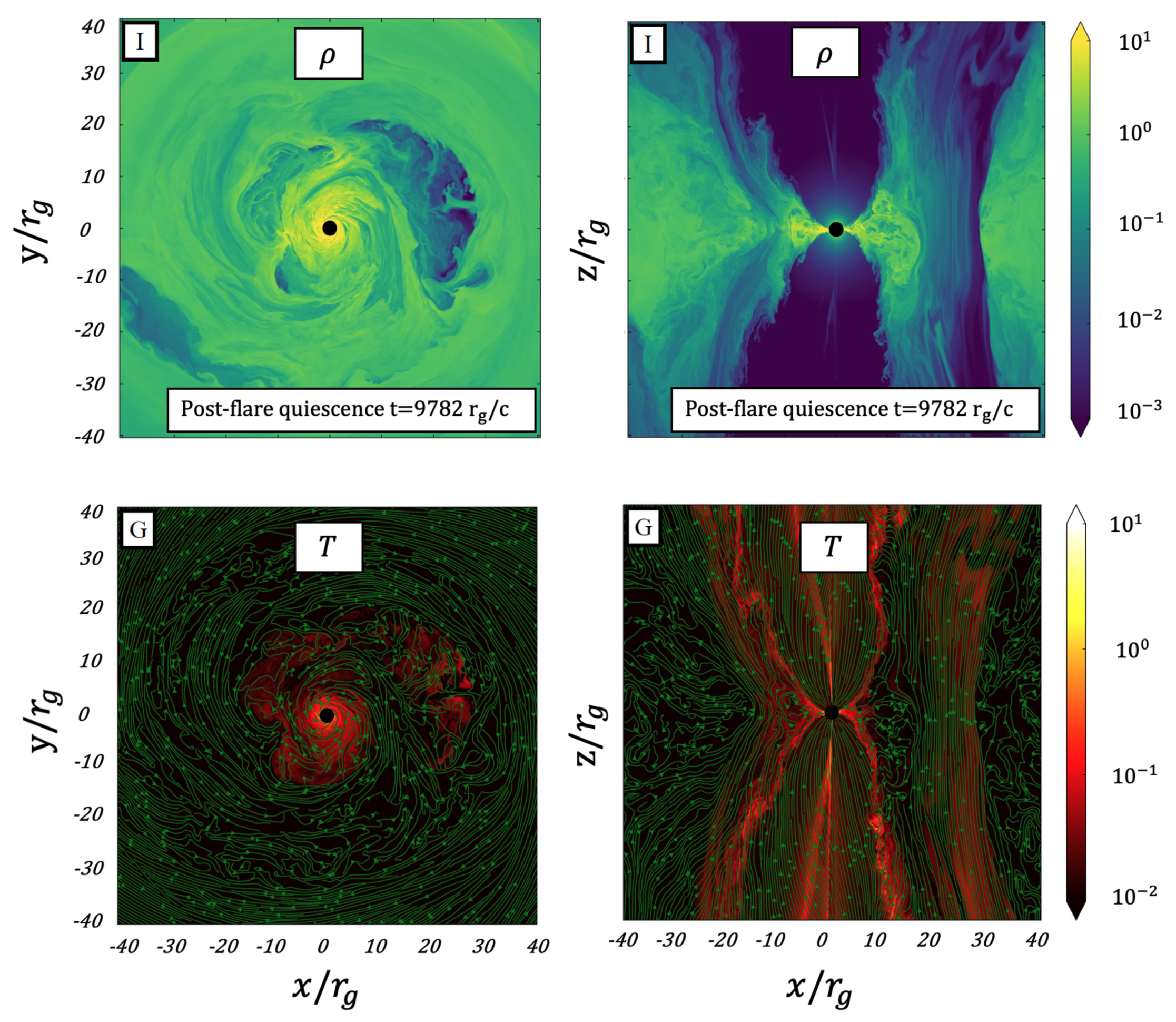
2. Dynamical Formation of a Hot Spot in the Simulations
3. Semi-Analytic Simulation of a Shearing Hot Spot
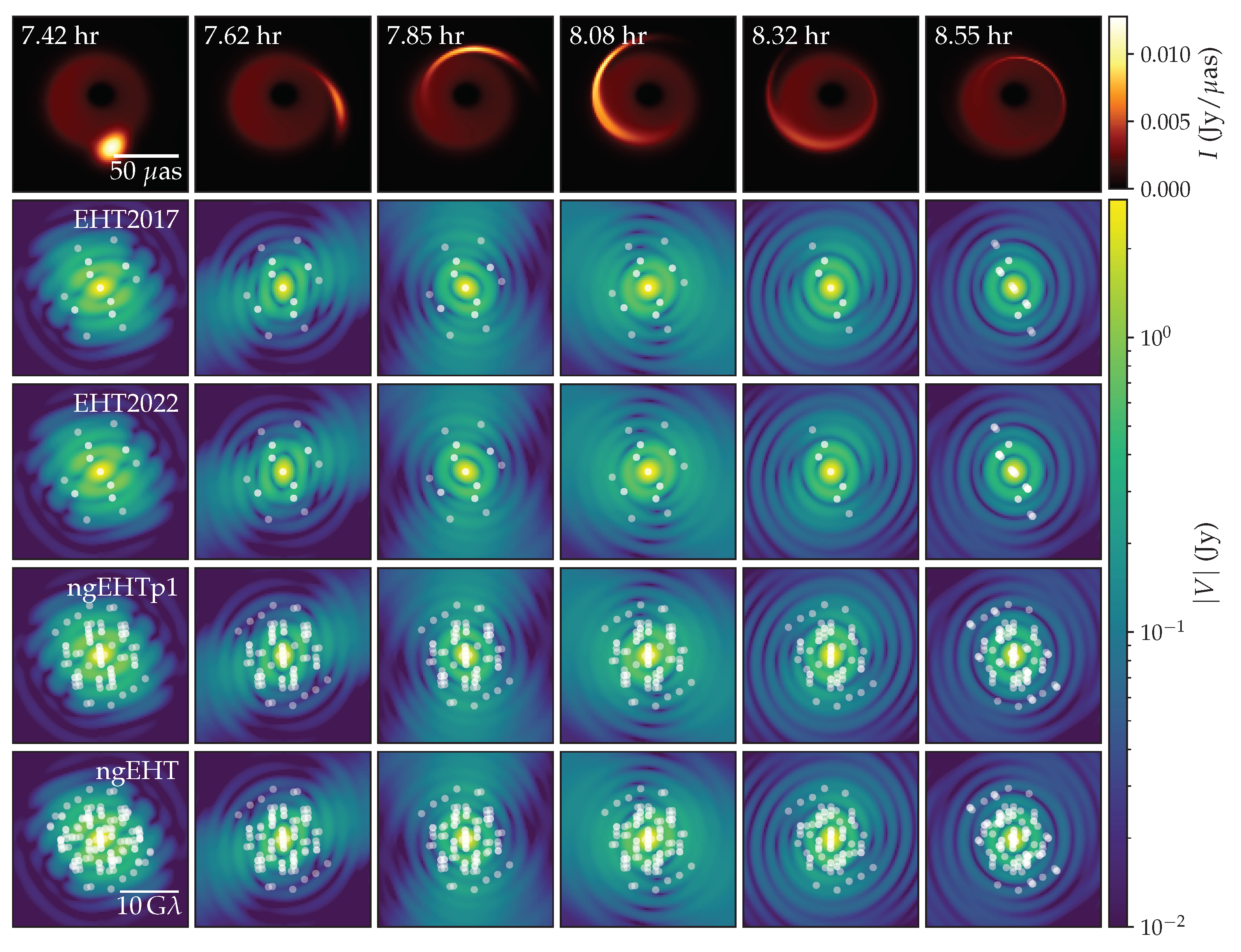
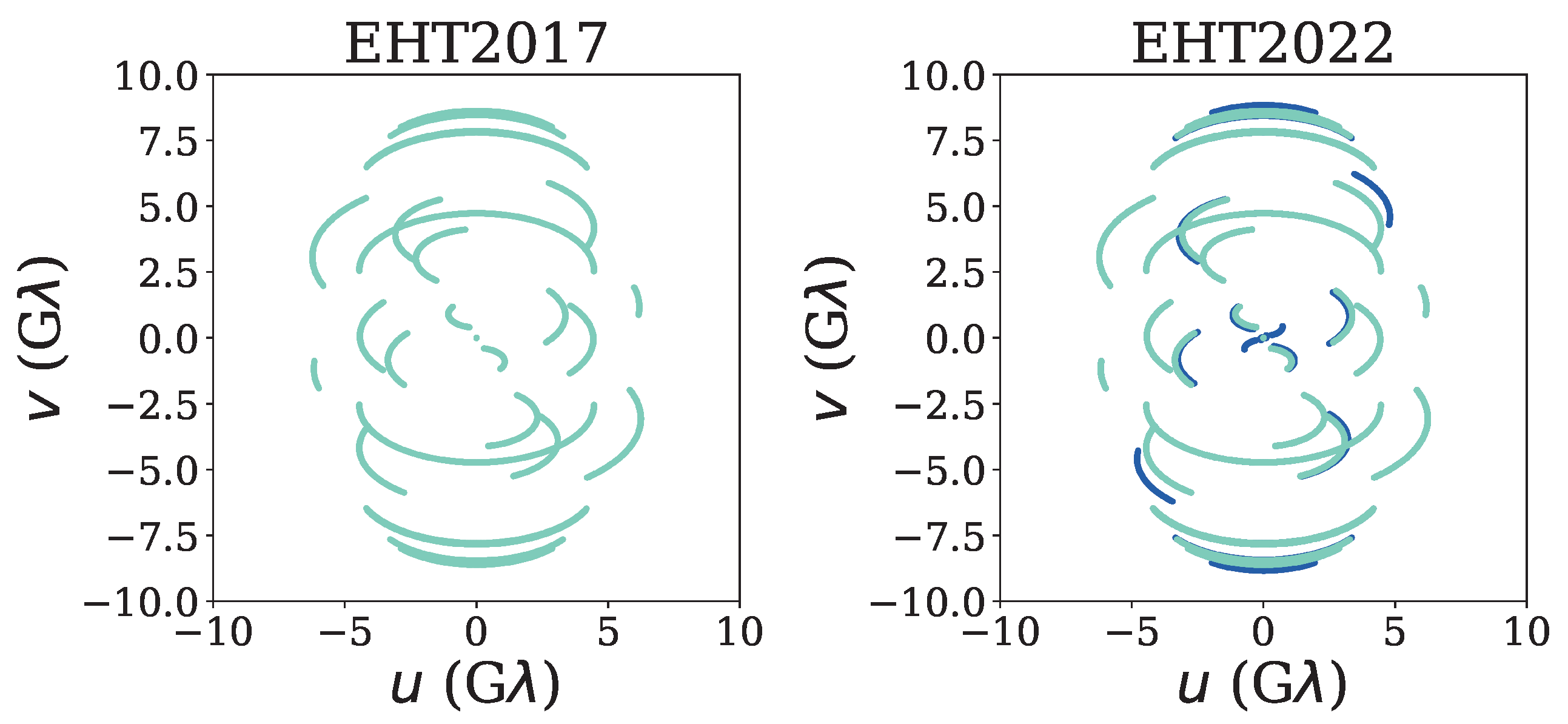
4. Creating Synthetic Data for EHT/ngEHT
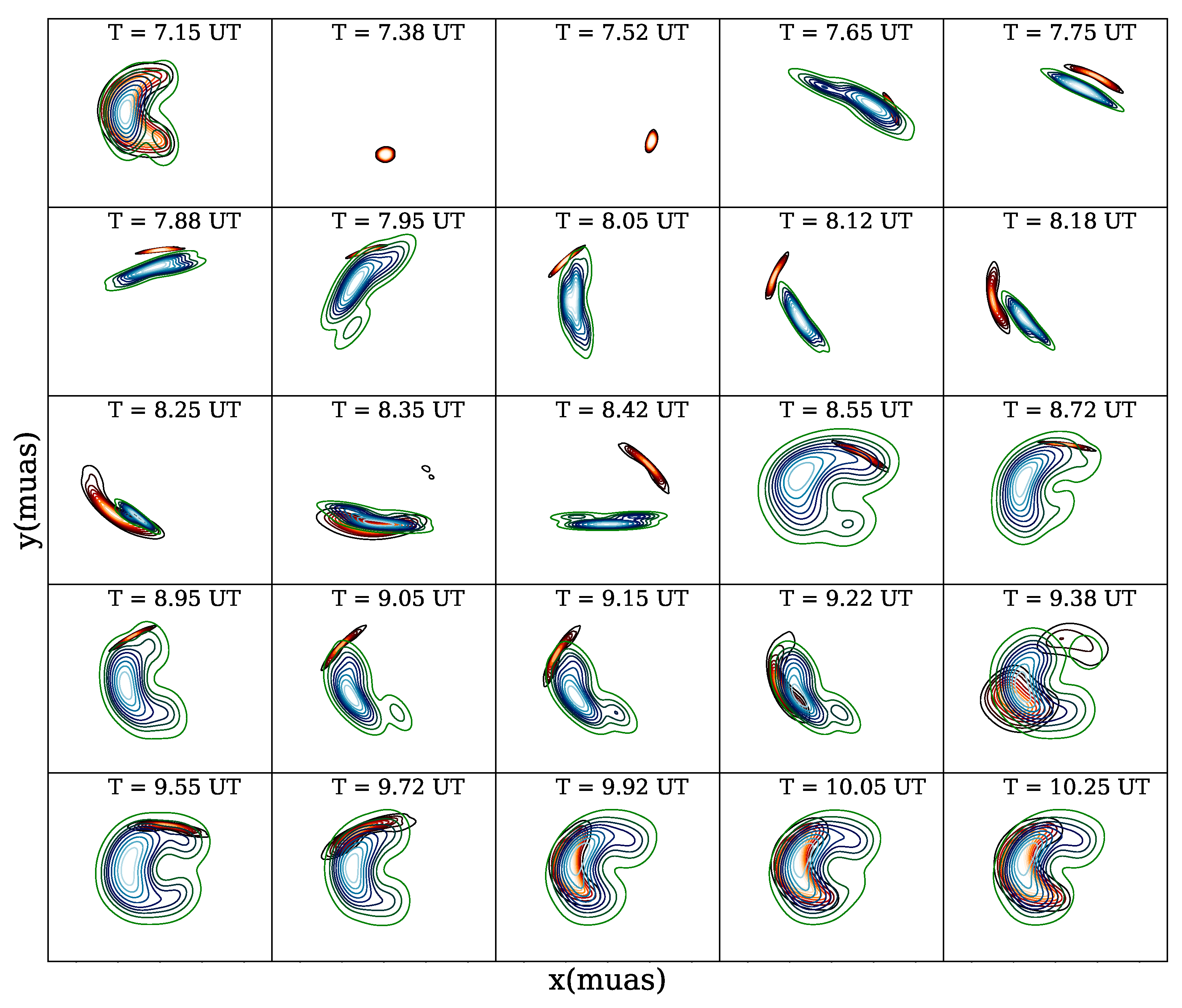
5. Dynamical Reconstruction Using the StarWarps Code
6. Reconstructing the Motion of the Hot Spot in Different Arrays
Nxcorr vs. Nrmse of the Reconstructed and Ground Truth Image
- : We make use of [67,70], defining the as:where X refers to the restructured image, while Y describes the ground truth image of the hot spot. Furthermore, N stands for the number of the pixels in the image, and refers to the mean pixel value of the image. Finally, describes the standard deviation of pixel values in image i. determines the similarities between two images. A perfect correlation between the images leads to 1, while a complete anti-correlation between them gives rise to a value of −1 for .
- is defined as [67]:where, unlike the case of , two completely similar (different) images X and Y have 0 (1) value .
- ∘
- Since the background RIAF is dominated in some snapshots, it is seen that we have a globally good correlation between the images.
- ∘
- This, however, becomes worse when the hot spot appears and becomes sheared down, in which it is seen that we have a some levels of suppression (enhancement) of () for some cases.
- ∘
- The aforementioned suppression (enhancement) is, however, minimal for the ngEHT array compared with the EHT(2017) and EHT(2022).
- ∘
- Consequently, we conclude that the ngEHT array helps a lot in improving the quality of the reconstructed image.
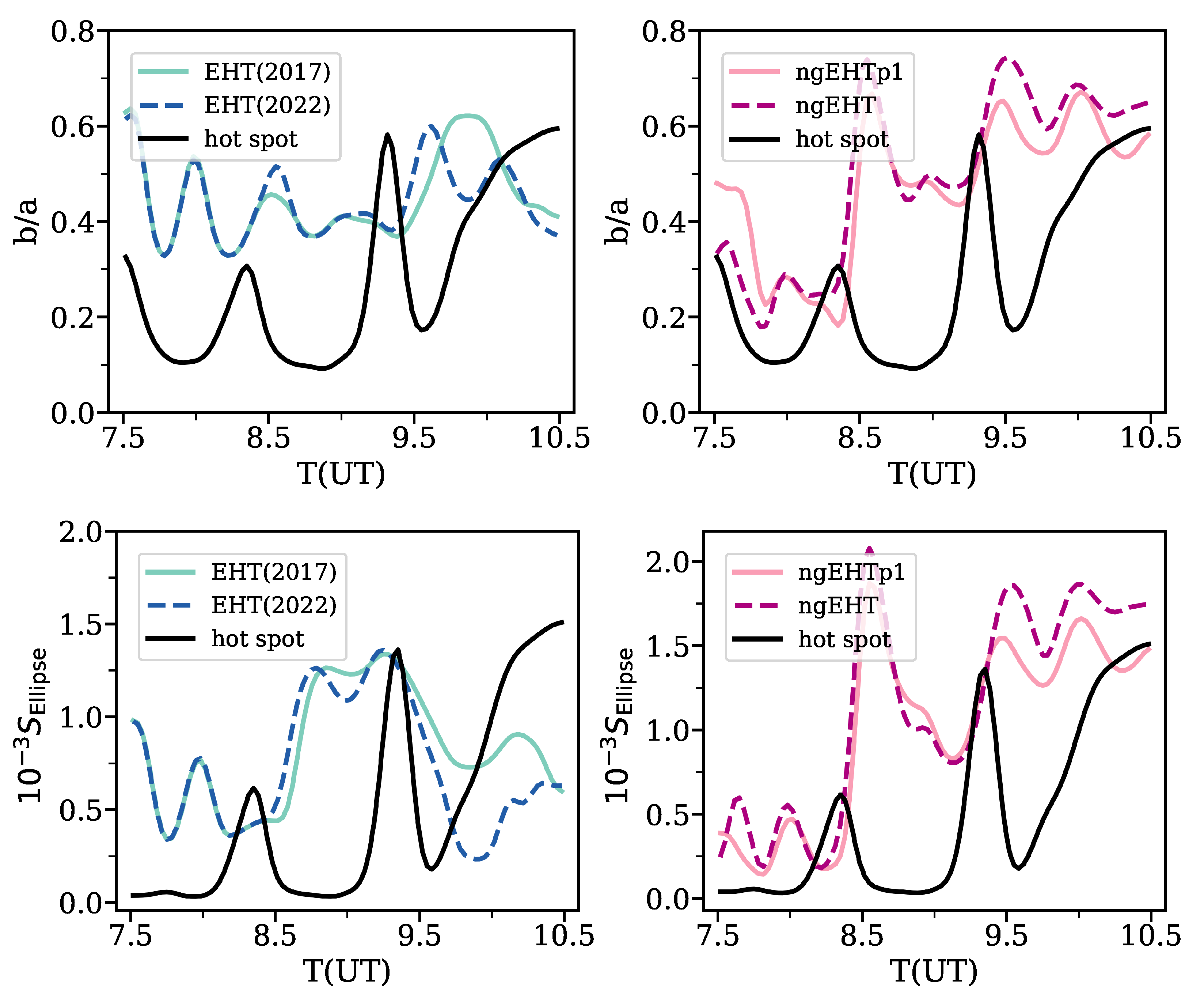
7. Tracking the Angular Location of the Hot Spot
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Akiyama, K.; Alberdi, A.; Alef, W.; Algaba, J.C.; Anantua, R.; Asada, K.; Azulay, R.; Bach, U.; Baczko, A.K.; Ball, D.; et al. First Sagittarius A* Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole in the Center of the Milky Way. Astrophys. J. Lett. 2022, 930, L12. [Google Scholar] [CrossRef]
- Akiyama, K.; Alberdi, A.; Alef, W.; Algaba, J.C.; Anantua, R.; Asada, K.; Azulay, R.; Bach, U.; Baczko, A.K.; Ball, D.; et al. First Sagittarius A* Event Horizon Telescope Results. II. EHT and Multiwavelength Observations, Data Processing, and Calibration. Astrophys. J. Lett. 2022, 930, L13. [Google Scholar] [CrossRef]
- Akiyama, K.; Alberdi, A.; Alef, W.; Algaba, J.C.; Anantua, R.; Asada, K.; Azulay, R.; Bach, U.; Baczko, A.K.; Ball, D.; et al. First Sagittarius A* Event Horizon Telescope Results. III. Imaging of the Galactic Center Supermassive Black Hole. Astrophys. J. Lett. 2022, 930, L14. [Google Scholar] [CrossRef]
- Akiyama, K.; Alberdi, A.; Alef, W.; Algaba, J.C.; Anantua, R.; Asada, K.; Azulay, R.; Bach, U.; Baczko, A.K.; Ball, D.; et al. First Sagittarius A* Event Horizon Telescope Results. IV. Variability, Morphology, and Black Hole Mass. Astrophys. J. Lett. 2022, 930, L15. [Google Scholar] [CrossRef]
- Akiyama, K.; Alberdi, A.; Alef, W.; Algaba, J.C.; Anantua, R.; Asada, K.; Azulay, R.; Bach, U.; Baczko, A.K.; Ball, D.; et al. First Sagittarius A* Event Horizon Telescope Results. V. Testing Astrophysical Models of the Galactic Center Black Hole. Astrophys. J. Lett. 2022, 930, L16. [Google Scholar] [CrossRef]
- Akiyama, K.; Alberdi, A.; Alef, W.; Algaba, J.C.; Anantua, R.; Asada, K.; Azulay, R.; Bach, U.; Baczko, A.K.; Ball, D.; et al. First Sagittarius A* Event Horizon Telescope Results. VI. Testing the Black Hole Metric. Astrophys. J. Lett. 2022, 930, L17. [Google Scholar] [CrossRef]
- Farah, J.; Galison, P.; Akiyama, K.; Bouman, K.L.; Bower, G.C.; Chael, A.; Fuentes, A.; Gómez, J.L.; Honma, M.; Johnson, M.D.; et al. Selective Dynamical Imaging of Interferometric Data. Astrophys. J. Lett. 2022, 930, L18. [Google Scholar] [CrossRef]
- Wielgus, M.; Marchili, N.; Martí-Vidal, I.; Keating, G.K.; Ramakrishnan, V.; Tiede, P.; Fomalont, E.; Issaoun, S.; Neilsen, J.; Nowak, M.A.; et al. Millimeter Light Curves of Sagittarius A* Observed during the 2017 Event Horizon Telescope Campaign. Astrophys. J. Lett. 2022, 930, L19. [Google Scholar] [CrossRef]
- Georgiev, B.; Pesce, D.W.; Broderick, A.E.; Wong, G.N.; Dhruv, V.; Wielgus, M.; Gammie, C.F.; Chan, C.k.; Chatterjee, K.; Emami, R.; et al. A Universal Power-law Prescription for Variability from Synthetic Images of Black Hole Accretion Flows. Astrophys. J. Lett. 2022, 930, L20. [Google Scholar] [CrossRef]
- Broderick, A.E.; Gold, R.; Georgiev, B.; Pesce, D.W.; Tiede, P.; Ni, C.; Moriyama, K.; Akiyama, K.; Alberdi, A.; Alef, W.; et al. Characterizing and Mitigating Intraday Variability: Reconstructing Source Structure in Accreting Black Holes with mm-VLBI. Astrophys. J. Lett. 2022, 930, L21. [Google Scholar] [CrossRef]
- Doeleman, S.; Blackburn, L.; Dexter, J.; Gomez, J.L.; Johnson, M.D.; Palumbo, D.C.; Weintroub, J.; Farah, J.R.; Fish, V.; Loinard, L.; et al. Studying Black Holes on Horizon Scales with VLBI Ground Arrays. arXiv 2019, arXiv:1909.01411. [Google Scholar]
- Johnson, M.; Haworth, K.; Pesce, D.W.; Palumbo, D.C.M.; Blackburn, L.; Akiyama, K.; Boroson, D.; Bouman, K.L.; Farah, J.R.; Fish, V.L.; et al. Studying black holes on horizon scales with space-VLBI. arXiv 2019, arXiv:1909.01405. [Google Scholar]
- Witzel, G.; Martinez, G.; Willner, S.P.; Becklin, E.E.; Boyce, H.; Do, T.; Eckart, A.; Fazio, G.G.; Ghez, A.; Gurwell, M.A.; et al. Rapid Variability of Sgr A* across the Electromagnetic Spectrum. Astrophys. J. 2021, 917, 73. [Google Scholar] [CrossRef]
- Doeleman, S.S.; Weintroub, J.; Rogers, A.E.E.; Plambeck, R.; Freund, R.; Tilanus, R.P.J.; Friberg, P.; Ziurys, L.M.; Moran, J.M.; Corey, B.; et al. Event-horizon-scale structure in the supermassive black hole candidate at the Galactic Centre. Nature 2008, 455, 78–80. [Google Scholar] [CrossRef]
- Doeleman, S. Approaching the event horizon: 1.3mmλ VLBI of SgrA*. J. Phys. Conf. Ser. 2008, 131, 012055. [Google Scholar] [CrossRef]
- Fish, V.L.; Doeleman, S.S.; Broderick, A.E.; Loeb, A.; Rogers, A.E.E. Detecting Flaring Structures in Sagittarius A* with (Sub)Millimeter VLBI. arXiv 2008, arXiv:0807.2427. [Google Scholar]
- Marrone, D.P.; Baganoff, F.K.; Morris, M.R.; Moran, J.M.; Ghez, A.M.; Hornstein, S.D.; Dowell, C.D.; Muñoz, D.J.; Bautz, M.W.; Ricker, G.R.; et al. An X-ray, Infrared, and Submillimeter Flare of Sagittarius A*. Astrophys. J. 2008, 682, 373–383. [Google Scholar] [CrossRef]
- Doeleman, S.S.; Fish, V.L.; Broderick, A.E.; Loeb, A.; Rogers, A.E.E. Detecting Flaring Structures in Sagittarius A* with High-Frequency VLBI. Astrophys. J. 2009, 695, 59–74. [Google Scholar] [CrossRef]
- Akiyama, K.; Kino, M.; Sohn, B.; Lee, S.; Trippe, S.; Honma, M. Long-term monitoring of Sgr A* at 7 mm with VERA and KaVA. Proc. Int. Astron. Union 2013, 9, 288–292. [Google Scholar] [CrossRef]
- Johnson, M.D.; Fish, V.L.; Doeleman, S.S.; Broderick, A.E.; Wardle, J.F.C.; Marrone, D.P. Relative Astrometry of Compact Flaring Structures in Sgr A* with Polarimetric Very Long Baseline Interferometry. Astrophys. J. 2014, 794, 150. [Google Scholar] [CrossRef]
- Fish, V.L.; Johnson, M.D.; Lu, R.S.; Doeleman, S.S.; Bouman, K.L.; Zoran, D.; Freeman, W.T.; Psaltis, D.; Narayan, R.; Pankratius, V.; et al. Imaging an Event Horizon: Mitigation of Scattering toward Sagittarius A*. Astrophys. J. 2014, 795, 134. [Google Scholar] [CrossRef]
- Genzel, R.; Schödel, R.; Ott, T.; Eckart, A.; Alexander, T.; Lacombe, F.; Rouan, D.; Aschenbach, B. Near-infrared flares from accreting gas around the supermassive black hole at the Galactic Centre. Nature 2003, 425, 934–937. [Google Scholar] [CrossRef] [PubMed]
- Eckart, A.; Schödel, R.; Meyer, L.; Trippe, S.; Ott, T.; Genzel, R. Polarimetry of near-infrared flares from Sagittarius A*. Astron. Astrophys. 2006, 455, 1–10. [Google Scholar] [CrossRef]
- Do, T.; Witzel, G.; Gautam, A.K.; Chen, Z.; Ghez, A.M.; Morris, M.R.; Becklin, E.E.; Ciurlo, A.; Hosek, M., Jr.; Martinez, G.D.; et al. Unprecedented Near-infrared Brightness and Variability of Sgr A*. Astrophys. J. Lett. 2019, 882, L27. [Google Scholar] [CrossRef]
- Baganoff, F.K.; Bautz, M.W.; Brandt, W.N.; Chartas, G.; Feigelson, E.D.; Garmire, G.P.; Maeda, Y.; Morris, M.; Ricker, G.R.; Townsley, L.K.; et al. Rapid X-ray flaring from the direction of the supermassive black hole at the Galactic Centre. Nature 2001, 413, 45–48. [Google Scholar] [CrossRef] [PubMed]
- Porquet, D.; Predehl, P.; Aschenbach, B.; Grosso, N.; Goldwurm, A.; Goldoni, P.; Warwick, R.S.; Decourchelle, A. XMM-Newton observation of the brightest X-ray flare detected so far from Sgr A*. Astron. Astrophys. 2003, 407, L17–L20. [Google Scholar] [CrossRef]
- Andrés, A.; van den Eijnden, J.; Degenaar, N.; Evans, P.A.; Chatterjee, K.; Reynolds, M.; Miller, J.M.; Kennea, J.; Wijnands, R.; Markoff, S.; et al. A Swift study of long-term changes in the X-ray flaring properties of Sagittarius A. Mon. Not. R. Astron. Soc. 2022, 510, 2851–2863. [Google Scholar] [CrossRef]
- Haggard, D.; Nynka, M.; Mon, B.; de la Cruz Hernandez, N.; Nowak, M.; Heinke, C.; Neilsen, J.; Dexter, J.; Fragile, P.C.; Baganoff, F.; et al. Chandra Spectral and Timing Analysis of Sgr A*’s Brightest X-ray Flares. Astrophys. J. 2019, 886, 96. [Google Scholar] [CrossRef]
- Kusunose, M.; Takahara, F. Synchrotron Blob Model of Infrared and X-ray Flares from Sagittarius A*. Astrophys. J. 2011, 726, 54. [Google Scholar] [CrossRef]
- Karssen, G.D.; Bursa, M.; Eckart, A.; Valencia-S, M.; Dovčiak, M.; Karas, V.; Horák, J. Bright X-ray flares from Sgr A*. Mon. Not. R. Astron. Soc. 2017, 472, 4422–4433. [Google Scholar] [CrossRef]
- Yusef-Zadeh, F.; Bushouse, H.; Dowell, C.D.; Wardle, M.; Roberts, D.; Heinke, C.; Bower, G.C.; Vila-Vilaró, B.; Shapiro, S.; Goldwurm, A.; et al. A Multiwavelength Study of Sgr A*: The Role of Near-IR Flares in Production of X-ray, Soft γ-ray, and Submillimeter Emission. Astrophys. J. 2006, 644, 198–213. [Google Scholar] [CrossRef]
- Yusef-Zadeh, F.; Roberts, D.; Wardle, M.; Heinke, C.O.; Bower, G.C. Flaring Activity of Sagittarius A* at 43 and 22 GHz: Evidence for Expanding Hot Plasma. Astrophys. J. 2006, 650, 189–194. [Google Scholar] [CrossRef]
- Eckart, A.; Schödel, R.; García-Marín, M.; Witzel, G.; Weiss, A.; Baganoff, F.K.; Morris, M.R.; Bertram, T.; Dovčiak, M.; Duschl, W.J.; et al. Simultaneous NIR/sub-mm observation of flare emission from Sagittarius A*. Astron. Astrophys. 2008, 492, 337–344. [Google Scholar] [CrossRef]
- Eckart, A.; Baganoff, F.K.; Morris, M.R.; Kunneriath, D.; Zamaninasab, M.; Witzel, G.; Schödel, R.; García-Marín, M.; Meyer, L.; Bower, G.C.; et al. Modeling mm- to X-ray flare emission from Sagittarius A*. Astron. Astrophys. 2009, 500, 935–946. [Google Scholar] [CrossRef]
- Boyce, H.; Haggard, D.; Witzel, G.; Fellenberg, S.v.; Willner, S.P.; Becklin, E.E.; Do, T.; Eckart, A.; Fazio, G.G.; Gurwell, M.A.; et al. Multiwavelength Variability of Sagittarius A* in 2019 July. Astrophys. J. 2022, 931, 7. [Google Scholar] [CrossRef]
- Dexter, J.; Tchekhovskoy, A.; Jiménez-Rosales, A.; Ressler, S.M.; Bauböck, M.; Dallilar, Y.; de Zeeuw, P.T.; Eisenhauer, F.; von Fellenberg, S.; Gao, F.; et al. Sgr A* near-infrared flares from reconnection events in a magnetically arrested disc. Mon. Not. R. Astron. Soc. 2020, 497, 4999–5007. [Google Scholar] [CrossRef]
- Gravity Collaboration; Abuter, R.; Amorim, A.; Bauböck, M.; Berger, J.P.; Bonnet, H.; Brandner, W.; Clénet, Y.; Coudé Du Foresto, V.; de Zeeuw, P.T.; et al. Detection of orbital motions near the last stable circular orbit of the massive black hole SgrA*. Astron. Astrophys. 2018, 618, L10. [Google Scholar] [CrossRef]
- Wielgus, M.; Moscibrodzka, M.; Vos, J.; Gelles, Z.; Martí-Vidal, I.; Farah, J.; Marchili, N.; Goddi, C.; Messias, H. Orbital motion near Sagittarius A*. Constraints from polarimetric ALMA observations. Astron. Astrophys. 2022, 665, L6. [Google Scholar] [CrossRef]
- Yuan, F.; Narayan, R. Hot Accretion Flows Around Black Holes. Annu. Rev. Astron. Astrophys. 2014, 52, 529–588. [Google Scholar] [CrossRef]
- Dovčiak, M.; Karas, V.; Yaqoob, T. An Extended Scheme for Fitting X-ray Data with Accretion Disk Spectra in the Strong Gravity Regime. Astrophys. J. Suppl. Ser. 2004, 153, 205–221. [Google Scholar] [CrossRef]
- Broderick, A.E.; Loeb, A. Imaging bright-spots in the accretion flow near the black hole horizon of Sgr A*. Mon. Not. R. Astron. Soc. 2005, 363, 353–362. [Google Scholar] [CrossRef]
- Broderick, A.E.; Loeb, A. Imaging optically-thin hotspots near the black hole horizon of Sgr A* at radio and near-infrared wavelengths. Mon. Not. R. Astron. Soc. 2006, 367, 905–916. [Google Scholar] [CrossRef]
- Tiede, P.; Pu, H.Y.; Broderick, A.E.; Gold, R.; Karami, M.; Preciado-López, J.A. Spacetime Tomography Using the Event Horizon Telescope. Astrophys. J. 2020, 892, 132. [Google Scholar] [CrossRef]
- Vos, J.; Mościbrodzka, M.A.; Wielgus, M. Polarimetric signatures of hot spots in black hole accretion flows. Astron. Astrophys. 2022, 668, A185. [Google Scholar] [CrossRef]
- Rees, M.J.; Begelman, M.C.; Blandford, R.D.; Phinney, E.S. Ion-supported tori and the origin of radio jets. Nature 1982, 295, 17–21. [Google Scholar] [CrossRef]
- Narayan, R.; Yi, I. Advection-dominated Accretion: A Self-similar Solution. Astrophys. J. Lett. 1994, 428, L13. [Google Scholar] [CrossRef]
- Narayan, R.; Yi, I. Advection-dominated Accretion: Self-Similarity and Bipolar Outflows. Astrophys. J. 1995, 444, 231. [Google Scholar] [CrossRef]
- Porth, O.; Chatterjee, K.; Narayan, R.; Gammie, C.F.; Mizuno, Y.; Anninos, P.; Baker, J.G.; Bugli, M.; Chan, C.k.; Davelaar, J.; et al. The Event Horizon General Relativistic Magnetohydrodynamic Code Comparison Project. Astrophys. J. Suppl. Ser. 2019, 243, 26. [Google Scholar] [CrossRef]
- Narayan, R.; Igumenshchev, I.V.; Abramowicz, M.A. Magnetically Arrested Disk: An Energetically Efficient Accretion Flow. Publ. Astron. Soc. Jpn. 2003, 55, L69–L72. [Google Scholar] [CrossRef]
- Ripperda, B.; Liska, M.; Chatterjee, K.; Musoke, G.; Philippov, A.A.; Markoff, S.B.; Tchekhovskoy, A.; Younsi, Z. Black Hole Flares: Ejection of Accreted Magnetic Flux through 3D Plasmoid-mediated Reconnection. Astrophys. J. Lett. 2022, 924, L32. [Google Scholar] [CrossRef]
- Liska, M.T.P.; Chatterjee, K.; Issa, D.; Yoon, D.; Kaaz, N.; Tchekhovskoy, A.; van Eijnatten, D.; Musoke, G.; Hesp, C.; Rohoza, V.; et al. H-AMR: A New GPU-accelerated GRMHD Code for Exascale Computing with 3D Adaptive Mesh Refinement and Local Adaptive Time Stepping. Astrophys. J. Suppl. Ser. 2022, 263, 26. [Google Scholar] [CrossRef]
- Broderick, A.E.; Loeb, A. Testing General Relativity with High-Resolution Imaging of Sgr A*. J. Phys. Conf. Ser. 2006, 54, 448–455. [Google Scholar] [CrossRef]
- Meyer, L.; Eckart, A.; Schodel, R.; Duschl, W.J., 3rd; Dovciak, M.; Karas, V. A two component hot spot/ring model for the NIR flares of Sagittarius A*. J. Phys. Conf. Ser. 2006, 54, 443–447. [Google Scholar] [CrossRef]
- Meyer, L.; Eckart, A.; Schödel, R.; Dovčiak, M.; Karas, V.; Duschl, W.J. The orbiting spot model gives constraints on the parameters of the supermassive black hole in the Galactic Center. Proc. Int. Astron. Union 2006, 2, 407–408. [Google Scholar] [CrossRef]
- Zamaninasab, M.; Eckart, A.; Meyer, L.; Schödel, R.; Dovciak, M.; Karas, V.; Kunneriath, D.; Witzel, G.; Gießübel, R.; König, S.; et al. An evolving hot spot orbiting around Sgr A*. J. Phys. Conf. Ser. 2008, 131, 012008. [Google Scholar] [CrossRef]
- Broderick, A.E.; Fish, V.L.; Doeleman, S.S.; Loeb, A. Evidence for Low Black Hole Spin and Physically Motivated Accretion Models from Millimeter-VLBI Observations of Sagittarius A*. Astrophys. J. 2011, 735, 110. [Google Scholar] [CrossRef]
- Younsi, Z.; Wu, K. Variations in emission from episodic plasmoid ejecta around black holes. Mon. Not. R. Astron. Soc. 2015, 454, 3283–3298. [Google Scholar] [CrossRef]
- Nathanail, A.; Fromm, C.M.; Porth, O.; Olivares, H.; Younsi, Z.; Mizuno, Y.; Rezzolla, L. Plasmoid formation in global GRMHD simulations and AGN flares. Mon. Not. R. Astron. Soc. 2020, 495, 1549–1565. [Google Scholar] [CrossRef]
- Ripperda, B.; Bacchini, F.; Philippov, A.A. Magnetic Reconnection and Hot Spot Formation in Black Hole Accretion Disks. Astrophys. J. 2020, 900, 100. [Google Scholar] [CrossRef]
- Moriyama, K.; Mineshige, S.; Honma, M.; Akiyama, K. Black Hole Spin Measurement Based on Time-domain VLBI Observations of Infalling Gas Clouds. Astrophys. J. 2019, 887, 227. [Google Scholar] [CrossRef]
- Zamaninasab, M.; Eckart, A.; Witzel, G.; Dovciak, M.; Karas, V.; Schödel, R.; Gießübel, R.; Bremer, M.; García-Marín, M.; Kunneriath, D.; et al. Near infrared flares of Sagittarius A*. Importance of near infrared polarimetry. Astron. Astrophys. 2010, 510, A3. [Google Scholar] [CrossRef]
- Roelofs, F.; Blackburn, L.; Lindahl, G.; Doeleman, S.S.; Johnson, M.D.; Arras, P.; Chatterjee, K.; Emami, R.; Fromm, C.; Fuentes, A.; et al. The ngEHT Analysis Challenges. Galaxies 2022, 11, 12. [Google Scholar] [CrossRef]
- Broderick, A.E.; Fish, V.L.; Johnson, M.D.; Rosenfeld, K.; Wang, C.; Doeleman, S.S.; Akiyama, K.; Johannsen, T.; Roy, A.L. Modeling Seven Years of Event Horizon Telescope Observations with Radiatively Inefficient Accretion Flow Models. Astrophys. J. 2016, 820, 137. [Google Scholar] [CrossRef]
- Bouman, K.L.; Johnson, M.D.; Dalca, A.V.; Chael, A.A.; Roelofs, F.; Doeleman, S.S.; Freeman, W.T. Reconstructing Video from Interferometric Measurements of Time-Varying Sources. arXiv 2017, arXiv:1711.01357. [Google Scholar] [CrossRef]
- Raymond, A.W.; Palumbo, D.; Paine, S.N.; Blackburn, L.; Córdova Rosado, R.; Doeleman, S.S.; Farah, J.R.; Johnson, M.D.; Roelofs, F.; Tilanus, R.P.J.; et al. Evaluation of New Submillimeter VLBI Sites for the Event Horizon Telescope. Astrophys. J. Suppl. Ser. 2021, 253, 5. [Google Scholar] [CrossRef]
- Palumbo, D.; Johnson, M.; Doeleman, S.; Chael, A.; Bouman, K. Next-Generation Event Horizon Telescope Developments: New Stations for Enhanced Imaging. American Astronomical Society Meeting Abstracts 2018. Volume 231, p. 347.21. Available online: https://ui.adsabs.harvard.edu/abs/2018AAS...23134721P/abstract (accessed on 1 January 2018).
- Chael, A.; Bouman, K.; Johnson, M.; Blackburn, L.; Shiokawa, H. Eht-Imaging: Tools For Imaging and Simulating Vlbi Data. Zenodo 2018. [Google Scholar] [CrossRef]
- Chael, A.; Chan, C.K.; Klbouman; Wielgus, M.; Farah, J.R.; Palumbo, D.; Blackburn, L.; Aviad; Dpesce; Quarles, G.; et al. achael/eht-imaging: V1.2.4. Zenodo. 2022. Available online: https://doi.org/10.5281/zenodo.6519440 (accessed on 12 November 2022).
- Johnson, M.D.; Narayan, R.; Psaltis, D.; Blackburn, L.; Kovalev, Y.Y.; Gwinn, C.R.; Zhao, G.Y.; Bower, G.C.; Moran, J.M.; Kino, M.; et al. The Scattering and Intrinsic Structure of Sagittarius A* at Radio Wavelengths. Astrophys. J. 2018, 865, 104. [Google Scholar] [CrossRef]
- Event Horizon Telescope Collaboration; Akiyama, K.; Alberdi, A.; Alef, W.; Asada, K.; Azulay, R.; Baczko, A.K.; Ball, D.; Baloković, M.; Barrett, J.; et al. First M87 Event Horizon Telescope Results. IV. Imaging the Central Supermassive Black Hole. Astrophys. J. Lett. 2019, 875, L4. [Google Scholar] [CrossRef]




| Array | Sites Used for Simulated Data | |||||||
|---|---|---|---|---|---|---|---|---|
| EHT(2017) | ALMA | APEX | SMA | JCMT | SMT | LMT | PV | SPT |
| EHT(2022) | EHT(2017)+ | KP | NOEMA | |||||
| ngEHTp1 | EHT(2022)+ | OVRO | HAY | CNI | BAJA | LAS | ||
| ngEHT | ngEHTp1+ | GARS | GAM | CAT | BOL | BRZ | ||
| Obs | Bs | logcam | cphase | |||
|---|---|---|---|---|---|---|
| EHT(2017) | 1.0 | 1.0 | 1.0 | 0.67 | 1.16 | 1.37 |
| EHT(2022) | 1.0 | 1.4 | 1.7 | 0.59 | 0.63 | 0.77 |
| ngEHTp1 | 1.2 | 1.5 | 1.5 | 1.14 | 1.5 | 1.84 |
| ngEHT | 1.0 | 1.0 | 1.0 | 1.17 | 1.51 | 1.90 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Emami, R.; Tiede, P.; Doeleman, S.S.; Roelofs , F.; Wielgus , M.; Blackburn , L.; Liska , M.; Chatterjee , K.; Ripperda, B.; Fuentes , A.; et al. Tracing Hot Spot Motion in Sagittarius A* Using the Next-Generation Event Horizon Telescope (ngEHT). Galaxies 2023, 11, 23. https://doi.org/10.3390/galaxies11010023
Emami R, Tiede P, Doeleman SS, Roelofs F, Wielgus M, Blackburn L, Liska M, Chatterjee K, Ripperda B, Fuentes A, et al. Tracing Hot Spot Motion in Sagittarius A* Using the Next-Generation Event Horizon Telescope (ngEHT). Galaxies. 2023; 11(1):23. https://doi.org/10.3390/galaxies11010023
Chicago/Turabian StyleEmami, Razieh, Paul Tiede, Sheperd S. Doeleman, Freek Roelofs , Maciek Wielgus , Lindy Blackburn , Matthew Liska , Koushik Chatterjee , Bart Ripperda, Antonio Fuentes , and et al. 2023. "Tracing Hot Spot Motion in Sagittarius A* Using the Next-Generation Event Horizon Telescope (ngEHT)" Galaxies 11, no. 1: 23. https://doi.org/10.3390/galaxies11010023
APA StyleEmami, R., Tiede, P., Doeleman, S. S., Roelofs , F., Wielgus , M., Blackburn , L., Liska , M., Chatterjee , K., Ripperda, B., Fuentes , A., Broderick, A. E., Hernquist , L., Alcock , C., Narayan, R., Smith , R., Tremblay, G., Ricarte, A., Sun, H., Anantua , R., ... Vogelsberger, M. (2023). Tracing Hot Spot Motion in Sagittarius A* Using the Next-Generation Event Horizon Telescope (ngEHT). Galaxies, 11(1), 23. https://doi.org/10.3390/galaxies11010023






