Cylindrical Gravastar Like-Structures in f(G) Gravity
Abstract
1. Introduction
- Inner sector ,
- Intermediate thin shell ,
- Outer sector .
2. Theory and Revised Field Equations
3. Composition of Gravastar
3.1. Zone (I)
3.2. Zone (II)
3.3. Zone (III)
4. Junction Conditions
5. Significant Attributes of Gravastar
5.1. Length of Shell
5.1.1. Energy Content
5.1.2. Entropy
5.1.3. Equation of State
6. Conclusions
- Characterization of density and pressure: (i) In the interior sector of gravastar, negative pressure will maintain its nature. Moreover, the values of pressure and energy density will be persistent. (ii) Figure 1 indicates the change in pressure of ultra relativistic fluid within the shell related to r which is the radial coordinate. Therefore, we can assume that the outer edge of shell will have more density than the inner one.
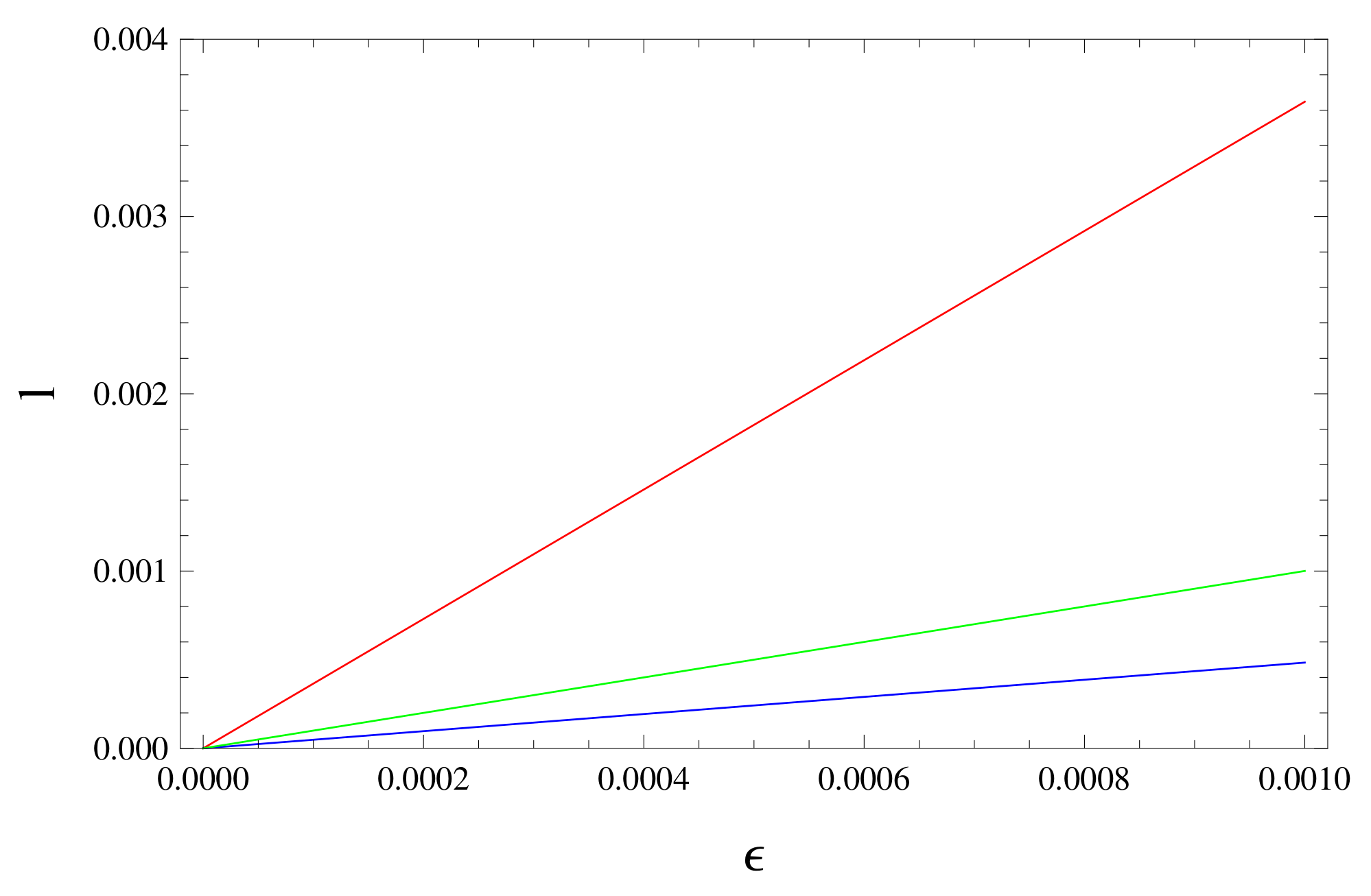
- Length of shell: We have examined the relation between of length of intermediate shell and shell thickness under the presence of constructive matter. Graph (Figure 2) between these two physical characteristics of model shows the continuous increase in length with the increase in its thickness.
- Entropy: (i) The area of event horizon of BH is used in order to determine its entropy. Because there is no event horizon in the case of gravastar, its entropy will be dependent on thickness of shell. (ii) Figure 3 specifies that by the increase (decrease) in shell thickness its entropy will also increase (decrease).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Nakao, K.I.; Yoo, C.M.; Harada, T. Gravastar formation: What can be the evidence of a black hole? Phys. Rev. D 2019, 99, 044027. [Google Scholar] [CrossRef]
- Arbañil, J.D.; Panotopoulos, G. Tidal deformability and radial oscillations of anisotropic polytropic spheres. Phys. Rev. D 2022, 105, 024008. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, Á.; Lopes, I. Slowly rotating dark energy stars. Phys. Dark Universe 2021, 34, 100885. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Tangphati, T.; Banerjee, A.; Jasim, M. Anisotropic quark stars in R2 gravity. Phys. Lett. B 2021, 817, 136330. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, Á.; Lopes, I. Interior solutions of relativistic stars with anisotropic matter in scale-dependent gravity. Eur. Phys. J. C 2021, 81, 63. [Google Scholar] [CrossRef]
- Tello-Ortiz, F.; Rincón, Á.; Bhar, P.; Gomez-Leyton, Y. Durgapal IV model considering the minimal geometric deformation approach. Chin. Phys. C 2020, 44, 105102. [Google Scholar] [CrossRef]
- Tello-Ortiz, F.; Malaver, M.; Rincón, Á.; Gomez-Leyton, Y. Relativistic anisotropic fluid spheres satisfying a non-linear equation of state. Eur. Phys. J. C 2020, 80, 371. [Google Scholar] [CrossRef]
- Mazur, P.O.; Mottola, E. Gravitational vacuum condensate stars. Proc. Natl. Acad. Sci. USA 2004, 101, 9545–9550. [Google Scholar] [CrossRef]
- Chirenti, C.B.; Rezzolla, L. How to tell a gravastar from a black hole. Class. Quantum Grav. 2007, 24, 4191. [Google Scholar] [CrossRef]
- Zeldovich, Y.B. A hypothesis, unifying the structure and the entropy of the Universe. Mon. Not. R. Astron. Soc. 1972, 160, 1P–3P. [Google Scholar] [CrossRef]
- Lobo, F.S.N.; Garattini, R. Linearized stability analysis of gravastars in noncommutative geometry. J. High Energy Phys. 2013, 2013, 65. [Google Scholar] [CrossRef]
- Nandi, K.K.; Zhang, Y.Z.; Cai, R.G.; Panchenko, A. Energetics in condensate star and wormholes. Phys. Rev. D 2009, 79, 024011. [Google Scholar] [CrossRef]
- Horvat, D.; Ilijić, S. Gravastar energy conditions revisited. Class. Quantum Grav. 2007, 24, 5637. [Google Scholar] [CrossRef]
- Furey, N.; DeBenedictis, A. Wormhole throats in Rm gravity. Class. Quantum Grav. 2004, 22, 313. [Google Scholar] [CrossRef]
- DeBenedictis, A.; Horvat, D.; Ilijić, S.; Kloster, S.; Viswanathan, K.S. Gravastar solutions with continuous pressures and equation of state. Class. Quantum Grav. 2006, 23, 2303. [Google Scholar] [CrossRef]
- Bilić, N.; Tupper, G.B.; Viollier, R.D. Born–Infeld phantom gravastars. J. Cosmol. Astropart. Phys. 2006, 2006, 013. [Google Scholar] [CrossRef]
- Visser, M.; Wiltshire, D.L. Stable gravastars an alternative to black holes? Class. Quantum Grav. 2004, 21, 1135. [Google Scholar] [CrossRef]
- Lobo, F.S.N.; Arellano, A.V.B. Gravastars supported by nonlinear electrodynamics. Class. Quantum Grav. 2007, 24, 1069. [Google Scholar] [CrossRef]
- Rocha, P.; Chan, R.; da Silva, M.; Wang, A. Stable and bounded excursion gravastars, and black holes in Einstein s theory of gravity. J. Cosmol. Astropart. Phys. 2008, 2008, 010. [Google Scholar] [CrossRef]
- Bhatti, M.Z.; Yousaf, Z.; Ajmal, M. Locally isotropic gravastars with cylindrical spacetime. Int. J. Mod. Phys. A 2019, 28, 1950123. [Google Scholar] [CrossRef]
- Bhatti, M.Z. Charged gravastars with cylindrical spacetime. Mod. Phys. Lett. A 2020, 35, 2050069. [Google Scholar] [CrossRef]
- Cattoen, C.; Faber, T.; Visser, M. Gravastars must have anisotropic pressures. Class. Quantum Grav. 2005, 22, 4189. [Google Scholar] [CrossRef]
- Bhatti, M.Z.; Yousaf, Z.; Rehman, A. Gravastars in f (R, G) gravity. Phys. Dark Universe 2020, 29, 100561. [Google Scholar] [CrossRef]
- Yousaf, Z.; Bhatti, M.Z.; Rehman, A. Electrically charged string-like axially symmetric object composition in f (R, G) gravity. Chin. J. Phys. 2021, 73, 493–502. [Google Scholar] [CrossRef]
- Horvat, D.; Ilijić, S.; Marunović, A. Radial stability analysis of the continuous pressure gravastar. Class. Quantum Grav 2011, 28, 195008. [Google Scholar] [CrossRef]
- Sakai, N.; Saida, H.; Tamaki, T. Gravastar shadows. Phys. Rev. D 2014, 90, 104013. [Google Scholar] [CrossRef]
- Rahaman, F.; Usmani, A.A.; Ray, S.; Islam, S. The (2+ 1)-dimensional charged gravastars. Phys. Lett. B 2012, 717, 1–5. [Google Scholar] [CrossRef]
- Pani, P.; Berti, E.; Cardoso, V.; Chen, Y.; Norte, R. Gravitational wave signatures of the absence of an event horizon: Nonradial oscillations of a thin-shell gravastar. Phys. Rev. D 2009, 80, 124047. [Google Scholar] [CrossRef]
- Yousaf, Z. Spatially Hyperbolic Gravitating Sources in Λ-Dominated Era. Universe 2022, 8, 131. [Google Scholar] [CrossRef]
- Yousaf, Z. Construction of charged cylindrical gravastar-like structures. Phys. Dark Universe 2020, 28, 100509. [Google Scholar] [CrossRef]
- Chirenti, C.B.; Rezzolla, L. Ergoregion instability in rotating gravastars. Phys. Rev. D 2008, 78, 084011. [Google Scholar] [CrossRef]
- Iorio, L. Editorial for the special issue 100 years of chronogeometrodynamics: The status of the Einstein s theory of gravitation in its centennial year. Universe 2015, 1, 38–81. [Google Scholar] [CrossRef]
- Debono, I.; Smoot, G.F. General relativity and cosmology: Unsolved questions and future directions. Universe 2016, 2, 23. [Google Scholar] [CrossRef]
- Vishwakarma, R.G. Einstein and beyond: A critical perspective on general relativity. Universe 2016, 2, 11. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Modified Gauss–Bonnet theory as gravitational alternative for dark energy. Phys. Lett. B 2005, 631, 1–6. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Future evolution and finite-time singularities in F (R) gravity unifying inflation and cosmic acceleration. Phys. Rev. D 2008, 78, 046006. [Google Scholar] [CrossRef]
- Lin, H.Y.; Deng, X.M. Rational orbits around 4D Einstein–Lovelock black holes. Phys. Dark Universe 2021, 31, 100745. [Google Scholar] [CrossRef]
- Deng, X.M.; Xie, Y. Study on an improved adaptive PSO algorithm for solving multi-objective gate assignment. Astrophys. Space Sci. 2017, 362, 1. [Google Scholar] [CrossRef]
- Bhatti, M.Z.; Yousaf, Z.; Khadim, A. Dynamical analysis of self-gravitating stars in modified Gauss-Bonnet gravity. Phys. Rev. D 2020, 101, 104029. [Google Scholar] [CrossRef]
- Easson, D.A. Modified gravitational theories and cosmic acceleration. Int. J. Mod. Phys. A 2004, 19, 5343–5350. [Google Scholar] [CrossRef]
- Neupane, I.P.; Carter, B.M. Towards inflation and dark energy cosmologies from modified Gauss–Bonnet theory. J. Cosmol. Astropart. Phys. 2006, 2006, 004. [Google Scholar] [CrossRef]
- Bhatti, M.Z.; Yousaf, Z. Dynamical variables and evolution of the universe. Int. J. Mod. Phys. D 2017, 26, 1750029. [Google Scholar] [CrossRef]
- Sharif, M.; Fatima, H.I. Thermodynamics with corrected entropies in f(G) gravity. Astrophys. Space Sci. 2014, 354, 507–515. [Google Scholar] [CrossRef]
- Cognola, G.; Elizalde, E.; Nojiri, S.I.; Odintsov, S.D.; Zerbini, S. String-inspired Gauss-Bonnet gravity reconstructed from the universe expansion history and yielding the transition from matter dominance to dark energy. Phys. Rev. D 2007, 75, 086002. [Google Scholar] [CrossRef]
- Sadjadi, H.M. On the second law of thermodynamics in modified Gauss–Bonnet gravity. Phys. Scr. 2011, 83, 055006. [Google Scholar] [CrossRef][Green Version]
- Chatterjee, S.; Parikh, M. The second law in four-dimensional Einstein–Gauss–Bonnet gravity. Class. Quantum Gravity 2014, 31, 155007. [Google Scholar] [CrossRef]
- Bhatti, M.Z.; Yousaf, Z. Dynamical instability of charged self-gravitating stars in modified gravity. Chin. J. Phys. 2021, 73, 115–135. [Google Scholar] [CrossRef]
- Li, B.; Barrow, J.D.; Mota, D.F. Cosmology of modified Gauss-Bonnet gravity. Phys. Rev. D 2007, 76, 044027. [Google Scholar] [CrossRef]
- Sharif, M.; Azam, M. Stability analysis of thin-shell wormholes from charged black string. J. Cosmol. Astropart. Phys. 2013, 2013, 023. [Google Scholar] [CrossRef]
- Madsen, M.S.; Mimoso, J.P. Evolution of the density parameter in inflationary cosmology reexamined. Phys. Rev. D 1992, 46, 1399. [Google Scholar] [CrossRef]
- Braje, T.M.; Romani, R.W. RX J1856–3754: Evidence for a stiff equation of state. Astrophys. J. 2002, 580, 1043. [Google Scholar] [CrossRef]
- Linares, L.P.; Malheiro, M.; Ray, S. The importance of the relativistic corrections in hyperon stars. Int. J. Mod. Phys. D 2004, 13, 1355. [Google Scholar] [CrossRef]
- Darmois, G. Les Equation de la Gravitation Einsteinnienne Memorial des Science Mathematiques Fasc; Gauthier-Villars: Paris, France, 1927; p. 25. [Google Scholar]
- Israel, W. Singular hypersurfaces and thin shells in general relativity. Il Nuovo Cimento B 1966, 44, 1. [Google Scholar] [CrossRef]
- Lanczos, K. Flächenhafte verteilung der materie in der einsteinschen gravitationstheorie. Ann. Phys. 1924, 379, 518–540. [Google Scholar] [CrossRef]
- Sen, N. Über die grenzbedingungen des schwerefeldes an unstetigkeitsflächen. Ann. Phys. 1924, 378, 365–396. [Google Scholar] [CrossRef]
- Rahaman, F.; Kalam, M.; Chakraborty, S. Thin shell wormholes in higher dimensional Einstein Maxwell theory. Gen. Relativ. Gravit. 2006, 38, 1687–1695. [Google Scholar] [CrossRef]
- Rahaman, F.; Rahman, K. Wormholes supported by polytropic phantom energy. Int. J. Theor. Phys 2010, 49, 2364. [Google Scholar] [CrossRef]
- Dias, G.A.S.; Lemos, J.P.S. Thin shell wormholes in d dimensional general relativity: Solutions, properties, and stability. Phys. Rev. D 2010, 82, 084023. [Google Scholar] [CrossRef]
- Rahaman, F.; Kuhfittig, P.K.F.; Kalam, M.; Usmani, A.A.; Ray, S. A comparison of Hořava–Lifshitz gravity and Einstein gravity through thin-shell wormhole construction. Class. Quantum Grav. 2011, 28, 155021. [Google Scholar] [CrossRef]
- Bhar, P. Higher dimensional charged gravastar admitting conformal motion. Astrophys. Space Sci. 2014, 354, 457–462. [Google Scholar] [CrossRef]
- Heiselberg, H.; Pandharipande, V. Recent progress in neutron star theory. Annu. Rev. Nucl. Part. Sci. 2000, 50, 481–524. [Google Scholar] [CrossRef]
- Lattimer, J.M.; Prakash, M. The equation of state of hot, dense matter and neutron stars. Phys. Rep. 2016, 621, 127–164. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhatti, M.Z.; Yousaf, Z.; Rehman, A. Cylindrical Gravastar Like-Structures in f(G) Gravity. Galaxies 2022, 10, 40. https://doi.org/10.3390/galaxies10020040
Bhatti MZ, Yousaf Z, Rehman A. Cylindrical Gravastar Like-Structures in f(G) Gravity. Galaxies. 2022; 10(2):40. https://doi.org/10.3390/galaxies10020040
Chicago/Turabian StyleBhatti, M. Z., Z. Yousaf, and A. Rehman. 2022. "Cylindrical Gravastar Like-Structures in f(G) Gravity" Galaxies 10, no. 2: 40. https://doi.org/10.3390/galaxies10020040
APA StyleBhatti, M. Z., Yousaf, Z., & Rehman, A. (2022). Cylindrical Gravastar Like-Structures in f(G) Gravity. Galaxies, 10(2), 40. https://doi.org/10.3390/galaxies10020040







