A Vibration Suppression Method for the Multistage Rotor of an Aero-Engine Based on Assembly Optimization
Abstract
1. Introduction
- The mathematical relationship between the assembly angles of the rotors at all stages and the vibration responses should be established;
- The vibration responses of the critical nodes on the rotor should be taken as the optimization objective to calculate the optimal assembly angles.
2. Methods
2.1. Single-Node Dynamics Model for a Rotor with Geometric and Mass Eccentricities
2.2. Dynamics Model of the Shaft Element of a Rotor
2.3. Error Transfer Model of the Geometric and Mass Eccentricities
2.3.1. Coordinate Transfer
2.3.2. Decomposition of the Geometric and Mass Eccentricities Errors
2.4. Dynamics Model of a Multistage Rotor System
2.5. Solution Method of the Nodal Vibration Responses
2.6. Optimization Objective
3. Results
3.1. Simulation
3.1.1. Finite Element Model of a 4-Stage Rotor
3.1.2. The Machined Rotor According to the 4-Stage Rotor
3.1.3. Optimization of the Assembly Angles
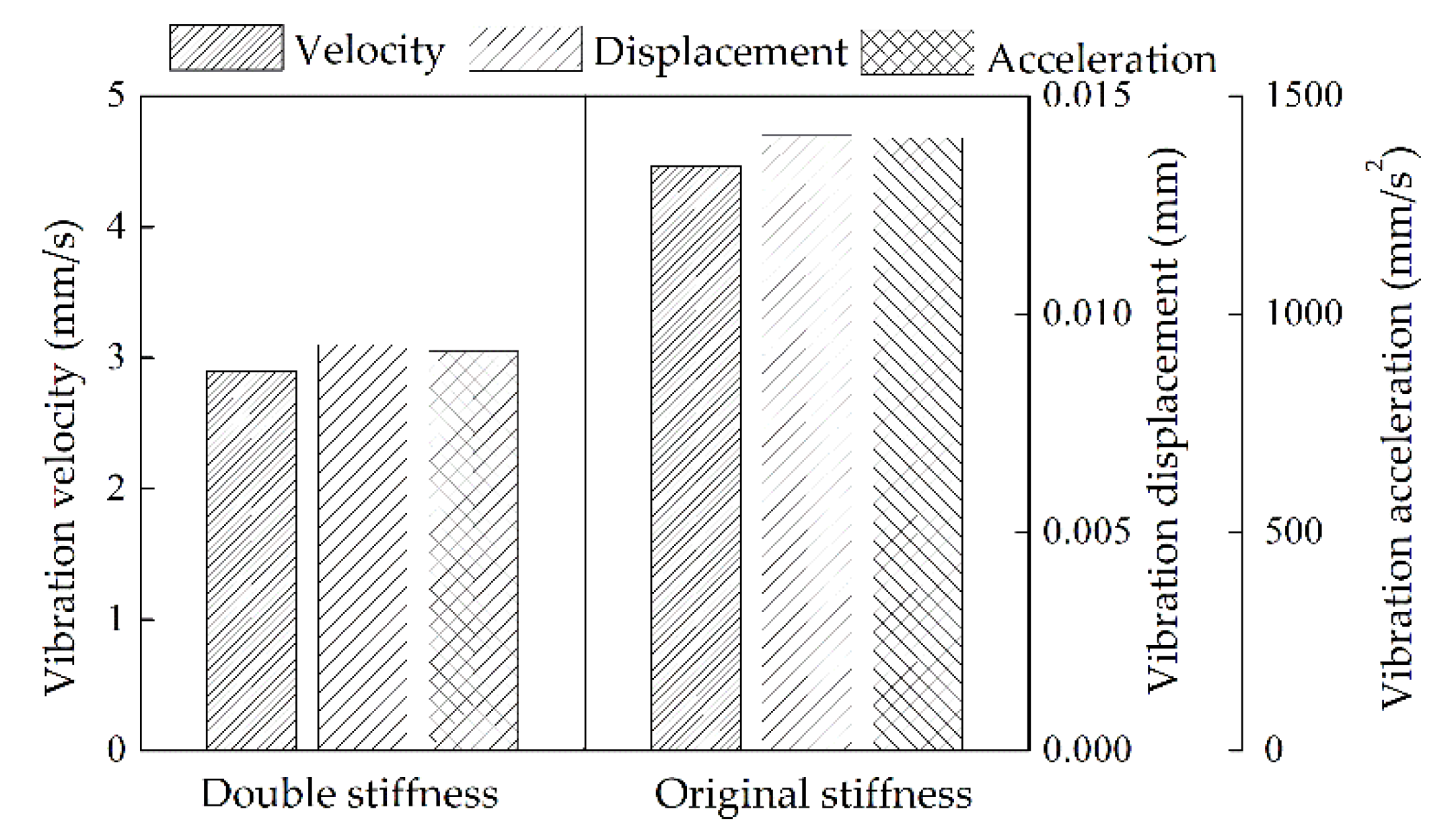
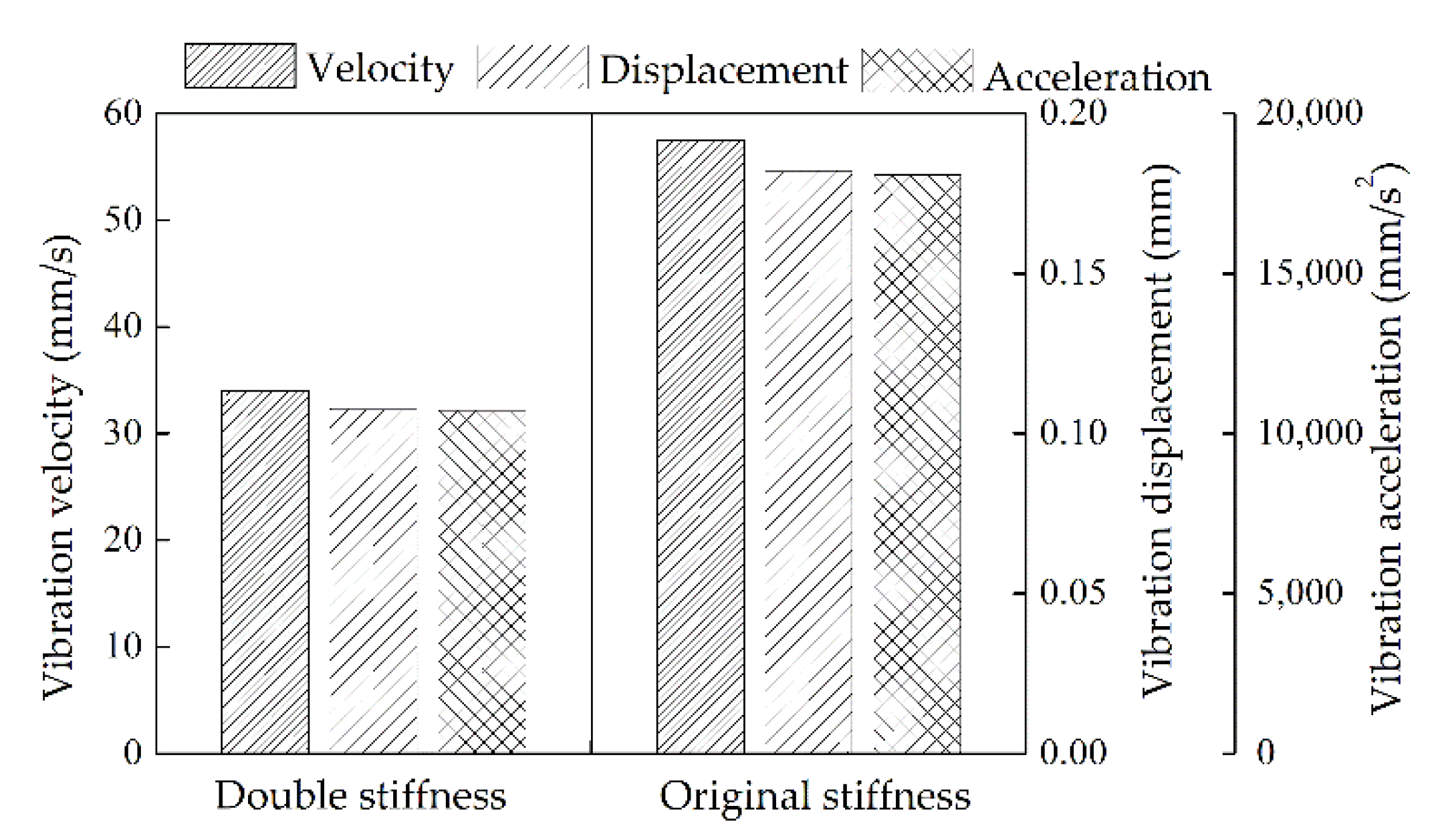
3.1.4. Effects of Stiffness and External Force on the Vibration Responses
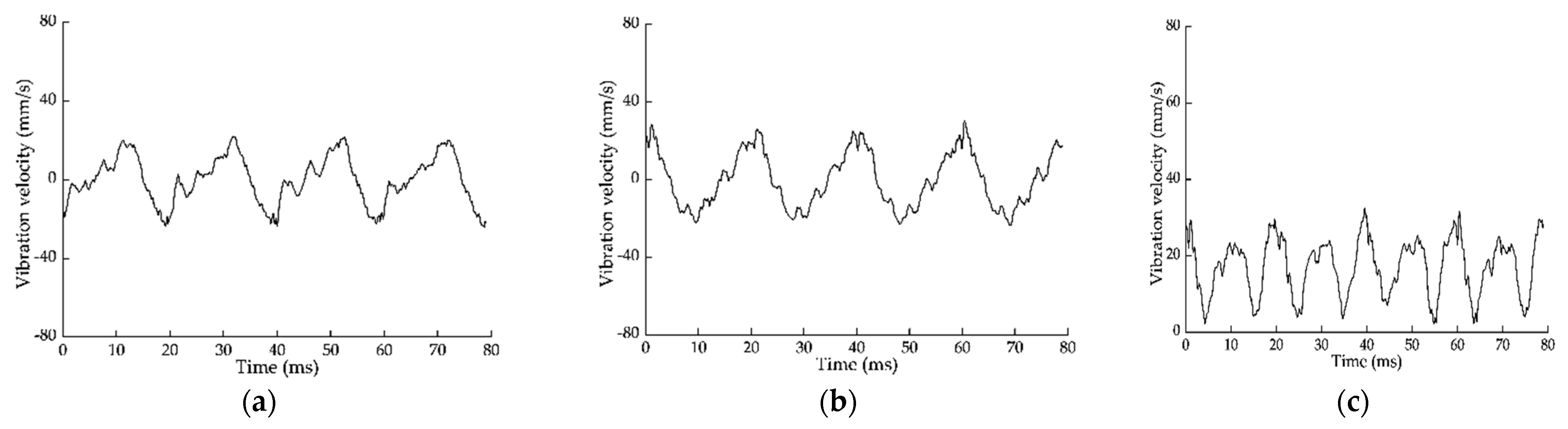
3.2. Experimental Verification
4. Discussion
5. Conclusions
- The mathematical relationship between the assembly angles of the rotors at all stages and the nodal vibration responses was established by combining the error transfer model of the geometric and mass eccentricities with the dynamics model of the multistage rotor system.
- An optimization function was developed, which takes the assembly angles as the optimization variables and the maximum vibration velocity at the bearings as the optimization objective. Then, the optimal assembly angles that can minimize the maximum vibration velocity at the bearings were calculated in the simulation.
- The experimental results showed that the maximum vibration velocity at the bearings of the four-stage rotor system under the optimal assembly was reduced by 69.6% and 45.5% compared with that under the worst assembly and default assembly.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Element | ln [mm] | Dn [mm] | dn [mm] |
|---|---|---|---|
| 1 | 2 | 20 | 0 |
| 2 | 16.5 | 33 | 0 |
| 3 | 5 | 33 | 0 |
| 4 | 3.5 | 33 | 0 |
| 5 | 8 | 35 | 0 |
| 6 | 5 | 35 | 0 |
| 7 | 2 | 39 | 0 |
| 8 | 2 | 42 | 0 |
| 9 | 26 | 42 | 27 |
| 10 | 24 | 42 | 27 |
| 11 | 3 | 58 | 36 |
| 12 | 3 | 58 | 0 |
| 13 | 8 | 82 | 0 |
| 14 | 15 | 192 | 0 |
| 15 | 5 | 82 | 0 |
| 16 | 3 | 82 | 0 |
| 17 | 3.5 | 82 | 54 |
| 18 | 24 | 82 | 72 |
| 19 | 50 | 82 | 72 |
| 20 | 3.5 | 102 | 72 |
| 21 | 3 | 110 | 72 |
| 22 | 7 | 110 | 74 |
| 23 | 19.5 | 82 | 74 |
| 24 | 3.5 | 82 | 56 |
| 25 | 3 | 128 | 56 |
| 26 | 10 | 128 | 48 |
| 27 | 34 | 128 | 112 |
| 28 | 46 | 128 | 112 |
| 29 | 7 | 128 | 48 |
| 30 | 5 | 128 | 50 |
| 31 | 3 | 128 | 33 |
| 32 | 6.5 | 68 | 33 |
| 33 | 30 | 42 | 33 |
| 34 | 20.5 | 42 | 33 |
| 35 | 11 | 42 | 0 |
| 36 | 2 | 39 | 0 |
| 37 | 5 | 35 | 0 |
| 38 | 8 | 35 | 0 |
| 39 | 3.5 | 33 | 0 |
| 40 | 5 | 33 | 0 |
| 41 | 16.5 | 33 | 0 |
References
- Chen, G. Vibration modelling and verifications for whole aero-engine. J. Sound Vib. 2015, 349, 163–176. [Google Scholar] [CrossRef]
- Cheng, W.; Guang, C. Wavelet correlation feature scale entropy and fuzzy support vector machine approach for aeroengine whole-body vibration fault diagnosis. Shock. Vib. 2013, 20, 341–349. [Google Scholar]
- Masrol, S.; Madlan, M.; Salleh, S. Vibration Analysis of Unbalanced and Misaligned Rotor System Using Laser Vibrometer. Appl. Mech. Mater. 2013, 315, 681–685. [Google Scholar] [CrossRef]
- Didier, J.; Sinou, J.; Faverjon, B. Study of the non-linear dynamic response of a rotor system with faults and uncertainties. J. Sound. Vib. 2012, 331, 671–703. [Google Scholar] [CrossRef]
- Ramlau, R.; Dicken, V.; Maa, P. Inverse imbalance reconstruction in rotordynamics. Zamm. J. Appl. Math. Mec. 2010, 86, 385–399. [Google Scholar] [CrossRef]
- Grobler, H.; Bright, G.; Loubser, R. Vibration analysis of a rotor by considering misalignment and unbalance, 18th ICSV. Sound Vibr. 2011, 3, 2151–2158. [Google Scholar]
- Juan, C.; Sanchez, L.; Hernandez, I. Classification of unbalance and misalignment in induction motors using orbital analysis and associative memories. Neurocomputing 2016, 175, 838–850. [Google Scholar]
- Hussain, T.; Yang, Z.; Popov, A.A. Straight-build assembly optimization: A method to minimize stage-by-stage eccentricity error in the assembly of axisymmetric rigid components (two-dimensional case study). J. Manuf. Sci. Eng. 2011, 133, 031014. [Google Scholar] [CrossRef]
- Hussain, T.; Mcwilliam, S.; Popov, A.A. Geometric error reduction in the assembly of axis-symmetric rigid components: A two-dimensional case study. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2012, 226, 1259–1274. [Google Scholar] [CrossRef]
- Hussain, T.; Yasinshaikh, G.; Shaikh, S.A. Variation propagation control in straight-build assemblies: 2d case study. Mehran Univ. Res. J. Eng. Technol. 2013, 32, 71–80. [Google Scholar]
- Zhang, Y.; Hussian, T.; Popov, A.A. Novel optimization technique for variation propagation control in an aero-engine assembly. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2010, 225, 100–111. [Google Scholar]
- Zhang, Y.; Popov, A.A.; Mcwilliam, S. Variation propagation control in mechanical assembly of cylindrical components. J. Manuf. Syst. 2012, 31, 162–176. [Google Scholar]
- Zhang, Y.; Hussian, T.; Popov, A.A. A comparison of different optimization techniques for variation propagation control in mechanical assembly. IOP Conf. 2011, 26, 012017. [Google Scholar]
- Zhang, Y.; Popov, A.A.; Mcwilliam, S.; Hussian, T. Dimensional variation propagation analysis in straight-build mechanical assemblies using a probabilistic approach. J. Manuf. Syst. 2013, 32, 348–356. [Google Scholar]
- Sun, J.; Ding, S.; Li, Z. Point-based solution using Jacobian-Torsor theory into partial parallel chains for revolving components assembly. J. Manuf. Syst. 2017, 46, 46–58. [Google Scholar]
- Sun, Y.; Guo, J.; Hong, J. Repair decision based on sensitivity analysis for aero-engine assembly. Int. J Precis. Eng. Man. 2019, 20, 347–362. [Google Scholar] [CrossRef]
- Wang, L.; Sun, C.; Tan, J. Improvement of location and orientation tolerances propagation control in cylindrical components assembly using stack-build assembly technique. Assem. Autom. 2015, 34, 358–366. [Google Scholar] [CrossRef]
- Sun, C.; Li, C.; Liu, Y. Prediction method of concentricity and perpendicularity of aero engine multistage rotors based on PSO-BP neural network. IEEE Access 2019, 7, 132271–132278. [Google Scholar] [CrossRef]
- Ganine, V.; Laxalde, D.; Michalska, H.; Pierre, C. Parameterized reduced order modeling of misaligned stacked disks rotor assemblies. J. Sound Vib. 2011, 330, 445–460. [Google Scholar] [CrossRef][Green Version]
- Liu, Y.; Zhang, M.; Sun, C. A method to minimize stage-by-stage initial unbalance in the aero engine assembly of multistage rotors. Aerosp. Sci. Technol. 2018, 85, 270–276. [Google Scholar] [CrossRef]
- Piskin, A.; Aktas, H.E.; Topal, A. Rotor balancing with turbine blade assembly using ant colony optimization for aero-engine applications. Int. J. Turbo Jet-Engines 2017, 8, 60. [Google Scholar] [CrossRef]
- Chen, Y.; Cui, J.; Sun, X. An unbalance optimization method for a multi-Stage rotor based on an assembly error propagation model. Appl. Sci. 2021, 11, 887. [Google Scholar] [CrossRef]
- Sun, C.; Liu, Z.; Liu, Y. An adjustment method of geometry and mass centers for precision rotors assembly. IEEE Access 2019, 7, 169992–170002. [Google Scholar] [CrossRef]
- Chen, Y.; Cui, J.; Sun, X. Research on multistage rotor assembly optimization methods for aeroengine based on the genetic algorithm. Complexity 2021, 2021, 8847690. [Google Scholar] [CrossRef]
- Chen, Y.; Cui, J.; Sun, X. An assembly method for the multistage rotor of an aero-engine based on the dual objective synchronous optimization for the coaxality and unbalance. Aerospace 2021, 8, 94. [Google Scholar] [CrossRef]
- Genta, G. Dynamics of Rotating Systems; Springer: New York, NY, USA, 2005; pp. 160–184. [Google Scholar]
- Guang, Z.; Yi, S.; Zhan, L. Application of a forecasting coupling method to the non-linear dynamic analysis of a flexible rotor supported by externally pressurized orifices hybrid gas bearings. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2011, 225, 704–717. [Google Scholar] [CrossRef]
- Tang, Y. Advanced Structural Dynamics; Tianjin University Press: Tianjin, China, 2002; pp. 115–119. [Google Scholar]

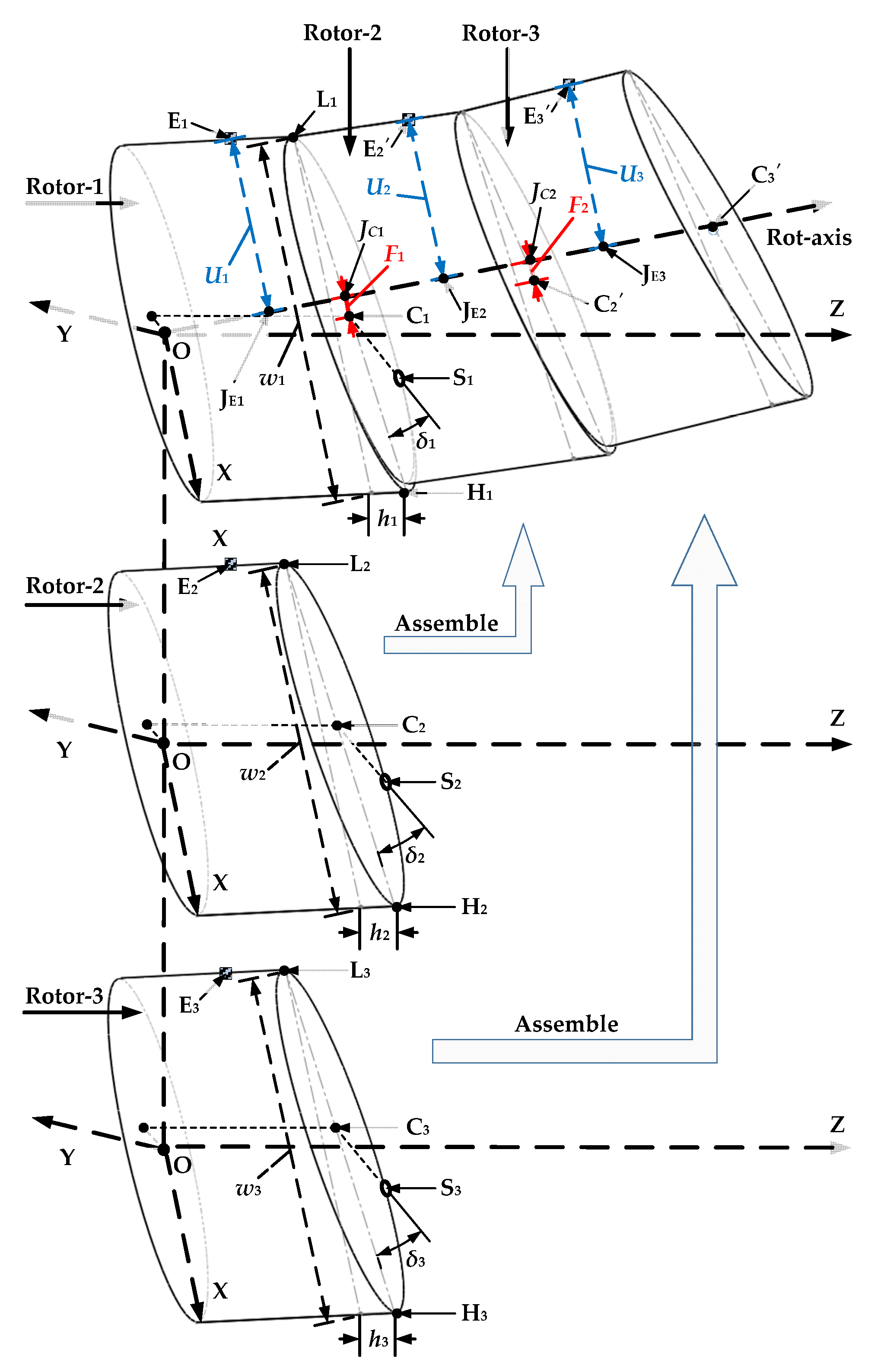


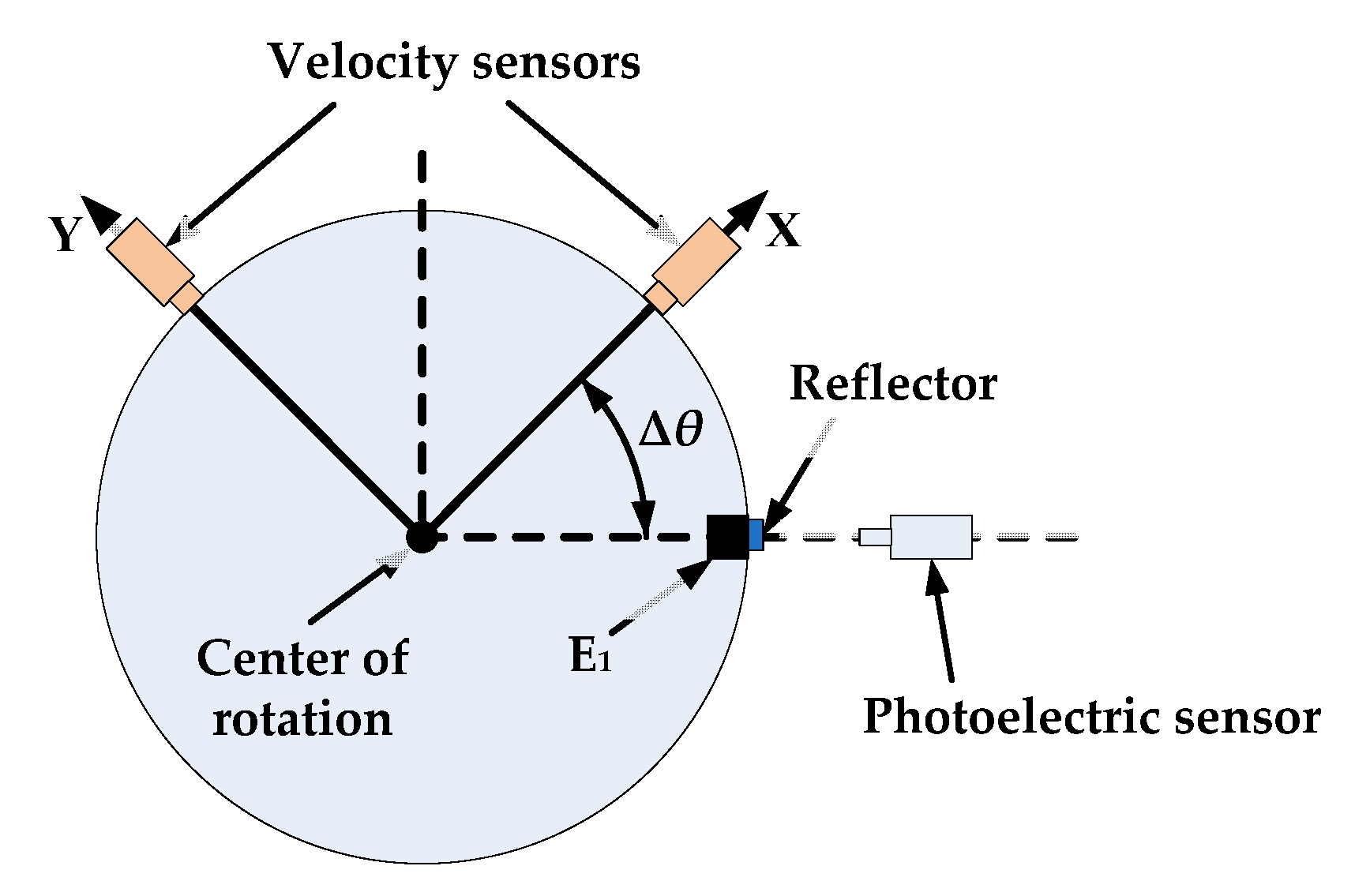

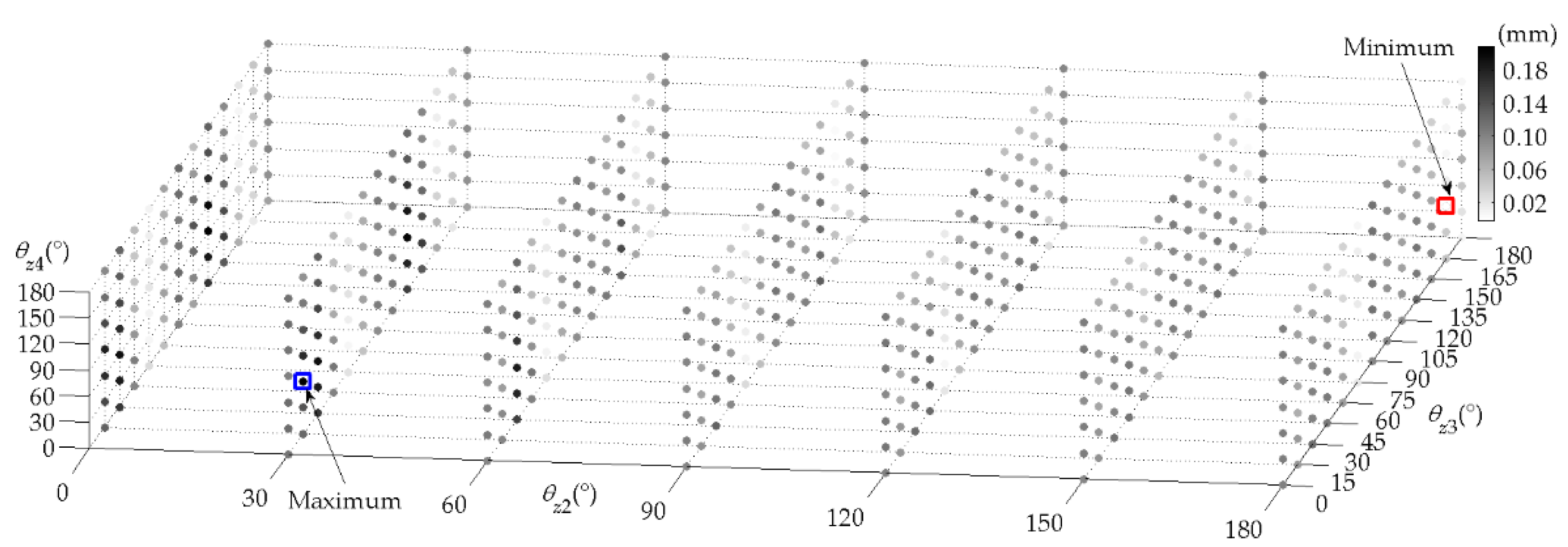
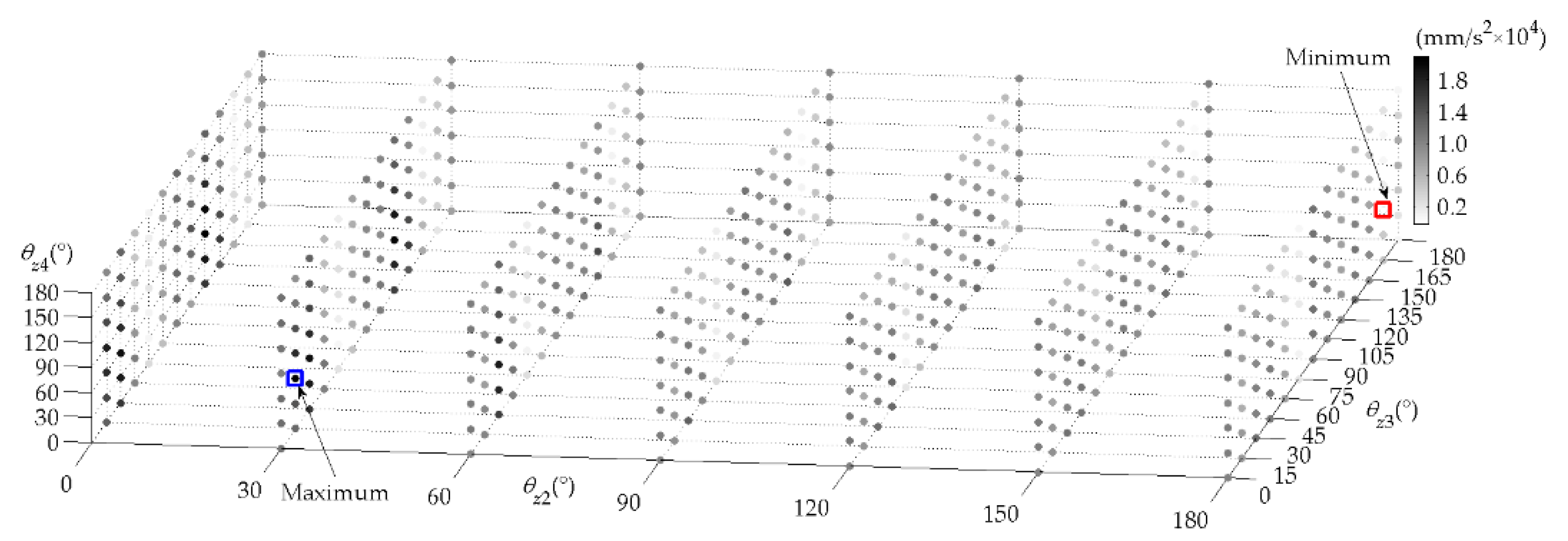


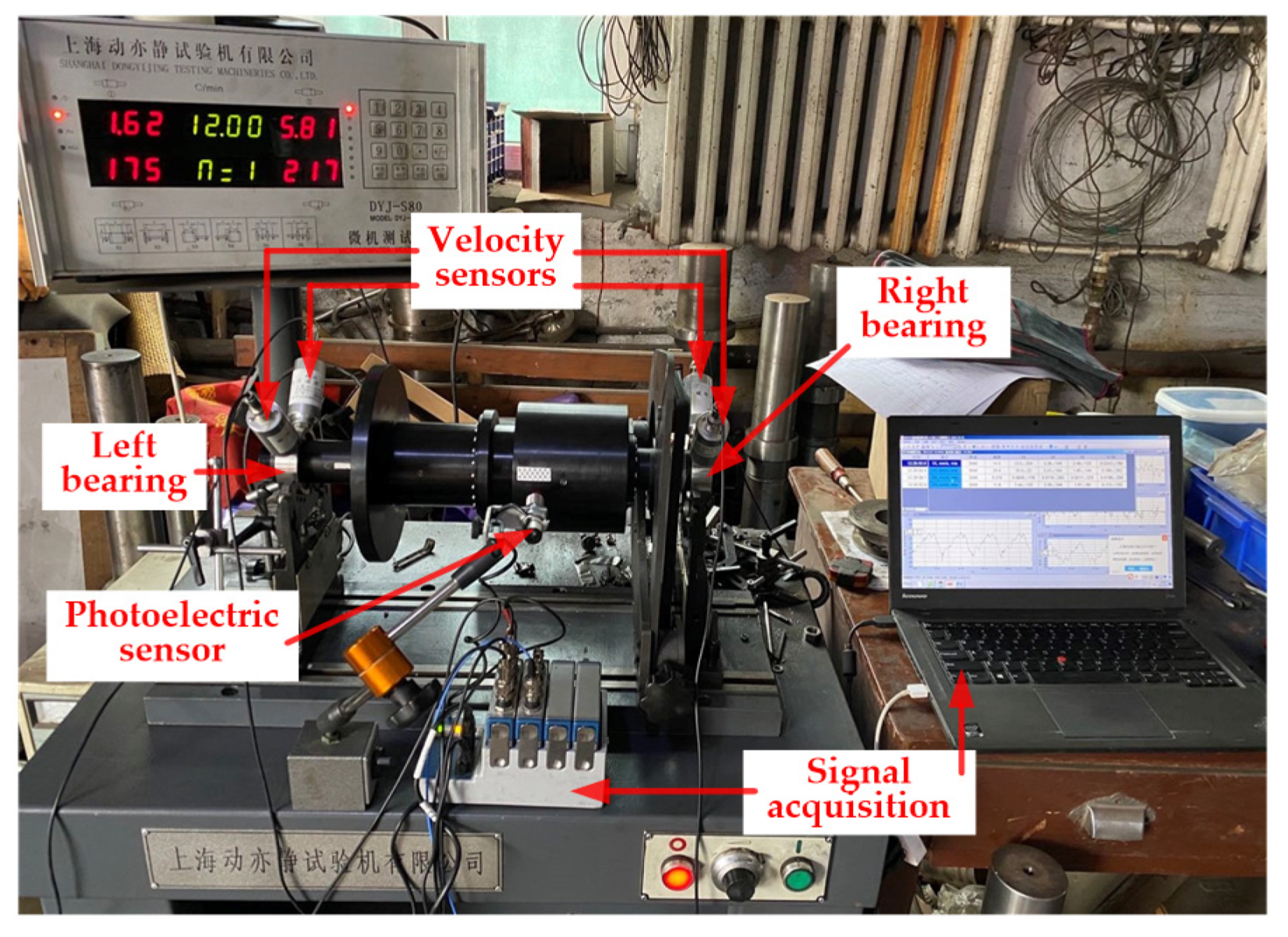
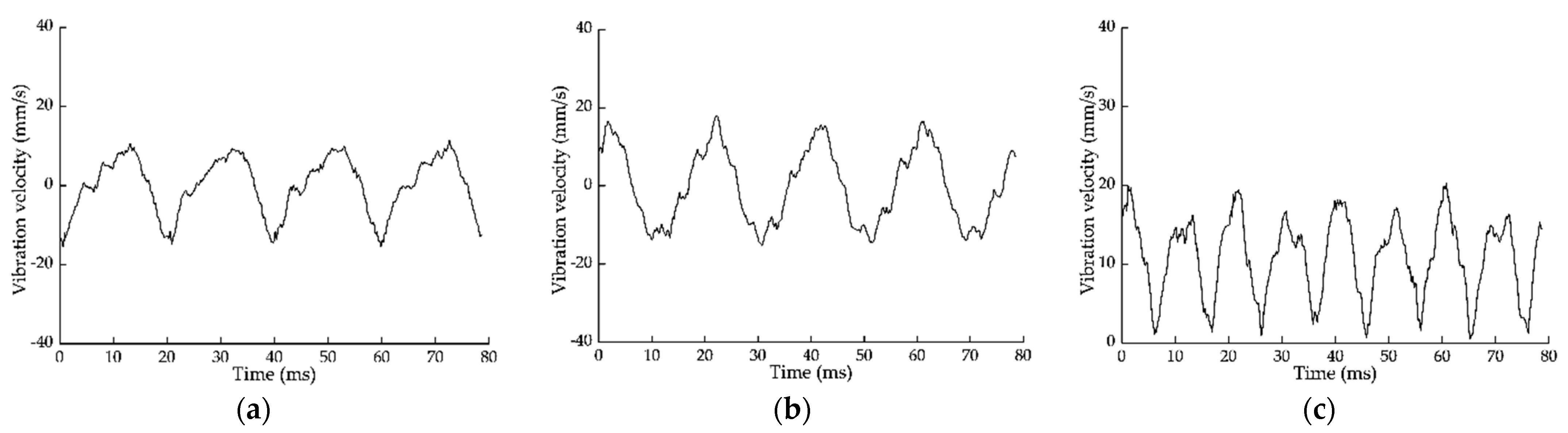

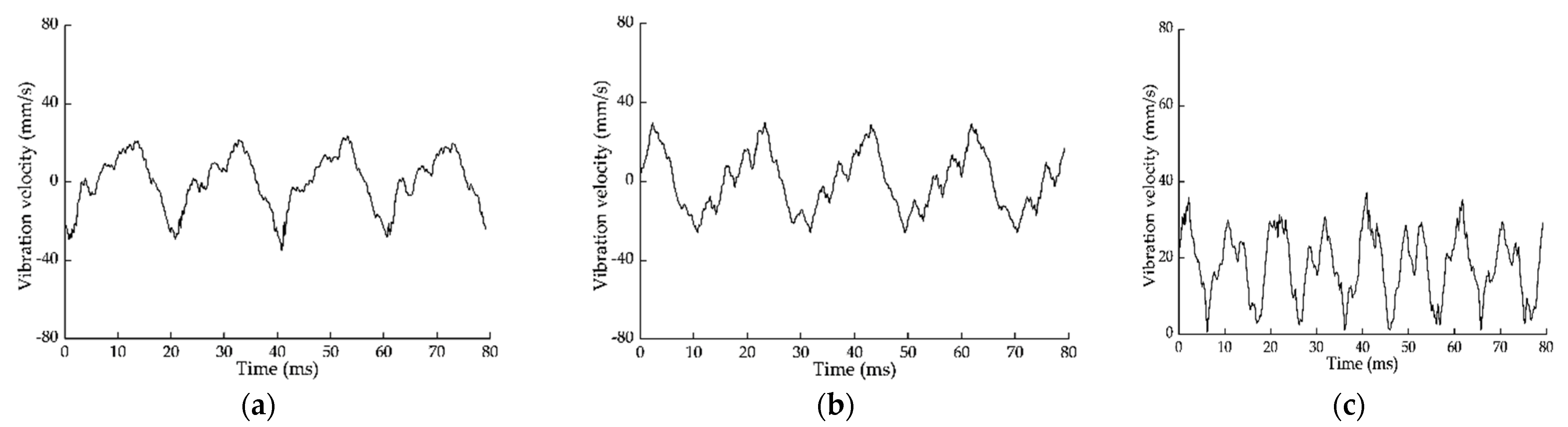
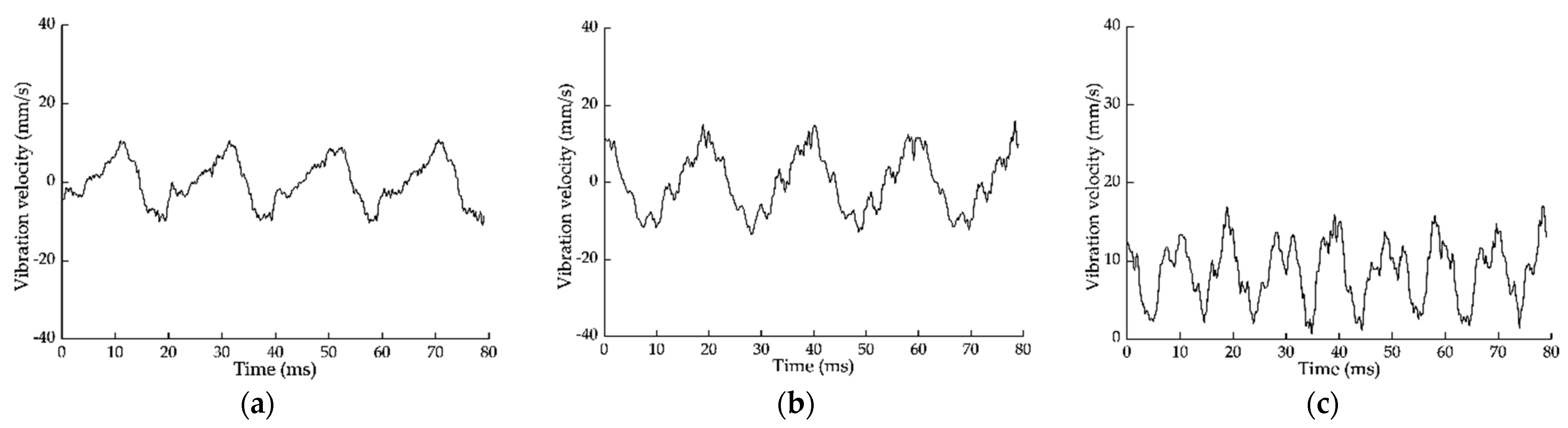

| Unbalanced Mass Points | un [g] | Coordinates of En [mm] |
|---|---|---|
| E1 | 0.296 | (17.25, 0, 35) |
| E2 | 0.197 | (38.5, 0, 58.5) |
| E3 | 0.314 | (60, 0, 77) |
| E4 | 0.178 | (18.75, 0, 36.5) |
| Components | ξn [mm] | h1 [mm] | w1 [mm] | δn [°] |
|---|---|---|---|---|
| Rotor-1 | (0.0181, 0.0062, 65.0152) | 0.0193 | 36.0220 | 113 |
| Rotor-2 | (−0.009, −0.0223, 115.0210) | 0.0235 | 101.9895 | 29 |
| Rotor-3 | (0.0056, 0.0244, 138.1047) | 0.0241 | 50.0149 | 242 |
| Rotor-4 | (0.0116, 0.0124, 74.9581) | - | - | 182 |
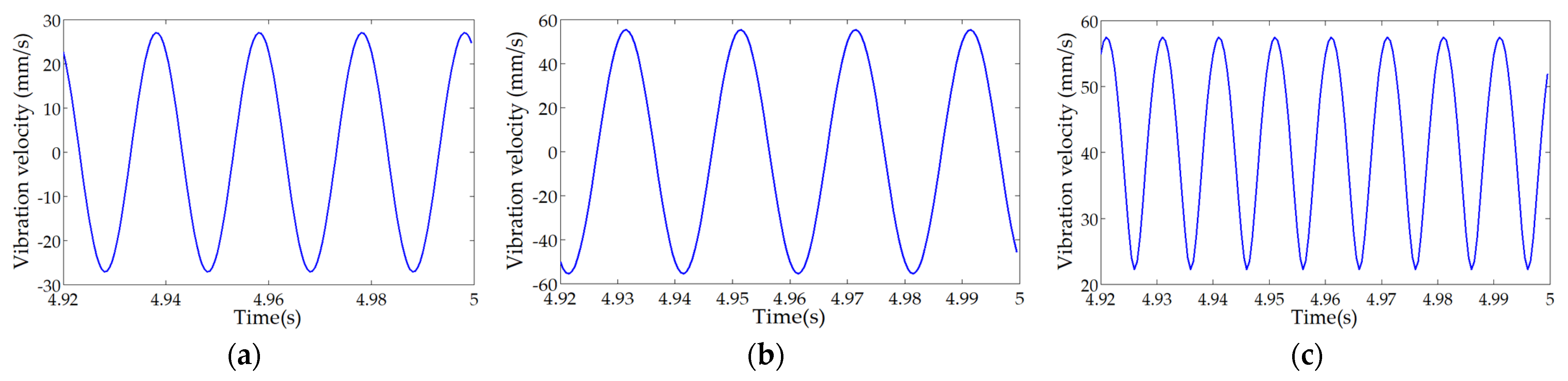
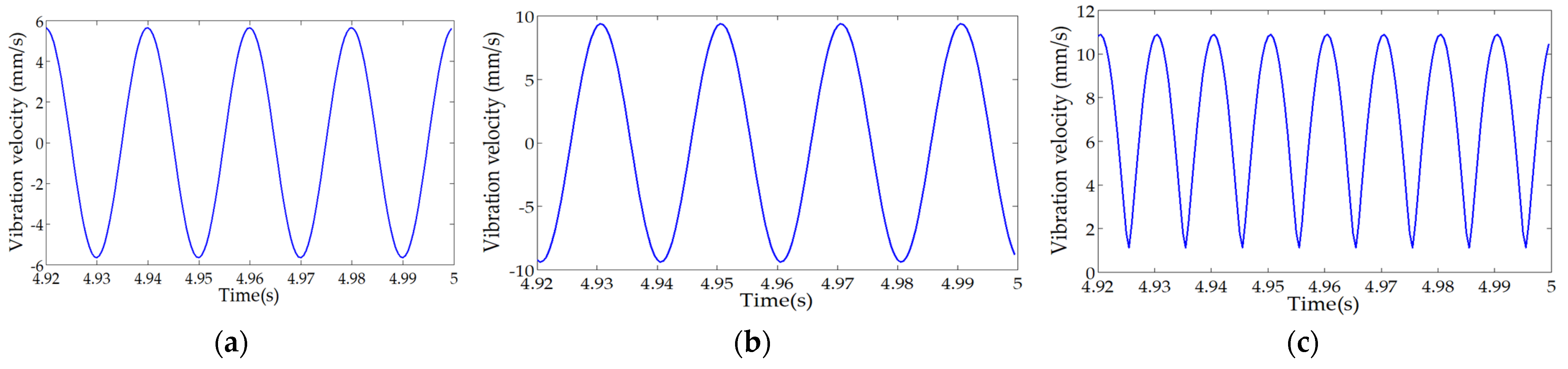
| Position | Vibration Velocity [mm/s] | Vibration Displacement [mm] | Vibration Acceleration [mm/s2] |
|---|---|---|---|
| Node-6 | 4.4630 | 0.0141 | 1.4044 × 103 |
| Node-38 | 3.5759 | 0.0113 | 1.1259 × 103 |
| Position | Vibration Velocity [mm/s] | Vibration Displacement [mm] | Vibration Acceleration [mm/s2] |
|---|---|---|---|
| Node-6 | 40.5936 | 0.1285 | 1.2785 × 104 |
| Node-38 | 57.4941 | 0.1820 | 1.8076 × 104 |
| Position | Vibration Velocity [mm/s] | Vibration Displacement [mm] | Vibration Acceleration [mm/s2] |
|---|---|---|---|
| Node-6 | 7.5441 | 0.0239 | 2.3778 × 103 |
| Node-38 | 10.8975 | 0.0345 | 3.4298 × 103 |
| Position | Vibration Velocity [mm/s] | Vibration Displacement [mm] | Vibration Acceleration [mm/s2] |
|---|---|---|---|
| Node-6 | 2.9001 | 0.0093 | 0.9149 × 103 |
| Node-38 | 2.1121 | 0.0074 | 0.8221 × 103 |
| Position | Vibration Velocity [mm/s] | Vibration Displacement [mm] | Vibration Acceleration [mm/s2] |
|---|---|---|---|
| Node-6 | 25.1136 | 0.0795 | 7.9071 × 103 |
| Node-38 | 34.0271 | 0.1077 | 1.0713 × 104 |
| Position | Vibration Velocity [mm/s] | Vibration Displacement [mm] | Vibration Acceleration [mm/s2] |
|---|---|---|---|
| Node-6 | 4.4776 | 0.0176 | 1.7205 × 103 |
| Node-38 | 7.9312 | 0.0284 | 2.4682 × 103 |
| External Force [N] | Vibration Velocity at Node-6 [mm/s] | Vibration Velocity at Node-38 [mm/s] |
|---|---|---|
| 0 | 4.4630 | 3.5759 |
| 5 | 4.3109 | 3.1741 |
| 10 | 4.1034 | 2.4683 |
| External Force [N] | Vibration Displacement at Node-6 [mm] | Vibration Displacement at Node-38 [mm] |
|---|---|---|
| 0 | 0.0141 | 0.0113 |
| 5 | 0.0128 | 0.0088 |
| 10 | 0.0117 | 0.0064 |
| External Force [N] | Vibration Acceleration at Node-6 [mm/s2] | Vibration Acceleration at Node-38 [mm/s2] |
|---|---|---|
| 0 | 0.9149 × 103 | 0.8221 × 103 |
| 5 | 0.8058 × 103 | 0.6072 × 103 |
| 10 | 0.7129 × 103 | 0.4305 × 103 |
| State | Maximum of fl(γ) [mm/s] | Maximum of fr(γ) [mm/s] |
|---|---|---|
| Optimal assembly | 20.2505 | 17.1042 |
| Worst assembly | 66.5348 | 55.5799 |
| Default assembly | 37.1793 | 32.4968 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Cui, J.; Sun, X. A Vibration Suppression Method for the Multistage Rotor of an Aero-Engine Based on Assembly Optimization. Machines 2021, 9, 189. https://doi.org/10.3390/machines9090189
Chen Y, Cui J, Sun X. A Vibration Suppression Method for the Multistage Rotor of an Aero-Engine Based on Assembly Optimization. Machines. 2021; 9(9):189. https://doi.org/10.3390/machines9090189
Chicago/Turabian StyleChen, Yue, Jiwen Cui, and Xun Sun. 2021. "A Vibration Suppression Method for the Multistage Rotor of an Aero-Engine Based on Assembly Optimization" Machines 9, no. 9: 189. https://doi.org/10.3390/machines9090189
APA StyleChen, Y., Cui, J., & Sun, X. (2021). A Vibration Suppression Method for the Multistage Rotor of an Aero-Engine Based on Assembly Optimization. Machines, 9(9), 189. https://doi.org/10.3390/machines9090189







