Influence of Aerodynamic Preloads and Clearance on the Dynamic Performance and Stability Characteristic of the Bump-Type Foil Air Bearing
Abstract
:1. Introduction
On the Stability and Dynamic Performance of Lobed Bump-Type Foil Air Bearings
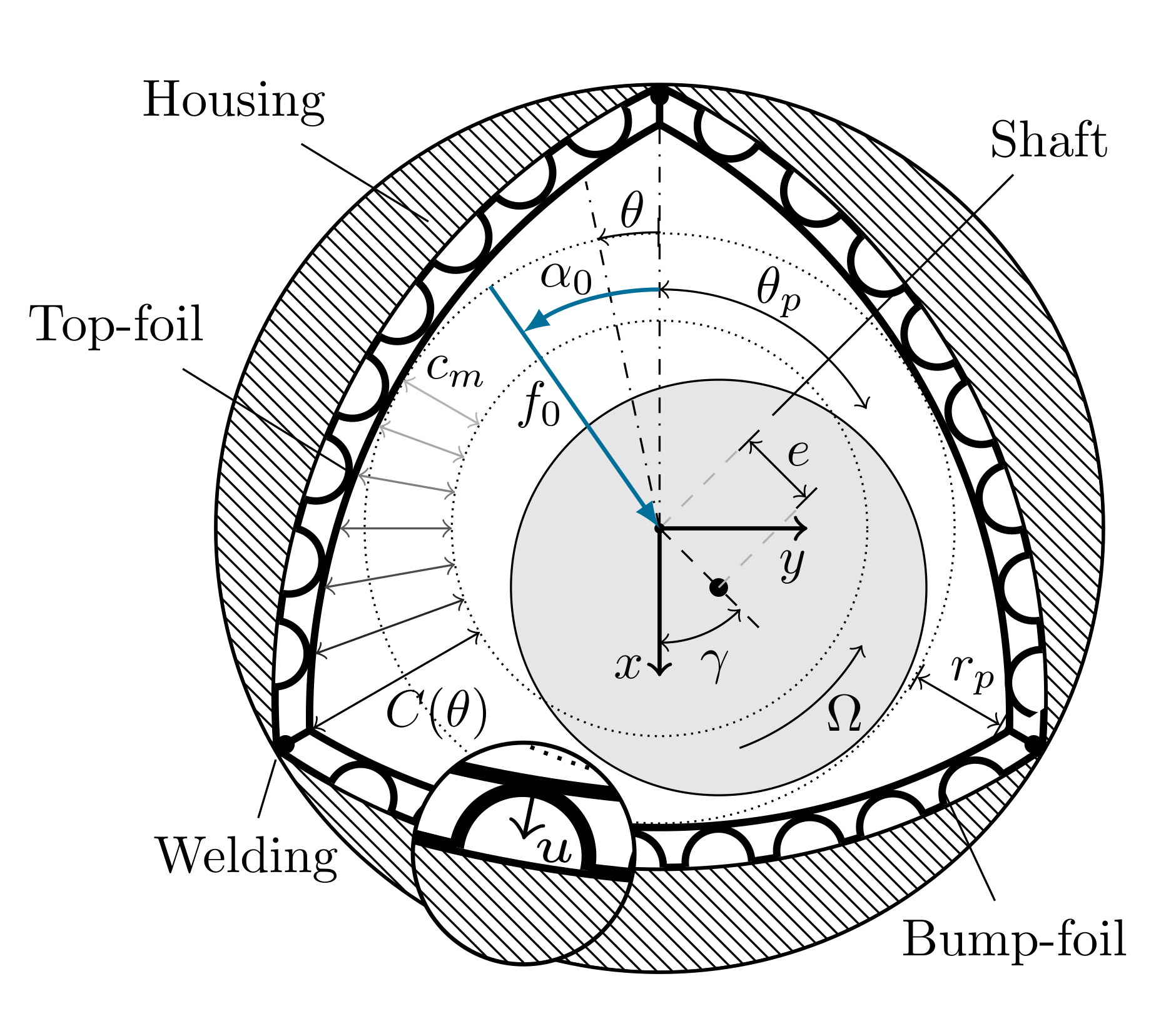
2. Bump-Type Foil Air Bearing Model
3. Methods of Solution
3.1. Steady-State Analysis
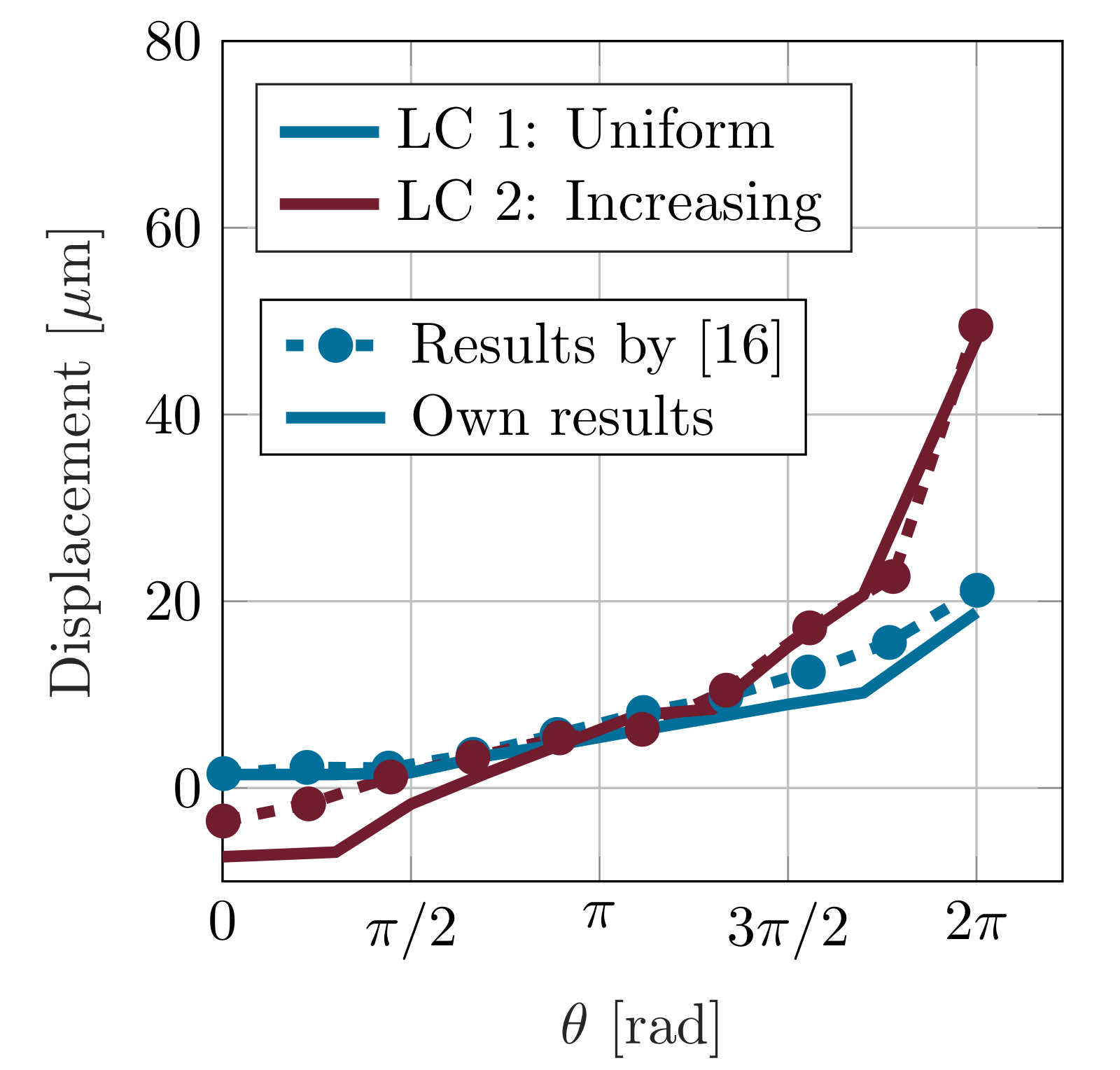
3.2. Transient Analysis
4. Parametric Study
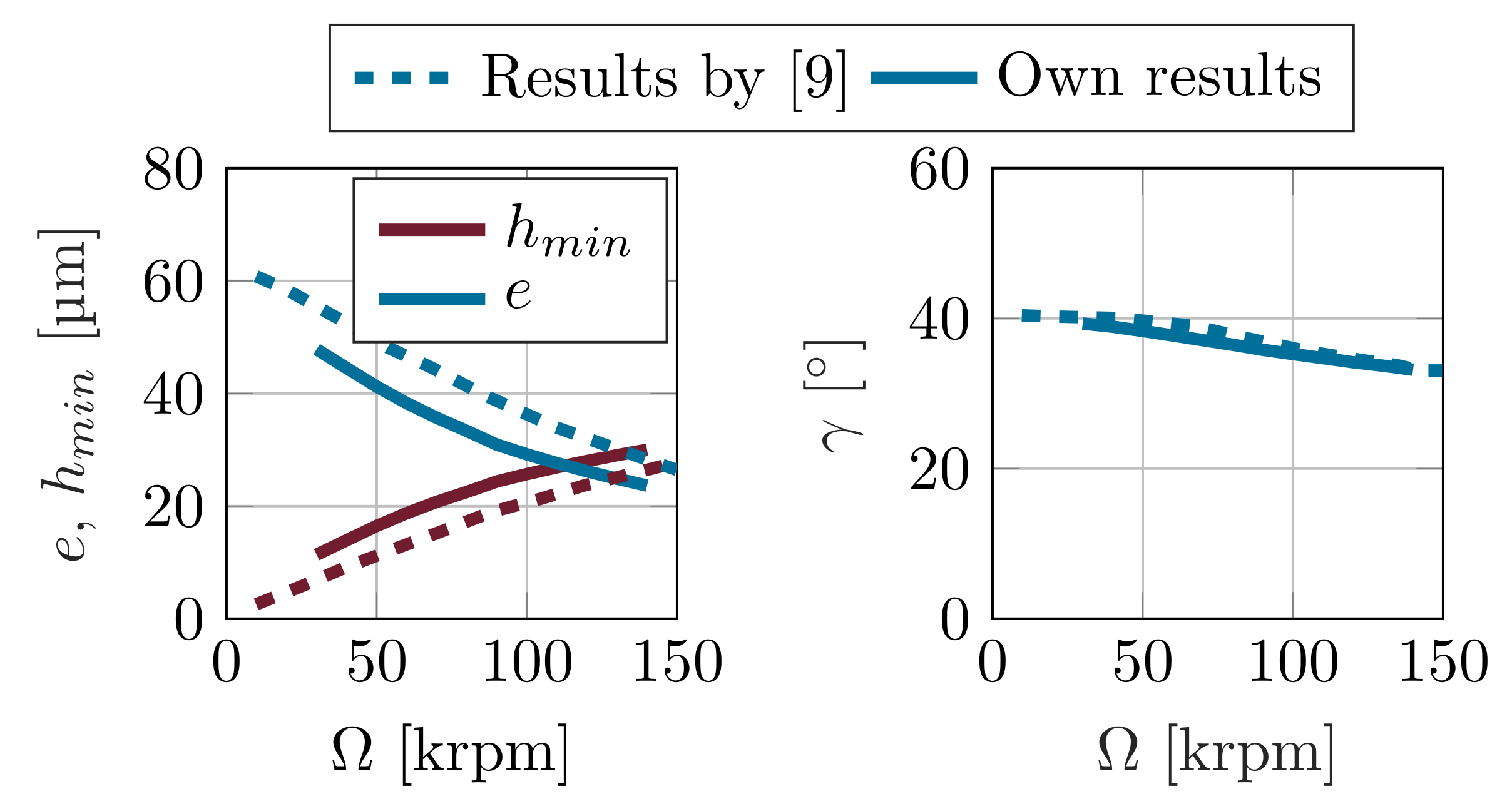
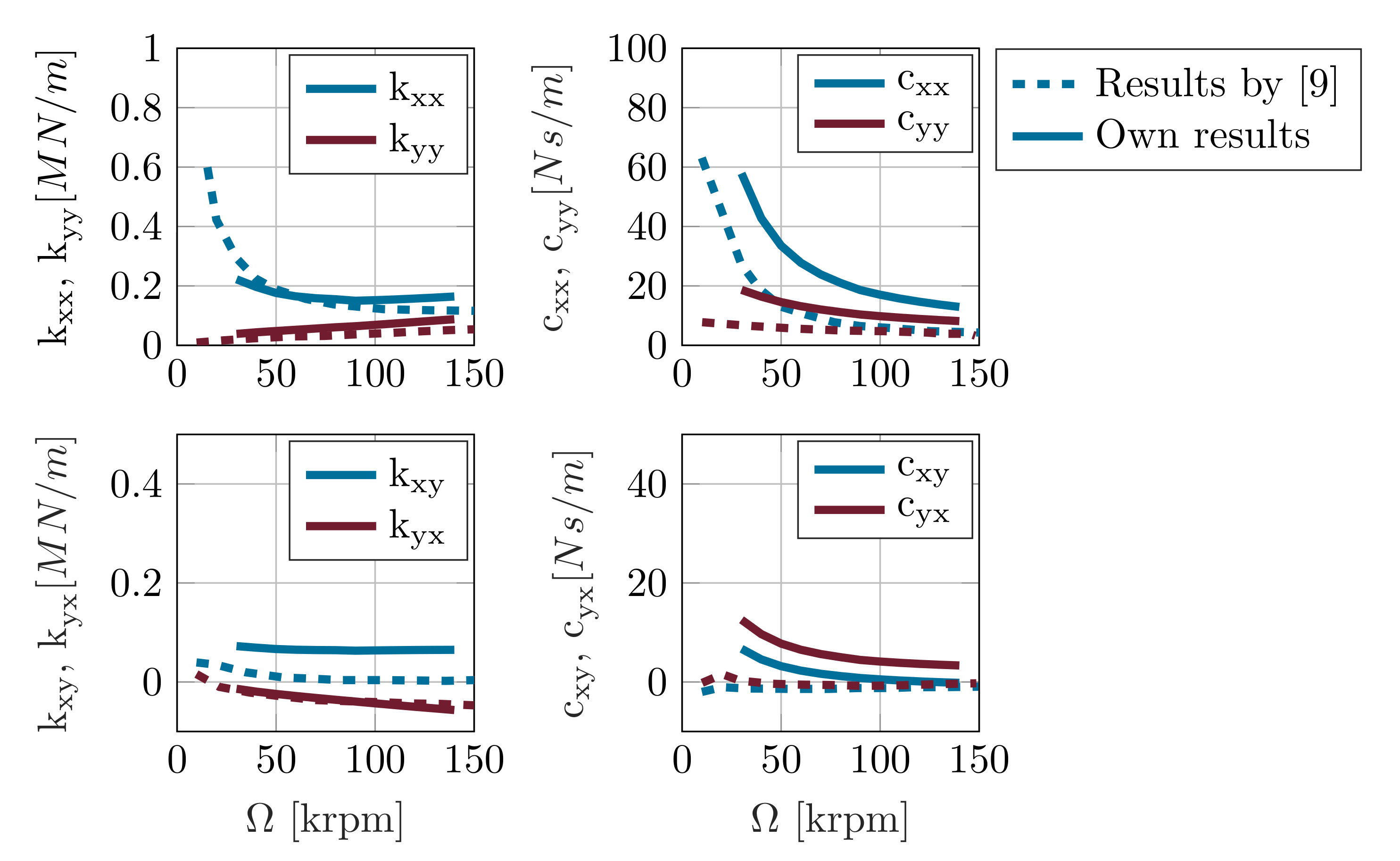
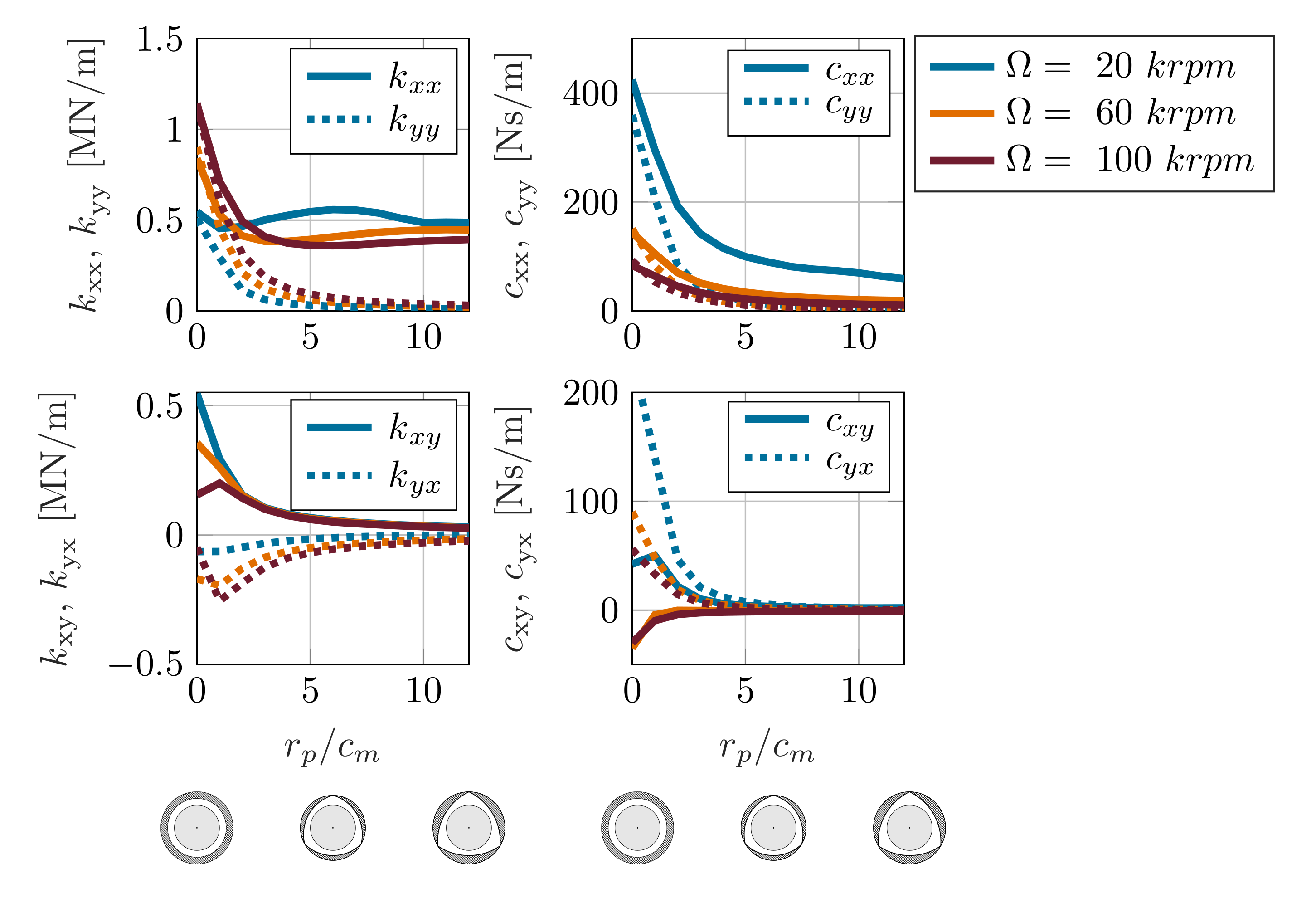
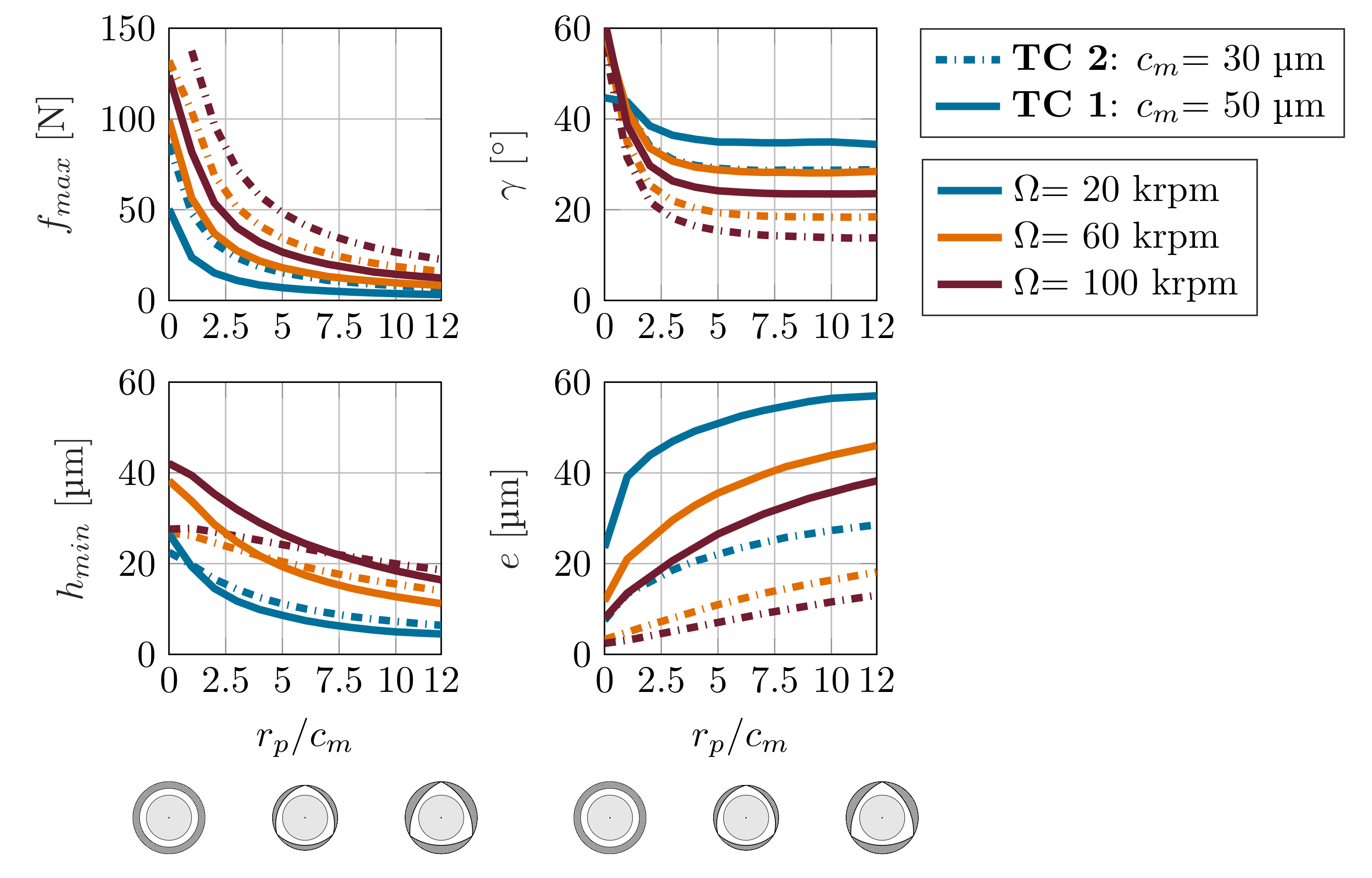
4.1. Dynamic Performance
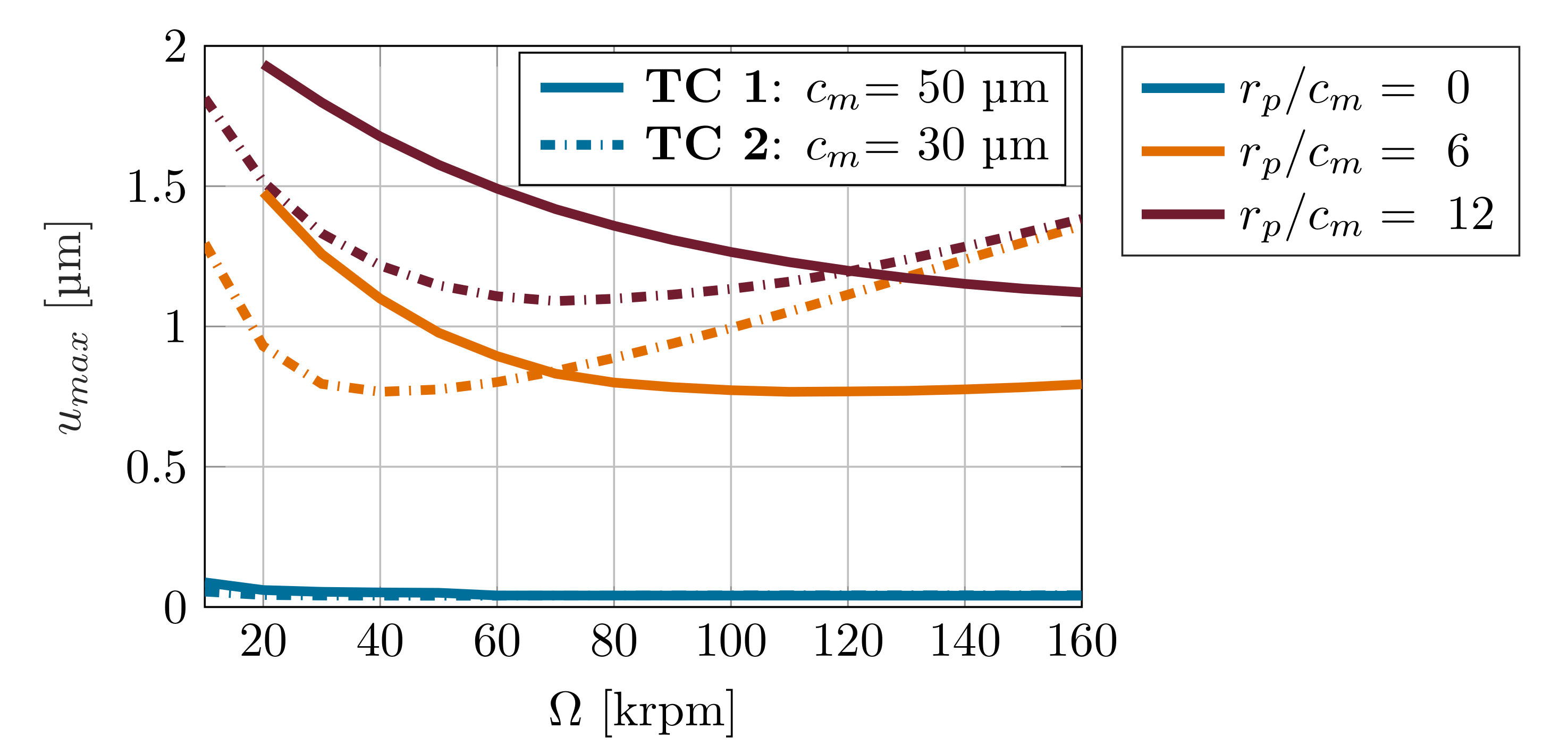
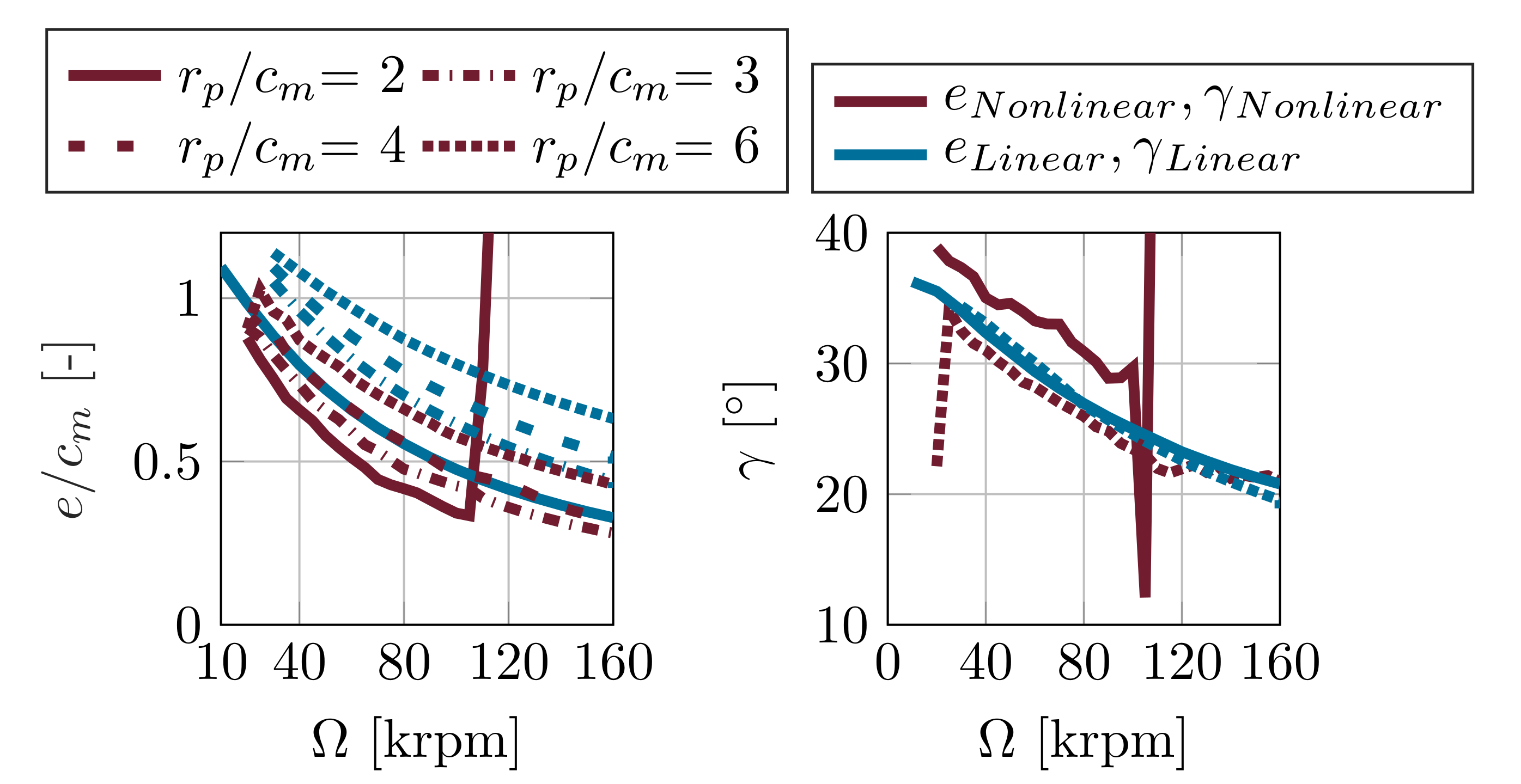
4.2. Stability Analysis
4.3. Parameter Optimization
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Amplitude [m] | |
| c | Damping [Ns/m] |
| Circumferential bearing clearance [m]/[-] | |
| Nominal bearing clearance [m] | |
| Minimum bearing clearance [-] | |
| Damping ratio [-] | |
| D | Bearing diameter [m] |
| E | Young’s modulus [Pa] |
| Eccentricity of shaft [m]/[-] | |
| Static load [N]/[-] | |
| f | Frequency [Hz] |
| Lubricant reaction force [N]/[-] | |
| Bump foil reaction force [N] | |
| Maximum load capacity [N] | |
| Eigenfrequency [Hz] | |
| Subsynchronous frequency [Hz] | |
| Film thickness [m]/[-] | |
| Minimum film thickness [m]/[-] | |
| k | Stiffness [Pa] |
| L | Length of bearing [m] |
| Half of bump length [m] | |
| Mass of the rotor [kg] | |
| n | Number of timesteps [-] |
| Number of foil segments [-] | |
| Number of bumps per segment [-] | |
| Pressure [Pa]/[-] | |
| Ambient pressure [Pa] | |
| Perturbed quantity [-] | |
| Bump radius [m] | |
| R | Bearing radius [m] |
| Preload factor [m] | |
| s | Bump pitch [m] |
| Time [s]/[-] | |
| Foil thickness [m] | |
| Foil deflection [m]/[-] | |
| v | Frequency ratio [-] |
| Vertical coordinate [m]/[-] | |
| Horizontal coordinate [m]/[-] | |
| Axial coordinate [m]/[-] | |
| Static force angle [°] | |
| Angle of lubricant reaction force [°] | |
| Structural loss factor [-] | |
| Attitude angle of journal [°] | |
| Circumferential coordinate [°] | |
| Pivot angle of foil segment [°] | |
| Eigenvalue [rad/s] | |
| Compressibility number [-] | |
| Frictional coefficient [-] | |
| Absolute viskosity [Pa s] | |
| Poisson ratio [-] | |
| Unbalance of shaft [g mm] | |
| Nondimensional state variable [-] | |
| Undamped angular eigenfrequency [rad/s] | |
| Excitation frequency [rad/s] | |
| Rotational speed [1/min] |
References
- Heshmat, H.; Shapiro, H.; Gray, S. Development of Foil Journal Bearings for High Load Capacity and High Speed Whirl Stability. J. Lubr. Tech. 1982, 104, 149–156. [Google Scholar] [CrossRef]
- Heshmat, H. Advancements in the Performance of Aerodynamic Foil Journal Bearings: High Speed and Load Capability. J. Tribol. 1994, 116, 287–294. [Google Scholar] [CrossRef]
- Kim, D. Parametric Studies on Static and Dynamic Performance of Air Foil Bearings with Different Top Foil Geometries and Bump Stiffnesses Distributions. J. Tribol. 2007, 129, 354–364. [Google Scholar] [CrossRef]
- Kim, T.H.; San Andrés, L. Effect of Mechanical Preloads on the Dynamic Performance of Gas Foil Bearings. In Proceedings of the STLE/ASME International Joint Tribology Conference, Miami, FL, USA, 20–22 October 2008; pp. 379–381. [Google Scholar]
- Sim, K.; Yong-Bok, L.; Ho Kim, T.; Lee, J. Rotordynamic Performance of Shimmed Gas Foil Bearings for Oil-Free Turbochargers. J. Tribol. 2012, 134, 031102. [Google Scholar] [CrossRef]
- Schiffmann, J.; Spakovszky, Z.S. Foil Bearing Design Guidelines for Improved Stability. J. Tribol. 2013, 135, 011103. [Google Scholar] [CrossRef] [Green Version]
- Sim, K.; Lee, Y.B.; Kim, T.H. Effects of Mechanical Preload and Bearing Clearance on Rotordynamic Performance of Lobed Gas Foil Bearings for Oil-Free Turbochargers. Tribol. Trans. 2013, 56, 224–235. [Google Scholar] [CrossRef]
- Sim, K.; Koo, B.; Lee, J.S.; Kim, T.H. Effects of Mechanical Preloads on the Rotordynamic Performance of a Rotor Supported on Three-Pad Gas Foil Journal Bearings. J. Eng. Gas Turbines Power 2014, 136, 122503. [Google Scholar] [CrossRef]
- Sim, K.; Lee, Y.B.; Kim, T.H. Rotordynamic Analysis of an Oil-Free Turbocharger Supported on Lobed Gas Foil Bearings—Predictions versus Test Data. Tribol. Trans. 2014, 57, 1086–1095. [Google Scholar] [CrossRef]
- Sadri, H.; Schlums, H.; Sinapius, M. Investigation of Structural Conformity in a Three-Pad Adaptive Air Foil Bearing With Regard to Active Control of Radial Clearance. J. Tribol. 2019, 141, 081701. [Google Scholar] [CrossRef]
- Sadri, H.; Schlums, H.; Sinapius, M. Design Characteristics of an Aerodynamic Foil Bearing With Adaptable Bore Clearance. In Proceedings of the ASME Turbo Expo: Turbomachinery Technical Conference and Exposition, Oslo, Norway, 11–15 June 2018. [Google Scholar]
- Feng, K.; Guan, H.Q.; Zhao, Z.L.; Liu, T.Y. Active bump-type foil bearing with controllable mechanical preloads. Tribol. Int. 2018, 120, 187–202. [Google Scholar] [CrossRef]
- Pinkus, O. The Reynolds Centennial: A Brief History of the Theory of Hydrodynamic Lubrication. J. Tribol. 1987, 109, 2–15. [Google Scholar] [CrossRef]
- Peng, J.P.; Carpino, M. Calculation of Stiffness and Damping Coefficients for Elastically Supported Gas Foil Bearings. J. Tribol. 1993, 115, 20–27. [Google Scholar] [CrossRef]
- Lee, J.S.; Kim, T.H. Analysis of Three-Pad Gas Foil Journal Bearing for Increasing Mechanical Preloads. J. Korean Soc. Tribol. Lubr. Eng. 2014, 30, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Sadri, H.; Sinapius, M.; Friedrichs, J. Active Shape Control of the Lubricant Film Geometry in Adaptive Air Foil Bearings; TU Braunschweig, Niedersächsisches Forschungszentrum für Luftfahrt: Braunschweig, Germany, 2020. [Google Scholar]
- Le Lez, S.; Arghir, M.; Frene, J. A New Bump-Type Foil Bearing Structure Analytical Model. J. Eng. Gas Turbines Power 2007, 129, 1047–1057. [Google Scholar] [CrossRef]
- Arghir, M.; Benchekroun, O. A simplified structural model of bump-type foil bearings based on contact mechanics including gaps and friction. Tribol. Int. 2019, 134, 129–144. [Google Scholar] [CrossRef]
- Arghir, M.; Benchekroun, O. A New Structural Bump Foil Model With Application From Start-Up to Full Operating Conditions. J. Eng. Gas Turbines Power 2019, 141, 101017. [Google Scholar] [CrossRef]
- Heshmat, H.; Walowit, J.A.; Pinkus, O. Analysis of Gas-Lubricated Foil Journal Bearings. J. Lubr. Technol. 1983, 105, 647–655. [Google Scholar] [CrossRef]
- Khonsari, M.M.; Peng, Z.C. Hydrodynamic Analysis of Compliant Foil Bearings With Compressible Air Flow. J. Tribol. 2004, 126, 542–546. [Google Scholar]
- Larsen, J.S.; Santos, I.F.; von Osmanski, S. Stability of rigid rotors supported by air foil bearings: Comparison of two fundamental approaches. J. Sound Vib. 2016, 381, 179–191. [Google Scholar] [CrossRef] [Green Version]
- Sternlicht, B. Elastic and Damping Properties of Cylindrical Journal Bearings. J. Basic Eng. 1959, 81, 101–107. [Google Scholar] [CrossRef]
- Lund, J.W. Calculation of Stiffness and Damping Properties of Gas Bearings. J. Lubr. Technol. 1968, 90, 793–803. [Google Scholar] [CrossRef]
- Lund, J.W. Review of the Concept of Dynamic Coefficients for Fluid Film Journal Bearings. J. Tribol. 1987, 109, 37–41. [Google Scholar] [CrossRef]
- Hunger, H. Berechnung der statischen und dynamischen Kennlinien Aerodynamischer Federlager; Universität Karlsruhe (TH): Karlsruhe, Germany, 1982. [Google Scholar]
- Iordanoff, I. Analysis of an Aerodynamic Compliant Foil Thrust Bearing: Method for a Rapid Design. J. Tribol. 1999, 121, 816–822. [Google Scholar] [CrossRef]
- Pan, C.H.T.; Kim, D. Stability Characteristics of a Rigid Rotor Supported by a Gas-Lubricated Spiral-Groove Conical Bearing. J. Tribol. 2007, 129, 375–383. [Google Scholar] [CrossRef]
- Vleugels, P.; Waumans, T.; Peirs, J.; Al-Bender, F.; Reynaerts, D. High-speed bearings for micro gas turbines: Stability analysis of foil bearings. J. Micromech. Microeng. 2006, 16, 282–289. [Google Scholar] [CrossRef]
- Pan, C.H.T. Spectral Analysis of Gas Bearing Systems for Stability Studies; Mechanical Technology Inc.: Latham, NY, USA, 1964. [Google Scholar]
- Le Lez, S.; Arghir, M.; Frêne, J. Nonlinear Numerical Prediction of Gas Foil Bearing Stability and Unbalanced Response. J. Eng. Gas Turbines Power 2009, 131, 012503. [Google Scholar] [CrossRef]
- Bonello, P.; Pham, H.M. Nonlinear Dynamic Analysis of High Speed Oil-Free Turbomachinery With Focus on Stability and Self-Excited Vibration. J. Tribol. 2014, 136, 041705. [Google Scholar] [CrossRef]
- Bonello, P.; Pham, H.M. The efficient computation of the nonlinear dynamic response of a foil–air bearing rotor system. J. Sound Vib. 2014, 333, 3459–3478. [Google Scholar] [CrossRef]
- Baum, C.; Hetzler, H.; Schröders, S.; Leister, T.; Seemann, W. A computationally efficient nonlinear foil air bearing model for fully coupled, transient rotor dynamic investigations. Tribol. Int. 2021, 153, 106434. [Google Scholar] [CrossRef]
- Baum, C.; Hetzler, H.; Seemann, W. On the stability of balanced rigid rotors in air foil bearings. In Proceedings of the International Conference on Vibrations in Rotating Machines, Magdeburg, Germany, 23–25 February 2015. [Google Scholar]
- Larsen, J.S.; Nielsen, B.B.; Santos, I.F. On the numerical Simulation of nonlinear transient Behavior of compliant air foil bearings. In Proceedings of the International Conference on Vibrations in Rotating Machines, Magdeburg, Germany, 23–25 February 2015. [Google Scholar]
- Guo, Z.; Feng, K.; Liu, T.; Lyu, P.; Zhang, T. Nonlinear dynamic analysis of rigid rotor supported by gas foil bearings: Effects of gas film and foil structure on subsynchronous vibrations. Mech. Syst. Signal Process. 2018, 107, 549–566. [Google Scholar] [CrossRef]
- Sadri, H.; Kyriazis, A.; Schlums, H.; Sinapius, M. A Semi-Analytical Model of Shape-Control in an Adaptive Air Foil Bearing. In Proceedings of the ASME 2018 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, San Antonio, TX, USA, 10–12 September 2018. [Google Scholar]

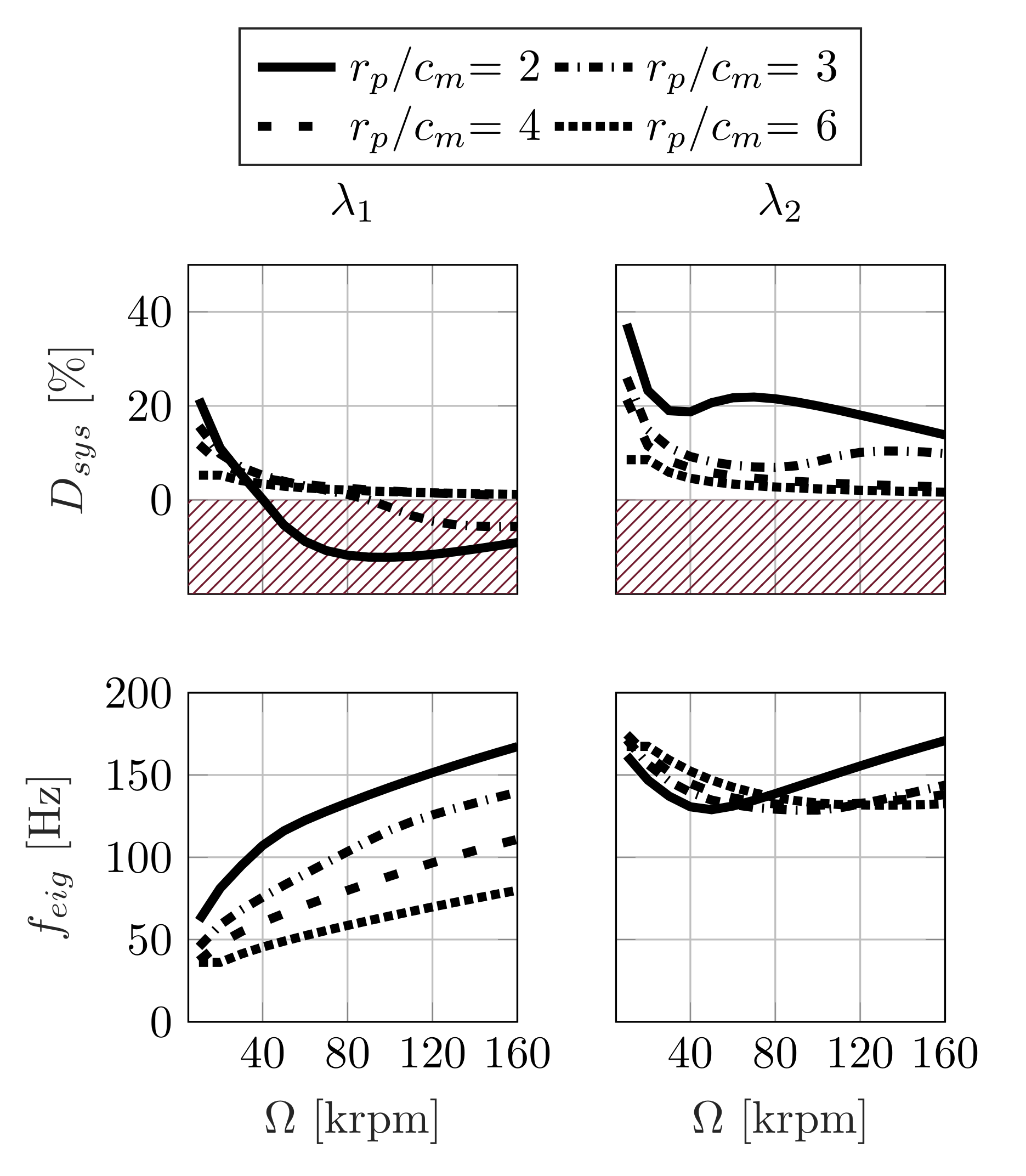
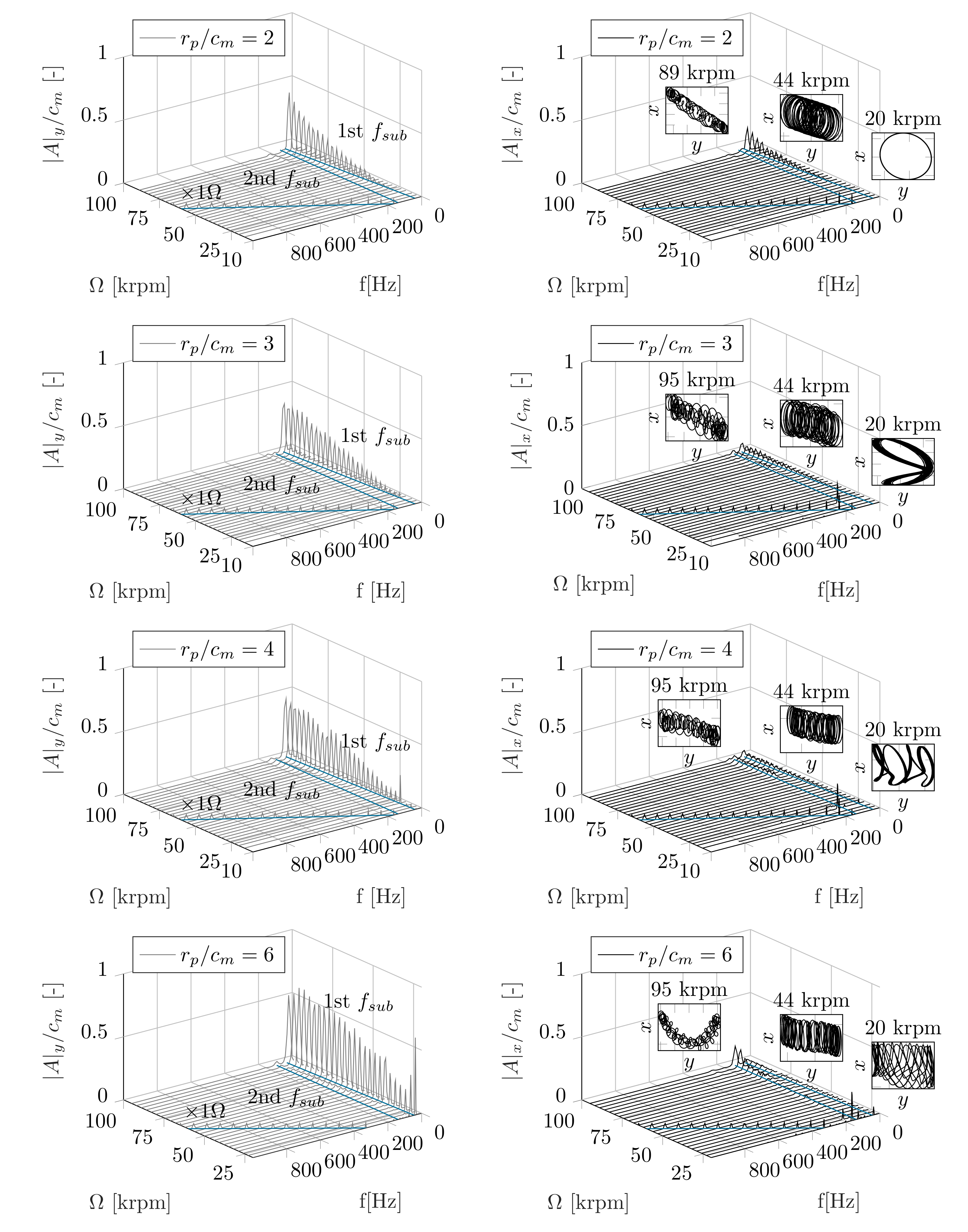
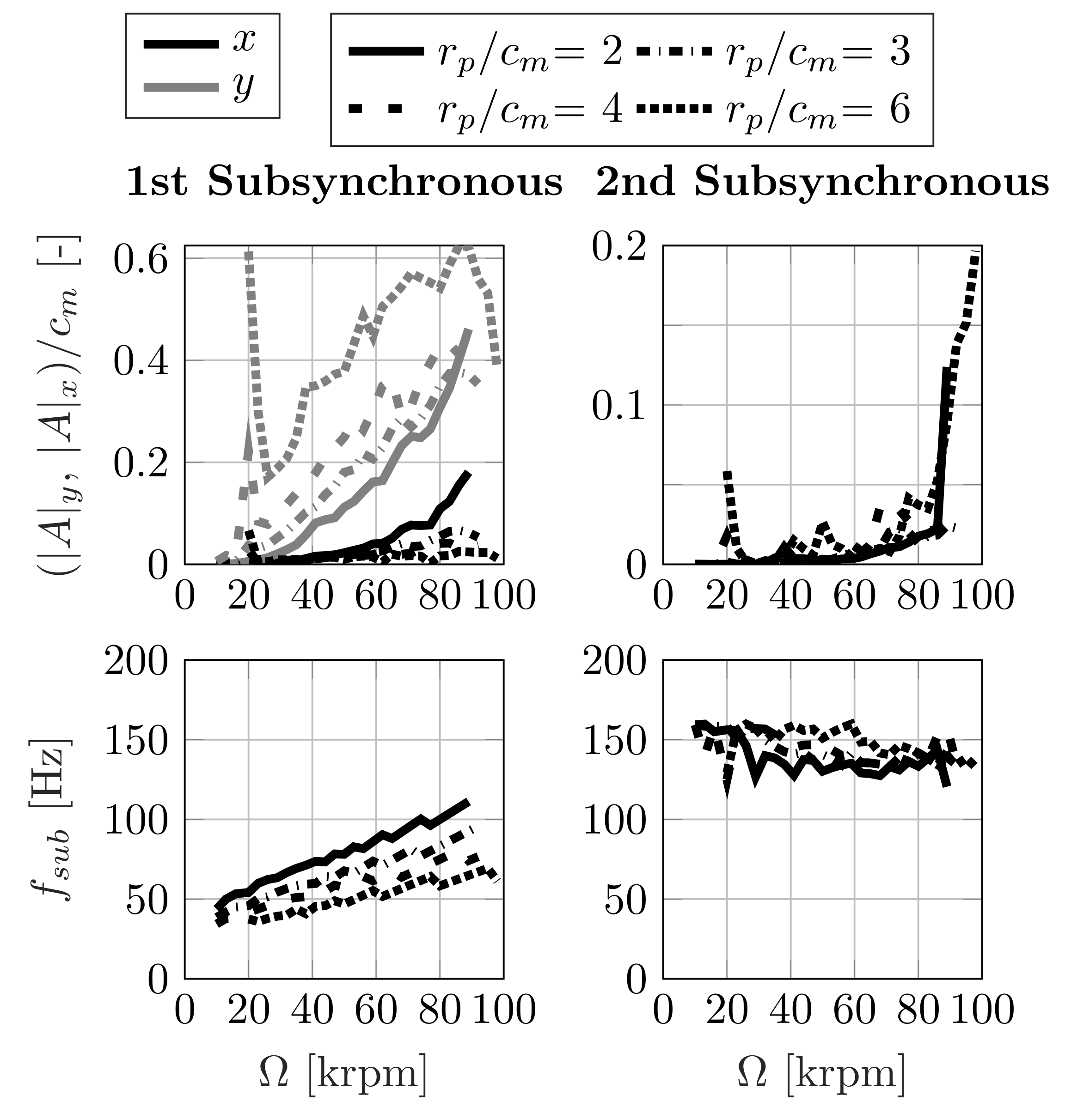
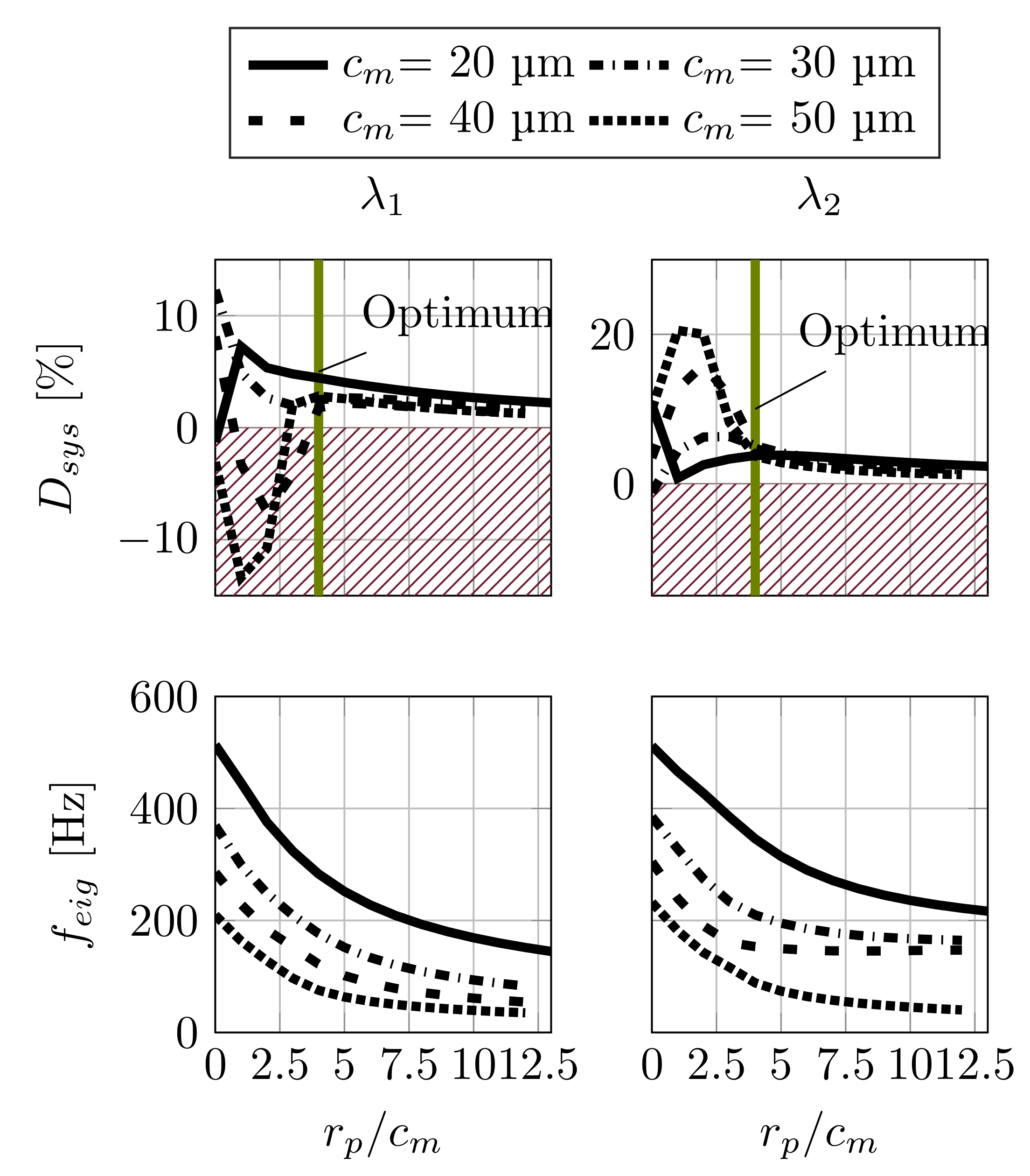

| Parameter | TC 1 | TC 2 | VC |
|---|---|---|---|
| Bore shape | |||
| [m] | 50 | 30 | 50 |
| [m] | 0 to 600 | 0 to 360 | 0, 100 |
| D [mm] | 38.5 | 25.6 | |
| L [mm] | 40 | 25.3 | |
| Bump foil | |||
| [-] | 3 | 3 | |
| [°] | 60 | 60 | |
| [-] | 9 | 6 | |
| [mm] | 2 | 2 | |
| [mm] | 1.81 | 1.2 | |
| s [mm] | 4.57 | 2.7 | |
| [mm] | 0.127 | 0.12 | |
| E [GPa] | 213 | 214 | |
| [-] | 0.29 | 0.29 | |
| [-] | 0.5 | 0.1 | |
| [-] | 0.3 | 0.4 | |
| Operational parameters | |||
| [N] | 5 | 1.9 | |
| [°] | 0 | 0 | |
| v (steady-state) [-] | 1 | 1 | |
| (transient) [gmm] | 1 | - | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Walter, F.; Sinapius, M. Influence of Aerodynamic Preloads and Clearance on the Dynamic Performance and Stability Characteristic of the Bump-Type Foil Air Bearing. Machines 2021, 9, 178. https://doi.org/10.3390/machines9080178
Walter F, Sinapius M. Influence of Aerodynamic Preloads and Clearance on the Dynamic Performance and Stability Characteristic of the Bump-Type Foil Air Bearing. Machines. 2021; 9(8):178. https://doi.org/10.3390/machines9080178
Chicago/Turabian StyleWalter, Fabian, and Michael Sinapius. 2021. "Influence of Aerodynamic Preloads and Clearance on the Dynamic Performance and Stability Characteristic of the Bump-Type Foil Air Bearing" Machines 9, no. 8: 178. https://doi.org/10.3390/machines9080178
APA StyleWalter, F., & Sinapius, M. (2021). Influence of Aerodynamic Preloads and Clearance on the Dynamic Performance and Stability Characteristic of the Bump-Type Foil Air Bearing. Machines, 9(8), 178. https://doi.org/10.3390/machines9080178







