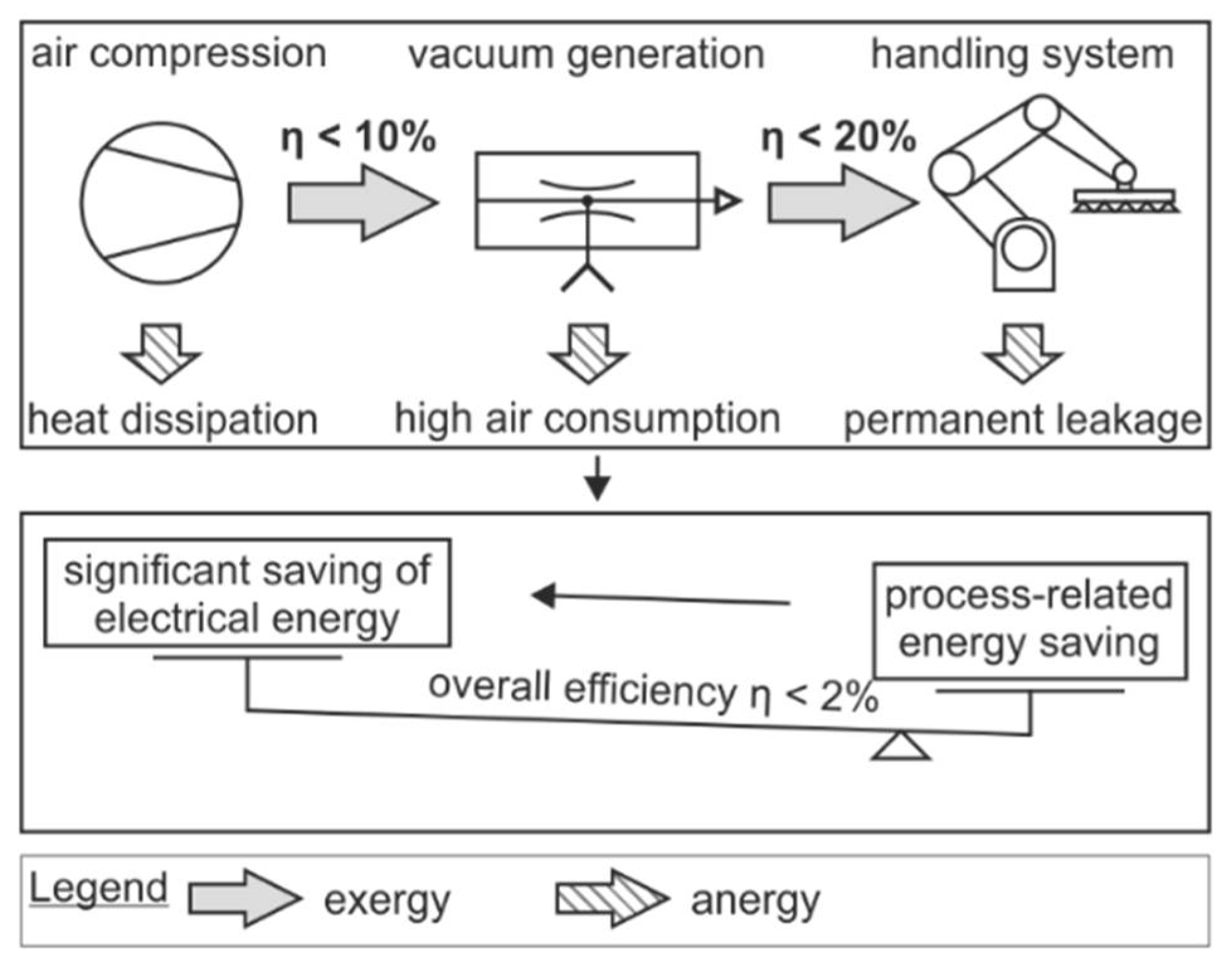
This section firstly elaborates the method for model-based grasp point optimization (
Section 2.1). In extension of the optimization method introduced in preliminary work of the authors, the influence of the surface roughness on the achievable holding force is examined in
Section 2.2. The influence of the surface roughness on the leakage between gripper and object is further investigated in
Section 2.3 as basis for the combined method for grasp optimization and gripping system dimensioning introduced in
Section 2.4.
2.1. Method for Model-Based Grasp Point Optimization
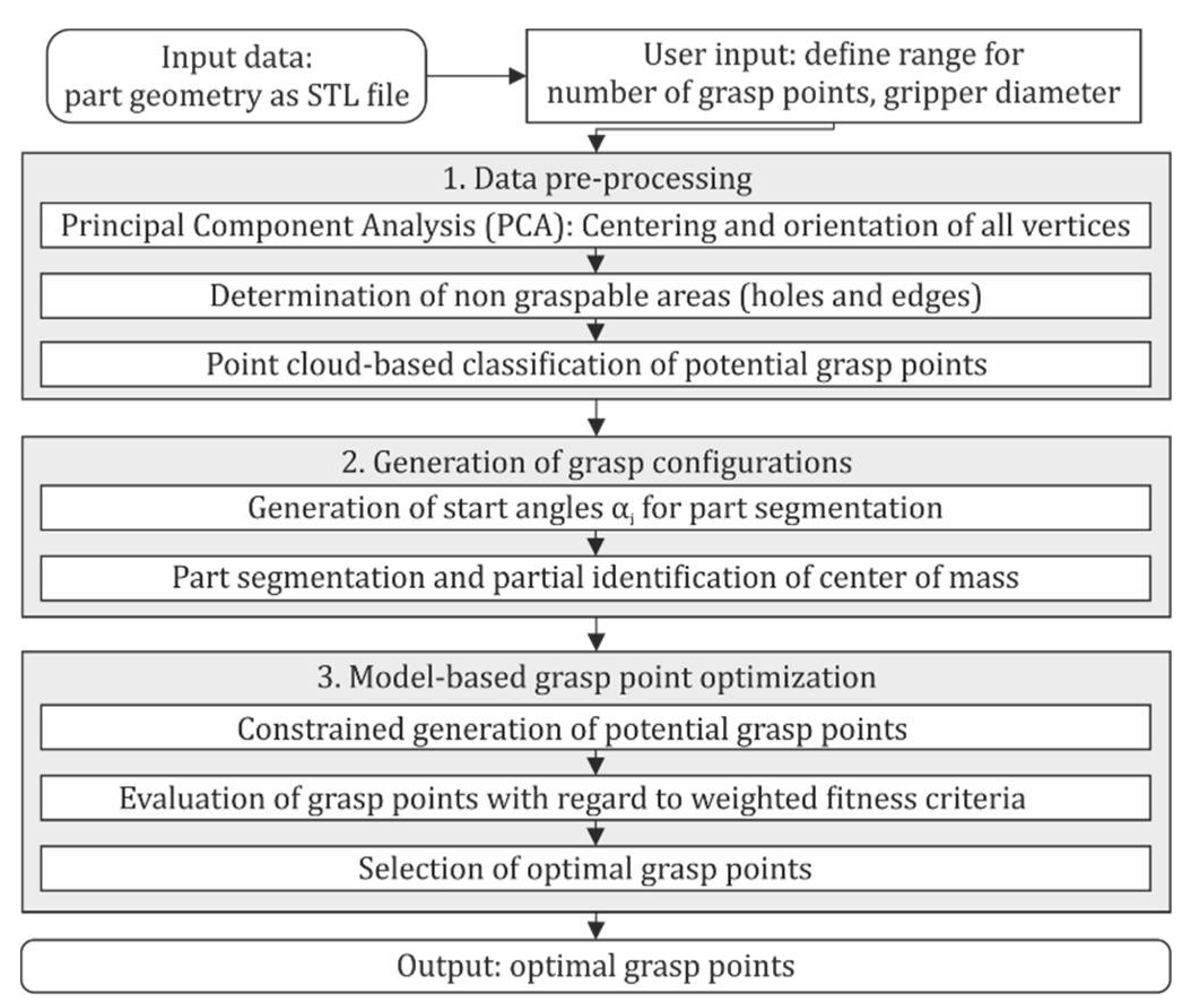
At a first step of the grasp optimization method (
Figure 2), the part geometry is input to the algorithm as STL file. Since the STL file may be generated by a 3D scan, the geometry can potentially be oriented arbitrarily in space and must therefore be centered and re-oriented, which is realized by a principal component analysis (PCA). This step also comprises a projection of the 3D geometry to a 2D polygon, as a simplification for shell-like parts such as car body sheet metal parts. Such parts have a large area of a low thickness and mostly only local three-dimensional surface geometries, such that the macroscopic geometry is still most similar to a plane. The optimization procedure begins with the identification of the graspable area. This excludes edges and holes from the overall part geometry. In addition, potential grasp points in the graspable area are classified with regard to the main geometry (e.g., cylinder or dome) in order to estimate the achievable holding force within the optimization procedure. After these preparatory steps, the part geometry is divided into
N segments, according to the gripper count
N.
The gripper count and diameter are defined based on the expected process-specific forces at the gripper-part-interface in order to provide the required holding force. The mass distribution of the part is approximated by the equidistant distribution of mesh vertices. Due to the 3D to 2D projection, information about the real three-dimensional mass distribution are lost. The segmentation is implemented in such a way, that it slices the part by counting the mesh vertices in polar coordinates from a start angle
α. If the part is graspable at the center of mass, the first segment (circular) is generated exactly at the center of mass, the remaining segments are generated as described above. The initial grasp points
PI are then positioned in the mass center points of each segment, as initial solution for the optimization algorithm. Regardless of the part’s surface geometry, this would distribute the part’s mass evenly over the vacuum grippers. In order to cover the entire global solution space, not only one specific part segmentation is done, but the start angle for the polar coordinate-based vertex counting procedure is varied, resulting in
P different segmentation variants. Further details on the segmentation can be found in [
6].
In rare cases, it may be possible that the initial grasp points are located in the graspable area, hence the grippers can be positioned exactly at the initial grasp points
PI. However, this is unlikely for most cases. Hence, the initial grasp points must be moved to the graspable area. To evaluate if a certain combination of moved grasp points is feasible, three fitness criteria are introduced. Firstly, it is crucial that the eccentricity of each grasp point to the segment-wise mass center point is minimal in order to avoid additional moments of inertia, which would require to provide a higher holding force. Hence, the first criterion (distance criterion) can be derived by applying the principle of virtual work [
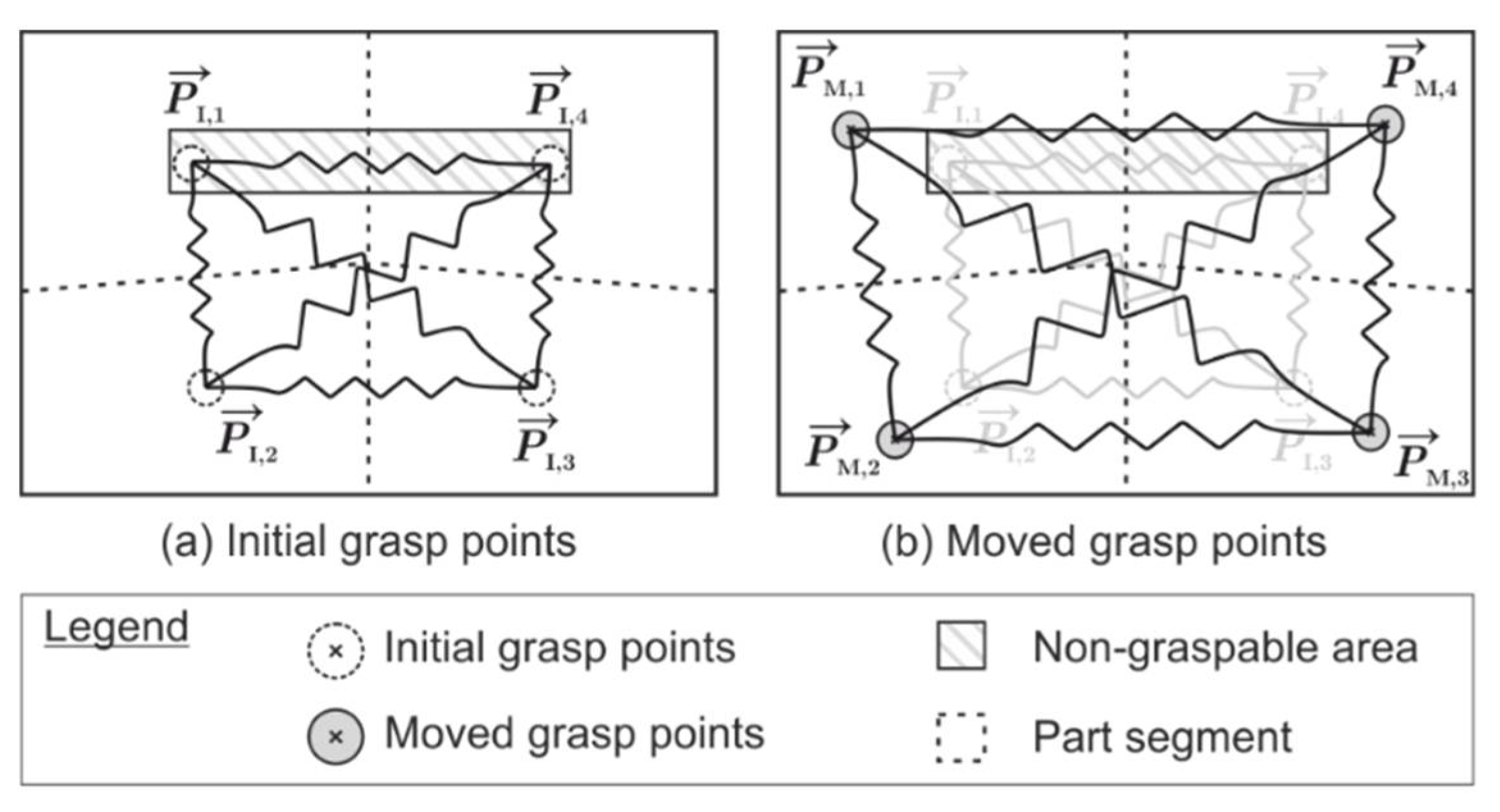
21]. In specific, all grasp points are interconnected by non-elongated virtual springs (
Figure 3a). If the position of any of the grasp points is changed, the virtual springs are elongated accordingly (
Figure 3b).
Applying a virtual force Fi in order to elongate the spring by Δli requires to perform virtual work , where and c is the spring stiffness. For all springs, the same stiffness c is selected in order to equally weight the influence of each spring for the fitness criterion and not prioritize certain point pairs. The spring elongation Δli can be represented by the distance between two grasp points that is added, when at least one of those two points is moved. Hence, in order to calculate the virtual work that must be performed by every grasp point PI in connection with all other grasp points, the following criterion is introduced (Equation (1)):
Criterion 1. Distance Criterion. Minimize the distance between every initial grasp point PI and the corresponding shifted grasp point PM.
Secondly, it is crucial that the eccentricity of common center point of all aggregated grasp points to the part’s mass center point is minimal in order to minimize additional moments of inertia. This is limited by the following criterion (Equation (2)):
Criterion 2. Center of Mass Criterion. Minimize the distance of the common center of mass of all moved grasp points to the center of mass.
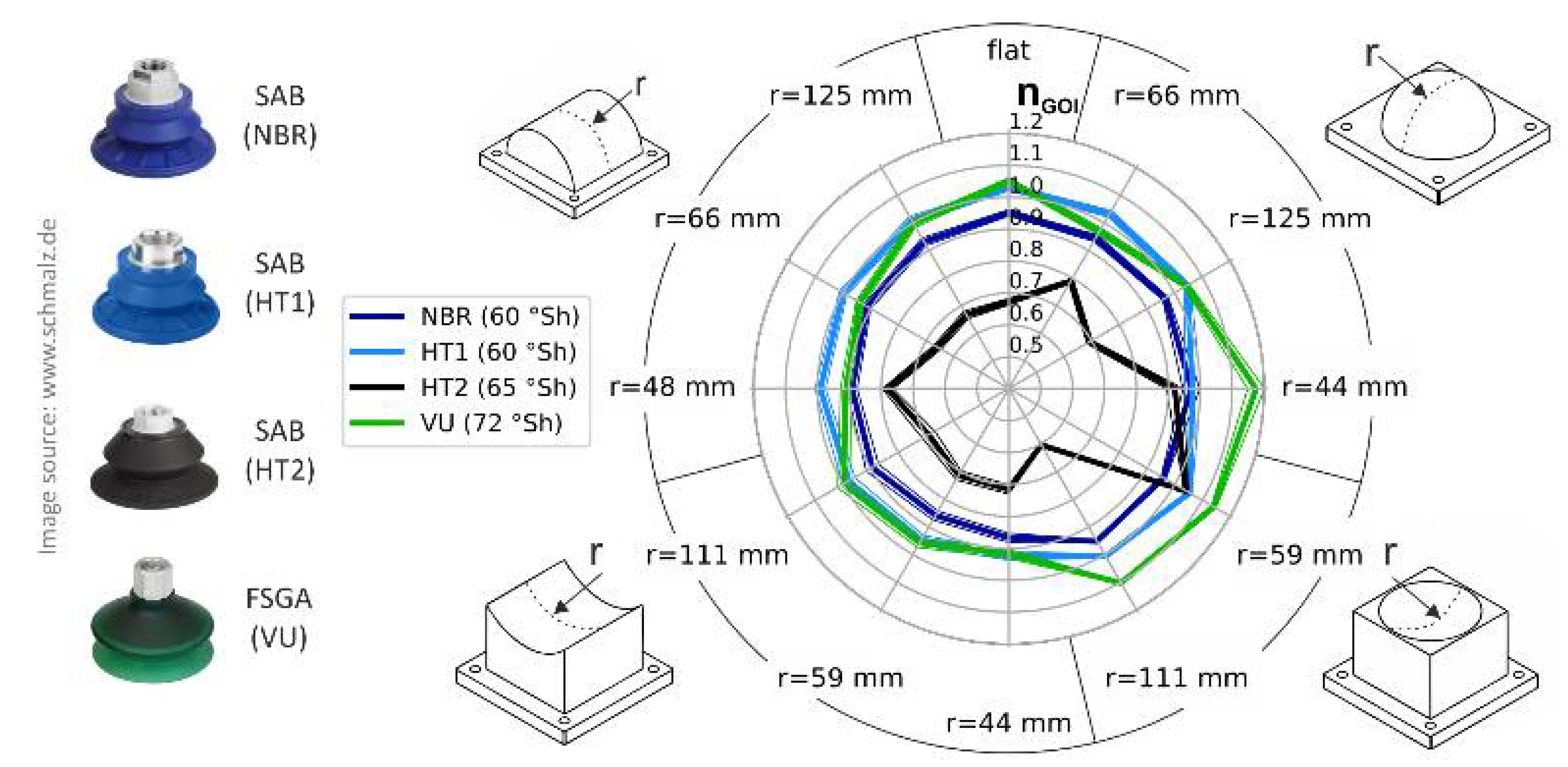
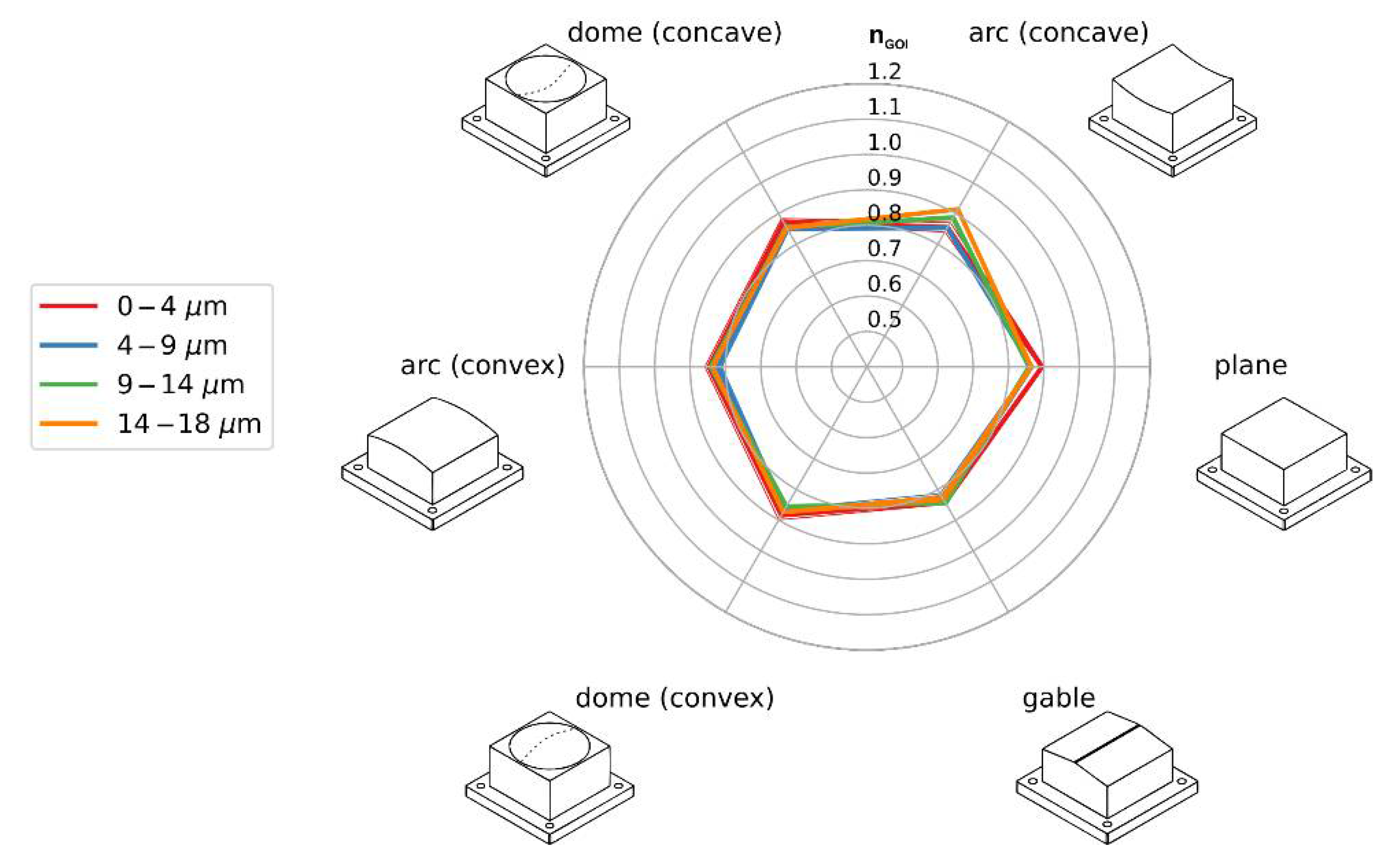
Thirdly, gripper-object-specific information considered in order to maximize the achievable holding force in normal load direction. In preliminary work, the obtained information about the achievable holding force was normalized by the maximum holding force information provided by the manufacturer, in order to quantify the actually achievable force for specific objects. The results of this preliminary work are depicted in
Figure 4. The parameter
nGOI describes the relation between the manufacturer provided values and the experimentally obtained information. For
nGOI = 1, the specified force matches the experimental values,
nGOI < 1 means that the experiments show smaller holding forces.
The third fitness criterion considers the gripper-object-specific values for nGOI and aims to maximize the achievable holding force for a given amount of grippers with a specified diameter (Equation (3)). The values of nGOI, that are specific to each possible grasp point within the graspable area, are provided as list to the algorithm.
Criterion 3. Formfit Criterion. Maximize the achievable holding force.
2.2. Investigation of the Roughness-Dependent Maximum Holding Force
In preliminary work, the achievable holding force was investigated for specific combinations of surface geometry and gripper type in [
3] and used in the optimization method [
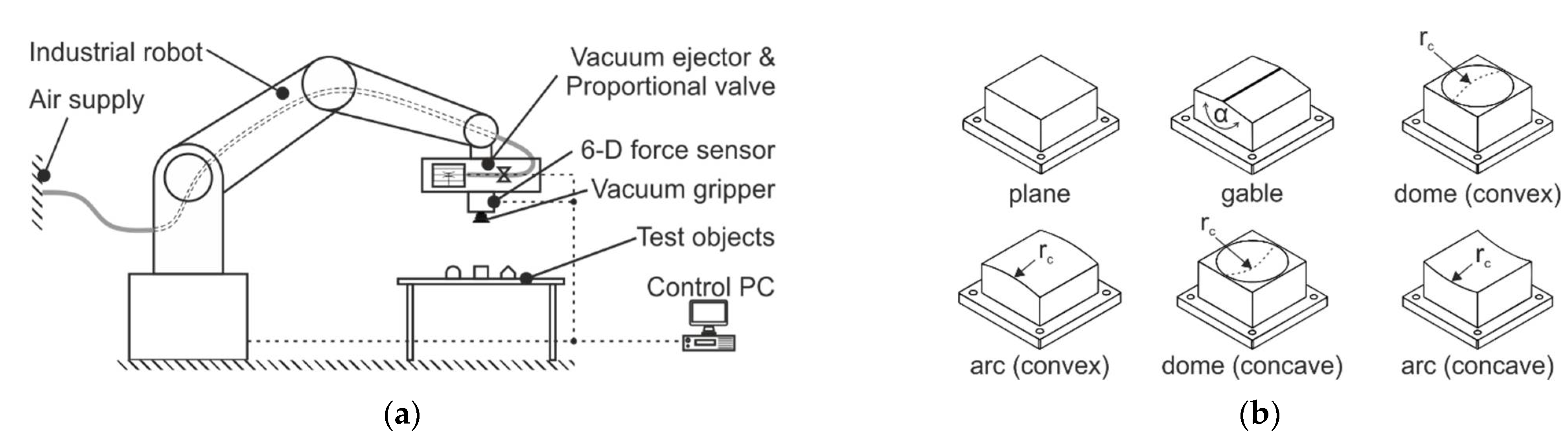
6] to evaluate the formfit criterion (Equation (3)). For the extended method introduced in this work, the influence of the object surface roughness is investigated. The used experimental setup is depicted in
Figure 5a. For the roughness-specific experiments, six test object geometries were selected (
Figure 5b) representing possible shapes of press-formed sheet metal: a plane as reference, a gable (
α = 135°) with a 5 mm round, a convex dome (
rc = 125 mm), a convex arc (
rc = 125 mm), a concave dome (
rc = 111 mm) and a concave arc (
rc = 125 mm).
rc is the curvature of the curved objects.
The experiments were conducted with one exemplary vacuum gripper with 1.5 foldings, made of SAB 60 nitrile butadiene rubber by J. Schmalz GmbH (Glatten, Germany). Since vacuum-based handling is extensively used in handling of sheet metal, e.g., in press shops in the automotive industry, a mean roughness range of 0.4 µm ≤ RZ ≤ 15 µm is taken into account, as this range is usually resulting from typical forming techniques such as deep-drawing [
22]. In order to create multiple different values of the surface roughness within the regarded range of 0.4 to 15 µm, the test objects (test area 80 × 80 mm) were manufactured from aluminum, steel and PA6 and then ground (WIWOX EK 080 with grain size 150–212 µm, WIXOX GmbH, Erkrath, Germany). Four different roughness ranges resulted from exemplary roughness measurements. Five measurements were conducted per material with a Hommel Wave S10 skidded gage (Hommel GmbH, Cologne, Germany), with a T1E 2 µm probe tip and a test distance of 15 mm. The resulting mean values for the surface roughness (±standard deviation) are given in
Table 1. From these measurements, discrete roughness ranges were derived for a straightforward generalization of the obtained results.
Resulting from the fabricated and grinded test objects, 24 different test objects are available for experiments (six object geometries, four roughness values). The objective of the experiments is to determine the maximum holding force. Firstly, the gripper is fully attached to the test object, evacuated by the used vacuum ejector and then pulled off completely at a velocity of 10 mm/s (in orientation to the test velocity for tensile testing of rubber [
23]). Thirty repetitions were scheduled for each object, resulting in a total of 720 repetitions. These 720 repetitions were randomized in batches of six repetitions and then carried out fully automated in the above introduced robot-based test setup.
Figure 6 shows the results of the roughness variation experiments. The comparison with the results obtained in [
3] indicates that the influence of the roughness on the maximum holding force is relatively small and can therefore be neglected in the regarded range of 0.4 µm ≤ R
Z ≤ 15 µm.
Although no significant influence of the surface roughness on the achievable holding force could be determined for the regarded roughness range, in the following section it is examined to what extent the surface roughness influences the leakage between gripper and object. This enables calculating the corresponding compressed air consumption for evacuating the dead volume (internal volume) of the gripping system and therefore dimensioning the gripping system in such a way, that the resulting compressed air consumption is minimized.
2.3. Investigation of the Influence of Surface Roughness on Leakage
Leakage is one major drawback for energy-efficient vacuum-based handling processes. Hence, it is particularly relevant to consider leakage in the process of optimizing the grasp positions. To quantify the leakage of the selected (SAB 60) vacuum gripper dependent of the four above introduced roughness ranges, further experiments are needed, since existing work [
17,
18] only focuses on relative changes of ventilation time in a predefined gripping system with fixed gripper count, but does not provide absolute information that are generally useable. The same experimental setup was used as in
Section 2.2. Based on the assumption, that for all different object geometries a fairly identical roughness range has been created by grinding, solely the plane objects with four different surface roughness are considered in the scope of the leakage experiments. The findings in [
18] indicate, that besides the gripper size and surface roughness, also the applied normal force influences the leakage between gripper and object. Hence, after the initial touch-down of the gripper on the test object, the gripper incl. a dead volume of 85 cm
3 (3 m tubing with 8 mm outer diameter) was first evacuated to a target pressure difference of
500 mbar and directly elongated by a certain distance. The measured initial pressure difference exceeding 500 mbar can be accounted to the dynamic closed-loop control behavior of the used compact ejectors. The distance was varied in steps of 2/4/6 mm. After a waiting time of 5 s, the system was ventilated. With four test objects, three distance steps, four gripper diameters (30/40/50/60 mm) and 10 repetitions per parameter set, 4 × 3 × 4 × 10 = 480 test runs were scheduled. For each gripper, the associated 120 test runs were fully randomized.
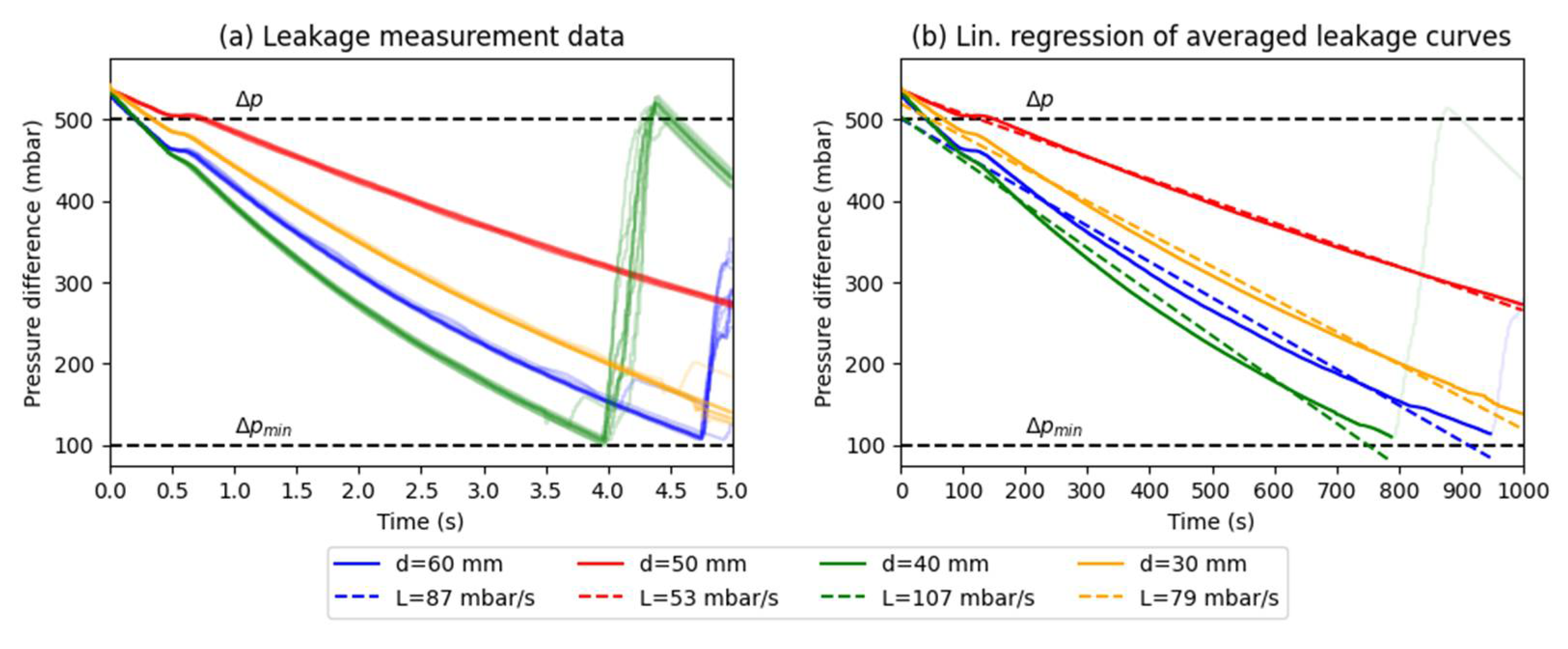
Figure 7a show the pressure difference profiles for all scheduled variations of normal force and surface roughness. The 10 repetitions for each respective setting were packed into one averaged profile. The influence of normal force and surface roughness may be present, but is significantly smaller than the influence of the gripper diameter. Therefore, all test runs for each gripper diameter were packed into one representative averaged pressure difference profile (
Figure 7b). For simplification, a linear regression was applied to these profiles to determine the leakage rate for the four different grippers in combination with the attached dead volume.
Normally, it would be plausible if a larger gripper diameter would correlate with a higher leakage rate, according to [
18]. The fact that the experimental results obtained in this work do not show this behavior may potentially be accounted to differences in the material batches utilized in gripper production. In [
18] it is shown, that a linear relation exists between leakage rate
L and dead volume
V. Therefore, the measured leakage that has been determined for a fixed dead volume of
V = 85 cm
3 can be directly calculated for different dead volumes. For an arbitrary gripping system with
N vacuum grippers, the resulting total dead volume
V divided by
N can be associated with each gripper. At the beginning of every process, the dead volume is initially evacuated in order to create the desired pressure difference
(
= atmospheric pressure,
= target pressure). The required evacuation time
with an ejector that supplies a suction flow
S can be computed by Equation (4). With the air consumption of the ejector
CEJ, the resulting compressed air use for evacuation
CE can be calculated by Equation (5):
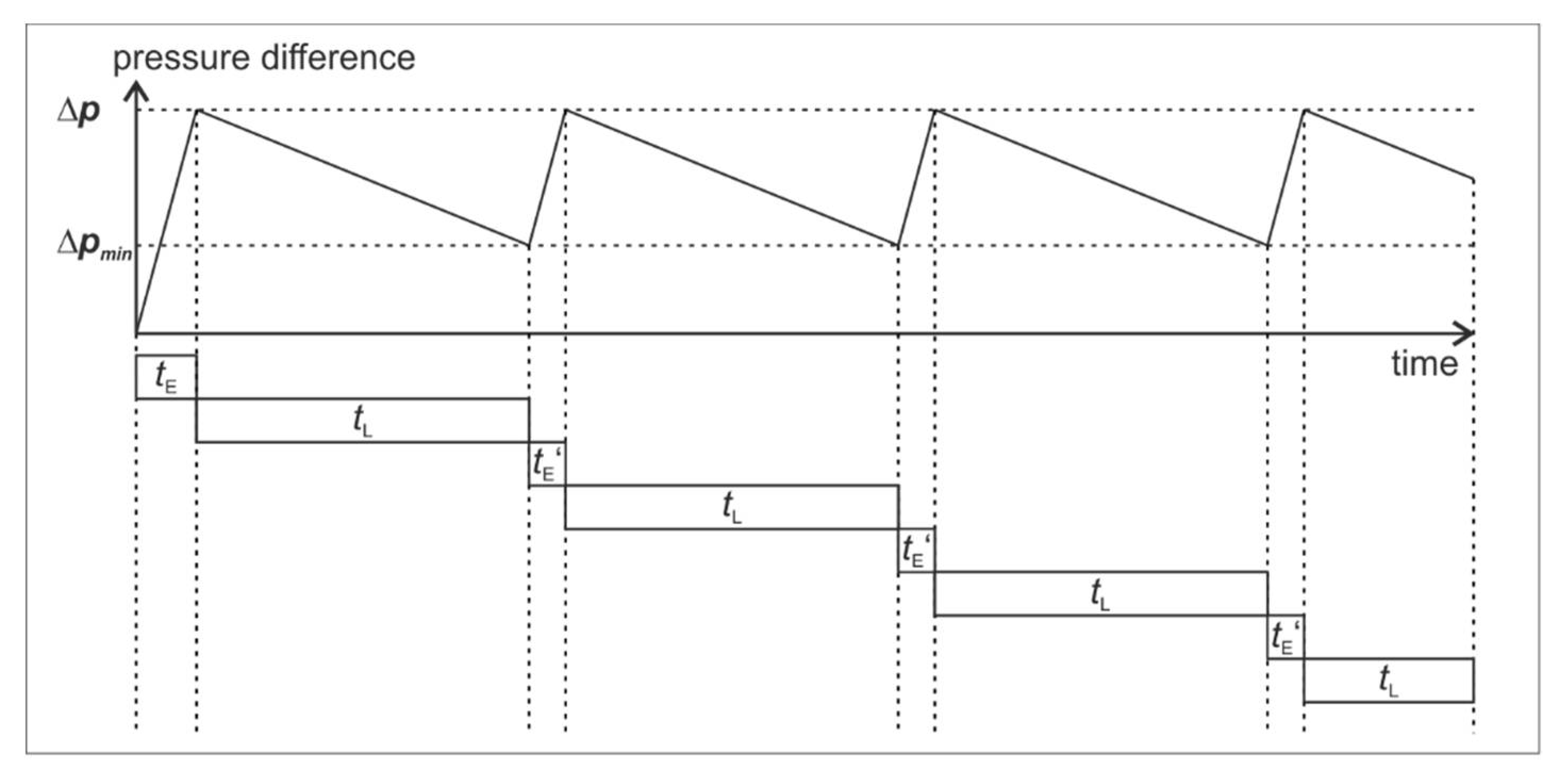
During the process, leakage causes a decrease of the pressure difference. Within a time interval of
t, the pressure difference drops by
from
to the minimally allowed pressure difference
(
Figure 8). Depending on the allowed lower limit for the pressure difference
, the ejector is operated for a duration of
in order to increase the pressure difference again up to
.
Hence, the duration of the entire process
is composed of the initial evacuation and multiple phases of leakage and re-evacuation, in the following (Equation (6)):
Firstly, it is determined how many complete cycles of leakage and re-evacuation are performed during the process. The remaining time interval
is computed in Equation (7):
Two different cases are possible. If the remainder is less than
, as shown in
Figure 8, Equation (8) can be used to determine the parameters
x,
y and
z in Equation (6). Otherwise, Equation (9) can be applied:
The required amount of compressed air for the entire process
C is composed of the air used for the initial evacuation
CE and for leakage-induced re-evacuation
CR during the process of duration
t. The used ejector supplies a suction flow
S at an air consumption of
CEJ. Equation (10) provides the required amount of compressed air for the entire process
C:
By this calculation scheme, depending on the specific leakage rate, the exact air consumption of arbitrarily configured gripping systems can be predicted with regard to a given process duration and the defined pressure difference values. Since the gripping system parameters are varied regardless of compressed air generation, in the proposed method, potential energy savings are approximated by the achieved savings in compressed air consumption.
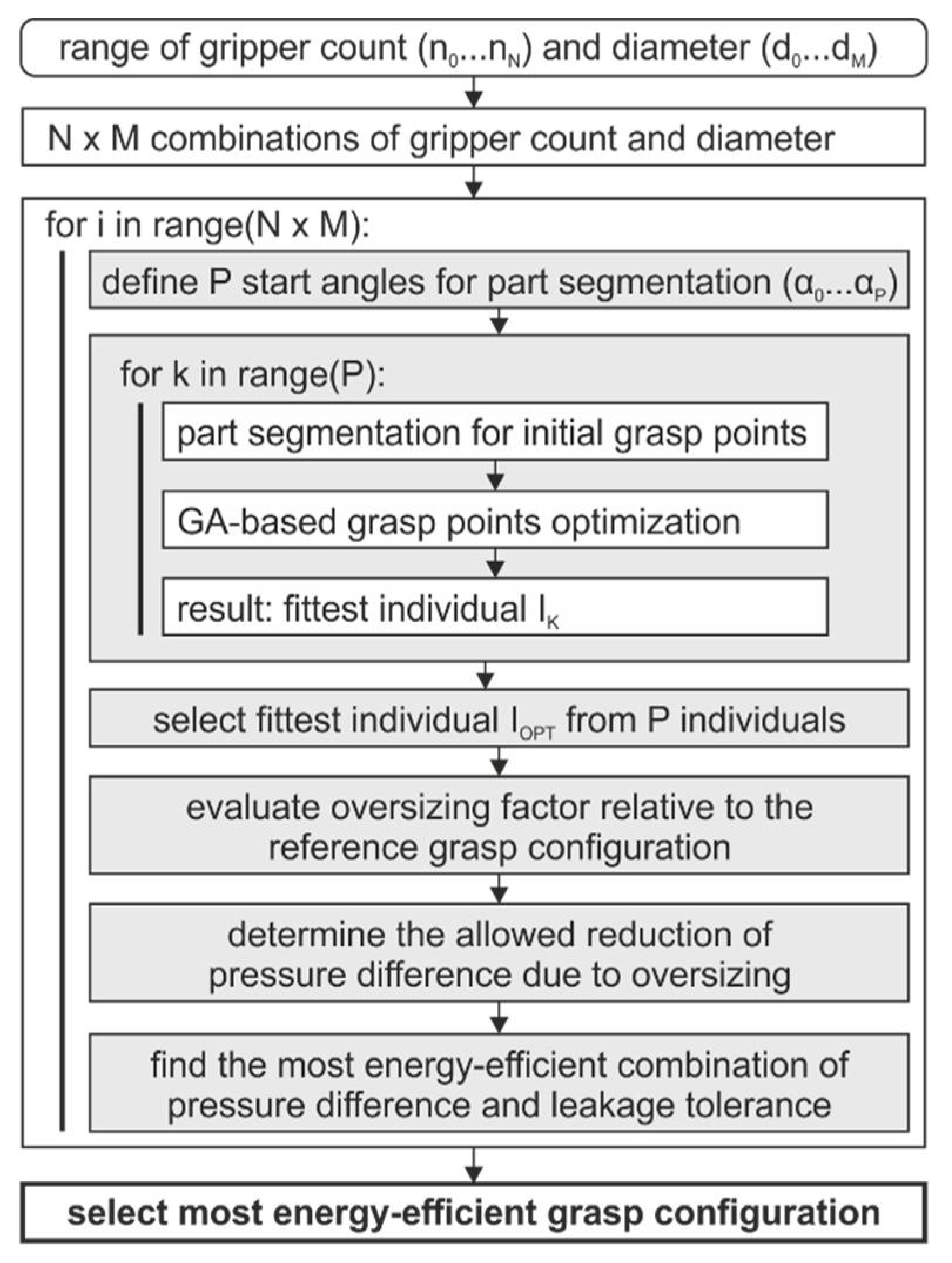
2.4. Combined Method for Grasp Optimization and Gripping System Dimensioning
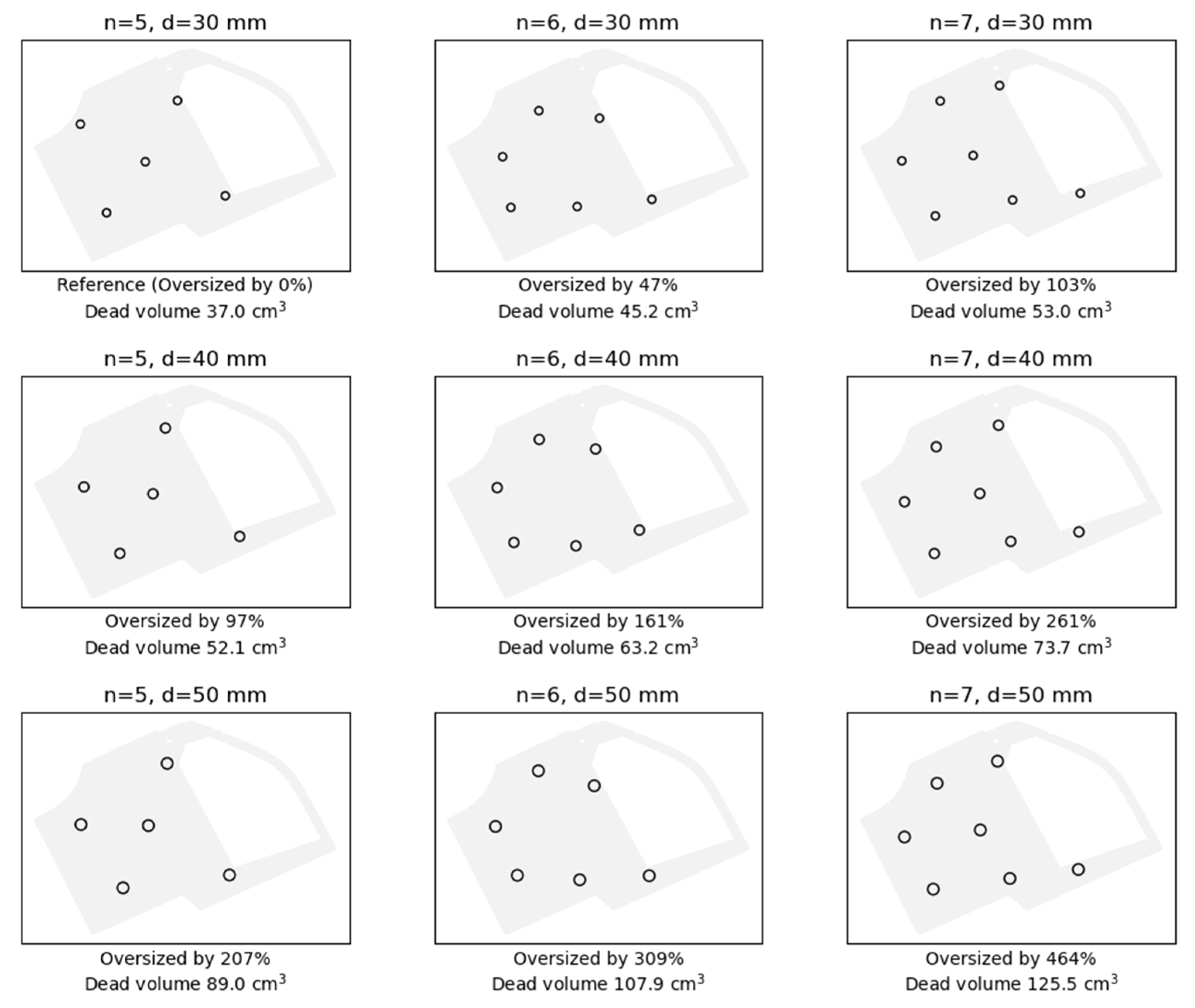
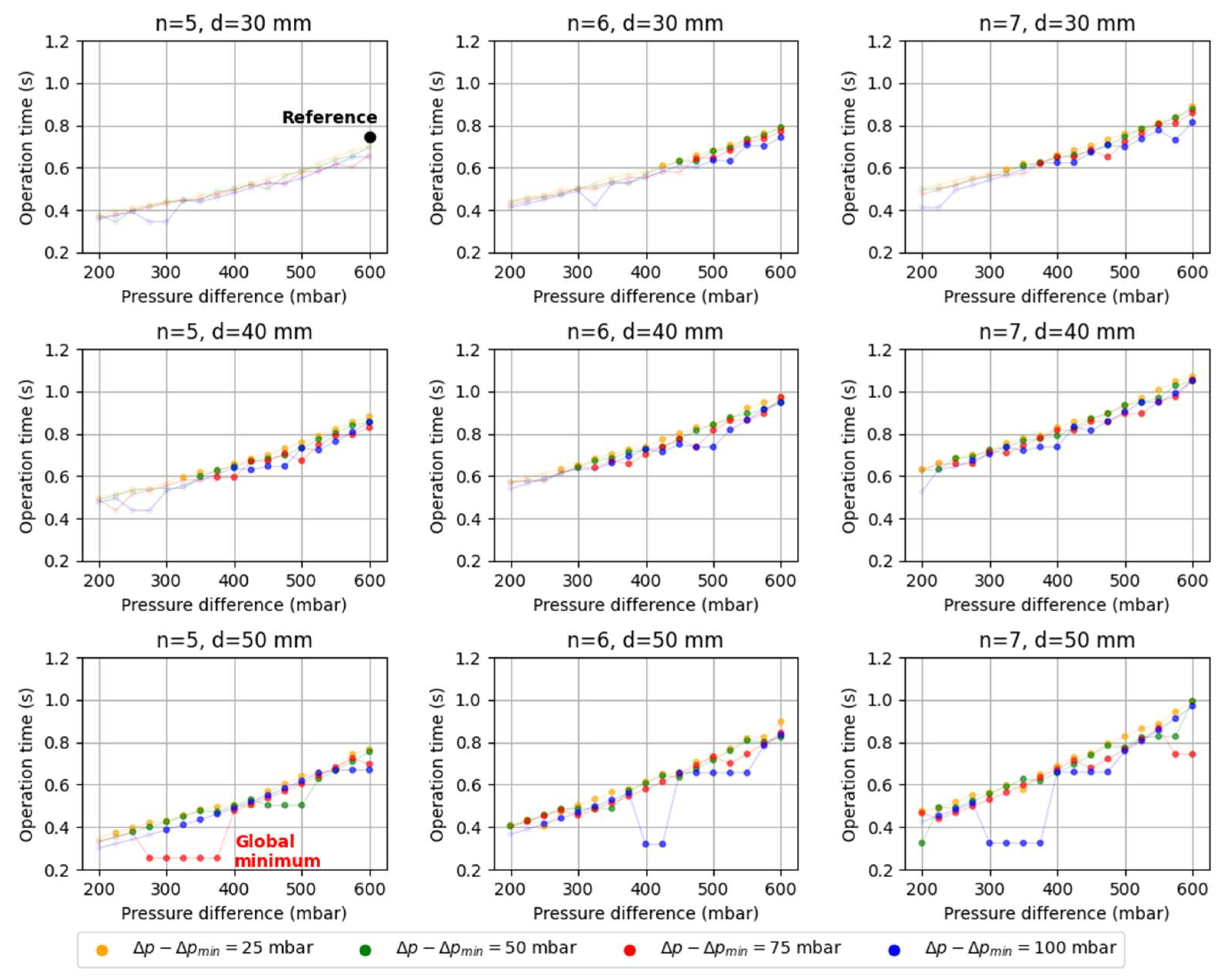
Based on the findings obtained in the above described investigations, the combined method for grasp optimization and gripping system dimensioning can be composed (
Figure 9). First, as alternatives to the reference grasp configuration with
n0 grippers of diameter
d0, a range of different values for the gripper count
n and the diameter
d is defined. For each of the resulting
Nx
M combinations, the grasp optimization is conducted. Subsequent to the grasp optimization, for each grasp configuration it is evaluated to what extent it is oversized relative to the reference grasp configurations. For example, if the gripper count is doubled relative to the reference configuration, the oversizing factor is 2. Hence, the target pressure difference could potentially be reduced to the half, since this would yield the same holding force. In combination with the target pressure difference, the leakage tolerance (i.e., the allowed drop in pressure difference, before the ejectors is operated again in order to reach the initially targeted pressure difference) can also be adjusted within the allowed range that is limited by the oversizing factor. By means of the gripper-specific leakage information and the calculation scheme for determining the air consumption depending on pressure difference
and leakage tolerance
, the resulting energy use can be predicted in the relevant value range for
and
. Finally, the most efficient set of gripper count, gripper diameter, target pressure difference and minimum pressure difference [
n,
d,
,
] is selected.