Analysis of the Torque Loss of High-Speed Transmission Mechanism with a Stacked Roller Set
Abstract
:1. Introduction
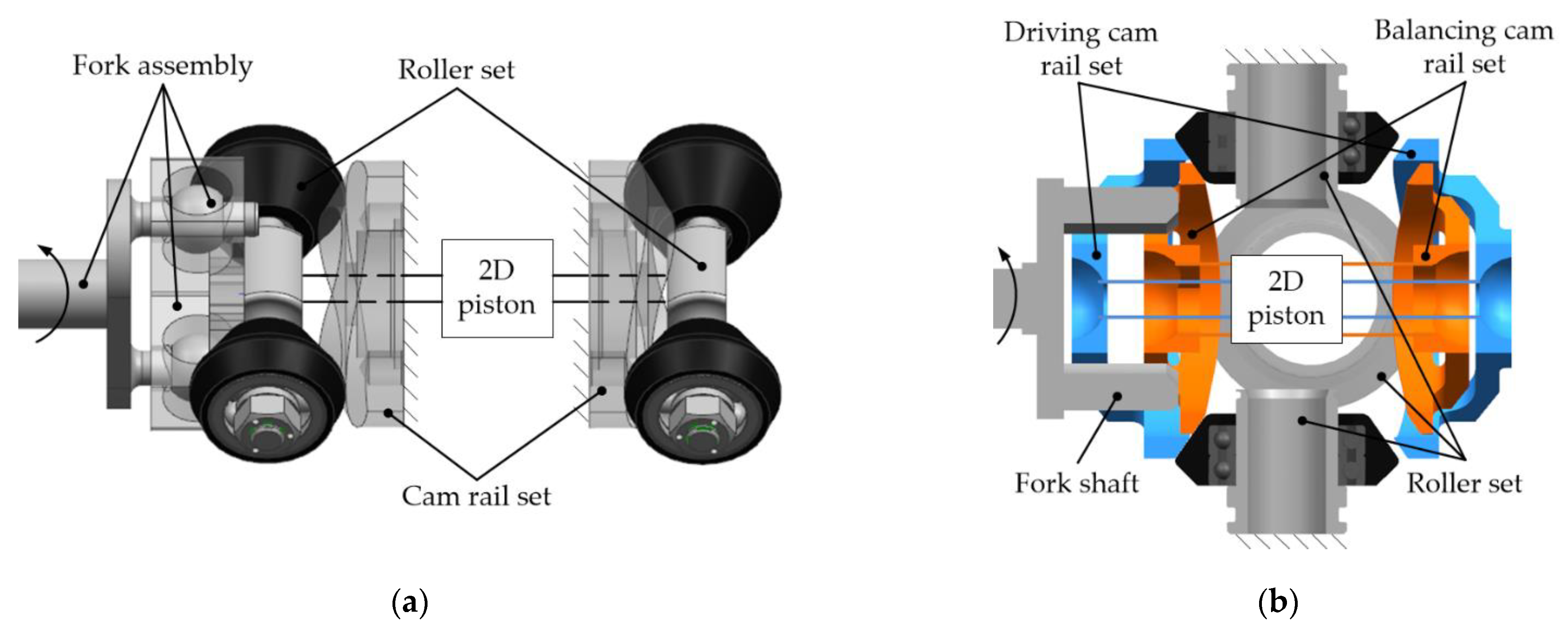
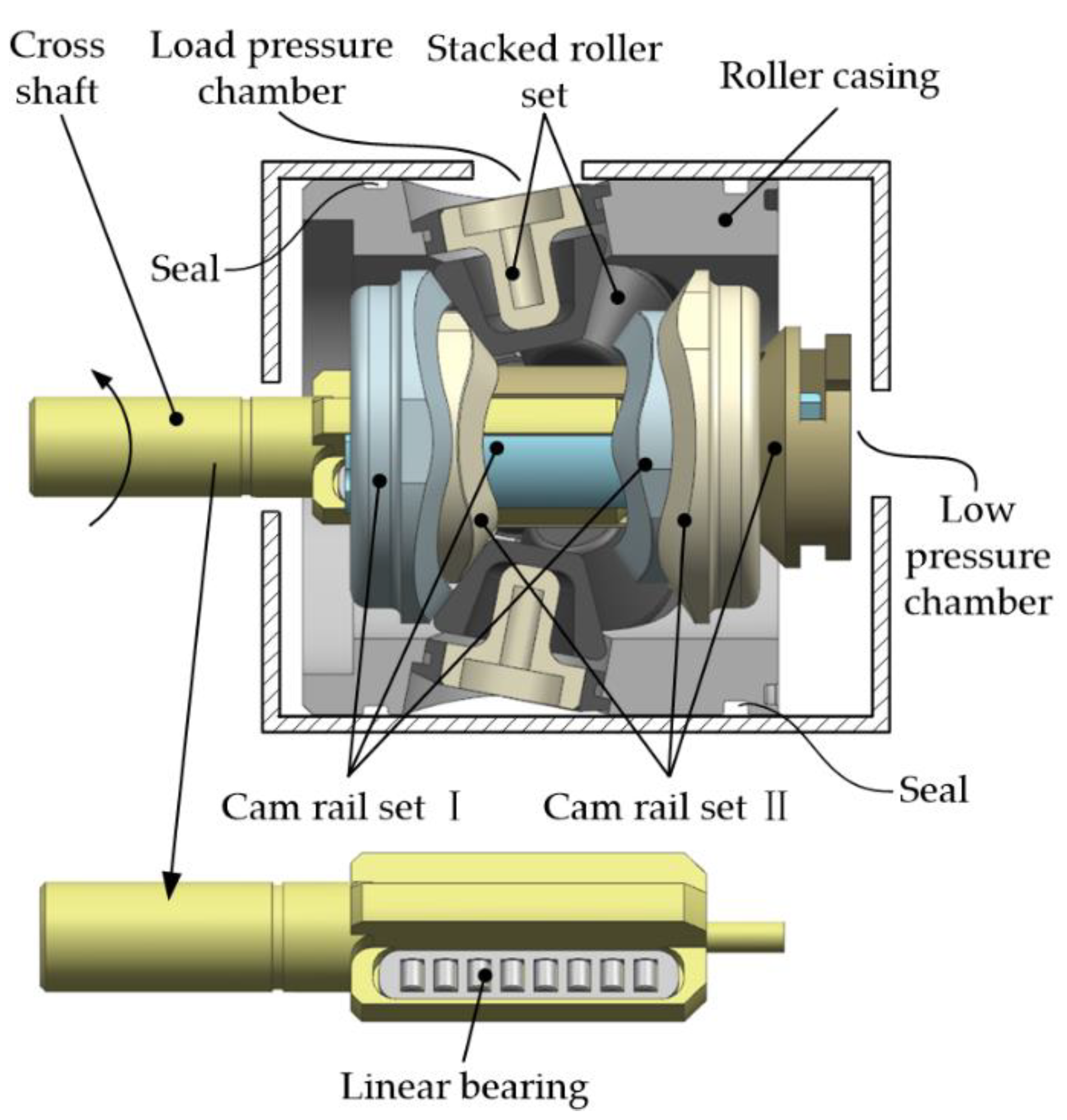
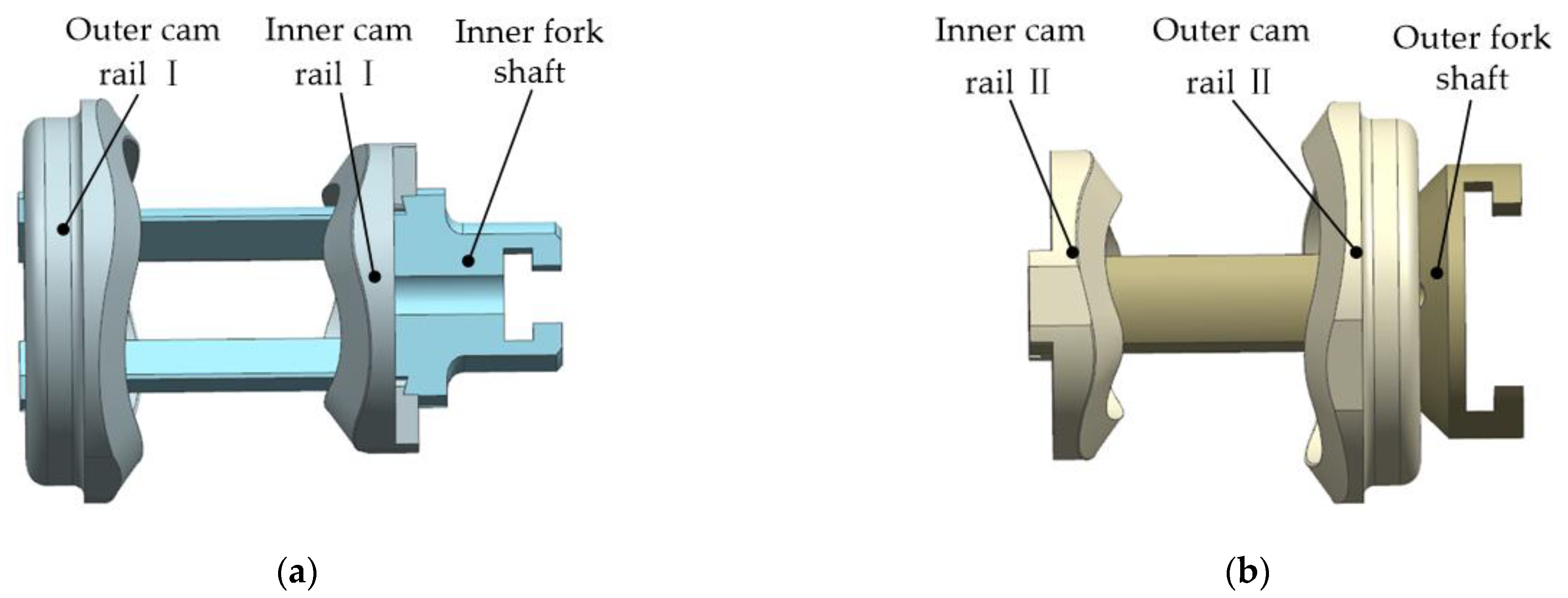
2. Structure and Working Principle
3. Mathematical Model
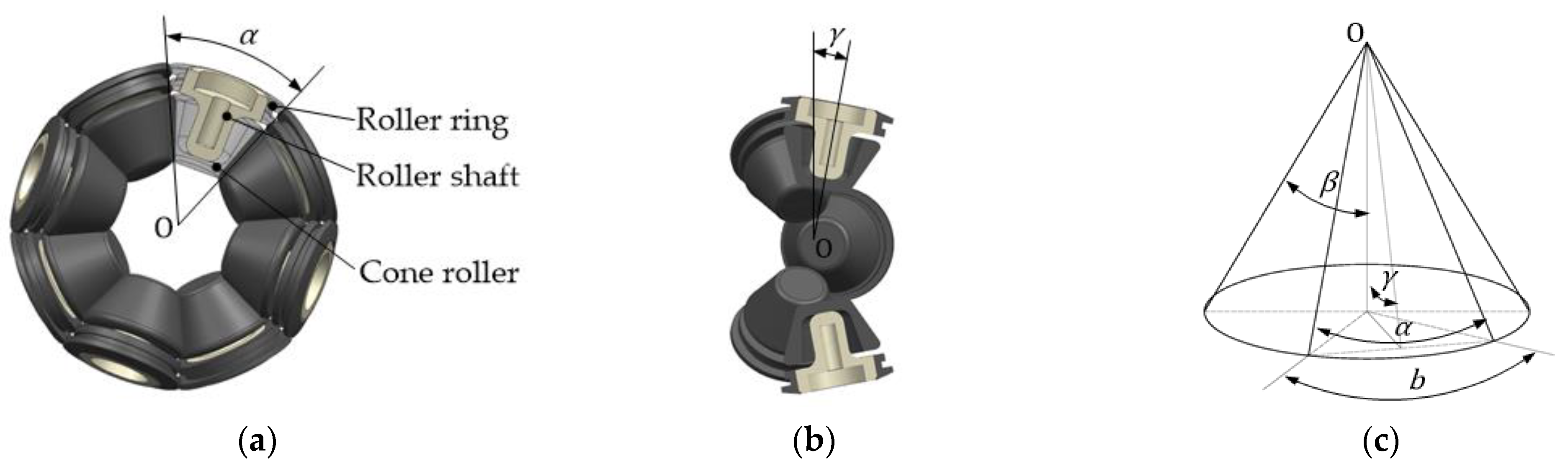
3.1. Cam Rail Surface Equation
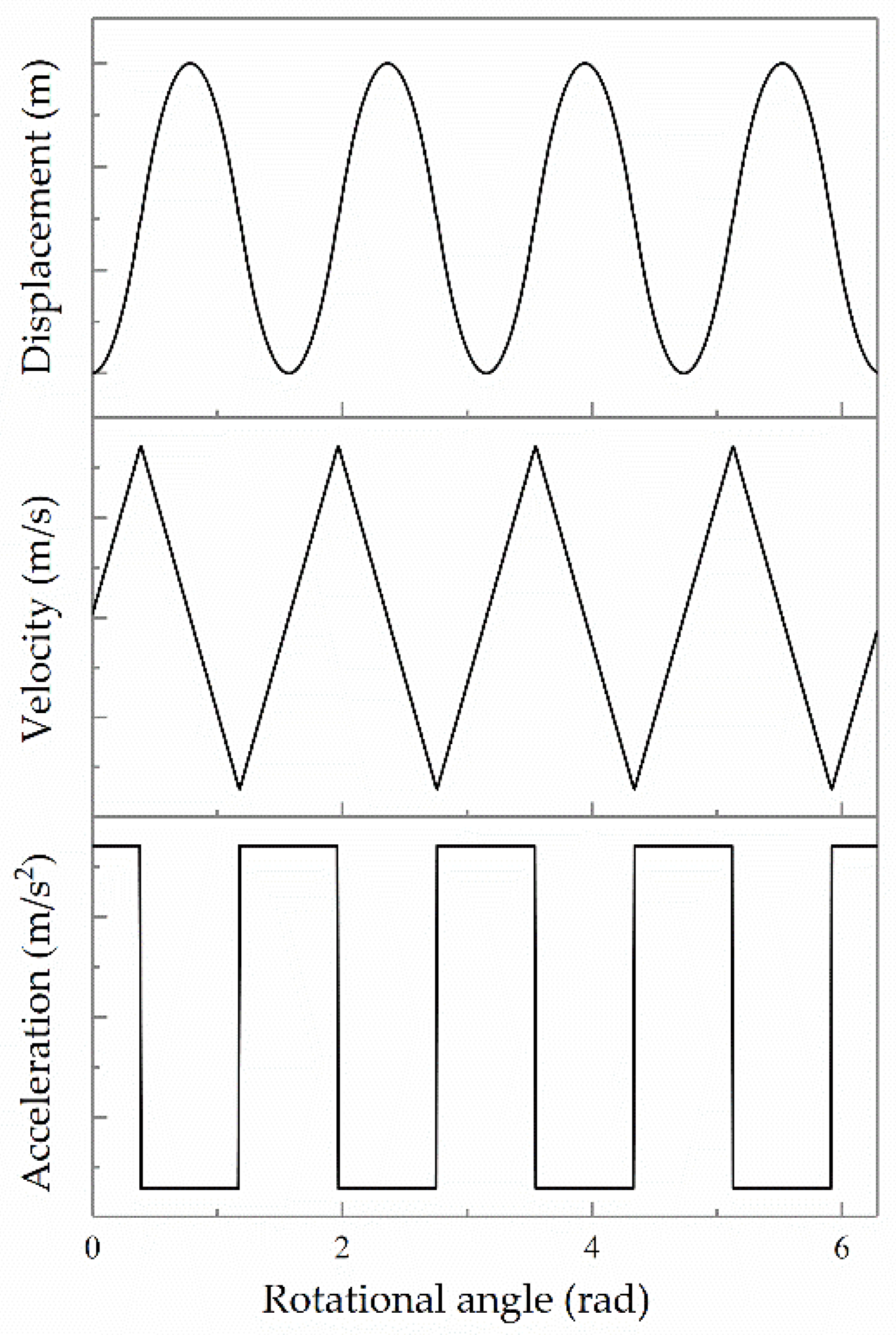
3.2. Space Angle Functions
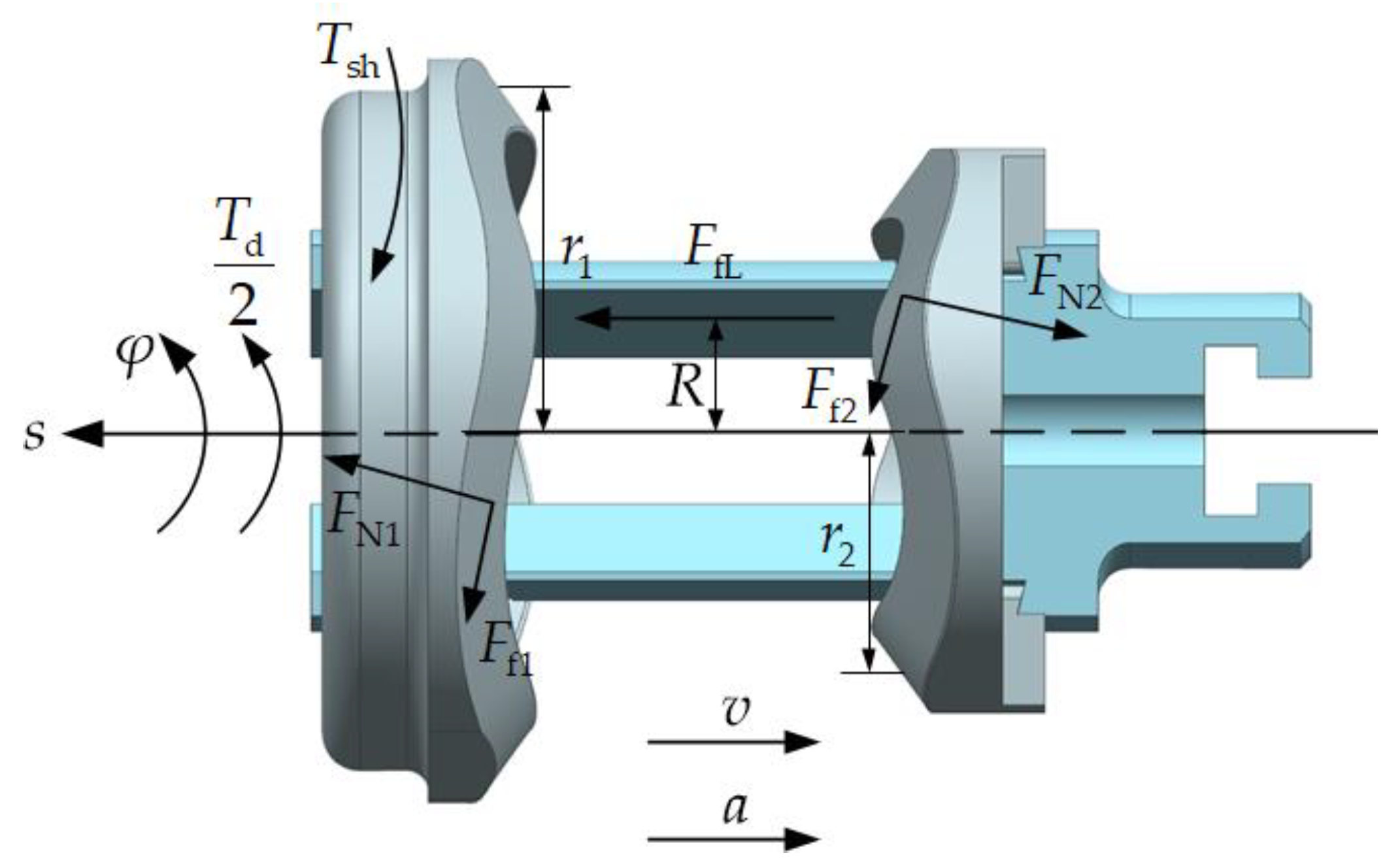
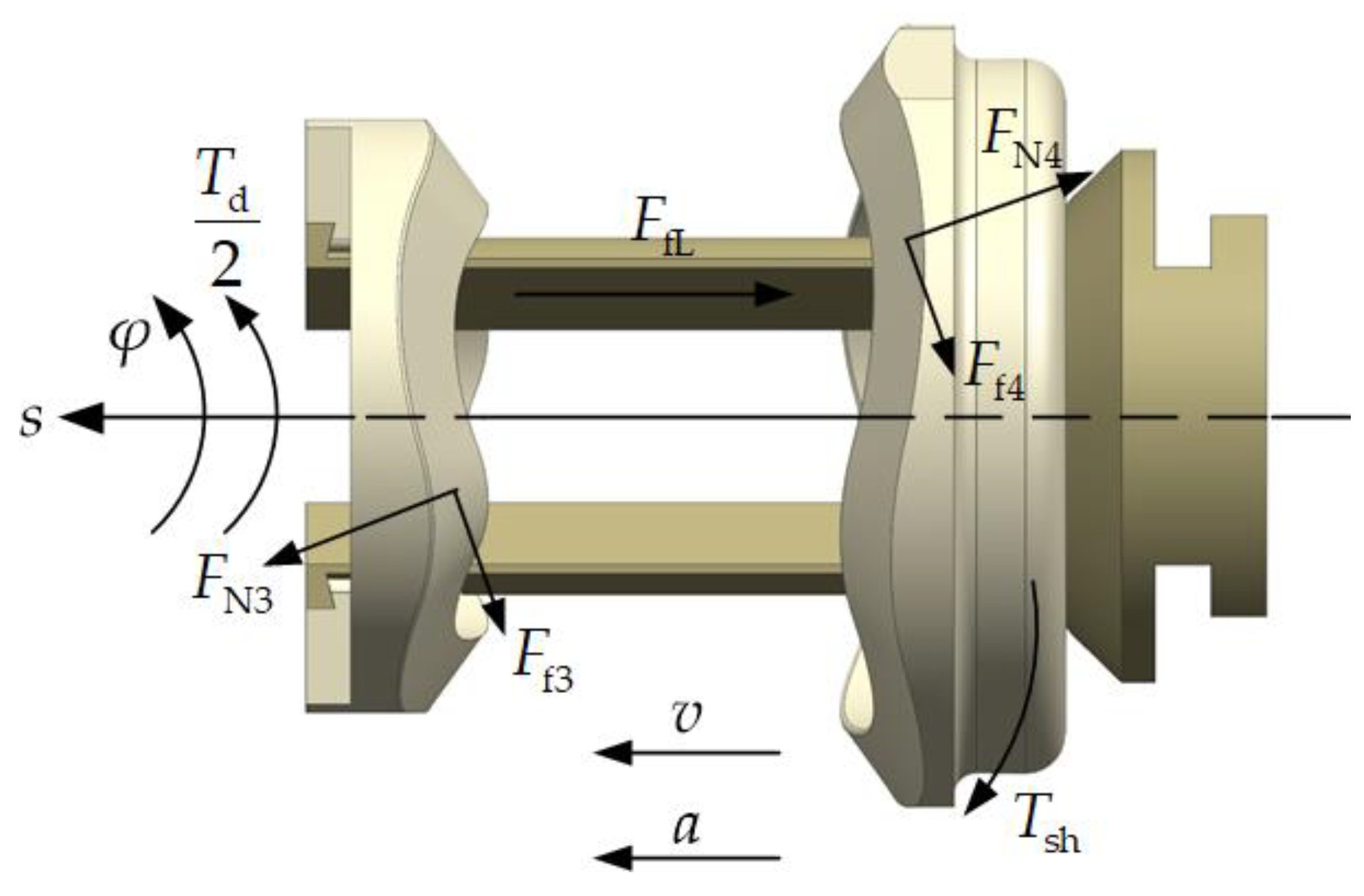
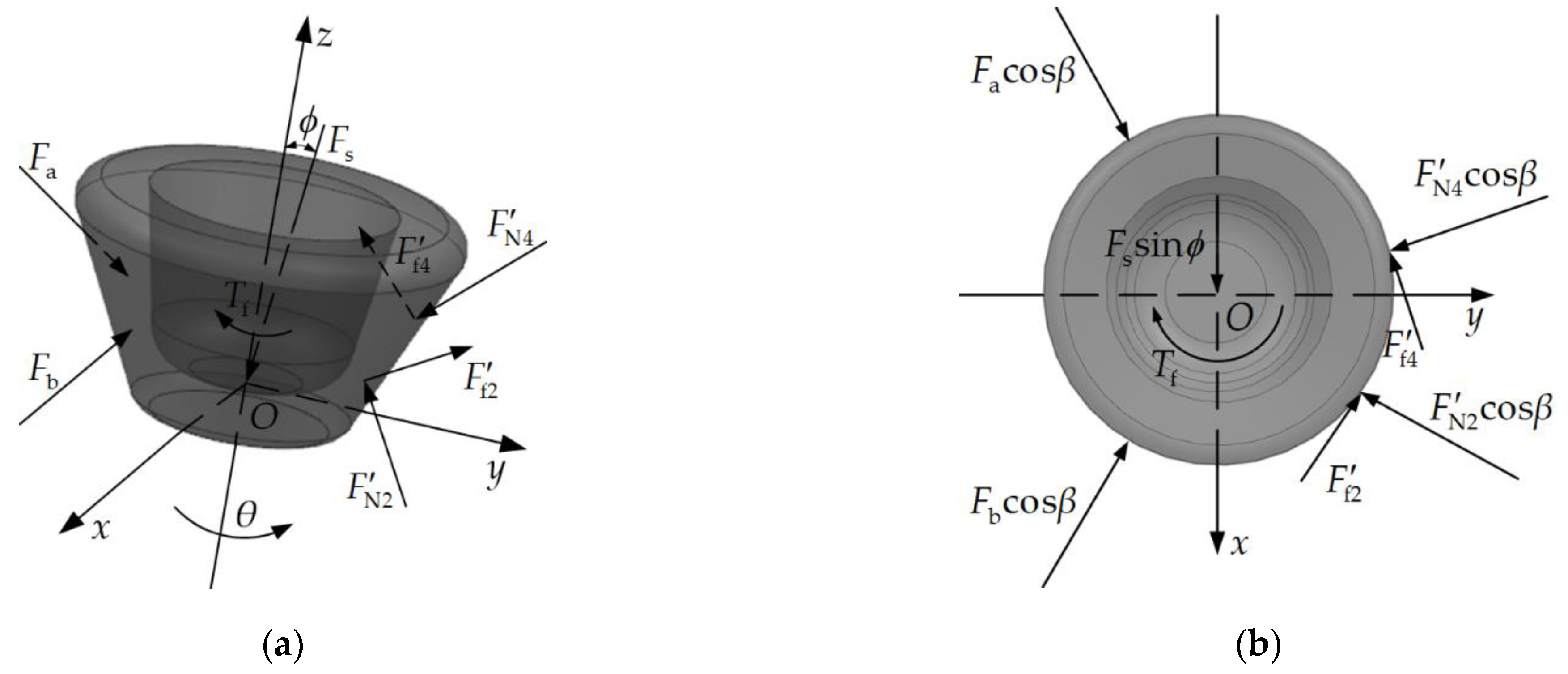
3.3. Dynamic Equilibrium Equations
4. Numerical Analysis
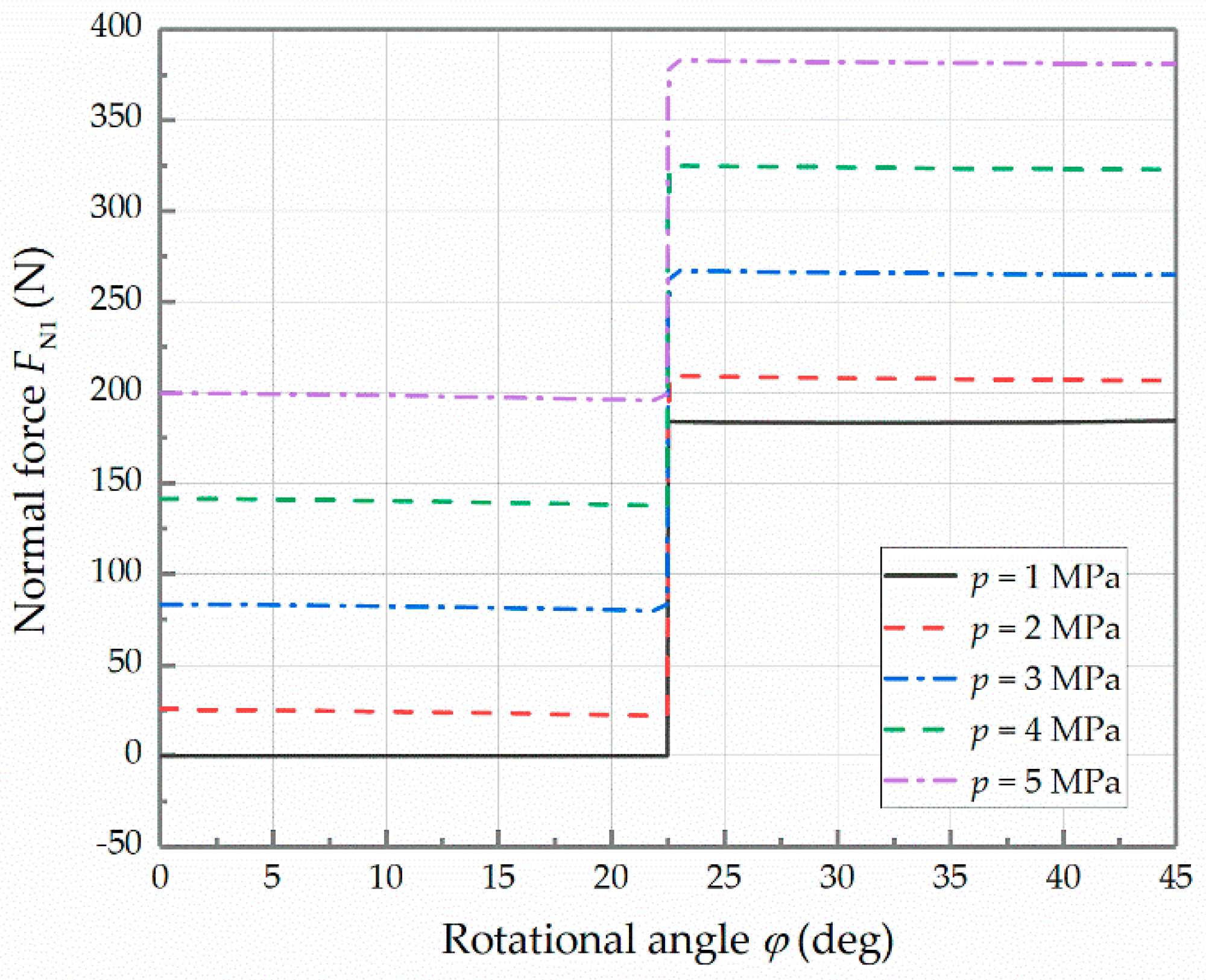
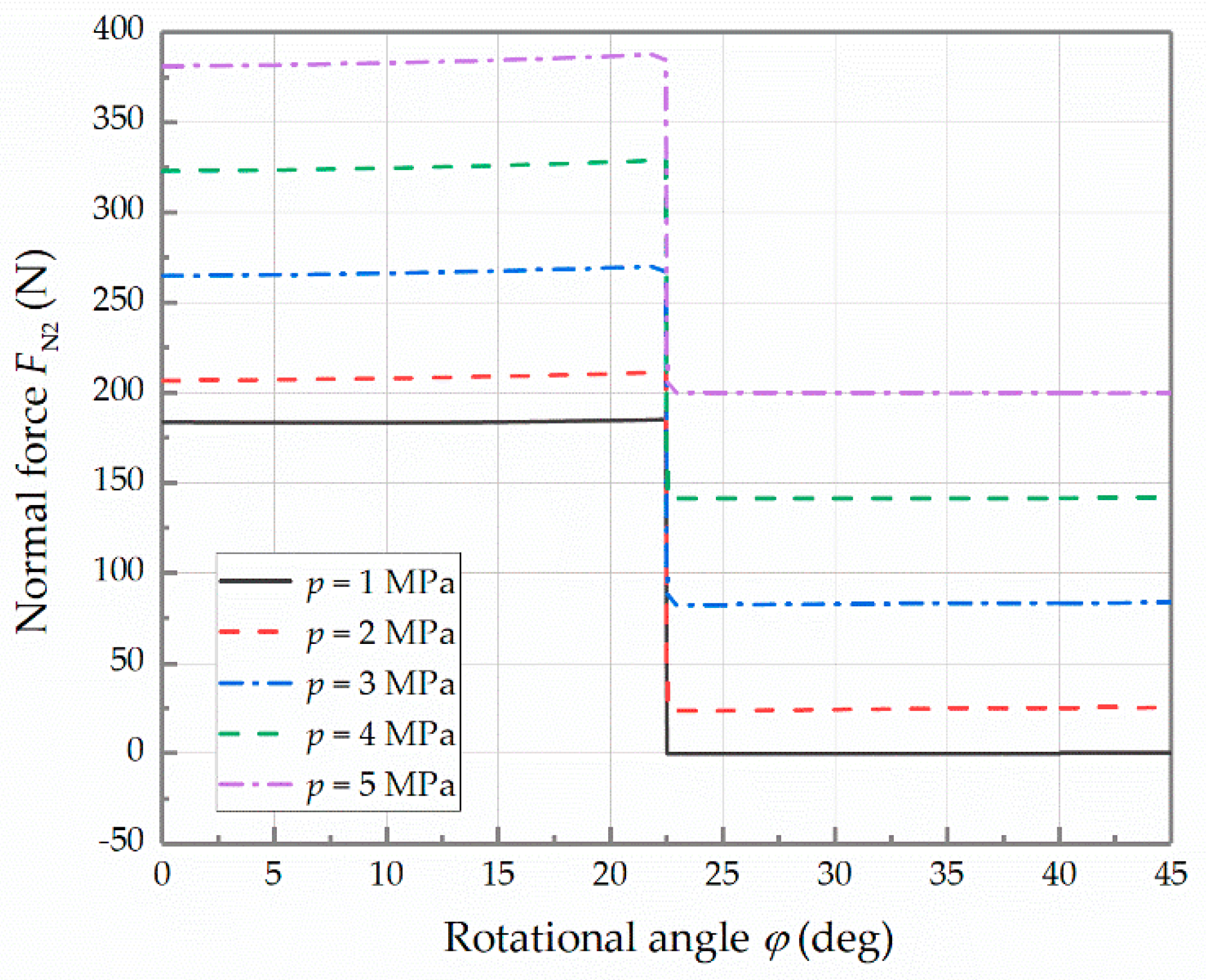
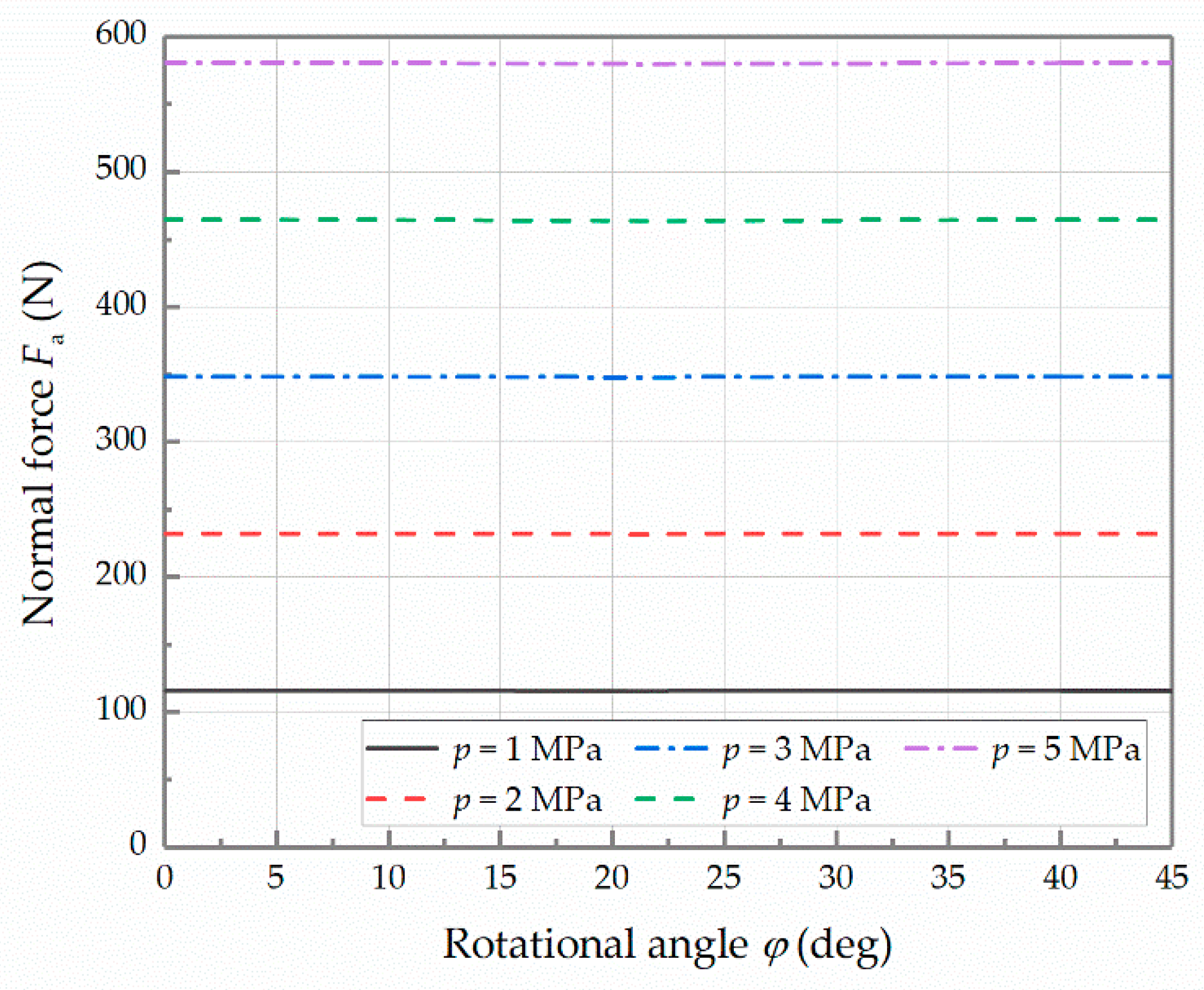
4.1. Analysis of Key Forces
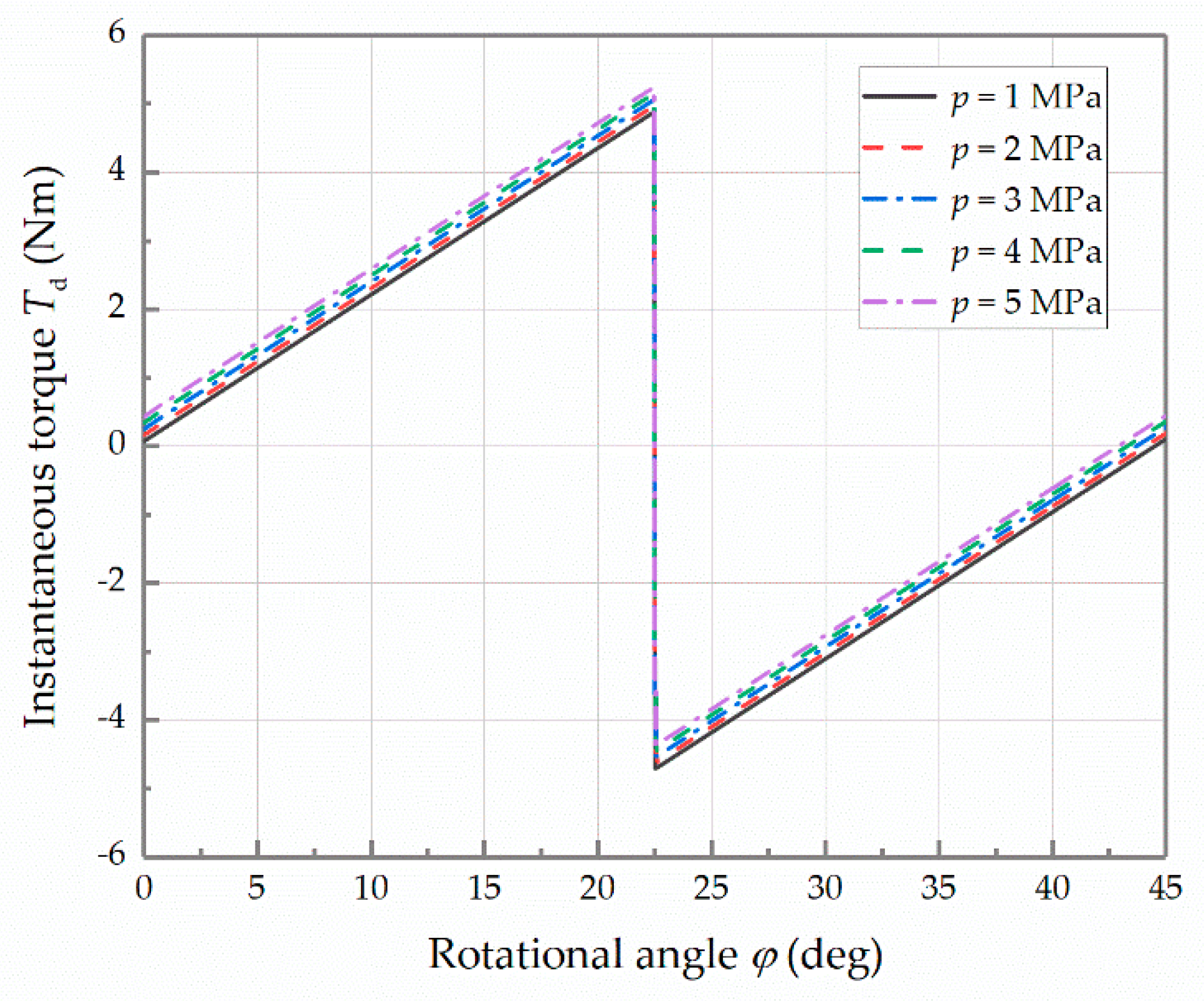
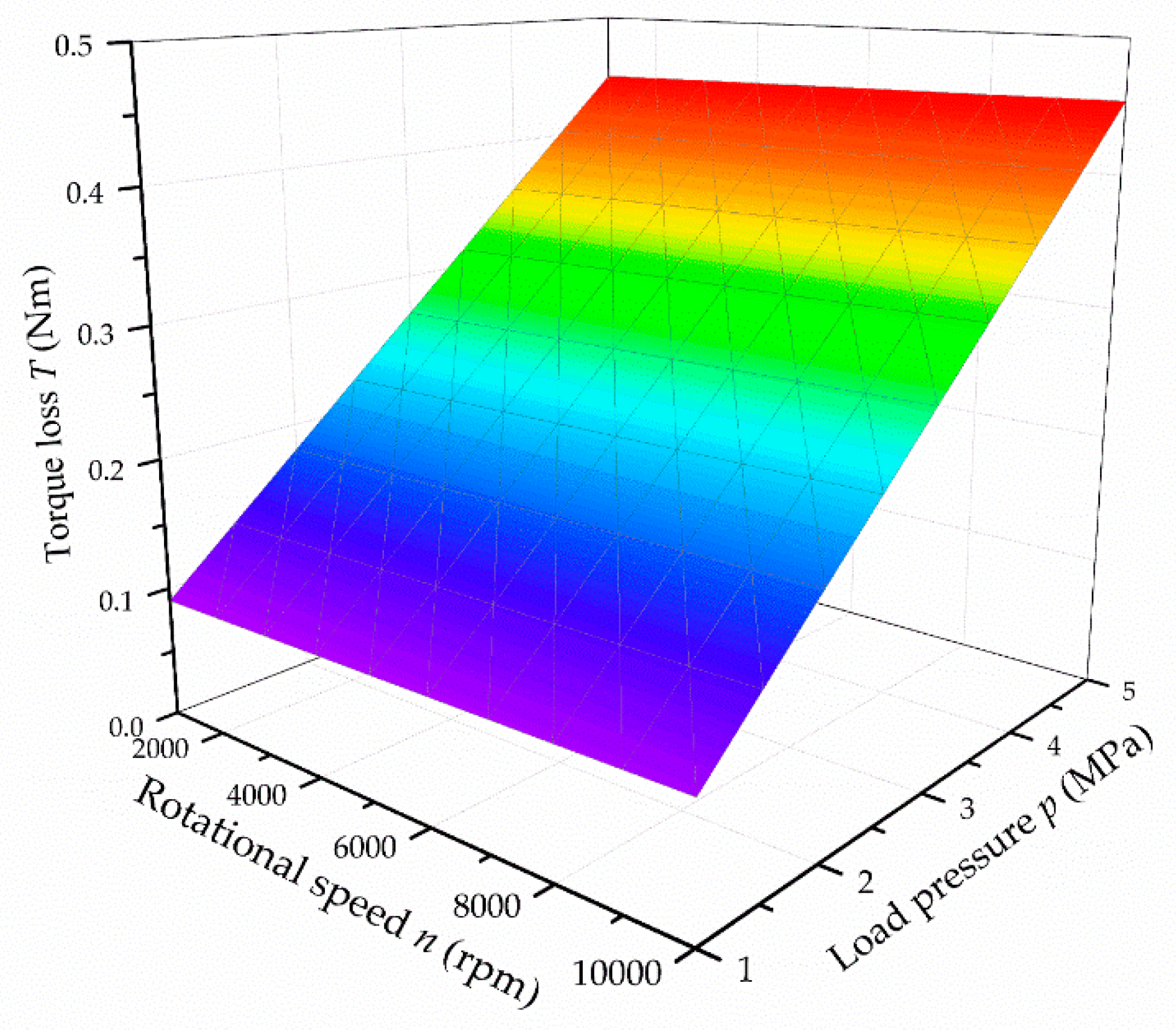
4.2. Analysis of the Torque Loss
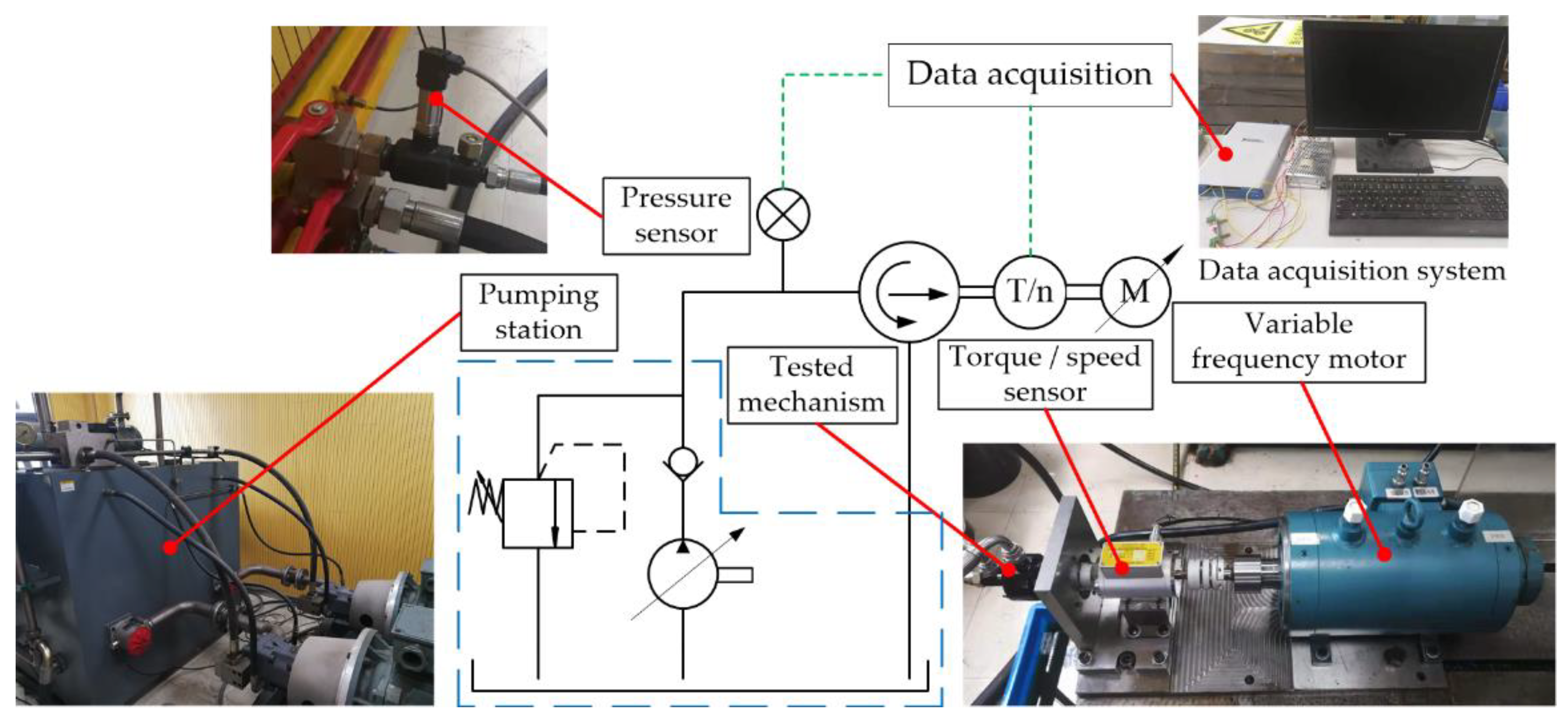
5. Experimental Research
6. Conclusions
- (1)
- According to the mathematical model, in the case of low load pressure, the normal forces, and , between the cam rail and the rollers have zero values. In this situation, there might be a contact clearance between the cam rail and the rollers on one side, which means it is invalid to press the stacked roller set to support the cam rail.
- (2)
- The validity of the mathematical model is verified by the experimental results. Combined the analytical results with the experimental results, the increment of the load pressure will enlarge the normal forces and the frictions, which raises the torque loss of the transmission mechanism significantly. Furthermore, the increase in the rotational speed will make the axial inertia forces of the cam rail sets increase. However, the influence of the rotational speed on the torque loss is slight, because the mass of the cam rail set is very small.
- (3)
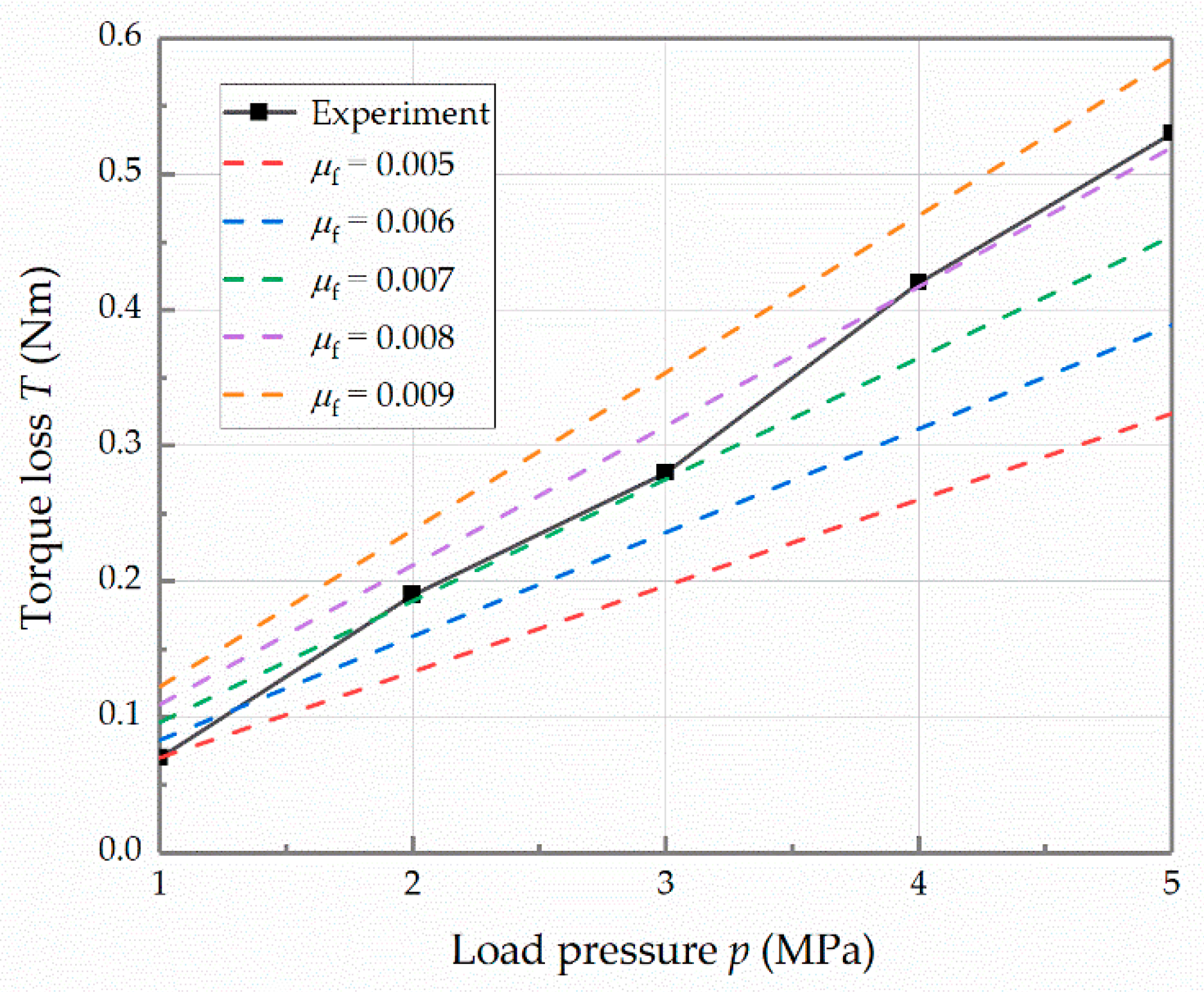
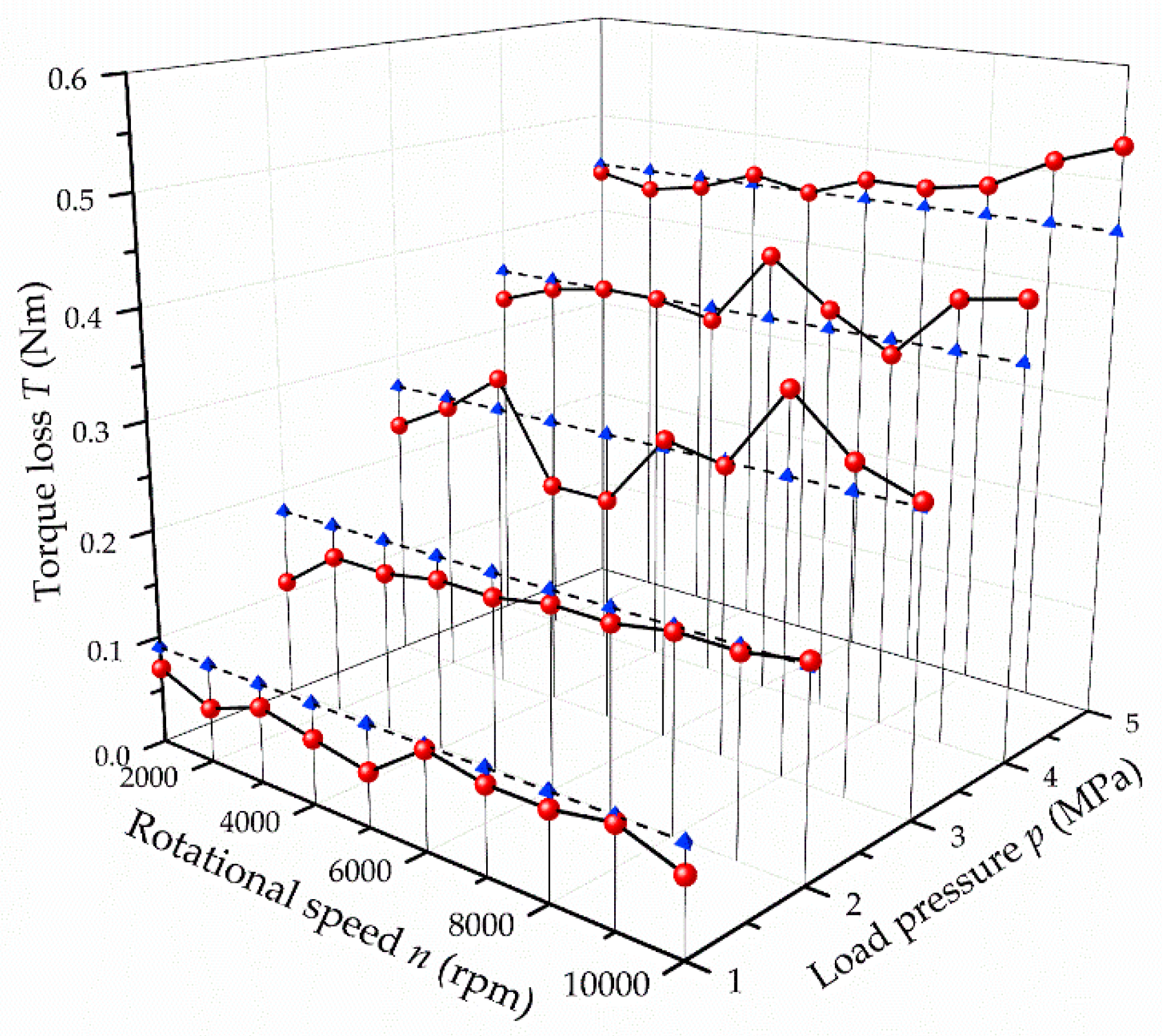
- In the case of high speed and high pressure, the viscosity of the oil decreases due to the temperature rise, which leads to the deterioration of the lubrication. Therefore, there is a growing trend of the friction coefficient. Overall, the average friction coefficient of the transmission mechanism is approximately 0.007. In addition, the torque loss at different load pressures and rotational speeds can be calculated based on the mathematical model. The errors between the theoretical torque loss and practical torque loss can be accepted when the load pressure is greater or equal to 2 MPa. Apart from the data when the load pressure is 1 MPa, the mean error is 8.9%. The overall mean error is 11.9%, which takes the data of the load pressure of 1 MPa into account.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| N | Number of cone rollers |
| α | Angle between two adjacent contact lines |
| β | Half cone angle of the cone roller |
| b | Angle between the projection lines of two adjacent contact lines |
| γ | Angle between the axis of the cone roller and the contact plane |
| s | Axial displacement of the cam rail |
| h | Stroke of the cam rail |
| φ | Rotational angle of the cam rail |
| L | Distance from any point on the surface of the cone roller to the plane X3O3Y3 |
| θ | Rotational angle of the cone roller |
| Cone roller surface in the moving coordinate system | |
| Transformation matrix from the coordinate system O0−X0Y0Z0 to the coordinate system O1−X1Y1Z1 | |
| Transformation matrix from the coordinate system O1−X1Y1Z1 to the coordinate system O2−X2Y2Z2 | |
| Transformation matrix from the coordinate system O2−X2Y2Z2 to the coordinate system O3−X3Y3Z3 | |
| Cone roller surface in the global coordinate system | |
| Vector function of the single-parameter surface family of the cone roller | |
| Parametric expression of the cam rail surface | |
| Direction of the circumferential friction acting on the cone roller | |
| Direction of the sliding friction along the generatrix of the cone roller | |
| Normal vector of the cone roller surface | |
| Projection of the normal vector on the bottom of the cone roller | |
| Unit vector along the circumference of the cam rail at the contact point | |
| Unit vector of the axis Y3 in the global coordinate system | |
| Unit vector of the axis Z0 in the global coordinate system | |
| Pressure angle of the cam rail surface | |
| Angle between the friction and the axial direction of the cam rail | |
| Angle between the normal force and the circumferential unit vector at the contact point | |
| Angle between the friction and the circumferential unit vector at the contact point | |
| Angle between the component of the normal force and the axis Y3 | |
| Angle with phase difference with | |
| Angle with phase difference with | |
| Angle with phase difference with | |
| Angle with phase difference with | |
| Angle with phase difference with | |
| Acceleration of the cam rail set | |
| n | Rotational speed of the cam rail set |
| Instantaneous torque | |
| Friction of the linear bearing | |
| Normal force acting on the outer cam rail I | |
| Friction acting on the outer cam rail I | |
| Normal force acting on the inner cam rail I | |
| Friction acting on the inner cam rail I | |
| Normal force acting on the inner cam rail II | |
| Friction acting on the inner cam rail II | |
| Normal force acting on the outer cam rail II | |
| Friction acting on the outer cam rail II | |
| Normal force between stacked rollers | |
| Normal force between stacked rollers | |
| Supporting force provided by the roller shaft | |
| Friction torque provided by the roller shaft | |
| Oil stirring shear moment | |
| Mass of the cam rail set I | |
| Force arm of the normal force and the friction | |
| Force arm of the normal force and the friction | |
| ϕ | Angle between the supporting force and the z-axis |
| Friction coefficient | |
| p | Load pressure |
| A | Area of the large end of the roller shaft |
| µ | Dynamic viscosity of the oil |
| Average diameter of the outer peripheral surface of the outer cam rail | |
| Average width of the outer peripheral surface of the outer cam rail | |
| Average gap between the outer peripheral surface of the outer cam rail and the inner wall of the roller casing | |
| Average torque |
References
- Alle, N.; Hiremath, S.S.; Makaram, S.; Subramaniam, K.; Talukdar, A. Review on Electro Hydrostatic Actuator for Flight Control. Int. J. Fluid Power 2016, 17, 125–145. [Google Scholar] [CrossRef]
- Zhu, Y.; Li, G.P.; Wang, R.; Tang, S.N.; Su, H.; Cao, K. Intelligent Fault Diagnosis of Hydraulic Piston Pump based on Wavelet Analysis and Improved AlexNet. Sensors 2021, 21, 549. [Google Scholar] [CrossRef]
- Hong, H.C.; Zhang, B.; Yu, M.; Zhong, Q.; Yang, H.Y. Analysis and Optimization on U-shaped Damping Groove for Flow Ripple Reduction of Fixed Displacement Axial-Piston Pump. Int. J. Fluid Mach. Syst. 2020, 13, 126–135. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, J.H.; Xu, B.; Chao, Q.; Liu, G. Multi-objective Optimization of Micron-Scale Surface Textures for the Cylinder/Valve Plate Interface in Axial Piston Pumps. Tribol. Int. 2019, 138, 316–329. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, B.L.; Lu, R.Q.; Yang, Q.F.; Dai, Q.M. Study on Oil Film Characteristics of Piston-Cylinder Pair of Ultra-High Pressure Axial Piston Pump. Processes 2020, 8, 16. [Google Scholar] [CrossRef] [Green Version]
- Tang, H.S.; Ren, Y.; Xiang, J.W. Power Loss Characteristics Analysis of Slipper Pair in Axial Piston Pump considering Thermoelastohydrodynamic Deformation. Lubr. Sci. 2019, 31, 381–403. [Google Scholar]
- Jiang, J.H.; Wang, Z.B.; Li, G.Q. The Impact of Slipper Microstructure on Slipper-Swashplate Lubrication Interface in Axial Piston Pump. IEEE Access 2020, 8, 222865–222875. [Google Scholar] [CrossRef]
- Xing, T.; Xu, Y.Z.; Ruan, J. Two-Dimensional Piston Pump: Principle, Design, and Testing for Aviation Fuel Pumps. Chin. J. Aeronaut. 2019, 33, 1349–1360. [Google Scholar] [CrossRef]
- Tong, C.W. Design and Research of Two-Dimensional (2D) Hydraulic Monomer Pump. Master’s Thesis, Zhejiang University of Technology, Hangzhou, China, 2016. [Google Scholar]
- Shentu, S.N.; Ruan, J.; Qian, J.Y.; Meng, B.; Wang, L.F.; Guo, S.S. Study of Flow Ripple Characteristics in an Innovative Two-Dimensional Fuel Piston Pump. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 1–15. [Google Scholar] [CrossRef]
- Li, J.Y.; Jin, D.C.; Tong, C.W.; Nansheng, S.; Meng, B.; Ruan, J. Design and an Error Compensation of End Cam for Two Dimensional (2D) Axial Piston Pump. Hydraul. Pneum. Seals 2017, 37, 18–22. [Google Scholar]
- Jin, D.C.; Ruan, J.; Li, S.; Meng, B.; Wang, L.F. Modelling and Validation of a Roller-Cam Rail Mechanism used in a 2D Piston Pump. J. Zhejiang Univ. Sci. A 2019, 20, 201–217. [Google Scholar] [CrossRef]
- Jin, D.C.; Ruan, J.; Xing, T.; Wang, L.F. Design and Experiment of Two-dimensional Cartridge Water Pump based on Mathematical Model. Trans. Chin. Soc. Agric. Mach. 2019, 50, 177–185. [Google Scholar]
- Qian, J.Y.; Shentu, S.N.; Ruan, J. Volumetric Efficiency Analysis of Two-Dimensional Piston Aviation Fuel Pump. Acta Aeronaut. Astronaut. Sin. 2020, 41, 423267. [Google Scholar]
- Huang, Y.; Ruan, J.; Zhang, C.C.; Ding, C.; Li, S. Research on the Mechanical Efficiency of High-Speed 2D Piston Pumps. Processes 2020, 8, 853. [Google Scholar] [CrossRef]
- Huang, Y.; Ruan, J.; Chen, Y.; Ding, C.; Li, S. Research on the Volumetric Efficiency of 2D Piston Pumps with a Balanced Force. Energies 2020, 13, 4796. [Google Scholar] [CrossRef]
- Wang, H.Y.; Li, S.; Ruan, J. Design and Research of Two-Dimensional Fuel Pump of Inertia Force Balanced. Acta Aeronaut. Astronaut. Sin. 2021, 4. [Google Scholar] [CrossRef]
- Huang, Y.; Ding, C.; Wang, H.Y.; Ruan, J. Numerical and Experimental Study on the Churning Losses of 2D High-Speed Piston Pumps. Eng. Appl. Comput. Fluid Mech. 2020, 14, 764–777. [Google Scholar] [CrossRef]
- Ding, C.; Huang, Y.; Zhang, L.C.; Ruan, J. Investigation of the Churning Loss Reduction in 2D Motion-converting Mechanisms. Energies 2021, 14, 1506. [Google Scholar] [CrossRef]
- Li, J.; Rao, X.; Tang, M.; Deng, Y.Y.; Xiong, M.L. Research of the Profile Surface Equation of Globoidal Indexing Cam based on Unified Mathematical Expression. J. Mech. Transm. 2018, 264, 29–34. [Google Scholar]
- Tsay, D.M.; Lin, B.J. Improving the Geometry Design of Cylindrical Cams Using Nonparametric Rational B-splines. Comput. Aided Des. 1996, 28, 5–15. [Google Scholar] [CrossRef]
- Zhang, J.H.; Li, Y.; Xu, B.; Pan, M.; Chao, Q. Experimental Study of an Insert and its Influence on Churning Losses in a High-Speed Electro-Hydrostatic Actuator Pump of an Aircraft. Chin. J. Aeronaut. 2019, 32, 2028–2036. [Google Scholar] [CrossRef]
- Li, D. Research on Frictional Properties and Temperature Distribution of High-Speed Railway Axle Box Bearing. Master’s Thesis, Dalian University of Technology, Dalian, China, 2017. [Google Scholar]
- Zhang, C.C.; Ruan, J.; Xing, T.; Li, S.; Meng, B.; Ding, C. Research on the Volumetric Efficiency of a Novel Stacked Roller 2D Piston Pump. Machines 2021, 9, 128. [Google Scholar] [CrossRef]




| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Stroke h (m) | 0.0015 | Force arm (m) | 0.01175 |
| Number of cone rollers N | 8 | Mass of the cam rail set I (kg) | 0.052 |
| Projection angle b (deg) | 120 | Area of the large end of the roller shaft A (m2) | 1.54 × 104 |
| Half cone angle (deg) | 26.2242 | Dynamic viscosity of oil (Pa∙s) | 0.03893 |
| Inclination angle (deg) | 13.8362 | Average diameter (m) | 0.0335 |
| Distance from middle contact points on the cam rails to the plane X3O3Y3 L (m) | 0.01217, 0.01632 | Average width (m) | 0.0065 |
| Force arm R (m) | 0.0045 | Average gap (m) | 0.0025 |
| Force arm (m) | 0.01575 |
| Description | Details |
|---|---|
| SL06 torque/speed sensor | Range of the torque: 0–20 Nm, precision: ±0.1%; Range of the rotational speed: 0–18,000 r/min. |
| MIK-P300 pressure sensor | Range: 0–10 MPa, precision: ±0.3%. |
| NI USB-6363 data acquisition card | Resolution: 16 bits; Maximum sampling frequency: 1 MHz. |
| Load Pressure (MPa) | Rotational Speed (rpm) | Practical Torque Loss (Nm) | Theoretical Torque Loss (Nm) | Error |
|---|---|---|---|---|
| 1 | 1000 | 0.07 | 0.09021 | 22.4% |
| 2000 | 0.05 | 0.09089 | 45.0% | |
| 3000 | 0.07 | 0.09157 | 23.6% | |
| 4000 | 0.06 | 0.09225 | 35.0% | |
| 5000 | 0.05 | 0.09293 | 46.2% | |
| 6000 | 0.09 | 0.09360 | 3.8% | |
| 7000 | 0.08 | 0.09428 | 15.1% | |
| 8000 | 0.08 | 0.09495 | 15.7% | |
| 9000 | 0.09 | 0.09562 | 5.9% | |
| 10,000 | 0.07 | 0.09629 | 27.3% | |
| 2 | 1000 | 0.11 | 0.17974 | 38.8% |
| 2000 | 0.15 | 0.18042 | 16.9% | |
| 3000 | 0.15 | 0.18110 | 17.2% | |
| 4000 | 0.16 | 0.18178 | 12.0% | |
| 5000 | 0.16 | 0.18246 | 12.3% | |
| 6000 | 0.17 | 0.18313 | 7.2% | |
| 7000 | 0.17 | 0.18380 | 7.5% | |
| 8000 | 0.18 | 0.18448 | 2.4% | |
| 9000 | 0.18 | 0.18514 | 2.8% | |
| 10,000 | 0.19 | 0.18581 | 2.3% | |
| 3 | 1000 | 0.23 | 0.26926 | 14.6% |
| 2000 | 0.26 | 0.26995 | 3.7% | |
| 3000 | 0.30 | 0.27063 | 10.8% | |
| 4000 | 0.21 | 0.27131 | 22.6% | |
| 5000 | 0.21 | 0.27198 | 22.9% | |
| 6000 | 0.28 | 0.27266 | 2.7% | |
| 7000 | 0.27 | 0.27333 | 1.2% | |
| 8000 | 0.35 | 0.27410 | 27.7% | |
| 9000 | 0.30 | 0.27467 | 9.2% | |
| 10,000 | 0.28 | 0.27534 | 1.7% | |
| 4 | 1000 | 0.33 | 0.35879 | 8.0% |
| 2000 | 0.35 | 0.35947 | 2.6% | |
| 3000 | 0.36 | 0.36015 | 0.0% | |
| 4000 | 0.36 | 0.36083 | 0.2% | |
| 5000 | 0.35 | 0.36151 | 3.2% | |
| 6000 | 0.42 | 0.36218 | 16.0% | |
| 7000 | 0.38 | 0.36286 | 4.7% | |
| 8000 | 0.35 | 0.36353 | 3.7% | |
| 9000 | 0.41 | 0.36420 | 12.5% | |
| 10,000 | 0.42 | 0.36487 | 15.1% | |
| 5 | 1000 | 0.44 | 0.44832 | 1.9% |
| 2000 | 0.43 | 0.44901 | 4.2% | |
| 3000 | 0.44 | 0.44968 | 2.2% | |
| 4000 | 0.46 | 0.45036 | 2.1% | |
| 5000 | 0.45 | 0.45104 | 0.2% | |
| 6000 | 0.47 | 0.45171 | 4.0% | |
| 7000 | 0.47 | 0.45238 | 3.9% | |
| 8000 | 0.48 | 0.45306 | 5.9% | |
| 9000 | 0.51 | 0.45372 | 12.4% | |
| 10,000 | 0.53 | 0.45439 | 16.6% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, K.; Ruan, C.; Wang, H.; Li, S.; Ruan, J. Analysis of the Torque Loss of High-Speed Transmission Mechanism with a Stacked Roller Set. Machines 2021, 9, 140. https://doi.org/10.3390/machines9080140
Zhu K, Ruan C, Wang H, Li S, Ruan J. Analysis of the Torque Loss of High-Speed Transmission Mechanism with a Stacked Roller Set. Machines. 2021; 9(8):140. https://doi.org/10.3390/machines9080140
Chicago/Turabian StyleZhu, Ke, Chuantan Ruan, Heyuan Wang, Sheng Li, and Jian Ruan. 2021. "Analysis of the Torque Loss of High-Speed Transmission Mechanism with a Stacked Roller Set" Machines 9, no. 8: 140. https://doi.org/10.3390/machines9080140
APA StyleZhu, K., Ruan, C., Wang, H., Li, S., & Ruan, J. (2021). Analysis of the Torque Loss of High-Speed Transmission Mechanism with a Stacked Roller Set. Machines, 9(8), 140. https://doi.org/10.3390/machines9080140






