Low-Order Radial Modal Test and Analysis of Drive Motor Stator
Abstract
:1. Introduction
2. Test Objects and Tools
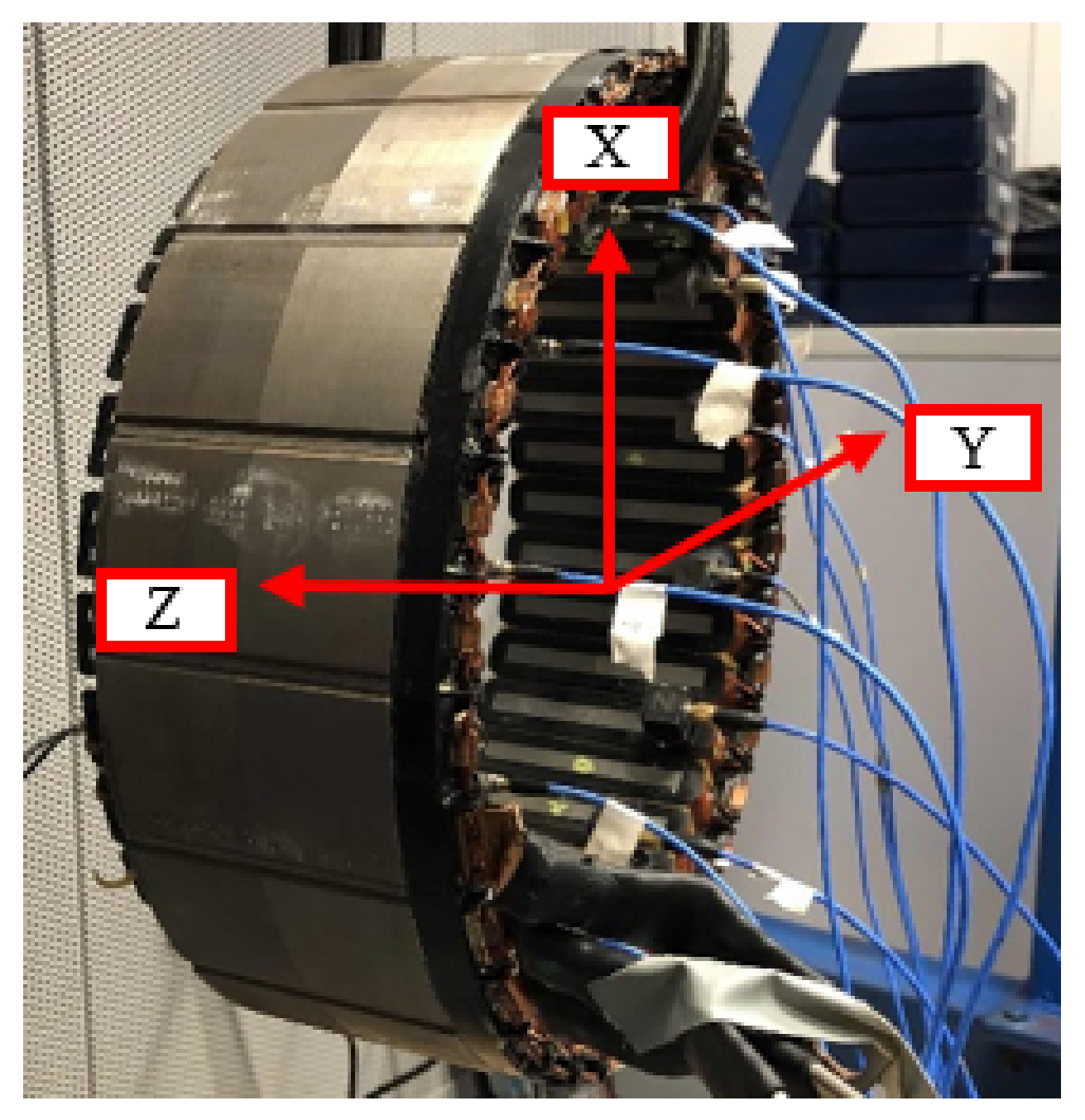
2.1. Motor Stator
2.2. Modal Test System
3. Test 1: Effect of Stator Shell on the Stator Modal
3.1. Sensor Layout and Parameter Setting
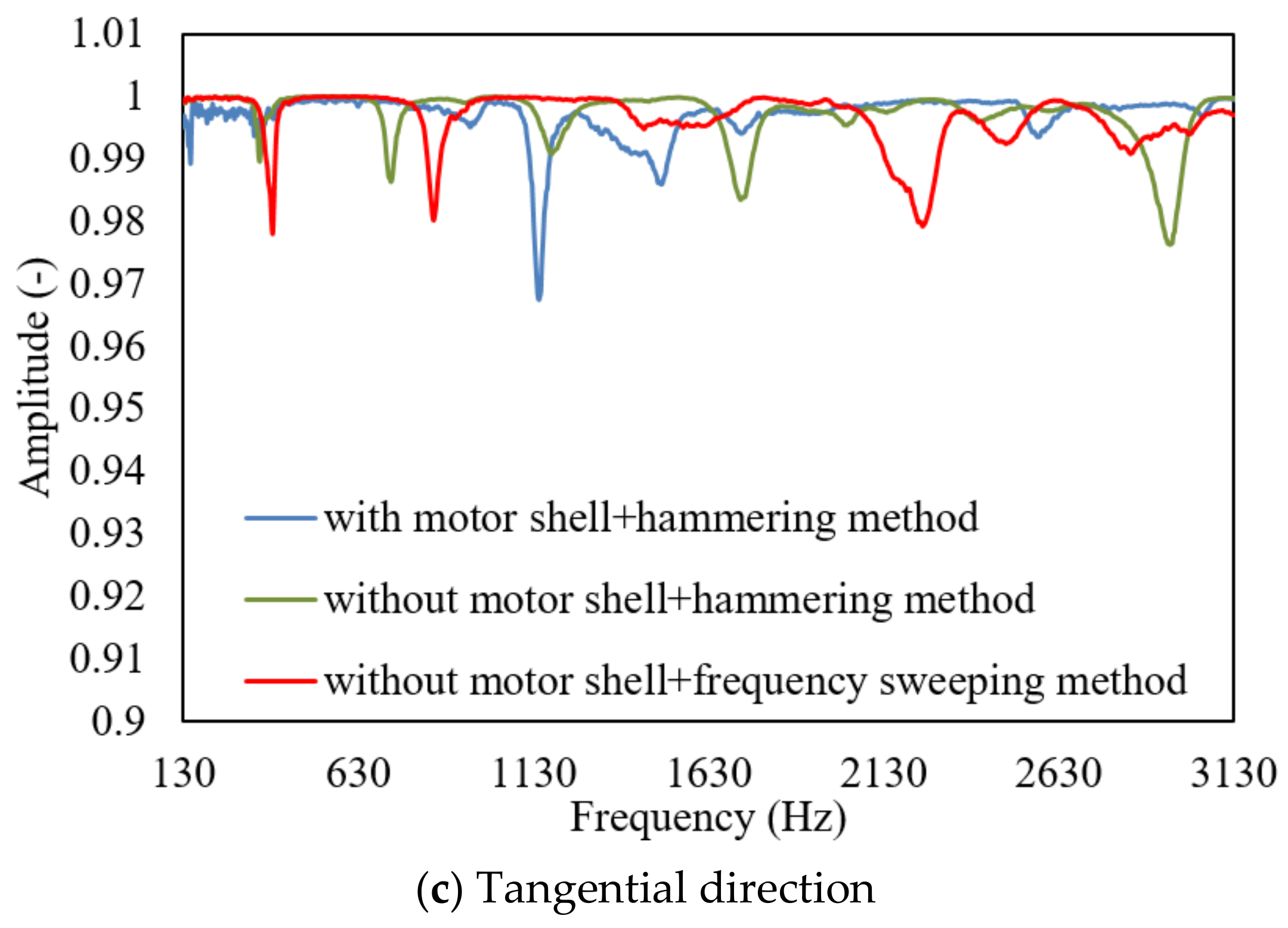
3.2. Results and Analysis
4. Test 2: Stator Modal Analysis under Different Excitation Sources
4.1. Sensor Layout and Parameter Setting
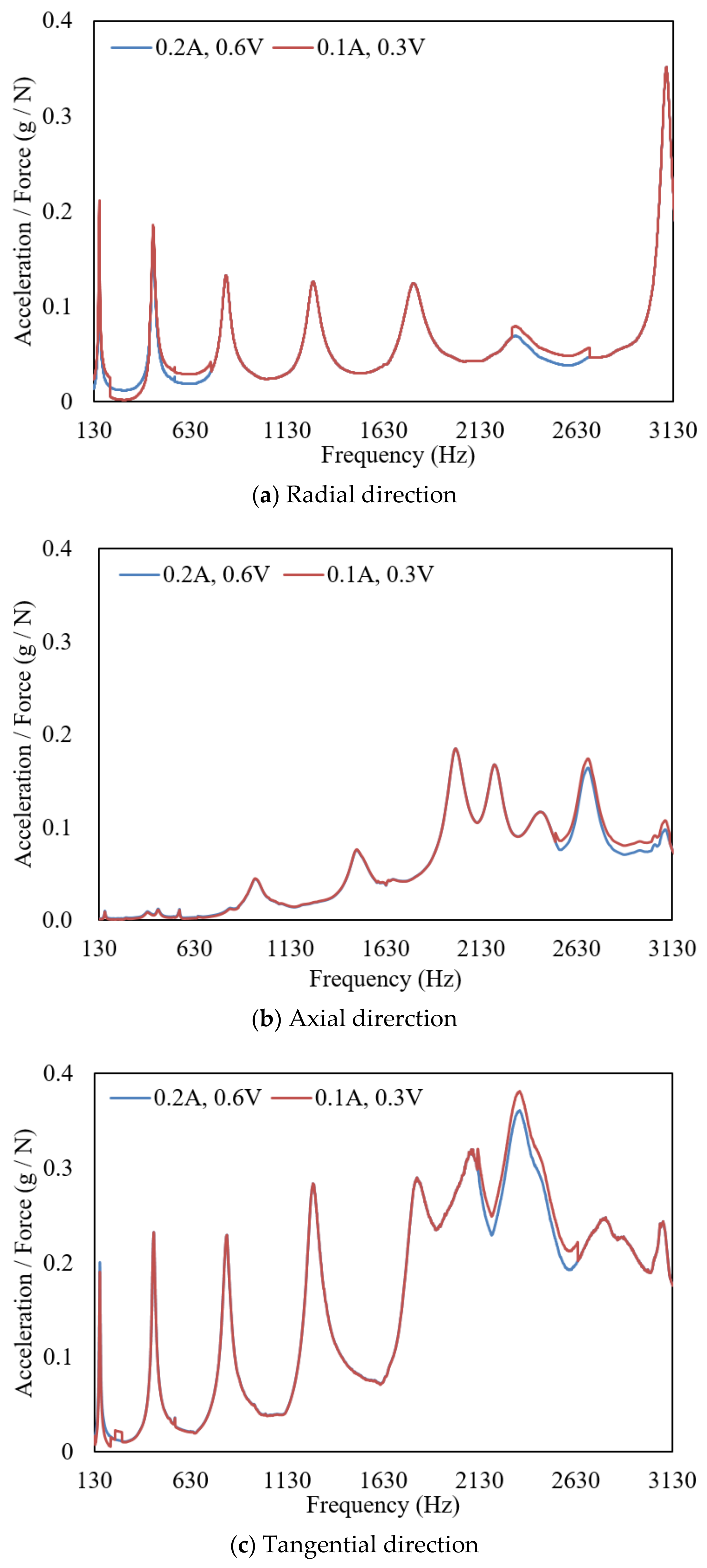
4.2. Results and Analysis
5. Conclusions
- (1)
- The coherence function of motor stator without shell is higher than that with shell, which indicates that the experimental modal of motor stator without shell is easier to be excited. The frequency response curve of motor stator with shell is lower than that without shell. The modal frequencies excited with shell are significantly higher than those without shell.
- (2)
- Under different output currents and voltages, the same excitation response point obtained by frequency sweeping method has the same frequency response function. The motor stator presents a linear structure in the frequency domain concerned. The frequency response functions of the motor stator obtained by the hammering method and frequency sweeping method have good consistency in the low frequency band. The consistency of the radial and axial frequency response functions is better than that of the tangential frequency response functions.
- (3)
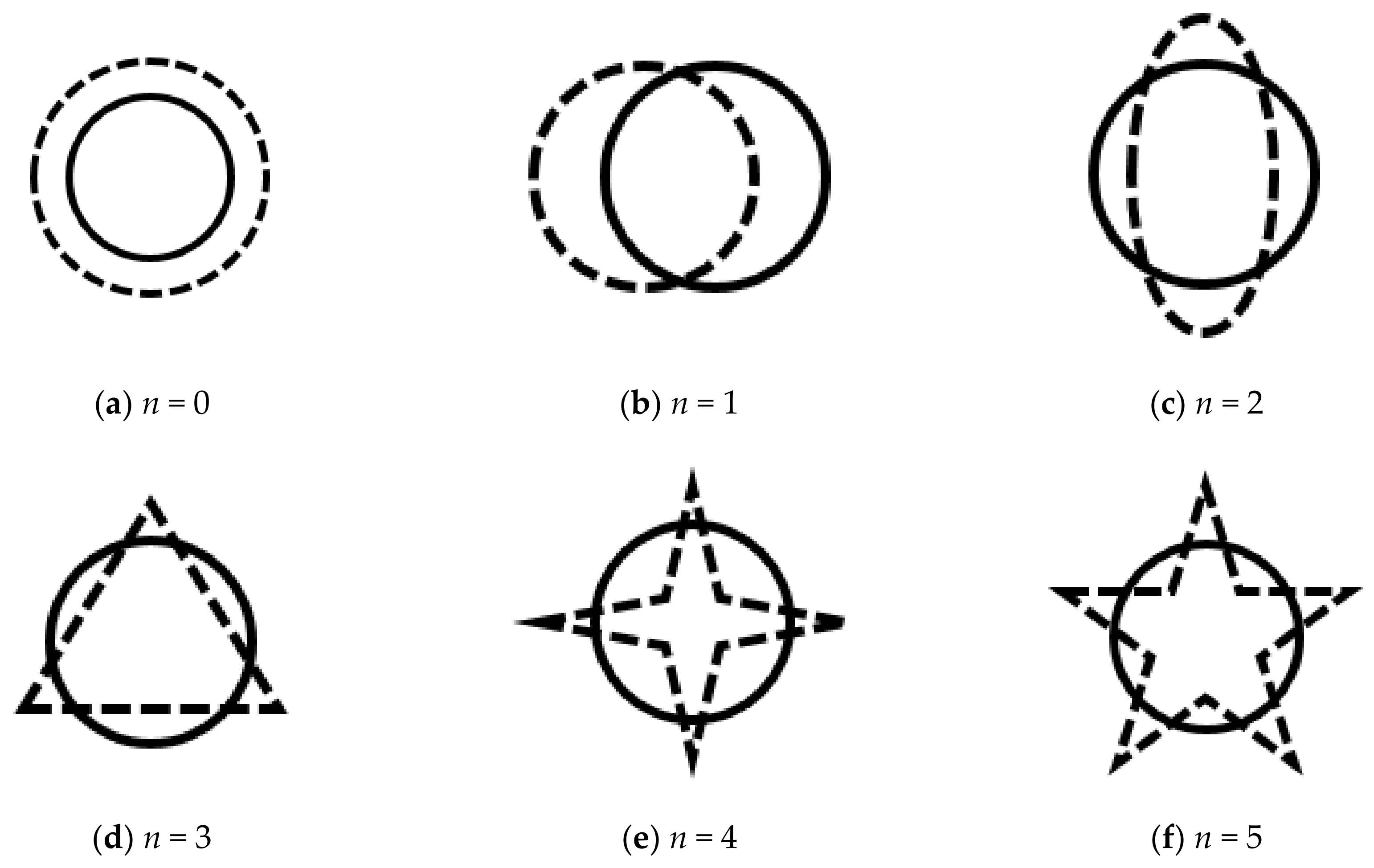
- The (1, 1), (1, 2) and (1, 3) modals of the motor stator with shell can not be excited by the hammering method. The modal frequencies obtained by the frequency sweeping method are slightly higher than those obtained by the hammering method. The motor stator without shell has multiple root modals. As the number of radial nodes is the same, a larger number of axial nodes results in a higher modal frequency.
- (4)
- The natural frequency and vibration mode of the motor stator are not determined by the stator alone. The stator shell also plays an important role. The NVH performance of the stator cannot be used for evaluating the NVH performance of the stator system, as the impact of the stator shell on the stator system NVH should also be considered.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Long, S.A.; Zhu, Z.Q.; Howe, D. Vibration behaviour of stators of switched reluctance motors. IEE Proc. Electr. Power Appl. 2001, 148, 257–264. [Google Scholar] [CrossRef]
- Sun, J.; Zhan, Q.; Huang, J. Modal anslysis of stator vibration for switched reluctance motors. Proc. Chin. Soc. Electr. Eng. 2005, 25, 148–152. [Google Scholar]
- He, G.; Huang, Z.; Qin, R.; Chen, D. Numerical prediction of electromagnetic vibration and noise of permanent-magnet direct current commutator motors with rotor eccentricies and golue effects. IEEE Trans. Magn. 2012, 48, 1924–1931. [Google Scholar] [CrossRef]
- Ko, H.S.; Kim, K.J. Characterization of noise and vibration sources in interior permanent-magnet brush-less DC motors. IEEE Trans. Magn. 2004, 40, 3482–3489. [Google Scholar] [CrossRef]
- Yu, S.B.; Tang, R.Y. Electromagnetic and mechanical characterizations of noise and vibration in permanent magnet synchronous machines. IEEE Trans. Magn. 2006, 42, 1335–1338. [Google Scholar]
- Timar, P.L.; Fazekas, A.; Kiss, J.; Miklos, A.; Vang, S. Noise and Vibration of Electrical Machines. In Studies in Electrical and Electronic Engineering; Elsevier: New York, NY, USA, 1989; pp. 53–62. [Google Scholar]
- Islam, R.; Hussain, I. Analytical model for predicting noise and vibration in permanet-magnet synchronous motors. IEEE Trans. Ind. Appl. 2010, 46, 2346–2354. [Google Scholar] [CrossRef]
- Han, W.; Jia, Q.; Qiu, J. Vibration and modal analysis for stator of an induction motor. J. Vib. Shock 2012, 31, 91–95. [Google Scholar]
- Gieras, J.F.; Wang, C.; Lai, J.C. Noise of Polyphase Electric Motors; CRC Press: Boca Raton, FL, USA, 2006; pp. 22–30. [Google Scholar]
- Neves, C.G.C.; Carlson, R.; Sadowski, N.; Bastos, J.P.A. A study on magnetic vibration sources identification in induction motors by FEM simulation and experimental procedures. In Proceedings of the Conference Record of 1998 IEEE Industry Applications Conference, Thirty-Third IAS Annual Meeting (Cat. No. 98CH36242), St. Louis, MO, USA, 12–15 October 1998; Volume 1, pp. 237–242. [Google Scholar]
- Neves, C.G.C.; Carlson, R.; Sadowski, N.; Bastos, J.P.A.; Soeiro, N.S.; Gerges, S.N.Y. Experimental and numerical analysis of induction motor vibrations. IEEE Trans. Magn. 1999, 35, 1314–1317. [Google Scholar] [CrossRef]
- Cai, W.; Pillay, P. Resonant frequencies and modal shapes of switched reluctance motors. IEEE Trans. Energy Convers. 2001, 16, 43–48. [Google Scholar] [CrossRef]
- Li, R.; Jia, Q.; Zhong, S. Vibration and modal analysis of induction motor. Appl. Mech. Mater. 2012, 226, 92–97. [Google Scholar] [CrossRef]
- Xie, Y.; Wang, Y.; Lv, S.; Ge, H.; Liu, H. Modal calculation and test of small asynchronous motor. Trans. China Electrotech. Soc. 2015, 30, 1–9. [Google Scholar]
- Liu, D. Modal analysis of induction motor stator. Drive Syst. Tech. 2016, 30, 24–29. [Google Scholar]
- Yu, S.; Li, L.; Cao, S. Modal analysis of stator and rotor in large capacity permanent magnet motor. Appl. Mech. Mater. 2013, 313–314, 41–44. [Google Scholar] [CrossRef]
- Liu, J.; Jin, J.; Ji, R.; Chen, D. A novel modal-independent ultrasonic motor with dual stator. Ultrasonics 2017, 76, 177–182. [Google Scholar] [CrossRef] [PubMed]
- Lei, L.; Qian, L. Modal analysis on linear ultrasonic motor. Appl. Mech. Mater. 2015, 742, 522–524. [Google Scholar]
- Yan, R.; Feng, X.; Zhu, L.; Yang, Q. Vibration Analysis of Motor Stator. Adv. Mater. Res. 2014, 902, 140–143. [Google Scholar] [CrossRef]
- Qiu, M.; Wang, D.; Wei, H.; Liang, X.; Ma, Y. Vibration modal analysis and optimization of the motor base. In Proceedings of the International Forum on Construction, Aviation and Environmental Engineering-Internet of Things (IFCAE-IOT 2018), Guangzhou, China, 11–13 May 2018; pp. 175–179. [Google Scholar]





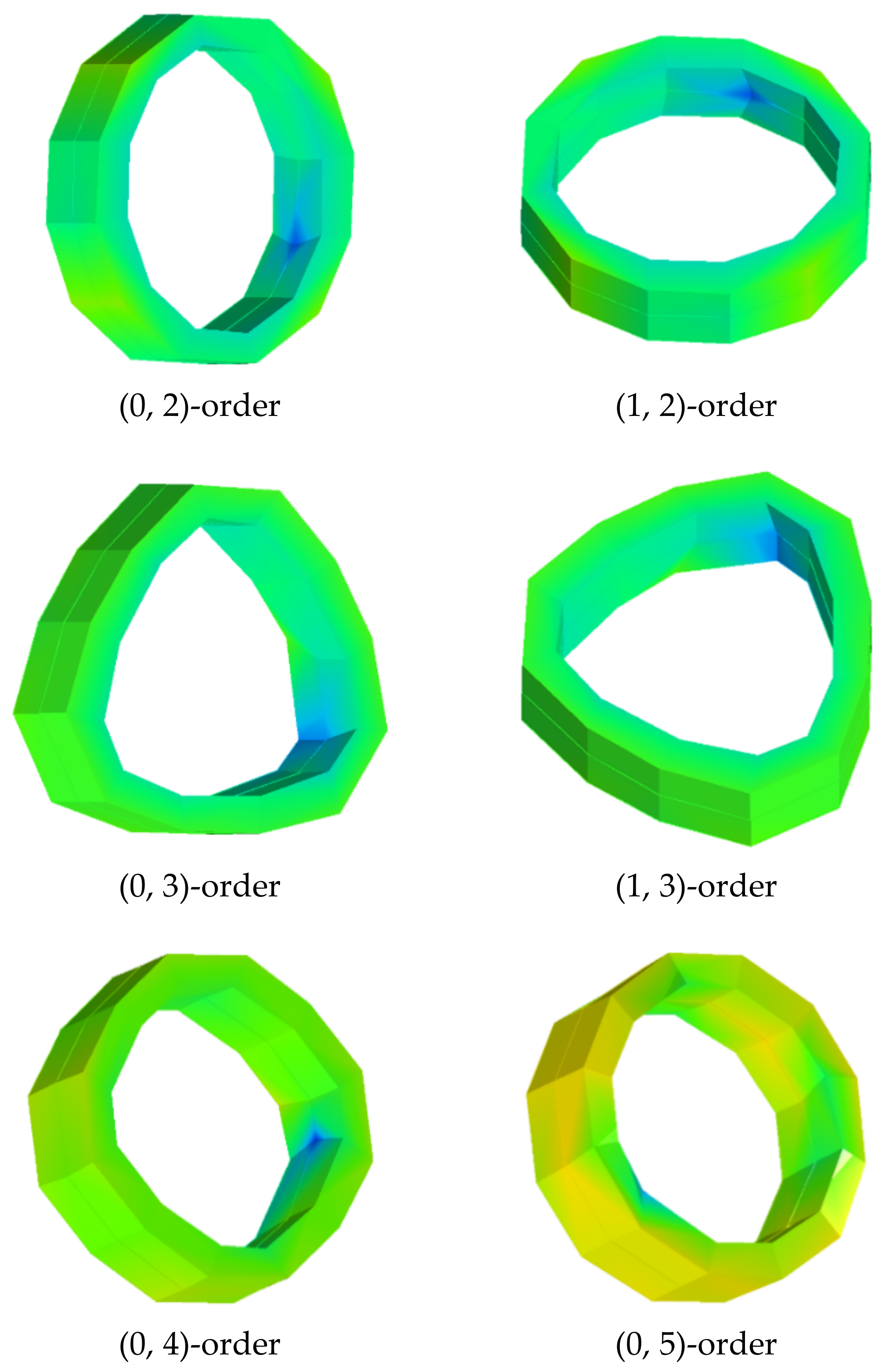
| Order | Hammering Method (with Shell) | Hammering Method (without Shell) | Frequency Sweeping Method (without Shell) | |||
|---|---|---|---|---|---|---|
| Frequency (Hz) | Damping Ratio | Frequency (Hz) | Damping Ratio | Frequency (Hz) | Damping Ratio | |
| (0, 0) | 3285 | 2.16% | 3073 | 1.04% | 3087 | 0.81% |
| (0, 1) | 4707 | 2.13% | 2176 | 1.38% | 2189 | 2.14% |
| (1, 1) | - | - | 2665 | 2.13% | 2681 | 1.41% |
| (0, 2) | 388 | 2.27% | 156 | 1.25% | 159 | 1.58% |
| (1, 2) | - | - | 370 | 2.68% | 381 | 2.21% |
| (0, 3) | 790 | 4.52% | 432 | 1.50% | 438 | 2.05% |
| (1, 3) | - | - | 938 | 2.76% | 957 | 3.21% |
| (0, 4) | 1311 | 4.99% | 798 | 1.93% | 815 | 2.40% |
| (0, 5) | 1972 | 2.99% | 1244 | 0.88% | 1263 | 2.68% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Yang, S.; Yang, J.; Li, F.; Zeng, Q.; Shao, J.; Chang, C.; Wu, N.; Chen, Y.; Li, K. Low-Order Radial Modal Test and Analysis of Drive Motor Stator. Machines 2021, 9, 97. https://doi.org/10.3390/machines9050097
Li J, Yang S, Yang J, Li F, Zeng Q, Shao J, Chang C, Wu N, Chen Y, Li K. Low-Order Radial Modal Test and Analysis of Drive Motor Stator. Machines. 2021; 9(5):97. https://doi.org/10.3390/machines9050097
Chicago/Turabian StyleLi, Jie, Shaobo Yang, Jincai Yang, Fengqin Li, Qingqiang Zeng, Junlong Shao, Chun Chang, Nian Wu, Ying Chen, and Keqiang Li. 2021. "Low-Order Radial Modal Test and Analysis of Drive Motor Stator" Machines 9, no. 5: 97. https://doi.org/10.3390/machines9050097
APA StyleLi, J., Yang, S., Yang, J., Li, F., Zeng, Q., Shao, J., Chang, C., Wu, N., Chen, Y., & Li, K. (2021). Low-Order Radial Modal Test and Analysis of Drive Motor Stator. Machines, 9(5), 97. https://doi.org/10.3390/machines9050097






