Full-Scale Train-to-Train Impact Test and Multi-Body Dynamic Simulation Analysis
Abstract
1. Introduction
2. Simulation
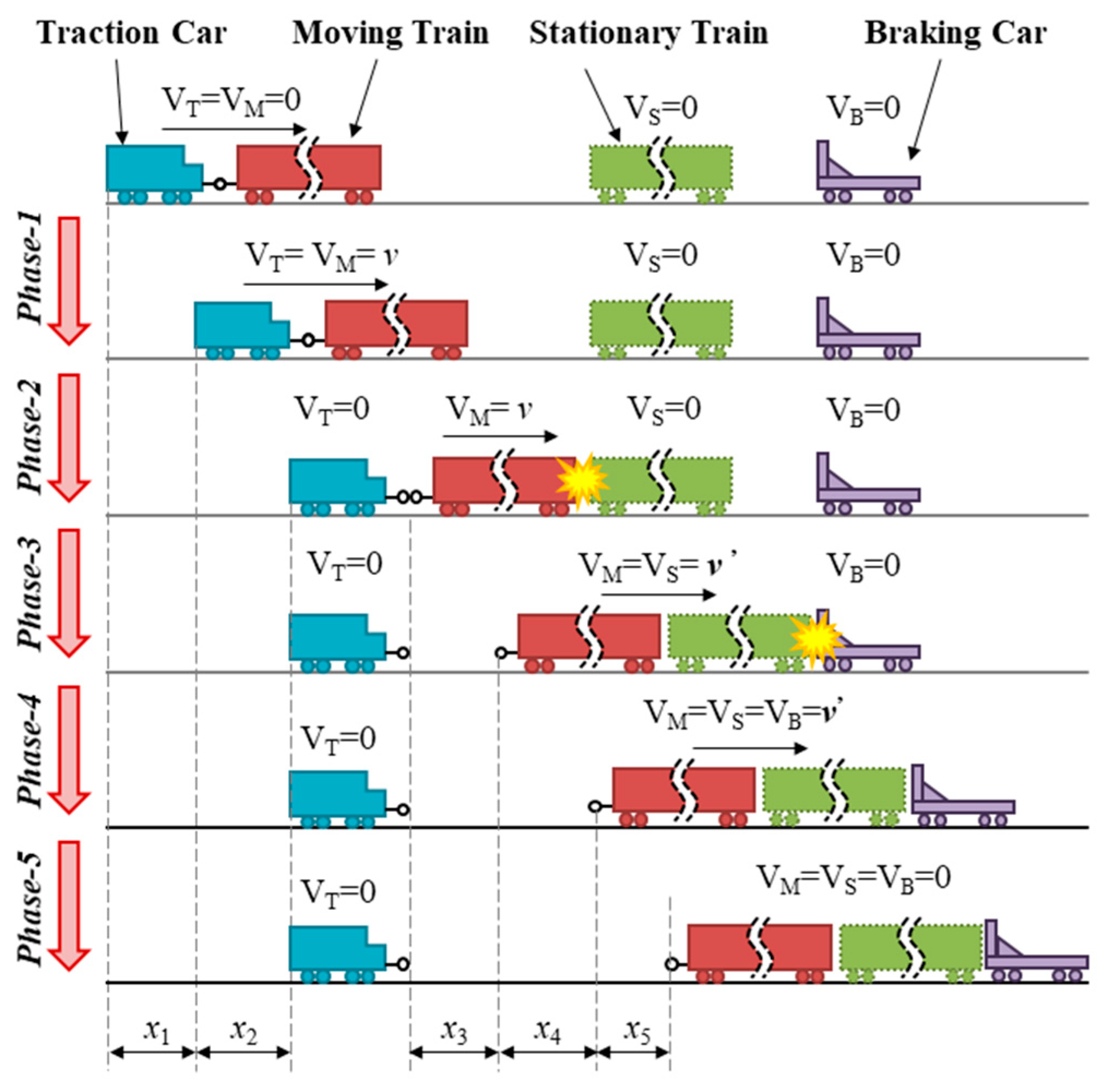
2.1. Vehicle Movement Process
- Phase 1: The traction car driving the moving test train accelerates to the target velocity of the crash test;
- Phase 2: The traction car releases the moving test train. While the moving test train continues to slide forward, the locomotive takes braking measures to protect the safety of the driver;
- Phase 3: Under the protection of multiple safety protection systems, the moving test train collides with a row of the identical stationary train. After the collision, part of the energy is absorbed by the plastic deformation of the energy-absorbing structure, but part of the energy is still stored in the form of kinetic energy, which makes the moving train and the stationary train continue to slide forward;
- Phase 4: After the train-to-train crash test, the sliding test train collides with the stationary braking car. After the collision, the braking car will slide forward together with the test train;
- Phase 5: Under the action of the braking force of the braking car, the test train and the braking car decelerate together until it stops.
2.2. 1D Dynamic Model of Train System
2.3. 3D Dynamic Model of Train System
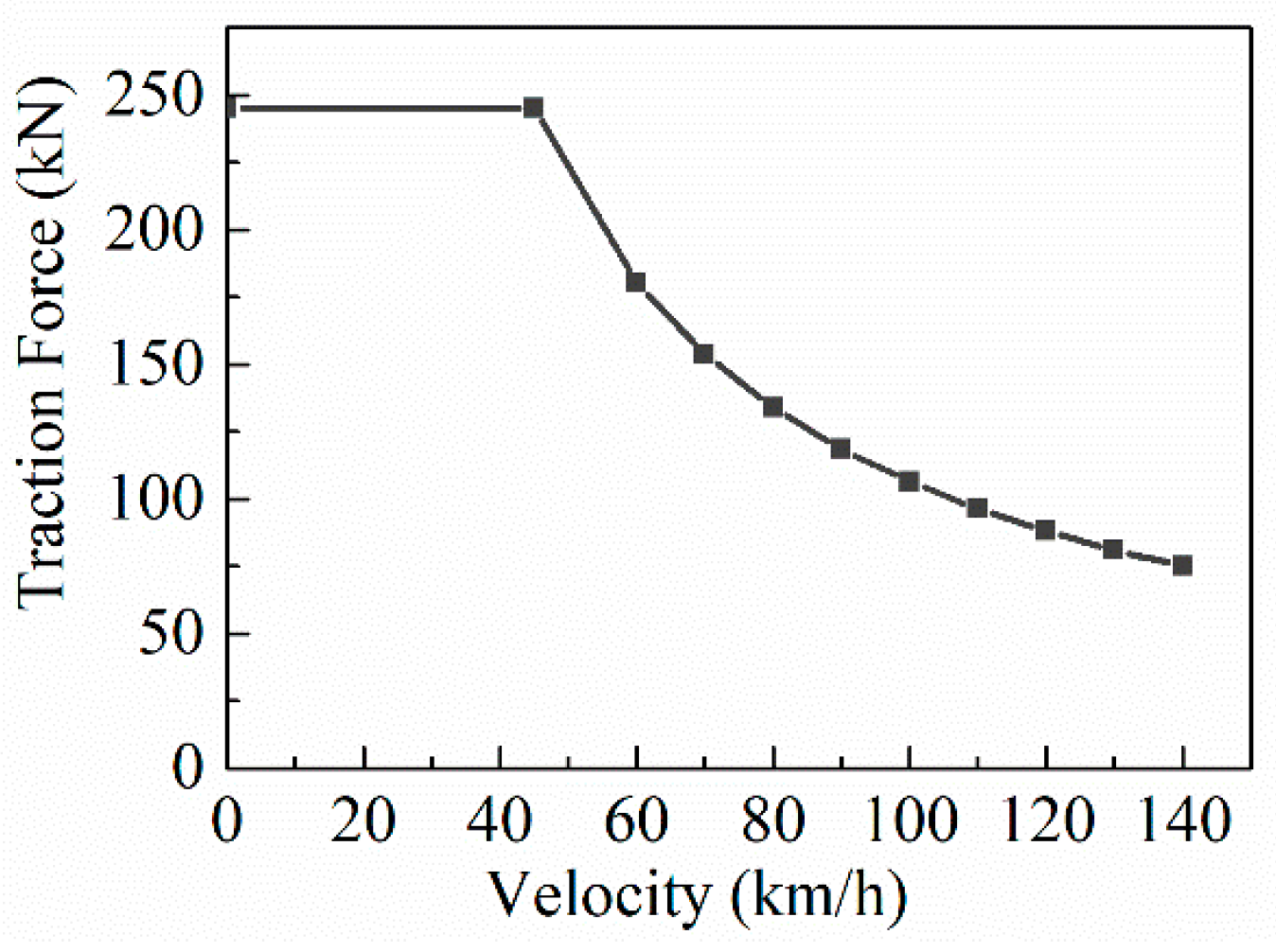
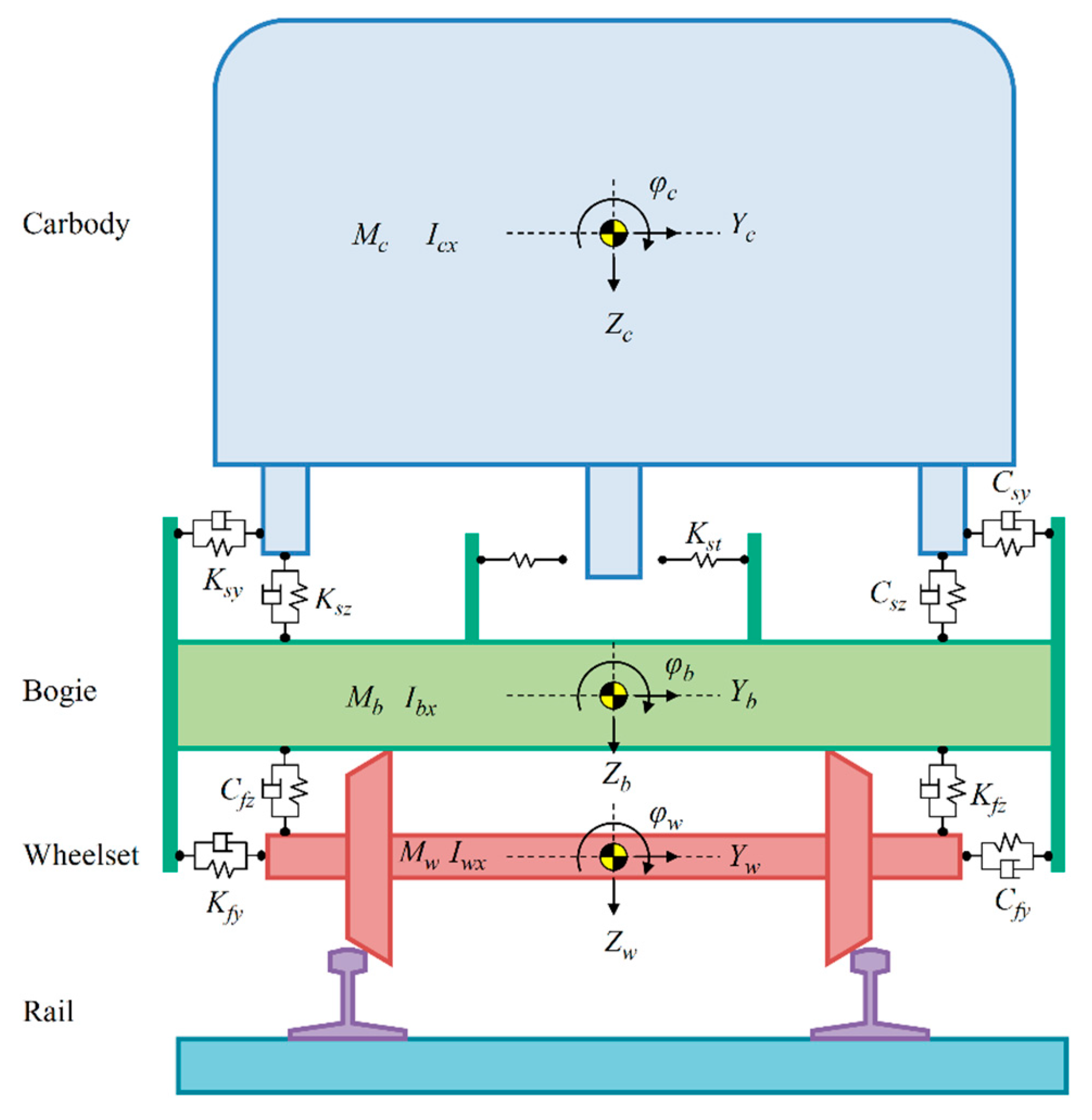
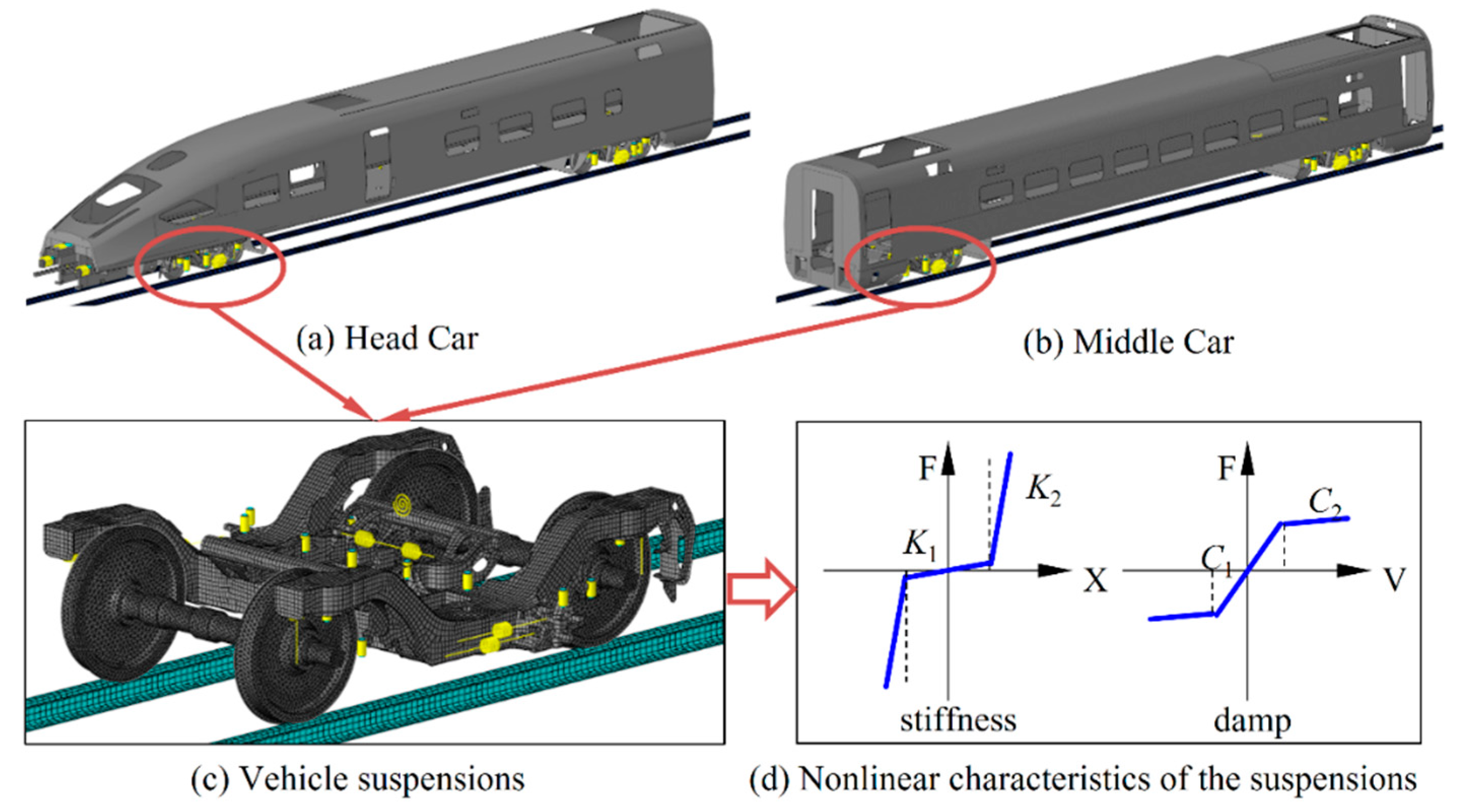
2.3.1. Modelling Vehicle Subsystem
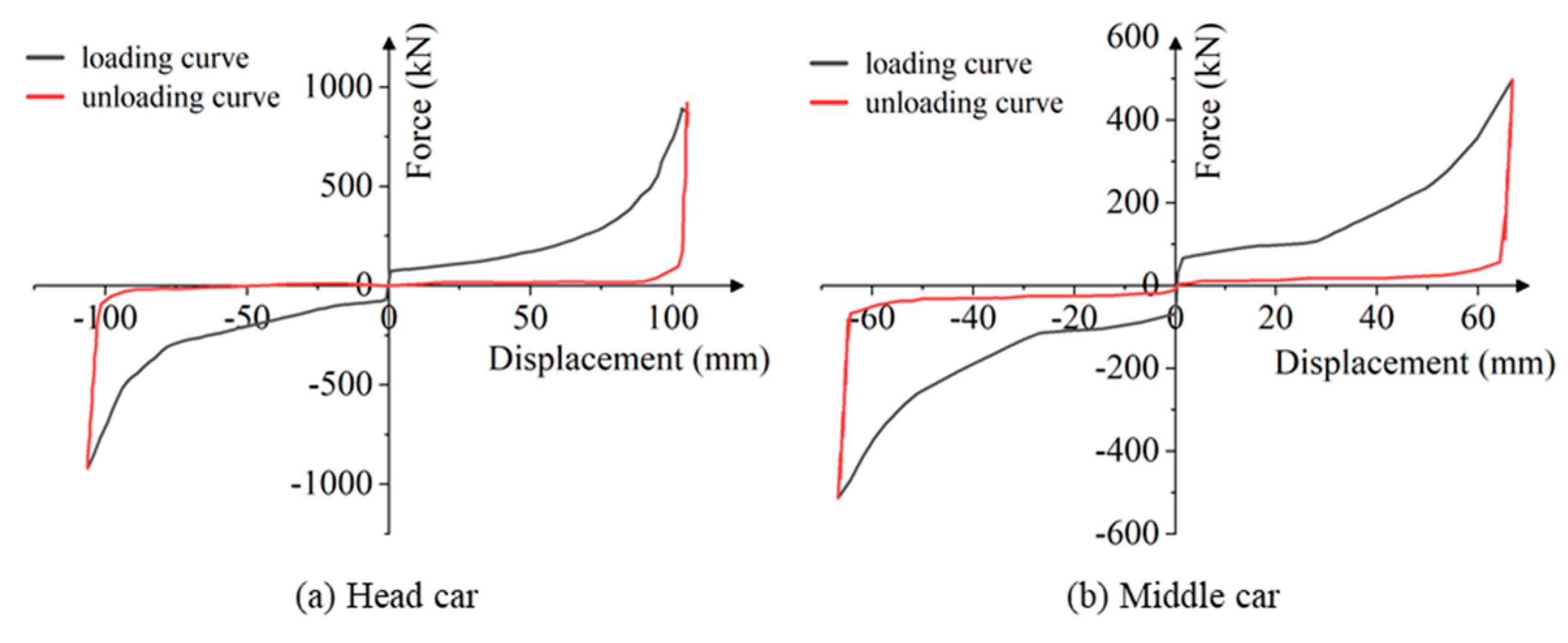
2.3.2. Modelling Energy-Absorbing Subsystem
2.3.3. Modelling Wheel/Rail Contact Aubsystem
2.4. Simulation Result
3. Test
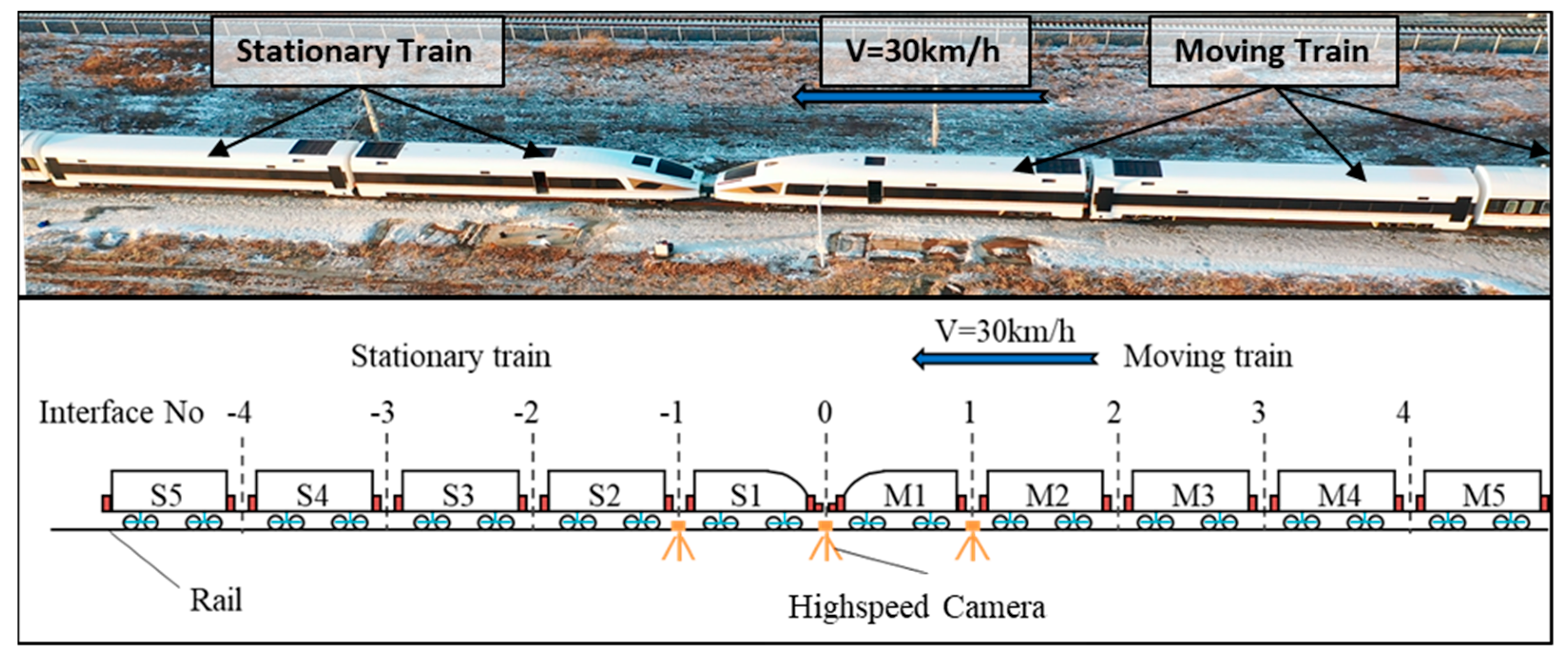
3.1. Test Setup
3.2. Test Result
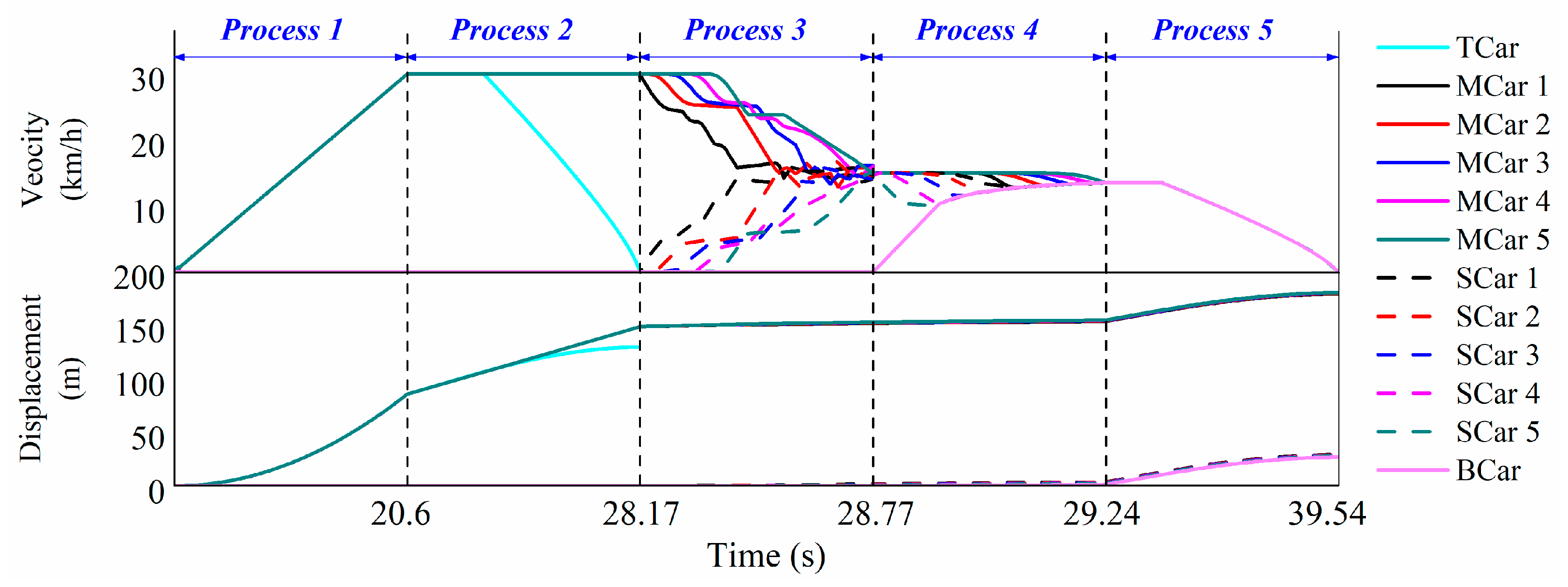
3.2.1. Vehicle Movement in Each Process
- Phase 1: In the process of the traction car pushing the five moving test train and accelerating to 30 km/h, the movement displacement of the driving car is about 90 m. During this test, the acceleration process of the driving car was manually controlled by the driver. The average traction power of the locomotive starting is 70% of the design maximum traction power of the locomotive. In future research and tests, the locomotive will start with full power.
- Phase 2: The braking process of the traction car first experienced a braking idling time of 2.5 s, and the braking idling distance is about 21 m; then it was decelerated to 0 km/h with a braking displacement of 23 m. The total braking distance of the driving car is 44 m.
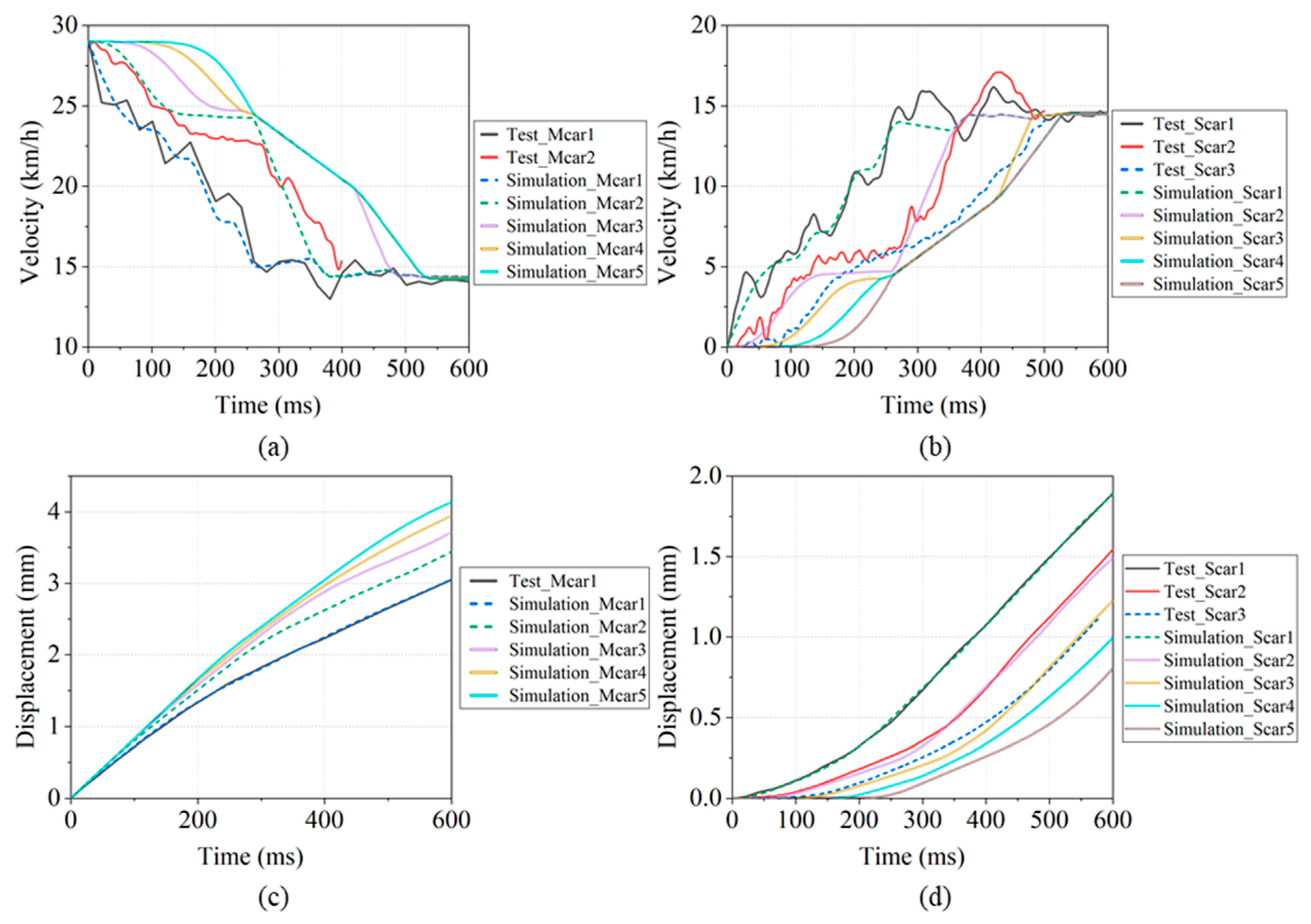
- Phase 3: The duration time of the impact process between the moving train and stationary train is about 0.6 s. During this process, the maximum running displacement of the moving train is 4 m. After the collision, the moving train and the stationary train glide forward together at a velocity of about 15 km/h.
- Phase 4: The moving train and the stationary train collided with the braking car, and the maximum running displacement is 2 m. After the collision, the moving train, the stationary train and the braking train glide forward together at a velocity of about 13.5 km/h.
- Phase 5: The braking process of the moving train, the stationary train and the braking train first experienced a braking idling time of 2.5 s, and the braking idling distance is 9 m; then it was decelerated to 0 km/h, and a braking displacement of 17 m is required for effective braking. The total braking distance of the driving car is 26 m.
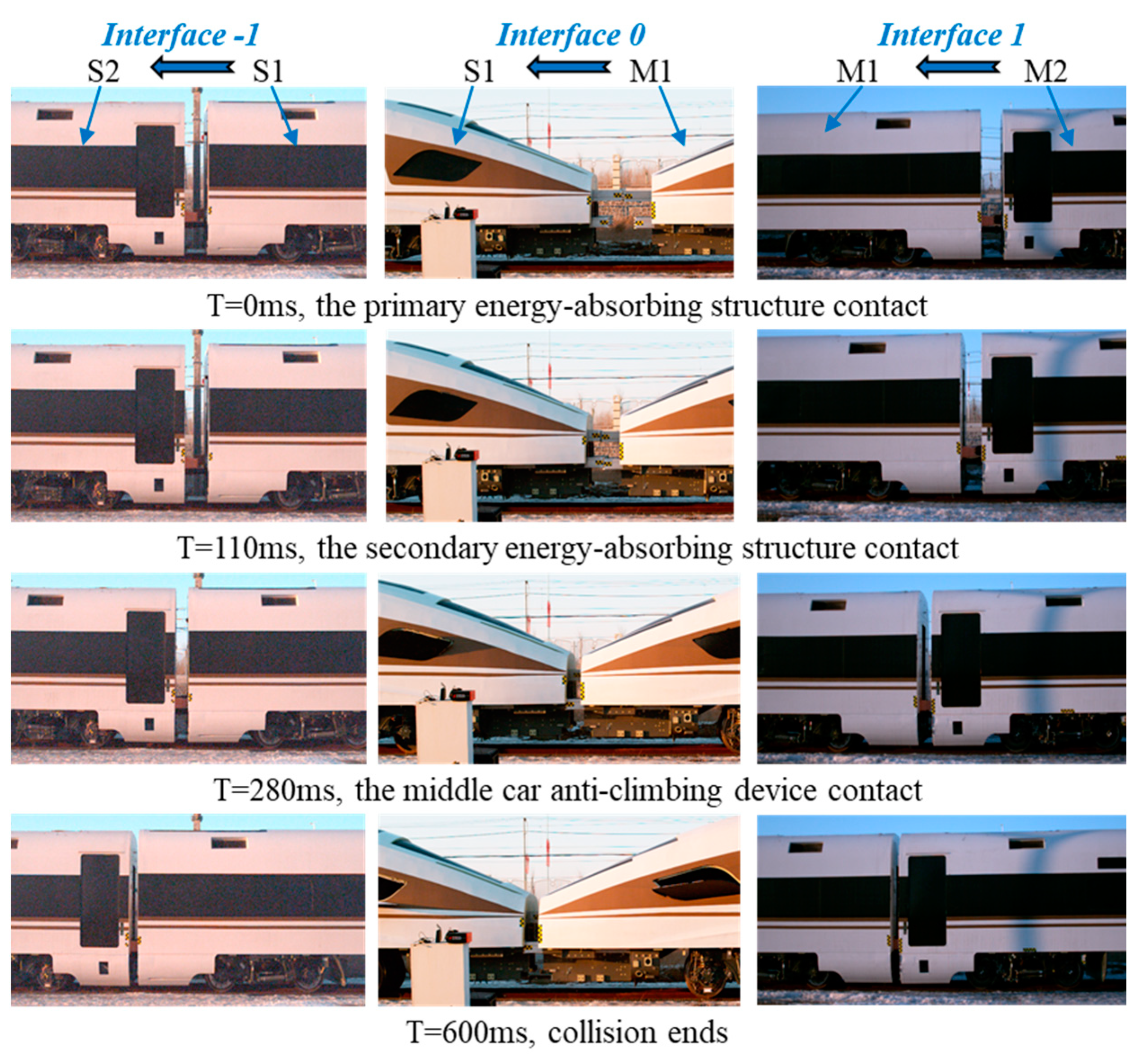
3.2.2. Collision Analysis
3.3. Validation of the Simulation Model
3.3.1. Vehicle Movement in Each Process
3.3.2. Collision Analysis
4. Parametric Analysis
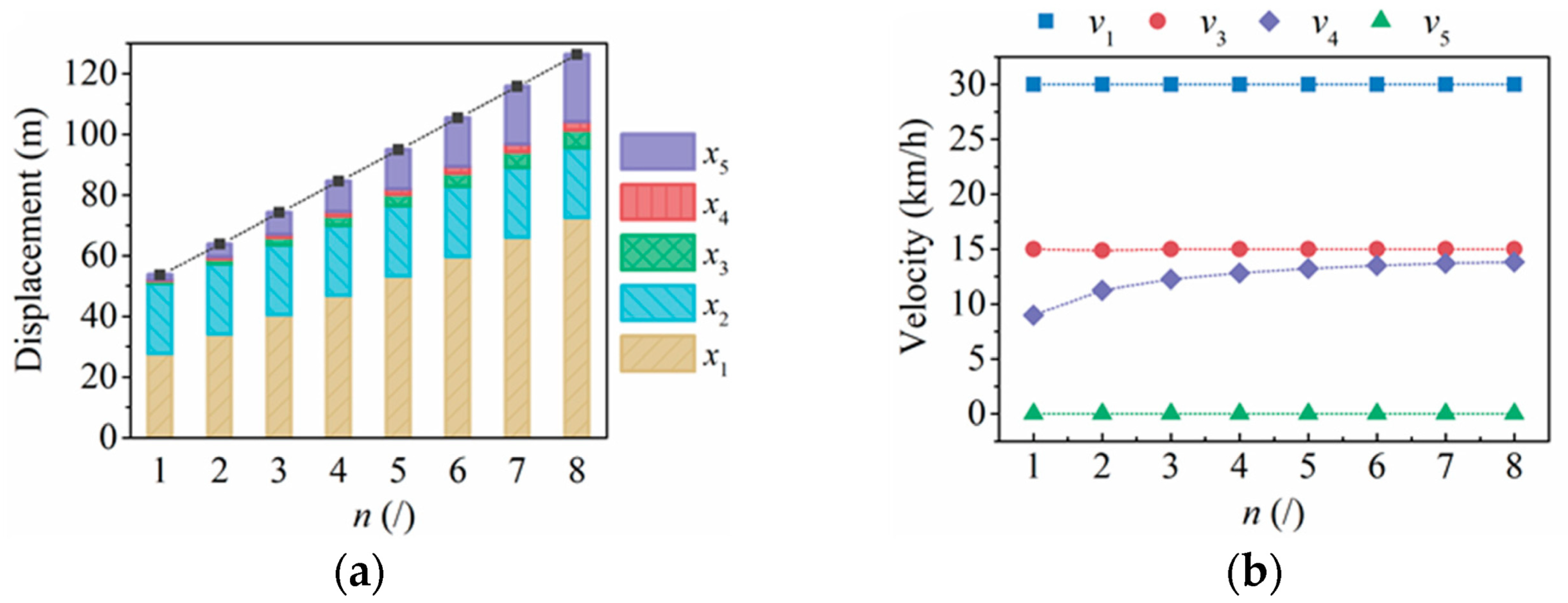
4.1. Effect of Train Formation
- x1: The displacement x1 increases linearly with the increase in the train formation. The displacement x1 was shown to increase from 27.6 m to 72.3 m when the train formation increased from 1 to 8. This is because when the traction force of the locomotive is constant (for the target velocity is less than 45 km/h), the mass of the object affected by the traction force increases linearly with the increase of the train formation, which causes the acceleration of the vehicle to decrease, so the distance x1 will increase accordingly.
- x2: Since the braking distance of the traction car is only related to its own initial state, changing the train formation of the test train that has been separated from the traction car has no effect on the value of x2.
- x3: As the number of test trains participating in the collision process increases, the system initial total kinetic energy of the collision increases linearly, and the maximum vehicle displacement x3 of the process also increases. However, as the number of train formation increases, the instantaneous velocity v3 of the vehicle at the end of the process does not change and is maintained at about 15 km/h (1/2 of the impact velocity).
- x4: As the train formation increases from 1 to 8, the duration time of the collision process between the test train and the braking car has gradually increased, and the velocity v4 after the collision increased significantly, from 9 km/h to 13.85 km/h. The movement displacement x4 of this process also increases.
- x5: For the post-collision braking process, the increase in the train formation not only increases the mass affected by the braking force, but also increases of the initial braking velocity v4, so x5 will gradually increase with the increase of train formation, and the increase velocity is getting faster and faster.
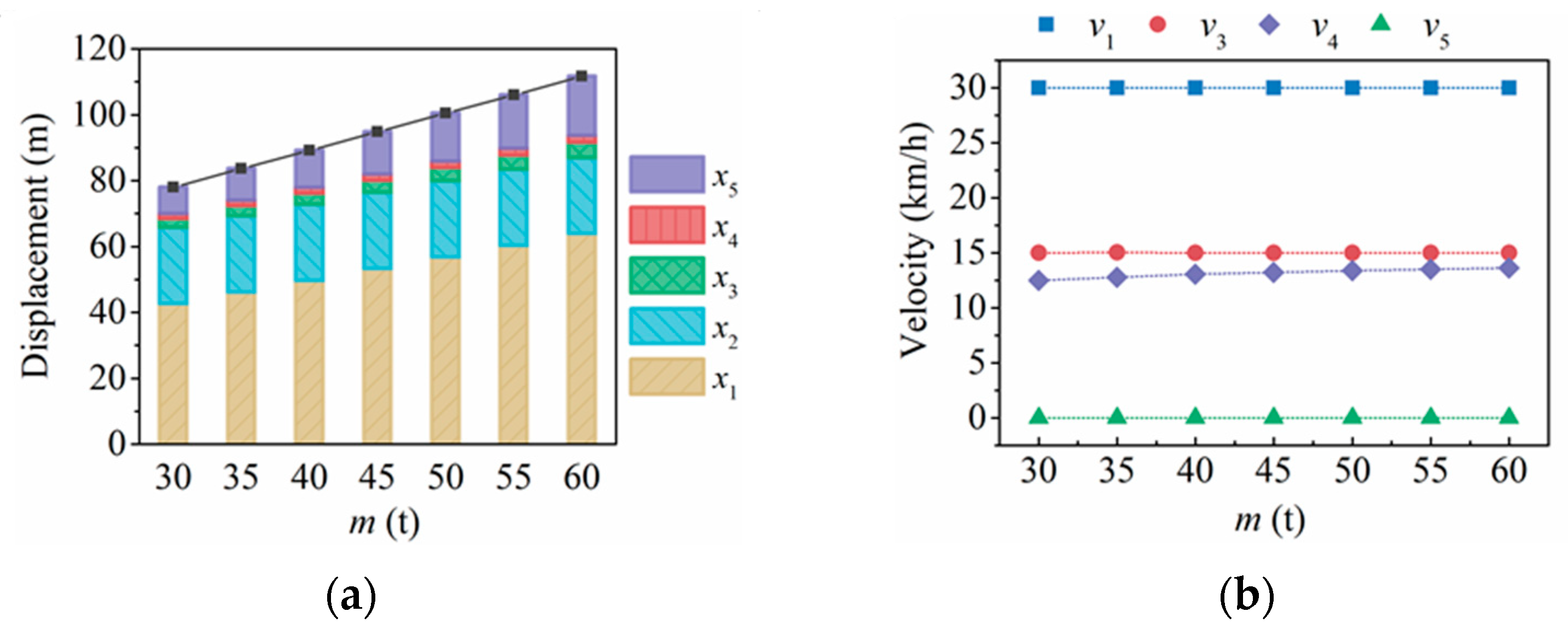
4.2. Effect of Vehicle Mass
- x1: The displacement x1 increases linearly with the increase in the vehicle mass. The displacement x1 was shown to increase from 42.52 m to 63.78 m when the vehicle mass increased from 30 t to 60 t. This is because when the traction force of the locomotive is constant (for the target velocity is less than 45 km/h), the mass of the object affected by the traction force increases linearly with the increase of the vehicle mass, which causes the acceleration of the vehicle to decrease, so the distance x1 will increase accordingly.
- x2: Since the braking distance of the traction car is only related to its own initial state, changing the vehicle mass of the test train that has been separated from the traction car has no effect on the value of x2.
- x3: As the train mass participating in the collision process increases, the system initial total kinetic energy of the collision increases linearly, and the maximum vehicle displacement x3 of the process also increases. However, as the vehicle mass increases, the instantaneous velocity v3 of the vehicle at the end of the process does not change, and is maintained at about 15 km/h (1/2 of the impact velocity).
- x4: As the vehicle mass increases from 30 t to 60 t, the duration time of the collision process between the test train and the braking trolley has slightly increased, and the velocity v4 after the collision increased slightly, from 12.48 km/h to 13.63 km/h. The movement displacement x4 of this process also increases.
- x5: According to the above analysis, as the increase of vehicle mass, the initial braking velocity v4 will increase, and at the same time the mass affected by the braking force will increase, so x5 will gradually increase with the increase of vehicle mass, and the increase and the increase velocity is getting faster and faster.
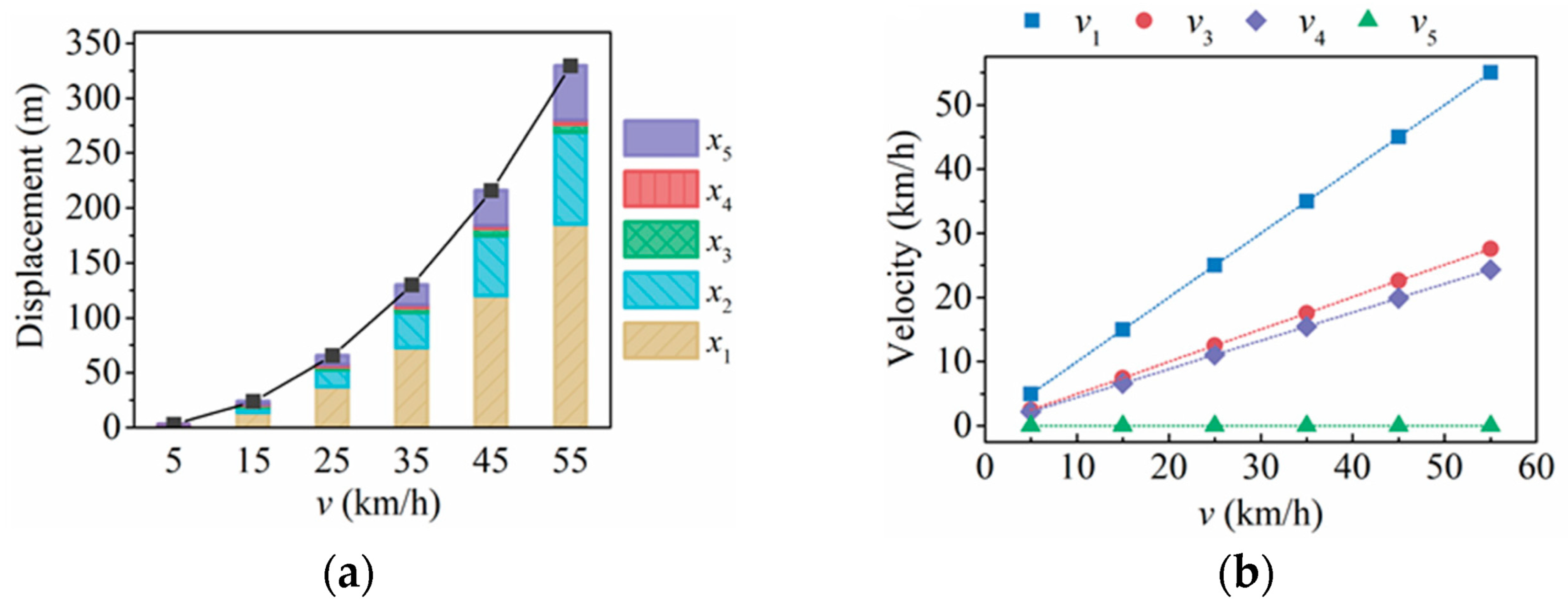
4.3. Effect of Impact Velocity
- x1: The displacement x1 increases approximately exponentially as the collision velocity increases. The displacement x1 was shown to increase from 1.48 m to 184.62 m when the collision impact increased from 5 km/h to 55 km/h.
- x2: When the impact velocity increases from 5 km/h to 55 km/h, the braking distance of the traction car increases exponentially from 0.42 m to 84.12 m.
- x3: As the impact velocity increases, the system initial total kinetic energy of the collision increases exponentially, and the maximum vehicle displacement x3 of the process also increases. As the impact velocity increases from 5 km/h to 55 km/h, the displacement x3 increases from 0.31 m to 7.39 m, and the instantaneous velocity v3 of the vehicle at the end of the process also increases, respectively 2.5 km/h, 7.5 km/h, 12.5 km/h, 17.5 km/h, 22.5 km/h and 27.5 km/h.
- x4: As the velocity v3 increases, the duration of the train collision process gradually increases, and the maximum movement displacement x4 of the train also increases dramatically, and the instantaneous velocity v4 of the vehicle at the end of the process increases, respectively 2.2 km/h, 6.6 km/h, 11.0 km/h, 15.4 km/h, 29.9 km/h and 24.3 km/h.
- x5: For the braking process, the change in the initial braking velocity v4 has a great influence on the braking process. x5 will gradually increase with the increase of the impact velocity, and the speed of increase will be faster and faster.
4.4. Sensitivity Analysis
5. Response Surface Models
5.1. Design of Experiment
5.2. Response Surface Models
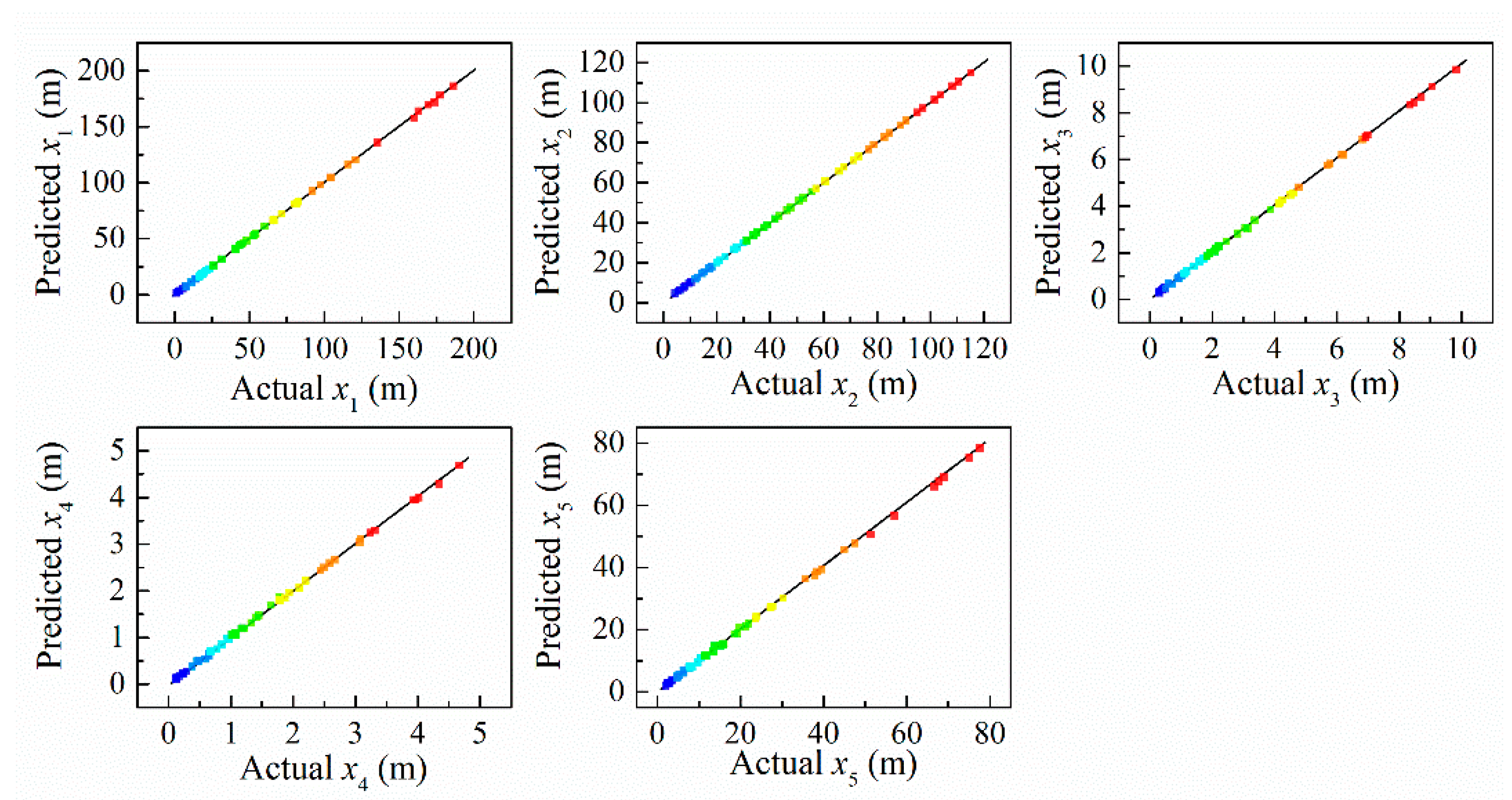
5.3. Error Estimation
6. Conclusions
- A 1D/3D co-simulation dynamics model of the train under various load conditions of driving, collision and braking has been established based on the MotionView dynamic simulation software. The accuracy of the numerical model is verified by comparing with a five-vehicle formations train-to-train crash test data.
- Based on the train dynamic model verified by test, this paper studied the effects of variable parameters on the train dynamic response at each stage of collision. The results show that the increase in the impact velocity has an increasing effect on the movement displacement of the vehicle in each process. However, increasing the vehicle mass and train formation has almost no effect on the running displacement of the braking process of the traction train. Through sensitivity analysis, we can find that the impact velocity has the greatest impact sensitivity for all test phase, followed by the train formation and vehicle mass;
- PRSM is used to construct the fitting relationship between the parameters and the responses, and step-wise regression method is adopted to simplify the PRSM. The fit-ting relationship can be applied to the design of the preliminary test plan for the train-to-train crash test, providing theoretical support for the test line length design, test equipment debugging and safety protection device installation, etc.
- According to the requirements of the standard EN15227 for train collision scenarios, the research completed in this paper only considers the first type of collision scenario. The future research will continue to conduct around the other two collision scenarios specified in the standard EN15227.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Guangjun, G. The Energy Distribution of a Train Impact Process Based on the Active–Passive Energy-Absorption Method. Transp. Saf. Environ. 2019, 1, 54–67. [Google Scholar] [CrossRef]
- Lu, G. Energy Absorption Requirement for Crashworthy Vehicles. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2002, 216, 31–39. [Google Scholar] [CrossRef]
- Lu, G.; Yu, T. 1—Introduction. In Energy Absorption of Structures and Materials; Lu, G., Yu, T., Eds.; Woodhead Publishing Series in Metals and Surface Engineering; Woodhead Publishing: Cambridge, UK, 2003; ISBN 978-1-85573-688-7. [Google Scholar]
- Crashworthiness Study of a Subway Vehicle Collision Accident Based on Finite-Element Methods. Available online: https://www.tandfonline.com/doi/epub/10.1080/13588265.2019.1699742?needAccess=true (accessed on 20 October 2021).
- Zhu, T.; Xiao, S.; Lei, C.; Wang, X.; Zhang, J.; Yang, B.; Yang, G.; Li, Y. Rail Vehicle Crashworthiness Based on Collision Energy Management: An Overview. Int. J. Rail Transp. 2021, 9, 101–131. [Google Scholar] [CrossRef]
- Scholes, A.; Lewis, J.H. Development of Crashworthiness for Railway Vehicle Structures. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 1993, 207, 1–16. [Google Scholar] [CrossRef]
- Shao, H.; Xu, P.; Yao, S.; Peng, Y.; Li, R.; Zhao, S. Improved Multibody Dynamics for Investigating Energy Dissipation in Train Collisions Based on Scaling Laws. Shock Vib. 2016, 2016, 3084052. [Google Scholar] [CrossRef]
- Kirkpatrick, S.W.; Schroeder, M.; Simons, J.W. Evaluation of Passenger Rail Vehicle Crashworthiness. Int. J. Crashworthiness 2001, 6, 95–106. [Google Scholar] [CrossRef]
- Wang, J.; Rakha, H.A. Longitudinal Train Dynamics Model for a Rail Transit Simulation System. Transp. Res. Part C Emerg. Technol. 2018, 86, 111–123. [Google Scholar] [CrossRef]
- Wu, Q.; Spiryagin, M.; Cole, C. Longitudinal Train Dynamics: An Overview. Veh. Syst. Dyn. 2016, 54, 1688–1714. [Google Scholar] [CrossRef]
- Wu, Q.; Cole, C.; Luo, S.; Spiryagin, M. A Review of Dynamics Modelling of Friction Draft Gear. Veh. Syst. Dyn. 2014, 52, 733–758. [Google Scholar] [CrossRef]
- Zhu, T.; Yang, B.; Yang, C.; Xiao, S.; Yang, G.; Yang, B. The Mechanism for the Coupler and Draft Gear and Its Influence on Safety during a Train Collision. Veh. Syst. Dyn. 2018, 56, 1375–1393. [Google Scholar] [CrossRef]
- Spiryagin, M.; Wu, Q.; Cole, C. International Benchmarking of Longitudinal Train Dynamics Simulators: Benchmarking Questions. Veh. Syst. Dyn. 2017, 55, 450–463. [Google Scholar] [CrossRef]
- Yang, L.; Kang, Y.; Luo, S.; Fu, M. Assessment of the Curving Performance of Heavy Haul Trains under Braking Conditions. J. Mod. Transport. 2015, 23, 169–175. [Google Scholar] [CrossRef]
- Severson, K.J. Volpe National Transportation Systems Center (U.S.) Development of Collision Dynamics Models to Estimate the Results of Full-Scale Rail Vehicle Impact Tests. Master’s Thesis, Tufts University, Atlanta, GA, USA, 2000. [Google Scholar]
- Milho, J.F.; Ambrósio, J.A.C.; Pereira, M.F.O.S. A Multibody Methodology for the Design of Anti-Climber Devices for Train Crashworthiness Simulation. Int. J. Crashworthiness 2002, 7, 7–20. [Google Scholar] [CrossRef]
- Milho, J.F.; Ambrósio, J.A.C.; Pereira, M.F.O.S. Design of Train Crash Experimental Tests by Optimization Procedures. Int. J. Crashworthiness 2004, 9, 483–493. [Google Scholar] [CrossRef]
- Milho, J.F.; Ambrósio, J.A.C.; Pereira, M.F.O.S. Validated Multibody Model for Train Crash Analysis. Int. J. Crashworthiness 2003, 8, 339–352. [Google Scholar] [CrossRef]
- Ambrósio, J. Crash Analysis and Dynamical Behaviour of Light Road and Rail Vehicles. Veh. Syst. Dyn. 2005, 43, 385–411. [Google Scholar] [CrossRef]
- Sun, Y.Q.; Cole, C.; Dhanasekar, M.; Thambiratnam, D.P. Modelling and Analysis of the Crush Zone of a Typical Australian Passenger Train. Veh. Syst. Dyn. 2012, 50, 1137–1155. [Google Scholar] [CrossRef][Green Version]
- Zhou, H.; Wang, W.; Hecht, M. Three-Dimensional Override Analysis of Crashed Railway Multiple Units. Veh. Syst. Dyn. 2012, 50, 663–674. [Google Scholar] [CrossRef]
- Zhou, H.; Zhang, J.; Hecht, M. Three-Dimensional Derailment Analysis of Crashed Freight Trains. Veh. Syst. Dyn. 2014, 52, 341–361. [Google Scholar] [CrossRef]
- Zhou, H.; Zhang, C.; Zhan, J.; Zhang, J. Research on the City Tram Collision at a Level Crossing. Adv. Mech. Eng. 2018, 10, 168781401879756. [Google Scholar] [CrossRef]
- Yang, C.; Li, Q.; Xiao, S.; Wang, X. On the Overriding Issue of Train Front End Collision in Rail Vehicle Dynamics. Veh. Syst. Dyn. 2018, 56, 506–528. [Google Scholar] [CrossRef]
- Ling, L.; Xiao, X.-B.; Jin, X.-S. Development of a Simulation Model for Dynamic Derailment Analysis of High-Speed Trains. Acta Mech. Sin. 2014, 30, 860–875. [Google Scholar] [CrossRef]
- Ling, L.; Dhanasekar, M.; Thambiratnam, D.P. Frontal Collision of Trains onto Obliquely Stuck Road Trucks at Level Crossings: Derailment Mechanisms and Simulation. Int. J. Impact Eng. 2017, 100, 154–165. [Google Scholar] [CrossRef]
- Huang, W.; Yang, N.; Huang, M.; Chen, S. Principle and Application of Equal Effective Conversion Calculation for Train Braking Force. Railway Locomotive & Car 2002, 1-7+4. [Google Scholar]
- Zhang, W. Dynamics of Coupled Systems in High-Speed Trains: Theory and Practice; China Science Publishing & Media Ltd.: Beijing, China, 2013. [Google Scholar]
- Zhang, H.; Peng, Y.; Hou, L.; Wang, D.; Tian, G.; Li, Z. Multistage Impact Energy Distribution for Whole Vehicles in High-Speed Train Collisions: Modeling and Solution Methodology. IEEE Trans. Ind. Inf. 2020, 16, 2486–2499. [Google Scholar] [CrossRef]
- Yao, S.; Yan, K.; Lu, S.; Xu, P. Energy-Absorption Optimisation of Locomotives and Scaled Equivalent Model Validation. Int. J. Crashworthiness 2017, 22, 441–452. [Google Scholar] [CrossRef]
- Zhao, H.; Xu, P.; Jiang, S.; Li, B.; Yao, S.; Xing, J.; Huang, Q.; Xu, K. A Novel Design Method of the Impact Zone of a High-Speed Train. Int. J. Crashworthiness 2020, 1–10. [Google Scholar] [CrossRef]
- Yao, S.; Zhu, H.; Liu, M.; Li, Z.; Xu, P.; Che, Q. A Study on the Frontal Oblique Collision-Induced Derailment Mechanism in Subway Vehicles. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2020, 234, 584–595. [Google Scholar] [CrossRef]
- Yao, S.; Zhu, H.; Yan, K.; Liu, M.; Xu, P. The Derailment Behaviour and Mechanism of a Subway Train under Frontal Oblique Collisions. Int. J. Crashworthiness 2021, 26, 133–146. [Google Scholar] [CrossRef]
- BSI British Standards Railway Applications. Crashworthiness Requirements for Rail Vehicles; The British Standards Institution: London, UK, 2020. [Google Scholar]
- Yao, S.; Yan, K.; Lu, S.; Xu, P. Prediction and Application of Energy Absorption Characteristics of Thin-Walled Circular Tubes Based on Dimensional Analysis. Thin-Walled Struct. 2018, 130, 505–519. [Google Scholar] [CrossRef]
- Yang, C.; Xu, P.; Yao, S.; Xie, S.; Li, Q.; Peng, Y. Optimization of Honeycomb Strength Assignment for a Composite Energy-Absorbing Structure. Thin-Walled Struct. 2018, 127, 741–755. [Google Scholar] [CrossRef]
- Xu, P.; Yang, C.; Peng, Y.; Yao, S.; Xing, J.; Li, B. Cut-out Grooves Optimization to Improve Crashworthiness of a Gradual Energy-Absorbing Structure for Subway Vehicles. Mater. Des. 2016, 103, 132–143. [Google Scholar] [CrossRef]


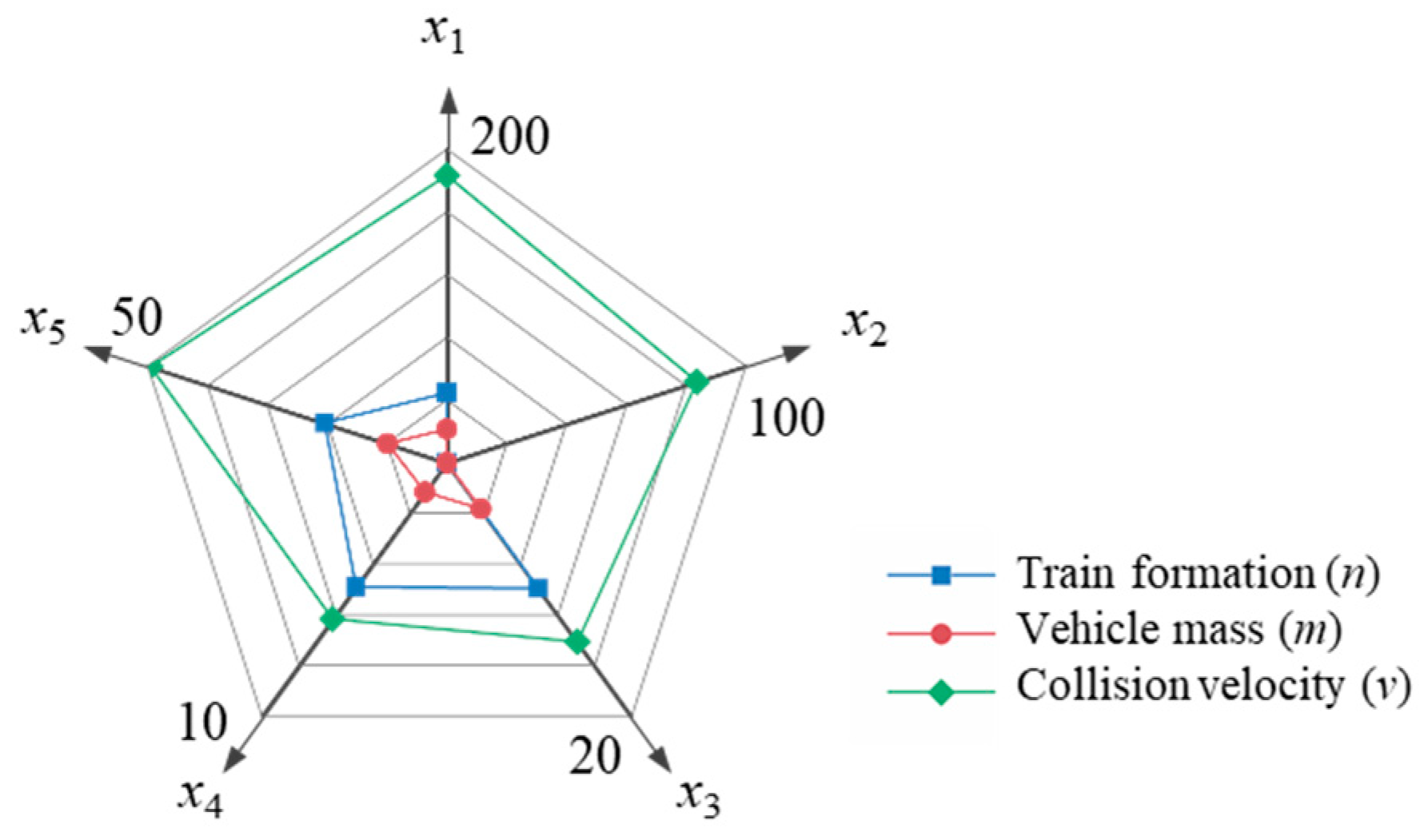
| Type | Parameters | Unit | Value |
|---|---|---|---|
| Geometric parameters | Vehicle distance | mm | 17,800 |
| Bogie axis distance | mm | 2500 | |
| Rail distance | mm | 1435 | |
| Wheel rolling circle diameter | mm | 860 | |
| Wheel profile shape | LMA | ||
| Rail profile shape | CHN60 | ||
| Inertia parameter | Carbody mass | t | 43.0 |
| Carbody moment of inertia-X | kg·m2 | 69,171 | |
| Carbody moment of inertia-Y | kg·m2 | 1,347,125 | |
| Carbody moment of inertia-Z | kg·m2 | 1,349,239 | |
| Bogie mass | t | 3.660 | |
| Bogie moment of inertia-X | kg·m2 | 3876 | |
| Bogie moment of inertia-Y | kg·m2 | 8098 | |
| Bogie moment of inertia-Z | kg·m2 | 10,248 | |
| Wheelset mass | t | 2.816 | |
| Wheelset moment of inertia-X | kg·m2 | 2024 | |
| Wheelset moment of inertia-Y | kg·m2 | 452 | |
| Carbody moment of inertia-Z | kg·m2 | 2024 | |
| Suspension parameters | Axlebox spring longitudinal stiffness | N/mm | 886 |
| Axlebox spring lateral stiffness | N/mm | 919 | |
| Axlebox spring vertical stiffness | N/mm | 919 | |
| Primary suspension vertical damper | N·s/mm | 20 | |
| Airspring longitudinal stiffness | N/mm | 107 | |
| Airspring lateral stiffness | N/mm | 107 | |
| Airspring vertical stiffness | N/mm | 173 | |
| Secondary suspension longitudinal damper | N·s/mm | 810 | |
| Secondary suspension lateral damper | N·s/mm | 15 | |
| Secondary suspension vertical damper | N·s/mm | 10 | |
| Train Type | Traction Car Mass | Moving/Stationary Train Mass | Braking Car Mass | ||||
|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | |||
| Mass (t) | 150 | 52.9 | 60.6 | 60.4 | 50 | 50 | 60 |
| Response | x1 | x2 | x3 | x4 | x5 | x–Total |
|---|---|---|---|---|---|---|
| Simulation (m) | 85.8 | 43.8 | 4.1 | 1.9 | 25.6 | 161.2 |
| Test (m) | 90 | 45 | 4 | 2 | 25 | 166 |
| Error (%) | 4.67 | 2.67 | 2.5 | 5 | 2.4 | 2.89 |
| Evaluation Type | RE (%) | RMSE (/) | R2 (/) |
|---|---|---|---|
| x1 | [–7.37, 8.40] | 0.598 | 0.9998437 |
| x2 | [–2.24, 0.43] | 0.085 | 0.9999935 |
| x3 | [–8.32, 9.23] | 0.051 | 0.9979243 |
| x4 | [–5.98, 9.09] | 0.027 | 0.9991360 |
| x5 | [–9.37, 5.79] | 0.406 | 0.9993867 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Xu, P.; Li, B.; Yao, S.; Yang, C.; Guo, W.; Xiao, X. Full-Scale Train-to-Train Impact Test and Multi-Body Dynamic Simulation Analysis. Machines 2021, 9, 297. https://doi.org/10.3390/machines9110297
Zhao H, Xu P, Li B, Yao S, Yang C, Guo W, Xiao X. Full-Scale Train-to-Train Impact Test and Multi-Body Dynamic Simulation Analysis. Machines. 2021; 9(11):297. https://doi.org/10.3390/machines9110297
Chicago/Turabian StyleZhao, Hui, Ping Xu, Benhuai Li, Shuguang Yao, Chengxing Yang, Wei Guo, and Xianliang Xiao. 2021. "Full-Scale Train-to-Train Impact Test and Multi-Body Dynamic Simulation Analysis" Machines 9, no. 11: 297. https://doi.org/10.3390/machines9110297
APA StyleZhao, H., Xu, P., Li, B., Yao, S., Yang, C., Guo, W., & Xiao, X. (2021). Full-Scale Train-to-Train Impact Test and Multi-Body Dynamic Simulation Analysis. Machines, 9(11), 297. https://doi.org/10.3390/machines9110297







